Rotational Doppler Velocimetry of a Surface at Larger Tilt Angles
Abstract
1. Introduction
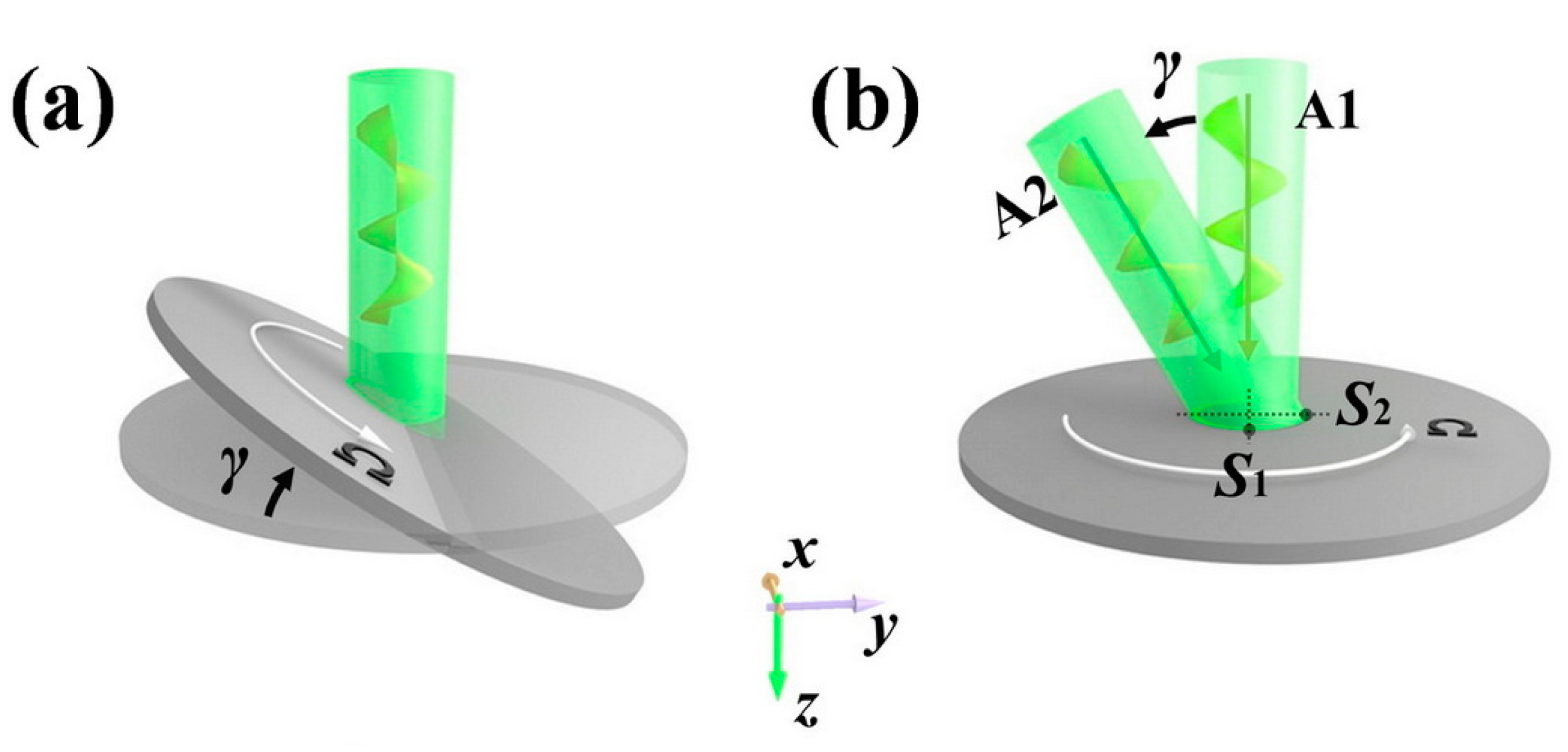
2. Theoretical Analyses
3. Experiment
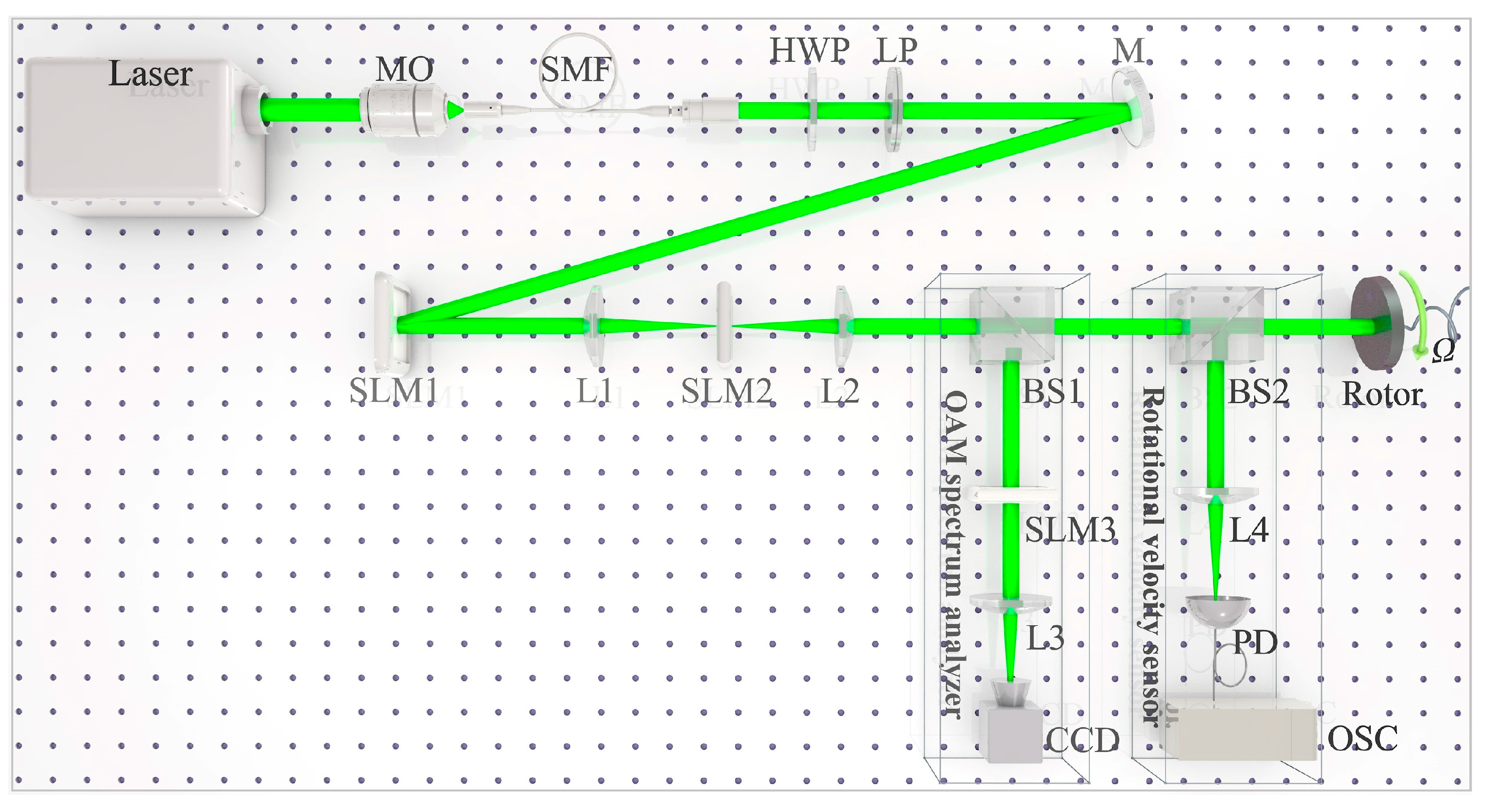
3.1. Experiment Setup
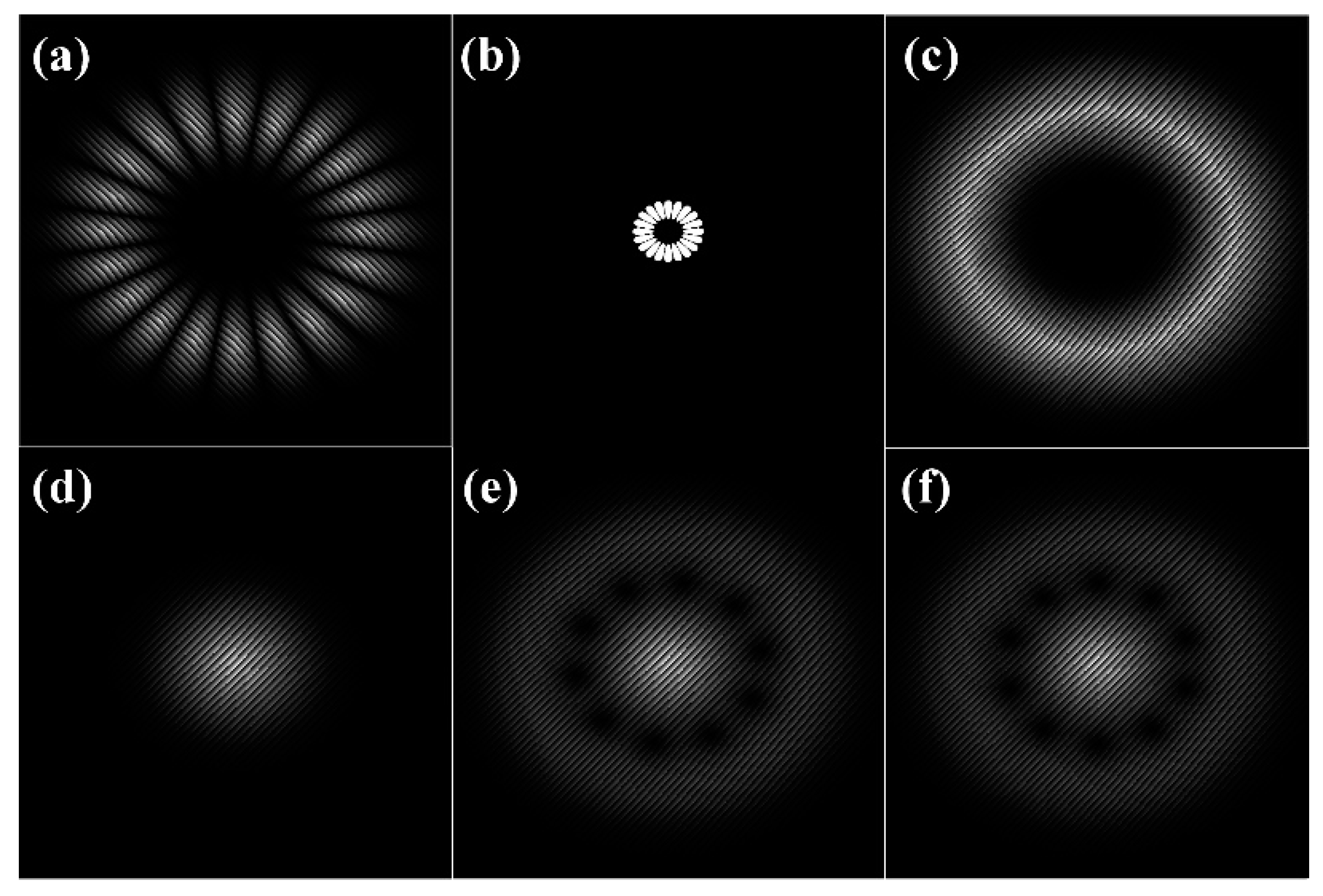
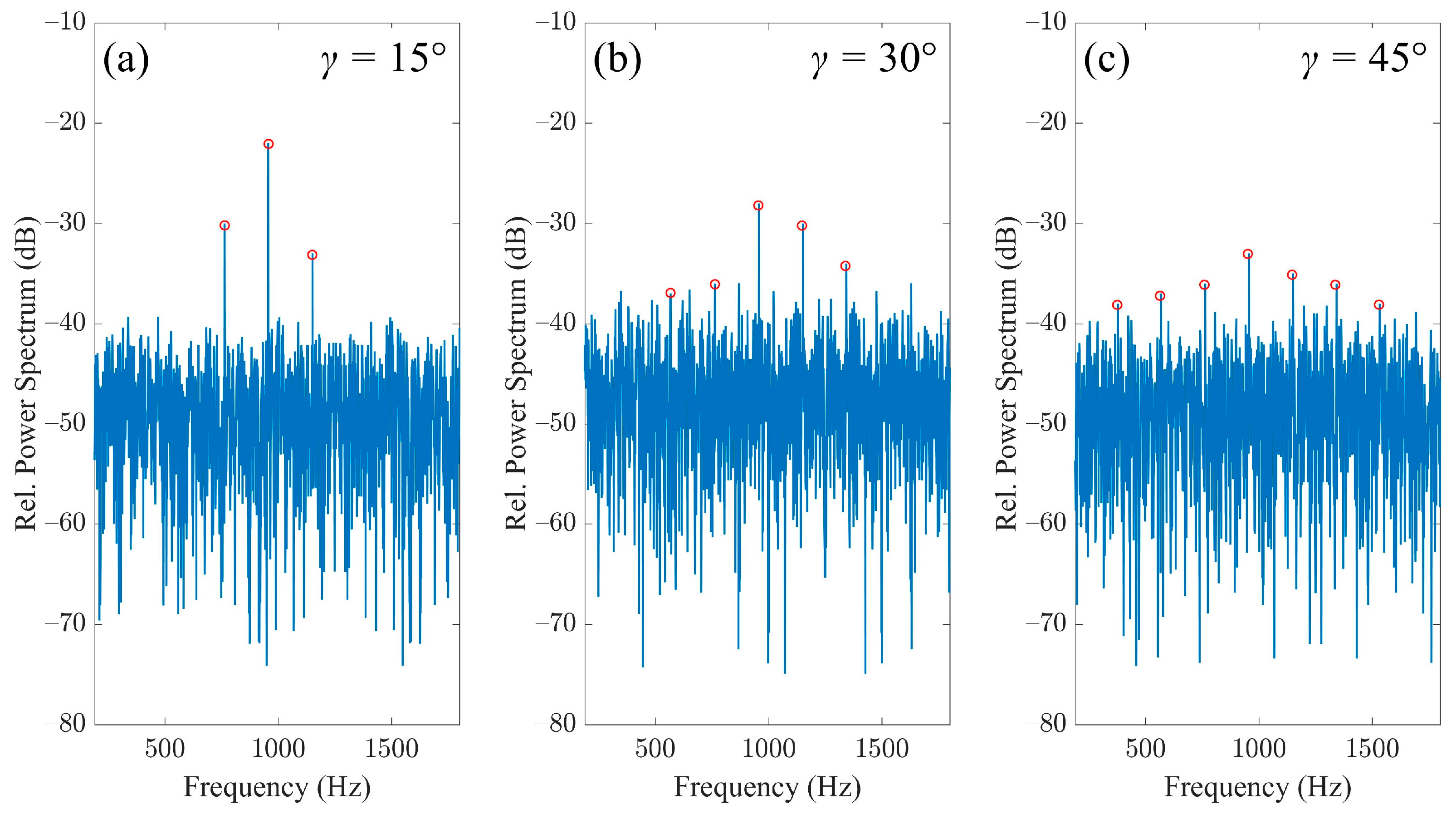
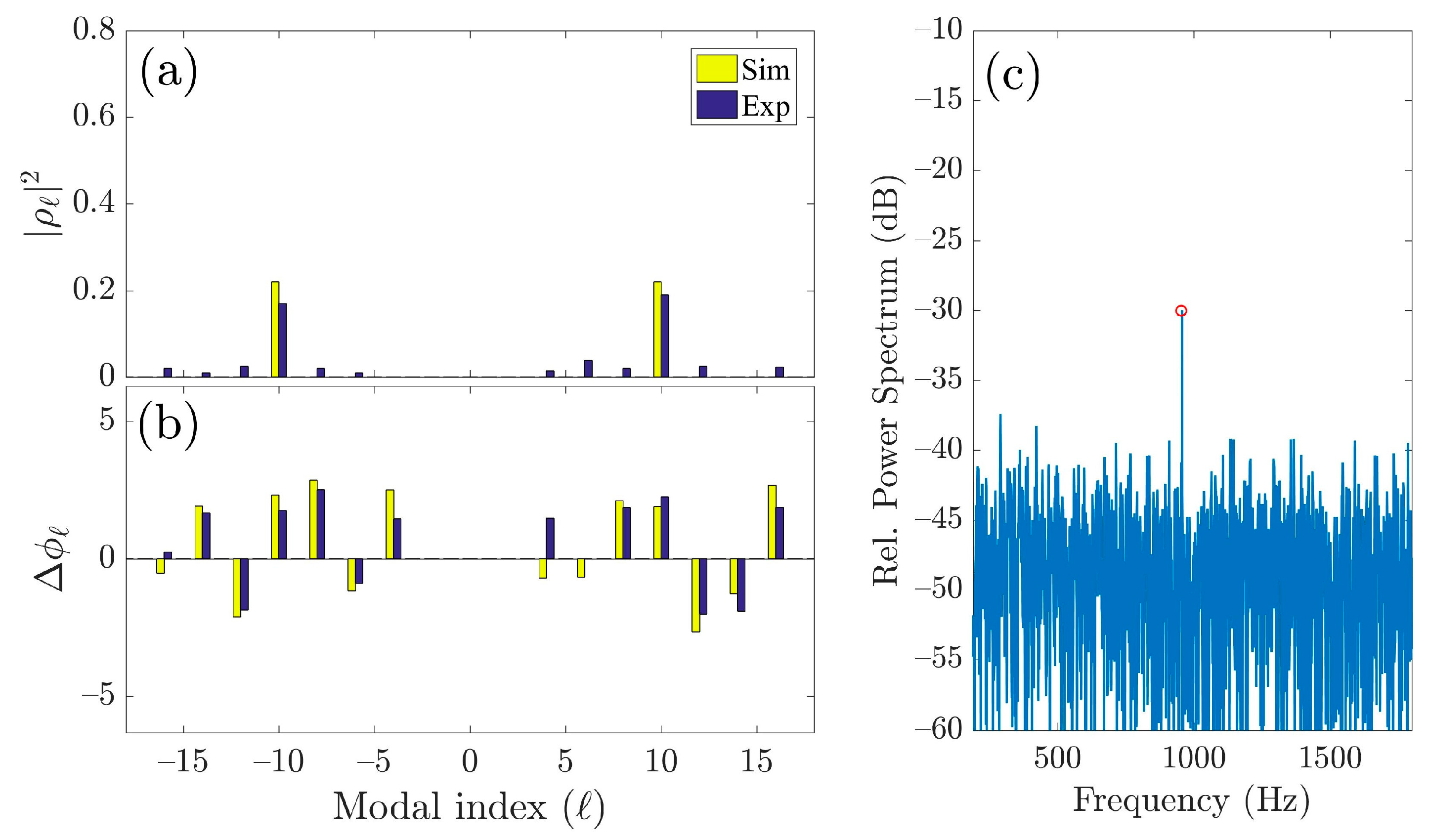
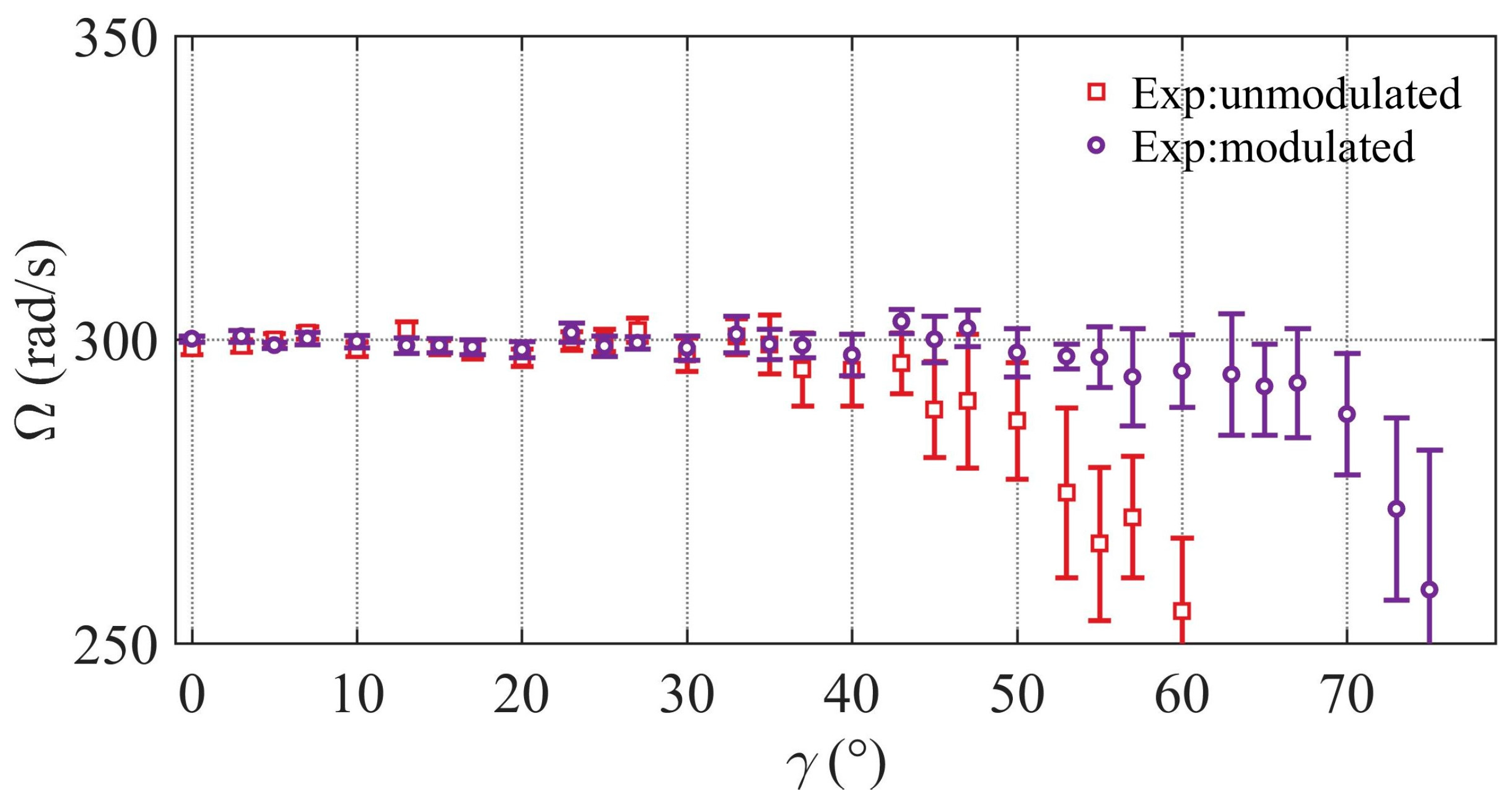
3.2. Experimental Results
4. Discussions
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Charrett, T.O.H.; James, S.W.; Tatam, R.P. Optical fibre laser velocimetry: A review. Meas. Sci. Technol. 2012, 23, 032001. [Google Scholar] [CrossRef]
- Yeh, Y.; Cummings, H.Z. Localised fluid flow measurements with a He–Ne spectrometer. Appl. Phys. Lett. 1964, 4, 176–178. [Google Scholar] [CrossRef]
- Mayor, M.; Lovis, C.; Santos, N.C. Doppler spectroscopy as a path to the detection of Earth-like planets. Nature 2014, 513, 328–335. [Google Scholar] [CrossRef]
- Doppler, C. Ueber das Farbige Licht der Doppelsterne und Einiger Anderer Gestirne des Himmels: Versuch Einer das Bradley’sche Aberrations-Theorem Als Integrirenden Theil in Sich Schliessenden Allgemeineren Theorie; Commission bei Borrosch & André: Prague, Czech Republic, 1842. [Google Scholar]
- Courtial, J.; Robertson, D.A.; Dholakia, K.; Allen, L.; Padgett, M.J. Rotational frequency shift of a light beam. Phys. Rev. Lett. 1998, 81, 4828–4830. [Google Scholar] [CrossRef]
- Amzajerdian, F.; Pierrottet, D.; Hines, G.D.; Petway, L.B.; Barnes, B.W. Doppler lidar sensor for precision navigation in GPS-deprived environment. Laser Radar Technology and Applications XVIII. Int. Soc. Opt. Photonics 2013, 8731, 87310G. [Google Scholar]
- Ristić, S. Laser Doppler anemometry and its application in wind tunnel tests. Sci. Technol. Rev. 2007, 57, 64. [Google Scholar]
- Watrasiewicz, B.M.; Rudd, M.J. Laser Doppler Measurements; Butterworths: London, UK, 1976. [Google Scholar]
- Fang, L.; Padgett, M.J.; Wang, J. Sharing a common origin between the rotational and linear Doppler effects. Laser Photonics Rev. 2017, 11, 1700183. [Google Scholar] [CrossRef]
- Nienhuis, G. Doppler effect induced by rotating lenses. Opt. Commun. 1996, 132, 8–14. [Google Scholar] [CrossRef]
- Belmonte, A.; Rosales-Guzmán, C.; Torres, J.P. Measurement of flow vorticity with helical beams of light. Optica 2015, 2, 1002–1005. [Google Scholar] [CrossRef]
- Vasnetsov, M.V.; Torres, J.P.; Petrov, D.V.; Torner, L. Observation of the orbital angular momentum spectrum of a light beam. Opt. Lett. 2003, 28, 2285–2287. [Google Scholar] [CrossRef]
- Rosales-Guzmán, C.; Hermosa, N.; Belmonte, A.; Torres, J.P. Experimental detection of transverse particle movement with structured light. Sci. Rep. 2013, 3, 2815. [Google Scholar] [CrossRef] [PubMed]
- Rosales-Guzmán, C.; Hermosa, N.; Belmonte, A.; Torres, J.P. Measuring the translational and rotational velocities of particles in helical motion using structured light. Opt. Express 2014, 22, 16504–16509. [Google Scholar] [CrossRef] [PubMed]
- Lavery, M.P.J.; Speirits, F.C.; Barnett, S.M.; Padgett, M.J. Detection of a spinning object using light’s orbital angular momentum. Science 2013, 341, 537–540. [Google Scholar] [CrossRef] [PubMed]
- Zhou, H.L.; Fu, D.; Dong, J.; Zhang, P.; Zhang, X. Theoretical analysis and experimental verification on optical rotational Doppler effect. Opt. Express 2016, 24, 10050–10056. [Google Scholar] [CrossRef] [PubMed]
- Li, G.; Zentgraf, T.; Zhang, S. Rotational Doppler effect in nonlinear optics. Nat. Phys. 2016, 12, 736–740. [Google Scholar] [CrossRef]
- Li, K.F.; Deng, J.H.; Liu, X.; Li, G. Observation of rotational doppler effect in second harmonic generation in reflection mode. Laser Photonics Rev. 2018, 12, 1700204. [Google Scholar] [CrossRef]
- Georgi, P.; Schlickriede, C.; Li, G.; Zhang, S.; Zentgraf, T. Rotational Doppler shift induced by spin-orbit coupling of light at spinning metasurfaces. Optica 2017, 4, 1000–1005. [Google Scholar] [CrossRef]
- Anderson, A.Q.; Strong, E.F.; Heffernan, B.M.; Siemens, M.E.; Rieker, G.B.; Gopinath, J.T. Detection technique effect on rotational Doppler measurements. Opt. Lett. 2020, 45, 2636–2639. [Google Scholar] [CrossRef]
- Zhou, H.L.; Fu, D.Z.; Dong, J.J.; Zhang, P.; Chen, D.X.; Cai, X.L.; Li, F.; Zhang, X.L. Orbital angular momentum complex spectrum analyzer for vortex light based on the rotational Doppler effect. Light Sci. Appl. 2017, 6, e16251. [Google Scholar] [CrossRef] [PubMed]
- Neo, R.; Leon-Saval, S.; Bland-Hawthorn, J.; Molina-Terriza, G. OAM interferometry: The detection of the rotational Doppler shift. Opt. Express 2017, 25, 21159–21170. [Google Scholar] [CrossRef]
- Zhang, W.; Gao, J.; Zhang, D.; He, Y.; Xu, T.; Fickler, R.; Chen, L. Free-space remote sensing of rotation at the photon-counting level. Phys. Rev. Appl. 2018, 10, 044014. [Google Scholar] [CrossRef]
- Zhai, Y.; Fu, S.; Zhang, J.; Lv, Y.; Zhou, H.; Gao, C. Remote detection of a rotator based on rotational Doppler effect. Appl. Phys. Express 2020, 13, 022012. [Google Scholar] [CrossRef]
- Fu, S.; Wang, T.; Zhang, Z.; Zhai, Y.; Gao, C. Nondiffractive Bessel-Gauss beams for the detection of rotating object free of obstructions. Opt. Express 2017, 25, 20098–20108. [Google Scholar] [CrossRef]
- Qiu, S.; Ren, Y.; Liu, T.; Chen, L.; Wang, C.; Li, Z.; Shao, Q. Spinning object detection based on perfect optical vortex. Opt. Lasers Eng. 2020, 124, 105842. [Google Scholar] [CrossRef]
- Qiu, S.; Ren, Y.; Sha, Q.; Ding, Y.; Wang, C.; Li, Z.; Liu, T. Observation of the rotational doppler shift of the ring airy gaussian vortex beam. Opt. Commun. 2021, 490, 126900. [Google Scholar] [CrossRef]
- Zhang, Y.; Zhang, Z.; Liu, L.; Zhao, Y. Measurement of rotational velocity enabled by novel perfect Laguerre-Gaussian modes. Opt. Express 2022. To be published. [Google Scholar]
- Lavery, M.P.; Barnett, S.M.; Speirits, F.C.; Padgett, M.J. Observation of the rotational Doppler shift of a white-light, orbital-angular-momentum-carrying beam backscattered from a rotating body. Optica 2014, 1, 1–4. [Google Scholar] [CrossRef]
- Rosales-Guzmán, C.; Hermosa, N.; Belmonte, A.; Torres, J.P. Direction-sensitive transverse velocity measurement by phase-modulated structured light beams. Opt. Lett. 2014, 39, 5415–5418. [Google Scholar] [CrossRef]
- Li, Z.; Liu, T.; Ren, Y.; Qiu, S.; Wang, C.; Wang, H. Direction-sensitive detection of a spinning object using dual-frequency vortex light. Opt. Express 2021, 29, 7453–7463. [Google Scholar] [CrossRef]
- Fang, L.; Wan, Z.; Forbes, A.; Wang, J. Vectorial doppler metrology. Nat. Commun. 2021, 12, 4186. [Google Scholar]
- Zhai, Y.; Fu, S.; Yin, C.; Zhou, H.; Gao, C. Detection of angular acceleration based on optical rotational Doppler effect. Opt. Express 2019, 27, 15518–15527. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Z.; Zhang, Y.; Wang, F.; Zhao, Y. Measuring the rotational velocity and acceleration based on orbital angular momentum modulation and time–frequency analysis method. Opt. Commun. 2022, 502, 127414. [Google Scholar] [CrossRef]
- Qiu, S.; Liu, T.; Li, Z.; Wang, C.; Ren, Y.; Shao, Q.; Xing, C. Influence of lateral misalignment on the optical rotational Doppler effect. Appl. Opt. 2019, 58, 2650–2655. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Z.; Cen, L.; Zhang, J.D.; Hu, J.; Wang, F.; Zhao, Y. Rotation velocity detection with orbital angular momentum light spot completely deviated out of the rotation center. Opt. Express 2020, 28, 6859–6867. [Google Scholar] [CrossRef]
- Qiu, S.; Liu, T.; Ren, Y.; Li, Z.; Wang, C.; Shao, Q. Detection of spinning objects at oblique light incidence using the optical rotational Doppler effect. Opt. Express 2019, 27, 24781–24792. [Google Scholar] [CrossRef]
- Ding, Y.; Ren, Y.; Liu, T.; Qiu, S.; Wang, C.; Li, Z.; Liu, Z. Analysis of misaligned optical rotational Doppler effect by modal decomposition. Opt. Express 2021, 29, 15288–15299. [Google Scholar] [CrossRef]
- Litvin, I.A.; Dudley, A.; Roux, F.S.; Forbes, A. Azimuthal decomposition with digital holograms. Opt. Express 2012, 20, 10996–11004. [Google Scholar] [CrossRef]
- Flamm, D.; Naidoo, D.; Schulze, C.; Forbes, A.; Duparré, M. Mode analysis with a spatial light modulator as a correlation filter. Opt. Lett. 2012, 37, 2478–2480. [Google Scholar] [CrossRef]
- Bolduc, E.; Bent, N.; Santamato, E.; Karimi, E.; Boyd, R.W. Exact solution to simultaneous intensity and phase encryption with a single phase-only hologram. Opt. Lett. 2013, 38, 3546–3549. [Google Scholar] [CrossRef]
- Schulze, C.; Dudley, A.; Flamm, D.; Duparre, M.; Forbes, A. Measurement of the orbital angular momentum density of light by modal decomposition. New J. Phys. 2013, 15, 073025. [Google Scholar] [CrossRef]
- D’Errico, A.; D’Amelio, R.; Piccirillo, B.; Cardano, F.; Marrucci, L. Measuring the complex orbital angular momentum spectrum and spatial mode decomposition of structured light beams. Optica 2017, 4, 1350–1357. [Google Scholar] [CrossRef]
- Fu, S.; Zhai, Y.; Zhang, J.; Liu, X.; Song, R.; Zhou, H.; Gao, C. Universal orbital angular momentum spectrum analyzer for beams. PhotoniX 2020, 1, 19. [Google Scholar] [CrossRef]
- Pinnell, J.; Klug, A.; Forbes, A. Spatial filtering of structured light. Am. J. Phys. 2020, 88, 1123–1131. [Google Scholar] [CrossRef]
- Neto, A.M.; Victorino, A.C.; Fantoni, I.; Zampieri, D.E.; Ferreira, J.V.; Lima, D.A. Image processing using Pearson’s correlation coefficient: Applications on autonomous robotics. In Proceedings of the IEEE International Conference on Autonomous Robot Systems and Competitions, 13th International Conference on Autonomous Robot Systems, Lisbon, Portugal, 24 April 2013; pp. 1–6. [Google Scholar]

| Items | Ref. [37] | This Work | |||
|---|---|---|---|---|---|
| Unmodulated | Modulated | ||||
| OAM indices of the probe beams | ±18 | ±10 | ±18 | ±10 | ±18 |
| The largest tilt angle of the object (°) | 49 | 50 | 51 | 70 | 72 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, Y.; Zhang, Z.; Liu, L.; Zhao, Y. Rotational Doppler Velocimetry of a Surface at Larger Tilt Angles. Photonics 2023, 10, 341. https://doi.org/10.3390/photonics10030341
Zhang Y, Zhang Z, Liu L, Zhao Y. Rotational Doppler Velocimetry of a Surface at Larger Tilt Angles. Photonics. 2023; 10(3):341. https://doi.org/10.3390/photonics10030341
Chicago/Turabian StyleZhang, Yanxiang, Zijing Zhang, Liping Liu, and Yuan Zhao. 2023. "Rotational Doppler Velocimetry of a Surface at Larger Tilt Angles" Photonics 10, no. 3: 341. https://doi.org/10.3390/photonics10030341
APA StyleZhang, Y., Zhang, Z., Liu, L., & Zhao, Y. (2023). Rotational Doppler Velocimetry of a Surface at Larger Tilt Angles. Photonics, 10(3), 341. https://doi.org/10.3390/photonics10030341





