Process Parameters Analysis of Laser Phase Transformation Hardening on the Raceway Surface of Shield Main Bearing
Abstract
:1. Introduction
2. Numerical Model for Laser Phase Hardening of Shield Main Bearing Raceway Surfaces
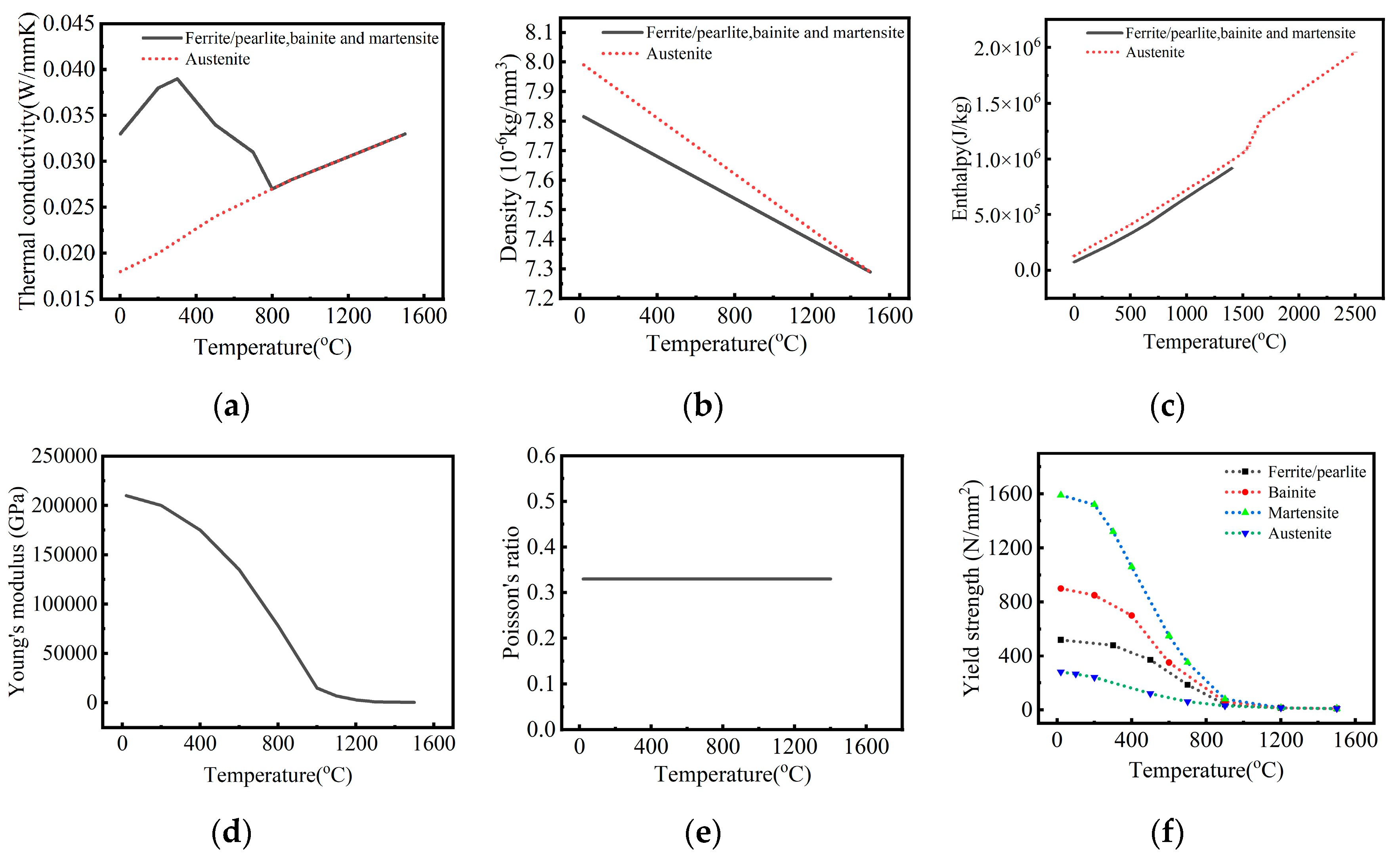
2.1. Material 42CrMo Parameters of Shield Main Bearing Raceway
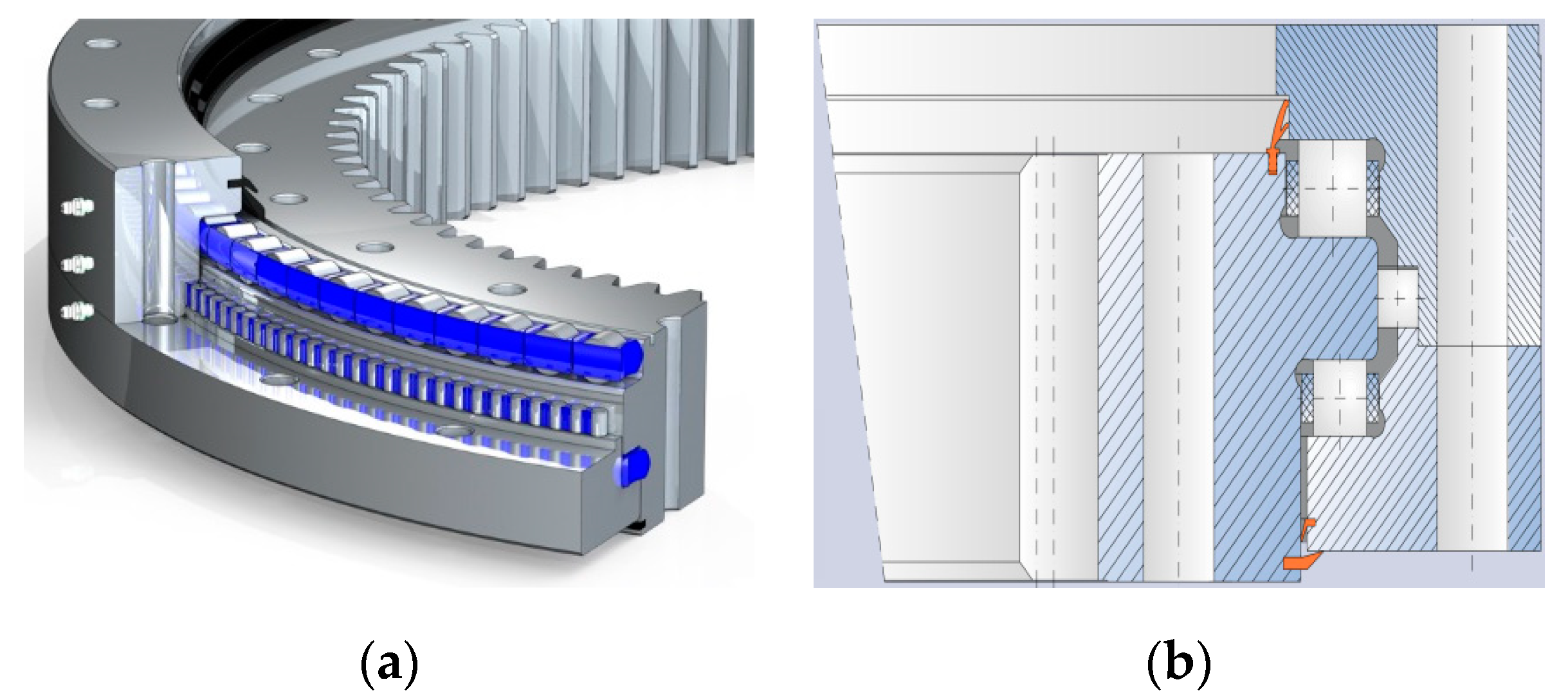
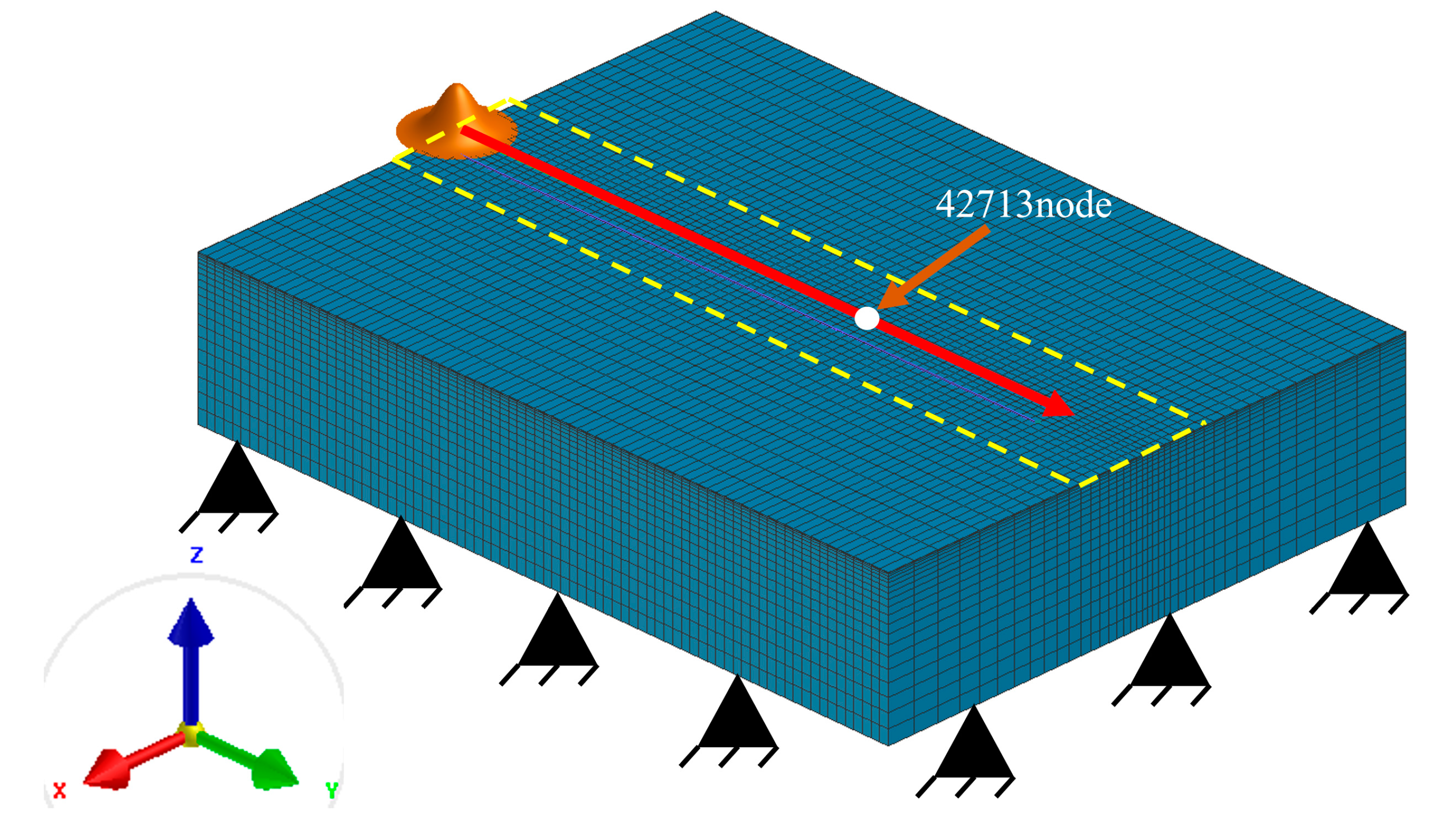
2.2. Numerical Model of Laser Phase Hardening of Shield Main Bearing Raceways
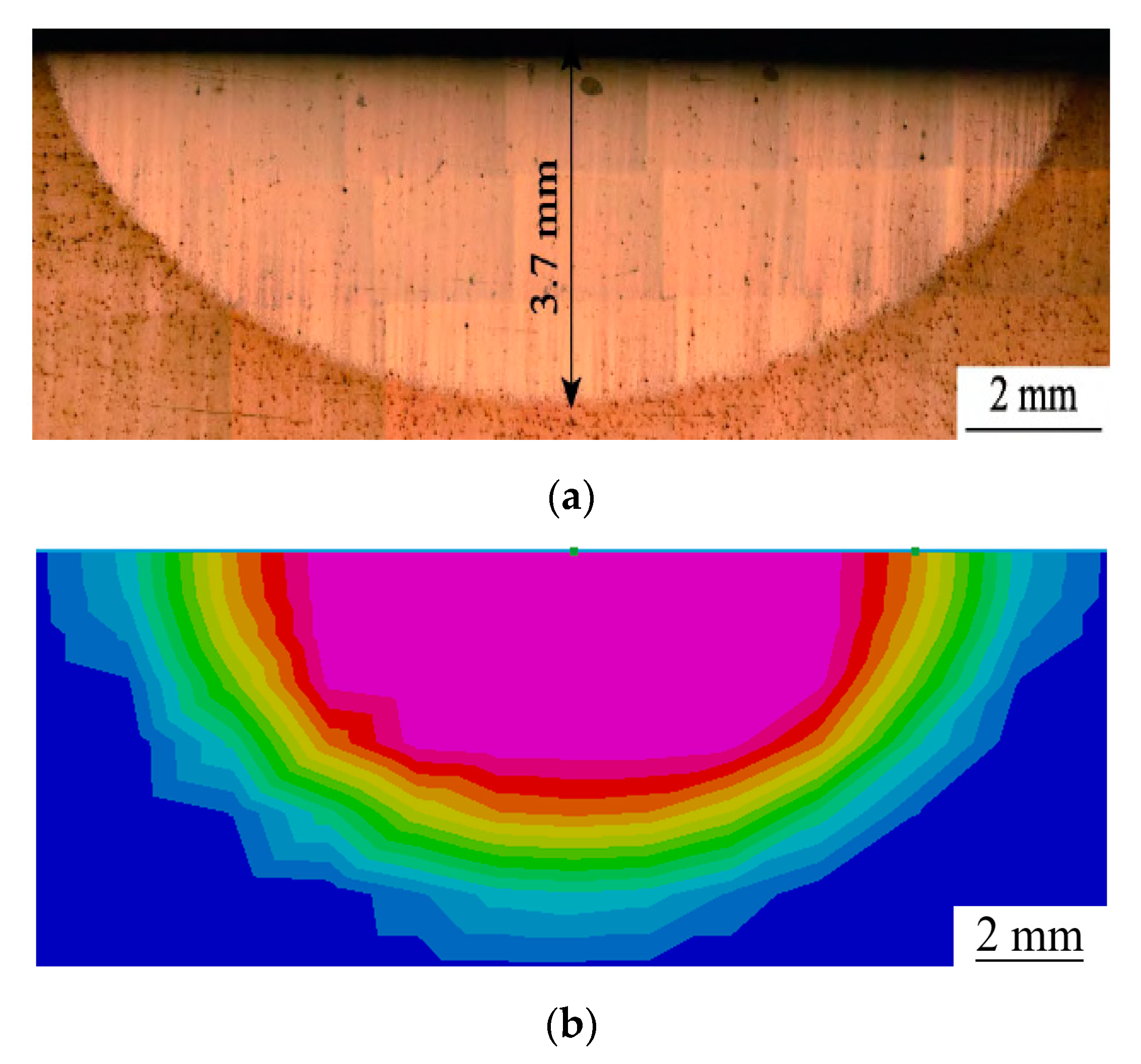
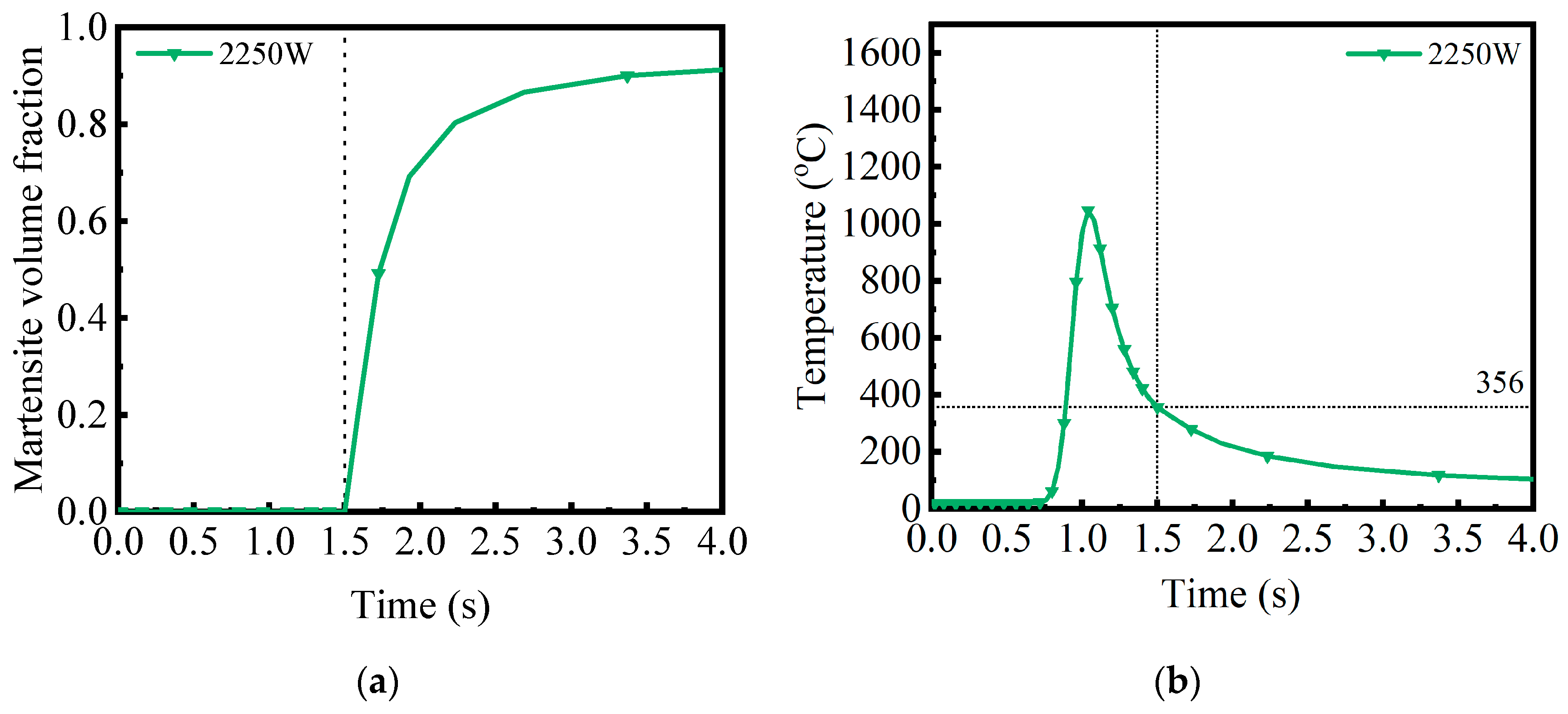
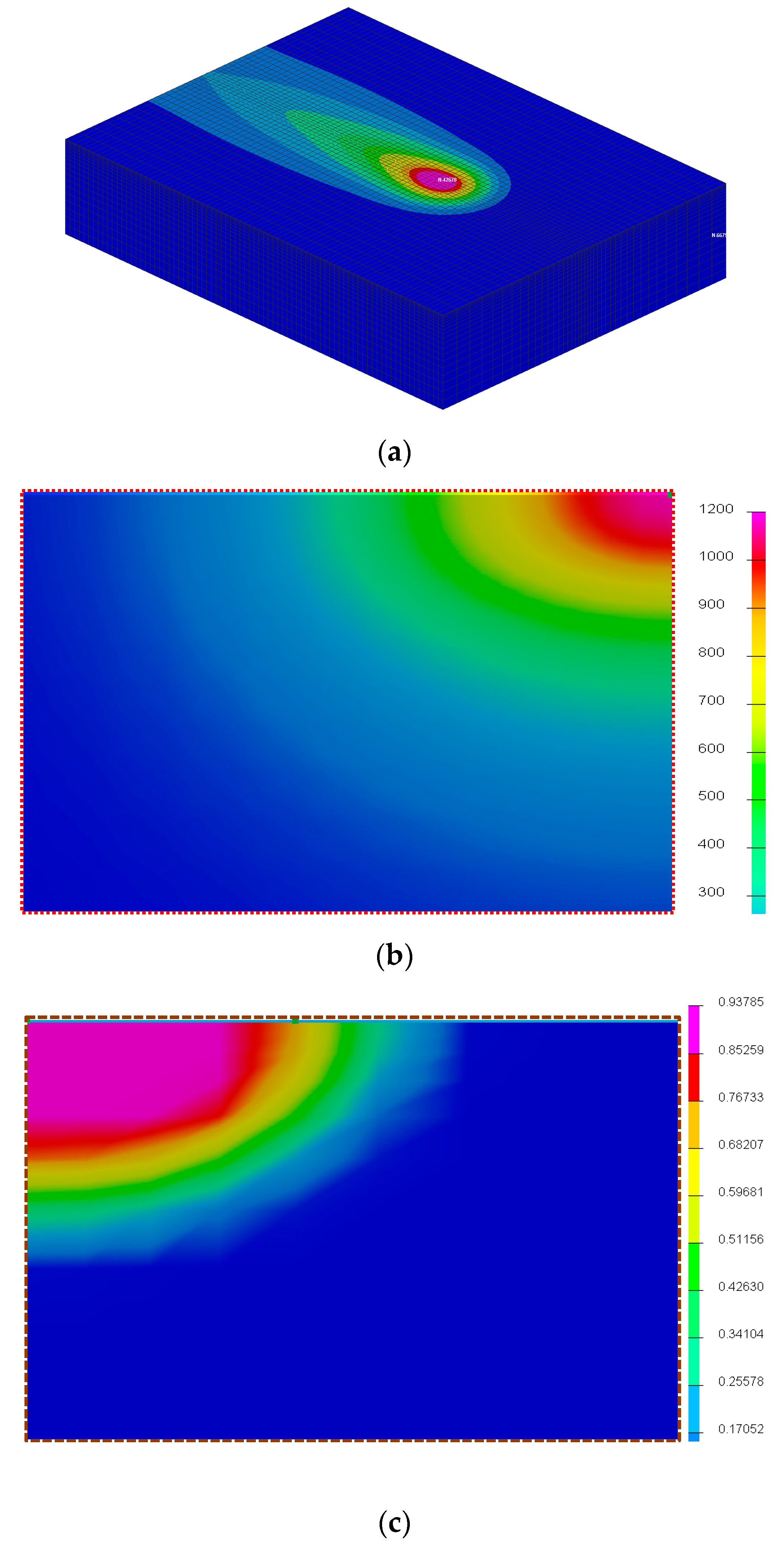
2.3. Numerical Model Validation of Laser Phase Hardening of Shield Main Bearing Raceways
3. Results and Discussion
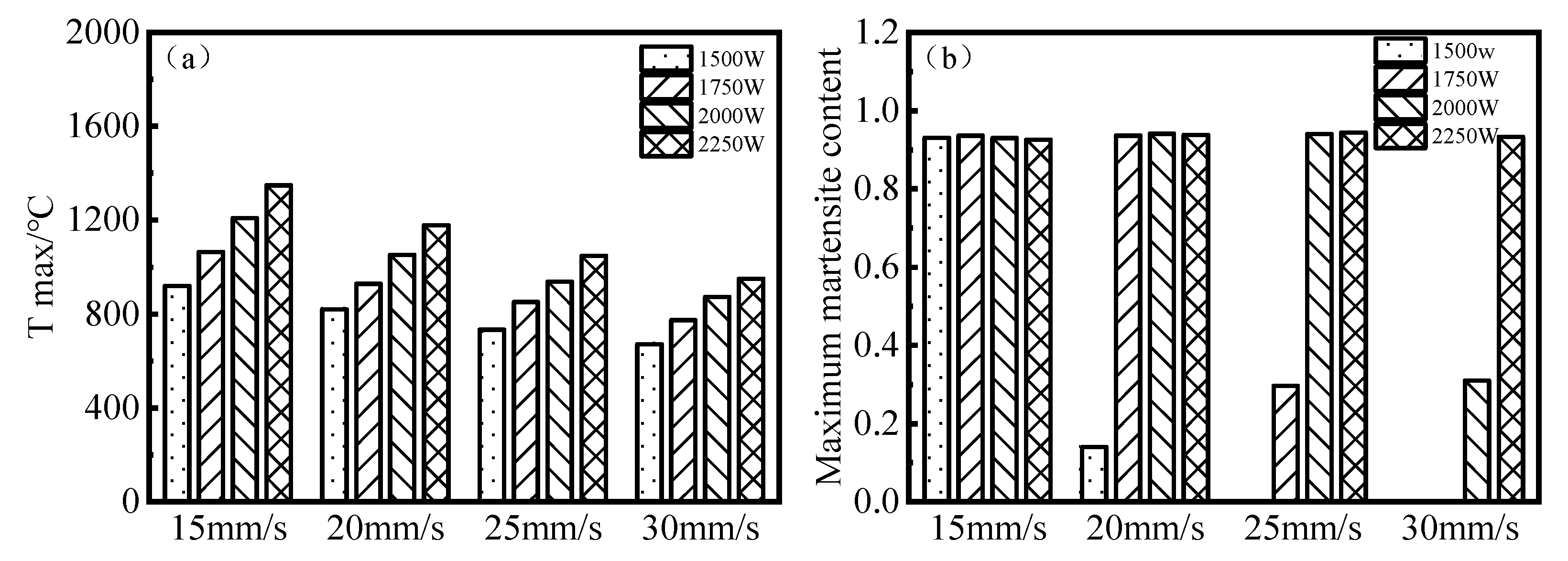
3.1. Power and Scanning Speed Effect of Laser Phase Hardening on the Surface of Shield Main Bearing Raceways
3.2. Primary and Secondary Factors Analysis of Laser Phase Change Hardening Process Parameters on Shield Main Bearing Raceway Surfaces
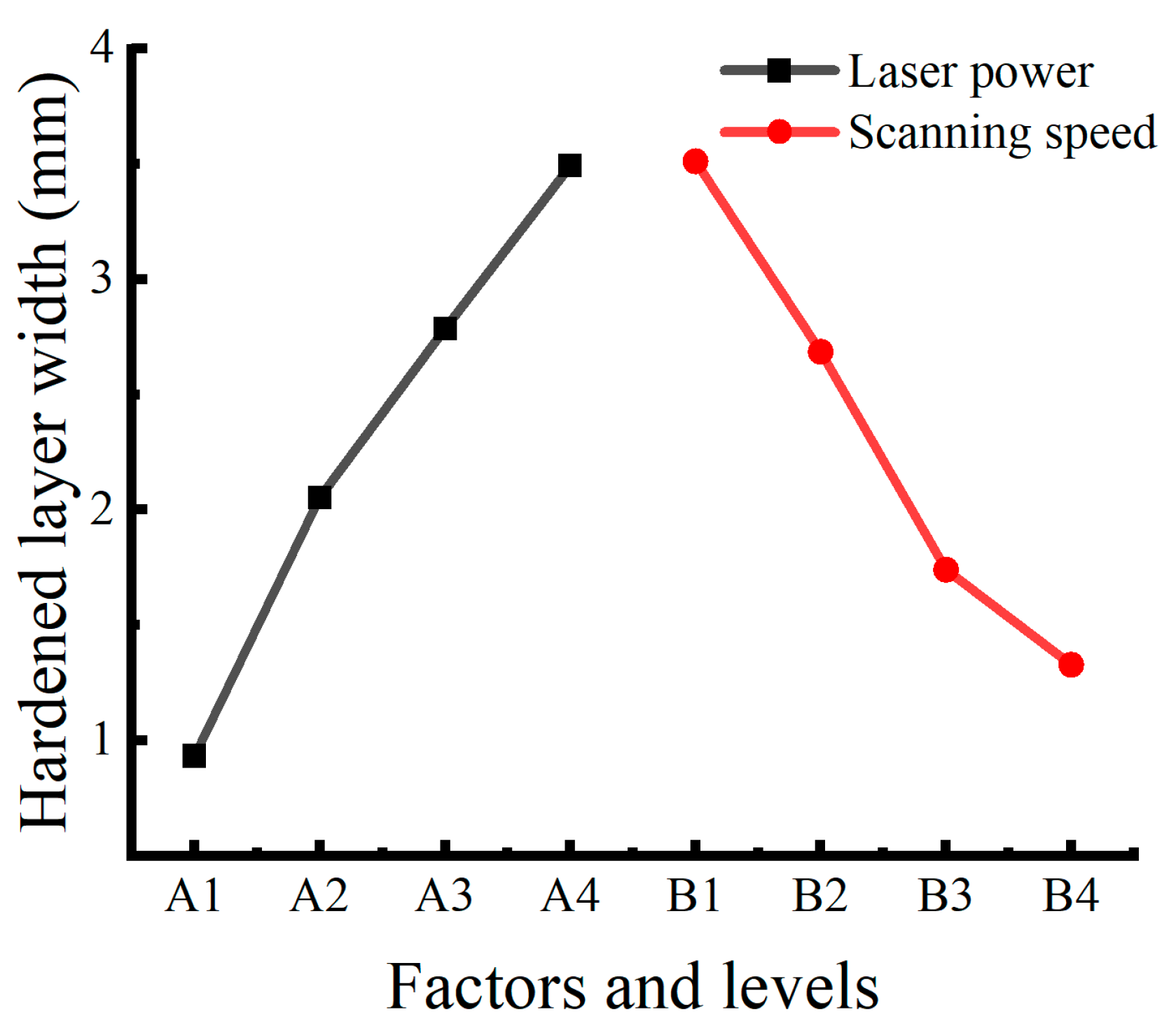
3.2.1. Effect on the Width of the Hardened Layer on the Surface of the Shield Main Bearing Raceway
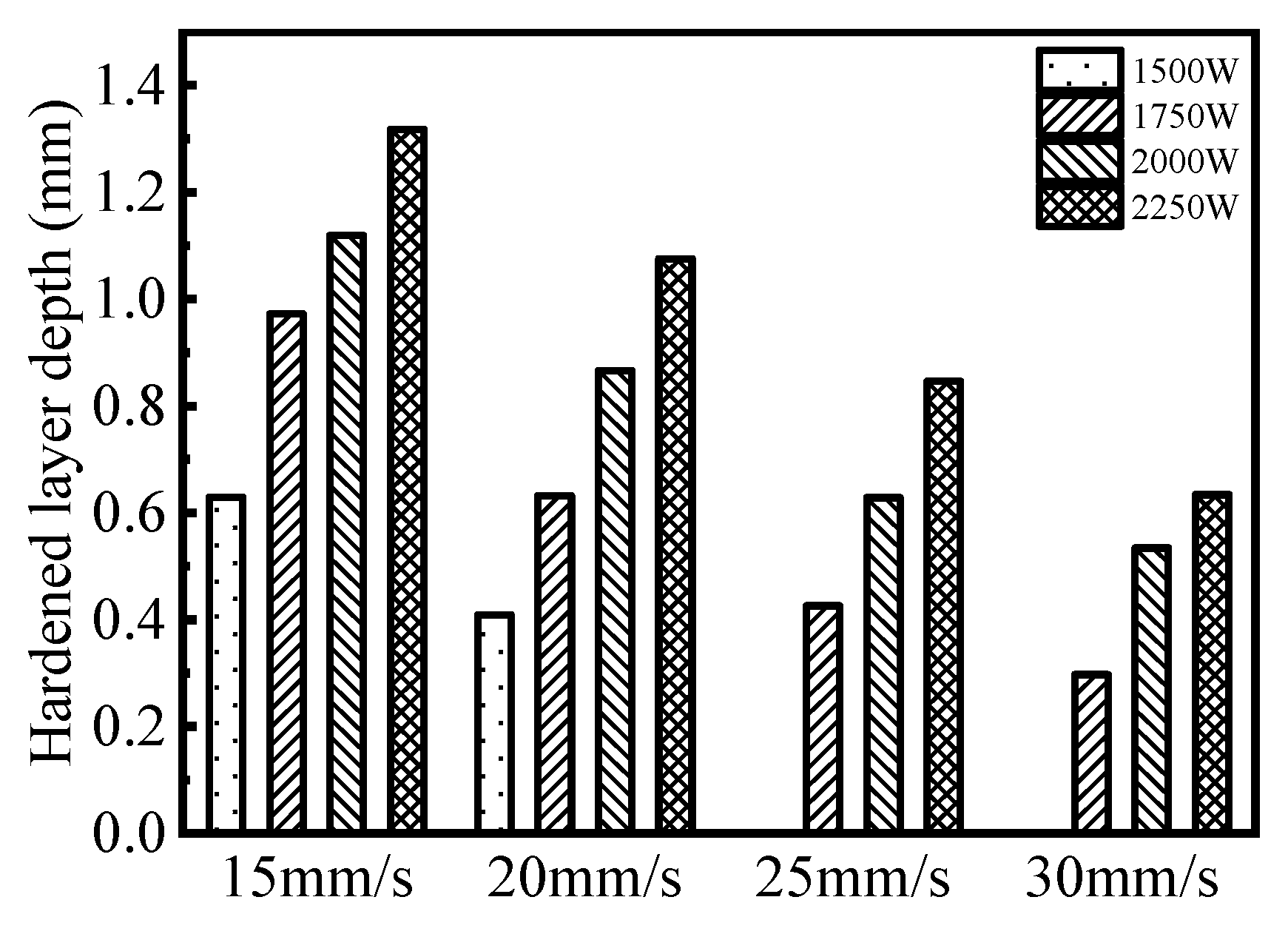
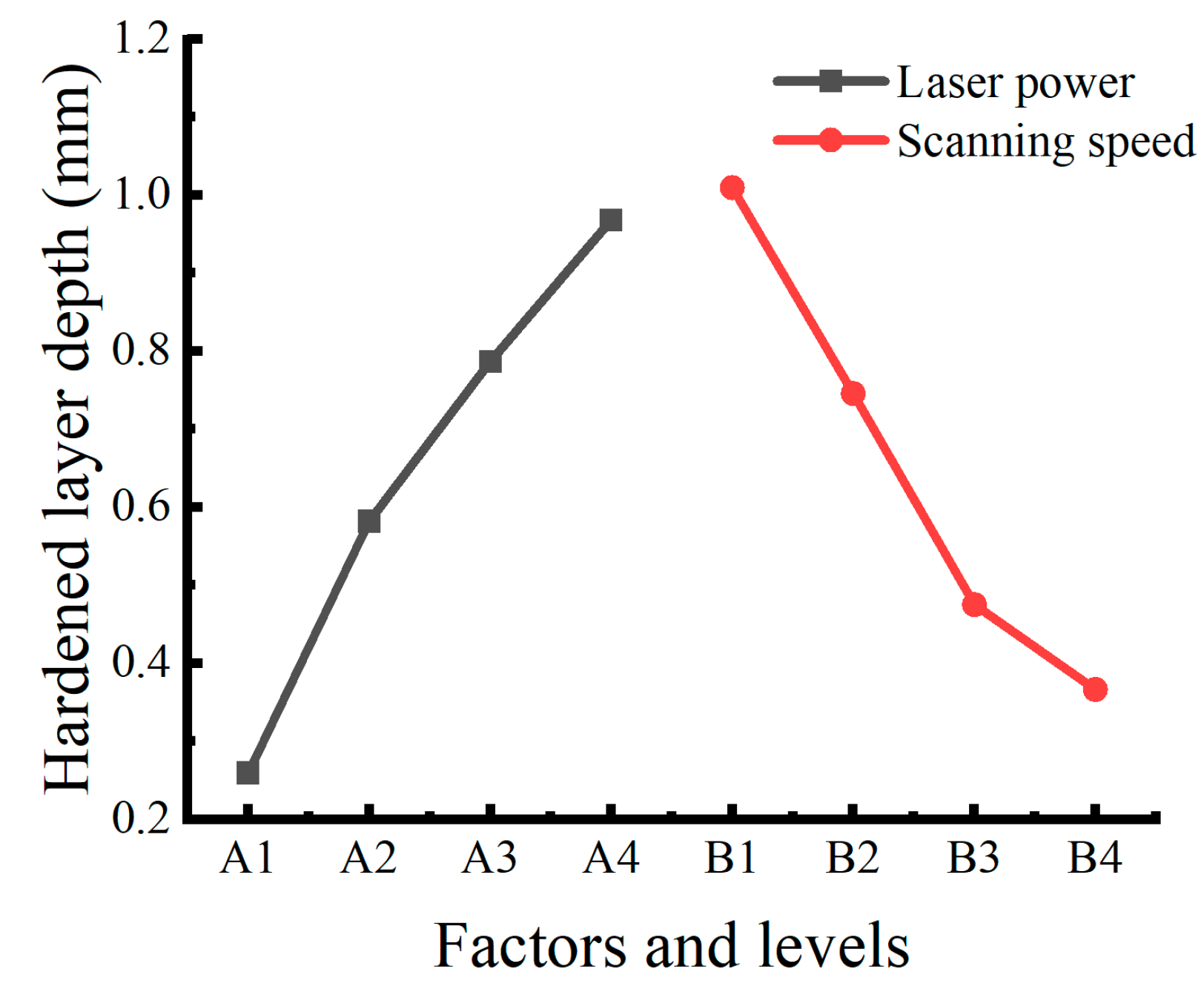
3.2.2. Effect on the Depth of the Hardened Layer on the Surface of the Shield Main Bearing Raceway
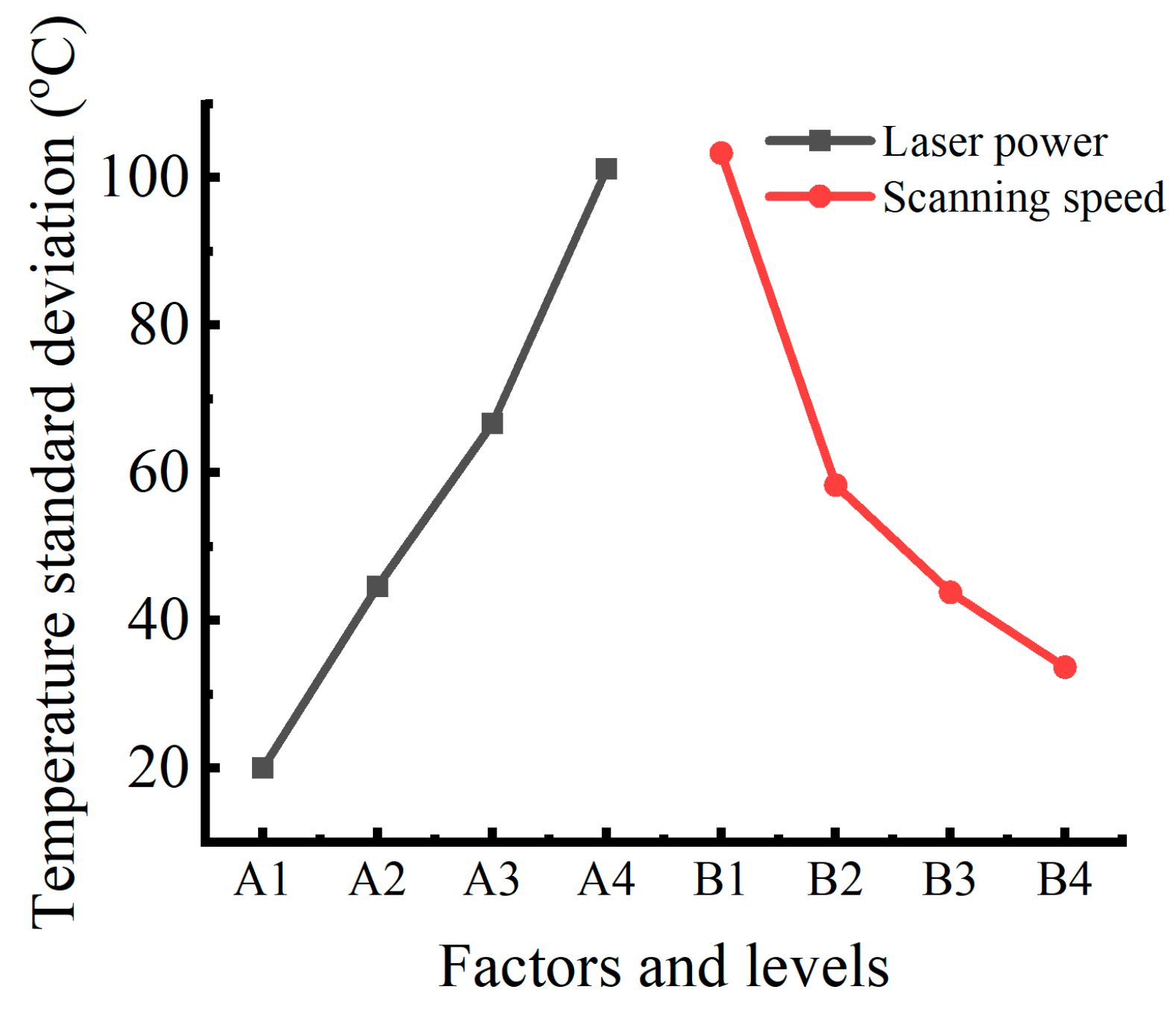
3.2.3. Effect on the Uniformity of the Hardened Layer on the Surface of the Shield Main Bearing Raceway
3.2.4. Multi-Indicator Evaluation of Laser Phase Change Hardening of Shield Main Bearing Raceway Surfaces
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Wang, X.; Lu, X.; Chen, G.; Hu, S.; Su, Y. Research on the temperature field in laser hardening. Opt. Laser Technol. 2006, 38, 8–13. [Google Scholar] [CrossRef] [Green Version]
- Soukieh, M.; Ghani, B.A.; Hammadi, M. Numerical calculations of temperature distribution of double layer metallic surface treated by laser beams. Opt. Laser Technol. 2004, 36, 523–528. [Google Scholar] [CrossRef]
- Amado, J.; Tobar, M.; Ramil, A.; Yáñez, A. Application of the Laplace transform dual reciprocity boundary element method in the modelling of laser heat treatments. Eng. Anal. Bound. Elements 2005, 29, 126–135. [Google Scholar] [CrossRef]
- Kusuhara, T.; Morimoto, J.; Abe, N.; Tsukamoto, M. 3-D Finite element temperature field modelling for direct diode laser hardening of carbon steel. In Proceedings of the 2011 Fourth International Conference on Modeling, Simulation and Applied Optimization, Kuala Lumpur, Malaysia, 19–21 April 2011; pp. 1–4. [Google Scholar] [CrossRef]
- Orazi, L.; Liverani, E.; Ascari, A.; Fortunato, A.; Tomesani, L. Laser surface hardening of large cylindrical components utilizing ring spot geometry. CIRP Ann. 2014, 63, 233–236. [Google Scholar] [CrossRef]
- Karabutov, A.; Sogoyan, M. Interaction of IR laser radiation with periodic metal surfaces. Infrared Phys. Technol. 1995, 36, 191–216. [Google Scholar] [CrossRef]
- Komanduri, R.; Hou, Z. Thermal analysis of laser surface transformation hardening—Optimization of process parameters. Int. J. Mach. Tools Manuf. 2004, 44, 991–1008. [Google Scholar] [CrossRef]
- Rana, J.; Goswami, G.; Jha, S.; Mishra, P.; Prasad, B. Experimental studies on the microstructure and hardness of laser-treated steel specimens. Opt. Laser Technol. 2007, 39, 385–393. [Google Scholar] [CrossRef]
- Kim, J.-D.; Lee, M.-H.; Lee, S.-J.; Kang, W.-J. Laser transformation hardening on rod-shaped carbon steel by Gaussian beam. Trans. Nonferr. Met. Soc. China 2009, 19, 941–945. [Google Scholar] [CrossRef]
- Mioković, T.; Schulze, V.; Vöhringer, O.; Löhe, D. Influence of cyclic temperature changes on the microstructure of AISI 4140 after laser surface hardening. Acta Mater. 2007, 55, 589–599. [Google Scholar] [CrossRef]
- Liu, A.; Previtali, B. Laser surface treatment of grey cast iron by high power diode laser. Phys. Procedia 2010, 5, 439–448. [Google Scholar] [CrossRef] [Green Version]
- Devgun, M.S.; Molian, P.A. Experimental study of la-ser heat-treated bearing steel. J. Mater. Process. Technol. 1990, 23, 41–54. [Google Scholar] [CrossRef]
- Lusquiños, F.; Conde, J.; Bonss, S.; Riveiro, A.; Quintero, F.; Comesaña, R.; Pou, J. Theoretical and experimental analysis of high power diode laser (HPDL) hardening of AISI 1045 steel. Appl. Surf. Sci. 2007, 254, 948–954. [Google Scholar] [CrossRef]
- Yánez, A.; Álvarez, J.; López, A.; Nicolás, G.; Pérez, J.; Ramil, A.; Saavedra, E. Modelling of temperature evolution on metals during laser hardening process. Appl. Surf. Sci. 2002, 186, 611–616. [Google Scholar] [CrossRef]
- Zhang, Q.; Tong, W.; Chen, Z.; Yao, J.; Li, Z.; Feng, K.; Kovalenko, V.S. Influence of spot size on geometric characteristics of laser deep quenching hardened layer of 42CrMo steel. Surf. Technol. 2020, 49, 254–261. [Google Scholar]
- Carslaw, H.S.; Jaeger, J.C. Conduction of Heat in Solids; Oxford University Press: Oxford, UK, 1959. [Google Scholar]
- Landau, L.D.; Lifshitz, E.M. Statistical Physics; Butterworth-Heinemann: Oxford, UK, 1980. [Google Scholar]
- Tani, G.; Fortunato, A.; Ascari, A.; Campana, G. Laser surface hardening of martensitic stainless steel hollow parts. CIRP Ann. 2010, 59, 207–210. [Google Scholar] [CrossRef]
- Zhao, J. High Energy Rate Heat Treatment; Ordnance Industry Press: Beijing, China, 1997. [Google Scholar]
- He, P.; Liu, R.; Hong, R.; Wang, H.; Yang, G.; Lu, C. Hardened raceway calculation analysis of a three-row roller slewing bearing. Int. J. Mech. Sci. 2018, 137, 133–144. [Google Scholar] [CrossRef]


| Element | C | Si | Mn | S | P | Cr | Ni | Cu | Mo |
|---|---|---|---|---|---|---|---|---|---|
| Content | 0.38~0.45 | 0.17~0.37 | 0.50~0.80 | ≤0.035 | ≤0.035 | 0.90~1.2 | ≤0.30 | ≤0.30 | 0.15~0.25 |
| Equipment Parameters | Numerical Value |
|---|---|
| Fiber core diameter | 1000 μm |
| Working temperature | 10–40 °C |
| Operating voltage | 380–480 V |
| Laser wavelength | 980–1020 nm |
| Maximum output power | 4400 W |
| Minimum beam quality | 30 mm.rad |
| Rated continuous operation time | 24 h |
| Level | Factor | |
|---|---|---|
| A: Laser Power P (W) | B: Scanning Speed v (mm/s) | |
| 1 | 1500 | 15 |
| 2 | 1750 | 20 |
| 3 | 2000 | 25 |
| 4 | 2250 | 30 |
| Factor | Evaluation Indicators | ||||||
|---|---|---|---|---|---|---|---|
| No | A: Laser Power P (W) | B: Scanning Speed v (mm/s) | Hardened Layer Width (mm) | Hardened Layer Depth (mm) | Temperature Standard Deviation | ||
| 1 | 1 | 1500 | 1 | 15 | 2.238 | 0.629 | 36.210 |
| 2 | 1 | 1500 | 2 | 20 | 1.492 | 0.409 | 3.882 |
| 3 | 1 | 1500 | 3 | 25 | 0 | 0 | / |
| 4 | 1 | 1500 | 4 | 30 | 0 | 0 | / |
| 5 | 2 | 1750 | 1 | 15 | 3.201 | 0.972 | 80.120 |
| 6 | 2 | 1750 | 2 | 20 | 2.275 | 0.632 | 39.701 |
| 7 | 2 | 1750 | 3 | 25 | 1.582 | 0.426 | 14.119 |
| 8 | 2 | 1750 | 4 | 30 | 1.155 | 0.297 | / |
| 9 | 3 | 2000 | 1 | 15 | 3.944 | 1.119 | 126.112 |
| 10 | 3 | 2000 | 2 | 20 | 3.126 | 0.866 | 77.313 |
| 11 | 3 | 2000 | 3 | 25 | 2.299 | 0.628 | 41.615 |
| 12 | 3 | 2000 | 4 | 30 | 1.774 | 0.534 | 21.596 |
| 13 | 4 | 2250 | 1 | 15 | 4.661 | 1.318 | 170.956 |
| 14 | 4 | 2250 | 2 | 20 | 3.846 | 1.075 | 112.383 |
| 15 | 4 | 2250 | 3 | 25 | 3.078 | 0.846 | 75.715 |
| 16 | 4 | 2250 | 4 | 30 | 2.382 | 0.634 | 45.757 |
| Level | A: Laser Power P (W) | B: Scanning Speed v (mm/s) | |
|---|---|---|---|
| Hardened layer width (mm) | K1 | 3.729455 | 14.04409 |
| K2 | 8.212545 | 10.73791 | |
| K3 | 11.14291 | 6.959182 | |
| K4 | 13.96691 | 5.310636 | |
| k1 | 0.932364 | 3.511023 | |
| k2 | 2.053136 | 2.684477 | |
| k3 | 2.785727 | 1.739795 | |
| k4 | 3.491727 | 1.327659 | |
| Polar difference R | 2.559364 | 2.183364 | |
| Sequence | 1 | 2 |
| Level | A: Laser Power P (W) | B: Scanning Speed v (mm/s) | |
|---|---|---|---|
| Hardened layer deptd (mm) | K1 | 1.038 | 4.038 |
| K2 | 2.327 | 2.982 | |
| K3 | 3.147 | 1.9 | |
| K4 | 3.873 | 1.465 | |
| k1 | 0.2595 | 1.0095 | |
| k2 | 0.58175 | 0.7455 | |
| k3 | 0.78675 | 0.475 | |
| k4 | 0.96825 | 0.36625 | |
| Polar difference R | 0.70875 | 0.64325 | |
| Sequence | 1 | 2 |
| Level | A: Laser Power P(W) | B: Scanning Speed v (mm/s) | |
|---|---|---|---|
| Hardened layer temperature uniformity | K1 | 40.092 | 413.397 |
| K2 | 133.940 | 233.280 | |
| K3 | 266.637 | 131.449 | |
| K4 | 404.811 | 67.353 | |
| k1 | 20.046 | 103.349 | |
| k2 | 44.647 | 58.320 | |
| k3 | 66.659 | 43.816 | |
| k4 | 101.203 | 33.677 | |
| Polar difference R | 81.157 | 69.673 | |
| Sequence | 1 | 2 |
| Evaluation Indicators | Yji | ||||||
|---|---|---|---|---|---|---|---|
| No | Hardened Layer Depth (mm) | Hardened Layer Width (mm) | Temperature Standard Deviation | Yj1 | Yj2 | Yj3 | Overall Rating |
| 1 | 2.238 | 0.629 | 36.21 | 0.318 | 0.429 | 0.663 | 49.498 |
| 2 | 1.492 | 0.409 | 3.882 | 0.181 | 0.238 | 0.790 | 44.440 |
| 3 | 0 | 0 | / | / | / | / | / |
| 4 | 0 | 0 | / | / | / | / | / |
| 5 | 3.201 | 0.972 | 80.12 | 0.550 | 0.748 | 0.450 | 57.914 |
| 6 | 2.275 | 0.632 | 39.701 | 0.326 | 0.432 | 0.647 | 49.174 |
| 7 | 1.582 | 0.426 | 14.119 | 0.195 | 0.250 | 0.754 | 43.783 |
| 8 | 1.155 | 0.297 | / | / | / | / | / |
| 9 | 3.944 | 1.119 | 126.112 | 0.720 | 0.842 | 0.246 | 57.307 |
| 10 | 3.126 | 0.866 | 77.313 | 0.531 | 0.660 | 0.463 | 54.929 |
| 11 | 2.299 | 0.628 | 41.615 | 0.332 | 0.428 | 0.638 | 48.817 |
| 12 | 1.774 | 0.534 | 21.596 | 0.227 | 0.340 | 0.725 | 46.555 |
| 13 | 4.661 | 1.318 | 170.956 | 0.840 | 0.922 | 0.117 | 57.973 |
| 14 | 3.846 | 1.075 | 112.383 | 0.700 | 0.818 | 0.300 | 58.103 |
| 15 | 3.078 | 0.846 | 75.715 | 0.520 | 0.642 | 0.471 | 54.317 |
| 16 | 2250 | 30 | 2.382 | 0.511 | 0.481 | 0.268 | 48.716 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
He, P.; Ding, Y.; Jiang, S.; Zhang, H.; Shen, T.; Wang, Y. Process Parameters Analysis of Laser Phase Transformation Hardening on the Raceway Surface of Shield Main Bearing. Photonics 2023, 10, 287. https://doi.org/10.3390/photonics10030287
He P, Ding Y, Jiang S, Zhang H, Shen T, Wang Y. Process Parameters Analysis of Laser Phase Transformation Hardening on the Raceway Surface of Shield Main Bearing. Photonics. 2023; 10(3):287. https://doi.org/10.3390/photonics10030287
Chicago/Turabian StyleHe, Peiyu, Yi Ding, Shiying Jiang, Hengjie Zhang, Tianyu Shen, and Yun Wang. 2023. "Process Parameters Analysis of Laser Phase Transformation Hardening on the Raceway Surface of Shield Main Bearing" Photonics 10, no. 3: 287. https://doi.org/10.3390/photonics10030287
APA StyleHe, P., Ding, Y., Jiang, S., Zhang, H., Shen, T., & Wang, Y. (2023). Process Parameters Analysis of Laser Phase Transformation Hardening on the Raceway Surface of Shield Main Bearing. Photonics, 10(3), 287. https://doi.org/10.3390/photonics10030287




