Experimental Investigation on the Mode Characteristics of an Excited-State Quantum Dot Laser under Concave Mirror Optical Feedback
Abstract
1. Introduction
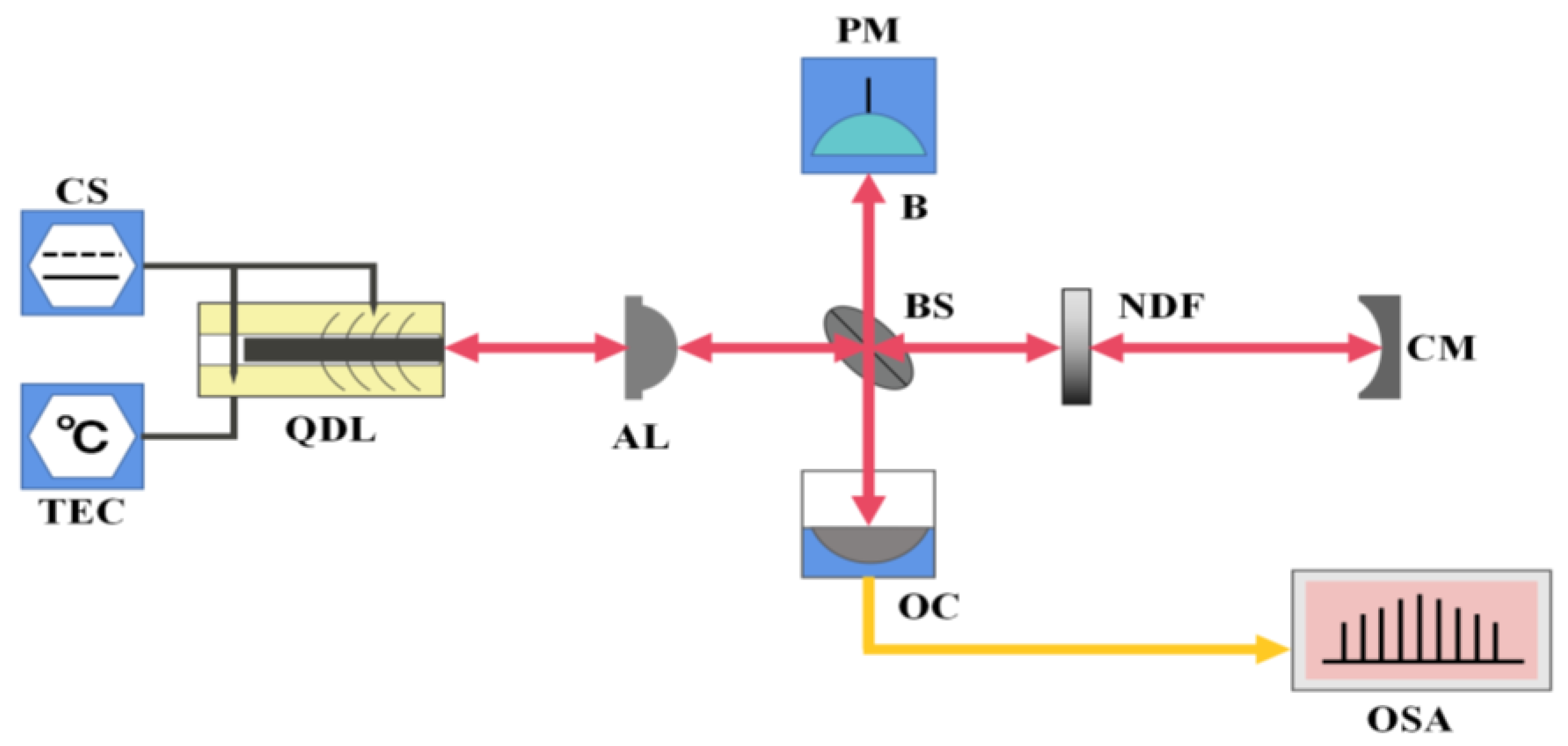
2. Experimental Setup
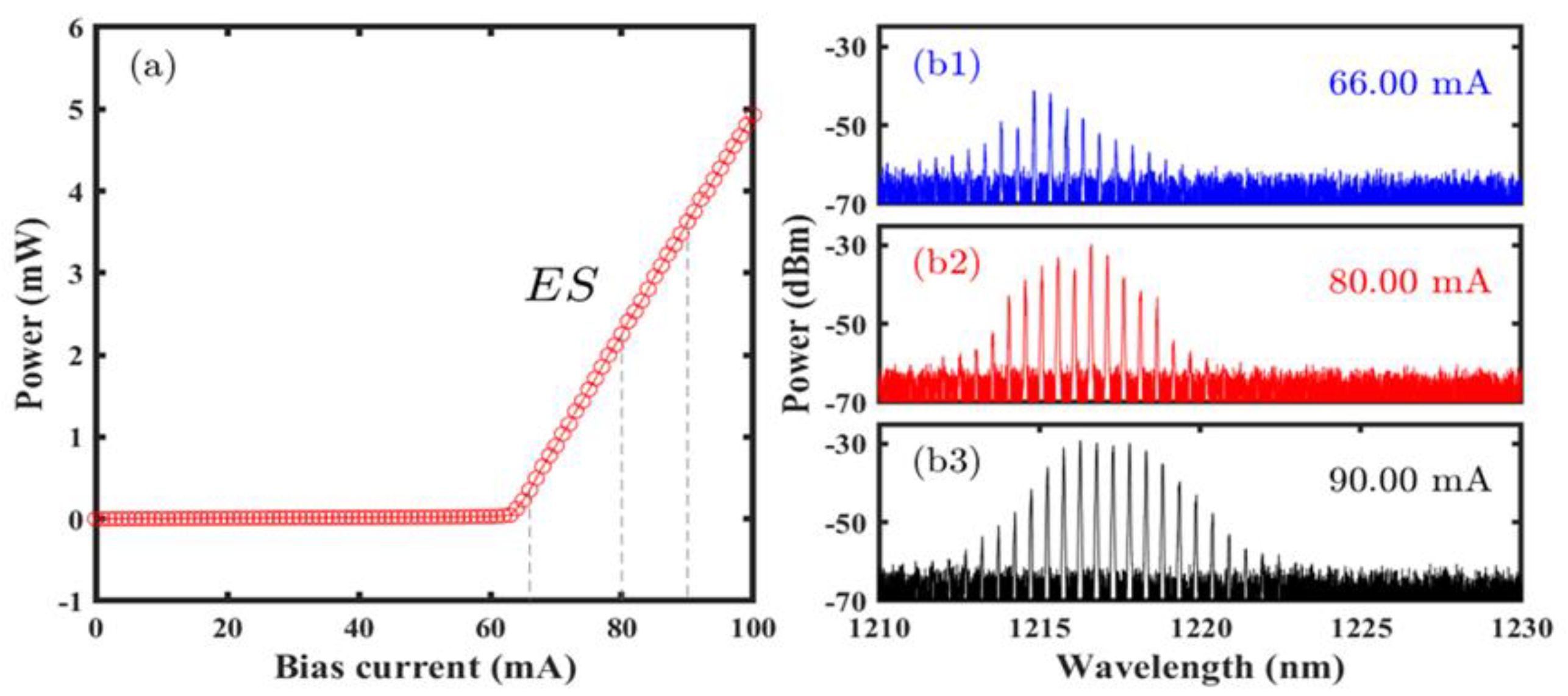
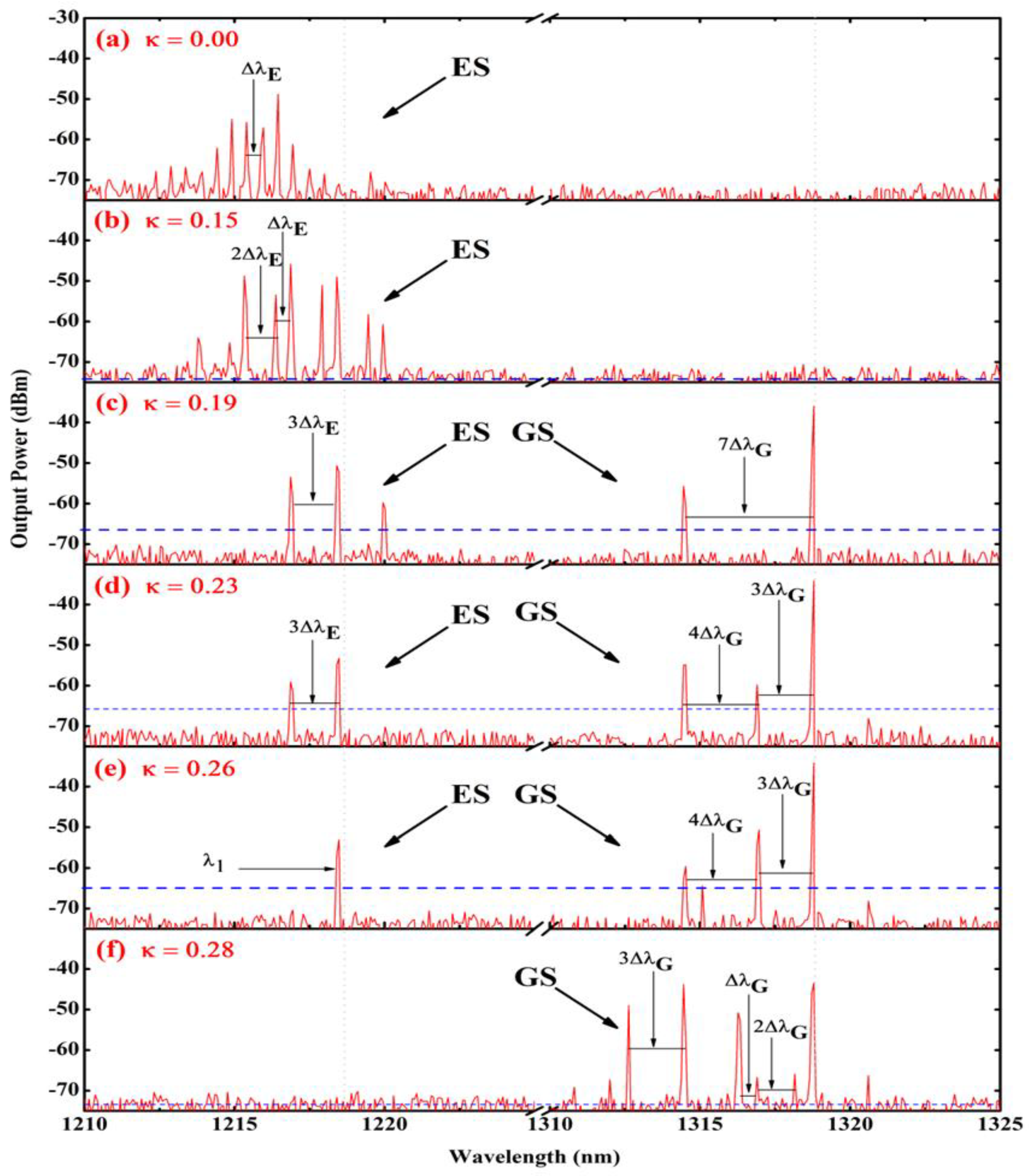
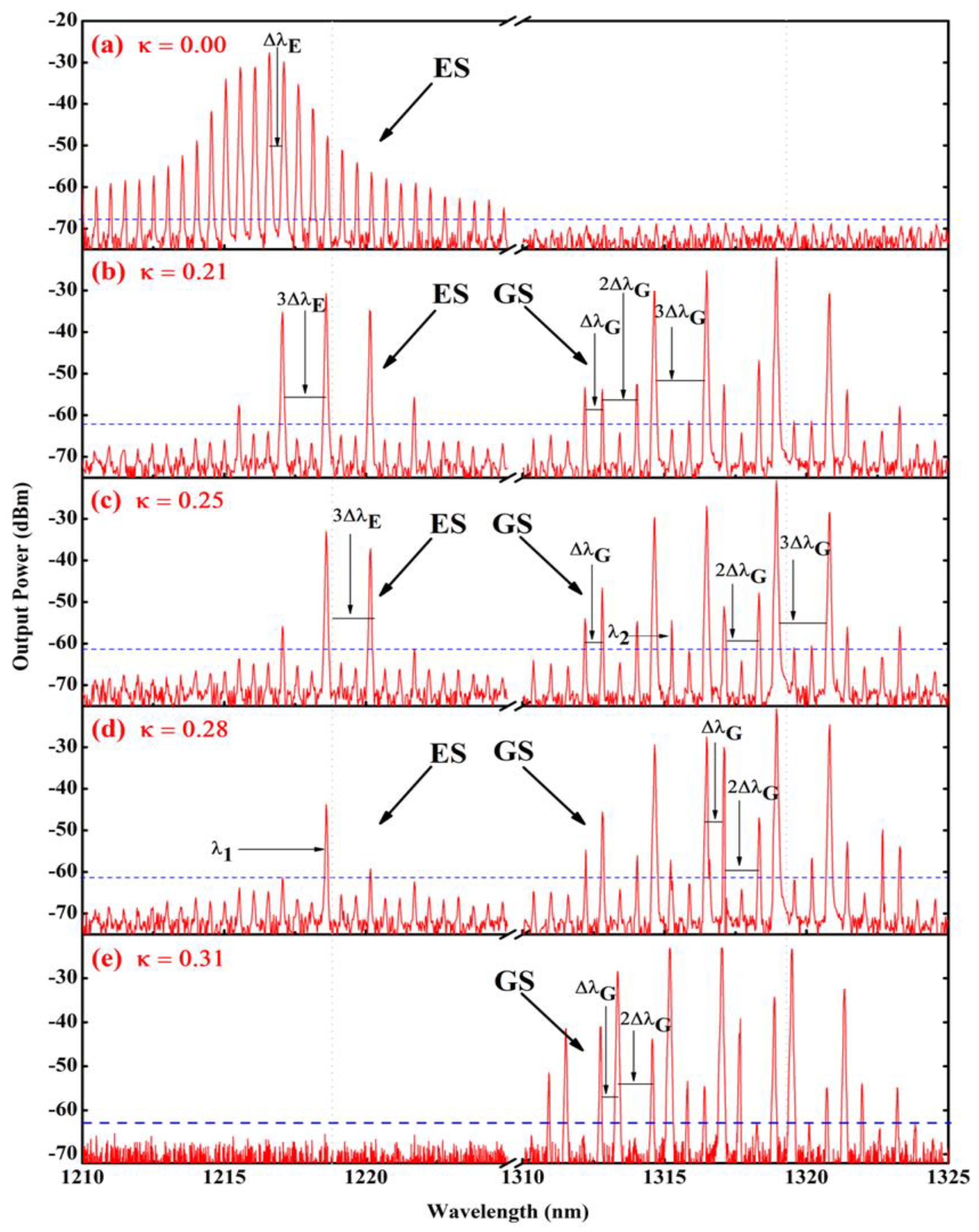
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Dillane, M.; Lingnau, B.; Viktorov, E.A.; Kelleher, B. Mapping the Stability and Dynamics of Optically Injected Dual State Quantum Dot Lasers. Photonics 2022, 9, 101. [Google Scholar] [CrossRef]
- Holzinger, S.; Redlich, C.; Lingnau, B.; Schmidt, M.; Helversen, M.V.; Beyer, J.; Schneider, C.; Kamp, M.; Hofling, S.; Ludge, K.; et al. Tailoring the mode-switching dynamics in quantum-dot micropillar lasers via time-delayed optical feedback. Opt. Express 2018, 26, 22457–22470. [Google Scholar] [CrossRef] [PubMed]
- Chengui, G.R.G.; Jacques, K.; Woafo, P.; Chembo, Y.K. Nonlinear dynamics in an optoelectronic feedback delay oscillator with piecewise linear transfer functions from the laser diode and photodiode. Phys. Rev. E 2020, 102, 042217. [Google Scholar] [CrossRef] [PubMed]
- Jiang, N.; Xue, C.P.; Lv, Y.X.; Qiu, K. Physically enhanced secure wavelength division multiplexing chaos communication using multimode semiconductor lasers. Nonlinear Dyn. 2016, 86, 1937–1949. [Google Scholar] [CrossRef]
- Kawaguchi, Y.; Okuma, T.; Kanno, K.; Uchida, A. Entropy rate of chaos in an optically injected semiconductor laser for physical random number generation. Opt. Express 2021, 29, 2442–2457. [Google Scholar] [CrossRef]
- Zhukovsky, S.V.; Chigrin, D.N. Optical memory based on ultrafast wavelength switching in a bistable microlaser. Opt. Lett. 2009, 34, 3310–3312. [Google Scholar] [CrossRef] [PubMed]
- Salvide, M.F.; Masoller, C.; Torre, M.S. All-Optical Stochastic Logic Gate Based on a VCSEL With Tunable Optical Injection. IEEE J. Quantum Electron. 2013, 49, 886–893. [Google Scholar] [CrossRef]
- Liu, A.Y.; Zhang, C.; Norman, J.; Snyder, A.; Lubyshev, D.; Fastenau, J.M.; Liu, A.W.K.; Gossard, A.C.; Bowers, J.E. High performance continuous wave 1.3 µm quantum dot lasers on silicon. Appl. Phys. Lett. 2014, 104, 041104. [Google Scholar] [CrossRef]
- Nishi, K.; Takemasa, K.; Sugawara, M.; Arakawa, Y. Development of Quantum Dot Lasers for Data-Com and Silicon Photonics Applications. IEEE J. Sel. Top. Quantum Electron. 2017, 23, 1901007. [Google Scholar] [CrossRef]
- Yamamoto, N.; Akahane, K.; Kawanishi, T.; Sotobayashi, H.; Yoshioka, Y.; Takai, H. Characterization of Wavelength-Tunable Quantum Dot External Cavity Laser for 1.3-µm-Waveband Coherent Light Sources. Jpn. J. Appl. Phys. 2012, 51, 02BG08. [Google Scholar] [CrossRef]
- Li, Q.Z.; Wang, X.; Zhang, Z.Y.; Chen, H.M.; Huang, Y.Q.; Hou, C.C.; Wang, J.; Zhang, R.Y.; Ning, J.Q.; Min, J.H.; et al. Development of Modulation p-Doped 1310 nm InAs/GaAs Quantum Dot Laser Materials and Ultrashort Cavity Fabry-Perot and Distributed-Feedback Laser Diodes. ACS Photonics 2018, 5, 1084–1093. [Google Scholar] [CrossRef]
- Virte, M.; Panajotov, K.; Sciamanna, M. Mode Competition Induced by Optical Feedback in Two-Color Quantum Dot Lasers. IEEE J. Quantum Electron. 2013, 49, 578–585. [Google Scholar] [CrossRef]
- Saito, H.; Nishi, K.; Kamei, A.; Sugou, S. Low chirp observed in directly modulated quantum dot lasers. IEEE Photonics Technol. Lett. 2000, 12, 1298–1300. [Google Scholar] [CrossRef]
- Xu, P.F.; Yang, T.; Ji, H.M.; Cao, Y.L.; Gu, Y.X.; Liu, Y.; Ma, W.Q.; Wang, Z.G. Temperature-dependent modulation characteristics for 1.3 µm InAs/GaAs quantum dot lasers. J. Appl. Phys. 2010, 107, 013102. [Google Scholar]
- Li, S.G.; Gong, Q.; Cao, C.F.; Wang, X.Z.; Chen, P.; Yue, L.; Liu, Q.B.; Wang, H.L.; Ma, C.H. Temperature dependent lasing characteristics of InAs/InP(100) quantum dot laser. Mater. Sci. Semicond. Process. 2012, 15, 86–90. [Google Scholar] [CrossRef]
- Wang, C.; Osinski, M.; Even, J.; Grillot, F. Phase-amplitude coupling characteristics in directly modulated quantum dot lasers. Appl. Phys. Lett. 2014, 105, 221114. [Google Scholar] [CrossRef]
- O’Brien, D.; Hegarty, S.P.; Huyet, G.; Uskov, A.V. Sensitivity of quantum-dot semiconductor lasers to optical feedback. Opt. Lett. 2004, 29, 1072–1074. [Google Scholar] [CrossRef]
- Azouigui, S.; Dagens, B.; Lelarge, F.; Provost, J.G.; Make, D.; Le Gouezigou, O.; Accard, A.; Martinez, A.; Merghem, K.; Grillot, F.; et al. Optical Feedback Tolerance of Quantum-Dot- and Quantum-Dash-Based Semiconductor Lasers Operating at 1.55 µm. IEEE J. Sel. Top. Quantum Electron. 2009, 15, 764–773. [Google Scholar] [CrossRef]
- Zhukov, A.E.; Kovsh, A.R. Quantum dot diode lasers for optical communication systems. Quantum Electron. 2008, 38, 409–423. [Google Scholar] [CrossRef]
- Norman, J.C.; Jung, D.; Zhang, Z.Y.; Wan, Y.T.; Liu, S.T.; Shang, C.; Herrick, R.W.; Chow, W.W.; Gossard, A.C.; Bowers, J.E. A Review of High-Performance Quantum Dot Lasers on Silicon. IEEE J. Quantum Electron. 2019, 55, 2000511. [Google Scholar] [CrossRef]
- Norman, J.C. The future of quantum dot photonic integrated circuits. APL Photonics 2018, 3, 030901. [Google Scholar] [CrossRef]
- Wang, C.; Raghunathan, R.; Schires, K.; Chan, S.C.; Lester, L.F.; Grillot, F. Optically injected InAs/GaAs quantum dot laser for tunable photonic microwave generation. Opt. Lett. 2016, 41, 1153–1156. [Google Scholar] [CrossRef] [PubMed]
- Jiang, Z.F.; Wu, Z.M.; Yang, W.Y.; Hu, C.X.; Jin, Y.H.; Xiao, Z.Z.; Xia, G.Q. Numerical investigation on photonic microwave generation by a sole excited-state emitting quantum dot laser with optical injection and optical feedback. Chin. Phys. B. 2021, 30, 050504. [Google Scholar] [CrossRef]
- Viktorov, E.A.; Mandel, P.; Tanguy, Y.; Houlihan, J.; Huyet, G. Electron-hole asymmetry and two-state lasing in quantum dot lasers. Appl. Phys. Lett. 2005, 87, 053113. [Google Scholar] [CrossRef]
- Meinecke, S.; Lingnau, B.; Rohm, A.; Ludge, K. Stability of Optically Injected Two-State Quantum-Dot Lasers. Ann. Phys. 2017, 529, 1600279. [Google Scholar] [CrossRef]
- Grillot, F.; Norman, J.C.; Duan, J.N.; Zhang, Z.Y.; Dong, B.Z.; Huang, H.M.; Chow, W.W.; Bowers, J.E. Physics and applications of quantum dot lasers for silicon photonics. Nanophotonics 2020, 9, 1271–1286. [Google Scholar] [CrossRef]
- Huyet, G.; O’Brien, D.; Hegarty, S.P.; McInerney, J.G.; Uskov, A.V.; Bimberg, D.; Ribbat, C.; Ustinov, V.M.; Zhukov, A.E.; Mikhrin, S.S.; et al. Quantum dot semiconductor lasers with optical feedback. Phys. Status Solidi A 2004, 201, 345–352. [Google Scholar] [CrossRef]
- O’Brien, D.; Hegarty, S.P.; Huyet, G.; McInerney, J.G.; Kettler, T.; Laemmlin, M.; Bimberg, D.; Ustinov, V.M.; Zhukov, A.E.; Mikhrin, S.S.; et al. Feedback sensitivity of 1.3 mu m InAs/GaAs quantum dot lasers. Electron. Lett. 2003, 39, 1819–1820. [Google Scholar] [CrossRef]
- Stevens, B.J.; Childs, D.T.D.; Shahid, H.; Hogg, R.A. Direct modulation of excited state quantum dot lasers. Appl. Phys. Lett. 2009, 95, 061101. [Google Scholar] [CrossRef]
- Lin, L.C.; Chen, C.Y.; Huang, H.M.; Arsenijevic, D.; Bimberg, D.; Grillot, F.; Lin, F.Y. Comparison of optical feedback dynamics of InAs/GaAs quantum-dot lasers emitting solely on ground or excited states. Opt. Lett. 2018, 43, 210–213. [Google Scholar] [CrossRef]
- Arsenijevic, D.; Schliwa, A.; Schmeckebier, H.; Stubenrauch, M.; Spiegelberg, M.; Bimberg, D.; Mikhelashvili, V.; Eisenstein, G. Comparison of dynamic properties of ground- and excited-state emission in p-doped InAs/GaAs quantum-dot lasers. Appl. Phys. Lett. 2014, 104, 181101. [Google Scholar] [CrossRef]
- Huang, H.M.; Lin, L.C.; Chen, C.Y.; Arsenijevic, D.; Bimberg, D.; Lin, F.Y.; Grillot, F. Multimode optical feedback dynamics in InAs/GaAs quantum dot lasers emitting exclusively on ground or excited states: Transition from short- to long-delay regimes. Opt. Express 2018, 26, 1743–1751. [Google Scholar] [CrossRef] [PubMed]
- Virte, M.; Breuer, S.; Sciamanna, M.; Panajotov, K. Switching between ground and excited states by optical feedback in a quantum dot laser diode. Appl. Phys. Lett. 2014, 105, 121109. [Google Scholar] [CrossRef]
- Tykalewicz, B.; Goulding, D.; Hegarty, S.P.; Huyet, G.; Byrne, D.; Phelan, R.; Kelleher, B. All-optical switching with a dual-state, single-section quantum dot laser via optical injection. Opt. Lett. 2014, 39, 4607–4610. [Google Scholar] [CrossRef]
- Virte, M.; Pawlus, R.; Sciamanna, M.; Panajotov, K.; Breuer, S. Energy exchange between modes in a multimode two-color quantum dot laser with optical feedback. Opt. Lett. 2016, 41, 3205–3208. [Google Scholar] [CrossRef]
- Tykalewicz, B.; Goulding, D.; Hegarty, S.P.; Huyet, G.; Dubinkin, I.; Fedorov, N.; Erneux, T.; Viktorov, E.A.; Kelleher, B. Optically induced hysteresis in a two-state quantum dot laser. Opt. Lett. 2016, 41, 1034–1037. [Google Scholar] [CrossRef]
- Kelleher, B.; Tykalewicz, B.; Goulding, D.; Fedorov, N.; Dubinkin, I.; Erneux, T.; Viktorov, E.A. Two-color bursting oscillations. Sci. Rep. 2017, 7, 8414. [Google Scholar] [CrossRef]
- Dillane, M.; Dubinkin, I.; Fedorov, N.; Erneux, T.; Goulding, D.; Kelleher, B.; Viktorov, E.A. Excitable interplay between lasing quantum dot states. Phys. Rev. E 2019, 100, 012202. [Google Scholar] [CrossRef]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zheng, Y.; Xia, G.; Lin, X.; Wang, Q.; Wang, H.; Jiang, C.; Chen, H.; Wu, Z. Experimental Investigation on the Mode Characteristics of an Excited-State Quantum Dot Laser under Concave Mirror Optical Feedback. Photonics 2023, 10, 166. https://doi.org/10.3390/photonics10020166
Zheng Y, Xia G, Lin X, Wang Q, Wang H, Jiang C, Chen H, Wu Z. Experimental Investigation on the Mode Characteristics of an Excited-State Quantum Dot Laser under Concave Mirror Optical Feedback. Photonics. 2023; 10(2):166. https://doi.org/10.3390/photonics10020166
Chicago/Turabian StyleZheng, Yanfei, Guangqiong Xia, Xiaodong Lin, Qingqing Wang, Hongpei Wang, Cheng Jiang, Hongmei Chen, and Zhengmao Wu. 2023. "Experimental Investigation on the Mode Characteristics of an Excited-State Quantum Dot Laser under Concave Mirror Optical Feedback" Photonics 10, no. 2: 166. https://doi.org/10.3390/photonics10020166
APA StyleZheng, Y., Xia, G., Lin, X., Wang, Q., Wang, H., Jiang, C., Chen, H., & Wu, Z. (2023). Experimental Investigation on the Mode Characteristics of an Excited-State Quantum Dot Laser under Concave Mirror Optical Feedback. Photonics, 10(2), 166. https://doi.org/10.3390/photonics10020166





