Optical Properties Analysis of Scattering Media Based on GI-OCT Imaging
Abstract
1. Introduction
2. Experiment and Methods
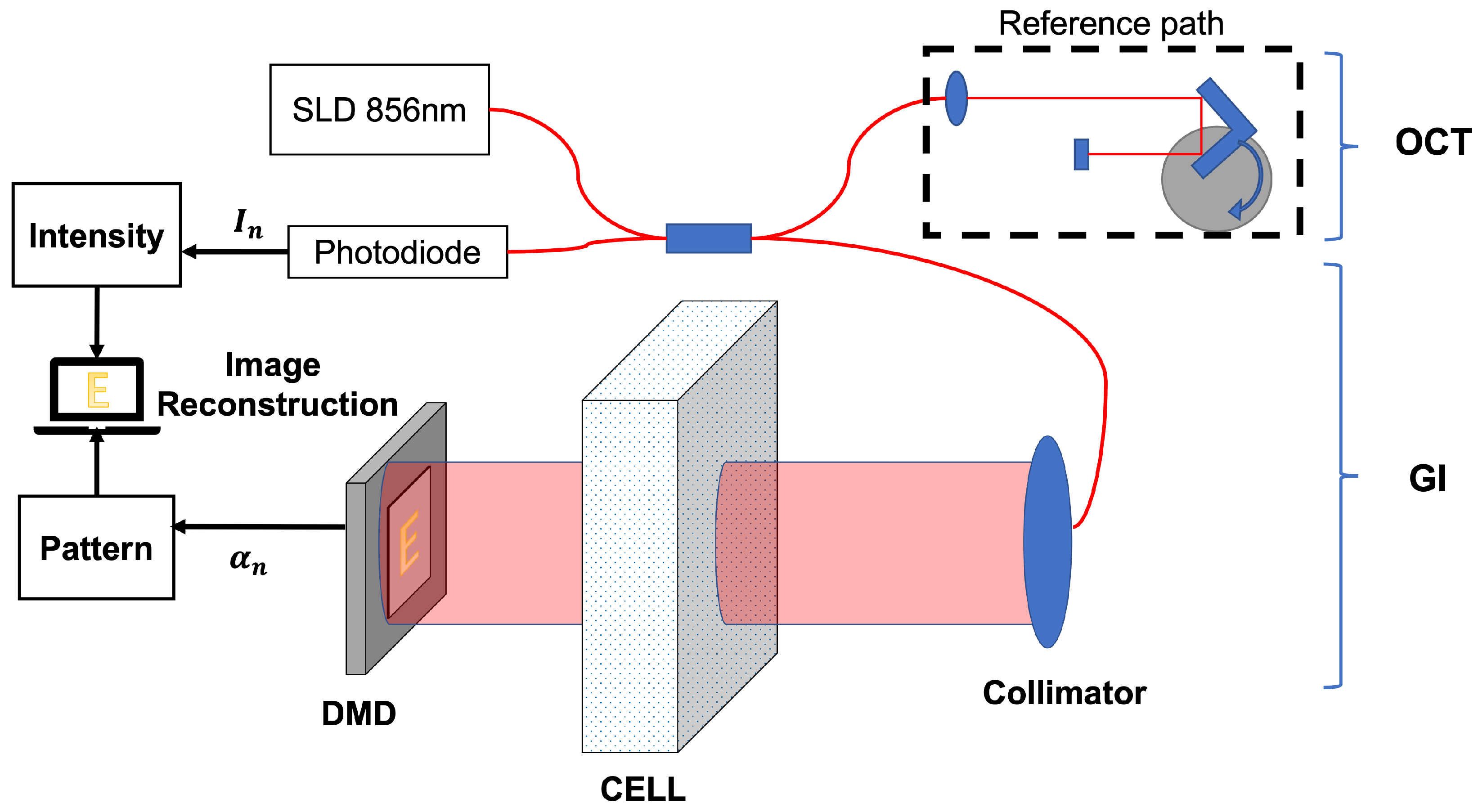
2.1. Experiment Setup and Sample Target
2.2. Method
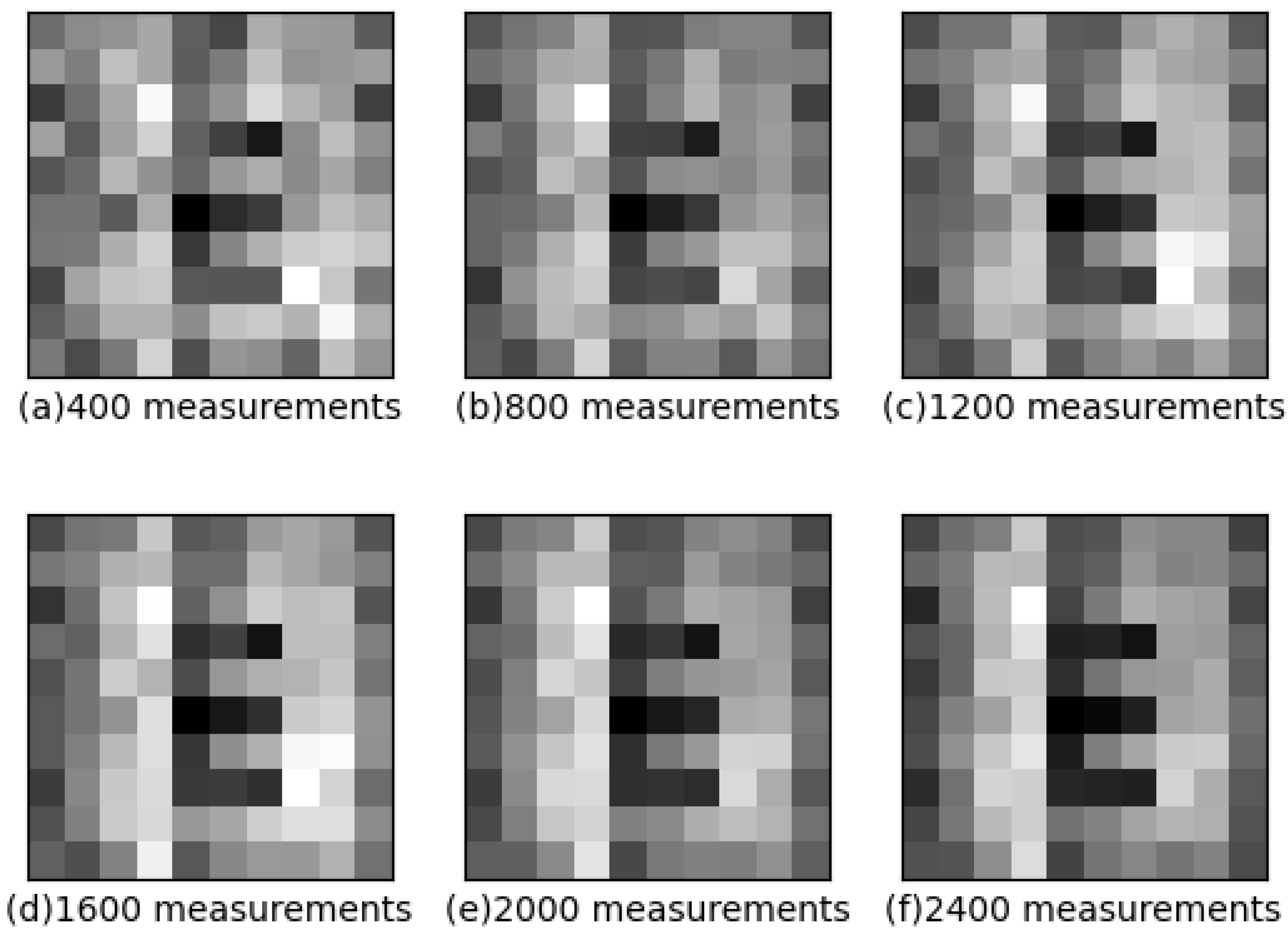
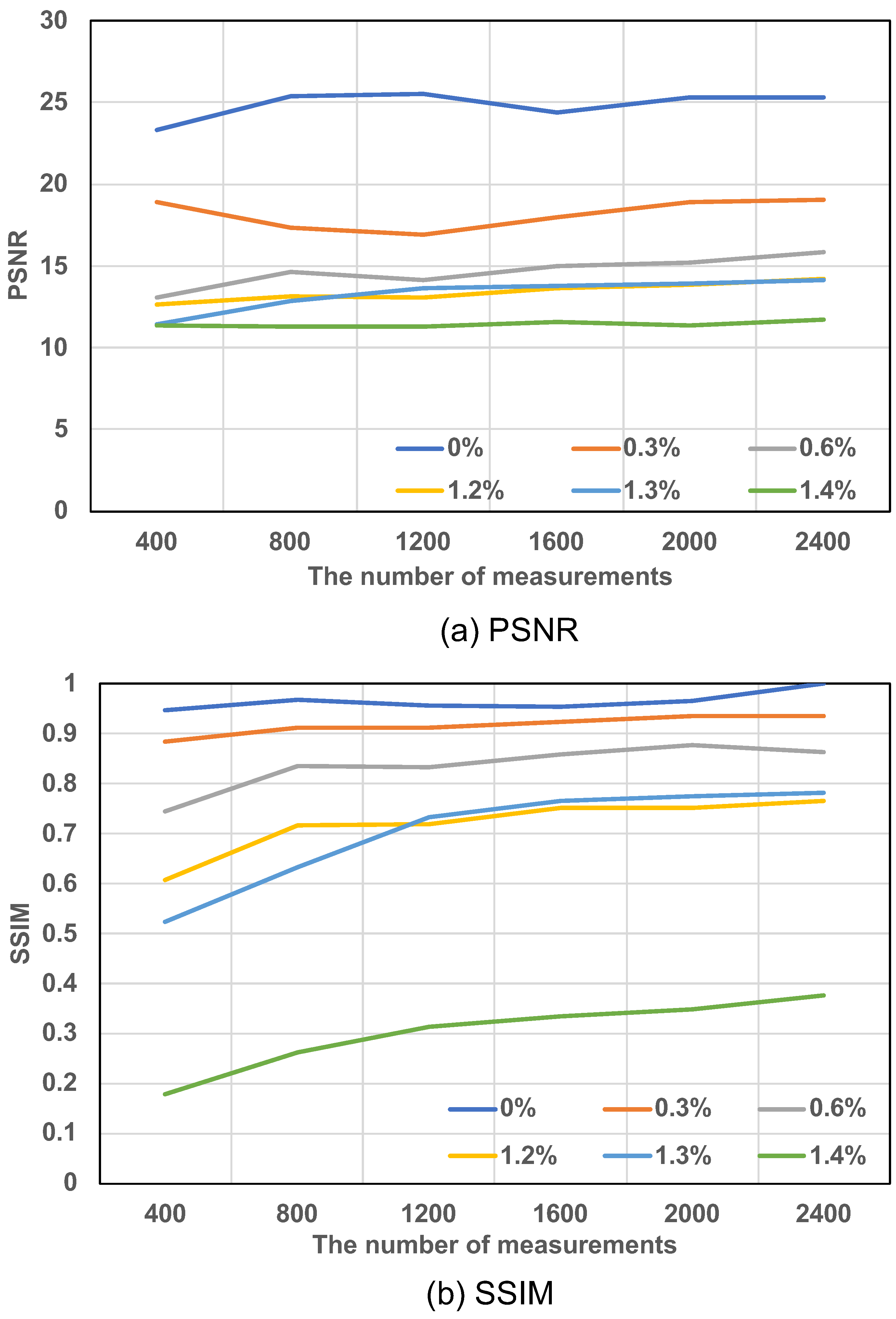
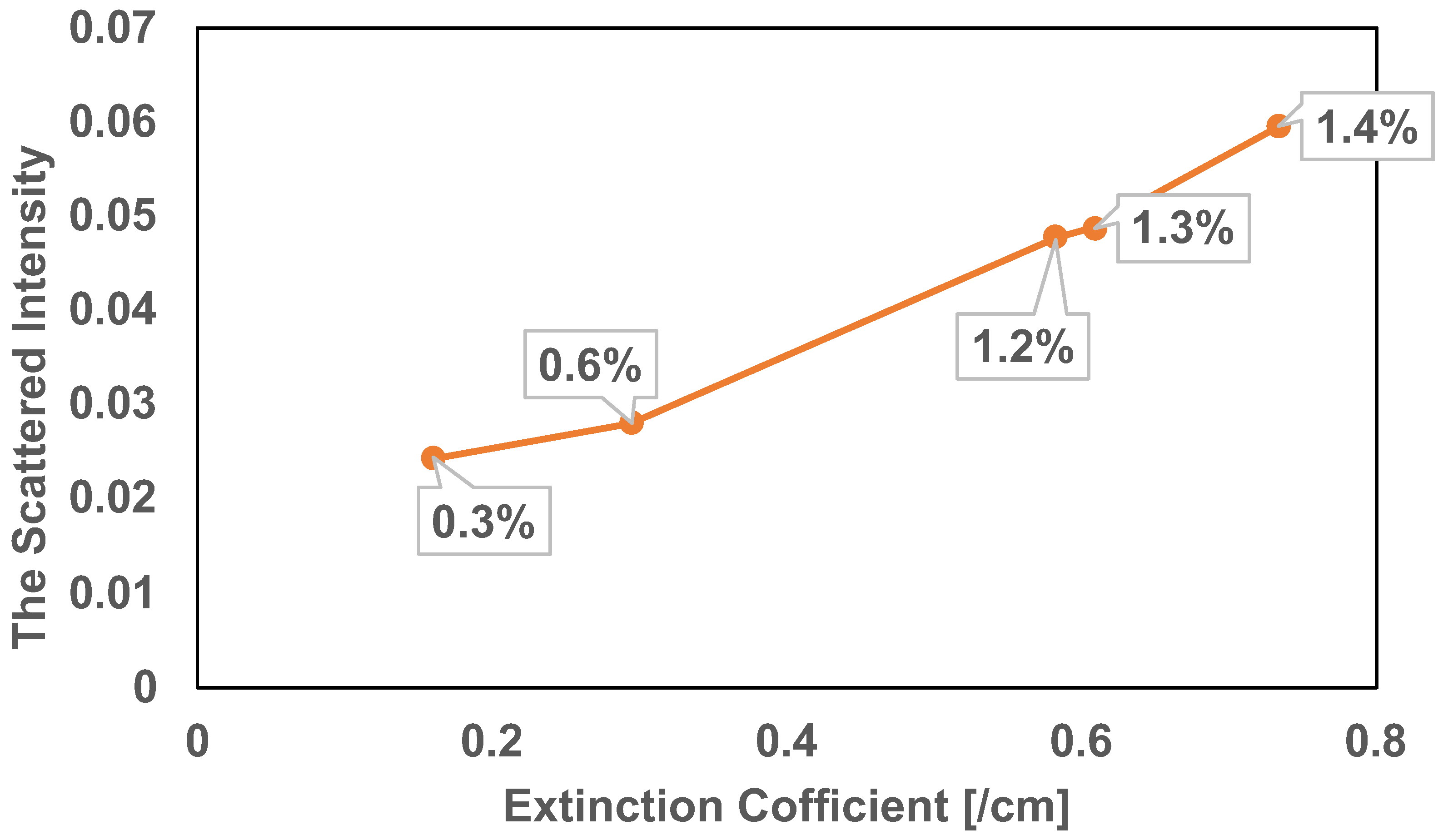
3. Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Huang, D.; Swanson, E.A.; Lin, C.P.; Schuman, J.S.; Stinson, W.G.; Chang, W.; Hee, M.R.; Flotte, T.; Gregory, K.; Puliafito, C.A.; et al. Optical coherence tomography. Science 1991, 254, 1178–1181. [Google Scholar] [CrossRef] [PubMed]
- Gambichler, T.; Moussa, G.; Sand, M.; Sand, D.; Altmeyer, P.; Hoffmann, K. Applications of optical coherence tomography in dermatology. J. Dermatol. Sci. 2005, 40, 85–94. [Google Scholar] [CrossRef]
- Sinclair, H.; Bourantas, C.; Bagnall, A.; Mintz, G.S.; Kunadian, V. OCT for the identification of vulnerable plaque in acute coronary syndrome. JACC Cardiovasc. Imaging 2015, 8, 198–209. [Google Scholar] [CrossRef] [PubMed]
- Schwartz, M.; Levine, A.; Markowitz, O. Optical coherence tomography in dermatology. Cutis 2017, 100, 163–166. [Google Scholar] [CrossRef] [PubMed]
- Spaide, R.F.; Fujimoto, J.G.; Waheed, N.K.; Sadda, S.R.; Staurenghi, G. Optical coherence tomography angiography. Prog. Retin. Eye Res. 2018, 64, 1–55. [Google Scholar] [CrossRef]
- Vabre, L.; Dubois, A.; Boccara, C.; Vabre, L.; Dubois, A.; Boccara, C.; Vabre, L.; Dubois, A.; Boccara, A.C. Thermal-light full-field optical coherence tomography. Opt. Lett. 2002, 27, 530–532. [Google Scholar]
- Kirillin, M.Y.; Priezzhev, A.V.; Myllylä, R. Role of multiple scattering in formation of OCT skin images. Quantum Electron. 2008, 38, 570–575. [Google Scholar] [CrossRef]
- Tamaki, T.; Yuan, B.; Raytchev, B.; Kaneda, K.; Mukaigawa, Y. Multiple-scattering optical tomography with layered material. In Proceedings of the 2013 International Conference on Signal-Image Technology and Internet-Based Systems, SITIS 2013, Kyoto, Japan, 2–5 December 2013; pp. 93–99. [Google Scholar] [CrossRef]
- Tearney, G.J.; Brezinski, M.E.; Bouma, B.E.; Hee, M.R.; Southern, J.F.; Fujimoto, J.G. Determination of the refractive index of highly scattering human tissue by optical coherence tomography. Opt. Lett. 1995, 20, 2258. [Google Scholar] [CrossRef]
- Levitz, D.; Thrane, L.; Frosz, M.H.; Andersen, P.E.; Andersen, C.B.; Andersson-Engels, S.; Valanciunaite, J.; Swartling, J.; Hansen, P.R. Determination of optical scattering properties of highly-scattering media in optical coherence tomography images. Opt. Express 2004, 12, 249. [Google Scholar] [CrossRef]
- Choi, Y.; Hillman, T.R.; Choi, W.; Lue, N.; Dasari, R.R.; So, P.T.; Choi, W.; Yaqoob, Z. Measurement of the time-resolved reflection matrix for enhancing light energy delivery into a scattering medium. Phys. Rev. Lett. 2013, 111, 243901. [Google Scholar] [CrossRef]
- Lindell, D.B.; Wetzstein, G. Three-dimensional imaging through scattering media based on confocal diffuse tomography. Nat. Commun. 2020, 11, 4517. [Google Scholar] [CrossRef]
- Shapiro, J.H. Computational ghost imaging. Phys. Rev. A 2008, 78, 061802. [Google Scholar] [CrossRef]
- Katkovnik, V.; Astola, J. Compressive sensing computational ghost imaging. J. Opt. Soc. Am. A 2012, 29, 1556. [Google Scholar] [CrossRef] [PubMed]
- Bromberg, Y.; Katz, O.; Silberberg, Y. Ghost imaging with a single detector. Phys. Rev. A 2009, 79, 053840. [Google Scholar] [CrossRef]
- Ryczkowski, P.; Barbier, M.; Friberg, A.T.; Dudley, J.M.; Genty, G. Ghost imaging in the time domain. Nat. Photonics 2016, 10, 167–170. [Google Scholar] [CrossRef]
- Devaux, F.; Moreau, P.A.; Denis, S.; Lantz, E. Computational temporal ghost imaging. Optica 2016, 3, 698. [Google Scholar] [CrossRef]
- Zhao, C.; Gong, W.; Chen, M.; Li, E.; Wang, H.; Xu, W.; Han, S. Ghost imaging lidar via sparsity constraints. Appl. Phys. Lett. 2012, 101, 141123. [Google Scholar] [CrossRef]
- Chen, W.; Chen, X. Ghost imaging for three-dimensional optical security. Appl. Phys. Lett. 2013, 103, 221106. [Google Scholar] [CrossRef]
- Olivieri, L.; Gongora, J.S.T.; Peters, L.; Cecconi, V.; Cutrona, A.; Tunesi, J.; Tucker, R.; Pasquazi, A.; Peccianti, M. Hyperspectral terahertz microscopy via nonlinear ghost imaging. Optica 2020, 7, 186. [Google Scholar] [CrossRef]
- Miot, C.A.G.A.; Yczkowski, P.I.R.; Riberg, A.R.I.T.F.; Udley, J.O.H.N.M.D.; Enty, G.O.G. Ghost optical coherence tomography. Opt. Express 2019, 27, 24114–24122. [Google Scholar]
- Li, F.; Zhao, M.; Tian, Z.; Willomitzer, F.; Cossairt, O. Compressive ghost imaging through scattering media with deep learning. Opt. Express 2020, 28, 17395. [Google Scholar] [CrossRef] [PubMed]
- Gong, W.; Han, S. Correlated imaging in scattering media. Opt. Lett. 2011, 36, 394. [Google Scholar] [CrossRef]
- Vellekoop, I.M.; Mosk, A.P. Focusing coherent light through opaque strongly scattering media. Opt. Lett. 2007, 32, 2309. [Google Scholar] [CrossRef] [PubMed]
- Wu, H.; Ryczkowski, P.; Friberg, A.; Dudley, J.M.; Genty, G. Temporal ghost imaging with wavelength conversion. In Proceedings of the 2019 Conference on Lasers and Electro-Optics Europe & European Quantum Electronics Conference (CLEO/Europe-EQEC), Munich, Germany, 23–27 June 2019; pp. 1–5. [Google Scholar]
- Popoff, S.M.; Lerosey, G.; Carminati, R.; Fink, M.; Boccara, A.C.; Gigan, S. Measuring the transmission matrix in optics: An approach to the study and control of light propagation in disordered media. Phys. Rev. Lett. 2010, 104, 100601. [Google Scholar] [CrossRef] [PubMed]
- Huyan, D.; Lagrosas, N.; Shiina, T. Target imaging in scattering media using ghost imaging optical coherence tomography. APL Photonics 2022, 7, 086104. [Google Scholar] [CrossRef]
- Dudley, D.; Duncan, W.M.; Slaughter, J. Emerging digital micromirror device (DMD) applications. MOEMS Disp. Imaging Syst. 2003, 4985, 14. [Google Scholar] [CrossRef]
- Hellman, B.; Takashima, Y. Angular and spatial light modulation by single digital micromirror device for multi-image output and nearly-doubled étendue. Opt. Express 2019, 27, 21477. [Google Scholar] [CrossRef]
- Zhang, Y.; Situ, G.; Pedrini, G.; Wang, D.; Javidi, B.; Osten, W. Application of short-coherence lensless Fourier-transform digital holography in imaging through diffusive medium. Opt. Commun. 2013, 286, 56–59. [Google Scholar] [CrossRef]
- Stsepuro, N.; Kovalev, M.; Krasin, G.; Podlesnykh, I.; Gulina, Y.; Kudryashov, S. Surface Depth-Mapping of Material via the Transport-of-Intensity Equation. Photonics 2022, 9, 815. [Google Scholar] [CrossRef]
- Li, J.; Zhou, N.; Sun, J.; Zhou, S.; Bai, Z.; Lu, L.; Chen, Q.; Zuo, C. Transport of intensity diffraction tomography with non-interferometric synthetic aperture for three-dimensional label-free microscopy. Light. Sci. Appl. 2022, 11, 154. [Google Scholar] [CrossRef]
- Chernomyrdin, N.V.; Skorobogatiy, M.; Gavdush, A.A.; Musina, G.R.; Katyba, G.M.; Komandin, G.A.; Khorokhorov, A.M.; Spektor, I.E.; Tuchin, V.V.; Zaytsev, K.I. Quantitative super-resolution solid immersion microscopy via refractive index profile reconstruction. Optica 2021, 8, 1471. [Google Scholar] [CrossRef]
- Wang, Z.; Bovik, A.C.; Sheikh, H.R.; Simoncelli, E.P. Image quality assessment: From error visibility to structural similarity. IEEE Trans. Image Process. 2004, 13, 600–612. [Google Scholar] [CrossRef] [PubMed]
- Shiina, T.; Moritani, Y.; Ito, M.; Okamura, Y. Long-optical-path scanning mechanism for optical coherence tomography. Appl. Opt. 2003, 42, 3795. [Google Scholar] [CrossRef] [PubMed]
- Don, M. An Introduction to Computational Ghost Imaging with Example Code; CCDC Army Research Laboratory: Aberdeen Proving Ground, MD, USA, 2019. [Google Scholar]
- Ferri, F.; Magatti, D.; Lugiato, L.A.; Gatti, A. Differential ghost imaging. Phys. Rev. Lett. 2010, 104, 253603. [Google Scholar] [CrossRef]
- Zhang, C.; Guo, S.; Cao, J.; Guan, J.; Gao, F. Object reconstitution using pseudo-inverse for ghost imaging. Opt. Express 2014, 22, 30063. [Google Scholar] [CrossRef]
- Agrawal, P.; Sanikop, S.; Patil, S. New developments in tools for periodontal diagnosis. Int. Dent. J. 2012, 62, 57–64. [Google Scholar] [CrossRef]
- Tanaka, M.; Hirano, M.; Murashima, K.; Obi, H.; Yamaguchi, R.; Hasegawa, T. 17-mm Spectroscopic Spectral-Domain Optical Coherence Tomography for Imaging Lipid Distribution Within Blood Vessel. Opt. Express 2015, 23, 6645. [Google Scholar] [CrossRef]
- Hsieh, Y.S.; Ho, Y.C.; Lee, S.Y.; Chuang, C.C.; che Tsai, J.; Lin, K.F.; Sun, C.W. Dental optical coherence tomography. Sensors 2013, 13, 8928–8949. [Google Scholar] [CrossRef]





| Manufacturer | THORLABS |
|---|---|
| Model number | SLD850S-A20W |
| Center wavelength | 860 nm |
| Spectral band width | 28 nm |
| Coherence length | 11.6 μm |
| Optical power | 50 mW |
| Manufacturer | TI |
|---|---|
| Model number | DLP2010LC |
| Illumination wavelength | 420∼700 nm (100%) 860 nm (90%) |
| Array diagonal | 5.29 mm |
| Output frame rate | 240 Hz |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Huyan, D.; Lagrosas, N.; Shiina, T. Optical Properties Analysis of Scattering Media Based on GI-OCT Imaging. Photonics 2023, 10, 146. https://doi.org/10.3390/photonics10020146
Huyan D, Lagrosas N, Shiina T. Optical Properties Analysis of Scattering Media Based on GI-OCT Imaging. Photonics. 2023; 10(2):146. https://doi.org/10.3390/photonics10020146
Chicago/Turabian StyleHuyan, Decai, Nofel Lagrosas, and Tatsuo Shiina. 2023. "Optical Properties Analysis of Scattering Media Based on GI-OCT Imaging" Photonics 10, no. 2: 146. https://doi.org/10.3390/photonics10020146
APA StyleHuyan, D., Lagrosas, N., & Shiina, T. (2023). Optical Properties Analysis of Scattering Media Based on GI-OCT Imaging. Photonics, 10(2), 146. https://doi.org/10.3390/photonics10020146






