4.1. Model Development
Up to this point, the analysis of the aberrations was conducted without considering the propagation of the wavefront. Further, the impact of the external and internal factors influencing the autocollimator measurements on the shaping of the aggregate wavefront will be considered.
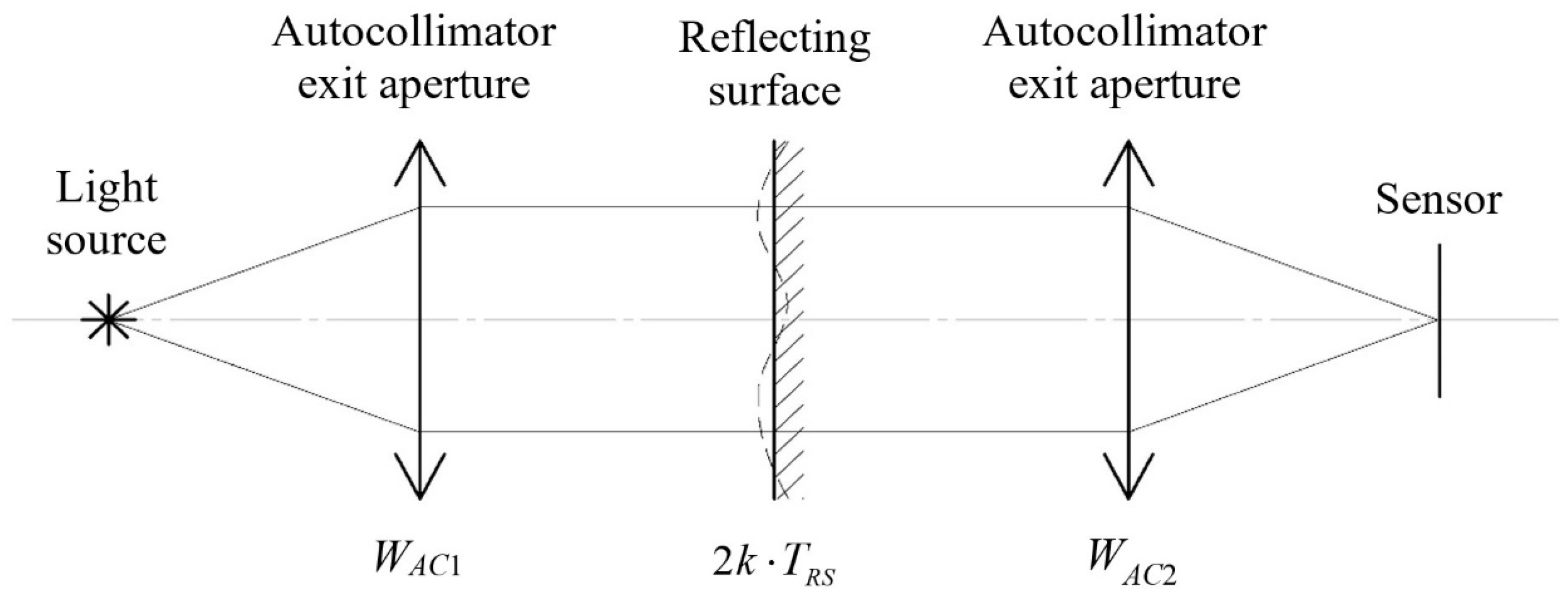
Figure 5 represents the unfolded optical scheme for the basic case where the reflecting surface is orthogonal to the autocollimator axis, and both the autocollimator exit aperture and the reflective surface are circular and of the same diameter.
TRS is the topography of the reflecting surface which is given in terms of height deviation relatively to the least squares approximating plane. The surface’s contribution to the wavefront aberration caused by the reflection will be defined as
2k·TRS, where k is the wave number of the propagating light. The influence of the autocollimator’s internal factors is presented by two wavefront aberration distributions
WAC1 and
WAC2 given in terms of wavefront phase deviation. The ideal wavefront is transformed as
WAC1 distribution while travelling from the light source to the autocollimator exit aperture, with
WAC2 aberrations added to the wavefront on the light’s way backwards from the exit aperture to the sensor. The common factor for these two distributions is the aberration brought by the autocollimator objective lens. Besides this,
WAC1 accounts for the illumination-related factors, while
WAC2 does the same for the influence of the sensing element’s parameters. The contribution of the beam-splitting element toward wavefront shaping also has to be accounted for separately, as it transmits the light in a forward direction and reflects it in a reverse direction. At this point, the upcoming logic demands an additional assumption. This assumption is that the plain or nearly plain wavefront stays invariant while travelling through free space. Research [
24] has shown that, in fact, the free space transformation of such a wavefront is very small, so it will be deemed negligible at this moment. Therefore, having incorporated the above assumption, the final wavefront
WΣ may be found as follows:
The basic case and formula (3) are presented in order to demonstrate how the aggregate wavefront builds up, regardless of the external measurement factors. The common measurement case including the reflecting surface inclination and the aperture stop is presented further in
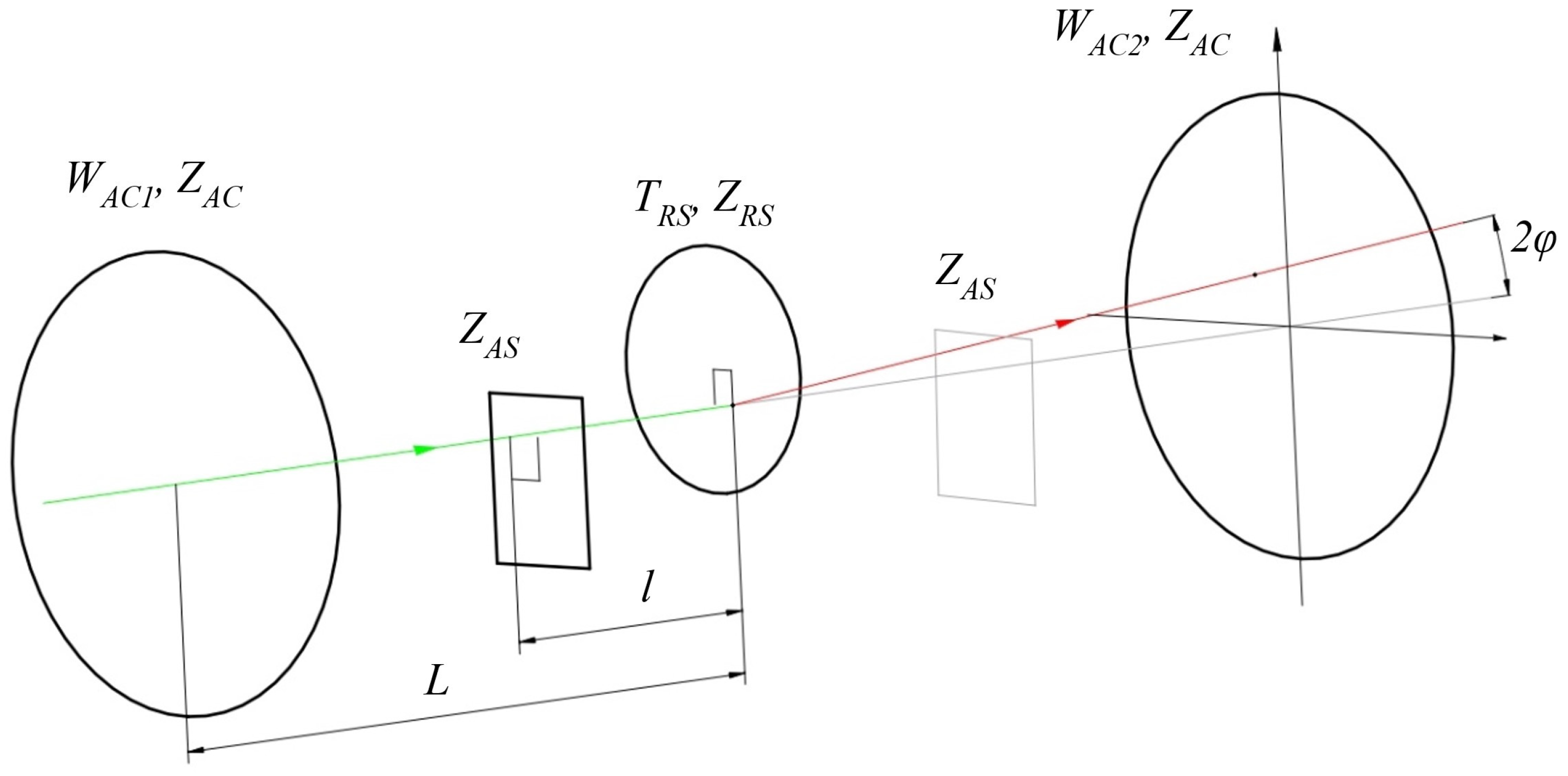
Figure 6.
Here, the colored lines with arrows indicate the propagating light beam. The green line is for the beam before reflection, and the red is for the beam after reflection. The autocollimator is the reference unit. The 0xy coordinate system is bound to the center of the autocollimator exit aperture, at the point where the wavefront returns to it. The system axes are parallel to the autocollimator axes, along which the image displacement is measured. As the overall inclination φ of the reflecting surface is small, it may be considered as a combination of two inclinations φx and φy under the condition that , where φx is the inclination around 0y, and vice versa. L is the distance from the autocollimator exit aperture to the reflecting surface, and l is the distance from the reflecting surface to the aperture stop. WAC1, WAC2 and TRS are defined relatively to the centers of the corresponding elements. The dimensions and shapes of the autocollimator exit aperture, reflecting surface and aperture stop are introduced through the special functions ZAC, ZRS and ZAS, which are also defined relatively to their element’s centers. The Z function is equal to 1 for all the points that are inside the bounds of the element and equal to 0 for the rest of the points. While the autocollimator exit aperture is circular, the shape of the reflecting surface and aperture stop may be random (it is also usually circular or rectangular). The lateral displacement of the reflecting surface and the aperture stop related to the autocollimator’s optical axis is set in terms of their center coordinates (xRS; yRS) and (xAS; yAS).
The inclination of the reflecting surface φ will also cause the general inclination of the propagating wavefront which has to bring the additional member to Equation (3). Inclination φ is, in fact, the quantity which is to be measured by the autocollimator and thus the additional member would represent the ideal wavefront. As the measurement model was developed to assess the errors that cause certain factors, this member will be omitted. The aggregate wavefront
WΣ will be substituted by the wavefront that contains only aberrations that cause measurement errors
WΣer. As a result, the wavefront
WΣer will be determined by:
The interpretation of this expression may be as follows. Three different distributions of the wavefront aberration are summed up depending on the overlap caused by their alignment properties, on the distance to the surface and on the actual angle that is being measured. Afterwards, the sum is cropped (multiplied by the
Z functions) depending on the same factors. It depends also on the shapes and dimensions of the considered elements, and the axial and lateral positions of the aperture stop. Ultimately, the resultant wavefront
WΣer has to be analyzed in order to determine the shift in the projected image centroid and the measurement error, respectively. It may be distributed in terms of Zernike aberrations to find the values of inclination and coma coefficients. Alternatively, the angular measurement error may be evaluated numerically as the general inclination in the wavefront border. Although the Zernike polynomials are quite common in aberration processing, it may be unfavorable to apply them to the problem at hand. This is mainly due to the area of the processed wavefront not being constant, which is caused by the cropping defined by the variable parameters of measurement. Supposing that there is an aberration distribution of a certain area with known Zernike expansion that is limited by some arbitrary aperture, the Zernike expansion for the derivative aberration distribution can never be obtained directly from the initial set of coefficients. Therefore, to obtain the Zernike expansion for the derivative distribution, the primary distribution would have to be reconstructed from the known expansion, cropped and expanded afterwards once again. This implies making a large number of calculations. The other problem is that the actual wavefront border may not be circular. In fact, taking into account all the overlapping and cropping from expression (4), the final wavefront outline will most definitely not be circular. There is a method for non-circular aberration distribution in terms of orthonormal polynomials [
25], but it also requires massive calculations.
The suggested model (4) provides an opportunity to evaluate the angular deviation of the real reflecting surface relatively to its least squares approximation plane measured by the autocollimator. This possibility is conditional upon the fact that the topography of the surface and the inner autocollimator aberrations are known, and the measurement setup parameters are defined and well controlled. Conventionally, the technical specifications for the autocollimators, provided by the manufacturers, determine both the angle measurement accuracy level with measurement range and the maximum distance to the reflecting surface. Some of these also specify the required minimum dimensions of the reflecting surface and the reflectivity. Manufacturers rarely specify the required surface flatness as the root mean square of the surface profile height. Usually, this is sufficient for common angle measurement applications that do not demand extreme accuracies. Yet, there are specific tasks such as the comparison measurements considered in the overview. Such measurements have to make use of the suggested model with all the listed parameters that influence the autocollimator operation. It is clear that these have to be the tasks that imply the major part of the influencing parameters to be constant in order to reduce the amount of numerical calculations.
4.2. Numerical Simulation of Angle Measurements
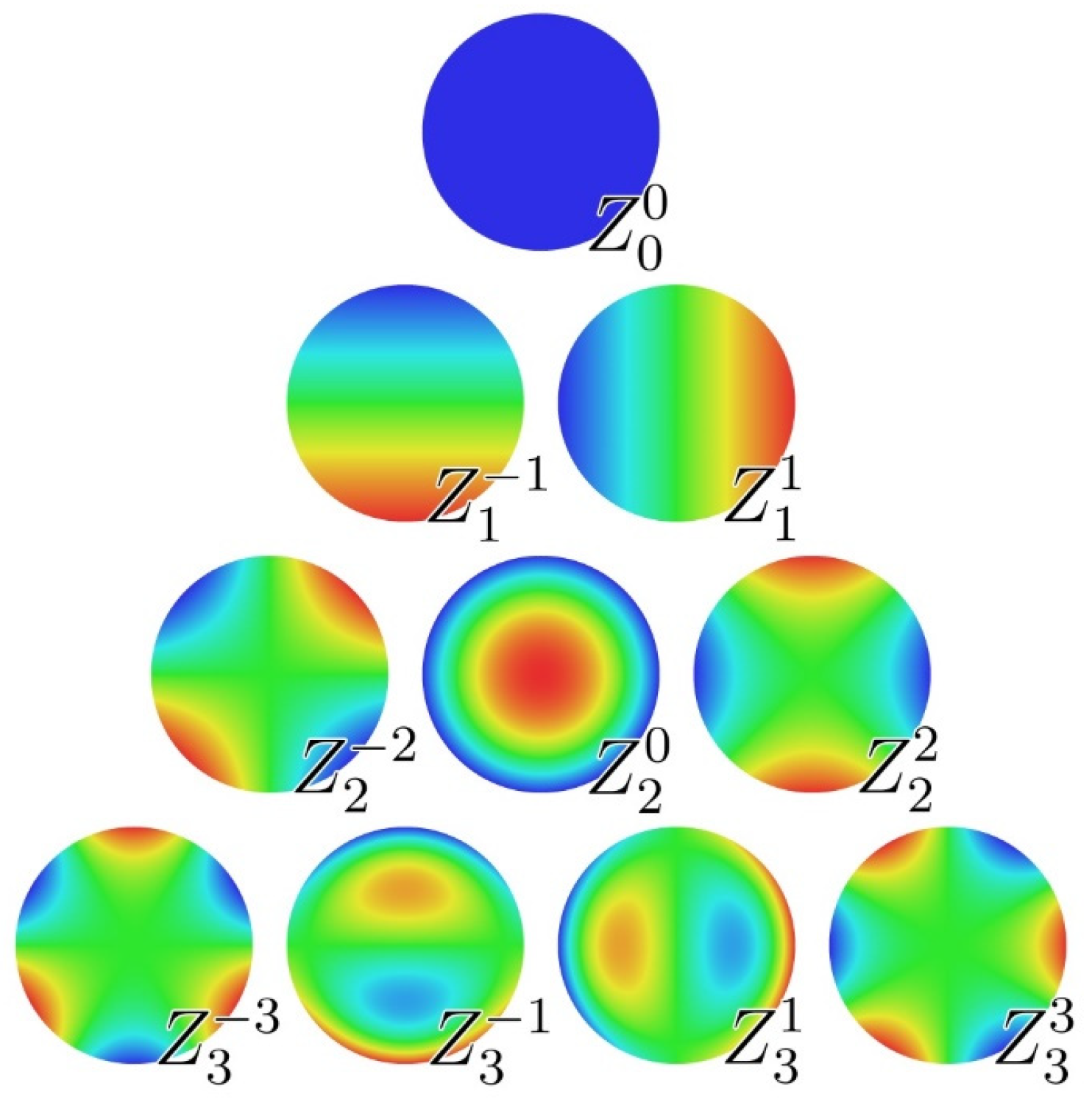
In order to estimate the accuracy level, at which the model application is essential, the following simplified simulation problem was considered. In principle, the deviation in autocollimator angle measurement arises due to various cropping of the cumulative wavefront. Thus, the point of the simulation was to generate a set of test wavefronts that would be close to what is found in practice. Then, the autocollimator’s angle deviations should be calculated when these wavefronts were cropped. As it was mentioned earlier, the impacts of the aberrations represented by different Zernike polynomials can be investigated separately. Therefore, the generated set consisted of wavefronts represented by the first-order Zernike aberrations of defocus, astigmatism, coma and spherical aberration. Commonly, the aberrations of these types bring the major contribution to overall wavefront topography. All generated wavefronts were defined inside the circular area of 20 mm diameter. To bring the values of the Zernike coefficients into compliance with the physical dimension of the wavefronts, they were selected as C3 = 10 nm for defocus, C4 = 10 nm for astigmatism, C6 = 10 nm for coma and C8 = 5 nm for spherical aberration. The choice of these values was based on face topographies measured for the 24-sided optical polygon which is regularly used in metrological routines. It was assumed that the autocollimator’s internal aberrations do not exceed those that are caused by the non-flatness of the reflecting surface.
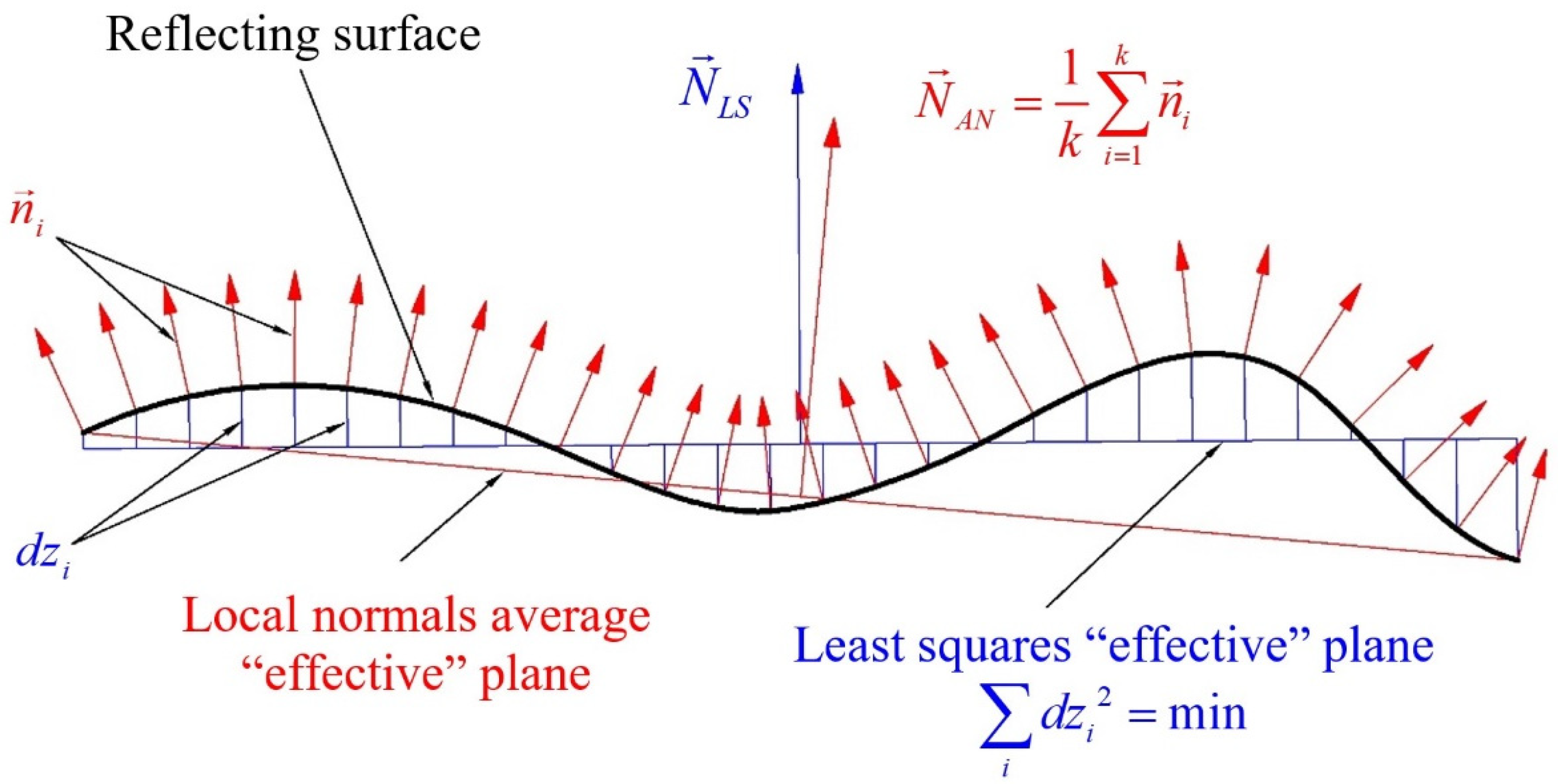
In order to estimate the angular deviations, each generated wavefront was masked with the circular aperture of 15 mm diameter. All deviations were calculated, applying the average normal approximation method for the cropped wavefront area to bring the result in accordance with what the autocollimator would register. The variation of the cropping area was realized by shifting the aperture relatively to the initial wavefront center along the axis of conjectural measurements (see
Figure 7). The maximum aperture shift considered was ± 2.5 mm.
All angle deviation values were calculated relatively to the initial wavefront of the least squares approximating plane.
Figure 8 represents the results combined in a single chart.
Naturally, any real wavefront is a combination of aberrations considered in the simulation case and much more. As the aberrations are summed up, they may build up the cumulative angle deviation or, alternatively, they may compensate each other, depending on the sign of the corresponding Zernike coefficients. The values of each Zernike coefficient applied in the simulation problem were selected at the upper margin of used measurement results. This means that summing up the maximum values of calculated angle deviations would correspond to some extreme case, which could hardly be found in practice. Having summarized all of the above and relying on the simulation results and common sense, the authors suggest to set the sought-for accuracy level at 0.2 arc seconds.
4.3. Experimental Verification of the Model Consistency
A large amount of variable parameters and unknown internal aberration distributions of the autocollimator
WAC1 and
WAC2 in model (4) significantly complicate the experimental research of this model. The only method that could resolve this issue is to eliminate the unknown distributions and to make the majority of the model variables constant. In practice, both these goals are attainable with a goniometric setup for optical polygon calibration which was generally utilized during the comparison measurements. An optical polygon is a regular prism with all the reflecting faces normal to its base. Polygon calibration consists in determining the angle deviation of each face normal relatively to its position, as defined by the corresponding ideal geometrical figure. The polygon angles themselves may be expressed with reference to the first face, so-called cumulative angles, or as the pitch angles that are measured sequentially between adjacent faces. The evaluation of the corresponding deviations for these polygon angles then continues as follows:
where β
i and α
i are cumulative and pitch polygon angles, Δβ
i and Δα
i are their deviations,
N is the number of polygon faces and γ
i is the angle position of each polygon face measured directly by the goniometric system relatively to the coordinate system set by the angle encoder of the index table. As it can be observed from expressions (5), each angle deviation depends on the measured angle position of two faces: the current and the first ones for the cumulative angle, or the current and the previous ones for the pitch angle. In the report to one of the first international comparison measurements (the Probst comparison report), it was proposed to apply reduced angle deviations to be able to investigate the deviation referring to a single face. These deviations
are derived from the cumulative ones:
The reduced deviation does not depend on any specific face, besides a current one to which it refers. In order to clarify the connection between the goniometric measurements and the model verification, the angle position of each polygon face has to be taken apart by its components. The goniometric system basically consists of the index table and the autocollimator mounted on the common base. The autocollimator implements an optical connection to the polygon reflecting faces so that their angular position could be measured relatively to the angular scale of the index table. During the measurement, each face is sequentially positioned against the autocollimator, so that the angle between the face normal and the autocollimator optical axis is within the autocollimator measurement range. After the index table stabilizes while positioning one of the faces, the readings from the autocollimator and the angular scale of the index table are taken, and the angle position of the reflecting face is evaluated as their difference. Following this, the index table rotates to position the next face. Taking into consideration the systematic errors of the autocollimator and the angular scale, the angle position of the
i-th face may be defined as:
where
ei is the current angle position of the index table presuming that its angular scale is ideally regular, Δ
ei is the systematic error of the scale for this position,
is the angle between the autocollimator optical axis and the normal to the least squares approximating plane of the current reflecting face,
is the angle deviation of the face caused by the internal aberrations of the autocollimator and
is the angle deviation of the face caused by its non-flatness. As a rule, in order to reduce the autocollimator measurement error, during each positioning, the polygon face is set as close to the normal position against the autocollimator as possible. Assuming that it is a sharp normal, the member
in expression (7) may be set to zero. Moreover, returning to expression (4), φ
x and φ
y may also be set to zero. For the former one, this is due to the same reason as for
, i.e., the angle that lies in the goniometric measurement plane. For the latter one, this is due to the polygon adjustment relatively to the rotation axis and the assumption that the polygon pyramidal error may be deemed as negligible. As for the shaping of the aggregate autocollimator wavefront, the pyramidal error gives rise to a variable shift of wavefront cropping defined by the parameter
. However, taking into account that for high-precision goniometer measurements, the distance between the reflecting face and the autocollimator exit aperture is typically below 20–30 cm and the polygon pyramidal error itself does not exceed a few arc seconds, this shift stays below 0.02 mm. Such values may indeed be considered as negligible.
The elimination of angle variables from expression (4) leads to the autocollimator’s internal aberrations (
WAC1 and
WAC2). In this situation the impact of these aberrations on the measured face angle position
is identical for all polygon faces, except for a certain case when disposing of angle variables is insufficient. This case occurs under the following conditions: the polygon is mounted eccentrically relatively to the index table rotation axis, the dimensions of the reflecting faces are smaller than the autocollimator exit aperture, and the aperture is absent. The above conditions make the overall wavefront cropping from expression (4) totally defined by the factor
. The eccentricity error makes this factor variable, depending on the magnitude of the eccentricity and reflecting face sequence number via the variance of the x
RS parameter. In practice, the eccentricity error can be reduced sufficiently by using special polygon housings and centering appliances. Proceeding to the reduced angle deviations of the polygon faces, they become independent of the autocollimator internal aberrations. Taking in account expressions (5) and (7) and all the considerations presented above, the reduced deviations may be expressed as follows:
Here, the first two brackets relate to the angular scale readings of the index table. The third one is defined by the number of the currently measured face and the total number of polygon faces, and the last one represents the influence of the polygon faces’ non-flatness on the autocollimator measurements. In order to use the measurement results for model verification, the autocollimator-related components have to be isolated. This can be achieved by running two similar calibrations of the same polygon with different apertures placed between the autocollimator and the polygon in the process. The fact that the calibrations are similar implies ruling out polygon reinstallation or changing any other measurement setup parameters, except inserting the apertures. Therefore, if the experiment is carried out correctly, the reduced angle deviations corresponding to different calibrations will have equal components represented by the first three brackets in expression (8). The difference can only be due to the way the reflecting faces are registered by the autocollimator. Therefore, the difference between the reduced deviations will result in the following:
where
and
are the angle deviations of the same face defined by its non-flatness and the apertures that were applied. It is important to note that the resulting difference depends not only on the current face deviation, but also on the change in the averaged deviations of the rest of the faces. This factor has to be accounted for while processing the measurement results.
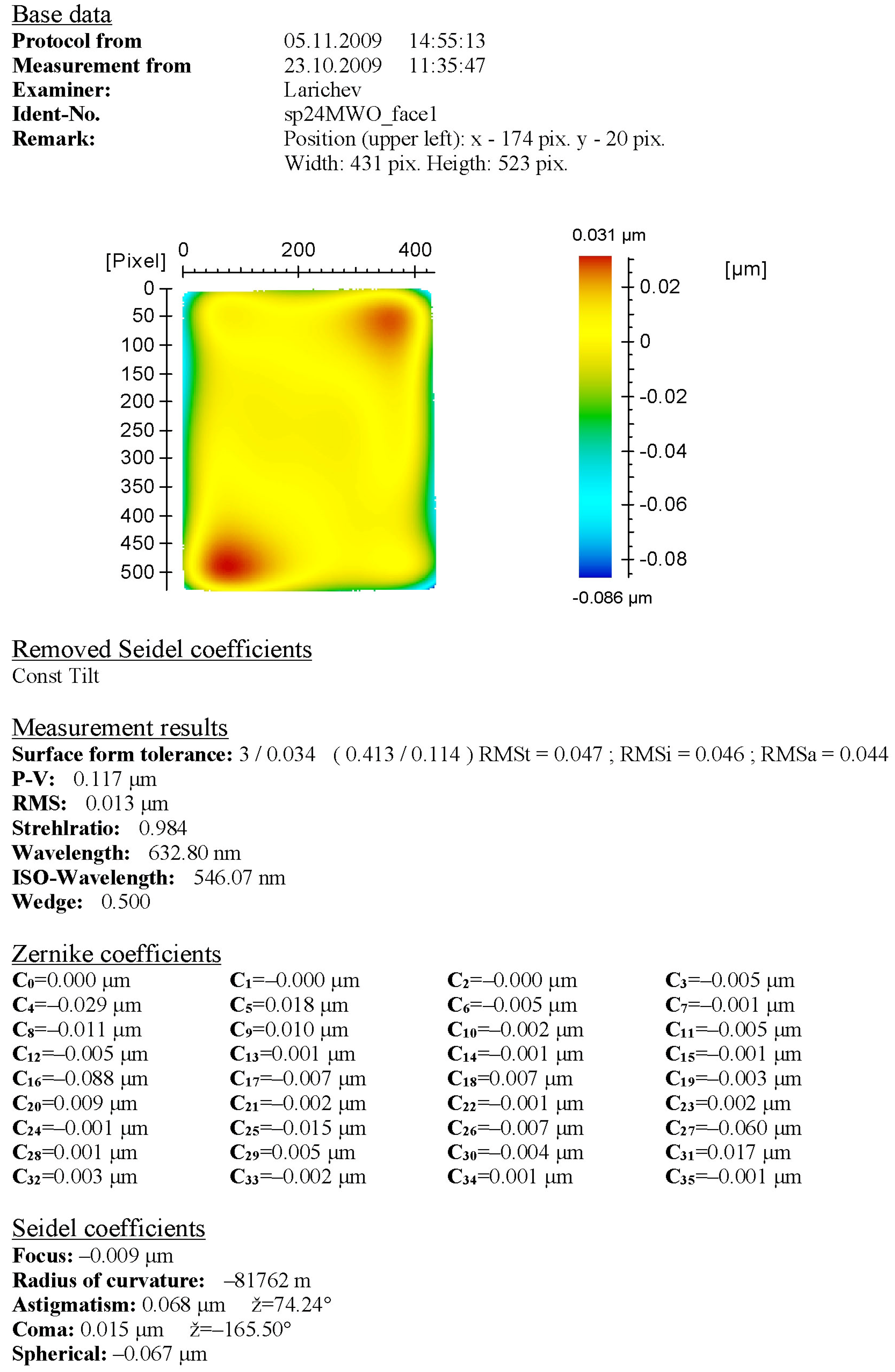
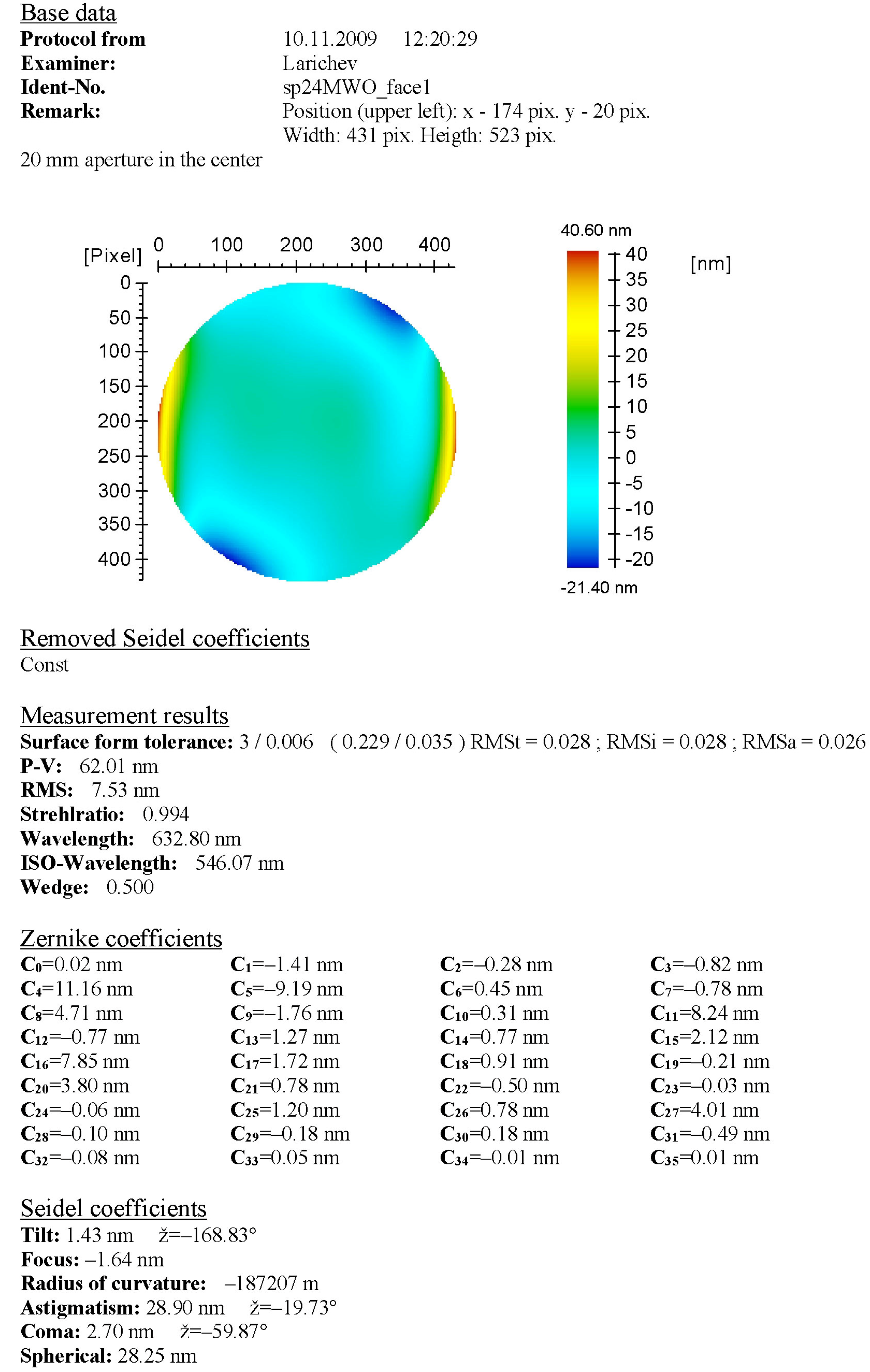
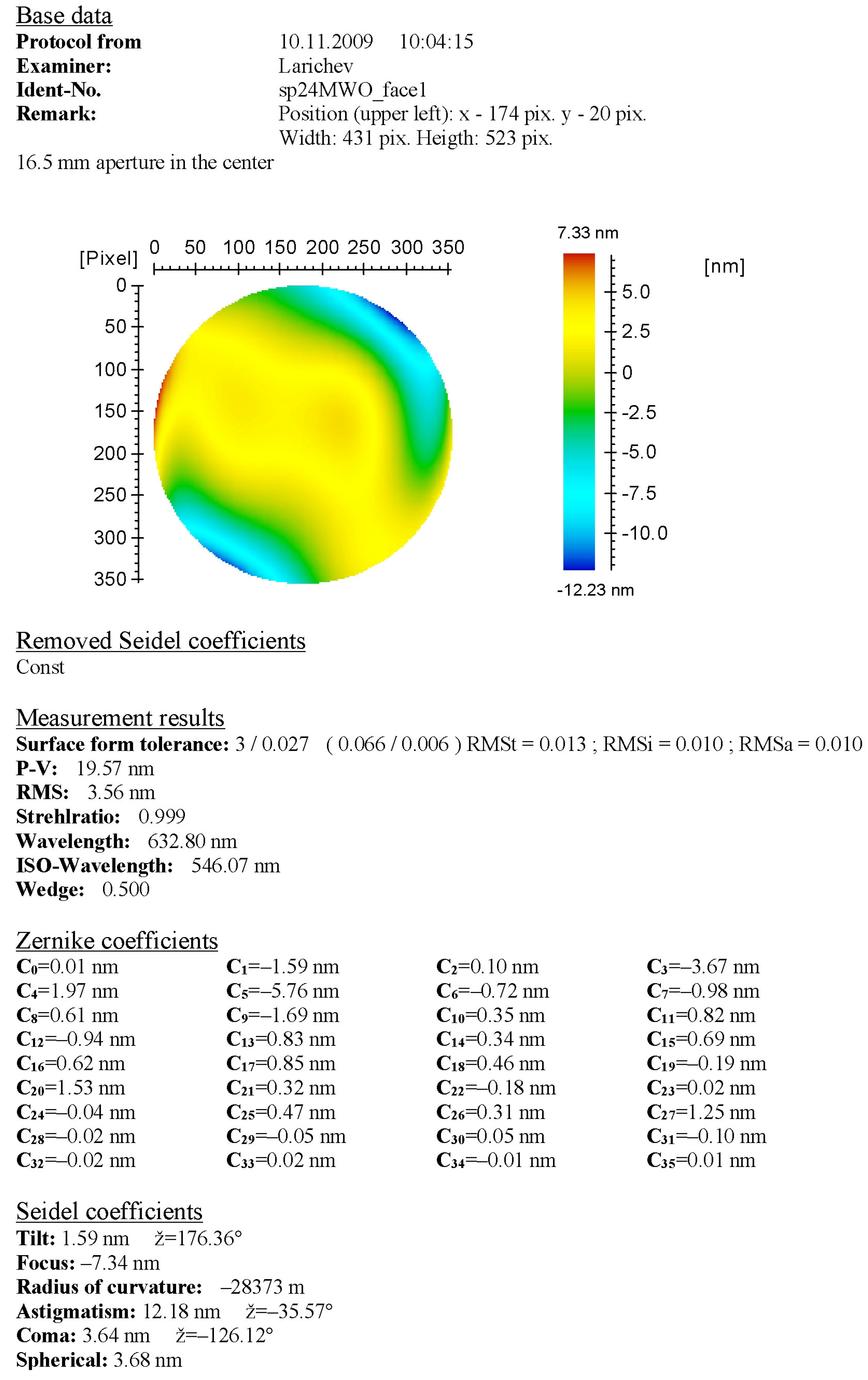
Ultimately, expression (9) demonstrates that the proposed experiment is in agreement with the goals set in the beginning of the section. After eliminating the unknown internal autocollimator aberrations, the final angular values consist only of members that are defined by the way the non-flat reflecting surfaces are limited by certain apertures. Given that the topography of the polygon faces and the aperture parameters are known, these members may be evaluated by using the suggested model, which gives an opportunity to verify it. In 2009, an experiment that met the presented description was carried out jointly with our colleagues from the “Length and angle graduations” working group (PTB, Germany) at their laboratory. A 24-sided polygon which was previously chosen for international comparison measurements, was selected as a subject of calibration. The angle comparator WMT 905 with an angle resolution of 0.035 arc seconds and a positioning accuracy of 0.03 arc seconds was used as the index table. The optical connection to the polygon faces was provided by the Moeller Wedel Elcomat HR autocollimator. The reflecting face topography was measured using the Moeller Wedel Interferometer V-100 phase-shifting interferometer.
After installing and adjusting the polygon on top of the angle comparator, the calibration was performed on the polygon with fully exposed reflecting faces and with several apertures inserted. The polygon faces were rectangular with dimensions of 20 × 25 mm, and the apertures that were applied were circular with diameters of 20, 18 and 16.5 mm. For each instance, angle measurements were conducted repeatedly during multiple revolutions of the polygon in order to reduce random error impact by averaging. The number of revolutions varied slightly around 40. Direct topography measurement involved saving raw measurement data (point-by-point height profile of the reflecting surface) and was conducted only for fully exposed polygon faces. Further, the statistical parameters (RMS, peak-to-valley, etc.) and Zernike expansions of each topography were evaluated via software processing. The same measurement data for the faces with reduced reflecting area were obtained not through direct measurement, but by the processing of the initially measured full topographies that were algorithmically trimmed by the corresponding virtual masks. The processing of the open reflecting faces was conducted by subtracting the general inclination (the 2nd and 3rd Zernike polynomials). Yet, this parameter was involved in the processing of the masked topographies in order to observe how the least squares approximating plane inclines due to the reflecting face trimming. The measurement protocols of the interferometer for the first polygon face are presented in
Appendix A as an example (
Figure A1,
Figure A2,
Figure A3 and
Figure A4).
The results of this experiment have already been reported previously [
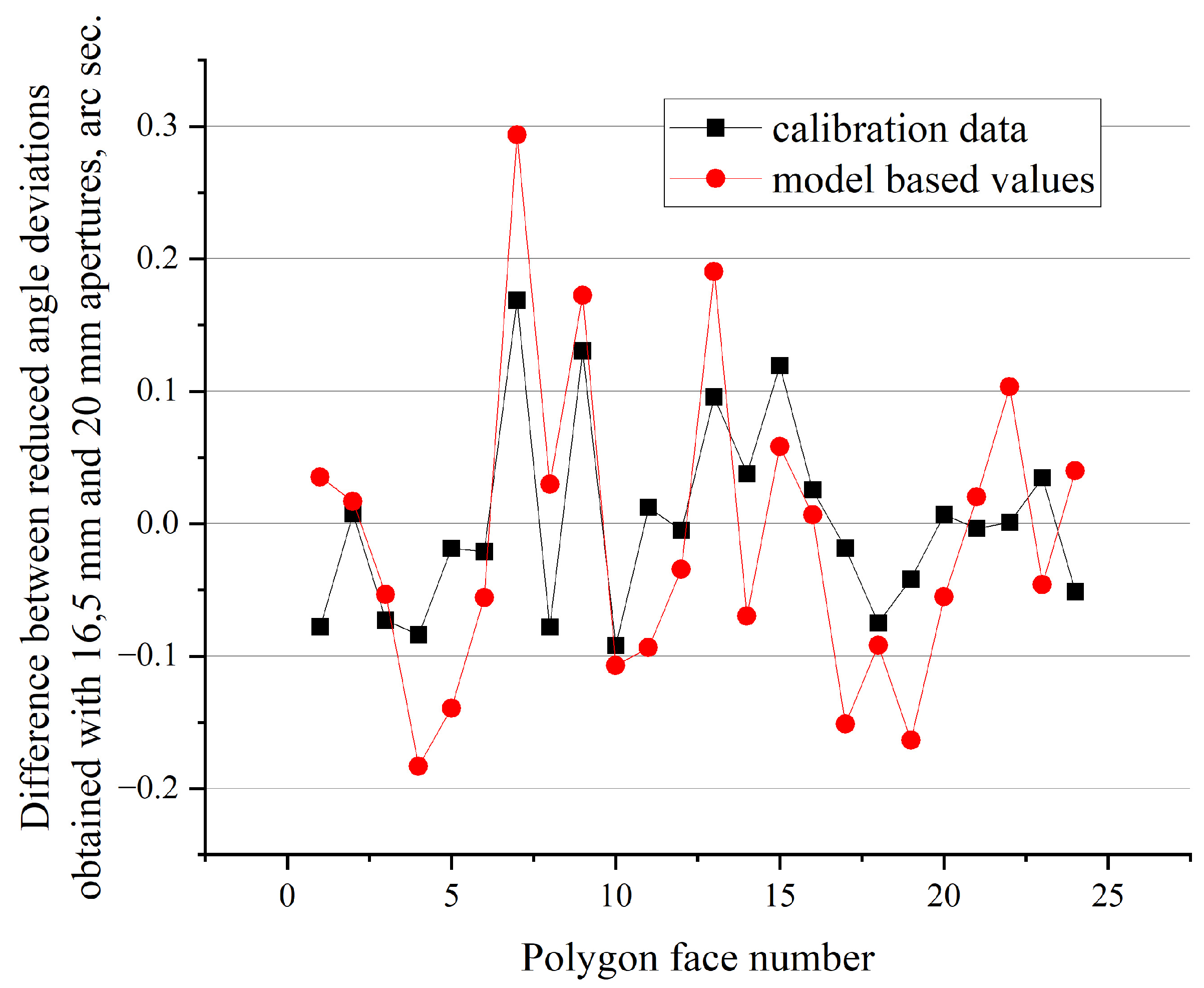
26]. However, these were analyzed based on the old assumption that the influence of non-flatness may be accounted for by its statistical parameters. As a part of the current research, in order to verify the proposed model, the old data were analyzed once again. Based on the raw topography data on the faces and the local normals’ approximation method, the difference between the reduced angular deviations (9) was calculated for each face of the 24-sided polygon for the cases of the 20 mm and 16.5 mm apertures. In addition, this difference was calculated based on the polygon calibration data obtained using the autocollimator with the corresponding apertures. Fully exposed faces were not considered in this comparison in order to avoid the influence of possible edge defects of the reflecting faces. The maximum and minimum were selected from the three apertures that were used, since the transition from one to the other provides the most noticeable change in the topography of the faces. The differences obtained are shown in
Figure 9.
To estimate the correlation between these two value sets, the Pearson correlation coefficient was calculated and found as 0.7 with a p-value below 0.0002. Although these numbers indicate good correlation, they are still not as good as the authors anticipated them to be. The probable reasons for this are discussed in the next section.