An Integrated Photoelasticity-Based Approach for the Reconstruction of Stress Profiles and Optical Anisotropy of GRIN Lenses

Abstract

The paper shows that it is possible to obtain reliable information on the dependence of the radial distribution of longitudinal birefringence in glass cylindrical elements with a radial distribution of refractive index (GRIN lenses) on the basis of transverse polarization tomography data on residual stresses. This does not require complicated procedures of sample preparation, as is necessary in the case of longitudinal translucency measurements. The approach developed was verified in the experiments with a set of different GRIN lenses formed with the ion exchange technique, and the closeness of the data obtained from transversal and conventional longitudinal transmission measurements was demonstrated.

1. Introduction

Gradient-index rods with a radial distribution of refractive index n(r) (GRIN lenses) are widely used in image translators in copiers and medical devices, as well as collimating and focusing lenses in fiber-optic passive and active connectors. In addition, GRIN lenses in combination with, for example, diffraction gratings are used in light flow splitters, optical switches, multiplexers, demultiplexers, etc. [1,2,3]. The most common technology for obtaining a given radial refractive index (RI) distribution in GRIN lenses is high-temperature ion exchange (IE) of alkaline cations in the glass–salt melt system. The IE synthesis of such GRINs is carried out at glass viscosities of 10⁸–10¹⁰ Pa·s, with the possible use of isothermal annealing outside the melt at various temperatures [4,5,6]. Due to diffusion, a gradient of the refractive index is formed across the cross section of the GRIN lens, corresponding to the substitution of alkali ions (e.g., Li (glass) ⇔ Na (salt melt)). When cooling GRIN lenses from the ion exchange temperature corresponding to the viscoelastic state of the material, residual stresses (RS) σ_ij(r) arise due to radial gradients of the temperature coefficient of linear expansion and glass transition temperature T_g. Because of the kinetic nature of the glass transition, the distribution of RS significantly depends on the temperature and duration of the sample cooling. RS hinder the creation of a given RI distribution in gradient rods and can lead to their bending due to the loss of stability and, ultimately, to destruction.

One of the most important manifestations of the RS in the GRIN lenses is radial birefringence, a consequence of elastic-optical (or photoelastic) effects. Birefringence in initially isotropic optical objects (in particular, those made of glass) is an inevitable consequence of RS. Accordingly, the birefringence distribution of such an object, in general, contains all the information about the RS distribution that caused it. That is, these two phenomena are inseparably interrelated. Radial birefringence causes polarization aberrations that, for example, degrade image quality in endoscopes. In fiber-optic sensors of stress, strain, or pressure, which use the modulation of optical signals directly using GRIN lenses, RS change the polarization of light, thereby limiting the sensitivity of the sensor to deformation [7,8,9]. The negative influence of RS can also be noted in GRIN lenses of large diameter used in macro-optics and in axisymmetric acoustic lenses with a gradient radial distribution of elastic properties [10,11,12]. It has recently been shown [13,14,15] that the combination of polarization characteristics of GRIN lenses (i.e., radial birefringence) and their focusing properties turned out to be very effective for the generation of optical vortex beams [16,17,18]. In this respect, it is noted [13,14,15] that it is relevant to increase the low level of optical anisotropy of GRIN lenses, that is, their birefringence. Additionally, the use of GRIN lenses with high birefringence has also enabled significant progress in polarization imaging of biological tissue structure, which is potentially a powerful tool for clinical diagnostics [3,19,20].

Thus, the development of gradient optical elements with both minimal and significant birefringence is of essential interest. In this regard, the issues of characterization of RS in GRIN lenses, determining the optimal modes of ion exchange, and heat treatment of the glass rods to obtain the required characteristics are relevant.

The method of integrated photoelasticity [21,22] is widely used to study the RS distribution in transparent gradient-free objects. Inverse (A) and direct (B) problems can be considered: A—reconstruction of RS distributions on the basis of measurements of the parameters of polarized light that passed through the object under study; B—determination of the birefringence distribution from known RS (e.g., obtained from the results of A or via modeling).

The analysis based on the integrated photoelasticity method proposed in this paper is based on the geometrical optics approximation, which is a special case of Maxwell’s equations. This approximation describes optical phenomena quite well if the light wavelength is negligibly small compared to the size of the optical devices used, in our case, GRIN lenses. These objects use radiation in the visible and near infrared range (wavelength 0.5–1.5 µm). This is due to the light sources used and the peculiarities of the absorption of radiation with glass and optical polymers. Typical sizes of gradient lenses are 1–10 mm, i.e., their sizes significantly exceed the specified wavelengths, which makes it possible to use special cases of Maxwell’s equations, in particular Fermat’s principle, in calculations [23].

The mathematical apparatus of the integrated photoelasticity method is well developed only for the rectilinear propagation of probing beams. However, in GRIN lenses, rays deviate significantly from straight-line propagation due to the gradient of refractive index (in high-aperture GRIN lenses, refractive index change can reach up to Δn ~ 0.14).

This raises the problem of generalizing the method of integrated photoelasticity to the case of cylindrical rods with radial RI distribution, which should allow for the adjustment of the parameters of technological processes to ensure the given distributions of RS and optical anisotropy.

Such a generalization of the relations in the inverse and direct problems of integral photoelasticity for the cases of transverse, longitudinal, and sagittal translucency GRIN lenses, taking into account the curvature of the trajectories of the rays propagating in them, was made in [24,25]. The analysis for the first two cases was also published in [26].

Both in these works and below, it is also assumed that in the considered weakly anisotropic gradient objects, the waves retain the transverse structure (quasi-isotropic approximation of geometrical optics for inhomogeneous media [27]), and the ray trajectories with mutually perpendicular polarization can be considered geometrically identical and corresponding to the initial axisymmetric RI distribution.

In this paper, new relations are obtained for the following:

(1)

Reconstruction of the radial rather than the axial component of the RS from the data of transverse translucence of GRIN lenses (inverse problem), which exclude the differentiation of experimental dependencies under the sign of the integral increasing the errors (as it takes place at reconstruction of the axial component of the RS);

(2)

Longitudinal birefringence (integral phase difference) calculations—direct problem from RS reconstruction data, based on the results of (1) for GRIN lens segments.

Using computational algorithms developed on the basis of relations (1) and (2), we obtained RS distributions for a number of GRIN lenses (the inverse problem of transversal translucency). Using these data as inputs for calculations (the direct problem in longitudinal incidence), the radial distributions of integral phase difference (birefringence) are determined for the case of meridional ray propagation in these GRIN lenses.

Examples of reconstruction of radial RS distributions and optical anisotropy profiles for glass GRIN lenses produced via high-temperature ion exchange are presented.

2. Methods

Entering a stressed medium, a polarized ray splits into two rays with mutually perpendicular polarizations. For these rays, due to the stress-induced optical anisotropy, the refractive index distributions are different, which causes a phase difference Δ after passing the rays through the medium. The relations connecting integral phase difference Δ (IPD) and the differences of quasi-principal RI values (n_n, n_l) or the differences of quasi-principal components (σ_n, σ_l) of the stress tensor in the plane perpendicular to the light ray (Wertheim’s integral law [28]) have the following form:

$$\mathrm{\Delta }=\frac{2\mathsf{\pi }}{\mathsf{\lambda }}{\int }_{\left(l\right)}(n_n-n_l)dl=C_0{\int }_{\left(l\right)}({\mathsf{\sigma }}_n-{\mathsf{\sigma }}_l)dl.$$

(1)

where C₀ is the optical stress coefficient, and λ is the wavelength. The integrals are taken over the ray path l.

Since a long rod, L > 2R (L and R are the length and radius of the cylinder, respectively), with radial RI distribution usually has no stress gradient along its axis (z coordinate), shear stresses σ_zr(r) in cylindrical coordinates are absent, and the stress state is characterized by 3 principal stress components: axial σ_zz(r), radial σ_rr(r), and tangential σ_θθ(r), depending only on the radial coordinate r [29,30]). Reconstructing the local distribution of principal stress components from translucency and Δ(x) measurements for different distances x from the optical axis is an incorrected (i.e., unstable with respect to input data errors) inverse problem of integral photoelasticity. The geometry of the longitudinal and transversal translucency is shown in Figure 1 and Figure 2.

As shown in [24,25,26], in the case of weakly and moderately gradient axisymmetric objects, the polarization of a probing beam is influenced by axial stresses σ_z(r) in transverse translucence and by the difference of tangential and radial stresses Δσ(r) = Δσ_θr(r) = σ_θ(r) − σ_r(r) in longitudinal translucence. RS difference distributions Δσ_θr(r) can be used to solve the direct problem of longitudinal translucence, that is, finding the radial distribution of the IPD and birefringence for GRIN segments of various lengths.

The derivation of a novel algorithm for solving the direct problem of longitudinal ray propagation in GRIN lenses and relations for the reconstruction of RS in their transverse translucency are presented in Section 2.1 and Section 2.2.

2.1. An Algorithm for Calculations of Longitudinal IPD (Direct Problem)

At the preliminary stage of the development of optimal modes of synthesis of GRIN lenses, it is usually limited to the search for conditions under which the parabolic (2) or paraxial refractive index profile is realized. To analyze IPD for longitudinal ray propagation in a GRIN lens (within the framework of a direct problem), it is more convenient to use the expression for the hypersecant refractive index profile (3).

$$n^2\left(r\right)=n_0^2\left(1-g^2{r}^2\right),$$

(2)

$$n^2\left(r\right)=n_0^2{\mathrm{sech}}^{2}gr,$$

(3)

where n₀ = n(0) − RI on the axis of the rod, and $g$ is power constant. When the input face of the GRIN lens at z = 0 is perpendicular to its axis and the rays fall at the input face parallel to the axis, the analytical expression of the trajectory equation at hypersecant RI distribution (3) is as follows [31]:

$$r\left(z\right)=\frac{1}{g}\mathrm{arcsh}\left(\mathit{sh}g{r}_0\cos gz\right)$$

(4)

At parabolic RI (2), it is as follows:

$$r\left(z\right)=r_0\cos \frac{g}{\sqrt{1-g^2{r}_0^2}}.$$

(5)

That is, the length of the periodicity L₀ depends on the $g$R constant:

$$L_0=\frac{2\mathsf{\pi }}{g}\sqrt{1-g^2{r}_0^2}.$$

(6)

Calculations of the relative differences in periodicity lengths depending on the ray entrance position x into the GRIN lens for $gR$ ≤ 0.3 show that the difference in ray propagation in GRIN lenses with parabolic and hypersecant index profiles is insignificant.

In the case of a longitudinal translucency GRIN lens (0.25 pitch), the relation (1) for the IPD Δ^L can be written as follows:

$$\begin{gathered} {\mathrm{\Delta }}^L={\mathrm{\Delta }}_0^L+{\mathrm{\Delta }}_1^L, \\ {\mathrm{\Delta }}_0^L={\int }_0^L{C}_0({\mathsf{\sigma }}_{\mathsf{\theta }}-{\mathsf{\sigma }}_r)\frac{n}{b}dz, \\ {\mathrm{\Delta }}_1^L={\int }_0^L{C}_0\left({\mathsf{\sigma }}_r-{\mathsf{\sigma }}_z\right)\left(1-\frac{b^2}{n^2}\right)\frac{n}{b}dz. \end{gathered}$$

(7)

For the direct problem, we also take into account the contribution to the IPD from the component ${\mathrm{\Delta }}_1^L$, which was neglected when considering the inverse problem for longitudinal translucency (i.e., the reconstruction of the RS from the corresponding experiments) [24,25,26].

As can be seen from expressions (7), it is required to perform integration along the GRIN lens axis, for which it is necessary to transform the input distributions (σ(r), n(r), C₀ (r)) into functions of z coordinate. In the case of the hypersecant distribution of RI, this problem is easily solved, since the explicit form of the trajectory equation r(z) (4) is known.

Let us denote the integrand in (7) as follows:

$$\begin{gathered} f_0\left(r\right)=C_0\left({\mathsf{\sigma }}_{\mathsf{\theta }}-{\mathsf{\sigma }}_r\right)\frac{n\left(r\right)}{n\left(x\right)}, \\ {f}_1\left(r\right)=C_0\left({\mathsf{\sigma }}_r-{\mathsf{\sigma }}_z\right)\left(\frac{n\left(r\right)}{n\left(x\right)}-\frac{n\left(x\right)}{n\left(r\right)}\right). \end{gathered}$$

(8)

If the initial stress values are given as an array of values for some points 0 = r₁ < r₂ < … < r_n = R, it is quite simple to express the function f₀ from the coordinate z, namely at the points r_i, where i = 1, 2, …, n, the values f_0j (j = n − i + 1), are calculated, and their corresponding values of z are found:

$$z_j=\frac{1}{g}\mathit{arc}\cos \left(\frac{\mathit{sh}\left(g{r}_i\right)}{\mathit{sh}\left(gx\right)}\right).$$

(9)

The integrand expressions in (7) are then approximated using splines with knots 0 = z₁ < z₂ < … < z_n = L₀/4, and the spline function can be integrated analytically:

$$\begin{gathered} {\int }_0^{L}s\left(z\right)dz=\sum _{i=1}^{m-1}I\left(z_i;z_{i+1}\right)+I\left(z_m,L\right), \\ I\left(z_i,z_{i+1}\right)={\int }_{z_i}^{z_{i+1}}s\left(z\right)dz, \\ f\left(z\right)\approx s\left(z\right)=a_i+b_i(z-z_i)+c_i(z-z_i{)}^2+d_i(z-z_i{)}^3. \end{gathered}$$

(10)

In this case, 0 < L < L₀/4, z_m < L < z_m+1.

The program based on this algorithm allows one to calculate Δ₀ and Δ₁ for lengths L ≤ L₀/4. For other lengths, one should take advantage of the fact that the ray propagates along a periodic trajectory and calculate Δ as follows:

$$\begin{gathered} {\mathrm{\Delta }}_{\mathrm{0,1}}=k{\int }_0^{\frac{L_0}{4}}{f}_{\mathrm{0,1}}\left(z\right)dz+{\int }_0^{L-k\frac{L_0}{4}}{f}_{\mathrm{0,1}}\left(z\right)dz,k-\mathit{is} \mathit{even}, \\ {\mathrm{\Delta }}_{\mathrm{0,1}}=(k+1){\int }_0^{L_0/4}{f}_{\mathrm{0,1}}(z)dz-{\int }_0^{L-k\frac{L_0}{4}}{f}_{\mathrm{0,1}}(z)dz,k-\mathit{is}\hspace{0.33em}\mathit{odd}. \end{gathered}$$

(11)

Here, k represents the number of quarters of a period on the trajectory.

2.2. Reconstruction of the Residual Stresses in Transversal Translucency (Inverse Problem)

When determining the stresses with this method, it is common to perform translucency on separate cross-sections of the object. As shown in [24,25], the integral equation for the phase difference (1) is reduced to the Abel integral equation for the case of transverse translucency of GRIN lenses with $gR$ ≤ 0.3:

$${\mathrm{\Delta }}^T(a)=2{\int }_a^{u_R}{C}_0{\mathsf{\sigma }}_z\frac{dr}{du}\frac{ndu}{\sqrt{u^2-a^2}},$$

(12)

which gives the following:

$${\mathsf{\sigma }}_z(r)=-(\mathsf{\pi }{C}_0{)}^{-1}\frac{du}{dr}{\int }_{u\left(r\right)}^{u_R}\frac{d{\mathrm{\Delta }}^T}{da}\frac{da}{\sqrt{a^2-u^2}},$$

(13)

where Δ^T(x) is the IPD of 2 plane-polarized oscillations in transverse translucence of the GRIN lens, a = xn_R, u = rn(r), and n_R ≡ n (R) is RI on the surface of the lens. As we see, expression (13) includes the singular convergent integral.

The stress components σ_θ(r) and σ_r(r) can be found using the so-called “sum rule” (14) [32], which is valid in the absence of an axial OH gradient, and the equilibrium condition for the cylinder (15) [29]:

$${\mathsf{\sigma }}_z\left(r\right)={\mathsf{\sigma }}_{\mathsf{\theta }}\left(r\right)+{\mathsf{\sigma }}_r\left(r\right),$$

(14)

$${\mathsf{\sigma }}_{\mathsf{\theta }}=d\left(r{\mathsf{\sigma }}_r\right)/dr.$$

(15)

Some properties of Δ^T(x) should be taken into account when analyzing experimental results and designing an algorithm for stress reconstruction. For example, due to the symmetry with respect to the rod axis (x = 0) it is obvious that

$$\frac{d{\mathrm{\Delta }}^T\left(x\right)}{dx}=0 \mathrm{at} x=0.$$

(16)

In addition, for solid rods, the following conditions must be satisfied for the stresses to remain finite at the axis of the rod; in other words, the total area under the Δ^T(x) curve must equal zero:

$${\int }_0^R{\mathrm{\Delta }}^T\left(x\right)dx=0.$$

(17)

This condition will not be fulfilled if, for example, the birefringence is not entirely caused by stress. This is possible if there is additional birefringence, e.g., induced by molecular orientation. The integral in the previous equation is a non-singular convergent integral, and there are difficulties in its numerical evaluation. In addition, there is one experimental limitation in the accuracy of Δ^T measurement. It can be shown that near the surface of the rod,

$${\left.\frac{d{\mathrm{\Delta }}^T\left(x\right)}{dx}\right|}_{x=R}=\infty .$$

(18)

As a result, Δ^T cannot be measured accurately near the surface, and the reconstruction of σ_z near the surface is inaccurate. In [33], the author overcomes this difficulty by finding the average birefringence along the light path, i.e., by extrapolating it to the outer surface of the rod. The rationale for this step lies in the fact that when the ray trajectory approaches the outer surface (i.e., x → R), the trajectory becomes shorter. Consequently, the mean value of σ_z tends to be its true value. In the limit, the path of light will be tangent to the rod, and Δ^T(x) = 0 because the path length goes to 0. However, the average birefringence approaches a certain value equal to the following:

$${\mathsf{\sigma }}_z(R)=\frac{1}{2C_0}\left\{\underset{x\to R}{lim}\frac{{\mathrm{\Delta }}^T\left(x\right)}{\sqrt{R^2-x^2}}\right\}=\frac{1}{C_0}\left\{\underset{x\to R}{lim}\frac{{\mathrm{\Delta }}^T\left(x\right)}{L\left(x\right)}\right\},$$

(19)

where $\hspace{0.33em}L(x)=2\sqrt{R^2-x^2}$, and L(x) is the geometric length of the probing ray path.

The relation to calculate the axial component RS on the surface of a cylinder accounting the curvature of the transmission rays is obtained in the following [24]:

$${\mathsf{\sigma }}_z\left(R\right)=\frac{3}{4}\frac{{\mathrm{\Delta }}^{Tmin}}{C_0\sqrt{R^2-x^2}}\frac{{\left[u^{\prime }\right]}_R}{n_R},$$

(20)

where x_m—is the translucency parameter corresponding to the IPD minimum near the cylinder surface; Δ^{T min}—IPD value at point x_m,$u^{\prime }\left(r\right)=\mathrm{d}u/\mathrm{d}r$.

Taking these into account, a regularized algorithm based on the spline approximation of Δ^T(x) and optimization of boundary conditions for ensuring the stability of the solution near the boundaries of the interval (0, R) was developed in [24,25,26].

It is important to note that the integral in (13) contains the derivative of the measured function, which leads to the enhancement of errors present in the experimental data.

In [34], it was shown how to avoid this in the case of a uniform (RI constant) glass cylinder with quenching stresses, which is used, in particular, in [35] for the reconstruction of RS in optical fiber preforms.

Let us find an analogous expression for the case of a cylindrical rod with an RI gradient. From relation (14), taking into account (15), we obtain the following:

$${\mathsf{\sigma }}_z(r)=\frac{1}{r}\frac{d}{dr}\left(r^2\mathsf{\sigma }r\right)$$

(21)

Substituting (21) into (12) and assuming that C₀(r)n(r) ~ C₀n_R, we obtain the Abel equation, the inversion of which gives the following:

$${\mathsf{\sigma }}_r(r)=-\frac{1}{\mathsf{\pi }{C}_0{n}_R}\frac{1}{r^2}{\int }_{u\left(r\right)}^{u_R}\frac{a{\mathrm{\Delta }}^T\left(a\right)da}{\sqrt{a^2-u^2}}.$$

(22)

The components σ_θ(r) and σ_z(r) can be found using (14) and (15).

In the limiting case of no RI gradient, (22) converges to the relation obtained in [34].

Thus, we eliminate the differentiation of the experimental function in the case of RS reconstruction for a cylinder with a RI gradient. Further, we use the algorithm based on relation (22) to reconstruct the RS in GRIN lenses from transverse translucency data.

The equations for the reconstruction of the axial and radial RS components (13) and (22), respectively, were obtained via Abel’s reversal (inversion) of the corresponding integral equations. To overcome the difficulties noted, robust solution algorithms have been developed. We used an algorithm based on approximating the original function with cubic splines, which could then be integrated analytically. The analytical expression of the spline allows us to easily express the derivative, which can be substituted into the integral (13).

Thus, computationally, the construction of the solution σ_z(r) at given spline coefficients is reduced to the summation of integrals expressed through elementary functions. Similar transformations were made for expression (22), which also produced a computationally simple solution algorithm. The algorithm was regularized by approximating the original function Δ(α) by smoothing splines with a reasonable choice of the vector of weight coefficients and boundary conditions of the spline approximation. The choice of boundary conditions was carried out taking into account the properties of the function under study (integral phase difference).

2.3. Measurements

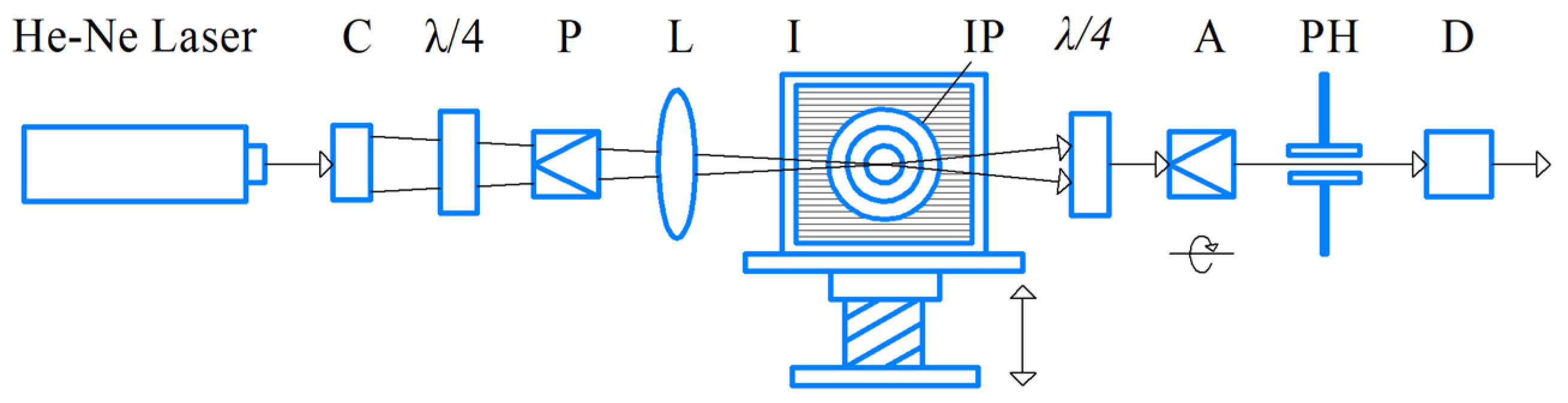
A polarization-optical setup with a He-Ne laser and a Senarmon compensator were used for the measurements (Figure 3).

The rotation angles of the polarizer, the λ/4 plate, and the analyzer were measured with an accuracy better than ±1°, i.e., the difference of the path between the radially and tangentially polarized beams could be measured with an accuracy of ±3.5 × 10⁻⁶ mm (λ = 0.633 µm). With a specimen length of 20 mm, this corresponds to a measurement accuracy of

$$\mathsf{\delta }\left(\mathrm{\Delta }{n}_{r\mathsf{\theta }}\right)=\pm 2\times 10^{-5}.$$

(23)

The specimen under study is immersed in a cuvette with an index-matching fluid (with a refractive index n_oil) to eliminate the refraction of light at the specimen surface. Compensation angles ψ_i = Δ_i(x_i) were recorded during the measurements for a given translucency parameter x_i, which was varied by precisely moving the cuvette stage. The proper choice of the n_oil allowed the refraction in the GRIN to be partially compensated by the refraction at the sample boundary. The errors in the illumination parameter introduced in this case are as follows:

$$\mathsf{\delta }x=\frac{n_{\mathit{oil}}-n_R}{n_R}\approx 10^{-2}.$$

(24)

To estimate the reconstruction error δσ_z from transverse translucency data of cylindrical lenses, we use the expression obtained for the gradient-free case [36]:

$$\mathsf{\delta }{\mathsf{\sigma }}_Z=\frac{\lambda /2\mathsf{\pi }}{\mathsf{\pi }C\mathsf{\delta }x}\mathsf{\delta }\mathrm{\Delta }\sqrt[ ]{\left(\mathsf{\delta }x/r\right)\ln \left(2r/\mathsf{\delta }x\right)},$$

(25)

where δx and δΔ are errors in the translucency parameter x and phase difference Δ. For the used polarimetric unit, it amounted to δΔ ~ 10′ (3 × 10⁻³ rad). The uncertainty of the parameter x is determined with the radius of the beam waist, Δx ~ 40 µm. Thus, at C₀ = 3.5 Br and r = 0.1 mm, the error is δσ_z ~ 0.06 kg/mm²; at r = 1 mm, it is δσ_z ~ 0.03 kg/mm² (0.6 and 0.3 MPa, respectively). These calculations do not account for uncertainties in the determination of RS, refractive index, and the influence of the gradient, but nevertheless allow us to conclude that the reconstruction technique used provides results with satisfactory accuracy.

2.4. Samples under Study

To illustrate and verify the approach developed, we have studied GRIN lenses of lithium zirconosilicate glass OPS [37,38] and alumosilicate glass TSM-412 [39] subjected to lithium-sodium ion exchange, elaborated and provided by S. I. Vavilov State Optical Institute Scientific Production Association and St. Petersburg Technological University of Plant Polymers. The characteristics of the investigated GRIN lenses are given in

Table 1. Characteristics of the investigated GRINs.

Specimen №	Glass	RI Original nD	Co, Br	Δn	Rod Diameter, mm	Temp. IE, °C	IE Duration, h	Content of Alkali Oxides, mol. % Li2O Na2O
1	OPS-83	1.61	2.8	0.0108	15	630	57	13 13
2	OPS-83	1.66	2.8	0.0134	16	630	60	13 13
3	TSM-412	1.524	2.7	0.008	9.9	660	24	11 10

.

In the present study, samples 1 and 2 were investigated via transverse translucency only, and sample 3 was investigated via both transverse and longitudinal translucency. Two types of characterization were used, i.e., a wide beam with scanning through a pinhole in the image plane of the object—longitudinal translucency (∆^L(x) and scanning of the object itself with a focused beam ~40 µm in diameter—transverse translucency (∆^T(x)). RS distributions σ(r) were reconstructed using a spline approximation Δ(x).

3. Results and Discussion

3.1. Residual Stresses

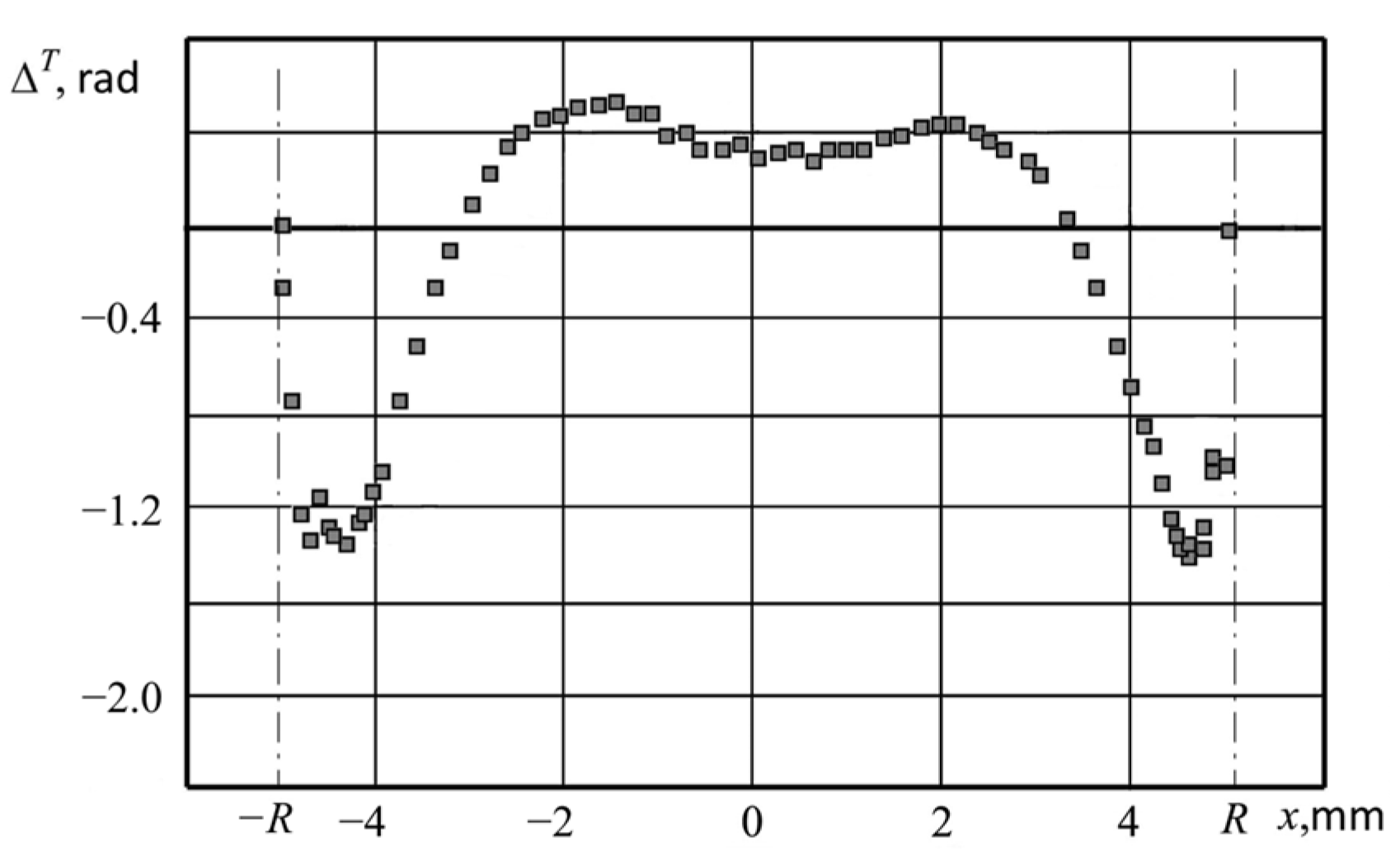
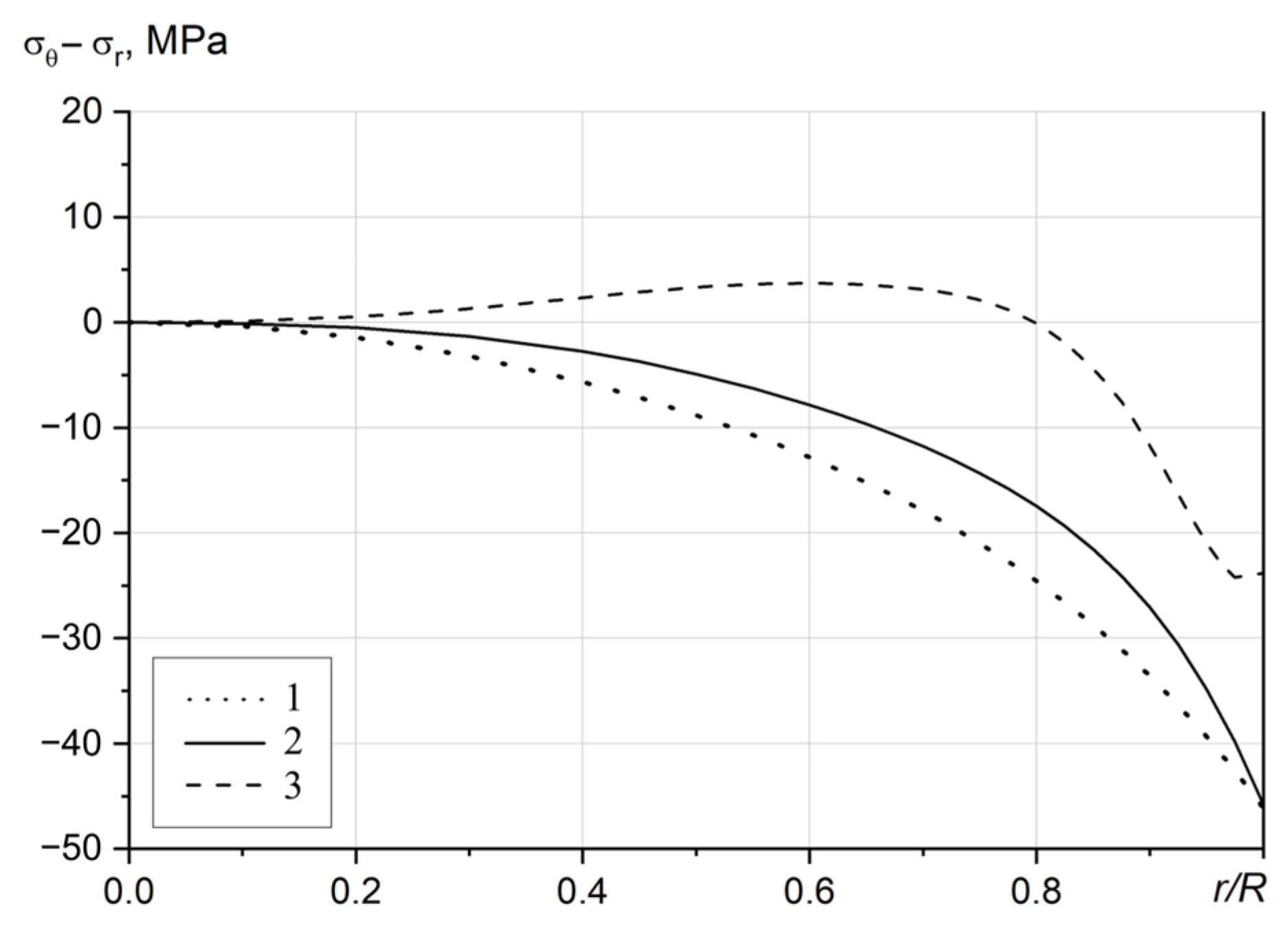
Figure 4 shows the phase difference vs. transverse translucency parameter for GRIN lens 3. Figure 5a gives the radial RS distributions of all the RS components for this GRIN. RS was reconstructed via calculations within the inverse problem based on the data in Figure 4.

Figure 5b presents similar dependencies on the RS reconstruction for GRIN 1.

In the RS reconstruction code, the experimental data were smoothed using splines.

It is necessary to note significantly higher values of the axial component of RS in GRIN 1 than in GRIN 3 (negative regions in RS dependencies correspond to compressive stresses). The reconstructed radial RS distributions of all RS components for samples 1, 2, and 3 are input arrays for calculations within the framework of the direct problem of birefringence characterization (functional optical anisotropy) under the longitudinal variant of rays passing through the corresponding GRIN lenses.

The radial distribution of differences in tangential and radial components Δσ_θr in specimens 1, 2, and 3 is shown in Figure 6.

As mentioned, the positive values of σ_z(r) correspond to tensile stresses, while negative values correspond to compressive ones.

3.2. Integral Phase Difference

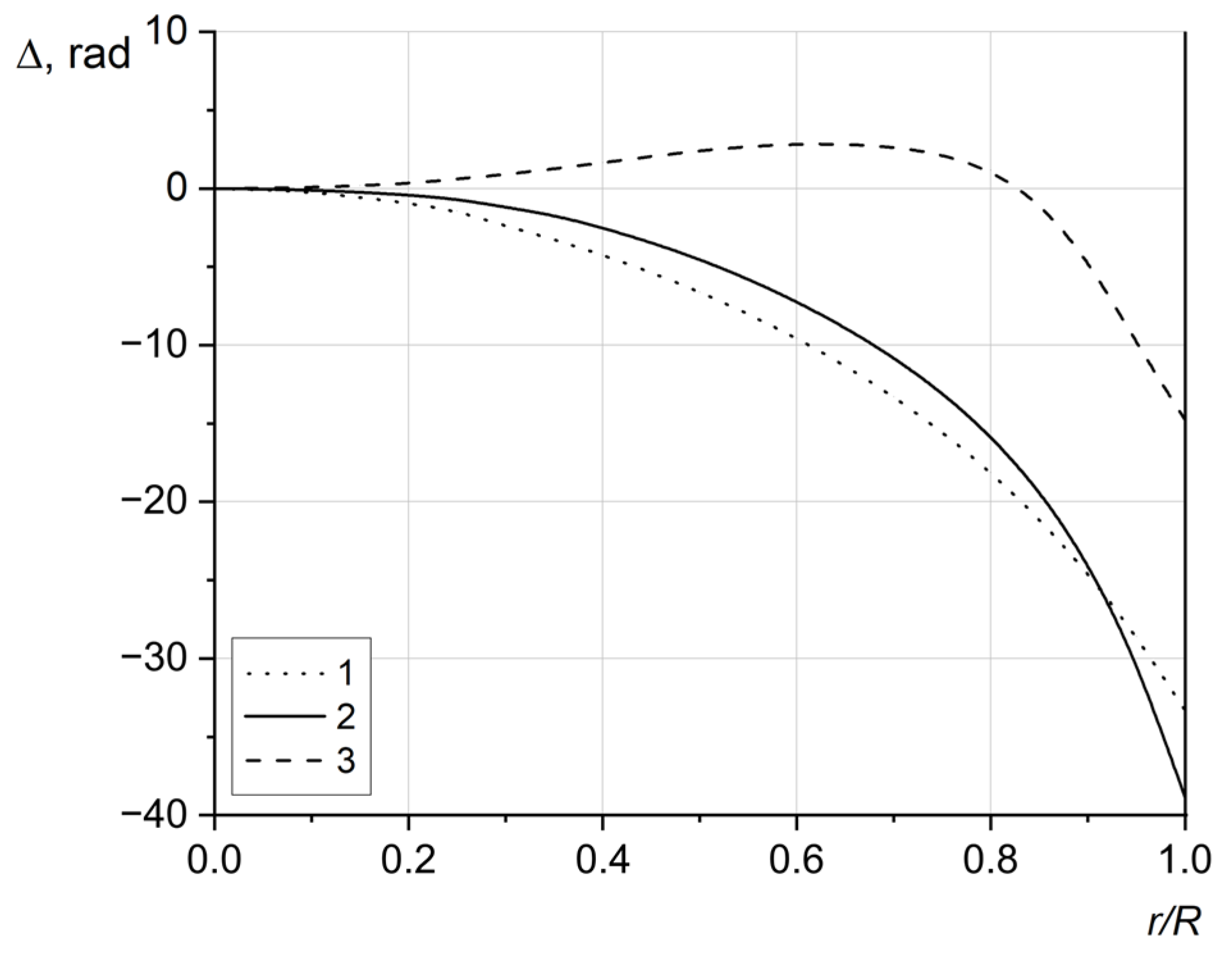
For GRIN lenses 1, 2, and 3, using the obtained RS distributions as inputs, the IPD was calculated for the hypersecant RI distribution using the “direct problem” solution algorithm (see Section 2.1). The results are presented in Figure 7.

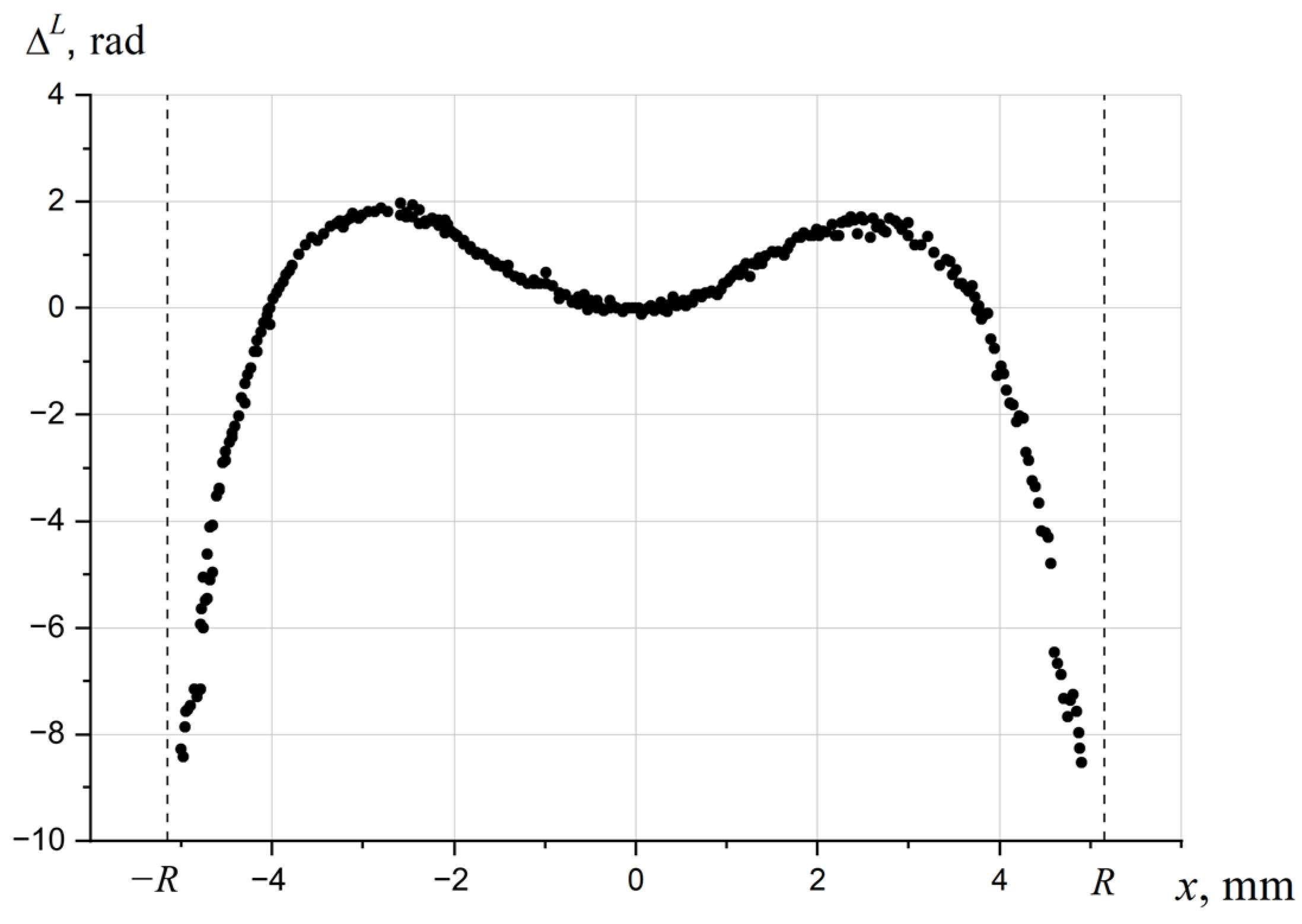
Using specimen 3, we studied the longitudinal translucency, which is presented in Figure 8.

As in the case of transverse translucency, the averaged experimental data were smoothed using splines.

Thus, the results show that all investigated GRIN lenses are characterized by sufficiently strong compressive stresses in the near-surface layers, which provides them with increased mechanical strength. A related effect is the large values of tangential and radial stress differences and, as a consequence, significant optical anisotropy in these GRIN lenses.

An example of a comparison of the IPD deduced from the transverse translucency data with experimental data on longitudinal translucency is shown in Figure 9 for specimen 3.

As can be seen, the calculated phase differences are quite close to the experimental ones, which confirms the applicability and accuracy of the developed algorithms.

The results obtained are applicable to the design of all GRIN lenses that are transparent to visible and near-infrared optical radiation (wavelength 0.5–1.5 µm). In this case, the diameters of the GRINs can range from 1 to 10 mm. The calculations of the optical characteristics of such GRIN lenses within the framework of the approach used are based on the geometrical optics approximation. In other spectral ranges, in particular at longer wavelengths, the relations presented are not valid.

4. Conclusions

Finally, in this paper, we present an algorithm allowing us to reconstruct the distribution of residual stresses and longitudinal optical anisotropy of GRIN lenses on the basis of transversal transmission measurements. Measurements of this kind, contrary to longitudinal transmission measurements, do not require precise segmentation of GRIN lenses, which essentially simplifies the lens characterization and, respectively, experimental studies aimed at the design of new gradient structures. The approach developed was verified in the experiments with a set of different GRIN lenses formed with the ion exchange technique, and the closeness of the data obtained from transversal and conventional longitudinal transmission measurements was demonstrated.

Modern gradient lenses with minimized birefringence are industrially produced and widely used in image transmission devices and fiber optic systems. At the same time, as noted in the Introduction, the combination of the focusing properties of GRIN lenses with radial birefringence has proven to be very effective for optical vortex generation in devices for polarization imaging of biological tissue structure, etc. [19,20,40,41,42]. Except for this, vortex beam generation systems are of interest for integrated photonics and communication systems using waves with a spiral wavefront. In these cases, it is relevant to increase the usually low level of optical anisotropy of GRIN lenses, i.e., their birefringence, and the models presented in this paper can be effectively used in the development of GRIN lenses with increased birefringence.