Electromagnetic Guided Wave in Goubau Line with Graphene Covering: TE Case

Abstract

This paper focuses on the problem of monochromatic terahertz TE-polarized wave propagation in a special type of circle cylindrical waveguides, the so-called Goubau line. The outer shell of the waveguide is covered with graphene characterized by complex surface conductivity. This covering affects electromagnetic wave propagation due to the generation of a surface current in graphene. The nonlinear interaction of graphene with the electromagnetic field is taken into account via a nonlinear term involving in graphene conductivity. Starting from the rigorous formulation for Maxwell’s equations with appropriate boundary and transmission conditions, we derive the dispersion equation for propagation constants. We discuss this result and point out some methods of studying the dispersion equation analytically. At the same time, we suggest numerical experiments shedding light on how cubic nonlinearity affects electromagnetic wave propagation.

1. Introductory Remarks

Two-dimensional materials and electronic components are actively studied nowadays [1,2]; among these two-dimensional materials, graphene, discovered in 2004 by A. Geim and K. Novoselov [3,4], occupies a special place. Graphene is a single sheet of carbon atoms in a hexagonal lattice; due to its special structure, graphene has linear, massless band structure, high carrier mobility and many other unusual electrical, thermal, mechanical and optical properties that make graphene promising for various applications [5]. For example, in photonics and optoelectronics, a great deal of attention has been paid to graphene-integrated waveguiding structures having different forms starting from simple rectangular and circular cylindrical waveguides to highky exotic complicated configurations that can serve as very effective photodetectors, modulators, polarizers, sensors, etc. [6].

One of the most important things about graphene is its ability to interact with electromagnetic waves in a wide frequency range, particularly in the so called «terahertz gap», which is the region from $0.1$ to 10 THz. As is known, terahertz technologies have extensive applicability in different areas of science, such as chemical and bio-sensing [7], near-field imaging [8] and spectroscopy and on-chip communication [9]. However, designing effective electrical components able to process and transmit THz waves is still a serious problem slowing down the development of THz technologies. Graphene, having almost purely imaginary surface conductivity in this frequency range, is believed to be beneficial for solving the mentioned problem [10,11].

In the papers in [12,13], it was theoretically predicted for the first time that graphene has strong cubic nonlinearity caused by the interaction of charge carriers in graphene with electromagnetic field. Since then, a plethora of studies revealed different nonlinear properties of graphene, including saturable absorption and nonlinear refraction [14,15], higher-harmonic generation [16,17] and wave-mixing processes [18,19]. In particular, at technologically important THz frequencies, saturable absorption in doped graphene [20,21] and the generation of the third harmonic [22] were experimentally detected. The mechanism of graphene’s nonlinear response at THz frequencies is discussed in [23]. The discovery of the nonlinear response of graphene has led to significant efforts to realize a new generation of nonlinear photonic guided-wave devices [24].

This paper focuses on the propagation of a monochromatic terahertz TE-polarized wave in the so-called Goubau line, which is a metallic wire surrounded by a dielectric layer; the outer boundary of the waveguide is covered with graphene. As is known, the guiding properties of graphene-integrated structures are crucial for different applications and have been studied by many authors [25,26,27,28,29,30,31]. The ability to propagate TM- and TE-polarized waves localized on a graphene monolayer with the dispersion in the terahertz range of electromagnetic radiation is shown in [25,26,27]. The ability of the structure formed by two graphene layers and a separating thin dielectric layer to hold the localized plasmon modes was studied in many papers [28,29,30]. In [31], the authors investigate the features of guided TE wave modes in a plane structure consisting of a set of alternating dielectric and graphene layers. The present study has the following important novel feature. We take into account the nonlinear interaction of graphene with electromagnetic waves. To be more precise, we assume that graphene’s conductivity is a sum of two terms: the first one is a constant and the second term depends on the modulus square of the tangential component of an electric field. Such nonlinearity corresponds to the so-called self-modulated effects in graphene. Other nonlinear effects such as higher harmonic generation are not considered in our study. We derive an explicit dispersion equation that allows us to determine the propagation constants of a waveguide with given structural (inner and outer radii) and material (permittivity of the medium in the waveguide and surrounding space and surface conductivity of the graphene covering) characteristics. It should be noted that by aiming to obtain a dispersion equation explicitly, we are forced to impose some restrictions on graphene conductivity, which will be discussed below in more detail. Nevertheless, the dispersion equation written in an explicit form is an important result. Studying this equation numerically or analytically, one can obtain crucial guiding properties of the considered waveguiding structure. A similar nonlinear problem for a plane dielectric waveguide covered with graphene is studied in [32].

Finally, it is worth noting beforehand that we consider the problem of finding the eigenmodes of the waveguide. In eigenwave problems, energy losses are often neglected assuming they are small and the propagation length of eigenwaves is sufficient for applications. Such an approach is applied in this paper as well: we neglect dielectric and absorption losses in graphene. We stress that although in general cases the absorption of graphene affects the wave propagation significantly and cannot be neglected, at THz frequencies it is small compared to the strong plasmonic response of graphene.

2. Statement of the Problem

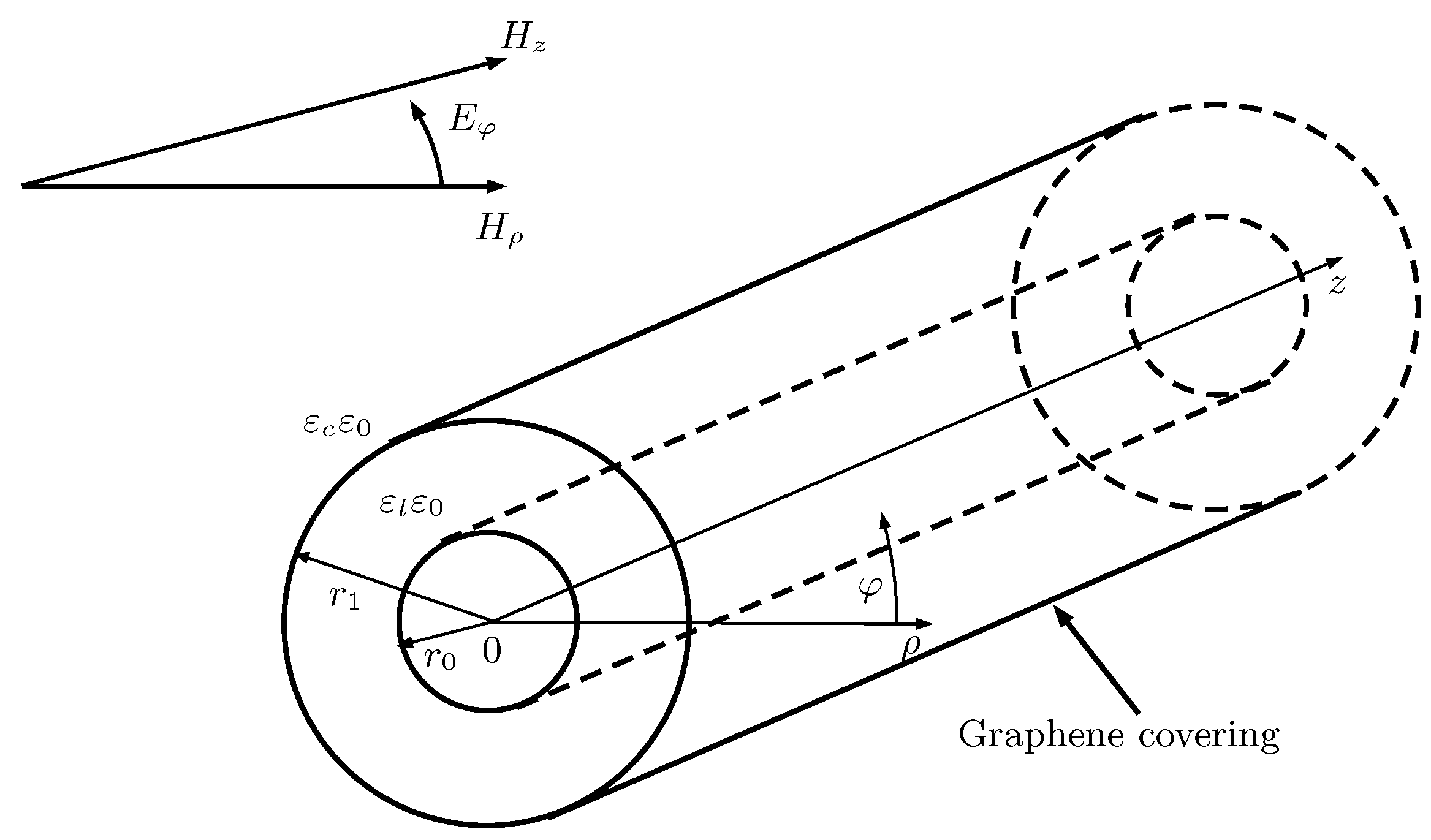
Let us consider a three-dimensional space ${\mathbb{R}}^3$ with a cylindrical coordinate system $O\rho \phi z$. The space is filled with an isotropic medium of dielectric permittivity ${\epsilon }_c{\epsilon }_0=const$, where ${\epsilon }_0$ is the permittivity of free space, without sources. The medium is assumed to be isotropic and nonmagnetic. A cylindrical dielectric waveguide of a circular cross section

$$W:=\left\{\left(\rho ,\phi ,z\right):r_0⩽\rho <r_1,0⩽\phi <2\pi \right\}$$

with a generating line parallel to the axis $Oz$ is placed in ${\mathbb{R}}^3$. It is supposed that, everywhere, $\mu ={\mu }_0$, where ${\mu }_0$ is the permeability of free space.

The cross section of the waveguide, which is perpendicular to its axis, consists of two concentric circles of radii: $r_0$ and $r_1$, see Figure 1. The inner cylinder (of radius $r_0$) is a metal rod that has perfectly conducted surface at $\rho =r_0$; $r_0$ is the radius of the inner cylinder; and $r_1-r_0$ is the thickness of the outer cylindrical shell.

The geometry of the problem is shown in Figure 1. The waveguide is unlimitedly continued in the z direction.

Let $\mathbf{E}$ and $\mathbf{H}$ be complex amplitudes of an electromagnetic field. The complex amplitudes $\mathbf{E}$ and $\mathbf{H}$ satisfy Maxwell’s equations

$$\left\{\begin{array}{c}\hfill \mathrm{rot}\mathbf{H}=-i\omega \epsilon \mathbf{E},\hfill \\ \hfill \mathrm{rot}\mathbf{E}=i\omega \mu \mathbf{H}.\hfill \end{array}\right.$$

(1)

Tangential electric field components vanish at $\rho =r_0$, and tangential field components satisfy transmission conditions at $\rho =r_1$ and obey the radiation condition at infinity; i.e., the electromagnetic field decays exponentially as $\rho \to \infty$ in the region $\rho >r_1$; $\omega$ is the circular frequency.

The permittivity in the entire space has the form $\epsilon =\tilde{\epsilon }{\epsilon }_0$, where

$$\tilde{\epsilon }=\left\{\begin{array}{cc}\hfill {\epsilon }_l,\hfill & \hfill r_0⩽\rho ⩽r_1,\hfill \\ \hfill {\epsilon }_c,\hfill & \hfill \rho >r_1,\hfill \end{array}\right.$$

(2)

and ${\epsilon }_l⩾1,{\epsilon }_c⩾1$ are real positive constants such that

$${\epsilon }_c<{\epsilon }_l.$$

The tangential component of the magnetic field has a jump at the boundary $\rho =r_1$ due to the surface current of charge carries in graphene (the current is induced by the electromagnetic wave) and the jump is equal to the surface current density $\mathbf{j}$; thus, at $\rho =r_1$, the discussed component satisfies the following condition:

$$[\mathrm{n},{\mathbf{H}}^{+}-{\mathbf{H}}^{-}]=\mathbf{j}={\sigma }_g\mathbf{E},$$

(3)

where $\mathbf{n}$ is a unit vector of the normal directed along the x axis, ${\mathbf{H}}^{+}$ and ${\mathbf{H}}^{-}$ are the values of magnetic field inside and outside the surface $\rho =r_1$, respectively, ${\sigma }_g$ is the surface conductivity of graphene and $[\ast ,\ast ]$ is the vector product.

The surface conductivity ${\sigma }_g$ characterizes the interaction of graphene with electromagnetic waves. It consists of two components related to the interband and intraband transitions in graphene caused by the excitation of graphene with light. Interband transitions lead to the creation of an electron–hole pair, whereas intraband transitions correspond to the free-carrier absorption. The significance of interband and intraband components depends on the photon energy $\hslash \omega$ and the Fermi energy $E_F$ of graphene. For low-energy photons (such that inequality $\hslash \omega <2E_F$ is fulfilled), as is usually the case at terahertz frequencies, the interband transitions are Pauli-blocked [33,34], and only intraband transitions are possible. In other words, at terahertz frequencies one can neglect the interband component in graphene conductivity and suppose that ${\sigma }_g$ equals its intraband component.

In addition, we suppose that surface conductivity, ${\sigma }_g$, of graphene depends on the intensity of electric field coupling to charge carriers in graphene. Taking into account the central symmetric structure of graphene, we assume that ${\sigma }_g$ has the form

$${\sigma }_g={\sigma }^{(1)}+{\sigma }^{(3)}{|\mathbf{E}|}^2,$$

(4)

where ${\sigma }^{(1)}$ and ${\sigma }^{(3)}$ are generally some complex quantities.

The linear part ${\sigma }^{(1)}$ of graphene’s electric conductivity is determined by the formula

$${\sigma }^{(1)}=i·\mathrm{Im}{\sigma }_{intra},$$

(5)

where

$${\sigma }_{intra}=\frac{2i{e}^2{k}_{b}T}{\pi {\hslash }^2(\omega +i{\tau }^{-1})}·ln\left[2cosh(\frac{{\mu }_c}{2k_{b}T})\right];$$

(6)

here, e is the electron charge, $k_b$ is the Boltzmann constant, ${\tau }^{-1}$ is the collision rate of carriers in graphene, ${\mu }_c$ is the chemical potential and T is the temperature [35,36]. We stress that we neglect the interband component in graphene conductivity as well as the real part of the intraband component. This is reasonable in the terahertz range, where graphene has a strong plasmonic response and much less loss. 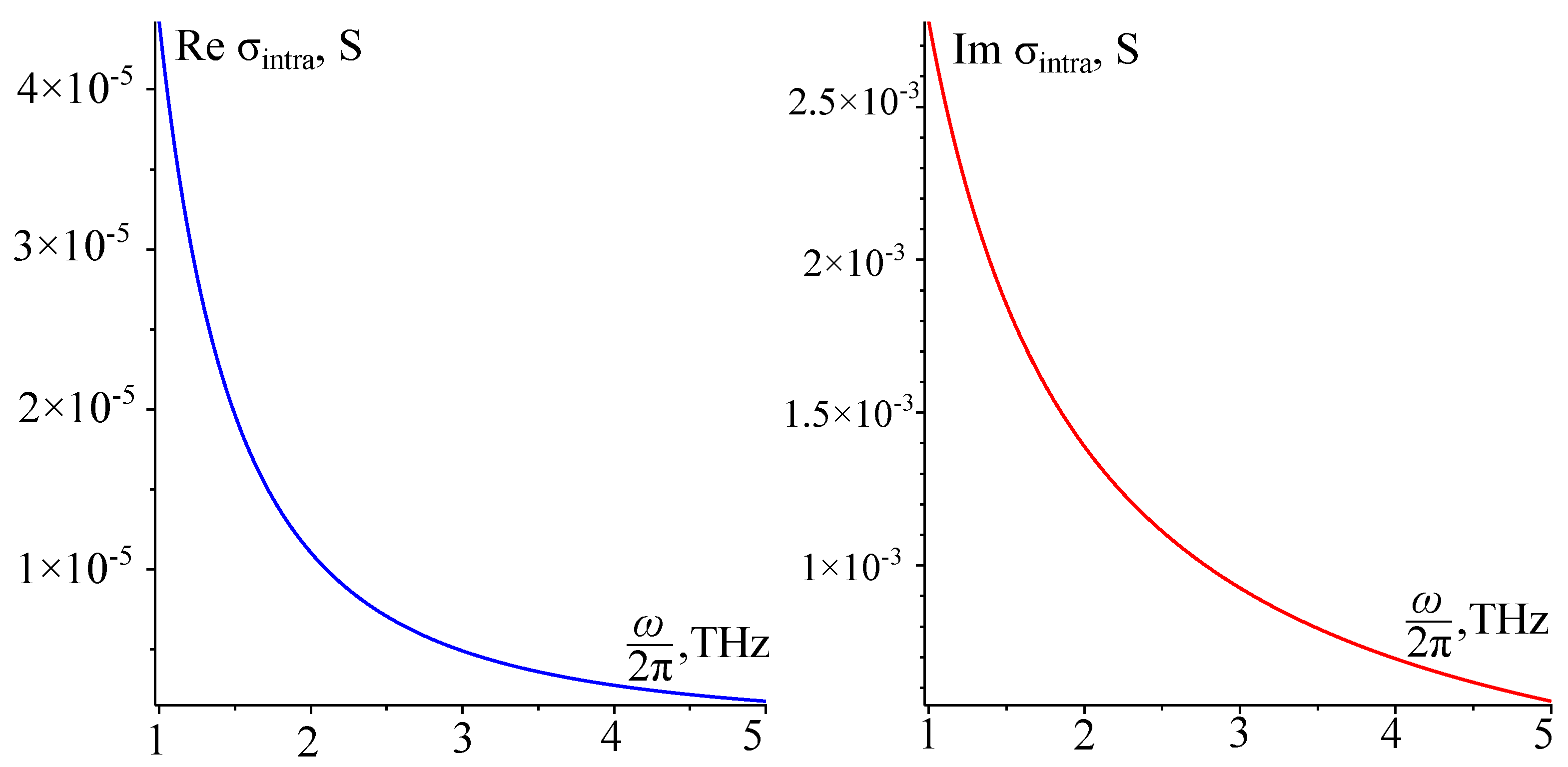Figure 2 show real and imaginary parts of ${\sigma }_{intra}$ at THz frequencies. One can see that imaginary part is two orders larger than the real one. We stress that the relation between the real and imaginary parts of ${\sigma }_{intra}$ depends significantly on the parameter $\tau$. To be more precise, the dominance of the imaginary part is valid for scattering time $\tau$ on the order of picoseconds or larger. We use $\tau =10\mathrm{ps}$ in our calculations [37]. A similar lossless model of graphene is considered in [38,39].

For determining the nonlinear coefficient ${\sigma }^{(3)}$ in (4) various formulas are proposed [12,13,40,41]. We use the one that can be acquired from [13] and suppose that

$${\sigma }^{(3)}=-i\frac{3e^4{v}_F^2}{32{\omega }^3{\hslash }^2{\mu }_c},$$

(7)

where $v_F\approx c_0/300$ is the Fermi velocity in graphene.

3. TE Waves

Let us consider TE-polarized waves in the harmonic mode

$$\mathbf{E}{e}^{-i\omega t}=e^{-i\omega t}{(0,E_{\phi },0)}^T,\mathbf{H}{e}^{-i\omega t}=e^{-i\omega t}{(H_{\rho },0,H_z)}^T,$$

(8)

where $\mathbf{E},\mathbf{H}$ are complex amplitudes and

$$E_{\phi }={\mathrm{E}}_{\phi }(\rho )e^{i\gamma z},H_{\rho }={\mathrm{H}}_{\rho }(\rho )e^{i\gamma z},H_z={\mathrm{H}}_z(\rho )e^{i\gamma z},$$

(9)

where $\gamma$ is a propagation constant. The polarization direction of the electromagnetic waves (8) is presented in Figure 1.

Let $k_0^2:={\omega }^2\mu {\epsilon }_0$. Thus, substituting the components in (9) into (1) and using the notation $u(\rho ;\gamma ):={\mathrm{E}}_{\phi }(\rho )$, we obtain

$${\left({\rho }^{-1}{\left(\rho u\right)}^{\prime }\right)}^{\prime }+\left(k_0^2\tilde{\epsilon }-{\gamma }^2\right)u=0,$$

(10)

where $\tilde{\epsilon }={\epsilon }_0^{-1}\epsilon$, and $\tilde{\epsilon }$ is defined by Formula (2).

We seek $\gamma$ under conditions ${\gamma }^2>{\epsilon }_c$.

Let

$$k_l^2:=k_0^2{\epsilon }_l-{\gamma }^2\mathrm{and}{k}_c^2:={\gamma }^2-k_0^2{\epsilon }_c.$$

In the domain $r_0⩽\rho ⩽r_1$, Equation (10) takes the form

$$u^{″}+{\rho }^{-1}{u}^{\prime }-{\rho }^{-2}u+k_l^{2}u=0.$$

(11)

In the domain $\rho >r_1$, Equation (10) takes the form

$$u^{″}+{\rho }^{-1}{u}^{\prime }-{\rho }^{-2}u-k_c^{2}u=0.$$

(12)

The necessary solutions to Equations (11) and (12) must be written in the following form:

$$\begin{array}{ccc}\hfill & \hfill u=c_1{J}_1(k_l\rho )+c_2{N}_1(k_l\rho ),\hfill & \hfill r_0<\rho <r_1,\end{array}$$

(13)

$$\begin{array}{ccc}\hfill & \hfill u=c_3{K}_1(k_c\rho ),\hfill & \hfill \rho >r_1.\end{array}$$

(14)

The functions $J_1$, $N_1$ and $K_1$ are the Bessel functions and $c_1,c_2,c_3$ are constants. The radiation condition is satisfied because $K_1(k_c\rho )\to 0$ exponentially as $\rho \to \infty$ [42].

4. Boundary and Transmission Conditions

The boundary conditions take the form

$${u|}_{\rho =r_0}=0.$$

(15)

Transmission conditions for the functions u and $u^{\prime }$ result from the continuity conditions for the tangential field components and have the form

$$\begin{array}{cc}\hfill {u|}_{\rho =r_1-0}{-u|}_{\rho =r_1+0}& \hfill =0,\hfill \end{array}$$

(16)

$$\begin{array}{cc}\hfill u^{\prime }{{|}_{\rho =r_1-0}-u^{\prime }|}_{\rho =r_1+0}& \hfill =-120\pi i{\sigma }_g{u|}_{\rho =r_1-0},\hfill \end{array}$$

(17)

where

$${\sigma }_g={\sigma }^{(1)}+{\sigma }^{(3)}{u}^2{|}_{\rho =r_1-0},$$

(18)

which results from (4), taking into account the form of field (8) and the realness of parameter $\gamma$.

The above listed conditions result from electromagnetic theory. However, since the main problem is nonlinear with respect to the sought-for function (see (17)), then in order to define discrete propagation constants one should impose one more condition. For this purpose, we use the following boundary condition:

$$u^{\prime }{|}_{\rho =r_0}=\frac{2a}{\pi r_0},$$

(19)

where a is supposed to be a known real parameter.

Let us formulate the transmission eigenvalue problem (problem P) to which the problem of surface waves propagating in a cylindrical waveguide has been reduced. The goal is to find quantities $\gamma$ such that for $a\ne 0$ there is a nonzero function $u(\rho ;\gamma )$ that solves Equation (11) for $r_0<\rho <+\infty$, which satisfies boundary conditions (15)–(17) and (19) and exponentially decays as $\rho \to +\infty$.

The quantities solving problem P are called eigenvalues, and the corresponding functions $u(\rho ;\gamma )$ are called eigenfunctions. It should be noted that the eigenvalue $\gamma$ depends on the value of the eigenfunction on one of the waveguide boundaries.

5. Dispersion Relation

Below, we use the following formulas:

$$\begin{array}{cc}\hfill J_1^{\prime }(ts)& \hfill =t{J}_0(ts)-\frac{1}{s}{J}_1(ts),\hfill \\ \hfill N_1^{\prime }(ts)& \hfill =t{N}_0(ts)-\frac{1}{s}{N}_1(ts),\hfill \\ \hfill K_1^{\prime }(ts)& \hfill =-t{K}_0(ts)+\frac{1}{s}{K}_1(ts),\hfill \end{array}$$

where ${(\dots )}^{\prime }=\frac{d}{ds}$ [43].

Substituting (13) into (15), one can choose

$$c_1=-c{N}_1(k_l{r}_0)\mathrm{and}{c}_2=c{J}_1(k_l{r}_0),$$

(20)

where c is an unknown and nonzero real constant.

Calculating $u^{\prime }$ at the point $\rho =r_0$, from Formulas (13) and (20) one obtains

$$\begin{array}{c}\hfill u^{\prime }{|}_{\rho =r_0}=c{\left.{\left(J_1(k_l{r}_0)N_1(k_l\rho )-N_1(k_l{r}_0)J_1(k_l\rho )\right)}^{\prime }\right|}_{\rho =r_0}=c{k}_l\left(J_1(k_l{r}_0)N_0(k_l{r}_0)-J_0(k_l{r}_0)N_1(k_l{r}_0)\right)=\frac{2c}{\pi r_0}.\end{array}$$

(21)

Now, substituting (21) into (19), one finds

$$c=a.$$

(22)

Taking into account the found results, solution (13) takes the form

$$u=av,$$

(23)

where

$$v=J_1(k_l{r}_0)N_1(k_l\rho )-N_1(k_l{r}_0)J_1(k_l\rho ).$$

(24)

Now, substituting (14) into (16) and (17), one obtains

$$\begin{array}{cc}\hfill {u|}_{\rho =r_1-0}-c_3{K}_1(k_c{r}_1)& \hfill =0,\hfill \end{array}$$

(25)

$$\begin{array}{cc}\hfill u^{\prime }{{|}_{\rho =r_1-0}-c_3{K}_1^{\prime }(k_c\rho )|}_{\rho =r_1+0}& \hfill =-120\pi i{\sigma }_g{u|}_{\rho =r_1-0},\hfill \end{array}$$

(26)

where ${\sigma }_g$ is defined by Formula (18).

Below, for brevity, we use the notation ${u|}_{r_1}$ instead of ${u|}_{\rho =r_1-0}$.

Expressing $c_3$ from (25), one finds

$$c_3=\frac{{u|}_{r_1}}{K_1(k_c{r}_1)}.$$

(27)

Substituting the found $c_3$ and $K_1^{\prime }(k_c\rho ){|}_{\rho =r_1+0}=-k_c{K}_0(k_c{r}_1)+\frac{1}{r_1}{K}_1(k_c{r}_1)$ into (26), one obtains

$$v^{\prime }{|}_{r_1}+\left(k_c\frac{K_0(k_c{r}_1)}{K_1(k_c{r}_1)}-\frac{1}{r_1}\right)v{{|}_{r_1}=-120\pi i{\sigma }_{g}v|}_{r_1},$$

(28)

where

$${\sigma }_g={\sigma }^{(1)}+{\sigma }^{(3)}{a}^2{v}^2{|}_{r_1},$$

(29)

Expression (28) is called the dispersion relation of problem P. Below, we consider the dispersion relation in different forms that are equivalent to relation (28).

One can rewrite relation (28) in the form

$$\frac{v^{\prime }{|}_{r_1}}{{v|}_{r_1}}=-k_c\frac{K_0(k_c{r}_1)}{K_1(k_c{r}_1)}+\frac{1}{r_1}-120\pi i{\sigma }_g.$$

(30)

Relation (30) is a form of the dispersion relation of the main problem. Below, we derive another form of this relation that can also be useful in analytical as well as numerical study.

Calculating $v^{\prime }{|}_{r_1}$ using the formulas in the beginning of this section, one obtains

$$\begin{array}{c}\hfill v^{\prime }{|}_{r_1}=J_1(k_l{r}_0)\left(k_l{N}_0(k_l{r}_1)-\frac{1}{r_1}{N}_1(k_l{r}_1)\right)-N_1(k_l{r}_0)\left(k_l{J}_0(k_l{r}_1)-\frac{1}{r_1}{J}_1(k_l{r}_1)\right)=\hfill \\ \hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}=k_l\left(J_1(k_l{r}_0)N_0(k_l{r}_1)-N_1(k_l{r}_0)J_0(k_l{r}_1)\right)-\frac{1}{r_1}\left(J_1(k_l{r}_0)N_1(k_l{r}_1)-N_1(k_l{r}_0)J_1(k_l{r}_1)\right)=\hspace{1em}\hspace{1em}\hspace{1em}\\ \hfill =k_l\left(J_1(k_l{r}_0)N_0(k_l{r}_1)-N_1(k_l{r}_0)J_0(k_l{r}_1)\right)-\frac{1}{r_1}{v|}_{r_1},\end{array}$$

(31)

Using (31), one obtains from (30)

$$k_l\frac{J_1(k_l{r}_0)N_0(k_l{r}_1)-N_1(k_l{r}_0)J_0(k_l{r}_1)}{J_1(k_l{r}_0)N_1(k_l{r}_1)-N_1(k_l{r}_0)J_1(k_l{r}_1)}=-k_c\frac{K_0(k_c{r}_1)}{K_1(k_c{r}_1)}+\frac{2}{r_1}-120\pi i{\sigma }_g,$$

(32)

Relation (32) is another form of the dispersion relation found above.

One can easily see that relation (30) (or (32)) is equivalent to the original problem; that is, if $\gamma$ is a propagation constant of the main problem, then this $\gamma$ is a solution to relation (30) (or (32)) and vice versa.

Dispersion relations for two special cases are presented below.

If ${\sigma }^{(3)}=0$, from (32) one obtains

$$k_l\frac{J_1(k_l{r}_0)N_0(k_l{r}_1)-N_1(k_l{r}_0)J_0(k_l{r}_1)}{J_1(k_l{r}_0)N_1(k_l{r}_1)-N_1(k_l{r}_0)J_1(k_l{r}_1)}=-k_c\frac{K_0(k_c{r}_1)}{K_1(k_c{r}_1)}+\frac{2}{r_1}-120\pi i{\sigma }^{(1)},$$

(33)

If ${\sigma }^{(1)}={\sigma }^{(3)}=0$, from (32) one obtains the classical dispersion equation for the Goubau line in the form

$$k_l\frac{J_1(k_l{r}_0)N_0(k_l{r}_1)-N_1(k_l{r}_0)J_0(k_l{r}_1)}{J_1(k_l{r}_0)N_1(k_l{r}_1)-N_1(k_l{r}_0)J_1(k_l{r}_1)}=-k_c\frac{K_0(k_c{r}_1)}{K_1(k_c{r}_1)}+\frac{2}{r_1},$$

(34)

In the next section, we numerically study relations (32)–(34) and plot the found results.

Now we discuss a few opportunities that can be used to analytically study relations (30), (32)–(34).

The first opportunity is in consideration of an auxiliary boundary value problem. Let us consider this auxiliary boundary value problem for Equation (11) with boundary conditions ${u|}_{r_0}{=u|}_{r_1}=0$. The first of these conditions coincides with condition (15), the second one gives that ${v|}_{r_1}$ in (30) vanishes. It follows from classical results [44] that zeros $\gamma$ of ${u|}_{r_1}$ (or zeros of ${v|}_{r_1}$) are simple (of multiplicity 1).

Looking on relation (30), one can see that if ${v|}_{r_1}$ vanishes for a some value of $\gamma$, this implies the existence of at least one solution to (30). Indeed, the numerator in the left-hand side is continuous and bounded for the considered range of $\gamma$; the same is true for the right-hand side. Since the denominator v vanishes at $r_0$ and $r_1$ and taking into account that zeros of ${v|}_{r_1}$ are simple, then the graph of the right-hand side necessarily intersects the graph of the left-hand side (as the graph of the left-hand side changes from $-\infty$ to $+\infty$). Thus, the above introduced auxiliary boundary value problem can be used to study the original problem in more detail.

The other opportunity is in detailed consideration of the expression for ${v|}_{r_1}$. Indeed, if we denote $k_l{r}_0$ as x and $\frac{r_0}{r_1}$ as k, we obtain for ${v|}_{r_1}$ the following expression:

$${v|}_{r_1}=J_1(kx)N_1(x)-N_1(kx)J_1(x),$$

(35)

where $0<k<1$. Using $k_l{r}_1$ as x and $\frac{r_1}{r_0}$ as k, we obtain a similar expression with $k>1$.

As is well known, the function $J_{\nu }(kx)N_{\nu }(x)-N_{\nu }(kx)J_{\nu }(x)$ for real $\nu$ and positive k has infinite real and simple (of multiplicity 1) zeros $x=x_0,x_1,\dots$ [45,46]. This means that one can always choose $k=\overline{k}$ and $x=\overline{x}$ and, therefore, the parameters $r_0$, $r_1$, $k_0$, ${\epsilon }_l,\dots$ of the main problem, such that ${v|}_{r_1}$ vanishes m times as x changes from 0 to $\overline{x}$. Obviously, this does not mean that for a prescribed set of parameters of the main problem function ${v|}_{r_1}$ vanishes. However, the above formulated fact can be used to find (or prove the existence of) solutions of the main problem.

6. Numerical Results

In the calculations below, we use the following parameters: ${\epsilon }_l=11.7$, ${\epsilon }_c=3.7$, ${\overline{\sigma }}^{(1)}=-0.35\mathrm{S}$, ${\overline{\sigma }}^{(3)}=1.3·{10}^{-14}\mathrm{S}·{\mathrm{m}}^2·{\mathrm{V}}^{-2}$, where ${\overline{\sigma }}_1=120\pi i{\sigma }^{(1)}$, ${\overline{\sigma }}_3=120\pi i{\sigma }^{(3)}$.

It is worth providing a comment concerning the chosen parameters. The elative dielectric permittivities ${\epsilon }_l=11.7$ and ${\epsilon }_c=3.7$ correspond to silicon (Si) and silicon dioxide (${\mathrm{SiO}}_2$), respectively [47,48]. The quantities ${\sigma }^{(1)}$ and ${\sigma }^{(3)}$ are obtained by virtue of Formulas (5) and (7), respectively, using the parameters $\omega =2\pi ·3·{10}^{12}\mathrm{Hz}$, ${\mu }_c=0.15\mathrm{eV}$, $T=300\mathrm{K}$ and $\tau =40\mathrm{ps}$.

For finding the propagation constants of the waveguide numerically, we use the following approach. We fix some segment on $\gamma$, say $\gamma \in [{\gamma }^{\prime },{\gamma }^{″}]$, and generate a grid with nodes ${\gamma }^{\prime }⩽{\gamma }_1<{\gamma }_2<\dots <{\gamma }_n⩽{\gamma }^{″}$. Let us rewrite dispersion Equation (32) in the form $F(\gamma )=0$, where

$$F(\gamma )=k_l\frac{J_1(k_l{r}_0)N_0(k_l{r}_1)-N_1(k_l{r}_0)J_0(k_l{r}_1)}{J_1(k_l{r}_0)N_1(k_l{r}_1)-N_1(k_l{r}_0)J_1(k_l{r}_1)}+k_c\frac{K_0(k_c{r}_1)}{K_1(k_c{r}_1)}-\frac{2}{r_1}+120\pi i{\sigma }_g;$$

remembering that $k_l\equiv k_l(\gamma )$, $k_c\equiv k_c(\gamma )$. Then, for each $\gamma ={\gamma }_i$, we can calculate $F({\gamma }_i)$. Finally going through all ${\gamma }_i$, we look at whether condition $F({\gamma }_i)·F({\gamma }_{i+1})<0$ is fulfilled. If it is fulfilled, then the segment $\gamma \in [{\gamma }_i,{\gamma }_{i+1}]$ definitely contains a propagation constant of the waveguide. The described approach is implemented using the mathematical package «Maple».

Analyzing the dimensions of quantities involved in problem $\mathcal{P}$, one can see that for the variable $\rho$ and parameter $\gamma$ it is convenient to use $k_0^{-1}$ and $k_0$, respectively, as units of measurement. Remember that $k_0=\omega \sqrt{\mu {\epsilon }_0}$; so, for $\omega =2\pi ·3·{10}^{12}\mathrm{Hz}$, we obtain $k_0\approx 2\pi ·{10}^5{\mathrm{m}}^{-1}$.

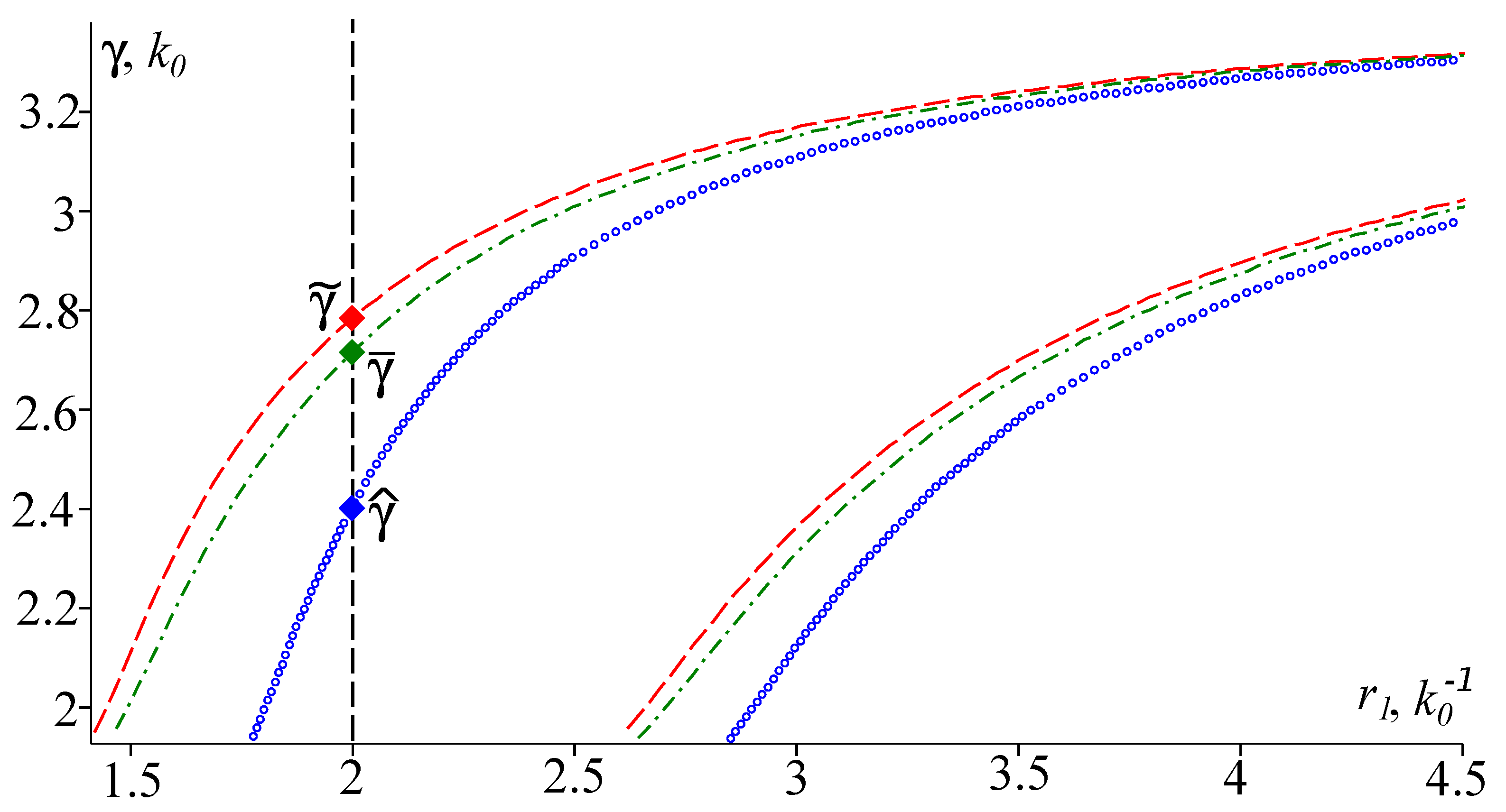
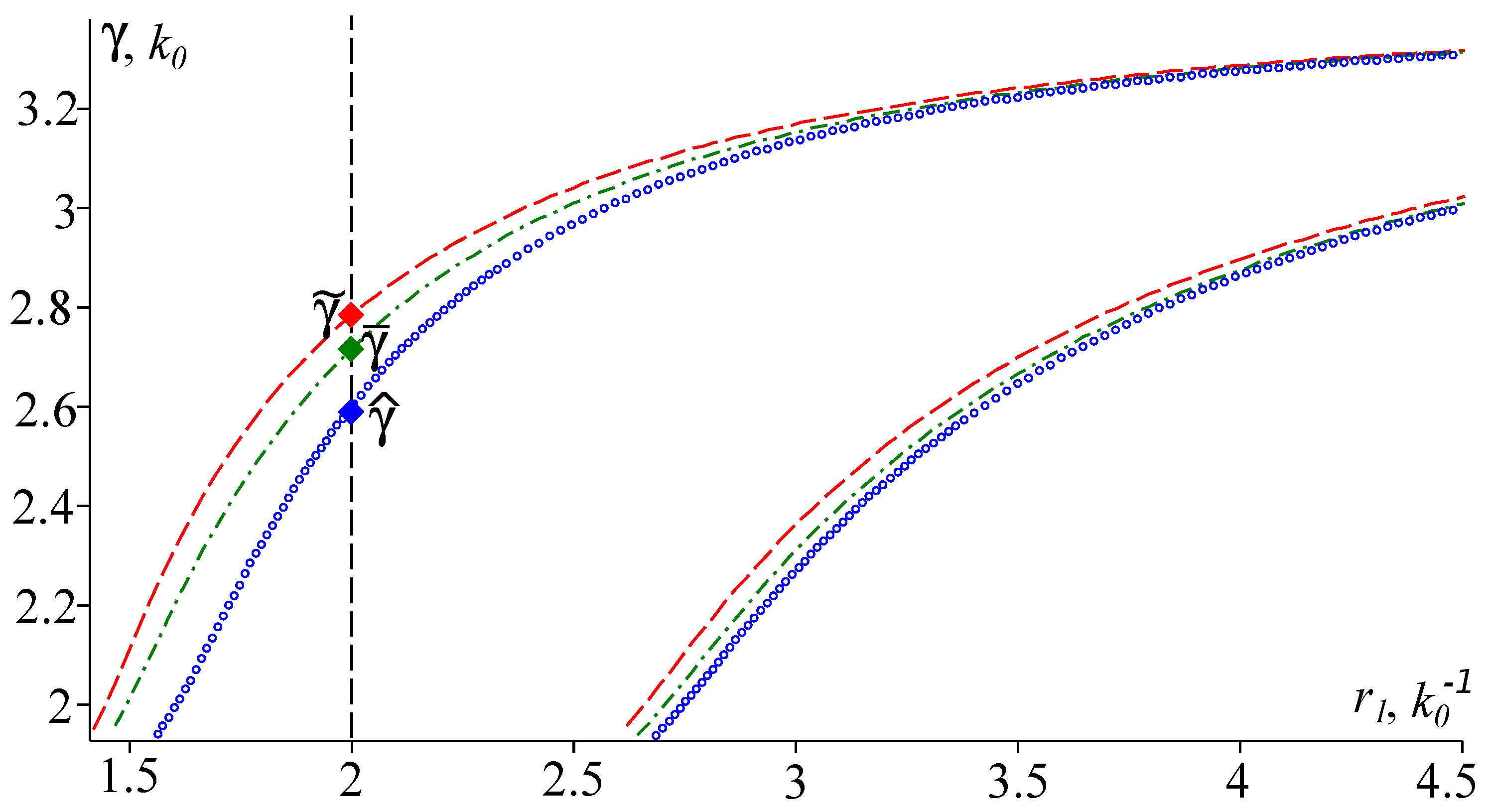
In Figure 3, the dispersion curves of problem $\mathcal{P}$ for nonlinear (${\sigma }_1\ne 0$, ${\sigma }_3\ne 0$), linear (${\sigma }_1\ne 0$, ${\sigma }_3=0$) and «no-graphene» (${\sigma }_1={\sigma }_3=0$) cases are presented. The dispersion curves are plotted as the dependence of a wave number (propagation constant) on either the wave frequency $\omega$ or radius $r_1$ of a waveguide. We chose the second option and plotted them as $\gamma$ vs. $r_1$.

The vertical line $r_1=2k_0^{-1}$ in Figure 3 corresponds to the waveguide of radius $r_1=2k_0^{-1}$. The intersection points of dispersion curves with this line denoted by diamonds are eigenvalues of problem $\mathcal{P}$ (propagation constants of the waveguide) in nonlinear, linear or «no-graphene» cases. One can see that for $r_1=2k_0^{-1}$, problem $\mathcal{P}$ has only one solution (in all three cases). From a physical point of view, it means that the corresponding waveguide has only one eigenmode.

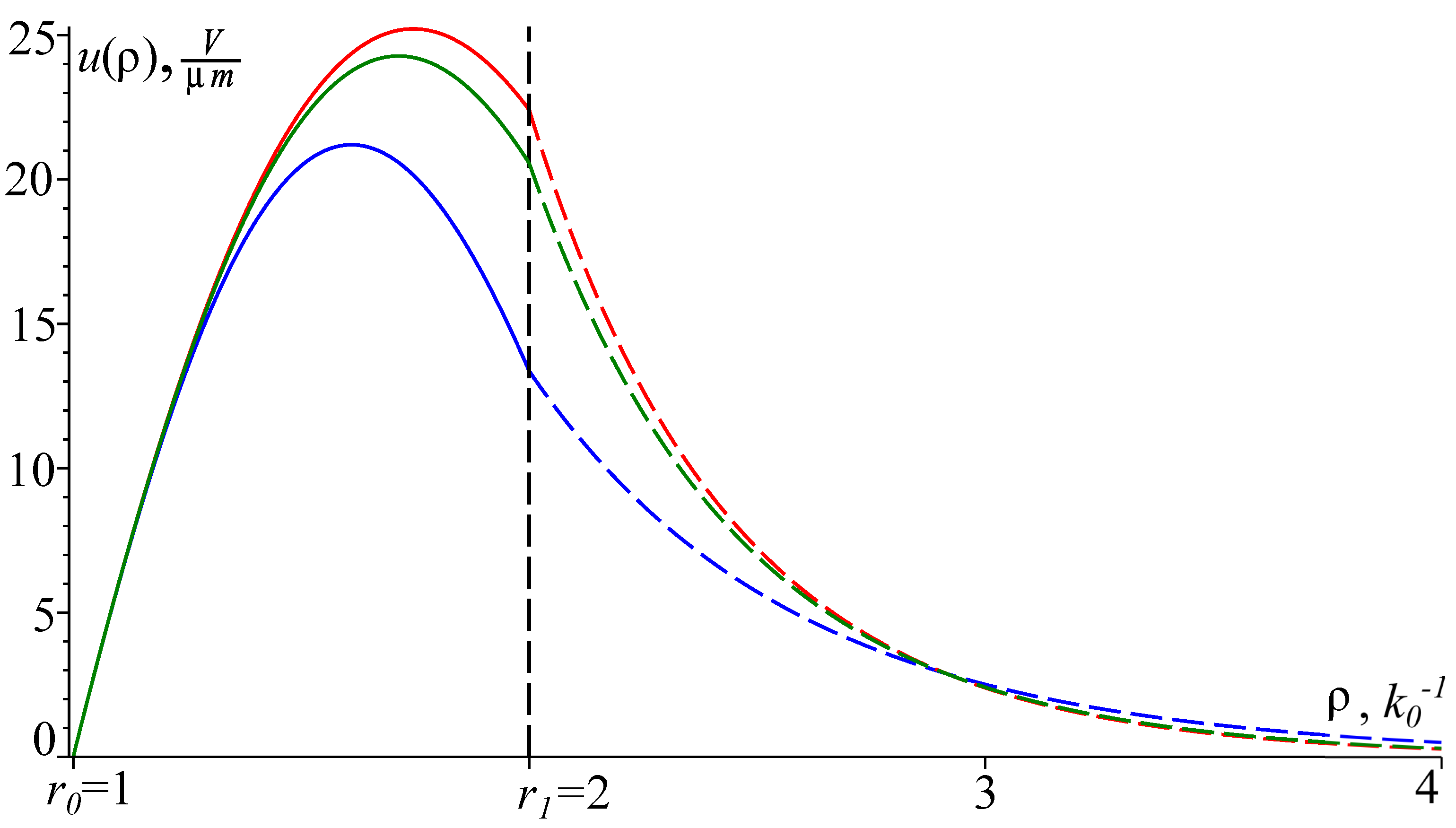
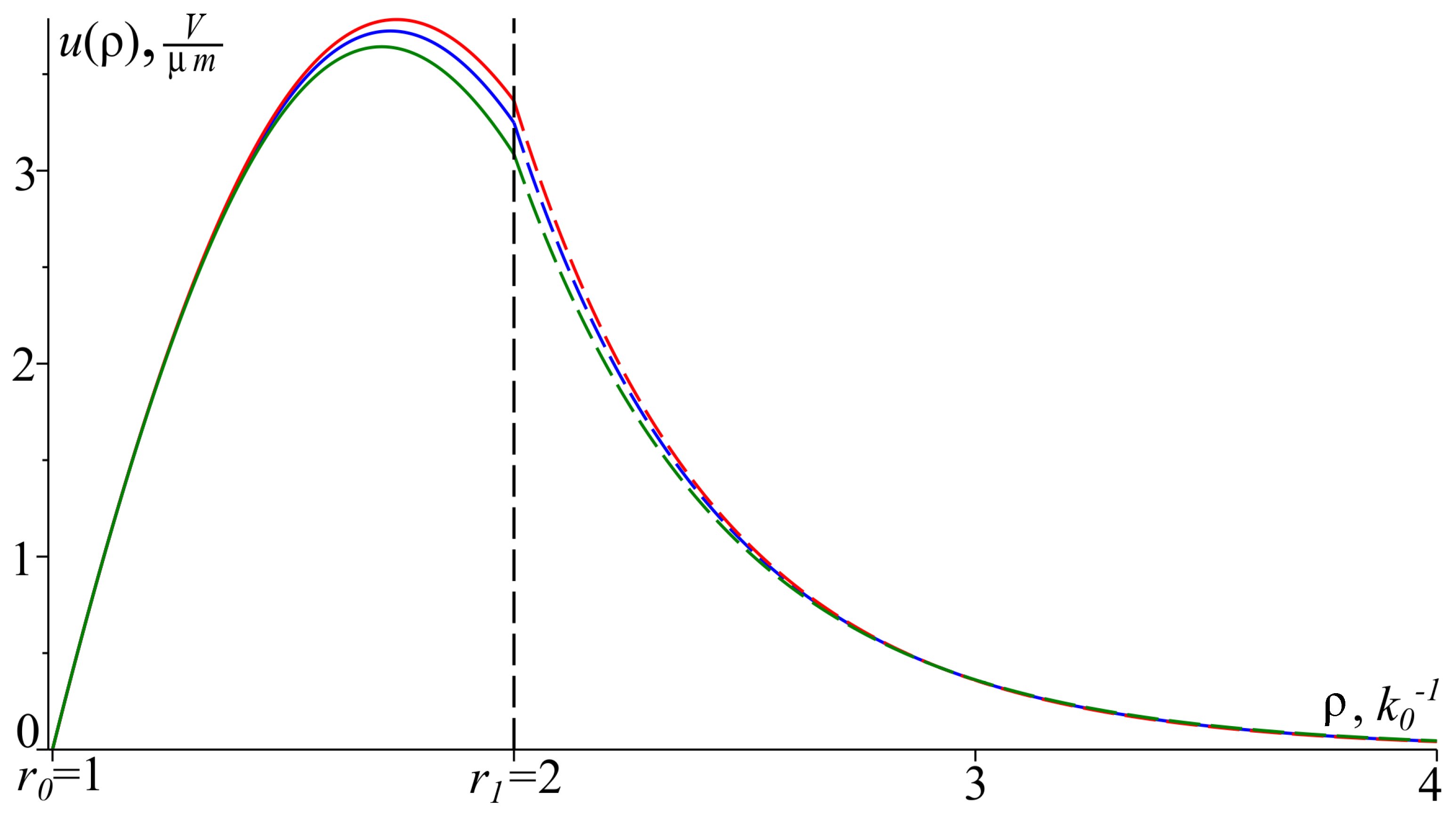
In Figure 4, we plot the eigenfunctions $\widehat{u}=u(\rho ;\widehat{\gamma })$, $\tilde{u}=u(\rho ;\tilde{\gamma })$ and $\overline{u}=u(\rho ;\overline{\gamma })$ of problem $\mathcal{P}$ corresponding to the eigenvalues $\widehat{\gamma }$, $\tilde{\gamma }$ and $\overline{\gamma }$ denoted in Figure 3. It can be shown that if ${\sigma }_3$ tends to zero, then $\widehat{\gamma }\to \tilde{\gamma }$, and if in addition ${\sigma }_1$ tends to zero, then $\tilde{\gamma }\to \overline{\gamma }$ and $\widehat{\gamma }\to \overline{\gamma }$. The same is true about the corresponding eigenfunctions, i.e., ${lim}_{{\sigma }_3\to 0}\widehat{u}=\tilde{u}$ and ${lim}_{{\sigma }_1\to 0,{\sigma }_3\to 0}\widehat{u}={lim}_{{\sigma }_1\to 0}\tilde{u}=\overline{u}$. So, as expected, in Figure 4 the eigenfunctions $\widehat{u}$, $\tilde{u}$ and $\overline{u}$ are similar to each other. Meanwhile, Figure 4 also demonstrates an important difference between the nonlinear case and two other ones. It can be seen that in the nonlinear case the absolute value of the eigenfunction (tangential component of the electric field) at the boundary $\rho =r_1$ is significantly smaller than in the linear and «no-graphene» cases. This means that the nonlinearity arising in graphene leads to a greater localization of the electromagnetic field within the waveguide. Beside this, the maximum value of the eigenfunction (tangential component of the electric field) in the nonlinear case is smaller than in two other cases, and the peak point shifts to the left relative to its position in the linear and «no-graphene» cases.

The real values (involving dimensions) of the propagation constants denoted in Figure 3, Figure 5 and Figure 6 are presented in

Table 1. The values of the propagation constants denoted in Figure 3, Figure 5 and Figure 6 (involving dimensions).

a, $\mathbf{V}·{\mathbf{m}}^{-1}$	$\overline{\mathit{\gamma }}$, ${\mathbf{m}}^{-1}$	$\tilde{\mathit{\gamma }}$, ${\mathbf{m}}^{-1}$	$\widehat{\mathit{\gamma }}$, ${\mathbf{m}}^{-1}$
${10}^8$	$170,274$	$174,672$	$150,796$
$5\times {10}^7$	$170,274$	$174,672$	$162,734$
$1.5\times {10}^7$	$170,274$	$174,672$	$172,787$

. The real sizes of inner and outer radii of the Goubau line are $r_0=k_0^{-1}\approx 1.6\mathsf{\mu }\mathrm{m}$ and $r_1=2k_0^{-1}\approx 3.2\mathsf{\mu }\mathrm{m}$.

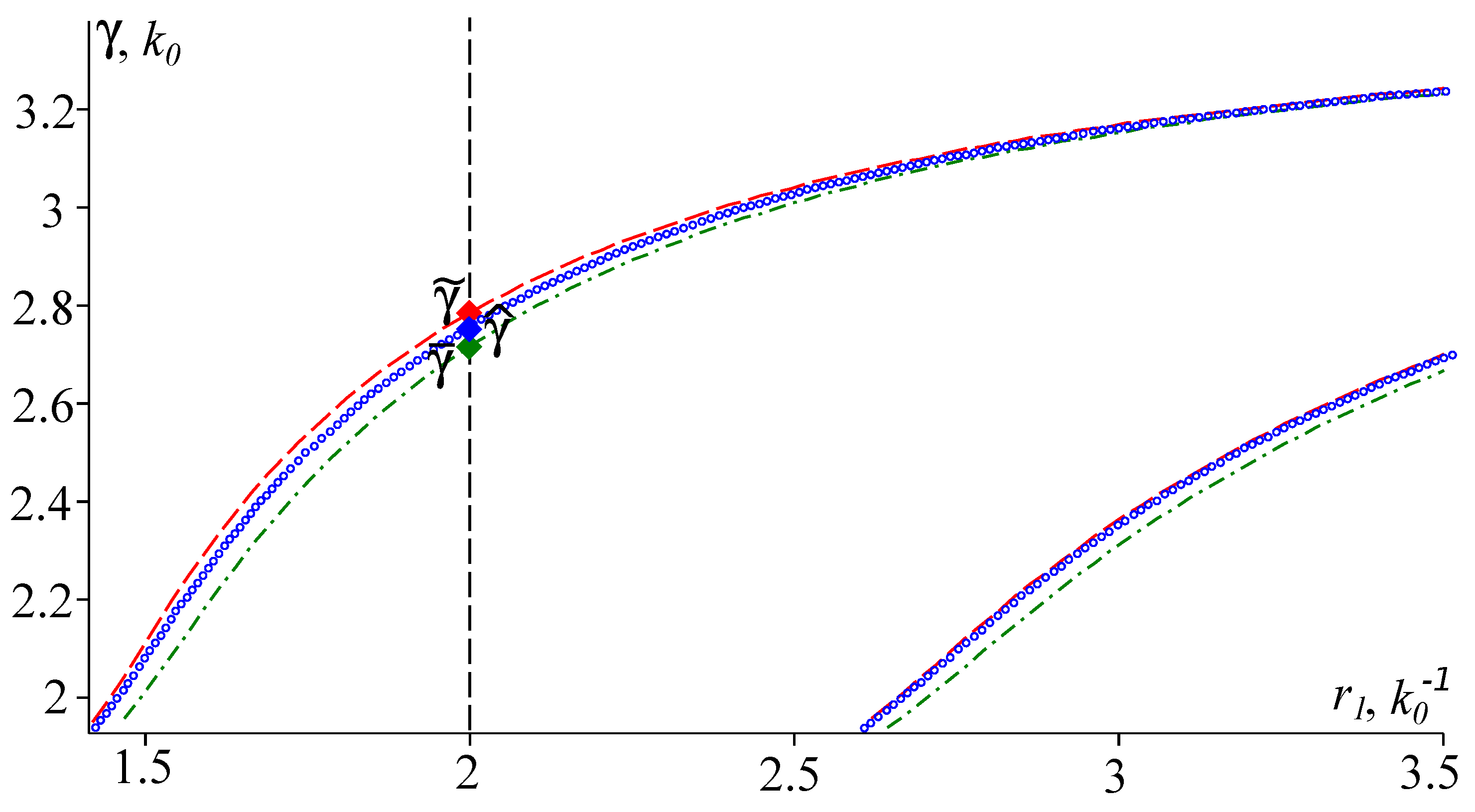
It also seems interesting to find at which conditions the discussed nonlinear effect in graphene becomes significant. In Figure 5 as well as in Figure 3, we plot dispersion curves of problem $\mathcal{P}$ for the nonlinear (${\sigma }_1\ne 0$, ${\sigma }_3\ne 0$), linear (${\sigma }_1\ne 0$, ${\sigma }_3=0$) and «no-graphene» (${\sigma }_1={\sigma }_3=0$) cases; however, in the calculations, we use smaller value for the amplitude of the electric field. In this case, the dispersion curves in the nonlinear case are no longer strongly different from those for the two other cases. In Figure 7, the eigenfunctions $\widehat{u}=u(\rho ;\widehat{\gamma })$, $\tilde{u}=u(\rho ;\tilde{\gamma })$ and $\overline{u}=u(\rho ;\overline{\gamma })$ of problem $\mathcal{P}$ corresponding to eigenvalues $\widehat{\gamma }$, $\tilde{\gamma }$ and $\overline{\gamma }$ denoted in Figure 5 are plotted. Here, again, one can see that the eigenmode corresponding to the nonlinear case is more localized within the waveguide than its linear and «no-graphene» counterparts; however, the effect is much weaker than the one demonstrated in Figure 4 due to the smaller amplitude of the electric field. Further reducing the amplitude of the electric field, we come to situation where the effect of localization in the nonlinear case is still better in comparison with the linear case but worse in comparison with the «no-graphene» case; see Figure 6 and Figure 8.

7. Conclusions

In this paper, using an analytical approach, we give a study of an important problem of guided-wave theory. We study the propagation of monochromatic TE-polarized electromagnetic waves in the so-called Goubau line covered with graphene. An important feature of this study is that we take into account the cubic nonlinearity of graphene corresponding to self-action effects, which do not affect the frequency of the incident electromagnetic wave. Using an analytical approach, we derive an explicit dispersion equation (involving a nonlinear term) that fully describes the guiding properties of the considered waveguiding structure. The numerical results presented in this paper give some insight into how the nonlinearity of graphene affects waveguide modes. For example, in Figure 3 and Figure 5, one can see that dispersion curves corresponding to the waveguide covered with graphene characterized by linear conductivity are located above the dispersion curves corresponding to the waveguide with no covering, whereas the dispersion curves corresponding to the waveguide covered with graphene characterized by nonlinear conductivity are located below them.

So, taking into account the relation between wave number and wavelength, one finds that in the Goubau line covered with graphene in the linear regime, electromagnetic waves with shorter wavelength can propagate more compared to the electromagnetic waves propagating in the Goubau line with no covering. This feature can be useful for some applications. However, in the strong nonlinear regime (for a sufficiently large electric field intensity, which is the case in Figure 3 and Figure 5), the opposite effect takes place, i.e., the electromagnetic waves with longer wavelength can propagate in the structure more compared to the ones propagating in the Goubau line with no covering. We stress that both these effects disappear as the thickness $r_1-r_0$ of the outer cylindrical shell increases, which can be clearly seen in Figure 3 and Figure 5 as well. Besides this, as mentioned in Section 6, the strong nonlinearity of graphene leads to a larger localization of the electromagnetic field within the waveguide; see Figure 4 and Figure 7.

This effect is caused by the fact that in the strong nonlinear regime waveguide modes have smaller wave numbers determined from dispersion Equation (32) involving a nonlinear term. Despite the above effects, we should say that there is no qualitative difference in the cases with a nonlinear graphene covering and without it.

Some of the theoretical results and approaches we used need to be developed further. In this direction, it is important to find analytical criteria allowing the conditions of existence-guided regimes to be derived. In the best case, these criteria must define guided regimes through basic parameters of the original problem. The possible methods of developing the theoretical results obtained in the paper are discussed at the end of Section 5.

It is interesting to note that this result is in agreement with a similar result for a plain waveguide with graphene covering [32] as well as for a plain waveguide filled with Kerr medium, where the Kerr law is of a special form [49]. Indeed, dispersion curves for these waveguiding structures look quite similar. In [32], the effect of larger localization of a monochromatic TE-polarized electromagnetic wave within a plain waveguide with graphene covering in the strong nonlinear regime is revealed as well. At the same time, in a waveguide (a plain one as well as a circle cylindrical one) filled with a nonlinear medium with the widely used Kerr law, novel guided regimes can be theoretically found [50,51].