Valley-Selective High Harmonic Generation and Polarization Induced by an Orthogonal Two-Color Laser Field
Abstract
:1. Introduction
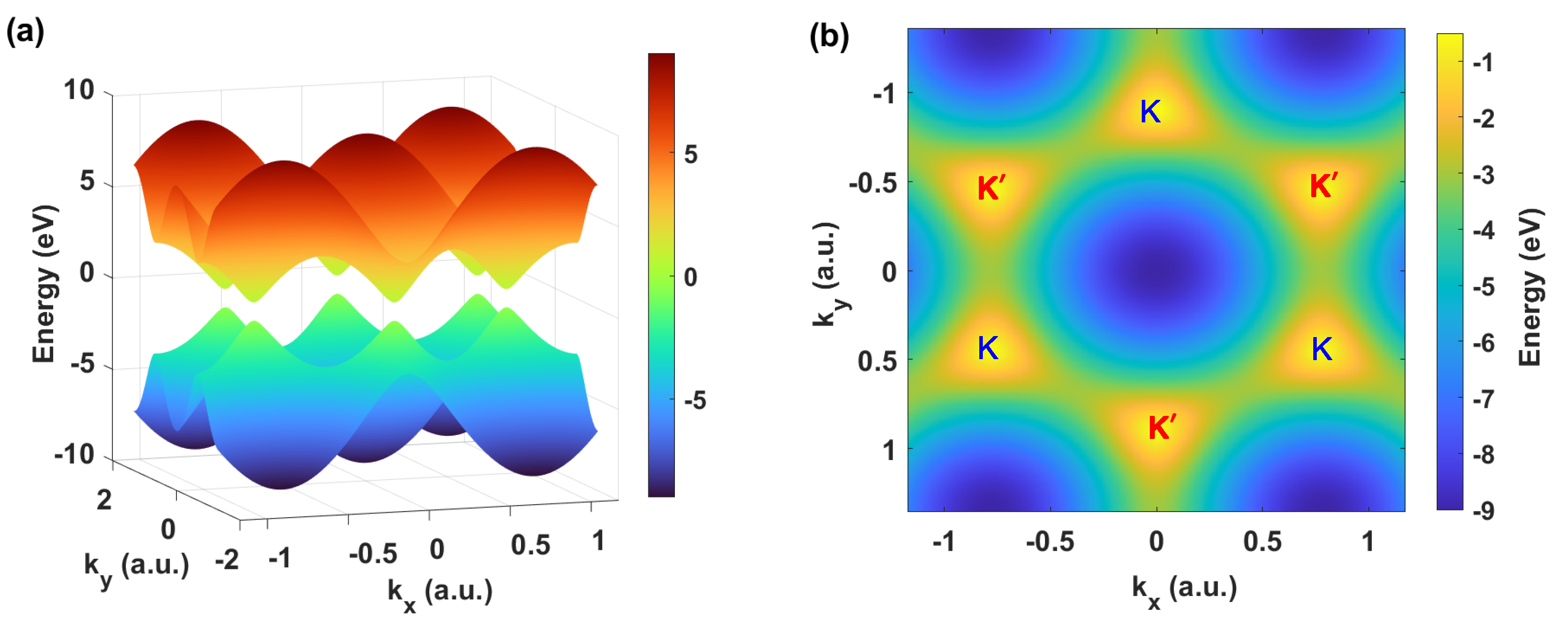
2. Theoretical Models
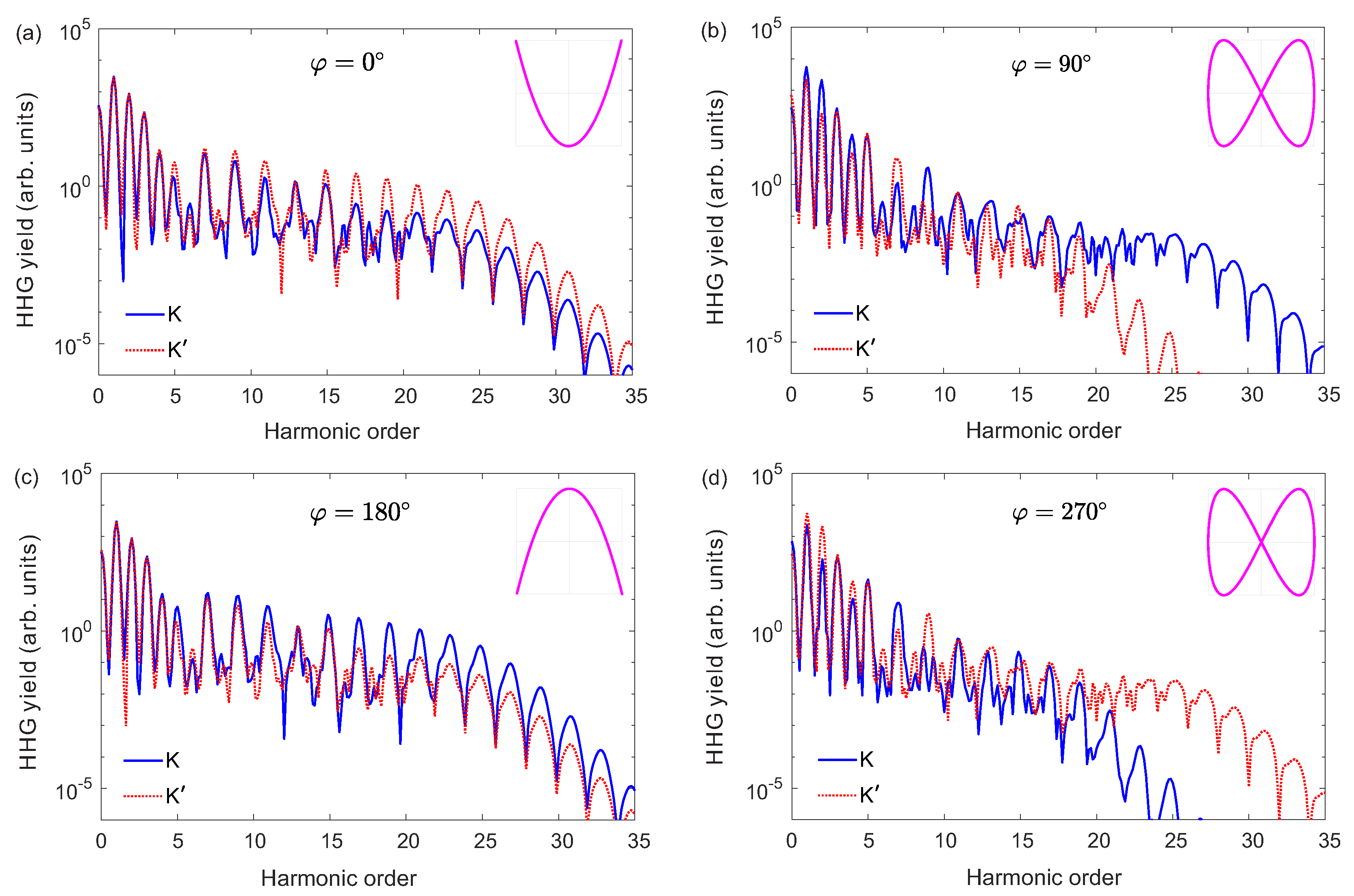
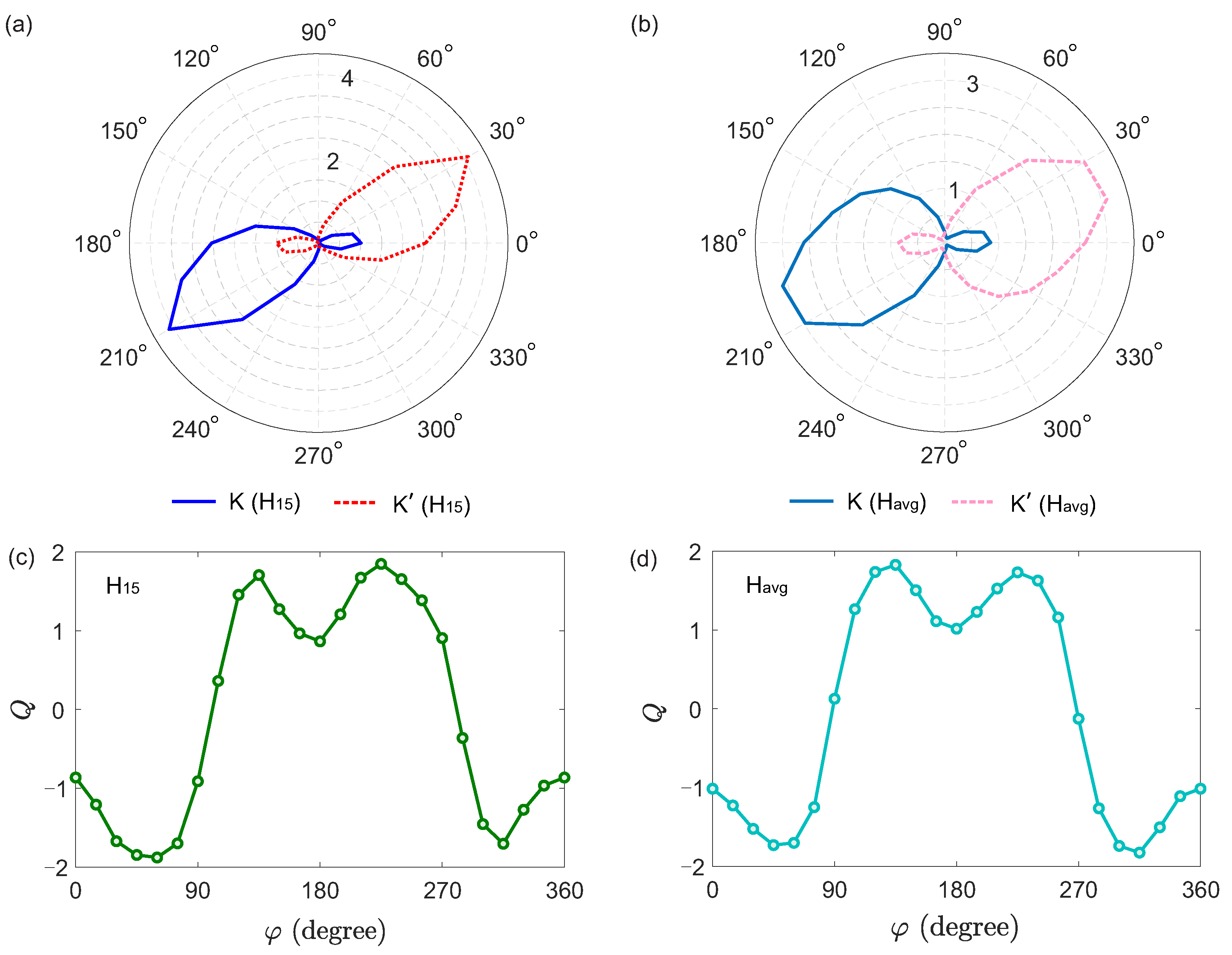
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Krausz, F.; Ivanov, M. Attosecond physics. Rev. Mod. Phys. 2009, 81, 163. [Google Scholar]
- Corkum, P.B.; Krausz, F. Attosecond science. Nat. Phys. 2007, 3, 381–387. [Google Scholar]
- Hentschel, M.; Kienberger, R.; Spielmann, C.; Reider, G.A.; Milosevic, N.; Brabec, T.; Corkum, P.; Heinzmann, U.; Drescher, M.; Krausz, F. Attosecond metrology. Nature 2001, 414, 509–513. [Google Scholar] [PubMed]
- Zhu, X.; Lu, P.; Lein, M. Control of the Geometric Phase and Nonequivalence between Geometric–Phase Definitions in the Adiabatic Limit. Phys. Rev. Lett. 2022, 128, 030401. [Google Scholar] [PubMed]
- Schafer, K.J.; Yang, B.; DiMauro, L.F.; Kulander, K.C. Above threshold ionization beyond the high harmonic cutoff. Phys. Rev. Lett. 1993, 70, 1599. [Google Scholar]
- Corkum, P.B. Plasma perspective on strong field multiphoton ionization. Phys. Rev. Lett. 1993, 71, 1994. [Google Scholar] [CrossRef]
- Lewenstein, M.; Balcou, P.; Ivanov, M.Y.; L’Huillier, A.; Corkum, P.B. Theory of high-harmonic generation by low–frequency laser fields. Phys. Rev. A 1994, 49, 2117. [Google Scholar] [PubMed]
- He, L.; Lan, P.; Le, A.; Wang, B.; Wang, B.; Zhu, X.; Lu, P.; Lin, C.D. Real–Time Observation of Molecular Spinning with Angular High–Harmonic Spectroscopy. Phys. Rev. Lett. 2018, 121, 163201. [Google Scholar] [PubMed]
- Ganeev, R.A. High–Order Harmonics Generation in Selenium–Containing Plasmas. Photonics 2023, 10, 854. [Google Scholar]
- Liu, X.; Zhu, X.; Li, L.; Li, Y.; Zhang, Q.; Lan, P.; Lu, P. Selection rules of high–order–harmonic generation: Symmetries of molecules and laser fields. Phys. Rev. A 2016, 94, 033410. [Google Scholar]
- Paul, P.M.; Toma, E.S.; Breger, P.; Mullot, G.; Augé, F.; Balcou, P.; Muller, H.G.; Agostini, P. Observation of a Train of Attosecond Pulses from High Harmonic Generation. Science 2001, 292, 1689–1692. [Google Scholar]
- Chatziathanasiou, S.; Kahaly, S.; Skantzakis, E.; Sansone, G.; Lopez-Martens, R.; Haessler, S.; Varju, K.; Tsakiris, G.D.; Charalambidis, D.; Tzallas, P. Generation of Attosecond Light Pulses from Gas and Solid State Media. Photonics 2017, 4, 26. [Google Scholar]
- Zhai, C.; Zhu, X.; Long, J.; Shao, R.; Zhang, Y.; He, L.; Tang, Q.; Li, Y.; Lan, P.; Yu, B.; et al. Generation of elliptically polarized attosecond pulses in mixed gases. Phys. Rev. A 2021, 103, 033114. [Google Scholar]
- Ghimire, S.; DiChiara, A.D.; Sistrunk, E.; Agostini, P.; DiMauro, L.F.; Reis, D.A. Observation of high–order harmonic generation in a bulk crystal. Nat. Phys. 2011, 7, 138–141. [Google Scholar]
- Kruchinin, S.Y.; Krausz, F.; Yakovlev, V.S. Colloquium: Strong–field phenomena in periodic systems. Rev. Mod. Phys. 2018, 90, 021002. [Google Scholar]
- Han, S. High–Harmonic Generation Using a Single Dielectric Nanostructure. Photonics 2022, 9, 427. [Google Scholar]
- Vampa, G.; Hammond, T.J.; Thiré, N.; Schmidt, B.E.; Légaré, F.; McDonald, C.R.; Brabec, T.; Corkum, P.B. Linking high harmonics from gases and solids. Nature 2015, 522, 462–464. [Google Scholar]
- Wu, M.; Browne, D.A.; Schafer, K.J.; Gaarde, M.B. Multilevel perspective on high-order harmonic generation in solids. Phys. Rev. A 2016, 94, 063403. [Google Scholar]
- Liu, X.; Zhu, X.; Lan, P.; Zhang, X.; Wang, D.; Zhang, Q.; Lu, P. Time–dependent population imaging for high–order–harmonic generation in solids. Phys. Rev. A 2017, 95, 063419. [Google Scholar]
- Fu, S.; Feng, Y.; Li, J.; Yue, S.; Zhang, X.; Hu, B.; Du, H. Recollision dynamics analysis of high–order harmonic generation in solids. Phys. Rev. A 2020, 101, 023402. [Google Scholar]
- Lanin, A.A.; Stepanov, E.A.; Fedotov, A.B.; Zheltikov, A.M. Mapping the electron band structure by intraband high–harmonic generation in solids. Optica 2017, 4, 516–519. [Google Scholar]
- Li, L.; Lan, P.; He, L.; Cao, W.; Zhang, Q.; Lu, P. Determination of Electron Band Structure using Temporal Interferometry. Phys. Rev. Lett. 2020, 124, 157403. [Google Scholar]
- Bauer, D.; Hansen, K.K. High–Harmonic Generation in Solids with and without Topological Edge States. Phys. Rev. Lett. 2018, 120, 177401. [Google Scholar] [PubMed]
- Heide, C.; Kobayashi, Y.; Baykusheva, D.R.; Jain, D.; Sobota, J.A.; Hashimoto, M.; Kirchmann, P.S.; Oh, S.; Heinz, T.F.; Reis, D.A.; et al. Probing topological phase transitions using high-harmonic generation. Nat. Photon. 2022, 16, 620–624. [Google Scholar]
- Schmid, C.P.; Weigl, L.; Grössing, P.; Junk, V.; Gorini, C.; Schlauderer, S.; Ito, S.; Meierhofer, M.; Hofmann, N.; Afanasiev, D.; et al. Tunable non-integer high-harmonic generation in a topological insulator. Nature 2021, 593, 385–390. [Google Scholar] [PubMed]
- Yue, L.; Hollinger, R.; Uzundal, C.B.; Nebgen, B.; Gan, Z.; Najafidehaghani, E.; George, A.; Spielmann, C.; Kartashov, D.; Turchanin, A.; et al. Signatures of Multiband Effects in High–Harmonic Generation in Monolayer MoS2. Phys. Rev. Lett. 2022, 129, 147401. [Google Scholar]
- Tamaya, T.; Akiyama, H.; Kato, T. Shear-strain controlled high-harmonic generation in graphene. Phys. Rev. B 2023, 107, L081405. [Google Scholar]
- Rana, N.; Mrudul, M.S.; Kartashov, D.; Ivanov, M.; Dixit, G. High-harmonic spectroscopy of coherent lattice dynamics in graphene. Phys. Rev. B 2022, 106, 064303. [Google Scholar]
- Yoshikawa, N.; Tamaya, T.; Tanaka, K. High-harmonic generation in graphene enhanced by elliptically polarized light excitation. Science 2017, 356, 736–738. [Google Scholar] [PubMed]
- Heide, C.; Kobayashi, Y.; Johnson, A.C.; Liu, F.; Heinz, T.F.; Reis, D.A.; Ghimire, S. Probing electron–hole coherence in strongly driven 2D materials using high–harmonic generation. Optica 2022, 9, 512–516. [Google Scholar]
- Cha, S.; Kim, M.; Kim, Y.; Choi, S.; Kang, S.; Kim, H.; Yoon, S.; Moon, G.; Kim, T.; Lee, Y.W.; et al. Gate–tunable quantum pathways of high harmonic generation in graphene. Nat. Commun. 2022, 13, 6630. [Google Scholar] [PubMed]
- Zheng, W.; Jiang, Y.; Wang, S.; Liu, C.; Bai, Y.; Liu, P.; Li, R. Frequency shift of even–order high harmonic generation in monolayer MoS2. Opt. Express 2023, 31, 27029–27040. [Google Scholar]
- Castro Neto, A.H.; Guinea, F.; Peres, N.M.R.; Novoselov, K.S.; Geim, A.K. The electronic properties of graphene. Rev. Mod. Phys. 2009, 81, 109. [Google Scholar]
- Geim, A.K. Graphene: Status and Prospects. Science 2009, 324, 1530–1534. [Google Scholar]
- Schaibley, J.R.; Yu, H.; Clark, G.; Rivera, P.; Ross, J.S.; Seyler, K.L.; Yao, W.; Xu, X. Valleytronics in 2D materials. Nat. Rev. Mater. 2016, 1, 16055. [Google Scholar]
- Vitale, S.A.; Nezich, D.; Varghese, J.O.; Kim, P.; Gedik, N.; Jarillo–Herrero, P.; Xiao, D.; Rothschild, M. Valleytronics: Opportunities, Challenges, and Paths Forward. Small 2018, 14, 1801483. [Google Scholar]
- Langer, F.; Schmid, C.P.; Schlauderer, S.; Gmitra, M.; Fabian, J.; Nagler, P.; Schüller, C.; Korn, T.; Hawkins, P.G.; Steiner, J.T.; et al. Lightwave valleytronics in a monolayer of tungsten diselenide. Nature 2018, 557, 76–80. [Google Scholar]
- Xiao, D.; Yao, W.; Niu, Q. Valley–Contrasting Physics in Graphene: Magnetic Moment and Topological Transport. Phys. Rev. Lett. 2007, 99, 236809. [Google Scholar]
- Mak, K.F.; Xiao, D.; Shan, J. Light–valley interactions in 2D semiconductors. Nat. Photon. 2018, 12, 451–460. [Google Scholar]
- Jiménez–Galán, Á.; Silva, R.E.F.; Smirnova, O.; Ivanov, M. Lightwave control of topological properties in 2D materials for sub–cycle and non-resonant valley manipulation. Nat. Photon. 2020, 14, 728–732. [Google Scholar]
- Jiménez–Galán, Á.; Silva, R.E.F.; Smirnova, O.; Ivanov, M. Sub-cycle valleytronics: Control of valley polarization using few-cycle linearly polarized pulses. Optica 2021, 8, 277–280. [Google Scholar]
- Sharma, S.; Elliott, P.; Shallcross, S. Valley control by linearly polarized laser pulses: Example of WSe2. Optica 2022, 9, 947–952. [Google Scholar]
- Golub, L.; Tarasenko, S. Valley polarization induced second harmonic generation in graphene. Phys. Rev. B 2014, 90, 201402. [Google Scholar] [CrossRef]
- Rana, N.; Dixit, G. All–Optical Ultrafast Valley Switching in Two-Dimensional Materials. Phys. Rev. Appl. 2023, 19, 034056. [Google Scholar]
- Mak, K.F.; McGill, K.L.; Park, J.; McEuen, P.L. The valley Hall effect in MoS2 transistors. Science 2014, 344, 1489–1492. [Google Scholar] [CrossRef]
- Mrudul, M.S.; Jiménez–Galán, Á.; Ivanov, M.; Dixit, G. Light–induced valleytronics in pristine graphene. Optica 2021, 8, 422–427. [Google Scholar]
- He, Y.; Guo, J.; Gao, F.; Liu, X. Dynamical symmetry and valley–selective circularly polarized high–harmonic generation in monolayer molybdenum disulfide. Phys. Rev. B 2022, 105, 024305. [Google Scholar]
- Chen, J.; Liu, C.; Li, R. Valley-Selective Polarization in Twisted Bilayer Graphene Controlled by a Counter-Rotating Bicircular Laser Field. Photonics 2023, 10, 516. [Google Scholar]
- Mrudul, M.S.; Dixit, G. Controlling valley–polarisation in graphene via tailored light pulses. J. Phys. B At. Mol. Opt. Phys. 2021, 54, 224001. [Google Scholar]
- Reich, S.; Maultzsch, J.; Thomsen, C.; Ordejon, P. Tight–binding description of graphene. Phys. Rev. B 2002, 66, 035412. [Google Scholar]
- Jiang, S.; Wei, H.; Chen, J.; Yu, C.; Lu, R.; Lin, C.D. Effect of transition dipole phase on high–order–harmonic generation in solid materials. Phys. Rev. A 2017, 96, 053850. [Google Scholar]
- Dimitrovski, D.; Madsen, L.B.; Pedersen, T.G. High–order harmonic generation from gapped graphene: Perturbative response and transition to nonperturbative regime. Phys. Rev. B 2017, 95, 035405. [Google Scholar]
- Avetissian, H.K.; Avetissian, A.K.; Avchyan, B.R.; Mkrtchian, G.F. Wave mixing and high harmonic generation at two–color multiphoton excitation in two–dimensional hexagonal nanostructures. Phys. Rev. B 2019, 100, 035434. [Google Scholar]
- Avetissian, H.K.; Mkrtchian, G.F.; Knorr, A. Efficient high–harmonic generation in graphene with two–color laser field at orthogonal polarization. Phys. Rev. B 2022, 105, 195405. [Google Scholar]
- Vampa, G.; McDonald, C.R.; Orlando, G.; Klug, D.D.; Corkum, P.B.; Brabec, T. Theoretical Analysis of High-Harmonic Generation in Solids. Phys. Rev. Lett. 2014, 113, 073901. [Google Scholar]
- Liu, X.; Li, L.; Zhu, X.; Huang, T.; Zhang, X.; Wang, D.; Lan, P.; Lu, P. Wavelength dependence of high–order harmonic yields in solids. Phys. Rev. A 2018, 98, 063419. [Google Scholar]
- Zhang, Y.; Li, J.; Li, L.; Huang, T.; Zhu, X.; Lan, P.; Lu, P. Enhancement of the photocurrents injected in gapped graphene by the orthogonally polarized two–color laser field. Opt. Express 2021, 29, 17387–17397. [Google Scholar] [PubMed]
- Vampa, G.; McDonald, C.R.; Orlando, G.; Corkum, P.B.; Brabec, T. Semiclassical analysis of high harmonic generation in bulk crystals. Phys. Rev. B 2015, 91, 064302. [Google Scholar] [CrossRef]
- Guan, Z.; Zhou, X.; Bian, X. High–order–harmonic generation from periodic potentials driven by few-cycle laser pulses. Phys. Rev. A 2016, 93, 033852. [Google Scholar] [CrossRef]
- Wang, H.; Feng, Y.; Fu, S.; Li, J.; Zhang, X.; Du, H. Complex carrier-envelope-phase effect of solid harmonics under nonadiabatic conditions. Phys. Rev. A 2019, 99, 023406. [Google Scholar]
- Liu, X.; Li, Y.; Liu, D.; Zhu, X.; Zhang, X.; Lu, P. Effects of quantum interferences among crystal–momentum–resolved electrons in solid high–order harmonic generation. Phys. Rev. A 2021, 103, 033104. [Google Scholar]
- Zhou, S.Y.; Gweon, G.H.; Fedorov, A.V.; First, P.N.; de Heer, W.A.; Lee, D.H.; Guinea, F.; Castro Neto, A.H.; Lanzara, A. Substrate–induced bandgap opening in epitaxial graphene. Nat. Mater. 2007, 6, 770–775. [Google Scholar]
- Yankowitz, M.; Xue, J.; Cormode, D.; Sanchez-Yamagishi, J.D.; Watanabe, K.; Taniguchi, T.; Jarillo-Herrero, P.; Jacquod, P.; LeRoy, B.J. Emergence of superlattice Dirac points in graphene on hexagonal boron nitride. Nat. Phys. 2012, 8, 382–386. [Google Scholar]
- Grujić, M.M.; Tadić, M.Ž.; Peeters, F.M. Spin-Valley Filtering in Strained Graphene Structures with Artificially Induced Carrier Mass and Spin-Orbit Coupling. Phys. Rev. Lett. 2014, 113, 046601. [Google Scholar] [PubMed]
- Brugnera, L.; Frank, F.; Hoffmann, D.J.; Torres, R.; Siegel, T.; Underwood, J.G.; Springate, E.; Froud, C.; Turcu, E.I.C.; Tisch, J.W.G.; et al. Enhancement of high harmonics generated by field steering of electrons in a two–color orthogonally polarized laser field. Opt. Lett. 2010, 35, 3994–3996. [Google Scholar] [PubMed]
- Cireasa, R.; Boguslavskiy, A.E.; Pons, B.; Wong, M.C.H.; Descamps, D.; Petit, S.; Ruf, H.; Thiré, N.; Ferré, A.; Suarez, J.; et al. Probing molecular chirality on a sub–femtosecond timescale. Nat. Phys. 2015, 11, 654–658. [Google Scholar]
- Smirnova, O.; Mairesse, Y.; Patchkovskii, S. Opportunities for chiral discrimination using high harmonic generation in tailored laser fields. J. Phys. B At. Mol. Opt. Phys. 2015, 48, 234005. [Google Scholar]
- Zhu, X.; Liu, X.; Lan, P.; Wang, D.; Zhang, Q.; Li, W.; Lu, P. Anomalous circular dichroism in high harmonic generation of stereoisomers with two chiral centers. Opt. Express 2016, 24, 24824–24835. [Google Scholar]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, X.; Liu, D.; Sun, Y.; Li, Y.; Zhang, C. Valley-Selective High Harmonic Generation and Polarization Induced by an Orthogonal Two-Color Laser Field. Photonics 2023, 10, 1126. https://doi.org/10.3390/photonics10101126
Liu X, Liu D, Sun Y, Li Y, Zhang C. Valley-Selective High Harmonic Generation and Polarization Induced by an Orthogonal Two-Color Laser Field. Photonics. 2023; 10(10):1126. https://doi.org/10.3390/photonics10101126
Chicago/Turabian StyleLiu, Xi, Dongdong Liu, Yan Sun, Yujie Li, and Cui Zhang. 2023. "Valley-Selective High Harmonic Generation and Polarization Induced by an Orthogonal Two-Color Laser Field" Photonics 10, no. 10: 1126. https://doi.org/10.3390/photonics10101126
APA StyleLiu, X., Liu, D., Sun, Y., Li, Y., & Zhang, C. (2023). Valley-Selective High Harmonic Generation and Polarization Induced by an Orthogonal Two-Color Laser Field. Photonics, 10(10), 1126. https://doi.org/10.3390/photonics10101126




