1. Introduction
In response to the growing demand for information transmission, optical fiber communication based on dense wavelength division multiplexing (WDM) technology has emerged to achieve higher network resource utilization. The core of next-generation communication networks is comprised of multi-dimensional optical cross-connects (OXCs) and reconfigurable optical add-drop multiplexers (ROADMs). The optical wavelength selective switches (WSSs), as the central device of ROADM, make it possible to match any input wavelength to any output port for the suggested input ports. The information bandwidth per channel in a WDM system is typically 50 to 100 GHz [
1], and wavelength demultiplexing or multiplexing is accomplished using a diffraction grating. One of the WSS design trends is moving towards building M × N WSS system based on 1 × N WSS and additional parts like couplers and optical switches [
2,
3,
4]. Since convergence of these discrete components results in systems becoming more complicated and unstable [
1,
5], the research on new type of the 1 × N WSS with higher compactness and lower loss plays a significant role to develop more complex and effective M × N WSS systems.
Commercially, micro-electromechanical systems (MEMS) and liquid crystal on silicon (LCoS) spatial light modulator (SLM) switches are two primary competing technologies used in 1 × N WSS systems [
6,
7,
8]. As early as in 2002, American researchers at Bell Laboratories combined multiplexer, demultiplexer, optical attenuator, and other optical components to create WSS based on planar lightwave circuit(PLC) [
9]. The system can output from 9 ports and has 1 input port, with an insertion loss of less than 7 dB. In 2004, Metconnex from Canada combined PLC and MEMS technology to develop WSS based on PLC and MEMS [
10]. In 2008, Finisar, the world’s largest manufacturer of optical communication devices, announced that they had developed the first LCoS-based WSS with a frequency interval of 50 GHz [
11]. The isolation degree of adjacent ports is greater than 25 dB, the insertion loss of polarized light is 8 dB, and the flexible configuration of ports is also supported. Nowadays, as a programmable diffractive optical element, LCoS SLM allows WSS systems to handle high-port-count networks and flex grid technology-enabled networks with good performance due to its high flexibility and structural stability [
12]. Considering that the unpolarized light transmitted in fibers is not applicable to LCoS, polarization splitter elements are usually introduced into the WSS system to achieve polarization splitting, modulation and polarization combination. They linearly polarize an input beam in the direction of the liquid-crystal director, modulating its phase in response [
13]. In this way, parallel-aligned LCoS devices are more widely employed to display phase-only patterns. Specifically, the reflective LCoS has many advantages such as integration of high performance driving circuitry on a silicon chip, high pixel fill factor and high quality process technology for excellent pixel mirror reflectivity [
14]. In a 1 × N WSS system, the input and output ports are often in the same array, corresponding to the same regions of LCoS, which increases the difficulty of optical path layout to a certain extent. Therefore, it is crucial for the design to consider how to eliminate the occlusion of the structure, which is why the off-axis structure is employed.
However, there was very few research of the 1 × N WSS system design from the standpoint of optical paths in earlier works [
15,
16]. Instead, the majority of the attention is placed on developing and testing them to obtain the desired performance, and only then the system structure altered to provide the best possible transmission performance, including insert loss and crosstalk [
17]. Skipping the design stages of the system creation is often blind, expensive, and inefficient.
Therefore, in this paper, a conceptual design method of a new type of LCoS-based WSS from the perspective of pure optical path simulation is proposed. This paper innovatively introduces a practical simulation method for WSS optical path design, providing detailed optical design principles for both the wavelength direction and LCoS deflection direction (which will be explained in detail later). Unlike previous polarization-related optical paths, a unique polarization-independent structure is ingeniously designed for the LCoS beam polarization requirement, simplifying the optical path by eliminating the need to polarize the light in the beginning. The entire system uses a minimal number of components, minimizing manufacturing costs and equipment weight, which is favorable for large-scale implementation. Through the detailed design description of the optical path simulation part and the error analysis of the designed optical system, the perfect performance with high pot-to-port coupling efficiency of the simulated system can be seen clearly.
The full paper frame is as follows. The design specifications for the WSS optical system are given in
Section 2, along with a detailed explanation of the design concept of the 2-f and 4-f systems described. In addition, the parameters of the system and optical components are also analyzed. Then, in
Section 3, we provide the design methods and detailed analysis of three key devices and give the calculation method of the crucial parameters of system performance evaluation. We also demonstrate the feasibility of employing ideal planar mirrors as substitutes for diffraction elements in the simulation of LCoS in this study. The design process and final performance of the 1 × 10 WSS optical system design with optical design software are shown at length in
Section 4. In
Section 5, a tolerance analysis of all configurations is displayed and the final section includes a summary of the WSS system design and a discussion on the unresolved issues that need further investigation.
2. Designing Principle and System Components
2.1. 4-f and 2-f Optical System
The specifications of the WSS system in this paper are listed in
Table 1. The input light source is Gaussian light with determined numerical aperture(NA) and the waist radius.
According to the following loss calculation formula as Equation (
1), the simulation coupling efficiency is more than 90%, which corresponds to a simulation coupling loss of at most −0.46 dB. It should be noted that the design and simulation presented in this paper are based on ideal diffraction elements and idealized analysis from an optical path perspective. Therefore, the coupling efficiency requirements are extremely strict, aiming to achieve a level of 90% or higher. In practical experiments, factors such as insertion loss, crosstalk, and diffraction efficiency cannot be replicated exactly as in the ideal design. This is precisely why such a high coupling efficiency is necessary, to allow for sufficient margin in the face of these unavoidable losses.
In simple terms, WSS is the WDM signal input to the fiber port, which is divided by a grating and subsequently focused on the focal plane of the lens, where LCoS is located. The wavelength direction (y-axis) and the switching direction (x-axis) describe two dimensions of the WSS system, and these two dimensions are based on different design strategy. The wavelength direction refers to the direction in which the light beam is spread and the switching direction refers to the arrangement direction of input/output single-mode fibers. These two directions are perpendicular to each other in the three-dimensional space of the system.
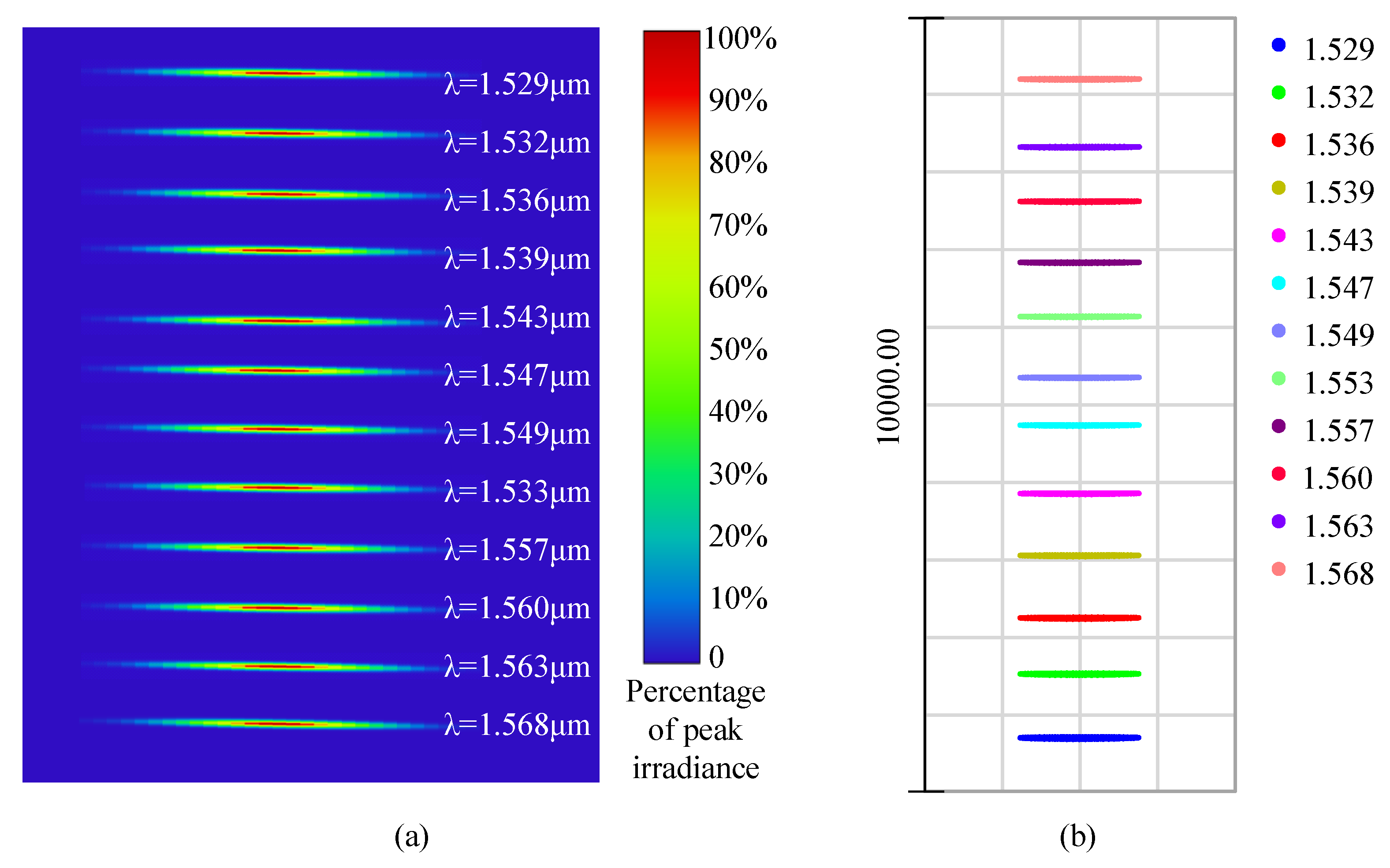
According to the distribution of optical power shown in
Figure 1a, the optical system in the wavelength direction can be regarded as a 4-f optical system where exist two lenses with the same optical power of
. The input Gaussian beam is firstly collimated by microlens array which is represented by Lens 1 in
Figure 1a, and the grating is located at the co-focal point of Lens 1 and Lens 2 as a dispersion elements. Then the light beam of different wavelengths is focused by Lens 2.
Similarly, the switching direction is a 2-f optical system as demonstrated in
Figure 1b. The input light beam is collimated by lens 3 with a focal length of
.
In this way, we can place the LCoS at the image plane, which is located at the exit pupil plane in the wavelength direction, and also is the focal plane in the switching direction. By this means, the channel conversion of the beam in the switching direction can be achieved without changing the conjugate position in the wavelength direction. As the length of the system is equal in both directions, the optical power of the element in the wavelength direction should be half of that in the switching direction, namely = 1/2.
2.2. System Parameter Analysis
The optical fiber we used in the simulation is the common single-mode fiber with a diameter of 250 m, and the ports are densely packed. So the fiber pitch is also 250 m. The beam waist is located at the exit end of the fiber.
According to the transmission properties of the Gaussian beam, the relationship between Gaussian beam power transmittance and aperture is listed in
Table 2. Therefore, in order to guarantee a high transmission efficiency of the system(greater than 98%), the beam waist radius of the Gaussian beam of each surface should not be greater than 1/3 of the surface aperture.
One of the challenges in this design is to reduce the number of components to make the system as compact as possible. We find that the two elements of the 4-f system, Lens 1 and Lens 2 in
Figure 1a, have the same optical power and the same distance from the grating, so they can be used for simplification. We replace them with a cylindrical mirror, which provides optical power and bends the light path as well.
Corresponding to this change, the grating is also replaced by a reflection one, which reflects the light beam back to the cylindrical mirror. As a result, the reflection system needs to be off-axis to eliminate light obscuration which will introduce greater aberrations and difficulty in assembly and make the system more complicated. Besides, we replace the plane diffraction grating with grating prism (hereinafter referred to as Grim) to maximize the dispersion angle differences of the light with different wavelengths. The detailed design method will be described in
Section 3.
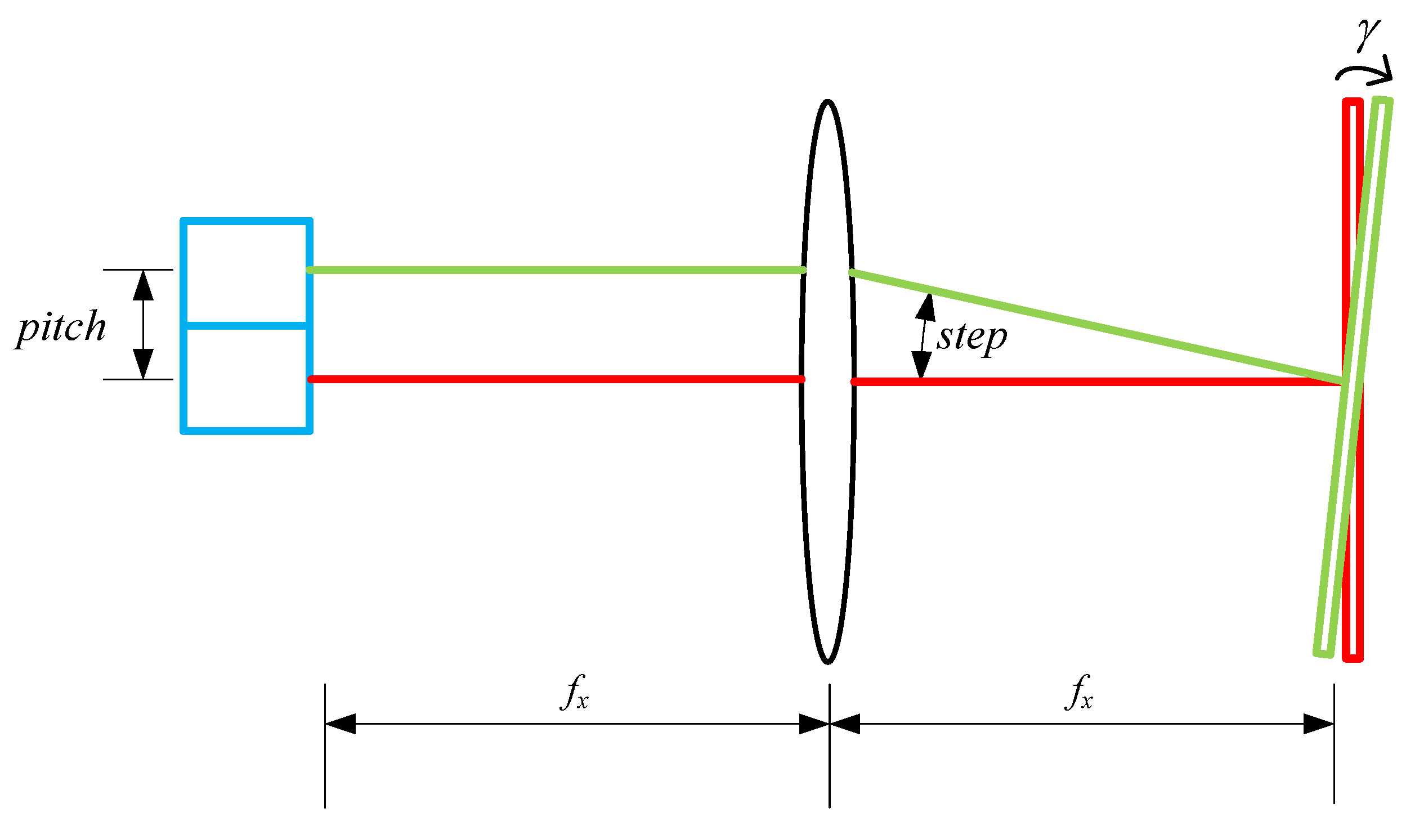
Based on the foregoing discussion, there are 48 wavelength channels in the C-band with a wavelength interval of 100 GHz. The relationship between the LCoS deflection angle and the power of switching direction is shown in
Figure 2,
is the LCoS deflection angle difference between every two adjacent channels, and
refers to the beam reflection angle difference between every two adjacent channels, which satisfies
= 2
. We have the geometric relationship:
where the pitch is the distance between the centers of adjacent fibers, which is equal to the fiber diameter of 250
m.
The maximum number of ports
can be calculated from the step value and the maximum diffraction angle
:
The beam focused on the LCoS through the cylindrical mirror forms a rectangular dispersion pattern in the entire active area of the chip. Phase grating holograms of different periods are uploaded on the pixel areas corresponding to different wavelength channels of the LCoS to complete the direction adjustment of the diffracted beams for the wavelength channels. Since the maximum deflection angle of LCoS cannot be too large, we take the total angle between the input port and the farthest output port within 1.2
[
18,
19]. After calculation we set the focal length of the element in the switching direction to 120 mm, then the corresponding power of the lens in the wavelength direction is 60 mm.
2.3. Components of the WSS System
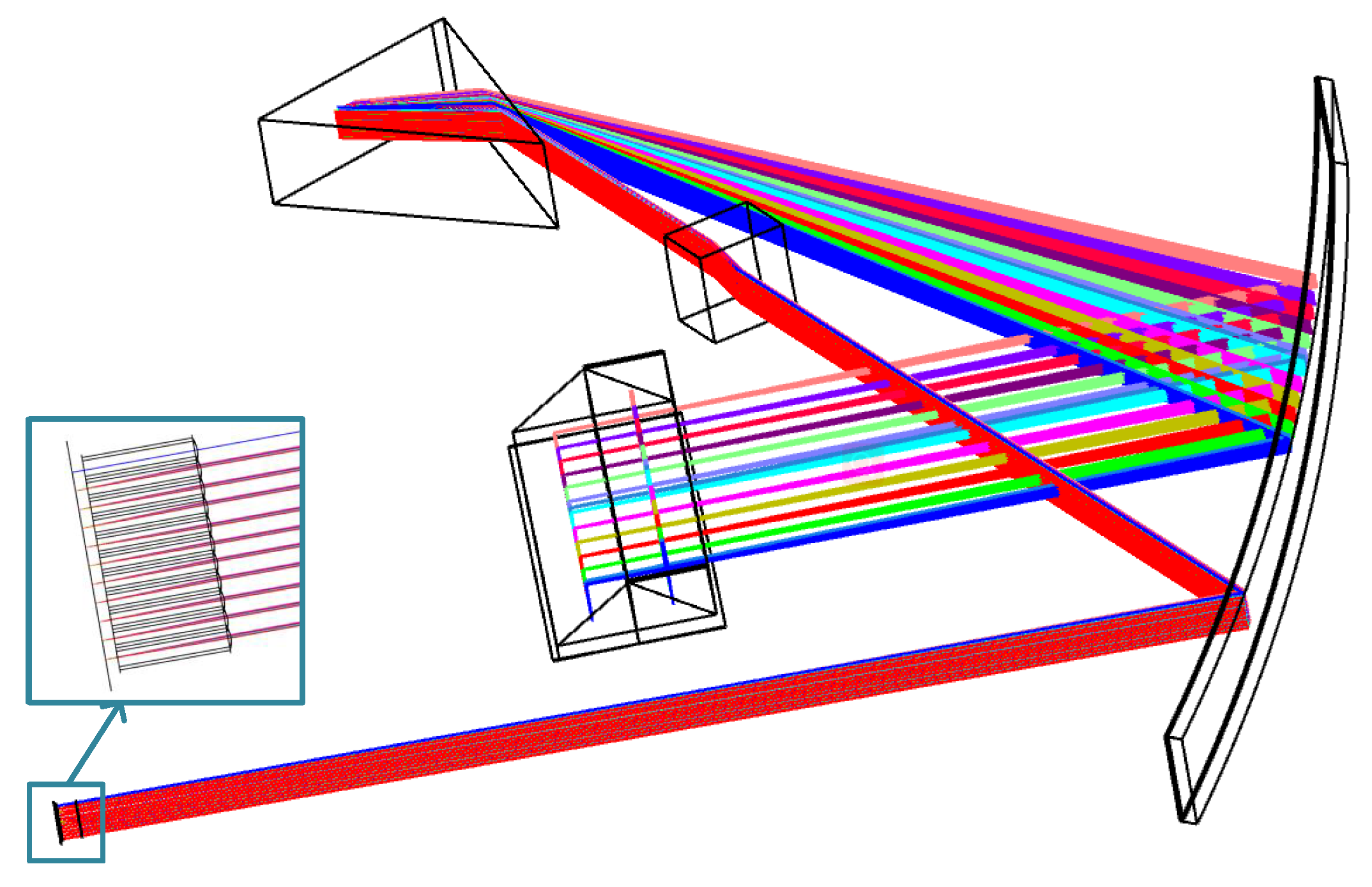
According to the above analysis, all elements except for the microlens array are cylindrical surfaces in the WSS system to control the optical power in two directions independently. Starting from the fiber output position, the components of this system are as shown in
Table 3 and
Figure 3.
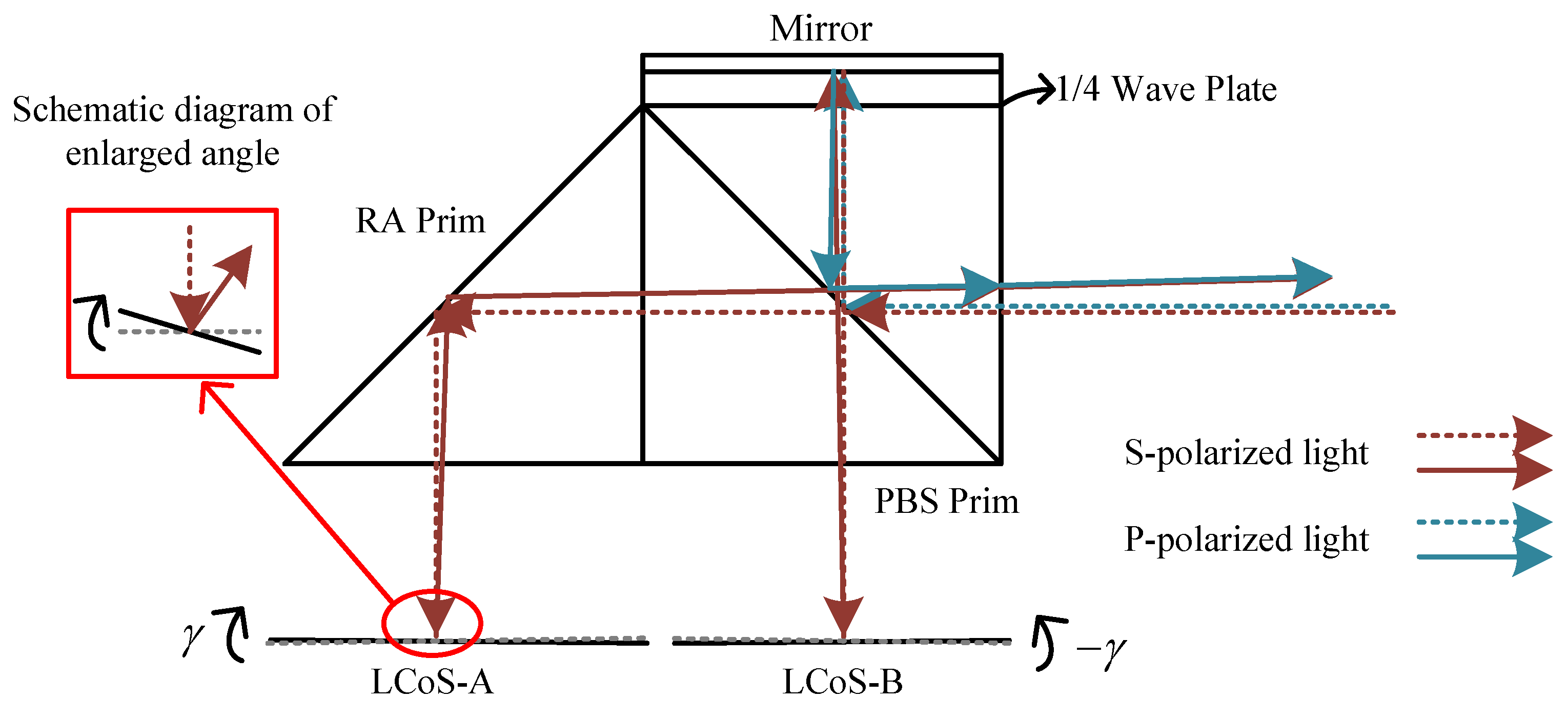
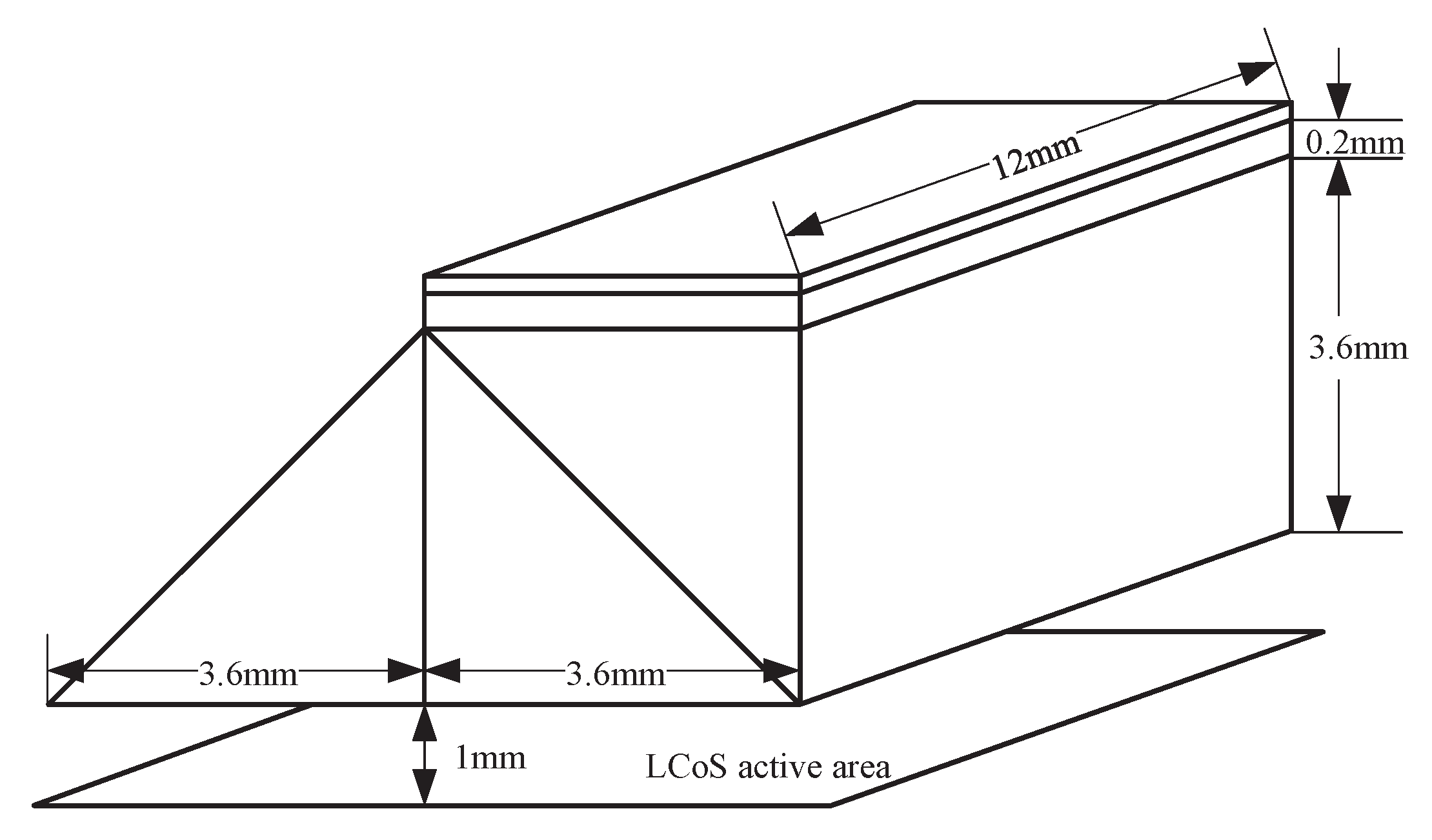
Polarization conversion element are needed due to the polarization-independent operating characteristics of LCoS. Only S-polarized light can be received and modulated by LCoS, so we need to convent P-polarized light into S-polarized light before LCoS. At the same time, it is necessary to control the optical paths in two directions to be consistent, so that S-polarized light and P-polarized light can be recombined into one when the optical path of the system returns. We combine the polarization conversion element and LCoS as Polarization Independent Structure (PIS) and the specific design scheme will be described in the next section.
5. Tolerance Analysis
The conceptual optical design of WSS system in this paper contains fewer components which are easy to obtain as well. However, this design employs an off-axis layout which is more difficult in fabrication and alignment than the ordinary coaxial optical system. Hence a detailed tolerance analysis should be implemented to demonstrated the feasibility of WSS system. The coupling efficiency of the output channels is selected as the performance criterion in tolerance analysis, and LCoS deflection angle is selected as compensator in Monte-Carlo analysis, since LCoS can be modulated by computer.
By comparison the data in
Table 6, we found that the performance of S-polarized light and P-polarized light for the same output channel is basically the same, that is, there is little difference with configurations 11–20 corresponding to configurations 1–10. Therefore, the error analysis for the first ten structures is reasonable to show the tolerance sensitivity of the whole system. In this paper, three representative configurations are selected from 1–10 configurations, namely, Config.1 with no deflection of LCoS, Config.5 with the middle deflection angle and Config.10 with the largest deflection angle. After sensitivity analysis, 500 trails of Monte Carlo analysis were performed to predict the average coupling efficiency of the as-built WSS system. The analysis results shows that the probable performance degradation in coupling efficiency is only 1%. The detailed tolerance allocation is listed in
Table 7 and
Table 8. The overall analysis results are listed in
Table 9 and shown in
Figure 13.
6. Discussion and Conclusions
In this paper, a new type of WSS system design is proposed from the perspective of optical path design. In the design approach, independent optical power distribution is carried out according to the 4-f system in the wavelength direction and the 2-f system in the switching direction. For the key devices, the Grim and polarization conversion element, detailed design schemes are described to meet the system performance requirements. Grim is made of a plane grating and a prim glued together, which can increase the maximum angle of light separation of different wavelengths by more than five times. The polarization conversion element is composed of a PBS prism and an RA prism in order to convert P-polarized light into S-polarized light and then modulated by LCoS. Instead of the traditional need for polarization splitting of the beam at the fiber location, the polarization conversion element innovatively provides a polarization-independent optical path solution for the WSS systems. In the simulation, an ideal model of a plane mirror was used to replace the LCoS, with a maximum switching angle of ±0.722, consistent with the maximum angle limitation found in relevant studies.
The WSS system designed in this paper has excellent performance in coupling efficiency ranging from 95.07 to 98.18%, corresponding to the best loss of −0.036 dB and worst loss of −0.220 dB, and the tolerance analysis demonstrates its good achievability. However, it is important to acknowledge the inherent limitations of the simulations presented in this study. LCoS is modeled as a flat mirror, effectively treated as an ideal diffractive element, while in practical experiments, errors and losses due to diffraction are unavoidable. Within the manuscript, we have addressed the LCoS-related errors, and it should be emphasized that the exceptionally high coupling efficiency observed in our simulations allows for a margin to accommodate losses in practical implementations. The optical path model proposed in this work for LCoS-based WSS design serves as an idealized representation, offering a novel perspective for WSS design. This idea and method can better inspire the integration and combination of different disciplines in optics.