Estimation of Tritium Concentration in the Rain- and Groundwater in the Dry River of Hafr Al Batin, Saudi Arabia
Abstract
:1. Introduction
2. Materials and Methods
2.1. Samples
2.2. Tritium Enrichment
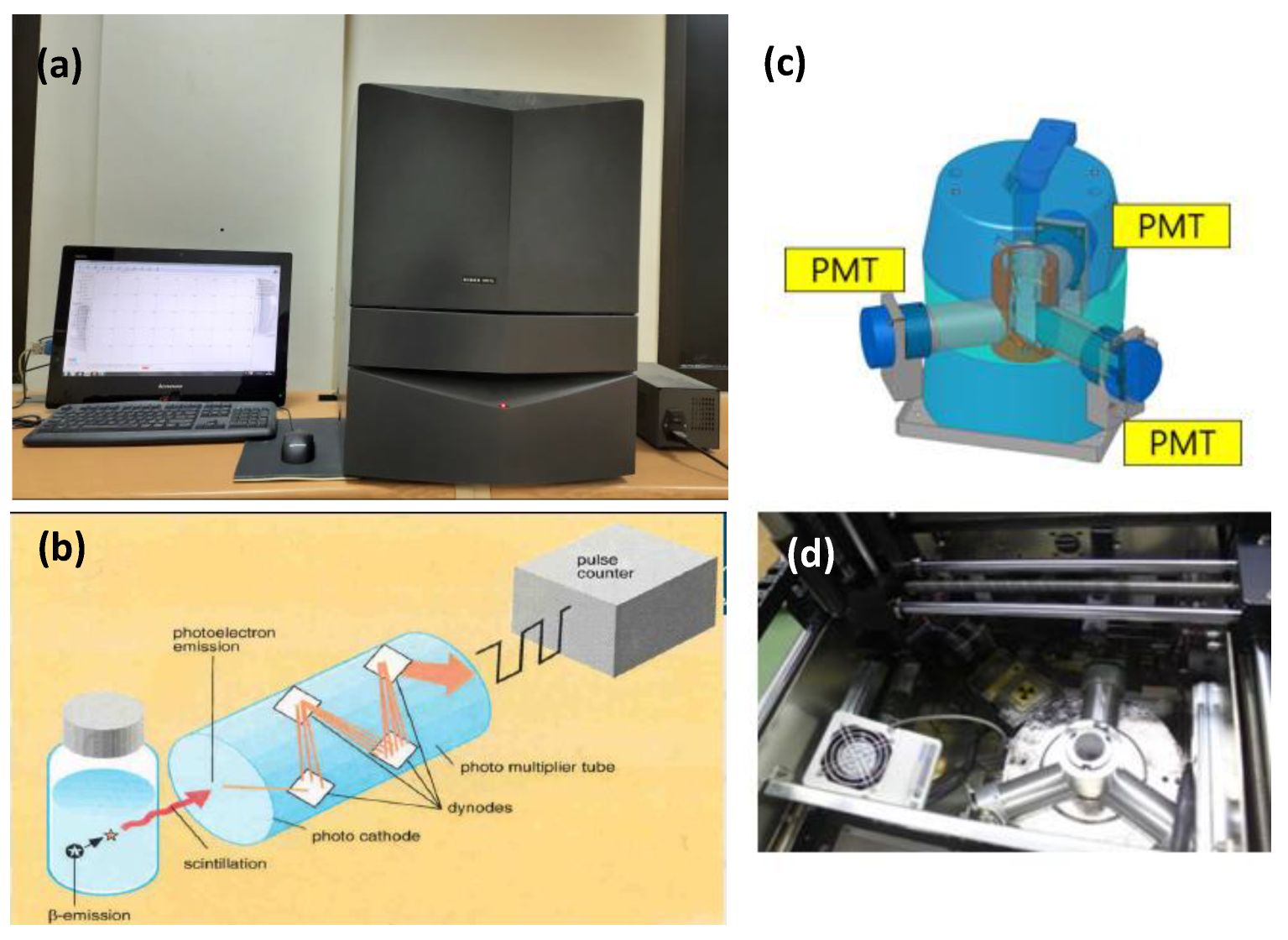
2.3. Tritium Measurement
2.4. Mathematical Formulation
2.4.1. Efficiency of the Scintillation Counter
2.4.2. Tritium Concentration
- c: activity concentration of tritium in Bq m−3;
- fA: correction factor for the decay;
- λ: decay constant of tritium in s−1;
- tA: time between sampling and beginning of the measurement in s;
- Rn: net count rate of the counting source in s−1; with Rn = Rg − Ro
- ε: detection efficiency in Bq−1 s−1;
- ρ: density of the groundwater in kg m−3;
- mn: mass of the solution remaining in the electrolysis cell after electrolysis, in g;
- mV: mass of the solution filled into the electrolysis cell before electrolysis, in g;
- mM: mass of distillate in the scintillation vial, in g;
- ϕ: procedural calibration factor Bq s m−3.
- Rg,j: gross count rate of the single measurement, j, of the counting source, in s−1;
- tm,j: duration of the single measurement, j, in s;
- ttot: sum of the durations of a single measurement in s.
2.4.3. Decision Threshold and Detection Limit
- c*: decision threshold in Bq m−3;
- c#: detection limit in Bq m−3;
- k1−α: quantile of the normal distribution for α = 0.0014;
- k1−β: quantile of the normal distribution for β = 0.05.
2.4.4. Standard Uncertainty
- to: is the sum of the time for a single background measurement, to,j, in s;
- urel(fA): relative standard uncertainty of the correction factor for the decay;
- urel(ηA,i): relative standard uncertainty of the tritium yield of electrolysis cell, i;
- urel(mn): relative standard uncertainty of the mass of solution remaining in the electrolysis cell before electrolysis;
- urel(mv): relative standard uncertainty of the mass of solution filled into the electrolysis cell before electrolysis;
- urel(mM): relative standard uncertainty of the mass of distillate in the scintillation vial;
- urel(ρ): relative standard uncertainty of the density of water;
- urel(ε): relative standard uncertainty of the detection efficiency.
2.4.5. Decay Correction
2.5. Data Validation by Comparing Results from Other Labs
3. Results
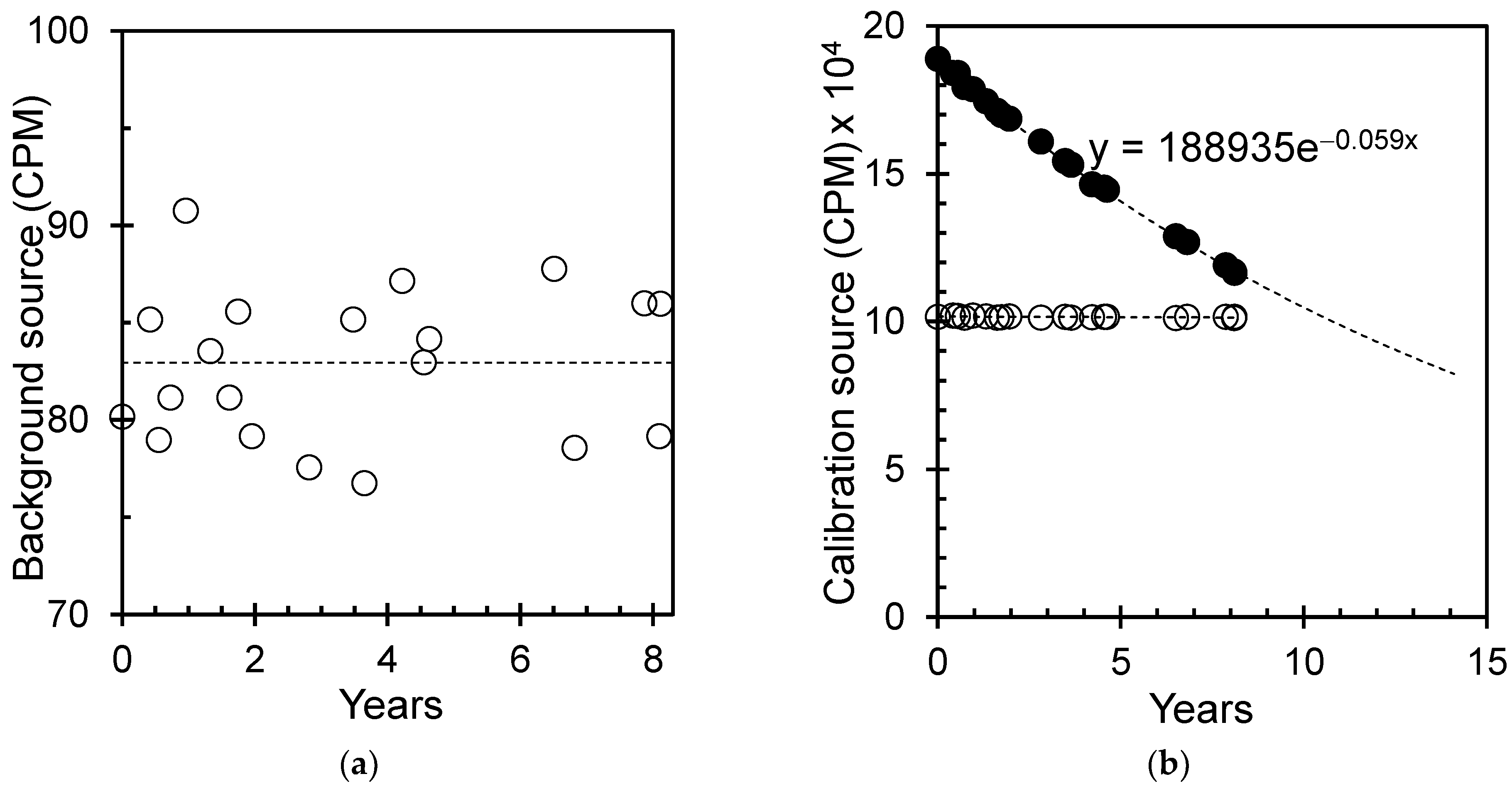
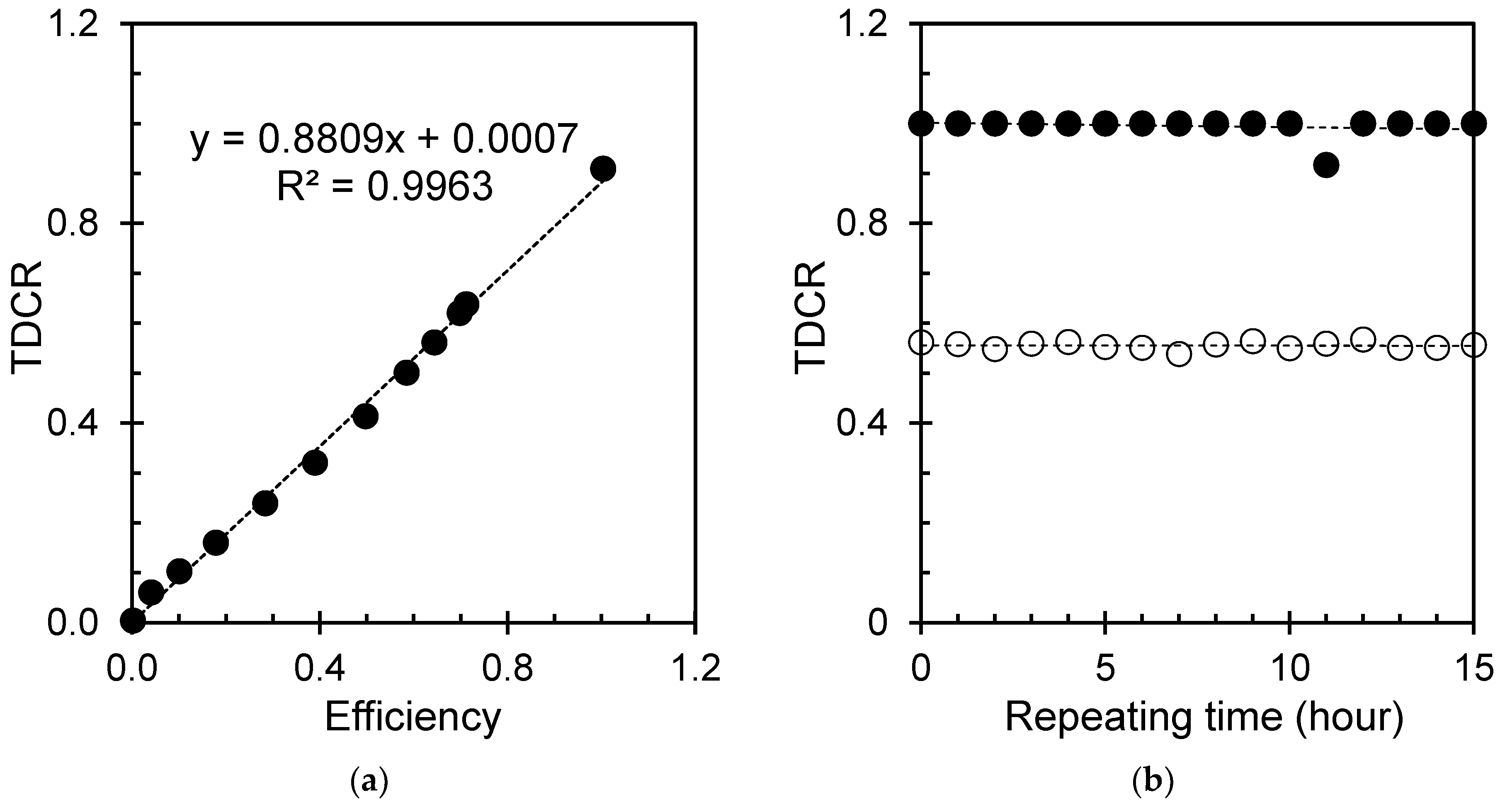
3.1. Efficiency and Background Calibration Using a Standard Sample
3.2. TDCR with Efficiency and Repeating Cycle
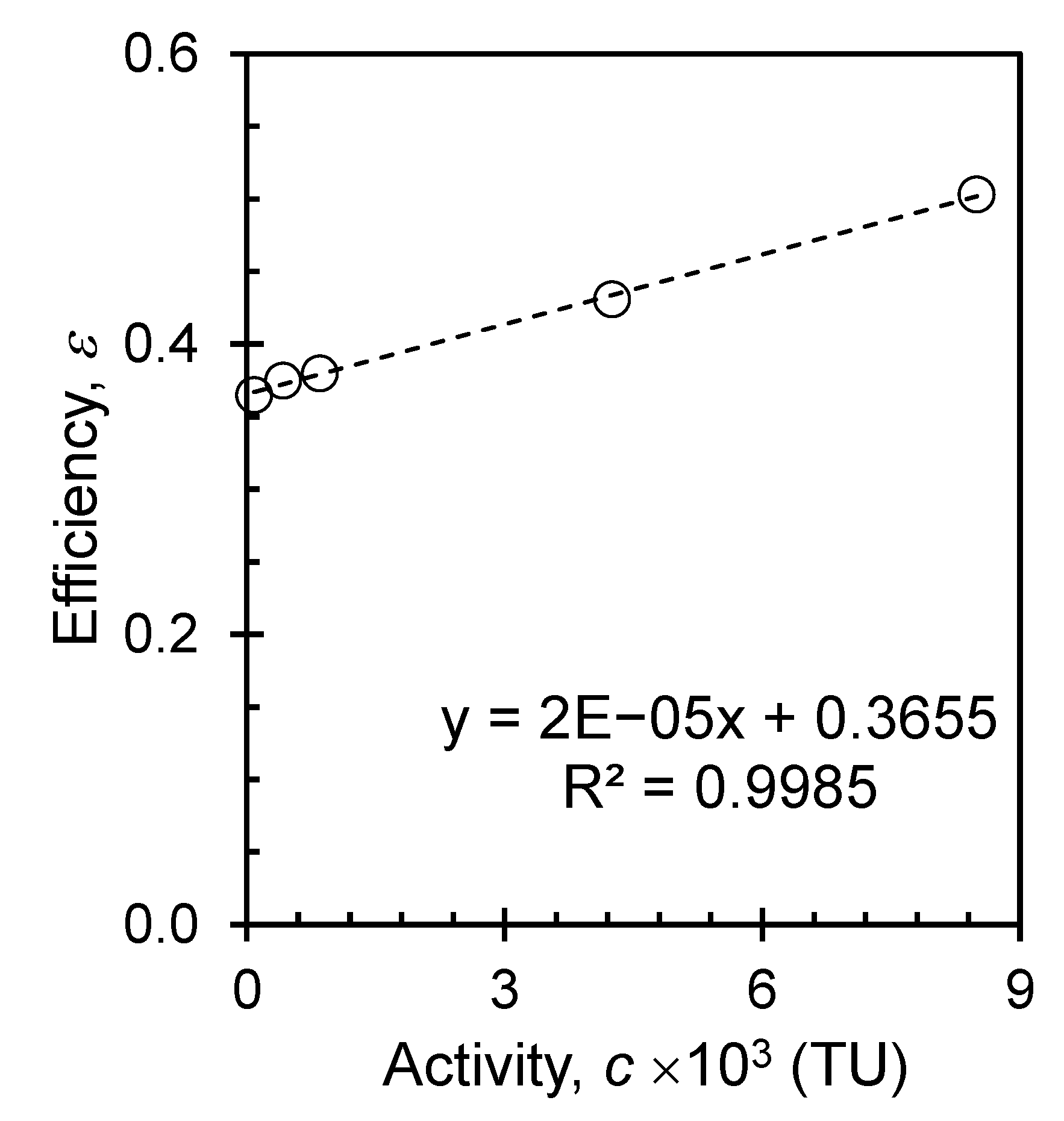
3.3. Activity-Dependent Efficiency
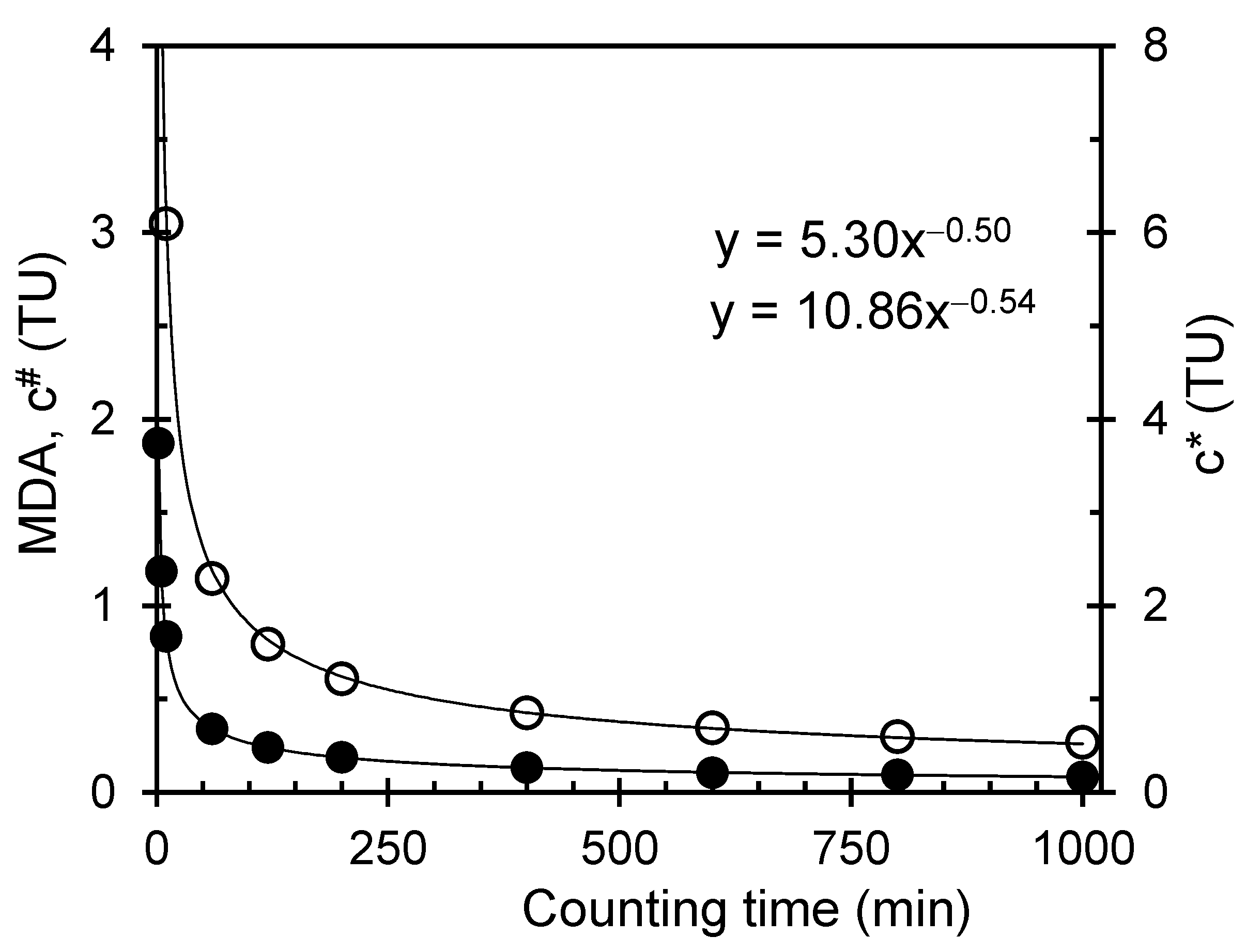
3.4. Counting Time-Dependent Decision Threshold and Detection Limit
3.5. Decay Correction Factor for Sampling Time
3.6. Tritium Activity Concentration
3.6.1. Reference Samples
3.6.2. Rainwater Samples
3.6.3. Groundwater Samples
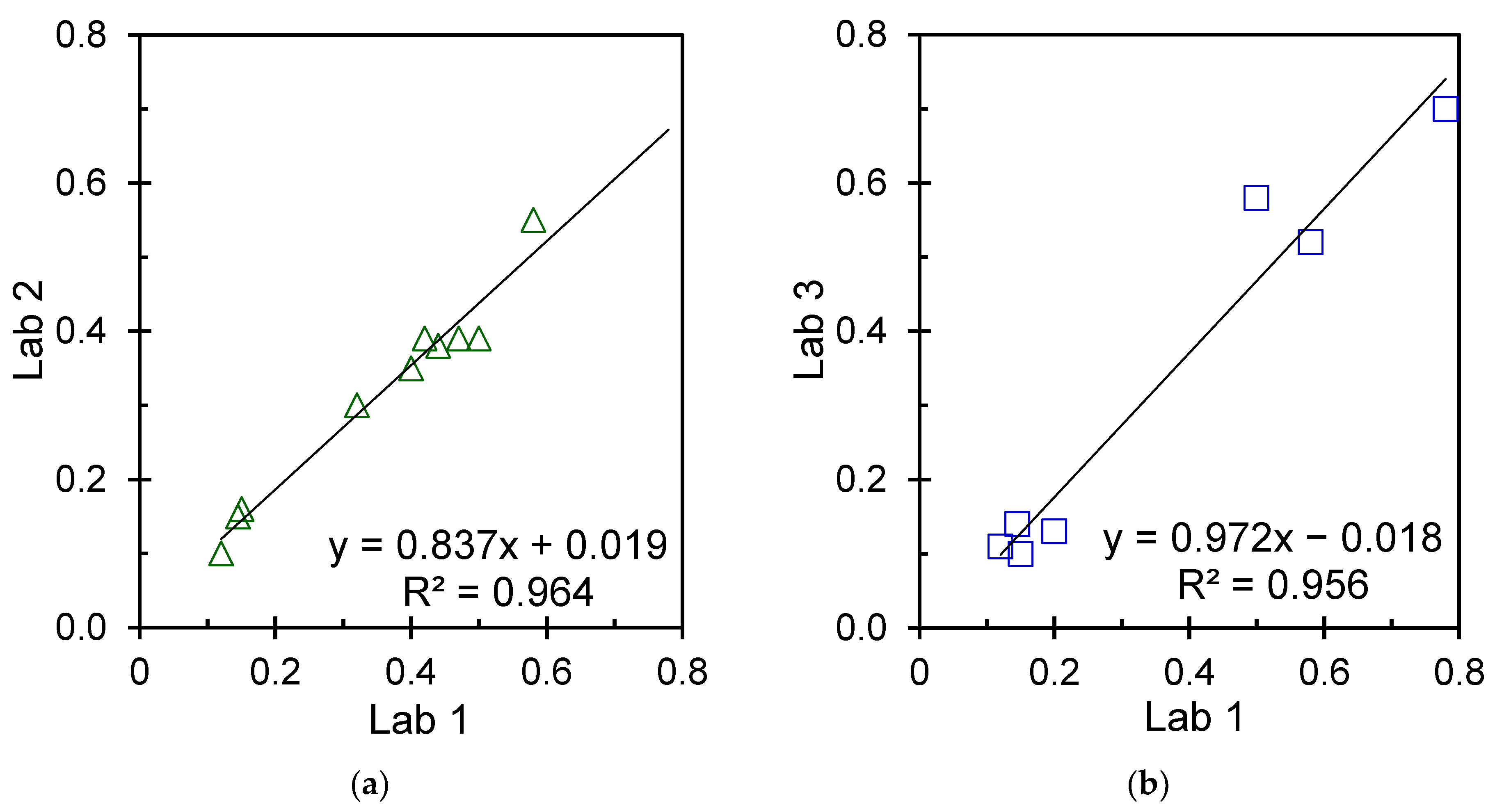
3.7. Data Validation
4. Conclusions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Lucas, L.L.; Unterweger, M.P. Comprehensive Review and Critical Evaluation of the Half-Life of Tritium. J. Res. Natl. Inst. Stand. Technol. 2000, 105, 541–549. [Google Scholar] [CrossRef] [PubMed]
- Canadian Nuclear Safety Commission. Standards and Guidelines for Tritium in Drinking Water (INFO-0766); Canadian Nuclear Safety Commission: Ottawa, ON, Canada, 2014.
- Commission, E.U. Recommendation on the protection of the public against exposure to radon in drinking water supplies. Off. J. Eur. Community 2001, 344, 85–88. [Google Scholar]
- Mittal, S.; Rani, A.; Mehra, R. Estimation of radon concentration in soil and groundwater samples of Northern Rajasthan, India. J. Radiat. Res. Appl. Sci. 2016, 9, 125–130. [Google Scholar] [CrossRef]
- Mehra, R.; Bala, P. Estimation of annual effective dose due to Radon level in indoor air and soil gas in Hamirpur district of Himachal Pradesh. J. Geochem. Explor. 2014, 142, 16–20. [Google Scholar] [CrossRef]
- Sharma, S.; Mehta, K.; Narang, S.; Kumar, A. Dose estimation from the exposure to radon, thoron and their progeny concentrations in the environs of Kangra area, Himachal Himalayas, India. Groundw. Sustain. Dev. 2020, 11, 100409. [Google Scholar] [CrossRef]
- EC. Radon in Workplaces: Implementing the Requirements in Council Directive; European Council: Luxembourg, 2019. [Google Scholar] [CrossRef]
- Tadros, C.V.; Hughes, C.E.; Crawford, J.; Hollins, S.E.; Chisari, R. Tritium in Australian precipitation: A 50 year record. J. Hydrol. 2014, 513, 262–273. [Google Scholar] [CrossRef]
- Gonfiantini, R. Standards for stable isotope measurements in natural compounds. Nature 1978, 271, 534–536. [Google Scholar] [CrossRef]
- Rittirong, A.; Noithong, P.; Hazama, R.; Sakuma, Y.; Saenboonruang, K.; Sudprasert, W. Determination of tritium levels in tap waters collected from various regions in Thailand using liquid scintillation counting. J. Phys. Conf. Ser. 2019, 1285, 012021. [Google Scholar] [CrossRef]
- Al-Shamma’a, A.; Ghafel, K.; Al, S.; Al, M. Estimating of groundwater age and quality of Al-Shanafiya Area Southwest Iraq. Iraqi J. Sci. 2014, 55, 1061–1070. [Google Scholar]
- Mamun, A.; Alazmi, A.S. Investigation of Radon in Groundwater and the Corresponding Human-Health Risk Assessment in Northeastern Saudi Arabia. Sustainability 2022, 14, 14515. [Google Scholar] [CrossRef]
- Mamun, A.; Alazmi, A.S. Advancement of a Liquid Scintillation Counter and Semiconductor Alpha Spectroscopy Detector to Estimate the Radon Concentration in Groundwater. Water 2022, 14, 3849. [Google Scholar] [CrossRef]
- Mamun, A. Enrichment of low-level tritium in groundwater by an electrolysis process for liquid scintillation counting applications. Submitt. Hydrol. 2023. [Google Scholar]
- Mamun, A. Enrichment of Low-Level Tritium in Groundwater of Arid Regions Using Electrolysis Process with Electrolytic Cells; University of Hafr Al Batin: Hafr Al Batin, Saudi Arabia, 2023. [Google Scholar]
- Salvato, C. The role of micro-strategies in the engineering of firm evolution. J. Manag. Stud. 2003, 40, 83–108. [Google Scholar] [CrossRef]
- Choppin, G.R.; Liljenzin, J.-O.; Rydberg, J.A.N. Chapter 5—Radionuclides in Nature. In Radiochemistry and Nuclear Chemistry, 3rd ed.; Choppin, G.R., Liljenzin, J.-O., Rydberg, J.A.N., Eds.; Butterworth-Heinemann: Woburn, MA, USA, 2002; pp. 94–122. [Google Scholar] [CrossRef]
- Broda, R.; Maletka, K. The TDCR Method as an Important Tool in Radionuclide Metrology; IAEA: Vienna, Austria, 2003. [Google Scholar]
- Arun, B.; Viswanathan, S.; Venkatesan, S.; Jose, M.T.; Balasubramaniam, V. Study of Triple to Double Coincidence Method for Tritium Measurements. Radiochemistry 2021, 63, 221–226. [Google Scholar] [CrossRef]
- Wanke, C.; Kossert, K.; Nähle, O.J. Investigations on TDCR measurements with the HIDEX 300 SL using a free parameter model. Appl. Radiat. Isot. 2012, 70, 2176–2183. [Google Scholar] [CrossRef]
- Broda, R. A review of the triple-to-double coincidence ratio (TDCR) method for standardizing radionuclides. Appl. Radiat. Isot. Incl. Data Instrum. Methods Use Agric. Ind. Med. 2003, 58, 585–594. [Google Scholar] [CrossRef]
- Feng, B.; Chen, B.; Zhao, C.; He, L.; Tang, F.; Zhuo, W. Application of a liquid scintillation system with 100-ml counting vials for environmental tritium determination: Procedure optimization, performance test, and uncertainty analysis. J. Environ. Radioact. 2020, 225, 106427. [Google Scholar] [CrossRef]
- USEPA. National Primary Drinking Water Regulations; Radionuclides; Final Rule. Fed. Reg. 2000, 65, 76708. [Google Scholar]
- UNSCEAR. The General Assembly with Scientific Annex; UNSCEAR: New York, NY, USA, 2000. [Google Scholar]
- WHO. Guidelines for Drinking-Water Quality; World Health Organization: Geneva, Switzerland, 2011; Volume 216, pp. 303–304. [Google Scholar]
- Aleissa, K.A.; Alghamdi, A.S.; Almasoud, F.I.; Islam, M.S. Measurement of radon levels in groundwater supplies of Riyadh with liquid scintillation counter and the associated radiation dose. Radiat. Prot. Dosim. 2013, 154, 95–103. [Google Scholar] [CrossRef]
- Abuelhia, E. Assessment of radiation dose from radon ingestion and inhalation in commercially bottled drinking water and its annual effective dose in Eastern Province, Saudi Arabia. Int. J. Environ. Health Res. 2019, 29, 164–172. [Google Scholar] [CrossRef]
- Abuelhia, E. Evaluation of annual effective dose from indoor radon concentration in Eastern Province, Dammam, Saudi Arabia. Radiat. Phys. Chem. 2017, 140, 137–140. [Google Scholar] [CrossRef]



| Sl No. | Sample Type | Total Sample | Tritium Level |
|---|---|---|---|
| Type 1 | Standard sample | 2 | Very high |
| Type 2 | Reference water | 5 | High to low |
| Type 3 | Rainwater | 4 | Low |
| Type 4 | Groundwater | 13 | Very low |
| Type 5 | Background water | 1 | ~Zero |
| Sample ID | Spiked Activity (TU) | ±Error (TU) | Measured Activity (TU) | ±Error (TU) | Z |
|---|---|---|---|---|---|
| Ref-1 | 8500 | 604.4 | 8760 | 560.9 | −0.346 |
| Ref-2 | 4250 | 182.7 | 4124 | 264.1 | 0.352 |
| Ref-3 | 850 | 166.2 | 820 | 52.42 | 0.160 |
| Ref-4 | 425 | 133.4 | 395 | 25.29 | 0.213 |
| Ref-5 | 85 | 12.29 | 89.5 | 5.740 | −0.352 |
| Sample ID | Tritium Activity (TU) | ±Uncertainty (TU) |
|---|---|---|
| Rain-1 | 3.37 | 0.23 |
| Rain-2 | 3.08 | 0.21 |
| Rain-3 | 3.48 | 0.24 |
| Rain-4 | 2.78 | 0.20 |
| Sample ID | Depth of Wells | Tritium Activity (TU) Lab 1 | ±Uncertainty (TU) |
|---|---|---|---|
| Gr-01 | Deep | 0.47 | 0.07 |
| Gr-02 | Deep | 0.58 | 0.08 |
| Gr-03 | Deep | 0.50 | 0.07 |
| Gr-04 | Deep | <MDA | - |
| Gr-05 | Shallow | 0.40 | 0.06 |
| Gr-06 | Shallow | 0.32 | 0.06 |
| Gr-07 | Shallow | 0.42 | 0.07 |
| Gr-08 | Deep | <MDA | - |
| Gr-09 | Deep | <MDA | - |
| Gr-10 | Shallow | 0.44 | 0.07 |
| Gr-11 | Deep | <MDA | - |
| Gr-12 | Deep | <MDA | - |
| Gr-13 | Shallow | 0.78 | 0.09 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mamun, A. Estimation of Tritium Concentration in the Rain- and Groundwater in the Dry River of Hafr Al Batin, Saudi Arabia. Limnol. Rev. 2023, 23, 93-107. https://doi.org/10.3390/limnolrev23020006
Mamun A. Estimation of Tritium Concentration in the Rain- and Groundwater in the Dry River of Hafr Al Batin, Saudi Arabia. Limnological Review. 2023; 23(2):93-107. https://doi.org/10.3390/limnolrev23020006
Chicago/Turabian StyleMamun, Al. 2023. "Estimation of Tritium Concentration in the Rain- and Groundwater in the Dry River of Hafr Al Batin, Saudi Arabia" Limnological Review 23, no. 2: 93-107. https://doi.org/10.3390/limnolrev23020006
APA StyleMamun, A. (2023). Estimation of Tritium Concentration in the Rain- and Groundwater in the Dry River of Hafr Al Batin, Saudi Arabia. Limnological Review, 23(2), 93-107. https://doi.org/10.3390/limnolrev23020006






