Go-MoS2/Water Flow over a Shrinking Cylinder with Stefan Blowing, Joule Heating, and Thermal Radiation
Abstract
1. Introduction
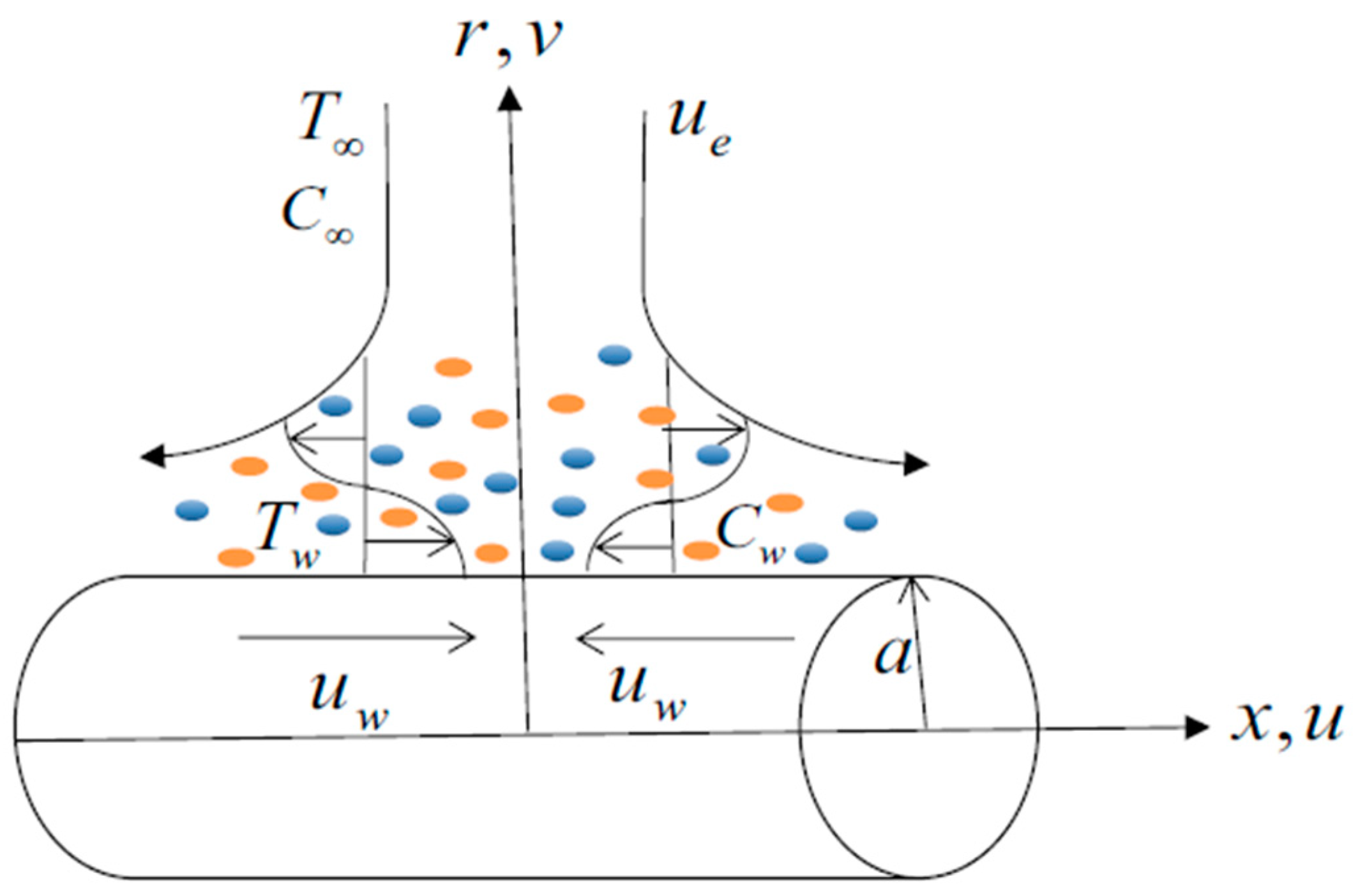
2. Mathematical Formulation
- The flow is steady, laminar, and 2D-dimensional.
- The flow is incompressible.
- The cylinder is shrinking with uniform velocity along the x-direction.
3. Numerical Method
4. Results and Discussion
5. Conclusions
- Since water is used as the base fluid in our model, the Schmidt number is fixed.
- Surface velocity is always less than zero.
- The Prandtl number has a range from 1.7 to 13.7 which is based on the base fluid.
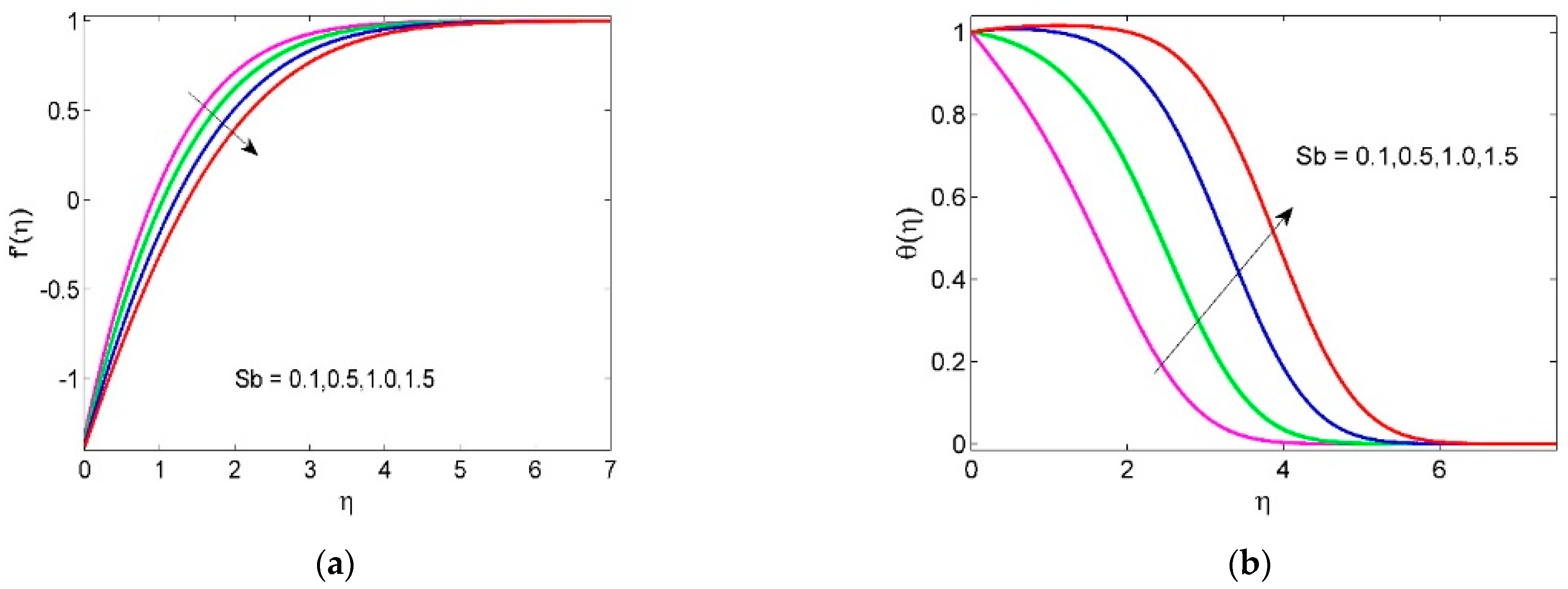
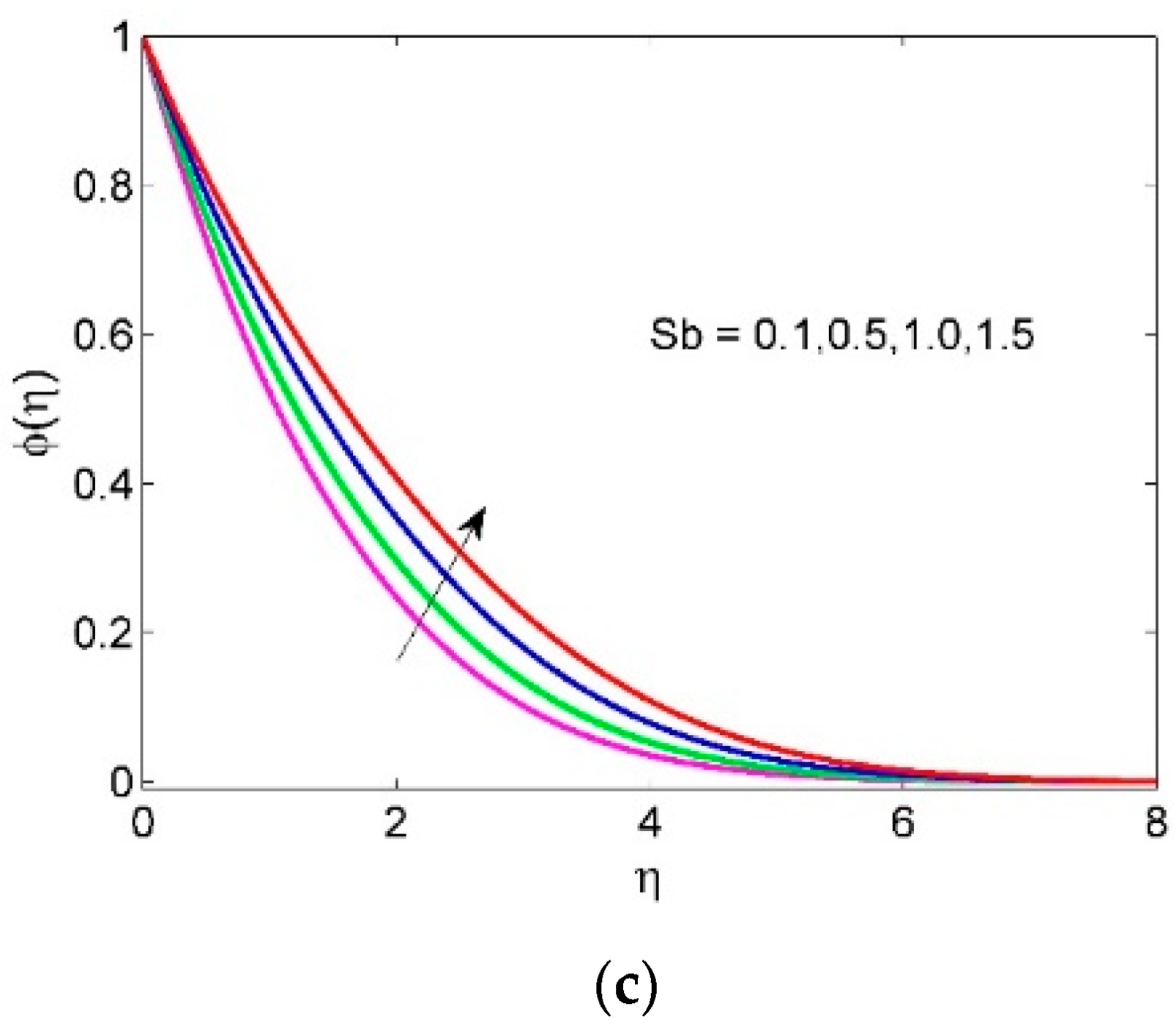
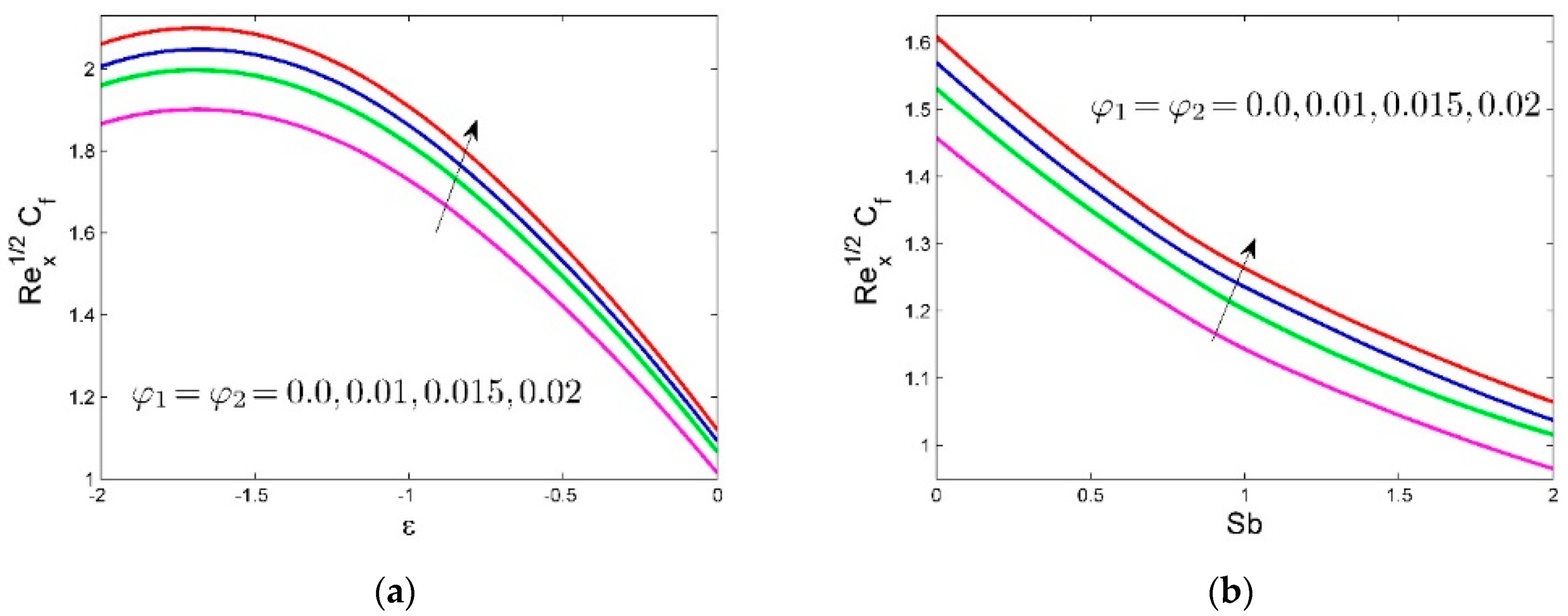
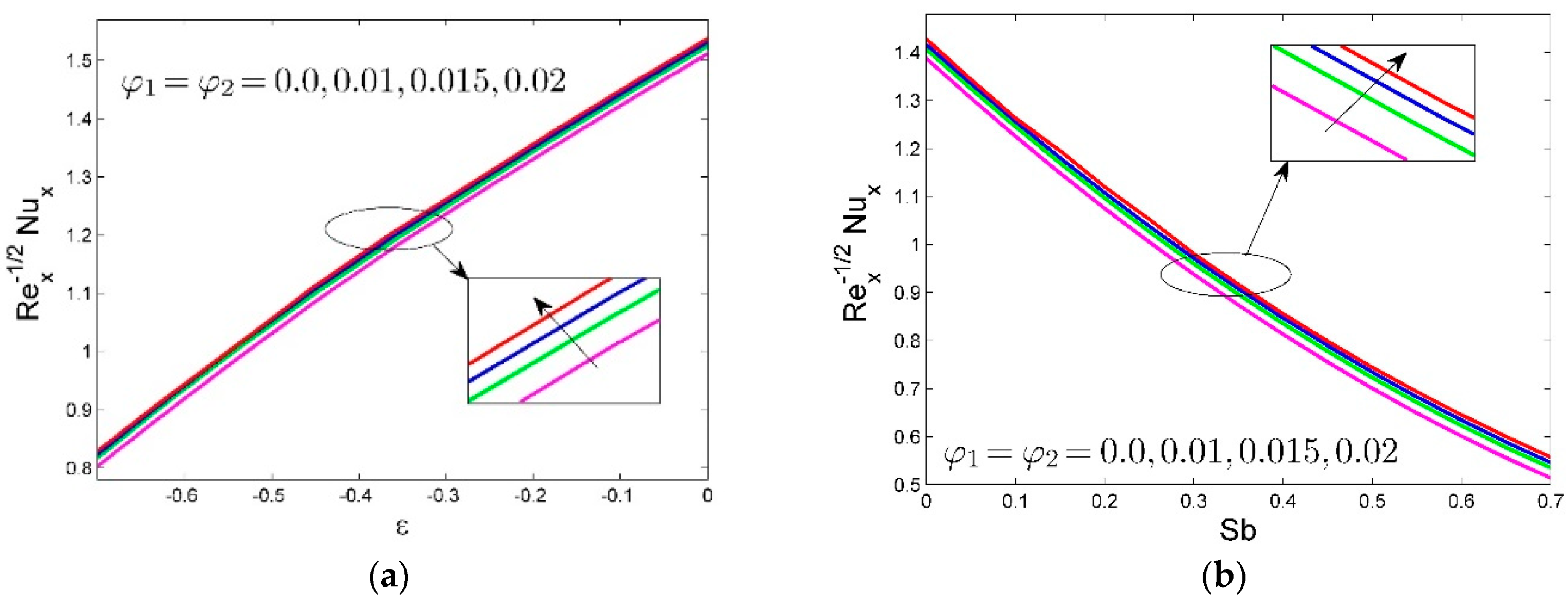
- There is an augmentation in temperature as well as concentration profiles due to the presence of Stefan blowing, but the velocity profile falls.
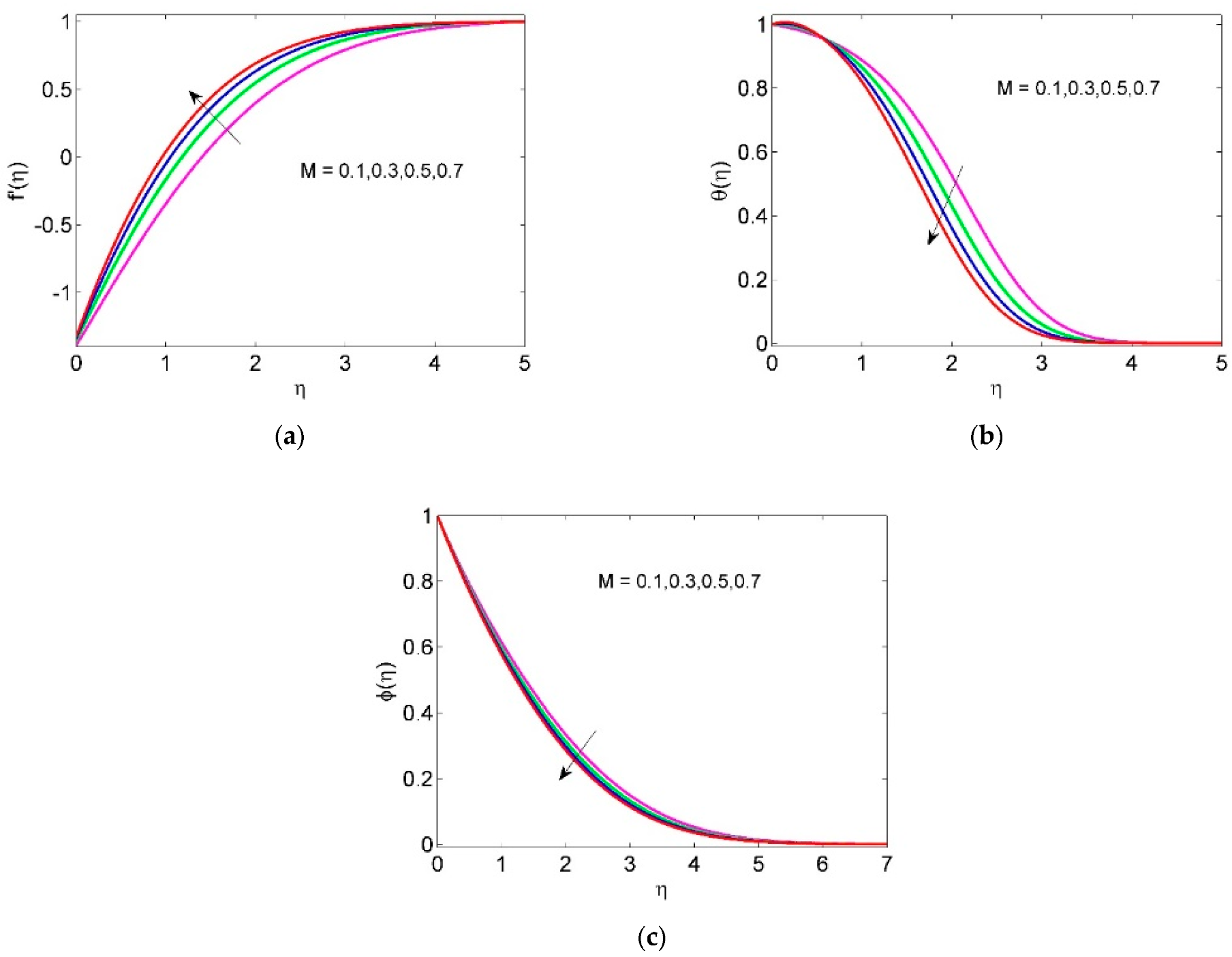
- The magnetic and curvature parameters cause a reduction in the temperature and concentration profiles and a rise in the velocity profile.
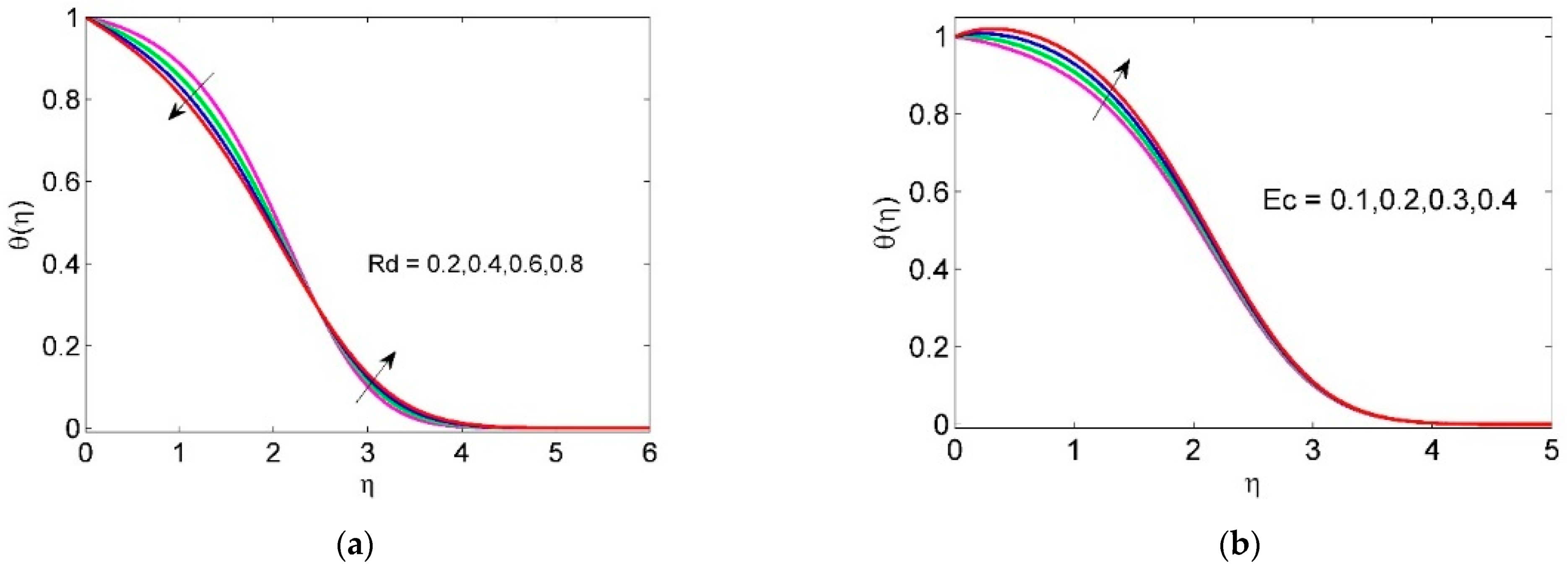
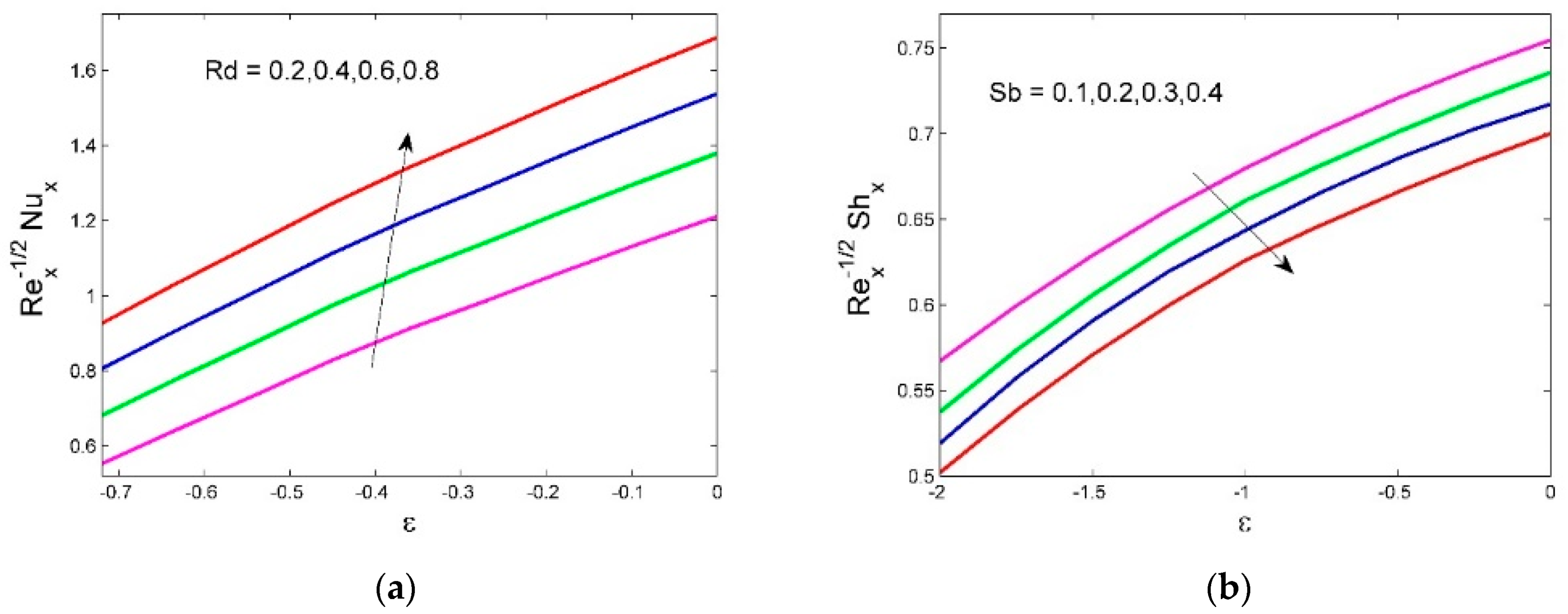
- The temperature profile rises due to an increase in Eckert number and thermal radiation parameter.
- The velocity profile rises as the magnetic field increases but decreases the thermal and concentration boundary layer.
- The heat transfer rate is improved by the thermal radiation parameter and shrinking case when ε < 0.
- With higher Stefan blowing, heat transmission increases but the mass transfer rate decreases in the presence of 2% of nanoparticles/HNF.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| HNF | hybrid nanofluid |
| MHD | Magnetohydrodynamics |
Nomenclature
| u,v | velocity components along x- and r-axes (m s−1) |
| L | characteristic length (m) |
| ww | surface velocity (m s−1) |
| ue | free stream velocity (m s−1) |
| Tw | surface temperature (K) |
| Cw | surface concentration (mol m3) |
| T∞ | ambient temperature (K) |
| D | mass diffusivity (m2 s−1) |
| C∞ | ambient concentration (mol m3) |
| Cs | concentration susceptibility |
| qr | heat flux (kg m2 s3) |
| kT | thermal diffusion ratio |
| Cp | specific heat (kg−1 J) |
| k* | mean absorption coefficient (cm−1) |
| T | the temperature of the fluid (K) |
| a | cylinder radius (cm) |
| k | thermal conductivity (W m−1 K−1) |
| C | fluid concentration (mol m3) |
| Rd | thermal radiation parameter |
| B0 | strength of magnetic field (A m−1) |
| Ec | Eckert number |
| M | Magnetic parameter |
| Pr | Prandtl number |
| Sc | Schmidt number |
| Cf | skin friction coefficient |
| Sb | Stefan blowing parameter |
| A | velocity slip parameter |
| Re | local Reynolds number |
| Nu | local Nusselt number |
| Sh | local Sherwood number |
| Greek symbols | |
| ν | kinematic viscosity (m2 s−1) |
| σ | electrical conductivity (S m−1) |
| μ | dynamic viscosity (m2 s−1) |
| ρ | the density of the fluid (kg m−3) |
| ε | shrinking parameter (<0) |
| σ* | Stefan-Boltzmann constant (W m−2 K−4) |
| Subscripts | |
| ∞ | ambient |
| f | base fluid |
| nf | nanofluid |
| hnf | hybrid nanofluid |
References
- Choi, S.U.; Eastman, J.A. Enhancing Thermal Conductivity of Fluids with Nanoparticles; Argonne National Lab (ANL): Argonne, IL, USA, 1995.
- Turcu, R.; Darabont, A.; Nan, A.; Aldea, N.; Macovei, D.; Bica, D.; Vekas, L. New polypyrrole-multiwall carbon nanotubes hybrid materials. J. Optoelectron. Adv. Mater. 2006, 8, 643–647. [Google Scholar]
- Jana, S.; Salehi Khojin, A.; Zhong, W.H. Enhancement of fluid thermal conductivity by the addition of single and hybrid nano-additives. Thermochim. Acta 2007, 462, 45–55. [Google Scholar] [CrossRef]
- Takabi, B.; Salehi, S. Augmentation of the heat transfer performance of a sinusoidal corrugated enclosure by employing hybrid nanofluid. Adv. Mech. Eng. 2014, 6, 147059. [Google Scholar] [CrossRef]
- Crane, L.J. Flow past a stretching plate. Z. Angew. Math. Phys. ZAMP 1970, 21, 645–647. [Google Scholar] [CrossRef]
- Waini, I.; Ishak, A.; Pop, I. Hybrid nanofluid flow towards a stagnation point on a stretching/shrinking cylinder. Sci. Rep. 2020, 10, 9296. [Google Scholar] [CrossRef]
- Wang, C.Y. Stagnation Flow towards a Shrinking Sheet. Int. J. Non-Linear Mech. 2008, 43, 377–382. [Google Scholar] [CrossRef]
- Waini, I.; Ishak, A.; Pop, I. Hybrid Nanofluid Flow on a Shrinking Cylinder with Prescribed Surface Heat Flux. Int. Numer. Methods Heat Fluid Flow 2020, 31, 1987–2004. [Google Scholar] [CrossRef]
- Awaludin, I.S.; Ahmad, R.; Ishak, A. On the stability of the flow over a shrinking cylinder with prescribed surface heat flux. Propuls. Power Res. 2020, 9, 181–187. [Google Scholar] [CrossRef]
- Ali, A.; Marwat, D.N.K.; Asghar, S. Viscous Flow over a Stretching (Shrinking) and Porous Cylinder of Non-Uniform Radius. Adv. Mech. Eng. 2019, 11, 168781401987984. [Google Scholar] [CrossRef]
- Jagan, K.; Sivasankaran, S.; Bhuvaneswari, M.; Rajan, S.; Makinde, O.D. Soret and Dufour effect on MHD Jeffrey nanofluid flow towards a stretching cylinder with triple stratification, radiation and slip. Defect Diffus. Forum 2018, 387, 523–533. [Google Scholar] [CrossRef]
- Sankar, M.; Kiran, S.; Sivasankaran, S. Natural Convection in a Linearly Heated Vertical Porous Annulus. J. Phys. Conf. Ser. 2018, 1139, 012018. [Google Scholar] [CrossRef]
- Rashid, U.; Liang, H.; Ahmad, H.; Abbas, M.; Iqbal, A.; Hamed, Y.S. Study of (Ag and TiO2)/Water Nanoparticles Shape Effect on Heat Transfer and Hybrid Nanofluid Flow toward Stretching Shrinking Horizontal Cylinder. Results Phys. 2021, 21, 103812. [Google Scholar] [CrossRef]
- Girish, N.; Sankar, M.; Makinde, O.D. Developing Buoyant Convection in Vertical Porous Annuli with Unheated Entry and Exit. Heat Transf. 2020, 49, 2551–2576. [Google Scholar] [CrossRef]
- Chu, Y.-M.; Nisar, K.S.; Khan, U.; Daei Kasmaei, H.; Malaver, M.; Zaib, A.; Khan, I. Mixed Convection in MHD Water-Based Molybdenum Disulfide-Graphene Oxide Hybrid Nanofluid through an Upright Cylinder with Shape Factor. Water 2020, 12, 1723. [Google Scholar] [CrossRef]
- Khan, U.; Zaib, A.; Ishak, A.; Waini, I.; Abdel-Aty, A.-H.; Sheremet, M.A.; Yahia, I.S.; Zahran, H.Y.; Galal, A.M. Agrawal Axisymmetric Rotational Stagnation-Point Flow of a Water-Based Molybdenum Disulfide-Graphene Oxide Hybrid Nanofluid and Heat Transfer Impinging on a Radially Permeable Moving Rotating Disk. Nanomaterials 2022, 12, 787. [Google Scholar] [CrossRef]
- Wahid, N.S.; Arifin, N.M.; Pop, I.; Bachok, N.; Hafidzuddin, M.E.H. MHD stagnation-point flow of nanofluid due to a shrinking sheet with melting, viscous dissipation and Joule heating effects. Alex. Eng. J. 2022, 61, 12661–12672. [Google Scholar] [CrossRef]
- Alarabi, T.H.; Rashad, A.M.; Mahdy, A. Homogeneous–Heterogeneous Chemical Reactions of Radiation Hybrid Nanofluid Flow on a Cylinder with Joule Heating: Nanoparticles Shape Impact. Coatings 2021, 11, 1490. [Google Scholar] [CrossRef]
- Khashi’ie, N.S.; Waini, I.; Arifin, N.M.; Pop, I. Unsteady Squeezing Flow of Cu–Al2O3/Water Hybrid Nanofluid in a Horizontal Channel with Magnetic Field. Sci. Rep. 2021, 11, 14128. [Google Scholar] [CrossRef]
- Jagan, K.; Sivasankaran, S. Soret & Dufour and Triple Stratification Effect on MHD Flow with Velocity Slip towards a Stretching Cylinder. Math. Comput. Appl. 2022, 27, 25. [Google Scholar] [CrossRef]
- Yashkun, U.; Zaimi, K.; Abu Bakar, N.A.; Ishak, A.; Pop, I. MHD Hybrid Nanofluid Flow over a Permeable Stretching/Shrinking Sheet with Thermal Radiation Effect. Int. J. Numer. Methods Heat Fluid Flow 2020, 31, 1014–1031. [Google Scholar] [CrossRef]
- Pal, D.; Mandal, G.; Vajravalu, K. Soret and Dufour Effects on MHD Convective–Radiative Heat and Mass Transfer of Nanofluids over a Vertical Non-Linear Stretching/Shrinking Sheet. Appl. Math. Comput. 2016, 287–288, 184–200. [Google Scholar] [CrossRef]
- Waini, I.; Khan, U.; Zaib, A.; Ishak, A.; Pop, I. Inspection of TiO2-CoFe2O4 Nanoparticles on MHD Flow toward a Shrinking Cylinder with Radiative Heat Transfer. J. Mol. Liq. 2022, 361, 119615. [Google Scholar] [CrossRef]
- Aladdin, N.A.L.; Bachok, N.; Rosali, H.; Wahi, N.; Abd Rahmin, N.A.; Arifin, N.M. Numerical Computation of Hybrid Carbon Nanotubes Flow over a Stretching/Shrinking Vertical Cylinder in Presence of Thermal Radiation and Hydromagnetic. Mathematics 2022, 10, 3551. [Google Scholar] [CrossRef]
- Eswaramoorthi, S.; Jagan, K.; Sivasankaran, S. MHD Bioconvective Flow of a Thermally Radiative Nanoliquid in a Stratified Medium Considering Gyrotactic Microorganisms. J. Phys. Conf. Ser. 2020, 1597, 012001. [Google Scholar] [CrossRef]
- Uddin, M.J.; Alginahi, Y.; Bég, O.A.; Kabir, M.N. Numerical Solutions for Gyrotactic Bioconvection in Nanofluid-Saturated Porous Media with Stefan Blowing and Multiple Slip Effects. Comput. Math. Appl. 2016, 72, 2562–2581. [Google Scholar] [CrossRef]
- Lund, L.A.; Omar, Z.; Raza, J.; Khan, I.; Sherif, E.-S.M. Effects of Stefan Blowing and Slip Conditions on Unsteady MHD Casson Nanofluid Flow over an Unsteady Shrinking Sheet: Dual Solutions. Symmetry 2020, 12, 487. [Google Scholar] [CrossRef]
- Ali, B.; Hussain, S.; Abdal, S.; Mehdi, M.M. Impact of Stefan Blowing on Thermal Radiation and Cattaneo–Christov Characteristics for Nanofluid Flow Containing Microorganisms with Ablation/Accretion of Leading Edge: FEM Approach. Eur. Phys. J. Plus 2020, 135, 821. [Google Scholar] [CrossRef]
- Rana, P.; Makkar, V.; Gupta, G. Finite element study of bio-convective Stefan blowing Ag-MgO/water hybrid nanofluid induced by stretching cylinder utilizing non-Fourier and non-Fick’s laws. Nanomaterials 2021, 11, 1735. [Google Scholar] [CrossRef]
- Raza, A.; Khan, S.U.; Khan, M.I.; Farid, S.; Muhammad, T.; Khan, M.I.; Galal, A.M. Fractional order simulations for the thermal determination of graphene oxide (GO) and molybdenum disulphide (MoS2) nanoparticles with slip effects. Case Stud. Therm. Eng. 2021, 28, 101453. [Google Scholar] [CrossRef]



| Properties | Go | MoS2 | H2O |
|---|---|---|---|
| ρ (kg m−3) | 1800 | 5060 | 997.1 |
| (J kg−1 K−1) | 717 | 397.21 | 4179 |
| (W m−1 K−1) | 5000 | 904.4 | 0.63 |
| σ (S m−1) | 6.30 × 107 | 2.09 × 104 | 0.05 |
| Properties | Correlations of HNF |
|---|---|
| Density | |
| Heat Capacity | |
| Dynamic Viscosity | |
| Thermal Conductivity | |
| Electric conductivity |
| ε | |||
|---|---|---|---|
| Wang [7] | Waini et al. [8] | Present Result | |
| −1 | 1.328820 | 1.328817 | 1.328820 |
| −0.5 | 1.495670 | 1.495670 | 1.495670 |
| 0 | 1.232588 | 1.232588 | 1.232588 |
| Sb | M | Ec | Rd | ε | ||||
|---|---|---|---|---|---|---|---|---|
| 0 | 0.1 | 0.1 | 0.1 | −0.5 | 0.02 | 1.578609 | 1.269266 | 0.471888 |
| 0.1 | - | - | - | - | - | 1.369317 | 1.098009 | 0.438235 |
| 1.0 | - | - | - | - | - | 1.180348 | 0.291620 | 0.308434 |
| 2.0 | - | - | - | - | - | 1.067028 | 0.072320 | 0.237721 |
| - | 0.1 | - | - | - | - | 1.067028 | 0.072320 | 0.237721 |
| - | 0.2 | - | - | - | - | 1.161345 | 0.032563 | 0.234640 |
| - | 0.3 | - | - | - | - | 1.205204 | 0.016897 | 0.237441 |
| - | - | 0.1 | - | - | - | 1.221111 | 0.008869 | 0.238595 |
| - | - | 0.2 | - | - | - | 1.172795 | −0.022768 | 0.240634 |
| - | - | 0.3 | - | - | - | 1.197174 | −0.101285 | 0.238227 |
| - | - | - | 0.2 | - | - | 1.139701 | −0.095667 | 0.24396 |
| - | - | - | 0.4 | - | - | 1.139701 | −0.078478 | 0.243946 |
| - | - | - | 0.6 | - | - | 1.139700 | −0.049477 | 0.243946 |
| - | - | - | - | −0.1 | - | 0.970726 | 0.054617 | 0.273412 |
| - | - | - | - | −0.2 | - | 1.014529 | 0.036342 | 0.267537 |
| - | - | - | - | −0.3 | - | 1.098488 | −0.022513 | 0.256132 |
| - | - | - | - | - | 0.01 | 1.115239 | −0.36832 | 0.254321 |
| - | - | - | - | - | 0.015 | 1.115239 | −0.36832 | 0.254321 |
| - | - | - | - | - | 0.02 | 1.115239 | −0.036832 | 0.254321 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Narayanaswamy, M.K.; Kandasamy, J.; Sivanandam, S. Go-MoS2/Water Flow over a Shrinking Cylinder with Stefan Blowing, Joule Heating, and Thermal Radiation. Math. Comput. Appl. 2022, 27, 110. https://doi.org/10.3390/mca27060110
Narayanaswamy MK, Kandasamy J, Sivanandam S. Go-MoS2/Water Flow over a Shrinking Cylinder with Stefan Blowing, Joule Heating, and Thermal Radiation. Mathematical and Computational Applications. 2022; 27(6):110. https://doi.org/10.3390/mca27060110
Chicago/Turabian StyleNarayanaswamy, Manoj Kumar, Jagan Kandasamy, and Sivasankaran Sivanandam. 2022. "Go-MoS2/Water Flow over a Shrinking Cylinder with Stefan Blowing, Joule Heating, and Thermal Radiation" Mathematical and Computational Applications 27, no. 6: 110. https://doi.org/10.3390/mca27060110
APA StyleNarayanaswamy, M. K., Kandasamy, J., & Sivanandam, S. (2022). Go-MoS2/Water Flow over a Shrinking Cylinder with Stefan Blowing, Joule Heating, and Thermal Radiation. Mathematical and Computational Applications, 27(6), 110. https://doi.org/10.3390/mca27060110







