Impacts of Casson Model on Hybrid Nanofluid Flow over a Moving Thin Needle with Dufour and Soret and Thermal Radiation Effects
Abstract
1. Introduction
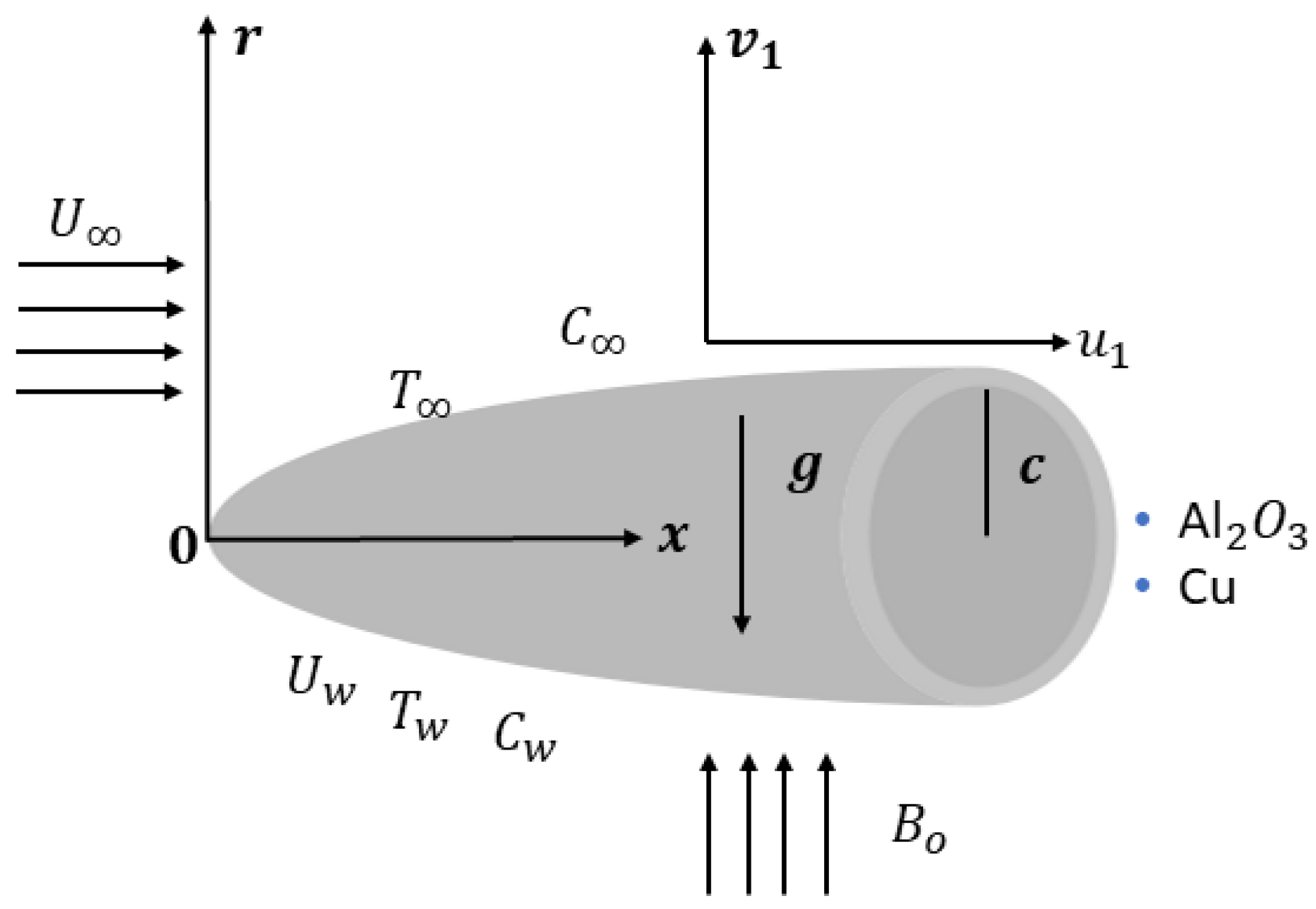
2. Mathematical Formulation of the Problem
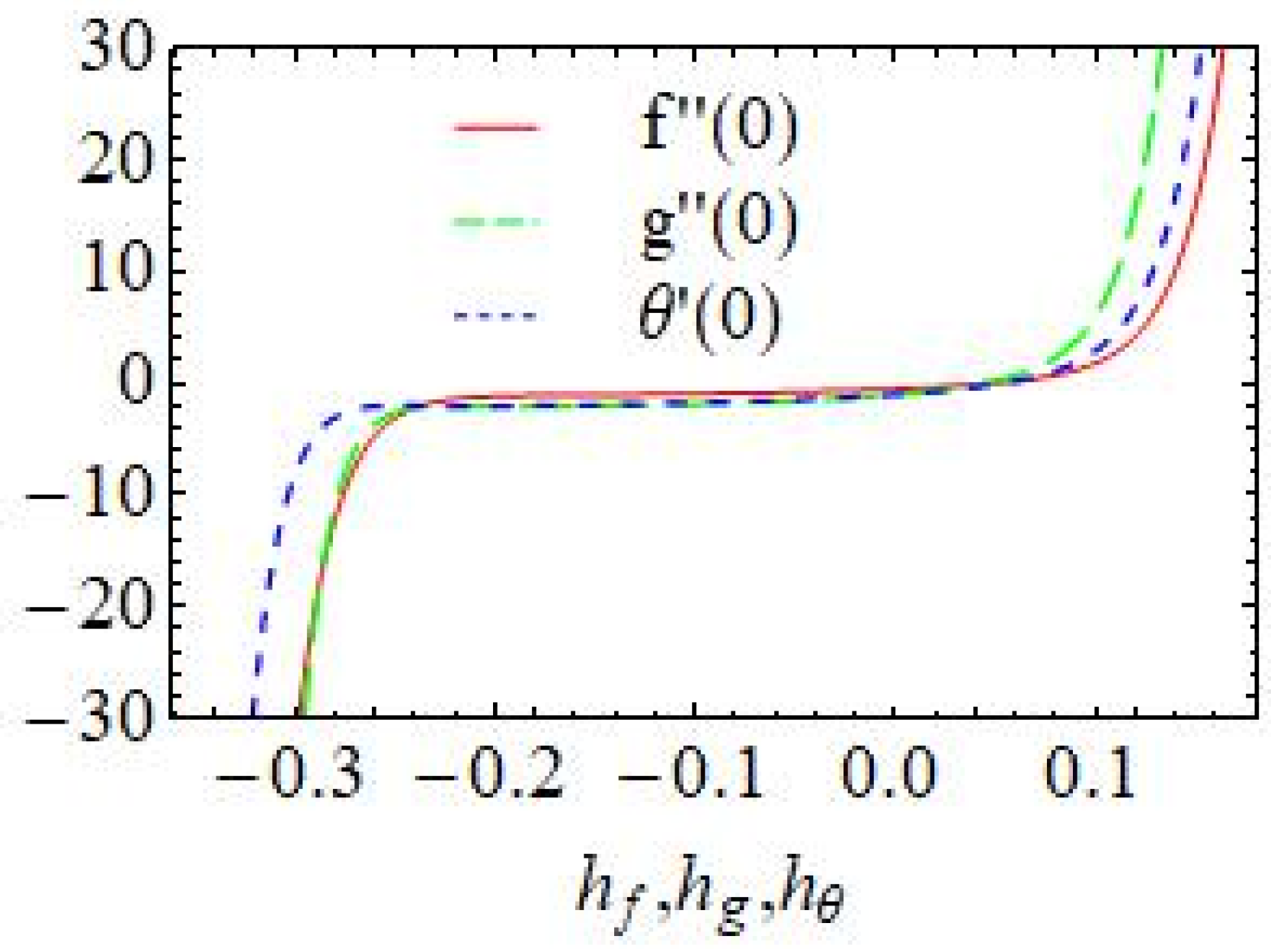
3. Method of Solution
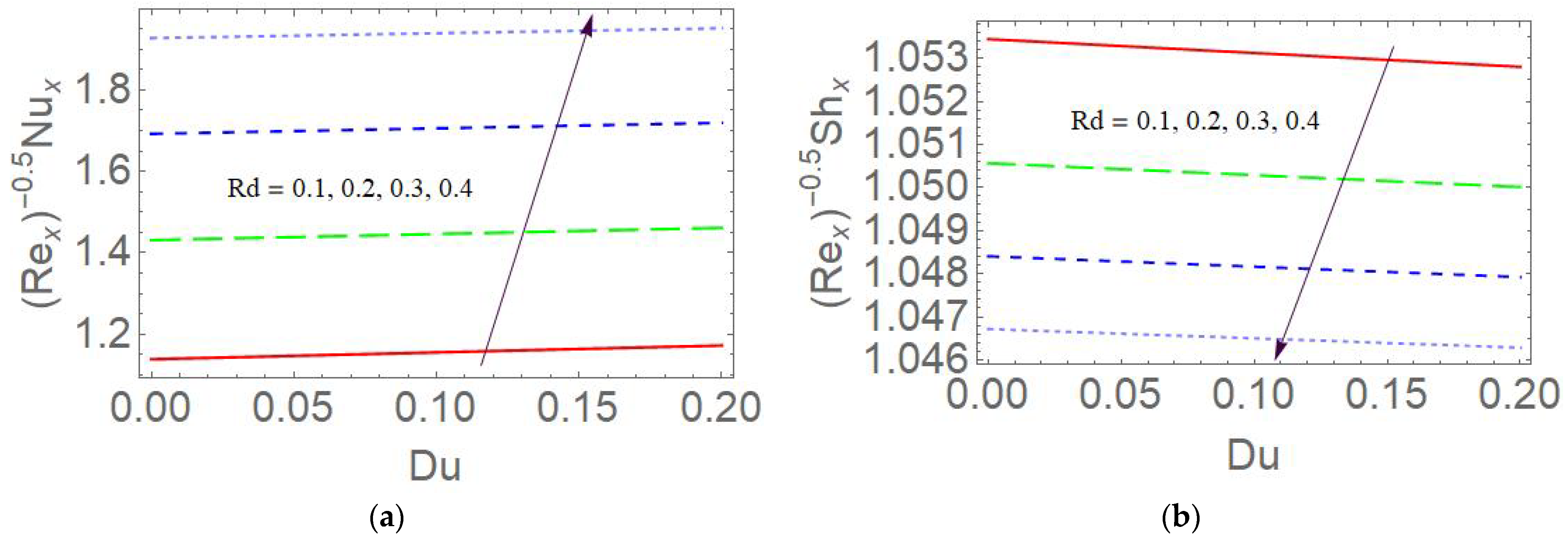
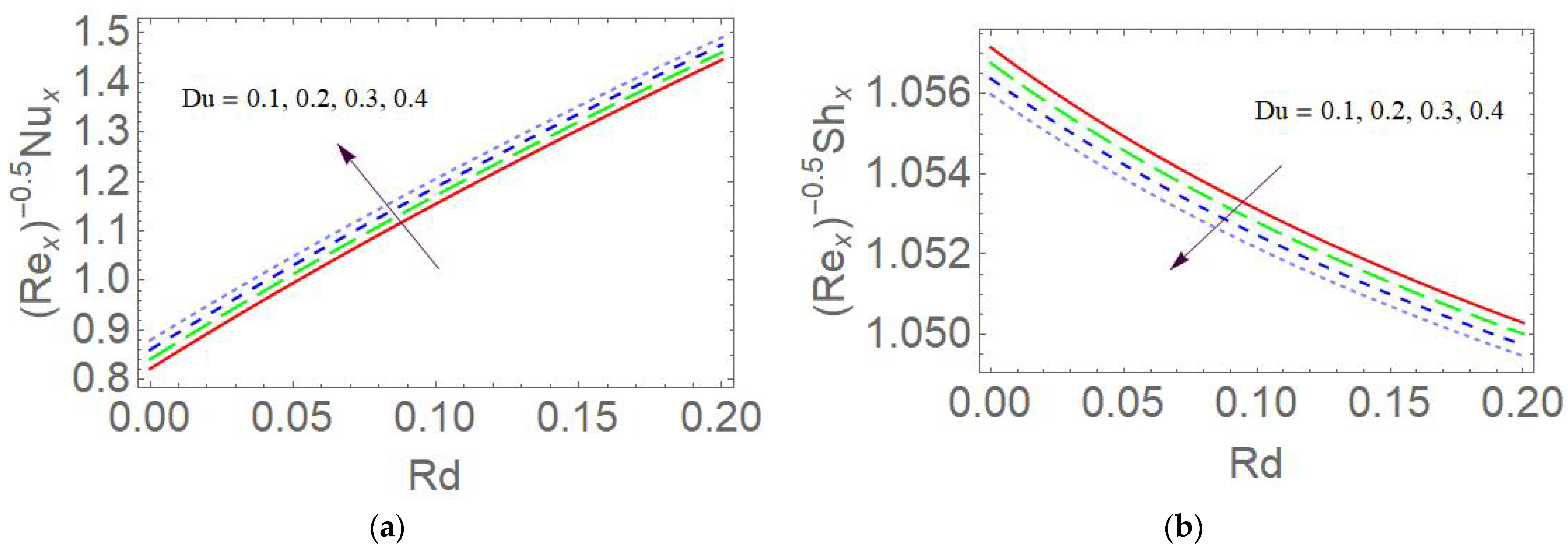
4. Results and Discussions
5. Conclusions
- The boundary layer that forms around the needle is equally tiny in size.
- The series solution is obtained with the proposed HAM method. HAM gives better approximations than other numerical methods.
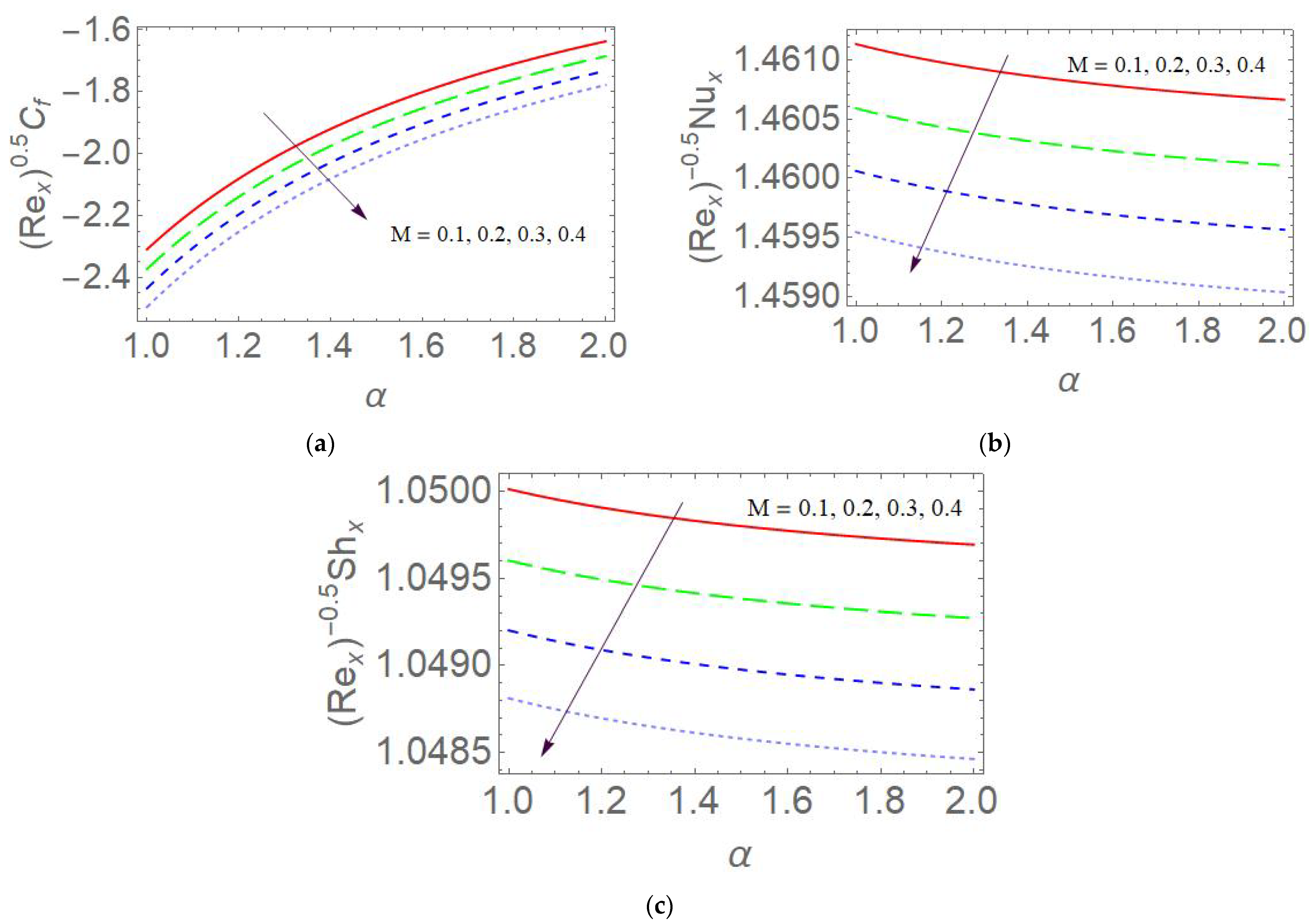
- In total, 2% nanoparticles are included and the rate of heat transfer increases up to 45%.
- The Casson parameter tends to makes the flow field smaller. Physically, increasing the Casson parameter causes an enhancement in the fluids dynamic viscosity, which reduces the fluid motion and results in a decrease in the profile of velocity.
- As the magnetic field strength increases, the velocity profiles become narrower, because magnetic fields produce Lorentz forces that oppose motion.
- As a result of the thermal radiation being included, heat is transferred more quickly.
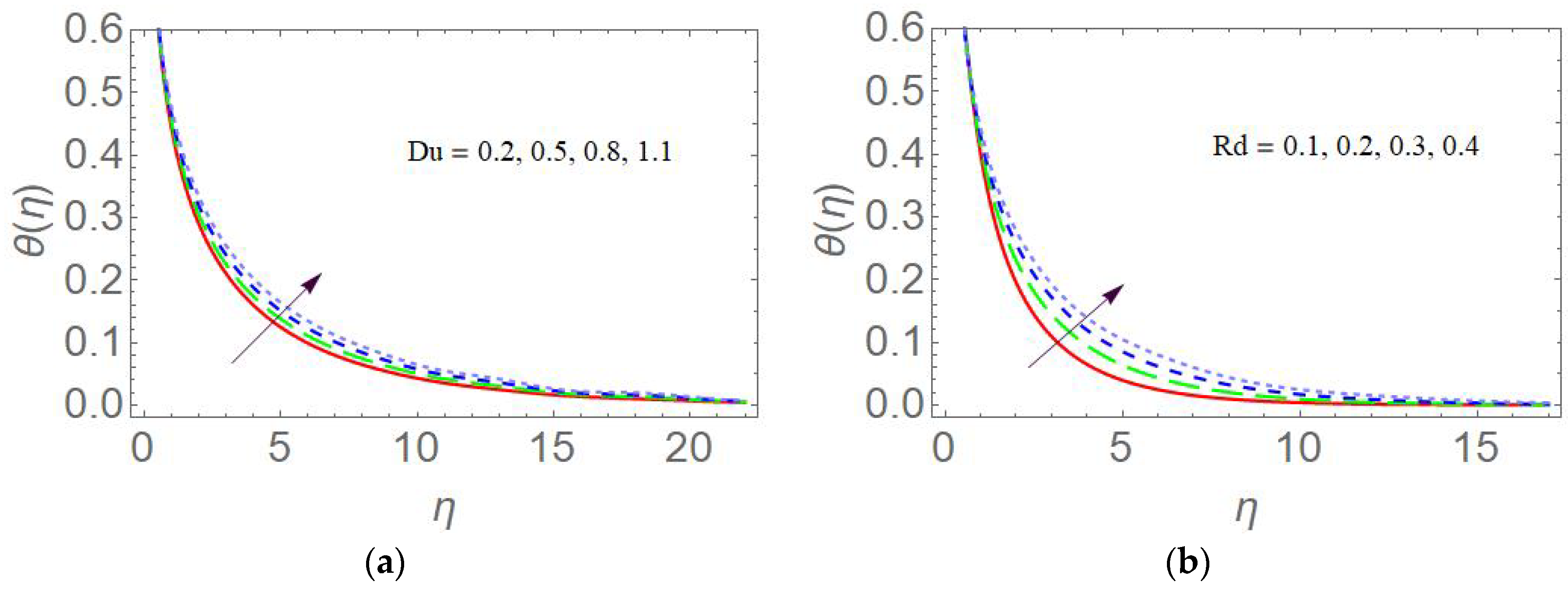
- As the radiation parameter is increased, the temperature within the boundary layer naturally increases, because thermal radiation is dominant over a thermal conduction heat transfer.
- The rates of mass and heat transfer as well as the Dufour and Soret effects are accelerated, because with the Dufour effect, the concentration gradient affects the flow of thermal energy flux, and higher Soret numbers correspond to a greater temperature gradient, resulting in a greater convective flow.
- The present results are useful in the cooling technology and thermal science community.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| MHD | Magnetohydrodynamics | |
| HAM | Homotopy Analysis Method | |
| c | needle radius (m) | |
| velocity at the surface (m.s−1) | ||
| wall concentration | ||
| temperature at the surface (K) | ||
| ambient concentration | ||
| temperature of mass fluid (K) | ||
| coefficient of mean absorption (c.m−1) | ||
| concentration susceptibility | ||
| free stream velocity (m.s−1) | ||
| specific heat (kg−1.J) | ||
| mass diffusion coefficient (m2.s−1) | ||
| ambient temperature (K) | ||
| velocity components along x and r directions (m.s−1) | ||
| radiative heat flux (kg.m2.s−3) | ||
| ratio of thermal diffusion | ||
| Skin friction | ||
| Sherwood number | ||
| C | fluid concentration | |
| Nusselt number | ||
| Greek symbols | ||
| kinematic viscosity (m2.s−1) | ||
| Casson parameter | ||
| fluid density (kg.m−3) | ||
| velocity ratio parameter | ||
| dynamic viscosity of a fluid (m2.s−1) | ||
| Stefan-Boltzmann constant (W.m−2.K−4) | ||
| Subscripts | ||
| hnf | hybrid nanofluid | |
| ‘ | differentiation w.r.t. | |
| nf | nanofluid | |
| f | fluid | |
| ambient | ||
References
- Wang, X.Q.; Mujumdar, A.S. A review on nanofluids—Part I: Theoretical and numerical investigations. Braz. J. Chem. Eng. 2008, 25, 613–630. [Google Scholar] [CrossRef]
- Wang, X.Q.; Mujumdar, A.S. A review on nanofluids—Part II: Experiments and applications. Braz. J. Chem. Eng. 2008, 25, 631–648. [Google Scholar] [CrossRef]
- Kamyar, A.; Saidur, R.; Hasanuzzaman, M. Application of Computational Fluid Dynamics (CFD) for nanofluids. Int. J. Heat Mass Transf. 2012, 55, 4104–4115. [Google Scholar] [CrossRef]
- Hayat, T.; Sabir Ali, S.; Qasim, M.; Asghar, S. Three-dimensional stretched flow via convective boundary condition and heat generation/absorption. Int. J. Numer. Methods Heat Fluid Flow 2012, 24, 342–358. [Google Scholar] [CrossRef]
- Sidik, N.A.C.; Adamu, I.M.; Jamil, M.M.; Kefayati, G.H.R.; Mamat, R.; Najafi, G. Recent progress on hybrid nanofluids in heat transfer applications: A comprehensive review. Int. Commun. Heat Mass Transf. 2016, 78, 68–79. [Google Scholar] [CrossRef]
- Rostami, M.N.; Dinarvand, S.; Pop, I. Dual solutions for mixed convective stagnation-point flow of an aqueous silica–alumina hybrid nanofluid. Chin. J. Phys. 2018, 56, 2465–2478. [Google Scholar] [CrossRef]
- Alwani, S.S.N.; Norfifah, B.; Norihan, A.; Fadzilah, A. Effect of Buoyancy force on the flow and heat transfer around the thin needle in Hybrid nanofluid. CFD Lett. 2020, 12, 22–36. [Google Scholar]
- Kavya, S.; Nagendramma, V.; Ameer Ahammad, N.; Sohail, A.; Raju, C.S.K. Magnetic-hybrid nanoparticles with stretching/shrinking cylinder in a suspension of MoS4 and copper nanoparticles. Int. Comm. Heat Mass Transf. 2022, 136, 106150. [Google Scholar] [CrossRef]
- Raju, C.S.K.; Ameer Ahammad, N.; Kiran, S.; Nehad Ali, S.; Se-Jin, Y.; Dinesh Kumar, M. Nonlinear movements of axisymmetric ternary hybrid nanofluids in a thermally radiated expanded or contracting permeable Darcy walls with different shapes and densities: Simple linear aggression. Int. Comm. Heat Mass Transf. 2022, 135, 106110. [Google Scholar] [CrossRef]
- Priyadarshini, P.; Vanitha Archana, N.; Ameer Ahammad, N.; Raju, C.S.K.; Se-Jin, Y.; Nehad Ali, S. Gradient descent machine learning regression for MHD flow: Metallurgy process. Int. Comm. Heat Mass Transf. 2022, 138, 106307. [Google Scholar] [CrossRef]
- Hu Ge, J.; Nehad Ali, S.; Mahrous, Y.M.; Pooja, S.; Raju, C.S.K.; Mamatha Upadhya, S. Radiated magnetic flow in a suspension of ferrous nanoparticles over a cone with Brownian motion and thermophoresis. Case Stud. Therm. Eng. 2021, 25, 100915. [Google Scholar]
- Basma, S.; Reddy, M.G.; Sreenivasulu, P.; Poornima, T.; Rahimi, G.M.; Alarifi, M.I. Comparative analysis on non-linear radiative heat transfer MHD Casson nanofluid past a thin needle. J. Mol. Liq. 2019, 284, 163–174. [Google Scholar]
- Bilal, M.; Urva, Y. Analysis of non-Newtonian fluid flow over a fine rotating thin needle for variable viscosity and activation energy. SN Appl. Sci. 2020, 2, 677. [Google Scholar] [CrossRef]
- Ibrar, N.; Reddy, M.G.; Shehzad, S.A.; Sreenivasulu, P.; Poornima, T. Interaction of single and multi -walls carbon nanotubes in magnetized- nano Casson fluid over radiated horizontal needle. SN Appl. Sci. 2020, 2, 677. [Google Scholar] [CrossRef]
- Jyothi, A.M.; Naveen Kumar, R.; Punith Gowda, R.J.; Prasanna kumara, B.C. Significance of Stefan blowing effect on flow and heat transfer of Casson nanofluid over a moving thin needle. Commun. Theor. Phys. 2021, 73, 0253–6102. [Google Scholar] [CrossRef]
- Mamatha Upadhya, S.; Shiva Rama Raju, S.V.; Raju, C.S.K.; Nehad Ali, S.; Jae Dong, C. Importance of entropy generation on Casson, Micropolar and Hybrid magneto-nanofluids in a suspension of cross section. Chin. J. Phys. 2021, 77, 1080–1101. [Google Scholar] [CrossRef]
- Sulochana, C.; Poornima, M.; Sandeep, N.; Gangadhar, P.A.K. Effect of variable heat generation/absorption on magnetohydrodynamic Sakiadis flow of Casson/Carreau hybrid nano liquid due to a persistently moving needle. Heat Transfer 2021, 50, 8354–8377. [Google Scholar]
- Raju, C.S.K.; Ibrahim, S.K.; Anuradha, S.; Priyadarshini, P. Bio-convection on non-linear radiative flow of a Carreau fluid over a moving wedge with suction or injection. Eur. Phy. J. Plus 2016, 131, 409. [Google Scholar] [CrossRef]
- Upadhya, S.M.; Mahesha; Raju, C.S.K. Cattaneo-Christov heat flux model for magnetohydrodynamic flow in a suspension of dust particles towards a stretching sheet. Nonlinear Eng. 2018, 3, 237–246. [Google Scholar] [CrossRef]
- Iskandar, W.; Anuar, I.; Pop, I. Hybrid nanofluid flow and heat transfer past a vertical thin needle with prescribed surface heat flux. Int. J. Numer. Methods Heat Fluid Flow 2019, 29, 4875–4894. [Google Scholar]
- Sulochana, C.; Aparna, S.R.; Sandeep, N. Impact of linear/nonlinear radiation on incessantly moving thin needle in MHD quiescent Al-Cu/methanol hybrid nanofluid. Int. Ambient. Energy 2020, 43, 2694–2700. [Google Scholar] [CrossRef]
- Madhukesh, K.; Ramesh, G.K.; Alsulami, M.D.; Prasanna kumara, B.C. Characteristic of thermophoretic effect and convective boundary thermal conditions on flow of hybrid nanofluid over a moving thin needle. In Waves in Random and Complex Media; Taylor & Francis: Abingdon, UK, 2021. [Google Scholar] [CrossRef]
- Zainal, N.A.; Roslinda, N.; Kohilvani, N.; Pop, I. Stability analysis of unsteady MHD Rear stagnation point flow of hybrid nanofluid. Mathematics 2021, 9, 2428. [Google Scholar] [CrossRef]
- Jagan, K.; Sivasankaran, S.; Bhuvaneswari, M.; Rajan, S. Effect of Thermal Radiation on Magneto-Convection of a Micropolar Nano liquid towards a Non-Linear Stretching Surface with Convective Boundary. Int. J. Eng. Technol. 2018, 7, 417–421. [Google Scholar] [CrossRef]
- Solomon, B.K.; Mitiku, D.; Abebe, G. Investigation of Effects of Thermal Radiation, Magnetic Field, Eckert Number and Thermal Slip on MHD Hiemenz Flow by Optimal Homotopy Asymptotic Method. J. Math. 2021, 2021, 5590657. [Google Scholar] [CrossRef]
- Zainal, N.A.; Roslinda, N.; Kohilvani, N.; Pop, I. Unsteady flow of a Maxwell hybrid nanofluid past a stretching/shrinking surface with thermal radiation effect. Appl. Math. Mech. 2021, 42, 1511–1524. [Google Scholar] [CrossRef]
- Zainal, N.A.; Roslinda, N.; Kohilvani, N.; Pop, I. Unsteady stagnation point flow past a permeable stretching/shrinking riga plate in Al2O3-Cu/water hybrid nanofluid with thermal radiation. Int. J. Numer. Methods Heat Fluid Flow 2021, 32, 2640–2658. [Google Scholar] [CrossRef]
- Zainal, N.A.; Roslinda, N.; Kohilvani, N.; Ioan Pop, I. The impact of thermal radiation on Maxwell hybrid nanofluids in the stagnation point. Nanomaterials 2022, 12, 1109. [Google Scholar] [CrossRef]
- Tayyaba, N.; Bhatti, M.M.; Efstathios Michaelides, E. Hybrid (Au-TiO2) nanofluid flow over a thin needle with magnetic field and thermal radiation: Dual solutions and stability analysis. Microfluid. Nano Fluid. 2022, 26, 2. [Google Scholar] [CrossRef]
- Iskandar, W.; Anuar, I.; Pop, I. Dufour and Soret effects on Al2O3-water nanofluid flow over a moving thin needle: Tiwari and Das model. Int. J. Numer. Methods Heat Fluid Flow 2020, 31, 766–782. [Google Scholar] [CrossRef]
- Umair, K.; Zaib, A.; Sakhinah Abu, B.; Anuar, I. Forced convection flow of water conveying AA7072 and AA7075 alloys-nanomaterials on variable thickness object experiencing Dufour and Soret effects. Sci. Rep. 2022, 12, 6940. [Google Scholar]



| Properties | Al2O3 | Cu | Ethylene Glycol |
|---|---|---|---|
| (kg.m−3) | 3970 | 8933 | 1190 |
| (W.m−1.K−1) | 40 | 400 | 0.258 |
| (J.kg−1.K−1) | 765 | 385 | 2400 |
| (.m−1) |
| Properties | Nanofluid | Hybrid Nanofluid |
|---|---|---|
| Density | ||
| Heat capacity | ||
| Dynamic viscosity | ||
| Thermal conductivity | ||
| Electrical Conductivity |
| Order | |||
|---|---|---|---|
| 1 | 0.50000 | 1.00001 | 1.00001 |
| 5 | 0.50002 | 1.00005 | 1.00004 |
| 10 | 0.50004 | 1.00011 | 1.00008 |
| 15 | 0.50006 | 1.00015 | 1.00012 |
| 20 | 0.50009 | 1.0002 | 1.00016 |
| 25 | 0.50011 | 1.00025 | 1.0002 |
| 30 | 0.50013 | 1.0003 | 1.00024 |
| 35 | 0.50016 | 1.00035 | 1.00029 |
| 40 | 0.50018 | 1.0004 | 1.00033 |
| M | Du | Rd | ||||
|---|---|---|---|---|---|---|
| 1 | 0.1 | 0.2 | 0.2 | −2.30857 | −1.92005 | −1.75259 |
| 1.4 | −1.63701 | 1.46098 | 1.46092 | |||
| 1.7 | 1.46086 | 1.04996 | 1.04996 | |||
| 2 | 1.04991 | 1.04987 | 1.04983 | |||
| 1 | 0.1 | 0.2 | 0.2 | −2.30857 | −2.37217 | −2.43422 |
| 0.2 | −2.4948 | 1.46113 | 1.46059 | |||
| 0.3 | 1.46006 | 1.45955 | 1.05001 | |||
| 0.4 | 1.0496 | 1.0492 | 1.04881 | |||
| 1 | 0.1 | 0.2 | 0.1 | −2.30857 | −2.30857 | −2.30857 |
| 0.2 | −2.30857 | 1.17214 | 1.46113 | |||
| 0.3 | 1.71912 | 1.9514 | 1.0528 | |||
| 0.4 | 1.05001 | 1.04792 | 1.04628 | |||
| 1 | 0.1 | 0.1 | 0.2 | −2.30857 | −2.30857 | −2.30857 |
| 0.2 | −2.30857 | 1.44608 | 1.46113 | |||
| 0.3 | 1.4762 | 1.49127 | 1.05029 | |||
| 0.4 | 1.05001 | 1.04974 | 1.04946 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Reddy, V.S.; Kandasamy, J.; Sivanandam, S. Impacts of Casson Model on Hybrid Nanofluid Flow over a Moving Thin Needle with Dufour and Soret and Thermal Radiation Effects. Math. Comput. Appl. 2023, 28, 2. https://doi.org/10.3390/mca28010002
Reddy VS, Kandasamy J, Sivanandam S. Impacts of Casson Model on Hybrid Nanofluid Flow over a Moving Thin Needle with Dufour and Soret and Thermal Radiation Effects. Mathematical and Computational Applications. 2023; 28(1):2. https://doi.org/10.3390/mca28010002
Chicago/Turabian StyleReddy, Vinodh Srinivasa, Jagan Kandasamy, and Sivasankaran Sivanandam. 2023. "Impacts of Casson Model on Hybrid Nanofluid Flow over a Moving Thin Needle with Dufour and Soret and Thermal Radiation Effects" Mathematical and Computational Applications 28, no. 1: 2. https://doi.org/10.3390/mca28010002
APA StyleReddy, V. S., Kandasamy, J., & Sivanandam, S. (2023). Impacts of Casson Model on Hybrid Nanofluid Flow over a Moving Thin Needle with Dufour and Soret and Thermal Radiation Effects. Mathematical and Computational Applications, 28(1), 2. https://doi.org/10.3390/mca28010002







