Counteracting Bleeding in Centrifugal Partition Chromatography: Redosing of the Stationary Phase
Abstract
1. Introduction
- Hydrodynamics during redosing. Disturbances during operation should be as confined as possible to not impact the separation task. With Computational Fluid Dynamics (CFD), flow regimes during the redosing of the stationary phase become investigable. Therefore, the simulation of redosing is a suitable tool for gaining knowledge about the hydrodynamics involved.
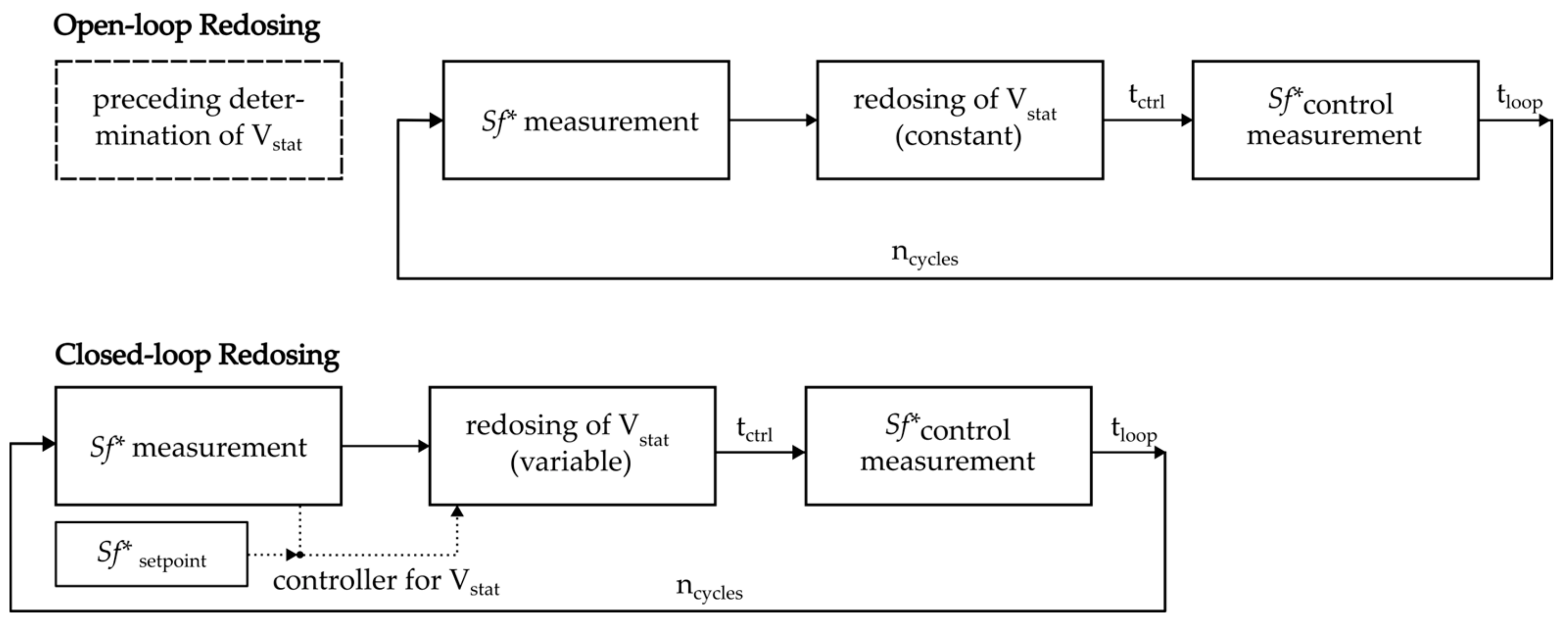
- Redosing: Experimental validation as a proof of concept. With the help of an adapted experimental setup with corresponding software implementation, the progression of retention factors over time will be investigated. Therefore, several variables must be defined: the volume of the redosed stationary phase, the interval of redosing, and the interval of retention measurements. Furthermore, a robust, precise, and reliable control system is a crucial target.
2. Materials and Methods
2.1. Computational Fluid Dynamics: Simulation of Redosing
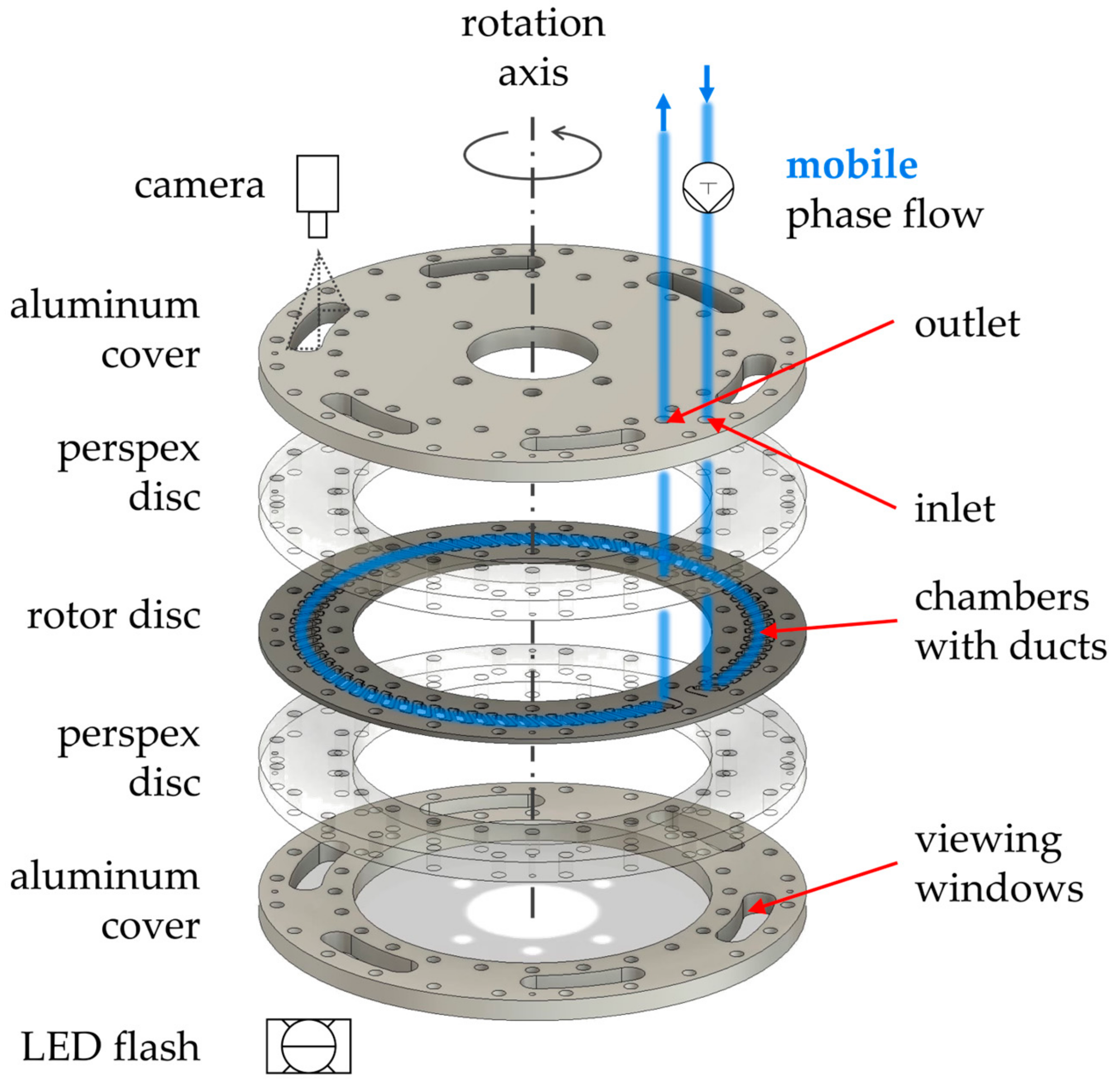
2.2. Centrifugal Partition Chromatograph
2.3. Phase System
2.4. Redosing Experiments
3. Results and Discussion
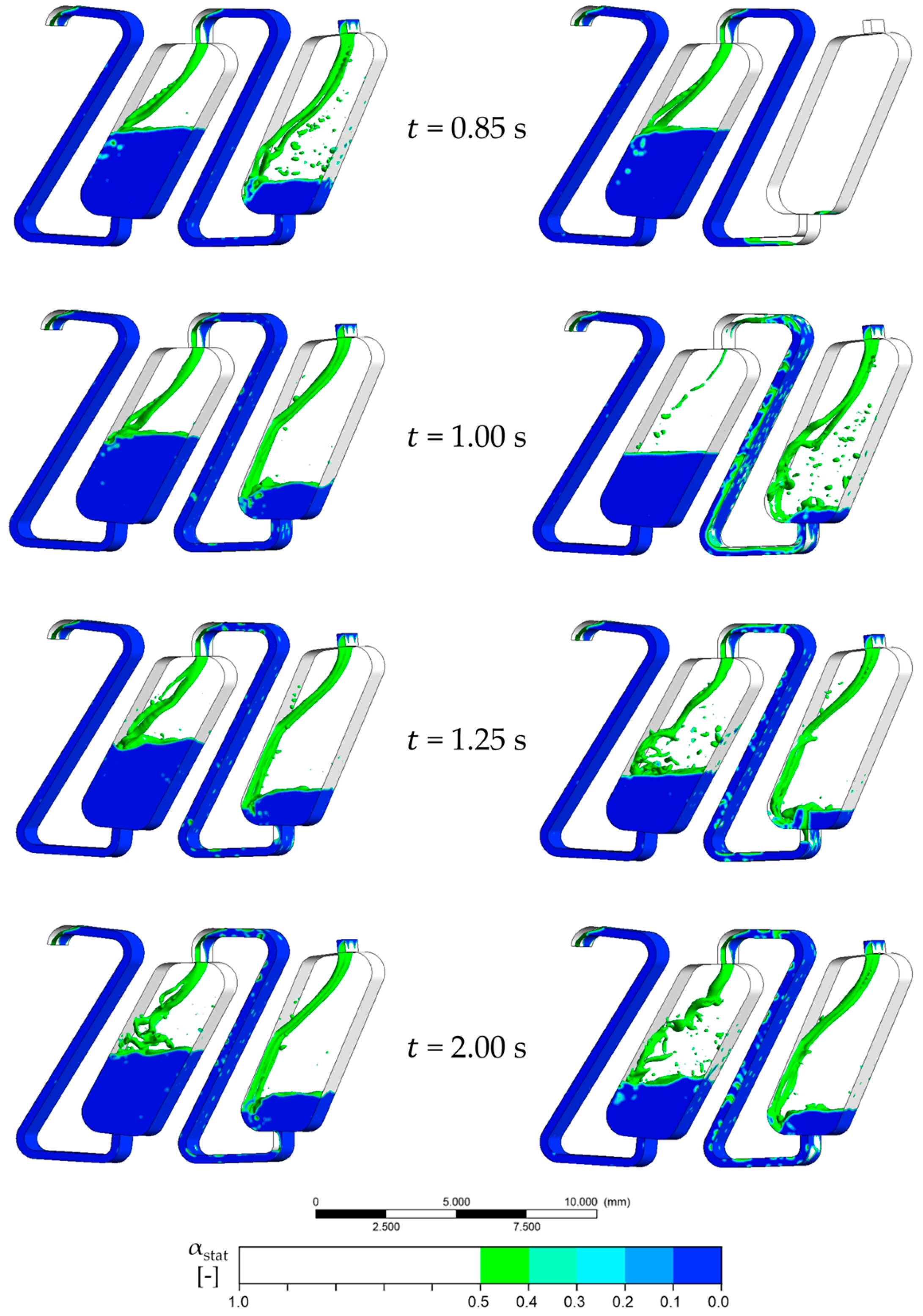
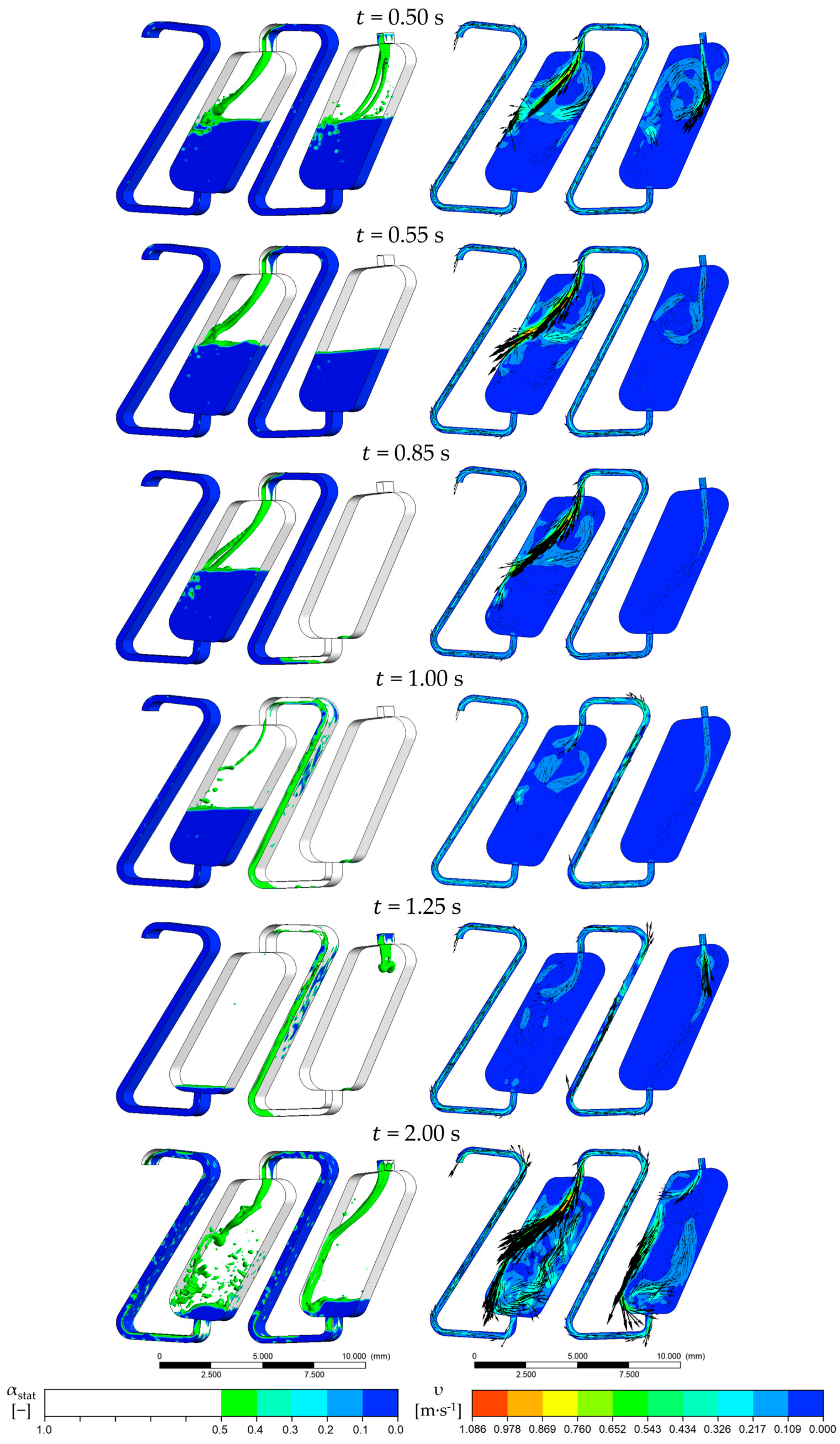
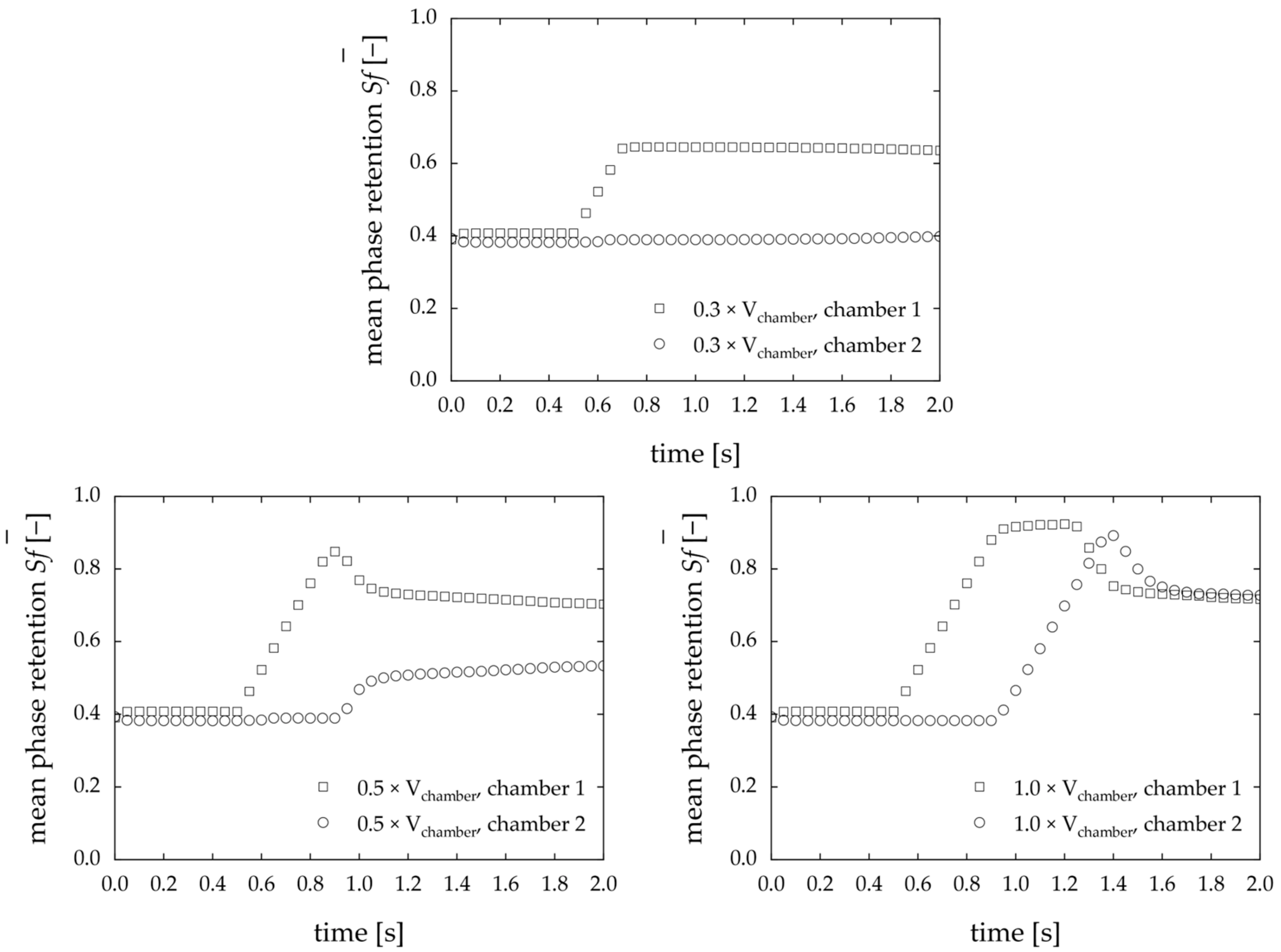
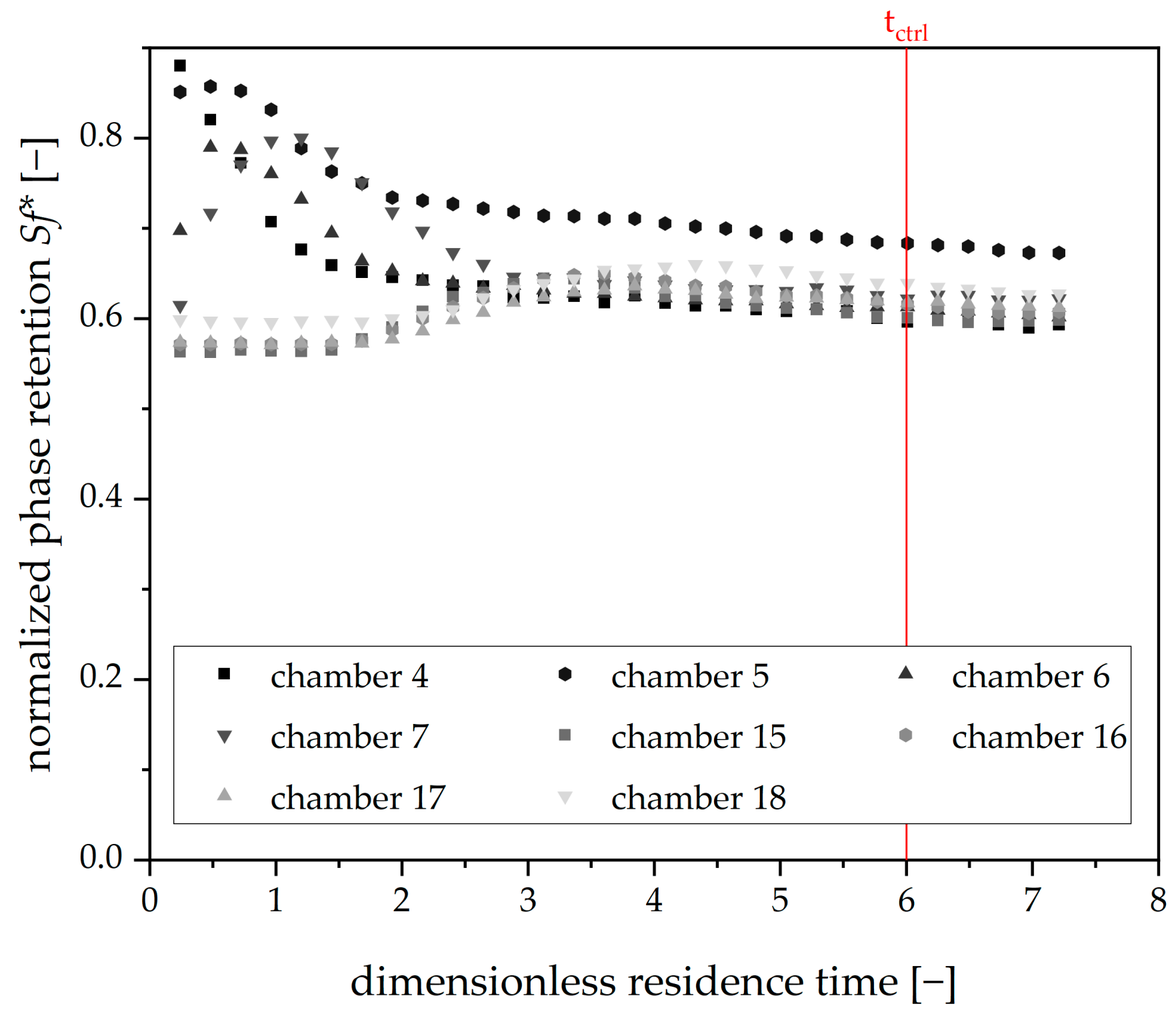
3.1. Simulation of Stationary Phase Redosing
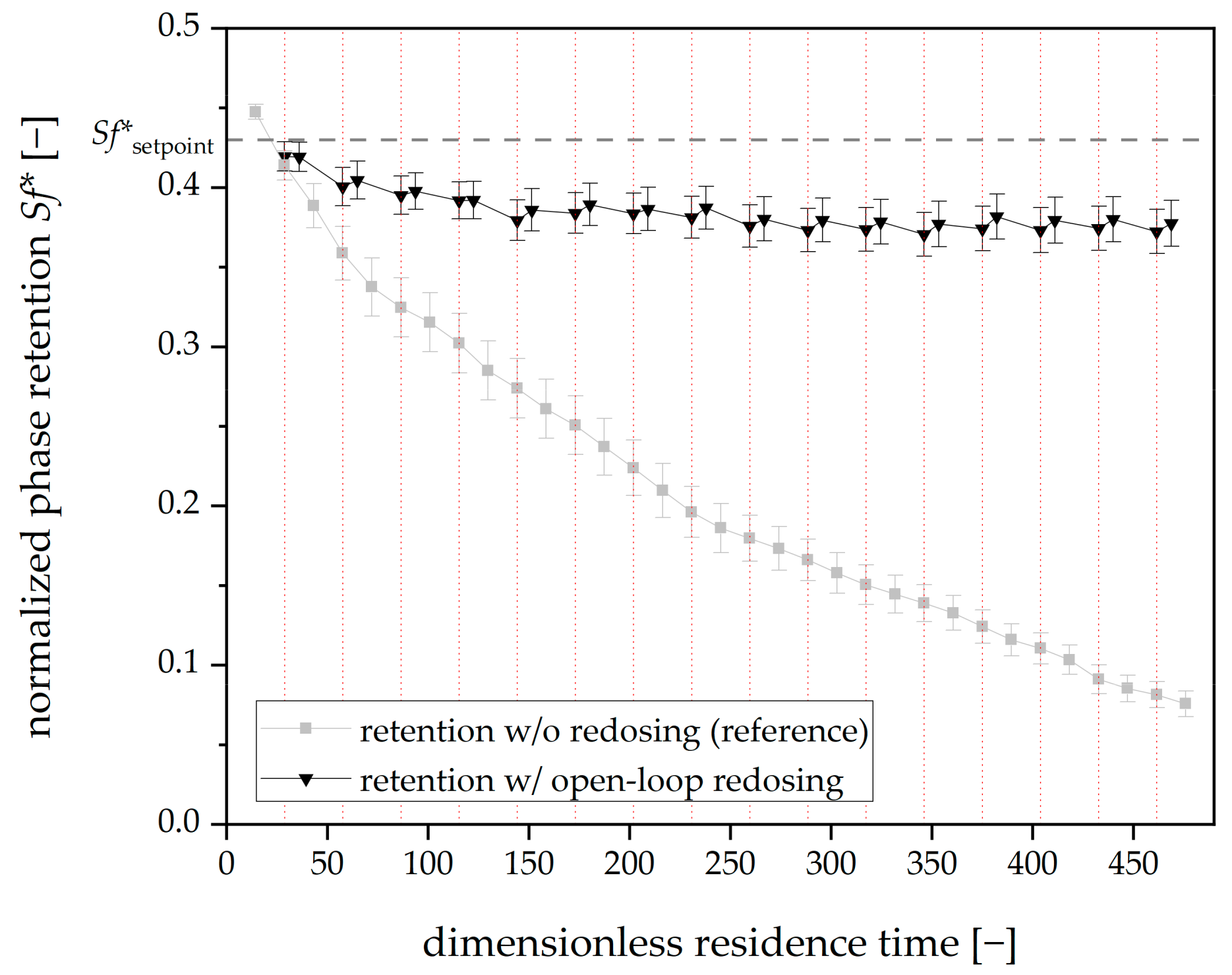
3.2. Open-Loop Redosing Experiments
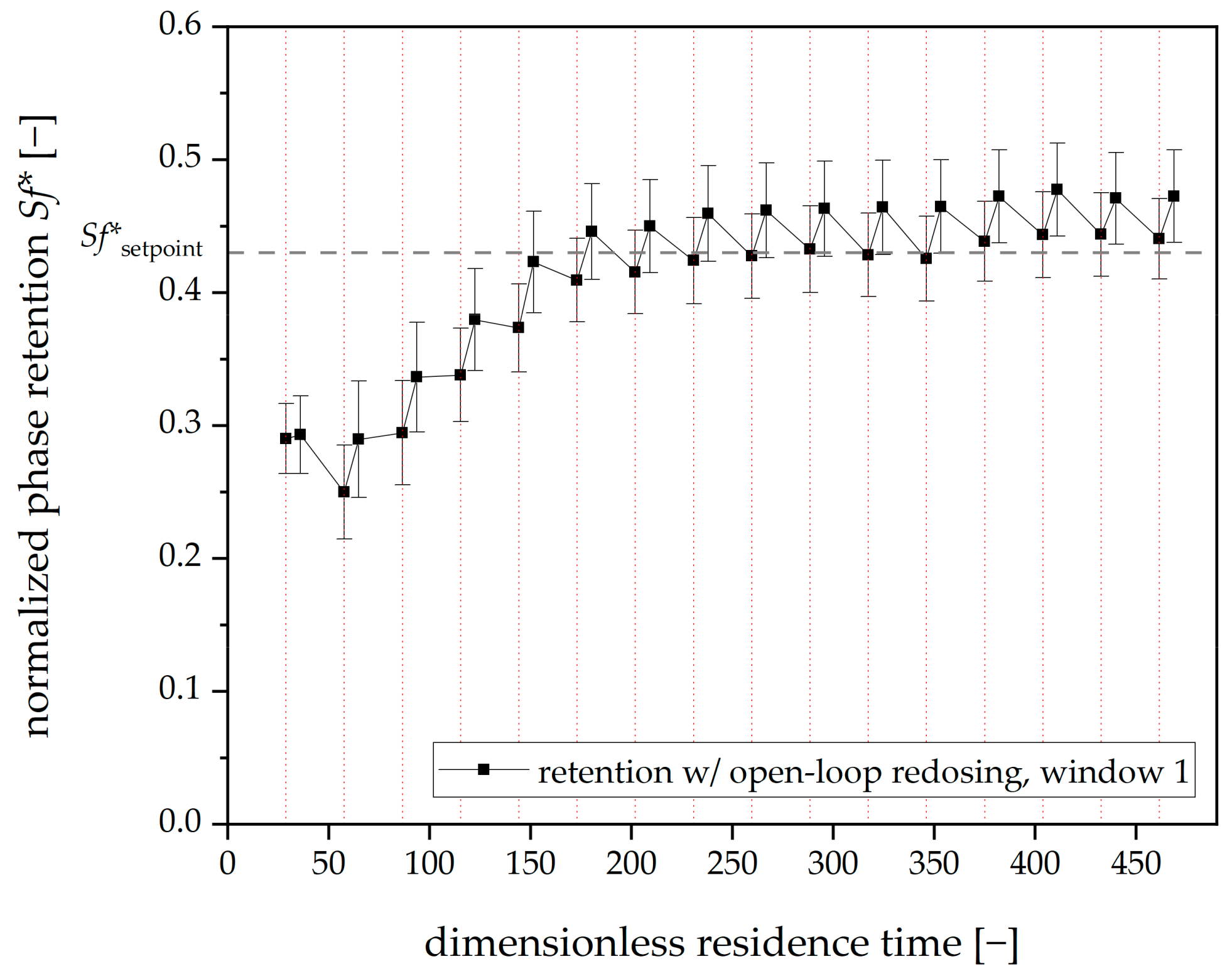
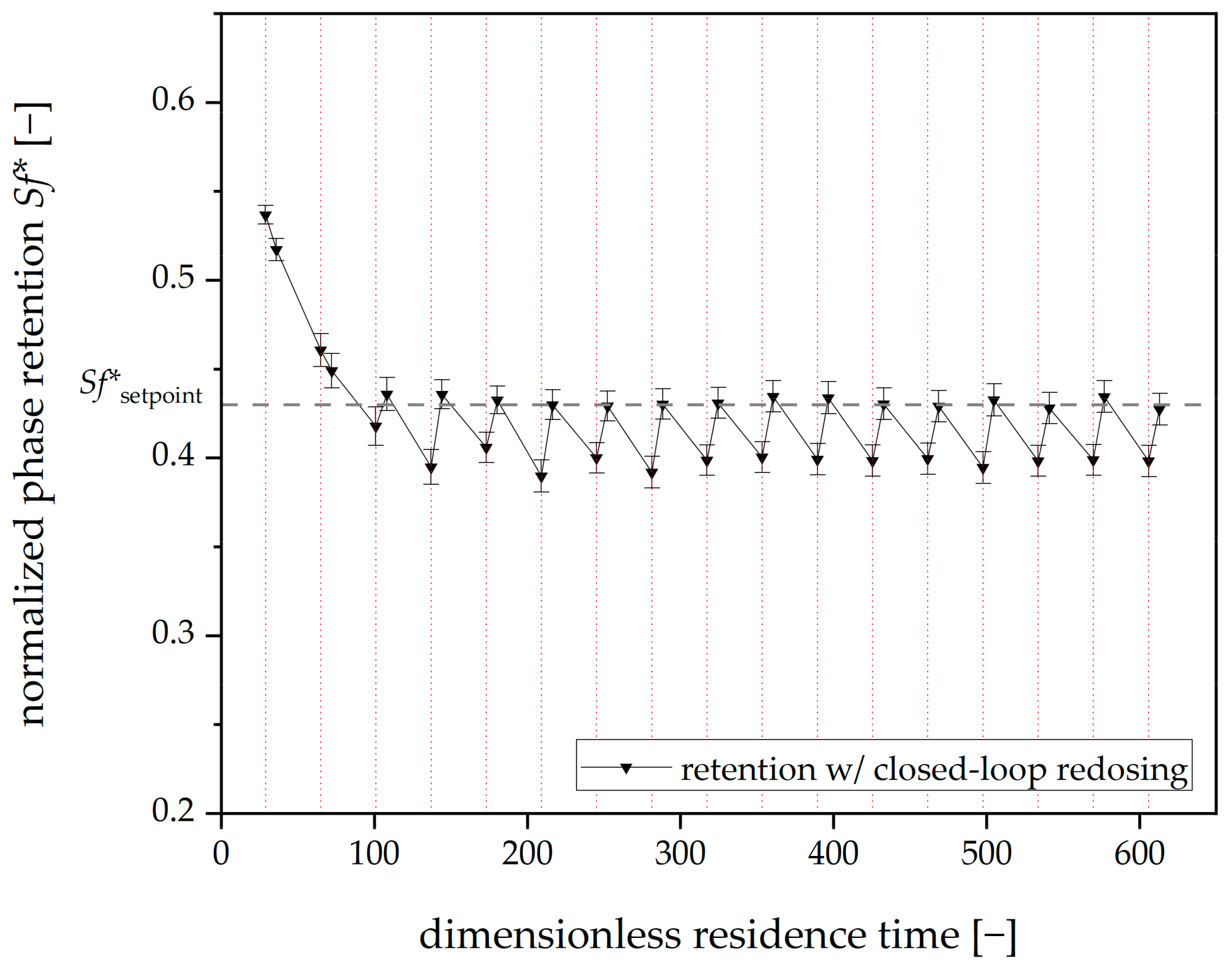
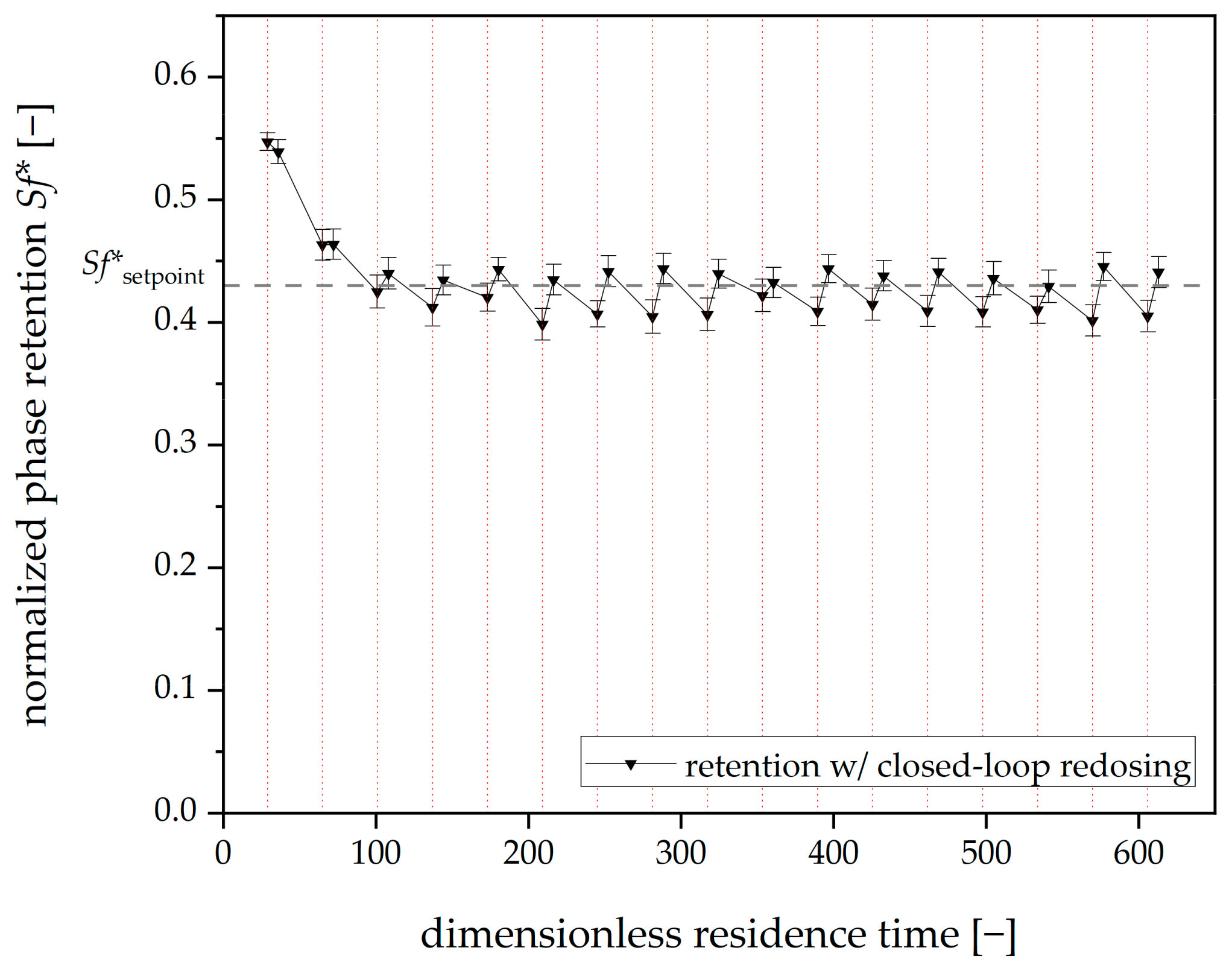
3.3. Closed-Loop Redosing Experiments
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A

References
- Marchal, L.; Legrand, J.; Foucault, A. Centrifugal partition chromatography: A survey of its history, and our recent advances in the field. Chem. Rec. 2003, 3, 133–143. [Google Scholar] [CrossRef] [PubMed]
- Chollet, S.; Marchal, L.; Jérémy, M.; Renault, J.H.; Legrand, J.; Foucault, A. Methodology for optimally sized centrifugal partition chromatography columns. J. Chromatogr. A 2015, 1388, 174–183. [Google Scholar] [CrossRef] [PubMed]
- Van Buel, M.J.; van Halsema, F.E.D.; van der Wielen, L.A.M.; Luyben, K.C.A.M. Flow regimes in centrifugal partition chromatography. AIChE J. 1998, 44, 1356–1362. [Google Scholar] [CrossRef]
- Adelmann, S. On Hydrodynamics in Centrifugal Partition Chromatography. Ph.D. Dissertation, TU Dortmund University, Dortmund, Germany, 2014. [Google Scholar]
- Buthmann, F.; Laby, P.; Hamza, D.; Koop, J.; Schembecker, G. Spatially and Temporally Resolved Analysis of Bleeding in a Centrifugal Partition Chromatography Rotor. Separations 2024, 11, 56. [Google Scholar] [CrossRef]
- Destandau, E.; Boukhris, M.A.; Zubrzycki, S.; Akssira, M.; Rhaffari, L.E.; Elfakir, C. Centrifugal partition chromatography elution gradient for isolation of sesquiterpene lactones and flavonoids from Anvillea radiata. J. Chromatogr. B 2015, 985, 29–37. [Google Scholar] [CrossRef] [PubMed]
- Fábryová, T.; Tůmová, L.; Da Silva, D.C.; Pereira, D.M.; Andrade, P.B.; Valentão, P.; Hrouzek, P.; Kopecký, J.; Cheel, J. Isolation of astaxanthin monoesters from the microalgae Haematococcus pluvialis by high performance countercurrent chromatography (HPCCC) combined with high performance liquid chromatography (HPLC). Algal Res. 2020, 49, 101947. [Google Scholar] [CrossRef]
- Bezold, F.; Roehrer, S.; Minceva, M. Ionic Liquids as Modifying Agents for Protein Separation in Centrifugal Partition Chromatography. Chem. Eng. Technol. 2019, 42, 474–482. [Google Scholar] [CrossRef]
- Krause, J.; Merz, J. Comparison of enzymatic hydrolysis in a centrifugal partition chromatograph and stirred tank reactor. J. Chromatogr. A 2017, 1504, 64–70. [Google Scholar] [CrossRef] [PubMed]
- Krause, J.F. Biocatalytic Conversions in a Centrifugal Partition Chromatograph. Ph.D. Dissertation, TU Dortmund University, Dortmund, Germany, 2017. [Google Scholar]
- Kotland, A.; Chollet, S.; Diard, C.; Autret, J.-M.; Meucci, J.; Renault, J.-H.; Marchal, L. Industrial case study on alkaloids purification by pH-zone refining centrifugal partition chromatography. J. Chromatogr. A 2016, 1474, 59–70. [Google Scholar] [CrossRef] [PubMed]
- Hopmann, E.; Minceva, M. Separation of a binary mixture by sequential centrifugal partition chromatography. J. Chromatogr. A 2012, 1229, 140–147. [Google Scholar] [CrossRef] [PubMed]
- Hopmann, E.; Goll, J.; Minceva, M. Sequential Centrifugal Partition Chromatography: A New Continuous Chromatographic Technology. Chem. Eng. Technol. 2012, 35, 72–82. [Google Scholar] [CrossRef]
- Goll, J.; Minceva, M. Continuous fractionation of multicomponent mixtures with sequential centrifugal partition chromatography. AIChE J. 2017, 63, 1659–1673. [Google Scholar] [CrossRef]
- Goll, J.; Morley, R.; Minceva, M. Trapping multiple dual mode centrifugal partition chromatography for the separation of intermediately-eluting components: Operating parameter selection. J. Chromatogr. A 2017, 1496, 68–79. [Google Scholar] [CrossRef] [PubMed]
- Morley, R.; Minceva, M. Trapping multiple dual mode centrifugal partition chromatography for the separation of intermediately-eluting components: Throughput maximization strategy. J. Chromatogr. A 2017, 1501, 26–38. [Google Scholar] [CrossRef] [PubMed]
- Schembecker, G.; Adelmann, S.; Schwienheer, C. Extractor for Centrifugal Partition Extraction and Method for Performing Centrifugal Partition Extraction. WO Patent 2012EP68999, 26 September 2012. [Google Scholar]
- Schwienheer, C. Advances in Centrifugal Purification Techniques for Separating (Bio-)chemical Compounds. Ph.D. Dissertation, TU Dortmund University, Dortmund, Germany, 2016. [Google Scholar]
- Buthmann, F.; Volpert, S.; Koop, J.; Schembecker, G. Prediction of Bleeding via Simulation of Hydrodynamics in Centrifugal Partition Chromatography. Separations 2024, 11, 16. [Google Scholar] [CrossRef]
- Ansys®. Fluent. 2021. Available online: https://forum.ansys.com/uploads/846/SCJEU0NN8IHX.pdf (accessed on 8 January 2024).
- Oka, F.; Oka, H.; Ito, Y. Systematic search for suitable two-phase solvent systems for high-speed counter-current chromatography. J. Chromatogr. A 1991, 538, 99–108. [Google Scholar] [CrossRef] [PubMed]
- Faure, K.; Bouju, E.; Doby, J.; Berthod, A. Limonene in Arizona liquid systems used in countercurrent chromatography. II Polarity and stationary-phase retention. Anal. Bioanal. Chem. 2014, 406, 5919–5926. [Google Scholar] [CrossRef] [PubMed]
- Berthod, A.; Hassoun, M.; Ruiz-Angel, M.J. Alkane effect in the Arizona liquid systems used in countercurrent chromatography. Anal. Bioanal. Chem. 2005, 383, 327–340. [Google Scholar] [CrossRef] [PubMed]
- Buthmann, F.; Pley, F.; Schembecker, G.; Koop, J. Automated Image Analysis for Retention Determination in Centrifugal Partition Chromatography. Separations 2022, 9, 358. [Google Scholar] [CrossRef]
- Schwarze, R. CFD-Modellierung: Grundlagen und Anwendungen bei Strömungsprozessen; Springer: Berlin/Heidelberg, Germany, 2012; ISBN 978-3-642-24378-3. [Google Scholar]
- Berthod, A. (Ed.) Countercurrent Chromatography: The Support-Free Liquid Stationary Phase; Elsevier: Amsterdam, The Netherlands, 2002; ISBN 9780444507372. [Google Scholar]
- Cushman-Roisin, B. Introduction to Geophysical Fluid Dynamics: Physical and Numerical Aspects, 2nd ed.; Academic Press: Waltham, MA, USA, 2011; ISBN 9780080916781. [Google Scholar]
- CFD in der Verfahrenstechnik: Allgemeine Grundlagen und mehrphasige Anwendungen; Wiley-VCH: Weinheim, Germany, 2005; ISBN 9783527603855.
- Lomax, H.; Pulliam, T.H.; Zingg, D.W.; Kowalewski, T.A. Fundamentals of Computational Fluid Dynamics. Appl. Mech. Rev. 2002, 55, B61. [Google Scholar] [CrossRef]
- Fromme, A. Systematic Approach towards Solvent System Selection for Ideal Fluid Dynamics in Centrifugal Partition Chromatography. Ph.D. Dissertation, TU Dortmund University, Dortmund, Germany, 2020. [Google Scholar]




Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Buthmann, F.; Hohlmann, J.; Volpert, S.; Neuwald, M.; Hamza, D.; Schembecker, G. Counteracting Bleeding in Centrifugal Partition Chromatography: Redosing of the Stationary Phase. Separations 2024, 11, 98. https://doi.org/10.3390/separations11040098
Buthmann F, Hohlmann J, Volpert S, Neuwald M, Hamza D, Schembecker G. Counteracting Bleeding in Centrifugal Partition Chromatography: Redosing of the Stationary Phase. Separations. 2024; 11(4):98. https://doi.org/10.3390/separations11040098
Chicago/Turabian StyleButhmann, Felix, Jan Hohlmann, Sophia Volpert, Mareen Neuwald, Djamal Hamza, and Gerhard Schembecker. 2024. "Counteracting Bleeding in Centrifugal Partition Chromatography: Redosing of the Stationary Phase" Separations 11, no. 4: 98. https://doi.org/10.3390/separations11040098
APA StyleButhmann, F., Hohlmann, J., Volpert, S., Neuwald, M., Hamza, D., & Schembecker, G. (2024). Counteracting Bleeding in Centrifugal Partition Chromatography: Redosing of the Stationary Phase. Separations, 11(4), 98. https://doi.org/10.3390/separations11040098





