RETRACTED: Finite Element Study of Magnetohydrodynamics (MHD) and Activation Energy in Darcy–Forchheimer Rotating Flow of Casson Carreau Nanofluid
Abstract
1. Introduction
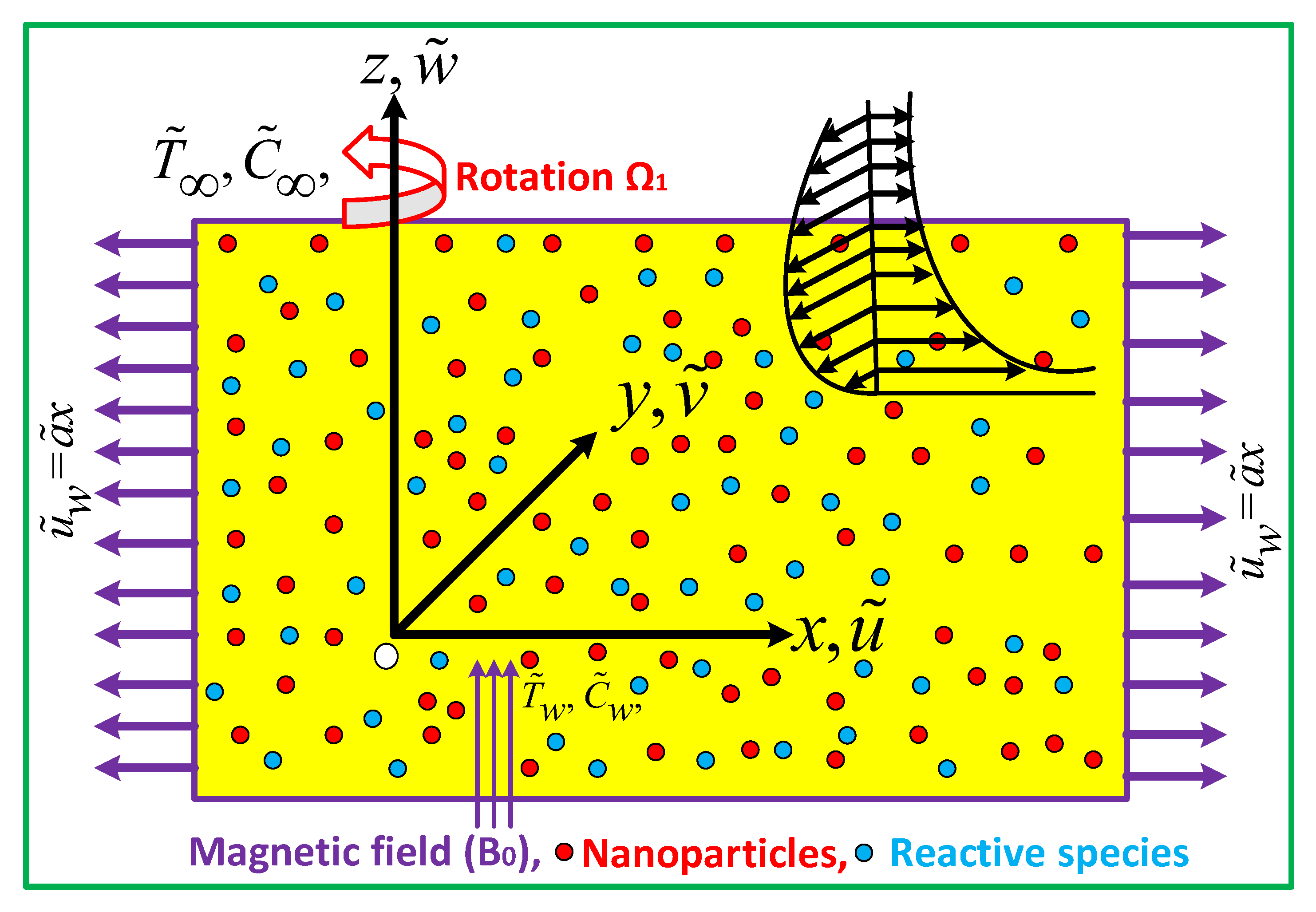
2. Statement of The Problem
3. Governing Equations
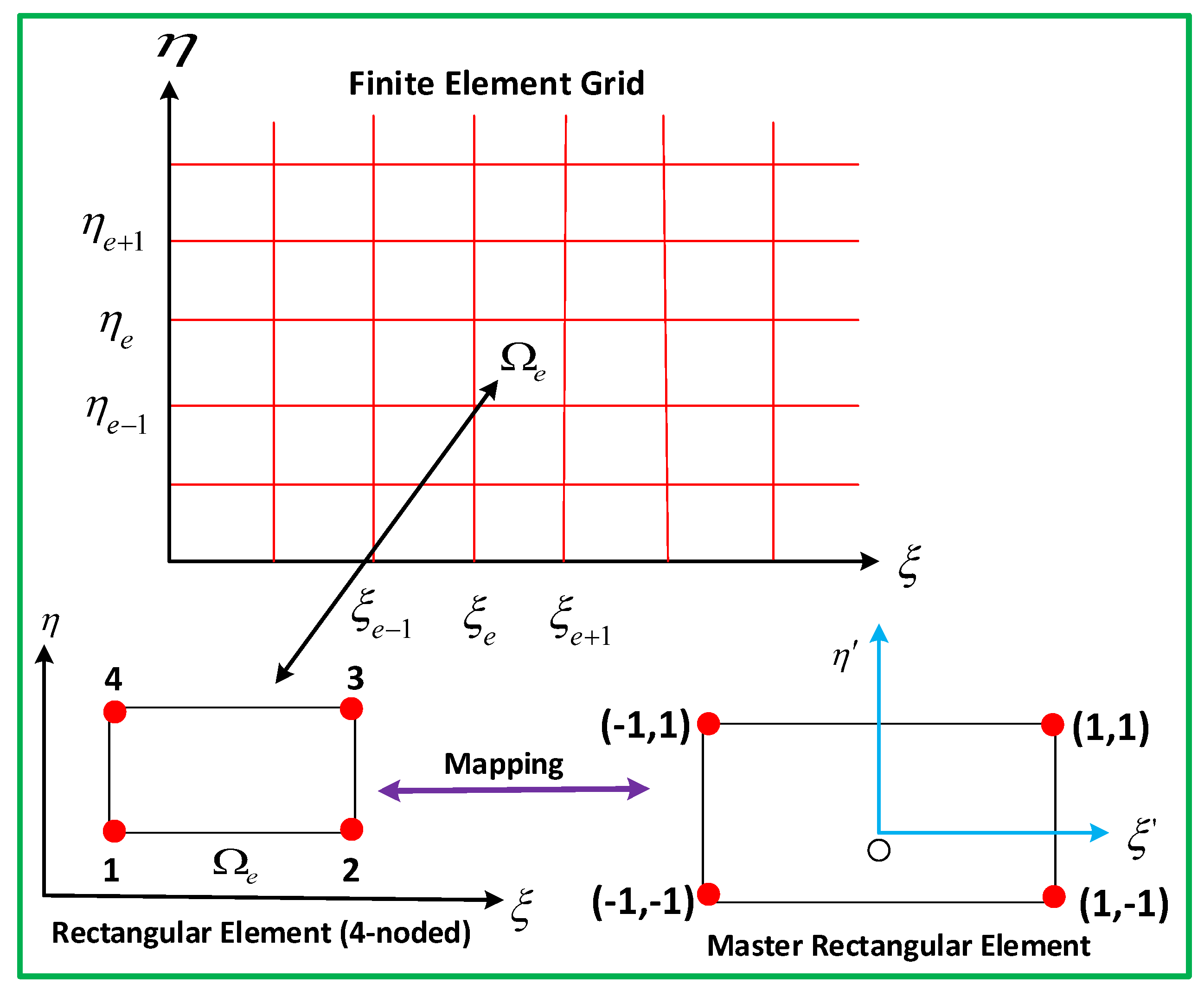
4. Finite Element Method Solutions
4.1. Variational Formulations
4.2. Finite Element Formulations
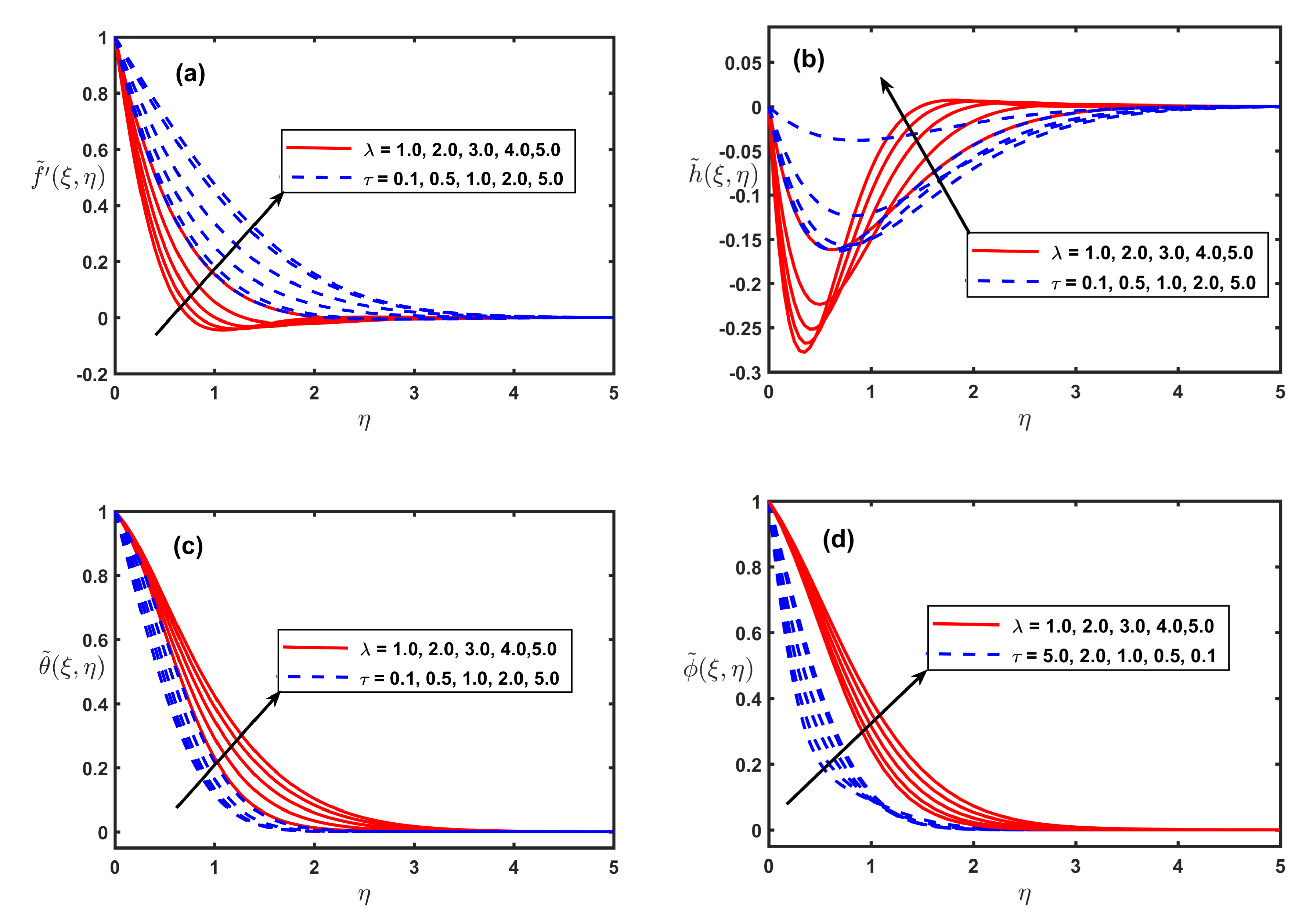
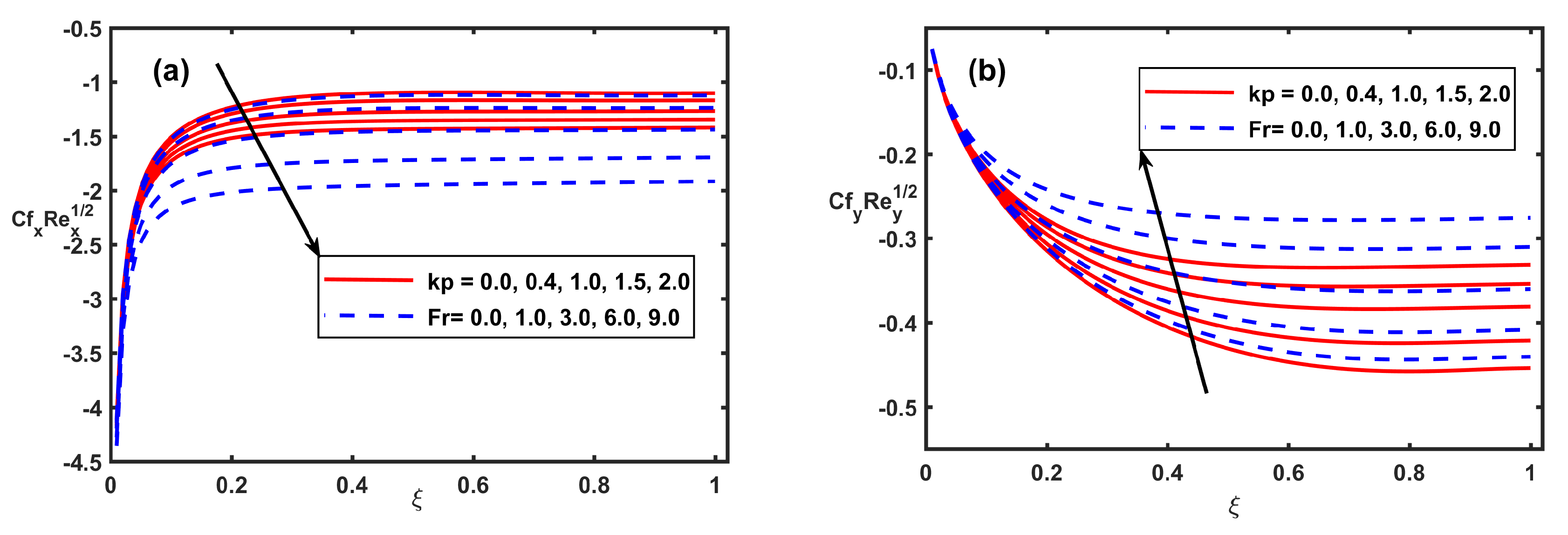
5. Results and Discussion
6. Conclusions
- The progressing values of Casson fluid parameter , magnetic parameter M, porosity , Forchheimer number (Fr), and Weissenberg number (We) reduced the magnitude of secondary velocity and the primary velocity but concentration and temperature are incremented.
- The concentration and temperature are incremented along with rising values of . The both components of velocity diminishes near the surface when is incremented.
- Improvement in temperature is reported when Nb, Nt, and are increased but inverse behaviour is noted for power-law index ().
- The higher values of Nb, power-law index (), and chemical reaction rate parameter recede the nanoparticle concentration but it increases against higher values of Nt and EE.
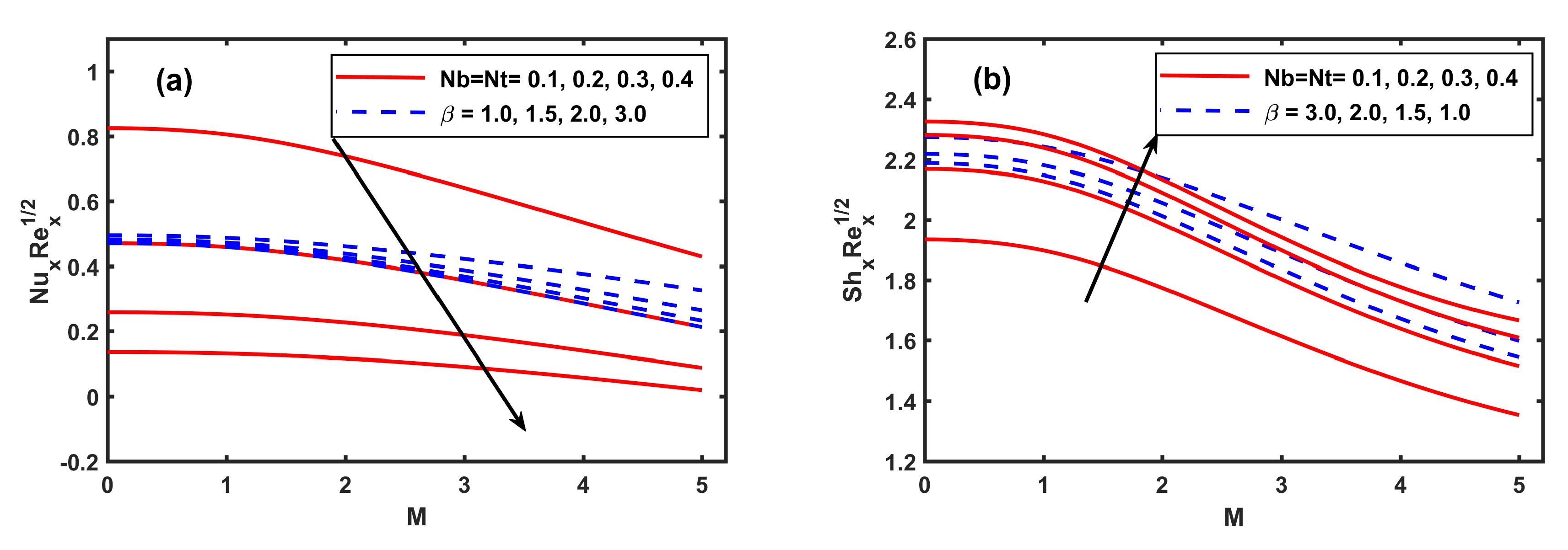
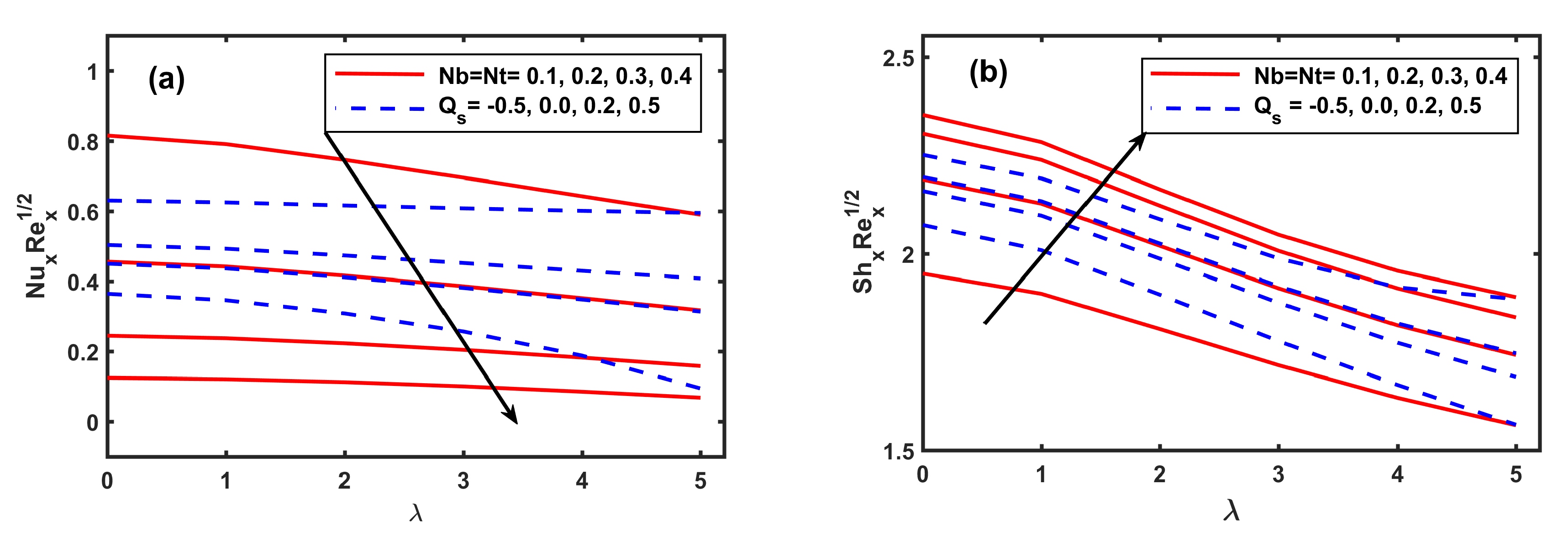
- The Nusselt number attains lower values for higher values of Casson fluid parameter , Brownian (Nb), thermophoresis (Nt), magnetic (M), heat source , and rotating ().
- The Sherwood number attains higher values for higher values of Lewis number (Le), Nb, and but the reduced Sherwood number exhibits an opposite trend for magnetic, rotational, porosity , Forchheimer number (Fr), and Casson fluid parameter parameters.
Author Contributions
Funding
Conflicts of Interest
References
- Choi, S.U.; Eastman, J.A. Enhancing Thermal Conductivity of Fluids with Nanoparticles; Technical Report; Argonne National Lab: Lemont, IL, USA, 1995. [Google Scholar]
- Makinde, O.D.; Aziz, A. Boundary layer flow of a nanofluid past a stretching sheet with a convective boundary condition. Int. J. Therm. Sci. 2011, 50, 1326–1332. [Google Scholar] [CrossRef]
- Sarafraz, M.; Arya, H.; Saeedi, M.; Ahmadi, D. Flow boiling heat transfer to MgO-therminol 66 heat transfer fluid: Experimental assessment and correlation development. Appl. Therm. Eng. 2018, 138, 552–562. [Google Scholar] [CrossRef]
- Uddin, M.J.; Khan, W.; Ismail, A.M. Free convection boundary layer flow from a heated upward facing horizontal flat plate embedded in a porous medium filled by a nanofluid with convective boundary condition. TRansport Porous Media 2012, 92, 867–881. [Google Scholar] [CrossRef]
- Khan, W.; Pop, I. Boundary-layer flow of a nanofluid past a stretching sheet. Int. J. Heat Mass Transf. 2010, 53, 2477–2483. [Google Scholar] [CrossRef]
- Turkyilmazoglu, M. Condensation of laminar film over curved vertical walls using single and two-phase nanofluid models. Eur. J. Mech. B Fluids 2017, 65, 184–191. [Google Scholar] [CrossRef]
- Mahanthesh, B.; Gireesha, B.J.; Thammanna, G.T.; Shehzad, S.A.; Abbasi, F.M.; Gorla, R.S.R. Nonlinear convection in nano Maxwell fluid with nonlinear thermal radiation: A three-dimensional study. Alex. Eng. J. 2018, 57, 1927–1935. [Google Scholar] [CrossRef]
- Shahzad, A.; Ali, R.; Hussain, M.; Kamran, M. Unsteady axisymmetric flow and heat transfer over time-dependent radially stretching sheet. Alex. Eng. J. 2017, 56, 35–41. [Google Scholar] [CrossRef]
- Nandy, S.K.; Mahapatra, T.R. Effects of slip and heat generation/absorption on MHD stagnation flow of nanofluid past a stretching/shrinking surface with convective boundary conditions. Int. J. Heat Mass Transf. 2013, 64, 1091–1100. [Google Scholar] [CrossRef]
- Rashid, S.; Hayat, T.; Qayyum, S.; Ayub, M.; Alsaedi, A. Three-dimensional rotating Darcy–Forchheimer flow with activation energy. Int. J. Numer. Methods Heat Fluid Flow 2019, 29, 935–948. [Google Scholar] [CrossRef]
- Hayat, T.; Ijaz, M.; Qayyum, S.; Ayub, M.; Alsaedi, A. Mixed convective stagnation point flow of nanofluid with Darcy-Fochheimer relation and partial slip. Results Phys. 2018, 9, 771–778. [Google Scholar] [CrossRef]
- Muhammad, R.; Khan, M.I.; Jameel, M.; Khan, N.B. Fully developed Darcy–Forchheimer mixed convective flow over a curved surface with activation energy and entropy generation. Comput. Methods Programs Biomed. 2020, 188, 105298. [Google Scholar] [CrossRef] [PubMed]
- Gireesha, B.; Mahanthesh, B.; Thammanna, G.; Sampathkumar, P. Hall effects on dusty nanofluid two-phase transient flow past a stretching sheet using KVL model. J. Mol. Liq. 2018, 256, 139–147. [Google Scholar] [CrossRef]
- Hayat, T.; Aziz, A.; Muhammad, T.; Alsaedi, A. Effects of binary chemical reaction and Arrhenius activation energy in Darcy–Forchheimer three-dimensional flow of nanofluid subject to rotating frame. J. Therm. Anal. Calorim. 2019, 136, 1769–1779. [Google Scholar] [CrossRef]
- Tiwana, M.H.; Mann, A.B.; Rizwan, M.; Maqbool, K.; Javeed, S.; Raza, S.; Khan, M.S. Unsteady Magnetohydrodynamic Convective Fluid Flow of Oldroyd-B Model Considering Ramped Wall Temperature and Ramped Wall Velocity. Mathematics 2019, 7, 676. [Google Scholar] [CrossRef]
- Naz, R.; Noor, M.; Hayat, T.; Javed, M.; Alsaedi, A. Dynamism of magnetohydrodynamic cross nanofluid with particulars of entropy generation and gyrotactic motile microorganisms. Int. Commun. Heat Mass Transf. 2020, 110, 104431. [Google Scholar] [CrossRef]
- Khan, M.; Salahuddin, T.; Yousaf, M.M.; Khan, F.; Hussain, A. Variable diffusion and conductivity change in 3d rotating Williamson fluid flow along with magnetic field and activation energy. Int. J. Numer. Methods Heat Fluid Flow 2019, 30, 2467–2484. [Google Scholar] [CrossRef]
- Alarifi, I.M.; Abokhalil, A.G.; Osman, M.; Lund, L.A.; Ayed, M.B.; Belmabrouk, H.; Tlili, I. MHD flow and heat transfer over vertical stretching sheet with heat sink or source effect. Symmetry 2019, 11, 297. [Google Scholar] [CrossRef]
- Thumma, T.; Wakif, A.; Animasaun, I.L. Generalized differential quadrature analysis of unsteady three-dimensional MHD radiating dissipative Casson fluid conveying tiny particles. Heat Transf. 2020, 49, 2595–2626. [Google Scholar] [CrossRef]
- Tlili, I.; Bilal, M.; Qureshi, M.Z.A.; Abdelmalek, Z. Thermal analysis of magnetized pseudoplastic nano fluid flow over 3D radiating non-linear surface with passive mass flux control and chemically responsive species. J. Mater. Res. Technol. 2020, 9, 8125–8135. [Google Scholar] [CrossRef]
- Khan, S.A.; Nie, Y.; Ali, B. Multiple slip effects on magnetohydrodynamic axisymmetric buoyant nanofluid flow above a stretching sheet with radiation and chemical reaction. Symmetry 2019, 11, 1171. [Google Scholar] [CrossRef]
- Aziz, A.; Muhammad, T.; Alsaedi, A.; Hayat, T. An optimal study for 3D rotating flow of Oldroyd-B nanofluid with convectively heated surface. J. Braz. Soc. Mech. Sci. Eng. 2019, 41, 236. [Google Scholar] [CrossRef]
- Mustafa, M.; Ahmad, R.; Hayat, T.; Alsaedi, A. Rotating flow of viscoelastic fluid with nonlinear thermal radiation: A numerical study. Neural Comput. Appl. 2018, 29, 493–499. [Google Scholar] [CrossRef]
- Wang, C. Stretching a surface in a rotating fluid. Z. Angew. Math. Phys. 1988, 39, 177–185. [Google Scholar] [CrossRef]
- Takhar, H.S.; Chamkha, A.J.; Nath, G. Flow and heat transfer on a stretching surface in a rotating fluid with a magnetic field. Int. J. Therm. Sci. 2003, 42, 23–31. [Google Scholar] [CrossRef]
- Ibrahim, W. Three dimensional rotating flow of Powell-Eyring nanofluid with non-Fourier’s heat flux and non-Fick’s mass flux theory. Results Phys. 2018, 8, 569–577. [Google Scholar] [CrossRef]
- Jusoh, R.; Nazar, R.; Pop, I. Magnetohydrodynamic rotating flow and heat transfer of ferrofluid due to an exponentially permeable stretching/shrinking sheet. J. Magn. Magn. Mater. 2018, 465, 365–374. [Google Scholar] [CrossRef]
- Jawad, M.; Shah, Z.; Islam, S.; Majdoubi, J.; Tlili, I.; Khan, W.; Khan, I. Impact of nonlinear thermal radiation and the viscous dissipation effect on the unsteady three-dimensional rotating flow of single-wall carbon nanotubes with aqueous suspensions. Symmetry 2019, 11, 207. [Google Scholar] [CrossRef]
- Christov, C. On frame indifferent formulation of the Maxwell–Cattaneo model of finite-speed heat conduction. Mech. Res. Commun. 2009, 36, 481–486. [Google Scholar] [CrossRef]
- Khan, M.I.; Alzahrani, F.; Hobiny, A.; Ali, Z. Modeling of Cattaneo-Christov double diffusions (CCDD) in Williamson nanomaterial slip flow subject to porous medium. J. Mater. Res. Technol. 2020, 9, 6172–6177. [Google Scholar] [CrossRef]
- Hayat, T.; Khan, M.I.; Farooq, M.; Alsaedi, A.; Waqas, M.; Yasmeen, T. Impact of Cattaneo–Christov heat flux model in flow of variable thermal conductivity fluid over a variable thicked surface. Int. J. Heat Mass Transf. 2016, 99, 702–710. [Google Scholar] [CrossRef]
- Rana, P.; Bhargava, R.; Bég, O.A. Finite element simulation of unsteady magneto-hydrodynamic transport phenomena on a stretching sheet in a rotating nanofluid. Proc. Inst. Mech. Eng. Part N J. Nanoeng. Nanosyst. 2013, 227, 77–99. [Google Scholar] [CrossRef]
- Raju, C.; Sandeep, N. Unsteady three-dimensional flow of Casson–Carreau fluids past a stretching surface. Alex. Eng. J. 2016, 55, 1115–1126. [Google Scholar] [CrossRef]
- Abbas, Z.; Javed, T.; Sajid, M.; Ali, N. Unsteady MHD flow and heat transfer on a stretching sheet in a rotating fluid. J. Taiwan Inst. Chem. Eng. 2010, 41, 644–650. [Google Scholar] [CrossRef]
- Babu, M.J.; Sandeep, N. 3D MHD slip flow of a nanofluid over a slendering stretching sheet with thermophoresis and Brownian motion effects. J. Mol. Liq. 2016, 222, 1003–1009. [Google Scholar] [CrossRef]
- Hayat, T.; Muhammad, T.; Alsaedi, A. On three-dimensional flow of couple stress fluid with Cattaneo–Christov heat flux. Chin. J. Phys. 2017, 55, 930–938. [Google Scholar] [CrossRef]
- Butt, A.S.; Ali, A.; Mehmood, A. Study of flow and heat transfer on a stretching surface in a rotating Casson fluid. Proc. Natl. Acad. Sci. India Sect. Phys. Sci. 2015, 85, 421–426. [Google Scholar] [CrossRef]
- Rudraswamy, N.; Shehzad, S.; Kumar, K.G.; Gireesha, B. Numerical analysis of MHD three-dimensional Carreau nanoliquid flow over bidirectionally moving surface. J. Braz. Soc. Mech. Sci. Eng. 2017, 39, 5037–5047. [Google Scholar] [CrossRef]
- Ramaiah K, D.; Kotha, G.; Thangavelu, K. MHD rotating flow of a Maxwell fluid with Arrhenius activation energy and non-Fourier heat flux model. Heat Transf. 2020, 49, 2209–2227. [Google Scholar] [CrossRef]
- Nazar, R.; Amin, N.; Pop, I. Unsteady boundary layer flow due to a stretching surface in a rotating fluid. Mech. Res. Commun. 2004, 31, 121–128. [Google Scholar] [CrossRef]
- Shafique, Z.; Mustafa, M.; Mushtaq, A. Boundary layer flow of Maxwell fluid in rotating frame with binary chemical reaction and activation energy. Results Phys. 2016, 6, 627–633. [Google Scholar] [CrossRef]
- Uddin, M.; Rana, P.; Bég, O.A.; Ismail, A.M. Finite element simulation of magnetohydrodynamic convective nanofluid slip flow in porous media with nonlinear radiation. Alex. Eng. J. 2016, 55, 1305–1319. [Google Scholar] [CrossRef]
- Rana, P.; Bhargava, R. Numerical study of heat transfer enhancement in mixed convection flow along a vertical plate with heat source/sink utilizing nanofluids. Commun. Nonlinear Sci. Numer. Simul. 2011, 16, 4318–4334. [Google Scholar] [CrossRef]
- Reddy, J.N. Solutions Manual for an Introduction to the Finite Element Method; McGraw-Hill: New York, NY, USA, 1993; p. 41. [Google Scholar]
- Jyothi, K.; Reddy, P.S.; Reddy, M.S. Carreau nanofluid heat and mass transfer flow through wedge with slip conditions and nonlinear thermal radiation. J. Braz. Soc. Mech. Sci. Eng. 2019, 41, 415. [Google Scholar] [CrossRef]
- Reddy, G.J.; Raju, R.S.; Rao, J.A. Influence of viscous dissipation on unsteady MHD natural convective flow of Casson fluid over an oscillating vertical plate via FEM. Ain Shams Eng. J. 2018, 9, 1907–1915. [Google Scholar] [CrossRef]
- Ali, B.; Yu, X.; Sadiq, M.T.; Rehman, A.U.; Ali, L. A Finite Element Simulation of the Active and Passive Controls of the MHD Effect on an Axisymmetric Nanofluid Flow with Thermo-Diffusion over a Radially Stretched Sheet. Processes 2020, 8, 207. [Google Scholar] [CrossRef]







| kp | Fr | Hayat et al. [14] | FEM (Our Results) | |||
|---|---|---|---|---|---|---|
| 0.0 | 0.1 | 0.2 | −1.06329 | −0.23769 | −1.063285 | −0.237676 |
| 0.1 | −1.10532 | −0.22319 | −1.105309 | −0.223185 | ||
| 0.2 | −1.14675 | −0.21087 | −1.146743 | −0.210873 | ||
| 0.2 | 0.1 | 0.2 | −1.14675 | −0.21087 | −1.146743 | −0.210873 |
| 0.2 | −1.17424 | −0.20994 | −1.174241 | −0.209930 | ||
| 0.3 | −1.20121 | −0.20905 | −1.201216 | −0.209058 | ||
| 0.2 | 0.1 | 0.05 | −1.12589 | −0.05425 | −1.125878 | −0.054246 |
| 0.1 | −1.13024 | −0.10786 | −1.130254 | −0.107849 | ||
| 0.2 | −1.14675 | −0.21087 | −1.146759 | −0.210873 | ||
| kp | Fr | Pr | Rashid et al. [10] | FEM (Our Results) | |
|---|---|---|---|---|---|
| 0.0 | 0.5 | 1.0 | 1.0 | 0.508972 | 0.508969 |
| 1.0 | 0.485852 | 0.485847 | |||
| 2.0 | 0.457520 | 0.457459 | |||
| 0.2 | 0.1 | 1.0 | 1.0 | 0.542198 | 0.542187 |
| 0.5 | 0.506965 | 0.506956 | |||
| 0.9 | 0.467794 | 0.467790 | |||
| 0.2 | 0.5 | 0.0 | 2.0 | 0.844615 | 0.844608 |
| 2.0 | 0.783173 | 0.783165 | |||
| 3.0 | 0.734272 | 0.734277 | |||
| 0.2 | 0.5 | 1.0 | 2.0 | 0.811336 | 0.811327 |
| 3.0 | 1.064610 | 1.064582 | |||
| 4.0 | 1.279790 | 1.279804 |
| Abbas et al. [34] | FEM (Our results) | |||||
|---|---|---|---|---|---|---|
| M = 0.5 | M = 1.0 | M = 2.0 | M = 0.5 | M = 1.0 | M = 2.0 | |
| 0.0 | 0.886 | 0.823 | 0.668 | 0.8862 | 0.8230 | 0.6682 |
| 0.5 | 0.841 | 0.800 | 0.663 | 0.8408 | 0.8003 | 0.6627 |
| 1.0 | 0.768 | 0.750 | 0.648 | 0.7684 | 0.7501 | 0.6483 |
| 2.0 | 0.641 | 0.643 | 0.603 | 0.6411 | 0.6429 | 0.6030 |
| 5.0 | 0.447 | 0.449 | 0.461 | 0.4467 | 0.4494 | 0.4612 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ali, B.; Rasool, G.; Hussain, S.; Baleanu, D.; Bano, S. RETRACTED: Finite Element Study of Magnetohydrodynamics (MHD) and Activation Energy in Darcy–Forchheimer Rotating Flow of Casson Carreau Nanofluid. Processes 2020, 8, 1185. https://doi.org/10.3390/pr8091185
Ali B, Rasool G, Hussain S, Baleanu D, Bano S. RETRACTED: Finite Element Study of Magnetohydrodynamics (MHD) and Activation Energy in Darcy–Forchheimer Rotating Flow of Casson Carreau Nanofluid. Processes. 2020; 8(9):1185. https://doi.org/10.3390/pr8091185
Chicago/Turabian StyleAli, Bagh, Ghulam Rasool, Sajjad Hussain, Dumitru Baleanu, and Sehrish Bano. 2020. "RETRACTED: Finite Element Study of Magnetohydrodynamics (MHD) and Activation Energy in Darcy–Forchheimer Rotating Flow of Casson Carreau Nanofluid" Processes 8, no. 9: 1185. https://doi.org/10.3390/pr8091185
APA StyleAli, B., Rasool, G., Hussain, S., Baleanu, D., & Bano, S. (2020). RETRACTED: Finite Element Study of Magnetohydrodynamics (MHD) and Activation Energy in Darcy–Forchheimer Rotating Flow of Casson Carreau Nanofluid. Processes, 8(9), 1185. https://doi.org/10.3390/pr8091185








