A Two-Patch Mathematical Model for Temperature-Dependent Dengue Transmission Dynamics
Abstract
1. Introduction
2. Materials and Methods
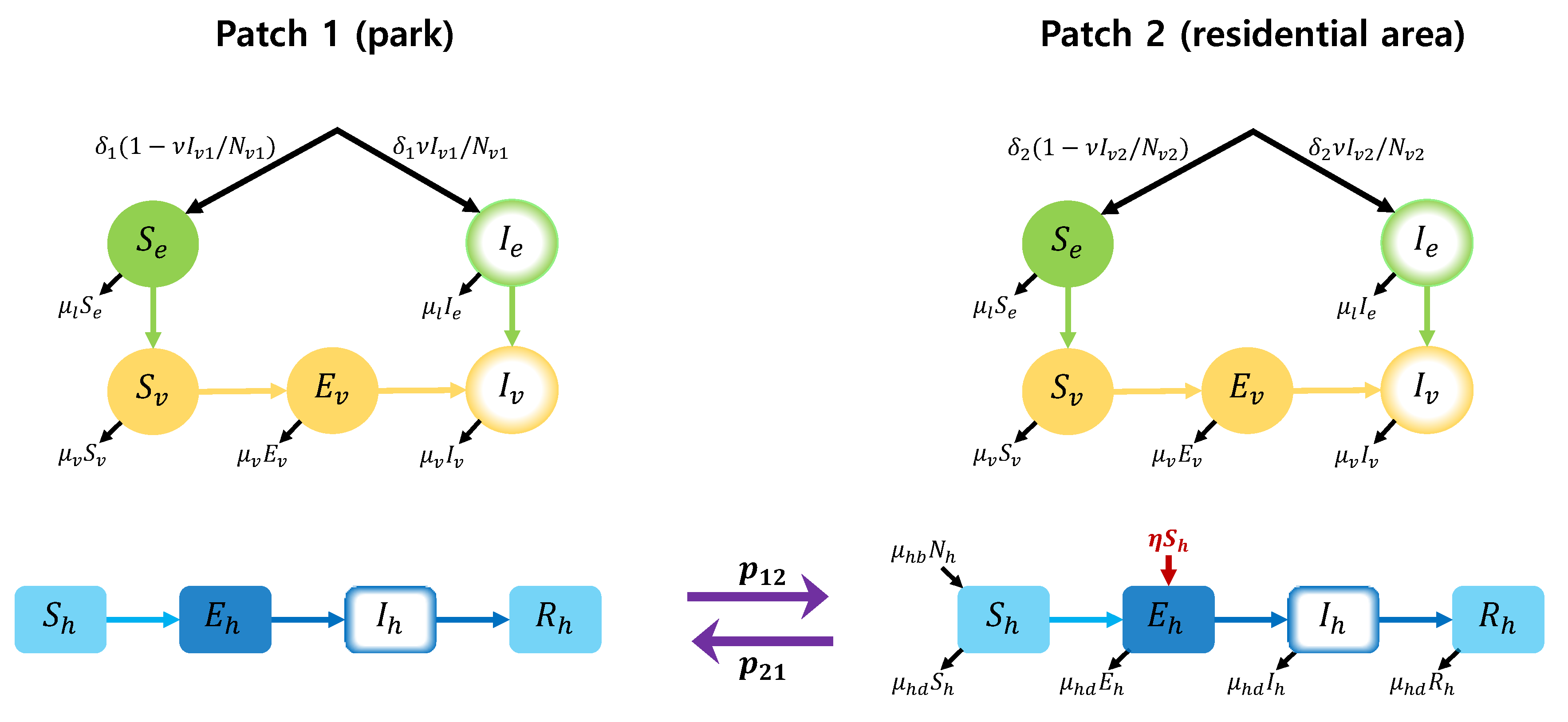
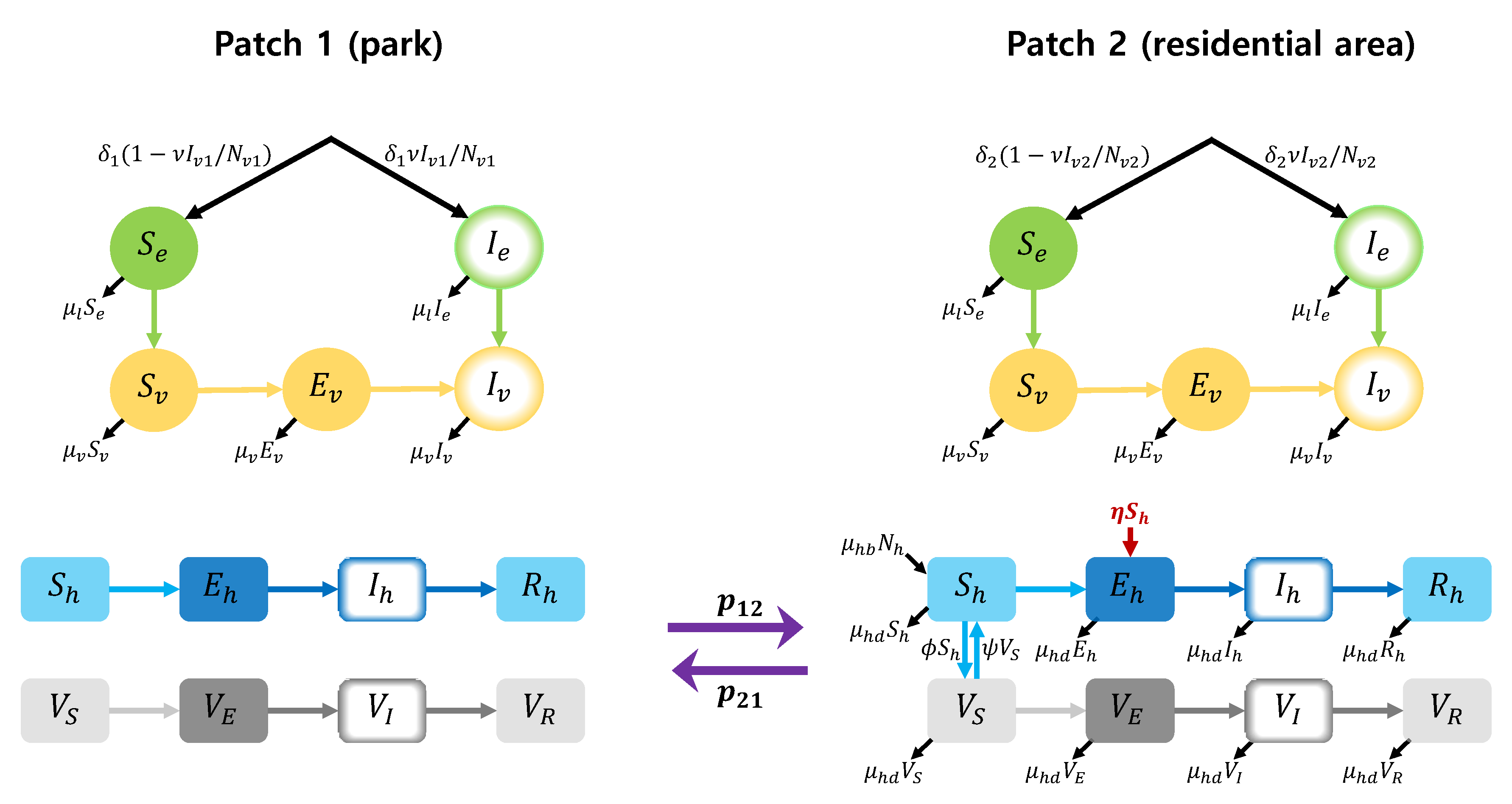
2.1. Two-Patch Dengue Transmission Model
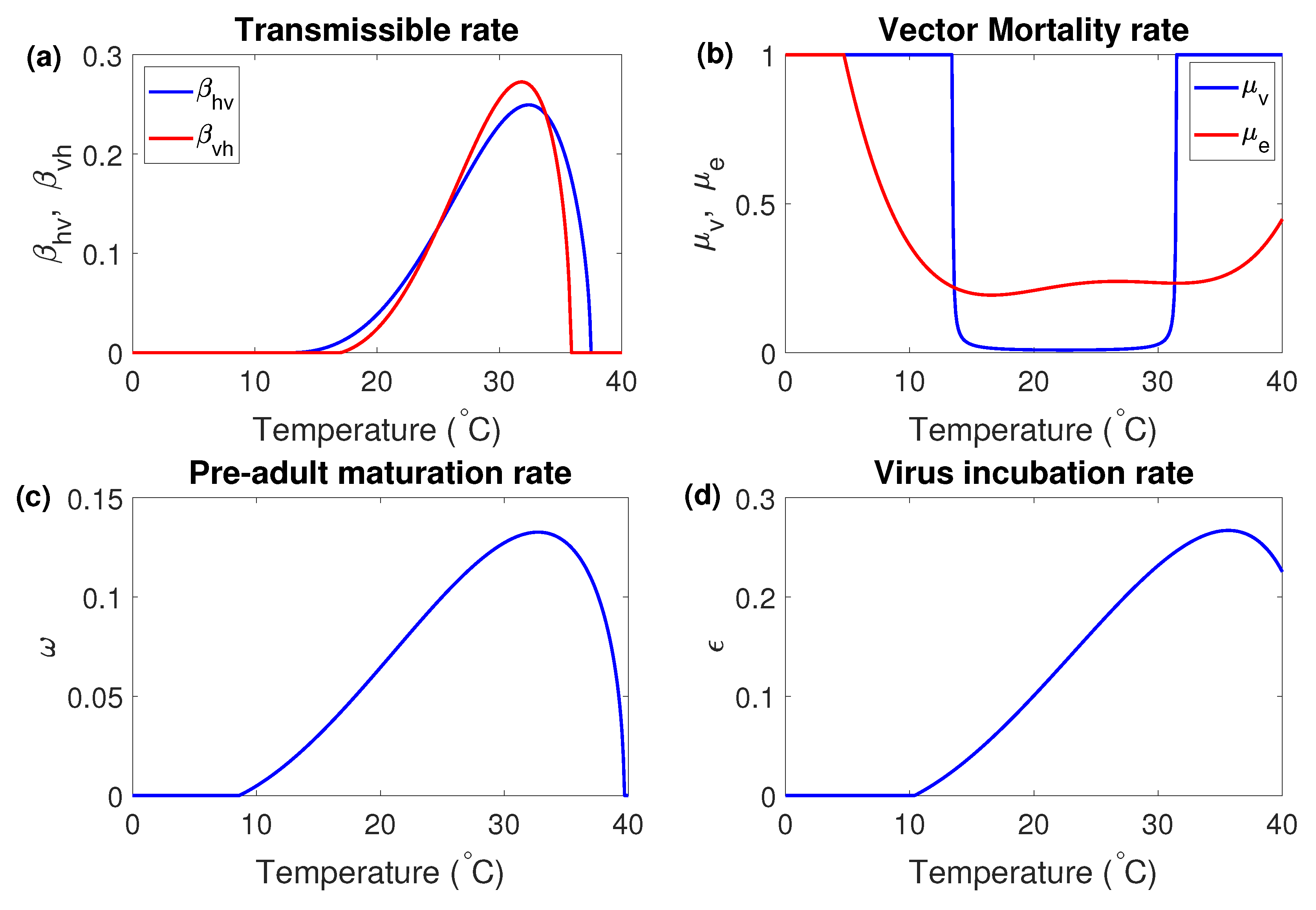
2.2. Parameter Estimation
- (1)
- (2)
- The probability of infection from mosquito to human per bite is [21]
- (3)
- The probability of infection from human to mosquito per bite is [21]
- (4)
- The mortality rate of the adult mosquito is [21]
- (5)
- Pre-adult maturation rate is [21]
- (6)
- Virus incubation rate is [21]
- (7)
- The mortality rate of larva (aquatic phase mortality rate) is [24]
- (8)
- The number of new recruits in the larvae stage for patch is computed as [14]
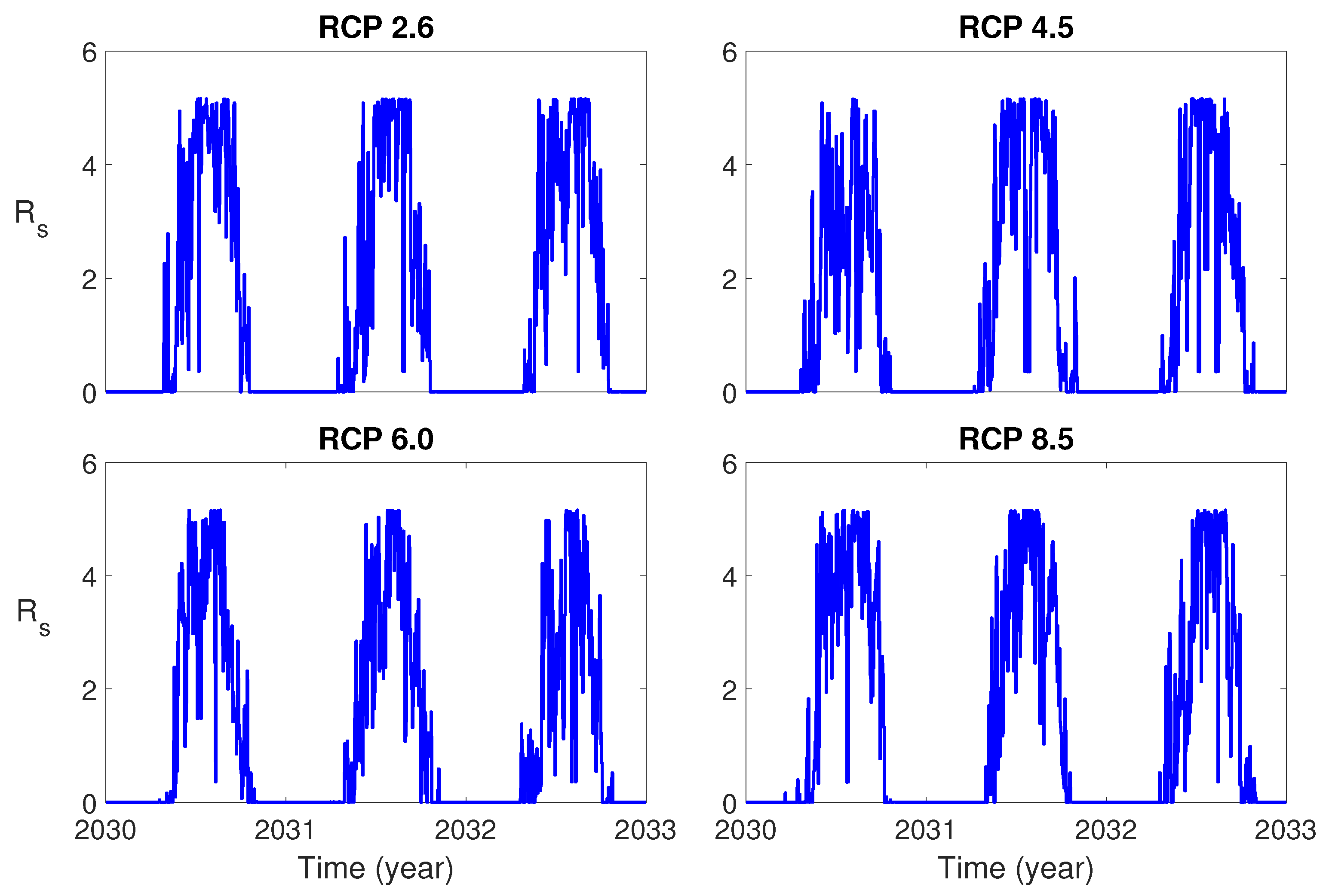
2.3. The Seasonal Reproduction Number
3. Results
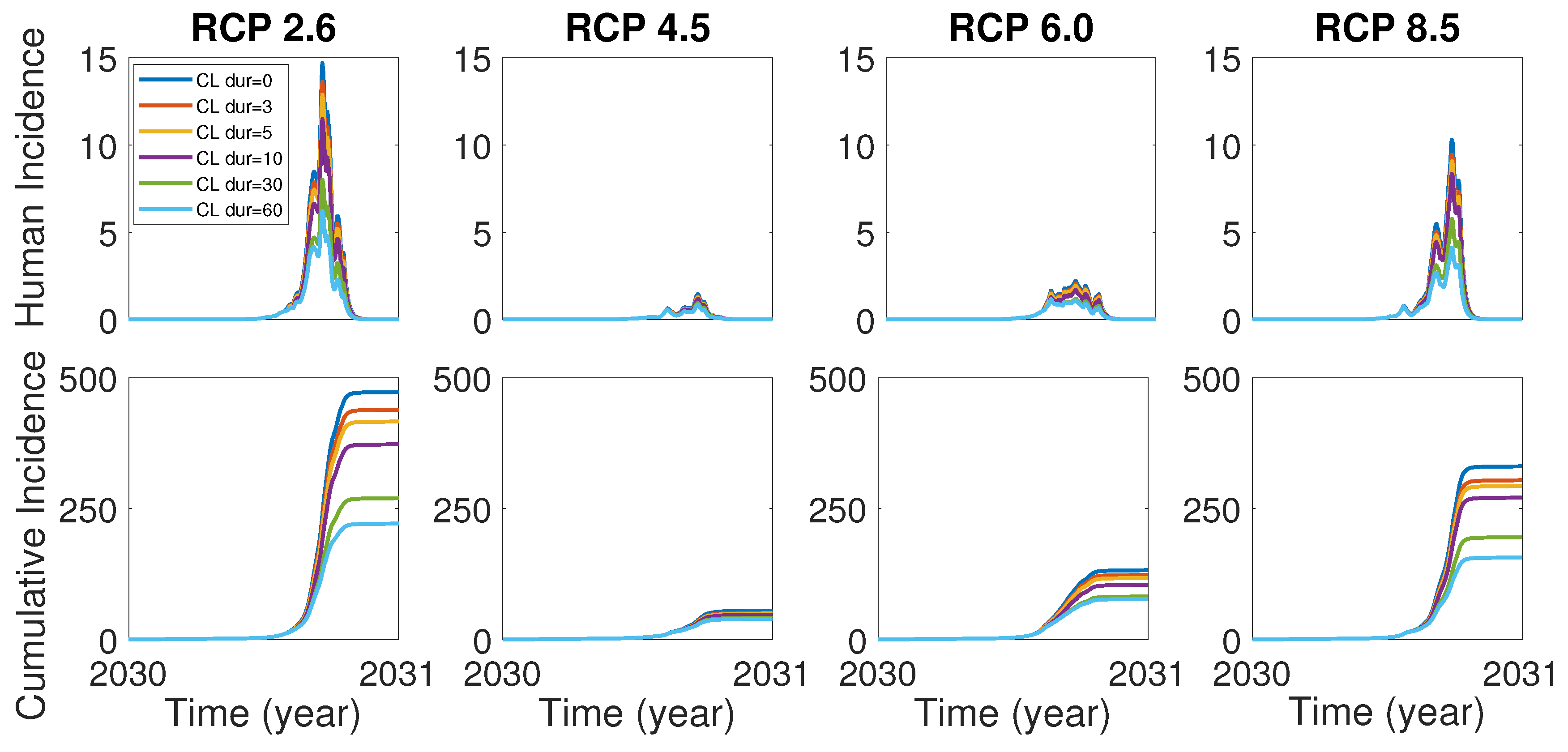
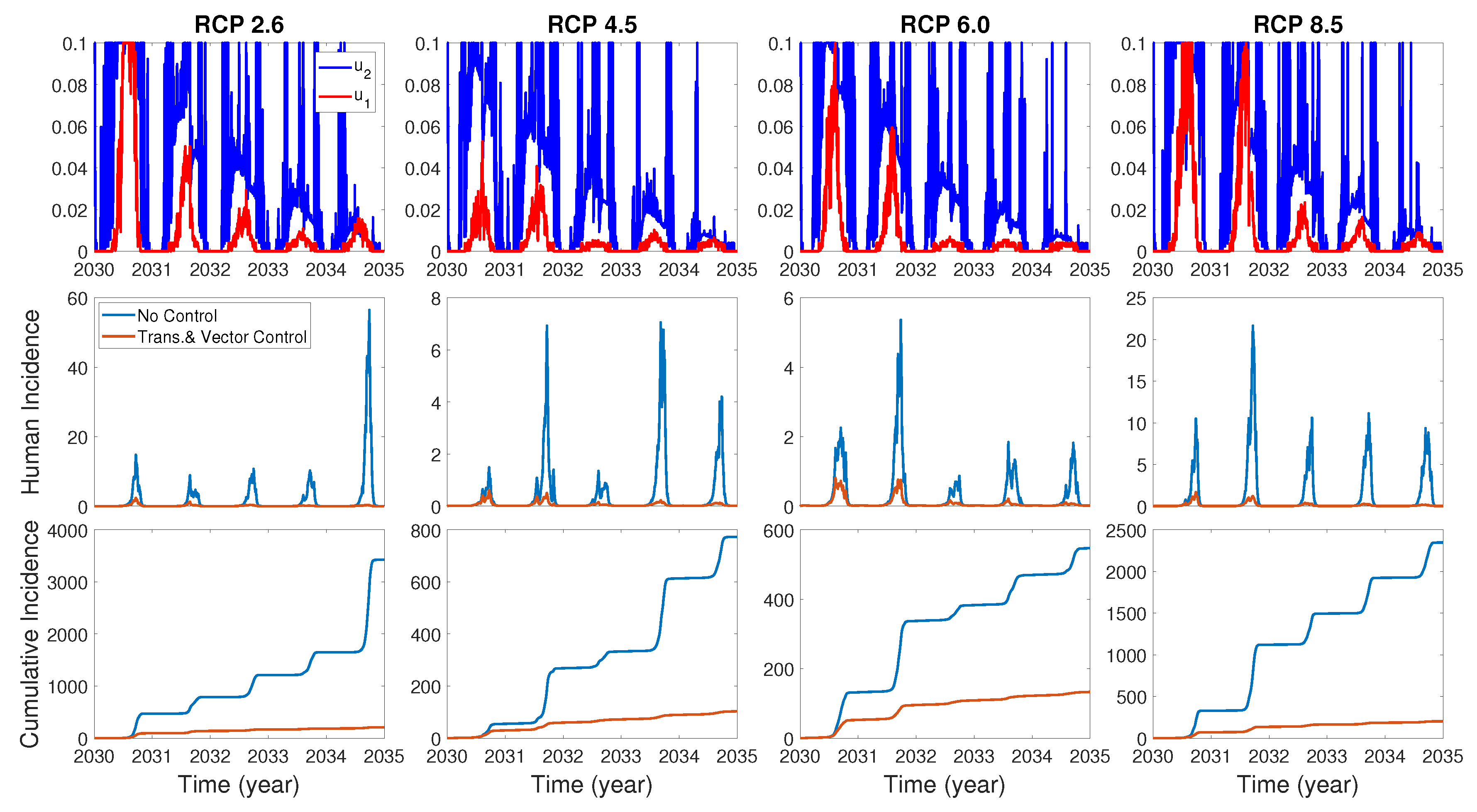
3.1. Dengue Transmission Dynamics Based on Rcp Scenarios
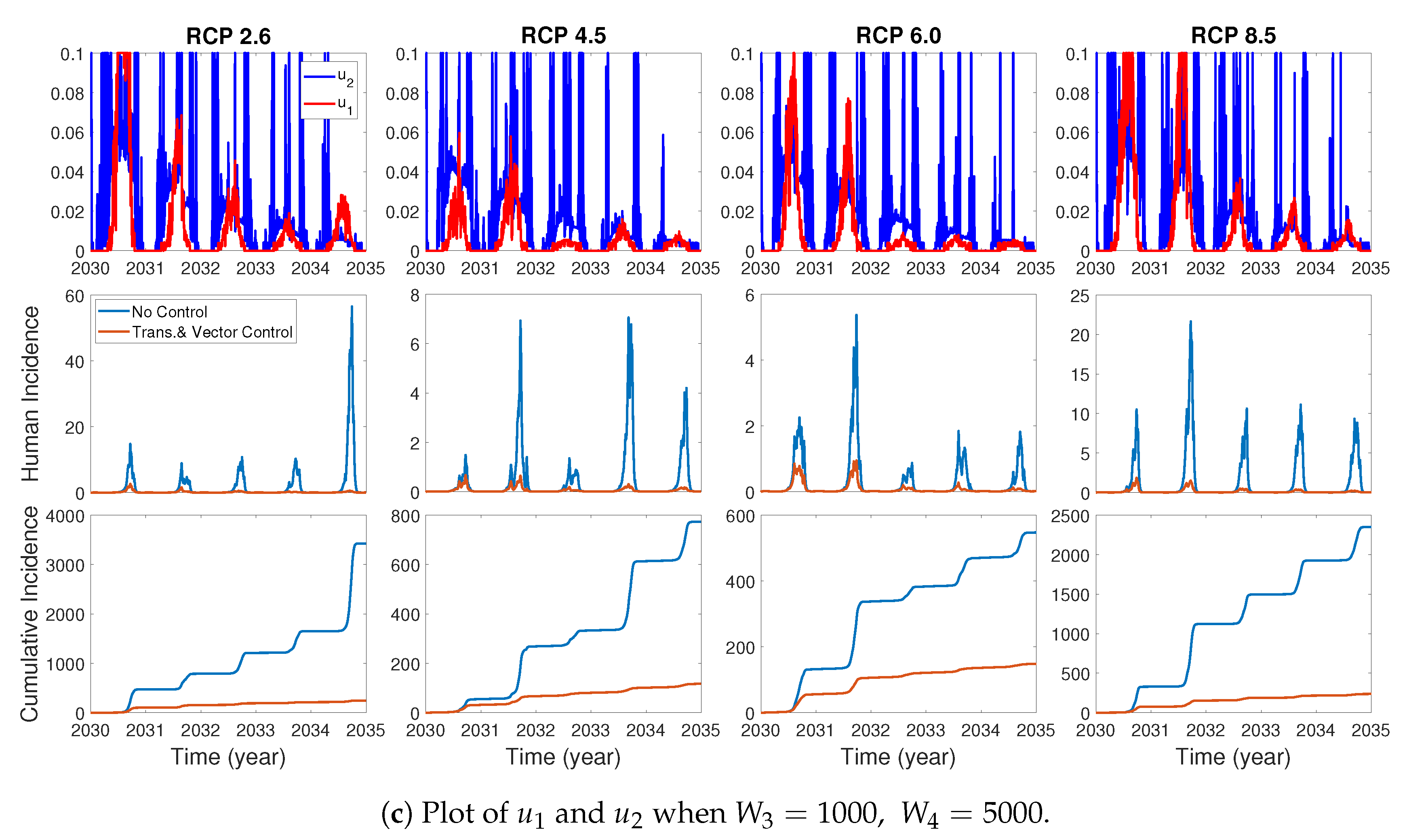
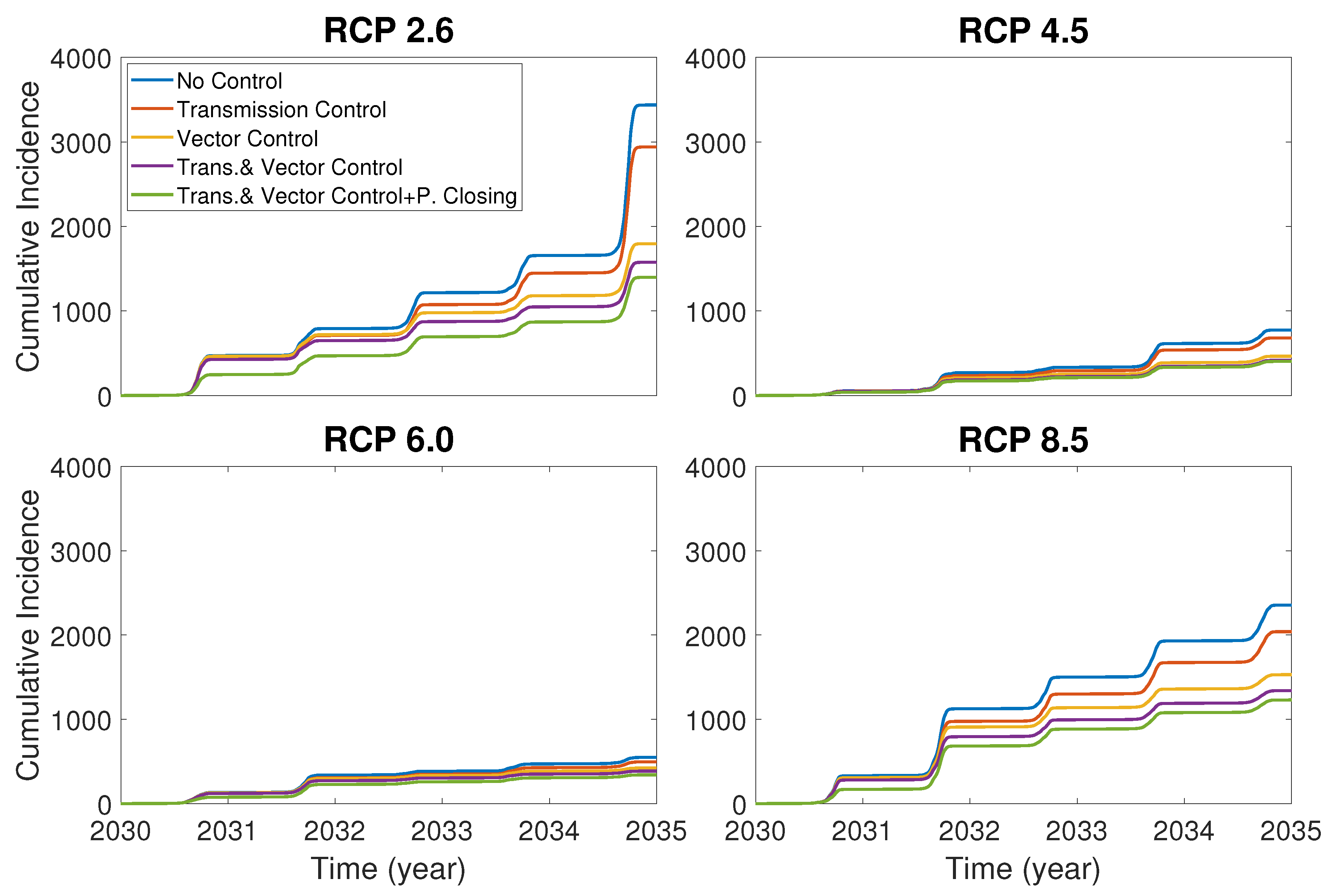
3.2. The Effects of Human and Vector Controls
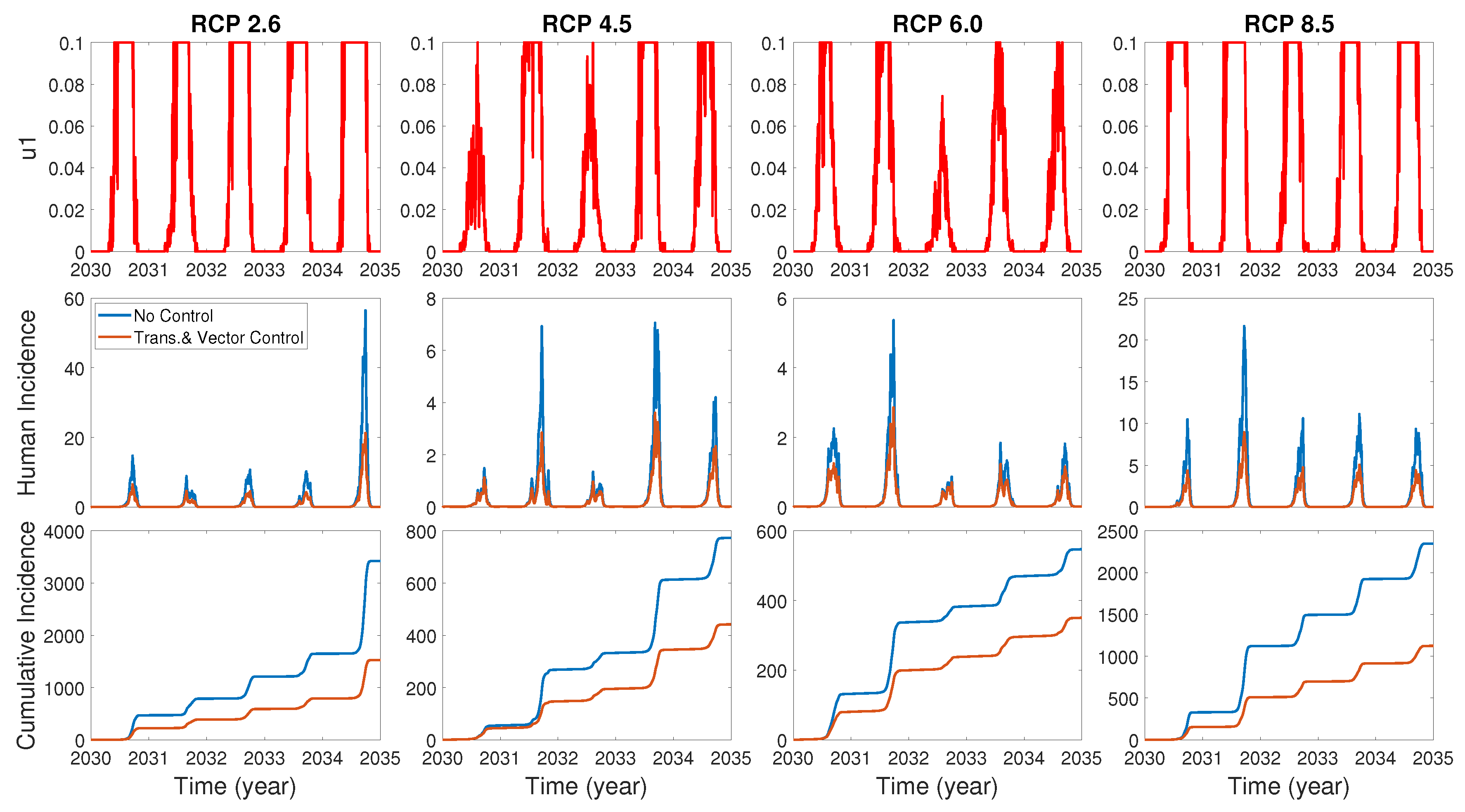
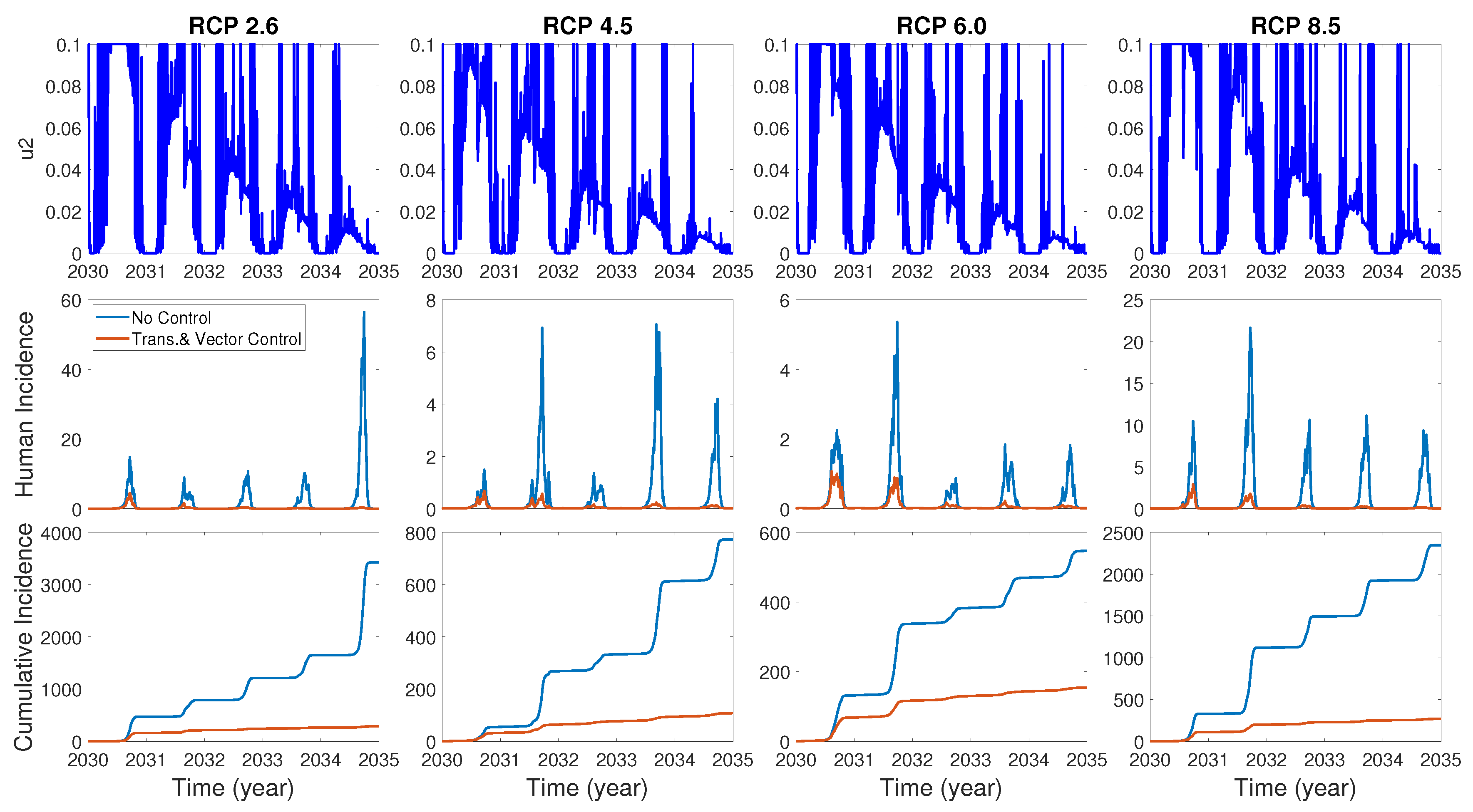
3.3. Optimal Control
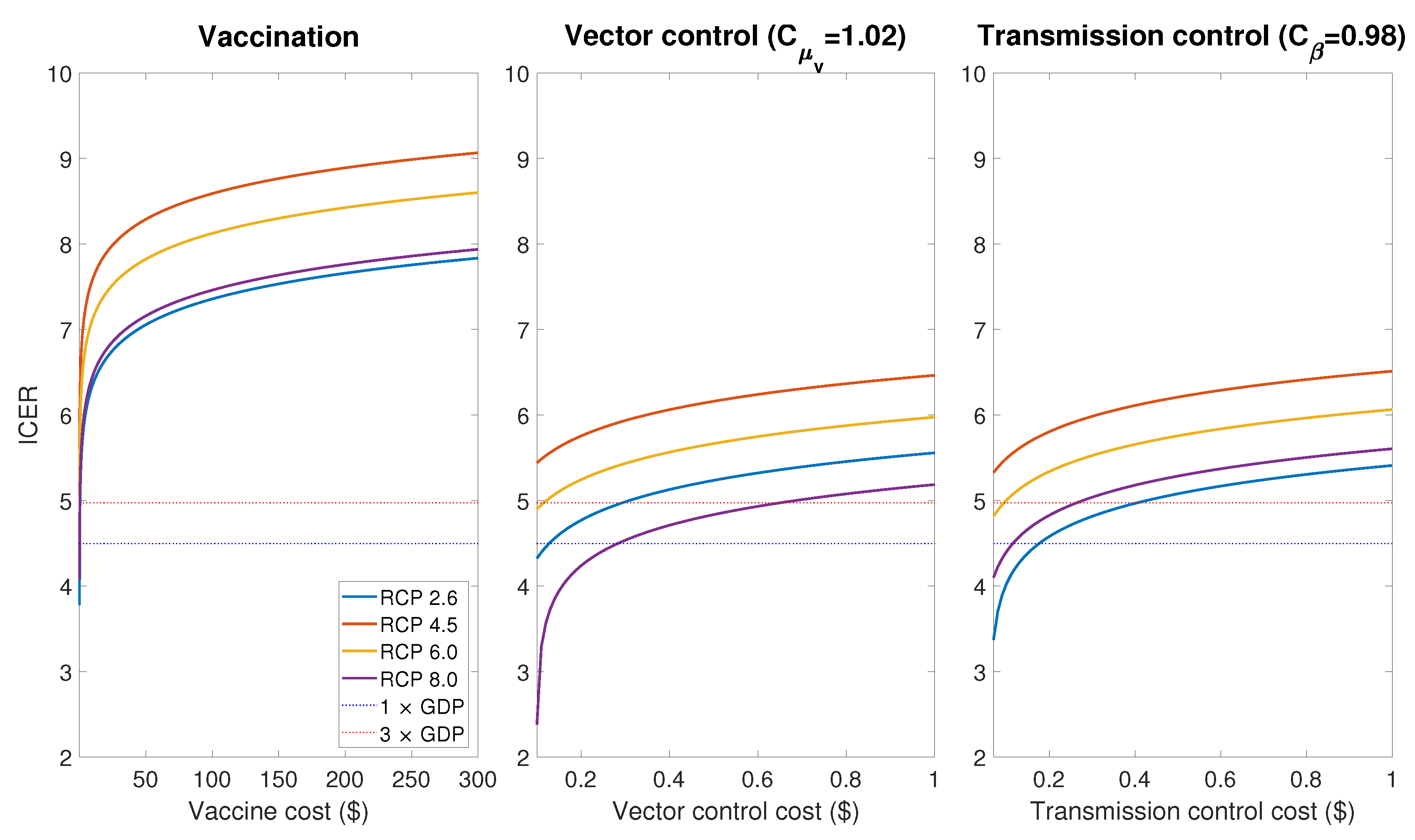
3.4. Vaccination Model and Cost-Effectiveness of Control Strategies
4. Discussion and Conclusions
Author Contributions
Funding
Conflicts of Interest
Appendix A. Temperature Data under RCP Scenarios

Appendix B. Proofs of Theorems 1, 2 and 3
Appendix C. Optimal Control Result with Different Weight Constants

References
- Cook, G.C.; Zumla, A. Manson’s Tropical Diseases; Elsevier Health Sciences: Amsterdam, The Netherlands, 2008. [Google Scholar]
- Martens, P. Health and Climate Change: Modelling the Impacts of Global Warming and Ozone Depletion; Routledge: Abingdon-on-Thames, UK, 2013. [Google Scholar]
- Teixeira, M.G.; Siqueira, J.B.; Germano, L., Jr.; Bricks, L.; Joint, G. Epidemiological trends of dengue disease in brazil (2000–2010): A systematic literature search and analysis. PLoS Negl. Trop. Dis. 2013, 7, e2520. [Google Scholar] [CrossRef] [PubMed]
- Morin, C.W.; Comrie, A.C.; Ernst, K. Climate and dengue transmission: Evidence and implications. Environ. Health Perspect. 2013, 121, 1264–1272. [Google Scholar] [CrossRef] [PubMed]
- Naish, S.; Dale, P.; Mackenzie, J.S.; McBride, J.; Mengersen, K.; Tong, S. Climate change and dengue: A critical and systematic review of quantitative modelling approaches. BMC Infect. Dis. 2014, 14, 167. [Google Scholar] [CrossRef]
- Liu-Helmersson, J.; Stenlund, H.; Wilder-Smith, A.; Rocklöv, J. Vectorial capacity of aedes aegypti: Effects of temperature and implications for global dengue epidemic potential. PLoS ONE 2014, 9, e89783. [Google Scholar] [CrossRef]
- Chen, S.-C.; Hsieh, M.-H. Modeling the transmission dynamics of dengue fever: Implications of temperature effects. Sci. Total Environ. 2012, 431, 385–391. [Google Scholar] [CrossRef] [PubMed]
- Kutsuna, S.; Kato, Y.; Moi, M.L.; Kotaki, A.; Ota, M.; Shinohara, K.; Kobayashi, T.; Yamamoto, K.; Fujiya, Y.; Mawatari, M.; et al. Autochthonous dengue fever, Tokyo, Japan, 2014. Emerg. Infect. Dis. 2015, 21, 517. [Google Scholar] [CrossRef]
- Yuan, B.; Lee, H.; Nishiura, H. Assessing dengue control in Tokyo, 2014. PLoS Negl. Trop. Dis. 2019, 13, e0007468. [Google Scholar] [CrossRef]
- Cho, H.-W.; Chu, C. A disease around the corner. Osong Public Health Res. Perspect. 2016, 7, 1–2. [Google Scholar] [CrossRef]
- Jeong, Y.E.; Lee, W.-C.; Cho, J.E.; Han, M.-G.; Lee, W.-J. Comparison of the epidemiological aspects of imported dengue cases between korea and japan, 2006–2010. Osong Public Health Res. Perspect. 2016, 7, 71–74. [Google Scholar] [CrossRef]
- Lim, S.-K.; Lee, Y.S.; Namkung, S.; Lim, J.K.; Yoon, I.-K. Prospects for dengue vaccines for travelers. Clin. Exp. Vaccine Res. 2016, 5, 89–100. [Google Scholar] [CrossRef]
- Lee, J.-S.; Farlow, A. The threat of climate change to non-dengue-endemic countries: Increasing risk of dengue transmission potential using climate and non-climate datasets. BMC Public Health 2019, 19, 934. [Google Scholar] [CrossRef] [PubMed]
- Lee, H.; Kim, J.E.; Lee, S.; Lee, C.H. Potential effects of climate change on dengue transmission dynamics in Korea. PLoS ONE 2018, 13, e0199205. [Google Scholar] [CrossRef]
- Landscape Architecture Korea (LAK). 2019. Available online: https://www.lak.co.kr/m/news/view.php?id=7248 (accessed on 20 June 2020).
- Adams, B.; Boots, M. How important is vertical transmission in mosquitoes for the persistence of dengue? insights from a mathematical model. Epidemics 2010, 2, 1–10. [Google Scholar] [CrossRef]
- Wearing, H.J.; Rohani, P. Ecological and immunological determinants of dengue epidemics. Proc. Natl. Acad. Sci. USA 2016, 103, 11802–11807. [Google Scholar] [CrossRef] [PubMed]
- Janreung, S.; Chinviriyasit, W. Dengue fever with two strains in thailand. Int. J. Appl. Phys. Math. 2014, 4, 55. [Google Scholar]
- Korean Statistical Information Service(KOSIS), Population Projections and Summary Indicators (Province). 2017. Available online: http://kosis.kr/eng/ (accessed on 29 May 2020).
- Shim, E. Cost-effectiveness of dengue vaccination in yucatán, mexico using a dynamic dengue transmission model. PLoS ONE 2017, 12, e0175020. [Google Scholar] [CrossRef]
- Mordecai, E.A.; Cohen, J.M.; Evans, M.V.; Gudapati, P.; Johnson, L.R.; Lippi, C.A.; Miazgowicz, K.; Murdock, C.C.; Rohr, J.R.; Ryan, S.J.; et al. Detecting the impact of temperature on transmission of zika, dengue, and chikungunya using mechanistic models. PLoS Negl. Trop. Dis. 2017, 11, e0005568. [Google Scholar] [CrossRef] [PubMed]
- Derouich, M.; Boutayeb, A.; Twizell, E. A model of dengue fever. BioMed. Eng. OnLine 2003, 2, 1. [Google Scholar] [CrossRef] [PubMed]
- Tran, A.; L’Ambert, G.; Lacour, G.; Benoît, R.; Demarchi, M.; Cros, M.; Cailly, P.; Aubry-Kientz, M.; Balenghien, T.; Ezanno, P. A rainfall-and temperature-driven abundance model for aedes albopictus populations. Int. J. Environ. Res. Public Health 2013, 10, 1698–1719. [Google Scholar] [CrossRef]
- Yang, H.; Macoris, M.d.L.d.G.; Galvani, K.; Andrighetti, M.; Wanderley, D. Assessing the effects of temperature on the population of aedes aegypti, the vector of dengue. Epidemiol. Infect. 2009, 137, 1188–1202. [Google Scholar] [CrossRef]
- Tjaden, N.B.; Thomas, S.M.; Fischer, D.; Beierkuhnlein, C. Extrinsic incubation period of dengue: Knowledge, backlog, and applications of temperature dependence. PLoS Negl. Trop. Dis. 2013, 7, e2207. [Google Scholar] [CrossRef] [PubMed]
- Korea Centers for Disease Control and Prevention (KCDC), Mathematical Modelling on p.vivax Malaria Transmission and Development of Its Application Program. 2009. Available online: http://www.cdc.go.kr/cdc_eng/ (accessed on 29 May 2020).
- Intergovernmental Panel on Climate Change (IPCC), Fifth Assessment Report. 2014. Available online: https://www.ipcc.ch/assessment-report/ar5/ (accessed on 29 May 2020).
- Brauer, F.; Castillo-Chavez, C. Mathematical Models in Population Biology and Epidemiology; Springer: New York, NY, USA, 2012; Volume 1. [Google Scholar]
- Kim, J.E.; Choi, Y.; Lee, C.H. Effects of climate change on plasmodium vivax malaria transmission dynamics: A mathematical modeling approach. Appl. Math. Comput. 2019, 347, 616–630. [Google Scholar] [CrossRef]
- Kim, J.E.; Lee, H.; Lee, C.H.; Lee, S. Assessment of optimal strategies in a two-patch dengue transmission model with seasonality. PLoS ONE 2017, 12, e0173673. [Google Scholar] [CrossRef] [PubMed]
- Pontryagin, L.S. Mathematical Theory of Optimal Processes; Routledge: Abingdon-on-Thames, UK, 2018. [Google Scholar]
- Fleming, W.H.; Rishel, R.W. Deterministic and Stochastic Optimal Control; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2012; Volume 1. [Google Scholar]
- Thomas, S.J.; Yoon, I.-K. A review of dengvaxia®: Development to deployment. Hum. Vaccines Immunother. 2019, 15, 2295–2314. [Google Scholar] [CrossRef]
- Hadinegoro, S.R.; Arredondo-García, J.L.; Capeding, M.R.; Deseda, C.; Chotpitayasunondh, T.; Dietze, R.; Ismail, H.H.M.; Reynales, H.; Limkittikul, K.; Rivera-Medina, D.M.; et al. Efficacy and long-term safety of a dengue vaccine in regions of endemic disease. N. Engl. J. Med. 2015, 373, 1195–1206. [Google Scholar] [CrossRef]
- Ferguson, N.M.; Rodríguez-Barraquer, I.; Dorigatti, I.; Teran-Romero, L.M.; Laydon, D.J.; Cummings, D.A. Benefits and risks of the sanofi-pasteur dengue vaccine: Modeling optimal deployment. Science 2016, 353, 1033–1036. [Google Scholar] [CrossRef]
- Sachs, J. Macroeconomics and Health: Investing in Health for Economic Development: Report of the Commission on Macroeconomics and Health; World Health Organization: Geneva, Switzerland, 2001. [Google Scholar]
- Murray, C.J. Quantifying the burden of disease: The technical basis for disability-adjusted life years. Bull. World Health Organ. 1994, 72, 429. [Google Scholar]
- Carrasco, L.R.; Lee, L.K.; Lee, V.J.; Ooi, E.E.; Shepard, D.S.; Thein, T.L.; Gan, V.; Cook, A.R.; Lye, D.; Ng, L.C.; et al. Economic impact of dengue illness and the cost-effectiveness of future vaccination programs in singapore. PLoS Negl. Trop. Dis. 2011, 5, e1426. [Google Scholar] [CrossRef]
- World Health Organization. The Global Burden of Disease: 2004 Update; World Health Organization: Geneva, Switzerland, 2008. [Google Scholar]
- Durham, D.P.; Mbah, M.L.N.; Medlock, J.; Luz, P.M.; Meyers, L.A.; Paltiel, A.D.; Galvani, A.P. Dengue dynamics and vaccine cost-effectiveness in brazil. Vaccine 2013, 31, 3957–3961. [Google Scholar] [CrossRef]
- Korea Centers for Disease Control and Prevention (KCDC), Infectious Disease Statistics System. 2020. Available online: https://www.cdc.go.kr/npt/biz/npp/nppMain.do (accessed on 29 May 2020).
- Gubler, D.J. Dengue and dengue hemorrhagic fever. Clin. Microbiol. Rev. 1998, 11, 480–496. [Google Scholar] [CrossRef]
- Joob, B.; Wiwanitkit, V. Cost and effectiveness of dengue vaccine: A report from endemic area, thailand. J. Med. Soc. 2018, 32, 163. [Google Scholar] [CrossRef]
- Darbro, J.; Halasa, Y.; Montgomery, B.; Muller, M.; Shepard, D.; Devine, G.; Mwebaze, P. An economic analysis of the threats posed by the establishment of aedes albopictus in brisbane, queensland. Ecol. Econ. 2017, 142, 203–213. [Google Scholar] [CrossRef]
- Packierisamy, P.R.; Ng, C.-W.; Dahlui, M.; Inbaraj, J.; Balan, V.K.; Halasa, Y.A.; Shepard, D.S. Cost of dengue vector control activities in malaysia. Am. J. Trop. Med. Hyg. 2015, 93, 1020–1027. [Google Scholar] [CrossRef] [PubMed]
- Centers for Disease Control and Prevention, Dengue Vaccine. 2019. Available online: https://www.cdc.gov/dengue/prevention/dengue-vaccine.html (accessed on 20 June 2020).
- World Health Organization. Questions and Answers on Dengue Vaccines. 2018. Available online: https://www.who.int/immunization/research/development/dengue_q_and_a/en/ (accessed on 20 June 2020).

| Symbol | Description | Value | Reference |
|---|---|---|---|
| Vertical infection rate of Aedes albopictus mosquitoes | 0.004 | [16] | |
| Latent period for human (day) | 5 | [17] | |
| Infectious period for human (day) | 7 | [7,16,18] | |
| Human birth rate (day) | 0.000022 | [19] | |
| Human death rate (day) | 0.000022 | Assumed | |
| Human movement rate from patch 2 to 1 (day) | 0.0411 | Estimated | |
| Human movement rate from patch 1 to 2 (day) | 0.999 | Assumed | |
| g | Proportion of dengue infections symptomatic in | 0.45 | [20] |
| b | Biting rate (day) | ** | [21] |
| Probability of infection per bite (v→h) | ** | [22] | |
| Probability of infection per bite (h→v) | ** | [22] | |
| Mortality rates of the larvae (day) | ** | [23] | |
| Mortality rates of the mosquitoes (day) | ** | [24] | |
| Pre-adult maturation rate (day) | ** | [24] | |
| Virus incubation rate (day) | ** | [25] | |
| Transmissible rate (v→h) (day) | [22] | ||
| Transmissible rate (h→v) (day) | [22] | ||
| Number of new recruits in the larvae stage | [16] | ||
| for patch (day) | |||
| Inflow rate of infection by international travelers (day) | ** | [14,26] |
| Symbol | Description | Value | Reference |
|---|---|---|---|
| Vaccine efficacy against infection | 0.616 | [34] | |
| Latent period for vaccinated human | 5 | Assumed | |
| Infectious period for vaccinated human | 7 | Assumed | |
| Proportion of symptomatic infection | 0.8 | [35] | |
| in the vaccinated class | |||
| Vaccination rate | 0.0030 | Estimated | |
| Immunity reduction rate | 0.0019 | Estimated |
| Symbol | Description | Value | Reference |
|---|---|---|---|
| r | Social discount rate for DALYs calculations | 0.03 | [37,38] |
| b | Parameter of the age-weighting function | 0.04 | [37,38] |
| h | Probability of developing DHF/DSS * | 0.045 × 0.25 | [20,35] |
| after symptomatic infection without vaccine | |||
| Probability of developing DHF/DSS | 0.045 | [35] | |
| after symptomatic infection with vaccine | |||
| C | Age-weighting correction constant | 0.16243 | [37,38] |
| Direct medical cost for DF | 293 | [20] | |
| Direct medical cost for DHF | 1171 | [20] | |
| Disability weight for death | 1 | [20] | |
| Disability weight for DF | 0.197 | [39,40] | |
| Disability weight for DHF | 0.545 | [39,40] | |
| Years of life lost due to death | 42 | [20] | |
| Time lost due to DF (years) | 0.019 | [40] | |
| Time lost due to DHF/DSS (years) | 0.0325 | [40] | |
| a | Average age of dengue exposure | 28 | [41] |
| Risk of death from DHF/DSS | 0.01 | [20,42] |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kim, J.E.; Choi, Y.; Kim, J.S.; Lee, S.; Lee, C.H. A Two-Patch Mathematical Model for Temperature-Dependent Dengue Transmission Dynamics. Processes 2020, 8, 781. https://doi.org/10.3390/pr8070781
Kim JE, Choi Y, Kim JS, Lee S, Lee CH. A Two-Patch Mathematical Model for Temperature-Dependent Dengue Transmission Dynamics. Processes. 2020; 8(7):781. https://doi.org/10.3390/pr8070781
Chicago/Turabian StyleKim, Jung Eun, Yongin Choi, James Slghee Kim, Sunmi Lee, and Chang Hyeong Lee. 2020. "A Two-Patch Mathematical Model for Temperature-Dependent Dengue Transmission Dynamics" Processes 8, no. 7: 781. https://doi.org/10.3390/pr8070781
APA StyleKim, J. E., Choi, Y., Kim, J. S., Lee, S., & Lee, C. H. (2020). A Two-Patch Mathematical Model for Temperature-Dependent Dengue Transmission Dynamics. Processes, 8(7), 781. https://doi.org/10.3390/pr8070781







