Finite Element Simulation of Multiple Slip Effects on MHD Unsteady Maxwell Nanofluid Flow over a Permeable Stretching Sheet with Radiation and Thermo-Diffusion in the Presence of Chemical Reaction
Abstract
1. Introduction
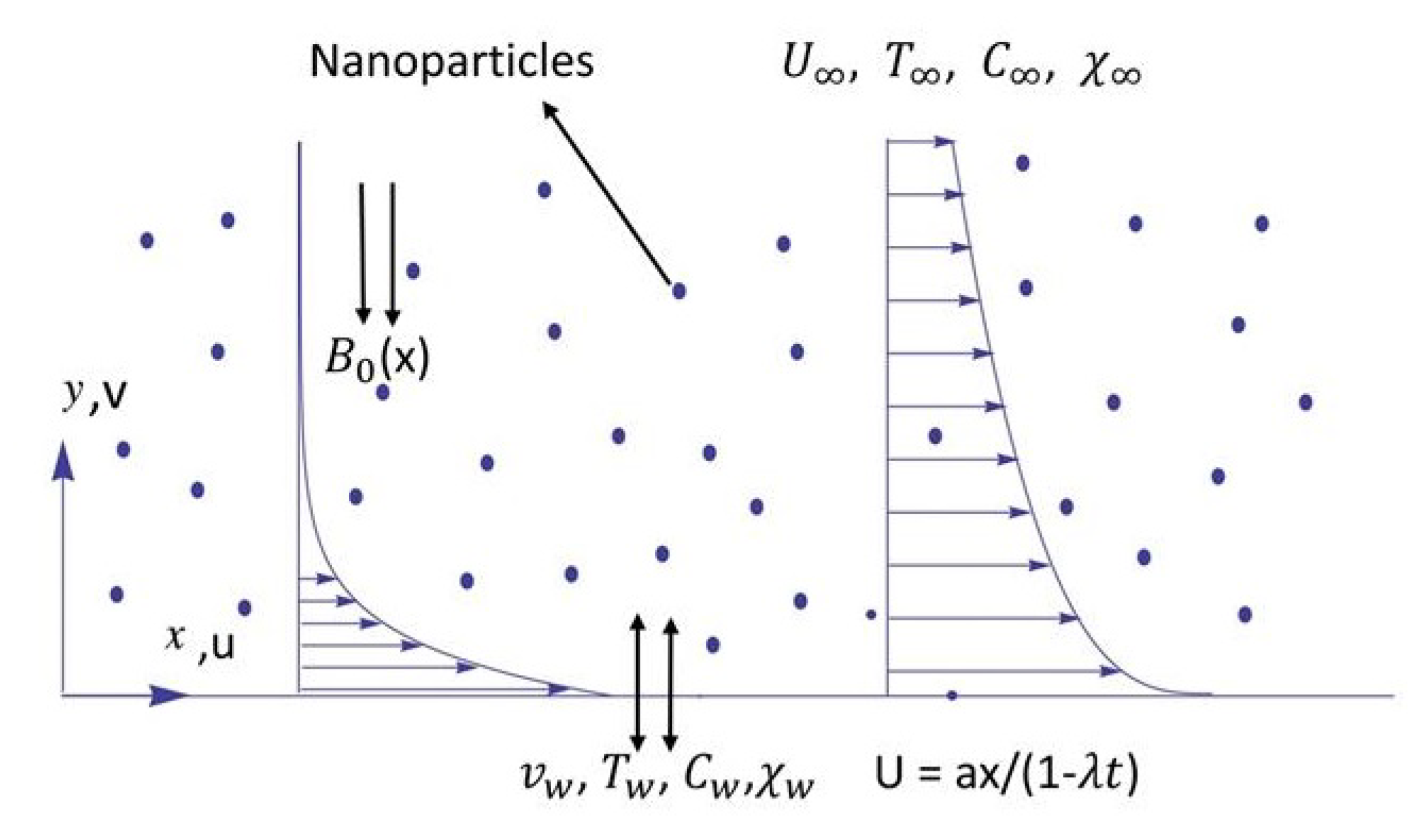
2. Mathematical Formulation
3. Finite Element Method Solutions
3.1. Variational Formulations
3.2. Finite Element Formulations
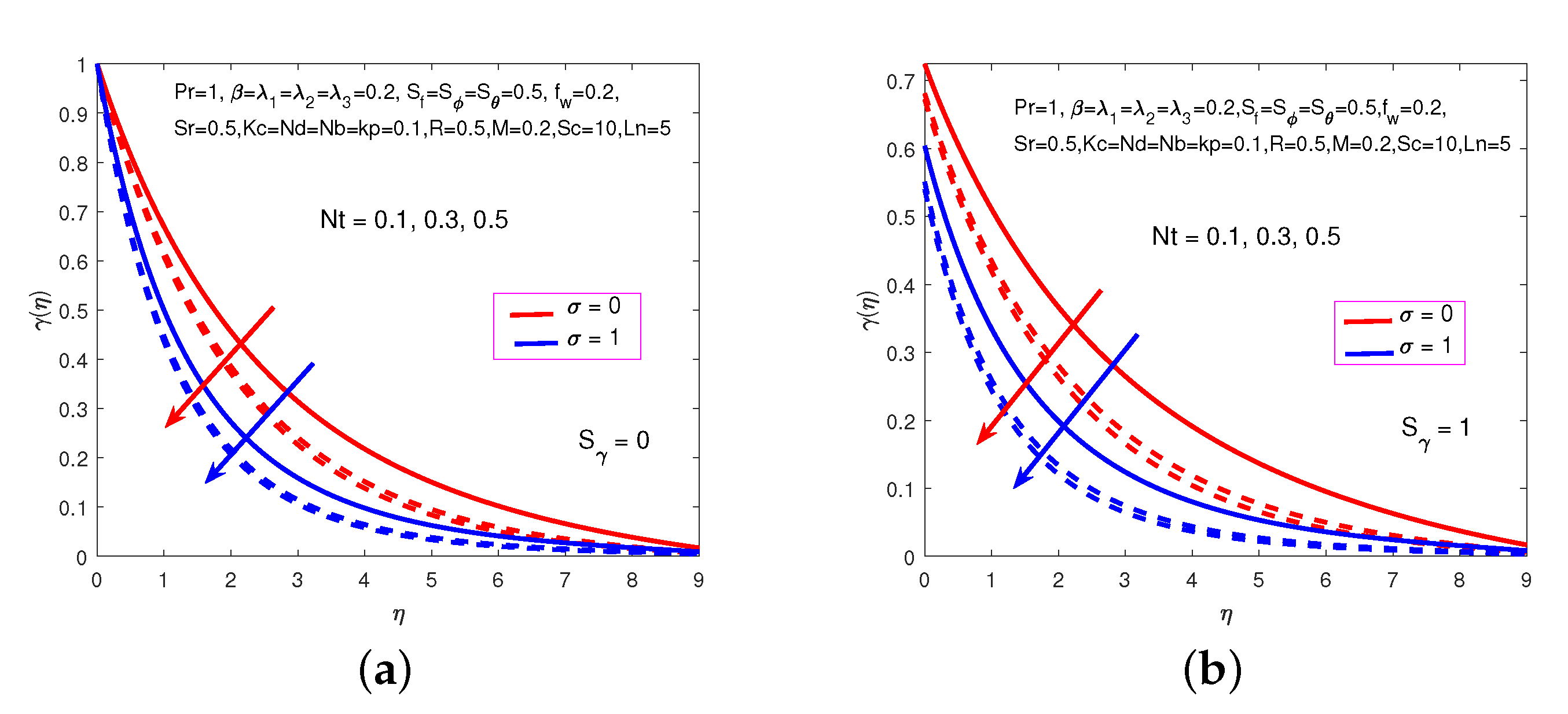
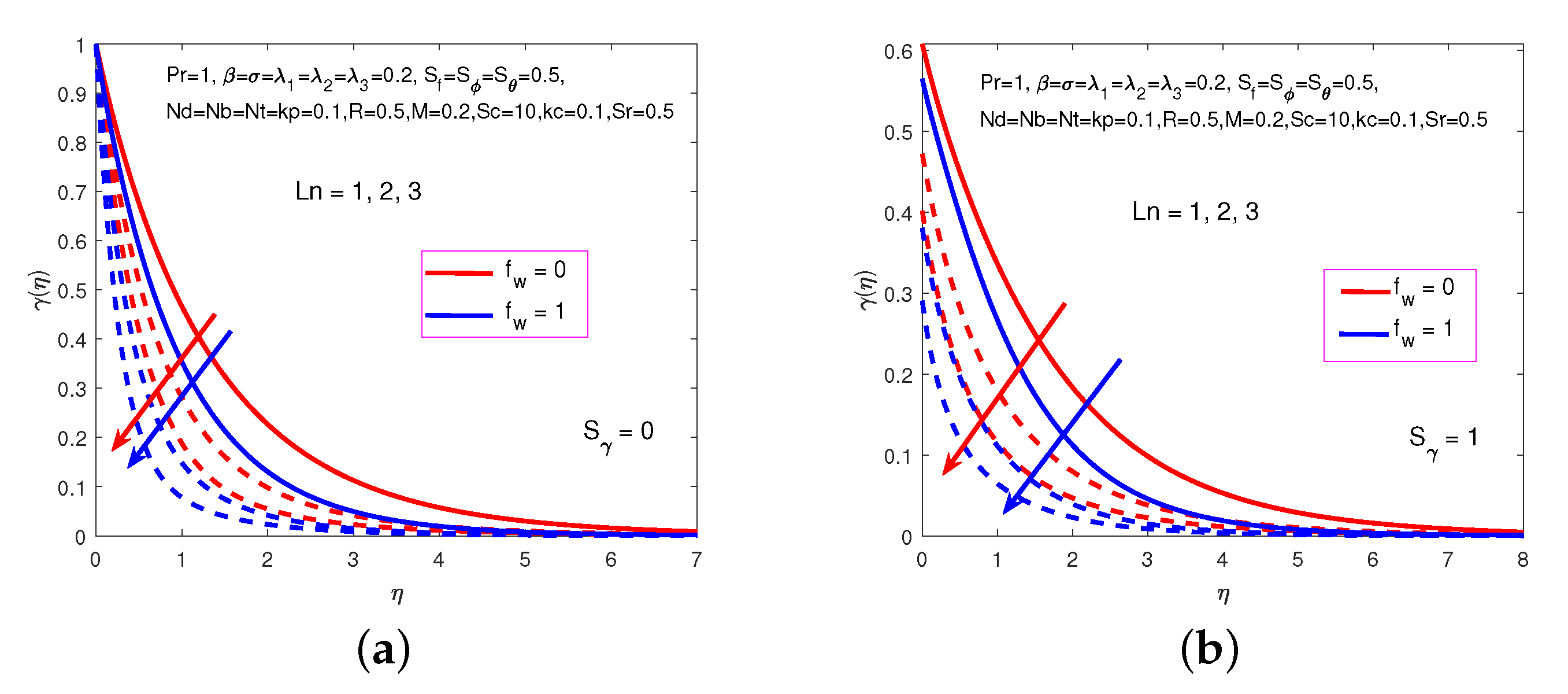
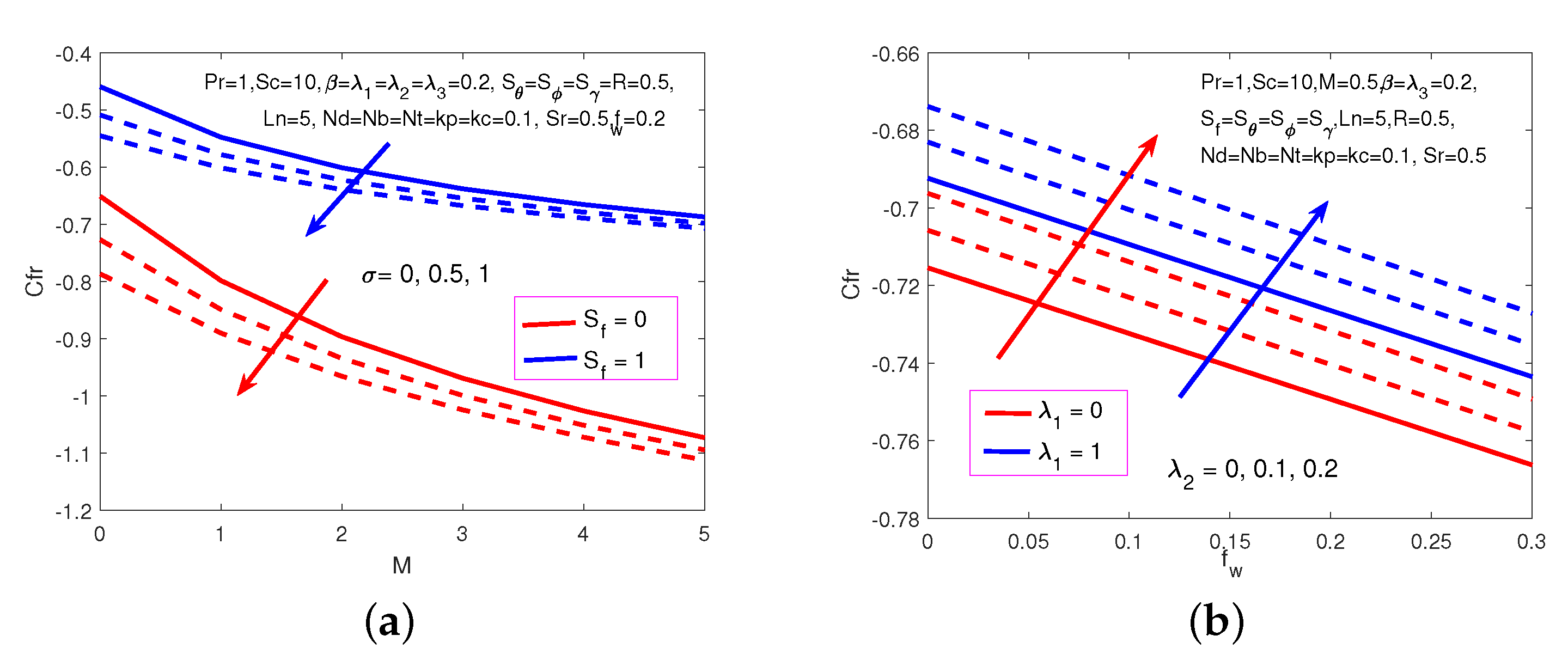
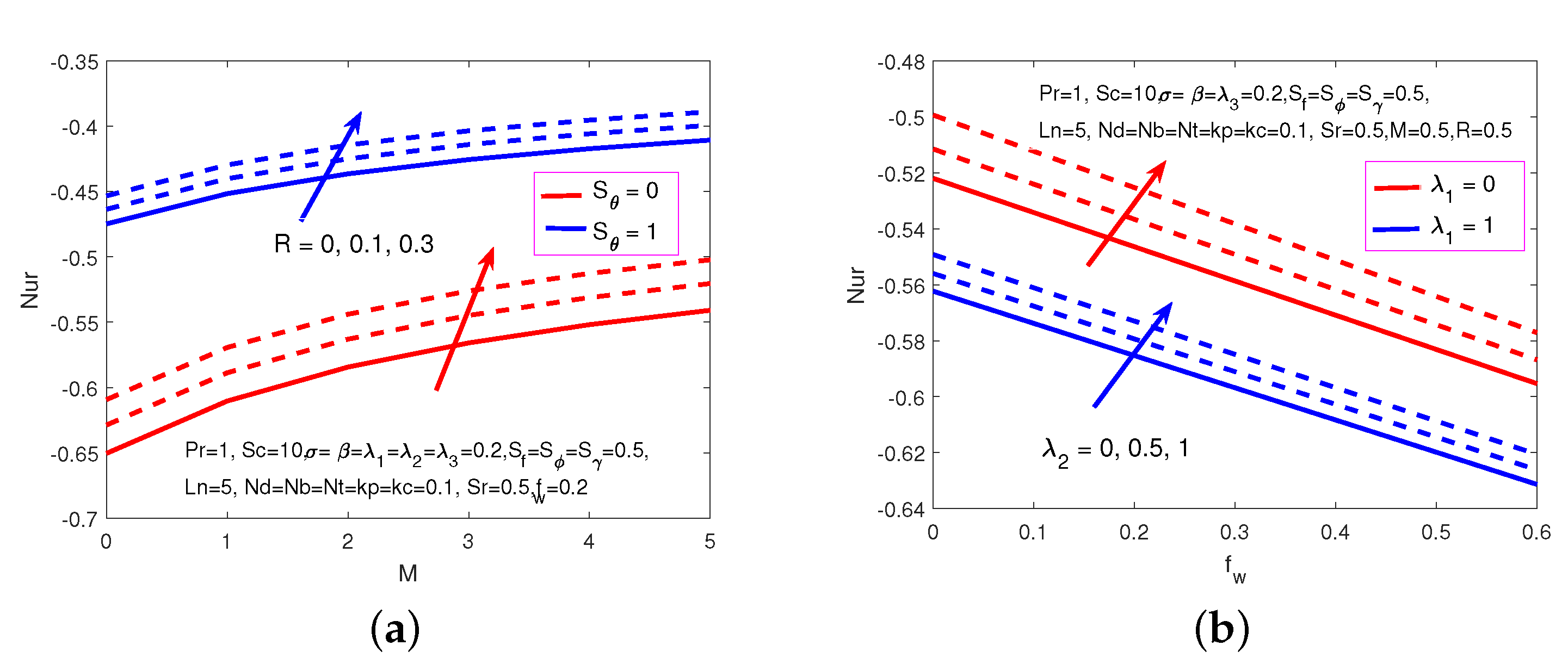
4. Results and Discussion
5. Conclusions
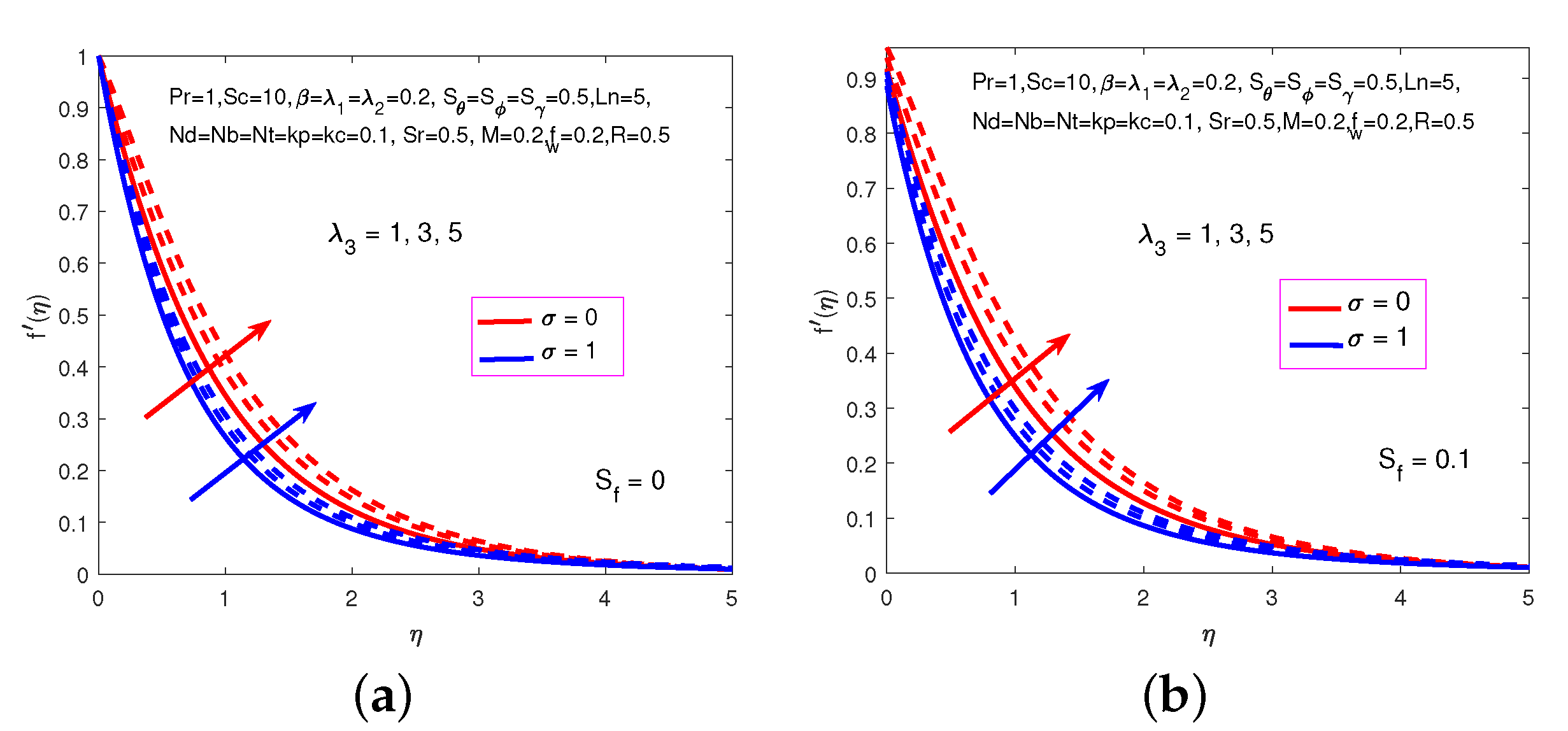
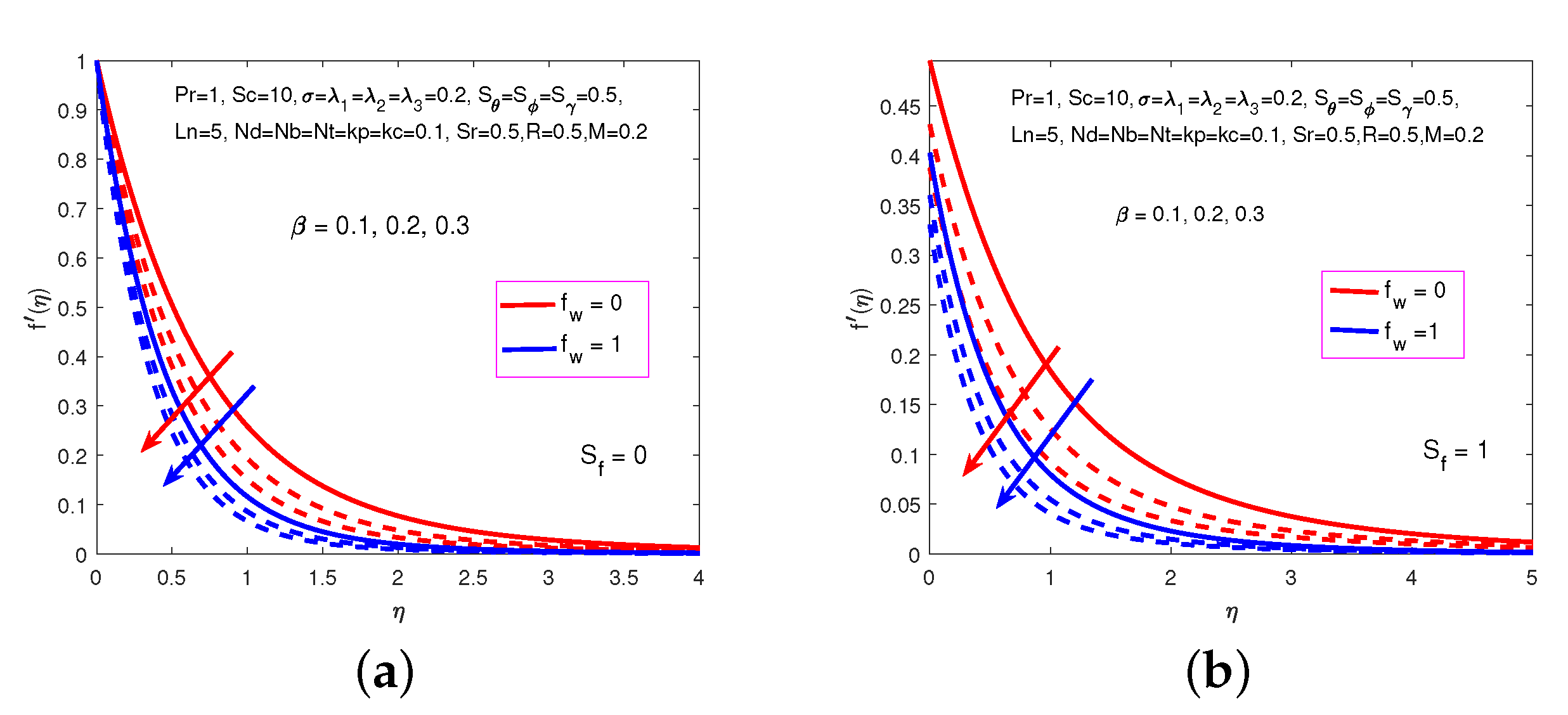
- The velocity profiles decrease with increasing values of suction, slip, unsteadiness, magnetic, Deborah number, and permeability parameters, but the influence is opposite for increasing values of buoyancy parameters and thermal radiation.
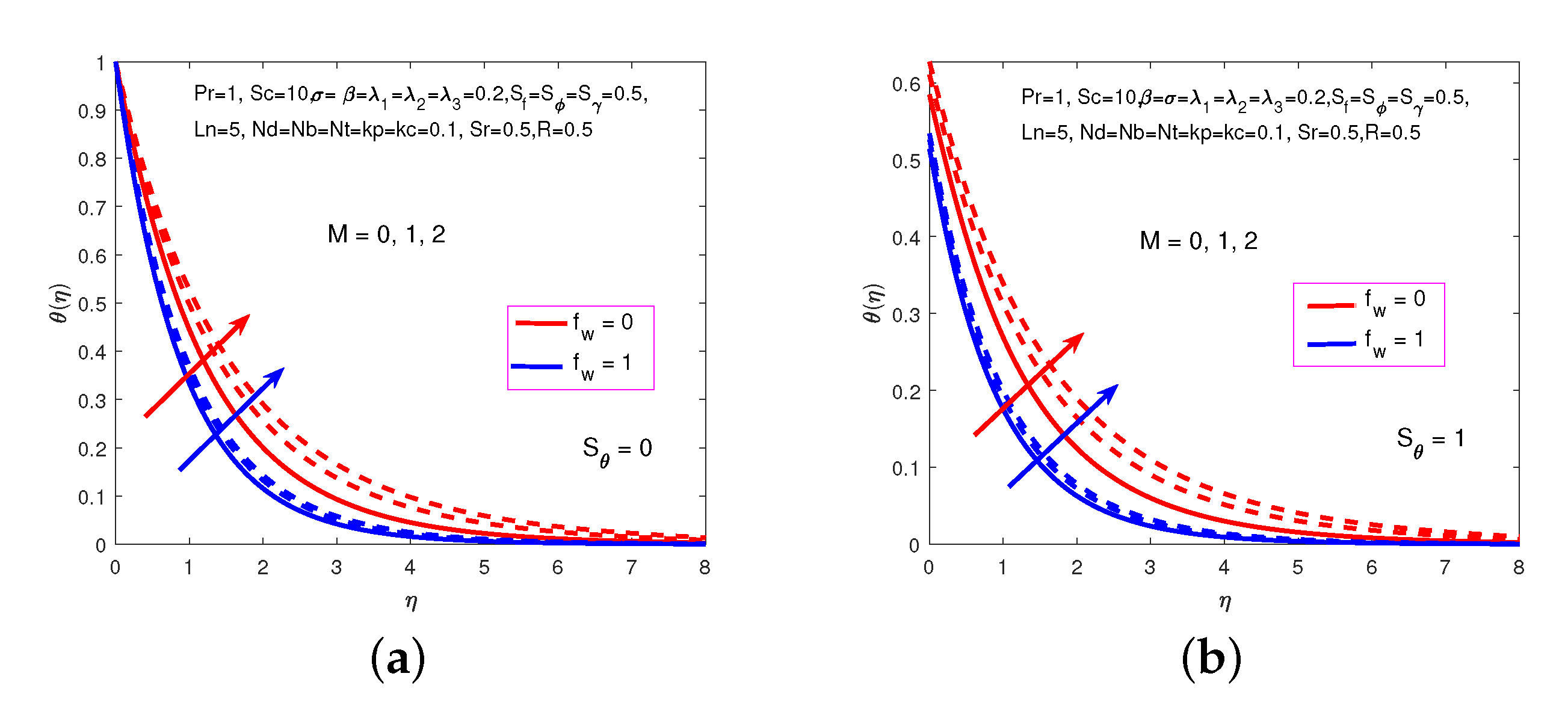
- Increments in magnetic field and Brownian motion parameters enhance the fluid temperature. However, the opposite influence is experienced with increasing values of suction and slip parameters.
- The solutal profle reduces with increasing values of the chemical reaction parameter, unsteadiness parameter, and slip parameter, but the solutal profile is enhanced with increasing values Soret number.
- Increasing the values of the thermophoresis, suction, slip, Lewis number, and unsteady parameters leads to the deceleration of the nanoparticles volume fraction profile.
- The skin friction coefficient increases with increasing rates of magnetic, suction, and unsteadiness parameters, whereas the skin friction coefficient decreases with the higher values of the slip and buoyancy parameters.
- Increments in magnetic field and thermal buoyancy parameters enhance the fluid Nusselt number but the opposite influence is experienced with increasing values of slip, solutal buoyancy, suction, and thermal radiation parameters.
- The Sherwood number increases with increasing values of Schmidt number, unsteadiness, and suction parameters, but reduces with increasing values of the Soret number, magnetic, and slip parameters.
Author Contributions
Funding
Conflicts of Interest
References
- Choi, S. Enhancing thermal conductivity of fluids with nanoparticle. In Development and Applications of Non-Newtonian Flow; 1995; Volume 31, pp. 99–105. Available online: https://pdfs.semanticscholar.org/06b5/8dc033ac26296c9231cc7b2b48611efb2ca8.pdf (accessed on 15 September 2019).
- Rao, A.S.; Prasad, V.R.; Nagendra, N.; Murthy, K.V.N.; Reddy, N.B. Numerical Modeling of Non-Similar Mixed Convection Heat Transfer over a Stretching Surface with Slip Conditions. World J. Mech. 2015, 5, 117–128. [Google Scholar] [CrossRef]
- Rana, P.; Bhargava, R. Numerical study of heat transfer enhancement in mixed convection flow along a vertical plate with heat source/sink utilizing nanofluids. Commun. Nonlinear Sci. Numer. Simul. 2011, 16, 4318–4334. [Google Scholar] [CrossRef]
- Hayat, T.; Khan, M.I.I.; Qayyum, S.; Alsaedi, A.; Khan, M.I.I. New thermodynamics of entropy generation minimization with nonlinear thermal radiation and nanomaterials. Phys. Lett. Sect. A Gen. At. Solid State Phys. 2018, 382, 749–760. [Google Scholar] [CrossRef]
- Mabood, F.; Ibrahim, S.M.; Khan, W.A. Effect of melting and heat generation/absorption on Sisko nano fluid over a stretching surface with nonlinear radiation. Phys. Scr. 2019, 94, 065701. [Google Scholar] [CrossRef]
- Rashidi, M.M.; Bagheri, S.; Momoniat, E.; Freidoonimehr, N. Entropy analysis of convective MHD flow of third grade non-Newtonian fluid over a stretching sheet. Ain Shams Eng. J. 2017, 8, 77–85. [Google Scholar] [CrossRef]
- Akbari, O.A.; Toghraie, D.; Karimipour, A.; Marzban, A.; Ahmadi, G.R. The effect of velocity and dimension of solid nanoparticles on heat transfer in non-Newtonian nanofluid. Phys. E Low-Dimens. Syst. Nanostructures 2017, 86, 68–75. [Google Scholar] [CrossRef]
- Mohyud-Din, S.T.; Khan, U.; Ahmed, N.; Rashidi, M.M. A study of heat and mass transfer on magnetohydrodynamic (MHD) flow of nanoparticles. Propuls. Power Res. 2018, 7, 72–77. [Google Scholar] [CrossRef]
- Hayat, T.; Ahmad, S.; Khan, M.I.; Alsaedi, A. Results in Physics Simulation of ferromagnetic nanomaterial flow of Maxwell fluid. Results Phys. 2018, 8, 34–40. [Google Scholar] [CrossRef]
- Pal, D. Combined effects of non-uniform heat source/sink and thermal radiation on heat transfer over an unsteady stretching permeable surface. Commun. Nonlinear Sci. Numer. Simul. 2011, 16, 1890–1904. [Google Scholar] [CrossRef]
- Raza, J. Thermal radiation and slip effects on magnetohydrodynamic (MHD) stagnation point flow of Casson fluid over a convective stretching sheet. Propuls. Power Res. 2019, 8, 138–146. [Google Scholar] [CrossRef]
- Mahanthesh, B.; Gireesha, B.J.; Thammanna, G.T.; Shehzad, S.A.; Abbasi, F.M.; Gorla, R.S.R. Nonlinear convection in nano Maxwell fluid with nonlinear thermal radiation: A three-dimensional study. Alex. Eng. J. 2018, 57, 1927–1935. [Google Scholar] [CrossRef]
- Ismail, A.I. Finite element simulation of magnetohydrodynamic convective nanofluid slip flow in porous media with nonlinear radiation. Alex. Eng. J. 2016, 55, 1305–1319. [Google Scholar] [CrossRef]
- Rana, P.; Dhanai, R.; Kumar, L. Radiative nanofluid flow and heat transfer over a nonlinear permeable sheet with slip conditions and variable magnetic field: Dual solutions. Ain Shams Eng. J. 2017, 8, 341–352. [Google Scholar] [CrossRef][Green Version]
- Ramya, D.; Raju, R.S.; Rao, J.A.; Rashidi, M.M. Boundary layer Viscous Flow of Nanofluids and Heat Transfer Over a Nonlinearly Isothermal Stretching Sheet in the Presence of Heat Generation/Absorption and Slip Boundary Conditions. Int. J. Nanosci. Nanotechnol. 2016, 12, 251–268. [Google Scholar]
- Amanulla, C.H.; Nagendra, N.; Reddy, M.S. Computational analysis of non-Newtonian boundary layer flow of nanofluid past a semi-infinite vertical plate with partial slip. Nonlinear Eng. 2018, 7, 29–43. [Google Scholar] [CrossRef]
- Bég, O.A.; Basir, M.F.M.; Uddin, M.J.; Ismail, A.I.M. Numerical Study of Slip Effects on Unsteady Asymmetric Bioconvective Nanofluid Flow in a Porous Microchannel With an Expanding/Contracting Upper Wall Using Buongiorno’s Model. J. Mech. Med. Biol. 2016, 17, 1750059. [Google Scholar] [CrossRef]
- Hayat, T.; Nassem, A.; Khan, M.I.; Farooq, M.; Al-Saedi, A. Magnetohydrodynamic (MHD) flow of nanofluid with double stratification and slip conditions. Phys. Chem. Liq. 2018, 56, 189–208. [Google Scholar] [CrossRef]
- Malarselvi, A.; Bhuvaneswari, M.; Sivasankaran, S.; Ganga, B.; Hakeem, A.K.A. Effect of Slip and Convective Heating on Unsteady MHD Chemically Reacting Flow Over a Porous Surface with Suction. In Applied Mathematics and Scientific Computing; Birkhauser: Cham, Switzerland, 2019; pp. 357–365. [Google Scholar]
- Rehman, K.U.; Malik, M.Y.; Zahri, M.; Tahir, M. Numerical analysis of MHD Casson Navier’s slip nanofluid flow yield by rigid rotating disk. Results Phys. 2018, 8, 744–751. [Google Scholar] [CrossRef]
- Ara, A.; Khan, N.A.; Khan, H.; Sultan, F. Radiation effect on boundary layer flow of an Eyring-Powell fluid over an exponentially shrinking sheet. Ain Shams Eng. J. 2014, 5, 1337–1342. [Google Scholar] [CrossRef]
- Chamkha, A.J.; Aly, A.M.; Mansour, M.A.; Education, A.; Valley, S. Similarity solution for unsteady heat and mass transfer from a stretching surface embedded in a porous medium with suction/injection and chemical reaction. Chem. Eng. Commun. 2010, 197, 37–41. [Google Scholar] [CrossRef]
- Daniel, Y.S.; Daniel, S.K. Effects of buoyancy and thermal radiation on MHD flow over a stretching porous sheet using homotopy analysis method. Alex. Eng. J. 2015, 54, 705–712. [Google Scholar] [CrossRef]
- Dhanai, R.; Rana, P.; Kumar, L. Multiple solutions of MHD boundary layer flow and heat transfer behavior of nanofluids induced by a power-law stretching/shrinking permeable sheet with viscous dissipation. Powder Technol. 2015, 273, 62–70. [Google Scholar] [CrossRef]
- Gireesha, B.J.; Ramesh, G.K.; Bagewadi, C.S. Heat transfer in MHD flow of a dusty fluid over a stretching sheet with viscous dissipation. J. Appl. Sci. Res. 2012, 3, 2392–2401. [Google Scholar]
- Haile, E.; Shankar, B. Heat and Mass Transfer in the Boundary Layer of Unsteady Viscous Nanofluid along a Vertical Stretching Sheet. J. Comput. Eng. 2014, 2014, 1–17. [Google Scholar] [CrossRef]
- Ashraf, M.; Bashir, S. Numerical simulation of MHD stagnation point flow and heat transfer of a micropolar fluid towards a heated shrinking sheet. Int. J. Numer. Methods Fluids 2012, 69, 384–398. [Google Scholar] [CrossRef]
- Dhanai, R.; Rana, P.; Kumar, L. Critical values in slip flow and heat transfer analysis of non-Newtonian nanofluid utilizing heat source/sink and variable magnetic field: Multiple solutions. J. Taiwan Inst. Chem. Eng. 2016, 58, 155–164. [Google Scholar] [CrossRef]
- Shahzad, A.; Ali, R.; Hussain, M.; Kamran, M. Unsteady axisymmetric flow and heat transfer over time-dependent radially stretching sheet. Alex. Eng. J. 2017, 56, 35–41. [Google Scholar] [CrossRef]
- Ashraf, M.; Batool, K. Mhd Flow and Heat Transfer of a Micropolar Fluid Over a Stretchable Disk. J. Theor. Appl. Mech. 2013, 51, 25–38. [Google Scholar]
- Shahzad, A.; Ahmed, J.; Khan, M. On heat transfer analysis of axisymmetric flow of viscous fluid over a nonlinear radially stretching sheet. Alex. Eng. J. 2016, 55, 2423–2429. [Google Scholar] [CrossRef]
- Chen, C.H. Laminar mixed convection adjacent to vertical, continuously stretching sheets. Heat Mass Transf.- Und Stoffuebertragung 1998, 33, 471–476. [Google Scholar] [CrossRef]
- Rana, P.; Bég, O.A. Mixed convection flow along an inclined permeable plate: Effect of magnetic field, nanolayer conductivity and nanoparticle diameter. Appl. Nanosci. 2015, 5, 569–581. [Google Scholar] [CrossRef]
- Thumma, T.; Anwar Bég, O.; Kadir, A. Numerical study of heat source/sink effects on dissipative magnetic nanofluid flow from a nonlinear inclined stretching/shrinking sheet. J. Mol. Liq. 2017, 232, 159–173. [Google Scholar] [CrossRef]
- Seth, G.S.; Sarkar, S. MHD natural convection heat and mass transfer flow past a time dependent moving vertical plate with ramped temperature in a rotating medium with hall effects, radiation and chemical reaction. J. Mech. 2015, 31, 91–104. [Google Scholar] [CrossRef]
- Takhar, H.S.; Agarwal, R.S.; Bhargava, R.; Jain, S. Mixed convection flow of a micropolar fluid over a stretching sheet. Heat Mass Transf.- Und Stoffuebertragung 1998, 34, 213–219. [Google Scholar] [CrossRef]
- Okedoye, A.M.; Akinrinmade, V.A. MHD Mixed Convection Heat and Mass Transfer Flow from Vertical Surfaces in Porous Media with Soret and Dufour Effects. J. Sci. Eng. Res. 2017, 4, 75–85. [Google Scholar]
- Ellahi, R.; Zeeshan, A.; Shehzad, N.; Alamri, S.Z. Structural impact of kerosene-Al2O3nanoliquid on MHD Poiseuille flow with variable thermal conductivity: Application of cooling process. J. Mol. Liq. 2018, 264, 607–615. [Google Scholar] [CrossRef]
- Hayat, T.; Waqas, M.; Khan, M.I.; Alsaedi, A.; Shehzad, S.A. Magnetohydrodynamic flow of burgers fluid with heat source and power law heat flux. Chin. J. Phys. 2017, 55, 318–330. [Google Scholar] [CrossRef]
- Baag, S.; Mishra, S.R.; Dash, G.C.; Acharya, M.R. Numerical investigation on MHD micropolar fluid flow toward a stagnation point on a vertical surface with heat source and chemical reaction. J. King Saud Univ.-Eng. Sci. 2017, 29, 75–83. [Google Scholar] [CrossRef]
- Singh, K. Effect of Viscous Dissipation on Double Stratified MHD Free Convection in Micropolar Fluid Flow in Porous Media with Chemical Reaction , Heat Generation and Ohmic Heating. Chem. Process. Eng. Res. 2015, 31, 75–81. [Google Scholar]
- Mabood, F.; Shateyi, S.; Rashidi, M.M.; Momoniat, E.; Freidoonimehr, N. MHD stagnation point flow heat and mass transfer of nanofluids in porous medium with radiation, viscous dissipation and chemical reaction. Adv. Powder Technol. 2016, 27, 742–749. [Google Scholar] [CrossRef]
- Hayat, T.; Khan, M.I.; Waqas, M.; Alsaedi, A. Mathematical modeling of non-Newtonian fluid with chemical aspects: A new formulation and results by numerical technique. Colloids Surf. A Physicochem. Eng. Asp. 2017, 518, 263–272. [Google Scholar] [CrossRef]
- Mahanthesh, B.; Gireesha, B.J.; Athira, P.R. Radiated flow of chemically reacting nanoliquid with an induced magnetic field across a permeable vertical plate. Results Phys. 2017, 7, 2375–2383. [Google Scholar] [CrossRef]
- Nayak, B.; Mishra, S.R.; Krishna, G.G. Chemical reaction effect of an axisymmetric flow over radially stretched sheet. Propul. Power Res. 2019, 8, 1–6. [Google Scholar] [CrossRef]
- Mabood, F. Multiple Slip Effects on MHD Unsteady Flow Heat and Mass. Model. Simul. Eng. 2019, 2019, 3052790. [Google Scholar]
- Uddin, M.; Alginahi, Y.; Bég, O.A.; Kabir, M. Numerical solutions for gyrotactic bioconvection in nanofluid-saturated porous media with Stefan blowing and multiple slip effects. Comput. Math. Appl. 2016, 72, 2562–2581. [Google Scholar] [CrossRef]
- Singh, B.; Gupta, D.; Kumar, L.; Anwar, O.B. Finite Element Analysis of MHD Flow of Micropolar Fluid over a Shrinking Sheet with a Convective Surface Boundary Condition. J. Eng. Thermophys. 2018, 27, 202–203. [Google Scholar] [CrossRef]
- Bhargava, R.; Rana, P. Finite element solution to mixed convection in MHD flow of micropolar fluid along a moving vertical cylinder with variable conductivity. Int. J. Appl. Math Mech. 2011, 7, 29–51. [Google Scholar]
- Rana, P.; Bhargava, R.; Bég, O.A. Finite element simulation of unsteady magneto-hydrodynamic transport phenomena on a stretching sheet in a rotating nanofluid. Proc. Inst. Mech. Eng. Part N J. Nanoeng. Nanosyst. 2013, 227, 77–99. [Google Scholar] [CrossRef]
- Reddy, J. Solutions manual for An introduction to the finite element method. In Essentials of Finite Element Method; Elsevier: Amsterdam, The Netherlands, 1993; p. 41. [Google Scholar]
- Swapna, G.; Kumar, L.; Rana, P.; Singh, B. Finite element modeling of a double-diffusive mixed convection flow of a chemically-reacting magneto-micropolar fluid with convective boundary condition. J. Taiwan Inst. Chem. Eng. 2015, 47, 18–27. [Google Scholar] [CrossRef]
- Kumar, L. Finite-element analysis of transient heat and mass transfer in microstructural boundary layer flow from a porous stretching sheet finite-element analysis of transient heat and mass transfer in microstructural boundary layer flow from a porous. Comput. Therm. Sci. Int. J. 2014, 6, 155–169. [Google Scholar] [CrossRef]
- Crane, L.J. Flow past a stretching plate. Z. Für Angew. Math. Und Phys. ZAMP 1970, 21, 645–647. [Google Scholar] [CrossRef]
- Jalil, M.; Asghar, S.; Yasmeen, S. An Exact Solution of MHD Boundary Layer Flow of Dusty Fluid over a Stretching Surface. Math. Probl. Eng. 2017, 2017, 1–5. [Google Scholar] [CrossRef]
- Aii, M.E.; Chamkha, A.J.; Aly, A.M.; Mansour, M.A.; Education, A.; Valley, S.; Mabood, F.; Das, K. Melting heat transfer on hydromagnetic flow of a nanofluid over a stretching sheet with radiation and second-order slip. Eur. Phys. J. Plus 2016, 234, 227–234. [Google Scholar] [CrossRef]
- Ishak, A.; Nazar, R.; Pop, I. Boundary layer flow and heat transfer over an unsteady stretching vertical surface. Meccanica 2009, 44, 369–375. [Google Scholar] [CrossRef]
- Aii, M.E. Heat transfer characteristics of a continuous stretching surface. Warme-und Stoffubertragung 1994, 29, 227–234. [Google Scholar]








| Number of Elements | ||||
|---|---|---|---|---|
| 300 | 0.12365 | 0.18517 | 0.06336 | 0.02773 |
| 600 | 0.12390 | 0.18506 | 0.06332 | 0.02772 |
| 900 | 0.12399 | 0.18503 | 0.06331 | 0.02771 |
| 1200 | 0.12403 | 0.18501 | 0.06330 | 0.02770 |
| 1500 | 0.12406 | 0.18500 | 0.06330 | 0.02770 |
| 1800 | 0.12408 | 0.18499 | 0.06330 | 0.02770 |
| 2100 | 0.12409 | 0.18499 | 0.06330 | 0.02770 |
| Crane [54] | FEM | Error in % | Crane [54] | FEM | Error in % | ||
|---|---|---|---|---|---|---|---|
| (a) Exact Solution | (b) (Our Results) | (a) Exact Solution | (b) (Our Results) | ||||
| 0 | 1.0000 | 1.00000 | 0.00000 | 5 | 0.0067 | 0.00670 | 0.00000 |
| 1 | 0.1379 | 0.13786 | 0.02901 | 6 | 0.0025 | 0.00251 | 0.40000 |
| 2 | 0.1353 | 0.13531 | 0.00739 | 7 | 0.0009 | 0.00091 | 1.11111 |
| 3 | 0.0498 | 0.04978 | 0.04016 | 8 | 0.0003 | 0.00033 | 3.33333 |
| 4 | 0.0183 | 0.01831 | 0.05464 | 9 | 0.0001 | 0.00011 | 10.0000 |
| M | Gireesha et al. [25] | Mudassar et al. [55] | FEM | Error in % |
|---|---|---|---|---|
| (a) Exact Dolution | (b) (Our Results) | |||
| 0.0 | 1.000 | 1.000000 | 1.0000080 | 0.00080 |
| 0.2 | 1.095 | 1.095445 | 1.0954458 | 0.00007 |
| 0.5 | 1.224 | 1.224745 | 1.2247446 | 0.00003 |
| 1.0 | 1.414 | 1.414214 | 1.4142132 | 0.00006 |
| 1.2 | 1.483 | 1.483240 | 1.4832393 | 0.00005 |
| 1.5 | 1.581 | 1.581139 | 1.5811384 | 0.00004 |
| 2.0 | 1.732 | 1.732051 | 1.7320504 | 0.00003 |
| M | [56] | [46] | FEM (Present) | [22] | [46] | FEM (Present) | |
|---|---|---|---|---|---|---|---|
| 0 | −1.000008 | −1.0000084 | −1.0000082 | 0.2 | - | - | 1.068027 |
| 1 | 1.4142135 | 1.41421356 | 1.41421353 | 0.4 | - | - | 1.134687 |
| 5 | 2.4494897 | 2.44948974 | 2.44948963 | 0.6 | - | - | 1.199118 |
| 10 | 3.3166247 | 3.31662479 | 3.31662463 | 0.8 | 1.261512 | 1.261042 | 1.261042 |
| 50 | 7.1414284 | 7.14142843 | 7.14142839 | 1.0 | - | - | 1.320522 |
| 100 | 10.049875 | 10.0498756 | 10.0498751 | 1.2 | 1.378052 | 1.377724 | 1.377724 |
| 500 | 22.383029 | 22.3830293 | 22.3830283 | 1.4 | - | - | 1.432836 |
| 1000 | 31.638584 | 31.6385840 | 31.6385833 | 1.6 | - | - | 1.486039 |
| Pr | Aii | Fazle et al. | Ishak et al. | Dulal Pal. | Haile et al. | Ishak et al. [57] | FEM | Error in % |
|---|---|---|---|---|---|---|---|---|
| [58] | [46] | [57] | [10] | [26] | (a) Exact solution | (b) (Our results) | ||
| 0.72 | 0.8058 | 0.8088 | - | - | - | 0.8086313498 | 0.8086339299 | 0.0003 |
| 1.00 | 0.9691 | 1.0000 | 1.0000 | 1.0000 | 1.0004 | 1.000000000 | 1.0000080213 | 0.0008 |
| 3.00 | 1.9144 | 1.9237 | 1.9237 | 1.9236 | 1.9234 | 1.923682594 | 1.9236777221 | 0.0003 |
| 10.0 | 3.7006 | 3.7207 | 3.7207 | 3.7207 | 3.7205 | 3.720673901 | 3.7206681683 | 0.0002 |
| 100 | - | - | 12.2941 | 12.2940 | 12.2962 | 12.294083260 | 12.294051659 | 0.0003 |
| Pr | Sc | Chamkha et al. [22] | FEM (Our Results) | ||||
|---|---|---|---|---|---|---|---|
| , | , | ||||||
| 0.71 | 0.22 | 0.27377 | 1.158393 | 0.740140 | 0.27371 | 1.158389 | 0.740160 |
| 0.60 | 0.49677 | 1.101087 | 1.312248 | 0.49669 | 1.101082 | 1.312192 | |
| 0.94 | 0.59941 | 1.076931 | 1.713443 | 0.59935 | 1.076899 | 1.713358 | |
| 0.3 | 0.62 | 0.38639 | 0.621980 | 1.355045 | 0.38655 | 0.621892 | 1.355036 |
| 0.71 | 0.50438 | 1.099032 | 1.337571 | 0.50429 | 1.099018 | 1.337267 | |
| 1.0 | 0.55244 | 1.384875 | 1.331027 | 0.55237 | 1.384753 | 1.331856 | |
| 3.0 | 0.69042 | 2.966999 | 1.316710 | 0.69036 | 2.966894 | 1.316382 | |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ali, B.; Nie, Y.; Khan, S.A.; Sadiq, M.T.; Tariq, M. Finite Element Simulation of Multiple Slip Effects on MHD Unsteady Maxwell Nanofluid Flow over a Permeable Stretching Sheet with Radiation and Thermo-Diffusion in the Presence of Chemical Reaction. Processes 2019, 7, 628. https://doi.org/10.3390/pr7090628
Ali B, Nie Y, Khan SA, Sadiq MT, Tariq M. Finite Element Simulation of Multiple Slip Effects on MHD Unsteady Maxwell Nanofluid Flow over a Permeable Stretching Sheet with Radiation and Thermo-Diffusion in the Presence of Chemical Reaction. Processes. 2019; 7(9):628. https://doi.org/10.3390/pr7090628
Chicago/Turabian StyleAli, Bagh, Yufeng Nie, Shahid Ali Khan, Muhammad Tariq Sadiq, and Momina Tariq. 2019. "Finite Element Simulation of Multiple Slip Effects on MHD Unsteady Maxwell Nanofluid Flow over a Permeable Stretching Sheet with Radiation and Thermo-Diffusion in the Presence of Chemical Reaction" Processes 7, no. 9: 628. https://doi.org/10.3390/pr7090628
APA StyleAli, B., Nie, Y., Khan, S. A., Sadiq, M. T., & Tariq, M. (2019). Finite Element Simulation of Multiple Slip Effects on MHD Unsteady Maxwell Nanofluid Flow over a Permeable Stretching Sheet with Radiation and Thermo-Diffusion in the Presence of Chemical Reaction. Processes, 7(9), 628. https://doi.org/10.3390/pr7090628







