Discrete Element Method (DEM) and Experimental Studies of the Angle of Repose and Porosity Distribution of Pellet Pile
Abstract
1. Introduction
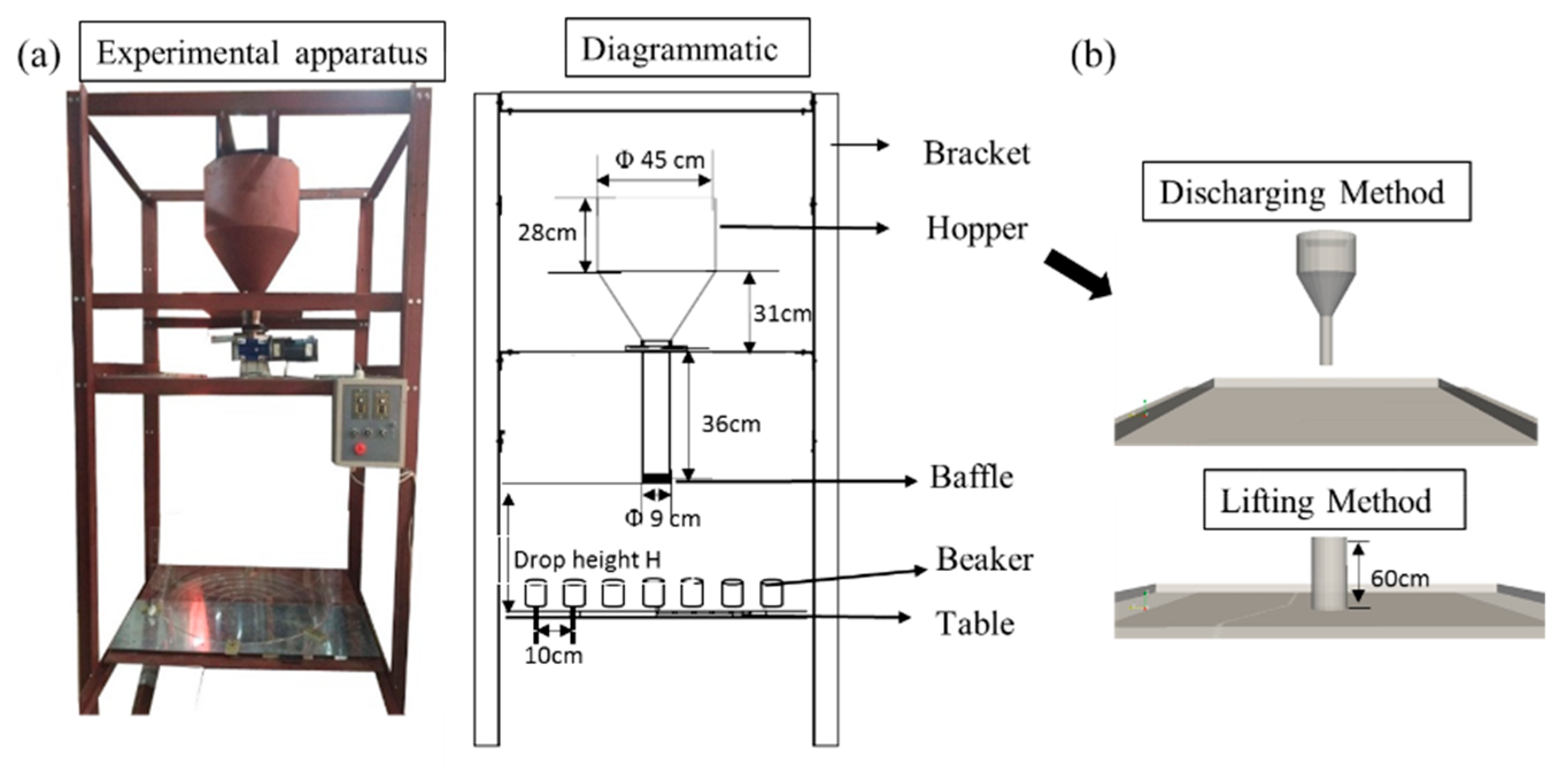
2. Experimental Work
3. Simulation Method and Conditions
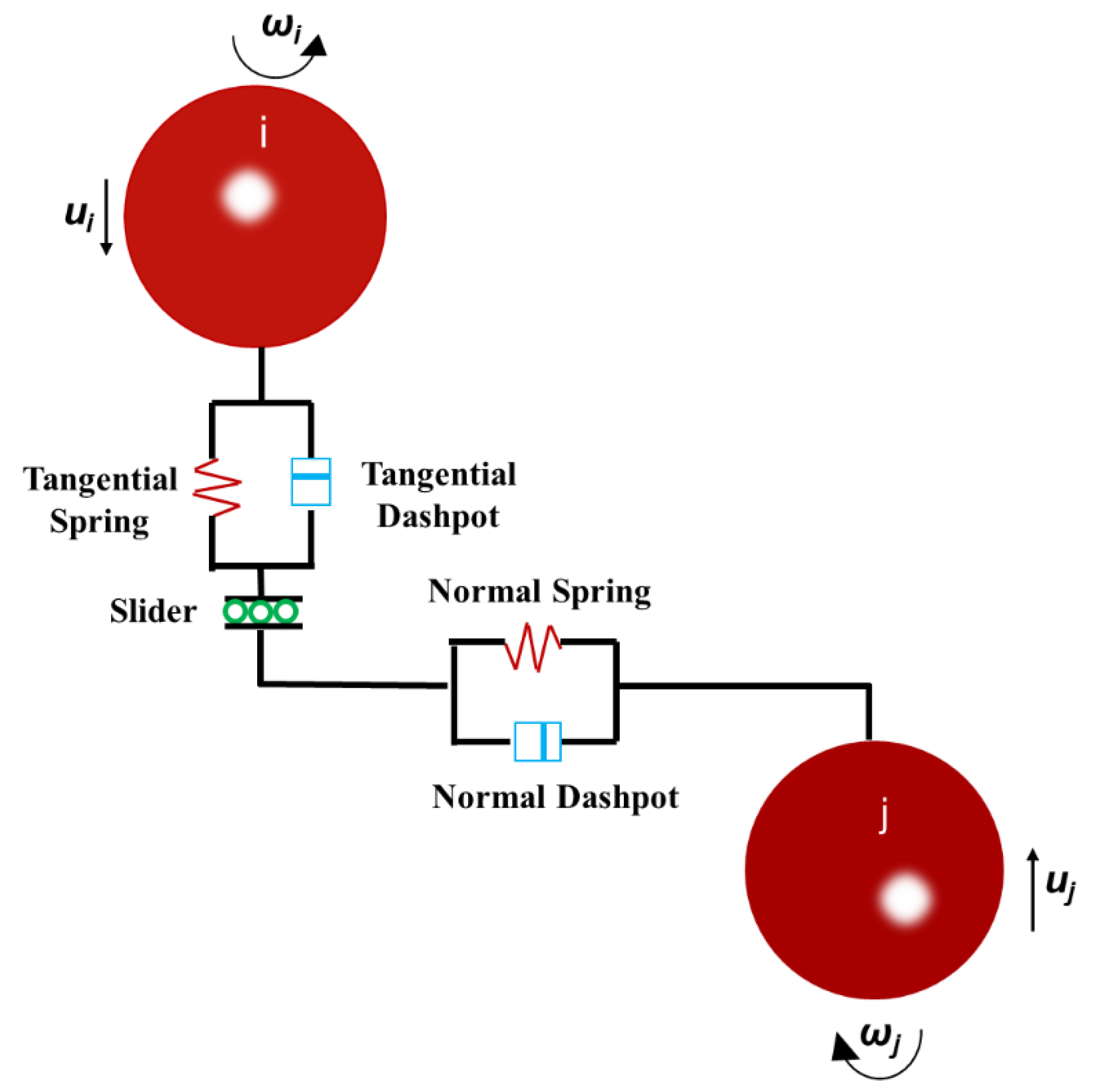
3.1. Discrete Element Method (DEM)
3.2. Simulation Conditions
4. Results and Discussion
4.1. Simulation and Experimental Study of Angle of Repose
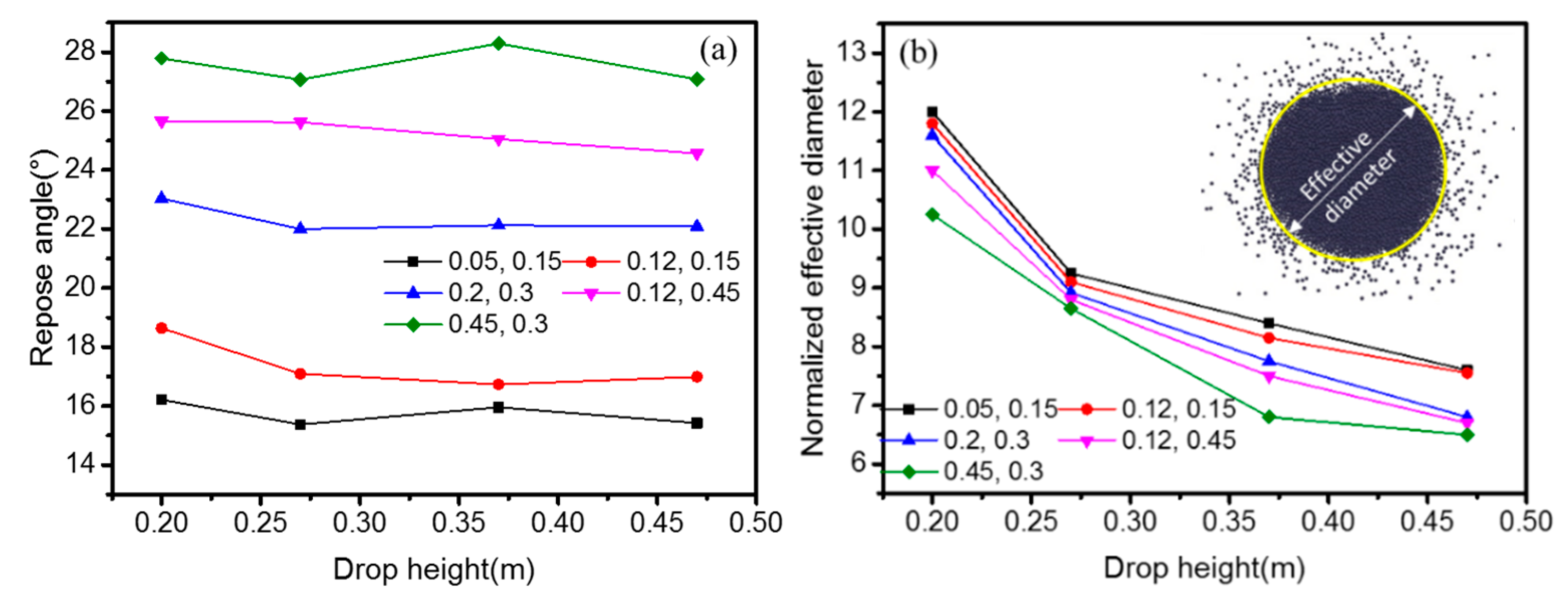
4.1.1. Angle of Repose by the Discharging Method
4.1.2. The angle of Repose by the Lifting Method
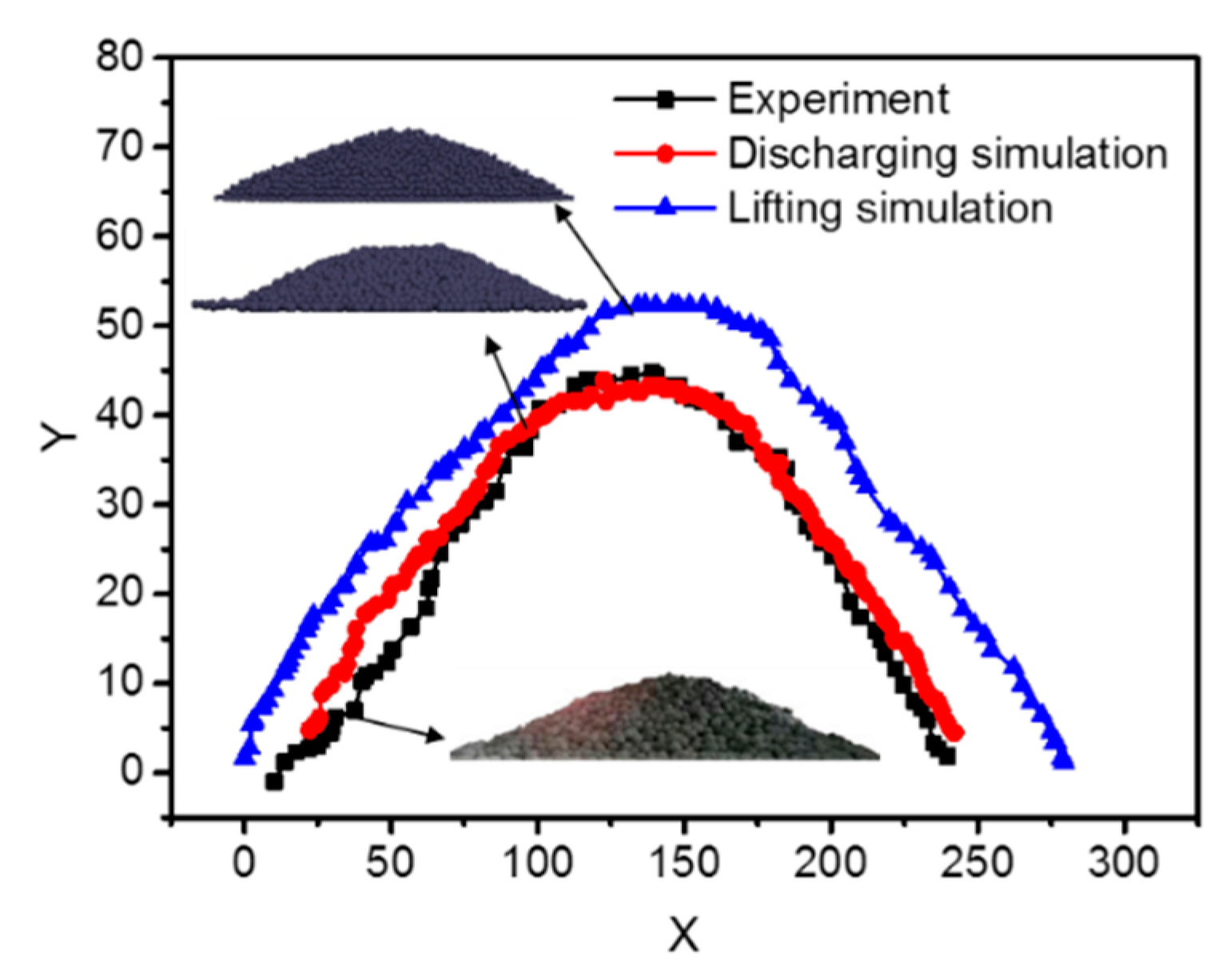
4.1.3. Simulated vs. Experimental Angles of Repose
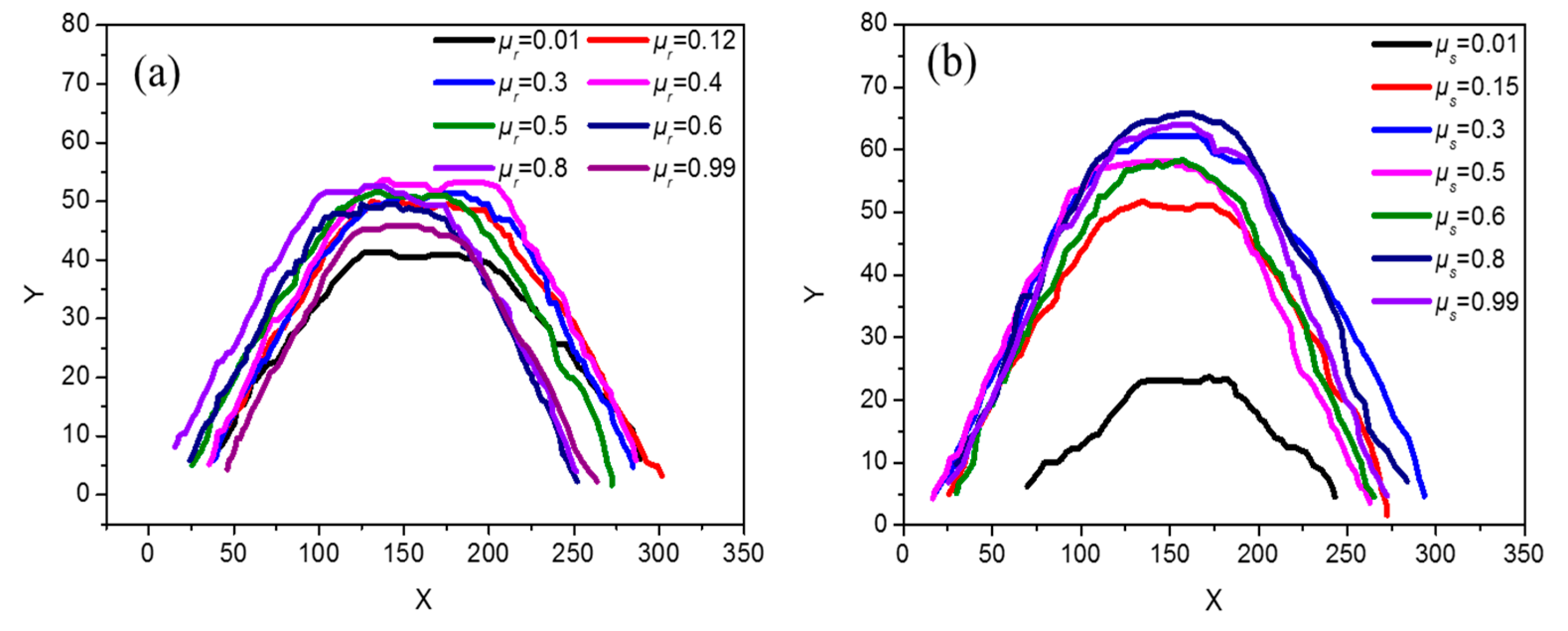
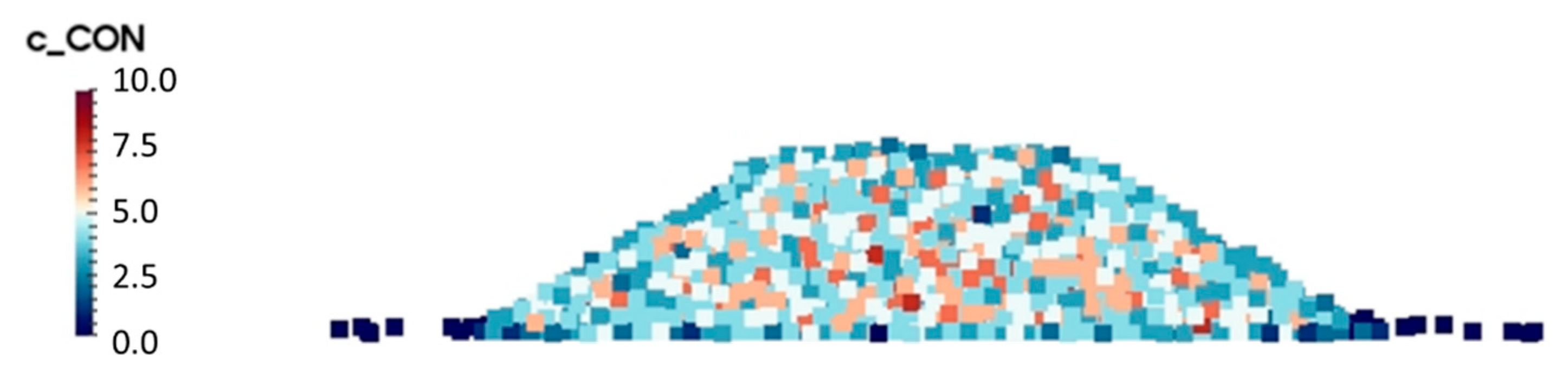
4.2. Porosity Distribution of Pellet Pile
4.2.1. Simulated vs. Experimental BPD
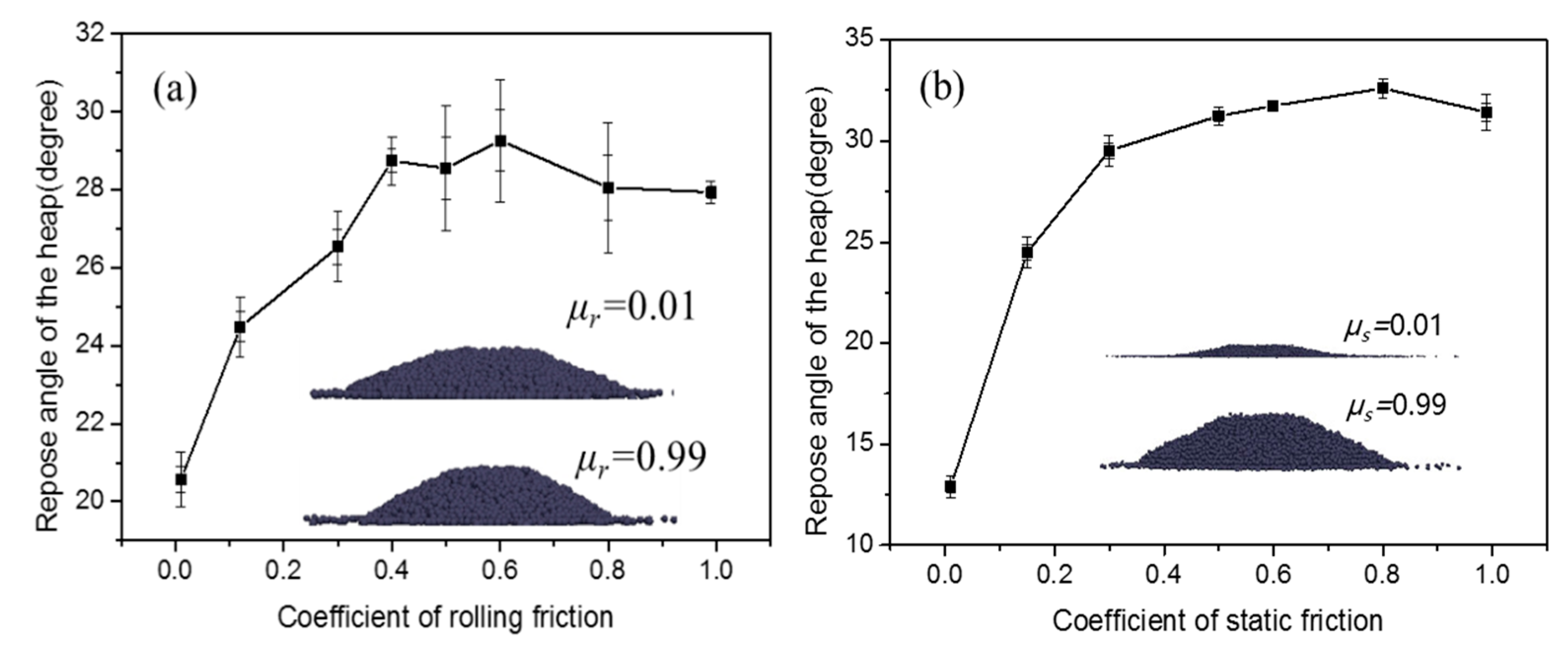
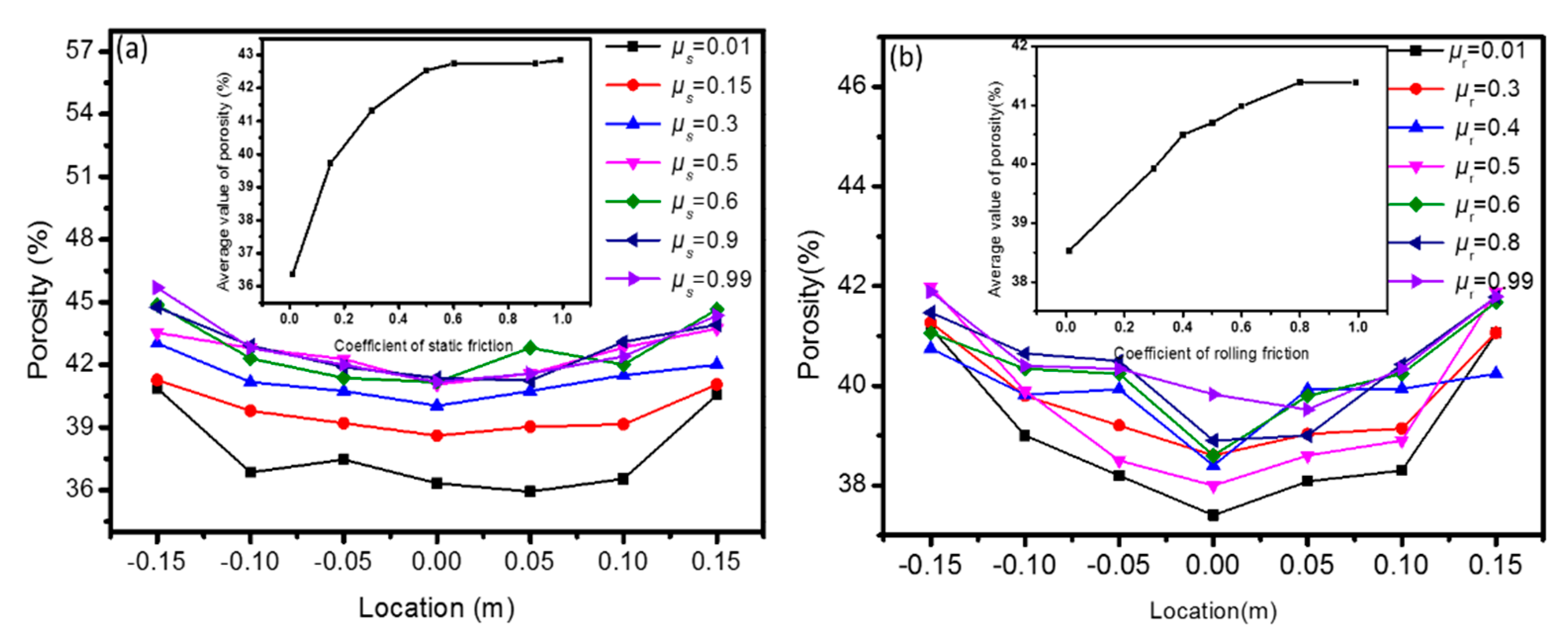
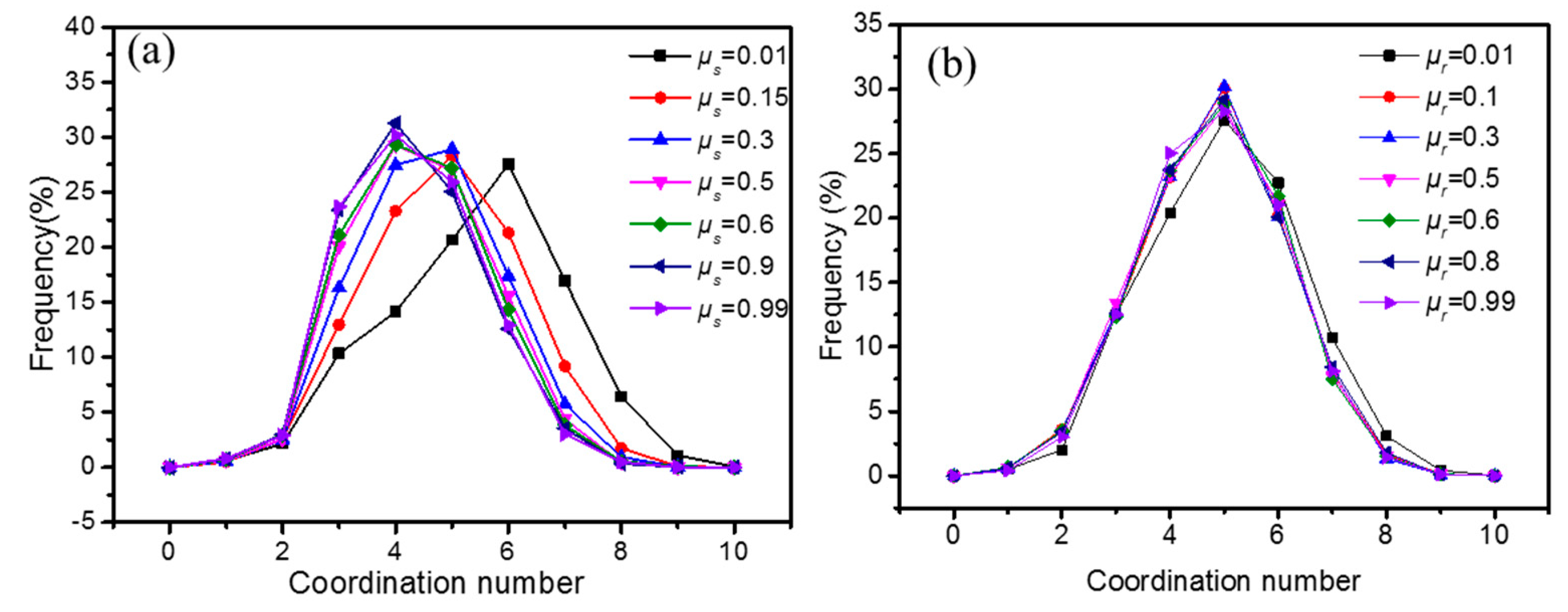
4.2.2. Effects of Rolling and Static Friction on BPD
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Kou, B.; Cao, Y.; Li, J.; Xia, C.; Li, Z.; Dong, H.; Zhang, A.; Zhang, J.; Kob, W.; Wang, Y. Granular materials flow like complex fluids. Nature 2017, 551, 360–363. [Google Scholar] [CrossRef] [PubMed]
- Zhou, Z.Y.; Zou, R.P.; Pinson, D.; Yu, A.B.; Zhou, Z. Angle of repose and stress distribution of sandpiles formed with ellipsoidal particles. Granul. Matter 2014, 16, 695–709. [Google Scholar] [CrossRef]
- Van Burkalow, A. Angle of Repose and Angle of Sliding Friction: AN Experimental Study. Geol. Soc. Am. Bull. 1945, 56, 669. [Google Scholar] [CrossRef]
- Elperin, T.; Golshtein, E. Comparison of different models for tangential forces using the particle dynamics method. Phys. A Stat. Mech. Appl. 1997, 242, 332–340. [Google Scholar] [CrossRef]
- Coetzee, C. Calibration of the discrete element method and the effect of particle shape. Powder Technol. 2016, 297, 50–70. [Google Scholar] [CrossRef]
- Alizadeh, M.; Hassanpour, A.; Pasha, M.; Ghadiri, M.; Bayly, A. The effect of particle shape on predicted segregation in binary powder mixtures. Powder Technol. 2017, 319, 313–322. [Google Scholar] [CrossRef]
- Dury, A.; Ristow, G.H.; Moss, J.L.; Nakagawa, M. Boundary Effects on the Angle of Repose in Rotating Cylinders. Phys. Rev. E Stat. Phys. Plasmas Fluids Relat. Interdiscip. Top. 1997, 57, 4491–4497. [Google Scholar] [CrossRef]
- Carstensen, J.; Chan, P.-C. Relation between particle size and repose angles of powders. Powder Technol. 1976, 15, 129–131. [Google Scholar] [CrossRef]
- Nan, W.; Wang, Y.; Ge, Y.; Wang, J. Effect of shape parameters of fiber on the packing structure. Powder Technol. 2014, 261, 210–218. [Google Scholar] [CrossRef]
- Zou, R.; Yu, A. Evaluation of the packing characteristics of mono-sized non-spherical particles. Powder Technol. 1996, 88, 71–79. [Google Scholar] [CrossRef]
- Gan, J.; Yu, A.; Zhou, Z. DEM simulation on the packing of fine ellipsoids. Chem. Eng. Sci. 2016, 156, 64–76. [Google Scholar] [CrossRef]
- Bernal, J.D.; Mason, J. Packing of Spheres: Co-ordination of Randomly Packed Spheres. Nature 1960, 188, 910–911. [Google Scholar] [CrossRef]
- Scott, G.D.; Kilgour, D.M. The density of random close packing of spheres. J. Phys. D Appl. Phys. 1969, 2, 863–866. [Google Scholar] [CrossRef]
- Mueller, G.E. Numerically packing spheres in cylinders. Powder Technol. 2005, 159, 105–110. [Google Scholar] [CrossRef]
- Zhao, J.; Li, S.; Jin, W.; Zhou, X. Shape effects on the random-packing density of tetrahedral particles. Phys. Rev. E 2012, 86, 03131–031306. [Google Scholar] [CrossRef] [PubMed]
- Tangri, H.; Guo, Y.; Curtis, J.S. Packing of cylindrical particles: DEM simulations and experimental measurements. Powder Technol. 2017, 317, 72–82. [Google Scholar] [CrossRef]
- Lu, P.; Li, S.; Zhao, J.; Meng, L. A computational investigation on random packings of sphere-spherocylinder mixtures. Sci. China Ser. G Phys. Mech. Astron. 2010, 53, 2284–2292. [Google Scholar] [CrossRef]
- Wouterse, A.; Williams, S.R.; Philipse, A.P. Effect of particle shape on the density and microstructure of random packings. J. Phys. Condens. Matter 2007, 19, 406215. [Google Scholar] [CrossRef]
- Kyrylyuk, A.V.; Philipse, A.P. Effect of particle shape on the random packing density of amorphous solids. Phys. Status Solidi A 2011, 208, 2299–2302. [Google Scholar] [CrossRef]
- Abreu, C.R.; Tavares, F.W.; Castier, M. Influence of particle shape on the packing and on the segregation of spherocylinders via Monte Carlo simulations. Powder Technol. 2003, 134, 167–180. [Google Scholar] [CrossRef]
- Natsui, S.; Ueda, S.; Nogami, H.; Kano, J.; Inoue, R.; Ariyama, T. Analysis on Non-Uniform Gas Flow in Blast Furnace Based on DEM-CFD Combined Model. Steel Res. Int. 2011, 82, 964–971. [Google Scholar] [CrossRef]
- Wei, H.; Zan, L.; Zhang, H.; Saxen, H.; Yu, Y. Two Methods of DEM Application on Charging System of Ironmaking Blast Furnace. In Proceedings of the 2018 37th Chinese Control Conference (CCC), Wuhan, China, 25–27 July 2018; pp. 3412–3415. [Google Scholar]
- Yang, W.; Zhou, Z.; Pinson, D.; Yu, A. A New Approach for Studying Softening and Melting Behavior of Particles in a Blast Furnace Cohesive Zone. Metall. Mater. Trans. B 2015, 46, 977–992. [Google Scholar] [CrossRef]
- Geleta, D.D.; Lee, J. Effects of Particle Diameter and Coke Layer Thickness on Solid Flow and Stress Distribution in BF by 3D Discrete Element Method. Met. Mater. Trans. A 2018, 49, 3594–3602. [Google Scholar] [CrossRef]
- Wei, H.; Tang, X.; Ge, Y.; Li, M.; Saxén, H.; Yu, Y. Numerical and experimental studies of the effect of iron ore particle shape on repose angle and porosity of a heap. Powder Technol. 2019, 353, 526–534. [Google Scholar] [CrossRef]
- Cundall, P.A.; Strack, O.D.L. Discussion: A discrete numerical model for granular assemblies. Geotechnique 2008, 29, 331–336. [Google Scholar] [CrossRef]
- Tsuji, Y.; Tanaka, T.; Ishida, T. Lagrangian numerical simulation of plug flow of cohesionless particles in a horizontal pipe. Powder Technol. 1992, 71, 239–250. [Google Scholar] [CrossRef]
- Goniva, C.; Kloss, C.; Hager, A.; Pirker, S. An Open Source CFD-DEM Perspective; JKU: Linz, Austria, 2010. [Google Scholar]
- Yu, Y.; Saxén, H. Experimental and DEM study of segregation of ternary size particles in a blast furnace top bunker model. Chem. Eng. Sci. 2010, 65, 5237–5250. [Google Scholar] [CrossRef]
- Li, C.; Honeyands, T.; O’Dea, D.; Moreno-Atanasio, R. The angle of repose and size segregation of iron ore granules: DEM analysis and experimental investigation. Powder Technol. 2017, 320, 257–272. [Google Scholar] [CrossRef]
- Zhou, Y.C.; Xu, B.H.; Yu, A.B.; Zulli, P. Numerical investigation of the angle of repose of monosized spheres. Phys. Rev. E 2001, 64, 021301. [Google Scholar] [CrossRef] [PubMed]
- Zhou, Y.; Xu, B.; Yu, A.; Zulli, P. An experimental and numerical study of the angle of repose of coarse spheres. Powder Technol. 2002, 125, 45–54. [Google Scholar] [CrossRef]
- Zhou, Y.; Wright, B.; Yang, R.; Xu, B.; Yu, A. Rolling friction in the dynamic simulation of sandpile formation. Phys. A Stat. Mech. Appl. 1999, 269, 536–553. [Google Scholar] [CrossRef]




| Parameters | Equations |
|---|---|
| Kn, Kt | , |
| γn, γt | |
| Sn, St | |
| β | |
| Parameters | Values |
|---|---|
| Particle number | 100,000 |
| Particle density | 4837 kg/m3 |
| Time step | 10−5 s |
| Young’s modulus | 2.5 × 1011 Pa (pellet), 2 × 1011 Pa (steel plane), 7.2 × 1010 (plexiglass) |
| Poisson ratio (p-p; p-w; p-g) | 0.25, 0.3, 0.2 |
| Coefficient of restitution (p-p; p-w; p-g) | 0.4, 0.35, 0.2 |
| Coefficient of friction (p-w; p-g) | 0.4, 0.25 |
| Rolling friction coefficient (p-w; p-g) | 0.4, 0.15 |
| Size of pellet | 8 mm, 14 mm, 20 mm |
| Cases | Rolling Friction Coefficient | Static Friction Coefficient | Barrel Size (Diameter: m) |
|---|---|---|---|
| Case1 | 0.05 | 0.15 | 0.1 |
| Case2 | 0.15 | ||
| Case3 | 0.12 | 0.15 | |
| Case4 | 0.12 | 0.45 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wei, H.; Li, M.; Li, Y.; Ge, Y.; Saxén, H.; Yu, Y. Discrete Element Method (DEM) and Experimental Studies of the Angle of Repose and Porosity Distribution of Pellet Pile. Processes 2019, 7, 561. https://doi.org/10.3390/pr7090561
Wei H, Li M, Li Y, Ge Y, Saxén H, Yu Y. Discrete Element Method (DEM) and Experimental Studies of the Angle of Repose and Porosity Distribution of Pellet Pile. Processes. 2019; 7(9):561. https://doi.org/10.3390/pr7090561
Chicago/Turabian StyleWei, Han, Meng Li, Ying Li, Yao Ge, Henrik Saxén, and Yaowei Yu. 2019. "Discrete Element Method (DEM) and Experimental Studies of the Angle of Repose and Porosity Distribution of Pellet Pile" Processes 7, no. 9: 561. https://doi.org/10.3390/pr7090561
APA StyleWei, H., Li, M., Li, Y., Ge, Y., Saxén, H., & Yu, Y. (2019). Discrete Element Method (DEM) and Experimental Studies of the Angle of Repose and Porosity Distribution of Pellet Pile. Processes, 7(9), 561. https://doi.org/10.3390/pr7090561







