Theoretical Analysis of Forced Segmented Temperature Gradients in Liquid Chromatography
Abstract
1. Introduction
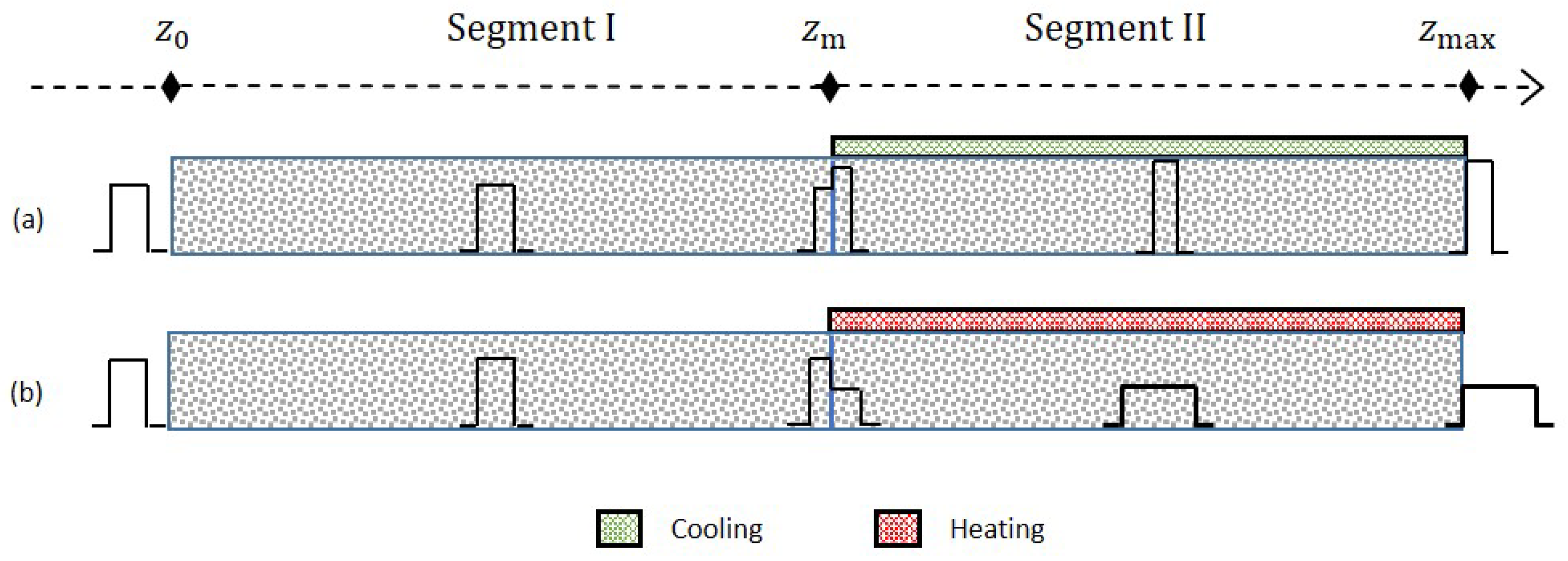
2. Non-Isothermal Equilibrium Model
3. Analytical Solution
Performance of the Chromatographic Process
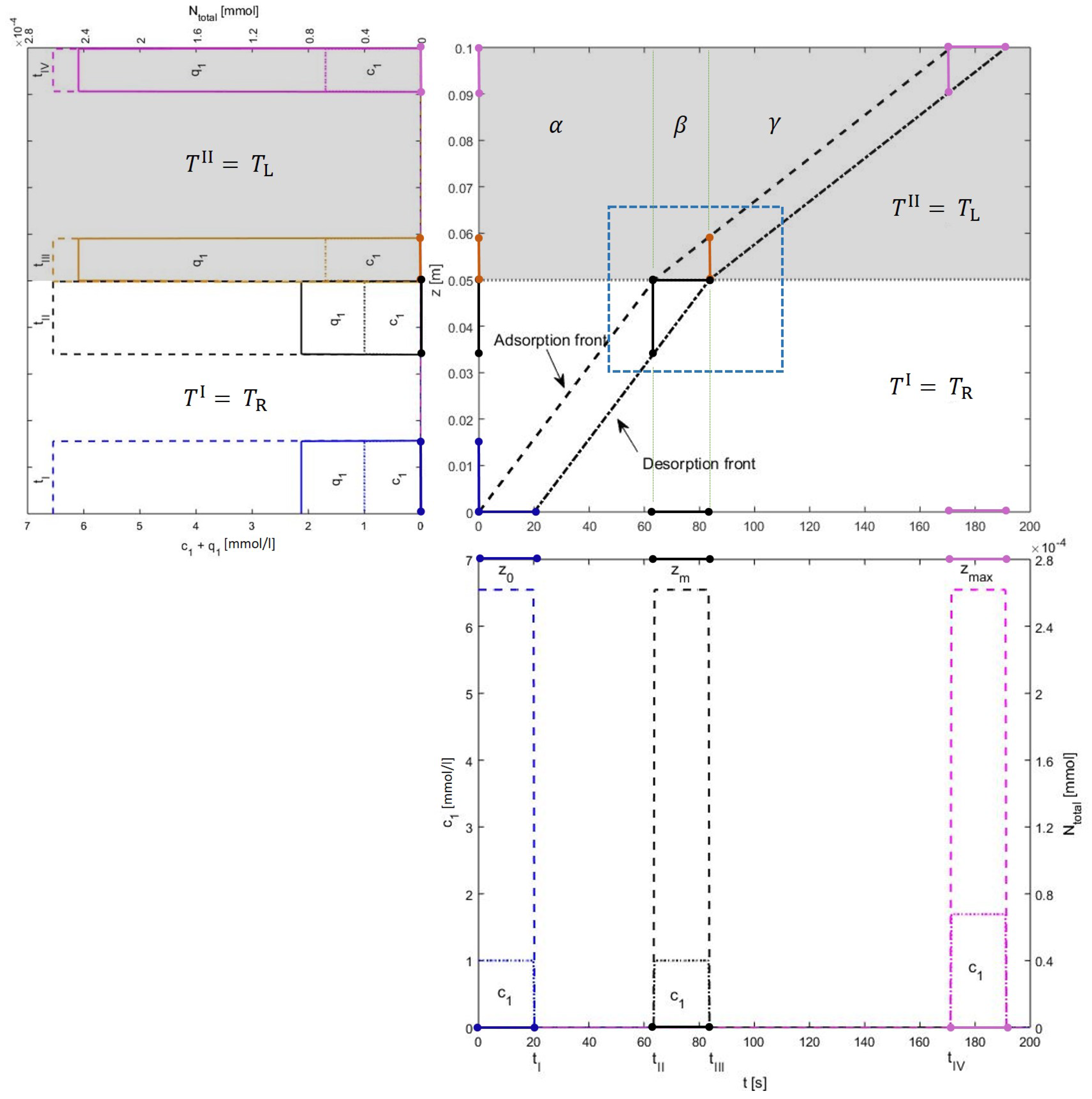
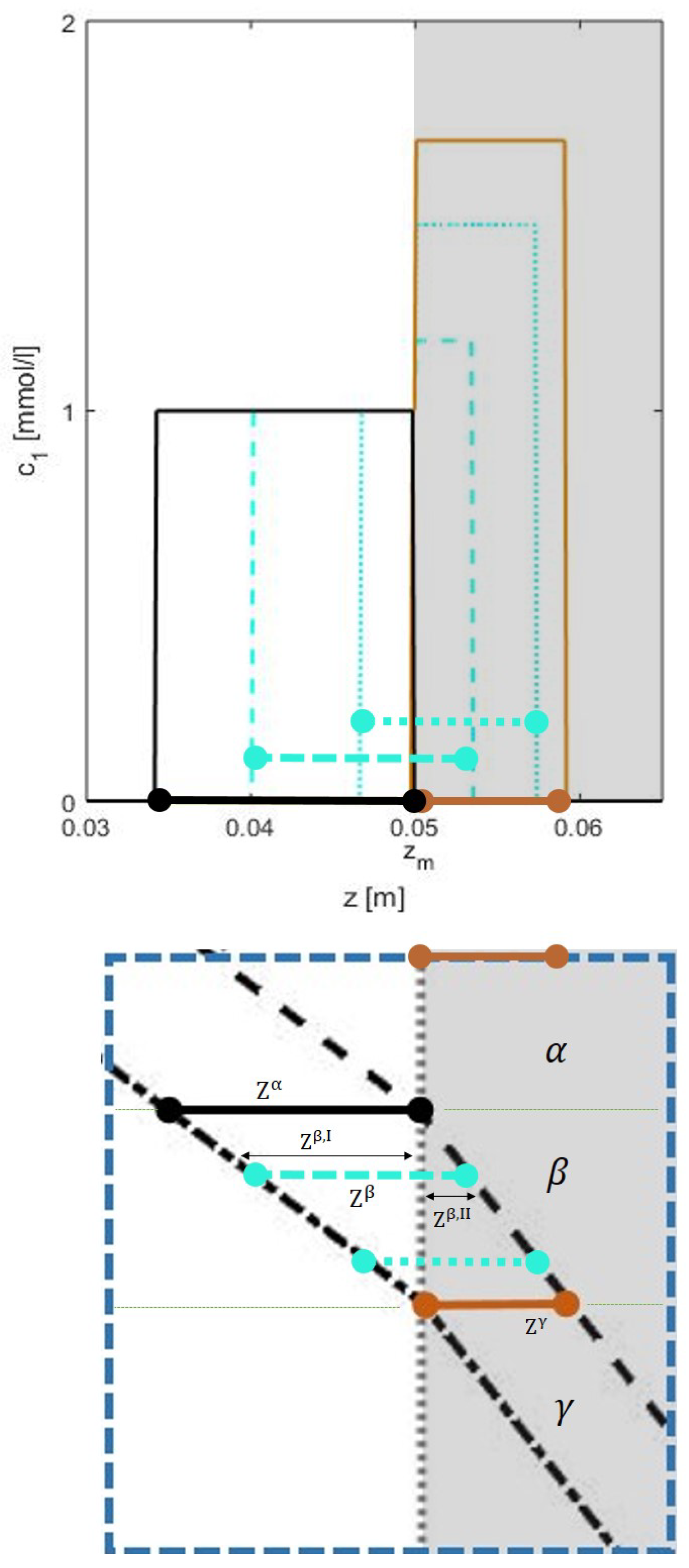
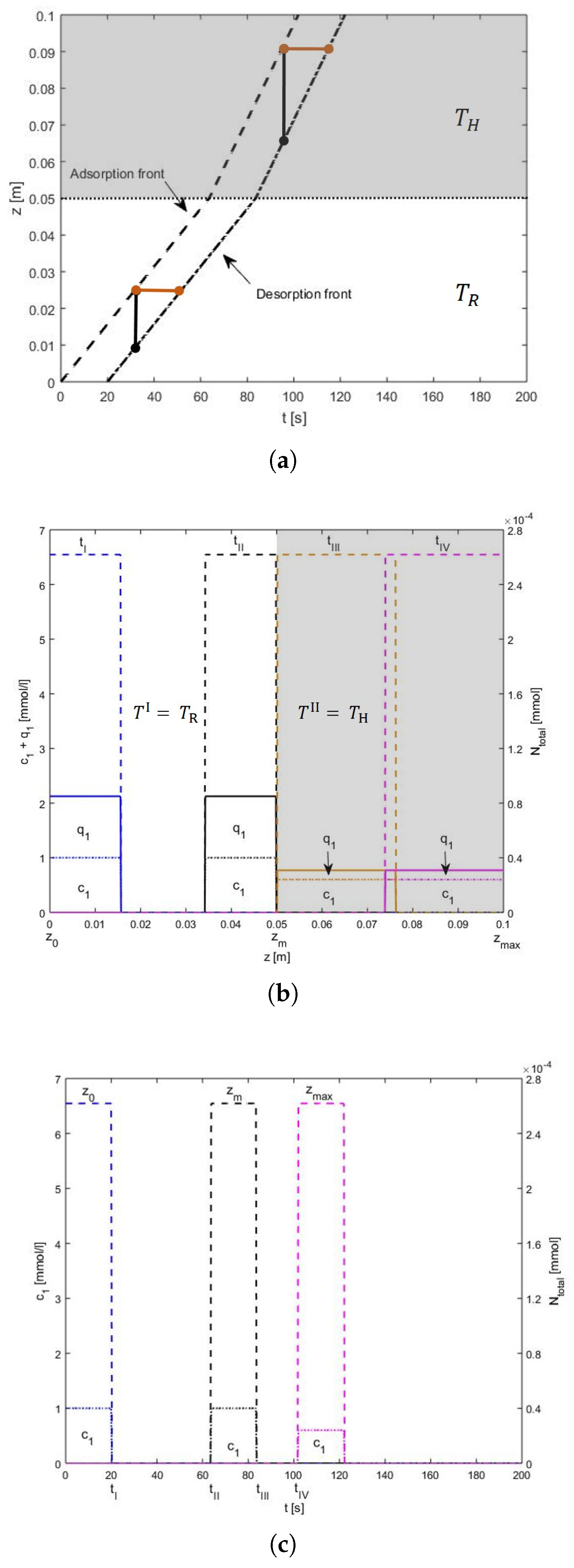
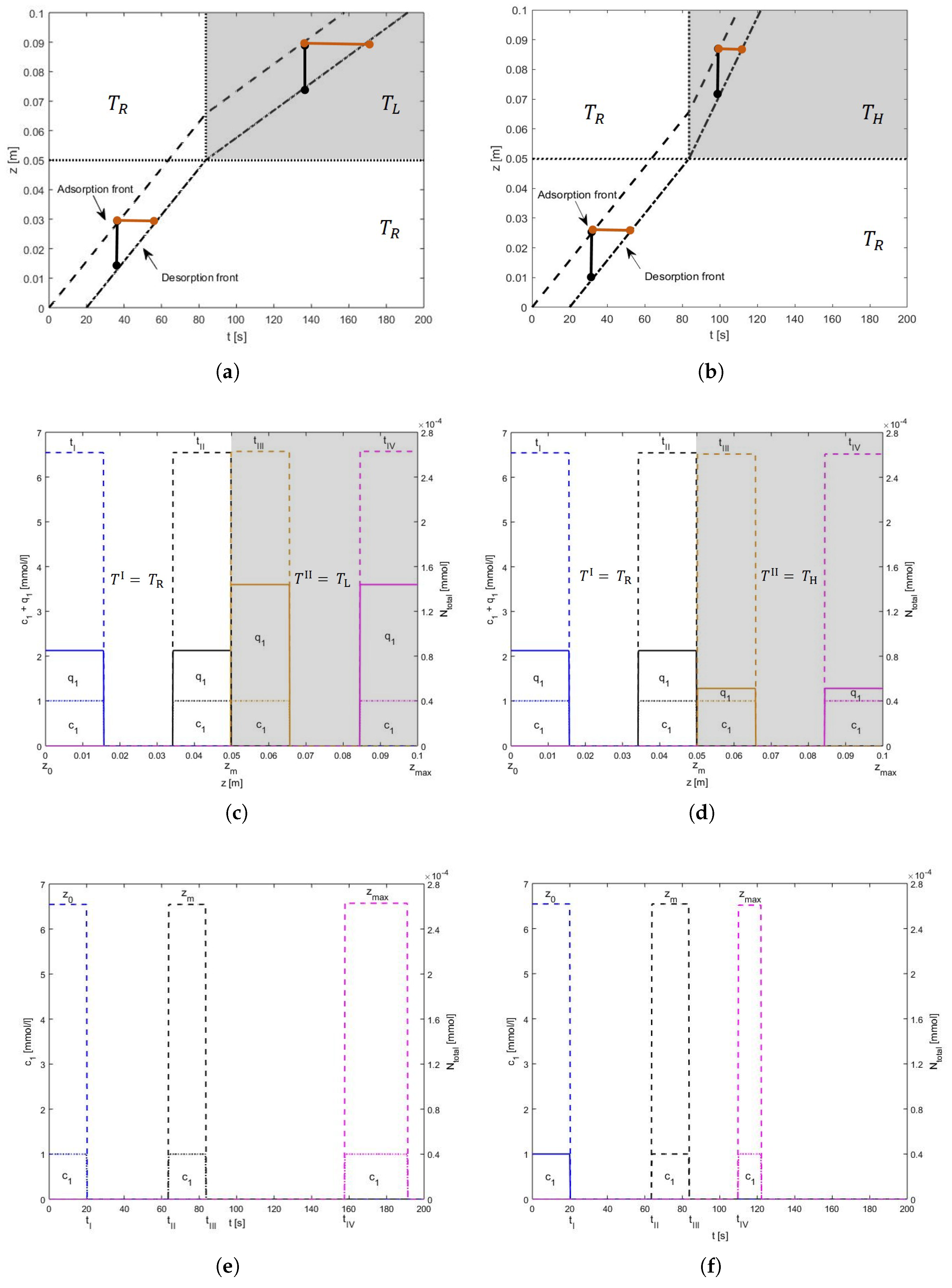
4. Illustration and Discussion of Results
4.1. Analysis of Single-Component Injection
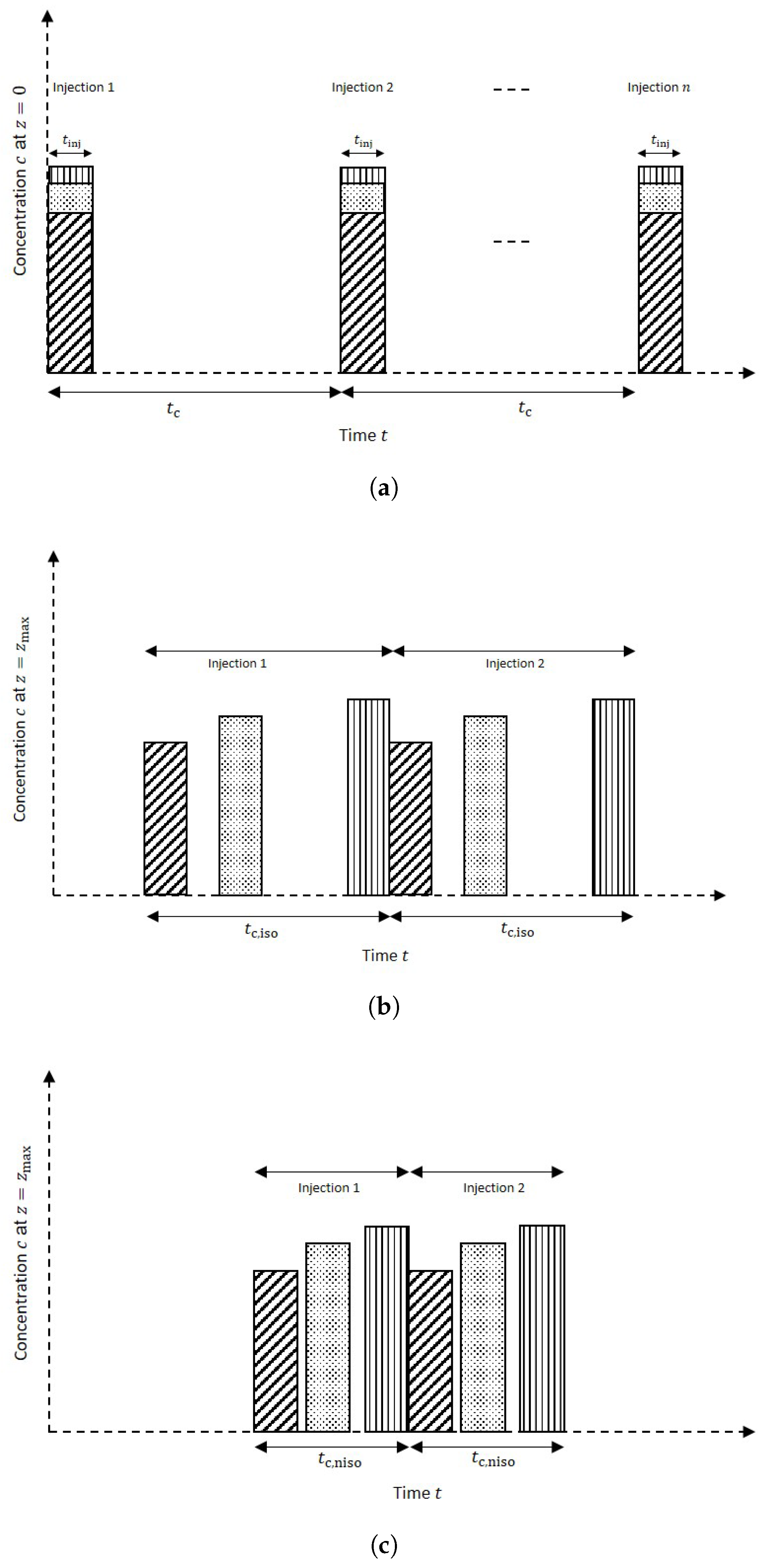
4.2. Analysis of Consecutive Injections of Multicomponent Mixtures
5. Materials and Methods
6. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Haynes, H.W., Jr. An Analysis of Sorption Heat Effects in the Pulse Gas Chromatography Diffusion Experiment. AIChE J. 1986, 32, 1750–1753. [Google Scholar] [CrossRef]
- Guillaume, Y.; Guinchard, C. Prediction of Retention Times, Column Efficiency, and Resolution in Isothermal and Temperature-programmed Gas Chromatography: Application for Separation of Four Psoalens. J. Chromatogr. Sci. 1997, 35, 14–18. [Google Scholar] [CrossRef][Green Version]
- Xiu, G.; Li, P.; Rodrigues, A.E. Sorption-enhanced Reaction Process with Reactive Regeneration. Chem. Eng. Sci. 2002, 57, 3893–3908. [Google Scholar] [CrossRef]
- Robinson, P.G.; Odell, A.L. Comparison of Isothermal and Non-linear Temperature Programmed Gas Chromatography the Temperature Dependence of the Retention Indices of a Number of Hydrocarbons on Squalane and SE-30. J. Chromatogr. A 1971, 57, 11–17. [Google Scholar] [CrossRef]
- Kaczmarski, K.; Gritti, F.; Guiochon, G. Prediction of the Influence of the Heat Generated by Viscous Friction on the Efficiency of Chromatography Columns. J. Chromatogr. A 2008, 1177, 92–104. [Google Scholar] [CrossRef] [PubMed]
- Gritti, F.; Michel Martin, M.; Guiochon, G. Influence of Viscous Friction Heating on the Efficiency of Columns Operated Under Very High Pressures. Anal. Chem. 2009, 81, 3365–3384. [Google Scholar] [CrossRef] [PubMed]
- Sainio, T.; Kaspereit, M.; Kienle, A.; Seidel-Morgenstern, A. Thermal Effects in Reactive Liquid Chromatography. Chem. Eng. Sci. 2007, 62, 5674–5681. [Google Scholar] [CrossRef]
- Sainio, T.; Zhang, L.; Seidel-Morgenstern, A. Adiabatic Operation of Chromatographic Fixed-bed Reactors. Chem. Eng. J. 2011, 168, 861–871. [Google Scholar] [CrossRef]
- Qamar, S.; Sattar, F.A.; Batool, I.; Seidel-Morgenstern, A. Theoretical Analysis of the Influence of Forced and Inherent Temperature Fluctuations in an Adiabatic Chromatographic Column. Chem. Eng. Sci. 2017, 161, 249–264. [Google Scholar] [CrossRef]
- Brandt, A.; Mann, G.; Arlt, W. Temperature Gradients in Preparative High-performance Liquid Chromatography Columns. J. Chromatogr. A 1997, 769, 109–117. [Google Scholar] [CrossRef]
- Vu, T.D.; Seidel-Morgenstern, A. Quantifying Temperature and Flow Rate Effects on the Performance of a Fixed-bed Chromatographic Reactor. J. Chromatogr. A 2011, 1218, 8097–8109. [Google Scholar] [CrossRef] [PubMed]
- Javeed, S.; Qamar, S.; Seidel-Morgenstern, A.; Warnecke, G. Parametric study of thermal effects in reactive liquid chromatography. Chem. Eng. J. 2012, 191, 426–440. [Google Scholar] [CrossRef]
- Teutenberg, T. High-Temperature Liquid Chromatography: A Users Guide for Method Development; RSC Chromatography Monographs; Royal Society of Chemistry: London, UK, 2010. [Google Scholar]
- Horváth, C.; Melander, W.; Molnar, I. Solvophobic Interactions in Liquid Chromatography with Nonpolar Stationary Phases. J. Chromatogr. A 1976, 125, 129–156. [Google Scholar] [CrossRef]
- Antia, F.D.; Horvath, C. High-performance Liquid Chromatography at Elevated Temperatures: Examination of Conditions for the Rapid Separation of Large Molecules. J. Chromatogr. A 1988, 435, 1–15. [Google Scholar] [CrossRef]
- Snyder, D.C.; Dolan, J.W. Initial Experiments in High-performance Liquid Chromatographic Method Development I. Use of a Starting Gradient Run. J. Chromatogr. A 1996, 721, 3–14. [Google Scholar] [CrossRef]
- Snyder, L.R.; Dolan, J.W.; Lommen, D.C. Drylab® Computer Simulation for High-performance Liquid Chromatographic Method Development: I. Isocratic Elution. J. Chromatogr. A 1989, 485, 45–89. [Google Scholar] [CrossRef]
- Poppe, H. Some Reflections on Speed and Efficiency of Modern Chromatographic Methods. J. Chromatogr. A 1997, 778, 3–21. [Google Scholar] [CrossRef]
- Poppe, H.; Kraak, J.C.; Huber, J.F.K.; Van den Berg, J.H.M. Temperature Gradients in HPLC Columns Due to Viscous Heat Dissipation. Chromatographia 1981, 14, 515–523. [Google Scholar] [CrossRef]
- Lee, H.; Yang, J.; Chang, T. Branching analysis of star-shaped polybutadienes by temperature gradient interaction chromatography-triple detection. Polymer 2017, 112, 71–75. [Google Scholar] [CrossRef]
- Ahn, S.; Lee, H.; Lee, S.; Chang, T. Characterization of Branched Polymers by Comprehensive Two-Dimensional Liquid Chromatography with Triple Detection. Macromolecules 2012, 45, 3550–3556. [Google Scholar] [CrossRef]
- Chen, X.; Rahman, M.S.; Lee, H.; Mays, J.; Chang, T.; Larson, R. Combined Synthesis, TGIC Characterization, and RheologicalMeasurement and Prediction of Symmetric H Polybutadienes and Their Blends with Linear and Star-Shaped Polybutadienes. Macromolecules 2011, 44, 7799–7809. [Google Scholar] [CrossRef]
- Lee, S.; Lee, H.; Chang, T.; Hirao, A. Synthesis and Characterization of an Exact Polystyrene-graftpolyisoprene: A Failure of Size Exclusion Chromatography Analysis. Macromolecules 2017, 50, 2768–2776. [Google Scholar] [CrossRef]
- Rhee, H.-K.; Aris, R.; Amundson, N.R. First-Order Partial Differential Equations, Vol. I; Prentice-Hall: Englewood Cliffs, NJ, USA, 1986. [Google Scholar]
- Rhee, H.-K.; Aris, R.; Amundson, N.R. First-Order Partial Differential Equations, Vol. II; Prentice-Hall: Englewood Cliffs, NJ, USA, 1989. [Google Scholar]
- Mazzotti, M.; Rajendran, A. Equilibrium Theory-Based Analysis of Non-linear Waves in Separation Processess. Annu. Rev. Chem. Biomol. Eng. 2013, 4, 119–141. [Google Scholar] [CrossRef] [PubMed]
- LeVeque, R.J. Numerical Methods for Conservation Laws, 2nd ed.; Birkhäuser Verlag: Basel, Switzerland; Boston, MA, USA; Berlin, Germany, 1992. [Google Scholar]

| Symbol | Quantity | Value Used in Simulation |
|---|---|---|
| Length of the column | 0.1 m | |
| A | Cross-sectional area of the column | 0.0000196 (diameter d = 0.5 cm) |
| Porosity of the column | 0.4 | |
| u | Interstitial velocity | 0.00167 m/s |
| Injection time | 20 s | |
| Feed concentrations | 1 mmol/L | |
| Henry constant at reference temperature, | 0.75 | |
| Henry constant at lower temperature, | 1.73 | |
| Henry constant at higher temperature, | 0.18 |
| Symbol | Quantity | Value Used in Simulation |
|---|---|---|
| Length of the column | 0.1 m | |
| A | Cross-sectional area of the column | 0.0000196 (diameter d = 0.5 cm) |
| Porosity of the column | 0.4 | |
| u | Interstitial velocity | 0.00167 m/s |
| Injection time | 10 s | |
| Feed concentrations | [1, 1, 1] mmol/L | |
| Henry constant for comp. 1 at | 0.25 | |
| Henry constant for comp. 1 at | 0.9 | |
| Henry constant for comp. 1 at | 0.06 | |
| Henry constant for comp. 2 at | 1.0 | |
| Henry constant for comp. 2 at | 3.6 | |
| Henry constant for comp. 2 at | 0.25 | |
| Henry constant for comp. 3 at | 3.0 | |
| Henry constant for comp. 3 at | 10.8 | |
| Henry constant for comp. 3 at | 0.74 |
| Symbol | Quantity | Value Obtained |
|---|---|---|
| Cycle time in isothermal case | 257 s | |
| Cycle time in non-isothermal case | 197 s | |
| Hypothetical cycle time in non-isothermal case | 129 s | |
| Time taken by the adsorption front component 1 to reach | 41 s | |
| Time taken by the desorption front component 1 to reach (non-isothermal case) | 120 s | |
| P | Production rate of each component under isothermal conditions | mmol/s |
| P | Production rate of each component under non-isothermal conditions | mmol/s |
| Increase in the overall production rate | 30% (approx.) |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hayat, A.; An, X.; Qamar, S.; Warnecke, G.; Seidel-Morgenstern, A. Theoretical Analysis of Forced Segmented Temperature Gradients in Liquid Chromatography. Processes 2019, 7, 846. https://doi.org/10.3390/pr7110846
Hayat A, An X, Qamar S, Warnecke G, Seidel-Morgenstern A. Theoretical Analysis of Forced Segmented Temperature Gradients in Liquid Chromatography. Processes. 2019; 7(11):846. https://doi.org/10.3390/pr7110846
Chicago/Turabian StyleHayat, Adnan, Xinghai An, Shamsul Qamar, Gerald Warnecke, and Andreas Seidel-Morgenstern. 2019. "Theoretical Analysis of Forced Segmented Temperature Gradients in Liquid Chromatography" Processes 7, no. 11: 846. https://doi.org/10.3390/pr7110846
APA StyleHayat, A., An, X., Qamar, S., Warnecke, G., & Seidel-Morgenstern, A. (2019). Theoretical Analysis of Forced Segmented Temperature Gradients in Liquid Chromatography. Processes, 7(11), 846. https://doi.org/10.3390/pr7110846





