Titanium Distribution Ratio Model of Ladle Furnace Slags for Tire Cord Steel Production Based on the Ion–Molecule Coexistence Theory at 1853 K
Abstract
1. Introduction
2. Materials and Methods
2.1. Production Procedure and Materials
2.2. Establishment of the IMCT Model
- (1)
- The constitutional units in the slag consist of simple ions, ordinary molecules, and complicated molecules;
- (2)
- Complex molecules are generated by the reactions of bonded ion couples and simple molecules under kinetic equilibrium;
- (3)
- The activity of each constituent in the slag equals the MAC of the structural unit at the steelmaking temperature;
- (4)
- The chemical reactions comply with the law of mass conservation.
3. Results and Discussion
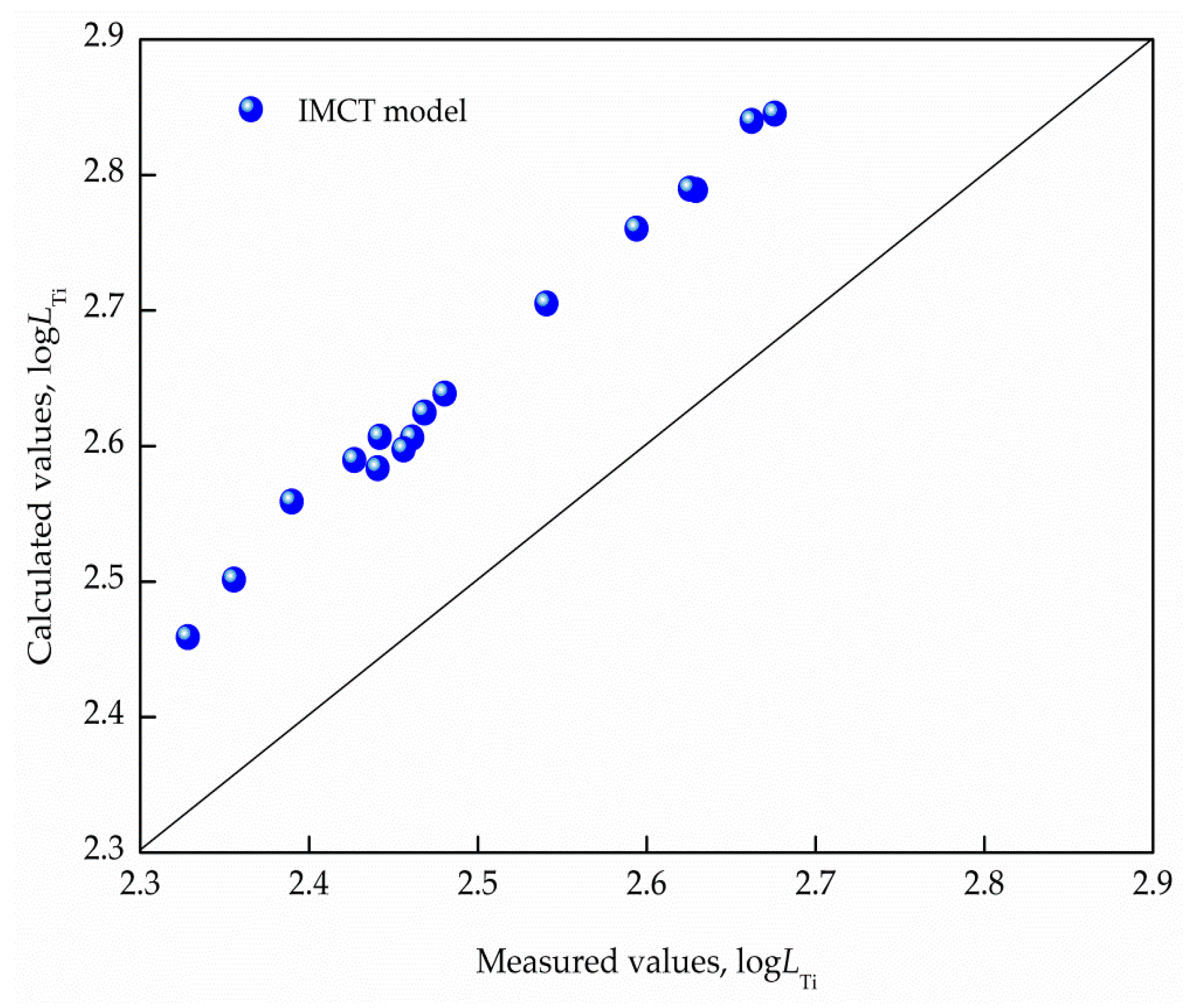
3.1. Comparison of Predicted and Measured Titanium Distribution Ratios
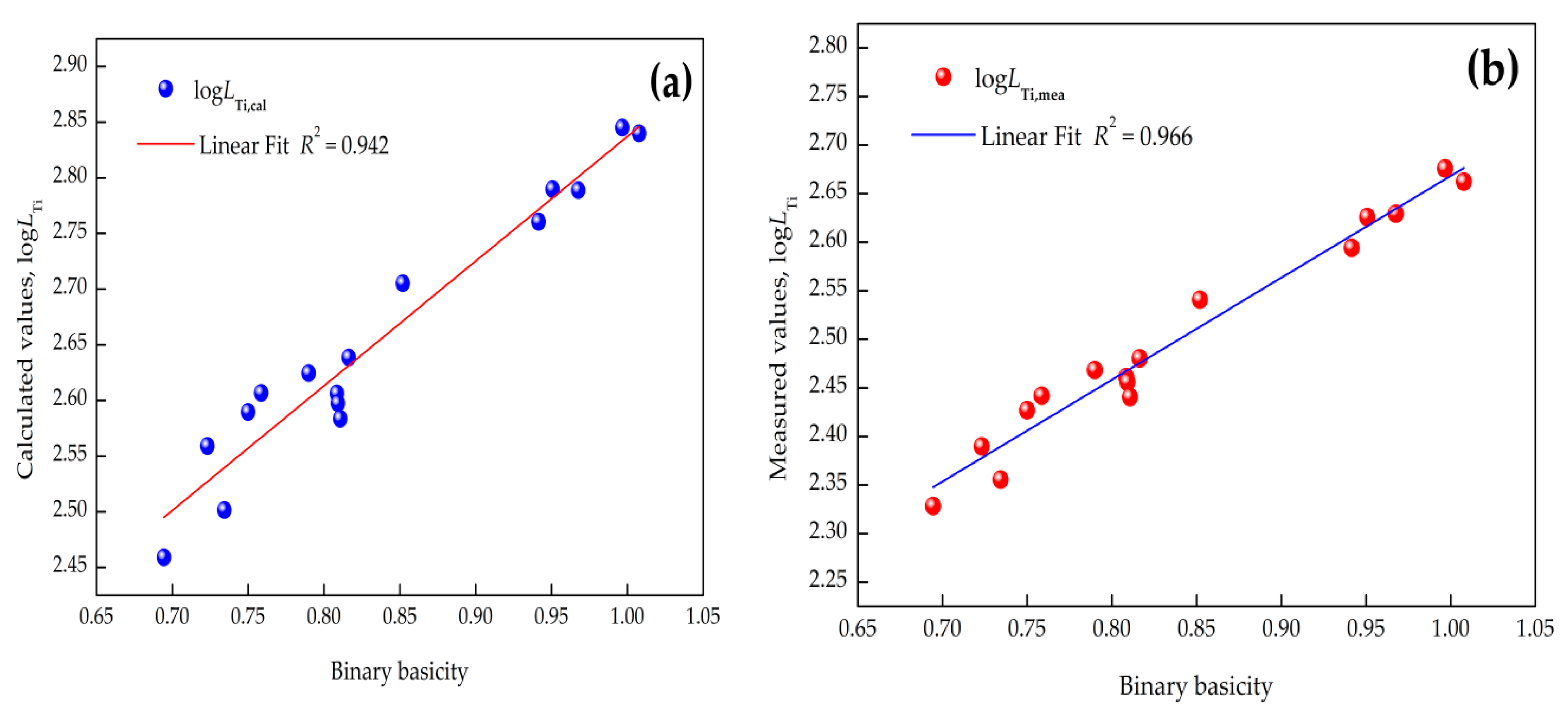
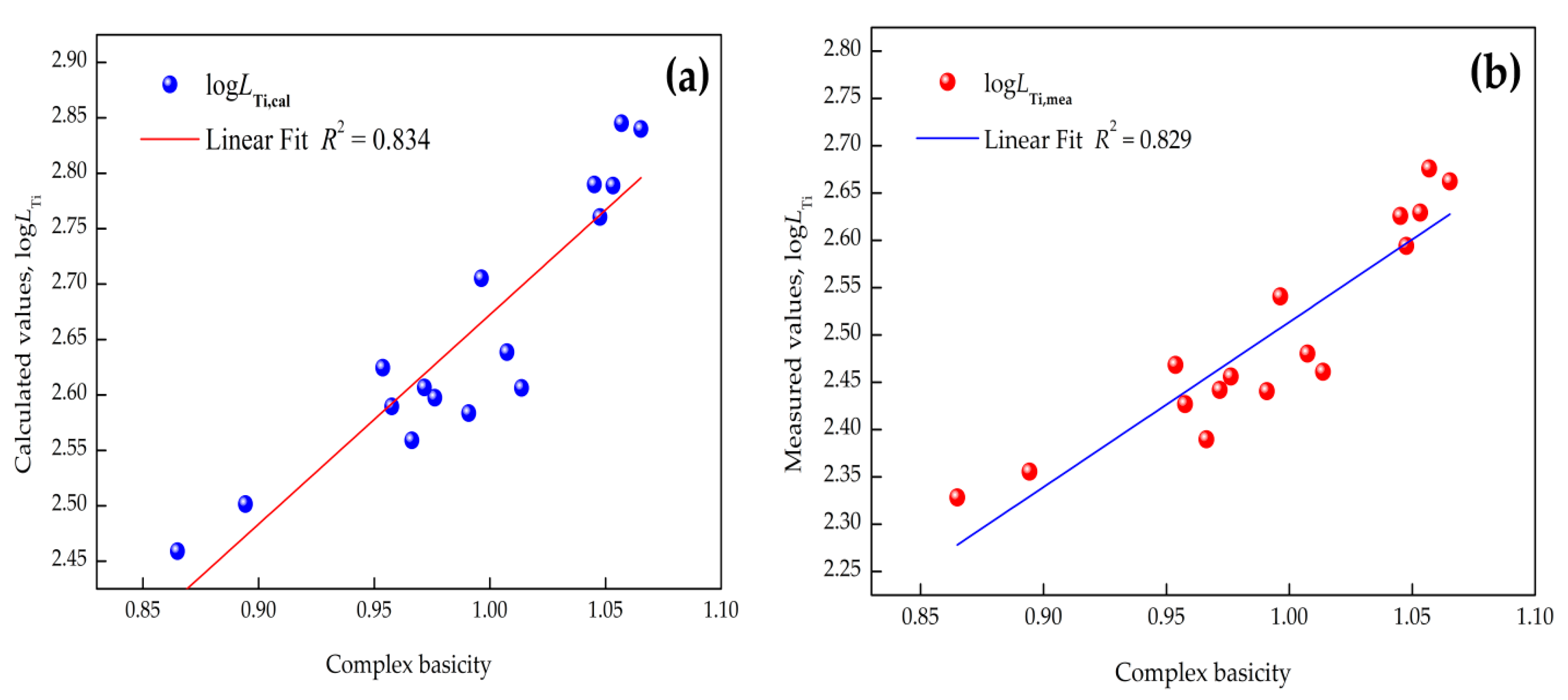
3.2. Influence of Basicity on the Titanium Distribution Ratio
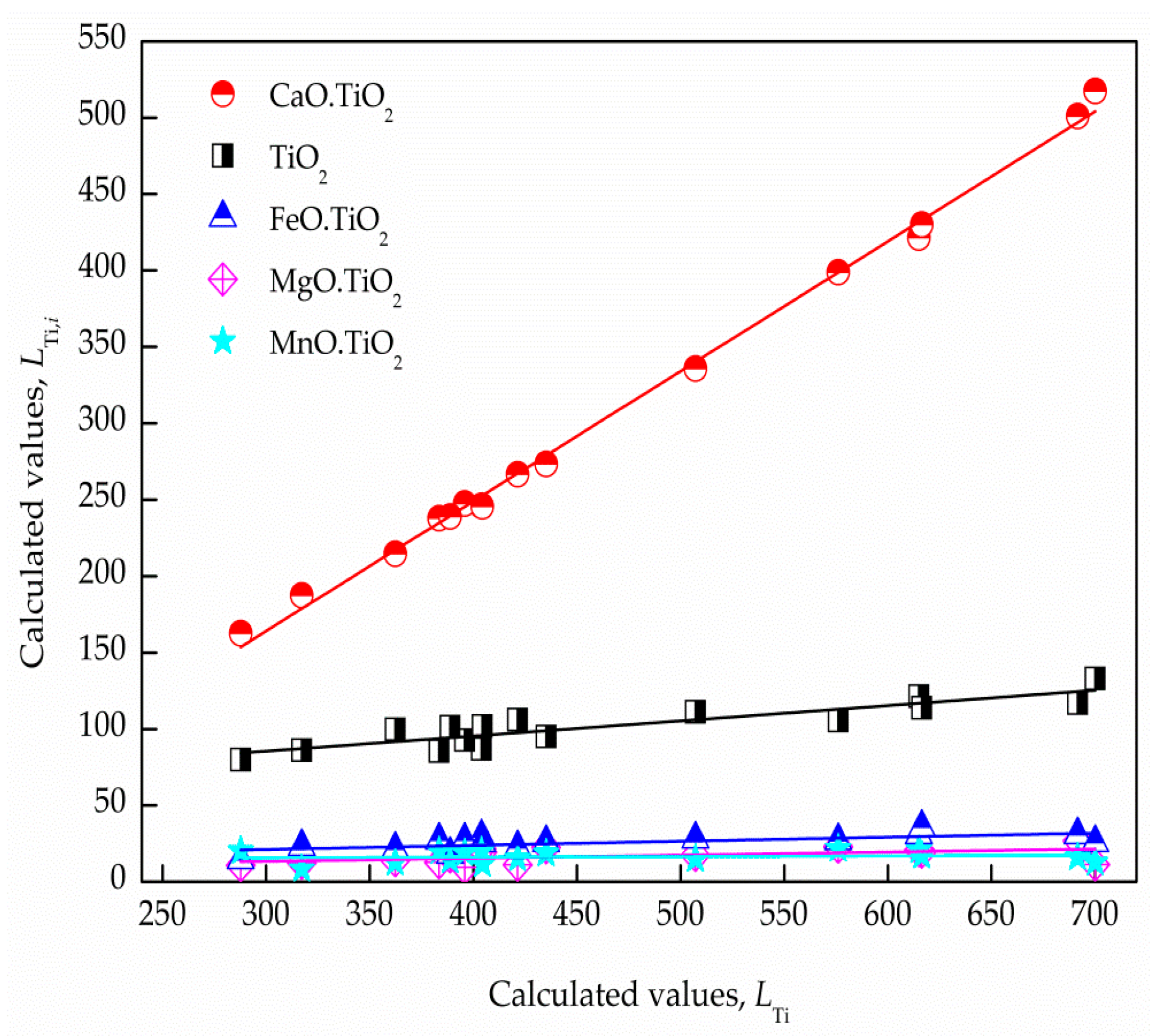
3.3. Contribution Ratio of the Respective Titanium Distribution Ratio Based on the IMCT
4. Conclusions
- (1)
- The established IMCT model for calculating the titanium distribution ratio exhibited a dependable agreement with the measurements, and the model can be responsibly applied to predict the maximum de-titanium potential in the LF process at metallurgical temperatures.
- (2)
- The titanium distribution ratio will increase with the rise of basicity, and the optical basicity is suggested to describe the correlation between basicity and de-titanium ability of the slag. Higher optical basicity is in favor of the de-titanium process.
- (3)
- The respective titanium distribution ratios of structural units containing TiO2 can be acquired by the built IMCT model. The contribution rates of , , , , and to total de-titanium potential were approximately 9.97%, 84.96%, 2.03%, 2.65%, and 0.39%, respectively, revealing that the structural unit CaO plays a pivotal role in the slags in the de-titanium process.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Abushosha, R.; Vipond, R.; Mintz, B. Influence of titanium on hot ductility of as cast steels. Mater. Sci. Technol. 1991, 7, 613–621. [Google Scholar] [CrossRef]
- Chen, Z.; Li, M.; Wang, X.; He, S.; Wang, Q. Mechanism of floater formation in the mold during continuous casting of Ti-stabilized austenitic stainless steels. Metals 2019, 9, 635. [Google Scholar] [CrossRef]
- Karmakar, A.; Kundu, S.; Roy, S.; Neogy, S.; Srivastava, D.; Chakrabarti, D. Effect of microalloying elements on austenite grain growth in Nb–Ti and Nb–V steels. Mater. Sci. Technol. A 2014, 30, 653–664. [Google Scholar] [CrossRef]
- Reyes-Calderón, F.; Mejía, I.; Boulaajaj, A.; Cabrera, J.M. Effect of microalloying elements (Nb, V and Ti) on the hot flow behavior of high-Mn austenitic twinning induced plasticity (TWIP) steel. Mater. Sci. Eng. A 2013, 560, 552–560. [Google Scholar] [CrossRef]
- Cui, H.Z.; Chen, W.Q. Effect of boron on morphology of inclusions in tire cord steel. J. Iron Steel Res. Int. 2012, 19, 22–27. [Google Scholar] [CrossRef]
- Wu, S.; Liu, Z.; Zhou, X.; Yang, H.; Wang, G. Precipitation behavior of Ti in high strength steels. J. Cent. South Univ. 2017, 24, 2767–2772. [Google Scholar] [CrossRef]
- Li, J.Y.; Zhang, W.Y. Effect of TiN inclusion on fracture toughness in ultrahigh strength steel. ISIJ Int. 1989, 29, 158–164. [Google Scholar] [CrossRef]
- Petit, J.; Sarrazin-Baudoux, C.; Lorenzi, F. Fatigue crack propagation in thin wires of ultra high strength steels. Procedia Eng. 2010, 2, 2317–2326. [Google Scholar] [CrossRef]
- Liu, H.Y.; Wang, H.L.; Li, L.; Zheng, J.Q.; Li, Y.H.; Zeng, X.Y. Investigation of Ti inclusions in wire cord steel. Ironmak. Steelmak. 2011, 38, 53–58. [Google Scholar] [CrossRef]
- Duan, S.C.; Guo, X.L.; Guo, H.J.; Guo, J. A manganese distribution prediction model for CaO–SiO2–FeO–MgO–MnO–Al2O3 slags based on IMCT. Ironmak. Steelmak. 2017, 44, 168–184. [Google Scholar] [CrossRef]
- Duan, S.C.; Li, C.; Guo, X.L.; Guo, H.J.; Guo, J.; Yang, W.S. A thermodynamic model for calculating manganese distribution ratio between CaO–SiO2–MgO–FeO–MnO–Al2O3–TiO2–CaF2 ironmaking slags and carbon saturated hot metal based on the IMCT. Ironmak. Steelmak. 2017, 45, 655–664. [Google Scholar] [CrossRef]
- Li, B.; Li, L.; Guo, H.; Guo, J.; Duan, S.; Sun, W. A phosphorus distribution prediction model for CaO–SiO2–MgO–FeO–Fe2O3–Al2O3–P2O5 slags based on the IMCT. Ironmak. Steelmak. 2019. [Google Scholar] [CrossRef]
- Yang, X.M.; Li, J.Y.; Chai, G.M.; Duan, D.P.; Zhang, J. A Thermodynamic model for predicting phosphorus partition between CaO–based slags and hot metal during hot metal dephosphorization pretreatment process based on the ion and molecule coexistence theory. Metall. Mater. Trans. B 2016, 47, 2279–2301. [Google Scholar] [CrossRef]
- Yang, X.M.; Duan, J.P.; Shi, C.B.; Zhang, M.; Zhang, Y.L.; Wang, J.C. A thermodynamic model of phosphorus distribution ratio between CaO–SiO2–MgO–FeO–Fe2O3–MnO–Al2O3–P2O5 slags and molten steel during a top–bottom combined blown converter steelmaking process based on the ion and molecule coexistence theory. Metall. Mater. Trans. B 2011, 42, 738–770. [Google Scholar] [CrossRef]
- Yang, X.M.; Zhang, M.; Chai, G.M.; Li, J.Y.; Liang, Q.; Zhang, J. Thermodynamic models for predicting dephosphorisation ability and potential of CaO–FeO–Fe2O3–Al2O3–P2O5 slags during secondary refining process of molten steel based on ion and molecule coexistence theory. Ironmak. Steelmak. 2016, 43, 663–687. [Google Scholar] [CrossRef]
- Li, J.Y.; Zhang, M.; Guo, M.; Yang, X.M. Enrichment mechanism of phosphate in CaO–SiO2–FeO–Fe2O3–P2O5 steelmaking slags. Metall. Mater. Trans. B 2014, 45, 1666–1682. [Google Scholar] [CrossRef]
- Yang, X.M.; Li, J.Y.; Zhang, M.; Yan, F.J.; Duan, D.P.; Zhang, J. A further evaluation of the coupling relationship between dephosphorization and desulfurization abilities or potentials for CaO–based slags: Influence of slag chemical composition. Metals 2018, 8, 1083. [Google Scholar] [CrossRef]
- Shi, C.B.; Yang, X.M.; Jiao, J.S.; Li, C.; Guo, H.J. A sulphide capacity prediction model of CaO–SiO2–MgO–Al2O3 ironmaking slags based on the ion and molecule coexistence theory. ISIJ Int. 2010, 50, 1362–1372. [Google Scholar] [CrossRef]
- Yang, X.M.; Shi, C.B.; Zhang, M.; Chai, G.M.; Wang, F. A thermodynamic model of sulfur distribution ratio between CaO–SiO2–MgO–FeO–MnO–Al2O3 slags and molten steel during LF refining process based on the ion and molecule coexistence theory. Metall. Mater. Trans. B 2011, 42, 1150–1180. [Google Scholar] [CrossRef]
- Yang, X.M.; Zhang, M.; Shi, C.B.; Chai, G.M.; Zhang, J. A sulfide capacity prediction model of CaO–SiO2–MgO–FeO–MnO–Al2O3 slags during the LF refining process based on the ion and molecule coexistence theory. Metall. Mater. Trans. B 2012, 43, 241–266. [Google Scholar] [CrossRef]
- Yang, X.M.; Li, J.Y.; Zhang, M.; Chai, G.M.; Zhang, J. Prediction model of sulfide capacity for CaO–FeO–Fe2O3–Al2O3–P2O5 slags in a large variation range of oxygen potential based on the ion and molecule coexistence theory. Metall. Mater. Trans. B 2014, 45, 2118–2137. [Google Scholar] [CrossRef]
- Yang, X.M.; Li, J.Y.; Zhang, M.; Zhang, J. Prediction model of sulphur distribution ratio between CaO–FeO–Fe2O3–Al2O3–P2O5 slags and liquid iron over large variation range of oxygen potential during secondary refining process of molten steel based on ion and molecule coexistence theory. Ironmak. Steelmak. 2016, 43, 39–55. [Google Scholar] [CrossRef]
- Yang, X.M.; Zhang, M.; Zhang, J.L.; Li, P.C.; Li, J.Y.; Zhang, J. Representation of oxidation ability for metallurgical slags based on the ion and molecule coexistence theory. Steel Res. Int. 2014, 85, 347–375. [Google Scholar] [CrossRef]
- Verein Deutscher Eisenhuttenleute (VDEh). Slag Atlas, 2nd ed.; Woodhead Publishing Limited: Cambridge, UK, 1995. [Google Scholar]
- Chen, J.X. Common Charts and Databook for Steelmaking, 2nd ed.; Metallurgical Industry Press: Beijing, China, 2010. [Google Scholar]
- Zhang, J. Computational Thermodynamics of Metallurgical Melts and Solutions; Metallurgical Industry Press: Beijing, China, 2007. [Google Scholar]
- Rein, R.H.; Chipman, J. Activities in the liquid solution SiO2–CaO–MgO–Al2O3 at 1600 °C. Trans. Met. Soc. AIME 1965, 233, 415–425. [Google Scholar]
- Turkdogan, E.T. Physical Chemistry of High Temperature Technology; Academic Press: New York, NY, USA, 1980; pp. 8–12. [Google Scholar]
- Gaye, H.; Welfringer, J. Proceedings of the Second International Symposium on Metallurgical Slags and Fluxes; Fine, H.A., Gaskell, D.R., Eds.; TMS–AIME: Lake Tahoe, NV, USA, 1984; pp. 357–375. [Google Scholar]
- Ban-ya, S.; Chiba, A.; Hikosaka, A. Thermodynamics of FetO–MxOy(MxOy=CaO, SiO2, TiO2, and Al2O3) binary melts in equilibrium with solid iron. Tetsu-To-Hagane 1980, 66, 1484–1493. [Google Scholar] [CrossRef]
- Timucin, M.; Muan, A. Activity–composition relations in NiAl2O4–MnAl2O4 solid solutions and stabilities of NiAl2O4 and MnAl2O4 at 1300 °C and 1400 °C. J. Am. Ceram. Soc. 1992, 75, 1399–1406. [Google Scholar] [CrossRef]
- Barin, I.; Knacke, O.; Kubaschewski, O. Thermochemical Properties of Inorganic Substances (Supplement); Springer: New York, NY, USA, 1977; pp. 392–445. [Google Scholar]
- Pauling, L. The Nature of Chemical Bond; Cornell University Press: Ithaca, NY, USA, 1960. [Google Scholar]

| Slag Systems | Applications | Ref. |
|---|---|---|
| CaO–SiO2–FeO–MgO–MnO–Al2O3 | A thermodynamic model for predicting the manganese distribution ratio and manganese capacity of the slags was developed based on the IMCT. The established model was successfully applied to not only manganese equilibrium experiments but also industrial production. | [10] |
| CaO–SiO2–MgO–FeO–MnO–Al2O3–TiO2–CaF2 | A thermodynamic model for calculating the manganese distribution ratio between the slags and carbon saturated liquid iron was built based on the IMCT. The predicted manganese distribution ratio by IMCT had a good linear relationship with measurements expect individual points. | [11] |
| CaO–SiO2–MgO–FeO–Fe2O3–Al2O3–P2O5 | A thermodynamic model for predicting the phosphorus distribution ratio of the slags was developed based on the IMCT. The developed model was successfully applied to not only phosphorus equilibrium experiments, but also industrial production in Hismelt smelting reduction vessels. | [12] |
| CaO-based Slags | A thermodynamic model for predicting phosphorus partition between CaO-based slags during hot metal dephosphorization pretreatment was established based on the IMCT. The established model was verified as effective through comparing with measured results and predicted ones by other models. | [13] |
| CaO–SiO2–MgO–FeO–Fe2O3–MnO–Al2O3–P2O5 | A thermodynamic model for calculating the phosphorus distribution ratio between steelmaking slags and molten steel was built based on the IMCT. The built IMCT prediction model was verified with measured and some other reported models. | [14] |
| CaO–FeO–Fe2O3–Al2O3–P2O5 | Thermodynamic models for predicting the phosphorus distribution ratio and phosphorus capacity of the slags during refining were developed based on the IMCT. The developed models were verified with experimental results and reported models. | [15] |
| CaO–SiO2–FeO–Fe2O3–P2O5 | Defined enrichment possibility and enrichment degree of solid solutions containing P2O5 from the developed IMCT model were verified from experimental results. | [16] |
| CaO-based Slags | Coupling relationships between dephosphorization and desulfurization abilities or potentials for CaO-based slags during the refining process of molten steel were proposed based on the IMCT. The proposed model was verified as effective and feasible through investigating the effect of slag composition. | [17] |
| CaO–SiO2–MgO–Al2O3 | A sulfide capacity prediction model of the slags was developed based on the IMCT. The developed model had a higher accuracy than other reported sulfide capacity prediction models. | [18] |
| CaO–SiO2–MgO–FeO–MnO–Al2O3 | A thermodynamic model for calculating the sulfur distribution ratio between ladle furnace (LF) refining slags and molten steel was established based on the IMCT. The model was verified with the measured and the calculated sulfur distribution ratio by Young’s model and the KTH model in LF refining. | [19] |
| CaO–SiO2–MgO–FeO–MnO–Al2O3 | A sulfide capacity prediction model of the LF refining slags was built based on the IMCT. The built sulfide capacity prediction model was verified with the measured and calculated by Young’s model and the KTH model in LF refining. | [20] |
| CaO–FeO–Fe2O3–Al2O3–P2O5 | A thermodynamic model for predicting the sulfide capacity of the slags at various oxygen potentials was developed based on the IMCT. The built model was verified through comparing the determined sulfide capacity, and could be applied to precisely predict sulfide capacity. | [21] |
| CaO–FeO–Fe2O3–Al2O3–P2O5 | A thermodynamic model for predicting the sulfur distribution ratio between the slags and liquid iron was built based on the IMCT. The developed model was verified with measured data of sulfur distribution equilibrium from the literatures. | [22] |
| CaO–SiO2–MgO–FeO–Fe2O3–MnO–Al2O3–P2O5 | The defined oxidation ability of metallurgical slags based on the IMCT was verified by comparisons with the reported activity in the selected FetO-containing slag systems. | [23] |
| Slag Composition | Metal Composition | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| CaO | SiO2 | Al2O3 | MgO | FeO | MnO | TiO2 | C | Si | Mn | Ti |
| 39.19 | 40.50 | 7.97 | 8.47 | 1.36 | 2.17 | 0.34 | 0.81 | 0.20 | 0.47 | 0.0008 |
| 38.56 | 40.95 | 7.73 | 8.89 | 1.27 | 2.28 | 0.31 | 0.80 | 0.19 | 0.48 | 0.0008 |
| 35.19 | 43.10 | 6.82 | 10.79 | 1.41 | 2.52 | 0.18 | 0.83 | 0.18 | 0.46 | 0.0006 |
| 34.70 | 42.92 | 6.46 | 10.97 | 1.81 | 2.95 | 0.19 | 0.80 | 0.20 | 0.46 | 0.0007 |
| 39.47 | 41.52 | 7.19 | 8.17 | 1.61 | 1.78 | 0.25 | 0.80 | 0.20 | 0.45 | 0.0006 |
| 37.08 | 45.82 | 3.78 | 8.10 | 1.61 | 3.40 | 0.20 | 0.82 | 0.20 | 0.46 | 0.0007 |
| 34.28 | 45.19 | 6.07 | 11.09 | 1.36 | 1.69 | 0.33 | 0.82 | 0.19 | 0.47 | 0.0012 |
| 36.11 | 44.54 | 4.79 | 9.12 | 1.73 | 3.49 | 0.22 | 0.81 | 0.18 | 0.46 | 0.0008 |
| 40.04 | 39.73 | 8.87 | 8.38 | 1.28 | 1.38 | 0.32 | 0.82 | 0.18 | 0.48 | 0.0007 |
| 33.25 | 45.28 | 7.74 | 10.12 | 1.62 | 1.71 | 0.27 | 0.80 | 0.19 | 0.47 | 0.0012 |
| 34.52 | 47.74 | 3.52 | 10.72 | 1.11 | 2.14 | 0.25 | 0.81 | 0.19 | 0.46 | 0.0010 |
| 37.23 | 43.69 | 6.48 | 9.12 | 1.34 | 1.86 | 0.28 | 0.82 | 0.20 | 0.47 | 0.0008 |
| 42.90 | 43.04 | 5.46 | 5.98 | 1.00 | 1.29 | 0.33 | 0.80 | 0.18 | 0.45 | 0.0007 |
| 36.52 | 46.23 | 4.75 | 8.65 | 1.14 | 2.44 | 0.26 | 0.83 | 0.20 | 0.46 | 0.0009 |
| 34.51 | 46.01 | 5.54 | 10.61 | 0.94 | 2.14 | 0.24 | 0.80 | 0.19 | 0.46 | 0.0009 |
| 31.73 | 45.69 | 6.82 | 9.78 | 1.08 | 4.70 | 0.21 | 0.81 | 0.20 | 0.48 | 0.0010 |
| Items | Constitutional Units | Balanced Mole Number | MACs |
|---|---|---|---|
| Simple cations and anions | |||
| Simple molecules | |||
| Complex molecules | |||
| Reaction Formulas | MACs | |
|---|---|---|
| 2 | ||
| Constitutional Units | Expression of | Average Contribution Rate/% |
|---|---|---|
| 9.97 | ||
| 84.96 | ||
| 2.03 | ||
| 2.65 | ||
| 0.39 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lei, J.; Zhao, D.; Feng, W.; Xue, Z. Titanium Distribution Ratio Model of Ladle Furnace Slags for Tire Cord Steel Production Based on the Ion–Molecule Coexistence Theory at 1853 K. Processes 2019, 7, 788. https://doi.org/10.3390/pr7110788
Lei J, Zhao D, Feng W, Xue Z. Titanium Distribution Ratio Model of Ladle Furnace Slags for Tire Cord Steel Production Based on the Ion–Molecule Coexistence Theory at 1853 K. Processes. 2019; 7(11):788. https://doi.org/10.3390/pr7110788
Chicago/Turabian StyleLei, Jialiu, Dongnan Zhao, Wei Feng, and Zhengliang Xue. 2019. "Titanium Distribution Ratio Model of Ladle Furnace Slags for Tire Cord Steel Production Based on the Ion–Molecule Coexistence Theory at 1853 K" Processes 7, no. 11: 788. https://doi.org/10.3390/pr7110788
APA StyleLei, J., Zhao, D., Feng, W., & Xue, Z. (2019). Titanium Distribution Ratio Model of Ladle Furnace Slags for Tire Cord Steel Production Based on the Ion–Molecule Coexistence Theory at 1853 K. Processes, 7(11), 788. https://doi.org/10.3390/pr7110788





