Applications of Water-Soluble Polymers in Turbulent Drag Reduction
Abstract
:1. Introduction
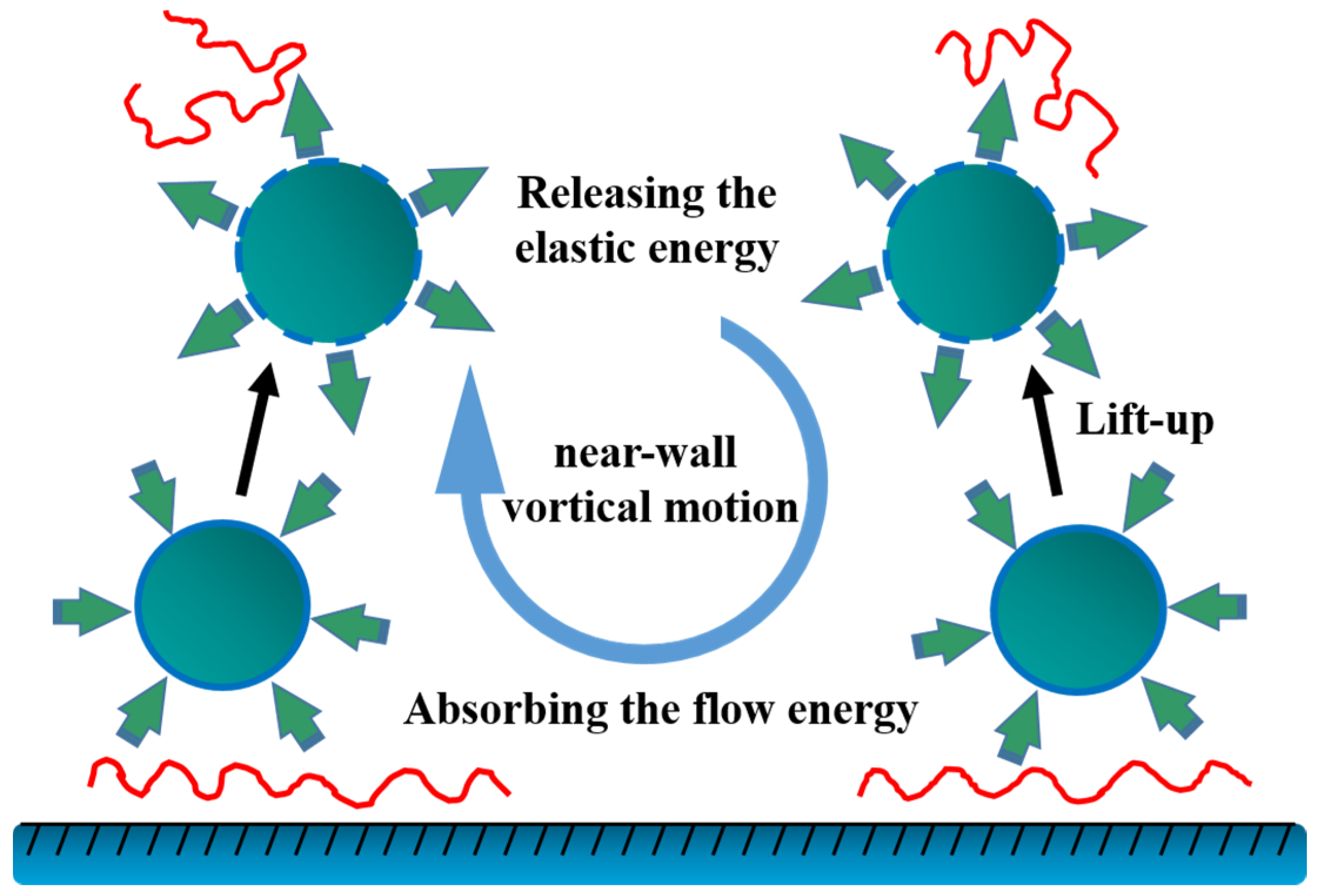
2. Mechanism of Turbulent Drag Reduction with Polymers
3. Synthetic Water-Soluble Polymers
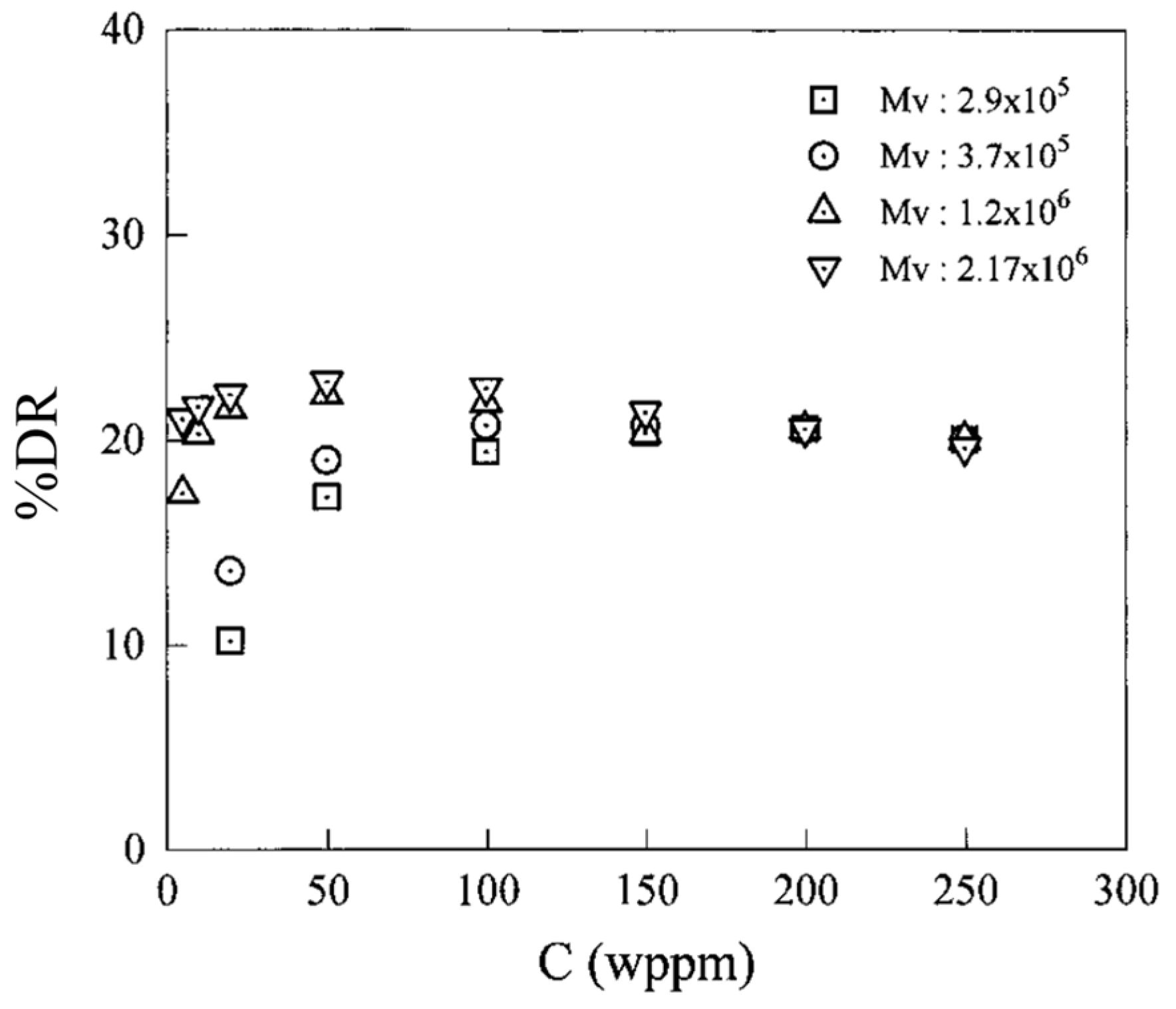
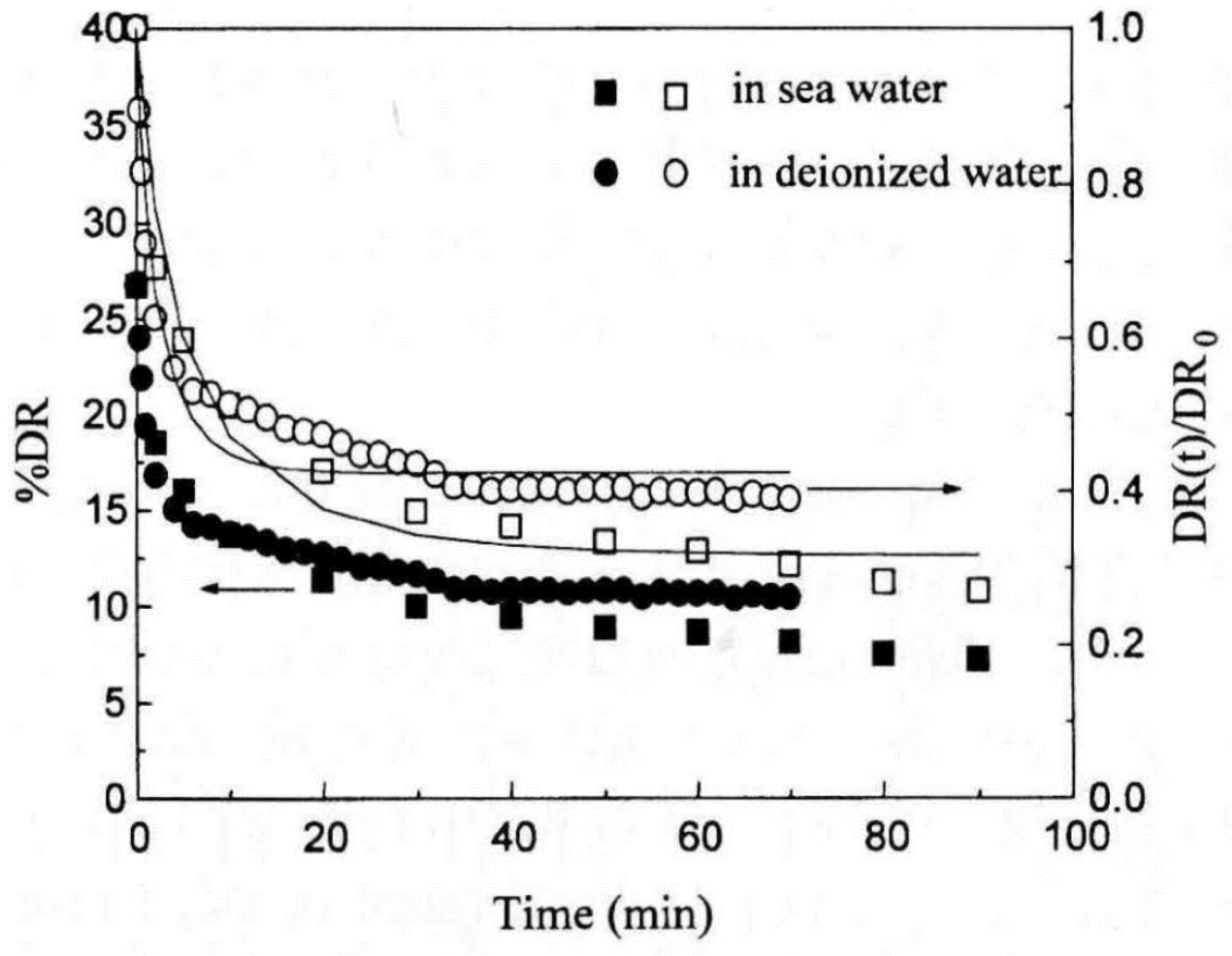
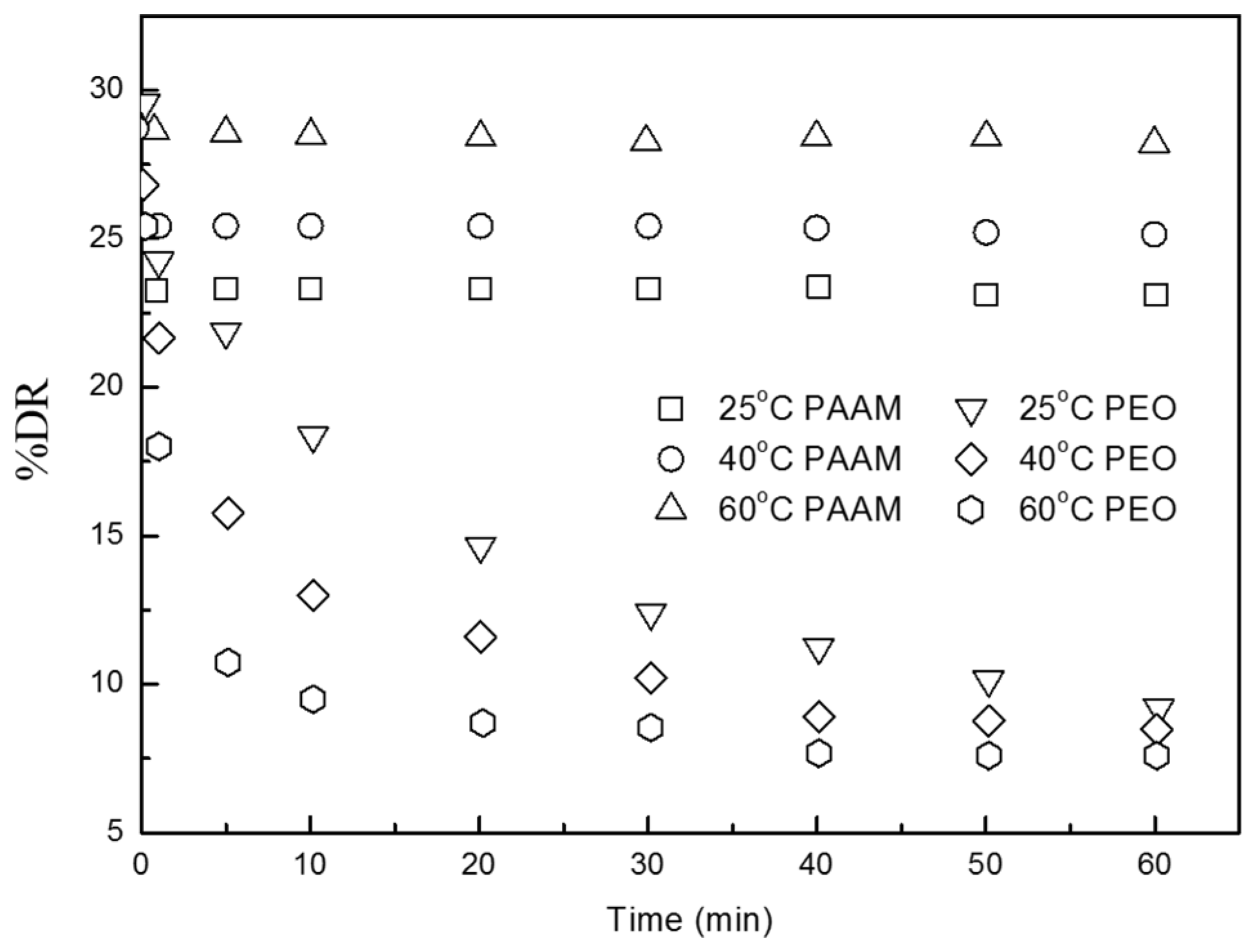
3.1. Poly(ethylene oxide)
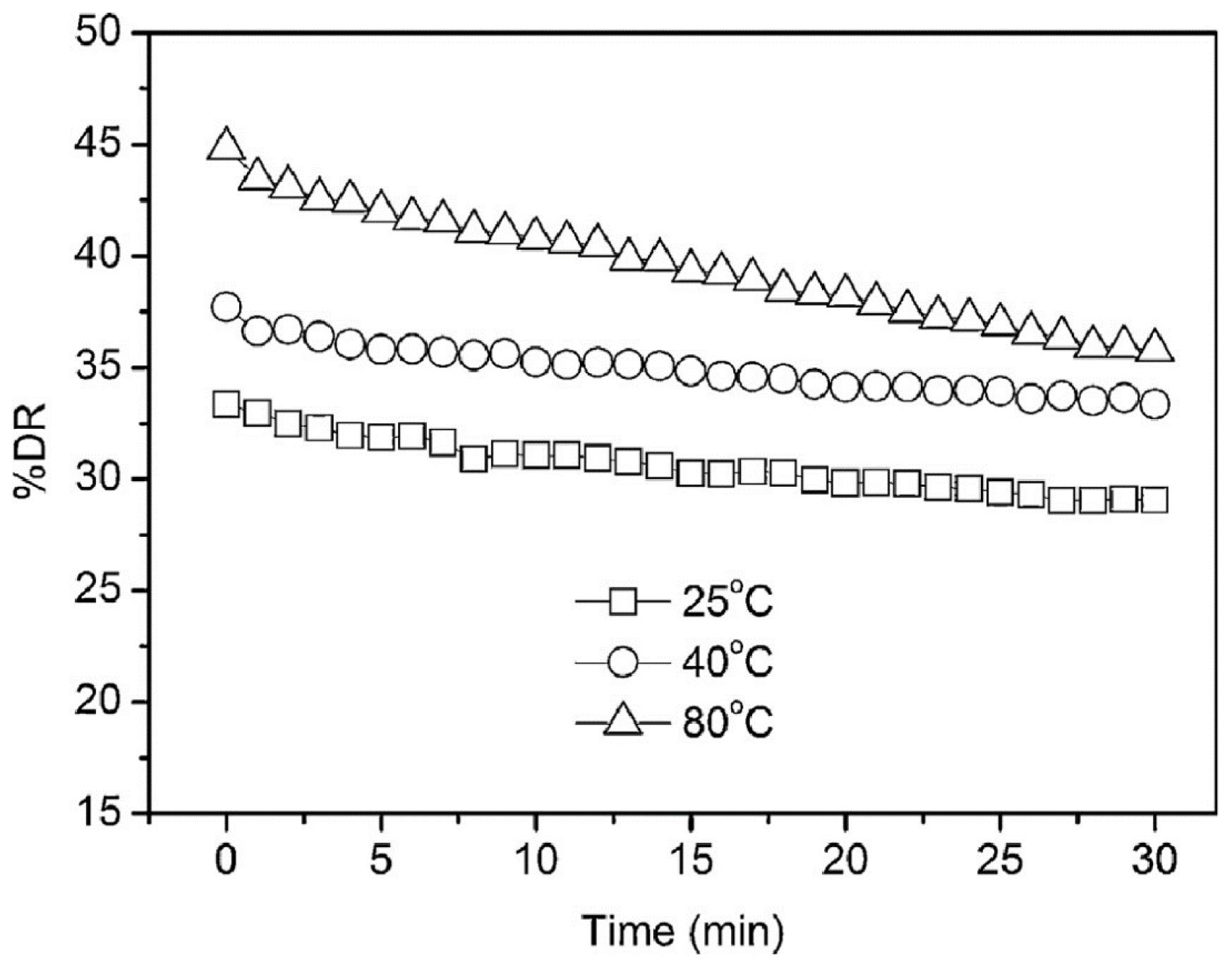
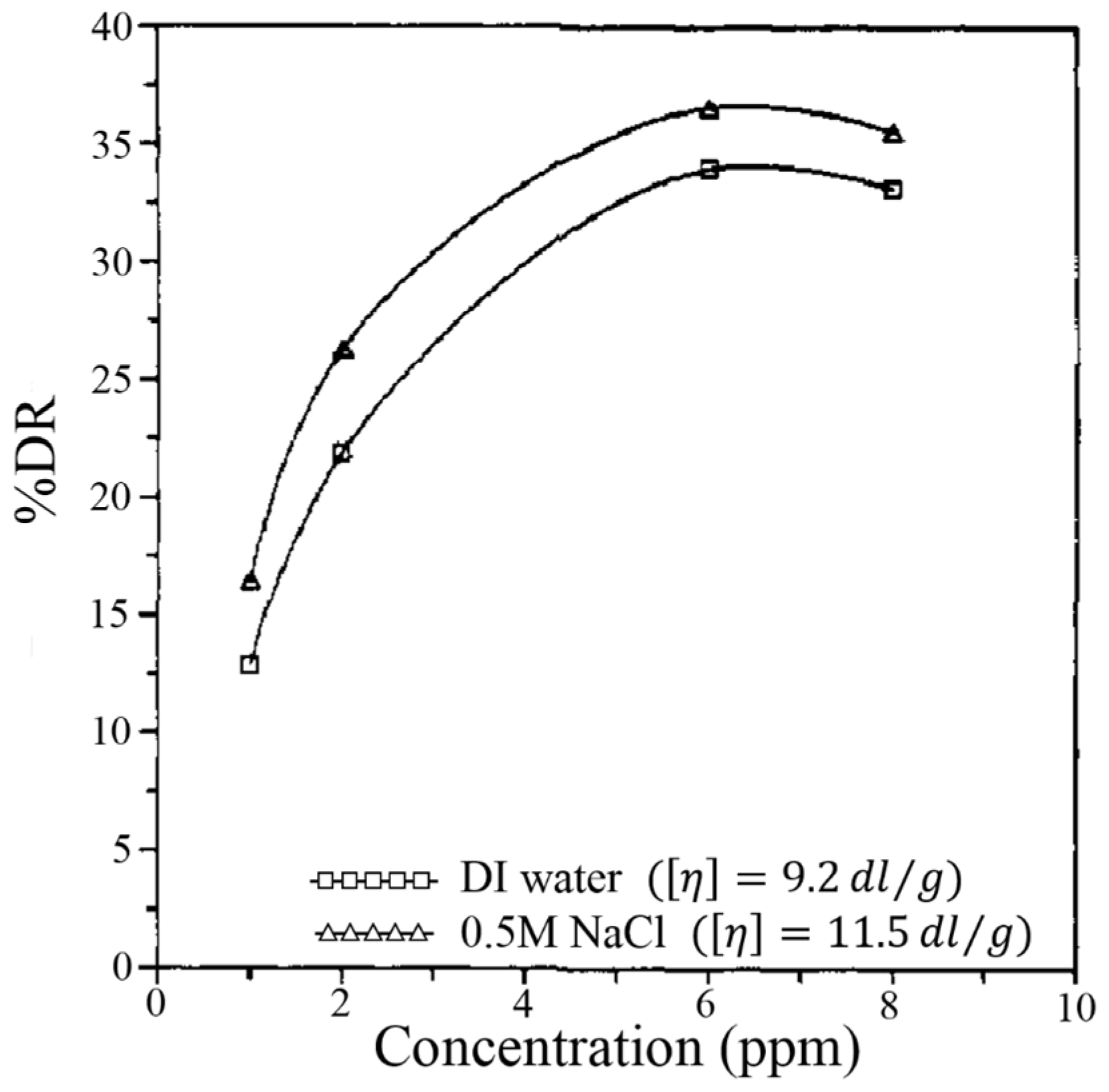
3.2. Polyacrylamide
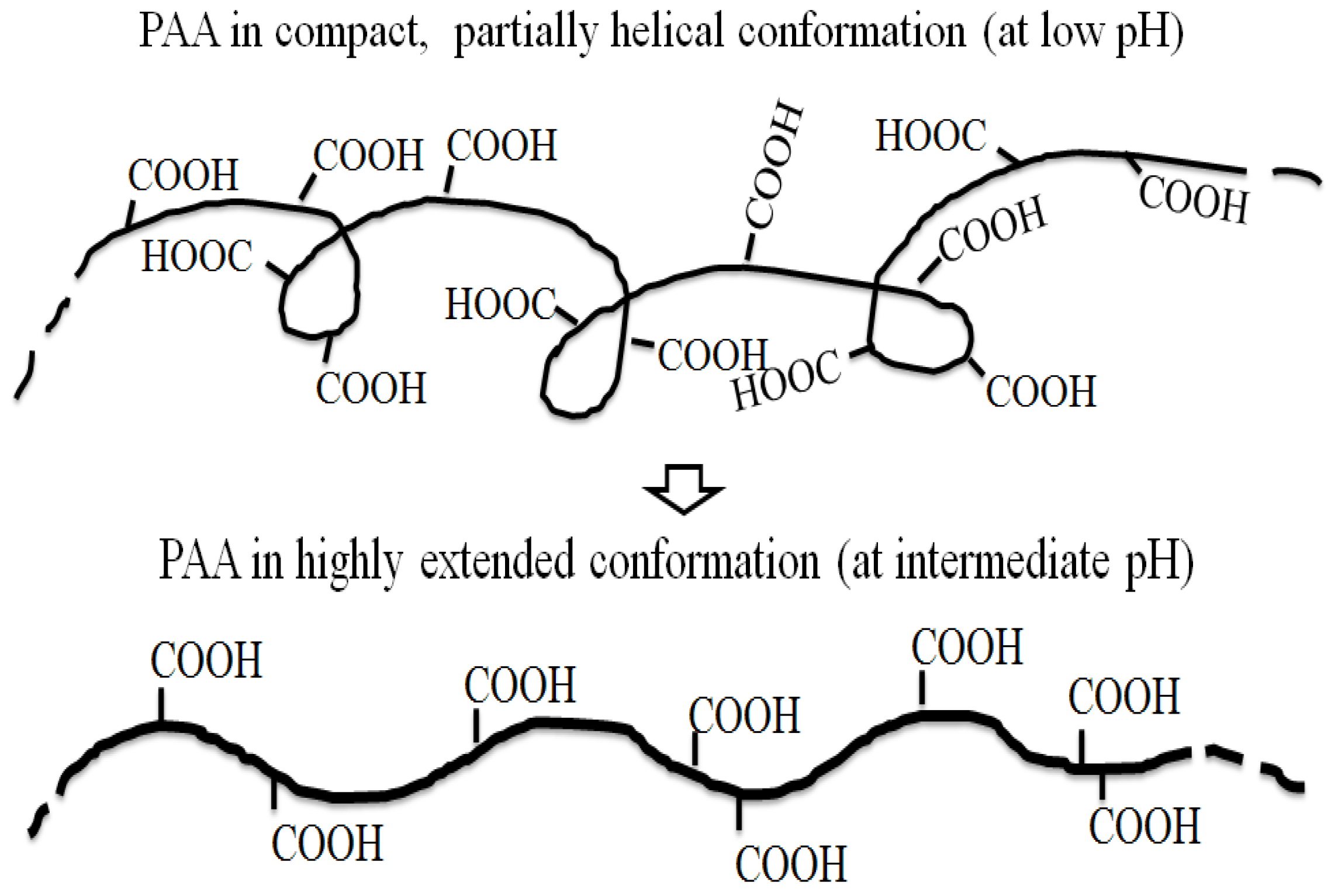
3.3. Poly(acrylic acid)
3.4. Poly(N-vinyl formamide)
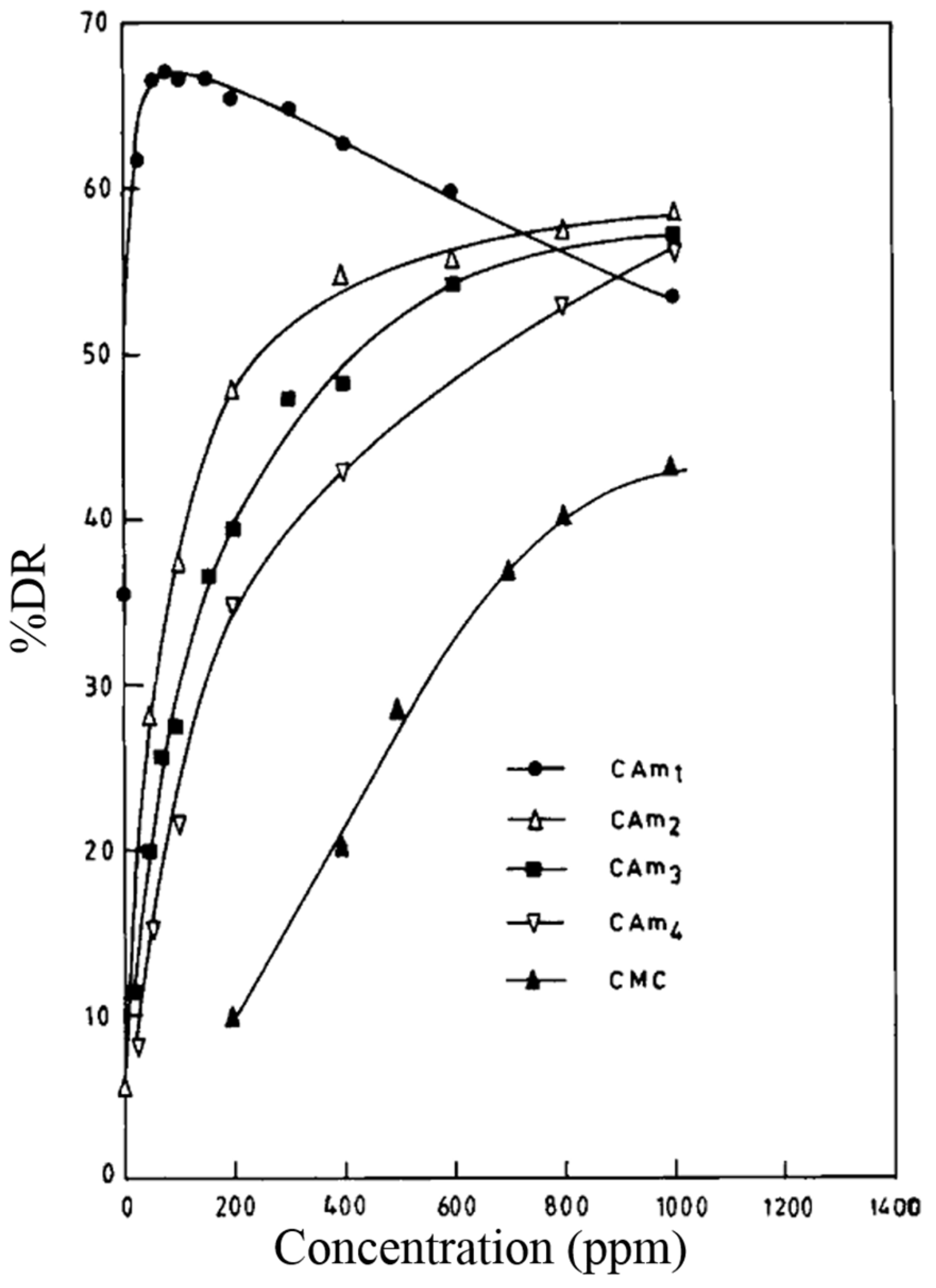
3.5. Water-Soluble Copolymer
4. Natural Water-Soluble Polymers
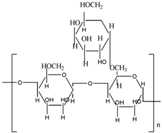
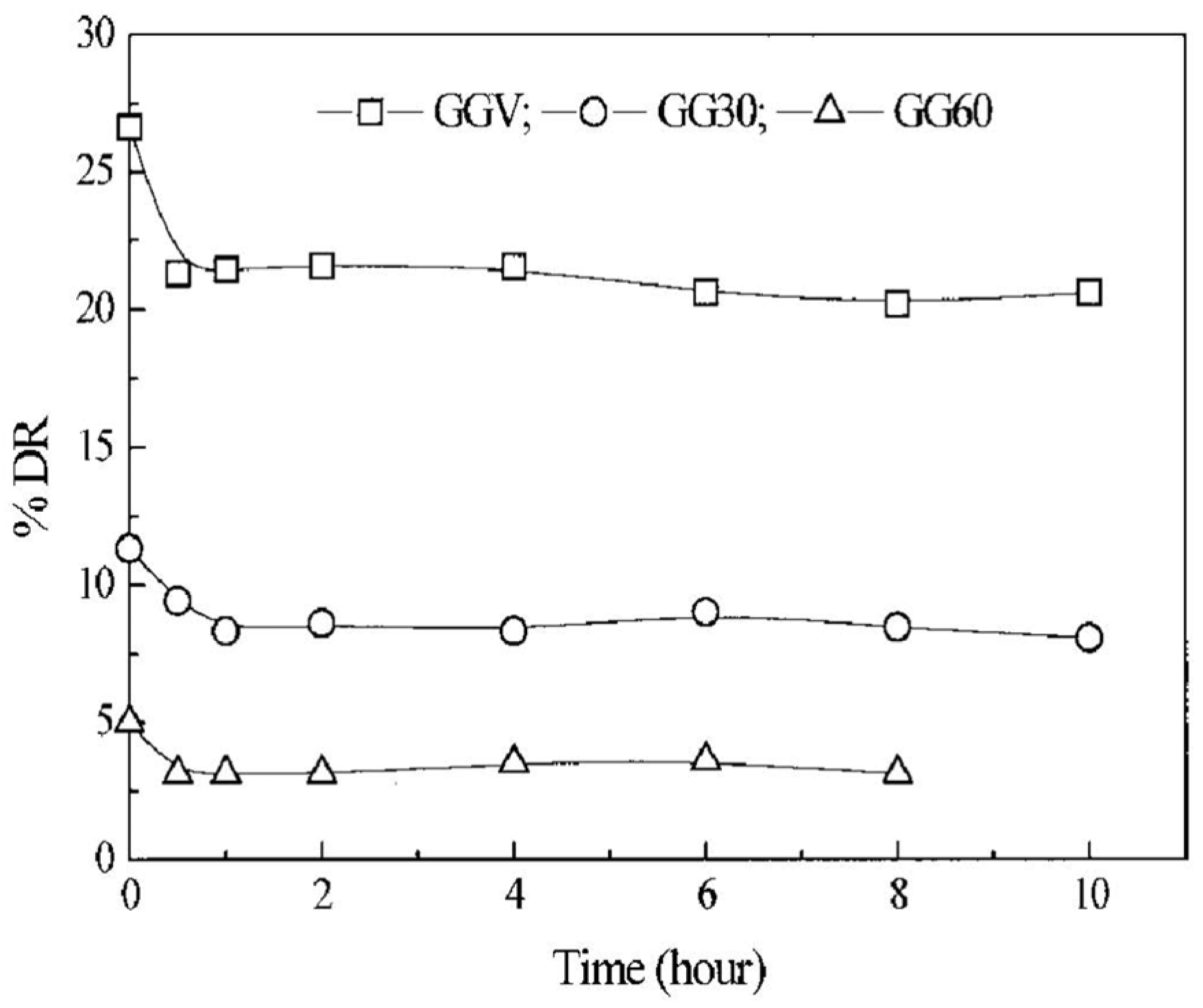
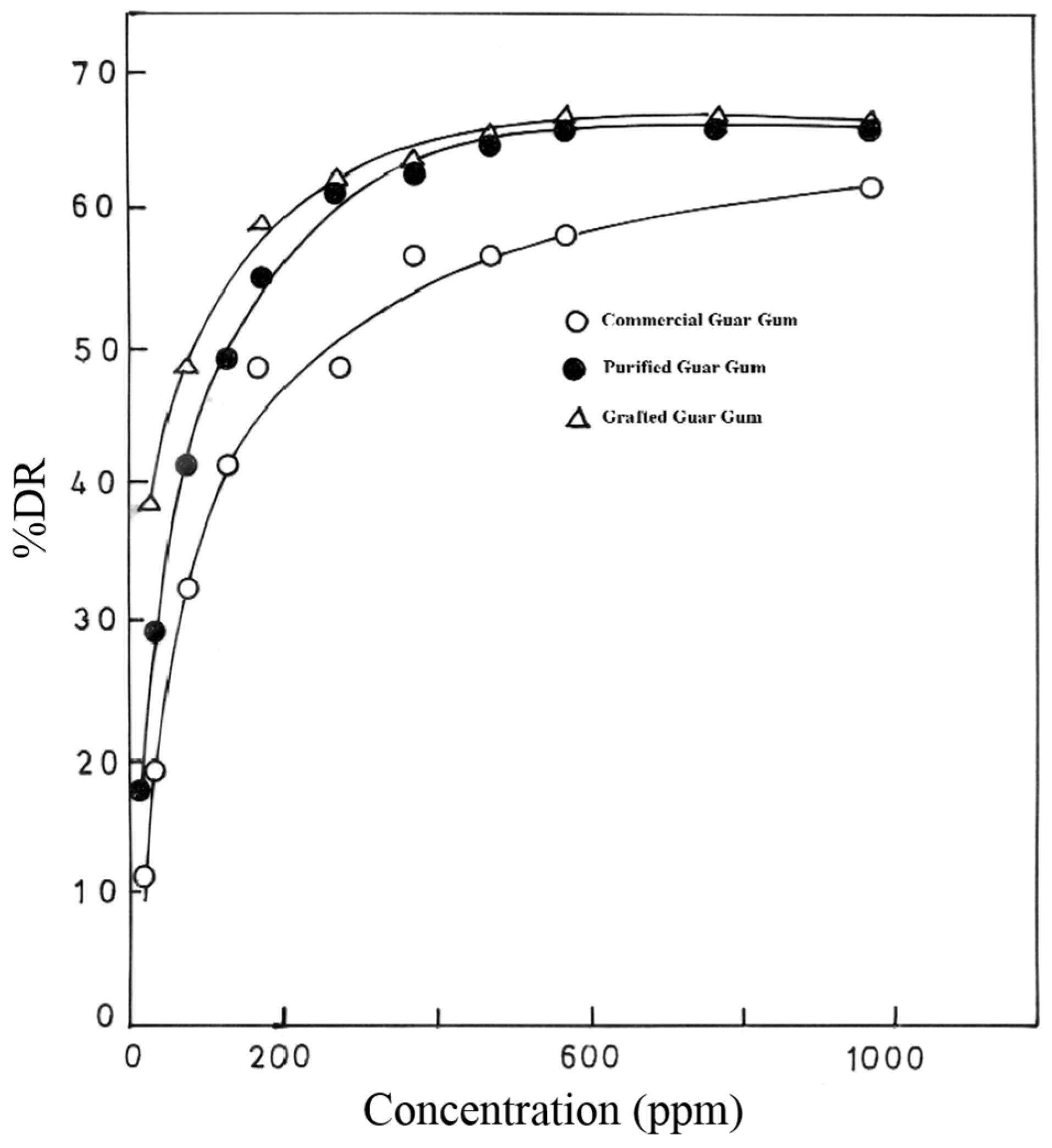
4.1. Guar Gum
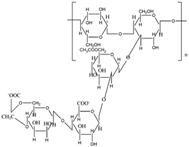
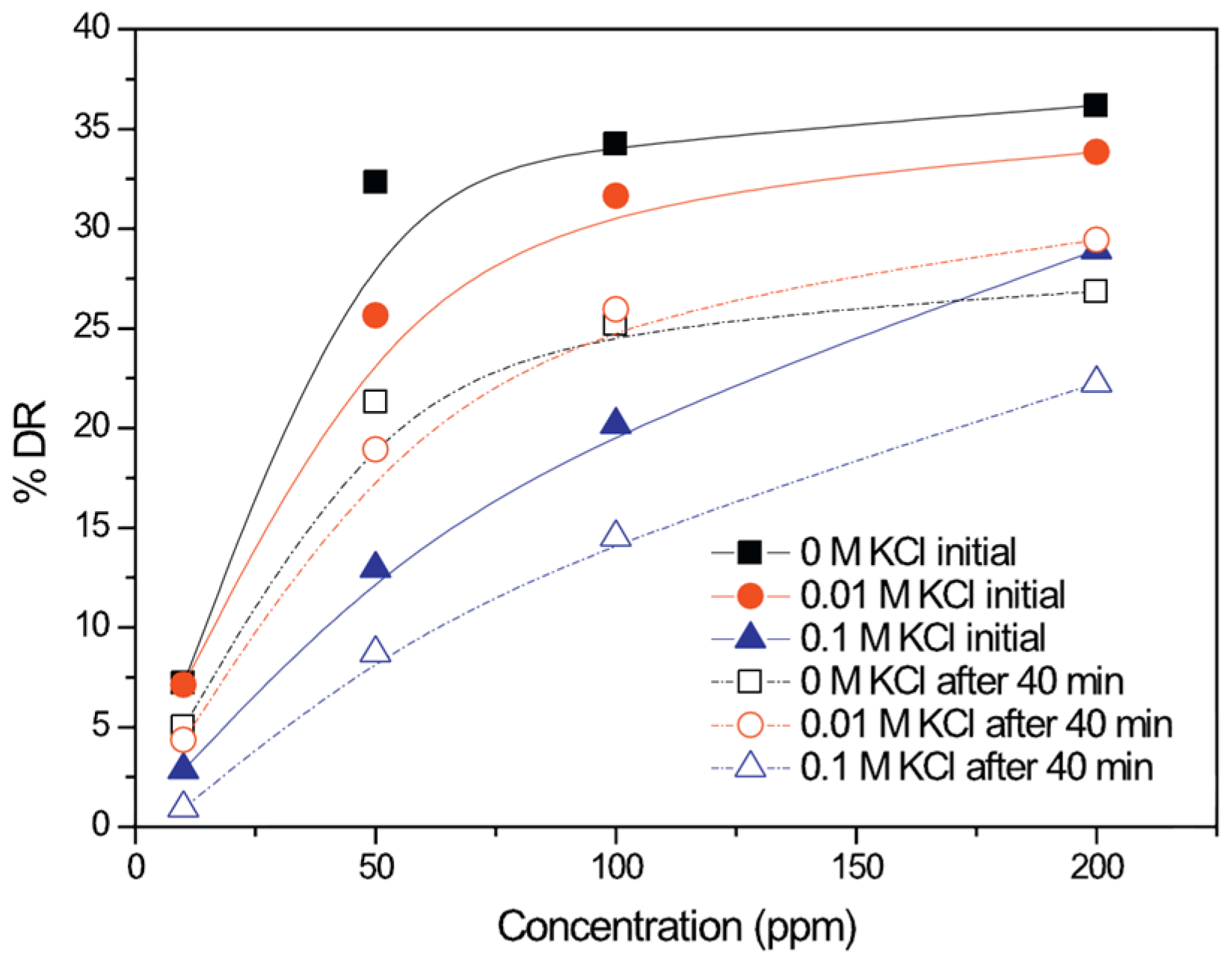
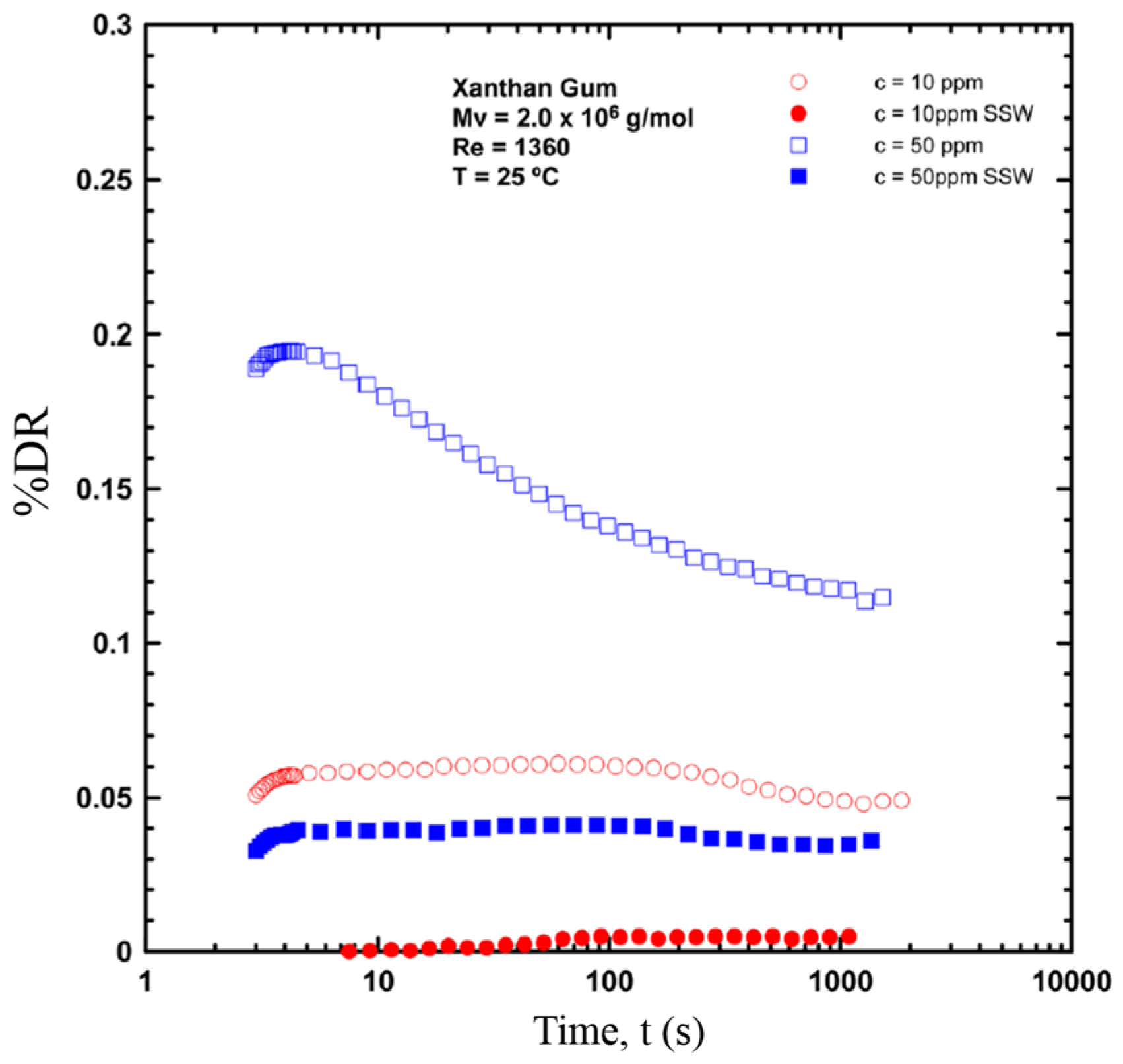
4.2. Xanthan Gum
4.3. Carboxymethyl Cellulose
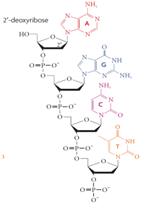
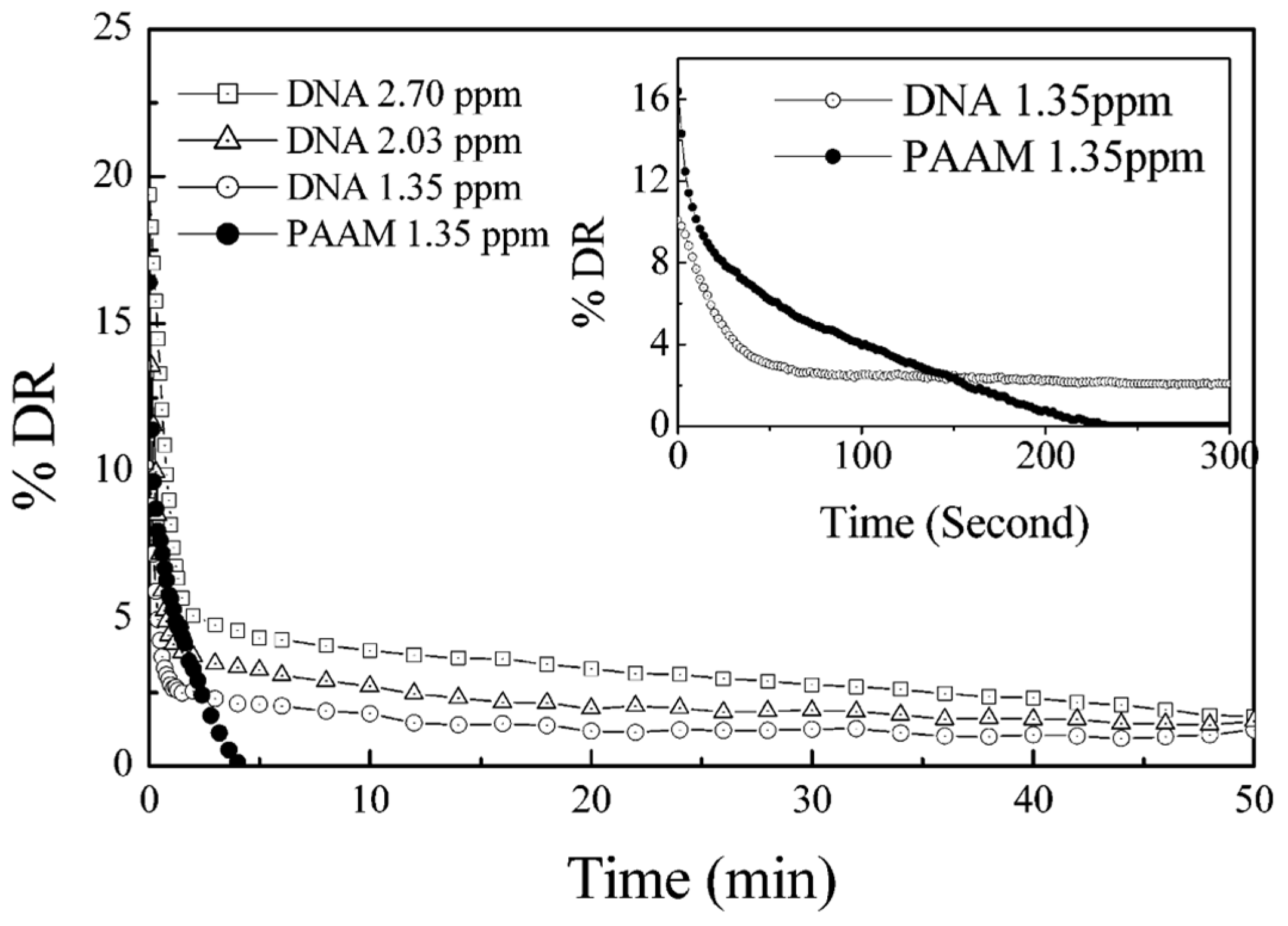
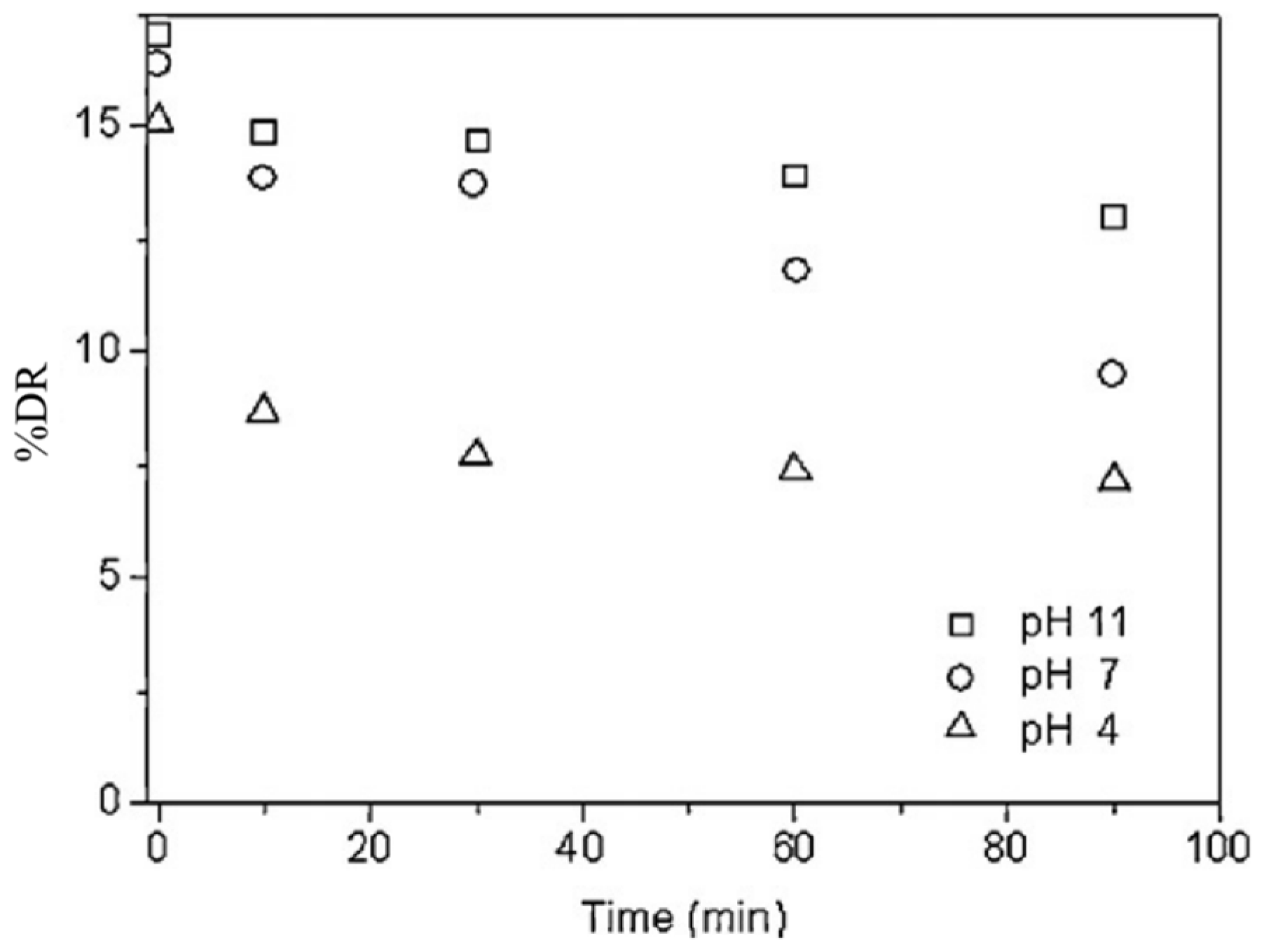
4.4. DNA
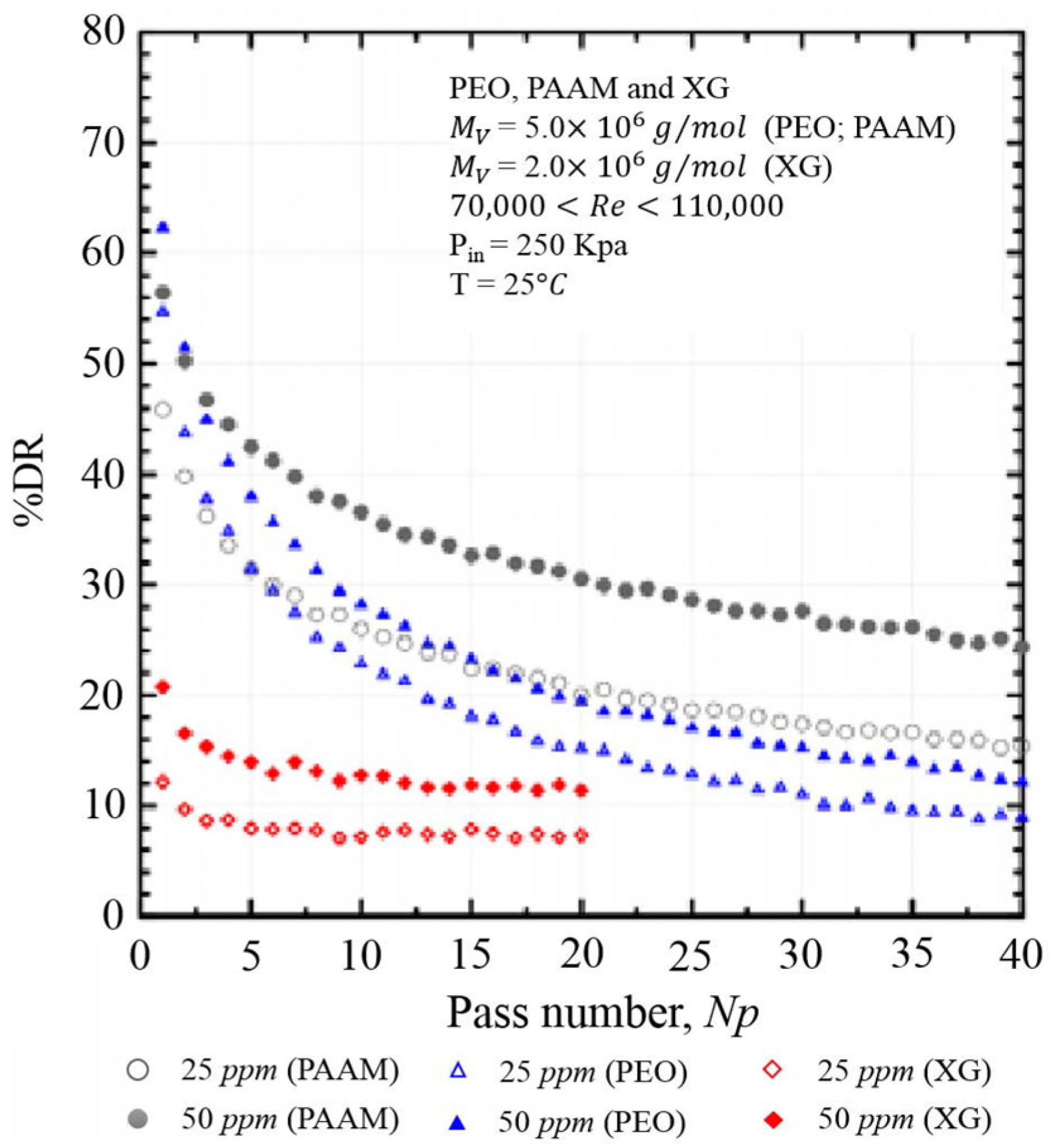
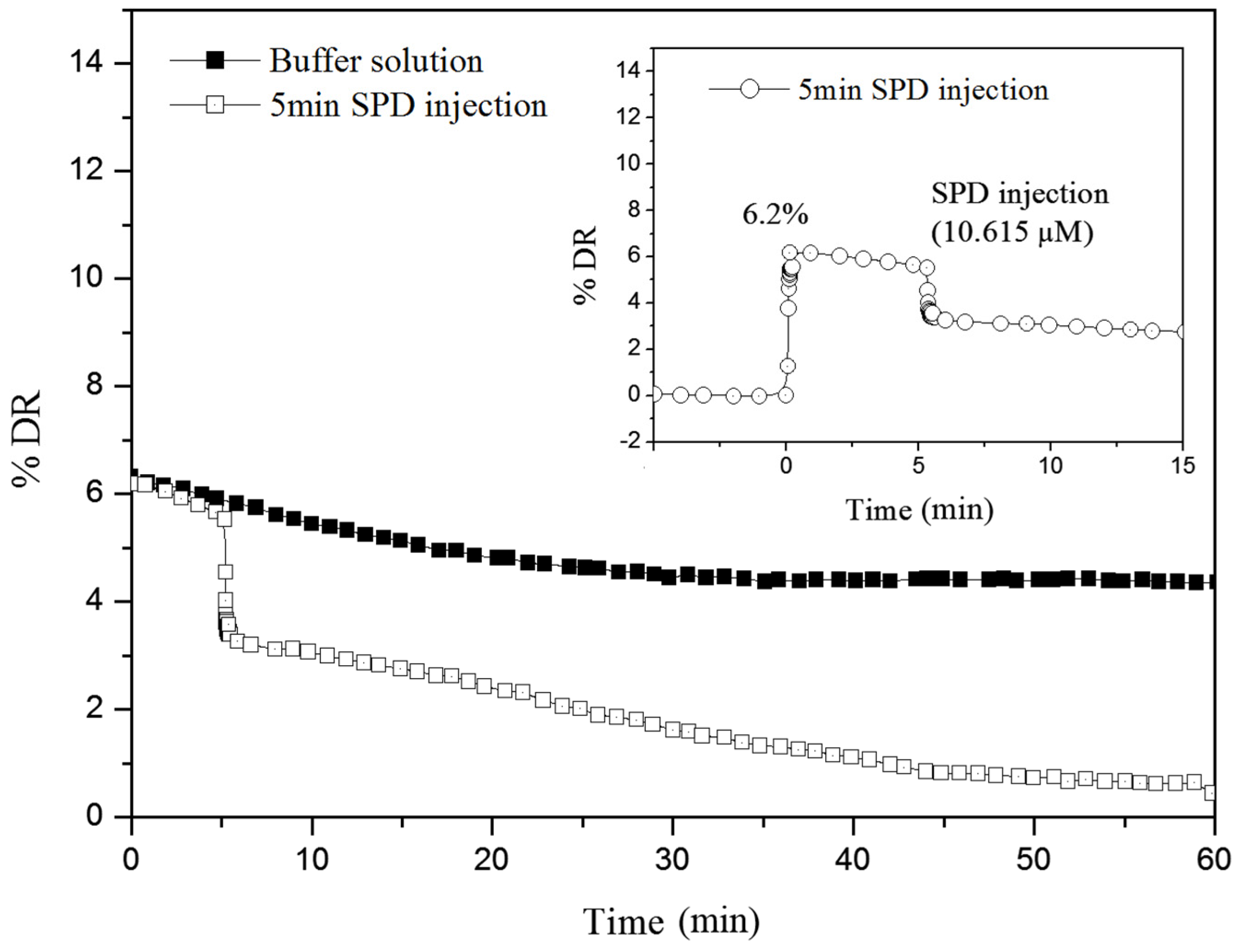
5. Applications of Polymer-Induced Drag Reduction
6. Conclusions
Acknowledgments
Conflicts of Interest
References
- White, C.M.; Mungal, M.G. Mechanics and prediction of turbulent drag reduction with polymer additives. Annu. Rev. Fluid Mech. 2008, 40, 235–256. [Google Scholar] [CrossRef]
- Ranade, V.V.; Mashelkar, R.A. Turbulent mixing in dilute polymer-solutions. Chem. Eng. Sci. 1993, 48, 1619–1628. [Google Scholar] [CrossRef]
- Abubakar, A.; Al-Wahaibi, T.; Al-Wahaibi, Y.; Al-Hashmi, A.R.; Al-Ajmi, A. Roles of drag reducing polymers in single- and multi-phase flows. Chem. Eng. Res. Des. 2014, 92, 2153–2181. [Google Scholar] [CrossRef]
- Toms, B.A. Some observations on the flow of linear polymer solutions through straight tubes at large Reynolds numbers. Proc. First Int. Congr. Rheol. 1948, 2, 135–141. [Google Scholar]
- Manzhai, V.N.; Nasibullina, Y.R.; Kuchevskaya, A.S.; Filimoshkin, A.G. Physico-chemical concept of drag reduction nature in dilute polymer solutions (the Toms effect). Chem. Eng. Process. 2014, 80, 38–42. [Google Scholar] [CrossRef]
- Brostow, W. Drag reduction in flow: Review of applications, mechanism and prediction. J. Ind. Eng. Chem. 2008, 14, 409–416. [Google Scholar] [CrossRef]
- Greene, H.L.; Mostardi, R.F.; Nokes, R.F. Effects of drag reducing polymers on initiation of atherosclerosis. Polym. Eng. Sci. 1980, 20, 499–504. [Google Scholar] [CrossRef]
- Usui, H.; Li, L.; Suzuki, H. Rheology and pipeline transportation of dense fly ash-water slurry. Korea-Aust. Rheol. J. 2001, 13, 47–54. [Google Scholar]
- Zhang, Y.; Schmidt, J.; Talmon, Y.; Zakin, J.L. Co-solvent effects on drag reduction, rheological properties and micelle microstructures of cationic surfactants. J. Colloid Interface Sci. 2005, 286, 696–709. [Google Scholar] [CrossRef] [PubMed]
- Wei, J.J.; Kawaguchi, Y.; Li, F.C.; Yu, B.; Zakin, J.L.; Hart, D.J.; Zhang, Y. Drag-reducing and heat transfer characteristics of a novel zwitterionic surfactant solution. Int. J. Heat Mass Tranf. 2009, 52, 3547–3554. [Google Scholar] [CrossRef]
- Li, F.C.; Wang, D.Z.; Kawaguchi, Y.; Hishida, K. Simultaneous measurements of velocity and temperature fluctuations in thermal boundary layer in a drag-reducing surfactant solution flow. Exp. Fluids 2004, 36, 131–140. [Google Scholar] [CrossRef]
- Mizunuma, H.; Kobayashi, T.; Tominaga, S. Drag reduction and heat transfer in surfactant solutions with excess counterion. J. Non-Newton. Fluid 2010, 165, 292–298. [Google Scholar] [CrossRef]
- Brunchi, C.E.; Morariu, S.; Bercea, M. Intrinsic viscosity and conformational parameters of xanthan inaqueous solutions: Salt addition effect. Colloid Surf. B 2014, 122, 512–519. [Google Scholar] [CrossRef] [PubMed]
- Qi, Y.; Kawaguchi, Y.; Lin, Z.; Ewing, M.; Christensen, R.N.; Zakin, J.L. Enhanced heat transfer of drag reducing surfactant solutions with fluted tube-in-tube heat exchanger. Int. J. Heat Mass Tranf. 2001, 44, 1495–1505. [Google Scholar] [CrossRef]
- Fu, Z.; Otsuki, T.; Motozawa, M.; Kurosawa, T.; Yu, B.; Kawaguchi, Y. Experimental investigation of polymer diffusion in the drag-reduced turbulent channel flow of inhomogeneous solution. Int. J. Heat Mass Transf. 2014, 77, 860–873. [Google Scholar] [CrossRef]
- Yang, J.W.; Park, H.; Chun, H.H.; Ceccio, S.L.; Perlin, M.; Lee, I. Development and performance at high Reynolds number of a skin-friction reducing marine paint using polymer additives. Ocean Eng. 2014, 84, 183–193. [Google Scholar] [CrossRef]
- Sadicoff, B.L.; Brandão, E.M.; Lucas, E.F. Rheological Behaviour of Poly (Acrylamide-G-Propylene Oxide) Solutions: Effect of Hydrophobic Content, Temperature and Salt Addition. Int. J. Polym. Mater. 2000, 47, 399–406. [Google Scholar] [CrossRef]
- Bhambri, P.; Narain, R.; Fleck, B.A. Thermo-responsive polymers for drag reduction in turbulent Taylor–Couette flow. J. Appl. Polym. Sci. 2016, 133, 44191. [Google Scholar] [CrossRef]
- Patterson, G.K.; Zakin, J.L.; Rodriguez, J.M. Drag Reduction-Polymer Solutions, Soap Solutions and Solid Particle Suspensions in Pipe Flow. Ind. Eng. Chem. 1969, 61, 22–30. [Google Scholar] [CrossRef]
- Wu, X.; Wang, D.; Gao, Y.; Zhao, S.S.; Zheng, W.L. Mechanism analysis on powerful chip and drag reduction of the polymer drilling fluid. Procedia Eng. 2014, 73, 41–47. [Google Scholar]
- Chagas, B.S., Jr.; Machado, D.L.P.; Haag, R.B.; Sousa, C.R.D.; Lucas, E.F. Evaluation of hydrophobically associated polyacrylamide-containing aqueous fluids and their potential use in petroleum recovery. J. Polym. Sci. Part A Polym. Chem. 2004, 91, 3686–3692. [Google Scholar] [CrossRef]
- Eshrati, M.; Al-Hashmi, A.R.; Al-Wahaibi, T.; Al-Wahaibi, Y.; Al-Ajmi, A.; Abubakar, A. Drag reduction using high molecular weight polyacrylamides during multiphase flow of oil and water: A parametric study. J. Petrol. Sci. Eng. 2015, 135, 403–409. [Google Scholar] [CrossRef]
- Yusuf, N.; Al-Wahaibi, T.; Al-Wahaibi, Y.; Al-Ajmi, A.; Al-Hashmi, A.R.; Olawale, A.S.; Mohammed, I.A. Experimental study on the effect of drag reducing polymer on flow patterns and drag reduction in a horizontal oil-water flow. Int. J. Heat Fluid Flow 2012, 37, 74–80. [Google Scholar] [CrossRef]
- Abubakar, A.; Al-Wahaibi, Y.; Al-Hashmi, A.R.; Al-Wahaibi, Y.; Al-Ajmi, A.; Eshrati, M. Empirical correlation for predicting pressure gradients of oil-water flow with drag-reducing polymer. Exp. Therm. Fluid Sci. 2016, 79, 275–282. [Google Scholar] [CrossRef]
- Astarita, G. Possible interpretation of mechanism of drag reduction in viscoelastic liquids. Ind. Eng. Chem. Fundam. 1965, 4, 354–356. [Google Scholar] [CrossRef]
- Gadd, G.E. Reduction of turbulent drag in liquids. Nature 1971, 230, 29–31. [Google Scholar] [CrossRef]
- Shenoy, A.V. A review on drag reduction with special reference to micellar systems. Colloid Polym. Sci. 1984, 262, 319–337. [Google Scholar] [CrossRef]
- Tulin, M.P.B. Hydrodynamic aspects of macromolecular solutions. In Proceedings of the 6th Symposium on Naval Hydrodynamics, Washington, DC, USA, 28 September–4 October 1966; pp. 3–18. [Google Scholar]
- Lumley, J.L. Drag reduction in turbulent flow by polymer additives. J. Polym. Sci. Macromol. Rev. 1973, 7, 263–290. [Google Scholar] [CrossRef]
- Min, T.; Yoo, J.Y.; Choi, H.; Joseph, D.D. Drag reduction by polymer additives in a turbulent channel flow. J. Fluid Mech. 2003, 486, 213–238. [Google Scholar] [CrossRef]
- Kostic, M. The ultimate asymptotes and possible causes of friction drag and heat transfer reduction phenomena. J. Energy Heat Mass Transf. 1994, 16, 1–14. [Google Scholar]
- Armstrong, R.; Jhon, M.S. Turbulence induced change in the conformation of polymer molecules. J. Chem. Phys. 1983, 79, 3143–3147. [Google Scholar] [CrossRef]
- Hidema, R.; Suzuki, H.; Hisamatsu, S.; Komoda, Y.; Furukawa, H. Effects of the extensional rate on two-dimensional turbulence of semi-dilute polymer solution flows. Rheol. Acta 2013, 52, 949–961. [Google Scholar] [CrossRef]
- Brostow, W. Drag reduction and mechanical degradation in polymer solutions in flow. Polymer 1983, 24, 631–638. [Google Scholar] [CrossRef]
- Pereira, A.S.; Soares, E.J. Polymer degradation of dilute solutions in turbulent drag reducing flows in a cylindrical double gap rheometer device. J. Non-Newton. Fluid Mech. 2012, 179–180, 9–22. [Google Scholar] [CrossRef]
- Den Toonder, J.M.J.; Hulsem, M.A.; Kuiken, G.D.C.; Nieuwstadt, F.M. Drag reduction by polymer additives in a turbulent pipe flow: numerical and laboratory experiments. J. Fluid Mech. 1997, 337, 193–231. [Google Scholar] [CrossRef]
- White, C.M.; Somandepalli, V.S.R.; Mungal, M.G. The turbulence structure of drag-reduced boundary layer flow. Exp. Fluids 2004, 36, 62–69. [Google Scholar] [CrossRef]
- Zadrazil, I.; Bismarck, A.; Hewitt, G.F.; Markides, C.N. Shear layers in the turbulent pipe flow of drag reducing polymer solutions. Chem. Eng. Sci. 2012, 72, 142–154. [Google Scholar] [CrossRef]
- Cai, W.H.; Li, F.C.; Zhang, H.N.; Li, X.B.; Yu, B.; Wei, J.J.; Kawaguchi, Y.; Hishida, K. Study on the characteristics of turbulent drag-reducing channel flow by particle image velocimetry combining with proper orthogonal decomposition analysis. Phys. Fluids 2009, 21, 115103. [Google Scholar] [CrossRef]
- Warholic, M.D.; Massah, H.; Hanratty, T.J. Influence of drag-reducing polymers on turbulence: effects of Reynolds number, concentration and mixing. Exp. Fluids 1999, 27, 461–472. [Google Scholar] [CrossRef]
- Itoh, M.; Tamano, S.; Yokota, K.; Ninagawa, M. Velocity measurement in turbulent boundary layer of drag-reducing surfactant solution. Phys. Fluids 2005, 17, 075107. [Google Scholar] [CrossRef]
- Wei, T.; Willmarth, W.W. Modifying turbulent structure with drag-reducing polymer additives in turbulent channel flows. J. Fluid Mech. 1992, 245, 619–641. [Google Scholar] [CrossRef]
- Wedgewood, L.E.; Ostrov, D.N.; Bird, B.R. A finitely extensible beadspring chain model for dilute polymer solutions. J. Non-Newton. Fluid Mech. 1991, 40, 119–139. [Google Scholar] [CrossRef]
- Li, F.C.; Cai, W.H.; Zhang, H.N.; Wang, Y. Inuence of polymer additives on turbulent energy cascading in forced homogeneous isotropic turbulence studied by direct numerical simulations. Chin. Phys. B 2012, 21, 114701. [Google Scholar] [CrossRef]
- Eshrati, M.; Al-Hashmi, A.R.; Ranjbaran, M.; Al-Wahaibi, T.; Al-Wahaibi, Y.; Al-Ajmi, A.; Abubakar, A. Prediction of water-soluble polymer drag reduction performance in multiphase flow using fuzzy logic technique. Int. J. Adv. Chem. Environ. Eng. Biol. Sci. 2016, 3, 1507–1515. [Google Scholar]
- Zadeh, L.A. Similarity relations and fuzzy orderings. Inform. Sci. 1971, 3, 177–200. [Google Scholar] [CrossRef]
- Amrouchene, Y.; Kellay, H. Polymer in 2D turbulence: Suppression of large scale fluctuation. Phys. Rev. Lett. 2002, 89, 088302. [Google Scholar] [CrossRef] [PubMed]
- Jun, Y.; Zhang, J.; Wu, X.L. Polymer effect on small and large scale two-dimensional turbulece. Phys. Rev. Lett. 2006, 96, 024502. [Google Scholar] [CrossRef] [PubMed]
- Kraichnan, R.H. Inertial ranges in Two-dimensional turbulence. Phys. Fluids 1967, 10, 1417–1423. [Google Scholar] [CrossRef]
- Hidema, R.; Suzuki, H.; Hisamatsu, S.; Komoda, Y. Characteristic scales of two-dimensional turbulence in polymer solutions. AIChE J. 2014, 60, 1854–1862. [Google Scholar] [CrossRef]
- Virk, P.S.; Merrill, E.W.; Mickley, H.S.; Smith, K.A. The Toms phenomenon: Turbulent pipe flow of dilute polymer solutions. J. Fluid Mech. 1967, 30, 305–328. [Google Scholar] [CrossRef]
- Little, R.C.; Patterson, R.L.; Ting, R.Y. Characterization of the Drag Reducing Properties of Poly(ethylene oxide) and Poly(acrylamide) Solutions in External Flows. J. Chem. Eng. Data 1976, 21, 281–283. [Google Scholar] [CrossRef]
- Little, R.C. Drag reduction in capillary tubes as a function of polymer concentration and molecular weight. J. Colloid Interface Sci. 1971, 37, 811–818. [Google Scholar] [CrossRef]
- Little, R.C. Flow Properties of Polyox Solutions. Ind. Eng. Chem. Fund. 1969, 8, 557–559. [Google Scholar] [CrossRef]
- Choi, H.J.; Jhon, M.S. Polymer induced Turbulent Drag Reduction. Ind. Eng. Chem. Res. 1996, 35, 2993–2998. [Google Scholar] [CrossRef]
- Kim, C.A.; Sung, J.H.; Choi, H.J.; Kim, C.B.; Chun, W.; Jhon, M.S. Drag Reduction and Mechanical Degradation of Poly(ethylene oxide) in Seawater. J. Chem. Eng. Jpn. 1999, 32, 803–811. [Google Scholar] [CrossRef]
- Choi, H.J.; Kim, C.A.; Sung, J.H.; Kim, C.B.; Chun, W.; Jhon, M.S. Universal drag reduction characteristics of saline water-soluble poly(ethylene oxide) in a rotating disk apparatus. Colloid Polym. Sci. 2000, 278, 701–705. [Google Scholar] [CrossRef]
- Lim, S.T.; Hong, C.H.; Choi, H.J.; Lai, P.Y.; Chan, C.K. Polymer turbulent drag reduction near the theta point. Eur. Phys. Lett. 2007, 80, 58003. [Google Scholar] [CrossRef]
- Sung, J.H.; Kim, C.A.; Choi, H.J.; Hur, B.K.; Kim, J.G.; Jhon, M.S. Turbulent drag reduction efficiency and mechanical degradation of poly(acrylamide). J. Macromol. Sci. Phys. 2004, B43, 507–518. [Google Scholar] [CrossRef]
- Sandoval, G.A.B.; Trevelin, R.; Soares, E.J.; Silveira, L.; Thomaz, F.; Pereira, A.S. Polymer degradation in turbulent drag reducing flows in pipes. Therm. Eng. 2015, 14, 03–06. [Google Scholar]
- Pereira, A.S.; Andrade, R.M.; Soares, E.J. Drag reduction induced by flexible and rigid molecules in a turbulent flow into a rotating cylindrical double gap device: Comparison between Poly(ethylene oxide), Polyacrylamide, and Xanthan Gum. J. Non-Newton. Fluid 2013, 202, 72–87. [Google Scholar] [CrossRef]
- Lim, S.T.; Choi, H.J. λ-DNA induced turbulent drag reduction and its characteristics. Macromolecules 2003, 36, 5348–5354. [Google Scholar] [CrossRef]
- Zhang, X.; Liu, L.; Cheng, L.; Guo, Q.; Zhang, N. Experimental study on heat transfer and pressure drop characteristics of air–water two-phase flow with the effect of polyacrylamide additive in a horizontal circular tube. Int. J. Heat Mass Tranf. 2013, 58, 427–440. [Google Scholar] [CrossRef]
- Terao, K. Poly(acrylic acid) (PAA). In Encyclopedia of Polymeric Nanomaterials; Springer: Berlin/Heidelberg, Germany, 2014; pp. 1–6. [Google Scholar]
- Montante, G.; Laurenzi, F.; Paglianti, A.; Magelli, F. A study on some effects of a drag-reducing agent on the performance of a stirred vessel. Chem. Eng. Des. Des. 2011, 89, 2262–2267. [Google Scholar] [CrossRef]
- Kim, J.T.; Kim, C.A.; Zhang, K.; Jang, C.H.; Choi, H.J. Effect of polymer–surfactant interaction on its turbulent drag reduction. Colloid Surf. A 2011, 391, 125–129. [Google Scholar] [CrossRef]
- Kim, O.K.; Choi, L.S.; Long, T.; McGrath, K.; Armistead, J.P.; Yoon, T.H. Unusual complexation behavior of poly(acry1ic acid) induced by shear. Macromolecules 1993, 26, 379–384. [Google Scholar] [CrossRef]
- Zhang, K.; Choi, H.J.; Jang, C.H. Turbulent drag reduction characteristics of poly(acrylamide-co-acrylic acid) in a rotating disk apparatus. Colloid Polym. Sci. 2011, 289, 821–1827. [Google Scholar] [CrossRef]
- Zhang, K.; Lim, G.H.; Choi, H.J. Mechanical degradation of water-soluble acrylamide copolymer under a turbulent flow: Effect of molecular weight and temperature. J. Ind. Eng. Chem. 2016, 33, 156–161. [Google Scholar] [CrossRef]
- Cole, D.P.; Khosravi, E.; Musa, O.M. Efficient water-Soluble drag reducing star polymers with improved mechanical stability. J. Polym. Sci. Polym. Chem. 2016, 54, 335–344. [Google Scholar] [CrossRef]
- Pinschmidt, R.K.; Renz, W.L.; Carroll, W.E.; Yocoub, K.; Drescher, J.; Nordquist, A.F.; Chen, N. N-Vinylformamide—Building Block for Novel Polymer Structures. J. Macromol. Sci. Pure Appl. Chem. 1997, A34, 1885–1905. [Google Scholar] [CrossRef]
- Gu, L.; Zhu, S.; Hrymak, A.N.; Pelton, R.H. Kinetics and modeling of free radical polymerization of N-vinylformamide. Polymer 2001, 42, 3077–3086. [Google Scholar] [CrossRef]
- Marhefka, J.N.; Marascalco, P.J.; Chapman, T.M.; Russell, A.J.; Kameneva, M.V. Poly(N-vinylformamide)—A drag-reducing polymer for biomedical applications. Biomacromolecules 2006, 2, 1597–1603. [Google Scholar] [CrossRef] [PubMed]
- Mishra, M.M.; Yadav, M.; Sand, A.; Tripathy, J.; Behari, K. Water soluble graft copolymer (κ-carrageenan-g-N-vinyl formamide): Preparation characterization and application. Carbohydr. Polym. 2010, 80, 235–241. [Google Scholar] [CrossRef]
- Mccormick, C.L.; Hester, R.D.; Morgan, S.E.; Safieddine, A.M. Water-Soluble Copolymers. 30. Effects of Molecular Structure on Drag Reduction Efficiency. Macromolecules 1990, 23, 2124–2131. [Google Scholar] [CrossRef]
- Mumick, P.S.; Welch, P.M.; Salazar, L.C.; Mccormick, C.L. Water-Soluble Copolymers. 56. Structure and Solvation Effects of Polyampholytes in Drag Reduction. Macromolecules 1994, 27, 323–331. [Google Scholar] [CrossRef]
- Brun, N.L.; Zadrazil, I.; Norman, L.; Bismarck, A.; Markides, C.N. On the drag reduction effect and shear stability of improved acrylamide copolymers for enhanced hydraulic fracturing. Chem. Eng. Sci. 2016, 146, 135–143. [Google Scholar] [CrossRef]
- Lumley, J.L. Drag reduction by additives. Ann. Rev. Fluid Mech. 1969, 1, 367–384. [Google Scholar] [CrossRef]
- Reis, L.G.; Oliveira, I.P.; Pires, R.V.; Lucas, E.F. Influence of structure and composition of poly(acrylamide-g-propylene oxide) copolymers on drag reduction of aqueous dispersions. Colloid Surf. A 2016, 502, 121–129. [Google Scholar] [CrossRef]
- Camail, M.; Margaillan, A.; Martin, I. Copolymers of N-alkyl- and N-arylalkylacrylamides with acrylamide: influence of hydrophobic structure on associative properties. Part I: viscometric behaviour in dilute solution and drag reduction performance. Polym. Int. 2009, 58, 149–154. [Google Scholar] [CrossRef]
- Al-Hashmi, A.; Al-Maamari, R.; Al-Shabibi, I.; Mansoor, A.; Al-Sharji, H.; Zaitoun, A. Mechanical stability of high-Molecular-weight polyacrylamides and an (acrylamido tert-butyl sulfonic acid)-acrylamide copolymer used in enhanced oil recovery. J. Appl. Polym. Sci. 2014, 131, 40921. [Google Scholar] [CrossRef]
- Akbar Ali, S.K.; Singh, R.P. An investigation of the flocculation characteristics of polyacrylamide-grafted chitosan. J. Appl. Polym. Sci. 2009, 114, 2410–2414. [Google Scholar]
- Singha, V.; Tiwaria, A.; Tripathia, D.N.; Sanghib, R. Microwave assisted synthesis of Guar-g-polyacrylamide. Carbohydr. Polym. 2004, 58, 1–6. [Google Scholar] [CrossRef]
- Kim, C.A.; Lim, S.T.; Choi, H.J.; Sohn, J.I.; Jhon, M.S. Characterization of drag reducing guar gum in a rotating disk flow. J. Appl. Polym. Sci. 2002, 83, 2938–3944. [Google Scholar] [CrossRef]
- Singh, R.P.; Pal, S.; Krishnamoorthy, S.; Adhikary, P.; Ali, S.A. High-technology materials based on modified polysaccharides. Pure Appl. Chem. 2009, 81, 525–547. [Google Scholar] [CrossRef]
- Deshmukh, S.R.; Chaturvedi, P.N.; Singh, R.P. The turbulent drag reduction by graft copolymers of guargum and polyacrylamide. J. Appl. Polym. Sci. 1985, 30, 4013–4018. [Google Scholar] [CrossRef]
- Behari, K.; Kumar, R.; Tripathi, M.; Pandey, P.K. Graft Copolymerization of Methacrylamide onto guar Gum using a potassium chromate/malonic acid redox pair. Macromol. Chem. Phys. 2001, 202, 1873–1877. [Google Scholar] [CrossRef]
- Bewersdorff, H.W.; Singh, R.P. Rheological and drag reduction characteristics of xanthan gum solutions. Rheol. Acta 1988, 27, 617–627. [Google Scholar] [CrossRef]
- Katzbauer, B. Properties and applications of xanthan gum. Polym. Degrad. Stabil. 1998, 59, 81–84. [Google Scholar] [CrossRef]
- Sohn, J.I.; Kim, C.A.; Choi, H.J.; Jhon, M.S. Drag-reduction effectiveness of xanthan gum in a rotating disk apparatus. Carbohydr. Polym. 2001, 45, 61–68. [Google Scholar] [CrossRef]
- Hong, C.H.; Choi, H.J.; Zhang, K.; Renou, F.; Grisel, M. Effect of salt on turbulent drag reduction of xanthan gum. Carbohydr. Polym. 2015, 121, 342–347. [Google Scholar] [CrossRef] [PubMed]
- Andrade, R.M.; Pereira, A.S.; Soares, E.J. Drag reduction in synthetic seawater by flexible and rigid polymer addition into a rotating cylindrical double gap device. J. Fluid Eng. 2016, 138, 021101. [Google Scholar] [CrossRef]
- Pushpamalar, V.; Langford, S.J.; Ahmad, M.; Lim, Y.Y. Optimization of reaction conditions for preparing carboxymethyl cellulose from sago waste. Carbohydr. Polym. 2006, 64, 312–318. [Google Scholar] [CrossRef]
- Biswal, D.R.; Singh, R.P. Characterisation of carboxymethyl cellulose and polyacrylamide graft copolymer. Carbohydr. Polym. 2004, 57, 379–387. [Google Scholar] [CrossRef]
- Abdulbari, H.A.; Shabirin, A.; Abdurrahman, H.N. Bio-polymers for improving liquid flow in pipelines—A review and future work opportunities. J. Ind. Eng. Chem. 2014, 20, 1157–1170. [Google Scholar] [CrossRef]
- Deshmukh, S.R.; Sudhakar, K.; Singh, R.P. Drag-reduction efficiency, shear stability, and biodegradation resistance of carboxymethyl cellulose-based and starch-based graft copolymers. J. Appl. Polym. Sci. 1991, 43, 1091–1101. [Google Scholar] [CrossRef]
- Rana, T.M. Illuminating the silence: Understanding the structure and function of small RNAs. Nat. Rev. Mol. Cell Biol. 2007, 8, 23–36. [Google Scholar] [CrossRef] [PubMed]
- Choi, H.J.; Lim, S.T.; Lai, P.Y.; Chan, C.K. Turbulent drag reduction and degradation of DNA. Phys. Rev. Lett. 2002, 89, 088302. [Google Scholar] [CrossRef] [PubMed]
- Lim, S.T.; Park, S.J.; Chan, C.K.; Choi, H.J. Turbulent drag reduction characteristics induced by calf-thymus DNA. Physica A 2005, 350, 84–88. [Google Scholar] [CrossRef]
- Lim, S.T.; Choi, H.J.; Chan, C.K. Effect of turbulent flow on coil-globule transition of lambda-DNA. Macromol. Rapid. Commun. 2005, 26, 1237–1240. [Google Scholar] [CrossRef]
- Ueberschär, O.; Wagner, C.; Stangner, T.; Kühne, K.; Gutsche, C.; Kremer, F. Drag reduction by DNA-grafting for single microspheres in a dilute lambda-DNA solution. Polymer 2011, 52, 4021–4032. [Google Scholar] [CrossRef]
- Evans, A.P. A new drag-reducing polymer with improved Shear Stability for nonaqueous systems. J. Appl. Polym. Sci. 1974, 18, 1919–1925. [Google Scholar] [CrossRef]
- Belokon, V.S.; Bespalova, N.B.; Vdovin, V.M.; Vlasov, S.A.; Kalashnikov, V.N.; Ushakov, N.V. Reduction of the hydrodynamic friction of hydrocarbons by means of small additions of certain organosilicon polymers. J. Eng. Phys. Thermophys. 1979, 36, 1–3. [Google Scholar] [CrossRef]
- Rodrigues, R.K.; Folsta, M.G.; Martins, A.L.; Sabadini, E. Tailoring of wormlike micelles as hydrodynamic drag reducers for gravel-pack in oil field operations. J. Petrol. Sci. Eng. 2016, 146, 142–148. [Google Scholar] [CrossRef]
- Mortazavi, S.M.M. Correlation of polymerization conditions with drag reduction efficiency of poly(1-hexene) in oil pipelines. Iran. Polym. J. 2016, 25, 731–737. [Google Scholar] [CrossRef]
- Wang, Z.H.; Yu, X.Y.; Li, J.X.; Wang, J.G.; Zhang, L. The use of biobased surfactant obtained by enzymatic syntheses for wax deposition inhibition and drag reduction in crude Oil Pipelines. Catalysts 2016, 6, 61. [Google Scholar] [CrossRef]
- Taylor, K.C.; Nasr-El-Din, H.A. Water-soluble hydrophobically associating polymers for improved oil recovery: A literature review. J. Petrol. Sci. Eng. 1998, 19, 265–280. [Google Scholar] [CrossRef]
- Wever, D.A.Z.; Picchioni, F.; Broekhuis, A.A. Polymers for enhanced oil recovery: A paradigm for structure–property relationship in aqueous solution. Prog. Polym. Sci. 2011, 36, 1558–1628. [Google Scholar] [CrossRef]
- Wang, L.; Wang, D.; Shen, Y.; Lai, X.; Guo, X. Study on properties of hydrophobic associating polymer as drag reduction agent for fracturing fluid. J. Polym. Res. 2016, 23. [Google Scholar] [CrossRef]
- Forester, R.H.; Larson, R.E.; Hayden, J.W.; Wetzel, J.M. Effects of polymer addition on friction in a 10-inch diameter pipe. J. Hydronaut. 1969, 3, 59–62. [Google Scholar] [CrossRef]
- Sellin, R.H.J. Increasing sewer capacity by polymer dosing. Proc. Inst. Civ. Eng. 1977, 63, 49–67. [Google Scholar] [CrossRef]
- Thurston, S.; Jones, R.D. Experimental model studies of non-newtonian soluble coatings for drag reduction. AIAA 1964. [Google Scholar] [CrossRef]
- Choi, K.S.; Yang, X.; Clayton, B.R.; Glover, E.J.; Altar, M.; Semenov, B.N.; Kulik, V.M. Turbulent drag reduction using compliant surfaces. Proc. Math. Phys. Eng. Sci. 1997, 453, 2229–2240. [Google Scholar] [CrossRef]
- Greene, H.L.; Nokes, R.F.; Thomas, L.C. Biomedical implications of drag reducing agents. Biorheology 1971, 7, 221–223. [Google Scholar] [PubMed]
- Polimeni, P.L.; Coleman, P. Enhancement of aortic blood flow with a linear anionic macropolymer of extraordinary molecular length. J. Mol. Cell. Cardiol. 1985, 17, 721–724. [Google Scholar] [CrossRef]
- Sawchuk, A.P.; Unthank, J.L.; Dalsing, M.C. Drag reducing polymers may decrease atherosclerosis by increasing shear in areas normally exposed to low shear stress. J. Vasc. Surg. 1999, 30, 761–764. [Google Scholar] [CrossRef]
- Kameneva, M.V.; Wu, Z.J.; Uraysh, A.; Repko, B.; Litwak, K.N.; Billiar, T.R.; Fink, M.P.; Simmons, R.L.; Griffith, B.P.; Borovetz, H. Blood soluble drag-reducing polymers prevent lethality from hemorrhagic shock in acute animal experiments. Biorheology 2004, 41, 53–64. [Google Scholar] [PubMed]





| No. | Name | Chemical Structure |
|---|---|---|
| 1. | Poly (ethylene oxide) | 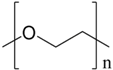 |
| 2. | Polyacrylamide | 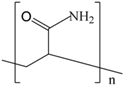 |
| 3. | Poly (acrylic acid) |  |
| 4. | Poly (N-vinyl formamide) | 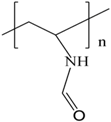 |
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Han, W.J.; Dong, Y.Z.; Choi, H.J. Applications of Water-Soluble Polymers in Turbulent Drag Reduction. Processes 2017, 5, 24. https://doi.org/10.3390/pr5020024
Han WJ, Dong YZ, Choi HJ. Applications of Water-Soluble Polymers in Turbulent Drag Reduction. Processes. 2017; 5(2):24. https://doi.org/10.3390/pr5020024
Chicago/Turabian StyleHan, Wen Jiao, Yu Zhen Dong, and Hyoung Jin Choi. 2017. "Applications of Water-Soluble Polymers in Turbulent Drag Reduction" Processes 5, no. 2: 24. https://doi.org/10.3390/pr5020024
APA StyleHan, W. J., Dong, Y. Z., & Choi, H. J. (2017). Applications of Water-Soluble Polymers in Turbulent Drag Reduction. Processes, 5(2), 24. https://doi.org/10.3390/pr5020024