The Control Effect of Equipment Operation on the Formation of Wellbore Hydrates Under the Production Conditions of Deepwater Gas Wells
Abstract
1. Introduction
2. Research Methodology
2.1. Heat Transfer Modelling for Deepwater Gas Well Operations
2.1.1. Modelling of Fluid Heat Transfer in Pipe Columns
2.1.2. Stratigraphic Heat Transfer Modelling
2.1.3. Modelling of Heat Transfer in Seawater Section
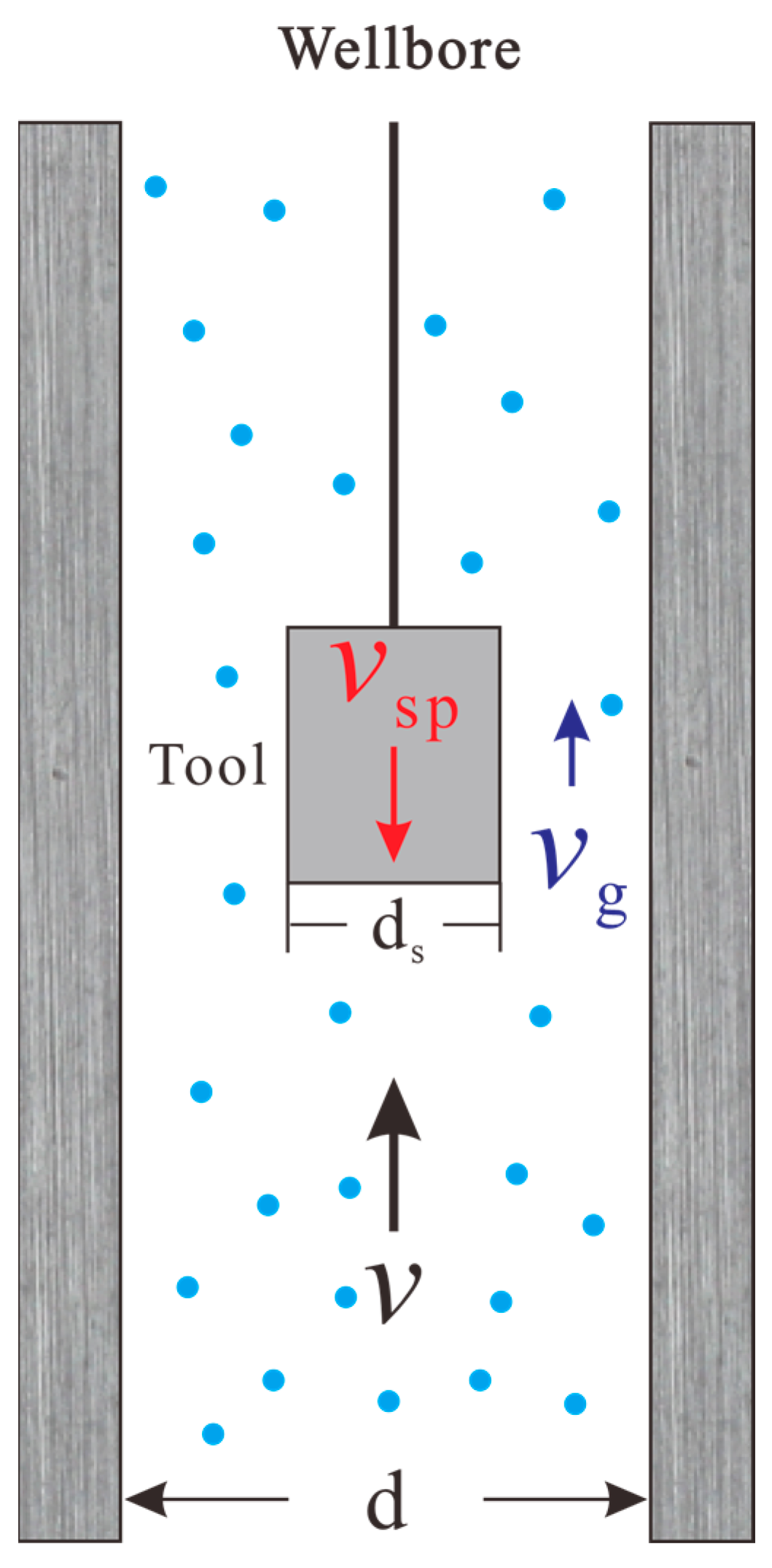
2.2. Wellbore Pressure Modelling for Deepwater Gas Well Operations
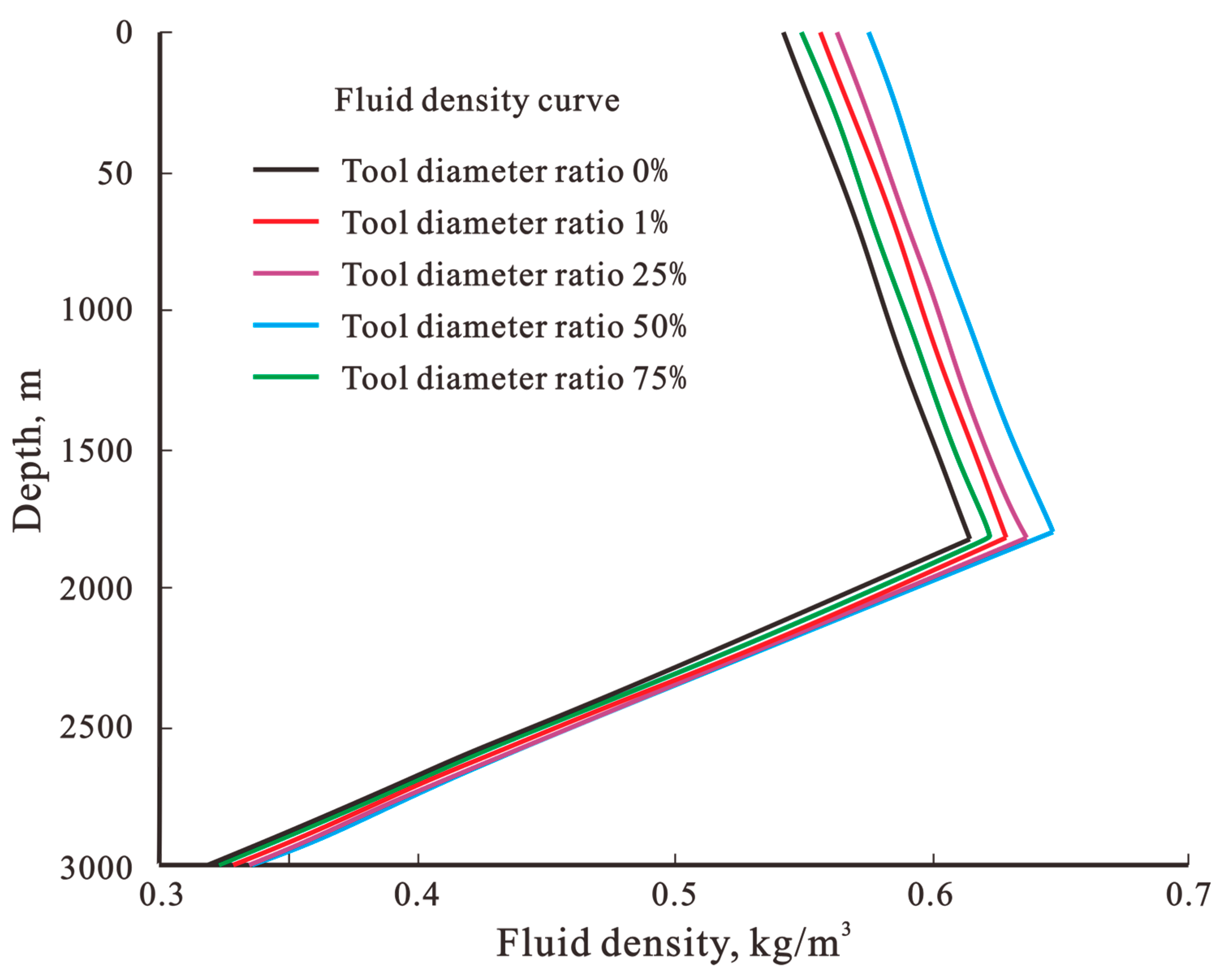
2.3. Wellbore Density Modelling for Deepwater Gas Well Operations
3. Results and Discussion
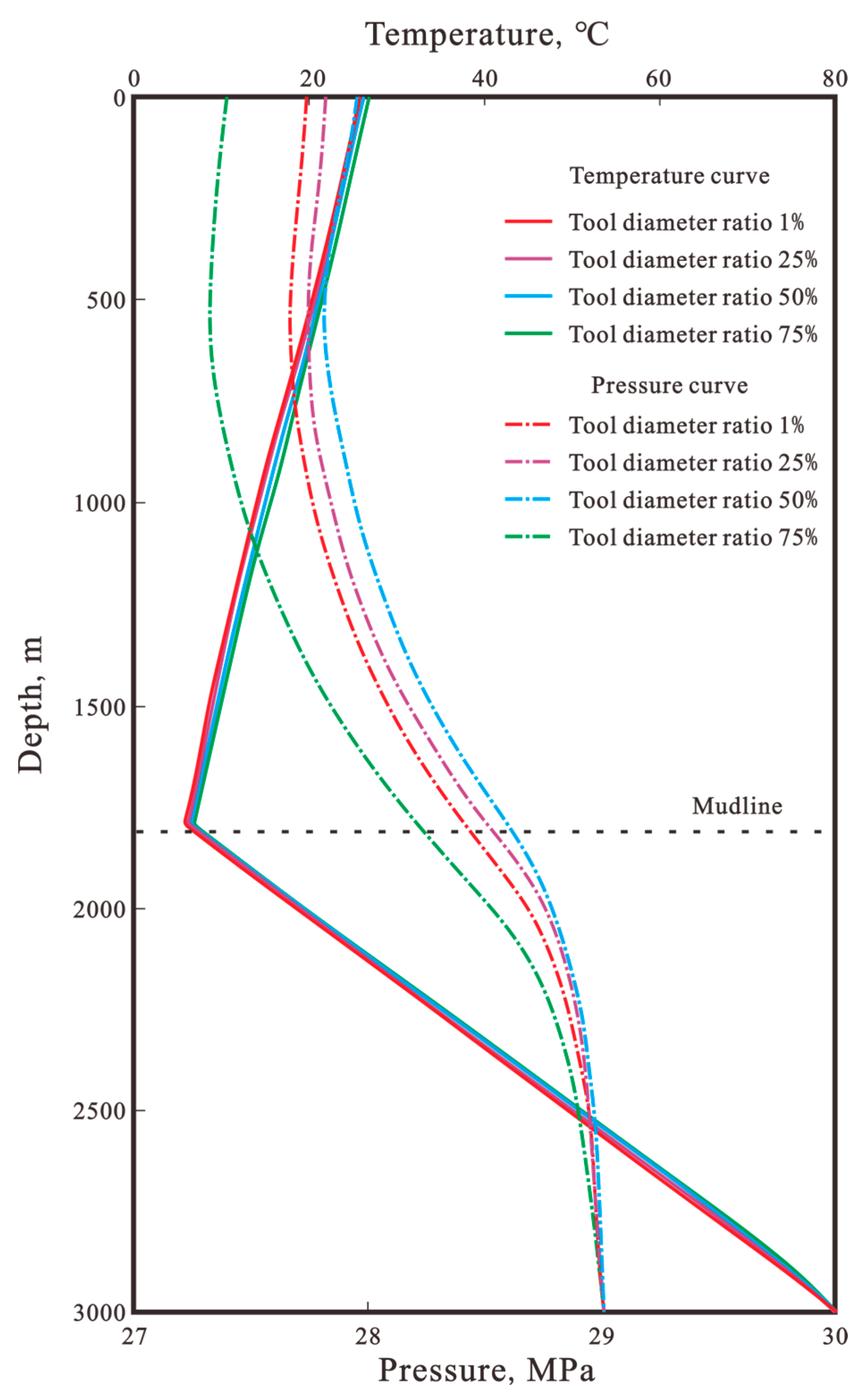
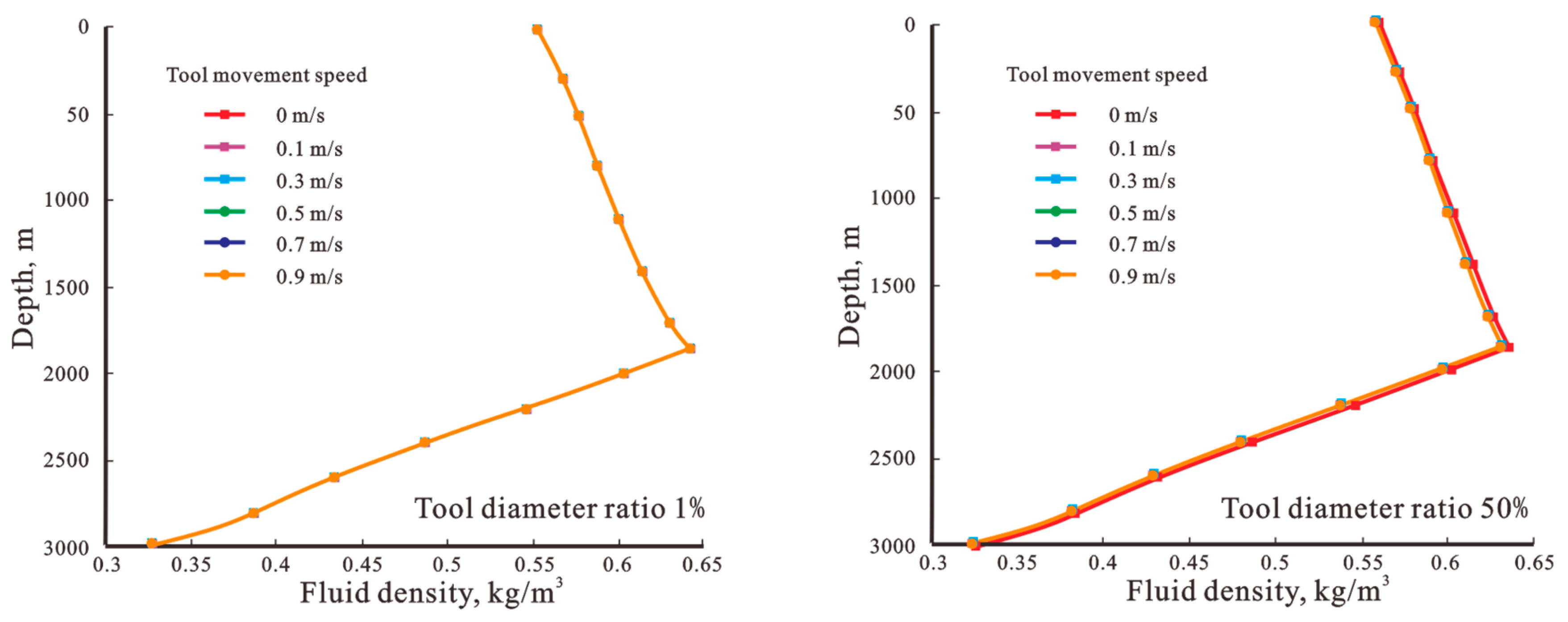
3.1. Evaluation of Tool Effects on Wellbore Fluid Density
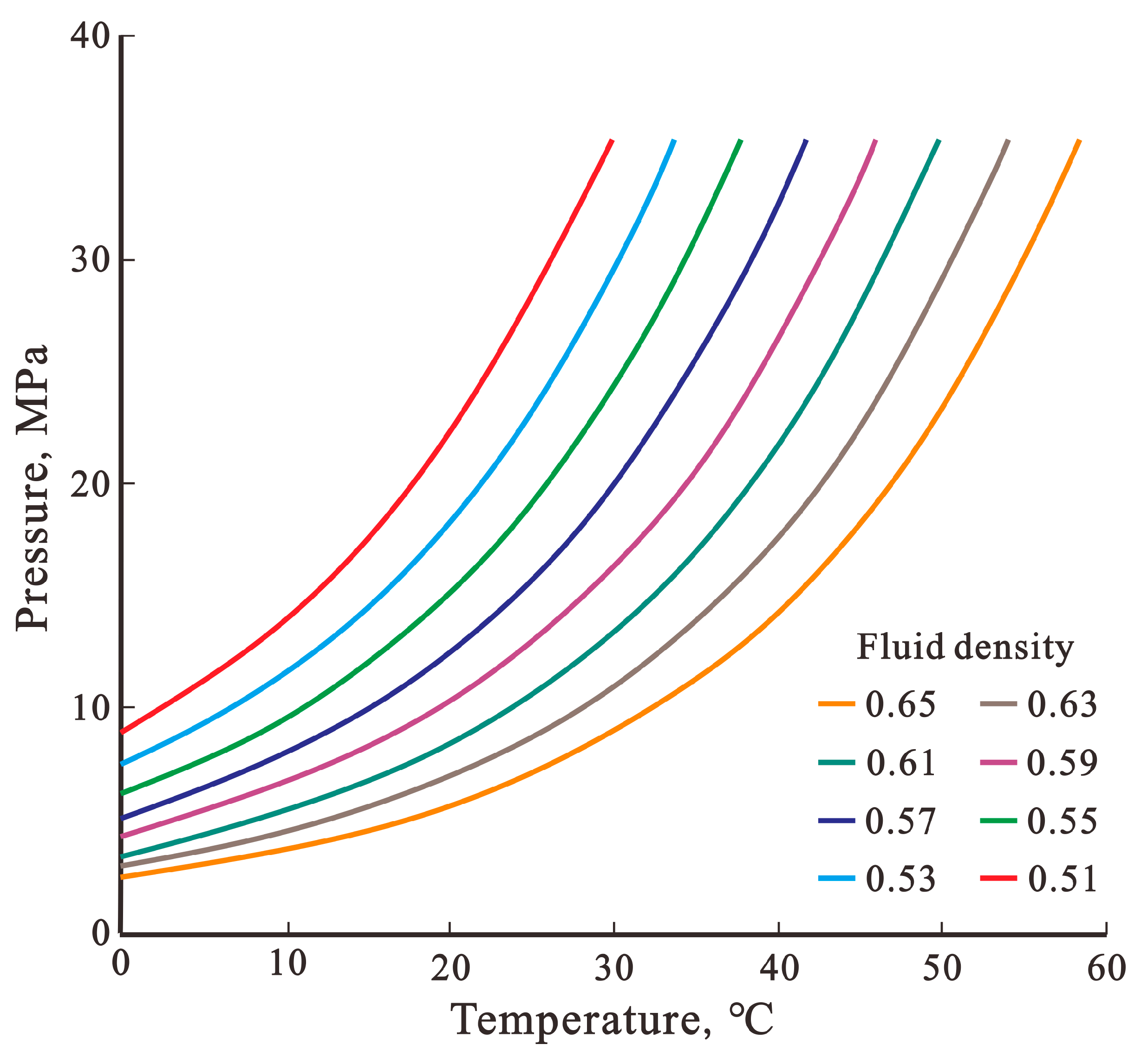
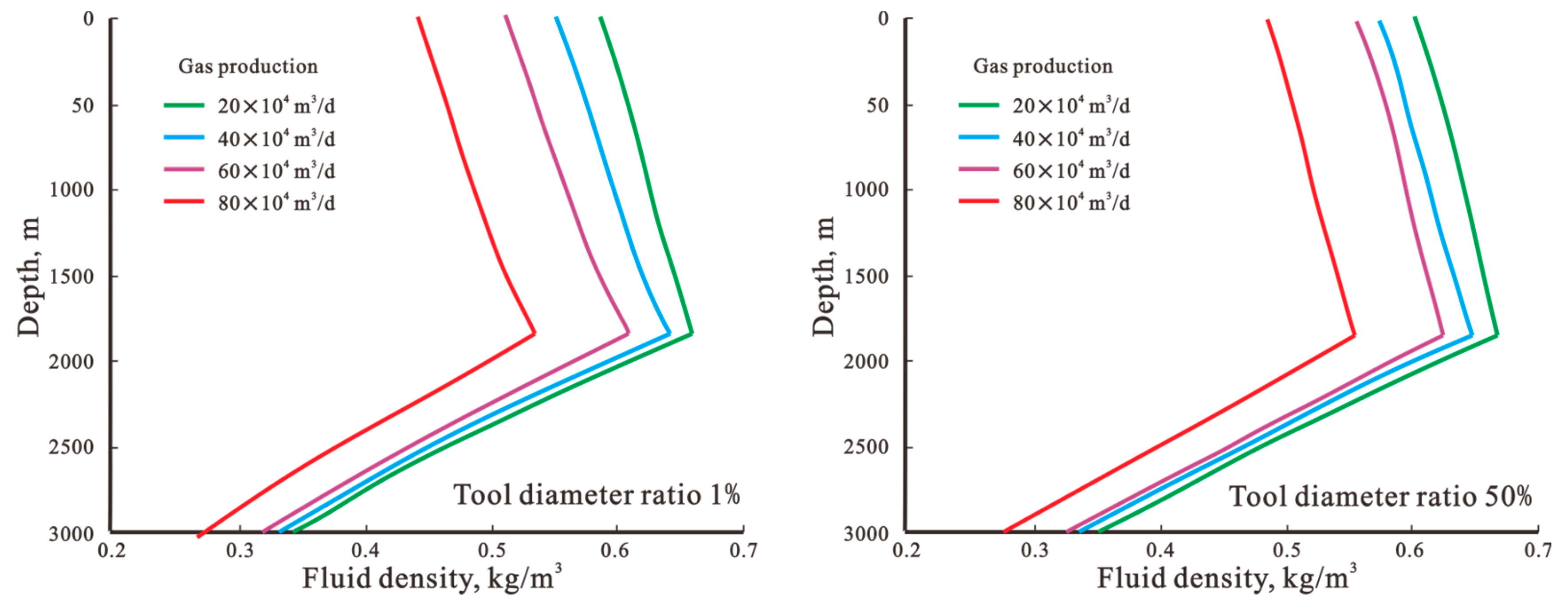
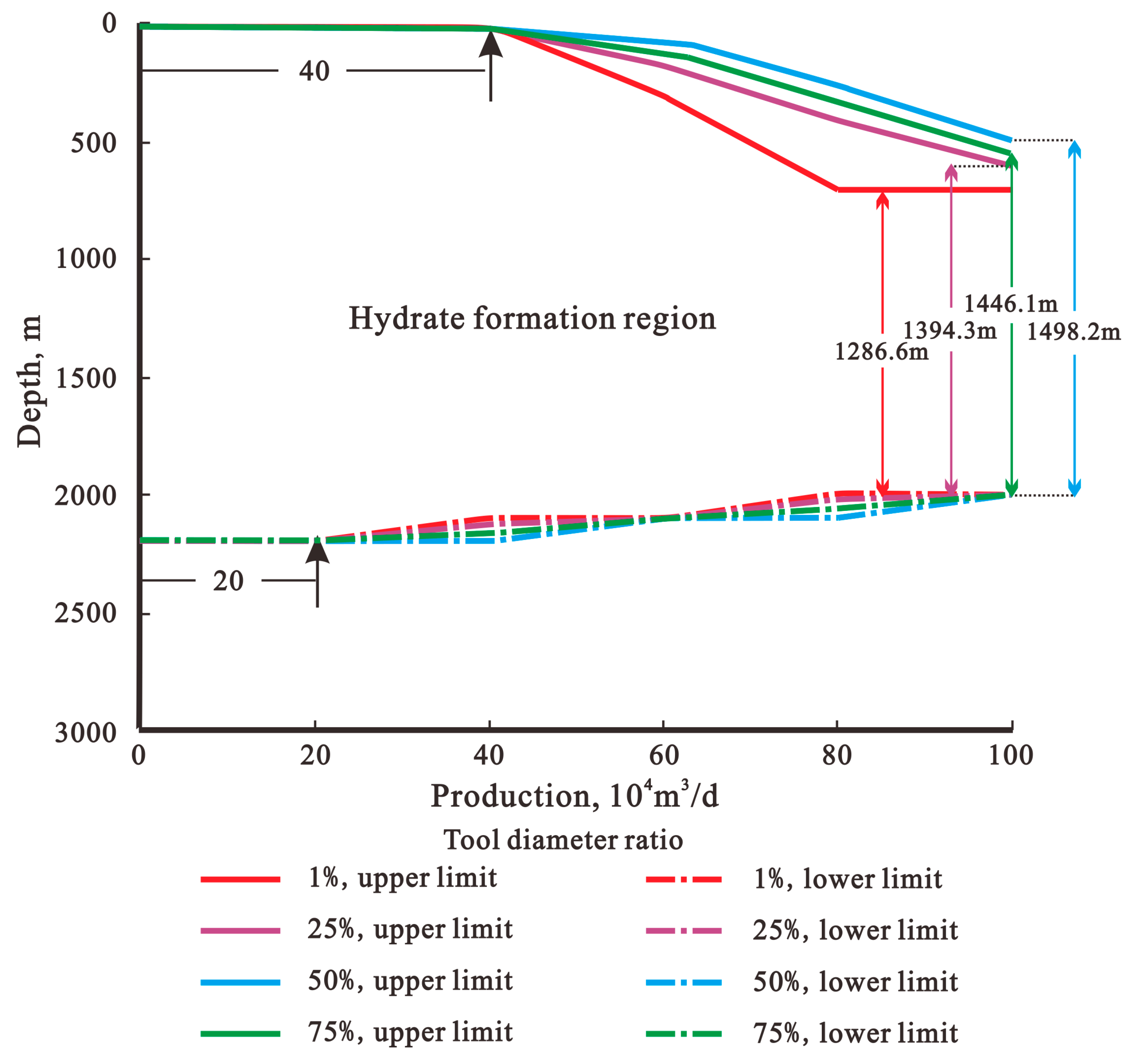
3.2. Regional Forecast of Wellbore Hydrate Generation Risks
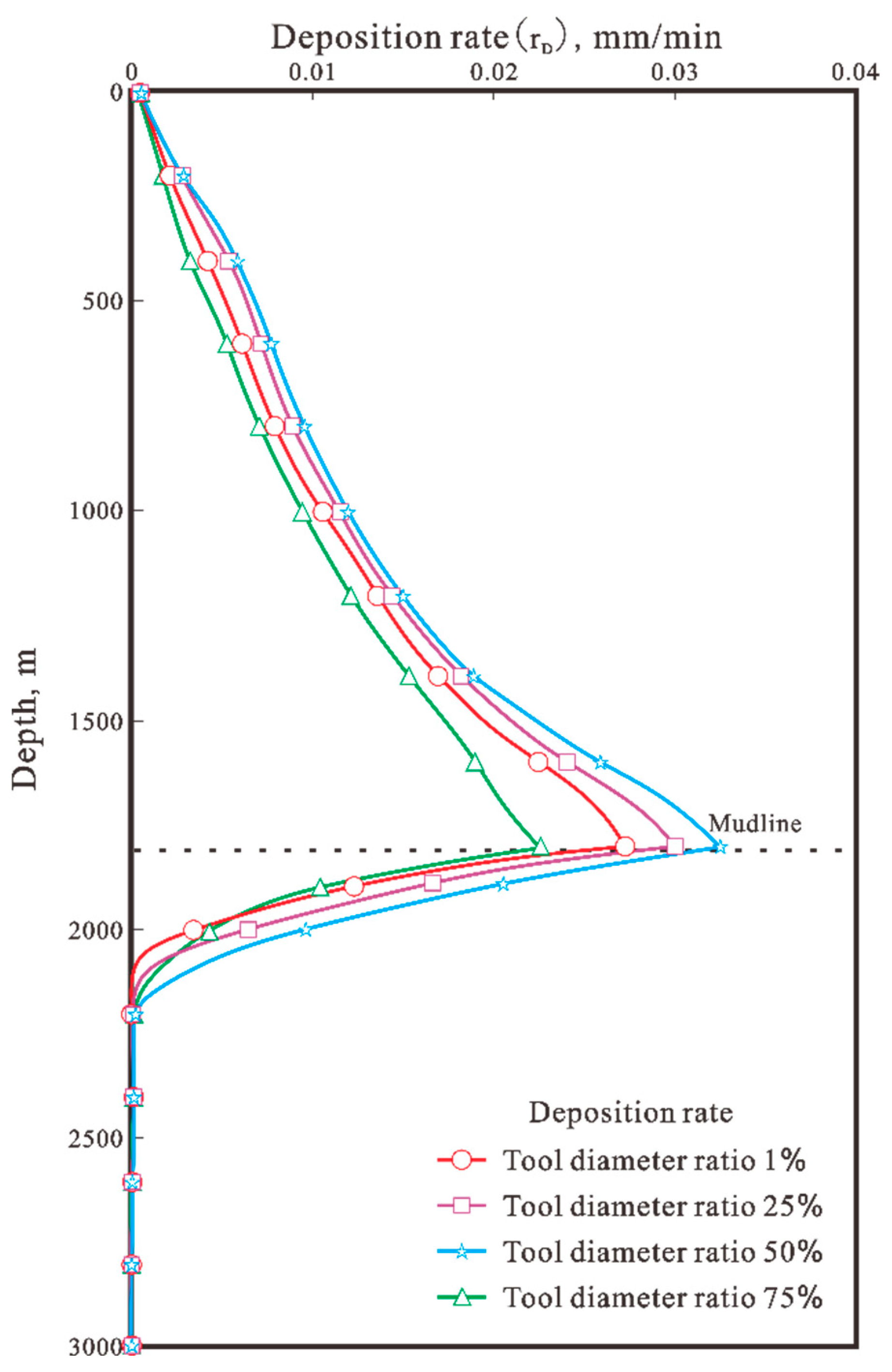
3.3. Prediction of Wellbore Hydrate Generation Rate
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Li, Q.; Li, Q.; Cao, H.; Wu, J.; Wang, F.; Wang, Y. The Crack Propagation Behaviour of CO2 Fracturing Fluid in Unconventional Low Permeability Reservoirs: Factor Analysis and Mechanism Revelation. Processes 2025, 13, 159. [Google Scholar] [CrossRef]
- Aman, Z.M.; Di Lorenzo, M.; Kozielski, K.; Koh, C.A.; Warrier, P.; Johns, M.L.; May, E.F. Hydrate formation and deposition in a gas-dominant flowloop: Initial studies of the effect of velocity and subcooling. J. Nat. Gas Sci. Eng. 2016, 35, 1490–1498. [Google Scholar]
- Andreussi, P.; Asali, J.C.; Hanratty, T.J. Initiation of roll waves in gas-liquid flows. AIChE J. 1985, 31, 119–126. [Google Scholar]
- Arrieta, V.V.; Torralba, A.O.; Hernandez, P.C.; García, E.R.; Maia, C.T.; Guajardo, M. Case History: Lessons Learned from Retrieval of Coiled Tubing Stuck by Massive Hydrate Plug When Well Testing in an Ultradeepwater Gas Well in Mexico. SPE Prod. Oper. 2011, 26, 337–342. [Google Scholar]
- Standing, M.B. Volumetric and Phase Behavior of Oil Field Hydrocarbon Systems; Society of Petroleum Engineers: Dallas, TX, USA, 1977; pp. 121–122. [Google Scholar]
- Bondarev, É.A.; Gabysheva, L.N.; Kanibolotskii, M.A. Simulation of the formation of hydrates during gas flow in tubes. Fluid Dyn. 1982, 17, 740–746. [Google Scholar]
- Bozorgian, A. Methods of Predicting Hydrates Formation. Adv. J. Sci. Eng. 2020, 1, 34–39. [Google Scholar]
- Di Lorenzo, M.; Aman, Z.M.; Kozielski, K.; Norris, B.W.E.; Johns, M.L.; May, E.F. Underinhibited Hydrate Formation and Transport Investigated Using a Single-Pass Gas-Dominant Flowloop. Energy Fuels 2014, 28, 7274–7284. [Google Scholar]
- Englezos, P.; Kalogerakis, N.; Dholabhai, P.D.; Bishnoi, P.R. Kinetics of formation of methane and ethane gas hydrates. Chem. Eng. Sci. 1987, 42, 2647–2658. [Google Scholar]
- Hall, K.; Yarborough, L. A new equation of state for Z-factor calculations. Oil Gas J. 1973, 71, 82–92. [Google Scholar]
- Li, J.; Gao, Y.; Zheng, Q.; Sun, B.; Li, G. Hydrate formation prediction in deepwater gas well testing. Oil Drill. Prod. Technol. 2012, 34, 77–80. (In Chinese) [Google Scholar]
- Li, X.-S.; Liu, Y.; Zeng, Z.-Y.; Chen, Z.; Li, G.; Wu, H.-J. Equilibrium Hydrate Formation Conditions for the Mixtures of Methane + Ionic Liquids + Water. J. Chem. Eng. Data 2010, 56, 119–123. [Google Scholar]
- Li, Z.; Xie, R.; Wu, Y.; Yuan, J. Progress and prospect of CNOOC’s oil and gas well drilling and completion technologies. Nat. Gas Ind. B 2022, 9, 209–217. [Google Scholar]
- Liu, W.; Hu, J.; Li, X.; Sun, F.; Sun, Z.; Zhou, Y. Research on evaluation method of wellbore hydrate blocking degree during deepwater gas well testing. J. Nat. Gas Sci. Eng. 2018, 59, 168–182. [Google Scholar] [CrossRef]
- Maekawa, T. Equilibrium conditions for gas hydrates of methane and ethane mixtures in pure water and sodium chloride solution. Geochem. J. 2001, 35, 59–66. [Google Scholar]
- Munck, J.; Skjold-Jørgensen, S.; Rasmussen, P. Computations of the formation of gas hydrates. Chem. Eng. Sci. 1988, 43, 2661–2672. [Google Scholar] [CrossRef]
- Sloan, E.D.; Koh, C.A. Clathrate Hydrates of Natural Gases, 3rd ed.; CRC Press: Boca Raton, FL, USA, 2007. [Google Scholar]
- Sloan, E.D. A changing hydrate paradigm—From apprehension to avoidance to risk management. Fluid Phase Equilibria 2005, 228–229, 67–74. [Google Scholar] [CrossRef]
- Sohn, Y.H.; Kim, J.; Shin, K.; Chang, D.; Seo, Y.; Aman, Z.M.; May, E.F. Hydrate plug formation risk with varying watercut and inhibitor concentrations. Chem. Eng. Sci. 2015, 126, 711–718. [Google Scholar]
- Suo, Y.; Dong, M.; Fu, X.; He, W.; Pan, Z. Experimental and numerical simulation research on hot dry rock wellbore stability under different cooling methods. Geothermics 2024, 119, 102977. [Google Scholar]
- Suo, Y.; Guan, W.; Dong, M.; Zhang, R.; Wang, K.; He, W.; Fu, X.; Pan, Z.; Guo, B. Study on the heat extraction patterns of fractured hot dry rock reservoirs. Appl. Therm. Eng. 2025, 262, 125286. [Google Scholar]
- Swinkels, W.J.A.M.; Drenth, R.J.J. Thermal Reservoir Simulation Model of Production From Naturally Occurring Gas Hydrate Accumulations. SPE Reserv. Eval. Eng. 2000, 3, 559–566. [Google Scholar]
- Turner, D.; Boxall, J.; Yang, S.; Kleehamer, D.; Koh, C.; Miller, K.; Sloan, E.D.; Xu, Z.; Matthews, P.; Talley, L. Development of a hydrate kinetic model and its incorporation into the OLGA2000-R transient multi-phase flow simulator. In Proceedings of the 5th International Conference on Gas Hydrates, Trondheim, Norway, 13–16 June 2005. [Google Scholar]
- Vysniauskas, A.; Bishnoi, P.R. A kinetic study of methane hydrate formation. Chem. Eng. Sci. 1983, 38, 1061–1072. [Google Scholar] [CrossRef]
- van der Waals, J.H.; Platteeuw, J.C. Clathrate Solutions. In Advances in Chemical Physics; Wiley: Hoboken, NJ, USA, 2007. [Google Scholar]
- Wang, Z.-Y.; Sun, B.-J.; Wang, X.-R.; Zhang, Z.-N. Prediction of natural gas hydrate formation region in wellbore during deep-water gas well testing. J. Hydrodyn. 2014, 26, 568–576. [Google Scholar] [CrossRef]
- Amin, J.S.; Bahadori, A.; Mohamadi, E.; Nia, B.H. Predicting natural gas hydrate formation temperature using Le-venberg-Marquardt algorithm. Pet. Sci. Technol. 2015, 33, 1038–1044. [Google Scholar] [CrossRef]
- Wang, Z.; Zhang, J.; Sun, B.; Chen, L.; Zhao, Y.; Fu, W. A new hydrate deposition prediction model for gas-dominated systems with free water. Chem. Eng. Sci. 2017, 163, 145–154. [Google Scholar] [CrossRef]
- Wang, Z.; Zhao, Y.; Sun, B.; Chen, L.; Zhang, J.; Wang, X. Modeling of Hydrate Blockage in Gas-Dominated Systems. Energy Fuels 2016, 30, 4653–4666. [Google Scholar] [CrossRef]
- Wang, Z.; Zhang, J.; Meng, W.; Sun, B.; Sun, J.; Wang, J.; Liu, D.; Wang, J. Formation, deposition characteristics and prevention methods of gas hydrates in deepwater gas wells. Acta Pet. Sin. 2021, 42, 776–790. (In Chinese) [Google Scholar]
- Zhang, Z.; Wang, J.; Li, Y.; Luo, M.; Zhang, C. Effects of instantaneous shut-in of high production gas well on fluid flow in tubing. Pet. Explor. Dev. 2020, 47, 642–650. [Google Scholar] [CrossRef]
| Ratio | (Be) Worth | Ratio | (Be) Worth |
|---|---|---|---|
| 5.3526 | −4.1947 | ||
| −5958.7076 | 21,106.237 | ||
| 1,542,927.6147 | −5,517,114.0404 | ||
| −147,104,751.5603 | 530,933,571.0111 | ||
| 5.859 | −2.6727 | ||
| −25,902.5287 | 10,642.7597 | ||
| 6,652,150.1513 | −2,662,933.0034 | ||
| -638,982,309.0002 | 254,335,889.8405 |
| Parametric | Numerical Value | Parametric | Numerical Value |
|---|---|---|---|
| Temperature of the formation at the bottom of the well, °C | 84.83 | Outer diameter of oil pipe, m | 0.1143 |
| Total heat transfer coefficient of seawater, W/(m2·°C) | 18 | Borehole size, m | 0.62 |
| Total stratigraphic heat transfer coefficient, W/(m2·°C) | 15 | Inner diameter of casing, m | 0.52 |
| Ground thermal conductivity, W/(m·°C) | 2.312 | Casing outer diameter, m | 0.54 |
| Stratigraphic heat diffusion coefficient, m2/s | 0.00005 | Thermal conductivity of oil pipe, W/(m·°C) | 40 |
| Well depth, m | 3000 | Thermal conductivity of casing, W/(m·°C) | 35 |
| Ground temperature gradient, °C/100 m | 2.55 | Thermal conductivity of cement ring, W/(m·°C) | 0.8 |
| Stratigraphic heat capacity, J/(kg·°C) | 1700 | Depth of sea water, m | 1800 |
| Relative density of gases | 0.684 | Gas viscosity, mPa-s | 0.033 |
| Ground pressure, MPa | 29.473 | Gas compression factor | 0.0056 |
| Ground density, kg/m3 | 2400 | gas volume factor | 0.0025 |
| Inner diameter of oil pipe, m | 0.086 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, D.; Gan, B.; Li, Y.; Li, Z.; Zhang, H.; Yu, F. The Control Effect of Equipment Operation on the Formation of Wellbore Hydrates Under the Production Conditions of Deepwater Gas Wells. Processes 2025, 13, 1028. https://doi.org/10.3390/pr13041028
Wang D, Gan B, Li Y, Li Z, Zhang H, Yu F. The Control Effect of Equipment Operation on the Formation of Wellbore Hydrates Under the Production Conditions of Deepwater Gas Wells. Processes. 2025; 13(4):1028. https://doi.org/10.3390/pr13041028
Chicago/Turabian StyleWang, Dianju, Bicheng Gan, Yihe Li, Zhandong Li, Haixiang Zhang, and Fahao Yu. 2025. "The Control Effect of Equipment Operation on the Formation of Wellbore Hydrates Under the Production Conditions of Deepwater Gas Wells" Processes 13, no. 4: 1028. https://doi.org/10.3390/pr13041028
APA StyleWang, D., Gan, B., Li, Y., Li, Z., Zhang, H., & Yu, F. (2025). The Control Effect of Equipment Operation on the Formation of Wellbore Hydrates Under the Production Conditions of Deepwater Gas Wells. Processes, 13(4), 1028. https://doi.org/10.3390/pr13041028





