Thermodynamic Simulations of a Single-Stage Absorption Heat Transformer Utilizing Low-Temperature Waste Heat (60 °C)
Abstract
1. Introduction
2. Materials and Methods
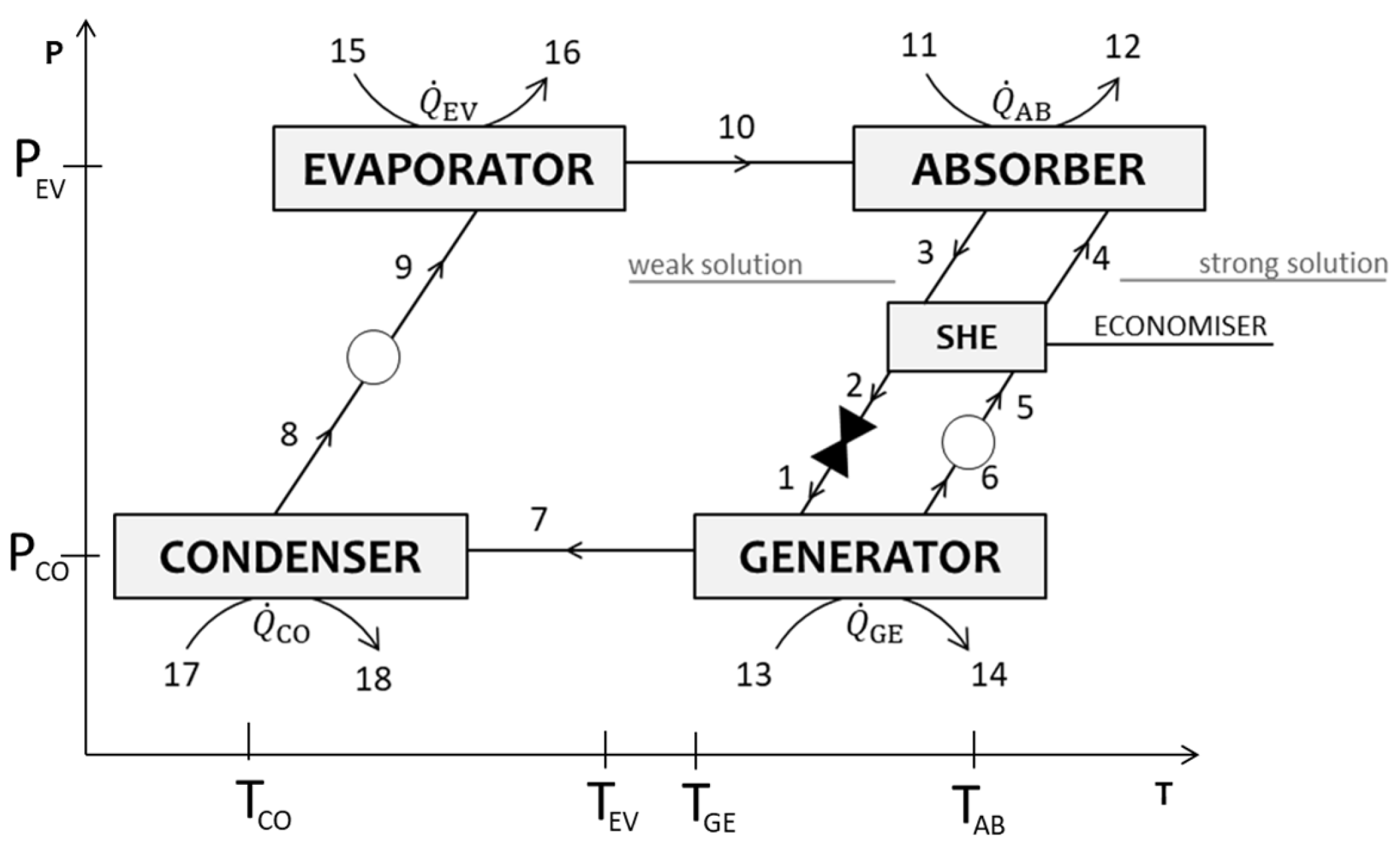
2.1. Single-Stage Absorption Heat Transformers
2.2. Thermodynamic Model
- The system is in a steady state;
- The refrigerant vapor/liquid leaving the evaporator/condenser is saturated;
- The expansion valve is adiabatic, and the pumps are isentropic;
- The generator and condenser are at a lower pressure, while the evaporator and absorber are at a higher pressure;
- There are no heat losses to the environment.
3. Results and Discussion
3.1. Base Model Performance
3.2. Sensitivity Analysis
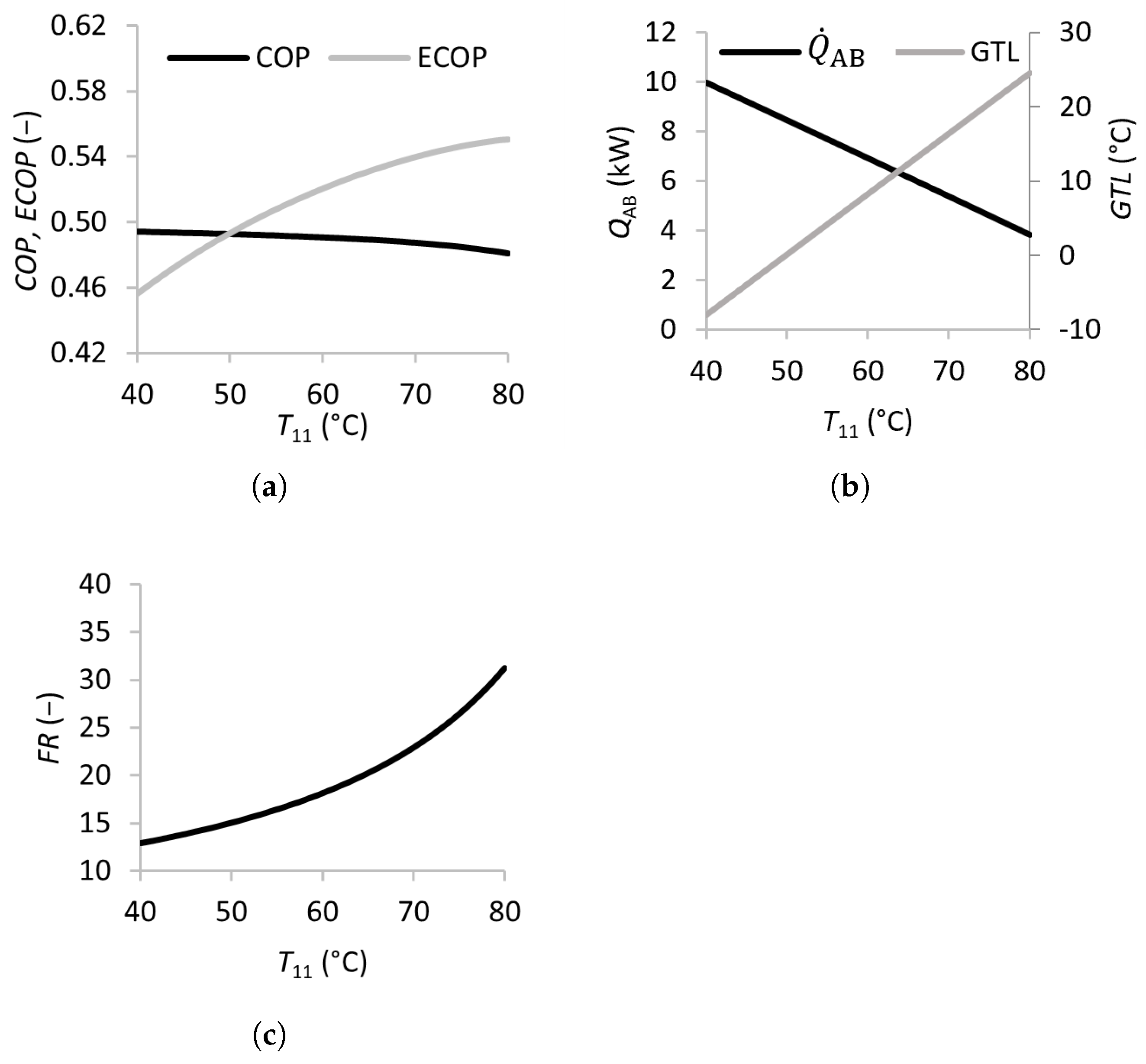
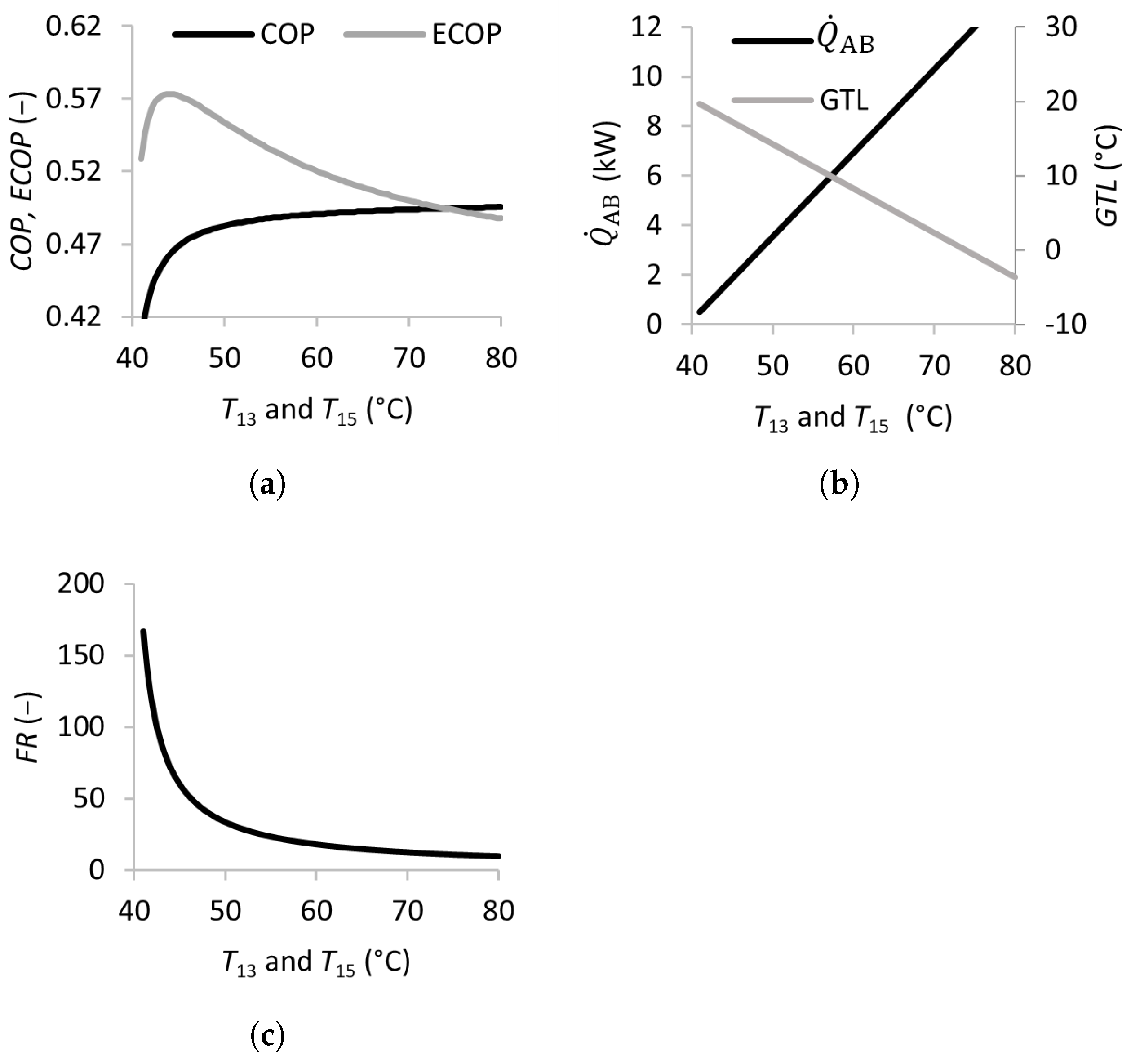
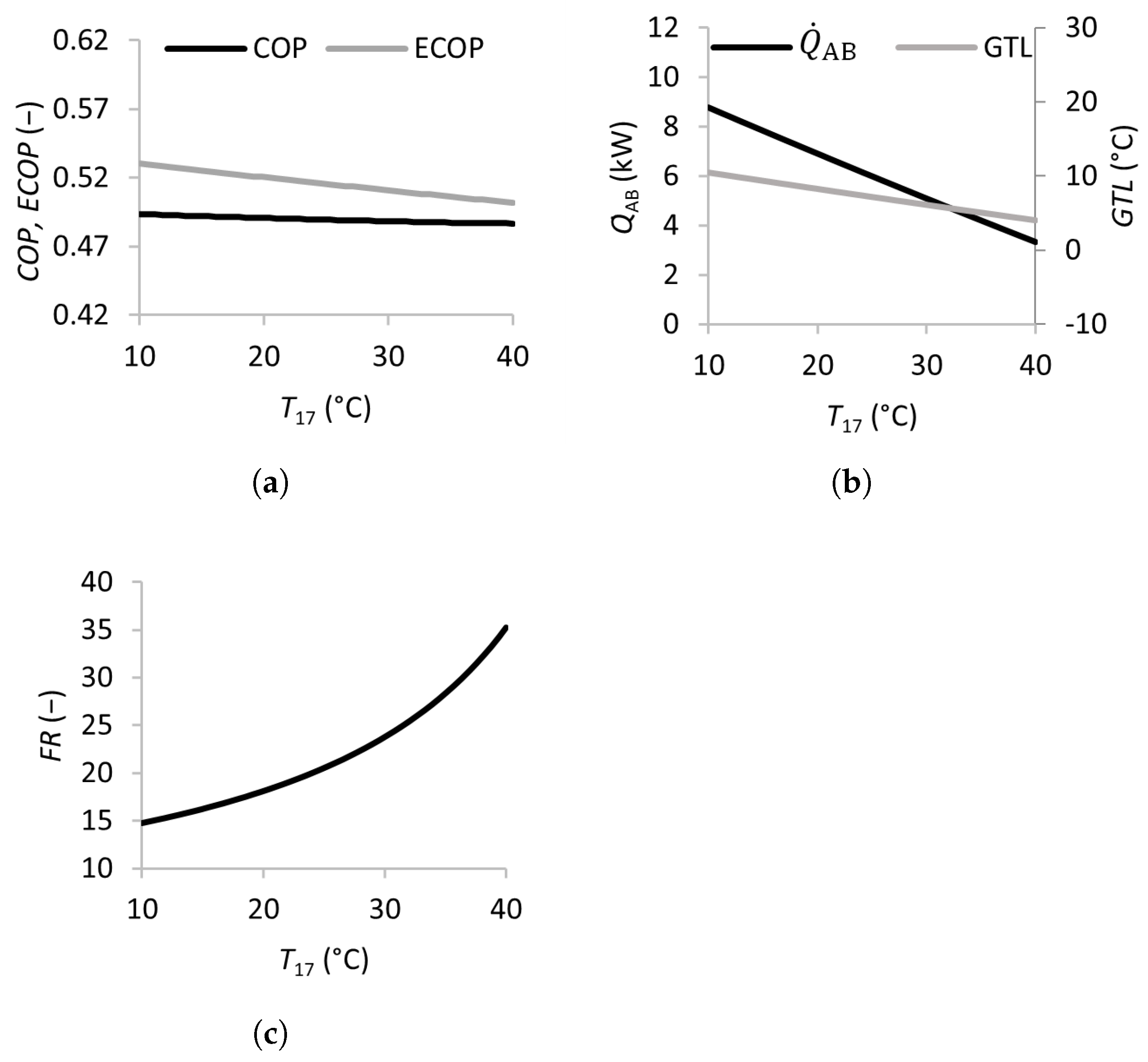
3.2.1. Effects of Inlet Temperatures
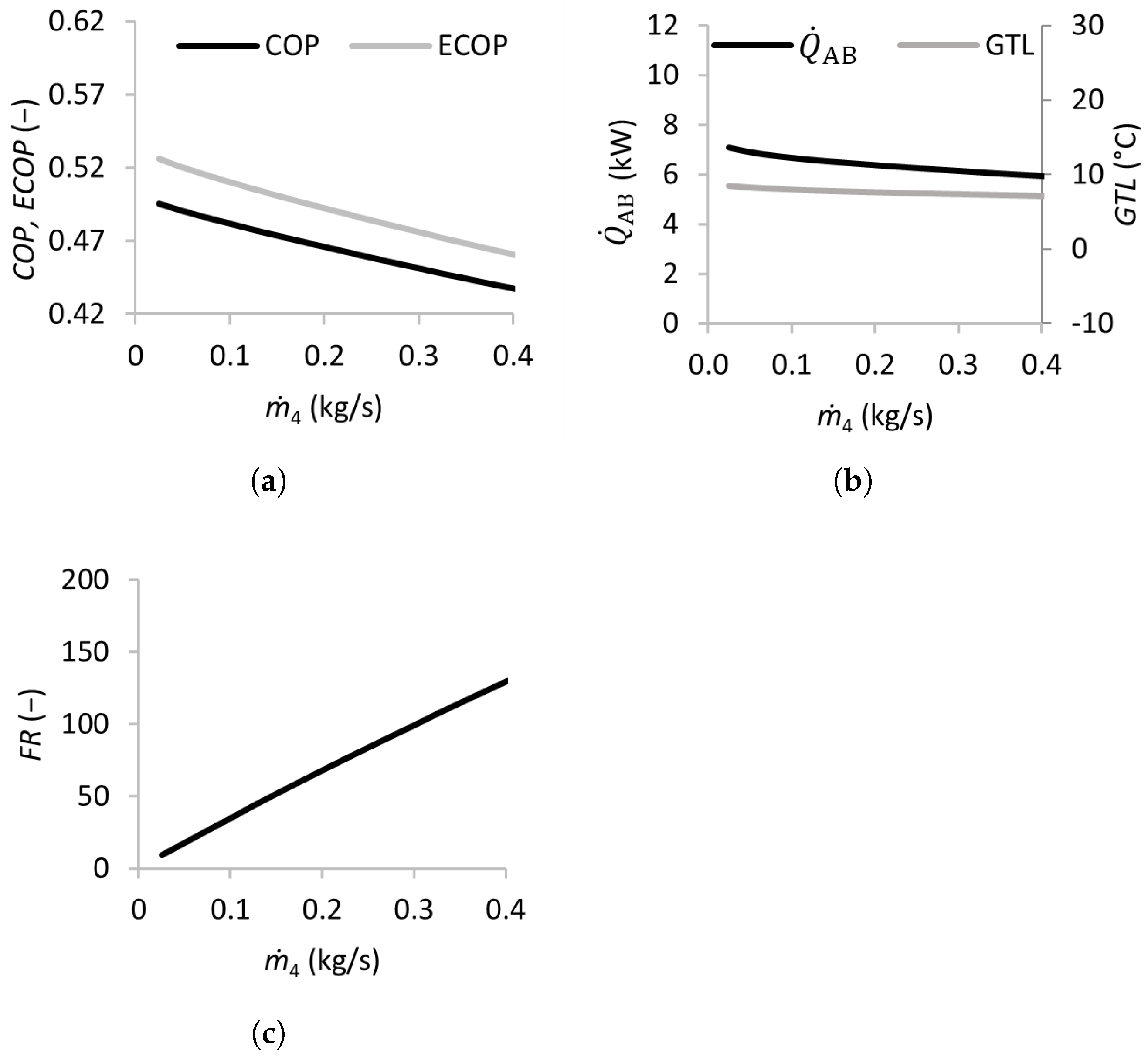
3.2.2. Effects of the Strong Solution Mass Flow Rate
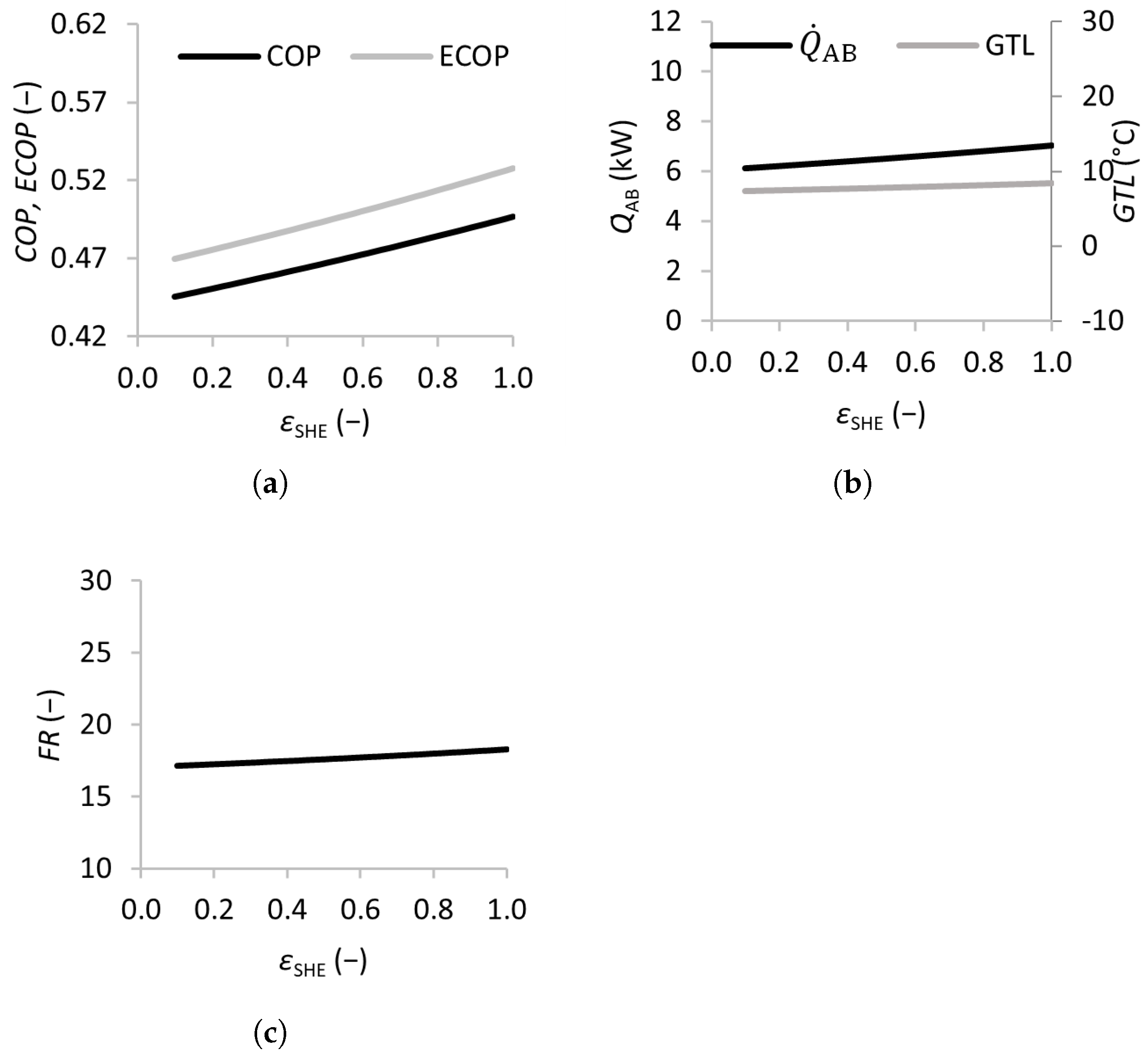
3.2.3. Heat Exchanger Effectiveness
3.3. Improved SSAHT Results
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| A | Area (m2) |
| AHT | Absorption heat transformer |
| c | Specific heat (J/(kg °C)) |
| Coefficient of performance (−) | |
| Exergetic coefficient (−) | |
| Correction factor for (−) | |
| Flow ratio (−) | |
| Gross temperature lift (°C) | |
| H2O/LiBr | Water/lithium bromide |
| NH3/H2O | Ammonia/water |
| h | Specific enthalpy (J/kg) |
| m | Mass flow rate (kg/s) |
| P | Pressure (kPa) |
| Heat transfer rate (W) | |
| Q | Vapor quality (−) |
| SSAHT | Single-stage absorption heat transformer |
| T | Temperature (°C) |
| Reference temperature (°C) | |
| U | Overall heat transfer coefficient (W/(m2 °C)) |
| Pump power (W) | |
| x | Solution mass concentration (−) |
| Heat exchanger effectiveness (−) | |
| Temperature difference (°C) | |
| Mean temperature difference (°C) | |
| Logarithmic mean temperature difference (°C) | |
| Subscripts | |
| AB | Absorber |
| CO | Condenser |
| EV | Evaporator |
| GE | Generator |
| i | Stream number index (1–18) |
| in | Inlet |
| out | Outlet |
| SHE | Solution heat exchanger |
References
- Cudok, F.; Giannetti, N.; Ciganda, J.L.C.; Aoyama, J.; Babu, P.; Coronas, A.; Fujii, T.; Inoue, N.; Saito, K.; Yamaguchi, S.; et al. Absorption heat transformer—state-of-the-art of industrial applications. Renew. Sustain. Energy Rev. 2021, 141, 110757. [Google Scholar] [CrossRef]
- Parham, K.; Khamooshi, M.; Tematio, D.B.K.; Yari, M.; Atikol, U. Absorption heat transformers—A comprehensive review. Renew. Sustain. Energy Rev. 2014, 34, 430–452. [Google Scholar] [CrossRef]
- Huicochea, A.; Siqueiros, J.; Romero, R.J. Portable water purification system integrated to a heat transformer. Desalination 2004, 165, 385–391. [Google Scholar] [CrossRef]
- Huicochea, A.; Siqueiros, J. Improved efficiency of energy use of a heat transformer using a water purification system. Desalination 2010, 257, 8–15. [Google Scholar] [CrossRef]
- Garone, S.; Toppi, T.; Guerra, M.; Motta, M. A water-ammonia heat transformer to upgrade low-temperature waste heat. Appl. Therm. Eng. 2017, 127, 748–757. [Google Scholar] [CrossRef]
- Kurem, E.; Horuz, I. A comparison between ammonia-water and water-lithium bromide solutions in absorption heat transformers. Int. Comm. Heat Mass Transfer 2001, 28, 427–438. [Google Scholar] [CrossRef]
- Rivera, W.; Romero, R.J.; Cardoso, M.J.; Aguillón, J.; Best, R. Theoretical and experimental comparison of the performance of a single-stage heat transformer operating with water/lithium bromide and water/Carrol ™. Int. J. Energy Res. 2002, 26, 747–762. [Google Scholar] [CrossRef]
- Yin, J.; Shi, L.; Zhu, M.S.; Han, L.Z. Performance analysis of an absorption heat transformer with different working fluid combinations. Appl. Energy 2000, 67, 281–292. [Google Scholar] [CrossRef]
- Sözen, A.; Yücesu, H.S. Performance improvement of absorption heat transformer. Renew. Energy 2007, 32, 267–284. [Google Scholar] [CrossRef]
- Horuz, I.; Kurt, B. Absorption heat transformers and an industrial application. Renew. Energy 2010, 35, 2175–2181. [Google Scholar] [CrossRef]
- Zhang, X.; Hu, D. Performance analysis of the single-stage absorption heat transformer using a new working pair composed of ionic liquid and water. Appl. Therm. Eng. 2012, 37, 129–135. [Google Scholar] [CrossRef]
- Herold, K.E.; Radermacher, R.; Klein, S.A. Absorption Chillers and Heat Pumps, 2nd ed.; CRC Press: Boca Raton, FL, USA, 2016; p. 386. [Google Scholar]
- López-Pérez, L.A.; Torres-Díaz, T.; Pérez Grajales, S.G.; Flores Prieto, J.J.; Juárez Romero, D.; Hernández Pérez, J.A.; Huicochea, A. Solar Water Heating System with Absorption Heat Transformer for Annual Continuous Water Heating. Processes 2024, 12, 1650. [Google Scholar] [CrossRef]
- Rivera, W.; Cardoso, M.J.; Romero, R.J. Single-stage and advanced absorption heat transformers operating with lithium bromide mixtures used to increase solar pond’s temperature. Sol. Energy Mater. Sol. Cells 2001, 70, 321–333. [Google Scholar] [CrossRef]
- Ibarra-Bahena, J.; Dehesa-Carrasco, U.; Montiel-González, M.; Romero, R.J.; Venegas-Reyes, E. Feasibility analysis of a hot water solar system coupled to an absorption heat transformer. Appl. Therm. Eng. 2017, 114, 1176–1185. [Google Scholar] [CrossRef]
- Siqueiros, J.; Romero, R.J. Increase of COP for heat transformer in water purification systems. Part I-Increasing heat source temperature. Appl. Therm. Eng. 2007, 27, 1043–1053. [Google Scholar] [CrossRef]
- ASHRAE. Ashrae ® Handbook-Fundamentals; ASHRAE: Atlanta, GA, USA, 2009; Volume 30329. [Google Scholar]
- Çengel, Y.A. Heat Transfer: A Practical Approach, 2nd ed.; Mcgraw-Hill: New York, NY, USA, 2002. [Google Scholar]
- Ibarra-Bahena, J.; Romero, R.J.; Velazquez-Avelar, L.; Valdez-Morales, C.V.; Galindo-Luna, Y.R. Experimental thermodynamic evaluation for a single stage heat transformer prototype build with commercial PHEs. Appl. Therm. Eng. 2015, 75, 1262–1270. [Google Scholar] [CrossRef]
- Romero, R.J.; Martínez, A.R.; Silva, S.; Cerezo, J.; Rivera, W. Comparison of double stage heat transformer with double absorption heat transformer operating with Carrol—Water for industrial waste heat recovery. Chem. Eng. Trans. 2011, 25, 129–134. [Google Scholar]
- Rivera, W.; Martínez, H.; Cerezo, J.; Romero, R.J.; Cardoso, M.J. Exergy analysis of an experimental single-stage heat transformer operating with single water/lithium bromide and using additives (1-octanol and 2-ethyl-1-hexanol). Appl. Therm. Eng. 2011, 31, 3525–3532. [Google Scholar] [CrossRef]
| State Point | (kJ/kg) | (kg/s) | (kPa) | (−) | (°C) | (kg/kg) |
|---|---|---|---|---|---|---|
| 1 | 120.7 | 0.053 | 4.840 | 0.006 | 50.74 | 0.4574 |
| 2 | 120.7 | 0.053 | 12.187 | 56.82 | 0.4574 | |
| 3 | 150.5 | 0.053 | 12.187 | 0.000 | 69.57 | 0.4574 |
| 4 | 146.4 | 0.050 | 12.187 | 68.02 | 0.4841 | |
| 5 | 114.8 | 0.050 | 12.187 | 54.03 | 0.4841 | |
| 6 | 114.8 | 0.050 | 4.840 | 0.000 | 54.03 | 0.4841 |
| 7 | 2594.8 | 0.003 | 4.840 | 50.74 | 0.0000 | |
| 8 | 135.3 | 0.003 | 4.840 | 0.000 | 32.30 | 0.0000 |
| 9 | 135.3 | 0.003 | 12.187 | 32.30 | 0.0000 | |
| 10 | 2590.8 | 0.003 | 12.187 | 1.000 | 49.73 | 0.0000 |
| 11 | 0.200 | 60.00 | ||||
| 12 | 68.26 | |||||
| 13 | 0.200 | 60.00 | ||||
| 14 | 51.73 | |||||
| 15 | 0.200 | 60.00 | ||||
| 16 | 51.44 | |||||
| 17 | 0.200 | 20.00 | ||||
| 18 | 28.57 | |||||
| (−) | 0.491 | (kW) | 6.9 | |||
| (−) | 0.581 | (kW) | 6.9 | |||
| (°C) | 19.84 | (kW) | 7.2 | |||
| (−) | 18.14 | (kW) | 7.2 | |||
| (W) | 0.022/0.25 | (kW) | 1.6 | |||
| State Point | (kJ/kg) | (kg/s) | (kPa) | (−) | (°C) | (kg/kg) |
|---|---|---|---|---|---|---|
| 1 | 126.8 | 0.028 | 4.243 | 0.010 | 48.96 | 0.4614 |
| 2 | 126.8 | 0.028 | 12.363 | 59.50 | 0.4614 | |
| 3 | 152.3 | 0.028 | 12.363 | 0.000 | 70.43 | 0.4614 |
| 4 | 150.4 | 0.025 | 12.363 | 68.98 | 0.5135 | |
| 5 | 122.1 | 0.025 | 12.363 | 55.95 | 0.5135 | |
| 6 | 122.1 | 0.025 | 4.243 | 0.000 | 55.95 | 0.5135 |
| 7 | 2591.6 | 0.003 | 4.243 | 48.96 | 0.0000 | |
| 8 | 125.7 | 0.003 | 4.243 | 0.000 | 29.98 | 0.0000 |
| 9 | 125.7 | 0.003 | 12.363 | 29.99 | 0.0000 | |
| 10 | 2591.3 | 0.003 | 12.363 | 1.000 | 50.02 | 0.0000 |
| 11 | 0.100 | 60.00 | ||||
| 12 | 76.35 | |||||
| 13 | 0.200 | 60.00 | ||||
| 14 | 51.83 | |||||
| 15 | 0.200 | 60.00 | ||||
| 16 | 51.68 | |||||
| 17 | 0.200 | 20.00 | ||||
| 18 | 28.32 | |||||
| (−) | 0.496 | (kW) | 6.9 | |||
| (−) | 0.596 | (kW) | 6.9 | |||
| (°C) | 20.41 | (kW) | 7.0 | |||
| (−) | 9.851 | (kW) | 7.0 | |||
| (W) | 0.023/0.13 | (kW) | 0.7 | |||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Santos, M.; Pinho, C.; Campos, J.B.L.M. Thermodynamic Simulations of a Single-Stage Absorption Heat Transformer Utilizing Low-Temperature Waste Heat (60 °C). Processes 2025, 13, 1001. https://doi.org/10.3390/pr13041001
Santos M, Pinho C, Campos JBLM. Thermodynamic Simulations of a Single-Stage Absorption Heat Transformer Utilizing Low-Temperature Waste Heat (60 °C). Processes. 2025; 13(4):1001. https://doi.org/10.3390/pr13041001
Chicago/Turabian StyleSantos, Marta, Carlos Pinho, and João B. L. M. Campos. 2025. "Thermodynamic Simulations of a Single-Stage Absorption Heat Transformer Utilizing Low-Temperature Waste Heat (60 °C)" Processes 13, no. 4: 1001. https://doi.org/10.3390/pr13041001
APA StyleSantos, M., Pinho, C., & Campos, J. B. L. M. (2025). Thermodynamic Simulations of a Single-Stage Absorption Heat Transformer Utilizing Low-Temperature Waste Heat (60 °C). Processes, 13(4), 1001. https://doi.org/10.3390/pr13041001







