A Study on the Failure Characteristics of Coal–Rock Structures with Different Bursting Liabilities
Abstract
1. Introduction
2. Experimental Procedure
2.1. Experimental Setup
2.2. Data Image Processing Method
3. Results
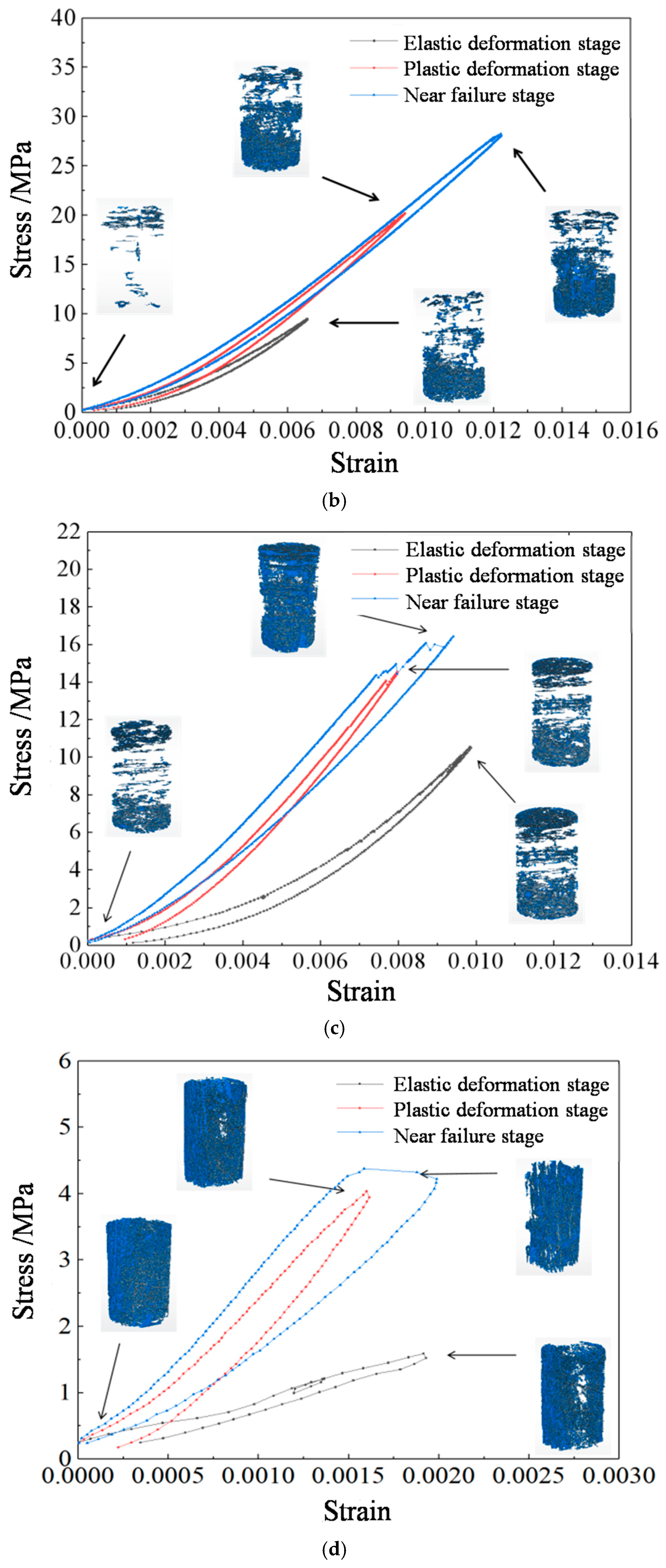
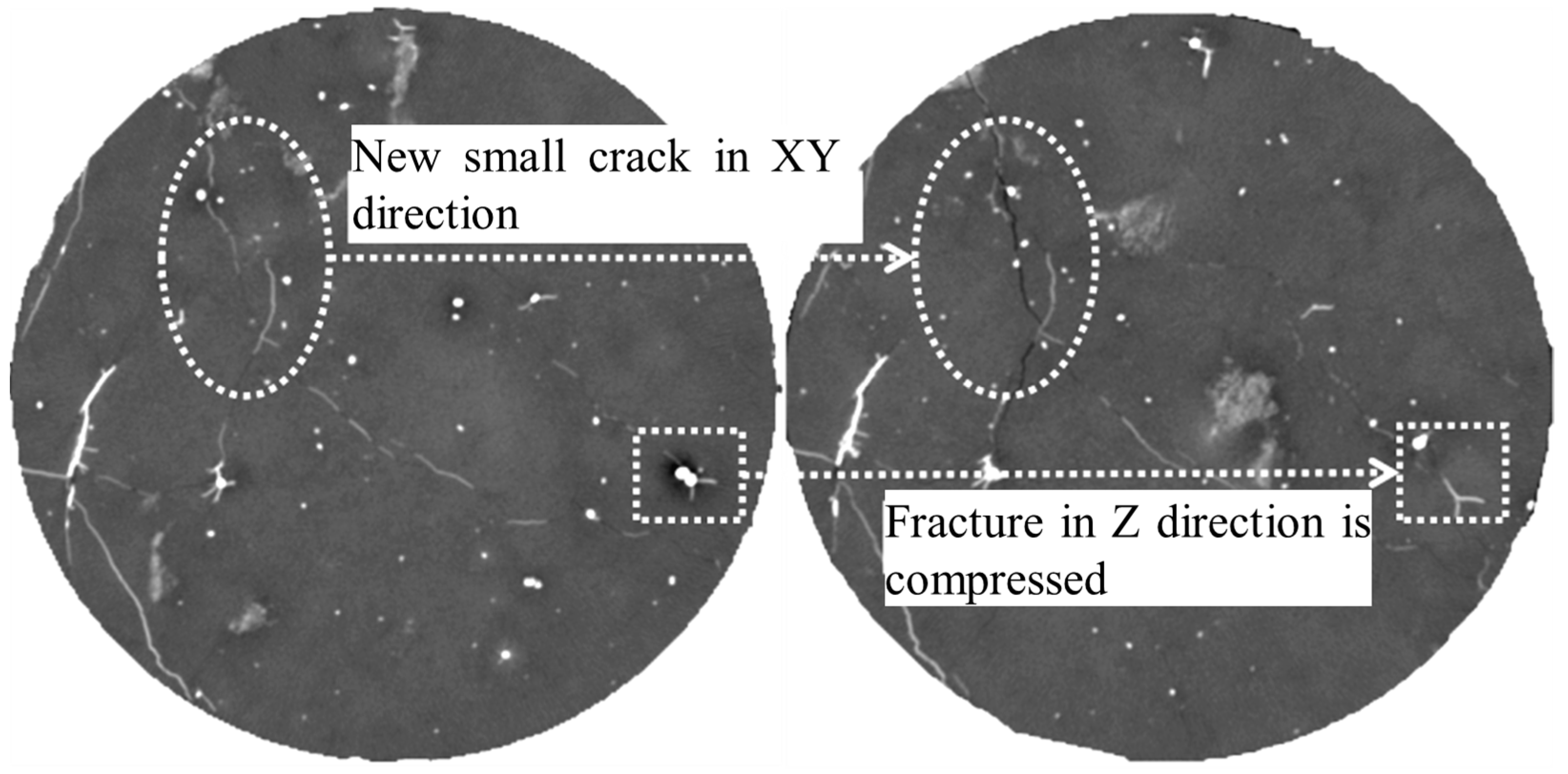
3.1. Evolution Process of Pre-Peak Deformation Fracture Structure of Coal and Rock
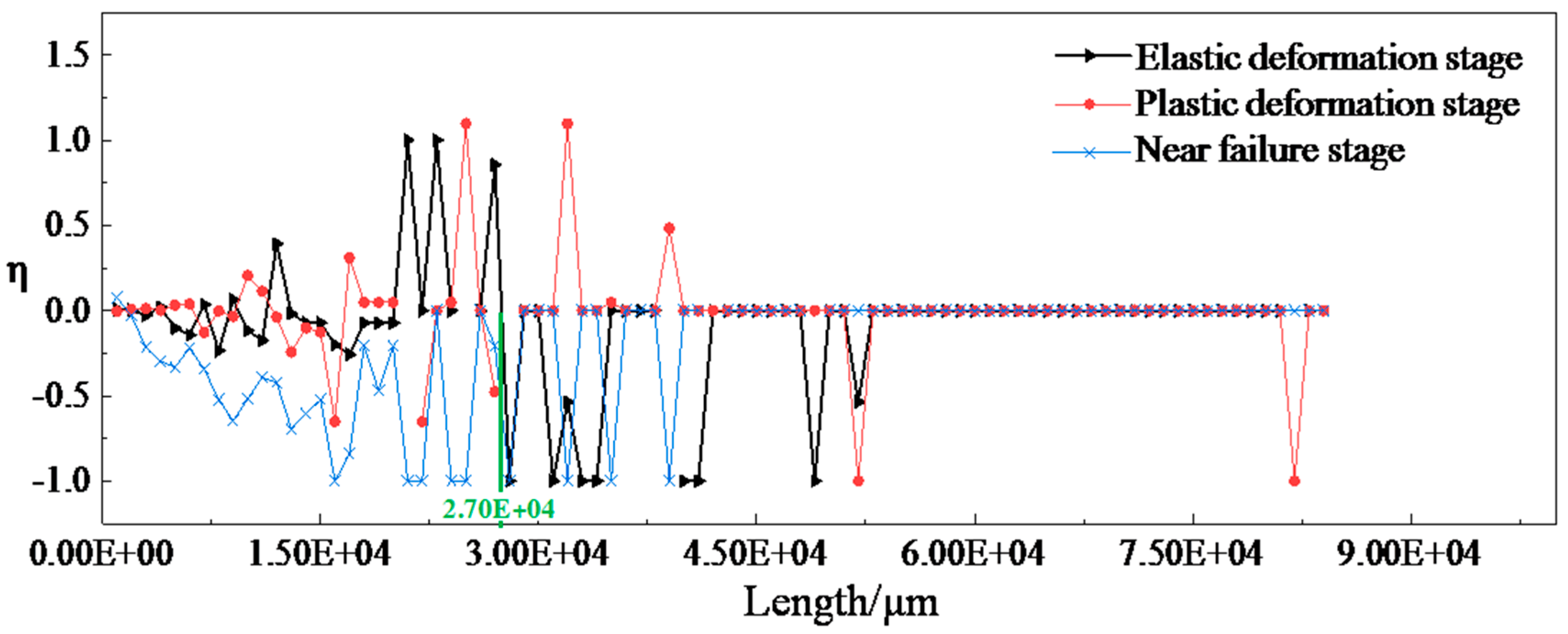
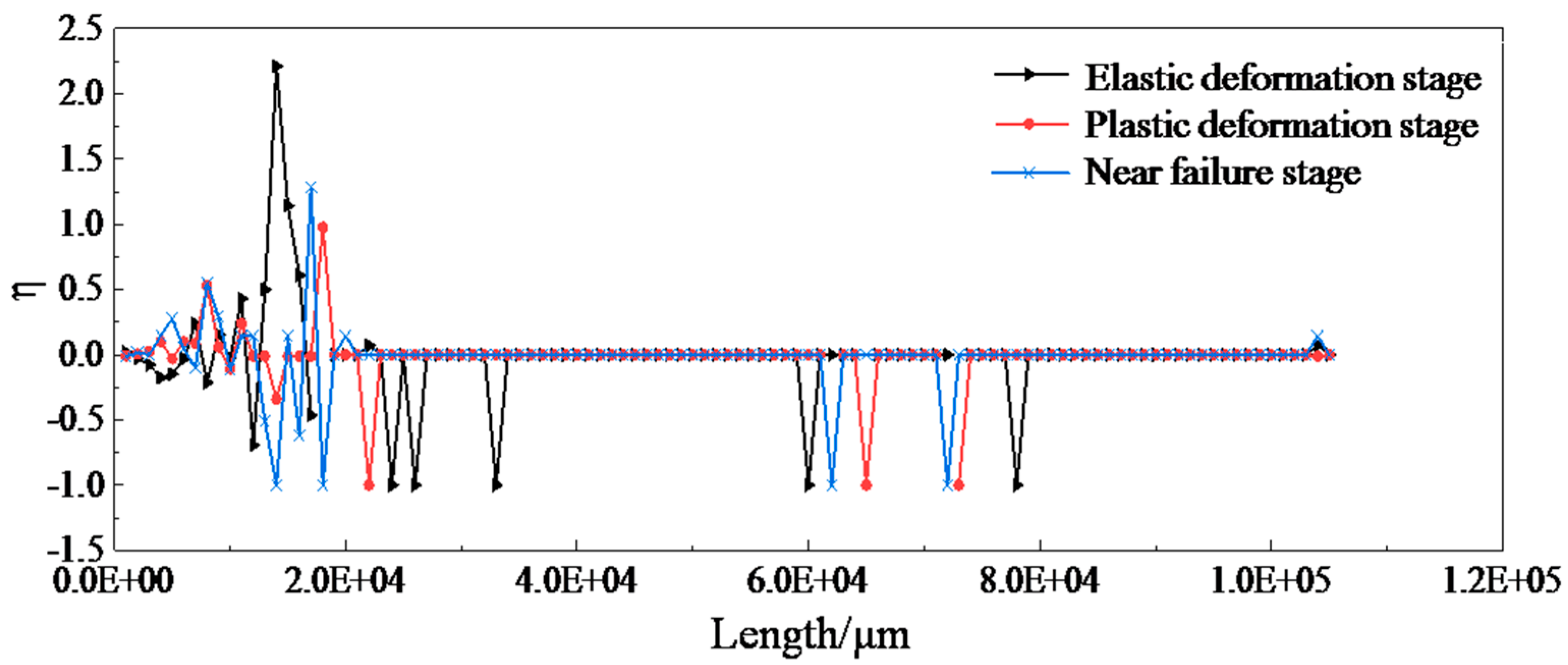
3.2. Evolution Behavior of Pre-Peak Deformation Fracture and Main Control Fracture Scale of Coal–Rock
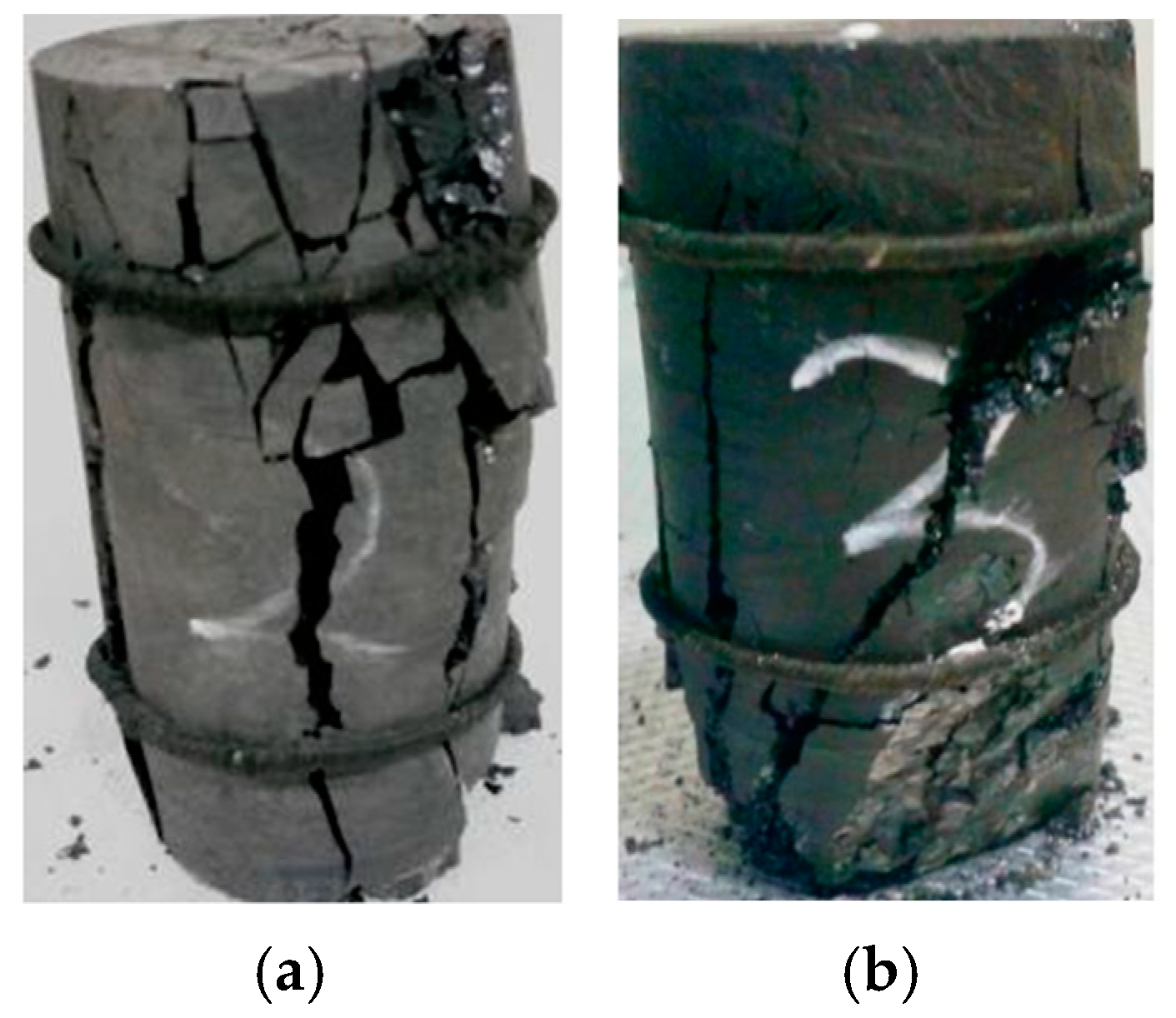
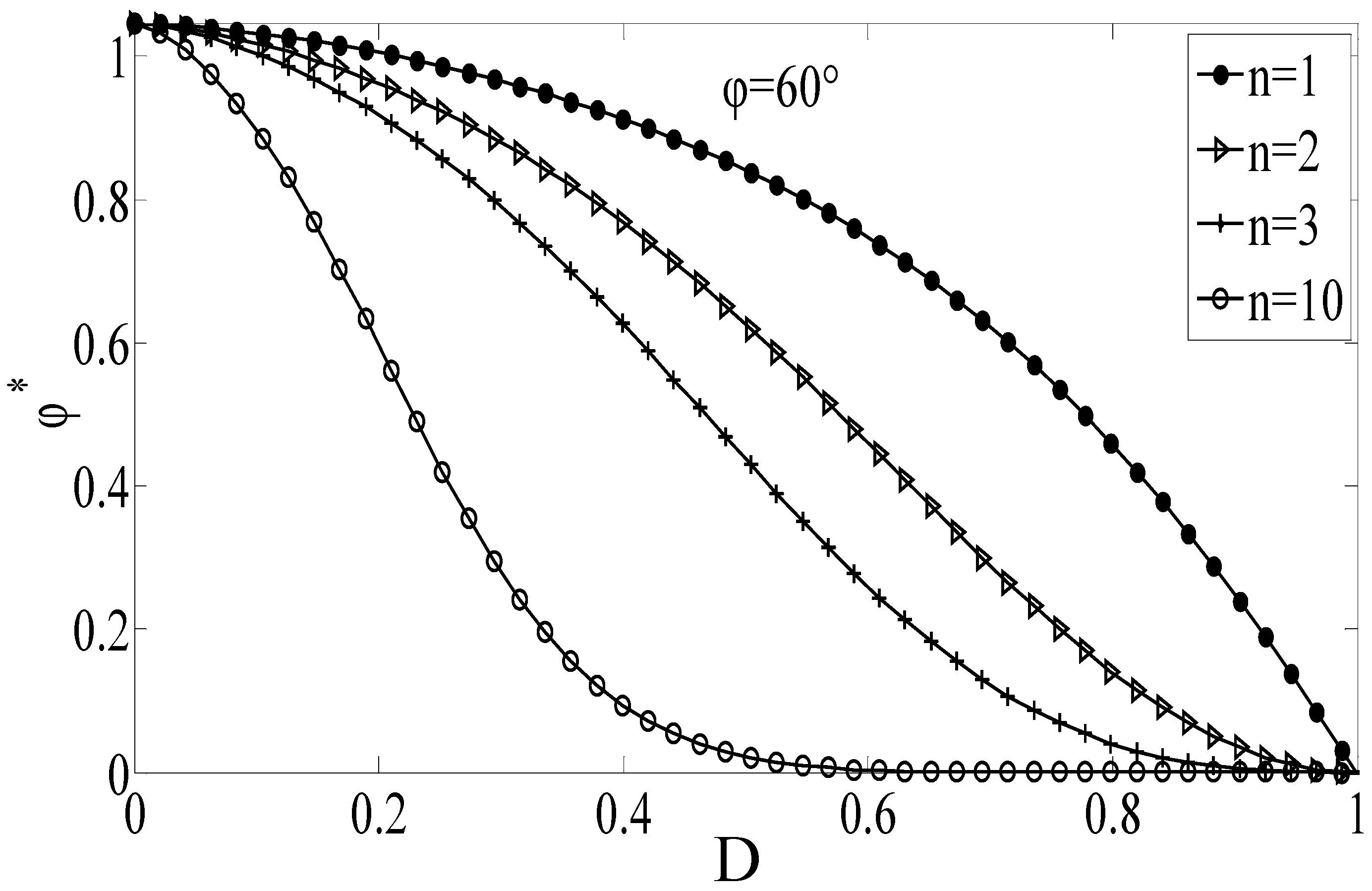
3.3. The Failure Characteristics Are Affected by the Fracture Development
4. Conclusions
- (1)
- The fracture evolution behavior of coal–rock specimens with strong bursting liability in their elastic deformation stage exhibits disorder and the scale is relatively dispersed. The boundary is about one-third of the specimen size; after entering the plastic deformation stage, the fracture evolution behavior becomes orderly, the continuity is improved, and the scale is gradually concentrated in the range of about one-third of the specimen size. When it is observed that the fractures in this range begin to develop concentratedly, the specimen can be considered to be in the “quasi-catastrophic” stage, and an early warning can be given.
- (2)
- Under the loading condition, the fracture evolution behavior of coal–rock with no bursting liability is relatively soft and orderly without a leap or sharp rise in the scale. The damage of the material is mainly controlled by the increase in small fractures, which contributes to the penetration of large fractures. The size of this small fracture is less than one-fifth of the specimen size.
- (3)
- The failure mechanism of coal–rock is affected by the fracture structure. Regarding the coal–rock media with different bursting liabilities, two different failure mechanisms under uniaxial loading will occur: tensile failure and shear failure/plastic deformation. The root cause lies in the relationship between Poisson’s ratio and the internal friction angle. Specifically, the property of the coal–rock fracture structure controlling the internal friction angle of the material makes the coal–rock failure mechanism transition from tensile failure to shear failure or plastic deformation as the bursting liability decreases.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Rong, F.; Wang, H.; Xia, M.; Ke, F.; Bai, Y. Catastrophic Rupture Induced Damage Coalescence in Heterogeneous Brittle Media. Pure Appl. Geophys. 2006, 163, 1847–1865. [Google Scholar] [CrossRef]
- He, X.; Wang, E.; Nie, B.; Liu, M.; Zhang, L. Electromagnetic Dynamics of Coal or Rock Rheology; Science Press: Beijing, China, 2003; pp. 12–28. [Google Scholar]
- Zhang, C.; Xu, J.; Yin, G.; Peng, S.; Li, Q.; Chen, Y. A Novel Large-Scale Multifunctional Apparatus to Study the Disaster Dynamics and Gas Flow Mechanism in Coal Mines. Rock Mech. Rock Eng. 2019, 52, 2889–2898. [Google Scholar] [CrossRef]
- Niu, Y.; Song, X.; Li, Z.; Wang, E.; Liu, Q.; Zhang, X.; Cai, G.; Zhang, Q. Experimental study and field verification of stability monitoring of gas drainage borehole in mining coal seam. J. Pet. Sci. Eng. 2020, 189, 106985. [Google Scholar] [CrossRef]
- Maleki, H. High stress gradient, critical factor affecting rock burst in the United States mines. In Proceedings of the SRM International Symposium (EUROCK 2021), Turin, Italy, 21–24 September 2021; Volume 833. [Google Scholar]
- Department of the Environment. Background Review: Subsidence from Coal Mining Activities; Department of the Environment: Canberra, Australia, 2005. [Google Scholar]
- Bai, Y.L.; Lu, C.S.; Ke, F.J.; Xia, M. Evolution induced catastrophe. Phys. Lett. 1994, 185, 196–200. [Google Scholar] [CrossRef]
- Xia, M.F.; Wei, Y.J.; Ke, F.J.; Bai, Y.L. Critical Sensitivity and Trans-scale Fluctuations in Catastrophic Rupture. Pure Appl. Geophys. 2002, 159, 2491–2509. [Google Scholar] [CrossRef]
- Wei, Y.J.; Xia, M.F.; Ke, F.J.; Yin, X.; Bai, Y. Evolution-induced catastrophe and its predictability. Pure Appl. Geophys. 2000, 157, 1945–1957. [Google Scholar] [CrossRef]
- Bazant, Z.P.; Chen, E.P. Scaling of Structural Failure. Appl. Mech. Rev. 1997, 50, 593–627. [Google Scholar] [CrossRef]
- Wang, H.Y.; He, G.W.; Xia, M.F.; Ke, F.; Bai, Y. Multiscale coupling in complex mechanical systems. Chem. Eng. Sci. 2004, 59, 1677–1686. [Google Scholar] [CrossRef]
- Rong, F.; Wang, H.Y.; Xia, M.F.; Ke, F.; Bai, Y. Trans-scale coupling in multi-scale simulation. Int. J. Multiscale Numer. Engrg. 2006, 4, 169–182. [Google Scholar] [CrossRef]
- Hao, S.W.; Wang, H.Y.; Xia, M.F.; Ke, F.; Bai, Y. Relationship between strain localization and catastrophic rupture. Theor. Appl. Fract. Mech. 2007, 48, 41–49. [Google Scholar] [CrossRef]
- Du, K.; Bi, R.; Khandelwal, M.; Li, G.; Zhou, J. Occurrence mechanism and prevention technology of rockburst, coal bump and mine earthquake in deep mining. Geomech. Geophys. Geo-Energy Geo-Resour. 2024, 10, 98. [Google Scholar] [CrossRef]
- Peng, S.; Wu, B.; Xu, J.; Shi, T.; Yan, F.; Wu, S. Research on 3D reconstruction method of rock fracture surface based on CGAL. Chin. J. Rock Mech. Eng. 2020, 39 (Suppl. S2), 3450–3463. [Google Scholar]
- Ding, X.; Liu, J.; Bai, S.; Sheng, Q.; Xu, P. Study on numerical simulation of structure effects of rock mass creep. Chin. J. Rock Mech. Eng. 2006, 25 (Suppl. S2), 3642–3649. [Google Scholar]
- Zhang, Z.; Zhao, Y.; Liu, Q.; Sheng, Q.; Yang, Y. Study of structure effect of jointed rock mass deformation modulus. Chin. J. Rock Mech. Eng. 2011, 31 (Suppl. S2), 4158–4166. [Google Scholar]
- Liu, C.; Ma, T.; Cheng, X. Failure characteristics of fractured coal rock under different angle discontinuities. Saf. Coal Mines 2015, 46, 218–220. [Google Scholar]
- Zheng, Y.; Kong, L. Geomechanics. Rock Plasticity Mechanics; Science Press: Beijing, China, 2010. [Google Scholar]
- Jaeger, J.C. Shear failure of anisotropic rocks. Geol. Mag. 1960, 97, 65–72. [Google Scholar] [CrossRef]
- Yang, Z.Y.; Chen, J.M.; Huang, T.H. Effect of joint sets on the strength and deformation of rock mass models. Int. J. Rock Mech. Min. Sci. 1998, 35, 75–84. [Google Scholar] [CrossRef]
- Nasseri, M.H.; Rao, K.S.; Ramamurthy, T. Failure mechanism in schistose rocks. Int. J. Rock Mech. Min. Sci. 1997, 34, 460–470. [Google Scholar] [CrossRef]
- Sun, G.; Sun, Y. Principle of Rock Mechanics; Science Press: Beijing, China, 2011. [Google Scholar]
- Han, J.; Li, S.; Li, S. Transformation mechanism and criterion model of structural control and stress control for rock mass failure. Sci. China Phys. Mech. Astron. 2013, 43, 177–185. [Google Scholar]
- Xiao, T.; Li, X.; Jia, S. Triaxial test research and mechanical analysis based on structure surface effect of deep rock mass with single fissure. Chin. J. Rock Mech. Eng. 2012, 31, 1666–1673. [Google Scholar]
- Du, S.; Lv, Y.; Luo, Z.; Huang, M. Combined test system for size effect of rock joint shear strength and its primary application research. Chin. J. Rock Mech. Eng. 2021, 40, 13. [Google Scholar]
- Zhang, L.; Kan, Z.; Xue, J.; Li, M. Study on permeability law of intact and fractured coal under cyclic loading and unloading. Chin. J. Rock Mech. Eng. 2021, 40, 2487–2499. [Google Scholar]
- Sun, B.; Wang, X.; Li, Z. Meso-scale image-based modeling of reinforced concrete and adaptive multi-scale analyses on damage evolution in concrete structures. Comput. Mater. Sci. 2015, 110, 39–53. [Google Scholar] [CrossRef]
- Li, B.; You, M. Nonlinear strength of rocks with cohesion and friction using the exponential criterion. Chin. J. Rock Mech. Eng. 2016, 35, 1945–1953. [Google Scholar]
- Gao, M.; Li, T.; Meng, L.; Chen, G. The method to identify characteristic stresses of rock in different stages during failure process. Chin. J. Rock Mech. Eng. 2016, 35 (Suppl. S2), 3577–3588. [Google Scholar]
- Feng, C.; Wu, Y.; Feng, F. Study of fatigue properties and critical stress measurement method of rock. Chin. J. Rock Mech. Eng. 2010, 2734–2740. [Google Scholar]
- Yuan, W.; Li, X.; He, J.; Li, S.; Cheng, G. Structure effect study of deformation and failure of rock and soil aggregate with CT technique. Chin. J. Rock Mech. Eng. 2013, 3134–3140. [Google Scholar]
- Wang, D.; Zeng, F.; Wang, J.; Wei, J.; Jiang, Z.; Wang, X.; Zhang, P.; Yu, C. Dynamic evolution characteristics and fractal law of loaded coal fractures by micro industrial CT. Chin. J. Rock Mech. Eng. 2020, 39, 90–99. [Google Scholar] [CrossRef]
- Lurie, A.I.; Belyaev, A. Theory of Elasticity; Springer: Berlin/Heidelberg, Germany, 2005. [Google Scholar]
- Brady, B.H.G.; Brown, E.T. Rock Mechanics and Mining Engineering. In Rock Mechanics; Springer: Dordrecht, The Netherlands, 1999. [Google Scholar]
- Liu, C.; Zhang, Z.; Yuan, J.; Liu, Y. Effect factors and determination methods of slope angle of rock slope stability. Hydro-Sci. Eng. 2016, 1, 23–29. [Google Scholar]
- Qiu, Z. Nonlinear Dynamic Damage Mechanics Theory and Numerical Analysis Model. Ph.D. Thesis, Zhejiang University, Hangzhou, China, 2005. [Google Scholar]




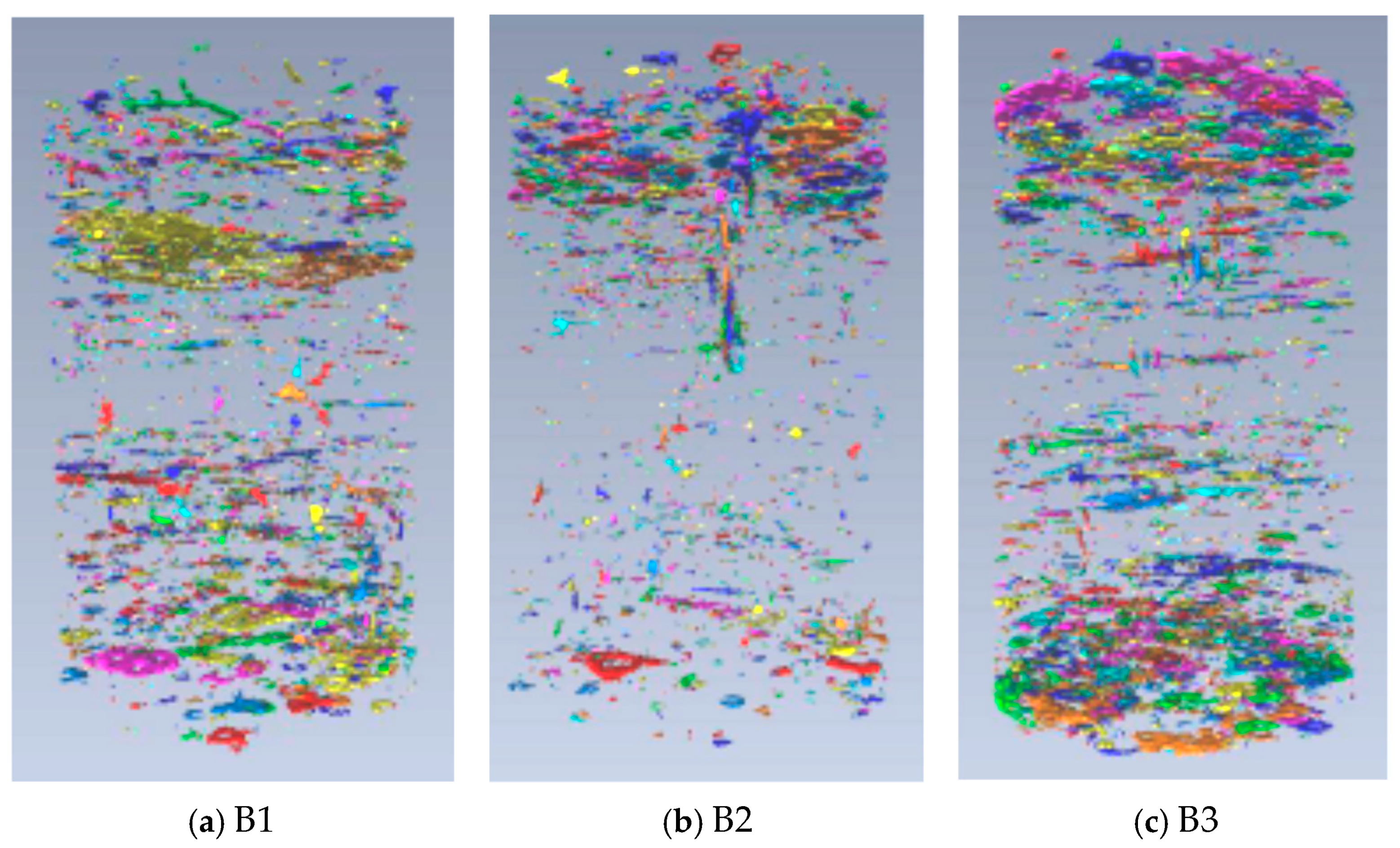
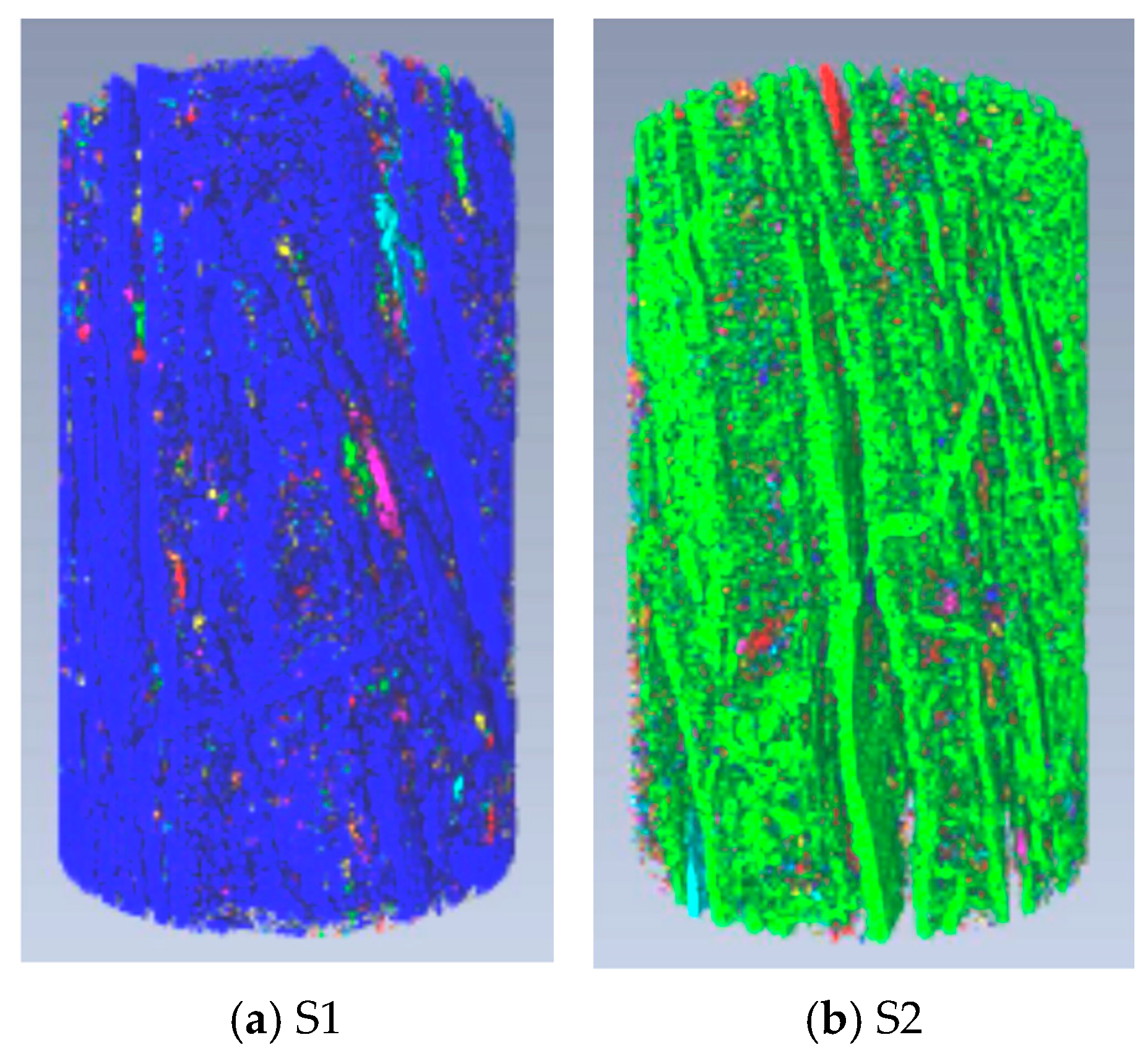

| Coal Mine Name | Elastic Modulus/(GPa) | Poisson’s Ratio | Tensile Strength/(MPa) | Uniaxial Compressive Strength/(MPa) | Elastic Energy Index—WET | Bursting Liability |
|---|---|---|---|---|---|---|
| Bayan Gaole | 2.216 | 0.28 | 1.158 | 28.568 | 17.603 | Strong |
| Shennan’ao | 0.802 | 0.38 | 0.56 | 5.929 | 1.82 | No |
| No. of Specimen | Bursting Liability | Loading Rate /(N/s) | Loading Stage | Actual Load /kN |
|---|---|---|---|---|
| B1 | Strong | 100 | Elastic deformation stage | 10.00 |
| Plastic deformation stage | 28.00 | |||
| Near-failure stage | 34.00 | |||
| B2 | Elastic deformation stage | 18.00 | ||
| Plastic deformation stage | 39.00 | |||
| Near-failure stage | 54.00 | |||
| B3 | Elastic deformation stage | 21.00 | ||
| Plastic deformation stage | 29.00 | |||
| Near-failure stage | 33.00 | |||
| S1 | No | 100 | Elastic deformation stage | 3.00 |
| Plastic deformation stage | 7.00 | |||
| Near-failure stage | 8.00 | |||
| S2 | Elastic deformation stage | 5.00 | ||
| Plastic deformation stage | 8.00 | |||
| Near-failure stage | 9.00 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, H.; He, S.; Mo, Y.; Sun, Z.; Li, L. A Study on the Failure Characteristics of Coal–Rock Structures with Different Bursting Liabilities. Processes 2025, 13, 652. https://doi.org/10.3390/pr13030652
Li H, He S, Mo Y, Sun Z, Li L. A Study on the Failure Characteristics of Coal–Rock Structures with Different Bursting Liabilities. Processes. 2025; 13(3):652. https://doi.org/10.3390/pr13030652
Chicago/Turabian StyleLi, Hongyan, Shi He, Yunlong Mo, Zhongxue Sun, and Lei Li. 2025. "A Study on the Failure Characteristics of Coal–Rock Structures with Different Bursting Liabilities" Processes 13, no. 3: 652. https://doi.org/10.3390/pr13030652
APA StyleLi, H., He, S., Mo, Y., Sun, Z., & Li, L. (2025). A Study on the Failure Characteristics of Coal–Rock Structures with Different Bursting Liabilities. Processes, 13(3), 652. https://doi.org/10.3390/pr13030652





