Energy Characteristics of the Compressor in a Heat Pump Based on Energy Conversion Theory
Abstract
1. Introduction
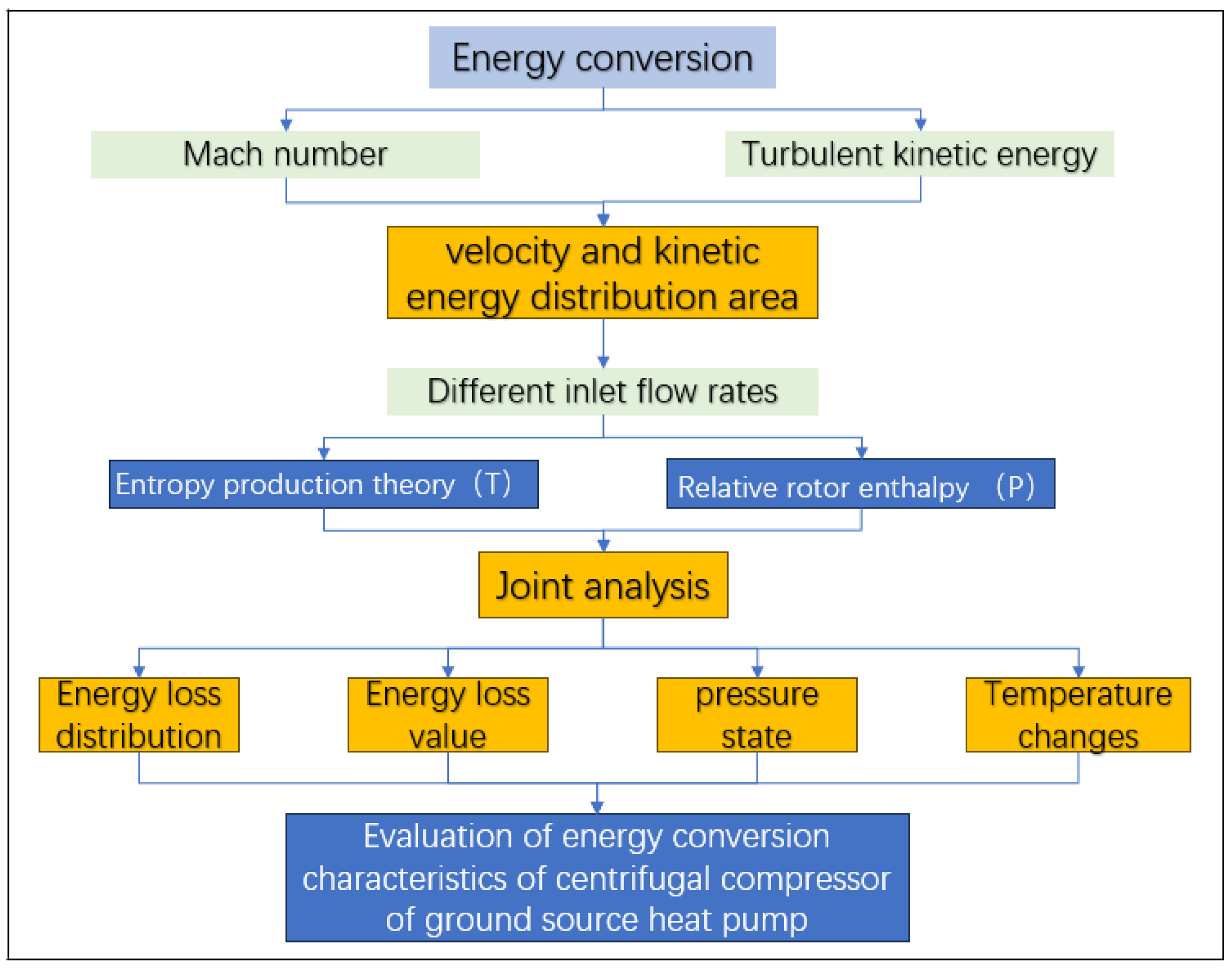
2. Methodology
2.1. Entropy Generation Theory
2.2. Relative Energy Rotor Enthalpy Theory
2.3. Energy Conversion Theory
3. Physical Model and Numerical Method
3.1. Physical Model
3.2. Mesh and Irrelevance Verification
3.3. Numerical Method
3.3.1. Governing Equations
- (1)
- Continuity equation
- (2)
- Momentum equation
- (3)
- Energy conservation equation
3.3.2. Turbulence Model Selection
- (1)
- turbulent kinetic energy equation (K equation)
- (2)
- specific dissipation rate equation (ω equation)
- (3)
- SST correction terms
3.3.3. Boundary Conditions and Convergence Criteria
4. Results and Discussion
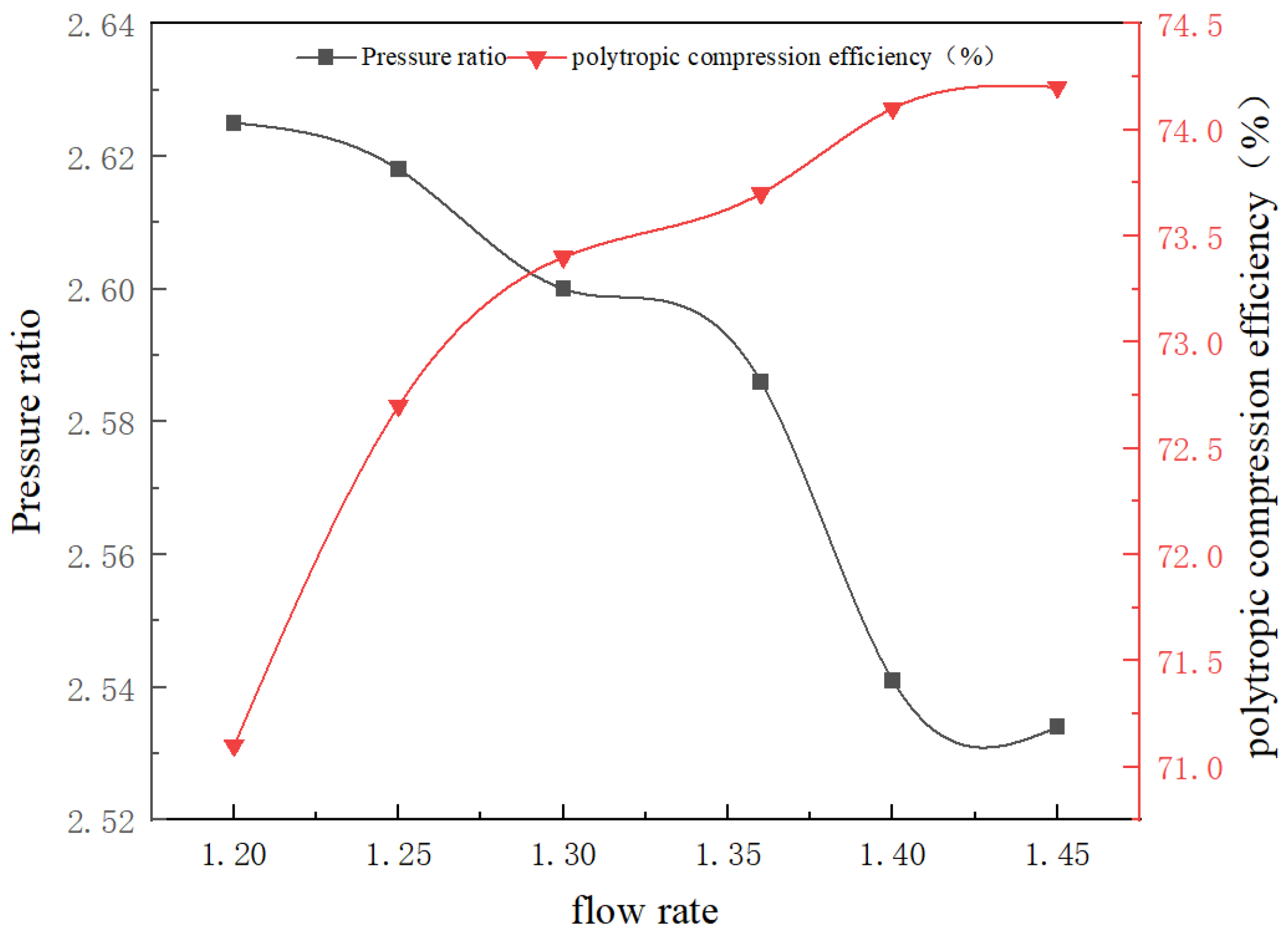
4.1. Aerodynamic Performance
4.2. Energy Characteristics of Internal Flow Field
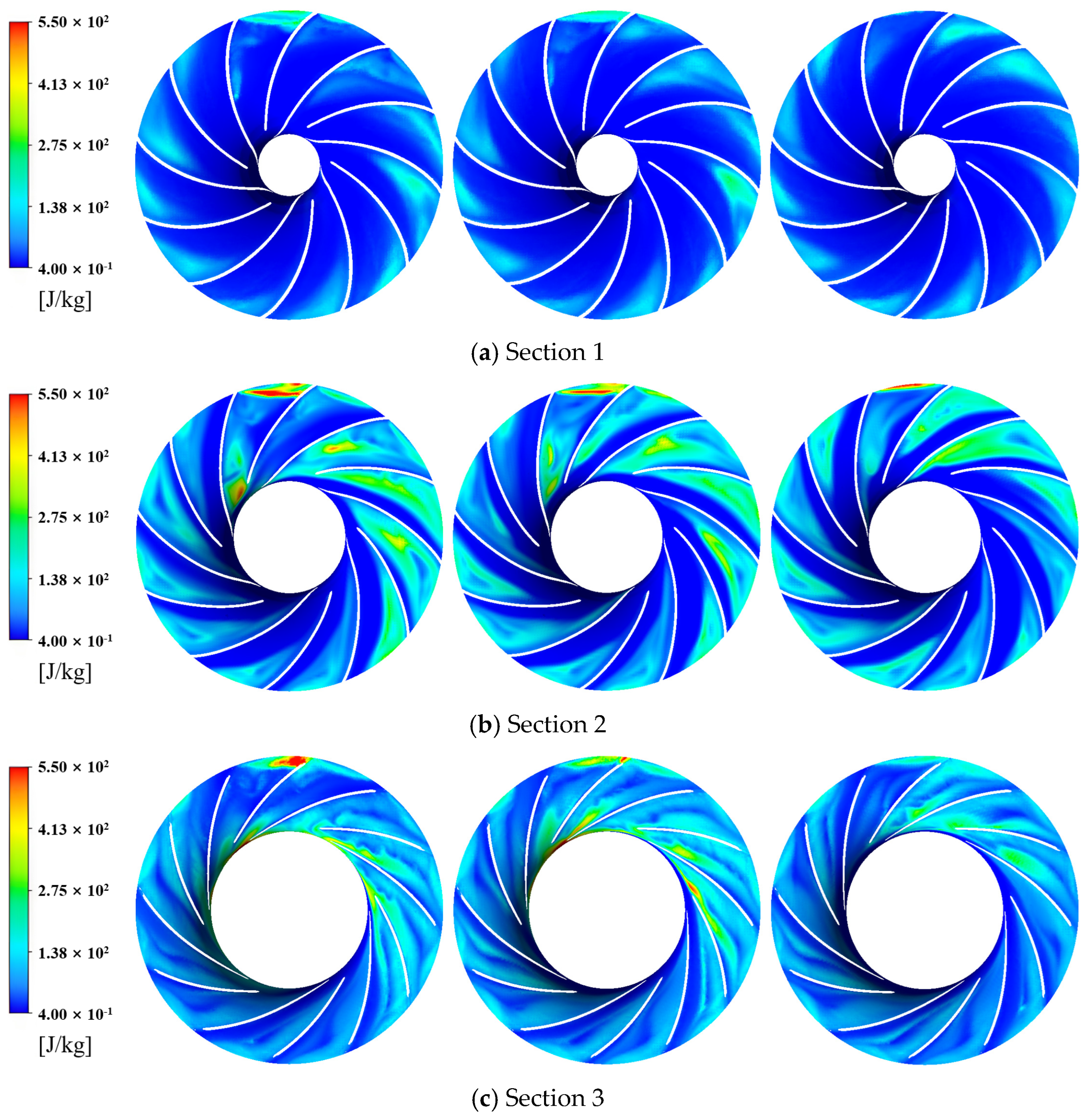
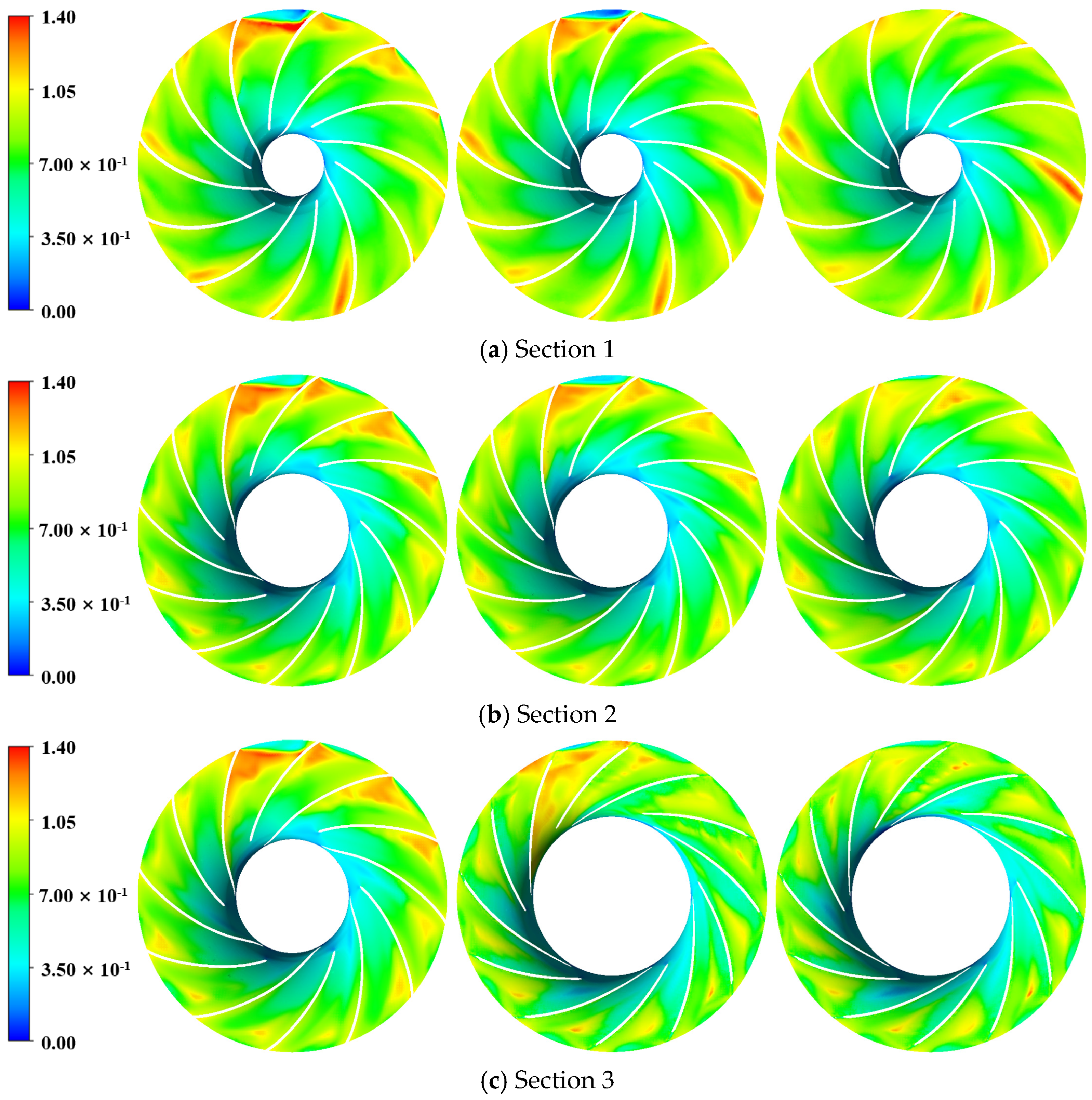
4.2.1. Turbulent Kinetic Energy Distribution
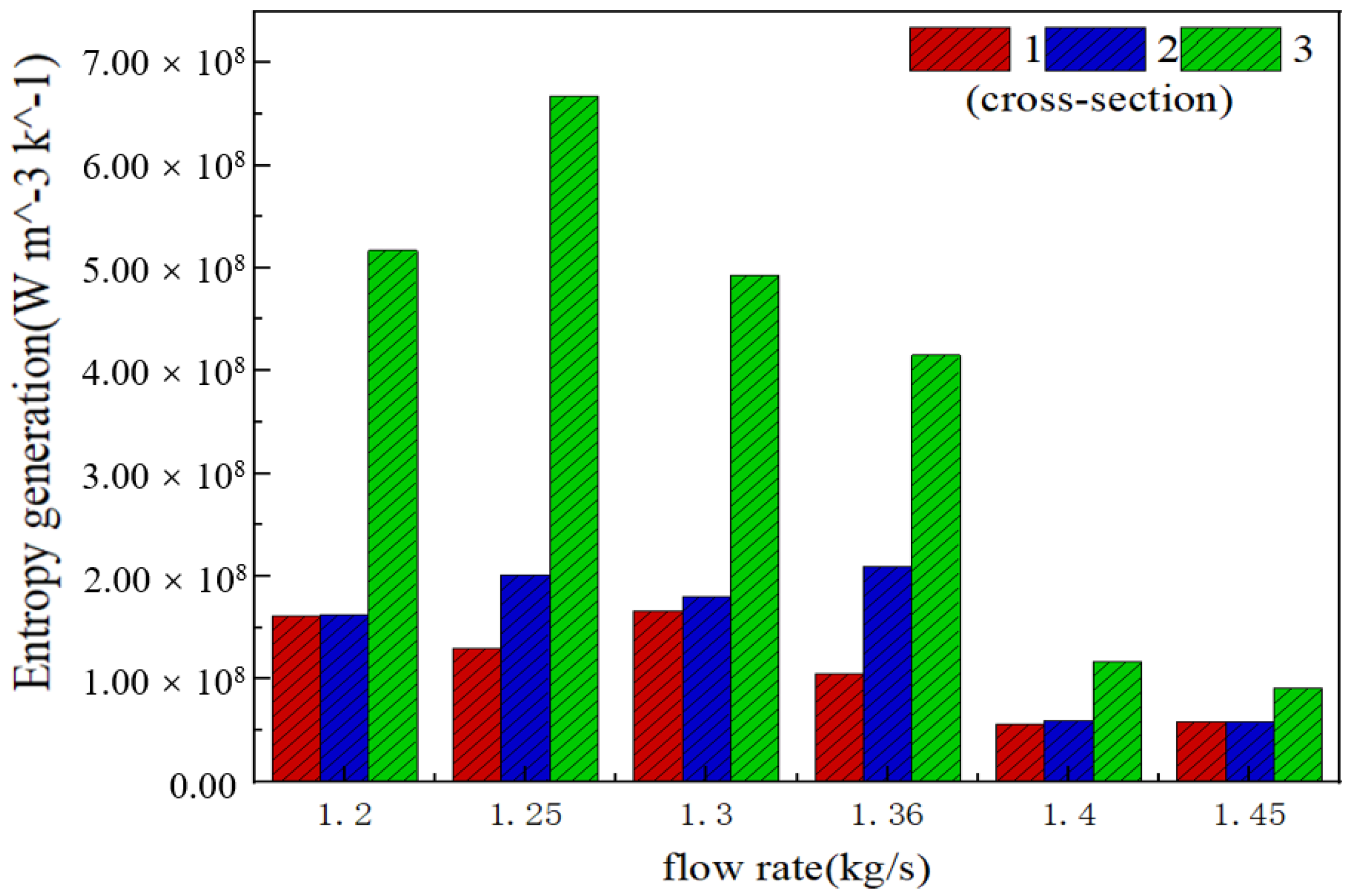
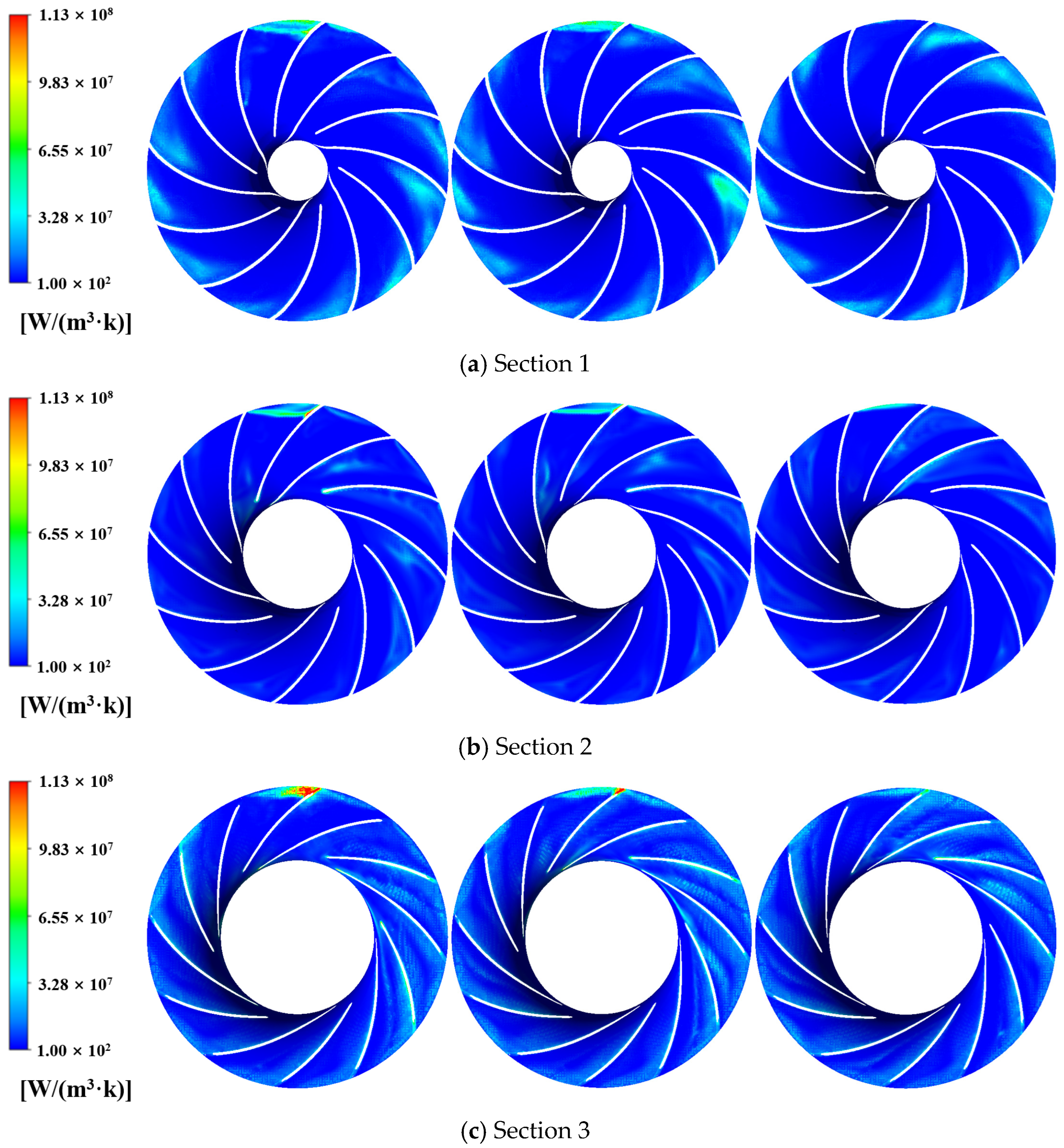
4.2.2. Entropy Generation Distribution
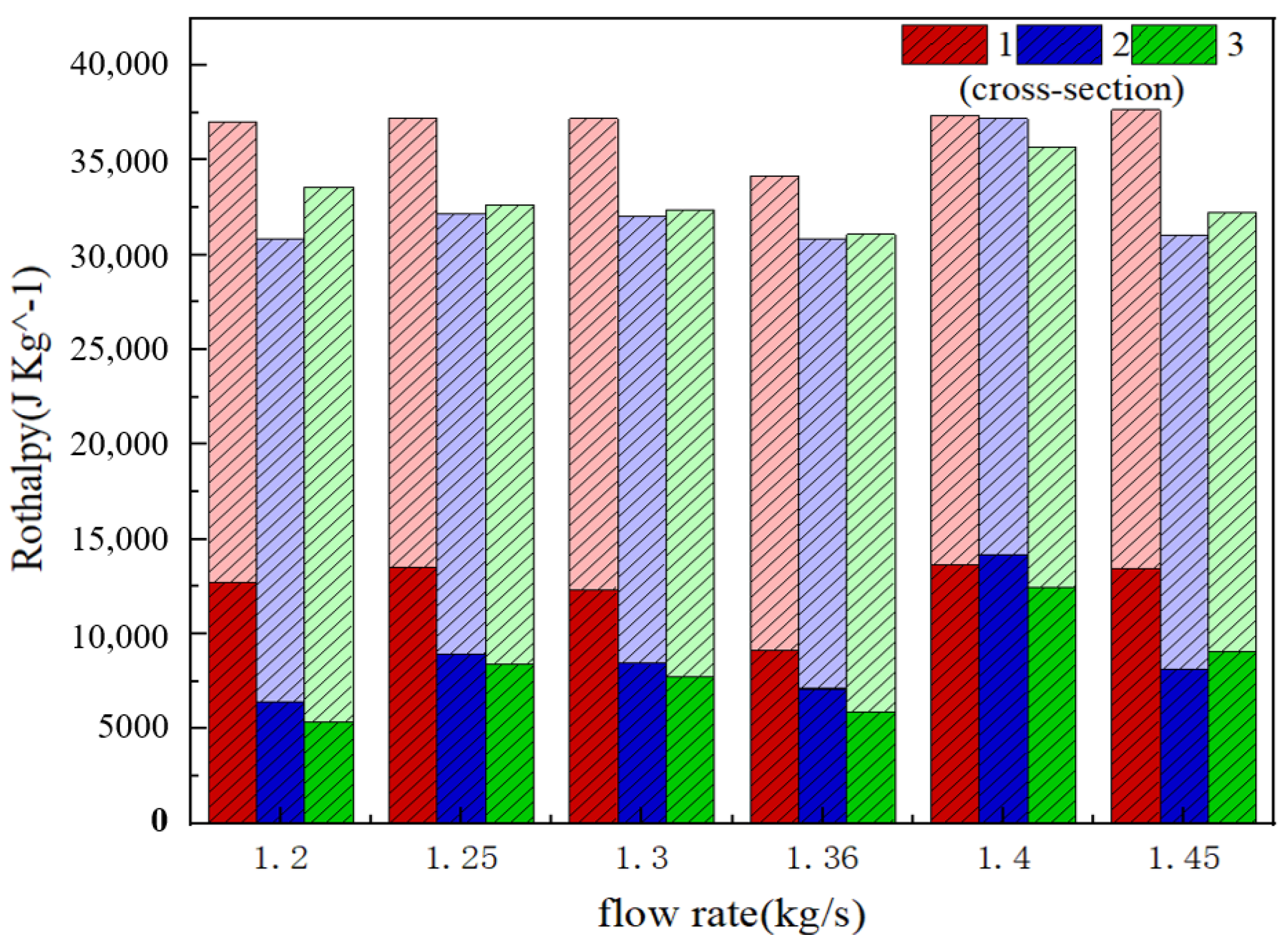
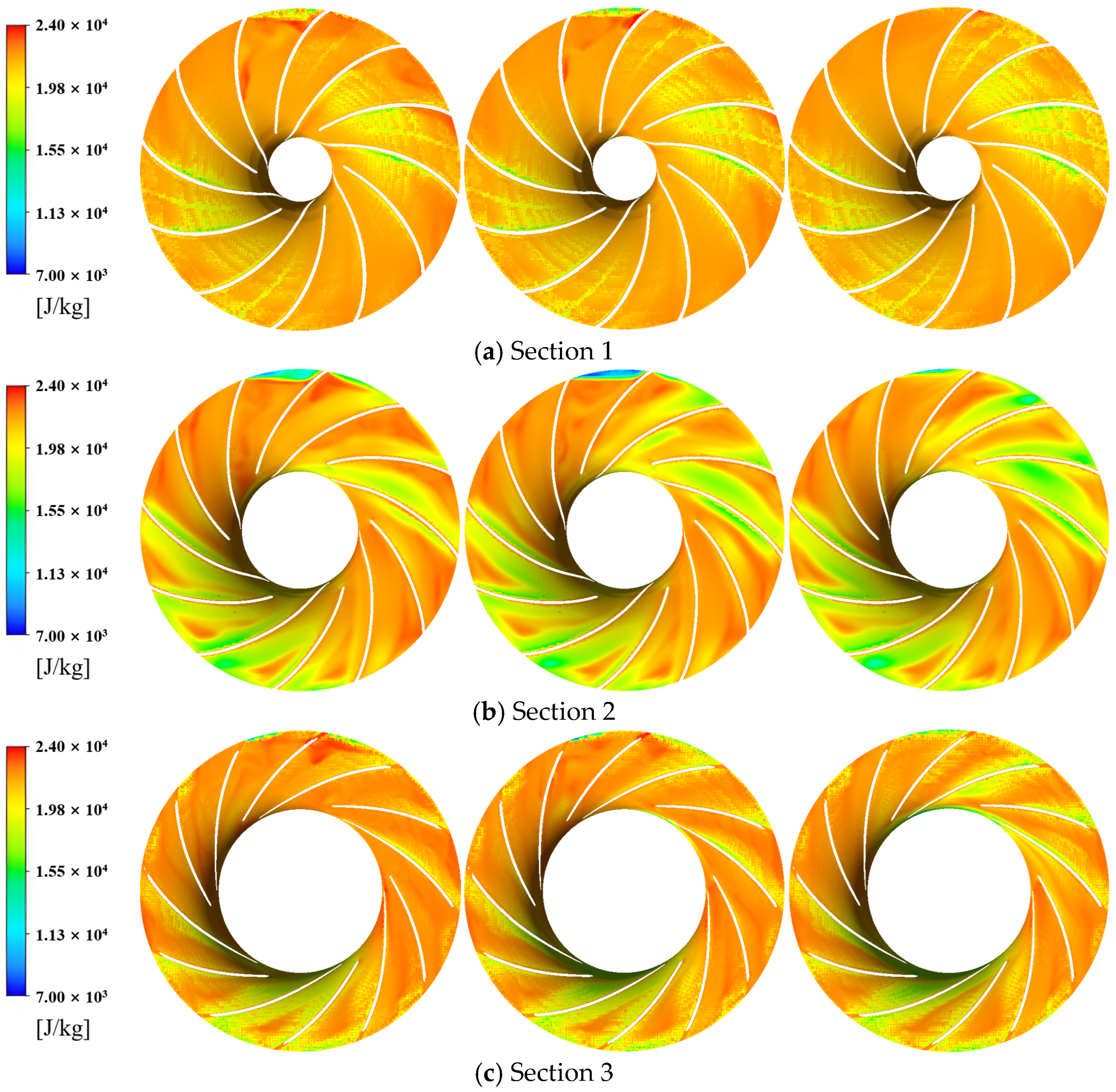
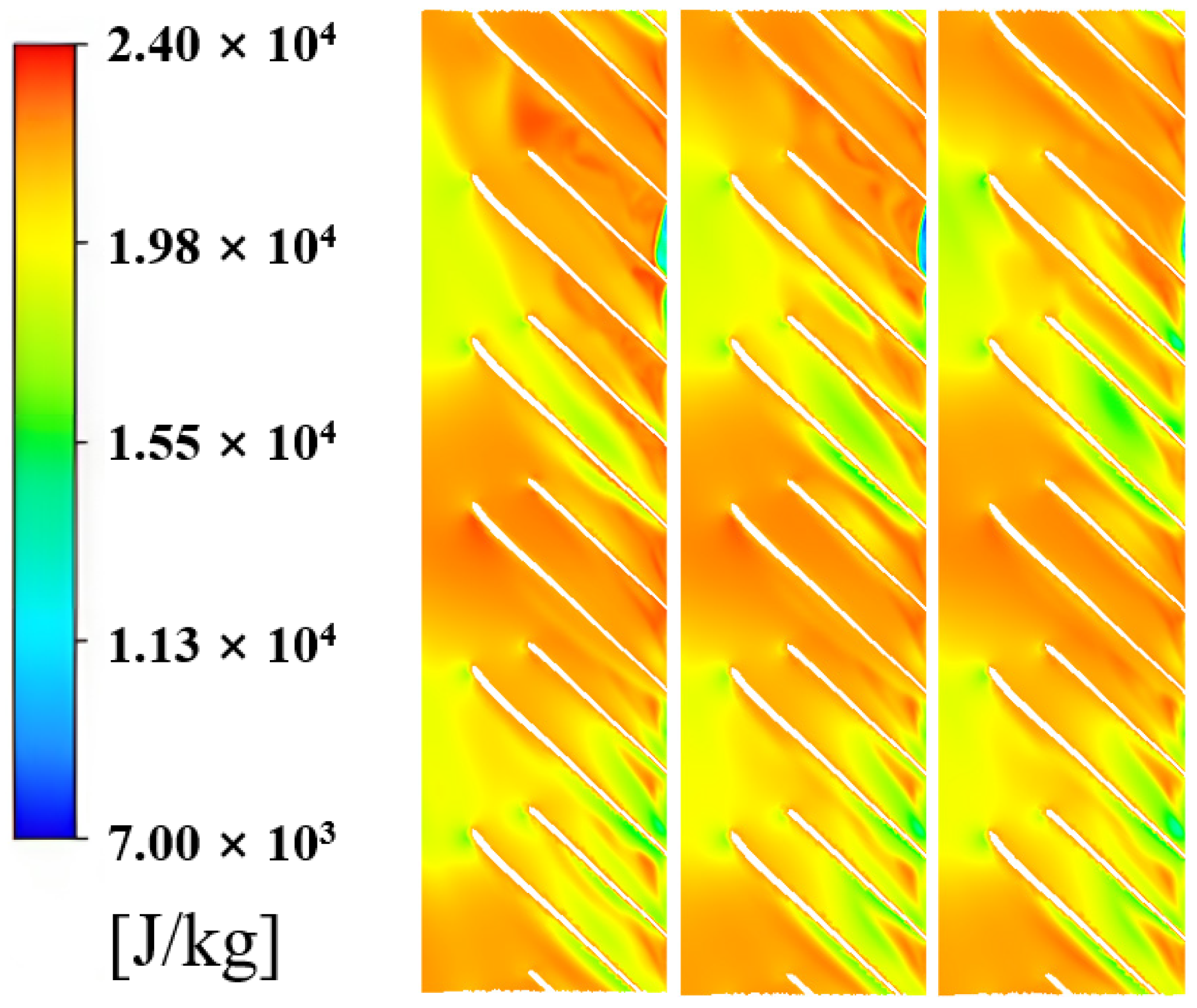
4.2.3. Rothalpy Distribution
5. Conclusions
- (1)
- In the flow range of 1.2–1.45 kg/s, the turbulent flow energy first decreases and then rises with the increase in flow, and the maximum value is concentrated at the tongue, mainly distributed in the impeller inlet and middle. The wave resistance loss reflected by the Mach number is mainly concentrated between the blades and near the blade root, and the Mach number drops sharply near the exit. Simultaneously, as the flow rate increases, the resistance within the impeller rises, leading to a decrease in the Mach number. The Mach number can be used to confirm the relationship between turbulent flow energy and velocity fluctuations from the side;
- (2)
- Across various flow rates, the primary areas of energy loss within the compressor are concentrated at the blade tips and in the vicinity of the tongue. When the flow rate increases from 1.2 kg/s to 1.45 kg/s, the maximum total entropy area decreases by 161%, and the minimum Rothalpy increases by about 90%. As the flow increases, the entropy generation initially rises and then falls, with the Rothalpy at the design operating point reaching its minimum. However, no clear trend is observed overall;
- (3)
- According to the analysis of the energy conversion theory, the high-entropy area can indicate a sharp change in temperature to a certain extent, which will not only lead to energy dissipation, but also affect the life of the device. The low Rothalpy can reflect the pressure situation in the area to a certain extent, which is usually manifested as negative pressure, which affects the compression efficiency.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Widiatmojo, A.; Chokchai, S.; Takashima, I.; Uchida, Y.; Yasukawa, K.; Chotpantarat, S.; Charusiri, P. Ground-Source Heat Pumps with Horizontal Heat Exchangers for Space Cooling in the Hot Tropical Climate of Thailand. Energies 2019, 12, 1274. [Google Scholar] [CrossRef]
- Wang, P.L.; Dong, F.; Jia, H.D.; Jiang, J.; Chen, S.; Hu, S.; Rao, J.; Huang, W. Influences of Centrifugal Compressor Impeller Design Based on Streamline Curvature Method. Fluid Mach. 2013, 41, 27–32. [Google Scholar] [CrossRef]
- He, X.; Gong, W.; Deng, J.; Li, J.; Liang, L. Influence of Impeller Tip Clearance on the Performance of Centrifugal Refrigeration Compresser. J. Xian Jiaotong Univ. 2019, 53, 30–37. [Google Scholar] [CrossRef]
- Li, S.; Ma, S.; Zhang, J. Characteristic Analysis of Flow Field in Centrifugal Impeller of High Temperature Heat Pump with Variable Blade Numbers. Fluid Mach. 2008, 10, 15–18+62. [Google Scholar] [CrossRef]
- Xu, C.; Amano, R.S. Effects of Asymmetric Radial Clearance on Performance of a Centrifugal Compressor. J. Energy Resour. Technol. 2018, 140, 052003. [Google Scholar] [CrossRef]
- Xu, C.; Amano, R.S. Centrifugal Compressor Performance Improvements Through Impeller Splitter Location. J. Energy Resour. Technol. 2018, 140, 051201. [Google Scholar] [CrossRef]
- Gao, F.; Zhu, D.; Pan, X. CFD Analysis of Stage Flow in Small Flow Centrifugal Refrigeration Compressor. Fluid Mach. 2017, 45, 78–82+57. [Google Scholar] [CrossRef]
- Gao, F.; Pan, X.; Zhu, D. Simulating Flow in Pump Impeller of Airborne Centrifugal Refrigeration Compressor. Mech. Sci. Technol. 2019, 38, 963–969. [Google Scholar] [CrossRef]
- Zhou, Y.; Sun, Y.; Hu, F.; Tan, J.; Yang, S.; Liu, X. Parameters Optimization and Performance Investigation of Centrifugal Compressor with Bionic Impeller Inspired by Owl Wing. J. Xian Jiaotong Univ. 2023, 7, 1–8. [Google Scholar] [CrossRef]
- Feng, R.; Lian, J.; Li, J. Analysis on influence of circumferential deviation of splitter blade on internal flow field and performance of high-speed centrifugal compressor. J. Mech. Des. 2022, 39, 85–91. [Google Scholar] [CrossRef]
- Hung, K.-S.; Ho, K.-Y.; Hsiao, W.-C.; Kuan, Y.-D. The Characteristic of High-Speed Centrifugal Refrigeration Compressor with Different Refrigerants via CFD Simulation. Processes 2022, 10, 928. [Google Scholar] [CrossRef]
- Shivani, S.; Amar Murthy, A.; Srinivas, G. Aerodynamic performance enhancement of centrifugal compressor using numerical techniques. F1000Research 2024, 13, 480. [Google Scholar] [CrossRef]
- Meng, C.; Zuo, Z.; Sun, J.; Guo, W.; Liang, Q.; Chen, H. Internal Inflow Study on a High-Pressure Centrifugal Compressor with Shroud and Backside Cavity in a Compressed Air Energy Storage System. Proc. Inst. Mech. Eng. A J. Power Energy 2022, 236, 1418–1432. [Google Scholar] [CrossRef]
- Wang, Y.; Liu, X.; Zhou, Y.; Fan, H.; Chen, Y.; Zhang, Z. Performance Analysis and Multi-Parameter Optimization of Centrifugal Impeller Configuration for the Centrifugal Compressor Used in Fuel Cells. J. Xian Jiaotong Univ. 2022, 56, 164–174. [Google Scholar] [CrossRef]
- Zhang, H.; Zhang, H.; Wang, Z. Effect on Vehicle Turbocharger Exhaust Gas Energy Utilization for the Performance of Centrifugal Compressors under Plateau Conditions. Energies 2017, 10, 2121. [Google Scholar] [CrossRef]
- Liu, S.; Wang, Q.; Ren, Y.; Zhang, K.; Jin, R. Simulation Analysis of Compressor Flow Field in Turbocharger. Fluid Mach. 2018, 46, 23–27+12. [Google Scholar] [CrossRef]
- Li, S.; Liu, Y.; Omidi, M.; Zhang, C.; Li, H. Numerical Investigation of Transient Flow Characteristics in a Centrifugal Compressor Stage with Variable Inlet Guide Vanes at Low Mass Flow Rates. Energies 2021, 14, 7906. [Google Scholar] [CrossRef]
- Li, Z.; Wu, Y.; Li, L.; Lu, X.; Han, G. Numerical Investigation of Low Reynolds Number Effects on Energy Loss inside Centrifugal Compressor with Different Inlet Conditions. Aerosp. Sci. Technol. 2024, 146, 108923. [Google Scholar] [CrossRef]
- Zhang, F.; Dong, Q.; Wang, P. Entropy Analysis of Thermodynamic Process for Centrifugal Compressors. Chin. J. Turbomach. 2011, 6, 29–31+43. [Google Scholar] [CrossRef]
- Kock, F.; Herwig, H. Local Entropy Production in Turbulent Shear Flows: A High-Reynolds Number Model with Wall Functions. Int. J. Heat Mass Transf. 2004, 47, 2205–2215. [Google Scholar] [CrossRef]
- Mathieu, J.; Scott, J. An Introduction to Turbulent Flow; Cambridge University Press: Cambridge, UK, 2000. [Google Scholar]
- Lyman, F.A. On the Conservation of Rothalpy in Turbomachines. ASME J. Turbomach. 1993, 115, 520–525. [Google Scholar] [CrossRef]
- Shao, D.W. Influence of Tip Clearance with Different Width on the Performance and Internal Fluid Flow of Centrifugal Impeller. J. Eng. Therm. Energy Power 2017, 32, 29–36+146. [Google Scholar] [CrossRef]
- Zhang, C.; Shi, J. Aerodynamic Design and Analysis of 20MW Class S-CO2 Cycle Centrifugal Compressor. J. Gas Turbine Technol. 2023, 65, 327–330+334. [Google Scholar] [CrossRef]
- Tian, Y.; Li, Y.; Hao, J.; Liu, J.; Kai, Y.; Yang, J.; Zhang, Z. Analysis of the Impact of Structural Parameters on the Performance of CO2 Centrifugal Compressor. Cryog. Eng. 2024, 5, 21–31. [Google Scholar] [CrossRef]
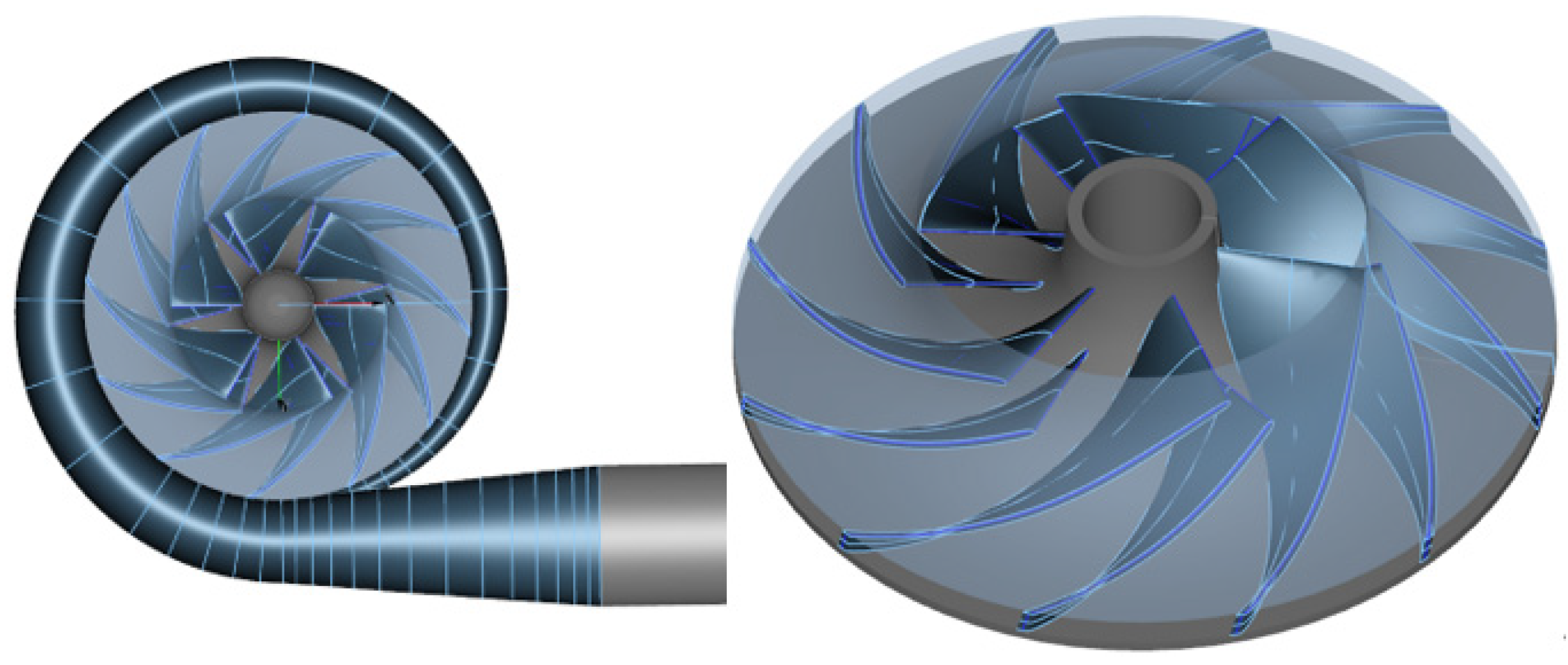




| Item | Parameters |
|---|---|
| Number of blades (main vane/shunt vane) | 6/6 |
| Blade outlet mounting angle | 40.1° |
| Impeller outlet width | 4 mm |
| Impeller outlet diameter | 110 mm |
| Tip clearance | 0.4 mm |
| Impeller inlet blade root diameter | 20 mm |
| Impeller inlet blade top diameter | 60 mm |
| Flow Rate (kg/s) | Section 1 | Section 2 | Section 3 | |||
|---|---|---|---|---|---|---|
| Min (J/kg) | Max (J/kg) | Min (J/kg) | Max (J/kg) | Min (J/kg) | Max (J/kg) | |
| 1.2 | 0.27982 | 456.416 | 0.018914 | 842.754 | 1.06374 | 1063.06 |
| 1.25 | 0.294949 | 309.635 | 0.020035 | 786.479 | 0.417929 | 793.432 |
| 1.3 | 0.306096 | 343.797 | 0.019535 | 707.702 | 0.433244 | 587.146 |
| 1.36 | 0.3441 | 290.132 | 0.020008 | 609.021 | 0.435938 | 600.269 |
| 1.4 | 0.285608 | 182.047 | 0.019188 | 405.969 | 0.435021 | 429.342 |
| 1.45 | 0.376257 | 220.174 | 0.019894 | 617.068 | 0.470167 | 315.719 |
| Flow Rate (kg/s) | Section 1 | Section 2 | Section 3 | |||
|---|---|---|---|---|---|---|
| Min [W/(m3·k)] | Max [W/(m3·k)] | Min [W/(m3·k)] | Max [W/(m3·k)] | Min [W/(m3·k)] | Max [W/(m3·k)] | |
| 1.2 | 1202.14 | 1.61 × 108 | 33.9084 | 1.62 × 108 | 2960.06 | 5.17 × 108 |
| 1.25 | 1352.12 | 1.30 × 108 | 34.6037 | 2.01 × 108 | 855.965 | 6.68 × 108 |
| 1.3 | 1435.8 | 1.66 × 108 | 38.4318 | 1.80 × 108 | 1012.05 | 4.93 × 108 |
| 1.36 | 1428.87 | 1.05 × 108 | 38.1503 | 2.09 × 108 | 938.577 | 4.15 × 108 |
| 1.4 | 1439.55 | 5.59 × 107 | 34.0638 | 5.91 × 107 | 1000.3 | 1.17 × 108 |
| 1.45 | 1910.04 | 5.78 × 107 | 43.337 | 5.75 × 107 | 1092.42 | 9.08 × 107 |
| Flow Rate (kg/s) | Section 1 | Section 2 | Section 3 | |||
|---|---|---|---|---|---|---|
| Min (J/kg) | Max (J/kg) | Min (J/kg) | Max (J/kg) | Min (J/kg) | Max (J/kg) | |
| 1.2 | 12,725.2 | 24,322.8 | 6436.21 | 24,429 | 5345 | 28,228.2 |
| 1.25 | 13,521 | 23,707.3 | 8930 | 23,222.4 | 8396.93 | 24,231.1 |
| 1.3 | 12,290 | 24,914.8 | 8436 | 23,633.7 | 7742 | 24,621.2 |
| 1.36 | 9151 | 25,027.1 | 7106 | 23,742.3 | 5890 | 25,197.4 |
| 1.4 | 13,668 | 23,690.6 | 14,183 | 23,008.1 | 12,443 | 23,240.3 |
| 1.45 | 13,436 | 24,230.3 | 8162 | 22,873.5 | 9072 | 23,148.4 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pei, Y.; Xue, Z.; Luo, X.; Zeng, L.; Xiao, X.; Xu, H.; Kou, L. Energy Characteristics of the Compressor in a Heat Pump Based on Energy Conversion Theory. Processes 2025, 13, 471. https://doi.org/10.3390/pr13020471
Pei Y, Xue Z, Luo X, Zeng L, Xiao X, Xu H, Kou L. Energy Characteristics of the Compressor in a Heat Pump Based on Energy Conversion Theory. Processes. 2025; 13(2):471. https://doi.org/10.3390/pr13020471
Chicago/Turabian StylePei, Yingju, Zhezhong Xue, Xu Luo, Lei Zeng, Xiaohua Xiao, Huyang Xu, and Li Kou. 2025. "Energy Characteristics of the Compressor in a Heat Pump Based on Energy Conversion Theory" Processes 13, no. 2: 471. https://doi.org/10.3390/pr13020471
APA StylePei, Y., Xue, Z., Luo, X., Zeng, L., Xiao, X., Xu, H., & Kou, L. (2025). Energy Characteristics of the Compressor in a Heat Pump Based on Energy Conversion Theory. Processes, 13(2), 471. https://doi.org/10.3390/pr13020471






