Numerical Simulation and Experimental Analysis of Mare’s Milk Sublimation Drying
Abstract
1. Introduction
2. Materials and Methods
2.1. Experimental Setup
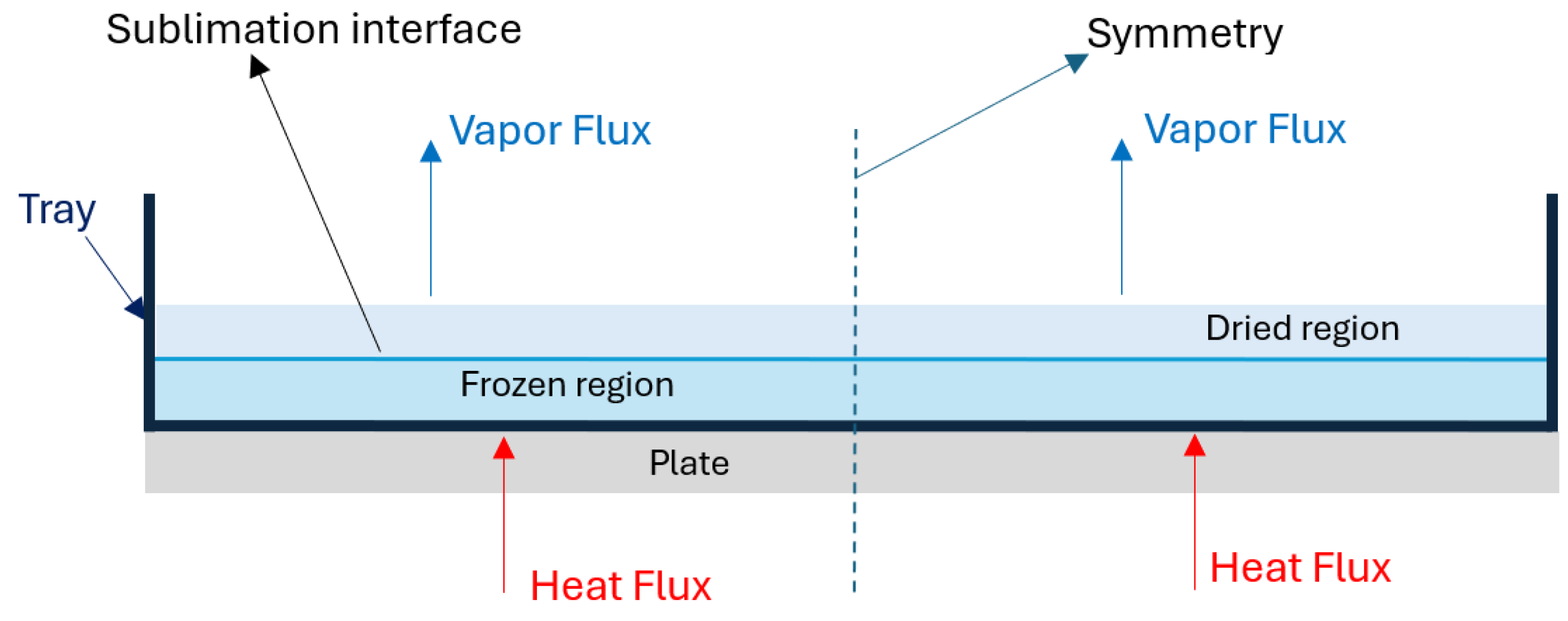
2.2. Numerical Modeling
- 1.
- Rigid Solid Matrix: The solid matrix structure of mare’s milk is considered rigid. This means that the matrix does not deform or change its structure during the sublimation drying process.
- 2.
- Infinitesimally Thin Interface: There exists a continuous, infinitesimally thin interface between the dried and frozen regions of the milk. This interface is used to track the progress of the sublimation front.
- 3.
- Water Vapor Transfer: Water vapor transfer in the dried region follows Darcy’s law. This implies that the movement of water vapor is driven by a pressure gradient, and the porous structure of the dried region is assumed to behave like a rigid porous medium.
- 4.
- Porous Matrix below Glass Transition: The porous matrix remains rigid as long as its temperature stays below the glass transition temperature. This assumption ensures that the physical structure of the dried region does not change during sublimation.
- 5.
- Thermal Properties Averaged by Composition: The thermal properties of mare’s milk (specific heat capacity, density, and thermal conductivity) are approximated using a weighted average of its individual components (water, fat, protein, lactose, and ash). This assumption is made due to the lack of precise data on the thermal properties of mare’s milk in the literature.
- :
- 1351.92 kg/
- :
- 2197.42 J/kg K
- :
- 0.350 W/m K
3. Results and Discussion
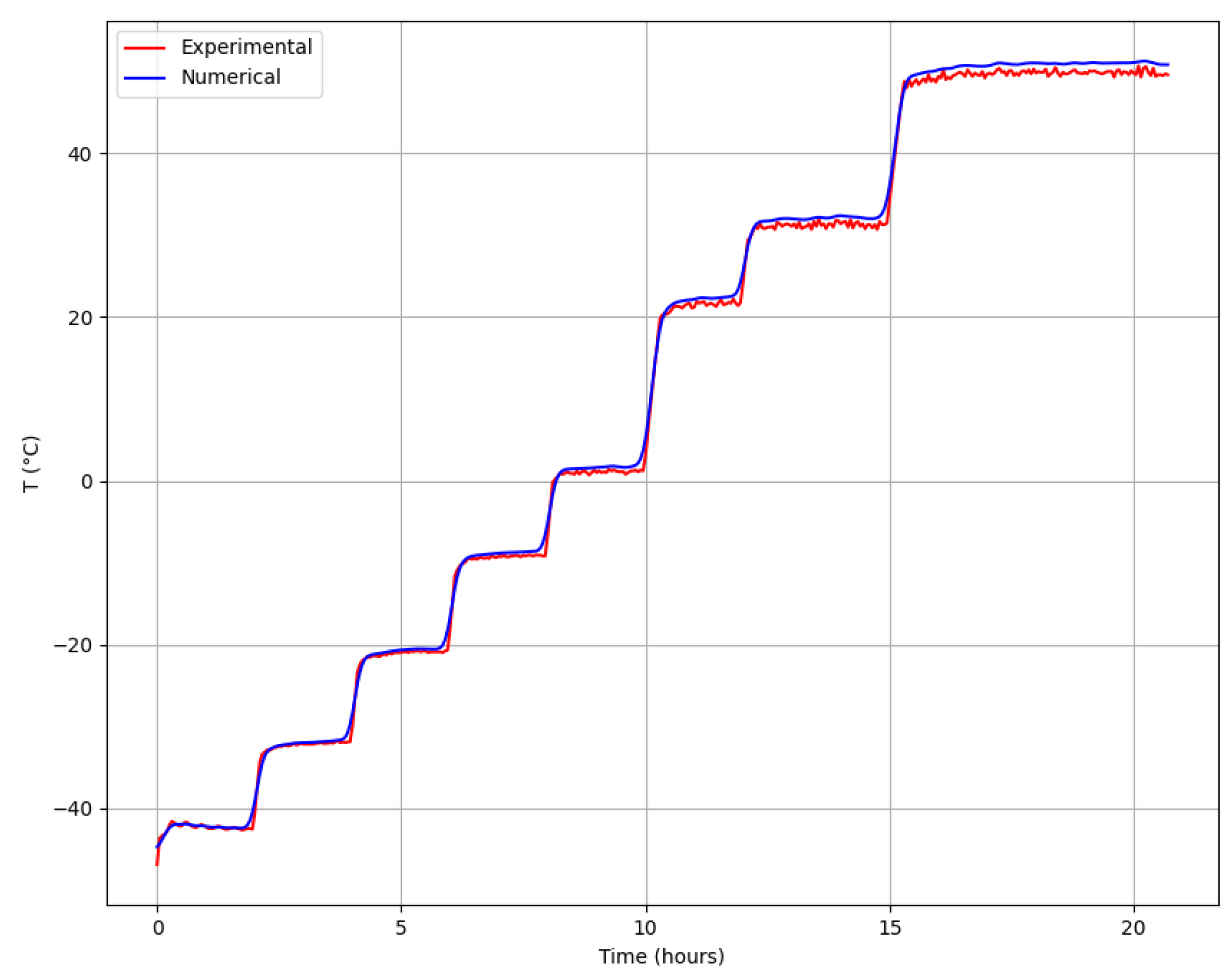
3.1. Experiment and Simulation Verification
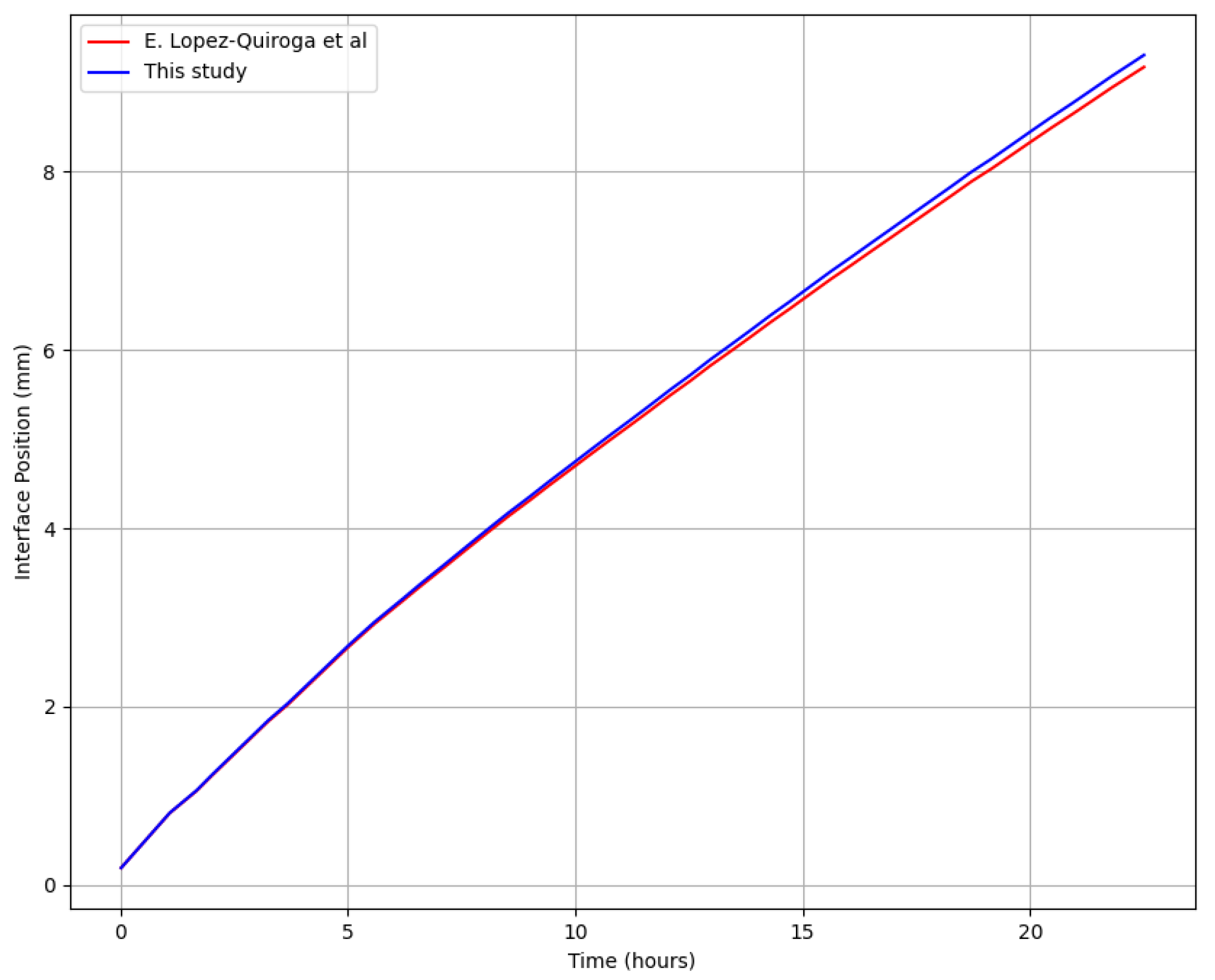
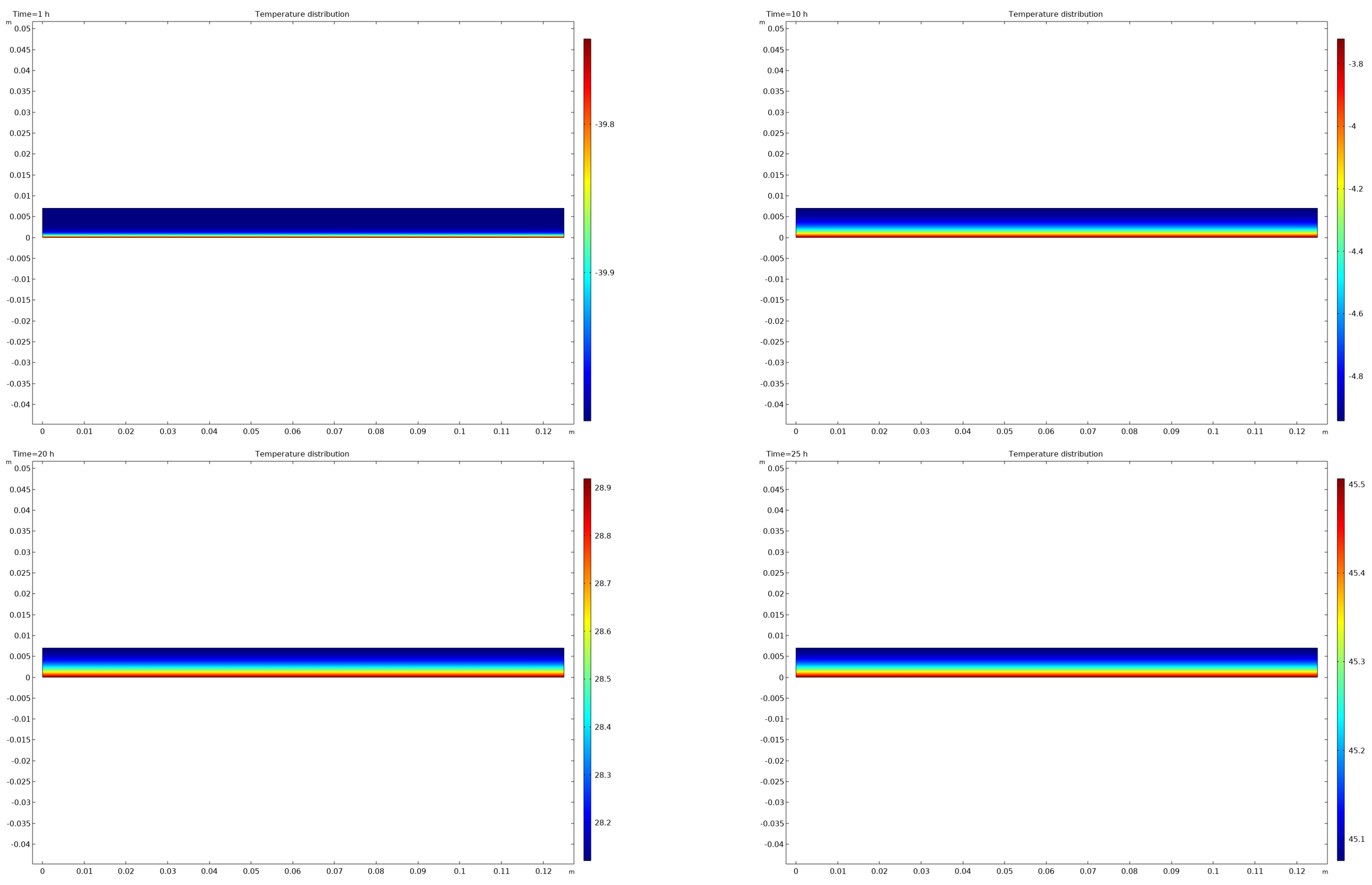
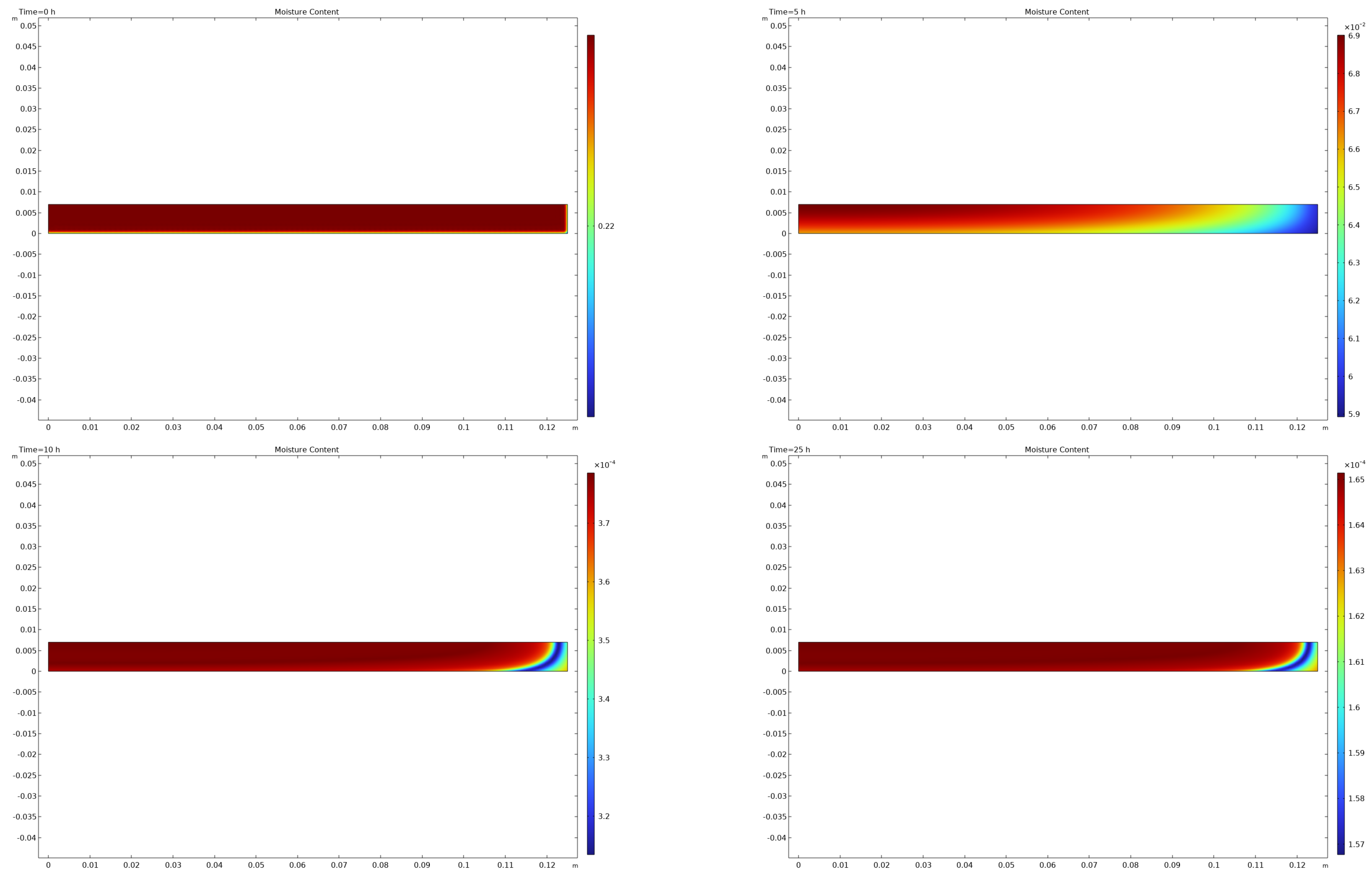
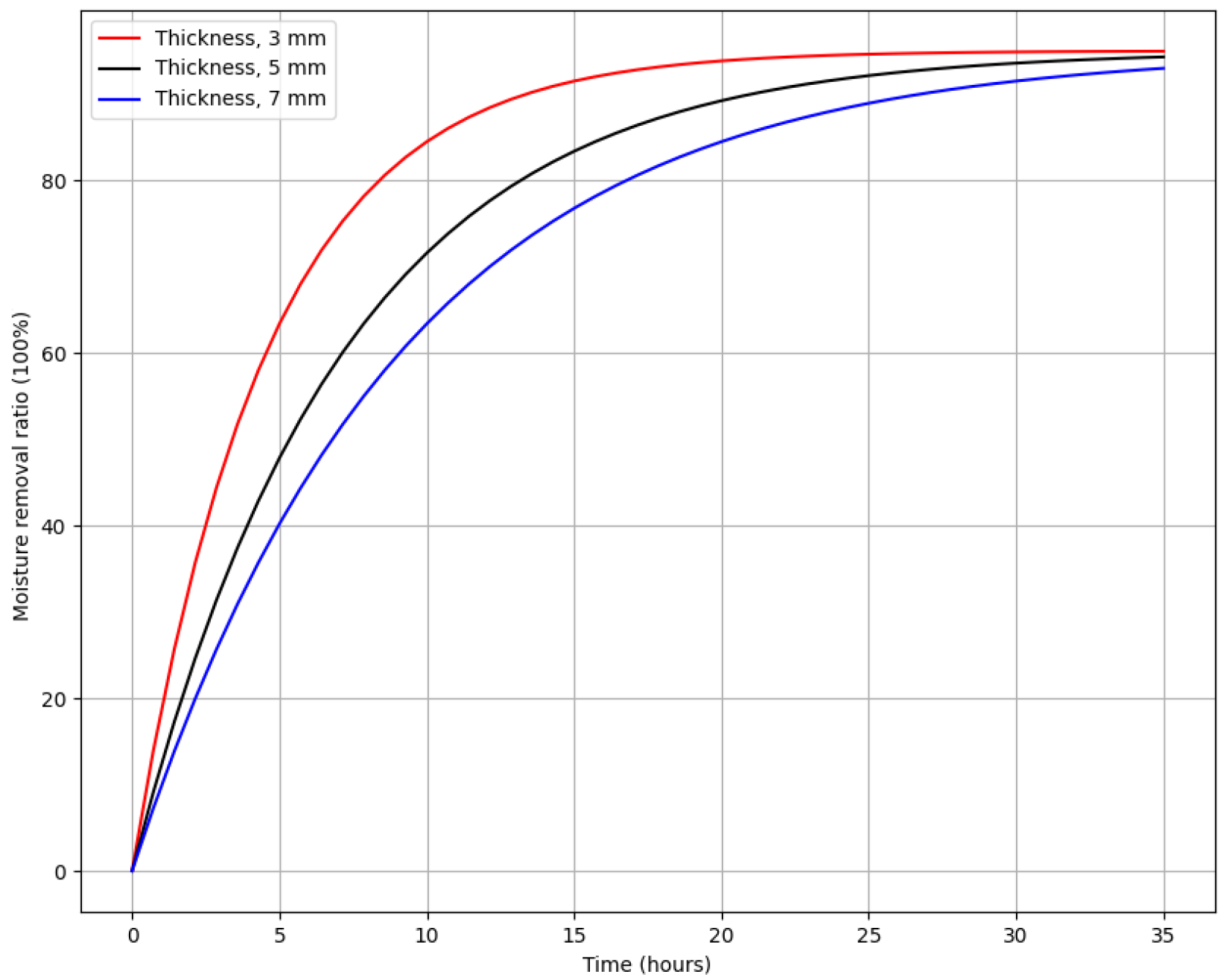
3.2. Sublimation Drying Process Analysis
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Pikal, M.J. Freeze Drying Encyclopedia of Pharmaceutical Technology, 3rd ed.; Taylor & Francis: London, UK, 2006; pp. 275–305. [Google Scholar]
- Musaev, A.; Sadykova, S.; Anambayeva, A.; Saizhanova, M.; Balkanay, G.; Kolbaev, M. Mare’s Milk: Composition, Properties, and Application in Medicine. Arch. Razi Inst. 2021, 76, 1125. [Google Scholar] [CrossRef] [PubMed]
- Kushugulova, A.; Kozhakhmetov, S.; Sattybayeva, R.; Nurgozhina, A.; Ziyat, A.; Yadav, H.; Marotta, F. Mare’s Milk as a Prospective Functional Product. Funct. Foods Health Dis. 2018, 8, 548. [Google Scholar] [CrossRef][Green Version]
- Ratti, C. Hot Air and Freeze-Drying of High-Value Foods: A Review. J. Food Eng. 2001, 49, 311–319. [Google Scholar] [CrossRef]
- Kremer, D.M.; Pikal, M.J.; Petre, W.J.; Shalaev, E.Y.; Gatlin, L.A.; Kramer, T. A Procedure to Optimize Scale-Up for the Primary Drying Phase of Lyophilization. J. Pharm. Sci. 2009, 98, 307–318. [Google Scholar] [CrossRef]
- Jastrzebska, E.; Wadas, E.; Daszkiewicz, T.; Pietrzak-Fiecko, R. Nutritional Value and Health-Promoting Properties of Mare’s Milk: A Review. Czech J. Anim. Sci. 2017, 62, 511–518. [Google Scholar] [CrossRef]
- Nastaj, J.F.; Witkiewicz, K. Mathematical Modeling of the Primary and Secondary Vacuum Freeze Drying of Random Solids at Microwave Heating. Int. J. Heat Mass Transf. 2009, 52, 4796–4806. [Google Scholar] [CrossRef]
- Nastaj, J.F.; Witkiewicz, K.; Wilczynska, B. Experimental and Simulation Studies of Primary Vacuum Freeze-Drying Process of Random Solids at Microwave Heating. Int. Commun. Heat Mass Transf. 2008, 35, 430–438. [Google Scholar] [CrossRef]
- Georgiev, K.; Kosturski, N.; Margenov, S.; Starý, J. On Adaptive Time Stepping for Large-Scale Parabolic Problems: Computer Simulation of Heat and Mass Transfer in Vacuum Freeze-Drying. J. Comput. Appl. Math. 2009, 226, 268–274. [Google Scholar] [CrossRef]
- Liapis, A.I.; Litchfield, R.J. Optimal Control of a Freeze Dryer-I: Theoretical Development and Quasi Steady State Analysis. Chem. Eng. Sci. 1979, 34, 975–981. [Google Scholar] [CrossRef]
- Liapis, A.I.; Litchfield, R.J. Numerical Solution of Moving Boundary Transport Problems in Finite Media by Orthogonal Collocation. Comput. Chem. Eng. 1979, 3, 615–621. [Google Scholar] [CrossRef]
- Tang, M.M.; Liapis, A.I.; Marchello, J.M. A Multi-Dimensional Model Describing the Lyophilization of a Pharmaceutical Product in a Vial. In Proceedings of the 5th International Drying Symposium, Cambridge, MA, USA, 13–16 August 1986; Mujumdar, A.S., Ed.; Hemisphere Publishing Corporation: New York, NY, USA, 1986; pp. 57–64. [Google Scholar]
- Mascarenhas, W.J.; Akay, H.U.; Pikal, M.J. A Computational Model for Finite Element Analysis of the Freeze-Drying Process. Comput. Methods Appl. Mech. Eng. 1997, 148, 105–124. [Google Scholar] [CrossRef]
- Scutella, B.; Plana-Fattori, A.; Passot, S.; Bourles, E.; Fonseca, F.; Flick, D.; Trelea, I.C. 3D Mathematical Modelling to Understand a Typical Heat Transfer Observed in Vial Freeze Drying. Appl. Therm. Eng. 2017, 126, 226–236. [Google Scholar] [CrossRef]
- Srinivasan, G.; Muneeshwaran, M.; Raja, B. Numerical Investigation of Heat and Mass Transfer Behavior of Freeze Drying of Milk in Vial. Heat Mass Transf. 2019, 55, 2073–2081. [Google Scholar] [CrossRef]
- Vilas, C.; Alonso, A.; Balsa-Canto, E.; López-Quiroga, E.; Trelea, I.C. Model-Based Real-Time Operation of the Freeze-Drying Process. Processes 2020, 8, 325. [Google Scholar] [CrossRef]
- Levin, P.; Buchholz, M.; Meunier, V.; Kessler, U.; Palzer, S.; Heinrich, S. Comparison of Knudsen Diffusion and the Dusty Gas Approach for the Modeling of the Freeze-Drying Process of Bulk Food Products. Processes 2022, 10, 548. [Google Scholar] [CrossRef]
- Shao, J.; Jiao, F.; Nie, L.; Wang, Y.; Du, Y.; Liu, Z. Study on Sublimation Drying of Carrot and Simulation by Using Cellular Automata. Processes 2023, 11, 2507. [Google Scholar] [CrossRef]
- Chen, B.-L.; Jang, J.-H.; Amani, M.; Yan, W.-M. Numerical and Experimental Study on the Heat and Mass Transfer of Kiwifruit during Vacuum Freeze-Drying Process. Alex. Eng. J. 2023, 73, 427–442. [Google Scholar] [CrossRef]
- Zhang, H.; Zhang, J.; He, L. Effects of the Sample’s Porous Structure on the Process of Vacuum Freeze Drying. In Proceedings of the 2010 4th International Conference on Bioinformatics and Biomedical Engineering, Chengdu, China, 18–20 June 2010. [Google Scholar] [CrossRef]
- Scutella, B.; Passot, S.; Bourles, E.; Fonseca, F.; Trelea, I.C. How Vial Geometry Influences Heat Transfer and Product Temperature during Freeze Drying. J. Pharm. Sci. 2016, 106, 770–778. [Google Scholar] [CrossRef]
- Altybay, A.; Ruzhansky, M.; Tokmagambetov, N. A parallel hybrid implementation of the 2D acoustic wave equation. Int. J. Nonlinear Sci. Numer. Simul. 2020, 21, 821–827. [Google Scholar] [CrossRef]
- Altybay, A.; Tokmagambetov, N. Numerical simulation and parallel computing of acoustic wave equation in isotropic heterogeneous media. CMES Comput. Model. Eng. Sci. 2024, 141, 1867–1881. [Google Scholar] [CrossRef]
- Altybay, A.; Darkenbayev, D.; Mekebayev, N. Parallel numerical simulation of the 2D acoustic wave equation. Int. J. Electr. Comput. Eng. (IJECE) 2024, 14, 6519–6525. [Google Scholar] [CrossRef]
- Experimental Setup. Available online: http://www.yuxiang17.com/productshow_161.html (accessed on 20 November 2024).
- Experimental Data. Available online: https://github.com/Arshynbek/Freeze-drying- (accessed on 20 November 2024).
- El-Maghlany, W.M.; Bedir, A.E.; Elhelw, M.; Attia, A. Freeze-Drying Modeling via Multi-Phase Porous Media Transport Model. Int. J. Therm. Sci. 2019, 135, 509–522. [Google Scholar] [CrossRef]
- Wang, W.; Hu, D.; Pan, Y.; Chen, G. Numerical Investigation on Freeze-Drying of Aqueous Material Frozen with Pre-Built Pores. Chin. J. Chem. Eng. 2016, 24, 116–125. [Google Scholar] [CrossRef]
- Nam, J.H.; Song, C.S. Numerical Simulation of Conjugate Heat and Mass Transfer during Multi-Dimensional Freeze Drying of Slab-Shaped Food Products. Int. J. Heat Mass Transf. 2007, 50, 4891–4900. [Google Scholar] [CrossRef]
- Warning, A.D.; Arquiza, J.M.R.; Datta, A.K. A Multiphase Porous Medium Transport Model with Distributed Sublimation Front to Simulate Vacuum Freeze Drying. Food Bioprod. Process. 2015, 94, 637–648. [Google Scholar] [CrossRef]
- Lopez-Quiroga, E.; Antelo, L.T.; Alonso, A.A. Time-Scale Modeling and Optimal Control of Freeze-Drying. J. Food Eng. 2012, 111, 655–666. [Google Scholar] [CrossRef]
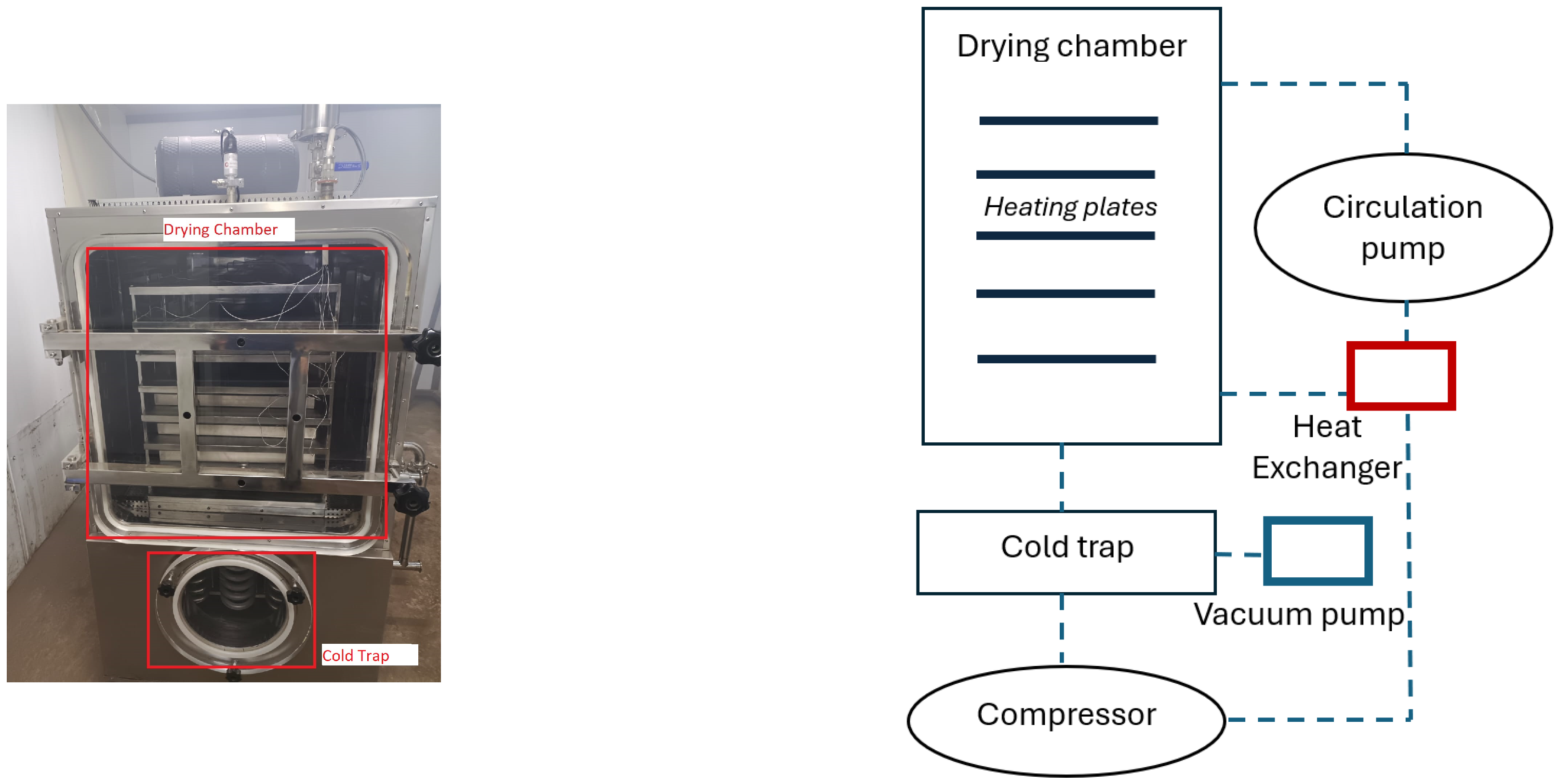

| Device | Specification |
|---|---|
| Drying chamber size | 900 mm × 2650 mm × 1580 mm |
| Shelf size (L × W × H) | 500 mm × 450 mm × 30 mm |
| Main power supply | 3-phase 5-wire 380 VAC 50 HZ |
| Cooling method | air cooling |
| Shelf temperature range | −50 °C∼70 °C |
| Cold trap coil temperature: | Minimum −75 °C |
| Pumping rate | 15 L/S |
| Ultimate vacuum degree | below 10 Pa (no load) |
| Parameter | Value | Units | Source |
|---|---|---|---|
| 0.85 | - | [28] | |
| 1351.92 | kg/ | ||
| 2197.42 | k · J/kg · K | ||
| W/m · K | |||
| 921 | kg/ | [28] | |
| 1.967 | kJ/kg · K | [28] | |
| 2.1 | W/m · K | [28] | |
| kg/ | [19] | ||
| 1.866 | kJ/kg · K | [29] | |
| 0.0022 | W/m · K | [29] | |
| kg/m · s | [29] | ||
| 0.018 | kg/mol | [27] | |
| R | 8.314 | J/mol · K | [27] |
| m2 | [30] | ||
| 2821 | kJ/kg | [29] |
| Parameter | Value |
|---|---|
| Total Elements | 1418 |
| Minimum Element Quality | 0.705 |
| Average Element Quality | 0.9052 |
| Element Area Ratio | 0.4022 |
| Mesh Area | 8.75 × m2 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rakhmatulina, A.; Altybay, A.; Imanbayeva, N.; Bagitova, S.; Baikonys, A. Numerical Simulation and Experimental Analysis of Mare’s Milk Sublimation Drying. Processes 2025, 13, 206. https://doi.org/10.3390/pr13010206
Rakhmatulina A, Altybay A, Imanbayeva N, Bagitova S, Baikonys A. Numerical Simulation and Experimental Analysis of Mare’s Milk Sublimation Drying. Processes. 2025; 13(1):206. https://doi.org/10.3390/pr13010206
Chicago/Turabian StyleRakhmatulina, Ayaulym, Arshyn Altybay, Nurbibi Imanbayeva, Saltanat Bagitova, and Anuar Baikonys. 2025. "Numerical Simulation and Experimental Analysis of Mare’s Milk Sublimation Drying" Processes 13, no. 1: 206. https://doi.org/10.3390/pr13010206
APA StyleRakhmatulina, A., Altybay, A., Imanbayeva, N., Bagitova, S., & Baikonys, A. (2025). Numerical Simulation and Experimental Analysis of Mare’s Milk Sublimation Drying. Processes, 13(1), 206. https://doi.org/10.3390/pr13010206






