An Investigation into the Creep Characteristics of Nylon Strings Used in Badminton Rackets
Abstract
1. Introduction
2. Materials and Methods
3. Results
3.1. Creep Model
3.2. The Effect of Processing Conditions on the Instantaneous Elastic Deformation Coefficient of Nylon Strings
3.3. The Effect of Processing Conditions on the Delayed Elastic Deformation Coefficient of Nylon Strings
3.4. The Effect of Processing Conditions on the Retardation Time of Nylon Strings
4. Conclusions
- (1)
- Highly accurate creep models were developed for nylon, all of which showed that the creep rate increased rapidly with time and then gradually became flat;
- (2)
- The instantaneous elastic deformation coefficient, delayed elastic deformation coefficient and retardation time all increased with increasing stress because of molecular chain movement and intermolecular forces;
- (3)
- With an increase in relative humidity, the instantaneous elastic deformation coefficient and delayed elastic deformation coefficient increased first and then decreased, while the retardation time of nylon decreased initially and then increased as the relative humidity rose;
- (4)
- In addition to the instantaneous elastic deformation coefficient, delayed elastic deformation coefficient and retardation time, there are other evaluation indicators, such as the delay time, tensile creep rate and viscosity coefficient. These can be further explored to describe the creep characteristics of nylon, especially under different processing conditions.
Funding
Data Availability Statement
Conflicts of Interest
References
- Collin, J.; Mackenzie, R. The World Cup, sport sponsorship, and health. Lancet 2006, 367, 1964–1966. [Google Scholar] [CrossRef] [PubMed]
- Wang, Z. A brief analysis of the origin, characteristics and technical training of badminton. Contemp. Sports Sci. Technol. 2012, 2, 28–31+69. [Google Scholar]
- Hastie, P.A.; Sinelnikov, O.A.; Guarino, A.J. The development of skill and tactical competencies during a season of badminton. Eur. J. Sport Sci. 2009, 9, 133–140. [Google Scholar]
- Nasruddin, F.A.; Syahrom, A.; Noor, H.M.; Abdul, K.M.R.; Hafidz, O.A. Finite-Element Study on Effect of String Tension Toward Coefficient of Restitution of a Badminton Racket String-Bed. Adv. Mater. Res. 2013, 845, 417–420. [Google Scholar]
- Ashofteh, R.S.; Khoramishad, H. The influence of hygrothermal ageing on creep behavior of nanocomposite adhesive joints containing multi-walled carbon nanotubes and graphene oxide nanoplatelets. Int. J. Adhes. Adhes. 2019, 94, 1–12. [Google Scholar]
- Qin, Z. Perceiving the affordance of string tension for power strokes in badminton: Expertise allows effective use of all string tensions. J. Sports Sci. 2013, 31, 1187–1196. [Google Scholar]
- Morishima, M.; Morikawa, A.; Gocho, H.; Jojima, E.; Shimizu, Y. Mechanical Property of Nylon 6 Fiber with Various Diameters in Standard, Wet and Water-Immersed Conditions. Seni Gakkaishi 2002, 58, 294–298. [Google Scholar] [CrossRef]
- Huynh, D.S.T.; Le, H.G.; Nguyen, T.N.H.; Minh, P.S. Enhancing the Fatigue Property of Nylon 6 by Using Glass-Fiber Reinforcement and Injection Molding. Mater. Sci. Forum 2019, 5939, 85–90. [Google Scholar] [CrossRef]
- Tang, H.; Wang, D.; Duan, Z. New Maxwell Creep Model Based on Fractional and Elastic-Plastic Elements. Adv. Civ. Eng. 2020, 2020, 9170706. [Google Scholar] [CrossRef]
- Junbao, W.; Tong, W.; Zhanping, S.; Yuwei, Z.; Qiang, Z. Improved Maxwell Model Describing the Whole Creep Process of Salt Rock and its Programming. Int. J. Appl. Mech. 2021, 13, 2150113. [Google Scholar]
- Wang, Y.; Pan, Z.; Zeng, B.; Xu, Q. Cyclic creep model of concrete based on Kelvin chain under fatigue loads. Constr. Build. Mater. 2024, 417, 135255. [Google Scholar]
- Songsong, S. Study on the creep behavior of recombinant bamboo based on a modified generalized Kelvin model. Wood Sci. Technol. 2022, 56, 589–601. [Google Scholar] [CrossRef]
- Cai, W.; Chen, W.; Xu, W. Characterizing the creep of viscoelastic materials by fractal derivative models. Int. J. Non-Linear Mech. 2016, 87, 58–63. [Google Scholar] [CrossRef]
- Shi, X.; Jiang, S.; Yang, L.; Tang, M.; Xiao, D. Modeling the viscoelasticity of shale by nanoindentation creep tests. Int. J. Rock Mech. Min. Sci. 2020, 127, 104210. [Google Scholar] [CrossRef]
- Wang, B.; Fancey, K.S. Viscoelastically prestressed polymeric matrix composites: An investigation into fibre deformation and prestress mechanisms. Compos. Part A Appl. Sci. Manuf. 2018, 111, 106–114. [Google Scholar]
- Neeraj, M.; Bharti, R.; Pooja, M.; Singh, B.B.; Mangala, J. Long-Term Prediction of Creep and Stress-Relaxation Behaviour in Synthetic Fabrics Using the Time—Temperature Superposition Principle. Fibers Polym. 2023, 24, 2195–2207. [Google Scholar]
- Mahdavipour, Z.; Jazini, F.; Karimi, M. Prediction of long-term creep response: Accelerated characterization of industrial nylon6,6 and HMLS PET filaments. J. Text. Inst. 2024, 1–10. [Google Scholar] [CrossRef]
- Habeger, C.C.; Coffin, D.W.; Hojjatie, B. Influence of humidity cycling parameters on the moisture-accelerated creep of polymeric fibers. J. Polym. Sci. Part B Polym. Phys. 2001, 39, 2048–2062. [Google Scholar] [CrossRef]
- Bell, G.A.; Bieliński, D.M.; Beake, B.D. Influence of water on the nanoindentation creep response of Nylon 6. J. Appl. Polym. Sci. 2008, 107, 577–582. [Google Scholar]
- Chevali, V.S.; Dean, D.R.; Janowski, G.M. Effect of environmental weathering on flexural creep behavior of long fiber-reinforced thermoplastic composites. Polym. Degrad. Stab. 2010, 95, 2628–2640. [Google Scholar]
- Xiaochao, D.; Bin, Y.; Tonghui, Y.; Yanpeng, W.; Hao, Y.; Tao, H. In Situ Polymerization of Nylon 66/Reduced Graphene Oxide Nanocomposites. J. Nanomater. 2018, 2018, 1047985. [Google Scholar]
- Iktisanov, V.A. Study of Rheological Properties of Liquid and Solidlike Materials Using Burgers’ Model. Colloid J. 2002, 64, 406–411. [Google Scholar] [CrossRef]
- Wang, X.; Wang, Y.; Qiang, H.; Bai, J.; Luo, C.; Zhao, Z. Initial stress approach-based finite element analysis for viscoelastic materials under generalized Maxwell model. AIP Adv. 2024, 14, 065132. [Google Scholar] [CrossRef]
- Vidal-Sallé, E.; Chassagne, P. Constitutive equations for orthotropic nonlinear viscoelastic behaviour using a generalized Maxwell model Application to wood material. Mech. Time-Depend. Mater. 2007, 11, 127–142. [Google Scholar] [CrossRef]
- Zhaolong, Z.; Dietrich, B.; Zhanwen, W.; Yinyue, Y.; Xiaolei, G. Frictional behaviour of wood-Plastic composites against cemented carbide during sliding contact. Wood Mater. Sci. Eng. 2023, 18, 1127–1133. [Google Scholar]
- Zhu, Y.; Buck, D.; Guan, J.; Song, M.; Tang, Q.; Guo, X.; Zhu, Z. Effects of Milling Methods on Cutting Performance of Wood–Plastic Composites Based on Principal Component Analysis. Forests 2024, 15, 1516. [Google Scholar] [CrossRef]

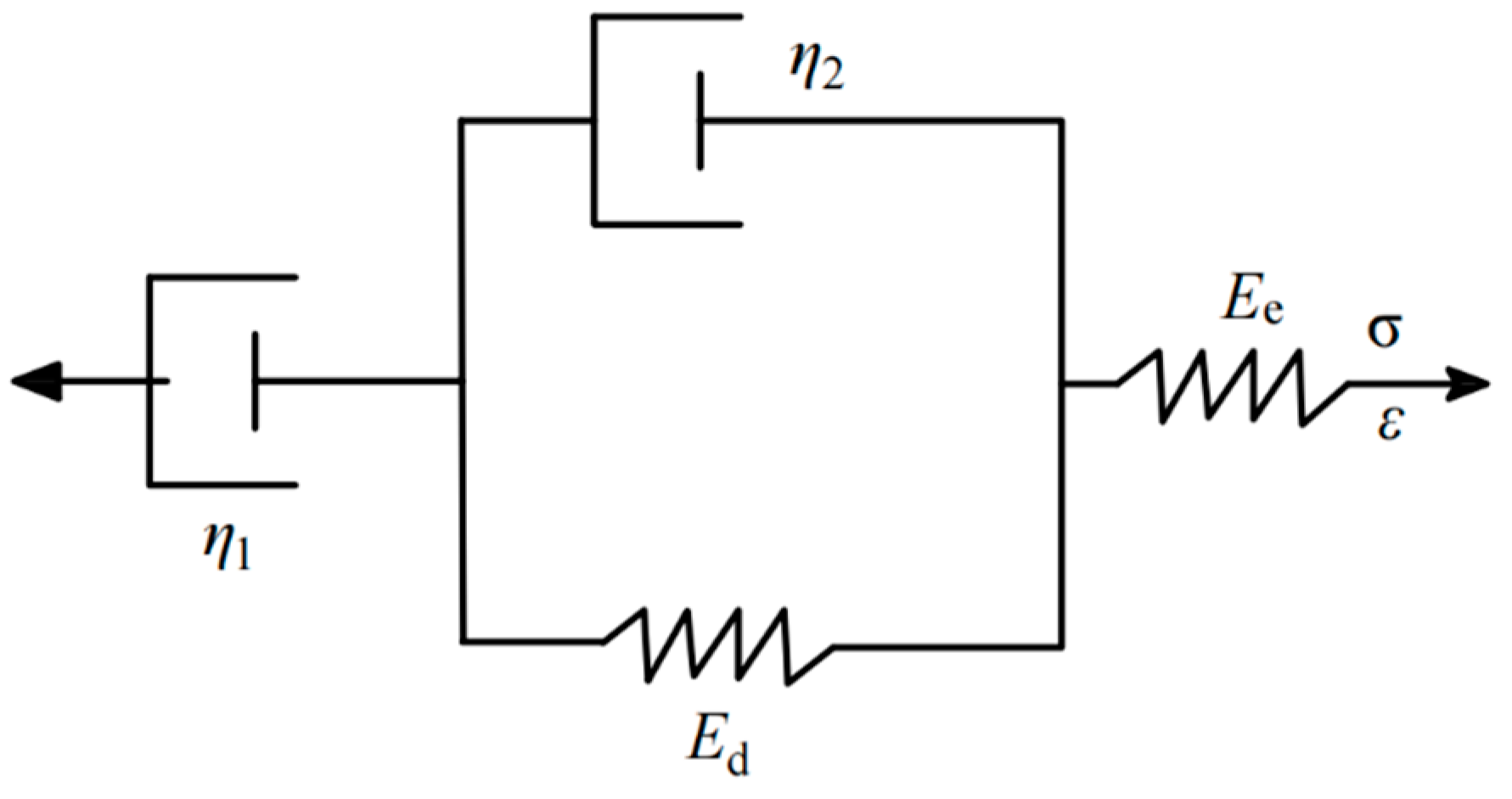
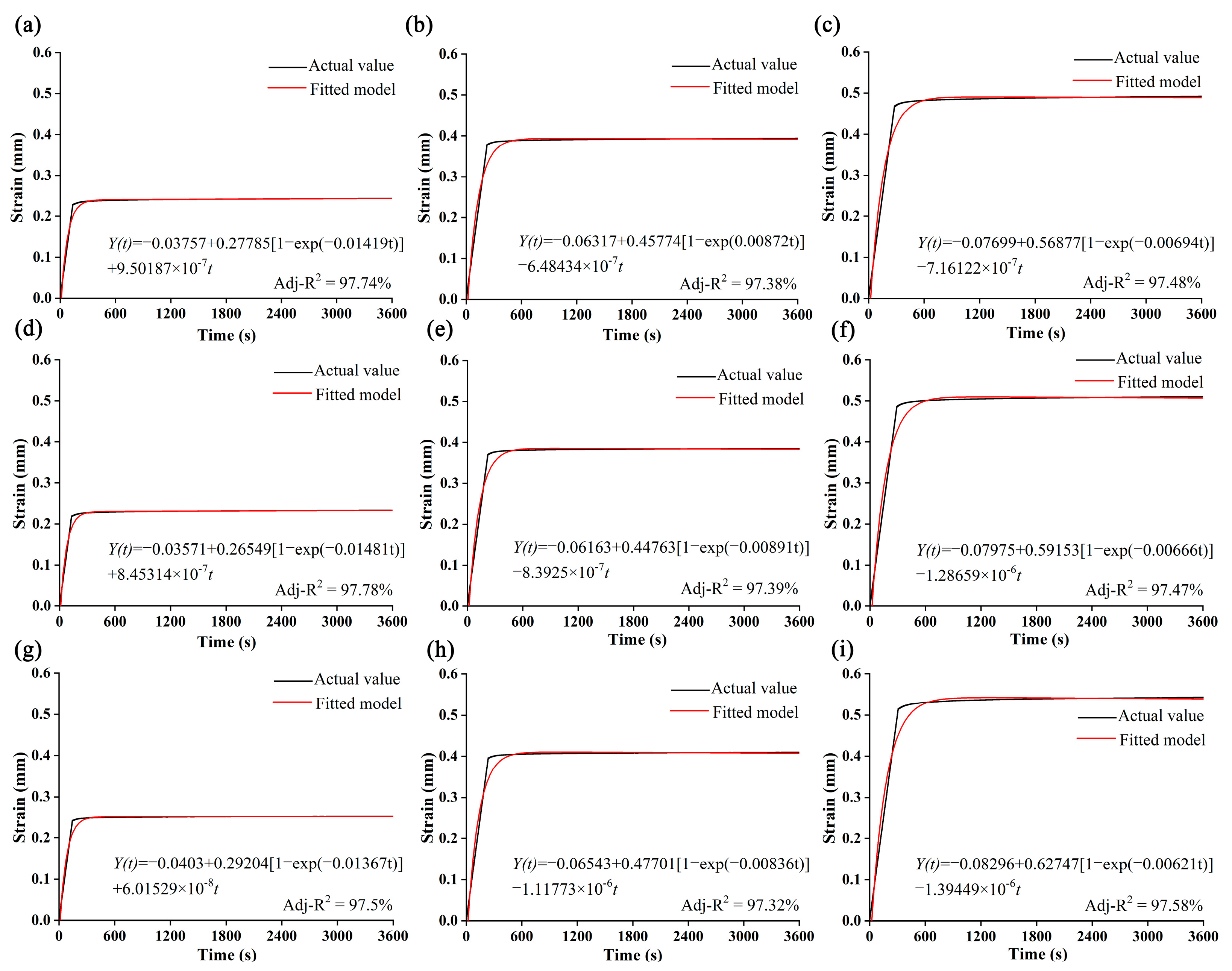



| No. | Relative Humidity | Stress Level |
|---|---|---|
| 1 | 30% | 20% |
| 2 | 30% | 40% |
| 3 | 30% | 60% |
| 4 | 60% | 20% |
| 5 | 60% | 40% |
| 6 | 60% | 60% |
| 7 | 90% | 60% |
| 8 | 90% | 60% |
| 9 | 90% | 60% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ji, F. An Investigation into the Creep Characteristics of Nylon Strings Used in Badminton Rackets. Processes 2024, 12, 2793. https://doi.org/10.3390/pr12122793
Ji F. An Investigation into the Creep Characteristics of Nylon Strings Used in Badminton Rackets. Processes. 2024; 12(12):2793. https://doi.org/10.3390/pr12122793
Chicago/Turabian StyleJi, Feng. 2024. "An Investigation into the Creep Characteristics of Nylon Strings Used in Badminton Rackets" Processes 12, no. 12: 2793. https://doi.org/10.3390/pr12122793
APA StyleJi, F. (2024). An Investigation into the Creep Characteristics of Nylon Strings Used in Badminton Rackets. Processes, 12(12), 2793. https://doi.org/10.3390/pr12122793





