Risk Assessment Method for Analyzing Borehole Instability Considering Formation Heterogeneity
Abstract
:1. Introduction
2. Prediction Model of Borehole Instability
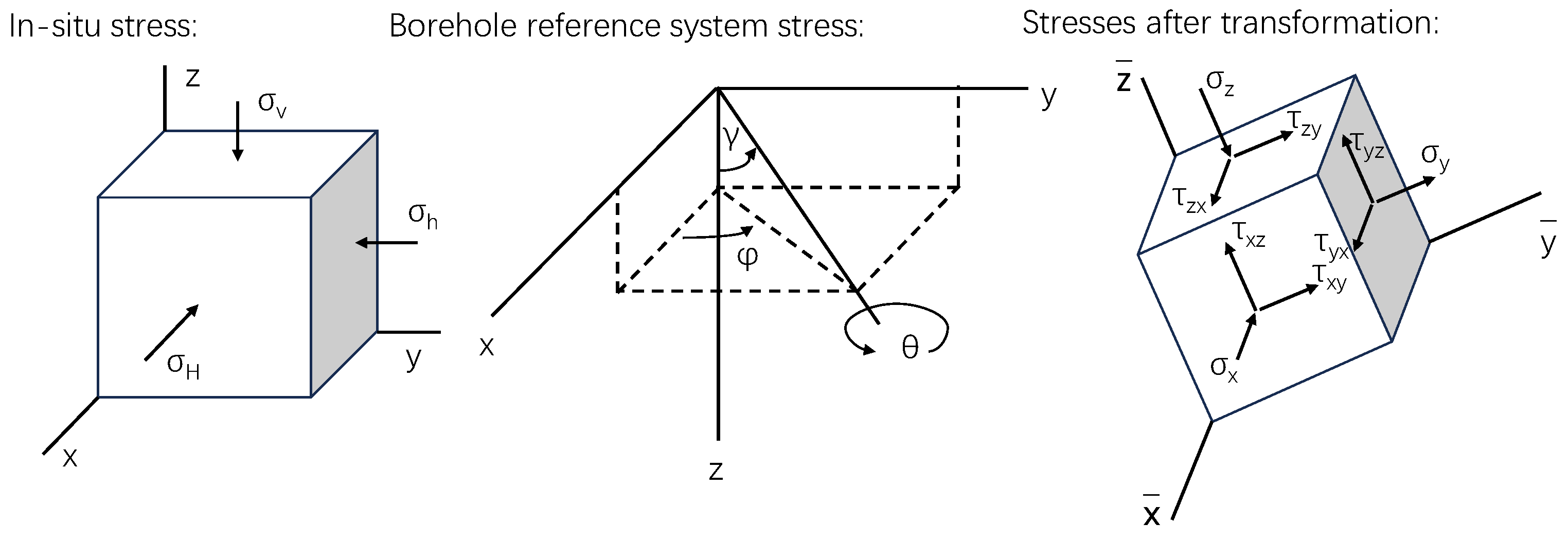
2.1. Stress Distribution around Borehole
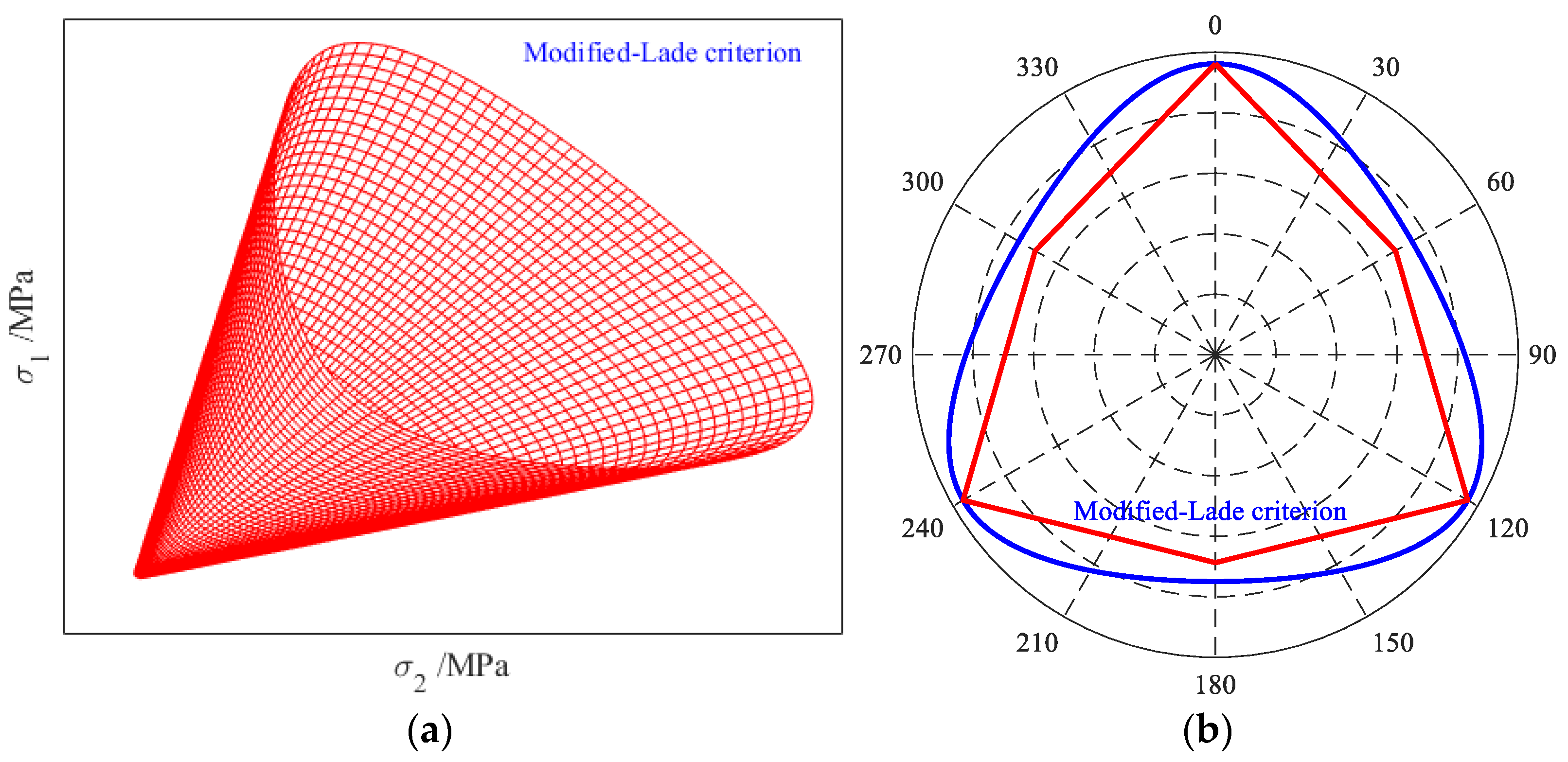
2.2. Strength Criterion
2.3. Model Solution
3. Quantitative Characterization of Stratigraphic Heterogeneity
4. Risk Analysis of Borehole Instability
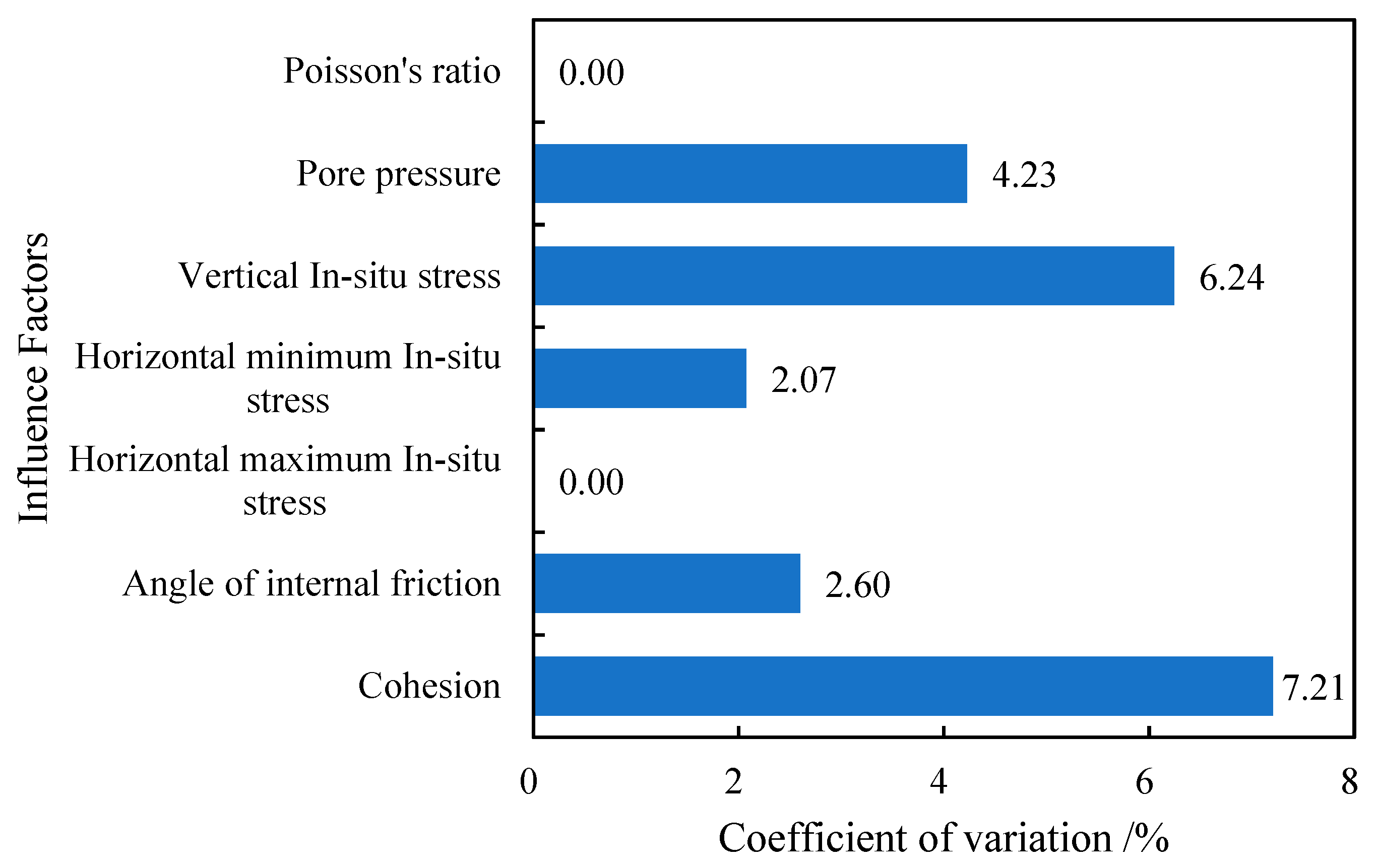
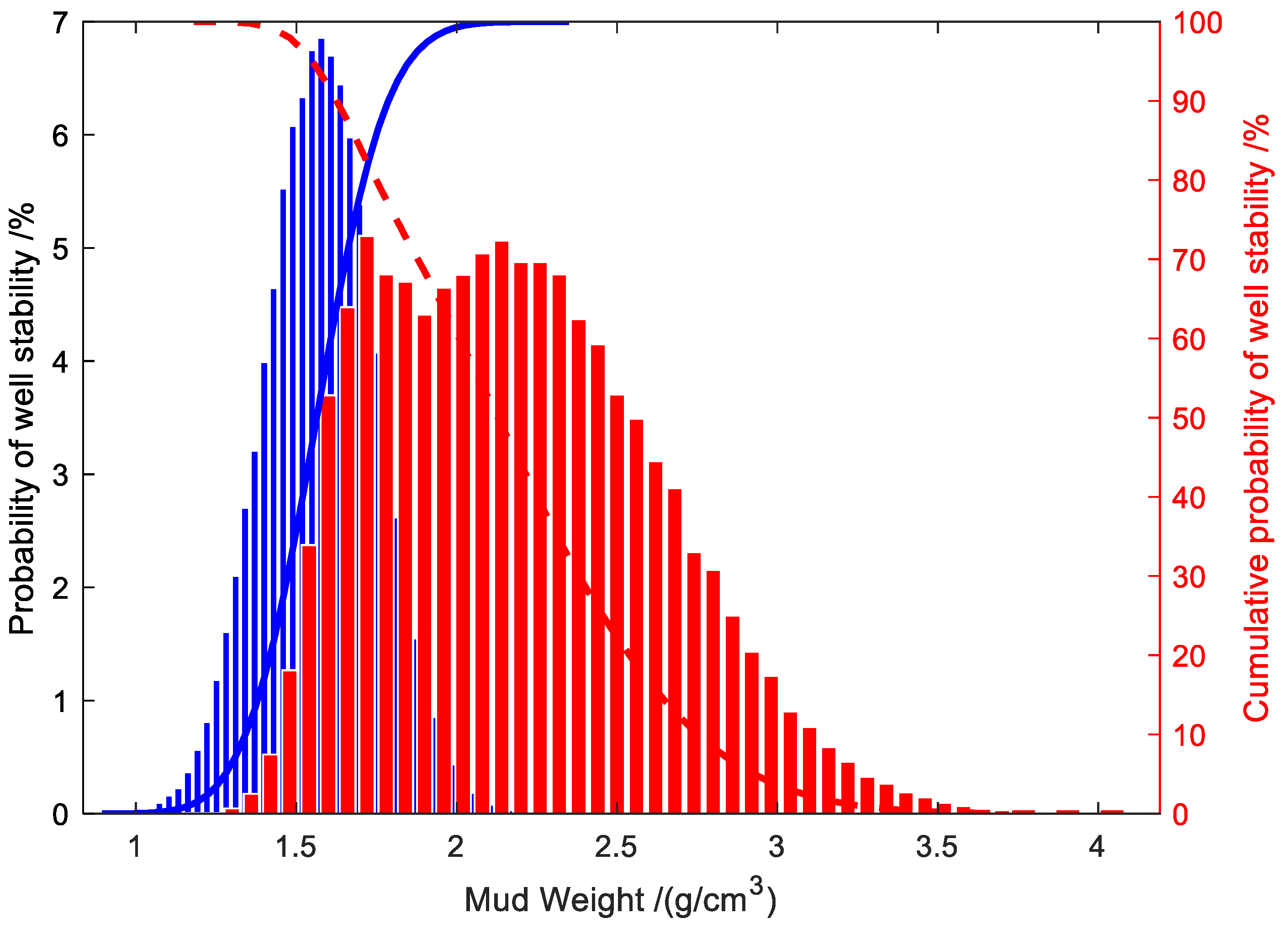
4.1. Influence of Single Parameter on the Risk of Borehole Instability
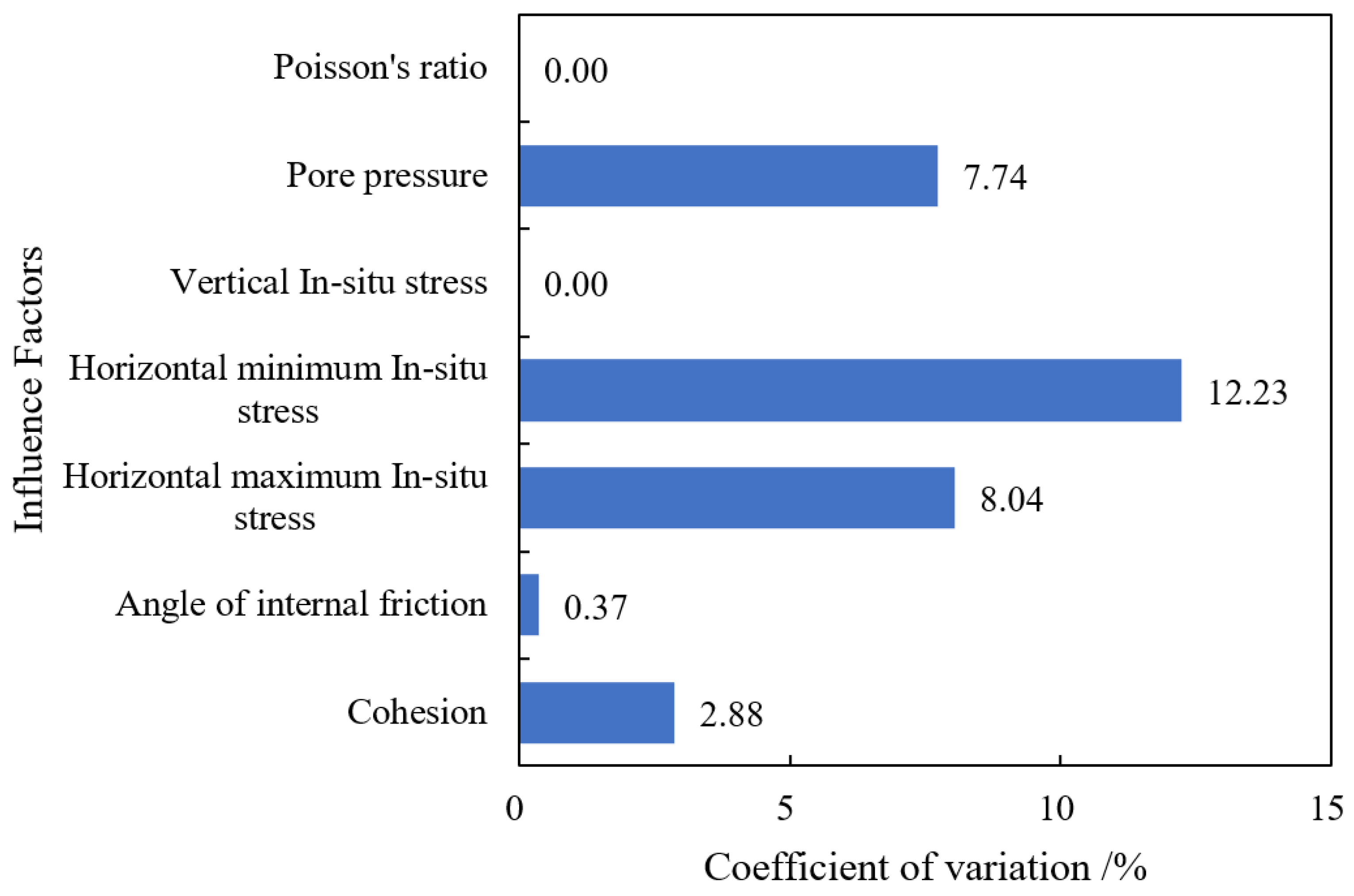
4.2. Influence of Multiple Parameters on the Risk of Borehole Instability
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Ebrahimi, M.A.; Ahmadi, M.; Ameri, M.J. Application of Unconditional Simulation Methods for Quantifying the Uncertainties in Mud Window Design of Gas Reservoirs Based on 3-Dimensional Mechanical Earth Modeling. J. Nat. Gas Sci. Eng. 2020, 76, 103186. [Google Scholar] [CrossRef]
- Denney, D. Safe Operating Window: Wellbore Stability is More Than Just Fluid Density. J. Pet. Technol. 2005, 57, 69–71. [Google Scholar] [CrossRef]
- Udegbunam, J.E.; Fjelde, K.K.; Arild, Ø.; Ford, E.; Lohne, H.P. Uncertainty-Based Approach for Predicting the Operating Window in UBO Well Design. SPE Drill. Complet. 2013, 28, 326–337. [Google Scholar] [CrossRef]
- Ma, T.S.; Zhang, Y.; Qiu, Y.; Liu, Y.; Chen, P. Risk Assessment Method for Instability of Inclined Well Lining Based on Reliability Theory. Acta Pet. Sin. 2021, 42, 1486–1498. [Google Scholar]
- Zhao, K.; Song, W.; Deng, J.; Tan, Q.; Wang, X. Evolution Law of Wellbore Instability Risk under Fluctuating Pressure. Energies 2023, 16, 2948. [Google Scholar] [CrossRef]
- Zhang, X.; Liu, J.; Sun, J.; Lv, K.; Wang, Z.; Xu, Z.; Sun, Y. Novel Modified Styrene-Based Microspheres for Enhancing the Performance of Drilling Fluids at High Temperatures. Gels 2023, 9, 763. [Google Scholar] [CrossRef] [PubMed]
- Zhang, M.; Li, D.; Liu, J.; Zhang, D.; Zhang, Y.; Cui, K. The Modification of Mohr-Coulomb Criteria Based on Shape Function and Determination Method of Undetermined Parameters. Mech. Mater. 2023, 185, 104772. [Google Scholar] [CrossRef]
- Cheng, Y.; Xue, M.; Shi, J.; Li, Y.; Yan, C.; Han, Z.; Yang, J. Numerical Simulating the Influences of Hydrate Decomposition on Wellhead Stability. Processes 2023, 11, 1586. [Google Scholar] [CrossRef]
- Al-Ajmi, A.; Zimmerman, R. A New 3D Stability Model for the Design of Non-Vertical Wellbores. In Proceedings of the 41st U.S. Symposium on Rock Mechanics (USRMS), Golden, CO, USA, 17–21 June 2006. [Google Scholar]
- Ewy, R.T. Wellbore-Stability Predictions by Use of a Modified Lade Criterion. SPE Drill. Complet. 1999, 14, 85–91. [Google Scholar] [CrossRef]
- Zhang, M.; Fan, X.; Zhang, Q.; Yang, B.; Zhao, P.; Yao, B.; He, L. Influence of Multi-Planes of Weakness on Unstable Zones Near Wellbore Wall in a Fractured Formation. J. Nat. Gas Sci. Eng. 2021, 93, 104026. [Google Scholar] [CrossRef]
- Zhang, M.; Fan, X.; Zhang, Q.; Yang, B.; Zhao, P.; Yao, B.; He, L. Study on Wellbore Stability of Multilateral Wells under Seepage-Stress Coupling Condition Based on Finite Element Simulation. Processes 2023, 11, 1651. [Google Scholar]
- Yang, B.; Xu, H. Analysis of Bottomhole Rock Stress in Deep-Well Drilling Considering Thermal-Hydro-Mechanical Coupling. Processes 2023, 11, 683. [Google Scholar] [CrossRef]
- Zhu, H.; Qi, Y.; Hu, H.; Zhang, F.; Jing, C.; Zhao, J. A Wellbore Pressure Control Method for Two-Layer Coal Seam Gas Coproduction Wells. Energies 2023, 16, 7148. [Google Scholar] [CrossRef]
- Feng, Y.; Tang, H.; Tang, H.; Leng, Y.; Shi, X.; Liu, J. Experimental Investigation of Stress Sensitivity of Elastic Wave Velocities for Anisotropic Shale in Wufeng–Longmaxi Formation. Processes 2023, 11, 2607. [Google Scholar] [CrossRef]
- Liu, W.; Qu, Z. Strain Field Evolution Analysis of Brittle Shale with Initial Fractures Based on DIC. Processes 2023, 11, 2319. [Google Scholar] [CrossRef]
- Chen, F.; Gao, J.; Feng, Y.; Lin, H.; Zhang, B.; Bian, G.; Yang, W.; Ouyang, H. Optimizing the Wellbore Trajectory of Directional Wells Considering Wellbore Stability Subjected to the Non-Independence and Uncertainty of Geomechanical Parameters. In Proceedings of the SPE Asia Pacific Unconventional Resources Conference and Exhibition, Brisbane, Australia, 14–15 November 2023; SPE: Kuala Lumpur, Malaysia, 2023. [Google Scholar]
- Galkin, V.I.; Martyushev, D.A.; Ponomareva, I.N.; Chernykh, I.A. Developing features of the near-bottomhole zones in productive formations at fields with high gas saturation of formation oil. J. Min. Inst. 2021, 249, 386–392. [Google Scholar] [CrossRef]
- Popov, S.N.; Chernyshov, S.E.; Krivoshchekov, S.N. Comparative analysis of the analytical and numerical methods for calculating the stress-strain state of the near-wellbore zone based on the elastic model taking into account the main structural elements of the well. Bull. Tomsk. Polytech. Univ. Geo Assets Eng. 2023, 334, 94–102. [Google Scholar] [CrossRef]
- Chen, Y.J.; Deng, C.G.; Ma, T.S. Reliability Theory Evaluation Method for Wellbore Instability Risk. Nat. Gas Ind. 2019, 39, 97–104. [Google Scholar]
- Wei, K.; Guan, Z.C.; Liao, H.L.; Shi, Y.C.; Liu, Y.W. Risk Assessment Method for Wellbore Instability. J. China Univ. Pet. Ed. Nat. Sci. 2013, 37, 62–66. [Google Scholar]
- Zhao, L.P.; Wang, Q.; Guo, Z.; Fang, C.; Cao, H. Evaluation of Wellbore Stability in Bedded Shale Formation Based on Limited Collapse Width. Sci. Technol. Rev. 2020, 38, 122–130. [Google Scholar]
- Sheng, Y.N.; Guan, Z.C.; Xu, Y.Q.; Wang, Q.; Zhang, B. Discussion on Uncertainty Analysis Method for Wellbore Stability Problems. Fault-Block Oil Gas Field 2017, 24, 847–850. [Google Scholar]
- Liu, X.; Yi, R.; Zhou, X.; Wang, R. Application of Bayesian Network Method in Optimization of Gas Well Completion Methods. In Proceedings of the 2018 National Natural Gas Academic Annual Conference (04 Engineering Technology), Washington, DC, USA, 25–29 June 2018. [Google Scholar]
- Xia, P.F. Study on Impact Crushing Law and Safety Analysis of Open-Hole Wellbore in Shale Gas Wells. Master’s Thesis, China University of Petroleum (East China), Qingdao, China, 2018. [Google Scholar]
- Ai, E.X. Research on Early Warning Theory and Method of Shale Wellbore Instability. Master’s Thesis, Xi’an Shiyou University, Xi’an, China, 2015. [Google Scholar]
- Al-Ajmi, A.M.; Al-Harthy, M.H. Probabilistic Wellbore Collapse Analysis. J. Pet. Sci. Eng. 2010, 74, 171–177. [Google Scholar] [CrossRef]
- De Fontoura, S.A.; Holzberg, B.B.; Teixeira, É.C.; Frydman, M. Probabilistic Analysis of Wellbore Stability during Drilling. In SPE/ISRM Rock Mechanics Conference; OnePetro: Richardson, TX, USA, 2002. [Google Scholar]
- Wen, Q.Y. Research on Risk Assessment of Drilling Systems in Deep Wells with Complex Formations Based on Soft Computing Theory. Ph.D. Dissertation, China University of Petroleum (East China), Qingdao, China, 2012. [Google Scholar]
- Hilgedick, S.A. Investigation of Wellbore Stability in a North Sea Field Development; Missouri University of Science and Technology: Rolla, MO, USA, 2012. [Google Scholar]
- Li, W.Q. Wellbore Stability Analysis and Risk Assessment Based on Lithological Parameter Uncertainty; China University of Petroleum (East China): Qingdao, China, 2020. [Google Scholar]
- Adams, A.J.; Parfitt, S.H.L.; Reeves, T.B.; Thorogood, J.L. Casing System Risk Analysis Using Structural Reliability. In Proceedings of the SPE/IADC Drilling Conference, Amsterdam, The Netherlands, 22–25 February 1993. [Google Scholar]
- Nilsen, T.; Sandoy, M.; Rommetveit, R.; Guarneri, A. Risk-Based Well Control Planning: The Integration of Random and Known Quantities in a Computerized Risk Management Tool. In Proceedings of the SPE/ICoTA Coiled Tubing Roundtable; Society of Petroleum Engineers, Houston, TX, USA, 7–8 March 2001. [Google Scholar]
- Payne, M.L.; Swanson, J.D. Application of Probabilistic Reliability Methods to Tubular Designs. SPE Drill. Eng. 1990, 5, 299–305. [Google Scholar] [CrossRef]
- Tabatabaee, M.S.S.; Nikolaev, N.; Khormali, A. A Comprehensive Uncertainty Assessment of Wellbore Stability Models. In Proceedings of the Saint Petersburg 2018: Innovations in Geosciences & Time for Breakthrough, St. Petersburg, FL, USA, 9–12 April 2018; p. 44298. [Google Scholar]
- Sheng, Y.; Reddish, D.; Lu, Z. Assessment of Uncertainties in Wellbore Stability Analysis. In Modern Trends in Geomechanics; Springer: Berlin/Heidelberg, Germany, 2006; pp. 541–557. [Google Scholar]
- Huang, Y.; Sheng, Y.N.; Guan, Z.C. Quantitative Risk Assessment of Drilling Wellbore Stability in Yingqiong Basin. Fault-Block Oil Gas Field 2019, 26, 380–384. [Google Scholar]
- Moos, D.; Peska, P.; Finkbeiner, T.; Zoback, M. Comprehensive Wellbore Stability Analysis Utilizing Quantitative Risk Assessment. J. Pet. Sci. Eng. 2003, 38, 97–109. [Google Scholar] [CrossRef]
- Guan, Z.C.; Sheng, Y.N. Study on Evaluation Method for Wellbore Stability Based on Uncertainty Analysis. J. Appl. Sci. Eng. 2017, 20, 453–457. [Google Scholar]
- Sheng, Y.N.; Guan, Z.C.; Luo, M. Sensitivity Analysis of Random Variables for Wellbore Stability Reliability Based on Monte Carlo Method. Pet. Drill. Tech. 2018, 40, 14–19. [Google Scholar]
- Qiu, K.; Chen, M.; Jin, Y. A Wellbore Collapse Pressure Model Based on Statistical Damage. Rock Soil Mech. 2011, 32, 2029–2033. [Google Scholar]
- Bai, G.B. Optimization Method for Drilling Fluid Density Design in Shale Gas Wells of Wei 202 and 204 Blocks. Drill. Fluid Complet. Fluid 2020, 37, 196–201. [Google Scholar]
- Lu, Y.H.; Xiao, X.H.; Zhao, L. Influence of Temperature on Wellbore Stability in Ultra-Deep Fractured Formations. Drill. Fluid Complet. Fluid 2020, 37, 160–167. [Google Scholar]
- Noohnejad, A.; Ahangari, K.; Goshtasbi, K. Integrated Mechanical Earth Model and Quantitative Risk Assessment to Successful Drilling. J. Pet. Explor. Prod. 2021, 11, 219–231. [Google Scholar] [CrossRef]
- Li, X.; Sun, B.; Ma, B.; Li, H.; Liu, H.; Cai, D.; Wang, X.; Li, X. Study on the Evolution Law of Wellbore Stability Interface during Drilling of Offshore Gas Hydrate Reservoirs. Energies 2023, 16, 7585. [Google Scholar] [CrossRef]
- Zhang, M.; Fan, X.; Zhang, Q.; Yang, B.; Zhao, P.; Yao, B.; Ran, J. Parametric Sensitivity Study of Wellbore Stability in Transversely Isotropic Medium Based on Poly-Axial Strength Criteria. J. Pet. Sci. Eng. 2020, 197, 108078. [Google Scholar] [CrossRef]
- Ye, Y.; Song, H.; Zhu, J.; Zheng, W.; Zhou, F.; Zhou, G.; Zhang, Q. Mechanism Study and Performance Evaluation of Nano-Materials Used to Improve Wellbore Stability. Sustainability 2023, 15, 5530. [Google Scholar] [CrossRef]
- Tran, N.H.; Do, D.P.; Vu, M.N.; Nguyen, T.T.N.; Pham, D.T.; Trieu, H.T. Combined effect of anisotropy and uncertainty on the safe mud pressure window of horizontal wellbore drilled in anisotropic saturated rock. Int. J. Rock Mech. Min. Sci. 2022, 152, 105061. [Google Scholar] [CrossRef]




| Inputting Parameters | Cohesion/MPa | Internal Friction Angle/° | Horizontal Maximum In Situ Stress/MPa | Horizontal Minimum In Situ Stress/MPa | Vertical In Situ Stress/MPa | Pore Pressure/MPa | Poisson’s Ratio |
|---|---|---|---|---|---|---|---|
| Mean value | 6.38 | 30.42 | 30.54 | 23.32 | 35.5 | 17.15 | 0.25 |
| Standard deviation | 2 | 2 | 2 | 2 | 2 | 2 | 2 |
| Well Type | Vertical Well | Horizontal Well | ||||
|---|---|---|---|---|---|---|
| Influencing Factors | Mean Value (g/cm3) | Standard Deviation (g/cm3) | Coefficient of Variation (%) | Mean Value (g/cm3) | Standard Deviation (g/cm3) | Coefficient of Variation (%) |
| Cohesion | 1.331 | 0.115 | 8.613 | 1.577 | 0.114 | 7.209 |
| Internal friction angle | 1.333 | 0.026 | 1.986 | 1.577 | 0.041 | 2.599 |
| Horizontal maximum in situ stress | 1.37 | 0.192 | 7.34 | 1.5 | 0.292 | 0.000 |
| Horizontal minimum in situ stress | 1.333 | 0.033 | 2.45 | 1.576 | 0.033 | 2.073 |
| Vertical in situ stress | 1.332 | 0 | 0 | 1.576 | 0.098 | 6.243 |
| Pore pressure | 1.333 | 0.067 | 5.023 | 1.576 | 0.067 | 4.227 |
| Poisson’s ratio | 1.127 | 0 | 0 | 1.126 | 0.00004 | 0.004 |
| Well Type | Vertical Well | Horizontal Well | ||||
|---|---|---|---|---|---|---|
| Influencing Factors | Mean Value (g/cm3) | Standard Deviation (g/cm3) | Coefficient of Variation (%) | Mean Value (g/cm3) | Standard Deviation (g/cm3) | Coefficient of Variation (%) |
| Cohesion | 1.63 | 0.05 | 2.88 | 1.74 | 0.19 | 10.63 |
| Internal friction angle | 1.63 | 0.01 | 0.37 | 1.74 | 0.02 | 1.38 |
| Horizontal maximum in situ stress | 1.62 | 0.3 | 8.04 | 1.74 | 0 | 0 |
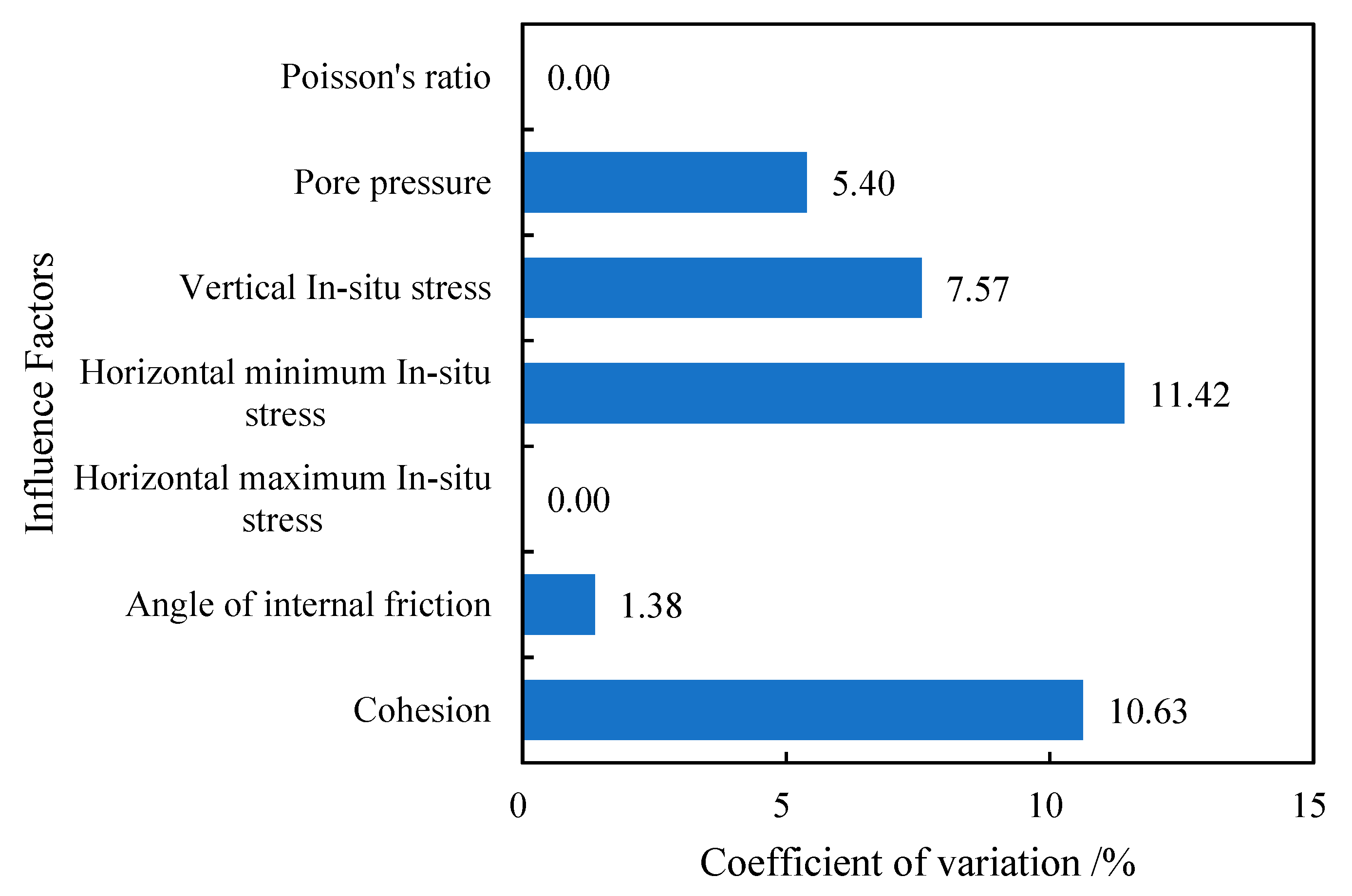
| Horizontal minimum in situ stress | 1.63 | 0.2 | 12.23 | 1.75 | 0.2 | 11.42 |
| Vertical in situ stress | 1.63 | 0 | 0 | 1.74 | 0.13 | 7.57 |
| Pore pressure | 1.63 | 0.13 | 7.74 | 1.74 | 0.09 | 5.4 |
| Poisson’s ratio | 1.63 | 0 | 0 | 1.74 | 0 | 0 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gao, X.; Wang, M.; Shi, X.; Li, C.; Zhang, M. Risk Assessment Method for Analyzing Borehole Instability Considering Formation Heterogeneity. Processes 2024, 12, 70. https://doi.org/10.3390/pr12010070
Gao X, Wang M, Shi X, Li C, Zhang M. Risk Assessment Method for Analyzing Borehole Instability Considering Formation Heterogeneity. Processes. 2024; 12(1):70. https://doi.org/10.3390/pr12010070
Chicago/Turabian StyleGao, Xiangsen, Min Wang, Xian Shi, Cui Li, and Mingming Zhang. 2024. "Risk Assessment Method for Analyzing Borehole Instability Considering Formation Heterogeneity" Processes 12, no. 1: 70. https://doi.org/10.3390/pr12010070
APA StyleGao, X., Wang, M., Shi, X., Li, C., & Zhang, M. (2024). Risk Assessment Method for Analyzing Borehole Instability Considering Formation Heterogeneity. Processes, 12(1), 70. https://doi.org/10.3390/pr12010070









