Numerical Study of a Heat Exchanger with a Rotating Tube Using Nanofluids under Transitional Flow
Abstract
1. Introduction
2. Numerical Model
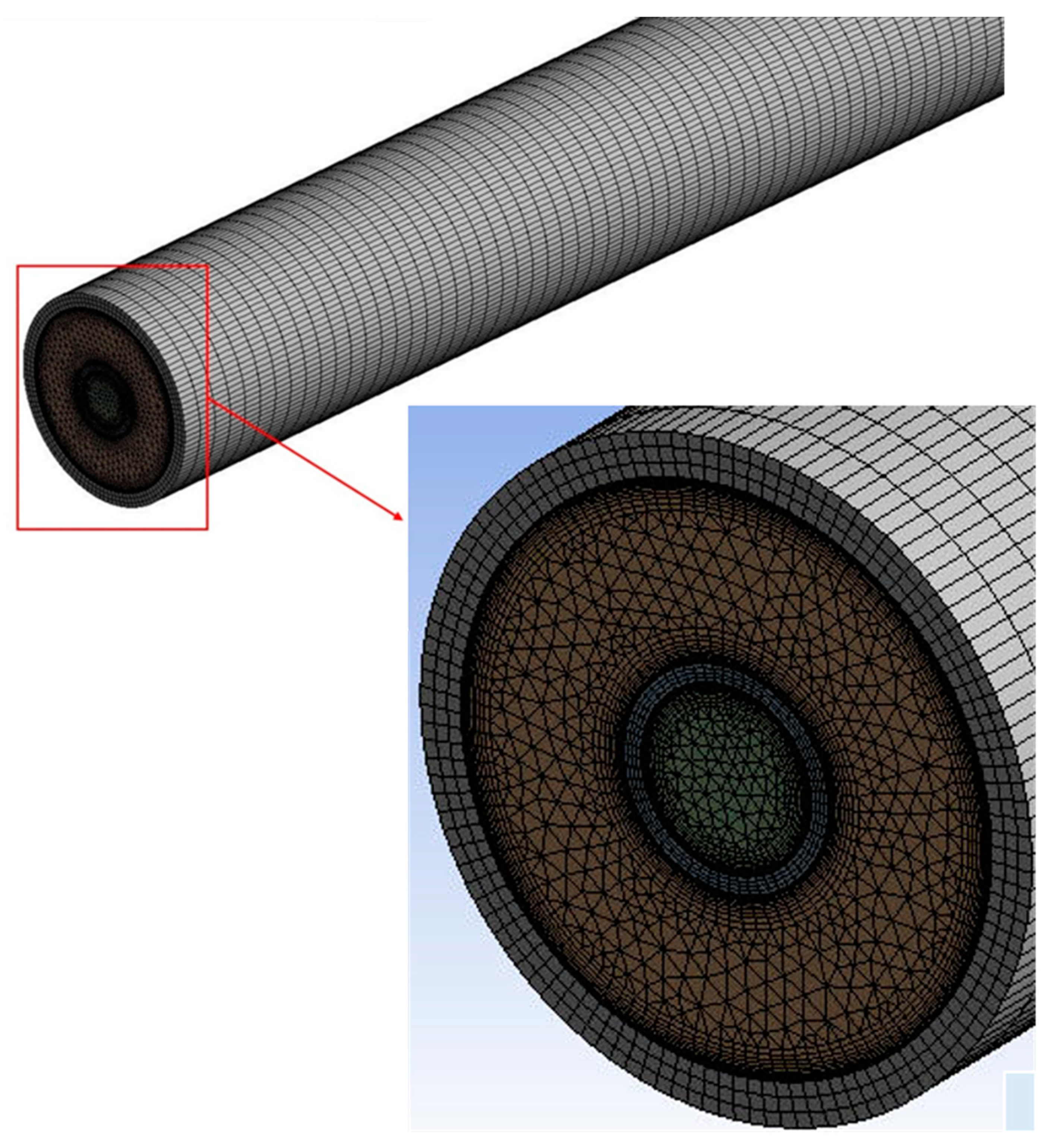
2.1. Geometry
2.2. Boundary Conditions
2.3. Governing Equations
2.4. Thermophysical Properties of Fluids
2.5. Numerical Method and Validation
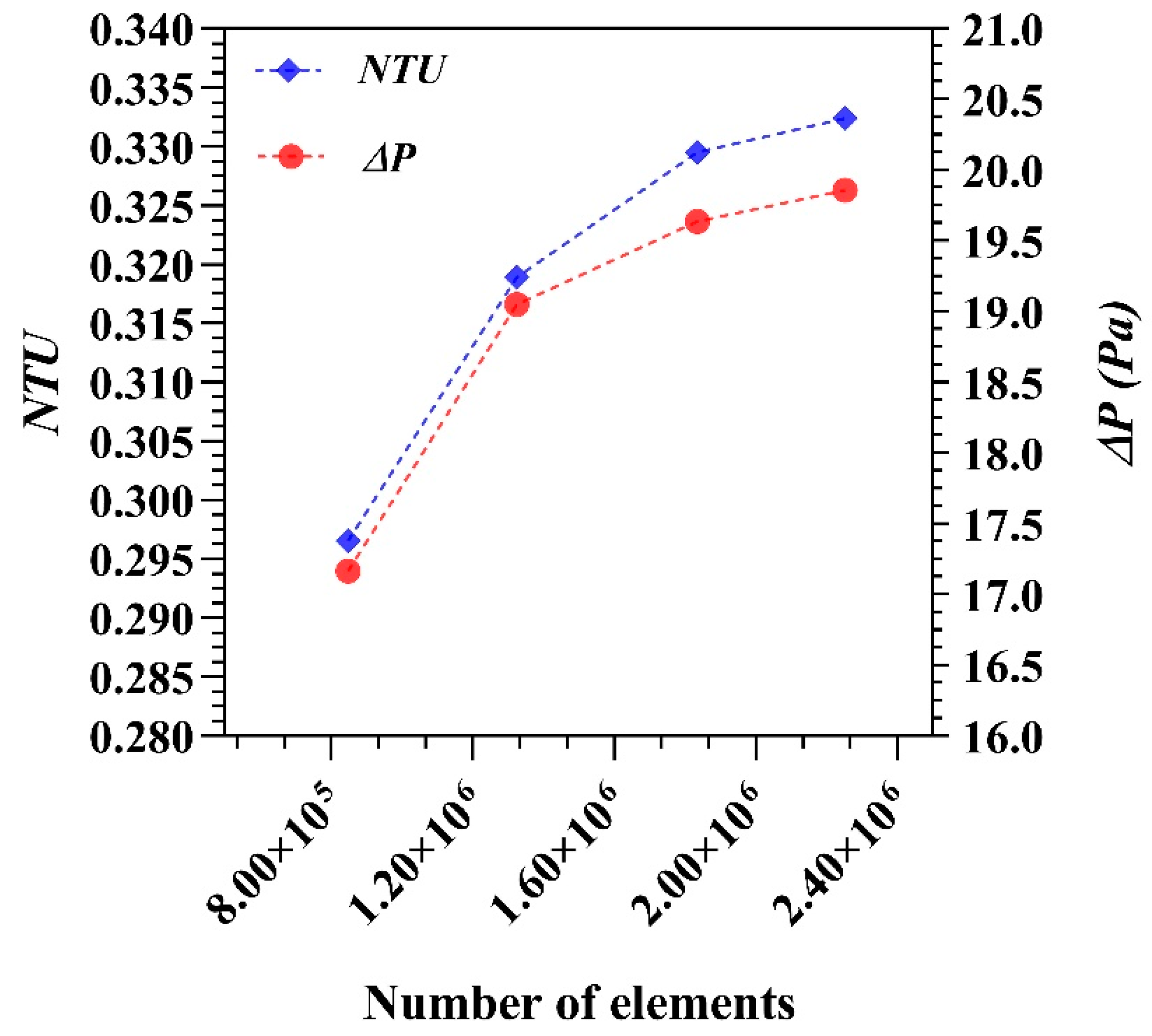
2.6. Grid Independence Study
2.7. Experimental Validation
3. Data Processing
4. Results and Discussion
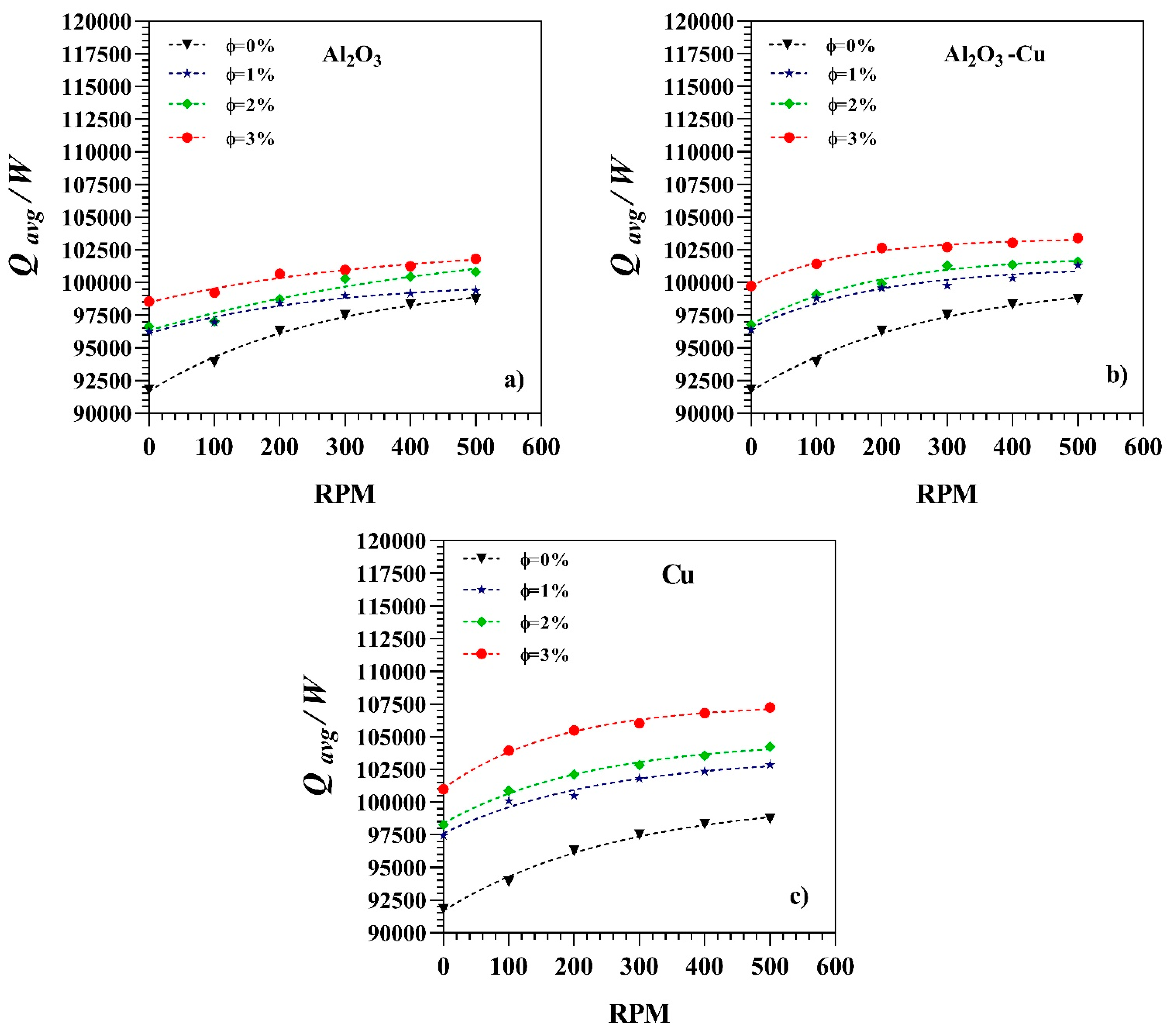
4.1. Flow Analysis
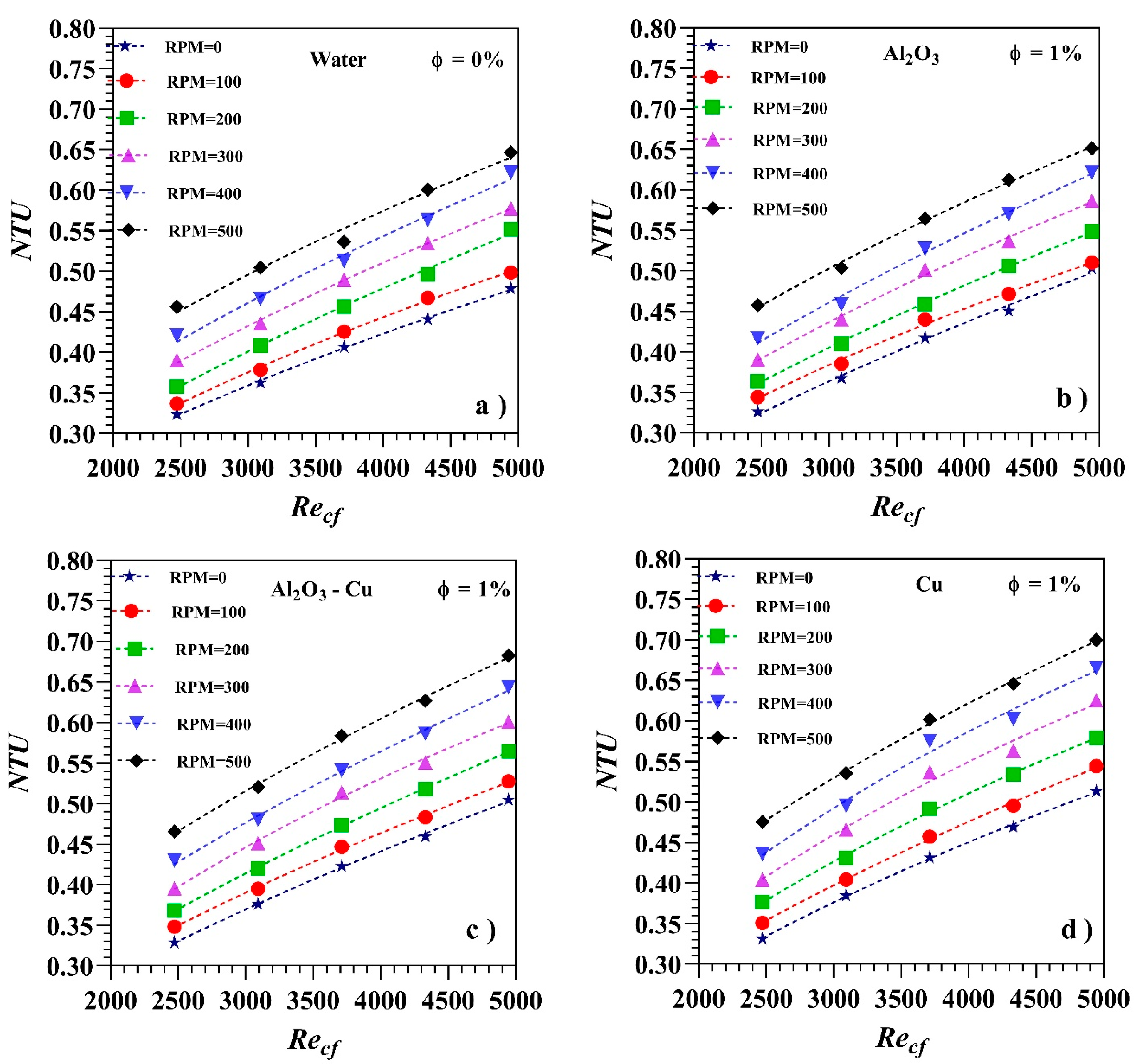
4.2. Number of Transfer Units (NTU)
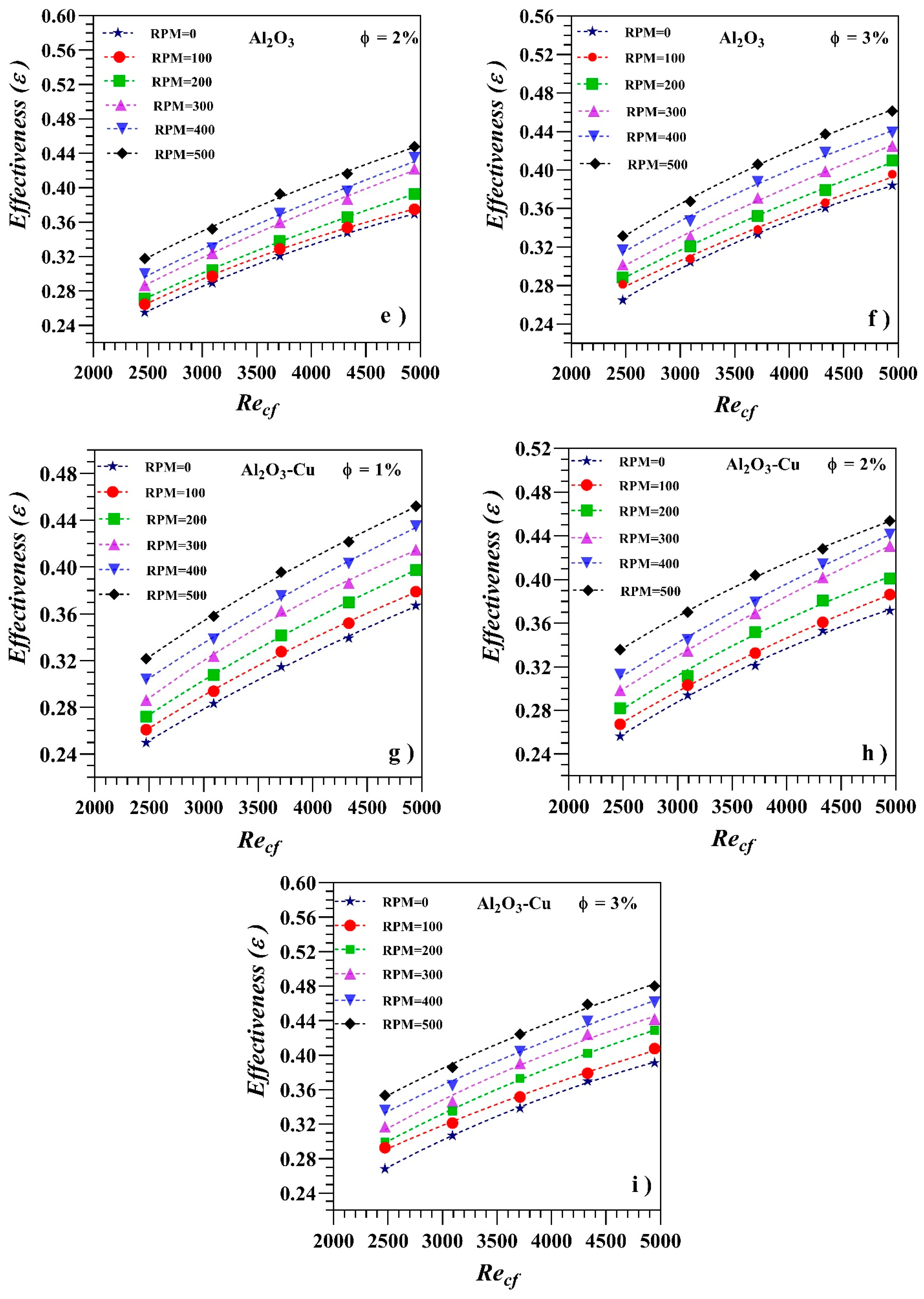
4.3. Effectiveness
4.4. Effect of Rotational Speed at Higher Reynolds Numbers
4.5. Local Heat Transfer Coefficients
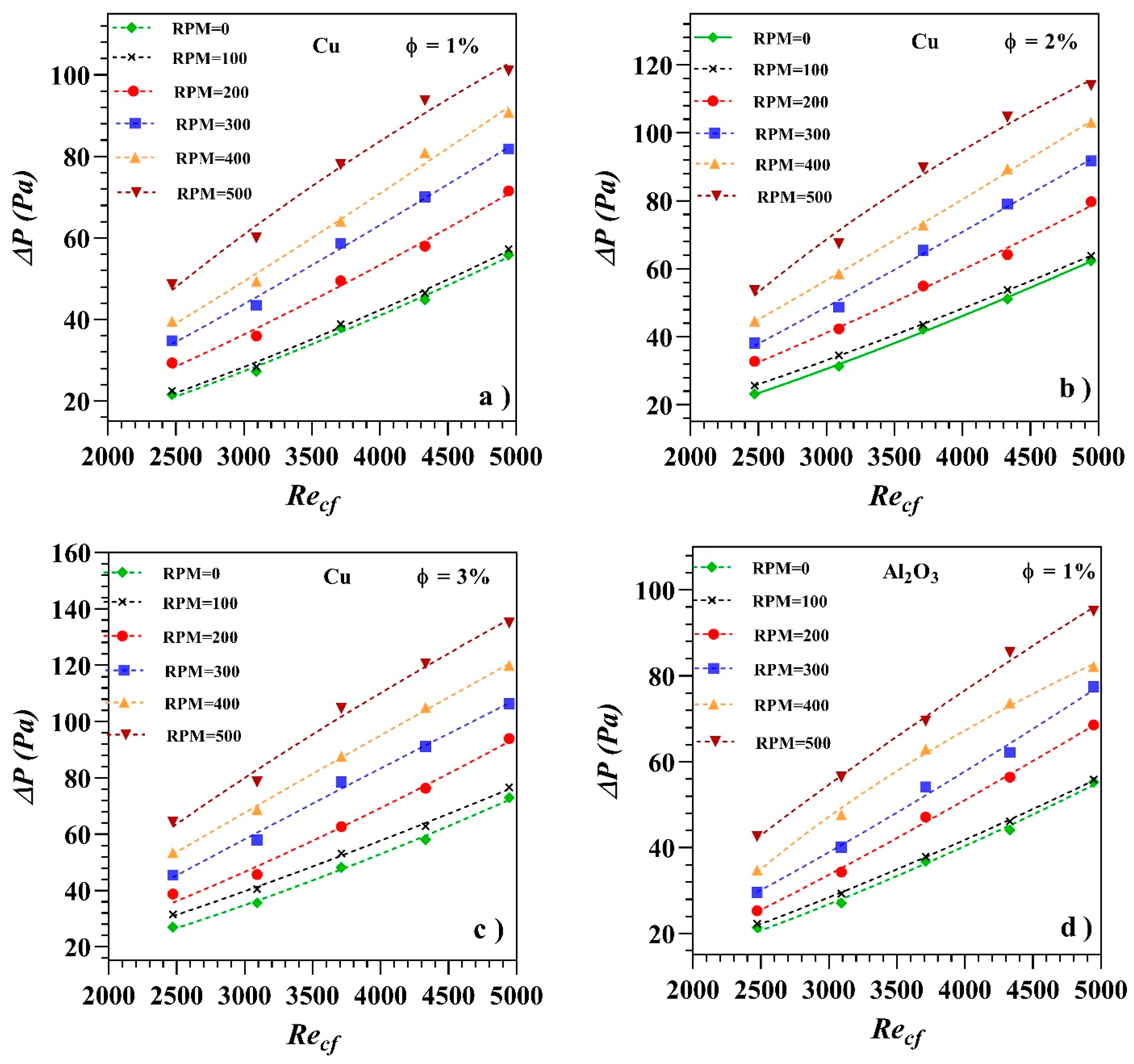
4.6. Pressure Drop
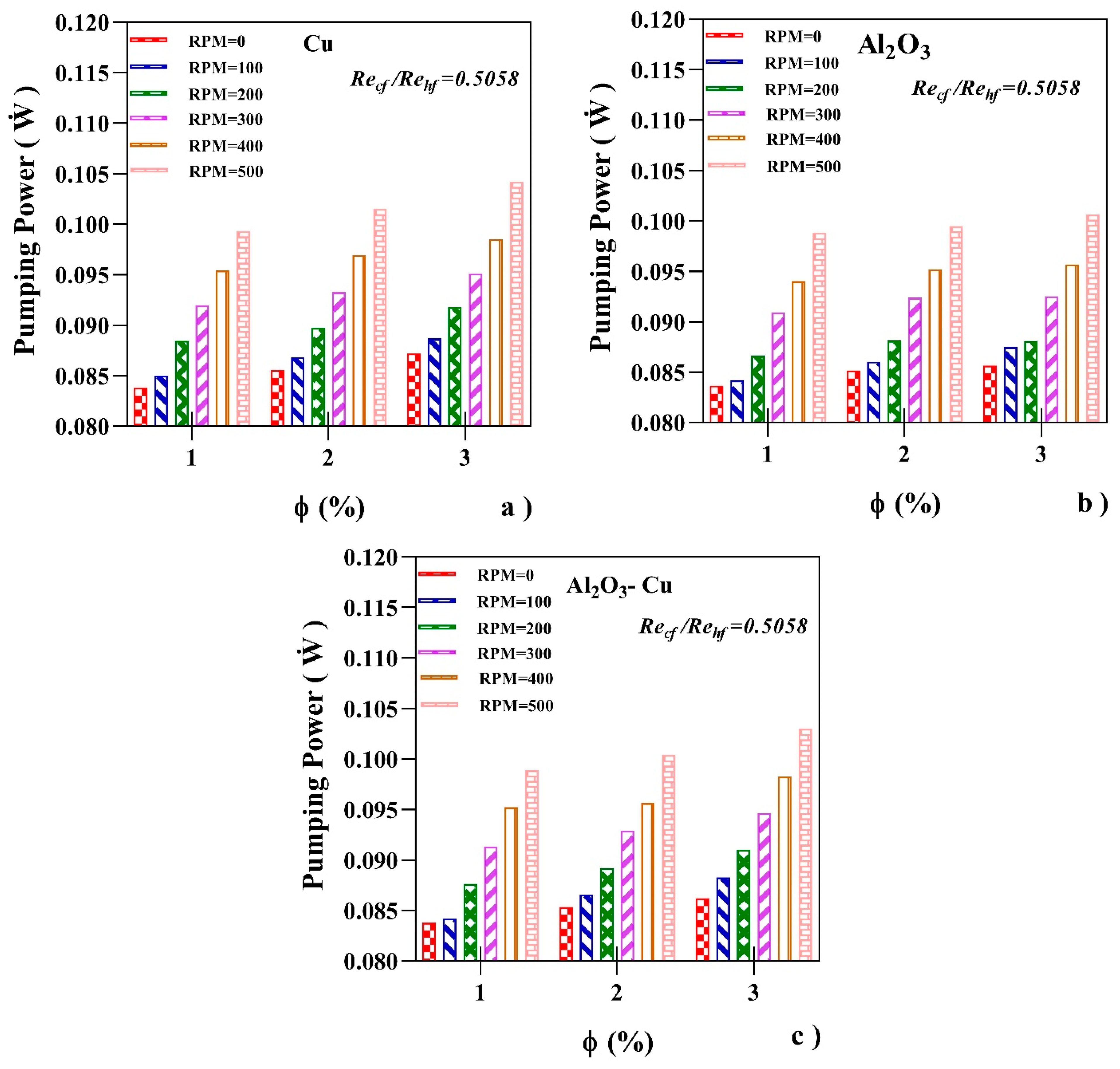
4.7. Pumping Power
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| s | Heat transfer surface area: m2 |
| c | Heat capacity rate, W/K |
| cp | Specific heat capacity, J/kg K |
| D | Diameter of outer tube, m |
| d | Diameter of inside tube, m |
| Generation rate | |
| k | Thermal conductivity, W/m K |
| L | Length of concentric tube, m |
| ṁ | Mass flow rate, kg/s |
| NTU | Number of Transfer Units |
| ρ | Density, kg/m3 |
| Heat transfer rate, W | |
| Re | Reynolds number |
| T | Temperature, K |
| Logarithmic mean temperature difference, K | |
| U | Overall heat transfer coefficient, W/m2 K |
| u | Axial flow velocity, velocity component, m/s |
| Z | Direction coordinates along the tube |
| Greek symbols | |
| Inverse effective turbulent Prandtl numbers | |
| ε | Effectiveness |
| ϕ | Solid volume fraction |
| μ | Dynamic viscosity, kg/m·s |
| ρ | Density, kg/m3 |
| Subscripts | |
| avg | Average |
| cf | Cold fluid |
| eff | Effective |
| f | Fluid |
| h | Hydraulic, hybrid |
| hf | Hot fluid |
| i | Inlet, inner, direction |
| max | Maximum |
| min | Minimum |
| nf | Nanofluid |
| o | Outlet, outer |
| p | Pressure |
| s | Nanoparticle |
| t | Turbulent |
References
- Bezaatpour, M.; Goharkhah, M. Convective heat transfer enhancement in a double pipe mini heat Exchanger by magnetic field induced swirling flow. Appl. Therm. Eng. 2020, 167, 114801. [Google Scholar] [CrossRef]
- Forooghi, P.; Flory, M.; Bertsche, D.; Wetzel, T.; Frohnapfel, B. Heat transfer enhancement on the liquid side of an industrially designed flat-tube heat exchanger with passive inserts—Numerical investigation. Appl. Therm. Eng. 2017, 123, 573–583. [Google Scholar] [CrossRef]
- Sonawane, S.S.; Khedkar, R.S.; Wasewar, K.L. Study on concentric tube heat exchanger heat transfer performance using Al2O3—Water based nanofluids. Int. Commun. Heat Mass Transf. 2013, 49, 60–68. [Google Scholar] [CrossRef]
- Bahmani, M.H.; Sheikhzadeh, G.; Zarringhalam, M.; Akbari, O.A.; Alrashed, A.A.A.A.; Shabani, G.; Goodarzi, M. Investigation of turbulent heat transfer and nanofluid flow in a double pipe heat exchanger. Adv. Powder Technol. 2018, 29, 273–282. [Google Scholar] [CrossRef]
- Rao, M.S.E.; Sreeramulu, D.; Rao, C.J.; Ramana, M.V. Experimental Investigation on Forced Convective Heat Transfer Coefficient of a Nano fluid. Mater. Today Proc. 2017, 4, 8717–8723. [Google Scholar] [CrossRef]
- Yang, C.; Li, W.; Nakayama, A. Convective heat transfer of nanofluids in a concentric annulus. Int. J. Therm. Sci. 2013, 71, 249–257. [Google Scholar] [CrossRef]
- Bajestan, E.E.; Moghadam, M.C.; Niazmand, H.; Daungthongsuk, W.; Wongwises, S. Experimental and numerical investigation of nanofluids heat transfer characteristics for application in solar heat exchangers. Int. J. Heat Mass Transf. 2016, 92, 1041–1052. [Google Scholar] [CrossRef]
- Korpyś, M.; Dzido, G.; Al-Rashed, M.H.; Wojcik, J. Experimental and numerical study on heat transfer intensification in turbulent flow of CuO–water nanofluids in horizontal coil. Chem. Eng. Process. Process Intensif. 2020, 153, 107983. [Google Scholar] [CrossRef]
- Saeedan, M.; Nazar, A.R.S.; Abbasi, Y.; Karimi, R. CFD Investigation and neutral network modeling of heat transfer and pressure drop of nanofluids in double pipe helically baffled heat exchanger with a 3-D fined tube. Appl. Therm. Eng. 2016, 100, 721–729. [Google Scholar] [CrossRef]
- Duangthongsuk, W.; Wongwises, S. An experimental study on the heat transfer performance and pressure drop of TiO2-water nanofluids flowing under a turbulent flow regime. Int. J. Heat Mass Transf. 2010, 53, 334–344. [Google Scholar] [CrossRef]
- Kumar, N.T.R.; Bhramara, P.; Addis, B.M.; Sundar, L.S.; Singh, M.K.; Sousa, A.C.M. Heat transfer, friction factor and effectiveness analysis of Fe3O4/water nanofluid flow in a double pipe heat exchanger with return bend. Int. Commun. Heat Mass Transf. 2017, 81, 155–163. [Google Scholar] [CrossRef]
- Esfe, M.H.; Saedodin, S.; Mahmoodi, M. Experimental studies on the convective heat transfer performance and thermophysical properties of MgO–water nanofluid under turbulent flow. Exp. Therm. Fluid Sci. 2014, 52, 68–78. [Google Scholar] [CrossRef]
- Halelfadl, S.; Estellé, P.; Maré, T. Heat transfer properties of aqueous carbon nanotubes nanofluids in coaxial heat exchanger under laminar regime. Exp. Therm. Fluid Sci. 2014, 55, 174–180. [Google Scholar] [CrossRef]
- Kumar, P.C.M.; Chandrasekar, M. CFD analysis on heat and flow characteristics of double helically coiled tube heat exchanger handling MWCNT/water nanofluids. Heliyon 2019, 5, e02030. [Google Scholar] [CrossRef] [PubMed]
- Goodarzi, M.; Kherbeet, A.S.; Afrand, M.; Sadeghinezhadd, E.; Mehrali, M.; Zahedi, P.; Wongwises, S.; Dahari, M. Investigation of heat transfer performance and friction factor of a counter-flow double-pipe heat exchanger using nitrogen-doped, graphene-based nanofluids. Int. Commun. Heat Mass Transf. 2016, 76, 16–23. [Google Scholar] [CrossRef]
- Hazbehian, M.; Maddah, H.; Mohammadiun, H.; Alizadeh, M. Experimental investigation of heat transfer augmentation inside double pipe heat exchanger equipped with reduced width twisted tapes inserts using polymeric nanofluid. Heat Mass Transf. 2016, 52, 2515–2529. [Google Scholar] [CrossRef]
- Madhesh, D.; Parameshwaran, R.; Kalaiselvam, S. Experimental investigation on convective heat transfer and rheological characteristics of Cu–TiO2 hybrid nanofluids. Exp. Therm. Fluid Sci. 2014, 52, 104–115. [Google Scholar] [CrossRef]
- Reddy, M.C.S.; Rao, V.V. Experimental investigation of heat transfer coefficient and friction factor of ethylene glycol water based TiO2 nanofluid in double pipe heat exchanger with and without helical coil inserts. Int. Commun. Heat Mass Transf. 2014, 50, 68–76. [Google Scholar] [CrossRef]
- Hormozi, F.; ZareNezhad, B.; Allahyar, H.R. An experimental investigation on the effects of surfactants on the thermal performance of hybrid nanofluids in helical coil heat exchangers. Int. Commun. Heat Mass Transf. 2016, 78, 271–276. [Google Scholar] [CrossRef]
- El Maakoul, A.; El Metoui, M.; Abdellah, A.F.B.; Saadeddine, S.; Meziane, M. Numerical investigation of thermohydraulic performance of air to water double-pipe heat exchanger with helical fins. Appl. Therm. Eng. 2017, 127, 127–139. [Google Scholar] [CrossRef]
- Nakhchi, M.E.; Hatami, M.; Rahmati, M. Experimental evaluation of performance intensification of double-pipe heat exchangers with rotary elliptical inserts. Chem. Eng. Process. Process Intensif. 2021, 169, 108615. [Google Scholar] [CrossRef]
- Vaisi, A.; Moosavi, R.; Lashkari, M.; Soltani, M.M. Experimental investigation of perforated twisted tapes turbulator on thermal performance in double pipe heat exchangers. Chem. Eng. Process. Process Intensif. 2020, 154, 108028. [Google Scholar] [CrossRef]
- Promvonge, P.; Promthaisong, P.; Skullong, S. Experimental and numerical heat transfer study of turbulent tube flow through discrete V-winglets. Int. J. Heat Mass Transf. 2020, 151, 119351. [Google Scholar] [CrossRef]
- Murali, G.; Nagendra, B.; Jaya, J. CFD analysis on heat transfer and pressure drop characteristics of turbulent flow in a tube fitted with trapezoidal-cut twisted tape insert using Fe3O4 nano fluid. Mater. Today Proc. 2020, 21, 313–319. [Google Scholar] [CrossRef]
- Watel, B.; Harmand, S.; Desmet, B. Influence of fin spacing and rotational speed on the convective heat exchanges from a rotating finned tube. Int. J. Heat Fluid Flow 2000, 21, 221–227. [Google Scholar] [CrossRef]
- Aliabadi, M.K.; Feizabadi, A. Performance intensification of tubular heat exchangers using compound twisted-tape and twisted-tube. Chem. Eng. Process. Process Intensif. 2020, 148, 107799. [Google Scholar] [CrossRef]
- Singh, S.K.; Sarkar, J. Improving hydrothermal performance of hybrid nanofluid in double tube heat exchanger using tapered wire coil turbulator. Adv. Powder Technol. 2020, 31, 2092–2100. [Google Scholar] [CrossRef]
- Karimi, A.; Al-Rashed, A.A.A.A.; Afrand, M.; Mahian, O.; Wongwises, S.; Shahsavar, A. The effects of tape insert material on the flow and heat transfer in a nanofluid-based double tube heat exchanger: Two-phase mixture model. Int. J. Mech. Sci. 2019, 156, 397–409. [Google Scholar] [CrossRef]
- Darzi, A.A.R.; Farhadi, M.; Sedighi, K.; Aallahyari, S.; Delavar, M.A. Turbulent heat transfer of Al2O3–water nanofluid inside helically corrugated tubes: Numerical study. Int. Commun. Heat Mass Transf. 2013, 41, 68–75. [Google Scholar] [CrossRef]
- Qi, C.; Luo, T.; Liu, M.; Fan, F.; Yan, Y. Experimental study on the flow and heat transfer characteristics of nanofluids in double-tube heat exchangers based on thermal efficiency assessment. Energy Convers. Manag. 2019, 197, 111877. [Google Scholar] [CrossRef]
- Chaurasia, S.R.; Sarviya, R.M. Thermal performance analysis of CuO/water nanofluid flow in a pipe with single and double strip helical screw tape. Appl. Therm. Eng. 2020, 166, 114631. [Google Scholar] [CrossRef]
- Nakhchi, M.E.; Esfahani, J.A. CFD approach for two-phase CuO nanofluid flow through heat exchangers enhanced by double perforated louvered strip insert. Powder Technol. 2020, 367, 877–888. [Google Scholar] [CrossRef]
- Karuppasamy, M.; Saravanan, R.; Chandrasekaran, M.; Muthuraman, V. Numerical exploration of heat transfer in a heat exchanger tube with cone shape inserts and Al2O3 and CuO nanofluids. Mater. Today Proc. 2020, 21, 940–947. [Google Scholar] [CrossRef]
- Saedodin, S.; Zaboli, M.; Rostamian, S.H. Effect of twisted turbulator and various metal oxide nanofluids on the thermal performance of a straight tube: Numerical study based on experimental data. Chem. Eng. Process. Process Intensif. 2020, 158, 108106. [Google Scholar] [CrossRef]
- Mohamed, M.A.; Trashorras, A.J.G.; Marigorta, E.B. Numerical Investigation of Heat Transfer with Nanofluids in Concentric Tube Heat Exchanger under Transitional Flow. Energy Perspect. 2020, 1, 34–56. Available online: http://globalpublisher.org/journals-1006/ (accessed on 17 January 2024).
- Arzani, H.K.; Amiri, A.; Kazi, S.N.; Chew, B.T.; Badarudin, A. Experimental and numerical investigation of thermophysical properties, heat transfer and pressure drop of covalent and noncovalent functionalized graphene nanoplatelet-based water nanofluids in an annular heat exchanger. Int. Commun. Heat Mass Transf. 2015, 68, 267–275. [Google Scholar] [CrossRef]
- Bahiraei, M.; Mazaheri, N.; Mohammadi, M.S.; Moayedi, H. Thermal performance of a new nanofluid containing biologically functionalized graphene nanoplatelets inside tubes equipped with rotating coaxial double-twisted tapes. Int. Commun. Heat Mass Transf. 2019, 108, 104305. [Google Scholar] [CrossRef]
- Shi, Z.; Dong, T. Thermodynamic investigation and optimization of laminar forced convection in a rotating helical tube heat exchanger. Energy Convers. Manag. 2014, 86, 399–409. [Google Scholar] [CrossRef]
- El-Maghlany, W.M.; Hanafy, A.A.; Hassan, A.A.; El-Magid, M.A. Experimental study of Cu–water nanofluid heat transfer and pressure drop in a horizontal double-tube heat exchanger. Exp. Therm. Fluid Sci. 2016, 78, 100–111. [Google Scholar] [CrossRef]
- Moayedi, H. Investigation of heat transfer enhancement of Cu-water nanofluid by different configurations of double rotating cylinders in a vented cavity with different inlet and outlet ports. Int. Commun. Heat Mass Transf. 2021, 126, 105432. [Google Scholar] [CrossRef]
- Abou-Ziyan, H.Z.; Helali, A.B.; Selim, M.Y.E. Enhancement of forced convection in wide cylindrical annular channel using rotating inner pipe with interrupted helical fins. Int. J. Heat Mass Transf. 2016, 95, 996–1007. [Google Scholar] [CrossRef]
- Ali, M.A.M.; El-Maghlany, W.M.; Eldrainy, Y.A.; Attia, A. Heat transfer enhancement of double pipe heat exchanger using rotating of variable eccentricity inner pipe. Alex. Eng. J. 2018, 57, 3709–3725. [Google Scholar] [CrossRef]
- Jasim, L.M.; Hamzah, H.; Canpolat, C.; Sahin, B. Mixed convection flow of hybrid nanofluid through a vented enclosure with an inner rotating cylinder. Int. Commun. Heat Mass Transf. 2021, 121, 105086. [Google Scholar] [CrossRef]
- Gomaa, A.; Halim, M.A.; Elsaid, A.M. Experimental and numerical investigations of a triple concentric-tube heat exchanger. Appl. Therm. Eng. 2016, 99, 1303–1315. [Google Scholar] [CrossRef]
- Abdollahzadeh, M.; Esmaeilpour, M.; Vizinho, R.; Younesi, A.; Pàscoa, J.C. Assessment of RANS turbulence models for numerical study of laminar-turbulent transition in convection heat transfer. Int. J. Heat Mass Transf. 2017, 115, 1288–1308. [Google Scholar] [CrossRef]
- Siavashi, M.; Jamali, M. Heat transfer and entropy generation analysis of turbulent flow of TiO2-water nanofluid inside annuli with different radius ratios using two-phase mixture model. Appl. Therm. Eng. 2016, 100, 1149–1160. [Google Scholar] [CrossRef]
- Ard, S.E.; Kiatkittipong, K. Heat transfer enhancement by multiple twisted tape inserts and TiO2/water nanofluid. Appl. Therm. Eng. 2014, 70, 896–924. [Google Scholar] [CrossRef]
- Fattahi, A. Numerical simulation of a solar collector equipped with a twisted tape and containing a hybrid nanofluid. Sustain. Energy Technol. Assess. 2021, 45, 101200. [Google Scholar] [CrossRef]
- YousefiMiab, E.; Islami, S.B.; Gharraei, R. Feasibility assessment of using nanofluids in shell and tube heat exchanger of gas pressure reducing stations through a new developed OpenFOAM solver. Int. J. Heat Fluid Flow 2022, 96, 108985. [Google Scholar] [CrossRef]
- Dbouk, T.; Habchi, C.; Harion, J.L.; Drikakis, D. Heat transfer and mixing enhancement by Poiseuille-Taylor-Couette flow between two rotating elliptically-deformed annular tubes. Int. J. Heat Fluid Flow 2022, 96, 109011. [Google Scholar] [CrossRef]
- Upadhya, S.M.; Raju, S.V.S.R.; Raju, C.S.K.; Shah, N.A.; Chung, J.D. Importance of entropy generation on Casson, Micropolar and Hybrid magneto-nanofluids in a suspension of cross diffusion. Chin. J. Phys. 2022, 77, 1080–1101. [Google Scholar] [CrossRef]
- Ghazanfari, V.; Taheri, A.; Amini, Y.; Mansourzade, F. Enhancing heat transfer in a heat exchanger: CFD study of twisted tube and nanofluid (Al2O3, Cu, CuO, and TiO2) effects. Case Stud. Therm. Eng. 2024, 53, 103864. [Google Scholar] [CrossRef]
- Sundar, L.S.; Mouli, K.V.V.C. Effectiveness and number of transfer units of plate heat exchanger with Fe3O4–SiO2/Water hybrid nanofluids: Experimental and artificial neural network predictions. Case Stud. Therm. Eng. 2024, 53, 103949. [Google Scholar] [CrossRef]
- Thirumalaisamy, K.; Sivaraj, R.; Reddy, A.S. Fluid flow and heat transfer analysis of a ternary aqueous Fe3O4 + MWCNT + Cu/H2O magnetic nanofluid in an inclined rectangular porous cavity. J. Magn. Magn. Mater. 2024, 589, 171503. [Google Scholar] [CrossRef]
- Hatami, M.; Jing, D. Nanofluids Mathematical, Numerical, and Experimental Analysis; Academic Press: Cambridge, MA, USA, 2020; ISBN 978-0-08-102933-6. [Google Scholar]
- Siadaty, M.; Kazazi, M. Study of water based nanofluid flows in annular tubes using numerical simulation and sensitivity analysis. Heat Mass Transf. 2018, 54, 2995–3014. [Google Scholar] [CrossRef]
- Babu, J.A.R.; Kumar, K.K.; Rao, S.S. State-of-art review on hybrid nanofluids. Renew. Sustain. Energy Rev. 2017, 77, 551–565. [Google Scholar] [CrossRef]









| Author | Heat Exchanger Morphology | Working Fluid | Main Results |
|---|---|---|---|
| Bahmani et al. [4] | Concentric tube | Al2O3-water | Increasing the maximum thermal efficiency and the average Nusselt number by 30% and 32.7%, respectively. |
| Saeedan et al. [9] | Double-pipe helically baffled | Cu, CuO and carbon nanotube | The heat transfer coefficient and pressure gradient were increased. |
| Kumar and Chandrasekar [14] | Double helically coiled tube | MWCNT-water | The Nusselt number increased by 20, 24 and 30% for 0.2, 0.4 and 0.6% concentrations. |
| Murali et al. [24] | Trapezoidal twisted tape | Fe3O4 | The heat transfer rate increased by 78.6%. |
| Watel et al. [25] | Rotating finned tube | Air | The convection of heat transfer was enhanced due to the rotation for each spacing fin. |
| Singh and Sarkar [27] | Tapered wire coils in a double tube | Al2O3-MgO hybrid nanofluid | The Nusselt numbers inreased by 84, 71 and 47%. |
| Qi et al. [30] | Corrugated double tube | TiO2-water | Improving the heat transfer rate by a 14.8% at 0.5% volume concentration and a higher pressure drop that increased by 6.5%. |
| Chaurasia and Sarviya [31] | Double and single strip helical screw | CuO-water | The Nusselt number was enhanced by 182 and 170% with the nanofluid for the double and single screw tapes. |
| Saedodin et al. [34] | Twisted turbulator | SiO2, Al2O3, CuO and TiO2 | The use of the turbulator increased the heat transfer rate and the CuO-water nanofluid had a higher heat transfer than the other nanofluids. |
| Bahiraei et al. [37] | Rotating coaxial tube with double twisted tapes | Graphene nanoplatelet (GNP) | Rotation of the tube improved the heat transfer coefficient and increased the pressure drop. |
| Shi and Dong [38] | Rotating helical tube | Water | A decrease in entropy generation due to heat transfer and an increase in entropy generation due to flow friction. |
| El-Maghlany et al. [39] | Rotating inner tube | Cu-water | NTU was increased by 51.4% and the exchanger effectiveness by 30.7% |
| Moayedi [40] | Double rotating cylinders in a vented cavity | Cu-water | Heat transfer was enhanced when the cylinders rotated and the mean Nusselt number, when cylinders rotated, was enhanced by 331.95%. |
| Ziyan et al. [41] | Rotating inner pipe with helical fins | Air | The Nusselt number for both fins and the rotation were enhanced by about 7.5 times compared to those with the stationary plain pipe. |
| Siavashi and Jamali [46] | Annulus with different radius ratios | TiO2-water | The performance increased with an increase in the Reynolds number at a radius ratio 0.2, 0.4 and 0.6 compared to that of 0.8. |
| Ard and Kiatkittipong [47] | Multiple twisted tapes | TiO2-water | Twisted tapes in counter arrangement had a higher thermal performance than that in co-counter. |
| Fattahi [48] | Cylindrical pipe | Al2O3-CuO-water hybrid | The Nusselt number at ϕ = 0.02 and ϕ = 0.04 was increased by 23% and 20%. |
| Physical Properties | ρ (kg/m3) | cp (J/kg·K) | k (W/m·K) | (Pa·s) |
|---|---|---|---|---|
| Pure water [55] | 997.1 | 4179 | 0.6 | 0.001 |
| Al2O3 [55] | 3970 | 765 | 36 | - |
| Cu [55] | 8933 | 385 | 385 | - |
| Nanofluid Properties | = 1% | = 2% | = 3% | ||||||
|---|---|---|---|---|---|---|---|---|---|
| Al2O3 | Cu | Al2O3-Cu | Al2O3 | Cu | Al2O3-Cu | Al2O3 | Cu | Al2O3-Cu | |
| ρ (kg/m3) | 1026.829 | 1076.45 | 1051.644 | 1056.558 | 1155.8 | 1106.188 | 1086.287 | 1235.1 | 1160.732 |
| Cp (J/kg·K) | 4047 | 3864.19 | 3953.42 | 3922.43 | 3592.6 | 3750.09 | 3804.69 | 3358.39 | 3565.896 |
| k (W/m·K) | 0.61729 | 0.6181 | 0.6180 | 0.6349 | 0.63656 | 0.63641 | 0.65289 | 0.6554 | 0.6551 |
| (Pa·s) | 0.001025 | 0.001025 | 0.00102562 | 0.001050 | 0.001050 | 0.00105248 | 0.001075 | 0.001075 | 0.00108058 |
| Water | = 3% | |||||
|---|---|---|---|---|---|---|
| Reynolds Number | ε (0 RPM) | ε (500 RPM) | Δε | ε (0 RPM) | ε (500 RPM) | Δε |
| 2473 | 0.247 | 0.317 | 0.070 | 0.275 | 0.371 | 0.096 |
| 3092 | 0.275 | 0.350 | 0.075 | 0.316 | 0.412 | 0.096 |
| 3712 | 0.306 | 0.373 | 0.067 | 0.346 | 0.449 | 0.103 |
| 4331 | 0.329 | 0.410 | 0.081 | 0.382 | 0.487 | 0.105 |
| 4946 | 0.353 | 0.436 | 0.083 | 0.401 | 0.499 | 0.098 |
| 10,500 | 0.370 | 0.439 | 0.069 | 0.425 | 0.517 | 0.092 |
| 14,000 | 0.426 | 0.499 | 0.073 | 0.461 | 0.537 | 0.076 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mohamed, M.A.E.-M.; Meana-Fernández, A.; González-Caballín, J.M.; Bowman, A.; Gutiérrez-Trashorras, A.J. Numerical Study of a Heat Exchanger with a Rotating Tube Using Nanofluids under Transitional Flow. Processes 2024, 12, 222. https://doi.org/10.3390/pr12010222
Mohamed MAE-M, Meana-Fernández A, González-Caballín JM, Bowman A, Gutiérrez-Trashorras AJ. Numerical Study of a Heat Exchanger with a Rotating Tube Using Nanofluids under Transitional Flow. Processes. 2024; 12(1):222. https://doi.org/10.3390/pr12010222
Chicago/Turabian StyleMohamed, Mohamed A. El-Magid, Andrés Meana-Fernández, Juan M. González-Caballín, Anthony Bowman, and Antonio José Gutiérrez-Trashorras. 2024. "Numerical Study of a Heat Exchanger with a Rotating Tube Using Nanofluids under Transitional Flow" Processes 12, no. 1: 222. https://doi.org/10.3390/pr12010222
APA StyleMohamed, M. A. E.-M., Meana-Fernández, A., González-Caballín, J. M., Bowman, A., & Gutiérrez-Trashorras, A. J. (2024). Numerical Study of a Heat Exchanger with a Rotating Tube Using Nanofluids under Transitional Flow. Processes, 12(1), 222. https://doi.org/10.3390/pr12010222