Natural Convection in a Circular Enclosure with Four Cylinders under Magnetic Field: Application to Heat Exchanger
Abstract
1. Introduction
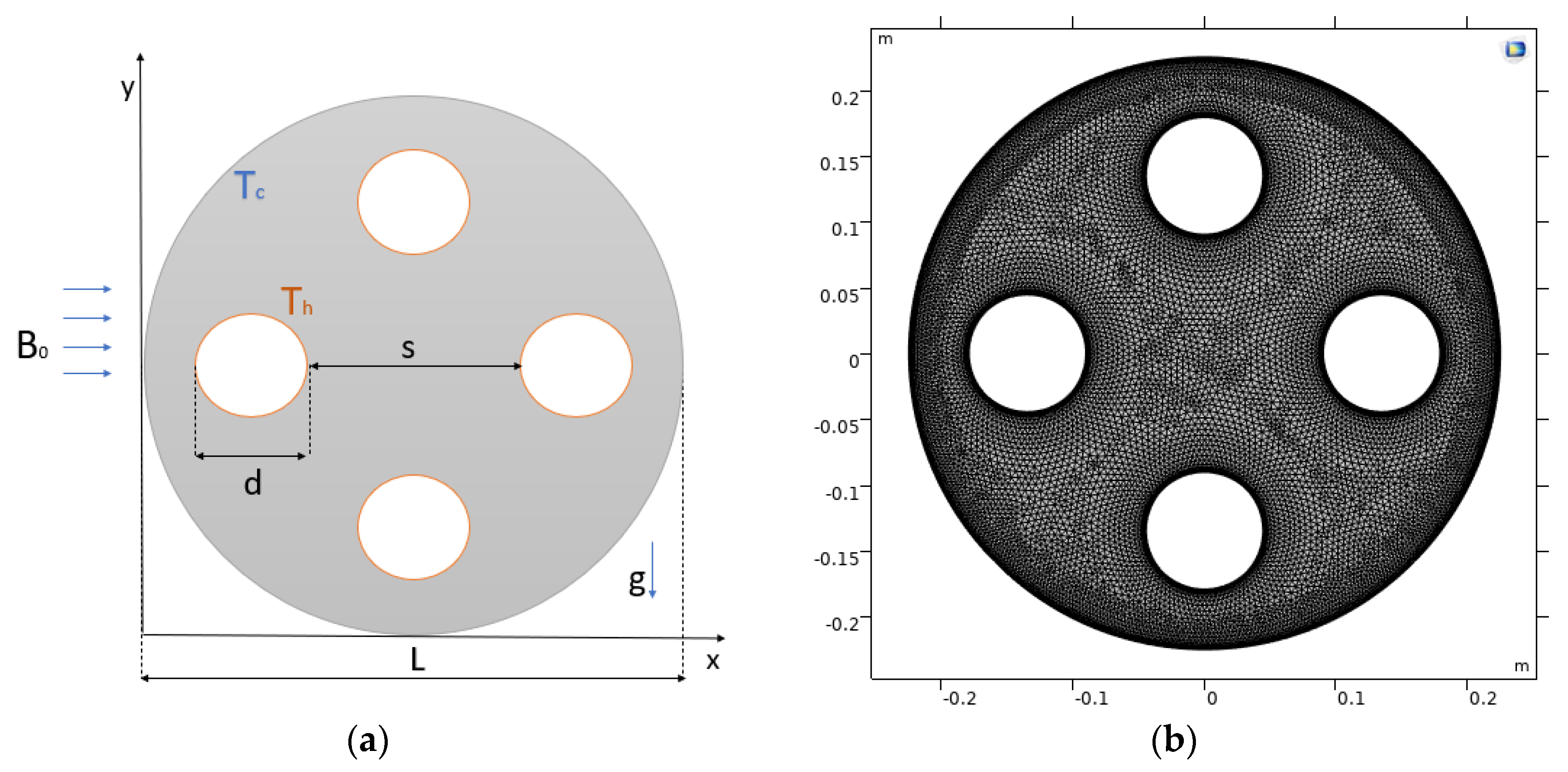
2. Numerical Model
2.1. Simplifying Assumptions
- The fluid used is considered incompressible and Newtonian fluid;
- The flow is thought to be stable, laminar, and two-dimensional;
- The effect of radiation considered minimal;
- Neglect is shown also for displacement currents, dissipation, and Joule heating.
2.2. Equations of the Model
2.2.1. Dimensional Form
2.2.2. Nondimensional Form
2.2.3. Boundary Conditions
3. Results and Discussion
3.1. Validation
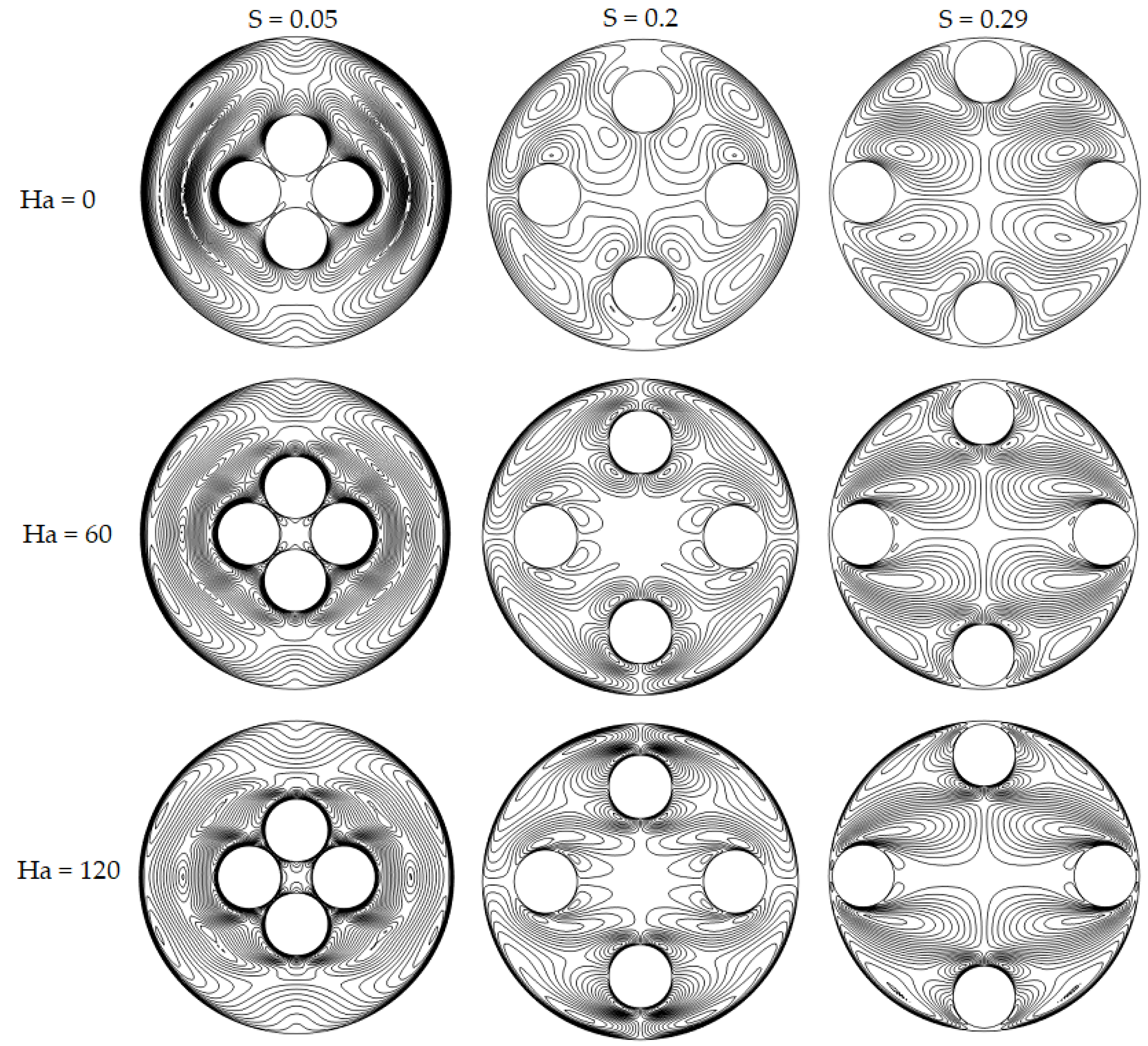
3.2. Flow Field
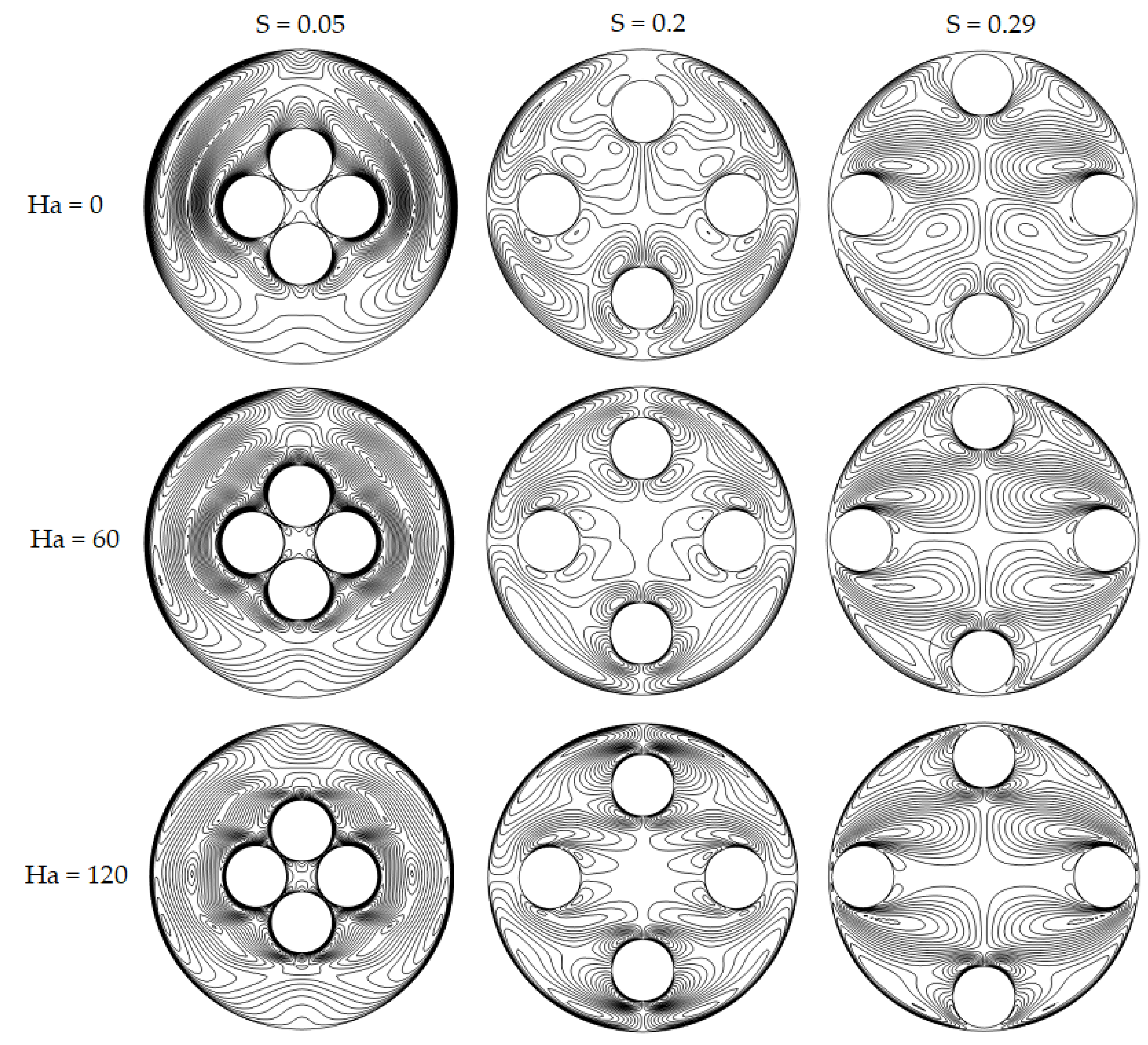
3.3. Temperature Field
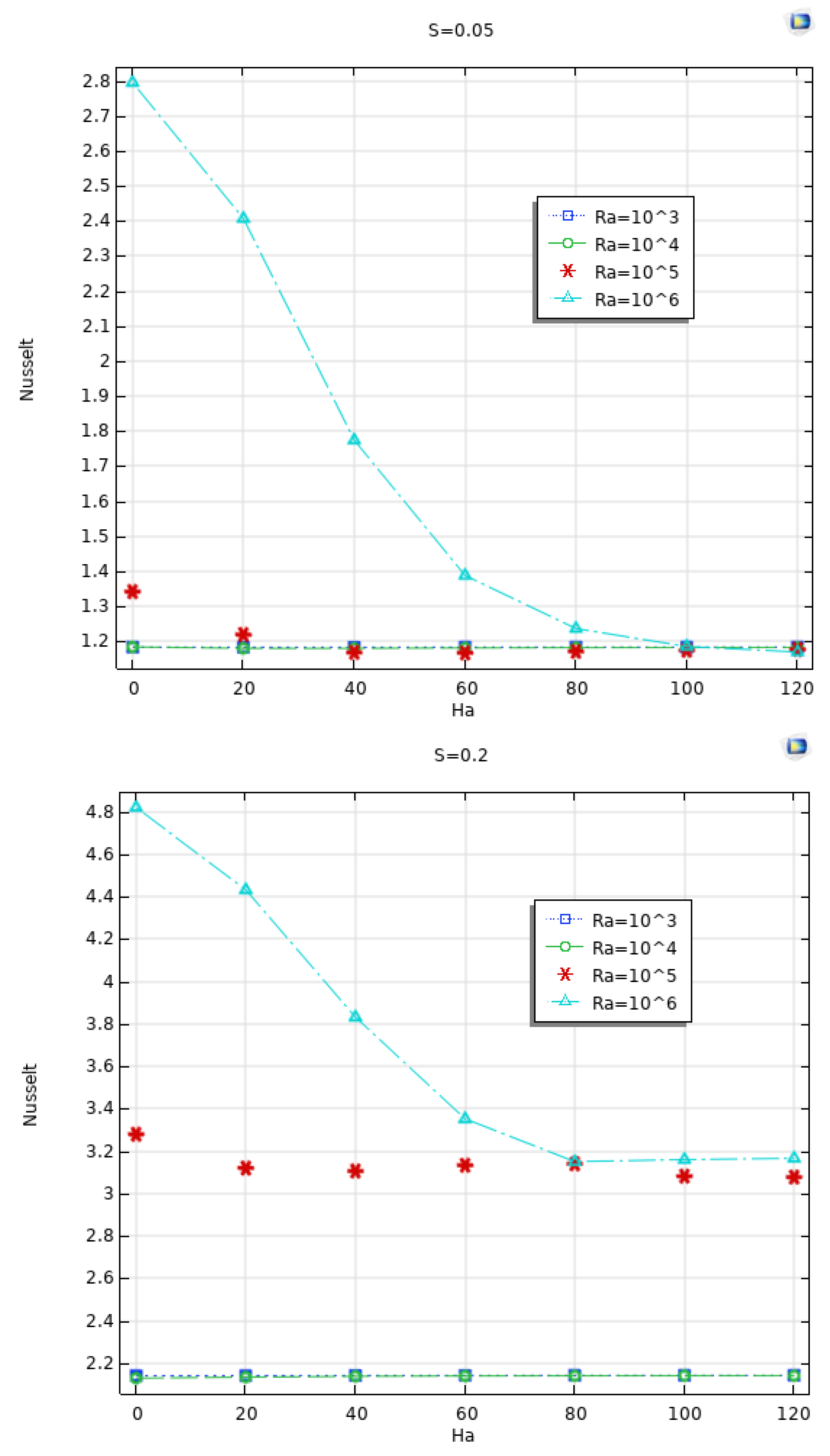
3.4. Heat Transfer
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| Magnetic field strength | |
| Gravitational acceleration, () | |
| Ha | Hartmann number |
| L | Length of cavity, (m) |
| Nu | Nusselt number |
| Fluid pressure, | |
| Dimensionless pressure | |
| Pr | Prandtl number |
| Ra | Rayleigh number |
| Temperature, (K) | |
| Dimensionless temperature | |
| s | Distance between inner cylinders (m) |
| S | Dimensionless distance between cylinders |
| d | Diameter of inner cylinder |
| Temperature of hot fluid, (K) | |
| Temperature of cold fluid, (K) | |
| MHDNC | magnetohydrodynamic natural convection |
| Velocity components in x, and y directions | |
| Dimensionless velocity components | |
| Cartesian coordinates | |
| Dimensionless coordinates | |
| α | Thermal diffusivity, |
| λ | Thermal conductivity, |
| Cp | Specific heat capacity, |
| σ | Electrical conductivity, |
| β | Expansion coefficient, |
| ρ | Density, |
| υ | Cinematic viscosity, |
| η | Normal direction dimensionless coordinate |
| c | Cold |
| h | Hot |
References
- Ben Hamida, M.B. Numerical analysis of tubular solar still with rectangular and cylindrical troughs for water production under vacuum. J. Taibah Univ. Sci. 2023, 7, 2159172. [Google Scholar] [CrossRef]
- Ben Hamida, M.B.; Al Shammari, F.; Altawi, I.; Alhadri, M.; Almeshaal, M.A.; Hajlaoui, K. Potential of tubular solar still with rectangular trough for water production under vacuum condition. Therm. Sci. 2022, 26, 4271–4283. [Google Scholar]
- Ben Hamida, M.B.; Belghaieb, J.; Hajji, N. Nnumerical study of heat and mass transfer enhancement for bubble absorption process ofammonia-water mixture without and with nanofluids. Therm. Sci. 2018, 22, 3107–3120. [Google Scholar]
- Tayebi, T.; Dogonchi, A.S.; Chamkha, A.J.; Ben Hamida, M.B.; El-Sapa, S.; Galal, A.M. Micropolar nanofluid thermal free convection and entropy generation through an inclined I-shaped enclosure with two hot cylinders. Case Stud. Therm. Eng. 2022, 31, 101813. [Google Scholar] [CrossRef]
- Massoudi, M.D.; Ben Hamida, M.B.; Mohammed, H.A.; Almeshaal, M.A. MHD Heat Transfer in W-Shaped Inclined Cavity Containing a Porous Medium Saturated with Ag/Al2O3 Hybrid Nanofluid in the Presence of Uniform Heat Generation/Absorption. Energies 2020, 13, 3457. [Google Scholar] [CrossRef]
- Massoudi, M.D.; Ben Hamida, M.B.; Almeshaal, M.A.; Hajlaoui, K. The influence of multiple fins arrangement cases on heat sink efficiency of MHD MWCNT-water nanofluid within tilted T-shaped cavity packed with trapezoidal fins considering thermal emission impact. Int. Commun. Heat Mass. Transf.sf. 2021, 126, 105468. [Google Scholar] [CrossRef]
- Ben Hamida, M.B.; Charrada, K. Natural convection heat transfer in an enclosure filled with an ethylene glycol—Copper nanofluid under magnetic fields. Numer. Heat Transf. A-Appl. 2015, 67, 902–920. [Google Scholar] [CrossRef]
- Baranwal, A.K.; Chhabra, R.P. Effect of fluid yield stress on natural convection from horizontal cylinders in a square enclosure. Heat Tran. Eng. 2017, 38, 557–577. [Google Scholar] [CrossRef]
- Park, Y.G.; Yoon, H.S.; Ha, M.Y. Natural convection in square enclosure with hot and cold cylinders at different vertical locations. Int. J. Heat Mass. Transf. 2012, 55, 7911–7925. [Google Scholar] [CrossRef]
- Park, Y.G.; Ha, M.Y.; Yoon, H.S. Study on natural convection in a cold square enclosure with a pair of hot horizontal cylinders positioned at different vertical locations. Int. J. Heat Mass Transf. 2013, 65, 696–712. [Google Scholar] [CrossRef]
- Roslan, R.; Saleh, H.; Hashim, I.; Bataineh, A.S. Natural convection in an enclosure containing a sinusoidally heated cylindrical source. Int. J. Heat Mass Transf. 2014, 70, 119–127. [Google Scholar] [CrossRef]
- Cho, H.W.; Seo, Y.M.; Mun, G.S.; Ha, M.Y.; Park, Y.G. The effect of instability flow for two-dimensional natural convection in a square enclosure with different arrays of two inner cylinders. Int. J. Heat Mass Transf. 2017, 114, 307–317. [Google Scholar] [CrossRef]
- Bhowmick, D.; Randive, P.; Pati, S.; Agrawal, H.; Kumar, A.; Srivastava, P.K. Natural convection heat transfer and entropy generation from a heated cylinder of different geometry in an enclosure with non-uniform temperature distribution on the walls. J. Therm. Anal. Calorim. 2020, 141, 839–857. [Google Scholar] [CrossRef]
- Deka, D.K.; Pal, G.C.; Pati, S.; Randive, P.R. Natural convection from two cylinders in an enclosure with sinusoidal bottom wall: A numerical study. In Recent Advances in Mechanical Engineering; Lecture Notes in Mechanical Engineering; Springer: Singapore, 2021; pp. 351–359. [Google Scholar]
- Goswami, N.; Randive, P.R.; Pati, S. Natural convection from a pair of heated cylinders in a square cavity with non-uniform temperature on the side walls. J. Inst. Eng. 2021, 102, 389–396. [Google Scholar] [CrossRef]
- Pirmohammadi, M.; Ghassemi, M. Effect of magnetic field on convection heat transfer inside a tilted square enclosure. Int. Commun. Heat Mass. Transf. 2009, 36, 776–780. [Google Scholar] [CrossRef]
- Ghasemi, B.; Aminossadati, S.M.; Raisi, A. Magnetic field effect on natural convection in a nanofluid-filled square enclosure. Int. J. Therm. Sci. 2011, 50, 1748–1756. [Google Scholar] [CrossRef]
- Turkyilmazoglu, M. MHD natural convection in saturated porous media with heat generation/absorption and thermal radiation: Closed-form solutions. Arch. Mech. 2019, 71, 49–64. [Google Scholar]
- Siddiqui, A.A.; Turkyilmazoglu, M. A new theoretical approach of wall transpiration in the cavity flow of the ferrofluids. Micromachines 2019, 10, 373. [Google Scholar] [CrossRef]
- Siddiqui, A.A.; Turkyilmazoglu, M. Natural convection in the ferrofluid enclosed in a porous and permeable cavity. Int. Commun. Heat Mass. Transf. 2020, 113, 104499. [Google Scholar] [CrossRef]
- Dutta, S.; Goswami, N.; Biswas, A.K.; Pati, S. Numerical investigation of magnetohydrodynamic natural convection heat transfer and entropy generation in a rhombic enclosure filled with Cu-water nanofluid. Int. J. Heat Mass. Transf. 2019, 136, 777–798. [Google Scholar] [CrossRef]
- Ben Hamida, M.B.; Almeshaal, M.A.; Hajlaoui, K. A Three-dimensional thermal analysis for cooling a square Light Emitting Diode by Multiwalled Carbon Nanotube-nanofluid-filled in a rectangular microchannel. Adv. Mech. Eng. 2021, 13, 16878140211059946. [Google Scholar] [CrossRef]
- Ben Hamida, M.B.; Hatami, M. Investigation of heated fins geometries on the heat transfer of a channel filled by hybrid nanofluids under the electric field. Case Stud. Therm. Eng. 2021, 28, 101450. [Google Scholar] [CrossRef]
- Khan, N.S.; Poom, K.; Thounthong, P. Mechanical aspects of Maxwell nanofluid in dynamic system with irreversible analysis. Int. J. Heat Mass Transf. 2019, 145, 118713. [Google Scholar] [CrossRef]
- Khan, N.S.; Shah, Q.; Sohail, A.; Poom, K. Renewable energy technology for the sustainable development of thermal system with en-tropy measures. ZAMM-J. Appl. Math. Mech./Z. Für Angew. Math. Und Mech. 2021, 101, e202000212. [Google Scholar]
- Kefayati, G.H.R. Lattice Boltzmann simulation of MHD natural convection in a nanofluid-filled cavity with sinusoidal temperature distribution. Powder Technol. 2013, 243, 171–183. [Google Scholar] [CrossRef]
- Al-Zamily, A.; Meerali, J. Effect of magnetic field on natural convection in a nanofluid-filled semi-circular enclosure with heat flux source. Comput. Fluids 2014, 103, 71–85. [Google Scholar] [CrossRef]
- Aglawe, K.R.; Yadav, R.K.; Thool, S.B. Preparation, applications and challenges of nanofluids in electronic cooling: A systematic review. Mater. Today Proc. 2021, 43, 366–372. [Google Scholar] [CrossRef]
- Ben Hamida, M.B.; Hatami, M. Optimization of fins arrangements for the square light emitting diode (LED) cooling through nanofluid-filled microchannel. Sci. Rep. 2021, 11, 12610. [Google Scholar] [CrossRef] [PubMed]
- Pal, G.C.; Nammi, G.; Pati, S.; Randive, P.R.; Baranyi, L. Natural convection in an enclosure with a pair of cylinders under magnetic field. Case Stud. Therm. Eng. 2022, 30, 101763. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Azzouz, R.; Hamida, M.B.B. Natural Convection in a Circular Enclosure with Four Cylinders under Magnetic Field: Application to Heat Exchanger. Processes 2023, 11, 2444. https://doi.org/10.3390/pr11082444
Azzouz R, Hamida MBB. Natural Convection in a Circular Enclosure with Four Cylinders under Magnetic Field: Application to Heat Exchanger. Processes. 2023; 11(8):2444. https://doi.org/10.3390/pr11082444
Chicago/Turabian StyleAzzouz, Raouia, and Mohamed Bechir Ben Hamida. 2023. "Natural Convection in a Circular Enclosure with Four Cylinders under Magnetic Field: Application to Heat Exchanger" Processes 11, no. 8: 2444. https://doi.org/10.3390/pr11082444
APA StyleAzzouz, R., & Hamida, M. B. B. (2023). Natural Convection in a Circular Enclosure with Four Cylinders under Magnetic Field: Application to Heat Exchanger. Processes, 11(8), 2444. https://doi.org/10.3390/pr11082444







