Energy Dissipation of Hydraulic Support Columns under Rockfall Impact Load in Steeply Dipping Coal Seams
Abstract
:1. Introduction
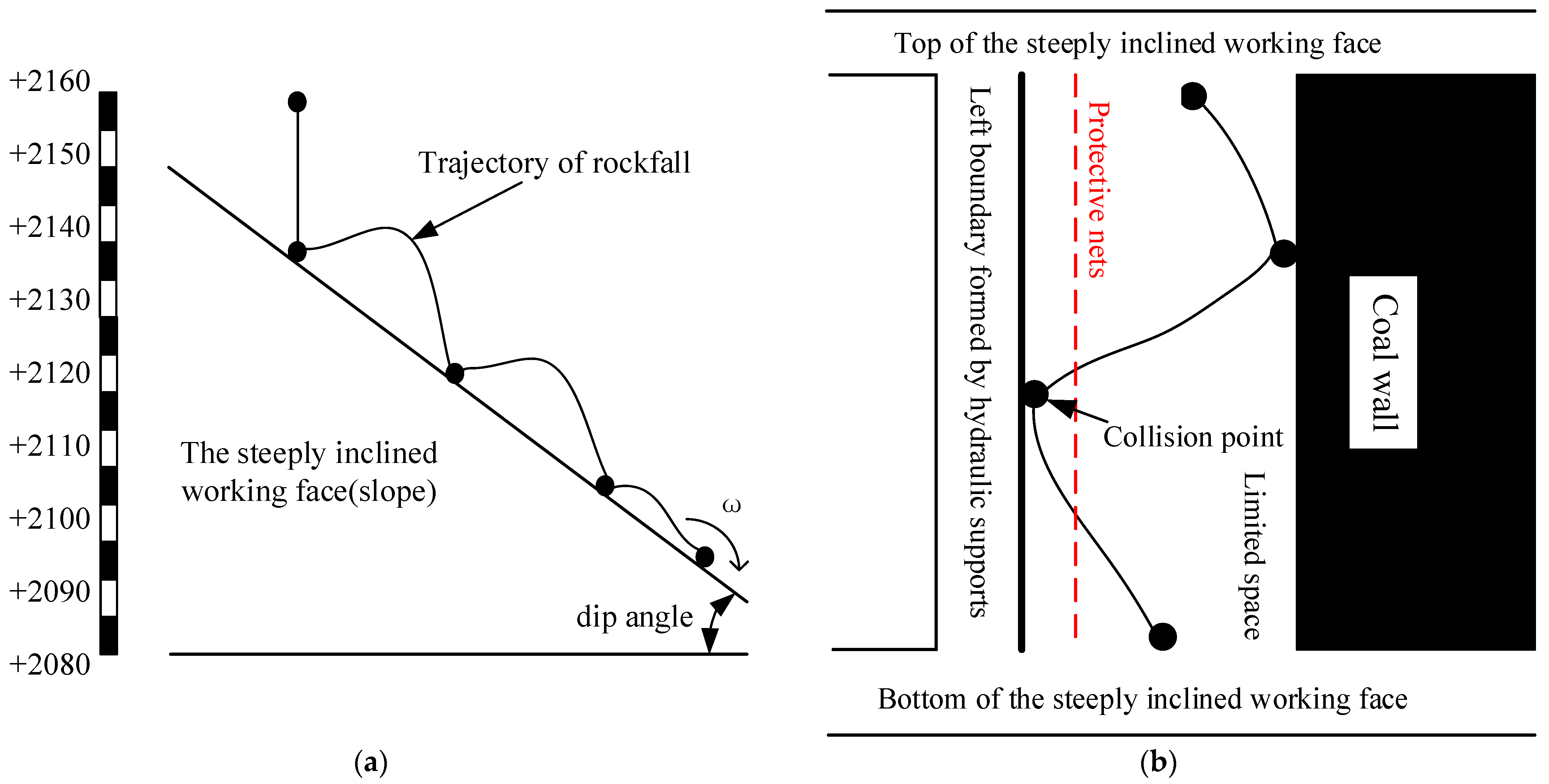
2. Rockfall Separation Mechanism
3. Particle Flow Method and Hertz Contact Theory
3.1. Particle Flow Code (PFC)
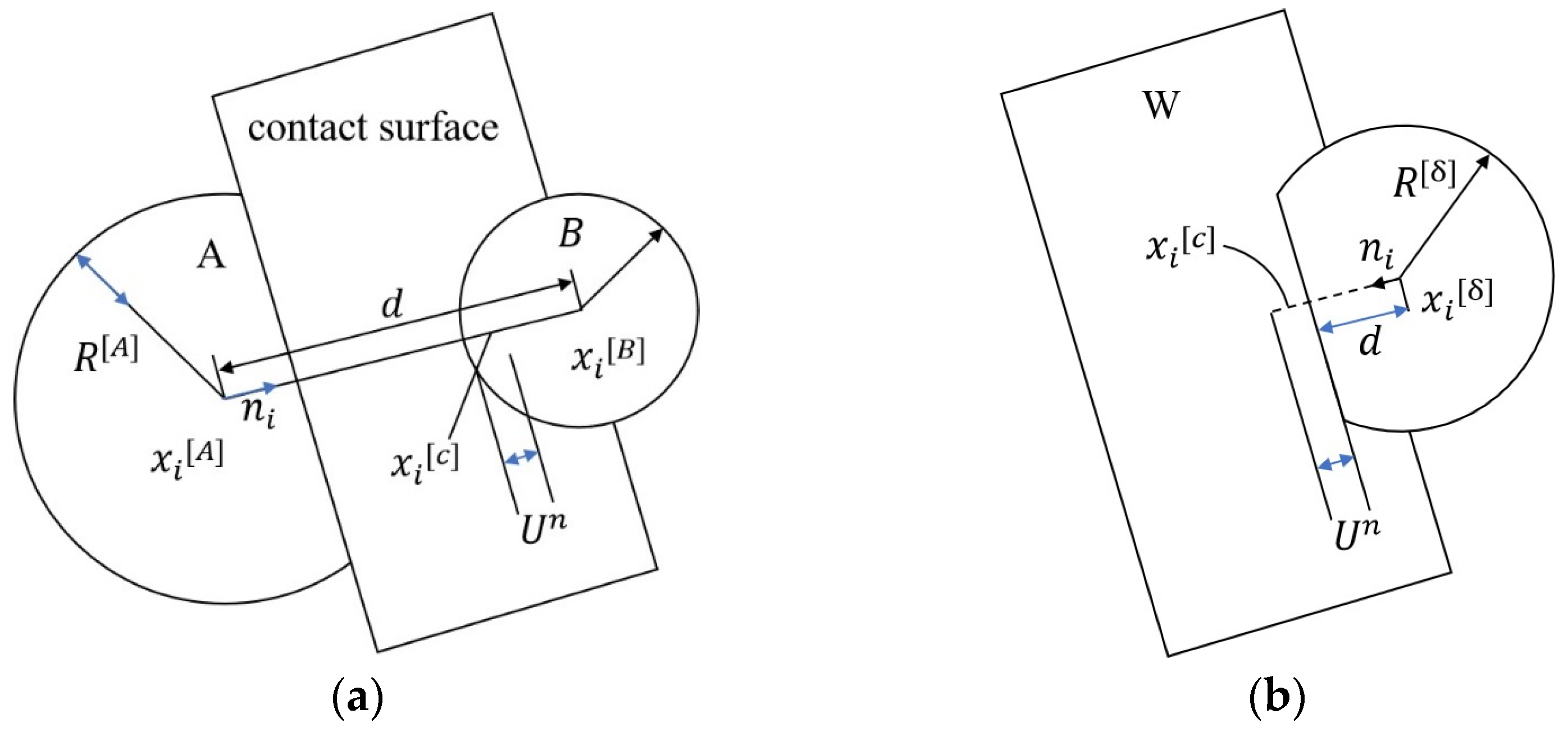
3.2. Hertz Contact Theory
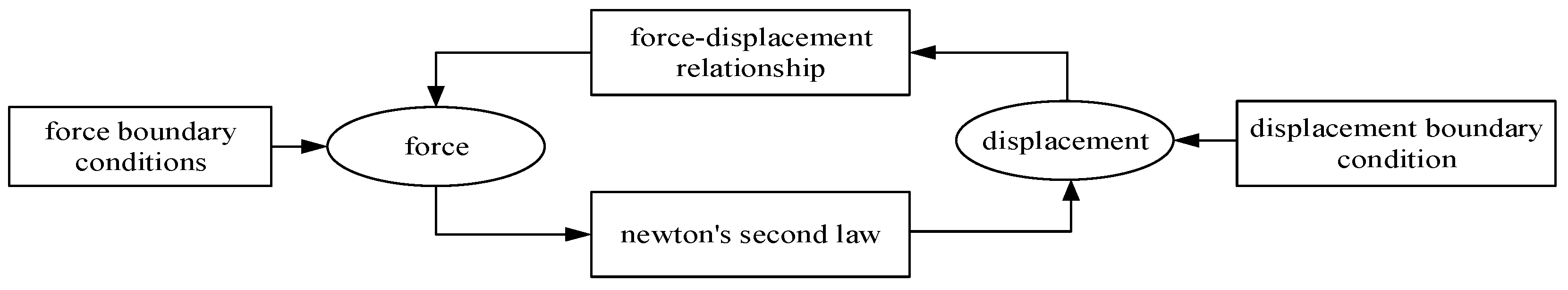
4. Energy Formula
- (1)
- Kinetic energy formula
- (2)
- Potential energy calculation
- (3)
- External force virtual work
- (4)
- Differential equations of motion
- (5)
- Galerkin discretization
5. Numerical Simulation
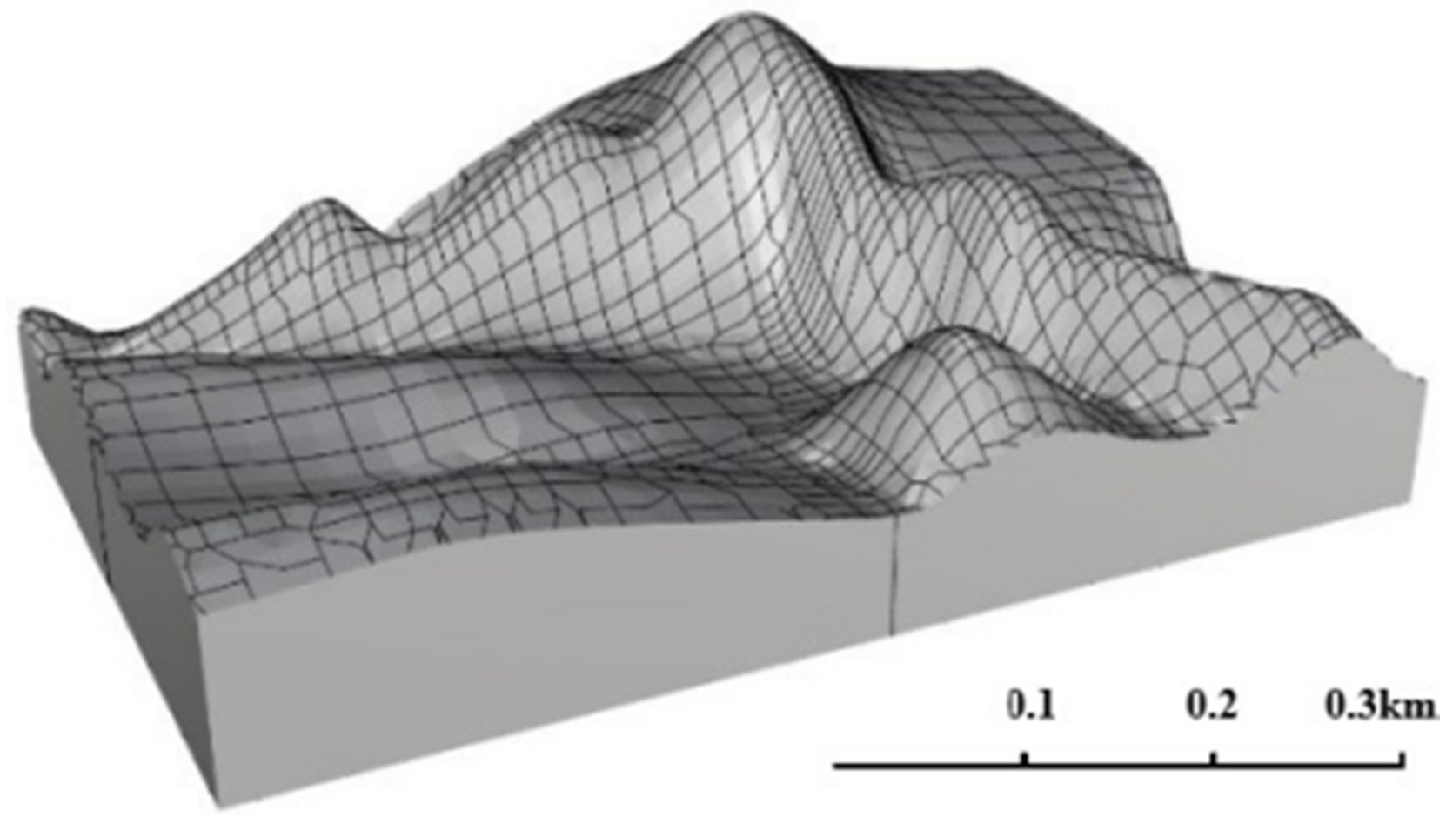
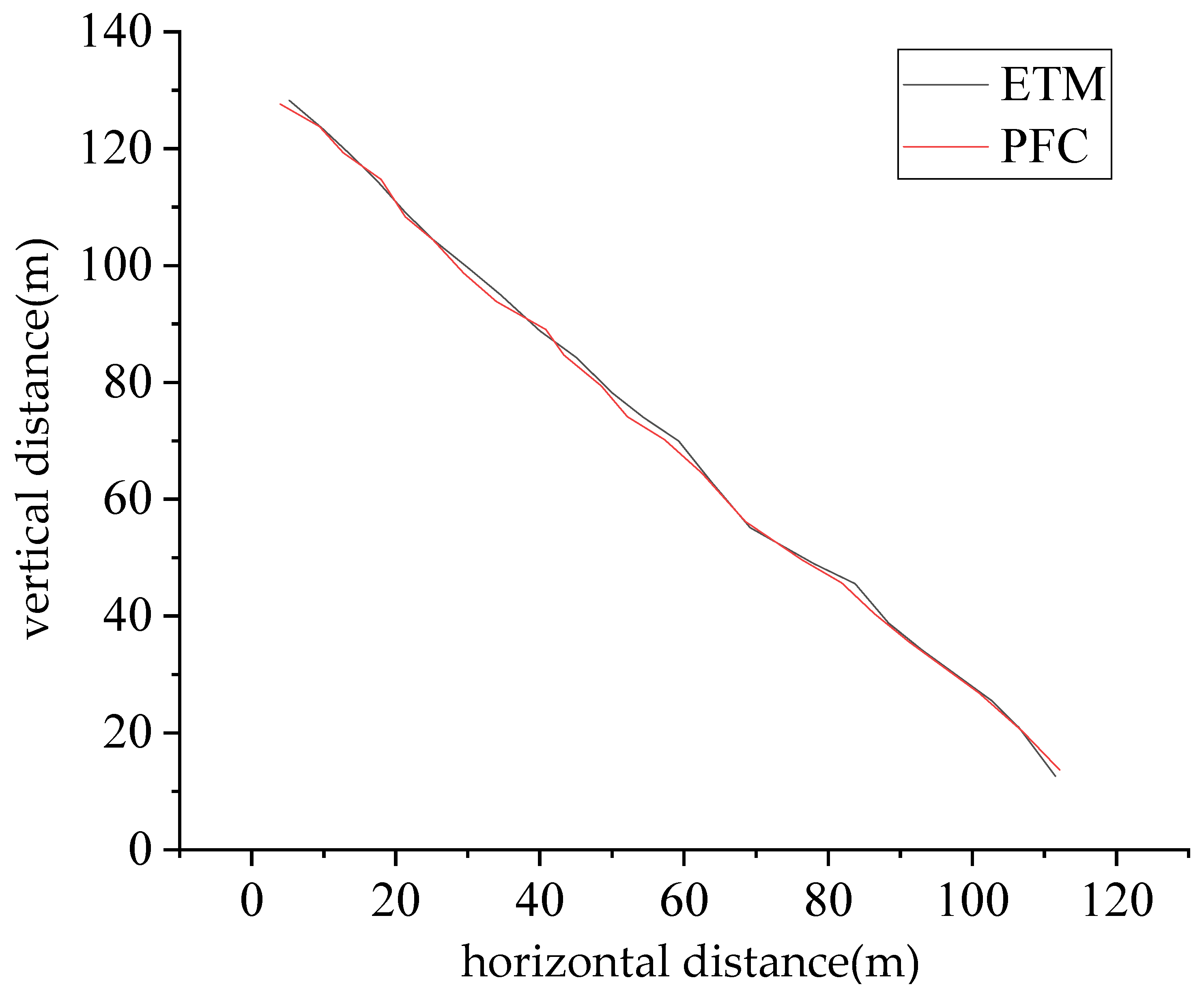
5.1. PFC3D Software to Simulate the Process of Rockfall Migration
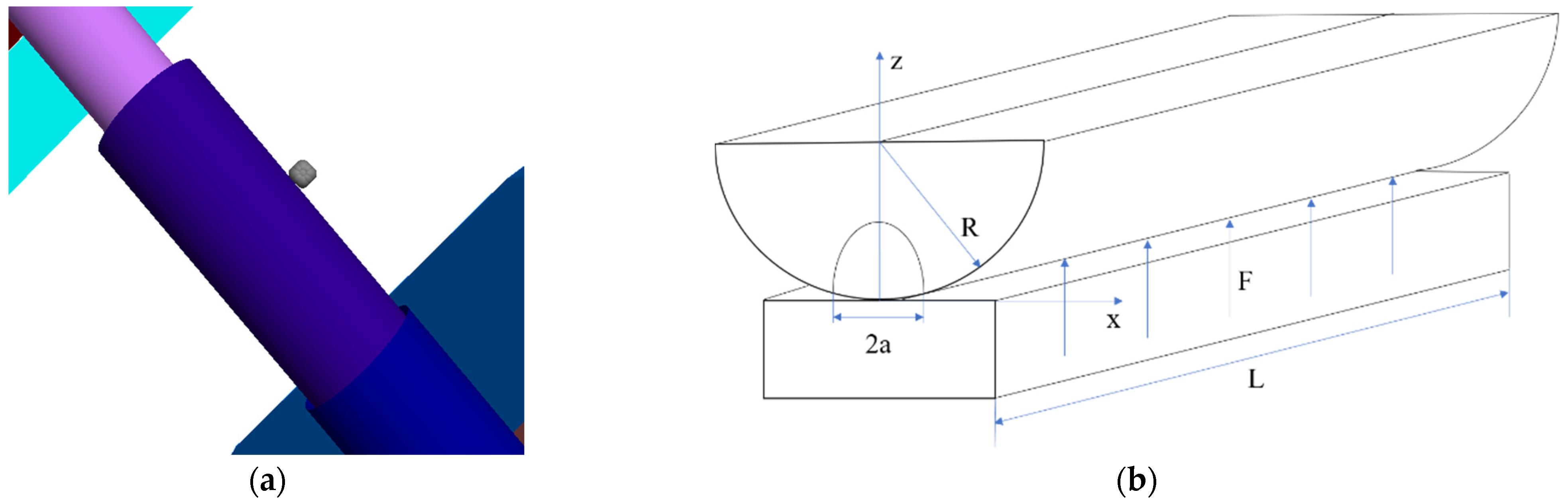
5.2. The Maximum Contact Deformation of the Collision Process between Rockfall and Hydraulic Support Column
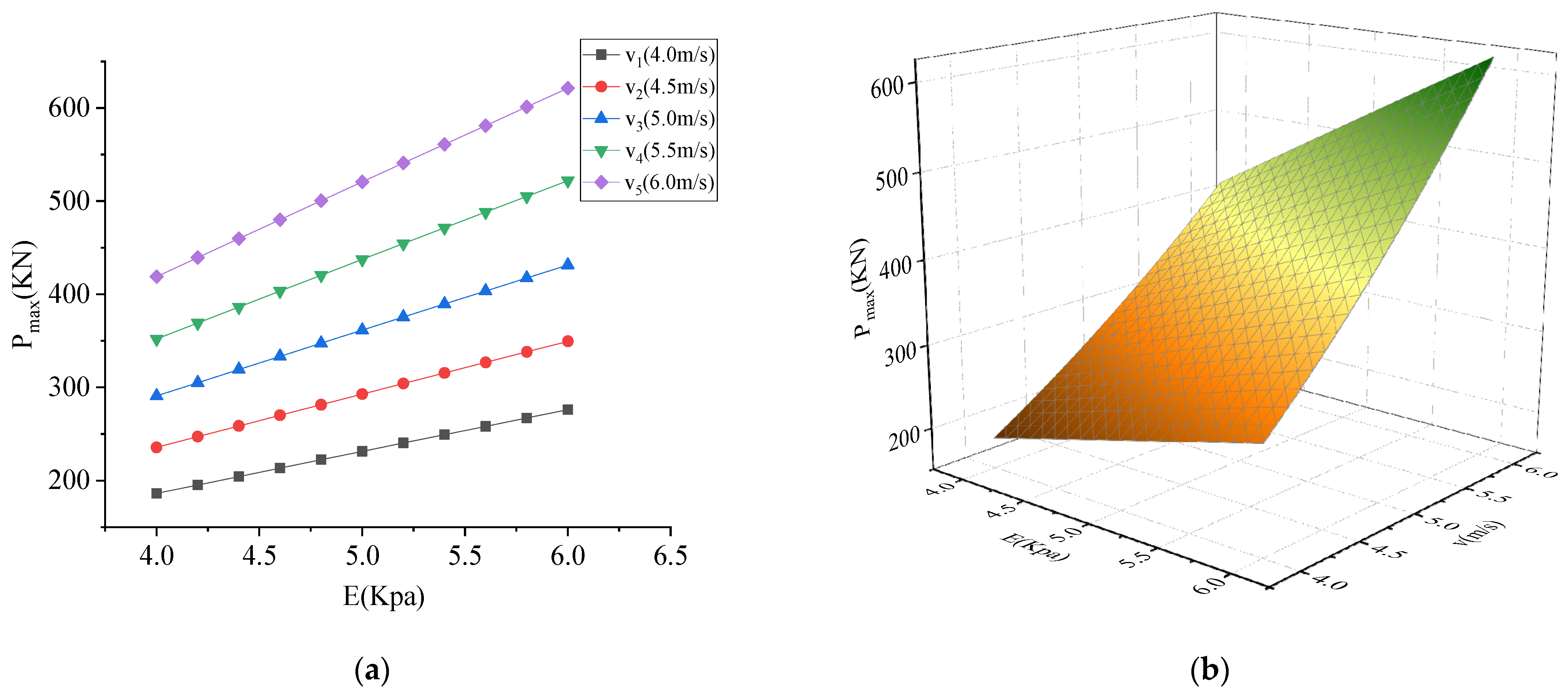
5.3. The Maximum Impact Force of the Collision Process between Rockfall and Hydraulic Support Column
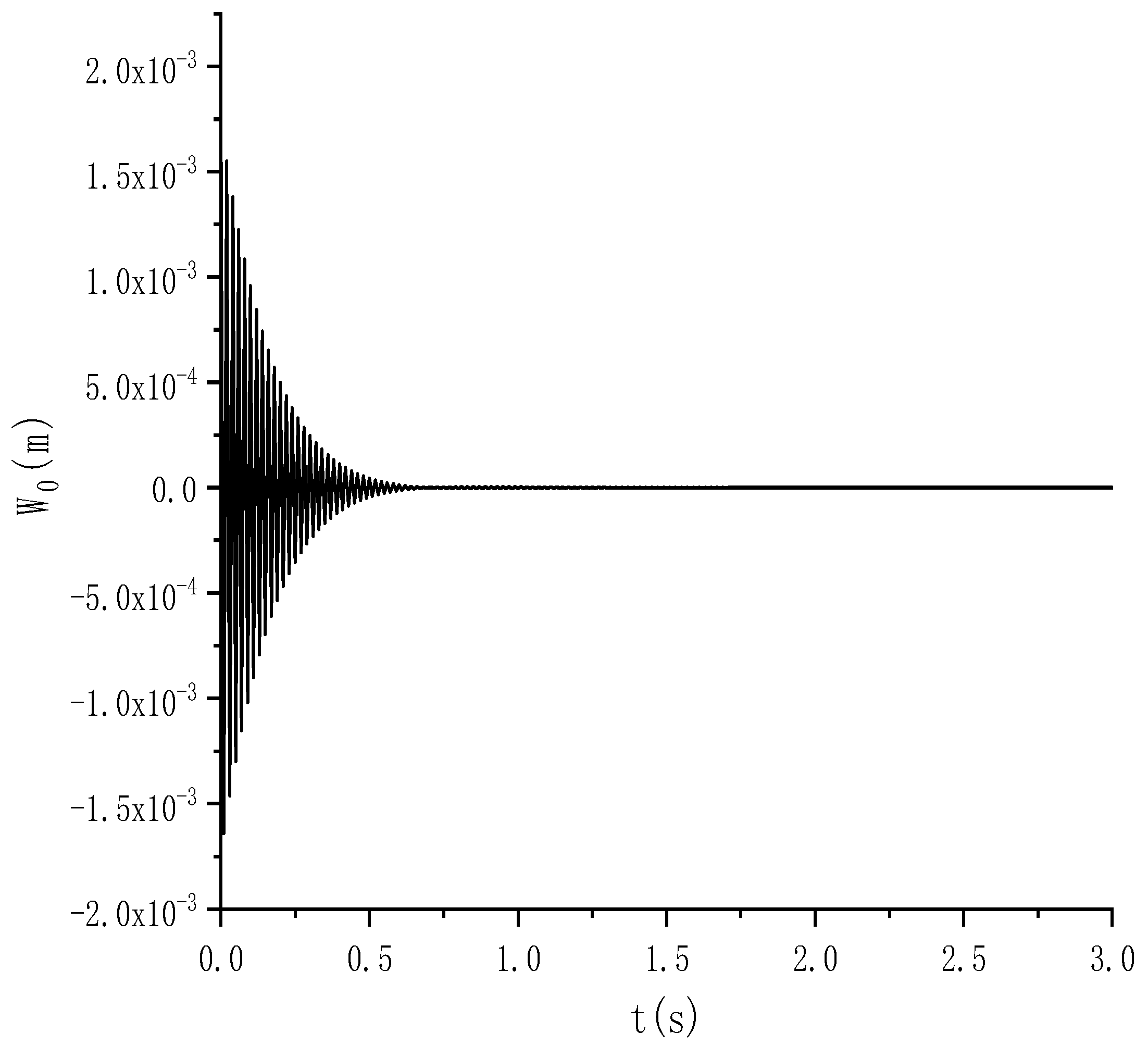
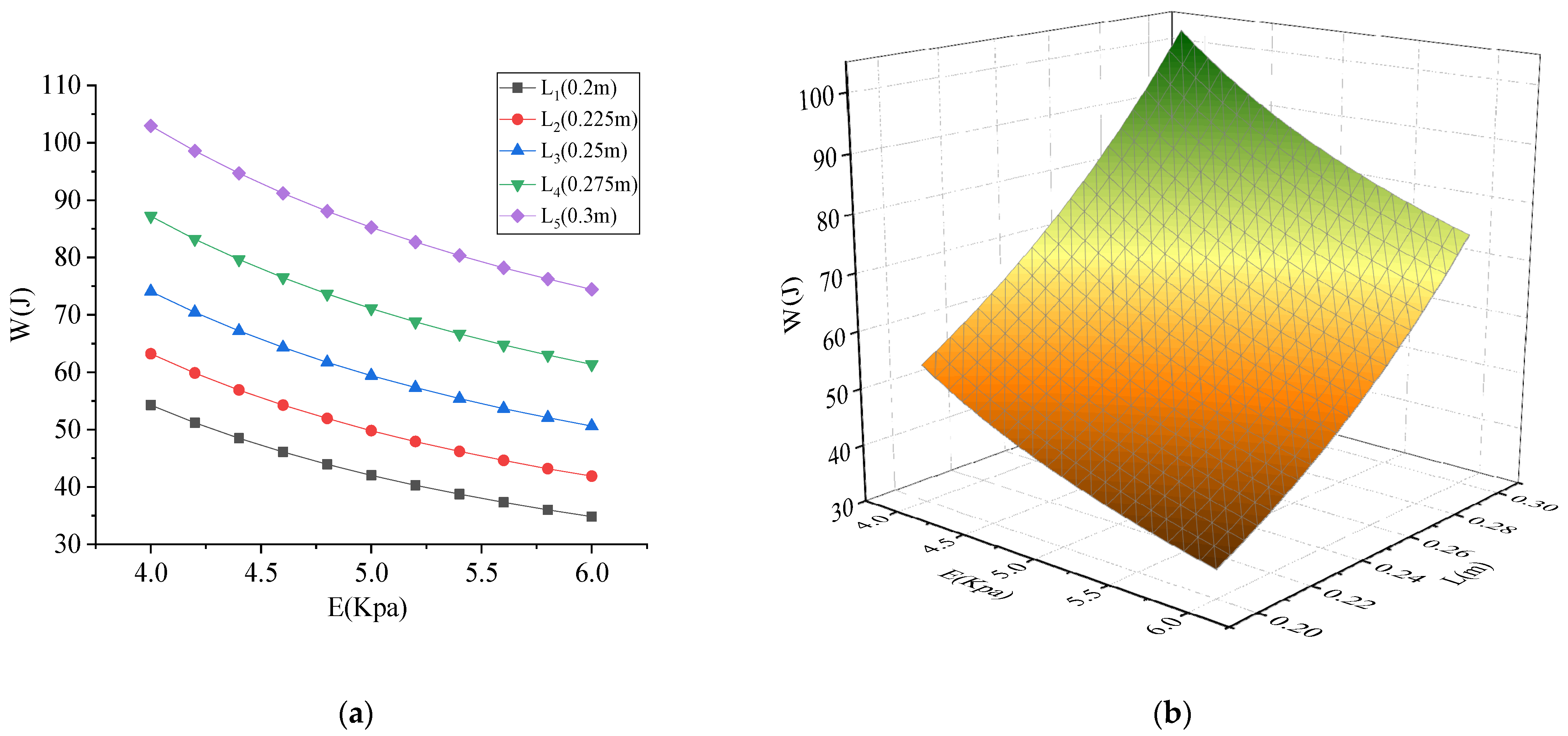
5.4. Absorbed Energy of the Collision Process between Rockfall and Hydraulic Support Column
5.5. Energy Distribution of the Collision Process between Rockfall and Hydraulic Support Column
6. Conclusions
- (1)
- Through the statistical analysis of the measured random separation in the working face, it can be seen that the mechanical state of the coal wall in the upper and lower parts of the inclined direction of the working face has a strong correlation with the number of randomly separated blocks, and the stress state of the coal wall in the middle has a weak correlation with the number of randomly separated blocks. According to the location of rockfall formation and the characteristics of rockfall trajectory, the principle of rockfall control can be determined as follows: the upper rockfall focuses on trajectory blocking, the middle rockfall emphasizes source control, and the lower rockfall prevents secondary disasters. The lower part of the working face is the core area of rockfall prevention and control, and the protection level should be strengthened.
- (2)
- Through the field measurements and statistics of the damage to key equipment in the working face, it was found that the frequency of damage to the hydraulic support column was higher. Therefore, a contact model which can describe the collision process between a polyhedron rockfall and hydraulic support column was constructed in this paper, and the maximum deformation and maximum impact force of the collision can be obtained. The results show that the rockfall velocity and block degree of rockfall have the greatest influence on the maximum deformation and maximum impact force.
- (3)
- In order to provide safer measures to protect against rockfall disasters, this study modeled the most dangerous situation, i.e., the maximum contact deformation between the rockfall and the column was inflicted on the column. Therefore, this study innovatively assigned the maximum contact deformation between the rockfall and the column to the initial displacement in the dynamic equation of the column, and the evolution law of the energy absorbed by the column was studied when the rockfall impacts the column. The results show that the rockfall velocity and block degree of rockfall have the greatest influence on the energy absorption and maximum impact force of rockfall. Measures should be taken to reduce the impact velocity of rockfall and prevent the occurrence of large-bulk rockfall in SDCSs.
- (4)
- An accurate description of the rockfall migration characteristics and energy dissipation process in the process of rockfall impact forms the basis for the design of rockfall protection devices. In practice, protection design can be carried out according to the impact position of the rockfall and the energy dissipation characteristics in the process of rockfall impact. The research results of this paper can provide a theoretical basis for the placement of rockfall protection devices and the design of protection device materials.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Cichowicz, A. Analysis of rockburst and rockfall accidents in relation to class of stope support, regional support, energy of seismic events and mining layout. Atherosclerosis 1994, 143, 99–104. [Google Scholar]
- Giacomini, A.; Thoeni, K.; Santise, M.; Diotri, F.; Booth, S.; Fityus, S.; Roncella, R. Temporal-spatial frequency rockfall data from open-pit highwalls using a low-cost monitoring system. Remote Sens. 2020, 12, 2459. [Google Scholar] [CrossRef]
- Ferrari, F.; Giacomini, A.; Thoeni, K.; Lambert, C. Qualitative evolving rockfall hazard assessment for highwalls. Int. J. Rock Mech. Min. Sci. 2017, 98, 88–101. [Google Scholar] [CrossRef]
- Zheng, D.; Frost, J.D.; Huang, R.D.; Liu, F.Z. Failure process and modes of rockfall induced by underground mining: A case study of Kaiyang Phosphorite Mine rockfalls. Eng. Geol. 2015, 197, 145–157. [Google Scholar] [CrossRef]
- Wu, Y.P.; Yun, D.F.; Xie, P.S.; Wang, H.W.; Lang, D. Progress, practice and scientific issues in steeply dipping seams fully-mechanized mining. J. China Coal Soc. 2020, 45, 24–34. [Google Scholar]
- Wu, Y.P.; Liu, K.Z.; Yun, D.F.; Xie, P.S.; Wang, H.W. Research progress on the safe and efficient mining technology of steeply dipping seam. J. China Coal Soc. 2014, 39, 1611–1618. [Google Scholar]
- Hu, B.S.; Wu, Y.P.; Wang, H.W.; Tang, Y.P.; Wang, C.R. Risk mitigation for rockfall hazards in steeply dipping seam: A case study in Xinjiang, northwestern China. Geomat. Nat. Hazards Risk 2021, 12, 988–1014. [Google Scholar] [CrossRef]
- Tu, H.S.; Tu, S.H.; Yuan, Y.; Wang, F.T.; Bai, Q.S. Present situation of fully mechanized mining technology for steeply inclined coal seams in China. Arab. J. Geosci. 2015, 8, 4485–4494. [Google Scholar] [CrossRef]
- Wu, Y.P.; Yun, D.F.; Xie, P.S.; Wang, H.W.; Luo, S.H. Theory and Technology of Fully Mechanized Longwall Mining in Steeply Inclined Seam; Science Press: Beijing, China, 2017. [Google Scholar]
- Wu, Y.P.; Hu, B.S.; Xie, P.S.; Li, S.H.; Huangfu, J.Y. Impact damage of flying gangue in steeply dipping seams and its control. J. China Coal Soc. 2018, 43, 2694–2702. [Google Scholar]
- Wu, Y.P.; Hu, B.S.; Lang, D.; Tang, Y.P. Risk assessment approach for rockfall hazards in steeply dipping coal seams. Int. J. Rock Mech. Min. Sci. 2021, 138, 104626. [Google Scholar] [CrossRef]
- Hu, B.S. Damage mechanism and control of flying-gangue hazard in longwall mining of steeply dipping coal seams. Chin. J. Rock Mech. Eng. 2022, 41, 2592. [Google Scholar]
- Wu, Y.P.; Wu, Y.P.; Xie, P.S.; Zeng, Y.F. Flying gangue regional control technology in longwall mining face of steeply dipping seam. Coal Sci. Technol. 2017, 45, 1–5. [Google Scholar]
- Liu, M.; Wu, Y.P.; Geng, S.; Duo, Y.L.; Lv, W.Y. Threat level assessment of flying gangue in steep seam mining. J. China Coal Soc. 2020, 45, 3688–3695. [Google Scholar]
- Liu, M.; Zhou, Y.T.; Lv, W.Y. Normal characteristic quantity theory of flying gangue motion in steeply dipping seam with stochastic parameters. J. China Coal Soc. 2021, 46, 2237–2244. [Google Scholar]
- Liu, M.; Chen, J.; Xiao, Y.; Lv, W.Y. Migration law for random parameters rockfall in steeply dipping coal seams. Front. Earth Sci. 2023, 11, 1088188. [Google Scholar] [CrossRef]
- Chen, J. Study on the Migration Law of Flying Gangue in Steeply Dipping Coal Seam Based on Energy Tracking Method; Liaoning Petrochemical University: Fushun, China, 2021. [Google Scholar]
- Wang, J.A.; Jiao, J.L. Criteria of support stability in mining of steeply inclined thick coal seam. Int. J. Rock Mech. Min. Sci. 2016, 82, 22–35. [Google Scholar] [CrossRef]
- Yao, Q.L.; Li, X.H.; Sun, B.Y.; Ju, M.H.; Chen, T.; Zhou, J.; Liang, S.; Qu, Q.D. Numerical investigation of the effects of coal seam dip angle on coal wall stability. Int. J. Rock Mech. Min. Sci. 2017, 100, 298–309. [Google Scholar] [CrossRef]
- Sławomir, B.; Stanisław, P. Numerical study of pressure on dams in a backfilled mining shaft based on PFC3D code. Comput. Geotech. 2015, 66, 230–244. [Google Scholar]
- Fu, G.H. An Extension of Hertz’s Theory in Contact Mechanics. J. Appl. Mech. 2006, 74, 373–374. [Google Scholar] [CrossRef]
- Margarida, M.; Pedro, M.; Paulo, F. Compliant contact force models in multibody dynamics: Evolution of the Hertz contact theory. Mech. Mach. Theory 2012, 53, 99–121. [Google Scholar]
- Zhupanska, O.I. Contact problem for elastic spheres: Applicability of the Hertz theory to non-small contact areas. Int. J. Eng. Sci. 2011, 49, 576–588. [Google Scholar] [CrossRef]
- Fu, X.L.; Liao, W.H. Nondimensional model and parametric studies of impact piezoelectric energy harvesting with dissipation. J. Sound Vib. 2018, 429, 78–95. [Google Scholar] [CrossRef]
- Chen, S.; Yao, Z.J.; Goldsmith, C.L. A new in situ residual stress measurement method for a MEMS thin fixed-fixed beam structure. J. Microelectromech. Syst. 2002, 11, 309–316. [Google Scholar] [CrossRef]
- Xu, J.J.; Tang, X.H.; Liu, Q.S.; Feng, Y.F. Investigation on trajectory of rolling rock affected by rock fragmentation based on energy tracking method. Rock Soil Mech. 2019, 40, 541–548. [Google Scholar]
- Paluszny, A.; Tang, X.H.; Zimmerman, R.W. Fracture and impulse based finite-discrete element modelling of fragmentation. Comput. Mech. 2013, 52, 1071–1084. [Google Scholar] [CrossRef]
- Tang, X.H.; Paluszny, A.; Zimmerman, R.W. Energy conservative property of impulse-based methods for collision resolution. Int. J. Numer. Methods Eng. 2013, 95, 529–540. [Google Scholar] [CrossRef]
- Tang, X.H.; Paluszny, A.; Zimmerman, R.W. An impulse-based energy tracking method for collision resolution. Comput. Methods Appl. Mech. Eng. 2014, 278, 160–185. [Google Scholar] [CrossRef]
- Paluszny, A.; Tang, X.H.; Nejati, M.; Zimmerman, R.W. A direct fragmentation method with Weibull function distribution of sizes based on finite- and discrete element simulations. Int. J. Solids Struct. 2016, 80, 38–51. [Google Scholar] [CrossRef]
- Xie, P.S.; Zhang, Y.Y.; Zhang, Y.L.; Chen, J.J.; Zhang, X.B.; Duan, J.J. Instability law of the coal-rock interbedded roof and its influence on supports in large mining height working face with steeply dipping coal seam. J. China Coal Soc. 2021, 46, 344–356. [Google Scholar]
- Yang, Y.; Zeng, Q.L. Influence analysis of the elastic supporting to the dynamic response when the spherical rock elastic impacting the metal plate and to the coal gangue impact differences. IEEE Access 2019, 7, 143347–143366. [Google Scholar] [CrossRef]
- Yang, Y.; Zeng, Q.L.; Wan, L. Dynamic response analysis of the vertical elastic impact of the spherical rock on the metal plate. Int. J. Solids Struct. 2019, 158, 287–302. [Google Scholar] [CrossRef]
- Yang, Y.; Zhang, Y.; Zeng, Q.L.; Wan, L.R.; Zhang, Q. Simulation research on impact contact behavior between coal gangue particle and the hydraulic support: Contact response differences induced by the difference in impacted location and impact material. Materials 2022, 15, 3890. [Google Scholar] [CrossRef] [PubMed]
- Yang, Y.; Zeng, Q.L.; Wan, L.R. Contact response analysis of vertical impact between elastic sphere and elastic half space. Shock. Vib. 2018, 2018, 1802174. [Google Scholar] [CrossRef]
- Yang, Y.; Wan, L.R. Study on the vibroimpact response of the particle elastic impact on the metal plate. Shock. Vib. 2019, 2019, 6325472. [Google Scholar] [CrossRef]
- Zeng, Q.L.; Yang, Y.; Zhang, X.; Wan, L.R.; Zhou, J.H.; Yin, G.J. Study on metal plate vibration response under coal–gangue impact based on 3D simulation. Arab. J. Sci. Eng. 2019, 44, 7567–7580. [Google Scholar] [CrossRef]

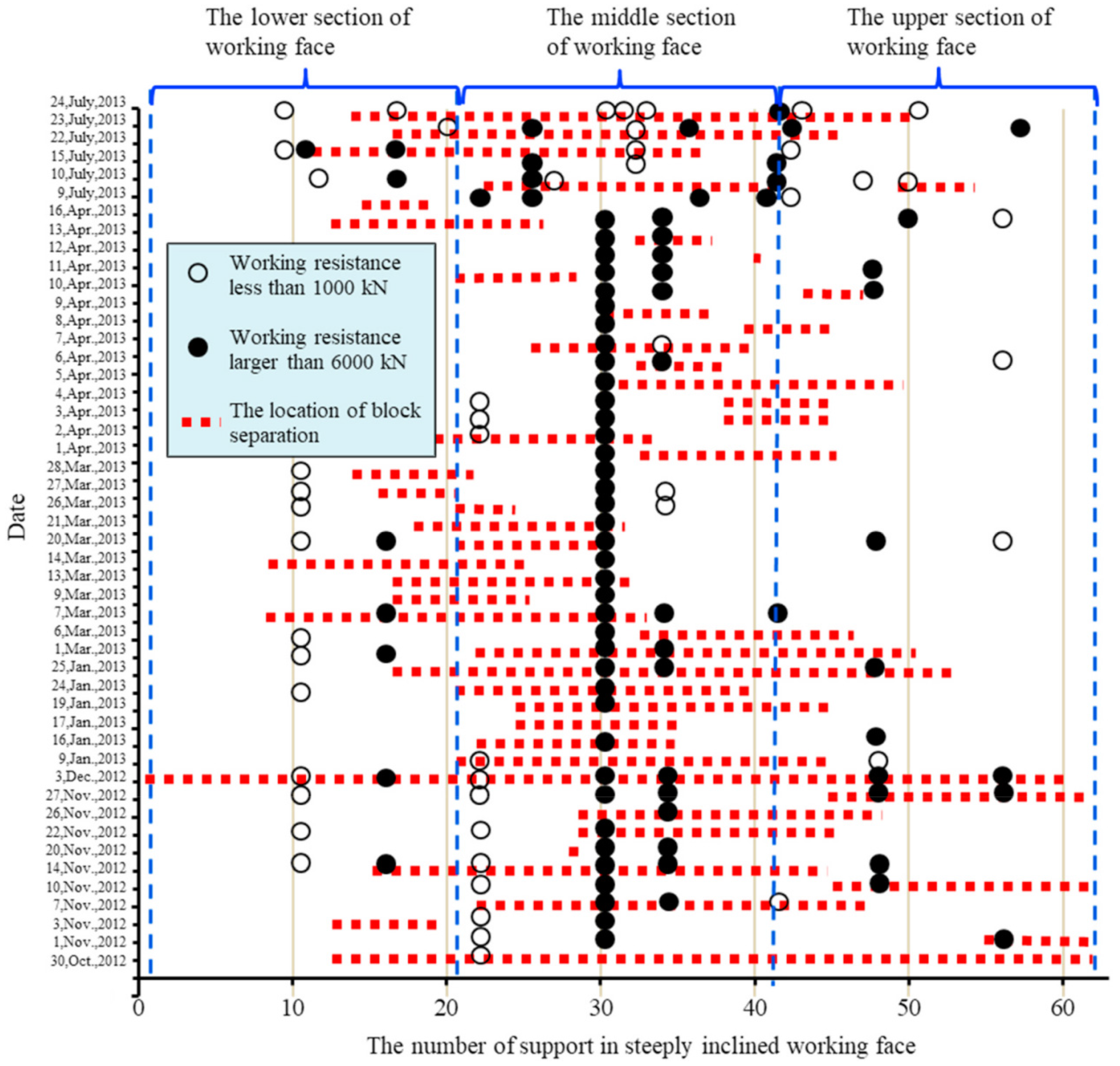



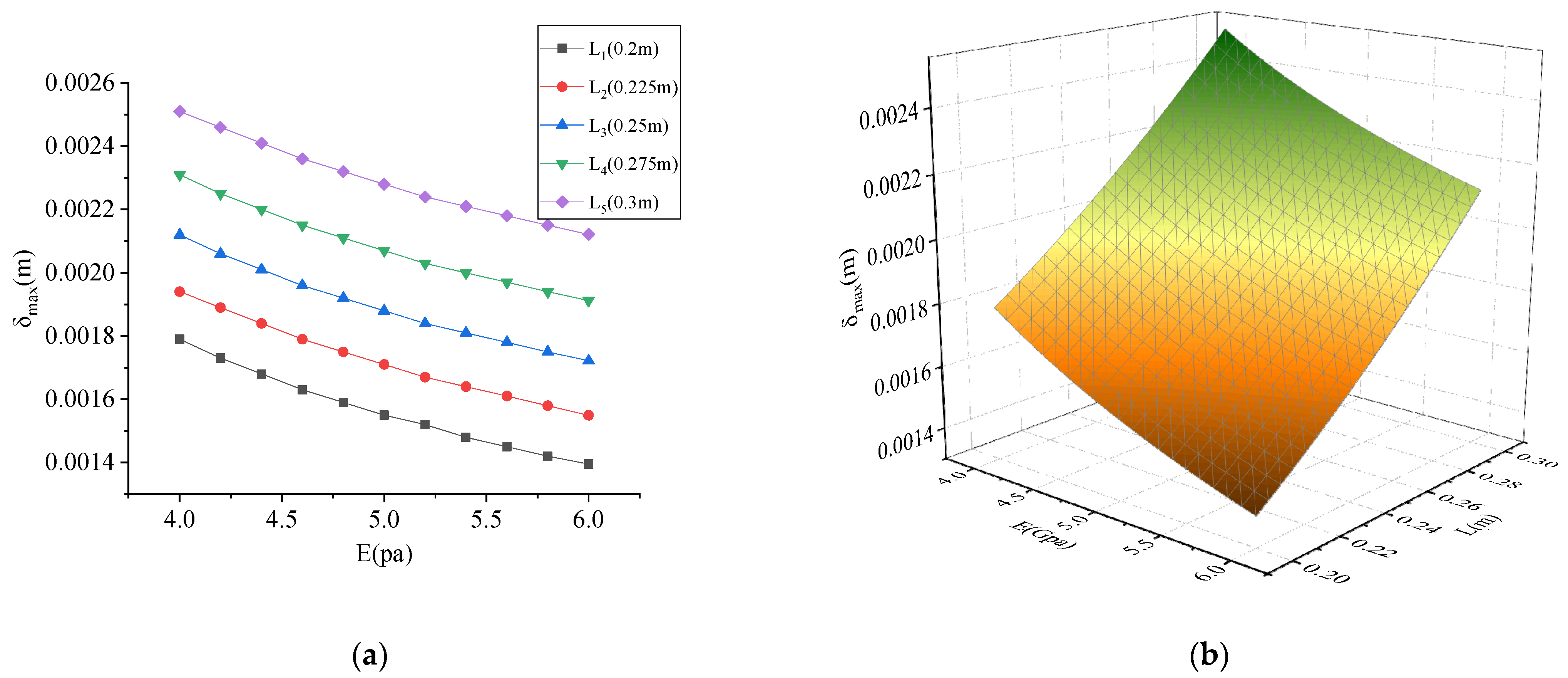
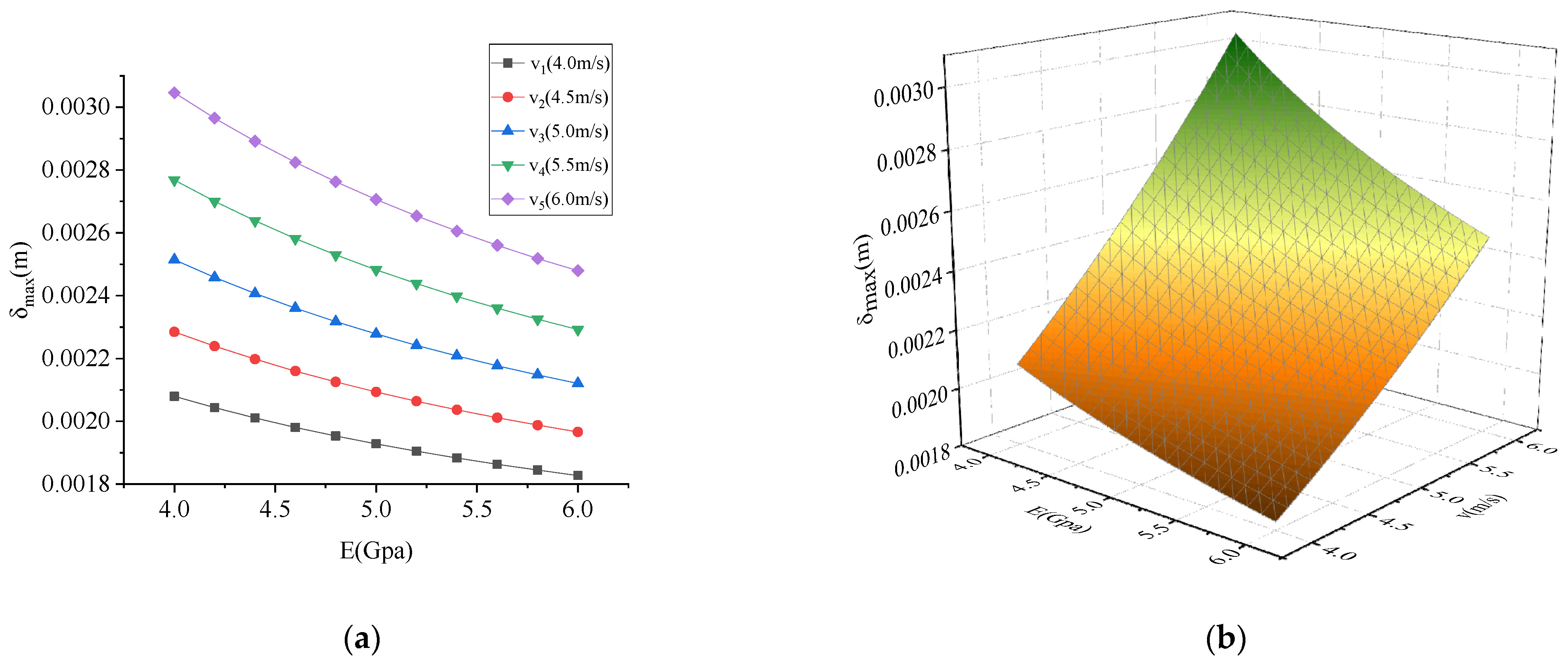
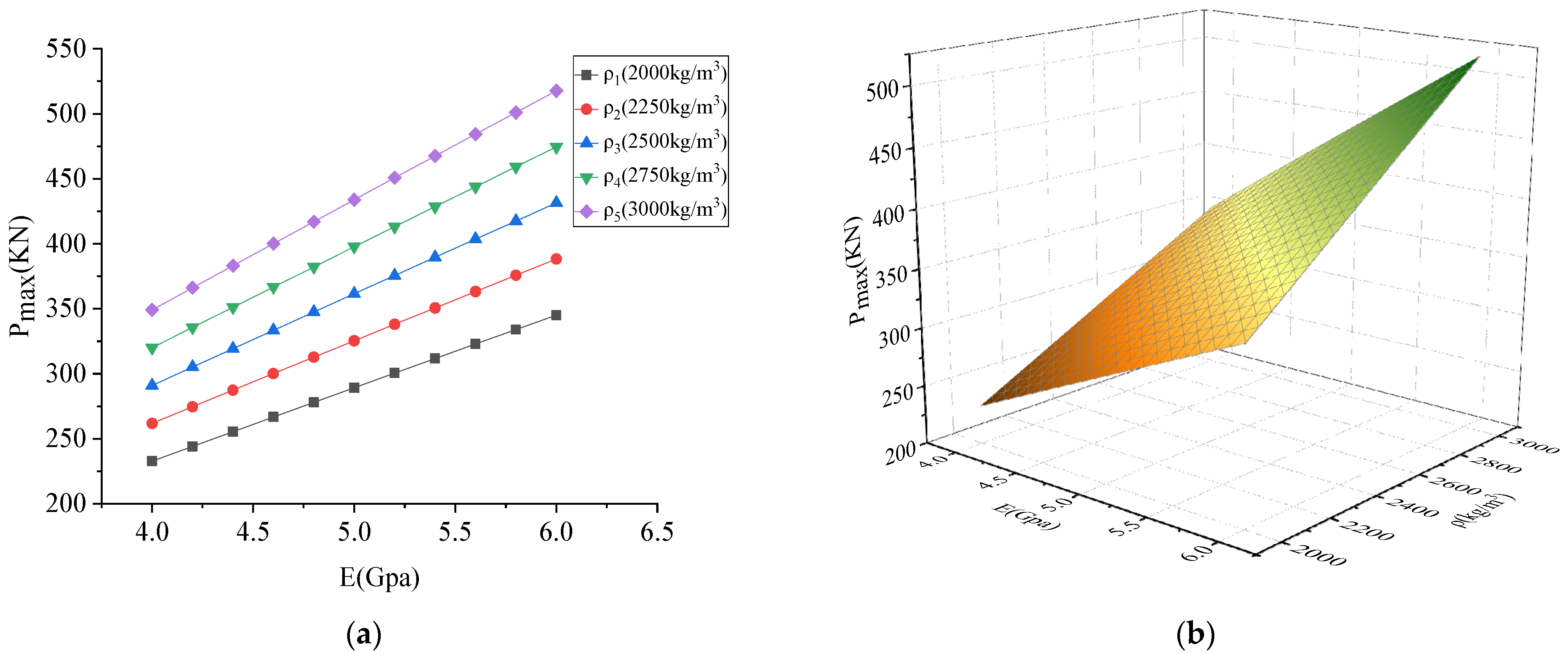
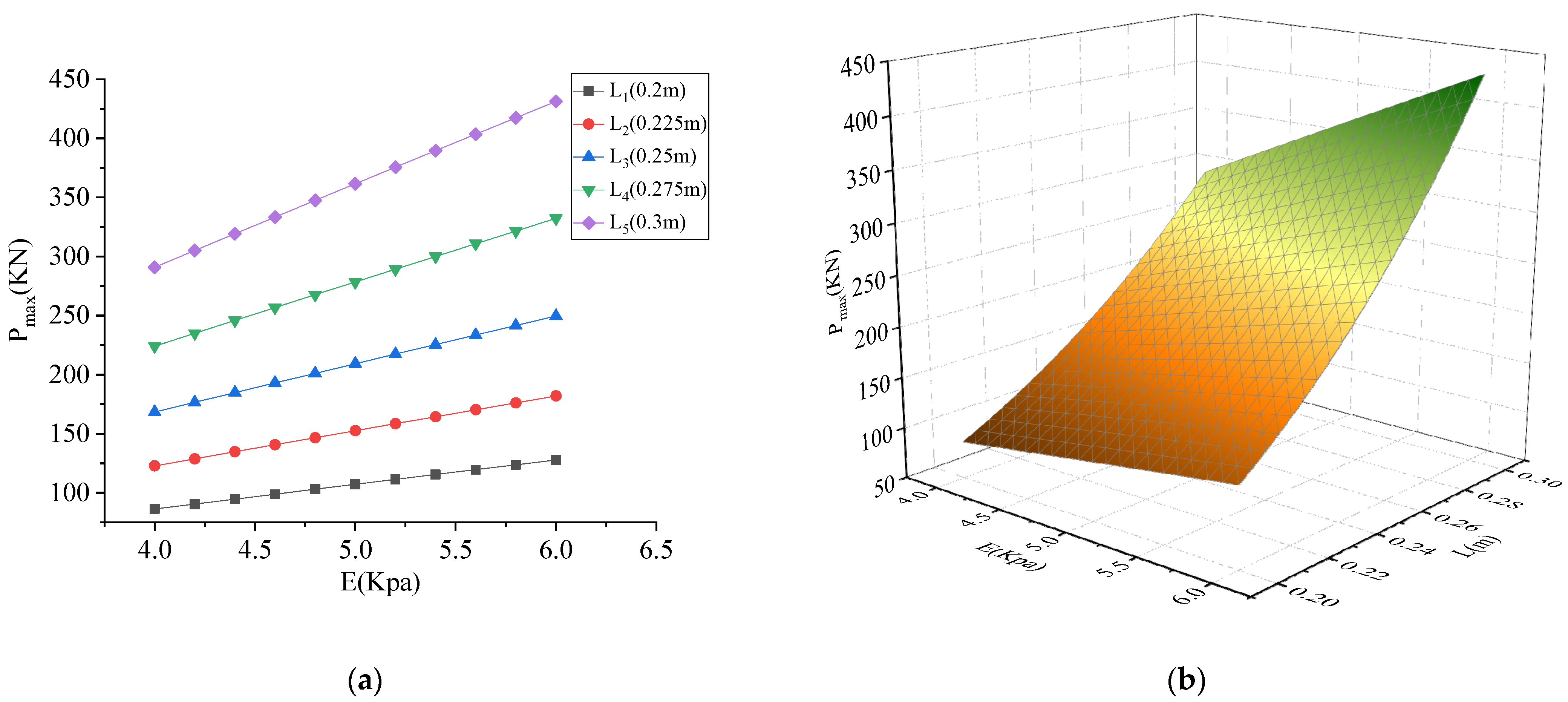



| Items | Lower Section | Middle Section | Upper Section | Total |
|---|---|---|---|---|
| Counts of coal wall spalling (A) | 118 | 375 | 150 | 643 |
| Counts of working resistance greater than 6000 kN (B) | 8 | 65 | 14 | 87 |
| Counts of working resistance less than 1000 kN (C) | 22 | 17 | 8 | 47 |
| A∩B | 5 | 32 | 9 | 46 |
| A∩C | 4.5 | 10.5 | 2 | 17 |
| P1(A∩B) | 62.5 | 49.2 | 64.3 | ∕ |
| P2(A∩C) | 20.5 | 61.8 | 25.0 | ∕ |
| Density (kg/m3) | Elasticity Modulus (Gpa) | Tangential Stiffness (N/m) | Normal Stiffness (N/m) | Poisson’s Ratio | Friction Coefficient | Side Length (m) |
|---|---|---|---|---|---|---|
| 2500 | 5 | 1 | 1 | 0.28 | 0.2 | 0.3 |
| Density (kg/m3) | Dip Angle (°) | Internal Friction Angle (°) | Elasticity Modulus (GPa) | Critical Damping | Working Face Length (m) |
|---|---|---|---|---|---|
| 2800 | 45 | 30 | 4.38 | 0.35 | 120 |
| Type of Hydraulic Cylinder | Cylinder Position | Piston Diameter (m) | Diameter of Piston Rod (m) | Oil Cavity Length (m) |
|---|---|---|---|---|
| Column hydraulic cylinder | First stage | 0.250 | 0.235 | 1.310 |
| Second stage | 0.180 | 0.160 | 1.285 |
| X Axis Coordinate Interval | Energy Proportion Sorting |
|---|---|
| (0.667, 0.833) | > > > |
| (0.833, 1.000000) | > > > |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, M.; Luan, B.; Xiao, Y. Energy Dissipation of Hydraulic Support Columns under Rockfall Impact Load in Steeply Dipping Coal Seams. Processes 2023, 11, 2497. https://doi.org/10.3390/pr11082497
Liu M, Luan B, Xiao Y. Energy Dissipation of Hydraulic Support Columns under Rockfall Impact Load in Steeply Dipping Coal Seams. Processes. 2023; 11(8):2497. https://doi.org/10.3390/pr11082497
Chicago/Turabian StyleLiu, Ming, Bohao Luan, and Yang Xiao. 2023. "Energy Dissipation of Hydraulic Support Columns under Rockfall Impact Load in Steeply Dipping Coal Seams" Processes 11, no. 8: 2497. https://doi.org/10.3390/pr11082497
APA StyleLiu, M., Luan, B., & Xiao, Y. (2023). Energy Dissipation of Hydraulic Support Columns under Rockfall Impact Load in Steeply Dipping Coal Seams. Processes, 11(8), 2497. https://doi.org/10.3390/pr11082497






