Effect of Non-Uniform Minerals Distribution on Hydraulic Fracture Evolution during Unconventional Geoenergy Exploration

Abstract

To study the effect of the non-uniform distribution of minerals on the development of microcracks within the rock during hydraulic fracturing, a novel numerical model considering multiple random mineral distributions was designed. The model investigated the impacts of mineral grain size, composition, and spatial arrangement on fracture initiation and propagation. The results indicate that the presence of the hard-phase mineral quartz can alter the propagation path of fractures, and increase the width of hydraulic fractures. In coarse-grained granite, the range of crack deflection is maximized, while in medium-grained granite, it is more prone to forming convoluted elongated cracks. A higher quartz content in granite further contributes to the formation of complex crack networks. Simultaneously, the evolution of granite fractures and variations in breakdown pressure in heterogeneous granite were investigated, considering the influence of core parameters such as fluid injection rate, fracturing fluid viscosity, and horizontal stress difference. The research reveals that a high injection rate promotes straight-line fracture expansion. Moreover, modest fluctuations in fracturing fluid viscosity have minimal effects on fracture morphology. When the fracture development avoids quartz, under the influence of high horizontal stress differential, it clearly turns toward the direction of the maximum principal stress. This study can offer insights into innovative and optimized deep reservoir fracturing techniques.

1. Introduction

Hydraulic fracturing (HF) is a pivotal technology for the stimulation of geoenergy reservoirs [1,2,3]. Nonetheless, for fracturing operations in deep reservoirs, several common challenges arise, such as the higher breakdown pressure (BP), the creation of a singular fracture network, and the lower mining efficiency [4,5,6]. Rock damage usually initiates at the mesoscale aspect [7]. Examining the above phenomena from a meso-mechanical perspective can improve our comprehension of the mechanisms underlying fracturing processes in reservoir stimulation. Notably, in geoenergy reservoirs predominantly composed of granite, factors such as mineral particle size, composition, and spatial arrangement exert significant influence on the evolution of hydraulic fractures [8]. Explaining the effects of mineral distribution on the fracturing processes, as well as analyzing the core determinants of BP and fracture network complexity, is crucial for deep reservoir stimulation.

Granite, predominantly comprised of minerals like feldspar, quartz, and mica, is inherently heterogeneous. Different from the anisotropy of rocks such as shale [9,10], the heterogeneity of granite arises from differences in the mechanical properties of these minerals, including strength, elastic modulus, permeability, and other factors, as well as from the non-uniform distribution of mineral components [11,12]. Quartz displays remarkable hardness, strength, and exceptionally low permeability, thereby contributing to the overall strength enhancement and porosity reduction of the rock. In contrast, mica, a mineral characterized by lower strength, exhibits higher permeability. The strength, hardness, permeability, and other parameters of feldspar are between the two minerals [13]. During the process of rock fracturing, microcracks initially emerge in mica and are subsequently observed in quartz. The primary crack propagates toward areas characterized by a greater abundance of mica [14]. In addition to variations in mineral mechanical properties, factors such as mineral grain size, relative content, and spatial arrangement have a direct influence on the macroscopic mechanical behavior of the rock. The distribution of diverse mineral grain sizes within granite plays a pivotal role in shaping its inherent structure. The presence of larger mineral particles can give rise to significant gaps and fractures among them, resulting in reduced macroscopic rock strength and increased permeability [15,16]. Additionally, differences in mineral particle sizes can lead to stress concentration within the rock, which in turn affects the fracture patterns and overall stability of the rock [17]. Granite with a higher quartz content generally displays a higher elastic modulus and greater strength, while the presence of mica tends to have an adverse correlation with the tensile and compressive strength of granite [18,19]. The spatial arrangement of minerals can influence fluid permeability pathways and stress distribution. If mineral particles are uniformly mixed, rocks generally exhibit greater strength and present a winding, uninterrupted pathway for crack propagation [20].

The propagation behavior of hydraulic fractures in granite is significantly influenced by mineral heterogeneity. Nonetheless, the mesoscopic rock structure has often been inadequately considered in the majority of HF experiments [21]. Faced with these time-consuming and labor-intensive challenges, numerical simulations have emerged as efficient tools for the investigation of mesoscale patterns in rock fracturing evolution during HF processes [22,23,24]. In essence, HF is a complex progressive failure behavior with fluid–solid interaction [4]. In comparison to traditional fracture problems, the interaction between crack propagation and fluid flow cannot be disregarded [25]. One of the simplest approaches to consider the influence of fluid flow in HF numerical simulations is by applying uniform fluid pressure to the crack surface [26]. However, this oversimplified approach is not applicable to the majority of scenarios. In comparison, it is more reasonable to characterize the fluid flow effect based on lubrication theory [27,28]. In this method, the relationship between flow rate and pressure gradient along a hydraulic fracture can be established using Poiseuille’s law. By considering flow velocity and pressure gradients in different directions, 3D discrete fracture analysis can be accomplished [29]. In conclusion, numerical simulation is an effective method to analyze fluid flow mechanisms.

In terms of mesoscopic numerical simulation, Farkas et al. [28] employed a modeling approach that integrated finite elements and discrete elements to construct models of both homogeneous and heterogeneous rock specimens. They investigated and compared the variations in fracturing behavior between constant injection and cyclic progressive injection. Guo et al. [30] developed a comprehensive coupled model to explore the effects of rock heterogeneity, Young’s modulus, injection rate, and various other factors on the fracturing process. The model integrated thermoelastic mechanics, Biot’s poroelasticity theory, and mesoscale damage mechanics. Wei et al. [31], considering the interparticle contact conditions of distinct mineral particles and utilizing mineral proportions derived from grayscale analysis of rock imagery, formulated a discrete element model to represent heterogeneous granite. Kong et al. [32] conducted simulations of the HF process in heterogeneous crystalline rock based on the discrete element method (DEM), and analyzed the effects of mesoscale mechanical parameters of mineral particles and grain boundaries. Li et al. [33], building upon the aforementioned foundation, introduced a porous network model to capture the interaction behavior between hydraulic fractures and the inherent meso-structure of rocks. Their observations demonstrated that distinct spatial arrangements of mineral particles result in variations in both the length and morphology of hydraulic fractures. In summary, previous research has illuminated the mesoscopic mechanisms of the fracturing process from different perspectives. However, at the mesoscale, there is a lack of systematic and comprehensive investigation on HF under non-uniform mineral distribution (e.g., grain size, composition, spatial arrangement, etc.) in granite. The intrinsic connection between microcracks and minerals at the mesoscopic level requires further elucidation. Additionally, existing studies often employ the computationally expensive and parameter-sensitive DEM. Furthermore, the scale of DEM models can significantly affect computational results. In recent years, significant progress has been made in addressing discontinuity issues through specific techniques in the finite element method (FEM) [34,35,36]. The calculation results exhibit good consistency at a macro/mesoscopic scale. More stable FEM has great potential for meso analysis of HF.

This paper established a two-dimensional planar strain numerical model of HF in granite, focusing on the influence of the non-uniform distribution of minerals on granite HF morphology, fracture parameters, and BP. The heterogeneous model adopted the global embedded method of cohesive elements to simulate the random distribution of various minerals. Based on the KGD model, the mineral particle size, composition, and spatial arrangement constituted the study clue of HF. At the same time, in granites, the damage mechanisms of fluid injection rate (IR), fracturing fluid viscosity (FFV), and horizontal stress difference (HSD) were analyzed. This study contributes to broadening the mesoscopic cognition of the fracturing behavior on heterogeneous hot dry rock.

2. Basic Principles of Mineral Non-Uniform Distribution Model Construction

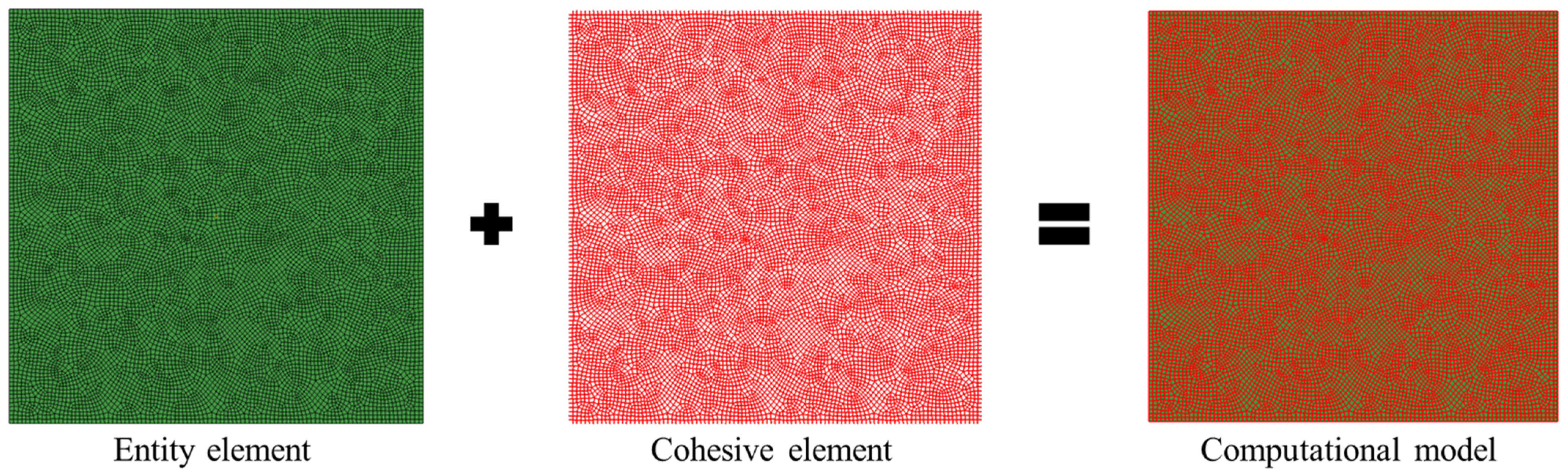
2.1. Global Cohesive Element Embedding Principle

The numerical simulation of the evolution process of hydraulic fractures can be achieved with the assistance of the built-in cohesion element in ABAQUS. This modeling method has satisfactory accuracy and reliability [37,38,39]. The cohesive element employs a traction–separation bilinear constitutive model (Figure 1a) to resist the tensile strength at the tip of the hydraulic fracture. The critical displacement corresponding to the tensile strength is ${\delta }_n^0$. When the displacement exceeds ${\delta }_n^0$, stress degradation occurs within the cohesive element. When the displacement surpasses the failure displacement δ_n, microcracks begin to propagate.

The initial damage criterion of the cohesive element in this study adopted the maximum nominal stress criterion, as shown in Equation (1):

$$\max \left\{\frac{\langle {\sigma }_n\rangle }{{\sigma }_n^0},\frac{{\sigma }_s}{{\sigma }_s^0},\frac{{\sigma }_t}{{\sigma }_t^0}\right\}=1,$$

(1)

where ${\sigma }_n^0$ is the normal stress critical value (i.e., tensile strength); ${\sigma }_s^0$ and ${\sigma }_t^0$ are the two shear stress critical values; and < > is the Macaulay bracket, which means that the cohesive element will not be damaged when it bears compressive stress. In the simulation calculation, the fluid is Newtonian fluid, and the tangential flow follows Equation (2):

$$q=-\frac{w^3}{12\mu }\nabla p_0,$$

(2)

where q is the flow rate, w is the crack width, μ is the fluid viscosity, and $\nabla$p₀ is the pressure gradient along the cohesive element. Part of the fluid in the hydraulic fracture seeps into nearby elements through normal flow, causing fluid leak-off and permeation (Figure 1b). The expression of normal flow is shown in (3):

$$\{\begin{array}{c}{q}_u=C_u\left(p_f-p_u\right)\\ q_l=C_l\left(p_f-p_l\right)\end{array},$$

(3)

where q_u and q_l are the flow rates into the top and bottom surfaces, respectively; C_u and C_l are the fluid leak-off coefficients at the top and bottom element surfaces, respectively; p_f is the midface pressure; and p_u and p_l are the pore pressures on the top and bottom surfaces, respectively.

Hydraulic fractures only propagate along the cohesive elements. Thus, simulate the authentic stochastic expansion behavior of fractures, the execution of a global embedding of zero-thickness cohesive elements was performed. It was accomplished by accessing the INP file of the ABAQUS finite element model through the Python. As depicted in Figure 2, the information related to elements and nodes obtained from the INP file was used to define the zero-thickness cohesive elements. Subsequently, these cohesive elements were integrated with solid elements to establish the computational model.

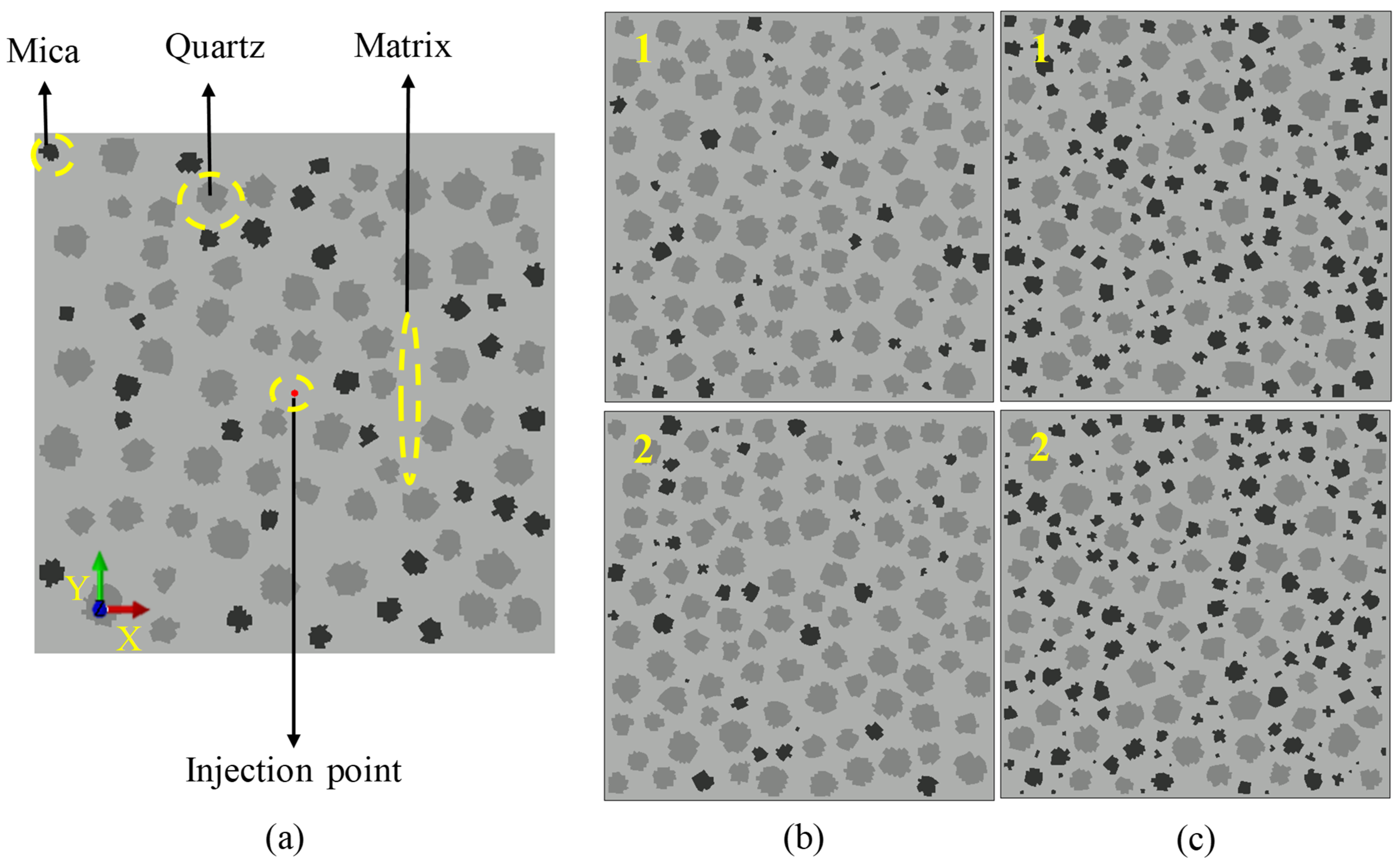
2.2. Methods for Implementing Non-Uniform Distribution of Minerals

The differences in the types, compositions, shapes, and sizes of minerals within granite have a direct influence on fluid flow. This, in turn, affects the propagation of hydraulic fractures, subsequently affecting the macroscopic outcomes of HF. This study, referred to a stochastic modeling approach, from Wang et al. [40], to simulate the embedding of random mineral particles. Initially, random parameters generated according to Equation (4) were used as vertex coordinates. When the number of vertices exceeded two, the triangle areas were calculated, and the area of the mineral particles can be obtained by accumulating the results. The generation of minerals ceased when the total area reached the preset range. There may be a slight deviation between the total mineral area and the required area. It can be adjusted by scaling the overall geometric size of the mineral particles in the library to match the required area. Finally, the generated mineral particles are randomly placed according to diameter from large to small, and the mineral-embedded plane model can be realized. The specific implementation process is depicted in Figure 3.

$$\{\begin{array}{l}r=ri\times \left[1+random\left(-1,1\right)\times f_r\right]\\ \theta =\frac{2\pi }{\alpha }\times \left[\beta +random\left(-1,1\right)\times f_{\theta }\right]\end{array},$$

(4)

where r_i represents the random radius at any point P_i, and it can be adjusted according to the specific conditions. f_r and f_θ denote the radius fluctuation ratio (ranging from 0 to 1) and the angular fluctuation ratio (ranging from 0 to 0.5), respectively. Larger values of these parameters indicate greater irregularity in the minerals. α represents the number of sides or edges of the mineral particles, while β signifies the number of corners or vertices of the mineral particles. The function random ( ) is used to generate a random number within the range of (−1, 1).

2.3. Model Design

In the mineral components of granites, feldspar content generally is the highest, followed by quartz, with mica content being relatively low [41,42]. In the field of igneous petrology, granite can be classified into four categories based on grain size: coarse-grained (grain size d > 5 mm), medium-grained (d = 2–5 mm), fine-grained (d = 0.2–2.0 mm), and micro-grained (d < 0.2 mm) [15]. Based on the morphological disparities, property variations, content differences, and distribution distinctions among different minerals, this study established two-dimensional plane strain models of granites. The model plane was predominantly composed of feldspar. Quartz and mica were embedded into the plane in the form of particles. The models represented coarse-grained, medium-grained, and fine-grained granites, as well as granites with varying mineral compositions. Within each category, two different spatial arrangement methods were implemented, as depicted in Figure 4.

Table 1. Hydraulic fracturing model grouping under non-uniform mineral distribution.

Category	Mineral Types	Particle Size (mm)	Content
Coarse-grained	Quartz	6–9	25%
	Mica	5–7	5%
Medium-grained	Quartz	3–5	25%
	Mica	2–3.5	5%
Fine-grained	Quartz	1–2	25%
	Mica	0.5–1.5	5%
High quartz content	Quartz	3–5	40%
	Mica	2–3.5	5%
High mica content	Quartz	3–5	25%
	Mica	2–3.5	20%

summarizes the model classification scheme, with distinct properties assigned to the matrix (i.e., feldspar), quartz, and mica. The grain boundaries maintain the properties of the matrix. When global properties are consistent, the entire model is considered isotropic. Model parameters were set based on relevant literature [13,43,44], and they are listed in

Table 2. Hydraulic fracturing simulation parameters.

Input Parameter	Value	Input Parameter	Value
Model dimensions (mm2)	50 × 50	Porosity	0.001
Matrix elastic modulus (GPa)	60	Matrix Poisson’s ratio	0.2
Matrix permeability coefficient (m/s)	1 × 10−10	Matrix tensile strength (MPa)	10
Matrix cohesive elastic modulus (GPa)	60	Matrix leak-off coefficient (m/(Pa·s))	1 × 10−14
Quartz elastic modulus (GPa)	72	Quartz Poisson’s ratio	0.17
Quartz permeability coefficient (m/s)	1 × 10−12	Quartz tensile strength (MPa)	50
Quartz cohesive elastic modulus (GPa)	72	Quartz leak-off coefficient (m/(Pa·s))	1 × 10−15
Mica elastic modulus (GPa)	30	Mica Poisson’s ratio	0.22
Mica permeability coefficient (m/s)	1 × 10−8	Mica tensile strength (MPa)	8
Mica cohesive elastic modulus (GPa)	30	Mica leak-off coefficient (m/(Pa·s))	1 × 10−13
Fluid specific gravity (kg/m3)	9800	Fluid dynamic viscosity (Pa·s)	1 × 10−3

.

The base model was a square with a side length of 50 mm. The injection point, located at the geometric center of the model, was defined as the origin of the coordinates. The mesh element length was divided into 0.5 mm, which met the requirement of not exceeding the minimum mineral particle size and ensured calculation accuracy. Cohesive elements were embedded between matrix elements in the entire model. The complete model comprised 12,349 matrix elements and 24,498 cohesive elements. The mineral embedding process occurred after the mesh division. The same base model was used for all classification schemes to avoid the influence of different mesh distributions on crack morphology. To improve model convergence during calculations, the viscosity regularization coefficient for cohesive elements was set to 0.01. Furthermore, two cohesive elements with initial damage were defined near the injection point, following previous research [35,45,46].

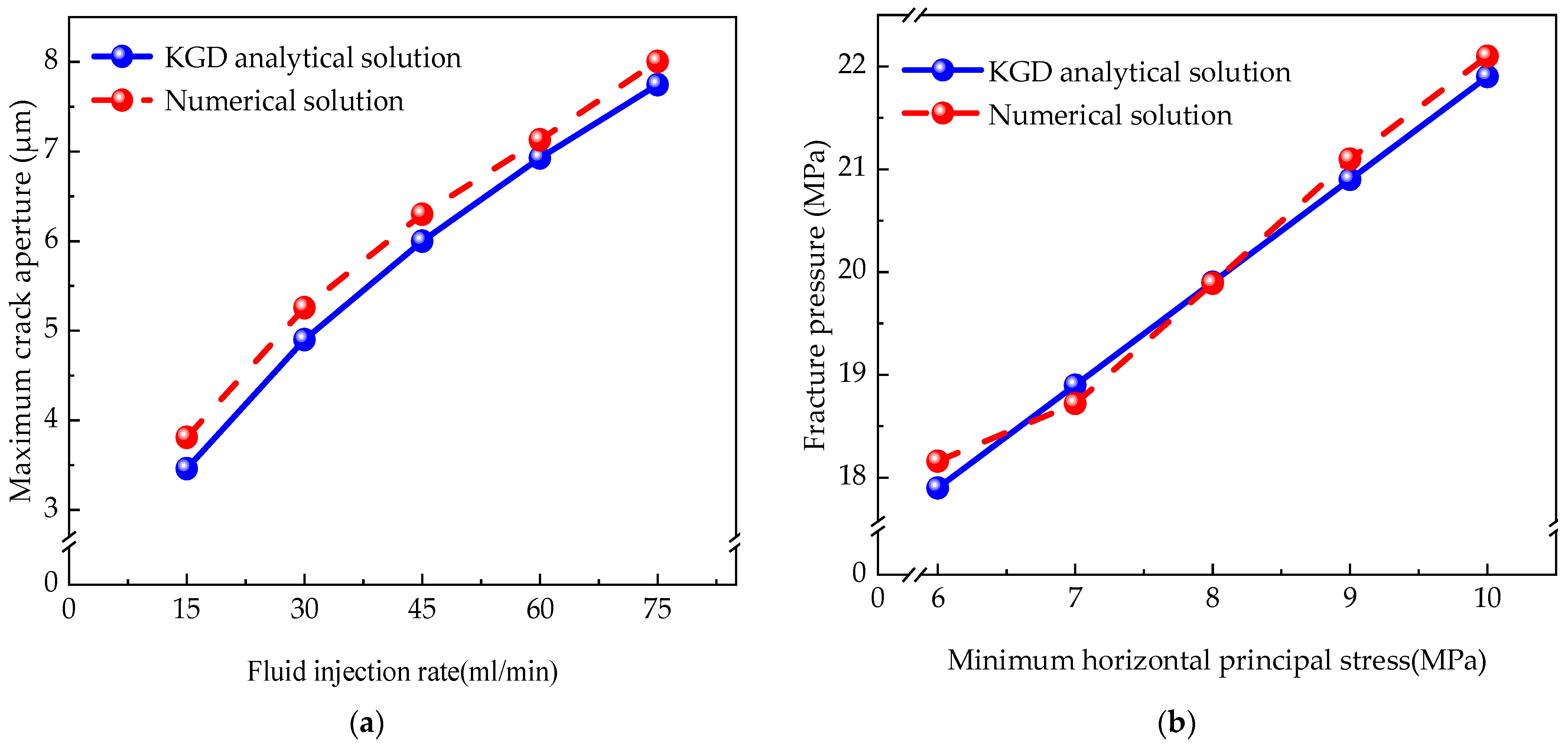
2.4. Model Validity Verification

Before investigating the influence of non-uniform mineral distribution on HF results, it was essential to ensure the validity of the model. The classical KGD (Khristianovic and Zheltov) model, first proposed in 1955, assumed that the rock mass is linear, elastic, and homogeneous. It also presumed that the flow within the fractures was laminar, simplifying the complex HF process [47,48]. Referring to previous studies [35,49,50], the 2D strain model established in this paper was compared with the results of the KGD model. In the KGD model, it was assumed that the fractures satisfied the plane strain requirements in the horizontal plane and the fracture tip was pointed, eliminating stress singularity at the fracture tip. The following approximate solution is obtained:

$$\{\begin{array}{l}{\omega }_0=2.36{\left[\frac{\left(1-{\upsilon }^2\right)\mu Q^3}{E}\right]}^{1/6}{t}^{1/3}\\ p_w={\sigma }_{\min }+1.09{\left[\frac{E^2\mu }{{\left(1-{\upsilon }^2\right)}^2}\right]}^{1/3}{t}^{-1/3}\end{array},$$

(5)

where ω₀ represents the maximum fracture aperture (MFA); p_w is the bottomhole pressure; E and υ denote the rock’s elastic modulus and Poisson’s ratio, respectively; μ represents the fracturing fluid viscosity; Q is the rate of fluid injection into the fracture; t stands for the fluid injection time; and σ_min is the minimum horizontal principal stress.

The KGD model did not account for the influence of heterogeneity on HF results. Therefore, the computational model utilized a simplified homogeneous approach, where all mineral assemblies were computed using the parameters of the matrix. According to Equation (5), it is evident that MFA is related to the IR, while the BP is influenced by the minimum horizontal stress. Horizontal stress levels of 8 MPa and 12 MPa were set to compare the differences in MFA under different IR conditions. Additionally, IR was set at 30 mL/min to compare BP variations under different horizontal stress levels. The calculation time for MFA was determined by the moment when the fracture extended to the model boundary at an IR of 75 mL/min. The corresponding bottomhole pressure value at the moment of fracturing initiation was considered the analytical solution for BP.

Figure 5 displays the comparison results, with the increase in IR, both the analytical solution and the numerical solution exhibited a gradual increase in MFA at the same time step. However, for each IR, the numerical solution was slightly greater than the analytical solution. It could be attributed to the limitations of the KGD model [34,35]. As the minimum horizontal principal stress increased, both the analytical solution and the numerical solution showed an increase in BP. It can be observed that the numerical solution fluctuated around the analytical solution. Notably, when the minimum principal stress was 8 MPa, the analytical BP was 19.90 MPa, while the numerical BP was 19.88 MPa, demonstrating a close alignment between the two. The above results showed that the overall trend of the simulation results was consistent with the KGD model results, and the numerical agreement was high. Therefore, this numerical simulation method was feasible.

3. Numerical Results and Analysis

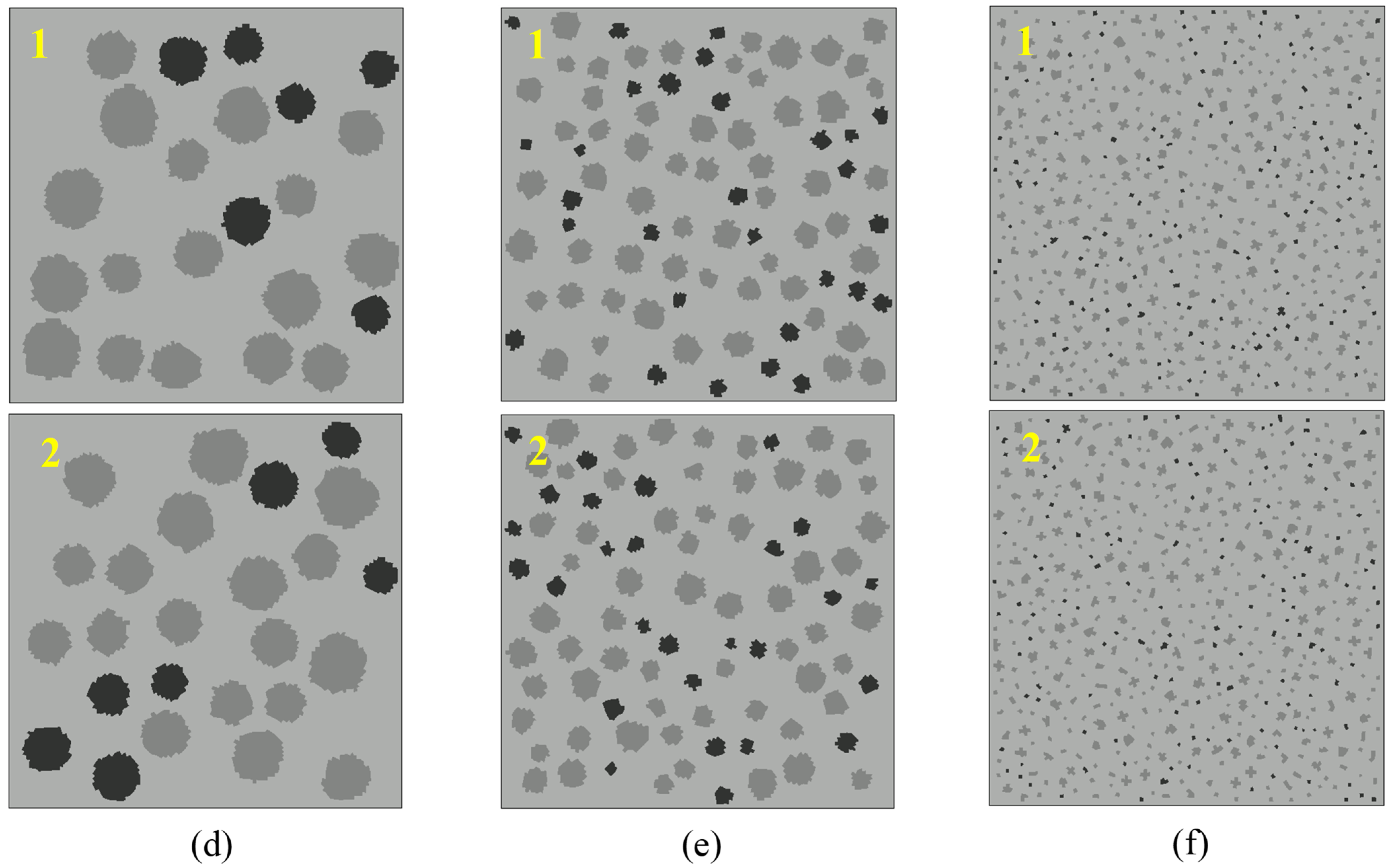
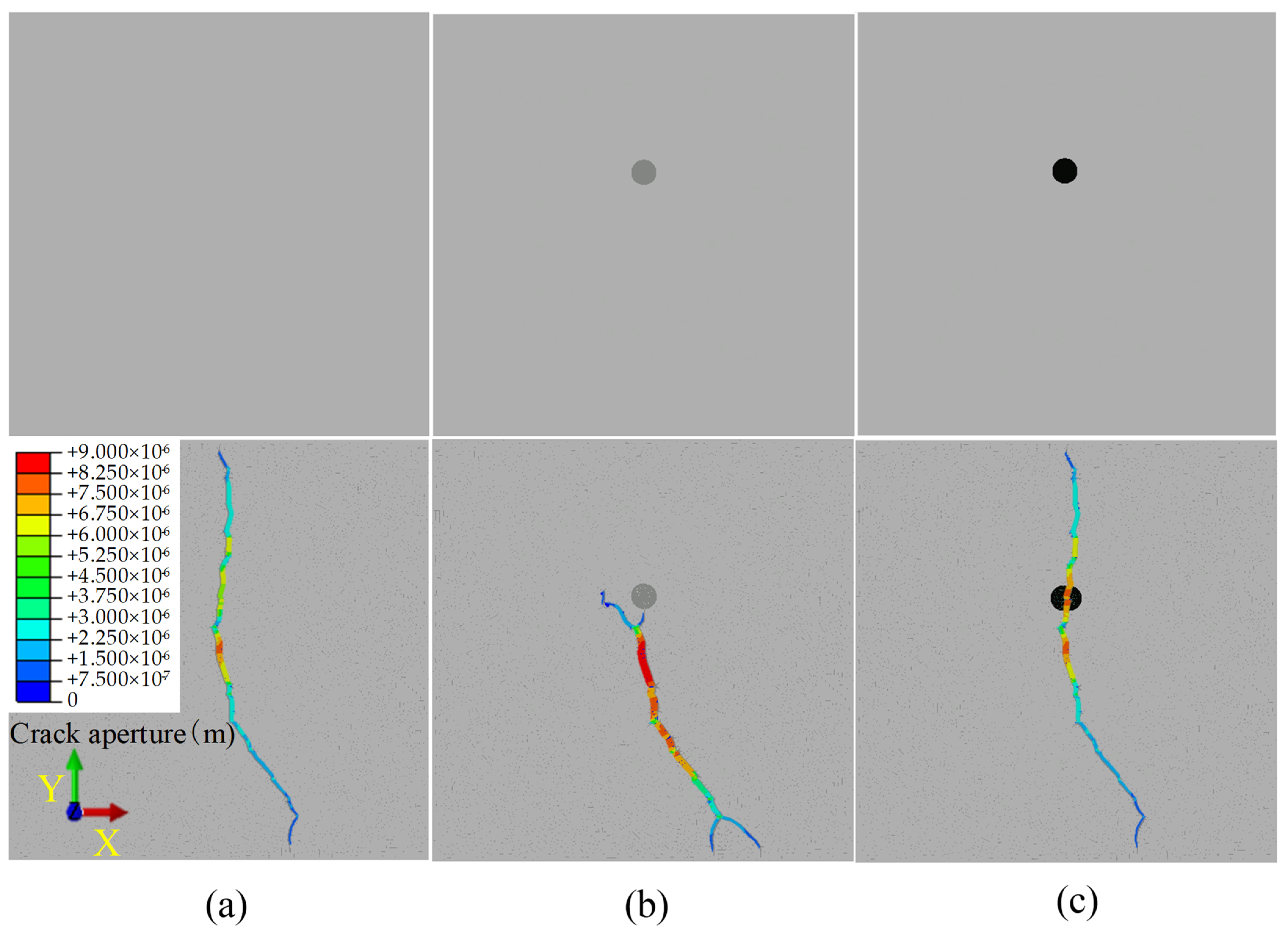
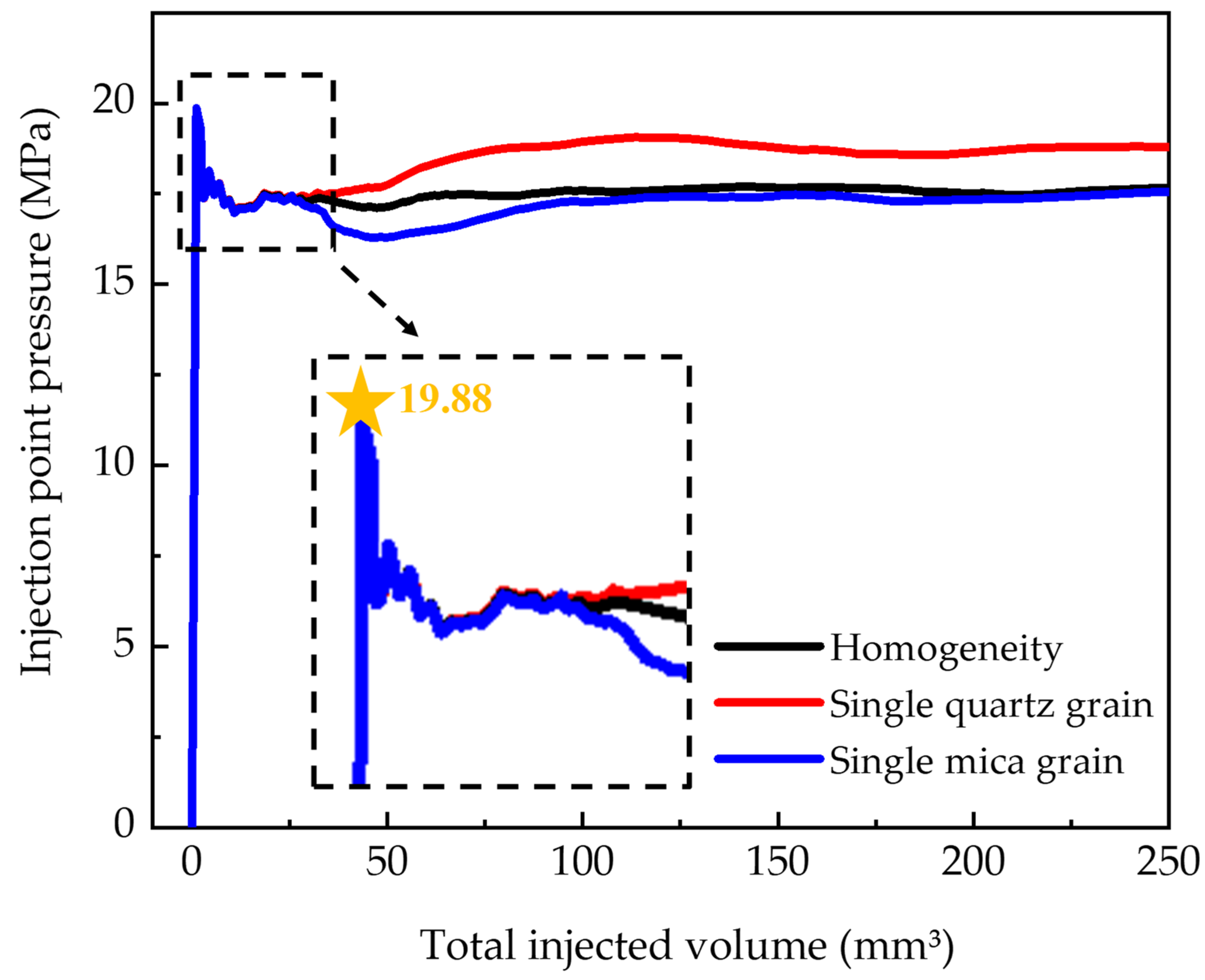
3.1. The Influence of Single Mineral

To visually compare the influence of quartz and mica particles on HF performance, it is necessary to analyze the fracturing models with the insertion of individual mineral particles. Previous studies have indicated a tendency for hydraulic fractures to propagate along the direction of maximum principal stress [51,52,53]. Inserting a circular block along the path of the maximum horizontal principal stress allowed for the simulation of individual mineral embedding. Subsequently, modifications to the properties of both the block’s solid elements and the related cohesive elements were implemented. When the properties of the block’s internal elements matched those of the matrix, the model exhibited homogeneous isotropy. Additionally, based on the comparative results in Section 2.4, horizontal stresses X and Y were set to 8 and 12 MPa, respectively. The IR was set to 30 mL/min, while other parameters were set according to Section 2.2. Different block-included plane strain models and the resulting fracture morphology are illustrated in Figure 6. In this figure, the gray block represents a quartz particle, the black block represents a mica particle, and the model without a displayed block is considered isotropic.

Based on the various fracture patterns observed, it was evident that under the influence of horizontal stress, fractures meandered in their propagation along the Y-axis. The fractures closest to the injection point displayed the largest apertures. The fracture aperture diminished progressively along the expansion path of hydraulic fractures, with the injection point serving as the center. In the initial stages, hydraulic fractures developed along similar trajectories under a geostress field. However, as fractures extended toward the vicinity of the inserted blocks, differences in fracture paths began to emerge due to the influence of different mineral properties.

Compared to the homogeneous model (Figure 6a), the presence of quartz particles hindered the original developing trajectory. Faced with higher tensile strength and lower permeability, the existing fluid pressure encountered challenges in inducing further fracturing. As fluid accumulated in the vicinity of quartz, the fluid pressure within the fractures substantially increased, and damage continued to accumulate [54], leading to a notable enlargement of the existing fracture apertures. When the fracture aperture reached a certain threshold without penetrating the quartz particles, primarily influenced by the maximum horizontal principal stress, the fracture path underwent alteration. The fluid chose a path with lower energy requirements for further fracturing [55], resulting in the branching and reduction in the overall length of the hydraulic fractures (Figure 6b). When hydraulic fractures approached mica, the weaker mineral mica was directly penetrated by the fluid (Figure 6c). Although the overall hydraulic fracture path remained consistent with the homogeneous model, the apertures of fractures that traversed the mica were significantly larger than those in the homogeneous model. In summary, quartz had a substantial impact on fracture morphology. When fractures extended toward hard-phase minerals, they preferred propagating along mineral boundaries, in alignment with previous research findings [56]. Different spatial arrangements of quartz may have led to distinct fracture paths and aperture distributions. Conversely, mica had a relatively minor influence on hydraulic fracture paths but could alter the distribution of fracture apertures.

Figure 7 displays the trend of fluid pressure at the injection point for three different HF models as a function of injected fluid volume. As injection commenced, fluid accumulated at the injection point, causing a rapid increase in fluid pressure. When the stress component reached the tensile strength of the cohesive elements, the model underwent fracturing. Hereafter, the concentrated fluid rapidly filled the fracture, leading to the dissipation of fluid pressure. After a certain degree of pressure drop and fluctuations, fluid pressure stabilized at a particular level, thereby providing the driving force for the extension and propagation of fractures. The highest point of the curve was marked with a five-pointed star, indicating the BP, while the stable pressure indicated its extension pressure. Due to the limited scope of influence of minerals, there was no significant variation in BP among the different models, all of which remained at around 19.88 MPa. However, during the process of fracture extension, there were differences in the extension pressures among the various models. In the model embedded with quartz particles, after the microcrack had developed stably for a period of time, the extension pressure began to rise. This higher level of extension pressure was subsequently maintained for fracture propagation. In the model embedded with mica particles, the early extension pressure showed no significant changes. When the cracks approached the mica, the extension pressure exhibited a decreasing trend and returned to the original extension pressure level after passing through the mica. In the homogeneous model, the extension pressure fluctuated less, with values falling between the two former scenarios, remaining at around 17.50 MPa.

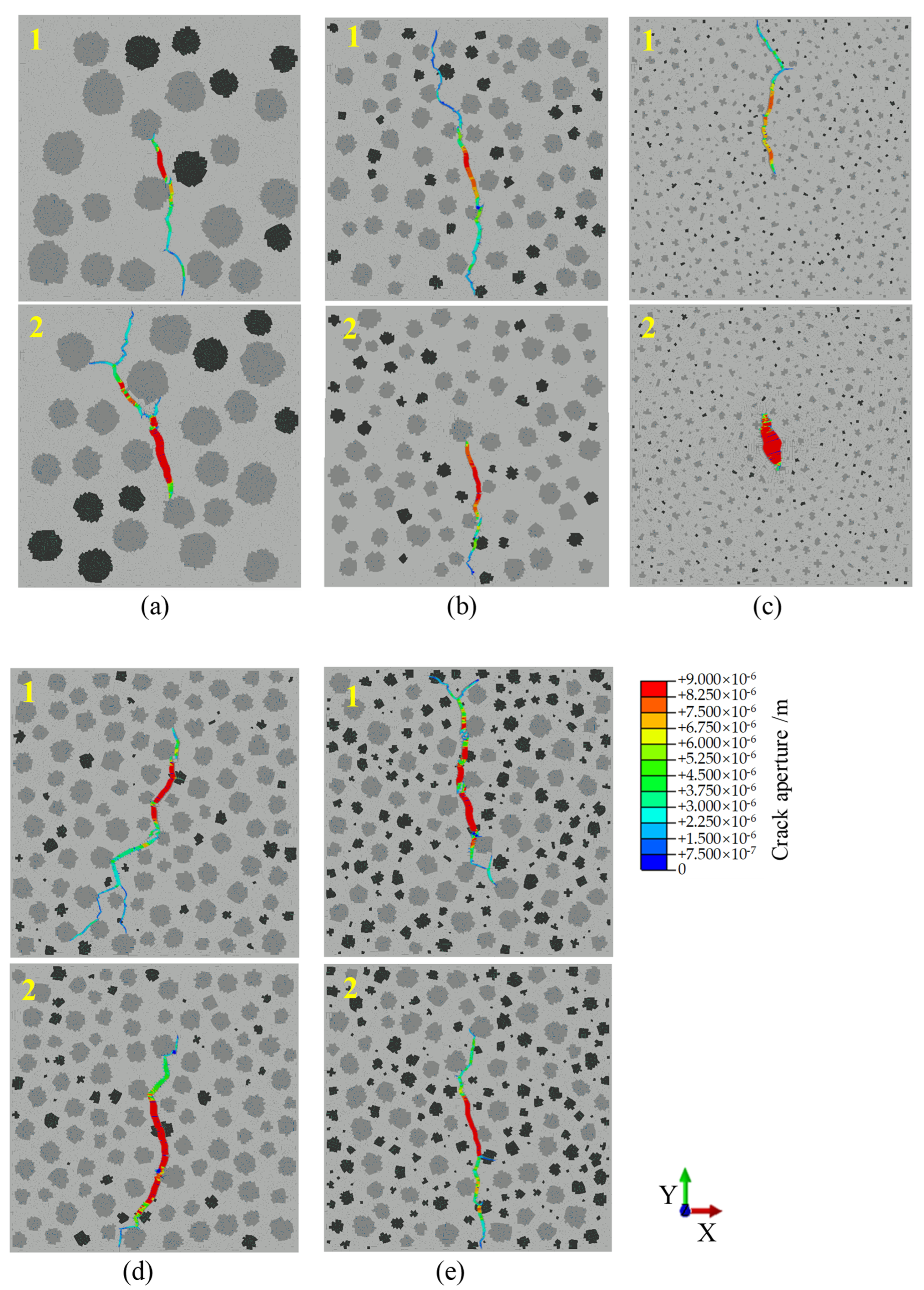
3.2. Effects of Particle Size, Composition, and Spatial Arrangement under Mineral Mixing

3.2.1. Fracture Morphology

Through the HF simulations under the influence of single quartz and mica particles, it was evident that the fracturing outcomes of single mineral embedding exhibited disparities when compared to the fracturing results achieved under homogeneous conditions. Furthermore, granite, comprising a mixture of various minerals, is profoundly influenced by the complex interplay of these minerals throughout each phase of fracture propagation [57]. Following the procedure outlined in Section 2.3, quartz and mica were embedded into the planar model, with model parameters consistent with those in Section 3.1. Numerical calculations were performed to obtain hydraulic fracture patterns under different conditions, as shown in Figure 8.

Under the influence of non-uniform mineral distribution, significant differences were observed in the fracture patterns of each model. The paths of fracture propagation and the distribution of fracture apertures consistently evolved in response to the varying mineral distribution. In the coarse-grain model, the larger particle size became an obstacle along the fracture propagation path. As quartz could alter the fracture’s direction and was influenced by the presence of large particles, the deflection path along the particle became longer. However, even after changing direction, the hydraulic fracture remained affected by nearby quartz particles. This made the smooth extension challenging, significantly weakening the dominant role of HSD in fracture propagation. Due to different quartz distribution regions, in Model 8(a)-1, the fractures mainly extended along the -Y direction without producing branching fractures. In Model 8(a)-2, most of the fractures extended along the +Y direction, with complex paths hindering the rapid dissipation of fluid pressure, consequently leading to increased fracture widths. In the medium-grain model, the overall width of the fractures decreased while the length increased. It was evident that fractures had more extension path options at this particle size, allowing them to extend more fully. Influenced by quartz distribution and horizontal stress, the fractures continuously changed direction, ultimately presenting a tortuous fracture pattern. In Model 8(b)-2, because quartz particles were positioned near the center of the extension path, fractures had difficulty making significant angular deviations in the +Y direction, leading to concentrated fractures extending in the -Y direction. In the fine-grain model, there was a higher probability of encountering the central area of quartz particles during the fracture propagation process. When the provided hydraulic pressure could not support significant angular deviations of fractures, fracture propagation terminated, even leading to the extreme case observed in Model 8(c)-2. In this scenario, the fracture propagation path was surrounded by tiny quartz particles. The optimal expansion path had not been found during the fluid accumulation process, and the fluid pressure was not enough to penetrate the quartz, resulting in an abnormal increase in fracture width.

In the medium-grain model, with a constant mica content of 5%, increasing the quartz content to 40% led to a noticeable increase in fracture complexity. Meandering fractures with accompanying branching fractures were observed, and the fracture tips underwent significant angular deflections due to the influence of quartz. The distribution range of wide fractures expanded, but instances of insufficient fracture extension persisted. The mica on the expansion path was directly penetrated, posing no hindrance to fracture propagation. The phenomenon was consistent with the phenomenon in Section 3.1. When maintaining a quartz content of 25% and increasing the mica content to 20%, numerous mica particles were present along the fracture path. Because less energy was required to fracture mica, fractures tended to approach mica [14], and even short secondary fractures penetrating the surrounding mica were observed.

3.2.2. Maximum Fracture Aperture

The evolution of MFA during the fracture propagation process in each fracturing model is illustrated in Figure 9. Overall, the rate of fracture growth gradually decreased, consistent with the results from traditional theoretical models [34,35]. Due to the influence of mineral grain size, composition, and spatial arrangement, there were some variations in the MFA curves under different conditions with increasing injection volumes. Notably, the influence of mineral spatial arrangement resulted in varying trends in MFA for fractures with the same grain size and composition. This phenomenon was particularly pronounced in the fine-grain model. As mineral grain size increased, the overall MEA gradually increased. It was observed that in the case of larger grain sizes, hydraulic fractures in coarse-grained granite were affected by the extended range of influence from quartz. This caused micro-fractures in coarse-grained granite to have difficulties extending smoothly along the direction of maximum horizontal stress. They could only develop further when fluid pressure reached a certain range, leading to an overall increase in fracture width. However, the different contents of quartz and mica particles in the composition had a relatively minor influence on the development of MFA. Combining these findings with the results of fracture morphology, it became clear that in the fine-grain Model 2, fractures encountered difficulties in maintaining sustained propagation. As a result, the curve for MFA was notably distinct from the others. In this case, it consistently maintained a higher growth rate. When quartz appeared in the fracture propagation path, during the fluid accumulation phase, the increase in fluid pressure led to a sustained high-rate increase in MFA. When fractures changed direction or bifurcated, fluid pressure dissipated, resulting in a brief decline in MFA, followed by a low-rate increase, as observed in the curves labeled L2 and Y1 in Figure 9.

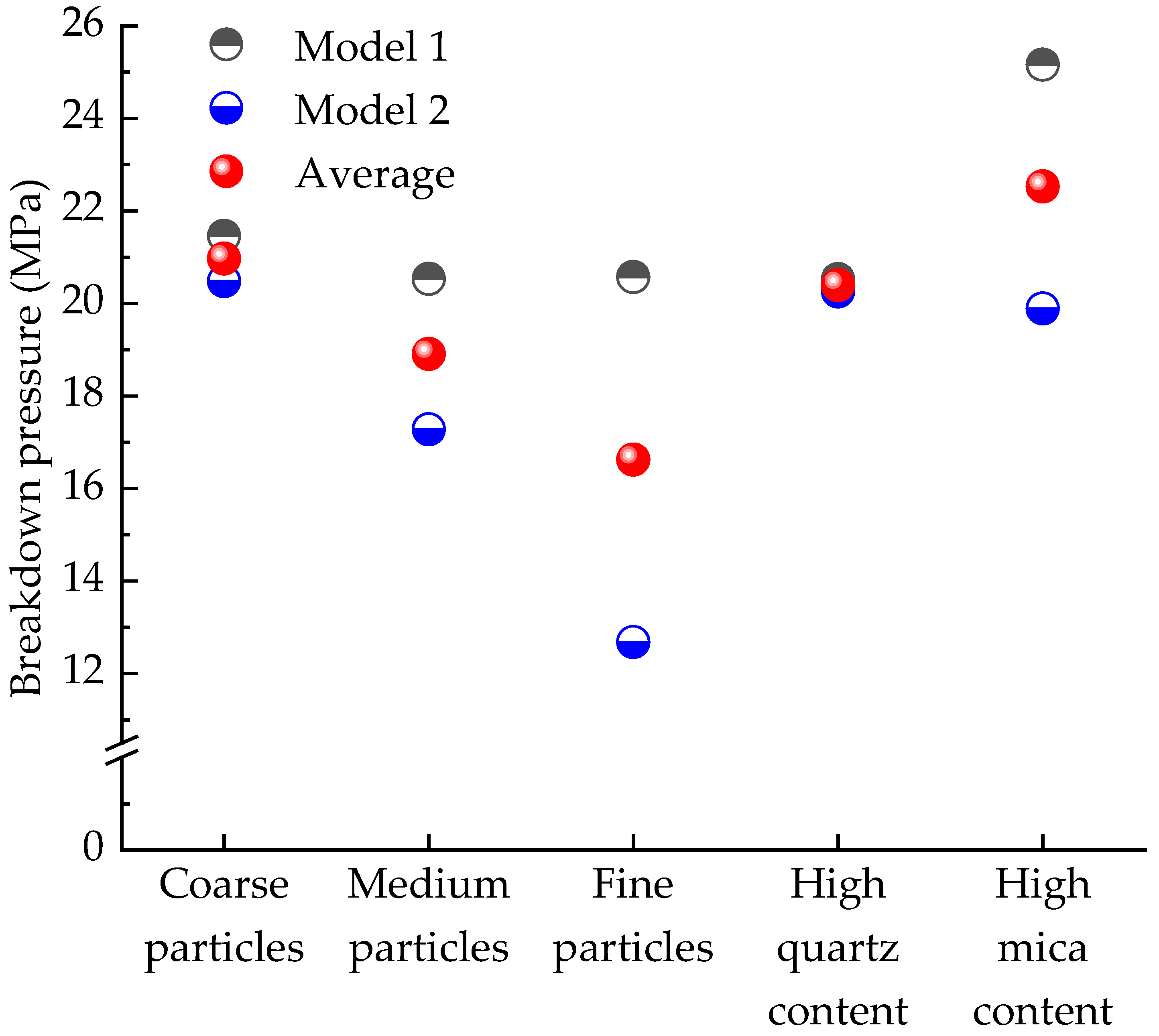
3.2.3. Breakdown Pressure

The extracted BP from the fluid pressure curves in each fracturing model is depicted in Figure 10. Due to the influence of non-uniform mineral distribution, the BPs in the models exhibited some fluctuations within a certain range. Notably, as mineral grain size decreased, the BP in the HF simulations gradually decreased. The extent of this change was associated with the spatial arrangement of minerals. When mineral grain size was relatively large, the models exhibited lower dispersion of BP under different spatial arrangements of minerals. However, as mineral grain size decreased, the effect of mineral spatial arrangement on BP became more pronounced. This phenomenon can be explained by the larger range of influence of larger mineral grain sizes in the rock, which affected fracturing near the injection point regardless of the distribution of minerals in the model plane. In contrast, smaller mineral grain sizes had a more limited range of influence, and the distribution of minerals near the injection point directly impacted the model’s BP. An analysis of the BP in the medium-grain model, high quartz content model, and high mica content model, it was evident that, in contrast to the mixed mineral content, the spatial arrangement exerted a more substantial influence on the BP. The presence of a higher content of quartz near the injection point directly increased BP.

Through a comprehensive analysis of the fracture morphology, MFA evolution, and BP in HF models with different mineral grain sizes, compositions, and spatial arrangements, it was observed that the bilateral hydraulic fractures did not propagate synchronously. Among these factors, the spatial arrangement of minerals had the most pronounced influence on fracture morphology, fracture width, and BP. Differences in mineral grain size and composition could affect the probability of fracture deflection events, resulting in certain regularities in the outcomes. HF results are closely related to working conditions [58]. Considering both the extent of fracture propagation and the range of BP fluctuations, medium-grain model 1 was selected as the reference model. Further investigations were conducted to explore the impact of different core fracturing parameters on the fracturing outcomes under this mineral distribution pattern.

4. Sensitivity Analysis of Fracturing Parameters for Heterogeneous Granite

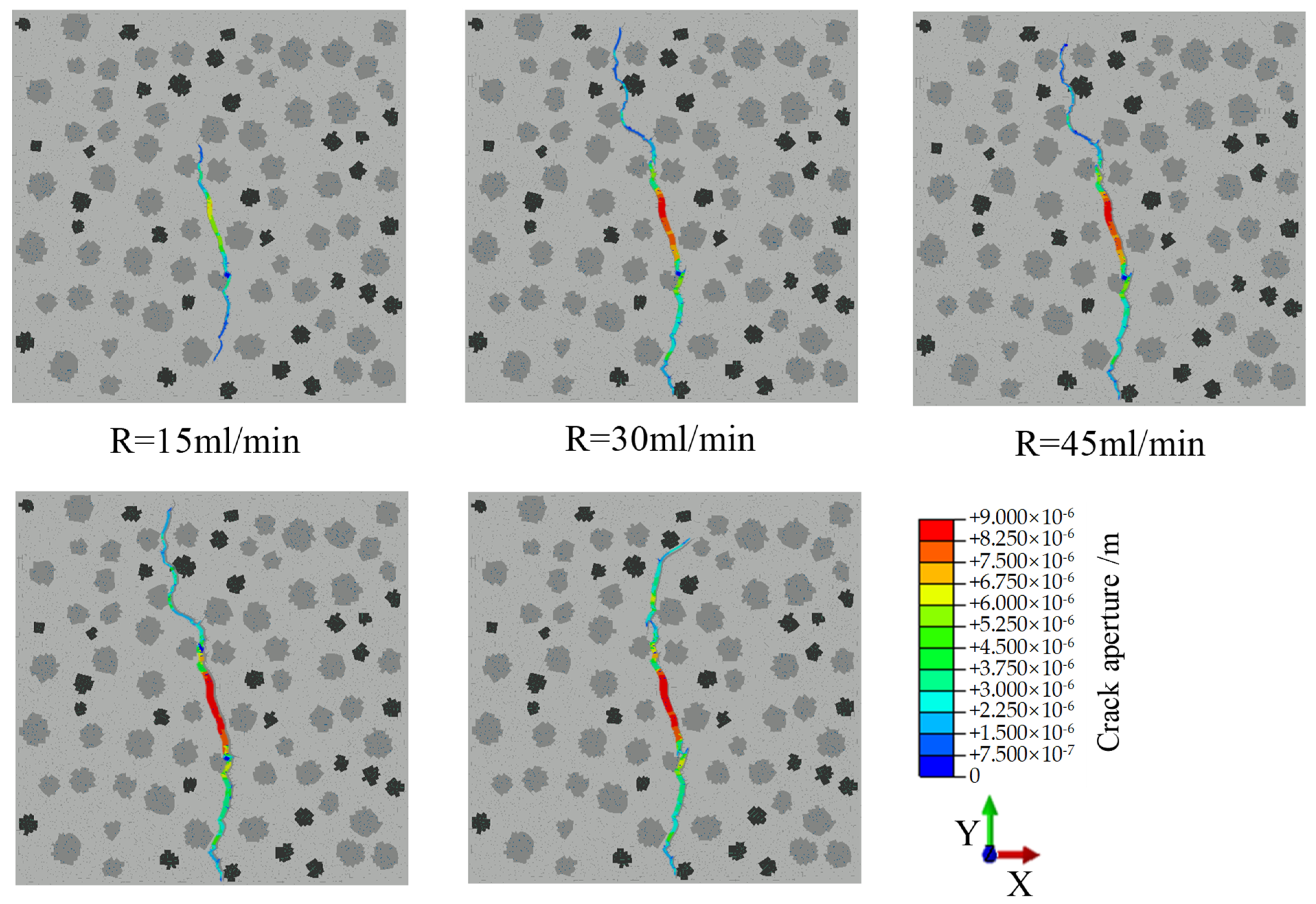
4.1. Injection Rate

Laboratory HF experiments are typically conducted under constant IR, and a correlation between BP and IR has been observed in these experiments [59,60]. To further investigate the role played by mineral particles in this process, based on medium-grain model 1, this section set IR values at 15 mL/min, 30 mL/min, 45 mL/min, 60 mL/min, and 75 mL/min, while keeping all other parameters consistent with those in Table 2.

Figure 11 displays the variations in fracture morphology under different IR conditions. Different IR values had a certain impact on hydraulic fracture width and propagation paths. When the IR was 15 mL/min, the lower fluid flow rate made it difficult to penetrate the model, resulting in a relatively smaller overall fracture width. With an IR of 30 mL/min, hydraulic fractures had already extended fully to the model boundaries, and the overall fracture width increased significantly. As the IR further increased, the hydraulic fracture propagation paths remained relatively unchanged, but the overall fracture width continued to grow. At the IR of 75 mL/min, while hydraulic fractures maintained a considerable width, there was a redirection at the +Y end. Influenced by the stress field distribution around the quartz, the higher fluid flow rate caused the fracture to undergo a large-angle deviation when obstructed in its expansion. Subsequently, it continued to develop away from the quartz.

Figure 12 depicts the trends in BP and MEA of the HF model at different IRs. As the IR increases, the BP of HF exhibits a continuous ascent. Specifically, the BP showed a significant increase when the injection rate exceeded 45 mL/min. The MFA was significantly influenced by mineral distribution but did not exhibit a consistent trend with increasing IR. The MFA at IRs of 15 mL/min, 30 mL/min, 45 mL/min, 60 mL/min, and 70 mL/min was 6.9 μm, 9.21 μm, 9.22 μm, 10.20 μm, and 10.22 μm, respectively. Notably, there were no substantial changes in MFA when the IR increased from 30 mL/min to 45 mL/min or from 60 mL/min to 75 mL/min. In combination with the analysis of fracture morphology in Figure 11, it became evident that once the hydraulic fracture had penetrated the model, minor fluctuations in IR lacked the capacity to bring about substantial alterations in fracture morphology. However, as the IR continued to increase, this state was disrupted. The MFA and BP continued to increase, ultimately resulting in noticeable changes in fracture morphology.

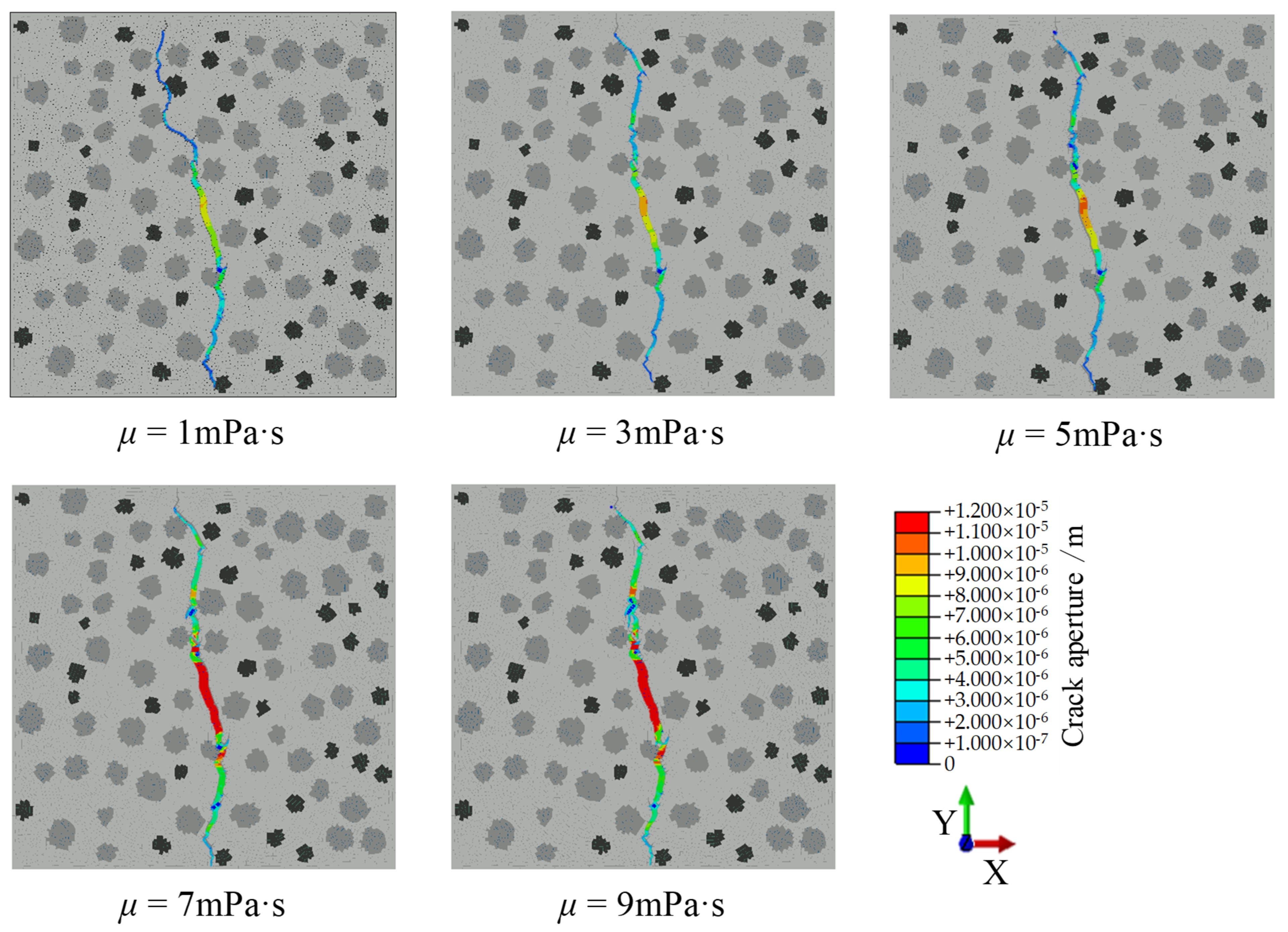
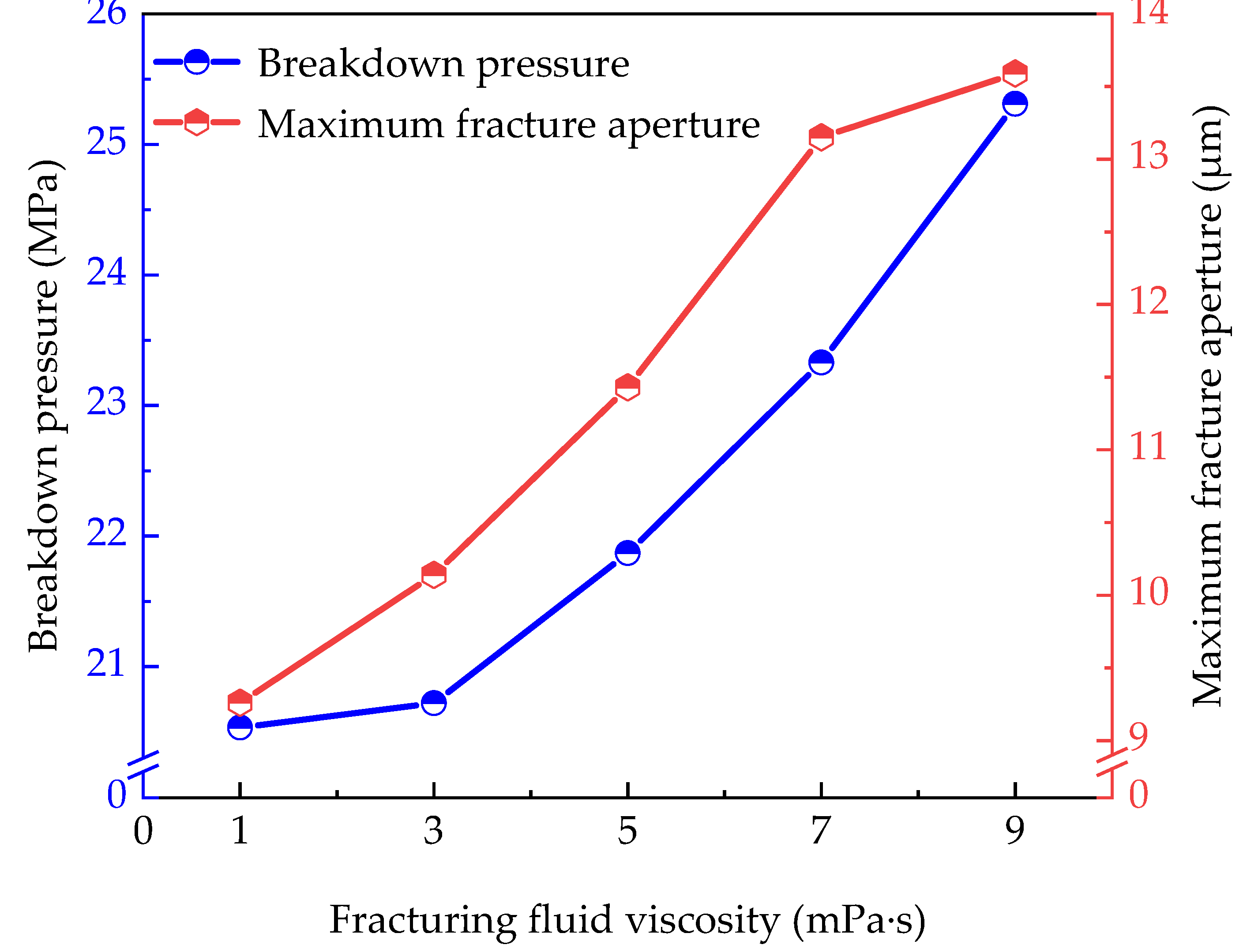
4.2. Fracturing Fluid Viscosity

FFV frequently plays a pivotal role in reservoir stimulation. Excessively low viscosity can make it challenging to create stable fractures, while excessively high viscosity can hinder fluid flow and even fail to propagate the tip of the fracture [61]. The objective of this section was to explore the influence of different FFVs in heterogeneous granite. The viscosities were specifically set at 1 mPa·s, 3 mPa·s, 5 mPa·s, 7 mPa·s, and 9 mPa·s. Simultaneously, a constant fracturing fluid flow rate of 30 mL/min was maintained. All other parameters remained identical to those mentioned earlier.

Under different FFVs, the hydraulic fracture morphology is depicted in Figure 13. When the FFV increased from 1 mPa·s to 3 mPa·s, the hydraulic fracture propagation path along the +Y-axis tended to become more linear. This change was accompanied by a slight increase in the crack width. However, the phenomenon of hydraulic fractures turning did not occur under these conditions. With further increases in the fracturing fluid viscosity, the hydraulic fracture propagation path remained consistent. The higher FFV led to the formation of a stronger liquid column, significantly increasing the fracture width. This trend aligns with the findings of Cong et al. [62]. Figure 14 illustrates the trends in BP and MFA under different FFVs. As FFV increased, both BP and MFA gradually increased. BP increased from 20.53 MPa to 25.31 MPa, while MFA increased from 9.26 μm to 13.59 μm. Notably, the change in FFV from 1 mPa·s to 3 mPa·s did not significantly elevate the fracturing BP, but it substantially increased MFA. Considering different fracture morphologies, one can infer the existence of an optimal viscosity within this range that maintains a relatively low BP, while ensuring complex growth of hydraulic fractures and larger fracture apertures. As FFV continued to increase, the MFA increased, but the BP also rose. Simultaneously, the fracture morphology remained relatively unchanged. This scenario is less conducive to efficient operations.

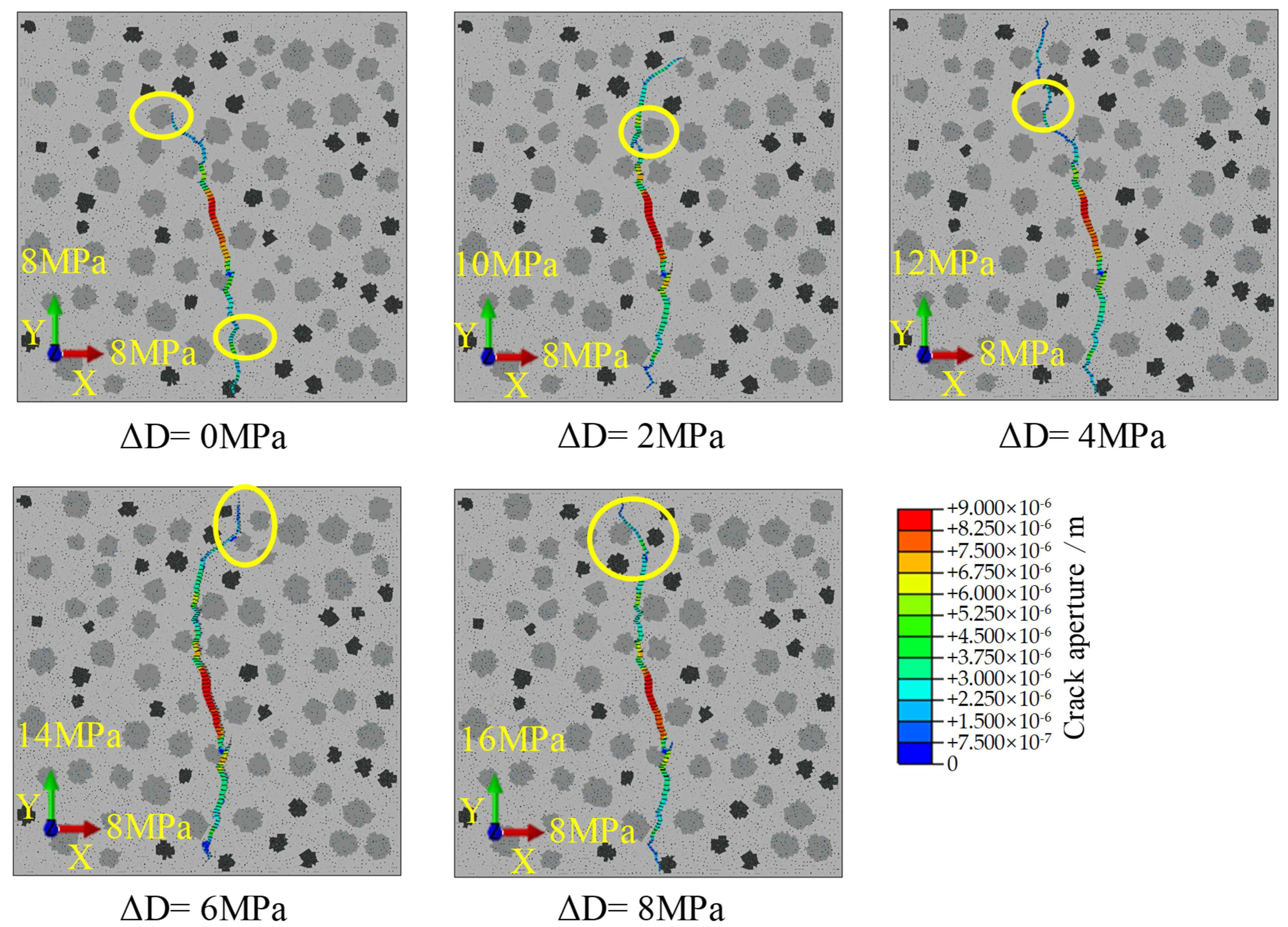
4.3. Horizontal Stress Difference

HSD directly influences the propagation path of fractures, with hydraulic fractures typically extending along the direction of maximum horizontal principal stress [51,52,53]. However, the presence of minerals can obstruct this propagation trend, causing deviations in the fracture path. To investigate the impact of minerals on fracturing under different HSD conditions, in this section, the HSDs were set to 0 MPa, 2 MPa, 4 MPa, 6 MPa, and 8 MPa, respectively. These HSDs were achieved by adjusting the maximum horizontal principal stress. The IR was kept at 30 mL/min, the FFV was maintained at 1 mPa·s, and other parameters remained the same as above.

The hydraulic fracture propagation and morphology under different HSDs are illustrated in Figure 15. The coupling effects of HSDs and non-uniform mineral distribution resulted in variations in the far-wellbore fracture morphology. When the HSD was 0 MPa, the fracture propagation was not driven by the stress difference. Under the influence of minerals, it propagated freely with a certain degree of tortuosity and eventually terminated at the edge of quartz particles in the far field. When the HSD increased to 2 MPa, at the same location, hydraulic fractures did not change their direction but propagated straight along the original path. However, a horizontal expansion trend appeared at the end of the +Y direction. When the HSD was 4 MPa, the influence of HSD became apparent as the fractures significantly deviated toward the direction of maximum principal stress upon bypassing the quartz. At an HSD of 6 MPa, the early-stage fracture propagation path remained consistent with that observed at the HSD of 2 MPa. However, under the influence of a larger HSD, there was a straight fracture path extending toward the +Y direction at the distal end. Further increasing the HSD to 8 MPa, a decrease in the overall tortuosity of the fracture was observed. Moreover, it could still maintain radial expansion after bypassing the quartz. Additionally, the region near the injection point maintained a larger fracture aperture. However, the aperture did not exhibit a consistent trend with changes in HSD due to the influence of fracture propagation paths. Figure 16 illustrates the trends in the model’s BP and MFA under different HSDs. With increasing HSD, BP decreased from 25.16 MPa to 17.47 MPa. This trend is consistent with previous experimental findings [23,63]. The MFA was influenced by fracture morphology, where MFA was slightly larger under the 2 MPa and 6 MPa HSD compared to other conditions.

5. Conclusions

In this paper, a two-dimensional granite model with non-uniform minerals was established, conducting HF to compare the failure behavior on different mineral distributions, and discussing the sensitivity of heterogeneous granite to the changes in key fracturing parameters. The following conclusions have been drawn:

(1)

The existence of hard mineral—quartz, in the crack propagation path, changes the direction of the crack and easily results in branch cracks. On the contrary, the crack can directly penetrate the weak mineral—mica. In addition, at the crack tip, the crack bypassing quartz tends to increase the extension pressure, while the crack passing through mica generates a lower extension pressure.

(2)

Coarse-grained granite has the widest range of crack deflection, while medium-grained granite is easier to lengthen and warp cracks. The failure result of fine-grained granite is determined by the spatial arrangement of minerals. Furthermore, the fractures in granite with a high content of quartz are more complex, and the hydraulic fractures in rock with a high content of mica clearly expand toward mica.

(3)

In granite with a non-uniform distribution of minerals, BP and MFA increase versus the IR and FFV increase. The morphology of hydraulic fractures is significantly influenced by the HSD. When cracks bypass quartz during their propagation, driven by high stress differences, they clearly expand in the direction of the maximum principal stress. In contrast, under low stress differences, cracks tend to expand laterally.