Battery Hybrid Energy Storage Systems for Full-Electric Marine Applications
Abstract
:1. Introduction
2. Target Ship and Requirements
- The battery system is integrated into the vessel through a DC link with a fixed voltage of 1000 V.
- The battery cells operate within the state of charge (SOC) of 90–10%. In other words, the maximum depth of discharge (DOD) is 80%.
- The battery system has to fulfill 10 years of operation.
3. Baseline Battery System and HESS Topologies
4. Specifications of Battery Cells and DC/DC Converter
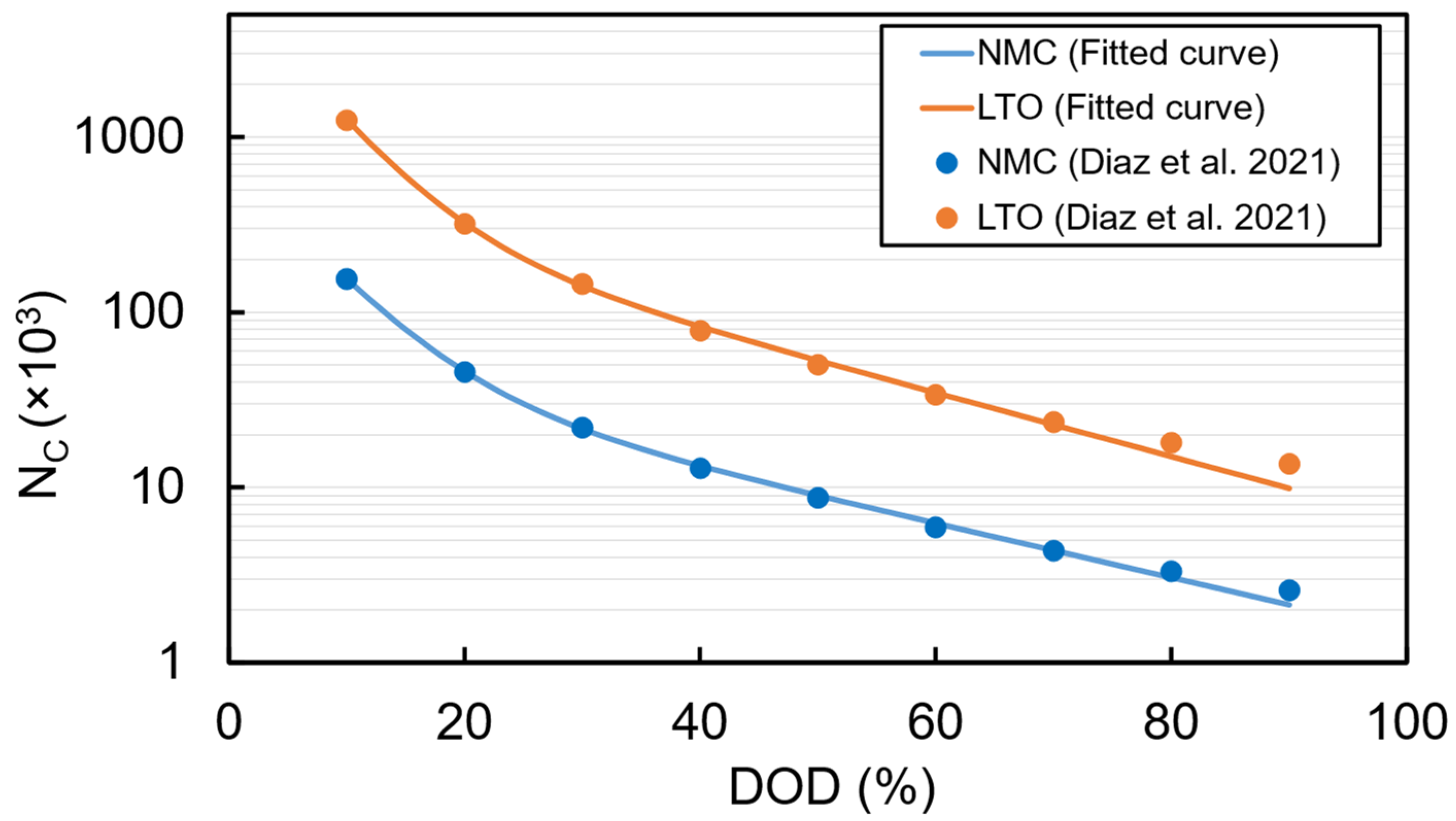
5. Battery Lifetime Model
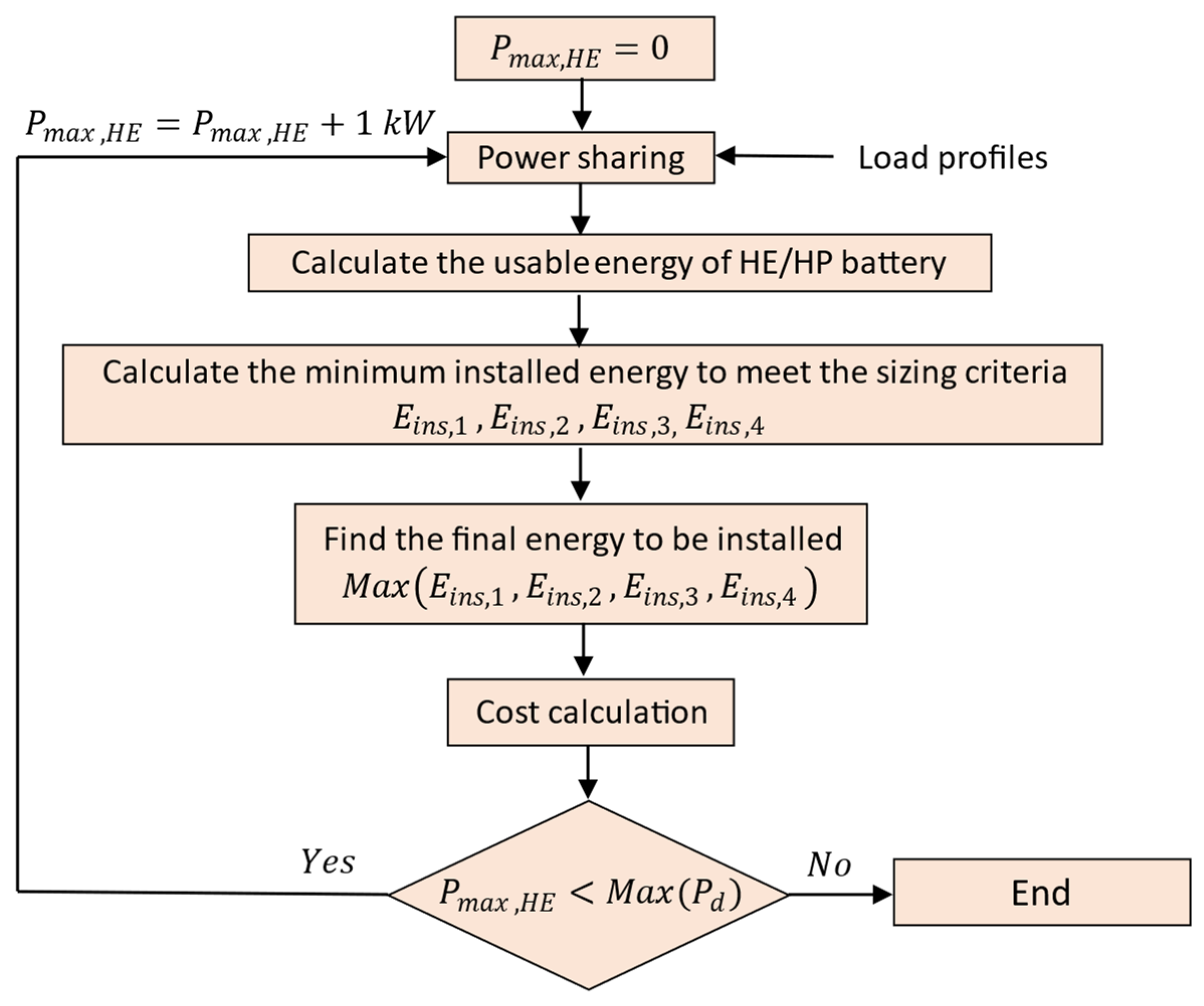
6. Sizing Methodology
6.1. Energy Management Strategy
6.2. Sizing Flowchart
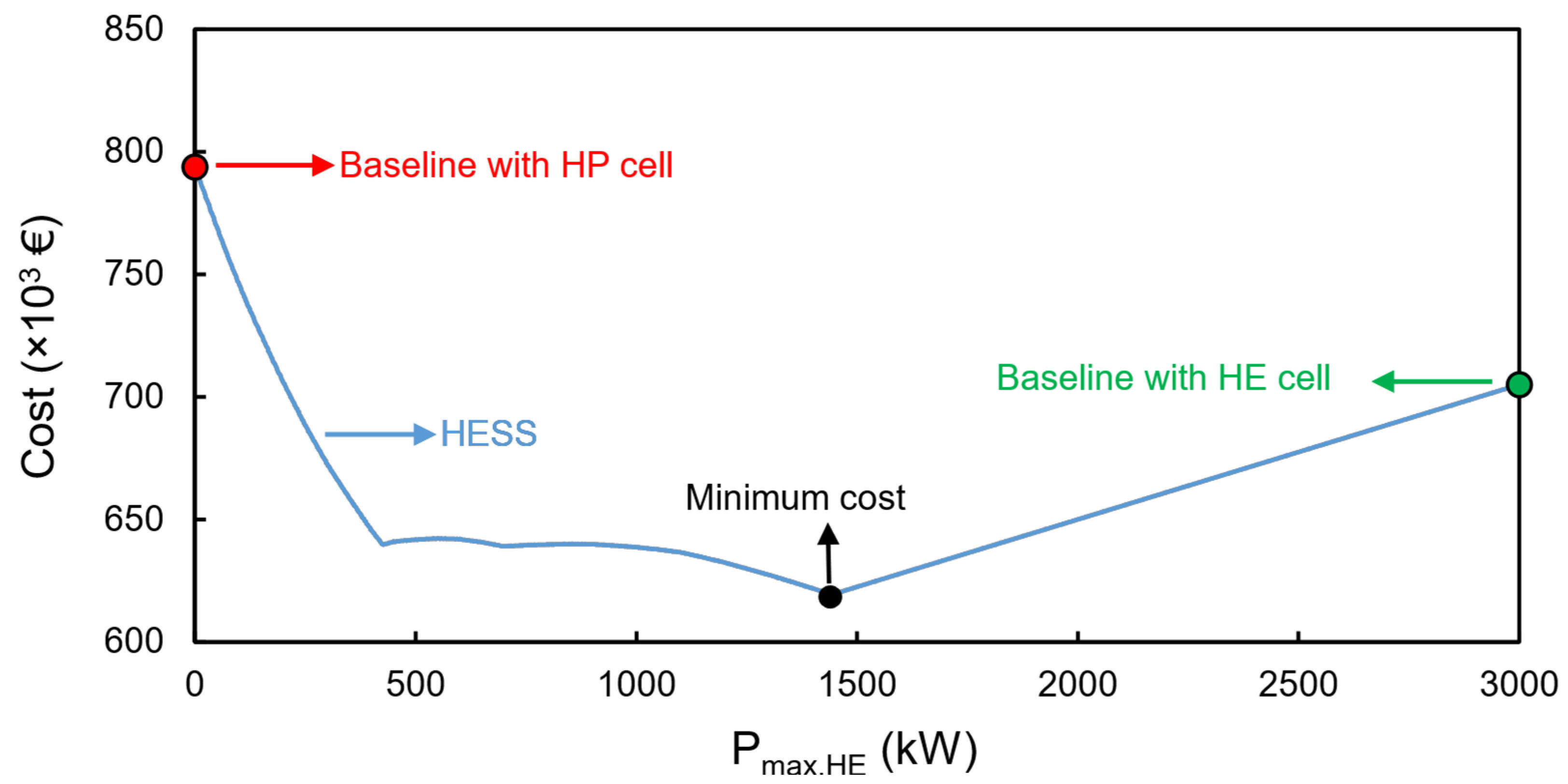
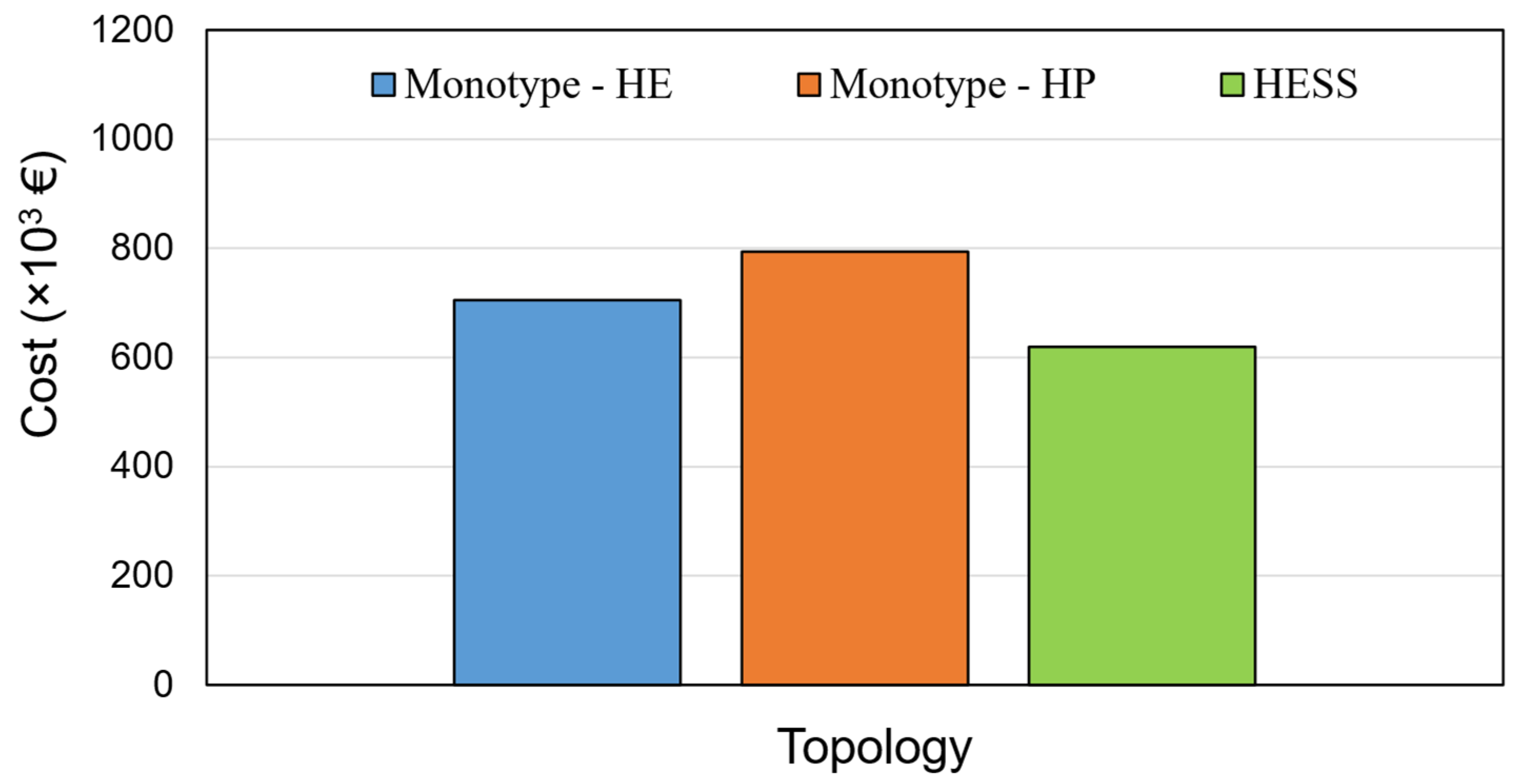
7. Results and Discussion
7.1. Battery Cost Analysis
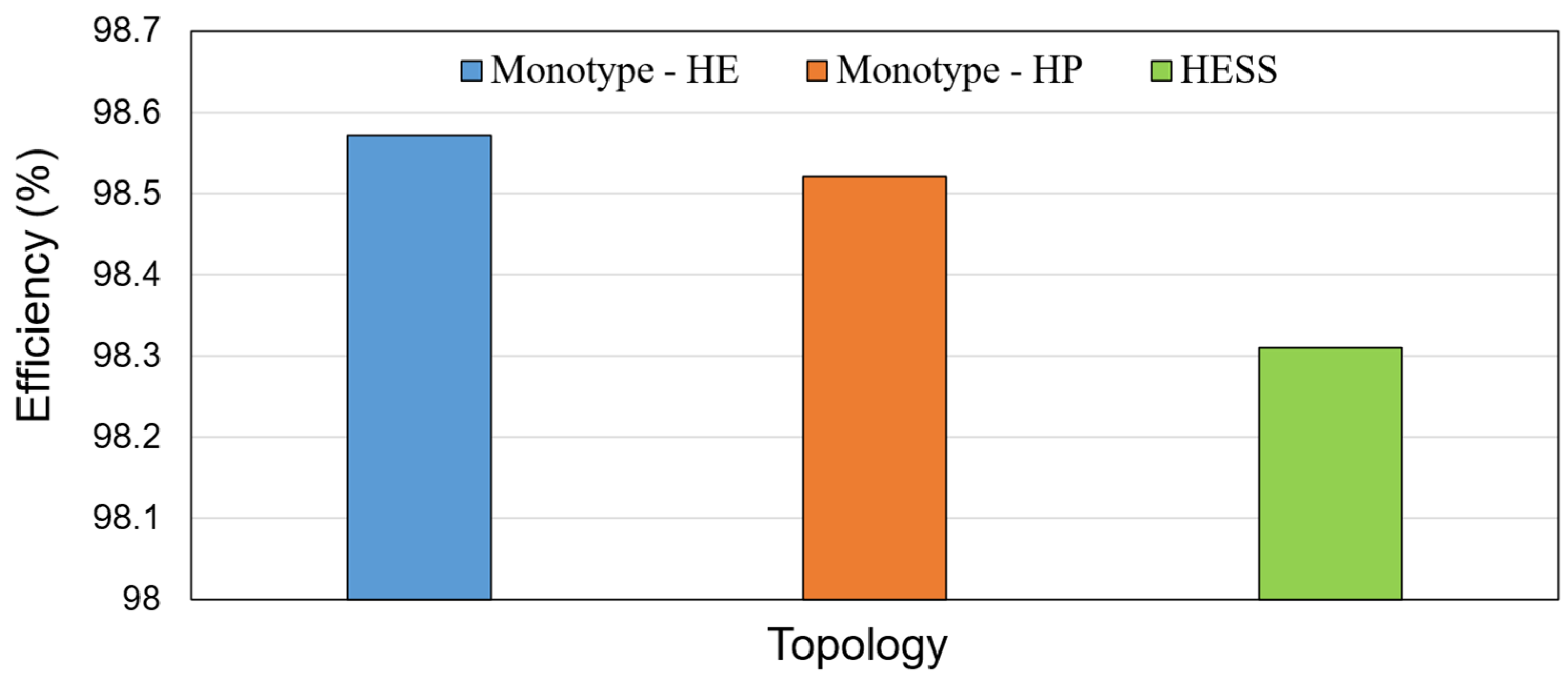
7.2. Battery System Losses
7.3. Battery Weight
8. Conclusions
Author Contributions
Funding

Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Enweremadu, C.; Samuel, O.; Rutto, H. Experimental Studies and Theoretical Modelling of Diesel Engine Running on Biodiesels from South African Sunflower and Canola Oils. Environ. Clim. Technol. 2022, 26, 630–647. [Google Scholar] [CrossRef]
- Elumalai, P.V.; Moorthy, R.K.; Parthasarathy, M.; Samuel, O.D.; Owamah, H.I.; Saleel, C.A.; Enweremadu, C.C.; Reddy, M.S.; Afzal, A. Artificial neural networks model for predicting the behavior of different injection pressure characteristics powered by blend of biofuel-nano emulsion. Energy Sci. Eng. 2022, 10, 2367–2396. [Google Scholar] [CrossRef]
- Chen, H.; Zhang, Z.; Guan, C.; Gao, H. Optimization of sizing and frequency control in battery/supercapacitor hybrid energy storage system for fuel cell ship. Energy 2020, 197, 117285. [Google Scholar] [CrossRef]
- Pan, P.; Sun, Y.; Yuan, C.; Yan, X.; Tang, X. Research progress on ship power systems integrated with new energy sources: A review. Renew. Sustain. Energy Rev. 2021, 144, 111048. [Google Scholar] [CrossRef]
- Mutarraf, M.U.; Terriche, Y.; Niazi, K.A.K.; Vasquez, J.C.; Guerrero, J.M. Energy Storage Systems for Shipboard Microgrids—A Review. Energies 2018, 11, 3492. [Google Scholar] [CrossRef] [Green Version]
- Yuan, Y.; Wang, J.; Yan, X.; Shen, B.; Long, T. A review of multi-energy hybrid power system for ships. Renew. Sustain. Energy Rev. 2020, 132, 110081. [Google Scholar] [CrossRef]
- Smith, T.W.P.; Jalkanen, J.P.; Anderson, B.A.; Corbett, J.J.; Faber, J.; Hanayama, S.; O’Keeffe, E.; Parker, S.; Johansson, L.; Aldous, L.; et al. Third IMO Greenhouse Gas Study 2014; International Maritime Organization: London, UK, 2014. [Google Scholar]
- Mokashi, I.; Afzal, A.; Khan, S.A.; Abdullah, N.A.; Azami, M.H.B.; Jilte, R.D.; Samuele, O.D. Nusselt number analysis from a battery pack cooled by different fluids and multiple back-propagation modelling using feed-forward networks. Int. J. Therm. Sci. 2021, 161, 106738. [Google Scholar] [CrossRef]
- Becker, J.; Nemeth, T.; Wegmann, R.; Sauer, D.U. Dimensioning and Optimization of Hybrid Li-Ion Battery Systems for EVs. World Electr. Veh. J. 2018, 9, 19. [Google Scholar]
- Syb ten Cate Hoedemaker. Solutions for Large Batteries for Waterborne Transport, D2.1–Application Matrix; SEABAT; Uniresearch BV: Delft, The Netherlands, 2021. [Google Scholar]
- Bocklisch, T. Hybrid energy storage approach for renewable energy applications. J. Energy Storage 2016, 8, 311–319. [Google Scholar] [CrossRef]
- Kouchachvili, L.; Yaïci, W.; Entchev, E. Hybrid battery/supercapacitor energy storage system for the electric. J. Power Sources 2018, 374, 237–248. [Google Scholar] [CrossRef]
- Song, Z.; Li, J.; Hou, J.; Hofmann, H.; Ouyang, M.; Du, J. The battery-supercapacitor hybrid energy storage system in electric vehicle applications: A case study. Energy 2018, 154, 433–441. [Google Scholar] [CrossRef]
- Nemeth, T.; Kollmeyer, P.J.; Emadi, A.; Sauer, D.U. Optimized Operation of a Hybrid Energy Storage System with LTO Batteries for High Power Electrified Vehicles. In Proceedings of the 2019 IEEE Transportation Electrification Conference and Expo (ITEC), Detroit, MI, USA, 19–21 June 2019. [Google Scholar]
- Zhang, X.; Peng, H.; Wang, H.; Ouyang, M. Hybrid Lithium Iron Phosphate Battery and Lithium Titanate Battery Systems for Electric Buses. IEEE Trans. Veh. Technol. 2017, 67, 956–965. [Google Scholar] [CrossRef]
- Degaa, L.; Rizoug, N.; Bendjedia, B.; Saidane, A.; Larouci, C. Sizing improvement of hybrid storage system composed with high energy and high power Li-ion batteries for automotive applications. J. Syst. Control Eng. 2019, 233, 870–876. [Google Scholar] [CrossRef]
- Min, H.; Lai, C.; Yu, Y.; Zhu, T.; Zhang, C. Comparison Study of Two Semi-Active Hybrid Energy Storage Systems for Hybrid Electric Vehicle Applications and Their Experimental Validation. Energies 2017, 10, 279. [Google Scholar] [CrossRef] [Green Version]
- Zhuanga, W.; Yea, J.; Songb, Z.; Yina, G.; Lia, G. Comparison of semi-active hybrid battery system configurations for electric taxis application. Appl. Energy 2020, 259, 11471. [Google Scholar] [CrossRef]
- Wieczorek, M.; Lewandowski, M.; Staroński, K.; Pierzchała, M. Experimental Validation of Energy Management Strategy in Hybrid Energy Storage System for Electric Vehicle. In Proceedings of the 2018 IEEE Transportation Electrification Conference and Expo (ITEC), Long Beach, CA, USA, 13–15 June 2018. [Google Scholar]
- Kim, K.; An, J.; Park, K.; Roh, G.; Chun, K. Analysis of a Supercapacitor/Battery Hybrid Power System for a Bulk Carrier. Appl. Sci. 2019, 9, 1547. [Google Scholar] [CrossRef] [Green Version]
- Balsamo, F.; Capasso, C.; Lauria, D.; Veneri, O. Optimal design and energy management of hybrid storage systems for marine propulsion applications. Appl. Energy 2020, 278, 115629. [Google Scholar] [CrossRef]
- Batteries Onboard Ocean-Going Vessels; MAN Energy Solutions: Copenhagen, Denmark, 2019.
- DAMEN RSD-E-Tug. Available online: https://products.damen.com/-/media/Products/Images/Clusters-groups/Tugs/RSD-Tugs/RSD-E-Tug/Documents/RSD_2513_Brochure.pdf (accessed on 7 October 2022).
- Zimmermann, T.; Keil, P.; Hofmann, M.; Horsche, M.F.; Pichlmaier, S. Review of system topologies for hybrid electrical energy storage systems. J. Energy Storage 2016, 8, 78–90. [Google Scholar] [CrossRef]
- Schaltz, E.; Khaligh, A.; Rasmussen, P.O. Influence of Battery/Ultracapacitor Energy-Storage Sizing on Battery Lifetime in a Fuel Cell Hybrid Electric Vehicle. IEEE Trans. Veh. Technol. 2009, 58, 3882–3891. [Google Scholar] [CrossRef]
- Stroe, D.I. Lifetime Models for Lithium-Ion Batteries Used in Virtual Power Plant Applications. Ph.D. Thesis, Department of Energy Technology, Aalborg University, Aalborg, Denmark, 2014. [Google Scholar]
- Stroe, D.I.; Świerczyński, M.; Stan, A.I.; Teodorescu, R.; Andreasen, S.J. Accelerated Lifetime Testing Methodology for Lifetime Estimation of Lithium-Ion Batteries Used in Augmented Wind Power Plants. IEEE Trans. Ind. Appl. 2014, 50, 4006–4017. [Google Scholar] [CrossRef]
- Bao, X.; Xu, X.; Zhang, Y.; Xiong, Y.; Shang, C. Optimal Sizing of Battery Energy Storage System in a Shipboard Power System with considering Energy Management Optimization. Discrete Dyn. Nat. Soc. 2021, 2021, 9032206. [Google Scholar] [CrossRef]
- Diaz, V.S.; Cantane, D.A.; Santos, A.Q.O.; Junior, O.H.A. Comparative Analysis of Degradation Assessment of Battery Energy Storage Systems in PV Smoothing Application. Energies 2021, 14, 3600. [Google Scholar] [CrossRef]







| Parameter | Value | |
|---|---|---|
| Chemistry | NMC (HE cell) | LTO (HP cell) |
| Capacity | 50 Ah | 23 Ah |
| Nominal voltage | 3.65 V | 2.3 V |
| Standard charge/discharge C-rate | 1/1 | 4/4 |
| Energy density | 206 Wh/kg | 96 Wh/kg |
| Weight | 0.885 kg | 0.55 kg |
| Internal resistance | 1.5 mΩ | 1.1 mΩ |
| Battery cost | 150 € | 380 € |
| DC/DC converter efficiency | 0.98 | |
| DC/DC cost | 85 €/kW | |
| Monotype | Monotype | HESS | ||
|---|---|---|---|---|
| Cell type | HE | HP | HE | HP |
| 274 | 435 | 274 | 435 | |
| 60 | 62 | 29 | 17 | |
| Total number of cells | 16,440 | 26,970 | 7946 | 7395 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Akbarzadeh, M.; De Smet, J.; Stuyts, J. Battery Hybrid Energy Storage Systems for Full-Electric Marine Applications. Processes 2022, 10, 2418. https://doi.org/10.3390/pr10112418
Akbarzadeh M, De Smet J, Stuyts J. Battery Hybrid Energy Storage Systems for Full-Electric Marine Applications. Processes. 2022; 10(11):2418. https://doi.org/10.3390/pr10112418
Chicago/Turabian StyleAkbarzadeh, Mohsen, Jasper De Smet, and Jeroen Stuyts. 2022. "Battery Hybrid Energy Storage Systems for Full-Electric Marine Applications" Processes 10, no. 11: 2418. https://doi.org/10.3390/pr10112418
APA StyleAkbarzadeh, M., De Smet, J., & Stuyts, J. (2022). Battery Hybrid Energy Storage Systems for Full-Electric Marine Applications. Processes, 10(11), 2418. https://doi.org/10.3390/pr10112418









