Wellbore Temperature and Pressure Calculation of Offshore Gas Well Based on Gas–Liquid Separated Flow Model
Abstract
:1. Introduction
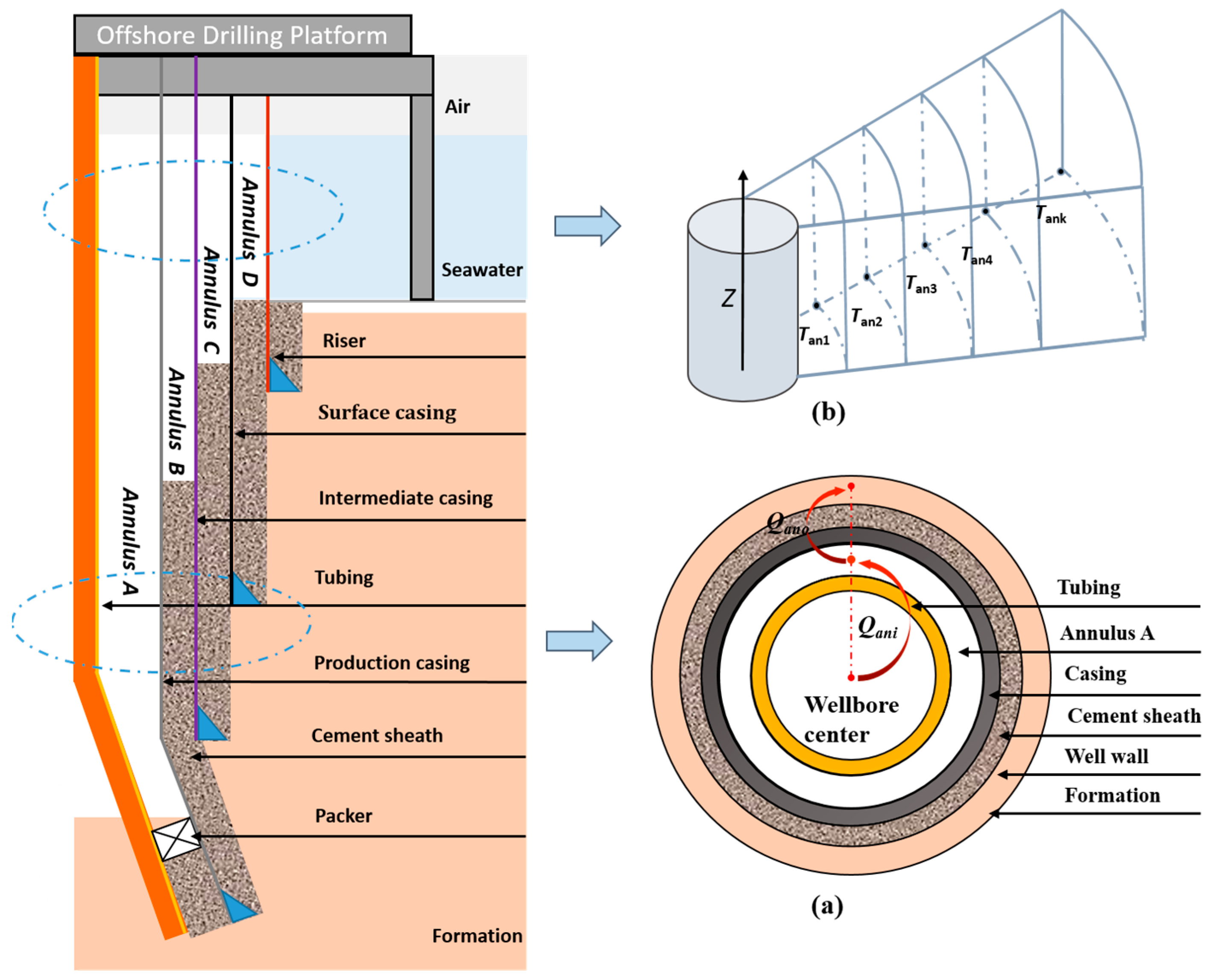
2. Calculation Model of Wellbore Temperature Field
- Assume that gas–liquid two-phase fluid flows in a one-dimensional steady direction in the tubing. The fluid flow is turbulent flow, and there is no phase change.
- Tubing is concentric with the riser, casing, formation, and cement sheath. Further, the cement sheath is well-bonded to the formation and casing.
- The temperature profile of the formation and seawater is approximately linear. Further, the air temperature is considered the same as the temperature of the sea level.
- When the fluid flows in the tubing, only the heat-transfer process occurs, and no energy exchange with the outside world occurs.
- Radial heat transfer occurs between the fluid in the tubing and the environment, and axial heat transfer in the flow direction is ignored.
2.1. Tubing Heat-Transfer Model
2.2. Wellbore Annulus Temperature Calculation
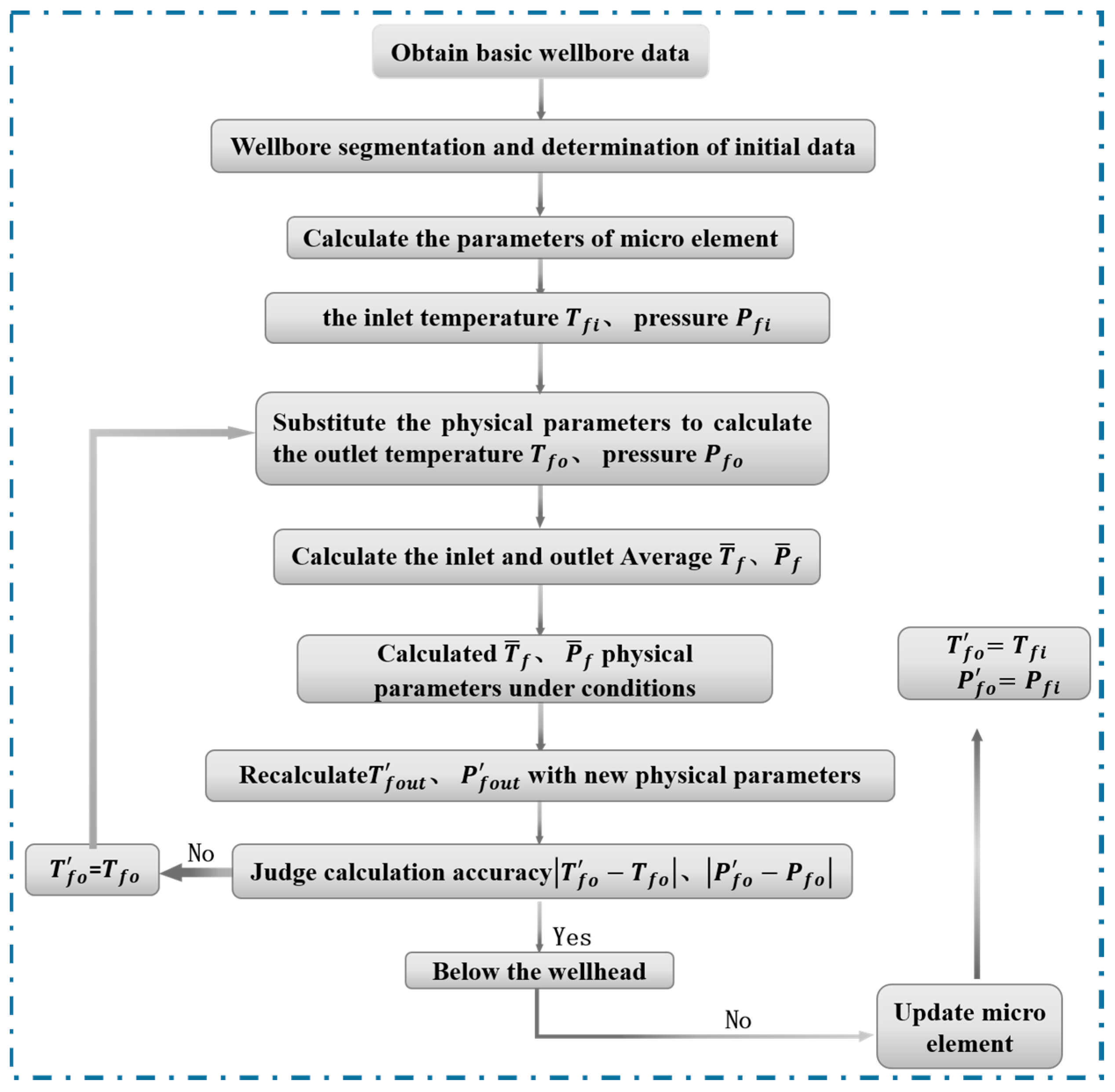
3. Wellbore Pressure Field Calculation Model
4. Example Calculation
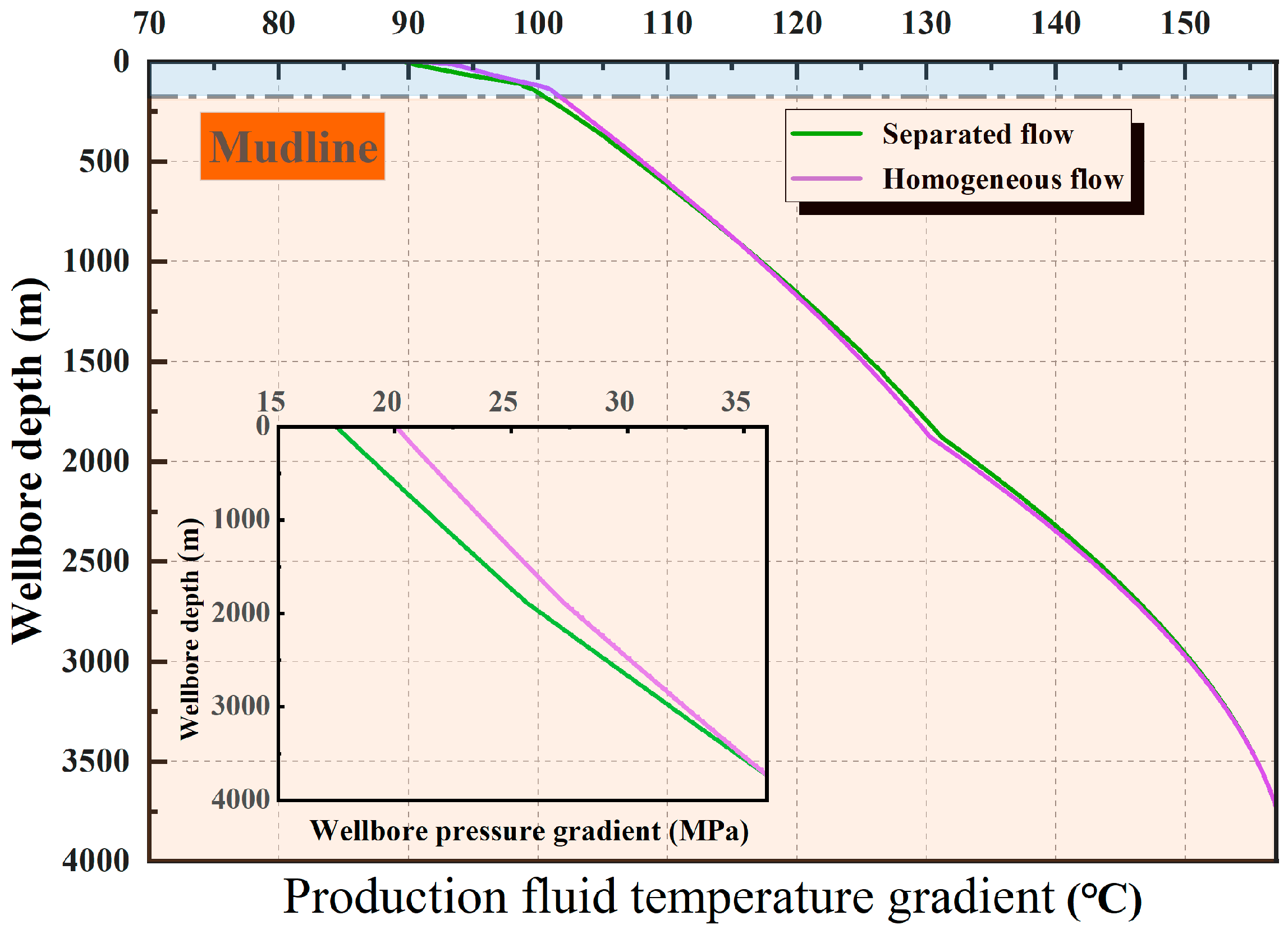
4.1. Model Verification
4.2. Wellbore Temperature Field
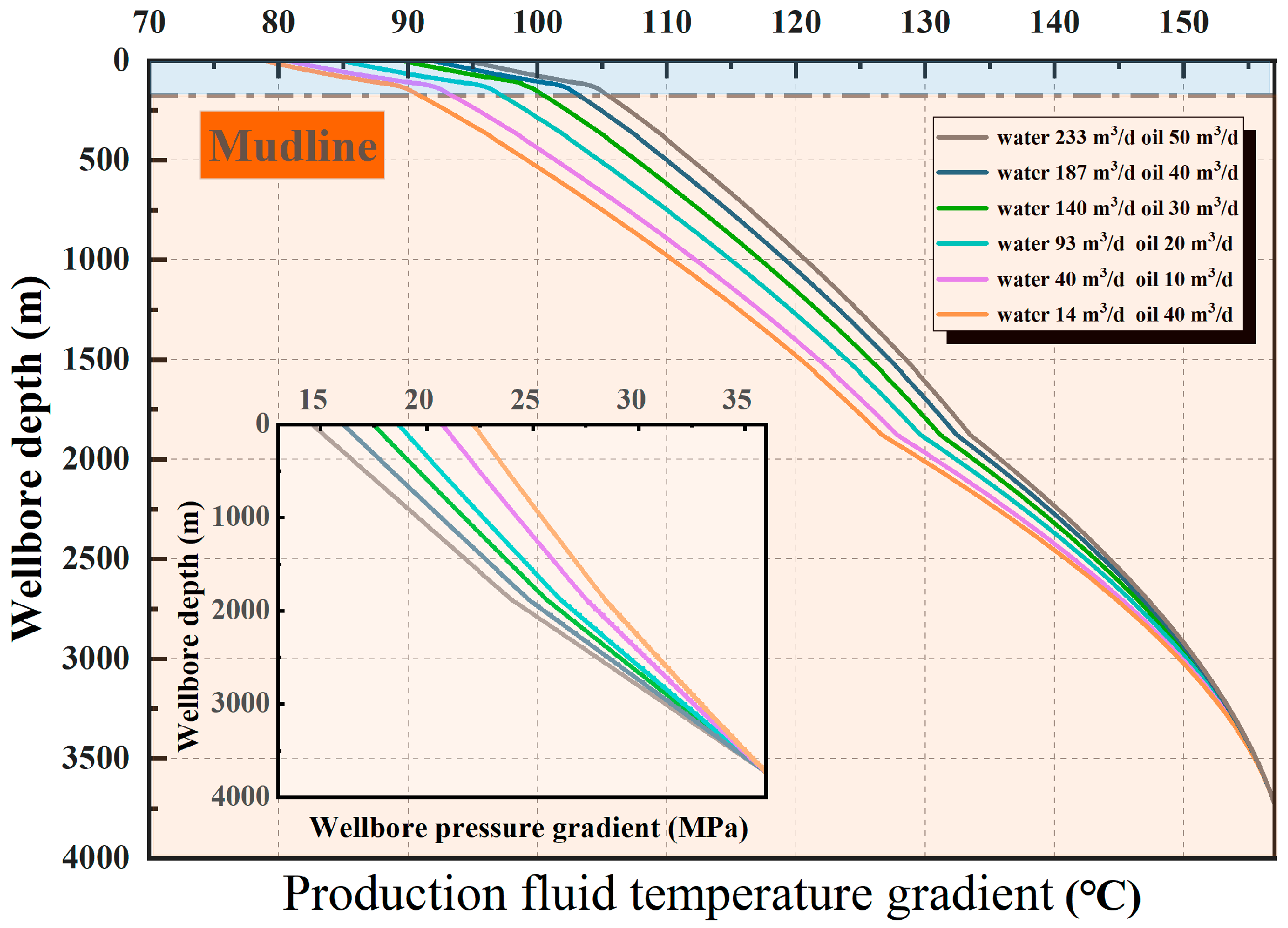
4.3. Gas–Liquid Ratio Production Fluid
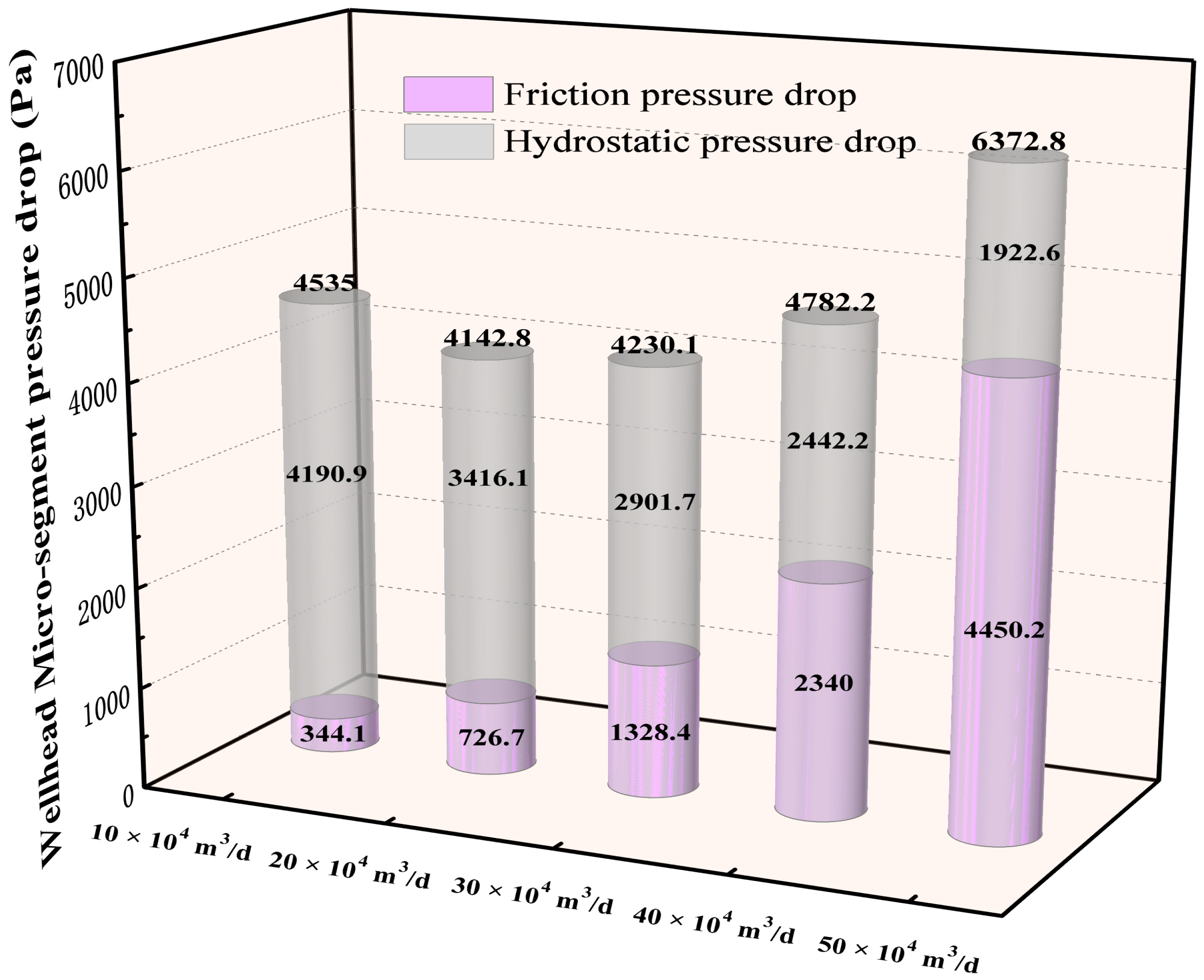
4.4. Production Conditions
5. Conclusions
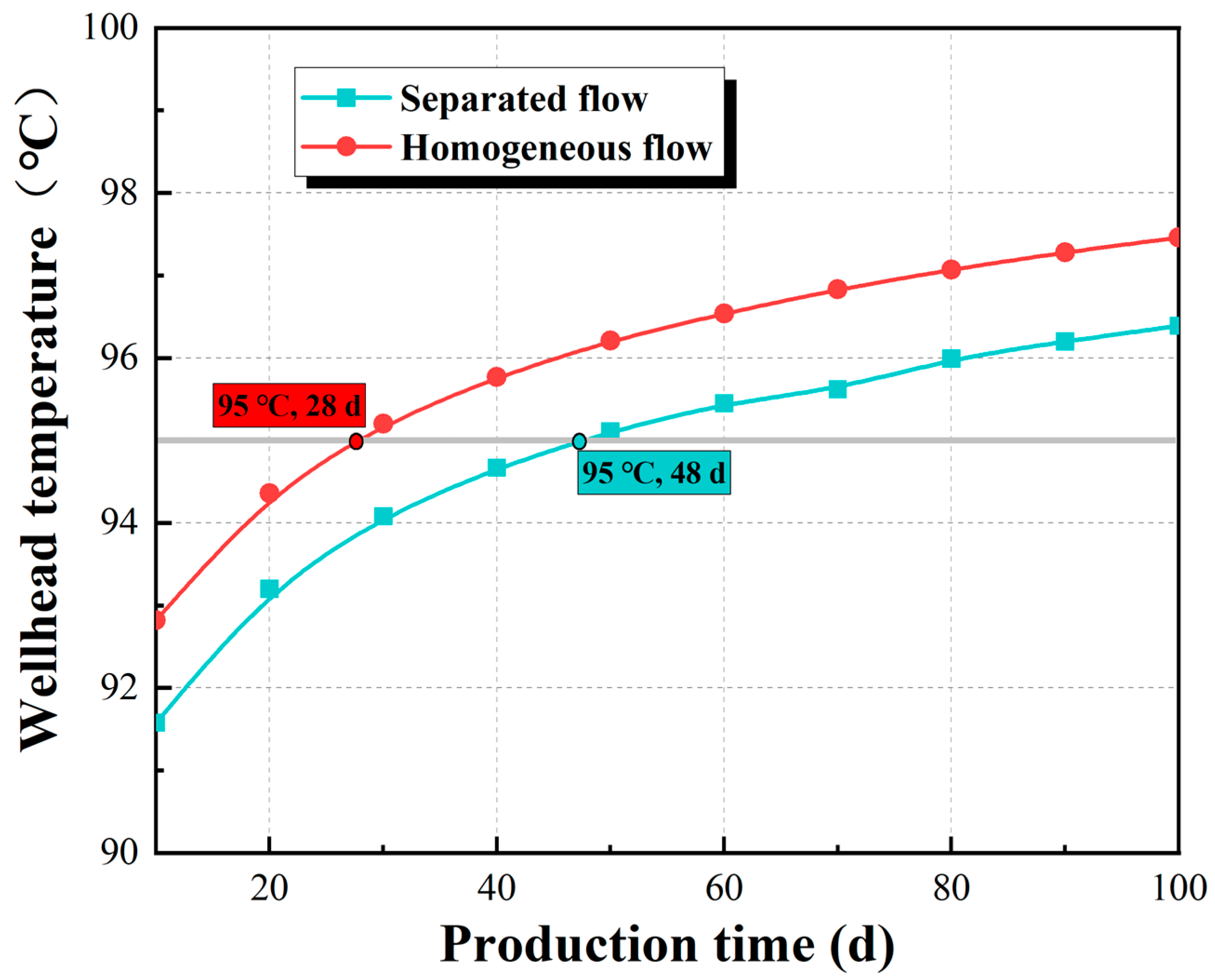
- Although the results of homogeneous flow and separated phase flow have the same trend, the errors of wellhead temperature and pressure obtained by homogeneous flow calculation are large compared with measured data, which are 2.33% and 17.45%. However, the errors of the improved model are only 0.87% and 2.46%, which greatly improves the accuracy of wellbore temperature and pressure calculation.
- Forced convection heat exchange between seawater–air and wellbore is stronger than that between wellbore and formation. As a result, the temperature drop rate of the tubing in the seawater and air sections is significantly accelerated. Therefore, the influence of the seawater and air sections on the wellbore temperature of offshore gas wells should be fully considered.
- Since the specific heat capacity of liquid phase is much larger than that of gas phase, the wellbore temperature becomes increasingly high as the gas–liquid ratio of the product decreases (liquid-phase content increases). However, it also leads to the increase in hydrostatic pressure and friction pressure loss as the mixed fluid density rises, causing the wellbore pressure decrease. Based on this trend, the liquid content of the product should be controlled in a reasonable range in order to avoid the risk of casing failure due to high wellbore pressure.
- Gas production is the key factor affecting wellbore temperature. When the gas production rises from 10 to 60 , the wellhead temperature rises from 63 to 99 . However, due to the mutual influence of friction pressure drop and hydrostatic pressure drop, wellbore pressure increases first and then decreases with the increase in gas production. It takes 28 days for the wellhead temperature to reach 95 in the model based on homogeneous-phase flow, which is much faster than 48 days in the separated model. Therefore, the improved model can provide a more accurate estimate of the time to reach the rated wellhead temperature and accurate theoretical support for the rational formulation of the production plan after the well opening, so as to avoid excessive restrictions on the initial production rate.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| HPHT | High temperature and high pressure |
| TOC | Top of cement |
References
- Luo, M.; Zhu, H.Y.; Liu, Q.Y.; Wang, Z.W.; Li, Y.J.; Han, C.; Zhang, C. A V-cutter PDC bit suitable for ultra-HTHP plastic mudstones. Nat. Gas Ind. 2021, 41, 97–106. [Google Scholar]
- Xu, M.G.; Fan, C.W.; Zhang, D.N.; Hu, G.W.; Tan, J.C. Formation condition and hydrocarbon accumulation model in Ledong 01 Gas Reservoir of super high temperature and high pressure in the Yinggehai Basin. Nat. Gas Ind. 2021, 41, 43–53. [Google Scholar]
- Chen, H.; Yang, C.; Datta-Gupta, A.; Zhang, J.; Chen, L.; Liu, L.; Chen, B.; Cui, X.; Shi, F.; Bahar, A. Fracture inference and optimal well placement using a multiscale history matching in a HPHT tight gas reservoir, Tarim Basin, China. Upstream Oil Gas Technol. 2020, 2, 100002. [Google Scholar] [CrossRef]
- Wang, Y.H.; Hao, Y.Z.; Li, F.; Lu, F.F.; Deng, K.H.; Lin, Y.H. Failure mechanism of wellbore integrity for HTHP gas wells, Shunnan block, Tarim Basin. Nat. Gas Explor. Dev. 2019, 42, 86–89. [Google Scholar]
- Xie, H.; Zhang, G.W.; Liu, X.Q.; Chen, G.M.; Chang, Y.J. Mechanism of Christmas tree rise in offshore oil and gas wells and the related calculation method. Nat. Gas Ind. 2013, 33, 80–84. [Google Scholar]
- Ramey, H.J. Wellbore Heat Transmission. J. Pet. Technol. 1962, 4, 427–435. [Google Scholar] [CrossRef]
- Shiu, K.C.; Begges, H. Predicting Temperatures in Flowing Oil Wells. J. Energy Resour. Technol. 1980, 102, 2–11. [Google Scholar] [CrossRef]
- Hasan, A.R.; Kabir, C.S. Heat transfer during two phase flow in wellbores. In Proceedings of the 66th Annual Technical Conference and ExhiMion of the Society of Petroleum Engineers, Dallas, TX, USA, 6–9 October 1991. [Google Scholar]
- Spindler, R. Analytical Models for Wellbore-Temperature Distribution. SPE J. 2011, 1, 125–133. [Google Scholar] [CrossRef]
- Wu, Z.; Xu, J.; Wang, X.; Chen, K.; Li, X.; Zhao, X. Predicting Temperature and Pressure in High-Temperature–High-Pressure Gas Wells. Petrol. Sci. Technol. 2011, 29, 132–148. [Google Scholar] [CrossRef]
- Xu, J.; Hu, J.; Wu, Z.; Wang, S.; Qi, B. Prediction of Temperature and Pressure Distribution in HTHP Injection Gas Wells with Thermal Effect of Wellbore. Petrol. Sci. Technol. 2013, 31, 1423–1438. [Google Scholar] [CrossRef]
- Martínez-Cuenca, R.; Mondragón, R.; Hernández, L.; Segarra, C.; Jarque, J.C.; Hibiki, T.; Juliá, J.E. Forced-convective heat-transfer coefficient and pressure drop of water-based nanofluids in a horizontal pipe. Appl. Therm. Eng. 2016, 98, 841–849. [Google Scholar] [CrossRef]
- Yin, B.; Li, X.; Qi, M. Study of Fluid Phase Behavior and Pressure Calculation Methods of a Condensate Gas Well. Petrol. Sci. Technol. 2013, 31, 1595–1607. [Google Scholar] [CrossRef]
- Gao, Y.; Cui, Y.; Xu, B.; Sun, B.; Zhao, X.; Li, H.; Chen, L. Two phase flow heat transfer analysis at different flow patterns in the wellbore. Appl. Therm. Eng. 2017, 117, 544–552. [Google Scholar] [CrossRef]
- Hou, Z.; Yan, T.; Li, Z.; Feng, J.; Sun, S.; Yuan, Y. Temperature prediction of two phase flow in wellbore using modified heat transfer model: An experimental analysis. Appl. Therm. Eng. 2019, 149, 54–61. [Google Scholar] [CrossRef]
- Song, X.C.; Wei, L.G.; He, L.; Feng, J.; Sun, S.; Gou, Y.B. Transient temperature field model of shut-in offshore wells. J. China Univ. Pet. 2013, 37, 94–99. [Google Scholar]
- Ma, Y.Q. Study on Law of Gas-Liquid Two-Phase Flow Heat Transfer in Deep-Water Wellbore; China University of Petroleum: Qingdao, China, 2010. [Google Scholar]
- Sivaramkrishnan, K.; Huang, B.; Jana, A.K. Predicting wellbore dynamics in a steam-assisted gravity drainage system: Numeric and semi-analytic model, and validation. Appl. Therm. Eng. 2015, 91, 679–686. [Google Scholar] [CrossRef]
- Hasan, A.R.; Jang, M. An analytic model for computing the countercurrent flow of heat in tubing and annulus system and its application: Jet pump. J. Petrol. Sci. Eng. 2021, 203, 108492. [Google Scholar] [CrossRef]
- Zhang, Z.; Wang, H. Effect of thermal expansion annulus pressure on cement sheath mechanical integrity in HPHT gas wells. Appl. Therm. Eng. 2017, 118, 600–611. [Google Scholar] [CrossRef]
- Hasan, A.R.; Lzgec, B.; Kabir, C.S. Sustained production by managing annular-pressure buildup. SPE J. 2010, 25, 195–202. [Google Scholar]
- Ghajar, A.J. Single-and Two-Phase Flow Pressure Drop and Heat Transfer in Tubes; Springer: Berlin/Heidelberg, Germany, 2021. [Google Scholar]
- Izgec, B.; Hasan, A.R.; Lin, D.; Kabir, C.S. Flow-rate estimation from wellhead-pressure and temperature data. In Proceedings of the SPE Annual Technical Conference and Exhibition (ATCE 2008), Denver, CO, USA, 21–24 September 2008; Volume 25, pp. 31–39. [Google Scholar]
- Popiel, C.O. Free Convection Heat Transfer from Vertical Slender Cylinders: A Review. Heat Transf. Eng. 2008, 29, 521–536. [Google Scholar] [CrossRef]
- Hasan, A.R.; Kabir, C.S. A Simple Model for Annular Two-Phase Flow in Wellbores. In Proceedings of the Annual Technical Conference and Exhibition of the Society of Petroleum Engineers 2005 (ATCE 2005), Dallas, TX, USA, 9–12 October 2005; pp. 168–175. [Google Scholar]
- Zhang, Z.; Wang, H. Sealed annulus thermal expansion pressure mechanical calculation method and application among multiple packers in HPHT gas wells. J. Nat. Gas Sci. Eng. 2016, 31, 692–702. [Google Scholar] [CrossRef]
- Bhagwat, S.M.; Ghajar, A.J. Experimental investigation of non-boiling gas-liquid two phase flow in upward inclined pipes. Exp. Therm. Fluid Sci. 2016, 79, 301–318. [Google Scholar] [CrossRef]
- Bhagwat, S.M. Experimental Measurements and Modeling of Void Fraction and Pressure Drop in Upward and Downward Inclined Non-Boiling Gas-Liquid Two Phase Flow; Oklahoma State University: Stillwater, OK, USA, 2015. [Google Scholar]






| Parameter | Value | Parameter | Value |
|---|---|---|---|
| Formation temperature | Cement sheath thermal conductivity | ||
| Formation pressure | 36 MPa | Formation thermal conductivity | |
| Mudline temperature | Annulus liquid thermal conductivity | ||
| Seawater temperature gradient | Annulus liquid density | ||
| Air temperature | Annulus liquid specific heat capacity | ||
| Formation thermal diffusivity | Annulus fluid viscosity | ||
| Gas production | Tubing thermal conductivity | ||
| Water production | Casing thermal conductivity | ||
| Oil production | Well inclination angle | ||
| Production time | 84 h | Seawater depth | 120 m |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jing, J.; Shan, H.; Zhu, X.; Huangpu, Y.; Tian, Y. Wellbore Temperature and Pressure Calculation of Offshore Gas Well Based on Gas–Liquid Separated Flow Model. Processes 2022, 10, 2043. https://doi.org/10.3390/pr10102043
Jing J, Shan H, Zhu X, Huangpu Y, Tian Y. Wellbore Temperature and Pressure Calculation of Offshore Gas Well Based on Gas–Liquid Separated Flow Model. Processes. 2022; 10(10):2043. https://doi.org/10.3390/pr10102043
Chicago/Turabian StyleJing, Jun, Hongbin Shan, Xiaohua Zhu, Yixiang Huangpu, and Yang Tian. 2022. "Wellbore Temperature and Pressure Calculation of Offshore Gas Well Based on Gas–Liquid Separated Flow Model" Processes 10, no. 10: 2043. https://doi.org/10.3390/pr10102043
APA StyleJing, J., Shan, H., Zhu, X., Huangpu, Y., & Tian, Y. (2022). Wellbore Temperature and Pressure Calculation of Offshore Gas Well Based on Gas–Liquid Separated Flow Model. Processes, 10(10), 2043. https://doi.org/10.3390/pr10102043








