Numerical Algorithms for Reflected Anticipated Backward Stochastic Differential Equations with Two Obstacles and Default Risk

Abstract

We study numerical algorithms for reflected anticipated backward stochastic differential equations (RABSDEs) driven by a Brownian motion and a mutually independent martingale in a defaultable setting. The generator of a RABSDE includes the present and future values of the solution. We introduce two main algorithms, a discrete penalization scheme and a discrete reflected scheme basing on a random walk approximation of the Brownian motion as well as a discrete approximation of the default martingale, and we study these two methods in both the implicit and explicit versions respectively. We give the convergence results of the algorithms, provide a numerical example and an application in American game options in order to illustrate the performance of the algorithms.

1. Introduction

The backward stochastic differential equation (BSDE) theory plays a significant role in financial modeling. Given a probability space $(\Omega ,\mathcal{F},\mathbb{P})$, where $B:={\left(B_t\right)}_{t\ge 0}$ is a d-dimensional standard Brownian motion, $\mathcal{F}:={\left({\mathcal{F}}_t\right)}_{t\ge 0}$ is the associated natural filtration of B, ${\mathcal{F}}_t=\sigma (B_s;0\le s\le t)$, and ${\mathcal{F}}_0$ contains all P-null sets of $\mathcal{F}$. We first consider the following form of BSDE with the generator f and the terminal value $\xi$:

$$Y_t=\xi +{\int }_t^{T}f(s,Y_s,Z_s)ds-{\int }_t^T{Z}_{s}d{B}_s,t\in [0,T].$$

(1)

The setting of this problem is to find a pair of ${\mathcal{F}}_t$-adapted processes $(Y,Z)\in {\mathcal{S}}_{\mathcal{F}}^2(0,T;\mathbb{R})\times {\mathcal{L}}_{\mathcal{F}}^2(0,T;{\mathbb{R}}^d)$ satisfying BSDE (1).

Linear BSDE was first introduced by Bismut (1973), when he studied maximum principle in stochastic optimal control. Pardoux and Peng (1990) studied the general nonlinear BSDEs under a smooth square integrability assumptions on the coefficient and the terminal value, and a Lipschitz condition for the generator f. Duffie and Epstein (1992) independently used a class of BSDEs to describe the stochastic differential utility function theory in uncertain economic environments. Tang and Li (1994) considered the BSDEs driven by a Brownian motion and an independent Poisson jump. Barles et al. (1997) completed the theoretical proofs of BSDE with Poisson jump. Cordoni and Di Persio (2014) studied the hedging, option pricing and insurance problems in a BSDE approach.

Cvitanic and Karatzas (1996) first studied reflected BSDEs with continuous lower obstacle and continuous upper obstacle under the smooth square integrability assumption and Lipschitz condition. A quadruple $(Y,Z,K^{+},K^{-}):={(Y_t,Z_t,K_t^{+},K_t^{-})}_{0\le t\le T}$ is a solution RBSDE with the generator f, the terminal value $\xi$ and the obstacles L and V:

$$\begin{array}{c}\hfill \left\{\begin{array}{c}{Y}_t=\xi +{\int }_t^{T}f(s,Y_s,Z_s)ds+(K_T^{+}-K_t^{+})-(K_T^{-}-K_t^{-})-{\int }_t^T{Z}_{s}d{B}_s,t\in [0,T];\hfill \\ V_t\ge Y_t\ge L_t,t\in [0,T];\hfill \\ {\int }_0^T(Y_t-L_t)d{K}_t^{+}={\int }_0^T(V_t-Y_t)d{K}_t^{-}=0.\hfill \end{array}\right.\end{array}$$

(2)

where $K^{+}$ and $K^{-}$ are continuous increasing processes, $K^{+}$ is to keep Y above L, while $K^{-}$ is to keep Y under V in a minimal way. When $V\equiv \infty$ and $K^{-}\equiv 0$ (resp. $L\equiv \infty$ and $K^{+}\equiv 0$), we obtain a reflected anticipated backward stochastic differential equation (RABSDE) with one lower (resp. upper) obstacle. The existence of the solution of RBSDE with two obstacles can be obtained under one of the following assumptions: (1) one of the obstacles L and V are regular (see e.g., Cvitanic and Karatzas 1996; Hamadene et al. 1997); (2) Mokobodski’s condition (see e.g., Hamadene and Lepeltier 2000; Lepeltier and Xu 2007), which means the existence of a difference of non-negative super-martingales between obstacles L and V. However, both of them have disadvantages, assumption (1) is somewhat restrictive, (2) is difficult to verify in practice. In this paper, we use the Assumption 5 for the obstacles.

Peng and Yang (2009) studied a new type of BSDE, anticipated BSDE (ABSDE) whose generator includes the values of both the present and the future,

$$\{\begin{array}{l}{Y}_t={\xi }_T+{\int }_t^{T}f\left(s,Y_s,{\mathbb{E}}^{{\mathcal{G}}_s}\left[Y_{s+\delta }\right],Z_s,{\mathbb{E}}^{{\mathcal{G}}_s}\left[Z_{s+\delta }\right]\right)ds-{\int }_t^T{Z}_{s}d{B}_s,t\in [0,T];\hfill \\ Y_t={\xi }_t,t\in (T,T+T^{\delta }];\\ Z_t={\alpha }_t,t\in (T,T+T^{\delta }].\end{array}$$

(3)

under the smooth square integrability assumption of the anticipated processes $\xi$ and $\alpha$, and Lipschitz condition of the generator f. Peng and Yang (2009) gave the existence and uniqueness theorem and the comparison theorem of anticipated BSDE (3). Øksendal et al. (2011) extended this topic to ABSDEs driven by a Brownian motion and an independent Poisson random measure. Jeanblanc et al. (2017) studied ABSDEs driven by a Brownian motion and a single jump process.

Default risk is the risk that an investor suffers a loss due to the inability of getting back the initial investment, it arises from a borrower failing to make required payments. This loss may be complete or partial (more see Kusuoka 1999). Peng and Xu (2009) introduced BSDE with default risk and gave the relative existence and uniqueness theorem and comparison theorem. Jiao and Pham (2011) studied the optimal investment with counterparty risk. Jiao et al. (2013) continued the research on the optimal investment under multiple default risk through a BSDE approach. Cordoni and Di Persio (2016) studied the BSDE with delayed generator in a defaultable setting. In this paper, we focus on the study of reflected anticipated BSDE with two obstacles and default risk.

For the numerical methods of BSDEs, Peng and Xu (2011) studied numerical algorithms for BSDEs driven by Brownian motion. Xu (2011) introduced a discrete penalization scheme and a discrete reflected scheme for RBSDE with two obstacles. Later Dumitrescu and Labart (2016) extended to RBSDE with two obstacles driven by Brownian motion and an independent compensated Poisson process. Lin and Yang (2014) studied the discrete BSDE with random terminal horizon.

The paper is organized as follows, we first introduce the basics of the defaultable model in Section 1.1 and the reflected anticipated BSDE (4) with two obstacles and default risk in Section 1.3. Section 2 illustrates the discrete time framework. We study the implicit and the explicit methods of two discrete schemes, i.e., the discrete penalization scheme in Section 3 and the discrete reflected scheme in Section 4. Section 5 completes the convergence results of the numerical algorithms which were provided in the previous sections. In Section 6, we illustrate the performance of the algorithms by a simulation example and an application in American game options in the defaultable setting. The proofs of the convergence results in Section 5 can be found in the Appendix A.

1.1. Basics of the Defaultable Model

Let $\tau =\{{\tau }_i;i=1,\dots k\}$ be k non-negative random variables on a probability space $(\Omega ,\mathcal{G},\mathbb{P})$ satisfying

$$\mathbb{P}({\tau }_i>0)=1;\mathbb{P}({\tau }_i>t)>0,\forall t>0;\mathbb{P}({\tau }_i={\tau }_j)=0,i\ne j.$$

For each $i=1\dots ,k$, we define a right-continuous default process $H^i:={\left(H_t^i\right)}_{t\ge 0}$, where $H_t^i:=1_{\{{\tau }_i\le t\}}$, denote by ${\mathcal{H}}^i:={\left({\mathcal{H}}_t^i\right)}_{t\ge 0}$ the associated filtration ${\mathcal{H}}_t^i:=\sigma (H_s^i;0\le s\le t)$. We assume that ${\mathcal{F}}_0$ is trivial (it follows that ${\mathcal{G}}_0$ is trivial as well). For a fixed terminal time $T\ge 0$, there are two kinds of information: one is from the asset prices, denoted by $\mathcal{F}:={\left({\mathcal{F}}_t\right)}_{0\le t\le T}$; the other is from the default times $\{{\tau }_i;i=1,\dots ,k\}$, denoted by $\{{\mathcal{H}}^i;i=1,\dots ,k\}$.

The enlarged filtration considered is denoted by $\mathcal{G}:={\left({\mathcal{G}}_t\right)}_{0\le t\le T}$, where ${\mathcal{G}}_t={\mathcal{F}}_t\vee {\mathcal{H}}_t^1\vee \dots \vee {\mathcal{H}}_t^k$. Generally, a $\mathcal{G}$-stopping time is not necessarily a $\mathcal{F}$-stopping time. Let $G:=G_t$, where $G_t=\mathbb{P}(\tau >t|{\mathcal{F}}_t)$, i.e., $G_t^i=\mathbb{P}({\tau }_i>t|{\mathcal{F}}_t)$, for each $i=1,\dots ,k$. In the following, $G^i$ is assumed to be continuous, then the random default time ${\tau }_i$ is totally inaccessible $\mathcal{G}$-stopping time. The processes $H^i$ $(i=1,\dots ,k)$ are obviously $\mathcal{G}$-adapted, but they are not necessarily $\mathcal{F}$-adapted. We need the following assumptions (see Kusuoka 1999; Bielecki et al. 2007):

Assumption 1.

There exist $\mathcal{F}$-adapted processes ${\gamma }^i\ge 0(i=1,\dots ,k)$ such that

$$M_t^i=H_t^i-{\int }_0^t{1}_{\{{\tau }_i>s\}}{\gamma }_s^{i}ds$$

are $\mathcal{G}$-martingales under $\mathbb{P}$. ${\gamma }^i$ is the $\mathcal{G}$-intensity of the default time ${\tau }_i$:

$${\gamma }_t^i=\underset{\Delta \to 0}{\lim }\frac{\mathbb{P}(t<{\tau }_i\le t+\Delta |{\mathcal{F}}_t)}{\Delta \mathbb{P}({\tau }_i>t|{\mathcal{F}}_t)},t\in [0,T];$$

Assumption 2.

Every $\mathcal{F}$-local martingale is a $\mathcal{G}$-local martingale.

1.2. Basic Notions

• ${\mathcal{L}}^2({\mathcal{G}}_T;\mathbb{R}):=\{\phi \in \mathbb{R}|\phi$ is a ${\mathcal{G}}_T$-measurable random variable and ${\mathbb{E}|\phi |}^2<\infty \}$;
• ${\mathcal{L}}_{\mathcal{G}}^2(0,t;{\mathbb{R}}^d):=\{\phi :\Omega \times [0,t]\to {\mathbb{R}}^d|{\phi }_t$ is ${\mathcal{G}}_t$-progressively measurable and $\mathbb{E}{{\int }_0^t|{\phi }_s|}^{2}ds<\infty \}$;
• ${\mathcal{S}}_{\mathcal{G}}^2(0,t;\mathbb{R}):=\{\phi :\Omega \times [0,t]\to \mathbb{R}|{\phi }_t$ is ${\mathcal{G}}_t$-progressively measurable rcll process and $\mathbb{E}\left[{{\sup }_{0\le s\le t}|{\phi }_s|}^2\right]<\infty \}$;
• ${\mathcal{L}}_{\mathcal{G}}^{2,\tau }(0,t;{\mathbb{R}}^k):=\{\phi :\Omega \times [0,t]\to {\mathbb{R}}^k|{\phi }_t$ is ${\mathcal{G}}_t$-progressively measurable and satisfies $\mathbb{E}\left[{{\int }_0^t|{\phi }_s|}^p{1}_{\{\tau >s\}}{\gamma }_{s}ds\right]=\mathbb{E}\left[{{\int }_0^t{\sum }_{i=1}^k|{\phi }_{i,s}|}^2{1}_{\{{\tau }_i>s\}}{\gamma }_s^{i}ds\right]<\infty \}$;
• ${\mathcal{A}}_{\mathcal{G}}^2(0,T;\mathbb{R}):=\{K:\Omega \times [0,T]\to \mathbb{R}|K_t$ is a ${\mathcal{G}}_t$-adapted rcll increasing process and $K_0=0$, $K_T\in {\mathcal{L}}^p({\mathcal{G}}_T;\mathbb{R})\}$;

1.3. Reflected Anticipated BSDEs with Two Obstacles and Default Risk

Consider the RABSDE below with two obstacles and default risk with coefficient $(f,\xi ,\delta ,L,V)$. $(Y,Z,U,K^{+},K^{-}):={(Y_t,Z_t,U_t,K_t^{+},K_t^{-})}_{0\le t\le T+\delta }$ is a solution for RABSDE with the generator f, the terminal value ${\xi }_T$, the anticipated processes $\xi$, the anticipated time $\delta$ ($\delta >0$ is a constant), and the obstacles L and V, such that

$$\{\begin{array}{l}{Y}_t={\xi }_T+{\int }_t^{T}f\left(s,Y_s,{\mathbb{E}}^{{\mathcal{G}}_s}\left[Y_{s+\delta }\right],Z_s,U_s\right)ds+(K_T^{+}-K_t^{+})-(K_T^{-}-K_t^{-})\\ -{\int }_t^T{Z}_{s}d{B}_s-{\int }_t^T{U}_{s}d{M}_s,t\in [0,T];\\ V_t\ge Y_t\ge L_t,t\in [0,T];\\ Y_t={\xi }_t,t\in (T,T+\delta ];\\ {\int }_0^T(Y_t-L_t)d{K}_t^{+}={\int }_0^T(V_t-Y_t)d{K}_t^{-}=0,\end{array}$$

(4)

where $Y\in {\mathcal{S}}_{\mathcal{G}}^2(0,T+\delta ;\mathbb{R})$, $Z\in {\mathcal{L}}_{\mathcal{G}}^2(0,T;{\mathbb{R}}^d)$, $U\in {\mathcal{L}}_{\mathcal{G}}^{2,\tau }(0,T;{\mathbb{R}}^k)$, $K^{\pm }\in {\mathcal{A}}_{\mathcal{G}}^2(0,T;\mathbb{R})$. We further state the following assumptions for RABSDE (4):

Assumption 3.

The anticipated process $\xi \in {\mathcal{L}}_{\mathcal{G}}^2(T,T+\delta ;{\mathbb{R}}^d)$, $\alpha \in {\mathcal{L}}_{\mathcal{G}}^2(T,T+T^{\delta };{\mathbb{R}}^d)$, here ξ is a given process, and ${\xi }_T$ is the terminal value;

Assumption 4.

The generator $f(w,t,y,{\overline{y}}_r,z,{\overline{z}}_r,u,{\overline{u}}_r):\Omega \times [0,T+T^{\delta }]\times \mathbb{R}\times {\mathcal{S}}_{\mathcal{G}}^2(t,T+T^{\delta };\mathbb{R})\times {\mathbb{R}}^d\times {\mathcal{L}}_{\mathcal{G}}^2(t,T+T^{\delta };{\mathbb{R}}^d)\times {\mathbb{R}}^k\to {\mathcal{L}}_{\mathcal{G}}^{2,\tau }(t,T+T^{\delta };{\mathbb{R}}^k)$ satisfies:

(a) 

$f(·,0,0,0,0)\in {\mathcal{L}}_{\mathcal{G}}^2(0,T+\delta ;\mathbb{R})$;

(b) 

Lipschitz condition: for any $t\in [0,T]$, $r\in [t,T+\delta ]$, y, $y^{\prime }\in \mathbb{R}$, z, $z^{\prime }\in {\mathbb{R}}^d$, u, $u^{\prime }\in {\mathbb{R}}^k$, $\overline{y}$, ${\overline{y}}^{\prime }\in {\mathcal{L}}_{\mathcal{G}}^2(t,T+\delta ;\mathbb{R})$, there exists a constant $L\ge 0$ such that

$$\begin{array}{c}|f(t,y,{\overline{y}}_r,z,u)-f(t,y^{\prime },{\overline{y}}_r^{\prime },z^{\prime },u^{\prime })|\le L\left(|y-y^{\prime }|+{\mathbb{E}}^{{\mathcal{G}}_t}|{\overline{y}}_r-{\overline{y}}_r^{\prime }|+|z-z^{\prime }|+|u-u^{\prime }|1_{\{\tau >t\}}\sqrt{{\gamma }_t}\right);\hfill \end{array}$$

(c) 

for any $t\in [0,T]$, $r\in [t,T+\delta ]$, y, $y^{\prime }\in \mathbb{R}$, z, $z^{\prime }\in {\mathbb{R}}^d$, u, $u^{\prime }\in {\mathbb{R}}^k$, $\overline{y}$, ${\overline{y}}^{\prime }\in {\mathcal{L}}_{\mathcal{G}}^2(t,T+T^{\delta };\mathbb{R})$, the following holds:

$$\begin{array}{c}\hfill \frac{f(t,y,{\overline{y}}_r,z,{\tilde{u}}^{i-1})-f(t,y,{\overline{y}}_r,z,{\tilde{u}}^i)}{(u^i-{\tilde{u}}^i)1_{\{{\tau }^i>t\}}{\gamma }_t^i}>-1,\end{array}$$

where ${\tilde{u}}^i=({\tilde{u}}^1,{\tilde{u}}^2,\dots ,{\tilde{u}}^i,u^{i+1},\dots ,u^k)$, $u^i$ is the i-th element of u.

Assumption 5.

The obstacle processes satisfy L, $V\in {\mathcal{S}}_{\mathcal{G}}^2(0,T+\delta ;\mathbb{R})$:

(a) 

for any $t\in [0,T]$, $V_T\ge \xi \ge L_T$, L and V are separated, i.e., $V_t>L_t$, $\mathbb{P}-a.s.$;

(b) 

L and V are rcll and their jumping times are totally inaccessible and satisfy

$$\mathbb{E}\left[\underset{0\le t\le T}{\sup }{\left(L_t^{+}\right)}^2\right]<\infty ,\mathbb{E}\left[\underset{0\le t\le T}{\sup }{\left(V_t^{-}\right)}^2\right]<\infty ;$$

(c) 

there exists a process of the following form:

$$X_t=X_0-{\int }_0^t{\sigma }_s^{\left(1\right)}d{B}_s-{\int }_0^t{\sigma }_s^{\left(2\right)}d{M}_s+A_t^{+}-A_t^{-},$$

where $X_T={\xi }_T$, ${\sigma }^{\left(1\right)}\in {\mathcal{L}}_{\mathcal{G}}^2(0,T;{\mathbb{R}}^d)$, ${\sigma }^{\left(2\right)}\in {\mathcal{L}}_{\mathcal{G}}^{2,\tau }(0,T;{\mathbb{R}}^k)$, $A^{+}$ and $A^{-}$ are $\mathcal{G}$-adapted increasing processes, $\mathbb{E}[|A_T^{+}{|}^2+|A_T^{-}{|}^2]<\infty$, such that

$$V_t\ge X_t\ge L_t,t\in [0,T],\mathbb{P}-a.s.$$

2. Discrete Time Framework

In order to discretize $[0,T]$, for $n\in \mathbb{N}$, we introduce ${\Delta }^n:=\frac{T}{n}$ and an equidistant time grid ${\left(t_i\right)}_{i=0,1,\dots n,\dots n^{\delta }}$ with step size ${\Delta }^n$, where $t_i:=i{\Delta }^n$, $n^{\delta }=n+\left[\frac{\delta }{{\Delta }^n}\right]$.

2.1. Random Walk Approximation of the Brownian Motion

We use a random walk to approximate the 1-dimensional standard Brownian motion:

$$\begin{array}{c}{B}_0^n=0;B_t^n:=\sqrt{{\Delta }^n}\sum _{j=1}^{[t/{\Delta }^n]}{ϵ}_j^n,t\in (0,T];\hfill \\ \Delta B_i^n:=B_i^n-B_{i-1}^n=\sqrt{{\Delta }^n}{ϵ}_i^n,i\in [1,n^{\delta }],\hfill \end{array}$$

where ${\left({ϵ}_i^n\right)}_{i=1,\dots n}$ is a $\{-1,1\}$-value i.i.d. Bernoulli sequence with $\mathbb{P}({ϵ}_i^n=1)=\mathbb{P}({ϵ}_i^n=-1)=\frac{1}{2}$. Denote ${\mathcal{F}}_i^n=\sigma \{{ϵ}_1^n,\dots {ϵ}_i^n\}$, for any $i\in [1,n^{\delta }]$. By Donsker’s invariance principle and the Skorokhod representation theorem, there exists a probability space, such that ${\sup }_{0\le t\le T+\delta }|B_t^n-B_t|\to 0$, in ${\mathcal{L}}^2\left({\mathcal{G}}_{T+\delta }\right)$, as $n\to \infty$.

2.2. Approximation of the Defaultable Model

We consider a defaultable model of a single uniformly distributed random default time $\tau \in (0,T]$. We define the discrete default process $h_i^n=h_{t_i}^n=1_{\{\tau \le t_i\}}$ ($i\in [1,n]$). Particularly, when $i\in [n+1,n^{\delta }]$, $h_i^n=1$ (since default case already happened). We have the conditional expectations of $h_i^n$ in ${\mathcal{G}}_{i-1}^n$:

$$\begin{array}{cc}\hfill \mathbb{E}\left[h_i^n=1|h_{i-1}^n=1\right]=& \mathbb{P}(\tau \le t_i|\tau \le t_{i-1})=1,\hfill \\ \hfill \mathbb{E}\left[h_i^n=1|h_{i-1}^n=0\right]=& \mathbb{P}(\tau \le t_i|\tau >t_{i-1})=\frac{{\Delta }^n}{T-t_{i-1}},\hfill \\ \hfill \mathbb{E}\left[h_i^n=0|h_{i-1}^n=0\right]=& \mathbb{P}(\tau >t_i|\tau >t_{i-1})=\frac{T-t_i}{T-t_{i-1}},i\in [1,n].\hfill \end{array}$$

We have the following approximation for the discrete martingale $M_t^n$ directly based on the definition of the martingale M (Assumption 1):

$$\begin{array}{cc}\hfill & M_0^n=0;M_t^n:=h_{[t/{\Delta }^n]}^n-{\Delta }^n\sum _{j=1}^{[t/{\Delta }^n]}(1-h_j^n){\gamma }_j^n,t\in (0,T];\hfill \\ \hfill & \Delta M_i^n:=h_i^n-h_{i-1}^n-{\Delta }^n(1-h_i^n){\gamma }_i^n,i\in [1,n],\hfill \end{array}$$

(5)

where the discrete intensity process ${\gamma }_i^n={\gamma }_{t_i}^n\ge 0$ is an ${\mathcal{F}}_i^n$-adapted process. Denote $\mathbb{G}:=\{{\mathcal{G}}_i^n;i\in [0,n^{\delta }]\}$, ${\mathcal{G}}_0^n=\{\Omega ,\varnothing \}$, for $i\in [1,n]$, ${\mathcal{G}}_i^n=\sigma \{{ϵ}_1^n,\dots {ϵ}_i^n,h_i^n\}$; for $i\in [n+1,n^{\delta }]$, ${\mathcal{G}}_i^n=\sigma \{{ϵ}_1^n,\dots {ϵ}_i^n,h_n^n\}$, where $h_i$ is independent from ${ϵ}_1^n$, …${ϵ}_i^n$. From the martingale property of $M_i$, we can get

$${\mathbb{E}}^{{\mathcal{G}}_{i-1}^n}\left[\Delta M_i^n\right]={\mathbb{E}}^{{\mathcal{G}}_{i-1}^n}\left[h_i^n-h_{i-1}^n-{\Delta }^n(1-h_i^n){\gamma }_i^n\right]=0,i\in [1,n],$$

therefore, the discrete intensity process has the following form (by the projection on ${\mathcal{F}}_{i-1}^n$):

$${\gamma }_i^n=\frac{\mathbb{P}(t_{i-1}<\tau \le t_i|{\mathcal{F}}_{i-1}^n)}{{\Delta }^n\mathbb{P}(\tau >t_i|{\mathcal{F}}_{i-1}^n)}=\frac{1}{T-t_i},i\in [1,\left[\frac{\tau }{{\Delta }^n}\right]].$$

Note that ${\gamma }_i^n=0$, when $i=0$ and $i\in [\left[\frac{\tau }{{\Delta }^n}\right]+1,n]$. If we set ${\widehat{\gamma }}_t^n={\gamma }_{[t/{\Delta }^n]}^n$ ($t\in [0,T]$), then as $n\to \infty$, it follows that ${\widehat{\gamma }}_t^n$ converges to ${\gamma }_t$.

2.3. Computing the Conditional Expectations

When $i\in [1,n-1]$, we use the following formula to compute the conditional expectation for the function $f:{\mathbb{R}}^{i+2}\to \mathbb{R}$:

$$\begin{array}{cc}& {\mathbb{E}}^{{\mathcal{G}}_i^n}\left[f\left({ϵ}_1^n,\dots ,{ϵ}_{i+1}^n,h_{i+1}^n\right)\right]\hfill \\ \hfill =& \frac{1}{2}\left(f\left({ϵ}_1^n,\dots ,{ϵ}_i^n,1,1\right){|}_{{ϵ}_{i+1}^n=1,h_i^n=1}+f\left({ϵ}_1^n,\dots ,{ϵ}_i^n,-1,1\right){|}_{{ϵ}_{i+1}^n=-1,h_i^n=1}\right)\hfill \\ & +\frac{{\Delta }^n}{2(T-t_i)}\left(f\left({ϵ}_1^n,\dots ,{ϵ}_i^n,1,1\right){|}_{{ϵ}_{i+1}^n=1,h_i^n=0,h_{i+1}^n=1}+f\left({ϵ}_1^n,\dots ,{ϵ}_i^n,-1,1\right){|}_{{ϵ}_{i+1}^n=-1,h_i^n=0,h_{i+1}^n=1}\right)\hfill \\ & +\frac{T-t_{i+1}}{2(T-t_i)}\left(f\left({ϵ}_1^n,\dots ,{ϵ}_i^n,1,0\right){|}_{{ϵ}_{i+1}^n=1,h_i^n=0,h_{i+1}^n=0}+f\left({ϵ}_1^n,\dots ,{ϵ}_i^n,-1,0\right){|}_{{ϵ}_{i+1}^n=-1,h_i^n=0,h_{i+1}^n=0}\right).\hfill \end{array}$$

When $i\in [n,n^{\delta }]$, we have the following conditional expectation for the function $f:{\mathbb{R}}^{i+2}\to \mathbb{R}$:

$$\begin{array}{c}\hfill {\mathbb{E}}^{{\mathcal{G}}_i^n}\left[f\left({ϵ}_1^n,\dots ,{ϵ}_{i+1}^n,h_n^n\right)\right]=\frac{1}{2}f\left({ϵ}_1^n,\dots {ϵ}_i^n,1,1\right){|}_{{ϵ}_{i+1}^n=1}+\frac{1}{2}f\left({ϵ}_1^n,\dots {ϵ}_i^n,-1,1\right){|}_{{ϵ}_{i+1}^n=-1}.\end{array}$$

2.4. Approximations of the Anticipated Processes and the Generator

Consider the approximation ${\xi }_n^n$ of the terminal value $\xi$, we have the following assumption:

Assumption 6.

${\left({\xi }_i^n\right)}_{i\in [n,n^{\delta }]}$ is ${\mathcal{G}}_i^n$-measurable, $\Psi :{\{1,-1\}}^n\to \mathbb{R}$ is a real analytic function, such that

$${\xi }_i^n=\Psi \left({ϵ}_1^n,\dots {ϵ}_i^n,h_i^n\right),i\in [n,n^{\delta }],$$

particularly, the terminal value ${\xi }_n^n=\Psi \left({ϵ}_1^n,\dots {ϵ}_n^n,h_i^n\right)$ is ${\mathcal{G}}_n^n$-measurable.

For the approximation ${\left(f^n(t_i,y,\overline{y},z,u)\right)}_{i\in [0,n]}$ of the generator f:

Assumption 7.

for any $i\in [0,n]$, $f^n(t_i,y,\overline{y},z,u)$ is ${\mathcal{G}}_i^n$-adapted, and satisfies:

(a) 

there exists a constant $C>0$, such that for all $n>1+2L+4L^2$,

$$\mathbb{E}\left[{\Delta }^n\sum _{i=0}^{n-1}{|f^n(·,0,0,0,0)|}^2\right]<C.$$

(b) 

for any $i\in [0,n-1]$, y, $y^{\prime }\in \mathbb{R}$, z, $z^{\prime }\in \mathbb{R}$, u, $u^{\prime }\in \mathbb{R}$, $\overline{y}$, ${\overline{y}}^{\prime }\in {\mathcal{S}}_{\mathcal{G}}^2(t,T+\delta ;\mathbb{R})$, there exists a constant $L\ge 0$, such that

$$\begin{array}{c}\hfill |f^n(t_i,y,{\overline{y}}_i,z,u)-f^n(t_i,y^{\prime },{\overline{y}}_i^{\prime },z^{\prime },u^{\prime })|\le L\left(|y-y^{\prime }|+{\mathbb{E}}^{{\mathcal{G}}_t}|{\overline{y}}_i-{\overline{y}}_i^{\prime }|+|z-z^{\prime }|+|u-u^{\prime }|1_{\{\tau >t_i\}}\sqrt{{\gamma }_i}\right),\end{array}$$

where ${\overline{y}}_i={\mathbb{E}}^{{\mathcal{G}}_i^n}\left[y_{\overline{i}}\right]$, $\overline{i}=i+\left[\frac{\delta }{{\Delta }^n}\right]$.

As $n\to \infty$, $f^n(\left[\frac{t}{{\Delta }^n}\right],y,\overline{y},z,u)$ converges to $f(t,y,\overline{y},z,u)$ in ${\mathcal{S}}_{\mathcal{G}}^2(0,T+\delta ;\mathbb{R})$.

2.5. Approximation of the Obstacles

${\left(L_i^n\right)}_{i\in [0,n]}$ and ${\left(V_i^n\right)}_{i\in [0,n]}$ are the discrete versions of L and V, by Assumption 5, we can have the following approximations:

$$\begin{array}{cc}\hfill L_i^n& =L_0+{\Delta }^n\sum _{j=0}^{i-1}{l}_j^{\left(1\right)}+\sum _{j=0}^{i-1}{l}_j^{\left(2\right)}\Delta B_{j+1}^n+\sum _{j=0}^{i-1}{l}_j^{\left(3\right)}\Delta M_{j+1}^n;\hfill \\ \hfill V_i^n& =V_0+{\Delta }^n\sum _{j=0}^{i-1}{v}_j^{\left(1\right)}+\sum _{j=0}^{i-1}{v}_j^{\left(2\right)}\Delta B_{j+1}^n+\sum _{j=0}^{i-1}{v}_j^{\left(3\right)}\Delta M_{j+1}^n,\hfill \end{array}$$

where $l_j^{\left(k\right)}=l_{t_j}^{\left(k\right)}$, $v_j^{\left(k\right)}=v_{t_j}^{\left(k\right)}$ ($k=1,2,3$). By the Burkholder–Davis–Gundy inequality, it follows

$$V_i^n\ge L_i^n,\underset{n}{\sup }\mathbb{E}\left[\underset{i}{\sup }{\left(L_i^n\right)}^{+2}+\underset{i}{\sup }{\left(V_i^n\right)}^{-2}\right]<\infty .$$

We introduce the discrete version of Assumption 5 (c):

Assumption 8.

There exists a process $X_i^n$ with the following form:

$$X_i^n=X_0^n-\sum _{j=0}^{i-1}{\sigma }_j^{\left(1\right)}\Delta B_{i+1}^n-\sum _{j=0}^{i-1}{\sigma }_j^{\left(2\right)}\Delta M_{i+1}^n+A_i^{+n}-A_i^{-n},$$

where $A_i^{+n}$ and $A_i^{-n}$ are ${\mathcal{G}}_i^n$-adapted increasing processes, $\mathbb{E}\left[|A_n^{+n}{|}^2+{|A_n^{-n}|}^2\right]<\infty$, such that

$$L_i^n\le X_i^n\le V_i^n,i\in [0,n].$$

We introduce two numerical algorithms below, discrete penalization scheme in Section 3 and discrete reflected scheme in Section 4. For each scheme, we study the implicit and explicit versions.

3. Discrete Penalization Scheme

We first use the methodology of penalization for the discrete scheme below. El Karoui et al. (1997a) proved the existence of RBSDE with one obstacle under a smooth square integrability assumption and Lipschitz condition through penalization method. Lepeltier and Martín (2004) used the similar penalization method to prove the existence theorem of RBSDE with two obstacles and Poisson jump. Similarly to Lemma 4.3.1 in Wang (2020), we consider the following special case of the penalized ABSDE for RABSDE (2):

$$\begin{array}{cc}\hfill -d{Y}_t^p=& f^n\left(t,Y_t^p,{\mathbb{E}}^{{\mathcal{G}}_t}\left[Y_{t+\delta }^p\right],Z_t^p,U_t^p\right)dt+d{K}_t^{+p}-d{K}_t^{-p}-Z_t^{p}d{B}_t-U_t^{p}d{M}_t,t\in [0,T],\hfill \\ \hfill Y_t^p=& {\xi }_t,t\in [T,T+\delta ],\hfill \end{array}$$

(6)

where

$$K_t^{+p}=p{\int }_t^T{(Y_s^p-L_s)}^{-}ds,K_t^{-p}=p{\int }_t^T{(Y_s^p-V_s)}^{+}ds.$$

By the existence and uniqueness theorem for ABSDEs with default risk (Theorem 4.3.3 in Wang 2020), there exists the unique solution for this penalized ABSDE (6). We will give the convergence of penalized ABSDE (6) to RABSDE (2) in Theorem 1 below.

3.1. Implicit Discrete Penalization Scheme

We first introduce the implicit discrete penalization scheme. In this scheme, p represents the penalization parameter. In practice, we can choose p which is independent of n and much larger than n, this will be illustrated in the simulation Section 6.

$$\begin{array}{c}\hfill \left\{\begin{array}{c}{y}_i^{p,n}=y_{i+1}^{p,n}+f^n(t_i,y_i^{p,n},{\overline{y}}_i^{p,n},z_i^{p,n},u_i^{p,n}){\Delta }^n+k_i^{+p,n}-k_i^{-p,n}\hfill \\ -z_i^{p,n}\Delta B_{i+1}^n-u_i^{p,n}\Delta M_{i+1}^n,i\in [0,n-1];\hfill \\ k_i^{+p,n}=p{\Delta }^n{(y_i^{p,n}-L_i^n)}^{-},i\in [0,n-1];\hfill \\ k_i^{-p,n}=p{\Delta }^n{(y_i^{p,n}-V_i^n)}^{+},i\in [0,n-1];\hfill \\ y_i^{p,n}={\xi }_i^n,i\in [n,n^{\delta }],\hfill \end{array}\right.\end{array}$$

(7)

where ${\overline{y}}_i^{p,n}={\mathbb{E}}^{{\mathcal{G}}_i^n}\left[y_{\overline{i}}^{p,n}\right]$, $\overline{i}=i+\left[\frac{\delta }{{\Delta }^n}\right]$.

For the theoretical convergence results in Section 5, we first prove the convergence (Theorem 2) of implicit discrete penalization scheme (7) to the penalized ABSDE (6), then combining with Theorem A2, we can get the convergence of the explicit discrete penalization scheme. By Theorem A3 and Theorem 1, we can prove the convergence of the implicit discrete reflected scheme (13).

From Section 2.3, taking conditional expectation in ${\mathcal{G}}_i^n$, we can calculate ${\overline{y}}_i^{p,n}$ as follows:

$$\begin{array}{c}\hfill {\mathbb{E}}^{{\mathcal{G}}_i^n}\left[y_{\overline{i}}^{p,n}\right]=\left\{\begin{array}{c}\frac{1}{2}\left(y_{\overline{i}}^{p,n}{|}_{{ϵ}_{\overline{i}}^n=1,h_i^n=1}+y_{\overline{i}}^{p,n}{|}_{{ϵ}_{\overline{i}}^n=-1,h_i^n=1}\right)\hfill \\ +\frac{\delta }{2(T-t_i)}\left(y_{\overline{i}}^{p,n}{|}_{{ϵ}_{\overline{i}}^n=1,h_i^n=0,h_{\overline{i}}^n=1}+y_{\overline{i}}^{p,n}{|}_{{ϵ}_{\overline{i}}^n=-1,h_i^n=0,h_{\overline{i}}^n=1}\right)\hfill \\ +\frac{T-t_i-\delta }{2(T-t_i)}\left(y_{\overline{i}}^{p,n}{|}_{{ϵ}_{\overline{i}}^n=1,h_i^n=0,h_{\overline{i}}^n=0}+y_{\overline{i}}^{p,n}{|}_{{ϵ}_{\overline{i}}^n=-1,h_i^n=0,h_{\overline{i}}^n=0}\right),\overline{i}\in [i,n-1];\hfill \\ \frac{1}{2}\left({\xi }_{\overline{i}}^n{|}_{{ϵ}_{\overline{i}}^n=1}+{\xi }_{\overline{i}}^n{|}_{{ϵ}_{\overline{i}}^n=-1}\right),\overline{i}\in [n,n^{\delta }].\hfill \end{array}\right.\end{array}$$

(8)

Similarly, $z_i^{p,n}$ and $u_i^{p,n}$ ($i\in [0,n-1]$) are given by

$$\begin{array}{cc}\hfill z_i^{p,n}=& \frac{{\mathbb{E}}^{{\mathcal{G}}_i^n}\left[y_{i+1}^{p,n}\Delta B_{i+1}^n\right]}{{\mathbb{E}}^{{\mathcal{G}}_i^n}\left[{(\Delta B_{i+1}^n)}^2\right]}=\frac{{\mathbb{E}}^{{\mathcal{G}}_i^n}\left[y_{i+1}^{p,n}\sqrt{{\Delta }^n}{ϵ}_{i+1}^n\right]}{{\mathbb{E}}^{{\mathcal{G}}_i^n}\left[{\left(\sqrt{{\Delta }^n}{ϵ}_{i+1}^n\right)}^2\right]}\hfill \\ \hfill =& \frac{1}{\sqrt{{\Delta }^n}}{\mathbb{E}}^{{\mathcal{G}}_i^n}\left[y_{i+1}^{p,n}{ϵ}_{i+1}^n\right]=\frac{y_{i+1}^{p,n}{|}_{{ϵ}_{i+1}=1}-y_{i+1}^{p,n}{|}_{{ϵ}_{i+1}=-1}}{2\sqrt{{\Delta }^n}};\hfill \\ \hfill u_i^{p,n}=& \frac{{\mathbb{E}}^{{\mathcal{G}}_i^n}\left[y_{i+1}^{p,n}\Delta M_{i+1}^n\right]}{{\mathbb{E}}^{{\mathcal{G}}_i^n}\left[{(\Delta M_{i+1}^n)}^2\right]}=\frac{{\mathbb{E}}^{{\mathcal{G}}_i^n}\left[y_{i+1}^{p,n}\left(h_{i+1}^n-h_i^n-{\Delta }^n(1-h_{i+1}^n){\gamma }_{i+1}\right)\right]}{{\mathbb{E}}^{{\mathcal{G}}_i^n}\left[{(h_{i+1}^n-h_i^n-{\Delta }^n(1-h_{i+1}^n){\gamma }_{i+1})}^2\right]}\hfill \\ \hfill =& \frac{y_{i+1}^{p,n}{\Delta }^n{|}_{h_i=0,h_{i+1}=1}-y_{i+1}^{p,n}(T-t_{i+1}){\Delta }^n{\gamma }_{i+1}{|}_{h_i=0,h_{i+1}=0}}{{\Delta }^n+(T-t_{i+1}){\left({\Delta }^n{\gamma }_{i+1}\right)}^2}.\hfill \end{array}$$

(9)

Note that $u_i^{p,n}$ only exists on $[0,\left[\frac{\tau }{{\Delta }^n}\right]-1]$ (i.e., before the default event happens). By taking the conditional expectation of (7) in ${\mathcal{G}}_i^n$, it follows:

$$\begin{array}{c}\hfill \left\{\begin{array}{c}{y}_i^{p,n}={\left({\Phi }^{p,n}\right)}^{-1}\left({\mathbb{E}}^{{\mathcal{G}}_i^n}\left[y_{i+1}^n\right]\right),i\in [0,n-1];\hfill \\ k_i^{+p,n}=p{\Delta }^n{(y_i^{p,n}-L_i^n)}^{-},i\in [0,n-1];\hfill \\ k_i^{-p,n}=p{\Delta }^n{(y_i^{p,n}-V_i^n)}^{+},i\in [0,n-1];\hfill \\ y_i^{p,n}={\xi }_i^n,i\in [n,n^{\delta }];\hfill \\ z_i^{p,n}=\frac{1}{\sqrt{{\Delta }^n}}{\mathbb{E}}^{{\mathcal{G}}_i^n}\left[y_{i+1}^{p,n}{ϵ}_{i+1}^n\right],i\in [0,n-1];\hfill \\ u_i^{p,n}=\frac{{\mathbb{E}}^{{\mathcal{G}}_i^n}\left[y_{i+1}^{p,n}\left(h_{i+1}^n-h_i^n-{\Delta }^n(1-h_{i+1}^n){\gamma }_{i+1}\right)\right]}{{\mathbb{E}}^{{\mathcal{G}}_i^n}\left[{(h_{i+1}^n-h_i^n-{\Delta }^n(1-h_{i+1}^n){\gamma }_{i+1})}^2\right]},i\in [0,\left[\frac{\tau }{{\Delta }^n}\right]-1].\hfill \end{array}\right.\end{array}$$

(10)

where ${\Phi }^{p,n}\left(y\right)=y-f^n(t_i,y,{\overline{y}}_i^{p,n},z_i^{p,n},u_i^{p,n}){\Delta }^n-p{\Delta }^n{(y-L_i^n)}^{-}+p{\Delta }^n{(y-V_i^n)}^{+}$. For the continuous time version ${(Y_t^{p,n},Z_t^{p,n},U_t^{p,n},K_t^{+p,n},K_t^{-p,n})}_{0\le t\le T}$:

$$\begin{array}{c}{Y}_t^{p,n}:=y_{[t/{\Delta }^n]}^{p,n},Z_t^{p,n}:=z_{[t/{\Delta }^n]}^{p,n},U_t^{p,n}:=u_{[t/{\Delta }^n]}^{p,n},\hfill \\ K_t^{+p,n}:=\sum _{i=0}^{[t/{\Delta }^n]}{k}_i^{+p,n},K_t^{-p,n}:=\sum _{i=0}^{[t/{\Delta }^n]}{k}_i^{-p,n}.\hfill \end{array}$$

3.2. Explicit Discrete Penalization Scheme

In many cases, the inverse of mapping $\Phi$ is not easy to get directly, for example, if f is not a linear function on y. We replace $y_i^{p,n}$ in $f^n$ by ${\mathbb{E}}^{{\mathcal{G}}_i^n}\left[y_{i+1}^{p,n}\right]$ in (7), it follows

$$\begin{array}{c}\hfill \left\{\begin{array}{c}{\tilde{y}}_i^{p,n}={\tilde{y}}_{i+1}^{p,n}+f^n(t_i,{\mathbb{E}}^{{\mathcal{G}}_i^n}\left[{\tilde{y}}_{i+1}^{p,n}\right],{\overline{\tilde{y}}}_i^{p,n},{\tilde{z}}_i^{p,n},{\tilde{u}}_i^{p,n}){\Delta }^n+{\tilde{k}}_i^{+p,n}-{\tilde{k}}_i^{-p,n}\hfill \\ -{\tilde{z}}_i^{p,n}\Delta B_{i+1}^n-{\tilde{u}}_i^{p,n}\Delta M_{i+1}^n,i\in [0,n-1];\hfill \\ {\tilde{k}}_i^{+p,n}=p{\Delta }^n{({\tilde{y}}_i^{p,n}-L_i^n)}^{-},i\in [0,n-1];\hfill \\ {\tilde{k}}_i^{-p,n}=p{\Delta }^n{({\tilde{y}}_i^{p,n}-V_i^n)}^{+},i\in [0,n-1];\hfill \\ {\tilde{y}}_i^{p,n}={\xi }_i^n,i\in [n,n^{\delta }],\hfill \end{array}\right.\end{array}$$

(11)

where ${\overline{\tilde{y}}}_i^{p,n}$, ${\tilde{z}}_i^{p,n}$ and ${\tilde{u}}_i^{p,n}$ can be calculated as (8) and (9). By Section 2.3, we computer ${\mathbb{E}}^{{\mathcal{G}}_i^n}\left[y_{i+1}^{p,n}\right]$ as follows:

$$\begin{array}{cc}\hfill {\mathbb{E}}^{{\mathcal{G}}_i^n}\left[{\tilde{y}}_{i+1}^{p,n}\right]=& \frac{1}{2}\left({\tilde{y}}_{i+1}^{p,n}{|}_{{ϵ}_{i+1}^n=1,h_i^n=1}+{\tilde{y}}_{i+1}^{p,n}{|}_{{ϵ}_{i+1}^n=-1,h_i^n=1}\right)\hfill \\ & +\frac{{\Delta }^n}{2(T-t_i)}\left({\tilde{y}}_{i+1}^{p,n}{|}_{{ϵ}_{i+1}^n=1,h_i^n=0,h_{i+1}^n=1}+{\tilde{y}}_{i+1}^{p,n}{|}_{{ϵ}_{i+1}^n=-1,h_i^n=0,h_{i+1}^n=1}\right)\hfill \\ & +\frac{T-t_{i+1}}{2(T-t_i)}\left({\tilde{y}}_{i+1}^{p,n}{|}_{{ϵ}_{i+1}^n=1,h_i^n=0,h_{i+1}^n=0}+{\tilde{y}}_{i+1}^{p,n}{|}_{{ϵ}_{i+1}^n=-1,h_i^n=0,h_{i+1}^n=0}\right).\hfill \end{array}$$

By taking the conditional expectation of (11) in ${\mathcal{G}}_i^n$, we have the following explicit penalization scheme:

$$\begin{array}{c}\hfill \left\{\begin{array}{c}{\tilde{y}}_i^{p,n}={\mathbb{E}}^{{\mathcal{G}}_i^n}\left[{\tilde{y}}_{i+1}^{p,n}\right]+f^n(t_i,{\mathbb{E}}^{{\mathcal{G}}_i^n}\left[{\tilde{y}}_{i+1}^{p,n}\right],{\overline{\tilde{y}}}_i^{p,n},{\tilde{z}}_i^{p,n},{\tilde{u}}_i^{p,n}){\Delta }^n+{\tilde{k}}_i^{+p,n}-{\tilde{k}}_i^{-p,n},i\in [0,n-1];\hfill \\ {\tilde{k}}_i^{+p,n}=\frac{p{\Delta }^n}{1+p{\Delta }^n}{\left({\mathbb{E}}^{{\mathcal{G}}_i^n}\left[{\tilde{y}}_{i+1}^{p,n}\right]+f^n(t_i,{\mathbb{E}}^{{\mathcal{G}}_i^n}\left[{\tilde{y}}_{i+1}^{p,n}\right],{\overline{\tilde{y}}}_i^{p,n},{\tilde{z}}_i^{p,n},{\tilde{u}}_i^{p,n}){\Delta }^n-L_i^n\right)}^{-},i\in [0,n-1];\hfill \\ {\tilde{k}}_i^{-p,n}=\frac{p{\Delta }^n}{1+p{\Delta }^n}{\left({\mathbb{E}}^{{\mathcal{G}}_i^n}\left[{\tilde{y}}_{i+1}^{p,n}\right]+f^n(t_i,{\mathbb{E}}^{{\mathcal{G}}_i^n}\left[{\tilde{y}}_{i+1}^{p,n}\right],{\overline{\tilde{y}}}_i^{p,n},{\tilde{z}}_i^{p,n},{\tilde{u}}_i^{p,n}){\Delta }^n-V_i^n\right)}^{+},i\in [0,n-1];\hfill \\ {\tilde{y}}_i^{p,n}={\xi }_i^n,i\in [n,n^{\delta }];\hfill \\ {\tilde{z}}_i^{p,n}=\frac{1}{\sqrt{{\Delta }^n}}{\mathbb{E}}^{{\mathcal{G}}_i^n}\left[{\tilde{y}}_{i+1}^{p,n}{ϵ}_{i+1}^n\right],i\in [0,n-1];\hfill \\ {\tilde{u}}_i^{p,n}=\frac{{\mathbb{E}}^{{\mathcal{G}}_i^n}\left[{\tilde{y}}_{i+1}^{p,n}\left(h_{i+1}^n-h_i^n-{\Delta }^n(1-h_{i+1}^n){\gamma }_{i+1}\right)\right]}{{\mathbb{E}}^{{\mathcal{G}}_i^n}\left[{(h_{i+1}^n-h_i^n-{\Delta }^n(1-h_{i+1}^n){\gamma }_{i+1})}^2\right]},i\in [0,\left[\frac{\tau }{{\Delta }^n}\right]-1].\hfill \end{array}\right.\end{array}$$

(12)

For the continuous time version ${({\tilde{Y}}_t^{p,n},{\tilde{Z}}_t^{p,n},{\tilde{U}}_t^{p,n},{\tilde{K}}_t^{+p,n},{\tilde{K}}_t^{-p,n})}_{0\le t\le T}$:

$$\begin{array}{c}{\tilde{Y}}_t^{p,n}:={\tilde{y}}_{[t/{\Delta }^n]}^{p,n},{\tilde{Z}}_t^{p,n}:={\tilde{z}}_{[t/{\Delta }^n]}^{p,n},{\tilde{U}}_t^{p,n}:={\tilde{u}}_{[t/{\Delta }^n]}^{p,n},\hfill \\ {\tilde{K}}_t^{+p,n}:=\sum _{i=0}^{[t/{\Delta }^n]}{\tilde{k}}_i^{+p,n},{\tilde{K}}_t^{-p,n}:=\sum _{i=0}^{[t/{\Delta }^n]}{\tilde{k}}_i^{-p,n}.\hfill \end{array}$$

Remark 1.

We give the following explanations of the derivation of ${\tilde{k}}_i^{+p,n}$ and ${\tilde{k}}_i^{-p,n}$:

• If $V_i^n>{\tilde{y}}_i^{p,n}>L_i^n$, we can get ${\tilde{k}}_i^{+p,n}={\tilde{k}}_i^{-p,n}=0$;
• If ${\tilde{y}}_i^{p,n}\le L_i^n$, we can get ${\tilde{k}}_i^{+p,n}=\frac{p{\Delta }^n}{1+p{\Delta }^n}({\mathbb{E}}^{{\mathcal{G}}_i^n}\left[{\tilde{y}}_{i+1}^{p,n}\right]+f^n(t_i,{\mathbb{E}}^{{\mathcal{G}}_i^n}\left[{\tilde{y}}_{i+1}^{p,n}\right],{\overline{\tilde{y}}}_i^{p,n},{\tilde{z}}_i^{p,n},$${\tilde{u}}_i^{p,n}){\Delta }^n-L_i^n{)}^{-}$, ${\tilde{k}}_i^{-p,n}=0$. From (12), we know that p should be much larger than n to keep ${\tilde{y}}_i^{p,n}$ above the lower obstacle $L_i^n$;
• If ${\tilde{y}}_i^{p,n}\ge V_i^n$, we can get ${\tilde{k}}_i^{-p,n}=\frac{p{\Delta }^n}{1+p{\Delta }^n}({\mathbb{E}}^{{\mathcal{G}}_i^n}\left[{\tilde{y}}_{i+1}^{p,n}\right]+f^n(t_i,{\mathbb{E}}^{{\mathcal{G}}_i^n}\left[{\tilde{y}}_{i+1}^{p,n}\right],{\overline{\tilde{y}}}_i^{p,n},{\tilde{z}}_i^{p,n},$${\tilde{u}}_i^{p,n}){\Delta }^n-V_i^n{)}^{+}$, ${\tilde{k}}_i^{+p,n}=0$. From (12), we know that p should be much larger than n to keep ${\tilde{y}}_i^{p,n}$ under the upper obstacle $V_i^n$.

4. Discrete Reflected Scheme

We can obtain the solution Y by reflecting between the two obstacles and get the increasing processes $K^{+}$ and $K^{-}$ directly.

4.1. Implicit Discrete Reflected Scheme

We have the following implicit discrete reflected scheme,

$$\begin{array}{c}\hfill \left\{\begin{array}{c}{y}_i^n=y_{i+1}^n+f^n(t_i,y_i^n,{\overline{y}}_i^n,z_i^n,u_i^n){\Delta }^n+k_i^{+n}-k_i^{-n}-z_i^n\Delta B_{i+1}^n-u_i^n\Delta M_{i+1}^n,i\in [0,n-1];\hfill \\ V_i^n\ge y_i^n\ge L_i^n,i\in [0,n-1];\hfill \\ k_i^{+n}\ge 0,k_i^{-n}\ge 0,k_i^{+n}{k}_i^{-n}=0,i\in [0,n-1];\hfill \\ (y_i^n-L_i^n)k_i^{+n}=(y_i^n-V_i^n)k_i^{-n}=0,i\in [0,n-1];\hfill \\ y_i^n={\xi }_i^n,i\in [n,n^{\delta }].\hfill \end{array}\right.\end{array}$$

(13)

where ${\overline{y}}_i^n$, $z_i^n$ and $u_i^n$ can be calculated as (8) and (9). By taking conditional expectation of (13) in ${\mathcal{G}}_i^n$, it follows

$$\begin{array}{c}\hfill \left\{\begin{array}{c}{y}_i^n={\mathbb{E}}^{{\mathcal{G}}_i^n}\left[y_{i+1}^n\right]+f^n(t_i,y_i^n,{\overline{y}}_i^n,z_i^n,u_i^n){\Delta }^n+k_i^{+n}-k_i^{-n},i\in [0,n-1];\hfill \\ V_i^n\ge y_i^n\ge L_i^n,i\in [0,n-1];\hfill \\ k_i^{+n}\ge 0,k_i^{-n}\ge 0,k_i^{+n}{k}_i^{-n}=0,i\in [0,n-1];\hfill \\ (y_i^n-L_i^n)k_i^{+n}=(y_i^n-V_i^n)k_i^{-n}=0,i\in [0,n-1];\hfill \\ y_i^n={\xi }_i^n,i\in [n,n^{\delta }];\hfill \\ z_i^n=\frac{1}{\sqrt{{\Delta }^n}}{\mathbb{E}}^{{\mathcal{G}}_i^n}\left[y_{i+1}^n{ϵ}_{i+1}^n\right],i\in [0,n-1];\hfill \\ u_i^n=\frac{{\mathbb{E}}^{{\mathcal{G}}_i^n}\left[y_{i+1}^n\left(h_{i+1}^n-h_i^n-{\Delta }^n(1-h_{i+1}^n){\gamma }_{i+1}\right)\right]}{{\mathbb{E}}^{{\mathcal{G}}_i^n}\left[{(h_{i+1}^n-h_i^n-{\Delta }^n(1-h_{i+1}^n){\gamma }_{i+1})}^2\right]},i\in [0,\left[\frac{\tau }{{\Delta }^n}\right]-1].\hfill \end{array}\right.\end{array}$$

(14)

If ${\Delta }^n$ is small enough, similarly to Section 4.1 in Xu (2011), (14) is equivalent to

$$\begin{array}{c}\hfill \left\{\begin{array}{c}{y}_i^n={\Phi }^{-1}\left({\mathbb{E}}^{{\mathcal{G}}_i^n}\left[y_{i+1}^n\right]+k_i^{+n}-k_i^{-n}\right),i\in [0,n-1];\hfill \\ k_i^{+n}={\left({\mathbb{E}}^{{\mathcal{G}}_i^n}\left[y_{i+1}^n\right]+f^n(t_i,L_i^n,{\overline{L}}_i^n,z_i^n,u_i^n){\Delta }^n-L_i^n\right)}^{-},i\in [0,n-1];\hfill \\ k_i^{-n}={\left({\mathbb{E}}^{{\mathcal{G}}_i^n}\left[y_{i+1}^n\right]+f^n(t_i,V_i^n,{\overline{V}}_i^n,z_i^n,u_i^n){\Delta }^n-V_i^n\right)}^{+},i\in [0,n-1];\hfill \\ y_i^n={\xi }_i^n,i\in [n,n^{\delta }];\hfill \\ z_i^n=\frac{1}{\sqrt{{\Delta }^n}}{\mathbb{E}}^{{\mathcal{G}}_i^n}\left[y_{i+1}^n{ϵ}_{i+1}^n\right],i\in [0,n-1];\hfill \\ u_i^n=\frac{{\mathbb{E}}^{{\mathcal{G}}_i^n}\left[y_{i+1}^n\left(h_{i+1}^n-h_i^n-{\Delta }^n(1-h_{i+1}^n){\gamma }_{i+1}\right)\right]}{{\mathbb{E}}^{{\mathcal{G}}_i^n}\left[{(h_{i+1}^n-h_i^n-{\Delta }^n(1-h_{i+1}^n){\gamma }_{i+1})}^2\right]},i\in [0,\left[\frac{\tau }{{\Delta }^n}\right]-1].\hfill \end{array}\right.\end{array}$$

(15)

here $\Phi \left(y\right)=y-f^n(t_i,y,{\overline{y}}_i^n,z_i^n,u_i^n){\Delta }^n$. For the continuous time version ${(Y_t^n,Z_t^n,U_t^n,K_t^{+n},K_t^{-n})}_{0\le t\le T}$:

$$\begin{array}{c}{Y}_t^n:=y_{[t/{\Delta }^n]}^n,Z_t^n:=z_{[t/{\Delta }^n]}^n,U_t^n:=u_{[t/{\Delta }^n]}^n,\hfill \\ K_t^{+n}:=\sum _{i=0}^{[t/{\Delta }^n]}{k}_i^{+n},K_t^{-n}:=\sum _{i=0}^{[t/{\Delta }^n]}{k}_i^{-n}.\hfill \end{array}$$

4.2. Explicit Discrete Reflected Scheme

We introduce the following explicit discrete reflected scheme by replacing $y_i^n$ in the generator $f^n$ by $\mathbb{E}[y_{i+1}^n|{\mathcal{G}}_i^n]$ in (13).

$$\begin{array}{c}\hfill \left\{\begin{array}{c}{\tilde{y}}_i^n={\tilde{y}}_{i+1}^n+f^n(t_i,{\mathbb{E}}^{{\mathcal{G}}_i^n}\left[{\tilde{y}}_{i+1}^n\right],{\overline{\tilde{y}}}_i^n,{\tilde{z}}_i^n,{\tilde{u}}_i^n){\Delta }^n+{\tilde{k}}_i^{+n}-{\tilde{k}}_i^{-n}-{\tilde{z}}_i^n\Delta B_{i+1}^n\hfill \\ -{\tilde{u}}_i^n\Delta M_{i+1}^n,i\in [0,n-1];\hfill \\ V_i^n\ge {\tilde{y}}_i^n\ge L_i^n,i\in [0,n-1];\hfill \\ {\tilde{k}}_i^{+n}\ge 0,{\tilde{k}}_i^{-n}\ge 0,{\tilde{k}}_i^{+n}{\tilde{k}}_i^{-n}=0,i\in [0,n-1];\hfill \\ ({\tilde{y}}_i^n-L_i^n){\tilde{k}}_i^{+n}=({\tilde{y}}_i^n-V_i^n){\tilde{k}}_i^{-n}=0,i\in [0,n-1];\hfill \\ {\tilde{y}}_i^n={\xi }_i^n,i\in [n,n^{\delta }].\hfill \end{array}\right.\end{array}$$

(16)

where ${\overline{\tilde{y}}}_i^n$, ${\tilde{z}}_i^n$ and ${\tilde{u}}_i^n$ can be calculated as (8) and (9). By taking conditional expectation of (16) in ${\mathcal{G}}_i^n$:

$$\begin{array}{c}\hfill \left\{\begin{array}{c}{\tilde{y}}_i^n={\mathbb{E}}^{{\mathcal{G}}_i^n}\left[{\tilde{y}}_{i+1}^n\right]+f^n(t_i,{\mathbb{E}}^{{\mathcal{G}}_i^n}\left[{\tilde{y}}_{i+1}^n\right],{\overline{\tilde{y}}}_i^n,{\tilde{z}}_i^n,{\tilde{u}}_i^n){\Delta }^n+{\tilde{k}}_i^{+n}-{\tilde{k}}_i^{-n},i\in [0,n-1];\hfill \\ V_i^n\ge {\tilde{y}}_i^n\ge L_i^n,i\in [0,n-1];\hfill \\ {\tilde{k}}_i^{+n}\ge 0,{\tilde{k}}_i^{-n}\ge 0,{\tilde{k}}_i^{+n}{k}_i^{-n}=0,i\in [0,n-1];\hfill \\ ({\tilde{y}}_i^n-L_i^n){\tilde{k}}_i^{+n}=({\tilde{y}}_i^n-V_i^n){\tilde{k}}_i^{-n}=0,i\in [0,n-1];\hfill \\ {\tilde{y}}_i^n={\xi }_i^n,i\in [n,n^{\delta }];\hfill \\ {\tilde{z}}_i^n=\frac{1}{\sqrt{{\Delta }^n}}{\mathbb{E}}^{{\mathcal{G}}_i^n}\left[{\tilde{y}}_{i+1}^n{ϵ}_{i+1}^n\right],i\in [0,n-1];\hfill \\ {\tilde{u}}_i^{p,n}=\frac{{\mathbb{E}}^{{\mathcal{G}}_i^n}\left[{\tilde{y}}_{i+1}^n\left(h_{i+1}^n-h_i^n-{\Delta }^n(1-h_{i+1}^n){\gamma }_{i+1}\right)\right]}{{\mathbb{E}}^{{\mathcal{G}}_i^n}\left[{(h_{i+1}^n-h_i^n-{\Delta }^n(1-h_{i+1}^n){\gamma }_{i+1})}^2\right]},i\in [0,\left[\frac{\tau }{{\Delta }^n}\right]-1].\hfill \end{array}\right.\end{array}$$

(17)

Similarly to the implicit reflected case, we can obtain

$$\begin{array}{c}\hfill \left\{\begin{array}{c}{\tilde{y}}_i^n={\mathbb{E}}^{{\mathcal{G}}_i^n}\left[{\tilde{y}}_{i+1}^n\right]+f^n(t_i,{\mathbb{E}}^{{\mathcal{G}}_i^n}\left[{\tilde{y}}_{i+1}^n\right],{\overline{\tilde{y}}}_i^n,{\tilde{z}}_i^n,{\tilde{u}}_i^n){\Delta }^n+{\tilde{k}}_i^{+n}-{\tilde{k}}_i^{-n},i\in [0,n-1];\hfill \\ {\tilde{k}}_i^{+n}={\left({\mathbb{E}}^{{\mathcal{G}}_i^n}\left[{\tilde{y}}_{i+1}^n\right]+f^n(t_i,{\mathbb{E}}^{{\mathcal{G}}_i^n}\left[{\tilde{y}}_{i+1}^n\right],{\overline{\tilde{y}}}_i^n,{\tilde{z}}_i^n,{\tilde{u}}_i^n){\Delta }^n-L_i^n\right)}^{-},i\in [0,n-1];\hfill \\ {\tilde{k}}_i^{-n}={\left({\mathbb{E}}^{{\mathcal{G}}_i^n}\left[{\tilde{y}}_{i+1}^n\right]+f^n(t_i,{\mathbb{E}}^{{\mathcal{G}}_i^n}\left[{\tilde{y}}_{i+1}^n\right],{\overline{\tilde{y}}}_i^n,{\tilde{z}}_i^n,{\tilde{u}}_i^n){\Delta }^n-V_i^n\right)}^{+},i\in [0,n-1];\hfill \\ {\tilde{y}}_i^n={\xi }_i^n,i\in [n,n^{\delta }];\hfill \\ {\tilde{z}}_i^n=\frac{1}{\sqrt{{\Delta }^n}}{\mathbb{E}}^{{\mathcal{G}}_i^n}\left[{\tilde{y}}_{i+1}^n{ϵ}_{i+1}^n\right],i\in [0,n-1];\hfill \\ {\tilde{u}}_i^{p,n}=\frac{{\mathbb{E}}^{{\mathcal{G}}_i^n}\left[{\tilde{y}}_{i+1}^n\left(h_{i+1}^n-h_i^n-{\Delta }^n(1-h_{i+1}^n){\gamma }_{i+1}\right)\right]}{{\mathbb{E}}^{{\mathcal{G}}_i^n}\left[{(h_{i+1}^n-h_i^n-{\Delta }^n(1-h_{i+1}^n){\gamma }_{i+1})}^2\right]},i\in [0,\left[\frac{\tau }{{\Delta }^n}\right]-1].\hfill \end{array}\right.\end{array}$$

(18)

For the continuous time version ${({\tilde{Y}}_t^n,{\tilde{Z}}_t^n,{\tilde{U}}_t^n,{\tilde{K}}_t^{+n},{\tilde{K}}_t^{-n})}_{0\le t\le T}$:

$$\begin{array}{c}{\tilde{Y}}_t^n:={\tilde{y}}_{[t/{\Delta }^n]}^n,{\tilde{Z}}_t^n:={\tilde{z}}_{[t/{\Delta }^n]}^n,{\tilde{U}}_t^n:={\tilde{u}}_{[t/{\Delta }^n]}^n,\hfill \\ {\tilde{K}}_t^{+n}:=\sum _{i=0}^{[t/{\Delta }^n]}{\tilde{k}}_i^{+n},{\tilde{K}}_t^{-n}:=\sum _{i=0}^{[t/{\Delta }^n]}{\tilde{k}}_i^{-n}.\hfill \end{array}$$

5. Convergence Results

We first state the convergence result from the Penalized ABSDE (19) to RABSDE (2) in Theorem 1, which is the basis of the following convergence results of the discrete schemes we have studied above. We prove the convergence (Theorem 2) from the implicit discrete penalization scheme (7) to the penalized ABSDE (6) with the help of Lemma 1. Combining with Theorem A2, we can get the convergence (Theorem 3) of the explicit discrete penalization scheme (11). By Theorem A3, Lemma 1 and Theorem 1, we can prove the convergence of the implicit discrete reflected scheme (13). By Theorem A3, Theorem A4 and Lemma A4, the convergence (Theorem 5) of the explicit penalization discrete scheme (16) then follows. The proofs of Theorem 1, Lemma 1, Theorem 2 and Theorem 4 can be seen in Appendix A.

5.1. Convergence of the Penalized ABSDE to RABSDE (2)

Theorem 1.

Suppose that the anticipated process ξ, the generator f satisfy Assumption 3 and Assumption 4, $f(t,y,{\overline{y}}_r,z,u)$ is increasing in $\overline{y}$, the obstacles L and V satisfy Assumption 5. We can consider the following special case of the penalized ABSDE for RABSDE (2):

$$\begin{array}{c}\hfill \left\{\begin{array}{c}-d{Y}_t^p=f^n\left(t,Y_t^p,{\mathbb{E}}^{{\mathcal{G}}_t}\left[Y_{t+\delta }^p\right],Z_t^p,U_t^p\right)dt+d{K}_t^{+p}-d{K}_t^{-p}-Z_t^{p}d{B}_t-U_t^{p}d{M}_t,t\in [0,T];\hfill \\ K_t^{+p}={\int }_0^{t}p{(Y_s^p-L_s)}^{-}ds,t\in [0,T];\hfill \\ K_t^{-p}={\int }_0^{t}p{(Y_s^p-V_s)}^{+}ds,t\in [0,T];\hfill \\ Y_t^p={\xi }_t,t\in [T,T+\delta ].\hfill \end{array}\right.\end{array}$$

(19)

Then we have the limiting process $(Y,Z,U,K^{+},K^{-})$ of $(Y^p,Z^p,U^p,K^{+p},K^{-p})$, i.e., as $p\to \infty$, $Y_t^p\to Y_t$ in ${\mathcal{S}}_{\mathcal{G}}^2(0,T+\delta ;\mathbb{R})$, $Z_t^p\to Z_t$ weakly in ${\mathcal{L}}_{\mathcal{G}}^2(0,T;\mathbb{R})$, $U_t^p\to U_t$ weakly in ${\mathcal{L}}_{\mathcal{G}}^{2,\tau }(0,T;\mathbb{R})$, $K_t^{+p}\left(K_t^{-p}\right)\to K_t^{+}\left(K_t^{-}\right)$ weakly in ${\mathcal{A}}_{\mathcal{G}}^2(0,T;\mathbb{R})$. Moreover, there exists a constant $C_{\xi ,f,L,V}$ depending on ξ, $f(t,0,0,0,0)$, L and V, such that

$$\begin{array}{c}\mathbb{E}[\underset{0\le t\le T}{\sup }|Y_t^p-Y_t{|}^2+{\int }_0^T|Z_t^p-Z_t{|}^{2}dt+{\int }_0^T{|U_t^p-U_t|}^2{1}_{\left\{\tau >t\right\}}{\gamma }_{t}dt\hfill \\ +\underset{0\le t\le T}{\sup }{|(K_t^{+p}-K_t^{+})-(K_t^{-p}-K_t^{-})|}^2]\le \frac{1}{\sqrt{p}}{C}_{\xi ,f,L,V}.\hfill \end{array}$$

5.2. Convergence of the Implicit Discrete Penalization Scheme

We first introduce the following lemma to prove the convergence result of the penalized ABSDE (19) to the implicit penalization scheme.

Lemma 1.

Under Assumption 6 and Assumption 7, $(Y_t^{p,n},Z_t^{p,n},U_t^{p,n})$ converges to $(Y_t^p,Z_t^p,U_t^p)$ in the following sense:

$$\begin{array}{c}\hfill \underset{n\to \infty }{\lim }\mathbb{E}\left[\underset{0\le t\le T}{\sup }|Y_t^{p,n}-Y_t^p{|}^2+{\int }_0^T|Z_t^{p,n}-Z_t^p{|}^{2}dt+{\int }_0^T{|U_t^{p,n}-U_t^p|}^2{1}_{\left\{\tau >t\right\}}{\gamma }_{t}dt\right]=0,\end{array}$$

(20)

for any $t\in [0,T]$, as $n\to \infty$, $K_t^{+p,n}-K_t^{-p,n}\to K_t^{+p}-K_t^{-p}$ in ${\mathcal{L}}_{\mathcal{G}}^2(0,T;\mathbb{R})$.

Theorem 2.

(Convergence of the implicit discrete penalization scheme) Under Assumption 3 and Assumption 7, $(Y_t^{p,n},Z_t^{p,n},U_t^{p,n})$ converges to $(Y_t,Z_t,U_t)$ in the following sense:

$$\begin{array}{c}\hfill \underset{p\to \infty }{\lim }\underset{n\to \infty }{\lim }\mathbb{E}\left[\underset{0\le t\le T}{\sup }|Y_t^{p,n}-Y_t{|}^2+{\int }_0^T|Z_t^{p,n}-Z_t{|}^{2}dt+{\int }_0^T{|U_t^{p,n}-U_t|}^2{1}_{\left\{\tau >t\right\}}{\gamma }_{t}dt\right]=0,\end{array}$$

(21)

for any $t\in [0,T]$, as $p\to \infty$, $n\to \infty$, $K_t^{+p,n}-K_t^{-p,n}\to K_t^{+}-K_t^{-}$ in ${\mathcal{L}}_{\mathcal{G}}^2(0,T;\mathbb{R})$.

5.3. Convergence of the Explicit Discrete Penalization Scheme

By Theorem 2 and Theorem A2, we can obtain the following convergence result of the explicit penalization discrete scheme.

Theorem 3.

(Convergence of the explicit discrete penalization scheme) Under Assumption 3 and Assumption 7, $({\tilde{Y}}_t^{p,n},{\tilde{Z}}_t^{p,n},{\tilde{U}}_t^{p,n})$ converges to $(Y_t,Z_t,U_t)$ in the following sense:

$$\begin{array}{c}\hfill \underset{n\to \infty }{\lim }\mathbb{E}\left[\underset{0\le t\le T}{\sup }|{\tilde{Y}}_t^{p,n}-Y_t{|}^2+{\int }_0^T|{\tilde{Z}}_t^{p,n}-Z_t{|}^{2}dt+{\int }_0^T{|{\tilde{U}}_t^{p,n}-U_t|}^2{1}_{\left\{\tau >t\right\}}{\gamma }_{t}dt\right]=0,\end{array}$$

(22)

for any $t\in [0,T]$, as $n\to \infty$, ${\tilde{K}}_t^{+p,n}-{\tilde{K}}_t^{-p,n}\to K_t^{+}-K_t^{-}$ in ${\mathcal{L}}_{\mathcal{G}}^2(0,T;\mathbb{R})$.

5.4. Convergence of the Implicit Discrete Reflected Scheme

Theorem 4.

(Convergence of the implicit discrete reflected scheme) Under Assumption 7 and Assumption 3, $(Y_t^n,Z_t^n,U_t^n)$ converges to $(Y_t,Z_t,U_t)$ in the following sense:

$$\begin{array}{c}\hfill \underset{n\to \infty }{\lim }\mathbb{E}\left[\underset{0\le t\le T}{\sup }|Y_t^n-Y_t{|}^2+{\int }_0^T|Z_t^n-Z_t{|}^{2}dt+{\int }_0^T{|U_t^n-U_t|}^2{1}_{\left\{\tau >t\right\}}{\gamma }_{t}dt\right]=0,\end{array}$$

(23)

and for any $t\in [0,T]$, as $n\to \infty$, $K_t^{+n}-K_t^{-n}\to K_t^{+}-K_t^{-}$ in ${\mathcal{L}}_{\mathcal{G}}^2(0,T;\mathbb{R})$.

5.5. Convergence of the Explicit Discrete Reflected Scheme

By Theorem A3, Theorem A4 and Lemma A4, we can get the convergence result of the explicit penalization discrete scheme.

Theorem 5.

(Convergence of the explicit discrete reflected scheme) Under Assumption 3 and Assumption 7, $({\tilde{Y}}_t^n,{\tilde{Z}}_t^n,{\tilde{U}}_t^n)$ converges to $(Y_t,Z_t,U_t)$ in the following sense:

$$\begin{array}{c}\hfill \underset{n\to \infty }{\lim }\mathbb{E}\left[\underset{0\le t\le T}{\sup }|{\tilde{Y}}_t^n-Y_t{|}^2+{\int }_0^T|{\tilde{Z}}_t^n-Z_t{|}^{2}dt+{\int }_0^T{|{\tilde{U}}_t^n-U_t|}^2{1}_{\left\{\tau >t\right\}}{\gamma }_{t}dt\right]=0,\end{array}$$

(24)

for any $t\in [0,T]$, as $n\to \infty$, ${\tilde{K}}_t^{+n}-{\tilde{K}}_t^{-n}\to K_t^{+}-K_t^{-}$ in ${\mathcal{L}}_{\mathcal{G}}^2(0,T;\mathbb{R})$.

6. Numerical Calculations and Simulations

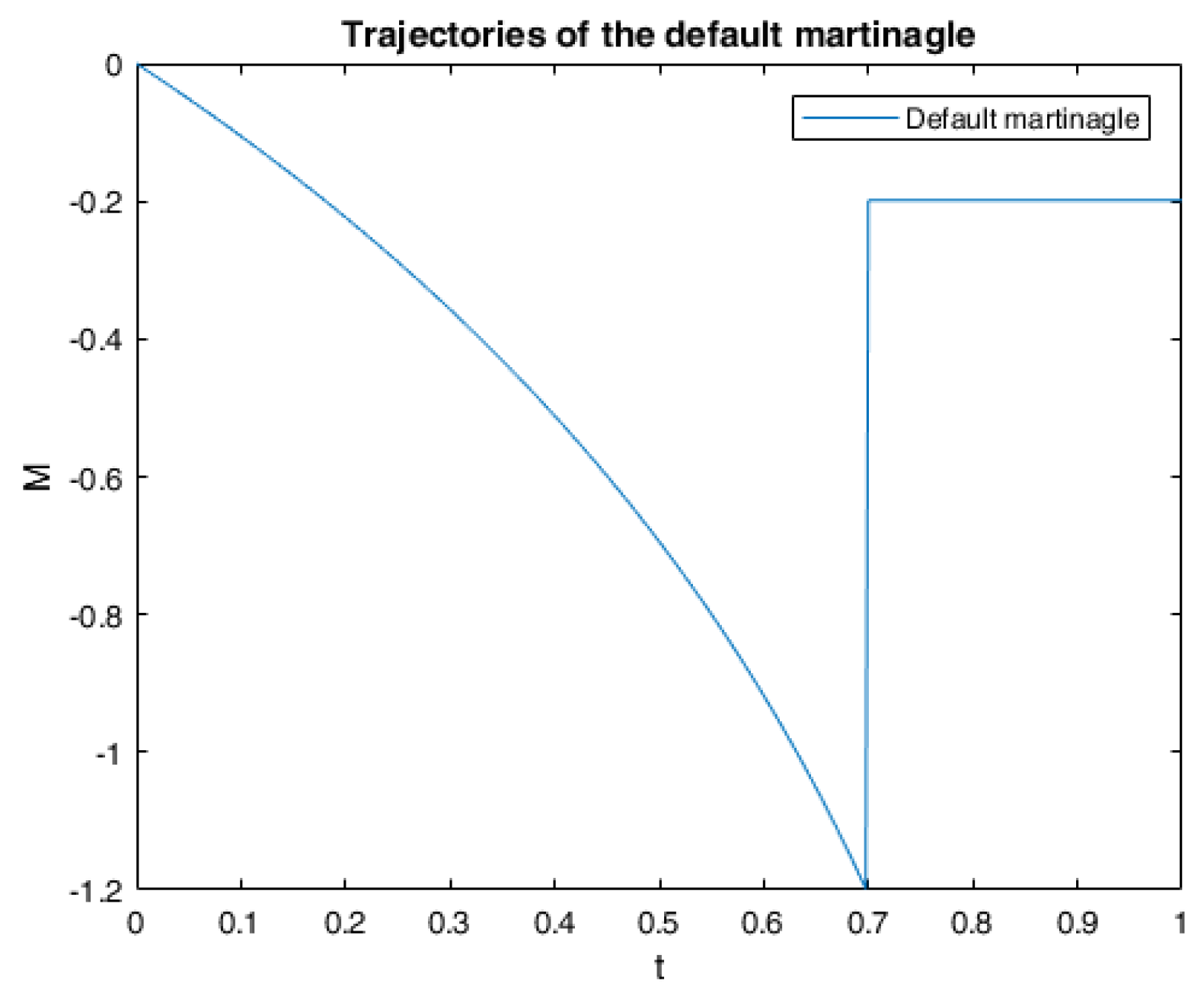
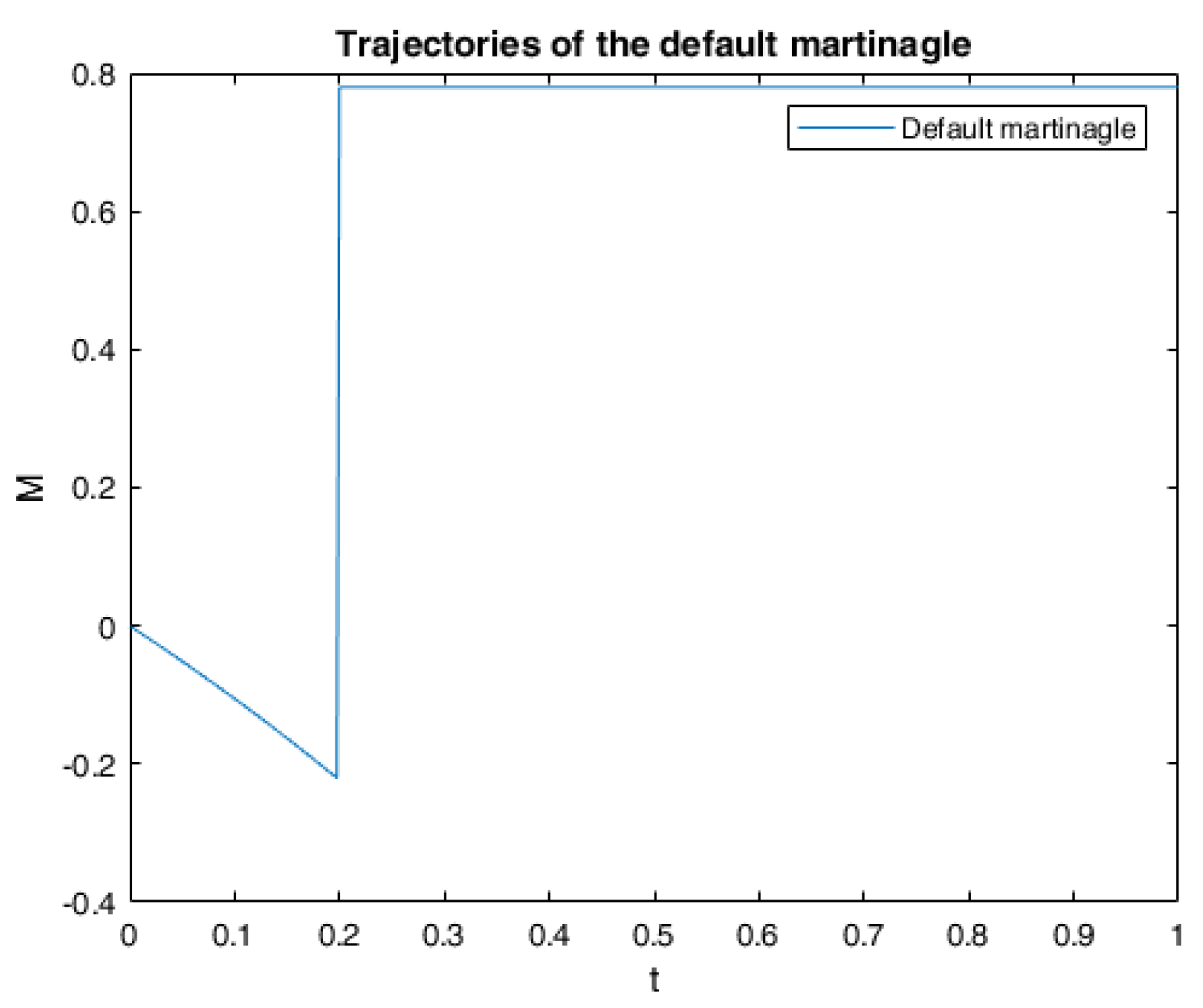
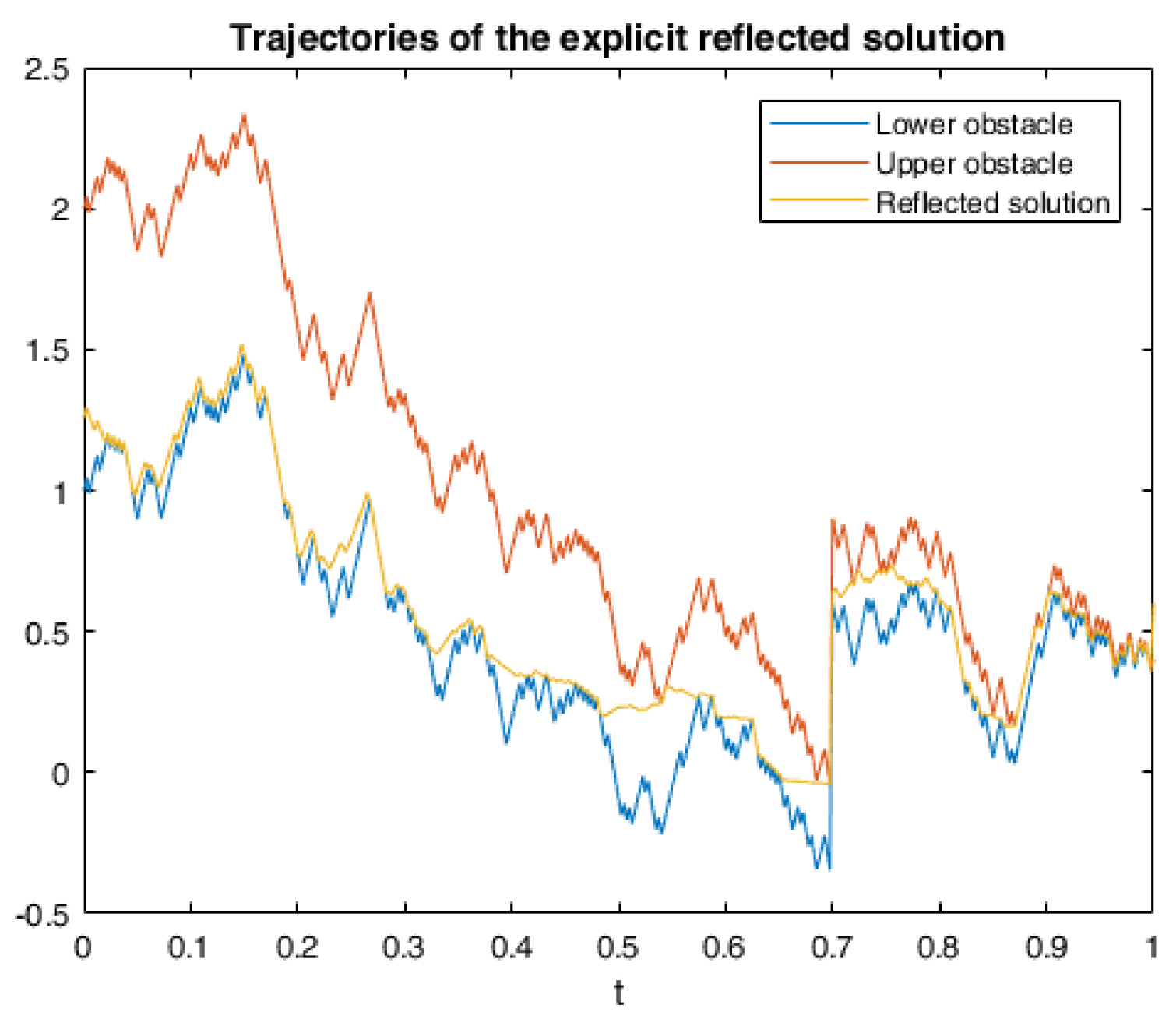
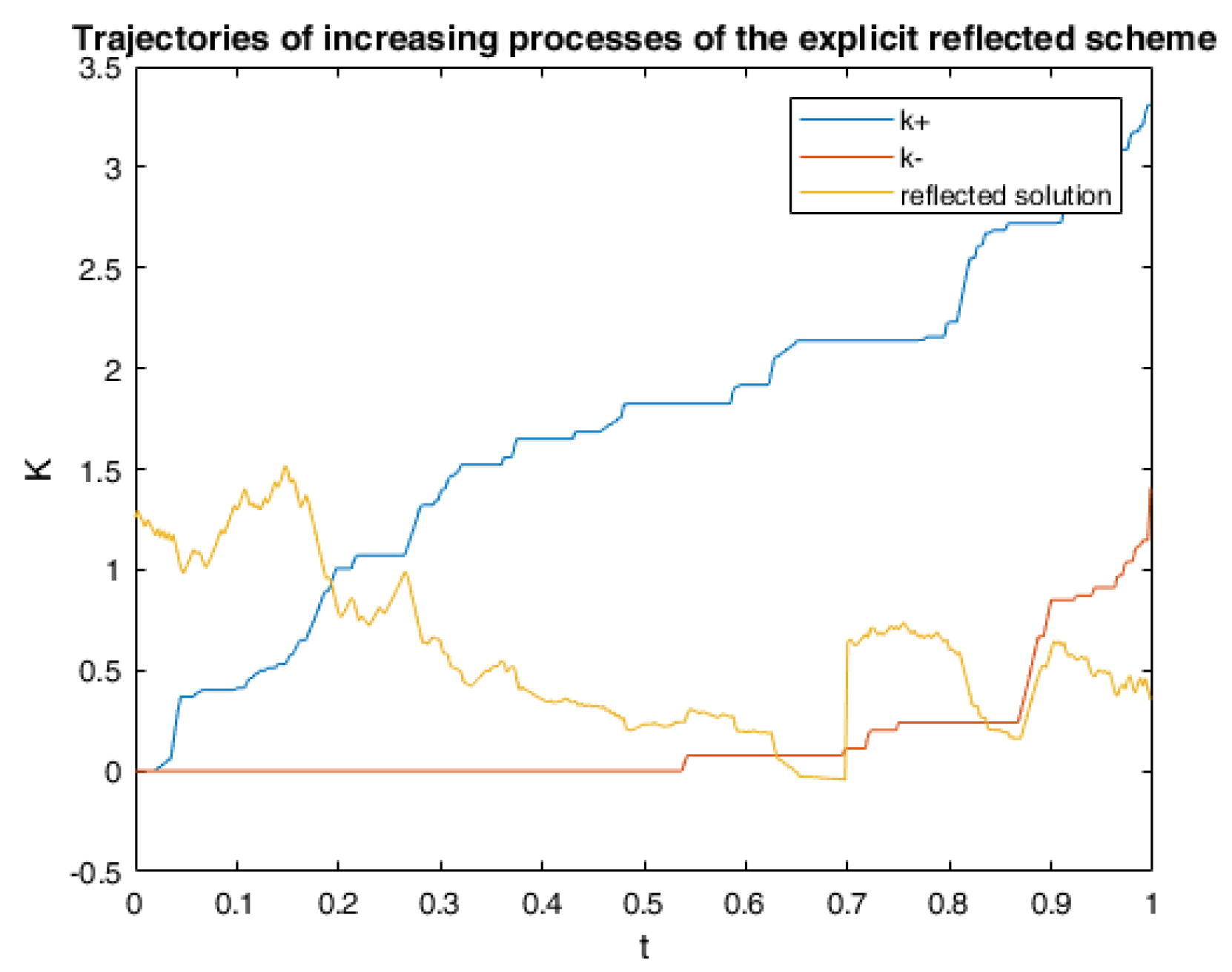
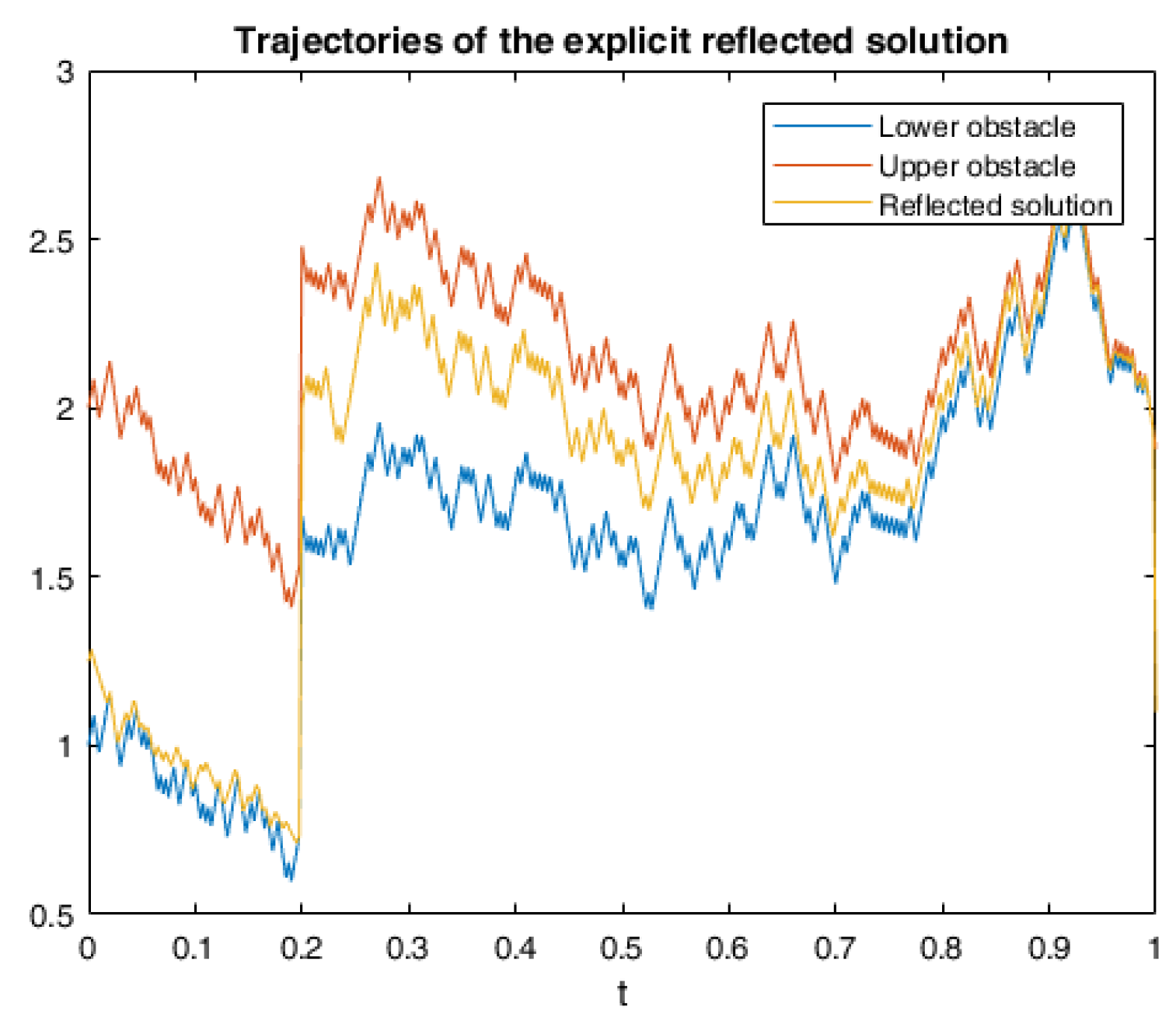
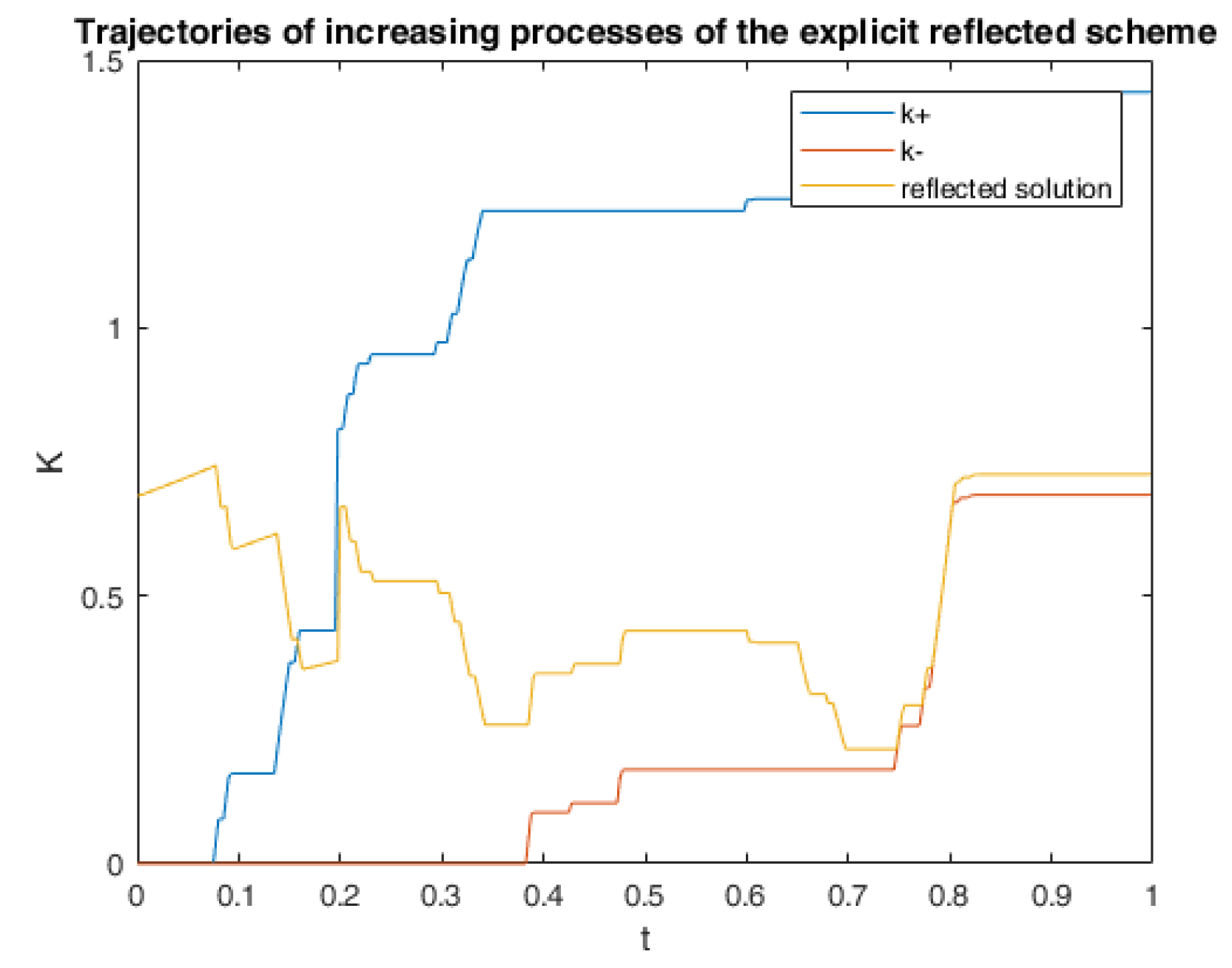
6.1. One Example of RABSDE with Two Obstacles and Default Risk

For the convenience of computation, we consider the case when the terminal time $T=1$, the calculation begins from $y_n^n={\xi }^n$, and proceeds backward to solve $(y_i^n,z_i^n,u_i^n,k_i^{+n},k_i^{-n})$ for $i=n-1,n-2,\dots ,1,0$. We use Matlab for the simulation. We consider a simple situation: the terminal value ${\xi }_T=\Phi (B_T,M_T)$ and anticipated process ${\xi }_t=\Phi \left(B_t\right)$ ($t\in (T,T+\delta ]$); the obstacles $L_t={\Psi }_1(t,B_t,M_t)$ and $V_t={\Psi }_2(t,B_t,M_t)$, where $\Phi$, ${\Psi }_2$ and ${\Psi }_3$ are real analytic functions defined on $\mathbb{R}$, $[0,T]\times \mathbb{R}$ and $[0,T]\times \mathbb{R}$ respectively. We take the following example ($n=200$, anticipated time $\delta =0.3$):

$$\begin{array}{c}f(t,y,\overline{y},z,u)=\left|\frac{y}{2}+\frac{\overline{y}}{2}\right|+z+u,t\in [0,T];\hfill \\ \Phi \left(B_t\right)=|B_t|+M_T,t\in [T,T+\delta ];\hfill \\ {\Psi }_1(t,B_t,M_t)=|B_t|+M_t+T-t,t\in [0,T];\hfill \\ {\Psi }_2(t,B_t,M_t)=|B_t|+M_t+2\left(T-t\right),t\in [0,T];\hfill \end{array}$$

This example satisfies the Assumption 3, Assumption 4 and Assumption 5 in the theoretical Section 1.3. We choose the default time $\tau$ as a uniformly distributed random variable.

As the inverse for both implicit schemes in (10) and (15) is not easy to get directly, we only use explicit schemes below. We are going to illustrate the behaviors of the explicit reflected scheme by looking at the pathwise behavior for $n=400$. Further, we will compare the explicit reflected scheme with the explicit penalization scheme for different values of the penalization parameter.

Figure 1 represents one path of the Brownian motion, Figure 2 and Figure 3 represent one path of the Brownian motion and one path of the default martingale when the default time $\tau =0.7$ and $0.2$ respectively.

Figure 4 and Figure 5 represent the paths of the solution ${\tilde{y}}^n$, increasing processes ${\tilde{K}}^{+n}$ and ${\tilde{K}}^{-n}$ in the explicit reflected scheme where the random default time $\tau =0.7$. We can see that for all i, ${\tilde{y}}_i^n$ stays between the lower obstacle $L_i^n$ and the upper obstacle $V_i^n$, the increasing process ${\tilde{K}}_i^{+n}$ (resp. ${\tilde{K}}_i^{-n}$) pushes ${\tilde{y}}_i^n$ upward (resp. downward), and they can not increase at the same time. In this example for $n=400$, default time $\tau =0.7$, we can get the reflected solution ${\tilde{y}}_0^n=1.2563$ from the explicit reflected scheme.

Figure 4 and Figure 6 illustrate the influence of the jump on the solution ${\tilde{y}}^n$ at the different random default times, the reflected solution ${\tilde{y}}^n$ moves downwards after the default time (which can not be shown in Figure 7). From the approximation of the default martingale (5), $M^n$ is larger with a larger default time.

Table 1. The values of the penalization solution ${\tilde{y}}_0^{p,n}$ ($\tau =0.7$).

${\tilde{\mathit{y}}}_0^{\mathit{p},\mathit{n}}$	$\mathit{p}={10}^3$	$\mathit{p}={10}^4$	$\mathit{p}={10}^5$	$\mathit{p}={10}^6$
$n=200$	$1.2369$	$1.2394$	$1.2428$	$1.2452$
$n=400$	$1.2458$	$1.2482$	$1.2496$	$1.2511$
$n=1000$	$1.2343$	$1.2497$	$1.2527$	$1.2630$

and contains the comparison between the explicit reflected scheme and the explicit penalization scheme by the values of ${\tilde{y}}_0^n$ and ${\tilde{y}}_0^{p,n}$ with respect to the parameters n and p. As n increases, the reflected solution ${\tilde{y}}_0^n$ increases because of the choice of the coefficient. For fixed n, as the penalization parameter p increases, the penalization solution ${\tilde{y}}_0^{p,n}$ converges increasingly to the reflected solution ${\tilde{y}}_0^n$, which is obvious from the comparison theorem of BSDE with default risk. If p and n have a smaller difference (when $n={10}^3$, $p={10}^3$), the penalization solution ${\tilde{y}}_0^{p,n}$ is far from the reflected solution ${\tilde{y}}_0^n$. Hence, the penalization parameter p should be chosen as large as possible.

Table 2. The values of the reflected solution ${\tilde{y}}_0^n$ ($\tau =0.7$) and ${\tilde{y}}_0^{n\ast }$.

	${\tilde{\mathit{y}}}_0^{\mathit{n}}$	${\tilde{\mathit{y}}}_0^{\mathit{n}\ast }$
$n=200$	$1.2469$	$1.5451$
$n=400$	$1.2563$	$1.5507$
$n=1000$	$1.2644$	$1.5614$

illustrates the comparison between the reflected solution ${\tilde{y}}_0^n$ and ${\tilde{y}}_0^{n\ast }$. Figure 7 represents the situation without the default risk, the reflected solution ${\tilde{y}}_0^{n\ast }$ has a larger value than in the situation when the default case happens (Figure 4).

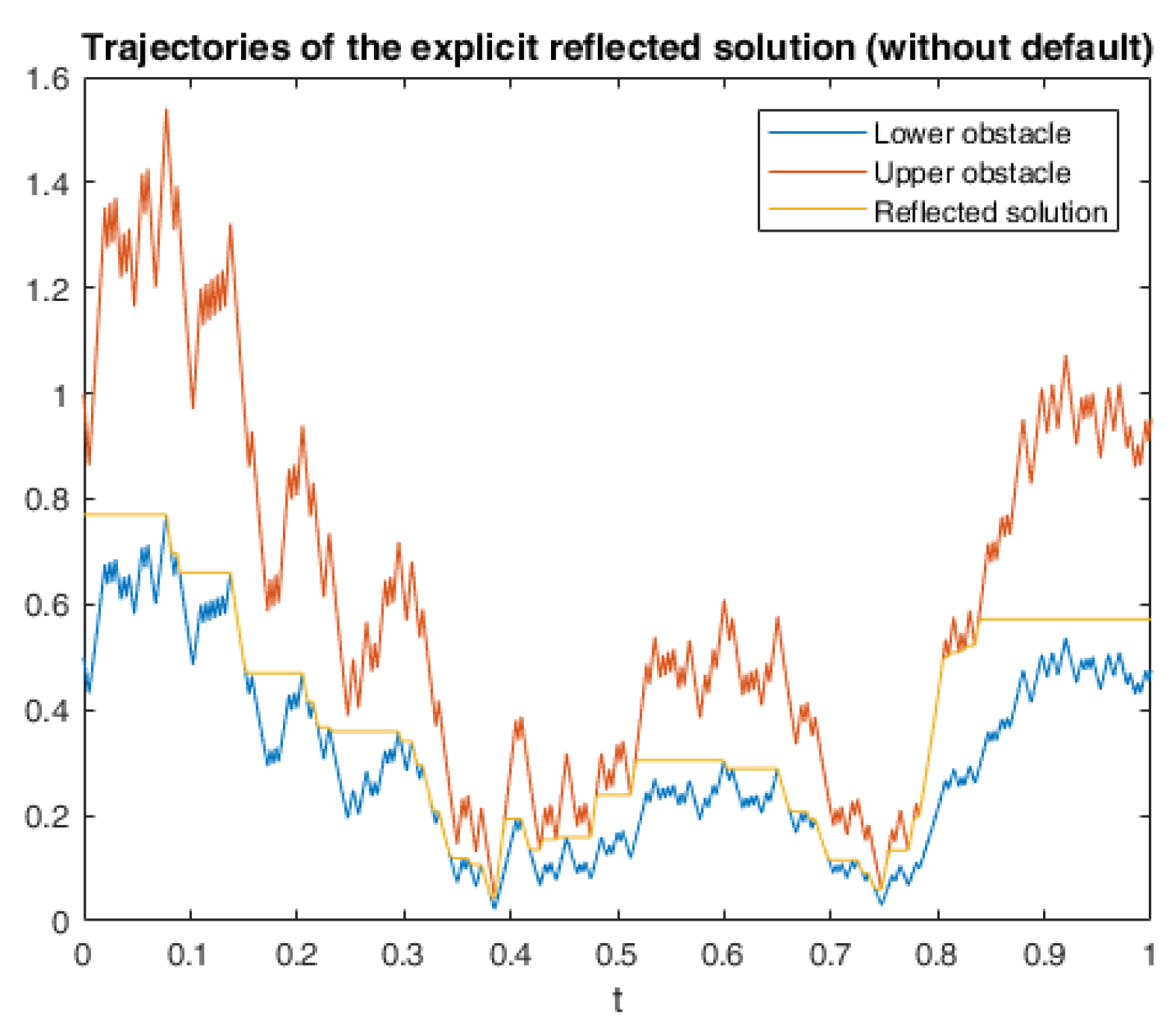
6.2. Application in American Game Options in a Defaultable Setting

6.2.1. Model Description

Hamadene (2006) studied the relation between American game options and RBSDE with two obstacles driven by Brownian motion. In our paper, we consider the case with default risk. An American game option contract with maturity T involves a broker $c_1$ and a trader $c_2$:

• The broker $c_1$ has the right to cancel the contract at any time before the maturity T, while the trader $c_2$ has the right to early exercise the option;
• the trader $c_2$ pays an initial amount (the price of this option) which ensures an income $L_{{\tau }_1}$ from the broker $c_1$, where ${\tau }_1\in [0,T]$ is an $\mathcal{G}$-stopping time;
• the broker has the right to cancel the contract before T and needs to pay $V_{{\tau }_2}$ to $c_2$. Here, the payment amount of the broker $c_1$ should be greater than his payment to the trader $c_2$ (if trader decides for early exercise), i.e., $V_{{\tau }_2}\ge L_{{\tau }_2}$, $V_{{\tau }_2}-L_{{\tau }_2}$ is the premium that the broker $c_1$ pays for his decision of early cancellation. ${\tau }_2\in [0,T]$ is an $\mathcal{G}$-stopping time;
• if $c_1$ and $c_2$ both decide to stop the contract at the same time $\tau$, then the trader $c_2$ gets an income equal to $Q_{\tau }{1}_{\{\tau <T\}}+\xi 1_{\{\tau =T\}}$.

6.2.2. The Hedge for the Broker

Consider a financial market $\mathcal{M}$, we have a riskless asset $C_t\in \mathbb{R}$ with risk-free rate r:

$$\begin{array}{c}\hfill \left\{\begin{array}{cc}d{C}_t\hfill & =r{C}_{t}dt,t\in (0,T],\hfill \\ C_0\hfill & =c_0,t=0;\hfill \end{array}\right.\end{array}$$

(25)

one risky asset $S_t\in \mathbb{R}$:

$$\begin{array}{c}\hfill \left\{\begin{array}{cc}d{S}_t\hfill & =S_t\left(\mu dt+\sigma d{B}_t+\chi d{M}_t\right),t\in (0,T],\hfill \\ S_0\hfill & =s_0,t=0,\hfill \end{array}\right.\end{array}$$

(26)

where $B_t$ is a 1-dimensional Brownian Motion, $\mu$ is the expected return, $\sigma$ is the volatility, $\chi$ is the parameter related to the default risk.

Consider a self-financing portfolio $\pi \in {\mathbb{R}}^d$ with strategy $\pi ={\left({\beta }_s^{\left(1\right)},{\beta }_s^{\left(2\right)}\right)}_{s\in [t,T]}$ trading on C and S respectively on the time interval $[t,T]$. $A^{\pi ,\alpha }$ is the wealth process with the value ${\alpha }^A$ at time t, here is a non-negative ${\mathcal{F}}_t$-measurable random variable.

$$\begin{array}{cc}\hfill & A_s^{\pi ,\alpha }={\beta }_s^{\left(1\right)}{C}_s+{\beta }_s^{\left(2\right)}{S}_s,s\in [t,T];\hfill \\ \hfill & A_s^{\pi ,\alpha }={\alpha }^A+{\int }_t^s{\beta }_u^{\left(1\right)}d{C}_u+{\int }_t^s{\beta }_u^{\left(2\right)}d{S}_u,s\in [t,T];\hfill \\ \hfill & {\int }_t^T\left[|{\beta }_u^{\left(1\right)}|+{\left({\beta }_u^{\left(2\right)}{S}_u\right)}^2\right]du<\infty ;\hfill \end{array}$$

(27)

Let $L^{\pi ,\alpha }$ be a positive local martingale with the following form:

$$\begin{array}{c}\hfill \left\{\begin{array}{cc}d{L}_t^{\pi ,\alpha }\hfill & =-L_{t-}^{\pi ,\alpha }{\sigma }^{-1}(\mu -r)d{B}_t,t\in (0,T],\hfill \\ L_0^{\pi ,\alpha }\hfill & =1,t=0,\hfill \end{array}\right.\end{array}$$

By Girsanov’s theorem, let ${\mathbb{P}}^{\pi ,\alpha }$ be the equivalent measure of $\mathbb{P}$:

$$\frac{{\mathbb{P}}^{\pi ,\alpha }}{\mathbb{P}}|{\mathcal{G}}_T=L_T^{\pi ,\alpha }=exp\left\{-{\sigma }^{-1}(\mu -r)B_T-\frac{1}{2}{\left(-{\sigma }^{-1}(\mu -r)\right)}^{2}T\right\},$$

here let ${\mathbb{E}}^{\pi ,\alpha }$ be the expectation, $B^{\pi ,\alpha }$ and $M^{\pi ,\alpha }$ be the Brownian motion and the default martingale under the measure ${\mathbb{P}}^{\pi ,\alpha }$:

$$\begin{array}{cc}\hfill B_t^{\pi ,\alpha }:=& B_t+{\sigma }^{-1}(\mu -r)t;\hfill \\ \hfill M_t^{\pi ,\alpha }:=& M_t.\hfill \end{array}$$

Hence, the risky asset $S_t$ defined in (26) can be converted into the following form under measure ${\mathbb{P}}^{\pi ,\alpha }$:

$$\begin{array}{c}\hfill \left\{\begin{array}{cc}d{S}_t\hfill & =S_t\left(rdt+\sigma d{B}_t^{\pi ,\alpha }+\chi d{M}_t^{\pi ,\alpha }\right),t\in (0,T],\hfill \\ S_0\hfill & =s_0,t=0.\hfill \end{array}\right.\end{array}$$

(28)

Denote by $(\pi ,\theta )$ a hedge for the broker against the American game option after t, where $\pi$ is defined in (27), $\theta \in [t,T]$ is a stopping time, satisfying

$$A_s^{\pi ,\alpha }\ge R(s,\theta ):=V_{\theta }{1}_{\{\theta <s\}}+L_s{1}_{\{s<\theta \}}+Q_s{1}_{\{\theta =s<T\}}+\xi 1_{\{\theta =s=T\}},s\in [t,T],\mathbb{P}-a.s.$$

(29)

here $R(s,\theta )$ is the amount that the broker $c_1$ has to pay if the option is exercised by $c_2$ at s or canceled at time $\theta$. Similarly to El Karoui et al. (1997b) and Karatzas and Shreve (1998), we define the value of the option at time t by $J_t$, where ${\left(J_t\right)}_{0\le t\le T}$ is an rcll (right continuous with left limits) process, for any $t\in [0,T]$,

$$\begin{array}{cc}\hfill J_t:=ess\inf \{{\alpha }^A\ge 0;& {\mathcal{G}}_t-\mathrm{measurable}\mathrm{such}\mathrm{that}\mathrm{there}\mathrm{exists}\mathrm{a}\mathrm{hedge}(\pi ,\theta )\mathrm{after}t,\hfill \\ \hfill & \mathrm{where}\pi \mathrm{is}\mathrm{a}\mathrm{self}-\mathrm{financing}\mathrm{portfolio}\mathrm{after}t\mathrm{whose}\mathrm{value}\mathrm{at}t\mathrm{is}{\alpha }^A.\}\hfill \end{array}$$

(30)

Consider the following RBSDE with two obstacles and default risk, for any $t\in [0,T]$, there exist a stopping time ${\theta }_t$, a process ${\left(Z_s^{\pi ,\alpha }\right)}_{t\le s\le T}$ and increasing processes ${\left(K_s^{\pi ,\alpha +}\right)}_{t\le s\le T}$ and ${\left(K_s^{\pi ,\alpha -}\right)}_{t\le s\le T}$, such that

$$\begin{array}{c}\hfill \left\{\begin{array}{c}{Y}_t^{\pi ,\alpha }=Y_{{\theta }_t}^{\pi ,\alpha }+\left(K_{{\theta }_t}^{\pi ,\alpha ,+}-K_s^{\pi ,\alpha ,+}\right)-\left(K_{{\theta }_t}^{\pi ,\alpha -}-K_s^{\pi ,\alpha -}\right)-{\int }_s^{{\theta }_t}{Z}_u^{\pi ,\alpha }d{B}_u^{\pi ,\alpha }\hfill \\ -{\int }_s^{{\theta }_t}{U}_u^{\pi ,\alpha }d{M}_u^{\pi ,\alpha },s\in [t,{\theta }_t];\hfill \\ Y_T^{\pi ,\alpha }=e^{-rT}\xi ;\hfill \\ e^{-rs}{L}_s\le Y_s^{\pi ,\alpha }\le e^{-rs}{V}_s,s\in [t,T];\hfill \\ {\int }_t^{{\theta }_t}(Y_u^{\pi ,\alpha }-e^{-ru}{L}_u)d{K}_u^{\pi ,\alpha +}={\int }_t^{{\theta }_t}(e^{-ru}{V}_u-Y_u^{\pi ,\alpha })d{K}_u^{\pi ,\alpha -}=0;\hfill \end{array}\right.\end{array}$$

(31)

For any $s\in [t,T]$, $e^{rt}{Y}_t^{\pi ,\alpha }$ is a hedge for the broker $c_1$ against the game option, i.e., $J_t=e^{rt}{Y}_t^{\pi ,\alpha }$ (see Theorem A5 in the Appendix). Similarly to Proposition 4.3 in Hamadene (2006), we set

$$\begin{array}{c}\hfill {\theta }_t^{\ast }:=\inf \left\{s\ge t;Y_t^{\pi ,\alpha }=e^{-rs}{V}_s\right\}\wedge T=\inf \left\{s\ge t;K_s^{\pi ,\alpha -}>0\right\};\\ \hfill v_t^{\ast }:=\inf \left\{s\ge t;Y_t^{\pi ,\alpha }=e^{-rs}{L}_s\right\}\wedge T=\inf \left\{s\ge t;K_s^{\pi ,\alpha +}>0\right\},\end{array}$$

(32)

therefore, we can get

$$\begin{array}{c}\hfill {\widehat{R}}_t(v,{\theta }_t^{\ast })\le Y_t^{\pi ,\alpha }={\widehat{R}}_t(v_t^{\ast },{\theta }_t^{\ast })\le {\widehat{R}}_t(v_t^{\ast },\theta ),\end{array}$$

where

$${\widehat{R}}_t(v,\theta ):={\mathbb{E}}^{\pi ,\alpha }\left[e^{-r\theta }{V}_{\theta }{1}_{\{\theta <v\}}+e^{-rv}{L}_v{1}_{\{v<\theta \}}+e^{-r\theta }{Q}_{\theta }{1}_{\{\theta =v<T\}}+e^{-rT}\xi 1_{\{\theta =v=T\}}|{\mathcal{G}}_t\right],\mathbb{P}-a.s.$$

6.2.3. Numerical Simulation

We use the same calculation method as in Section 6.1, starting from $Y_n^{\pi ,\alpha }=\xi$, and proceeding backward to solve $(Y_i^{\pi ,\alpha },Z_i^{\pi ,\alpha },U_i^{\pi ,\alpha },K_i^{\pi ,\alpha +},K_i^{\pi ,\alpha -})$ for $i=n-1,\dots ,1,0$ with step size ${\Delta }^n$. The forward SDEs (25) and (26) can be numerically approximated by the Euler scheme on the time grid ${\left(t_i\right)}_{i=0,1,\dots n}$:

$$\begin{array}{cc}\hfill C_{i+1}=& C_i+r{C}_i{\Delta }^n;\hfill \\ \hfill S_{i+1}=& S_i+S_i\left(\mu {\Delta }^n+\sigma \Delta B_i^n+\chi \Delta M_i^n\right).\hfill \end{array}$$

In this case, we consider parameters as below:

$$\begin{array}{cc}\hfill s_0=& 1.5,T=1,r=1.1,\mu =1.5,\sigma =0.5,\chi =0.2,\hfill \\ \hfill L_t=& {\left(S_t-1\right)}^{+},V_t=2{\left(S_t-1\right)}^{+},\xi =1.2{\left(S_T-1\right)}^{+},\hfill \end{array}$$

In the case $n=400$, Figure 8 represents one path of the Brownian motion, Figure 9 and Figure 10 represent the paths of the solution $Y^{\pi ,\alpha }$, increasing processes $K^{\pi ,\alpha -}$ and $K^{\pi ,\alpha -}$ in the explicit reflected scheme where the random default time $\tau =0.2$. We can see that $Y_t^{\pi ,\alpha }$ stays between the lower obstacle $e^{-rt}{L}_t$ and the upper obstacle $e^{-rt}{V}_t$. In this example for $n=400$, default time $\tau =0.2$, we can get the solution $Y_0^{\pi ,\alpha }=0.6857$ from the explicit reflected scheme, i.e., the hedge for the broker $c_1$ against the game option at $t=0$ in the defaultable model. In the case without the default risk, $Y_0^{\pi ,\alpha }=0.7704$, which means the occurrence of the default event could reduce the value of $Y^{\pi ,\alpha }$. Figure 11 represents the situation without the default risk, the solution $Y^{\pi ,\alpha }$ has a larger value than in the situation when the default case happens (Figure 9).