1. Introduction
The 1988 Basel accord ties bank capitalization to portfolio risk by introducing the Capital Adequacy Requirement (CAR). Subsequently, Basel II obliges banks to maintain sufficient loss-absorbing capital on an annual basis. However, several studies on the 2008 financial crisis, such as
Flannery (
2014) and
Duffie (
2010) reveal that, in practice, regulators are unable to force banks to maintain adequate loss-absorbing capital. To alleviate banks under-capitalization,
Flannery (
2005) introduced Contingent Convertibles, hereafter referred to as CoCo in accordance with most of the main-related studies. CoCo is a hybrid debt with a specific clause stipulating that the issuer would either convert it to equity or write down its face value if the level of loss-absorbing capital falls below a certain threshold. This is supposed to help with a firm’s recapitalization under distress, while equity holders would be reluctant to raise capital voluntarily by issuing new shares. Basel III recommends large financial institutions to issue CoCo as a part of their capital adequacy requirements (CAR).
CoCo carries two major risks: the risk of default, which threatens any type of debt instrument, plus the exclusive risk of mandatory conversion. CoCo differs greatly from a traditional convertible bond, which the conversion is optional and generally rewards the bondholder; hence, it is not a risk. Mandatory conversion of CoCo is a punishing mechanism which decreases the value of the bondholder in most scenarios; hence, it is a risk factor.
Most literature employs the structural approach to model CoCo dynamics. Studies generally define a trigger threshold as the barrier, then calculate the conditional probability of hitting the barrier. What makes this group of studies different is the choice of underlying instrument that triggers the conversion and the dynamics of the underlying trigger. The trigger is the book-equity-to-book-asset ratio in
Glasserman and Nouri (
2012), where they model the book asset process using a geometric Brownian motion (GBM). Conversion occurs if the book-equity-to-book-asset ratio exceeds a predetermined exogenous ratio.
Chen et al. (
2013) use the same underlying instrument while they model the book asset process using a jump-diffusion. Conversion is triggered if the asset value goes over a predetermined exogenous threshold. In
Brigo et al. (
2015), firm asset value is a GBM process and the conversion barrier is a linear function of the asset-to-equity ratio.
De Spiegeleer et al. (
2017), in a more recent study, come closer to the Basel III accord and define the implied Common Equity Tier 1 (CET1) volatility as the conversion trigger while modelling the CET1 capital ratio as a GBM. Structural modelling based on a GBM makes the conversion time predictable, while being counterfactual. Uncertainty with respect to the conversion is due not only to the use of accounting capital ratio as the conversion trigger, but also to the stipulations in Basel III that allow regulators to choose the conversion time at their discretion. Studies such as
Albul et al. (
2013) and
Hilscher and Raviv (
2014) model the CoCo as a contingent claim on the value of the bank’s assets. The results are relatively tractable models in which the bank’s incentive to issue CoCo voluntarily could be examined. Once again, the main challenge of these approaches is in triggering the conversion by the unobservable market value of assets.
In
Cheridito and Xu (
2015), the CoCo price is modelled using a pure reduced form approach where the conversion and default events are modelled with a time-changed Poisson process. However, the reduced form approach is less intuitive by ignoring the link between the capital ratio and the trigger event (
Brigo et al. 2015).
Chung and Kwok (
2016) use a structural approach to model the conversion when the capital ratio falls below a certain threshold and also use a Poisson process to model the potential unexpected conversion imposed by the regulator.
Conversion price is also a matter of debate in the literature. As a basic design,
Flannery (
2005) proposes that the number of shares received by CoCo holders at conversion is determined by the face value of the CoCo bonds divided by the market price of stock on the day of conversion. However, this basic conversion mechanism gives an opportunity to short sellers to bid down the share price, force conversion and dilute the market by increasing the number of outstanding shares. To avoid share price manipulation,
Duffie (
2010) argues that the number of shares should be based on a multi-day average of closing prices. Other studies such as
Flannery (
2016) and
Pennacchi (
2010) propose converting the CoCo into a predetermined number of shares at a fixed price. However, there is a high risk that CoCo investors will suffer some value losses upon conversion due to a jump in the market price of shares.
Regulators insist that the CoCo conversion trigger should be based on the accounting capital ratio. Indeed, this is determined by the Basel Committee on Banking Supervision (BCBS) at a global level in Basel III, the European Banking Authority through the Capital Requirements Directive IV/Capital Requirements Regulation (CRD IV/CRR) and the Office of the Superintendent of Financial Institutions (OSFI) in Canada through the Capital Adequact Requirements (CAR) guidelines. The Common Equity Tiers 1 (CET1) should not fall bellow a certain percent of risk-weighted asset (RWA). Although accounting measures are not forward looking and can be manipulated by managers, they ensure that CoCo conversion occurs when a firm encounters serious financial problems.
Glasserman and Nouri (
2012) and
Chen et al. (
2013) choose asset book value as the trigger through the book-equity-to-book-asset ratio, claiming that the book asset value truly approximates the market asset value. Many studies are against employing book value as a basis of conversion because it generates a delayed signal of financial distress.
McDonald (
2013) and
Bolton and Samama (
2012) assume that the market price of equity can measure a bank’s loss-absorbing capacity. They propose a CoCo design in which the share price functions as the conversion trigger.
Sundaresan and Wang (
2015) point out two shortcomings of employing the market price of shares as the conversion trigger within their framework, both of which are linked to the fact that CoCo conversion generates a value exchange between CoCo holders and initial equity holders. First, if the value is transferred from shareholders to CoCo investors at conversion, there can be more than one rational expected equilibrium price for both the stocks and CoCos. Second, if the value is transferred to shareholders, the model sometimes lacks an equilibrium share price.
Sundaresan and Wang (
2015) conclude that a unique competitive equilibrium exists if the conversion does not induce a value transfer.
Glasserman and Nouri (
2012) maintain that this multiple equilibrium problem is a feature of discrete-time models and can be alleviated in a continuous time framework.
Closer to our paper,
Chen et al. (
2017) study the design and incentive effects of contingent convertible debt on optimal capital structure. The asset value is modelled as a jump diffusion, default and conversion occur whenever the firm value triggers some critical value. The default threshold is determined by the equity holder in such a way to maximize the equity value. They conclude that equity holders often have a positive incentive of issuing CoCos because the presence of CoCos reduces the bankruptcy costs when the conversion trigger is large enough.
In this paper, we also study the impact of having a contingent debt instrument in the debt structure in the perspective of equity holders. Based on a discrete time dynamic optimization approach, we reach similar conclusions as
Chen et al. (
2017). Indeed, our framework differs from the latter in various ways. First, the endogenous floating coupon rates of the standard and the CoCo debts account for the indebtedness of the firm. Second, the number of shares received by CoCo debt holders at conversion time is also designed differently. In our study, the optimal dividend stream is solved through a dynamic optimization approach: the equity holders have an incentive to control the firm debt ratio to maximize their share of the firm equity value, avoiding the dilution effect caused by conversion. It also benefits the bondholder as its mitigates the default risk.
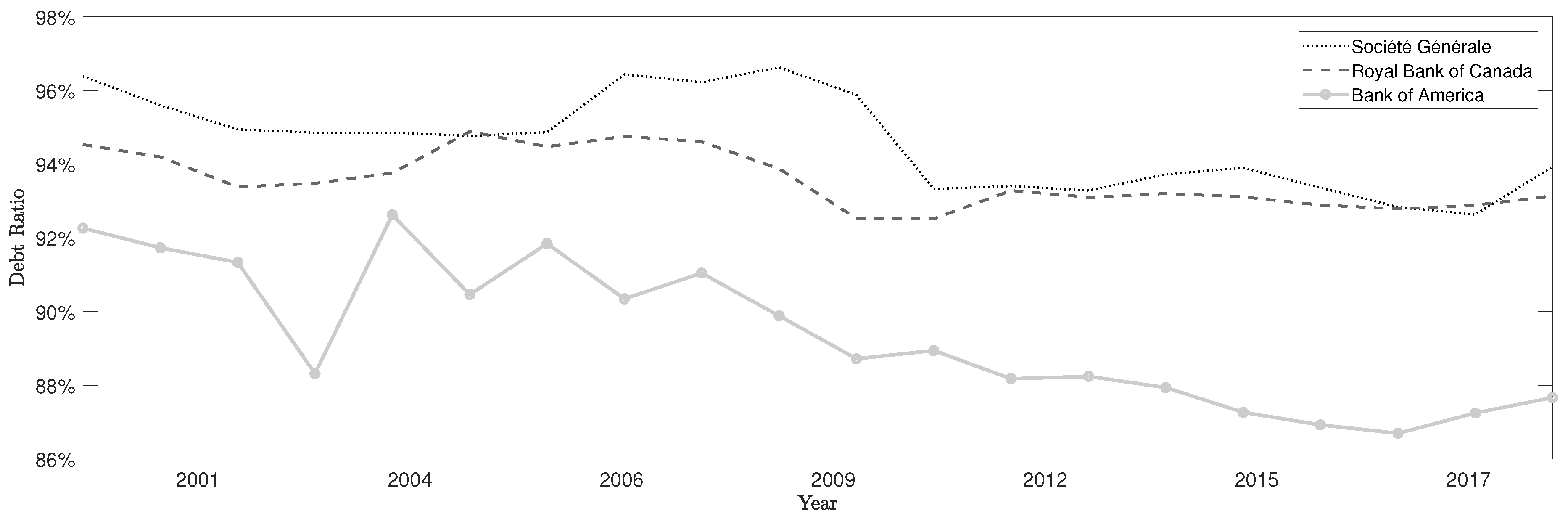
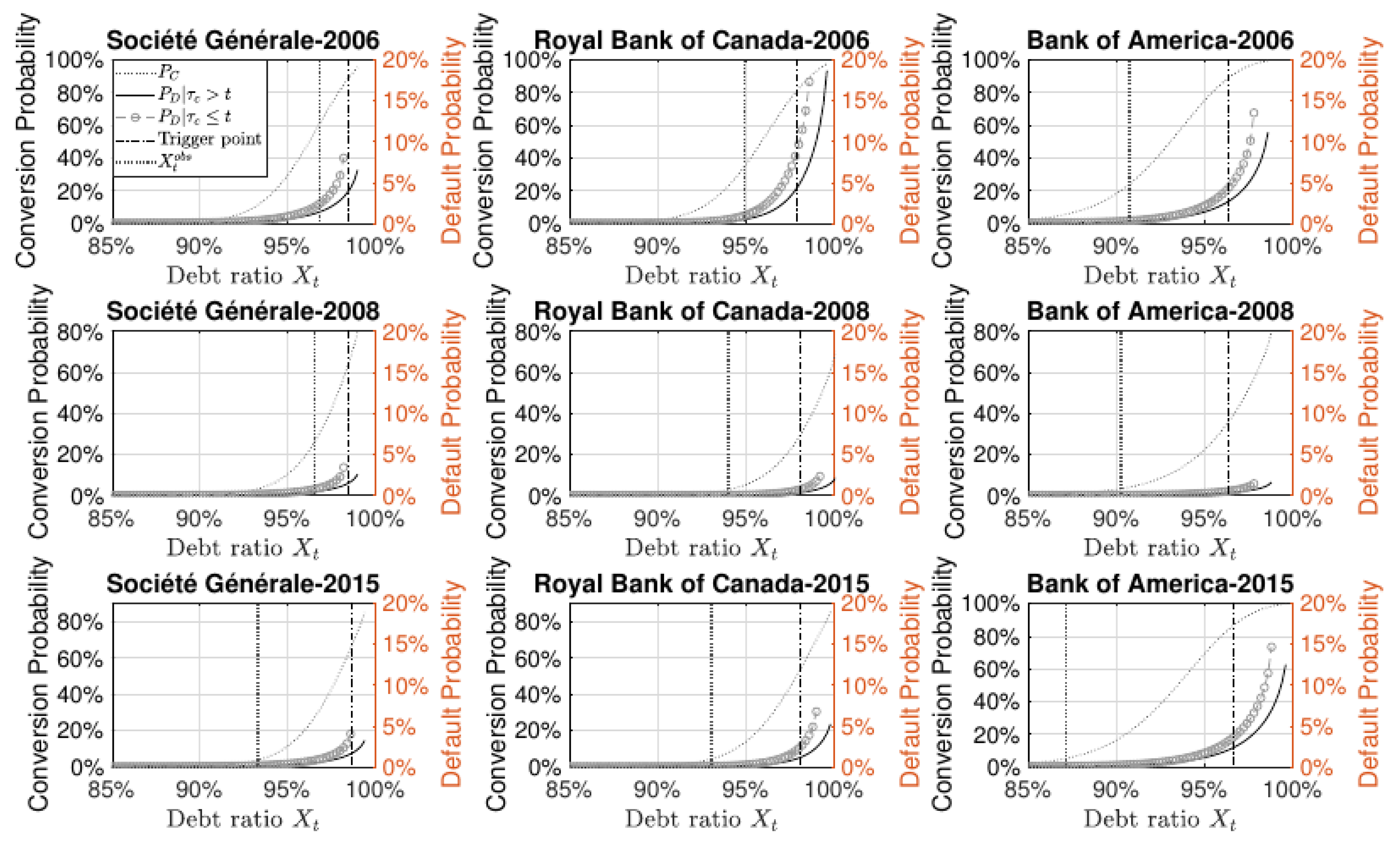
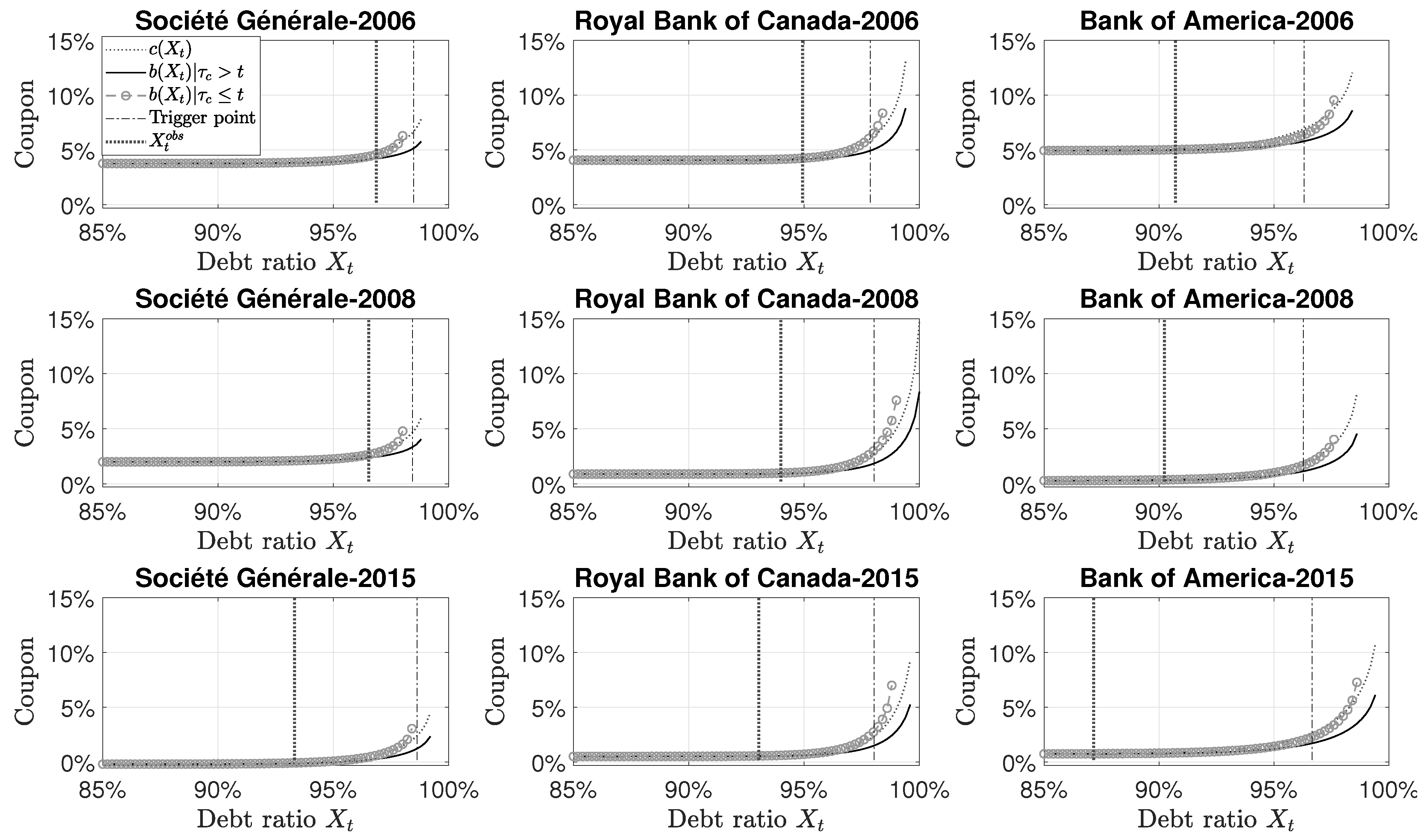
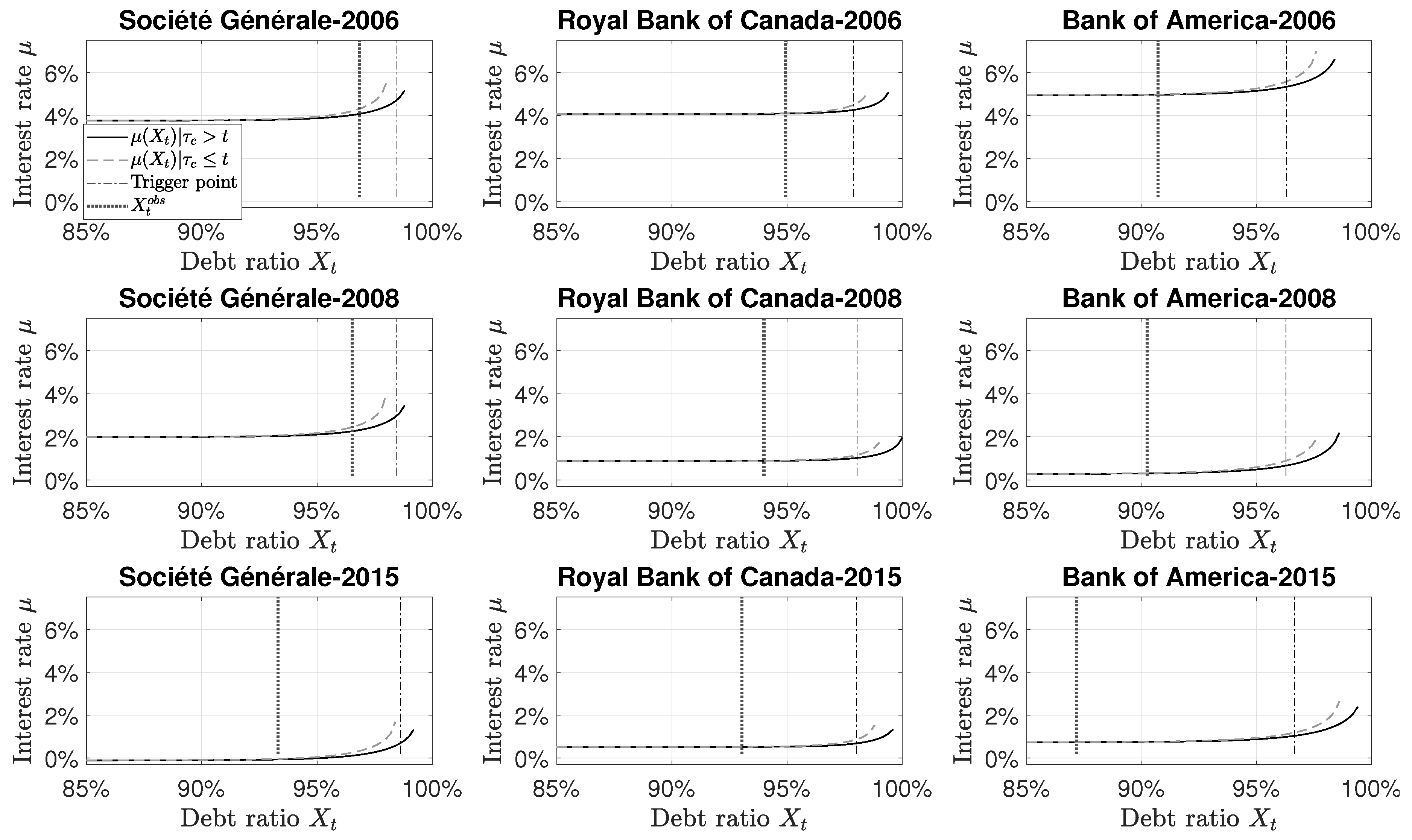
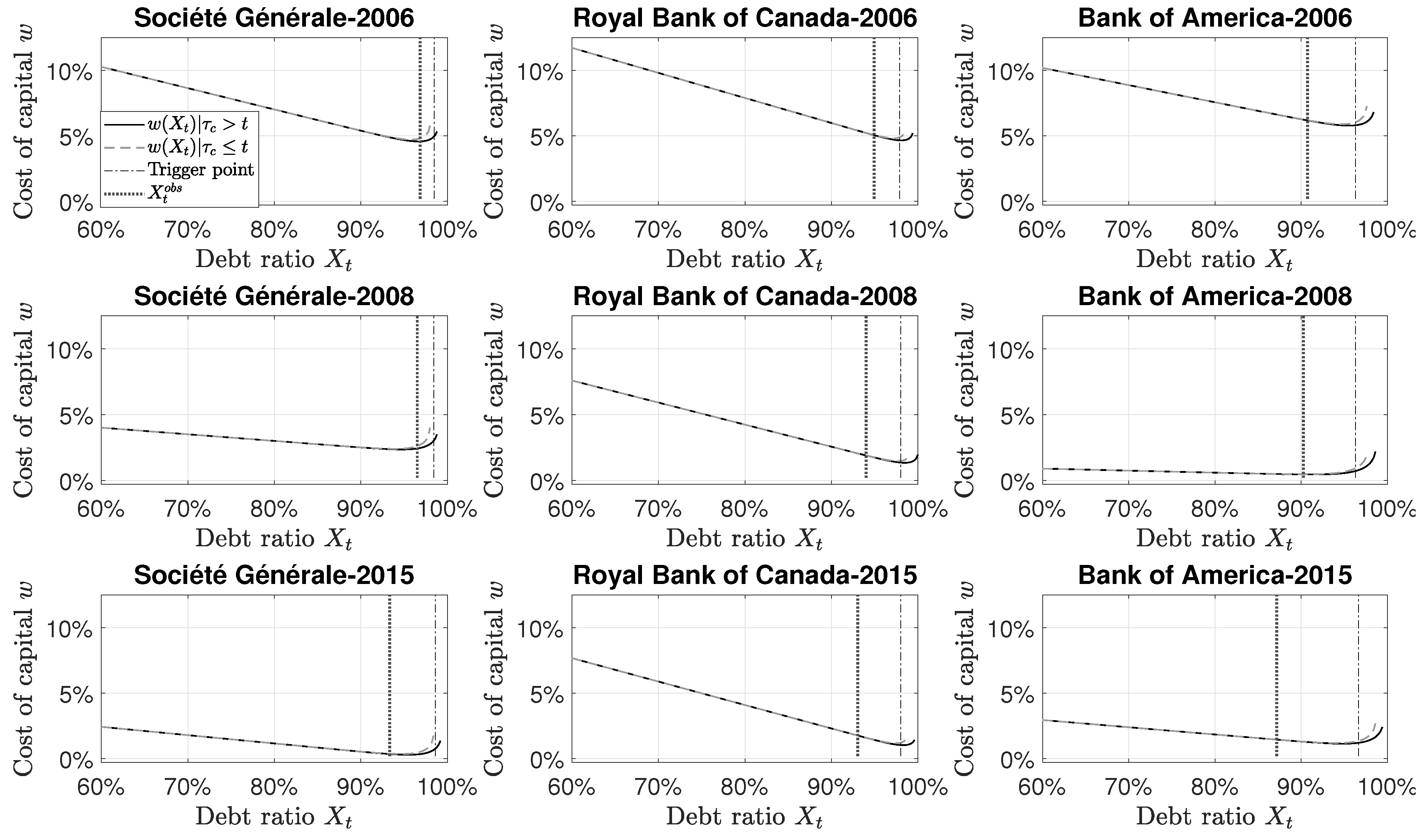
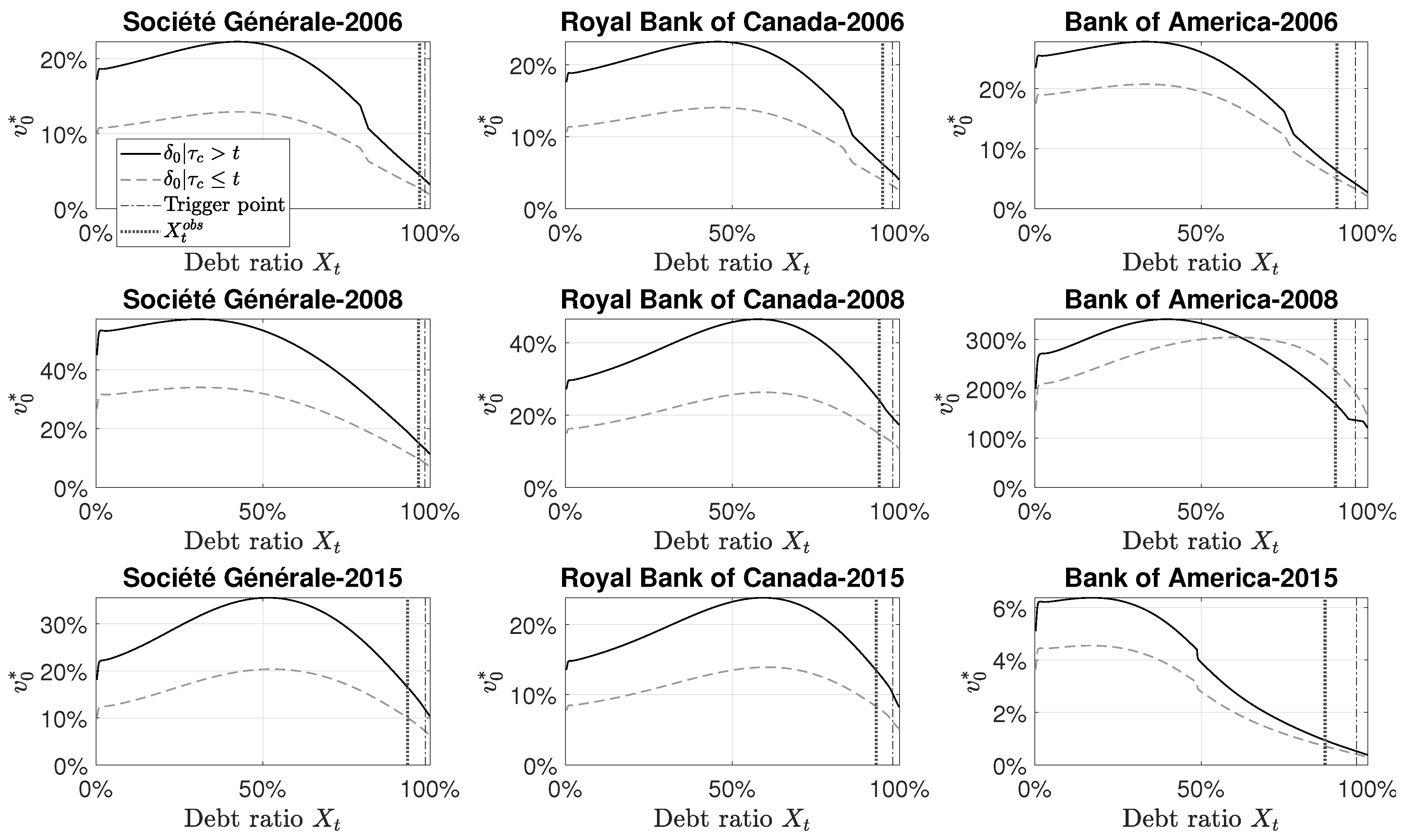
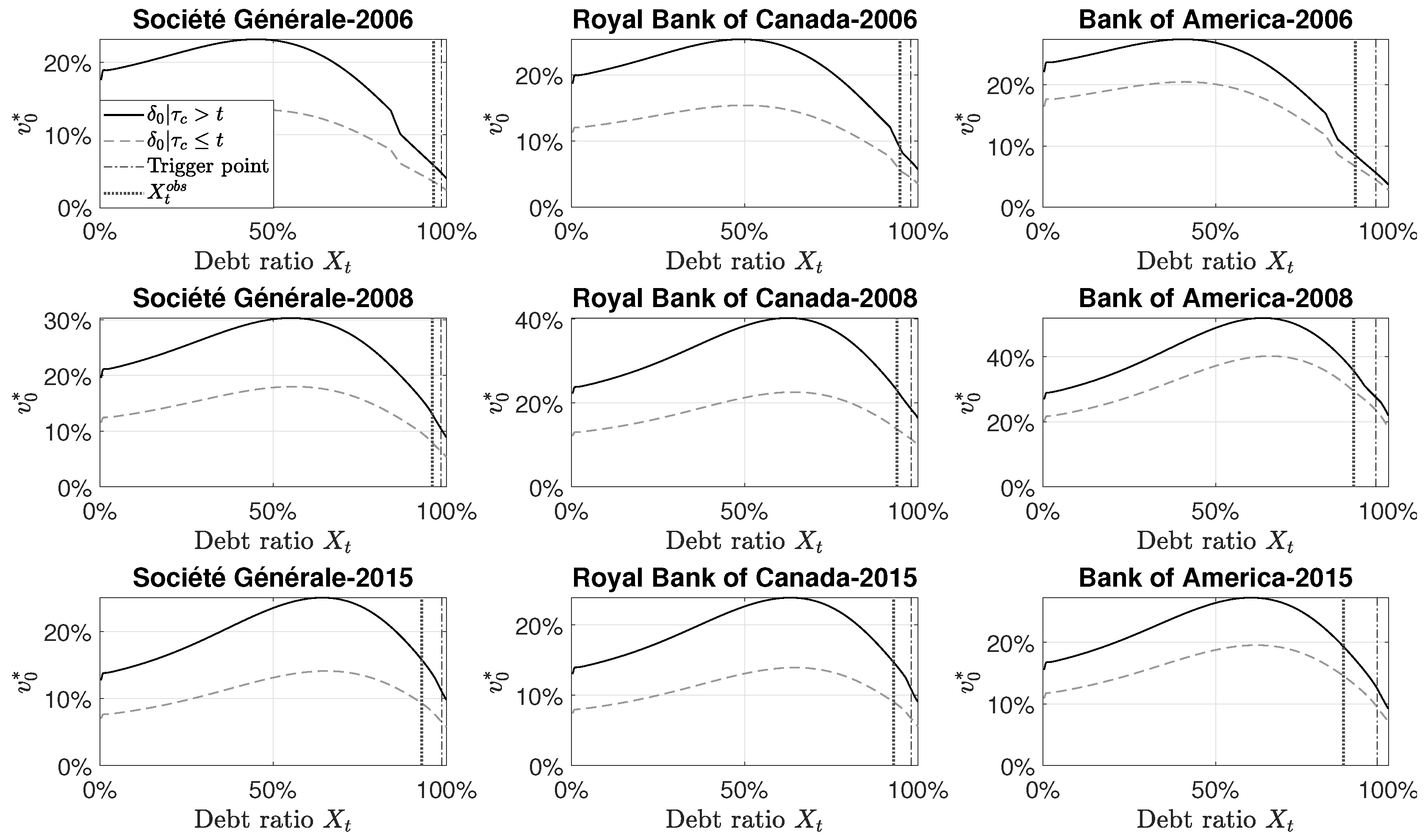
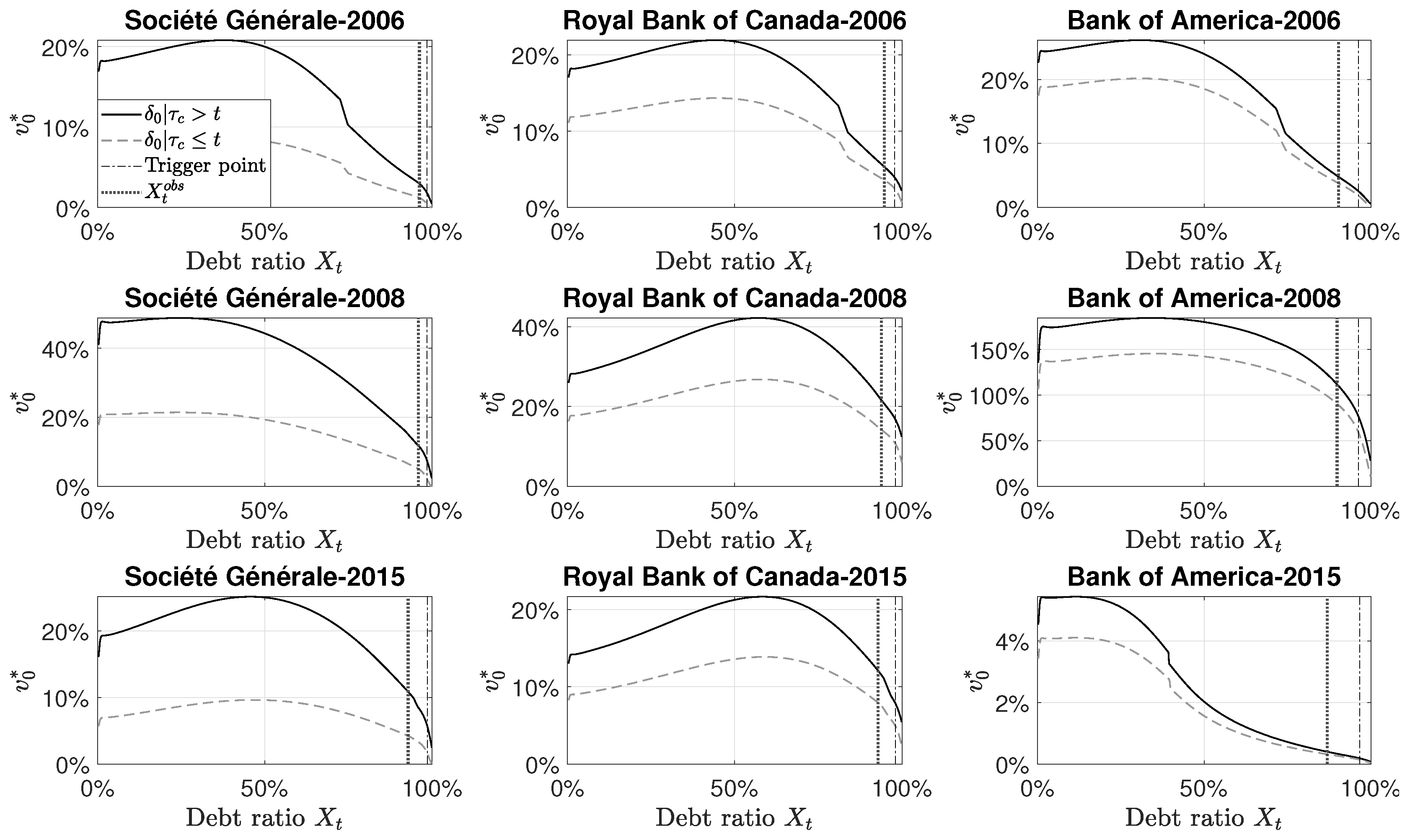
A numerical simulation based on realistic data evaluates the benefits and the costs of having CoCos in the bank’s debt structure. The parameters are estimated using three banks from three different regions (Europe, Canada and the United States), for three different periods of time (pre-crisis, crisis, post-crisis). The results help to understand how CoCos can help “Too big to fail” banks in different economic conditions. Although there are important differences among these three cases, common behaviours are observed: the presence of CoCos in the debt structure reduces the probability of default, the coupon of the standard debt, the cost of debt and capital. However, the CoCo is a more expensive instrument than the standard debt, mainly because the investor bears more risk. This study contributes to the literature by evaluating the effectiveness of adding CoCos to the financial firm’s capital structure. We do not only evaluate the CoCo debt itself, but also examine its impact on the firm’s management strategy by optimizing the per-share value of the cumulated dividend stream. Equity holders modify the optimal dividend policy to account for the conversion risk that affect them through the dilution effect (after conversion, there are more equity shares).
This paper is structured as follows.
Section 2 presents the model to value CoCo and defines the conversion as well as the default intensity.
Section 3 presents the dynamic programming model use to examine how CoCo impact the firm’s management strategy.
Section 4 is decomposed into three parts. First, the data used to have realistic scenarios are presented. Second, the results are presented for each bank and each year. Third, a sensitivity analysis follows.
Section 5 presents conclusions.
2. The Model
The asset value satisfies
where
and
are the equity and debt values, respectively. The debt is decomposed into three main components: the deposit whose time
t value is
, the coupon-paying bonds and other debt instruments for a value of
and a convertible contingent instrument (CoCo) whose value is
:
The debt ratio is defined as
The presence of the CoCo debt alleviates the default risk since, in case of financial distress, the CoCo debt is converted into equity, leading to a smaller debt ratio. CoCo debt holders bear not only a default risk, but also a conversion risk; therefore, they require compensation in the form of a coupon payment that differs from that of a standard debt, which is subject only to credit risk. More precisely, once converted into equity, the CoCo debt has a zero recovery rate in case of default. However, if the firm survives after the conversion, then it is not clear whether the proportion of equity value held by the CoCo debt holders will be more or less profitable than for a standard debt.
The default time is denoted
and the conversion time satisfies
, which means that the CoCo debt is always converted before the default event. Because other factors may influence the conversion decision, it does not necessarily occur as soon as the leverage ratio triggers
, but it is very likely to occur. Consequently, the conversion intensity driving
,
is a positive and convex function of
, taking large values whenever
is above
and small values otherwise.
should be close to
. The parameter
is usually a large positive constant. Therefore,
Intuitively, the conversion should occur whenever the leverage ratio is close to the critical level
determined by the regulator. If the CoCo debt still exists at time
t, then the superscript
denotes the pre-conversion values of the considered variables. The convertible contingent instrument is a coupon-paying bond with the floating coupon rate
. At conversion, CoCo debt holders receive, in the form of equity shares, an amount equivalent to the debt’s face value
and a fraction
of the coupon. In other words, at conversion, the convertible debt holders receive
equity shares where
is the post-conversion price per share. Assuming that initially there were
N outstanding equity shares, the post-conversion price per share becomes
Multiplying both sides by
and isolating
leads to
which implies that the price per share is not affected by the conversion. Then, the equity value becomes
Letting
be the proportion of convertible debt in the total debt value. Because there are always deposits, then the convertible instrument represents less than 100% of the debt value, which implies that
. The number of additional shares issued at conversion satisfies
The standard debt pays the floating coupon rate of
unless default occurs. Interestingly, the debt coupon is affected by the presence of the CoCo instrument because the latter mitigates both the default and recovery risks. If
denotes the proportion of the non-convertible contingent debt value that consists of deposits, then
2.1. The Debt Ratio -Dynamics
Assume that the firm survives up to time
At the beginning of the
period, the invested capital yields returns:
where
m and
are predictable processes and the sequence of
is formed with independent standard normal random variables. The information structure is provided by the filtration
where
is the
-field modelling the information available at time
t. Throughout the period, decisions about the convertible contingent debt conversion and the dividend payment affect the debt and debt ratio values. At time
free cash flow (
) is
The financial flow (
) is decomposed into the deposit interest payment
where
is the predictable risk-free rate, the interest payment on standard and convertible debts,
and
respectively, the dividend payment
and the debt structure variation,
The floating interest rates reflect the risk embedded in both standard and CoCo debts. Both types of debts contain credit risk because the firm may default. See
Appendix A.
The debt structure variation is expressed as a ratio:
New debt issuance makes
, whereas debt reaching maturity or CoCo debt conversion leads to
.
Assumption 1. Let where . In other words, whenever the contingent convertible debt exists, its proportion of the total debt value remains constant.
Similarly, assuming that the proportion of deposits with respect to the non-convertible debt value remains constant over time, and
Assumption 2. At conversion time, if there is no variation in the other types of debt, then In the numerical implementation, we assume that . In other words, the debt value at conversion is modified only by the conversion of the CoCo debt to equity.
Thus, the weighted average interest rate is
Since
the financial flow satisfies
The bank’s profit is the difference
between the free cash flow and the financial flow. Therefore,
which is equivalent to
Dividing both sides of Equation (
8) by
Since
comparing both equations and isolating
implies that the (post-dividend) debt ratio must satisfy
Note that
where
denotes the pre-dividend debt ratio
2.2. Conversion
The conversion decision is taken under the assumption that
,
(no debt issuing or refunding) and that the full interest payment includes the CoCo debt coupon:
The conversion intensity
is a positive, increasing, and convex function of the debt ratio
which is a particular case of Equation (
9). The parameter
is the critical level from which the conversion probability grows fast beyond this threshold. Because of the convex relation between the conversion intensity and the conversion probability,
is not exactly equal to the regulator critical level
, but it is in its neighborhood. The parameter
captures the growth speed. In the numerical implementation, both parameters are obtained through a calibration approach based on the regulator critical level
. For this reason,
denotes the conversion time characterized by the conversion intensity
. The conditional conversion probability at time
triggered by the critical level
(letting
, we recover the case where conversion occurs as soon as
) is
Because immediate conversion may also arise as a consequence of an unpredicted default, the survival conversion probability is
Then, the conditional conversion probability arising from both the critical level and the potential default is
where the default intensity
is defined at Equation (
17).
2.3. Default
Since default occurs after conversion, the interest payment does not include the CoCo debt coupon. Indeed, a fraction of the CoCo coupon is paid back to CoCo debt holders in the form of equity shares. This means that
The default intensity is based on the pre-dividend debt ratio. More precisely, assuming that there are no dividends,
and that the only debt variation considered is the one arising from an immediate conversion,
, the pre-dividend debt ratio is
Since a debt ratio augmentation has more impact on the default probability when the debt ratio is already high, the default intensity
is a positive, increasing, and convex function of the debt ratio. More precisely,
where
,
. Indeed,
represents the critical debt ratio from which the increasing behaviour of the default probability (seen as a function of the debt ratio) accelerates. Because the CoCo instrument provides the standard debt holders with an additional protection against default risk, the critical debt ratio is
slightly higher whenever the CoCo debt is present in the debt structure. Consequently, the conditional default probabilities are
and
3. Stochastic Optimum Control Problem
The period
discount factor is
where the cost of capital is a weighted average of the cost of equity,
, and the cost of debt:
The current equity holders want to maximize their share of dividends. More precisely, given a stream of dividend rates
, the expected value of the discounted dividends at time
t is
since Assumption 1 and Equation (
4) imply that
Since
V allows for the decomposition
the optimal dividend rate sequence
can be constructed recursively using backward recursion over time:
See
Appendix D. Because
,
and
Therefore, we work with a standardized version of the primary equity holders’ share of cumulated discounted dividends. Indeed, under this form, the dynamic optimization does not require the modelling of the dynamics of A.
The dividend rate is bounded above. Indeed, if the dividend payment is too large, the equity value will drop below its current level. More precisely, noting that
, the expected variation of the pre-dividend equity is (see
Appendix B.3)
We restrict
, making the right-hand side bound positive. Now, let
be the solution of
. Indeed, since
is an increasing function of
x starting at 0, a unique solution exists. Since equity holders do not reduce the expected equity value deliberately, it follows that
In addition, since
as
, this upper dividend rate bound is not active for small debt ratio values. However, the dividend rate is generally lower than the expected asset returns, which leads to
, where
Dynamic programming optimization allows using a recursive method starting from T with backward induction. At each time period, the value function is the sum of the immediate dividend and the expected future dividend gain. To initialize the recursion, a long time horizon T is chosen for which some simplifications are made. Because the model is Markovian, after some iterations, the terminal conditions vanish and, for that reason, the following assumption has no impact on our numerical results. The algorithm stops when the variations in the optimal dividend become very small. Then, for time T, the following simplifications are to be assumed.
Assumption 3. For any ,
- 1.
The asset returns are no longer uncertain, that is, ,
- 2.
There is no more possibility of conversion, that is, the CoCo debt becomes a standard debt;
- 3.
The dividends are the remaining part of the returns once the interest rate payment on the debts is deducted: - 4.
If the dividend payment is positive, then there is no variation of the debt value, that is, or, equivalently,
- 5.
The risk-free rate is constant and equal to
Since a potential conversion is no longer possible (Assumption 3-2), for all
, the coupon on the CoCo debt is the same as the one on the ordinary debt, that is,
Assume for a moment that
. As a consequence of Assumption 3-3,
,
which implies that
Therefore,
Moreover, from Assumption 3-4,
it follows that
and
In addition, a recursive argument leads to the following conclusion: if the dividend rate is positive, that is
then for all
,
,
,
and
The discount factor then becomes
If
, then not only is there no dividend payment, but also the firm needs to issue more debt to cover the interest expenses:
In that case,
and
Therefore, the debt is growing and the asset value is stable, which implies that the debt ratio will increase until default.
Lemma 1. Under Assumption 3, the expected value of the discounted dividends at time T satisfieswhere , and
See proof in
Appendix B.4.