The Impact of Reinsurance Strategies on Capital Requirements for Premium Risk in Insurance

Abstract

New risk-based solvency requirements for insurance companies across European markets have been introduced by Solvency II and will come in force from 1 January 2016. These requirements, derived by a Standard Formula or an Internal Model, will be by far more risk-sensitive than the required solvency margin provided by the current legislation. In this regard, a Partial Internal Model for Premium Risk is developed here for a multi-line Non-Life insurer. We follow a classical approach based on a Collective Risk Model properly extended in order to consider not only the volatility of aggregate claim amounts but also expense volatility. To measure the effect of risk mitigation, suitable reinsurance strategies are pursued. We analyze how naïve coverage as conventional Quota Share and Excess of Loss reinsurance may modify the exact moments of the distribution of technical results. Furthermore, we investigate how alternative choices of commission rates in proportional treaties may affect the variability of distribution. Numerical results are also figured out in the last part of the paper with evidence of different effects for small and large companies. The main reasons for these differences are pointed out.

1. Introduction

On 10 October 2014, the European Commission adopted a Delegated Act (see [1]) regarding implementing rules for Solvency II. This document was published in the Official Journal of European Union on 17 January 2015, as Commission Delegated Regulation n. 2015/35, after approval of the European Parliament and Council. The new system will lay down new quantitative requirements and a proper methodology to evaluate them. These new criteria will come in force from next 1 January 2016.

Usually for a Non-Life insurer, the Underwriting Risk module (and, in particular, Premium and Reserve sub-module) has the greatest impact on Solvency II Capital Requirement (SCR—Solvency Capital Requirement). In the valuation of these requirements, the risk mitigation effect of proportional and non-proportional reinsurance will be recognized. In this framework, the aim of this paper is to describe the risk profile of a general multi-line insurer in order to show the effect of reinsurance, one of the most crucial aspects of risk management strategies.

In order to describe the risk profile of a general multi-line insurer, we start by adopting the structure of a classical risk theoretical model where, by known relations in actuarial literature1, only premium risk is dealt with. We extend the relation to consider also the volatility of expenses to evaluate the impact on both exact moments of technical results and capital requirements.

Furthermore, to analyze the effect on both profit and losses and capital requirement of alternative reinsurance strategies2, two classical proportional and non-proportional treaties have been introduced by extending classical relations. We derive moments of combined ratios by considering both the cases of Quota-Share (QS) and Excess of Loss (XL) treaties (see [2,3,4]). According to Quota-Share, we analytically describe how alternative methodologies3 to identify ceding commissions have an effect on the moments of the probability distribution of combined ratios and on the capital requirements for Premium Risk.

Indeed, in the management practice, the insurer must usually choose among different efficient reinsurance strategies, taking into account either profitability sacrifice or capital saving.

A case study based on two different multi-line Non-Life insurers allows the comparison of the effect of wide strategies on both profitability and allocated capital. Parameters of the model have been calibrated in order to assure a realistic and consistent comparison between insurers and alternative reinsurance strategies.

In particular, Section 2 briefly describes the structure of the Standard Formula defined by Delegated Acts [1] focusing only on a sub-module of Premium and Reserve risk. In Section 3, we provide the general framework needed to develop the internal model. Section 4 analytically shows the effect of two alternative reinsurance strategies as Quota-Share and Excess of Loss. Finally, in Section 5, numerical results are reported by focusing on the capital requirements derived by applying both the Internal Model and the market-wide approach of the Standard Formula. Main results have been extended in Section 6 to evaluate the effect of reinsurance. The conclusion follows.

2. A Brief Description of the Delegated Acts (DA) Standard Formula

We give a brief description of the main elements of Standard Formula for Premium and Reserve Risk defined by Delegated Acts (see [1]). Since Quantitative Impact Studies 3 (QIS3), a unique sub-module for the joined valuation of risks related both to future claims arising during and after the period until the one-year time horizon for the solvency assessment (Premium Risk) and the risk related to a non-sufficient amount of the technical provisions (Reserve Risk) has been introduced. The derived capital charge must be then aggregated to lapse and cat risk to quantify the capital requirement for Non-Life Underwriting Risk.

Focusing on Premium and Reserve risk, we have that the capital requirement $SC{R}_{NL}^{SF}$ is equal to the following formula:

$$SC{R}_{NL}^{SF}=3·{\text{σ}}_{NL}·V_{NL}$$

(1)

where $V_{NL}$ is the volume measure net of reinsurance and ${\text{σ}}_{NL}$ is the standard deviation for non-life premium and reserve. ${\text{σ}}_{NL}$ can be described as the standard deviation of the ratio between the aggregate claims amount of premium and reserve risk and the volume measure, and it is then strictly related to the variability coefficient (CV) of aggregate claim amount.

In particular, Equation (1) assumes to measure the distance between the 99.5% quantile and the mean of the probability distribution of aggregate claims amount by using a fixed multiplier of the standard deviation equal to 3. This choice has replaced the $\text{ρ}\left({\text{σ}}_{NL}\right)$ function used since QIS5 [16], which was based on the assumption of a LogNormal distribution of total losses. From a practical point of view, skewness of distribution is not directly taken into account anymore. The drawback is a potential underestimation of capital requirement for small insurers and an overestimation for big insurers. We have indeed found that the LogNormal assumption (and then ρ(σ_NL)) returns a multiplier equal to 3 only when ${\text{σ}}_{NL}$ is roughly 14.47%. For smaller volatilities coefficients (as it usually happens for big insurers), Equation (1) leads to a capital requirement that is larger than QIS5.

The net volume measure $V_{NL}$ is equal to the sum of all the lines of business (LoBs) of net best estimate of claims reserve at the valuation date plus net premium volume. This volume is equal to the maximum between last year and next year earned premiums plus the expected present value of future premiums after one-year for existing contracts and contracts of the following year. Finally, in the valuation of $V_{NL}$, it is allowed to take into account a geographical diversification of business held in different macro-geographical regions of world, through the Herfindahl Index.

With regard to ${\text{σ}}_{NL}$, the overall volatility is derived by a double steps aggregation process based on an initial aggregation of standard deviation of premium and reserve risk of single LoB assuming a linear correlation coefficient equal to 0.5. Then, the standard deviation will be aggregated between different lines of business by using a given correlation matrix (see Annex IV of Delegated Acts [1] for details).

In order to quantify the standard deviation of premium or reserve risk of a single LoB, two different approaches are provided. The first one is based on fixed volatility factors and it is defined as a “market-wide approach”, while the second one is based on methodologies (see Annex XVII of [1]) that take into account the specific technical data of the company (“undertaking-specific approach”). Adoption of the latter approach must be approved by the supervising authority. The differences between market-wide and the undertaking-specific approach may be noticeable in the single-LoB volatility valuation. The market approach is based on a market-wide estimate of the standard deviation for premium risk, determined by a specific volatility factor given as input (see Annex II and XIV of [1]). For instance, similarly to the main non-life LoB analyzed in the next, these factors for Premium risk are reported in Table 1.

Table 1. Volatility factor, ${\text{σ}}_{prem,lob}$ (DA—Premium Risk).

LoBs	Accident4	Motor Damages	Property	Motor Third-Party Liab.	General Third-Party Liab.
Volatility Factor	8.5%	8%	8%	10%	14%

Table 1. Volatility factor, ${\text{σ}}_{prem,lob}$ (DA—Premium Risk).

LoBs	Accident4	Motor Damages	Property	Motor Third-Party Liab.	General Third-Party Liab.
Volatility Factor	8.5%	8%	8%	10%	14%

Only for Premium Risk, values of ${\text{σ}}_{prem,lob}$ can be multiplied by a non-proportional factor $N{P}_{lob}$ in order to take into account existing Excess of Loss treaties. This factor is set out at 80% for Property, Motor Third Party Liabilities (MTPL) and General Third Party Liabilities (GTPL) and at 100% for other LoBs.

It is, however, allowed to use an undertaking specific approach (see Annex XVII of [1]) to also derive an alternative estimate of $N{P}_{lob}$ based on the valuation of the reducing effect of XL treaty on the variability coefficient of the aggregate claim amount. In this case, the final value of $N{P}_{lob}$ will be a weighted average of fixed $N{P}_{lob}$ and the corresponding estimate. The weight of the factor estimated by data is given by a fixed credibility factor increasing the larger the available time-series. For all LoBs, the weights are greater than zero if data of at least last five years are available and tend to one. MTPL, GTPL and Credit and Suretyship should have data over 15 years and other LoBs at least over 10 years.

3. General Framework

We present an Internal Model for Premium Risk for a multi-line Non-Life insurer to take into account the characteristics of each line of business (LoB) and the diversification effect due to the aggregation of them. To introduce the framework, let ${\tilde{Y}}_{t+1}$ be the random variable (r.v.5) of a one-year technical result for the period $\left(t, t+1\right)$, evaluated at the end of time $t$, as the difference between earned premium of the total portfolio with $h=1,\dots , L$ LoBs and total amount of claims and expenses of the year. For the sake of simplicity, we will start by considering a gross of reinsurance technical result. Generalizations to also include the reinsurance effect are in the next Section6.

By considering the main sources of risks, we may decompose ${\tilde{Y}}_{t+1}$ as follows:

$${\tilde{Y}}_{t+1}={\displaystyle \sum }_{h=1}^L\left(B_{t+1,h}^{writt}+V_{t,h}^P-{\tilde{V}}_{t+1,h}^P-{\tilde{E}}_{t+1,h}-{\tilde{X}}_{t+1,h}^{paid,CY}-{\tilde{V}}_{t+1,h}^{S,CY}\right)$$

(2)

Earned premiums of a single LoB are here described as the difference between written premium of the year $B_{t+1,h}^{writt}$ and the one-year change in premium reserve (${\tilde{V}}_{t+1,h}^P-V_{t,h}^P$) for unearned premiums and unexpired risks evaluated under Solvency II criteria. In the same way we take into account the claim cost of the year, by considering both payments ($\tilde{X}$) for claims and the provisions for outstanding claims (${\tilde{V}}_{t+1}^S$). Regarding premium risk, we consider only payment for losses of claims incurred during the year $t+1$ $\left({\tilde{X}}_{t+1,h}^{paid,CY}\right)$ and the reserve at the end of year $t+1$ for new claims $\left({\tilde{V}}_{t+1,h}^{S,CY}\right)$. Both payments and reserves for claims incurred in previous years are necessarily covered by initial claims reserve and their volatility attains to reserve risk. Finally we assume random the expenses ${\tilde{E}}_{t+1,h}$ too.

Formula (2) may be rewritten as follows:

$${\tilde{Y}}_{t+1}={\displaystyle \sum }_{h=1}^L\left(P_{t+1,h}+{\text{λ}}_h{P}_{t+1,h}+c_h{B}_{t+1,h}+V_{t,h}^P-{\tilde{V}}_{t+1,h}^P-{\tilde{E}}_{t+1,h}-{\tilde{X}}_{t+1,h}^{paid,CY}-{\tilde{V}}_{t+1,h}^{S,CY}\right)$$

(3)

In Equation (3), gross premiums of the $h$-th LoB are represented by risk premiums split into three components: the expected amount for claims of current year $P_h=E\left({\tilde{X}}_h^{paid,CY}+{\tilde{V}}_h^{S,CY}\right)$, the safety loadings (${\text{λ}}_h·P_h$) and the expense loading equal to the expected amount of expenses, i.e., $c_h{B}_{t+1,h}=E\left({\tilde{E}}_{t+1,h}\right)$.

For sake of simplicity, we can assume that earned premiums and written premiums are equal7 and recalling a classical notation in Risk Theory, we can identify the aggregate claim amount by a generic random variable $\tilde{X}={\tilde{X}}^{paid,CY}+{\tilde{V}}^{S,CY}$ independent by paid or reserved claims:

$${\tilde{Y}}_{t+1}={\displaystyle \sum }_{h=1}^L\left(P_{t+1,h}+{\text{λ}}_h{P}_{t+1,h}+c_h{B}_{t+1,h}-{\tilde{E}}_{t+1,h}-{\tilde{X}}_{t+1,h}\right)$$

(4)

where ${\tilde{X}}_{t+1,h}$ describes the aggregate claim amount of next year related to new business.

To evaluate characteristics of ${\tilde{Y}}_{t+1}$, we can make some assumptions about aggregate claim amounts and expenses. Following the collective approach (e.g., see [5,8,17]), for each LoB, the aggregate claims amount is given by a mixed compound process:

$${\tilde{X}}_{t+1,h}={\displaystyle \sum }_{j=1}^{{\tilde{K}}_{t+1,h}}{\tilde{Z}}_{j,t+1,h}$$

where the number of claims distribution, ${\tilde{K}}_{t+1,h}$, follows the Poisson law, with parameter, $n$, increasing year by year according to the real growth rate $g$ (i.e., $n_{t+1,h}=n_{t,h}\left(1+g_h\right))$. We are assuming that the expected number of claims grows along with the number of contracts. Frequency is then constant in period $\left(t,t+1\right)$. In the present paper, trends as well as long-term cycles are not considered and only short-term fluctuations that may affect the volatility of the number of claims are taken into account. For this purpose, a structural variable ${\tilde{q}}_h$ will be introduced to represent short-term fluctuations in the number of claims. Then we have that $n$ turns out to be a stochastic parameter $\left(n_{t+1,h}·{\tilde{q}}_h\right)$ where ${\tilde{q}}_h$ has its own probability distribution depending on the short-term fluctuations it is going to represent. In Section 5, we will assume that ${\tilde{q}}_h$ is Gamma distributed with mean equal to one. Standard results from mathematical statistics imply that the mixture Poisson-Gamma leads to a Negative Binomial r.v. for the number of claims.

The claim size amounts ${\tilde{Z}}_{j,t+1,h}$ are assumed i.i.d. and scaled by the claim inflation rate $i_h$. In other words, we have that simple moments of order r of severity distribution are equal to $E({\tilde{Z}}_{t+1,h}^r)={\left(1+i_h\right)}^r·E\left({\tilde{Z}}_{t,h}^r\right).$ Different distributional assumptions (for details see [19]) may be considered for claim size but for sake of simplicity and without loss of generalization, only the results under LogNormal assumption will be reported below.

In order to take into account expense volatility, we will assume that acquisition and management expenses are described by two random variables with mean and standard deviation equal to $\left(c_h^A{B}_{t+1,h},{\text{σ}}_h^A{B}_{t+1,h}\right)$ and $\left(c_h^M{B}_{t+1,h},{\text{σ}}_h^M{B}_{t+1,h}\right)$ respectively, with $c_h^A+c_h^M=c_h$. The coefficients $c_h^A$ and $c_h^M$ represent the percentages of gross premiums used to cover respectively acquisition and management expenses. ${\text{σ}}_h^A$ and ${\text{σ}}_h^M$ describe the standard deviation of expense ratios considering only acquisition or management expenses.

To simulate expenses, a LogNormal distribution has been used in the next case study. It will be assumed that expenses are not correlated to the claim amount. However, the distributional and dependence assumptions do not have a great impact on the capital charge (except for specific lines as Credit and Suretyship or Financial Losses for some specialist insurers).

Under these assumptions, main cumulants of ${\tilde{X}}_{t+1,h}$ and ${\tilde{E}}_{t+1,h}$ may be derived to obtain exact formulae for cumulants of technical results of a single line of business.

The cumulant generating function (f.g.c.), ${\text{Ψ}}_{{\tilde{Y}}_{t+1,h}}\left(s\right)$, of technical result of the $h$-th single LoB is:

$${\text{Ψ}}_{{\tilde{Y}}_{t+1,h}}\left(s\right)=s·B_{t+1,h}-{\text{Ψ}}_{{\tilde{E}}_{t+1,h}}\left(s\right)-{\text{Ψ}}_{{\tilde{X}}_{t+1,h}}\left(s\right)=$$

where $M_{{\tilde{Z}}_{,t+1,h}}\left(s\right)$ is the moment generating function of claim-size.

Then, the mean, variance and skewness of ${\tilde{Y}}_{h,t+1}$ are:

$$E\left({\tilde{Y}}_{h,t+1}\right)={\text{λ}}_h{P}_{t+1,h}$$

$${\text{σ}}^2\left({\tilde{Y}}_{t+1,h}\right)=\left({\left({\text{σ}}_h^A\right)}^2+{\left({\text{σ}}_h^M\right)}^2\right)B_{t+1,h}^2+n_{t+1,h}{a}_{2,{\tilde{Z}}_{t+1,h}}+n_{t+1,h}^2{a}_{1,{\tilde{Z}}_{t+1,h}}^2{\text{σ}}_{{\tilde{q}}_h}^2$$

$$\begin{array}{l}\gamma ({\stackrel{\sim }{Y}}_{t+1,h})=\\ =\frac{{\mu }_3({\stackrel{\sim }{E}}_{{}_{t+1,h}}^A)+{\mu }_3({\stackrel{\sim }{E}}_{{}_{t+1,h}}^M)+n_{t+1,h}{a}_{3,{\tilde{z}}_{t+1,h}}+3n_{t+1,h}^2{a}_{1,{\tilde{Z}}_{t+1,h}}{a}_{2,{\tilde{Z}}_{t+1,h}}{\sigma }_{\tilde{q}h}^2+n_{t+1,h}^3{a}_{1,{\tilde{Z}}_{t+1,h}}^3{\mu }_3(\tilde{q}h)}{{\left(\left({\left({\sigma }_h^A\right)}^2+{\left({\sigma }_h^M\right)}^2\right)B_{t+1,h}^2+n_{t+1,h}{a}_{2,{\tilde{Z}}_{t+1,h}}+n_{t+1,h}{a}_{1,{\tilde{Z}}_{t+1,h}}^2{\sigma }_{\tilde{q}h}^2\right)}^{3/2}}\end{array}$$

where $a_{r,{\tilde{Z}}_{t+1,h}}$ are non-central moments of ${\tilde{Z}}_{t+1,h}$ of order $r$ and ${\text{μ}}_3\left(·\right)$ describes the central moment of order 3.

Aggregated technical results will depend instead on the dependence assumed between several lines of business. According to the VaR risk measure (see [20]) at confidence level α = 99.5% as defined by Solvency II ([1]), the capital requirement (SCR) for Premium could be derived as:

$$SC{R}_{\text{α}}=-Va{R}_{1-\text{α}}\left({\tilde{Y}}_{t+1}\right)=Va{R}_{\text{α}}\left({\displaystyle \sum }_{h=1}^L{\tilde{E}}_{t+1,h}+{\tilde{X}}_{t+1,h}\right)-{\displaystyle \sum }_{h=1}^L{B}_{t+1,h}$$

It is noteworthy that we recognize expected profits/losses in the capital requirement evaluation by considering safety loadings. From our point of view, safety loading should be regarded, but it is not clear if it will be allowed in Internal Model by the supervisor, because QIS5 [16] and Delegated Acts [1] Standard Formula do not mention it in the evaluation (see Section 2). This solution in the Standard Formula is coming from the QIS5 multiplier of standard deviation found as the distance between the desired quantile (at 99.5% level) and the expected losses. It is worth pointing out that this approach would be not conservative if underpricing was in force, and a negative technical result would be expected implying a consequent higher risk profile.

4. Reinsurance Effect

In order to consider the effect of reinsurance treaties, Formula (4) may be enriched as follows:

$${\tilde{Y}}_{t+1}^{net}={\displaystyle \sum }_{h=1}^L\left[\left(P_{t+1,h}+{\text{λ}}_h{P}_{t+1,h}+c_h{B}_{t+1,h}-{\tilde{E}}_{t+1,h}-{\tilde{X}}_{t+1,h}\right)-\left(B_{t+1,h}^{RE}-{\tilde{X}}_{t+1,h}^{RE}-{\tilde{C}}_{t+1,h}^{RE}\right)\right]$$

where $B_{t+1,h}^{RE}$ describes premiums paid to the reinsurer, while ${\tilde{X}}_{t+1,h}^{RE}$ is the amount of claims paid or reserved born by the reinsurer. Finally, we consider stochastic ceding commissions ${\tilde{C}}_{t+1,h}^{RE}$ that the reinsurer usually pays in proportional treaties to the ceding company for the afforded commercial expenses.

We will consider in the next Section either the case of fixed commissions equal to a deterministic percentage of premiums or the case of “sliding scale” commissions. A sliding scale commission is a percent of premium paid by the reinsurer to the ceding company, which “slides” with the actual loss experience, usually subjected to minimum and maximum amounts.

We start by considering the effect of two global Quota Share treaties, with either fixed commissions or sliding commissions.

As is well known, in the case of a Quota Share reinsurance treaty, with an insurer’s retention quota ${\text{β}}_h\in \left(0,1\right)$, the aggregate claim amount charged to reinsurer is equal to ${\tilde{X}}_{t+1,h}^{RE}=\left(1-{\beta }_h\right){\tilde{X}}_{t+1,h}$. On the other hand, the gross premiums ceded to the reinsurer are:

$$B_{t+1,h}^{RE}=\left(1-{\text{β}}_h\right)B_{t+1,h}$$

In proportional treaties, the reinsurer pays the cedant a commission on the premiums it receives to compensate for the cost of acquiring the business and maintaining the portfolio. To describe commissions, we have assumed ${\tilde{C}}_{t+1,h}^{RE}={\tilde{c}}_h^{RE}{B}_{t+1,h}^{RE}$. In this regard, we consider two alternative ways. On one hand we assume a fixed percentage of ceded premiums as commission: $C_{t+1,h}^{RE}=c_h^{RE}{B}_{t+1,h}^{RE}$ (i.e., ${\tilde{c}}_h^{RE}=c_h^{RE}$). On the other hand, we consider a sliding commission that rewards or penalizes the insurer according to the quality of portfolio protected by the treaty. The system consists of a variable commission whose value depends by the observed loss ratio (see [15]).

We assume to describe the random commission rate according to the next formula:

$${\tilde{c}}_h^{RE}=c_h^{RE}\left[1+\left(1-\frac{{\widetilde{LR}}_{h,t+1}}{E\left({\widetilde{LR}}_{h,t+1}\right)}\right)\right]$$

(5)

where ${\widetilde{LR}}_{h,t+1}$ is the loss ratio at time $t+1$. Sliding commissions are here assumed not subjected to minimum and maximum amounts. In Section 6, we will also test numerically the effect of a different structure where a minimum and maximum commission is provided when observed loss ratio falls out of a certain range. For each line of business, we can easily derive the characteristics of technical result net of reinsurance ${\tilde{Y}}_{t+1,h}^{net}$ and of aggregate claim amount retained by ceding company.

We report exact cumulants of combined ratio net of reinsurance ${\widetilde{CR}}_{t+1,h}^{net}$ for both cases of fixed (${\widetilde{CR}}_{t+1,h}^{net,QSF})$ and scaling commissions (${\widetilde{CR}}_{t+1,h}^{net,QSS})$.

First of all, the expected combined ratio net of Quota Share treaty, ${\widetilde{CR}}_{t+1,h}^{net,QS}$, for both fixed and scaling commissions is:

$$E\left({\widetilde{CR}}_{t+1,h}^{net,QS}\right)=E\left(\frac{{\tilde{E}}_{t+1,h}+{\tilde{X}}_{t+1,h}-{\tilde{C}}_{t+1,h}^{RE}-{\tilde{X}}_{t+1,h}^{RE}}{B_{t+1,h}-B_{t+1,h}^{RE}}\right)=\frac{1+c_h}{1-{\text{λ}}_h}+\frac{c_h-c_h^{RE}\left(1-{\text{β}}_h\right)}{{\text{β}}_h}$$

Note that the net combined ratio is equal to $E\left({\widetilde{CR}}_{t+1,h}^{gross,QS}\right)$ when commission rate $c_h^{RE}$ is equal to the expenses loading coefficient $c_h$.

Furthermore the standard deviation is:

$$\begin{array}{l}\sigma ({\stackrel{\sim }{CR}}_{t+1,h}^{net,QS})\\ =\sqrt{\frac{{\sigma }^2({\stackrel{\sim }{E}}_{t+1,h})+{\sigma }^2({\stackrel{\sim }{X}}_{t+1,h}-{\stackrel{\sim }{X}}_{t+1,h}^{RE})+{\sigma }^2({\stackrel{\sim }{C}}_{t+1,h}^{RE})+2Cov({\stackrel{\sim }{X}}_{t+1,h}-{\stackrel{\sim }{X}}_{t+1,h}^{RE},-{\stackrel{\sim }{C}}_{t+1,h}^{RE})}{{\beta }_h^2{B}_{t+1,h}^2}}\\ =\sqrt{\frac{1}{{\beta }_h^2}{\sigma }^2({\stackrel{\sim }{ER}}_{t+1,h}^{gross})+{\sigma }^2({\stackrel{\sim }{LR}}_{t+1,h}^{gross})+\frac{{\sigma }^2({\stackrel{\sim }{C}}_{t+1,h}^{RE})-2{\beta }_{h}Cov({\stackrel{\sim }{X}}_{t+1,h},{\stackrel{\sim }{C}}_{t+1,h}^{RE})}{{\beta }_h^2{B}_{t+1,h}^2}}\end{array}$$

where, in the case of fixed commissions, we have:

$$\text{σ}\left({\widetilde{CR}}_{t+1,h}^{net,QSF}\right)=\sqrt{\frac{1}{{\text{β}}_h^2}{\text{σ}}^2\left({\widetilde{ER}}_{t+1,h}^{gross}\right)+{\text{σ}}^2\left({\widetilde{LR}}_{t+1,h}^{gross}\right)}$$

with variability greater than the corresponding value for the gross of reinsurance case because of a higher volatility of net expense ratio.

For sliding commissions, we have instead:

$$\begin{array}{l}\sigma ({\stackrel{\sim }{CR}}_{t+1,h}^{net,QS})=\\ =\sqrt{\frac{1}{{\beta }_h^2}{\sigma }^2({\stackrel{\sim }{ER}}_{t+1,h}^{gross})+{\sigma }^2({\stackrel{\sim }{LR}}_{t+1,h}^{gross})+\frac{{(C_h^{RE}{B}_{t+1,h}^{RE}CV({\stackrel{\sim }{X}}_{t+1,h}^{RE}))}^2-2{\beta }_{h}Cov({\stackrel{\sim }{X}}_{t+1,h},-\frac{C_h^{RE}{B}_{t+1,h}^{RE}}{E({\stackrel{\sim }{X}}_{t+1,h}^{RE})}{\stackrel{\sim }{X}}_{t+1,h}^{RE})}{{\beta }_h^2{B}_{t+1,h}^2}}\\ =\sqrt{\frac{1}{{\beta }_h^2}{\sigma }^2({\stackrel{\sim }{ER}}_{t+1,h}^{gross})+{\sigma }^2({\stackrel{\sim }{LR}}_{t+1,h}^{gross})+{(\frac{(1-{\beta }_h)}{{\beta }_h}{C}_h^{RE}CV({\stackrel{\sim }{X}}_{t+1,h}))}^2+2\frac{C_h^{RE}{B}_{t+1,h}^{RE}}{{\beta }_{h}E({\stackrel{\sim }{X}}_{t+1,h})}{\sigma }^2({\stackrel{\sim }{LR}}_{t+1,h}^{gross})}\end{array}$$

where we observe the effects of both variability of commissions and negative dependency between commissions and aggregate claims amount. We have indeed that the correlation coefficient is equal to:

$$\text{ρ}\left({\tilde{X}}_{t+1,h}^{RE},{\tilde{C}}_{t+1,h}^{RE}\right)=-\frac{\frac{\left(1-{\text{β}}_h\right)c_h^{RE}{B}_{t+1,h}^{RE}{\text{σ}}^2\left({\tilde{X}}_{t+1,h}\right)}{E\left({\tilde{X}}_{t+1,h}\right)}}{\text{σ}\left({\tilde{X}}_{t+1,h}^{RE}\right)\text{σ}\left({\tilde{C}}_{t+1,h}^{RE}\right)}=-1$$

and also $\text{ρ}\left({\tilde{X}}_{t+1,h},{\tilde{C}}_{t+1,h}^{RE}\right)=-1$, i.e., they are negatively linear dependent, where we remind that ${\text{β}}_h$ denotes the insurer’s retention quota for line $h$.

Furthermore, we will consider Excess of Loss treaty, with a retention for claim unit and no limit to reinsurer exposure. In the case of an Excess of Loss treaty, the stochastic claim amount charged to the reinsurer for year $t$ is:

$${\stackrel{\sim }{X}}_{t+1,h}^{RE}=\underset{j=1}{\overset{{\stackrel{\sim }{K}}_{t+1,h}}{\Sigma }}{\stackrel{\sim }{Z}}_{j,t+1,h}^{RE}=\underset{j=1}{\overset{{\stackrel{\sim }{K}}_{t+1,h}}{\Sigma }}Max(0,{\stackrel{\sim }{Z}}_{j,t+1,h}^{RE}-M_{t+1,h})$$

having denoted by $M_{t+1,h}$ the insurer’s retention limit for year $t+1$. The reinsurer risk premium $P_{t+1,h}^{RE}$ is given by the well-known relationship:

$$P_{t+1,h}^{RE}=E\left({\stackrel{\sim }{X}}_{t+1,h}^{RE}\right)=n_{t+1,h}E\left({\stackrel{\sim }{Z}}_{t+1,h}^{RE}\right)$$

No explicit commission and loss participations are usually provided in the case of the Excess of Loss coverage, so that we get: $B_{t+1,h}^{RE}=P_{t+1,h}^{RE}\left(1+{\text{λ}}_h^{RE}\right)$ and ${\tilde{C}}_{t+1,h}^{RE}=0$, with ${\text{λ}}_h^{RE}$ being the safety loading coefficient applied by reinsurer, usually greater than the safety loading coefficient ${\text{λ}}_h$, as increasing as the insurer’s retention limit is growing up.

In general, the f.g.c. net of XL is equal to:

$${\text{Ψ}}_{{\tilde{Y}}_{t+1,h}^{net}}\left(s\right)=s·\left(B_{t+1,h}-B_{t+1,h}^{RE}\right)-{\text{Ψ}}_{{\tilde{E}}_{t+1,h}}\left(s\right)-{\text{Ψ}}_{{\tilde{X}}_{t+1,h}^{net}}\left(s\right)=$$

from which we can derive cumulants of technical result net of XL in a similar way as the Quota Share case.

5. Numerical Analysis

To show the effect of an Internal Model (IM) based on a Collective Risk Model for Premium risk, two non-life insurance companies with a different dimension are considered (their figures are summed up in Table 2). It is assumed that both insurers underwrite business in the same five lines of business (Accident, Motor Other Damages (MOD), Property, Motor Third-Party Liability and General Third-Party Liability) with the same mix of portfolio (the proportions used resemble the real proportions in the Italian insurance market). The comparison of results will allow us to describe the effect of a different portfolio size on the aggregate claims amount distribution and on the capital requirements.

Table 2. Gross premium volumes of both insurers (amounts in mln of Euro).

LoBs	Omega		Epsilon		Both Insurers
	Bt	Bt+1	Bt	Bt+1	Bt,h/Ʃh Bt,h
Accident	100.0	105.0	10.0	10.5	10.0%
MOD	100.0	105.0	10.0	10.5	10.0%
Property	150.0	157.5	15.0	15.8	15.0%
MTPL	550.0	577.5	55.0	57.8	55.0%
GTPL	100.0	105.0	10.0	10.5	10.0%
TOTAL	1000.0	1050.0	100.0	105.0	100.0%

Table 2. Gross premium volumes of both insurers (amounts in mln of Euro).

LoBs	Omega		Epsilon		Both Insurers
	Bt	Bt+1	Bt	Bt+1	Bt,h/Ʃh Bt,h
Accident	100.0	105.0	10.0	10.5	10.0%
MOD	100.0	105.0	10.0	10.5	10.0%
Property	150.0	157.5	15.0	15.8	15.0%
MTPL	550.0	577.5	55.0	57.8	55.0%
GTPL	100.0	105.0	10.0	10.5	10.0%
TOTAL	1000.0	1050.0	100.0	105.0	100.0%

The main parameters of Collective Risk Model are in Table 3. As we can see, both insurers have the same characteristics apart from the expected number of claims. Omega is assumed to be ten times larger than Epsilon. Safety loading coefficient (λ) and the standard deviation of structure variable (${\text{σ}}_q$) are obtained mainly by Italian market Loss Ratios and Combined Ratios. About $\text{λ}$, it depends by the mean of the empirical combined ratios. It shows a negative value for LoBs where the observed combined ratios are on average greater than one e.g., in GTPL. Furthermore, it is noteworthy to recall that the safety loading is here expressed as a percentage of risk premium. Expense parameters (see $c^M, c^A, {\text{σ}}^M,{\text{σ}}^A$ defined in Section 3) have been calibrated by using the historical pattern of both management and acquisition expenses in the same period. The small values of ${\text{σ}}^M$ and ${\text{σ}}^A$ that will lead to a low variability of expenses producing a low additional capital requirement for expense risk could be noticed. The CV of claim size ($c_z$) is fixed, for each LoB, and calibrated on the Italian market data. Moreover, the expected number of claims ($n_t$) and the expected claim cost ($m_t$) reported in Table 3 for each LoB are referred to the initial year $t$; they will increase in the examined year $t$+1 as described in the previous Section for the dynamic portfolio, according to the annual rate of real growth of portfolio ($g$) as well as to the number of claims and the annual claim inflation rate ($i$) and to claim size, assumed to be almost 2% and 3% respectively for all LoBs in the simulations.

Table 3. Parameters for premium risk.

Insurers	LoBs	$n_t$	${\sigma }_q$	$g$	$m_t$	$c_z$	$i$	$\lambda$	$c^M,$	$c^A,$	${\sigma }^M$	${\sigma }^A$
Omega	Accid.	16,428	15.2%	1.9%	3200	3	3%	27.7%	4.6%	28.2%	0.3%	0.8%
	MOD	25,900	11.1%	1.9%	2500	2	3%	13.9%	4.7%	21.5%	0.4%	1.4%
	Prop.	18,849	6.9%	1.9%	6000	8	3%	−6.4%	4.7%	24.8%	0.6%	0.6%
	MTPL	116,509	8.6%	1.9%	4000	4	3%	−4.0%	4.7%	14.0%	0.7%	0.8%
	GTPL	8225	12.8%	1.9%	10,000	12	3%	−13.1%	4.5%	24.0%	0.8%	1.5%
Epsilon	Accid.	1643	15.2%	1.9%	3200	3	3%	27.7%	4.6%	28.2%	0.3%	0.8%
	MOD	2590	11.1%	1.9%	2500	2	3%	13.9%	4.7%	21.5%	0.4%	1.4%
	Prop.	1885	6.9%	1.9%	6000	8	3%	−6.4%	4.7%	24.8%	0.6%	0.6%
	MTPL	11,651	8.6%	1.9%	4000	4	3%	−4.0%	4.7%	14.0%	0.7%	0.8%
	GTPL	823	12.8%	1.9%	10,000	12	3%	−13.1%	4.5%	24.0%	0.8%	1.5%

Table 3. Parameters for premium risk.

Insurers	LoBs	$n_t$	${\sigma }_q$	$g$	$m_t$	$c_z$	$i$	$\lambda$	$c^M,$	$c^A,$	${\sigma }^M$	${\sigma }^A$
Omega	Accid.	16,428	15.2%	1.9%	3200	3	3%	27.7%	4.6%	28.2%	0.3%	0.8%
	MOD	25,900	11.1%	1.9%	2500	2	3%	13.9%	4.7%	21.5%	0.4%	1.4%
	Prop.	18,849	6.9%	1.9%	6000	8	3%	−6.4%	4.7%	24.8%	0.6%	0.6%
	MTPL	116,509	8.6%	1.9%	4000	4	3%	−4.0%	4.7%	14.0%	0.7%	0.8%
	GTPL	8225	12.8%	1.9%	10,000	12	3%	−13.1%	4.5%	24.0%	0.8%	1.5%
Epsilon	Accid.	1643	15.2%	1.9%	3200	3	3%	27.7%	4.6%	28.2%	0.3%	0.8%
	MOD	2590	11.1%	1.9%	2500	2	3%	13.9%	4.7%	21.5%	0.4%	1.4%
	Prop.	1885	6.9%	1.9%	6000	8	3%	−6.4%	4.7%	24.8%	0.6%	0.6%
	MTPL	11,651	8.6%	1.9%	4000	4	3%	−4.0%	4.7%	14.0%	0.7%	0.8%
	GTPL	823	12.8%	1.9%	10,000	12	3%	−13.1%	4.5%	24.0%	0.8%	1.5%

Characteristics of simulated distribution of losses for Premium risk and for each LoB are reported in Table 4. One million simulations have been applied in order to assure stable convergence. Premium risk, CV, and skewness of the Aggregate amount of next-year claims plus expenses (${\tilde{X}}_{t+1}$ + ${\tilde{E}}_{t+1}$) are figured out.

The high variability of GTPL because of a large variability coefficient of claim-size is noteworthy. Furthermore, the effect of non-pooling risk is significant for MOD and Property. As expected, we have indeed that the bigger insurer shows for several LoB a CV of ${\tilde{X}}_{t+1}$ slightly greater than the value of the standard deviation of ${\text{σ}}_q$ because of the relevant diversification effect. The effect of size is indeed noticeable for Epsilon company where LoBs with high $c_Z$ as Property and GTPL show the greater increase of variability with respect to Omega.

Finally, the aggregate distribution has been derived by assuming a Gaussian Copula function whose parameters have been calibrated by using the correlation matrix proposed by the standard Formula in Technical Specifications of QIS5 (see [16]) and Delegated Acts [1]. We limited the analysis to this simple choice of copula having at its disposal correlation coefficient provided by the Standard Formula, but the evaluation may be properly extended in order to consider both a more significant tail dependency between several LoBs and hierarchical structure based on Archimedean Copulas to aggregate LoBs (see at this regard [21]). Despite the positive correlation provided by Solvency II, we observe in Table 4 a, diversification effect between LoBs.

Table 4. CV and skewness of simulated distribution for each LoB (Gross of Reinsurance).

LoBs	Omega						Epsilon
	${\tilde{X}}_{t+1}$		${\tilde{E}}_{t+1}$		${\tilde{X}}_{t+1}+{\tilde{E}}_{t+1}$		${\tilde{X}}_{t+1}$		${\tilde{E}}_{t+1}$		${\tilde{X}}_{t+1}+{\tilde{E}}_{t+1}$
	CV	Skew.	CV	Skew.	CV	Skew.	CV	Skew.	CV	Skew.	CV	Skew.
Accident	15.34%	0.30	2.53%	0.08	9.49%	0.30	17.01%	0.37	2.53%	0.08	10.52%	0.37
MOD	11.15%	0.22	5.45%	0.18	8.09%	0.21	11.89%	0.23	5.44%	0.18	8.60%	0.22
Property	9.00%	0.95	2.88%	0.15	6.52%	0.92	19.66%	6.56	2.88%	0.15	14.16%	6.52
MTPL	8.68%	0.18	5.40%	0.19	7.18%	0.17	9.39%	0.21	5.39%	0.20	7.76%	0.21
GTPL	18.26%	2.84	5.87%	0.18	13.65%	2.79	42.14%	12.87	5.87%	0.18	31.34%	12.82
Total	5.87%	0.24	2.62%	0.15	4.55%	0.23	7.86%	2.73	2.62%	0.15	6.07%	2.69

Table 4. CV and skewness of simulated distribution for each LoB (Gross of Reinsurance).

LoBs	Omega						Epsilon
	${\tilde{X}}_{t+1}$		${\tilde{E}}_{t+1}$		${\tilde{X}}_{t+1}+{\tilde{E}}_{t+1}$		${\tilde{X}}_{t+1}$		${\tilde{E}}_{t+1}$		${\tilde{X}}_{t+1}+{\tilde{E}}_{t+1}$
	CV	Skew.	CV	Skew.	CV	Skew.	CV	Skew.	CV	Skew.	CV	Skew.
Accident	15.34%	0.30	2.53%	0.08	9.49%	0.30	17.01%	0.37	2.53%	0.08	10.52%	0.37
MOD	11.15%	0.22	5.45%	0.18	8.09%	0.21	11.89%	0.23	5.44%	0.18	8.60%	0.22
Property	9.00%	0.95	2.88%	0.15	6.52%	0.92	19.66%	6.56	2.88%	0.15	14.16%	6.52
MTPL	8.68%	0.18	5.40%	0.19	7.18%	0.17	9.39%	0.21	5.39%	0.20	7.76%	0.21
GTPL	18.26%	2.84	5.87%	0.18	13.65%	2.79	42.14%	12.87	5.87%	0.18	31.34%	12.82
Total	5.87%	0.24	2.62%	0.15	4.55%	0.23	7.86%	2.73	2.62%	0.15	6.07%	2.69

Table 5 shows SCR ratio obtained by IM as the capital requirement for Premium risk divided by initial gross premium volume. According to Omega, as expected, the highest ratios are registered for the line GTPL (65.3%) due mainly to its large variability (CV = 13.7%). Property and MTPL show high ratios too (respectively 26.7% and 24.8%). The large safety loadings lead to lower ratios for MOD (11.9%) and Accident (9.1%). Focusing on Epsilon, the effect of pooling risk is clearly noticeable on Premium risk capital charges.

Table 5. SCR ratio $\left(\frac{SC{R}_{99.5\text{\%}}}{B_t}\right)$ (Gross of Reinsurance).

LoBs	Omega				Epsilon
	SCR Ratio	SCR Ratio (λ = 0)	SCR Ratio (No Exp. Risk)	SCR Ratio (SF)	SCR Ratio	SCR Ratio (λ = 0)	SCR Ratio (No Exp. Risk)	SCR Ratio (SF)
Accident	9.08%	24.4%	8.99%	26.78%	12.19%	27.5%	12.11%	26.78%
MOD	11.93%	21.4%	11.59%	25.20%	13.41%	22.9%	13.07%	25.20%
Property	26.65%	21.6%	26.53%	25.20%	66.58%	61.5%	66.50%	25.20%
MTPL	24.81%	21.3%	24.68%	31.50%	26.81%	23.3%	26.64%	31.50%
GTPL	65.32%	54.0%	65.27%	44.10%	168.82%	157.5%	168.79%	44.10%
Total	19.35%	17.0%	19.25%	22.78%	30.76%	28.2%	30.66%	22.78%

Table 5. SCR ratio $\left(\frac{SC{R}_{99.5\text{\%}}}{B_t}\right)$ (Gross of Reinsurance).

LoBs	Omega				Epsilon
	SCR Ratio	SCR Ratio (λ = 0)	SCR Ratio (No Exp. Risk)	SCR Ratio (SF)	SCR Ratio	SCR Ratio (λ = 0)	SCR Ratio (No Exp. Risk)	SCR Ratio (SF)
Accident	9.08%	24.4%	8.99%	26.78%	12.19%	27.5%	12.11%	26.78%
MOD	11.93%	21.4%	11.59%	25.20%	13.41%	22.9%	13.07%	25.20%
Property	26.65%	21.6%	26.53%	25.20%	66.58%	61.5%	66.50%	25.20%
MTPL	24.81%	21.3%	24.68%	31.50%	26.81%	23.3%	26.64%	31.50%
GTPL	65.32%	54.0%	65.27%	44.10%	168.82%	157.5%	168.79%	44.10%
Total	19.35%	17.0%	19.25%	22.78%	30.76%	28.2%	30.66%	22.78%

As expected, the effect of expenses is not significant on the capital requirement for Premium risk. Finally, neglecting safety loading (i.e., assuming λ = 0), SCR is significantly greater for Accident and MOD (where λ > 0). By contrast, the choice of Standard Formula to not consider safety loading seems to be less prudential for most important LoBs, but it is influenced by the phase of the underwriting cycle. The SCR ratio for only Premium Risk, derived by applying the “market-wide approach” of the Standard Formula (SF) (see Section 2), is also reported in Table 5.

Both insurers have the same ratios for each LoB when SF is applied because of the lack of a size factor. The total SCR ratio, derived by the SF, is also equal for both insurers having assumed the same mix of portfolio. It is interesting to compare this ratio to the results obtained by the IM. A consistent comparison could be developed only by considering the case of λ = 0 because, as previously mentioned, the Standard Formula neglects safety loading in capital requirement evaluation. We observe a saving of capital by using the Internal Model for Omega, while a significant increase of capital is requested for the smaller insurer if IM is used.

Main differences are justified by considering that volatility factor used in the Standard Formula have been calibrated on the European market, while main parameters of the Internal Model have been derived by considering the risk profile of each specific insurer.

Exploring deeply the differences between IM and SF, some key points could be captured.

(a)

In the Internal Model, we are considering also the volatility of expenses, neglected by the Standard Formula. Main results confirm that the effect of expenses is not very significant for the LoB analyzed.

(b)

For Omega, the standard deviations of ($X/B$) of Accident (8.1%), MOD (7.2%), MTPL (7.4%) and Property (6.8%) are lower than volatility factors provided by the Standard Formula (see Table 1). A greater value is indeed observed for GTPL (15.1% against a volatility factor of 14%). For Epsilon, the high variability coefficient of severity distribution and a low expected number of claims lead to very high standard deviation of ($X/B$) for Property (15%) and GTPL (36%) when IM is applied.

(c)

Because of the skewness of the overall aggregate distribution, for both insurers, the ratio between 99.5% quantile less the mean and the standard deviation is very far from the multiplier equal to 3 fixed by the Standard Formula. The implicit multiplier, derived by IM as $\frac{Va{R}_{0.995}\left({{\displaystyle \sum }}_{h=1}^L{\tilde{X}}_{t+1,h}\right)-{{\displaystyle \sum }}_{h=1}^L{P}_{t+1,h}}{\sigma \left({{\displaystyle \sum }}_{h=1}^L{\tilde{X}}_{t+1,h}\right)}$, is equal to 2.76 for Omega and to 3.15 for Epsilon.

6. The Effect of Alternative Reinsurance Strategies

The model has been also applied net of reinsurance in order to compare the effect on capital requirement of different reinsurance treaties. For each line of business, we assume evaluating the following reinsurance strategies:

-

QSF1: Quota Share treaties with a retention ${\text{β}}_h$ equal to 90% for Accident and MOD, 80% for Property, 95% for MTPL and 85% for GTPL and a fixed commission applied to reinsurer premiums and equal to the expected expense ratio. In this case we have $c_h=c_h^{RE}$.

-

QSF2: Quota Share treaties with the same retentions ${\text{β}}_h$ of QSF1 and a fixed commission applied to reinsurer premiums and equal to 80% of the expected expense ratio. In this case we have $c_h^{RE}=0.8 c_h$.

-

QSS1: Quota Share treaties with the same retentions ${\text{β}}_h$ of QSF1 and a sliding commission applied to reinsurer premiums. Provisional and expected commission rate is equal to 80% of the expected expense ratio $E\left(c_h^{RE}\right)=0.8 c_h$, while the effective percentage varies according to the observed loss experience as provided by Formula (5).

-

QSS2: Quota Share treaties with the same retentions ${\text{β}}_h$ of QSF1 and a sliding commission applied to reinsurer premiums. Provisional and expected commission rate is equal to 80% of the expected expense ratio $E\left(c_h^{RE}\right)=0.8 c_h$. The commissions are adjusted also in this case according to the observed loss ratio. We build up five bins of width 10% and we modify the percentage according to the ratio between the average value of the classes where the observed loss ratio falls and the expected loss ratio. According to this classification, we assume a maximum value equal to the expected loss ratio plus 25% and a minimum value equal to the expected loss ratio less 25%. The excesses due to loss ratios outside the limits of the scale (above or below) are not taken into account in the calculation of commission rate. This structure implicitly defines a minimum and a maximum commission.

-

XL: an XL treaty for each LoB with a retention limit equal to $M_{t+1,h}=E\left({\tilde{Z}}_{t+1,h}\right)+15\text{σ}\left({\tilde{Z}}_{t+1,h}\right)$. Safety loading coefficient ${\text{λ}}_h^{RE}$ of the reinsurer is equal to the safety loading coefficient of insurer, proportionally increased to take into account the savings of variability coefficient of the insurer because of reinsurance.

-

XLQS: a QS treaty with retention and sliding commissions equal to QSS2 for Accident, MOD and Property and a XL treaty with retention limit and safety loadings equal to XL1 for MTPL and GTPL.

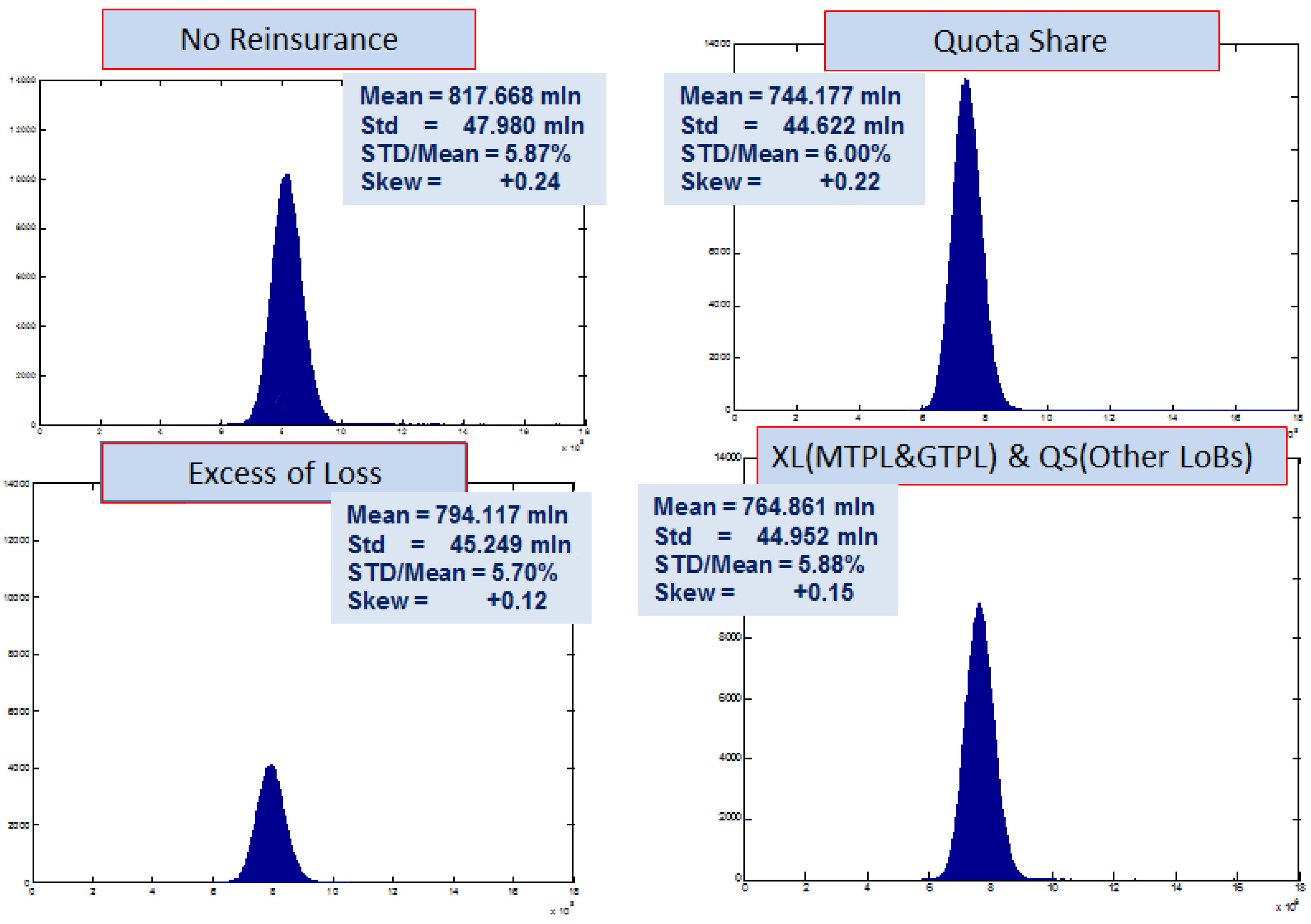
Figure 1. Distribution of aggregate claim amount of Total Portfolio for Omega according to different reinsurance strategies.

Figure 1. Distribution of aggregate claim amount of Total Portfolio for Omega according to different reinsurance strategies.

For the sake of simplicity, we report in Figure 1 and Figure 2 only the aggregated distribution of aggregate claim amount of gross and net of reinsurance respectively in order to catch the effect of several treaties on the shape of distribution. Quota Share treaty intuitively leads to a variability coefficient and a skewness similar to the gross reinsurance case. We do not have the same CV because the different retentions between Lines of business lead to a different mix of portfolio with respect to reinsurance cases. We have instead a greater effect on CV and skewness when a XL treaty is used. Finally, the choice of different treaties between long-tail business and other LoBs leads to results similar to XL because of the high weight of MTPL on the total portfolio.

Figure 2. Distribution of aggregate claim amount of Total Portfolio for Epsilon according to different reinsurance strategies.

Figure 2. Distribution of aggregate claim amount of Total Portfolio for Epsilon according to different reinsurance strategies.

Table 6. CV and skewness of simulated distribution for each LoB (Gross and Net of Reinsurance).

LoBs	Omega
	$CV\left({\tilde{X}}_{t+1}\right)$				$\gamma \left({\tilde{X}}_{t+1}\right)$
	No Reins	QS	XL	XLQS	No Reins	QS	XL	XLQS
Accident	15.34%	15.34%	15.29%	15.34%	0.30	0.30	0.30	0.30
MOD	11.15%	11.15%	11.14%	11.15%	0.22	0.22	0.22	0.22
Property	9.00%	9.00%	7.69%	9.00%	0.95	0.95	0.17	0.95
MTPL	8.68%	8.68%	8.65%	8.65%	0.18	0.18	0.18	0.18
GTPL	18.26%	18.26%	14.27%	14.27%	2.84	2.84	0.18	0.18
Total	5.87%	6.00%	5.70%	55.88%	0.24	0.22	0.12	0.15
LoBs	Epsilon
	$CV\left({\tilde{X}}_{t+1}\right)$				$\gamma \left({\tilde{X}}_{t+1}\right)$
	No Reins	QS	XL	XLQS	No Reins	QS	XL	XLQS
Accident	17.01%	17.01%	16.54%	17.01%	0.37	0.37	0.32	0.37
MOD	11.89%	11.89%	11.79%	11.89%	0.23	0.23	0.22	0.23
Property	19.66%	19.66%	12.92%	19.66%	6.56	6.56	0.34	6.56
MTPL	9.39%	9.39%	9.09%	9.09%	0.21	0.21	0.17	0.17
GTPL	42.14%	42.14%	23.28%	23.28%	12.87	12.87	0.22	0.22
Total	7.86%	7.75%	6.37%	6.69%	2.73	2.31	0.06	0.12

Table 6. CV and skewness of simulated distribution for each LoB (Gross and Net of Reinsurance).

LoBs	Omega
	$CV\left({\tilde{X}}_{t+1}\right)$				$\gamma \left({\tilde{X}}_{t+1}\right)$
	No Reins	QS	XL	XLQS	No Reins	QS	XL	XLQS
Accident	15.34%	15.34%	15.29%	15.34%	0.30	0.30	0.30	0.30
MOD	11.15%	11.15%	11.14%	11.15%	0.22	0.22	0.22	0.22
Property	9.00%	9.00%	7.69%	9.00%	0.95	0.95	0.17	0.95
MTPL	8.68%	8.68%	8.65%	8.65%	0.18	0.18	0.18	0.18
GTPL	18.26%	18.26%	14.27%	14.27%	2.84	2.84	0.18	0.18
Total	5.87%	6.00%	5.70%	55.88%	0.24	0.22	0.12	0.15
LoBs	Epsilon
	$CV\left({\tilde{X}}_{t+1}\right)$				$\gamma \left({\tilde{X}}_{t+1}\right)$
	No Reins	QS	XL	XLQS	No Reins	QS	XL	XLQS
Accident	17.01%	17.01%	16.54%	17.01%	0.37	0.37	0.32	0.37
MOD	11.89%	11.89%	11.79%	11.89%	0.23	0.23	0.22	0.23
Property	19.66%	19.66%	12.92%	19.66%	6.56	6.56	0.34	6.56
MTPL	9.39%	9.39%	9.09%	9.09%	0.21	0.21	0.17	0.17
GTPL	42.14%	42.14%	23.28%	23.28%	12.87	12.87	0.22	0.22
Total	7.86%	7.75%	6.37%	6.69%	2.73	2.31	0.06	0.12

Analyzing the effects on aggregate claim amount for each LoB (see Table 6), we observe a similar behavior of proportional and non-proportional treaties for Accident and MOD while a greater saving of variability and skewness is provided by XL for LoBs with a greater $c_Z$ as MTPL, GTPL and Property. Because of a higher pooling risk, the relative effect of non-proportional treaties is higher for Epsilon. We have in this case that aggregated CV moves from 7.9% to 6.3% and aggregated skewness varies from 2.73 to 0.06 when a XL treaty is applied.

In order to consider the effect of pricing of the treaties, we evaluate the characteristics of Combined Ratio distribution. As previously described, several QS treaties are considered with the same retention and different commission rates. We report in Table 7 simulated characteristics of Combined Ratio (CR) of total portfolio for both insurers. It is noteworthy that the high number of simulations (1 million) assured a strong convergence of simulated moments to the exact ones. Some negligible differences are observed for high skewed LoB (as GTPL) of small companies.

Table 7. Characteristics of Combined Ratio distribution for both insurers (Total Portfolio—Gross and Net Reinsurance).

LoBs	Stats	No Reins	QSF1 $c^{re}=c$ Fixed Comm.	QSF2 $c^{re}=0.8c$ Fixed Comm.	QSS1 $E\left(c^{re}\right)=0.8c$ Sliding Comm.	QSS2 $E\left(c^{re}\right)=0.8c$ Classes (Min, Max)	XL	QSXL
Omega	Mean	101.29%	101.24%	101.77%	101.77%	101.77%	101.81%	102.20%
	St. Dev.	4.61%	4.73%	4.73%	4.81%	4.80%	4.47%	4.67%
	Skew.	0.23	0.21	0.21	0.22	0.20	0.11	0.14
Epsilon	Mean	101.29%	101.24%	101.77%	101.77%	101.77%	102.21%	102.40%
	St. Dev.	6.15%	6.09%	6.09%	6.27%	6.20%	5.01%	5.33%
	Skew.	2.69	2.27	2.25	2.39	2.15	0.05	0.11

Table 7. Characteristics of Combined Ratio distribution for both insurers (Total Portfolio—Gross and Net Reinsurance).

LoBs	Stats	No Reins	QSF1 $c^{re}=c$ Fixed Comm.	QSF2 $c^{re}=0.8c$ Fixed Comm.	QSS1 $E\left(c^{re}\right)=0.8c$ Sliding Comm.	QSS2 $E\left(c^{re}\right)=0.8c$ Classes (Min, Max)	XL	QSXL
Omega	Mean	101.29%	101.24%	101.77%	101.77%	101.77%	101.81%	102.20%
	St. Dev.	4.61%	4.73%	4.73%	4.81%	4.80%	4.47%	4.67%
	Skew.	0.23	0.21	0.21	0.22	0.20	0.11	0.14
Epsilon	Mean	101.29%	101.24%	101.77%	101.77%	101.77%	102.21%	102.40%
	St. Dev.	6.15%	6.09%	6.09%	6.27%	6.20%	5.01%	5.33%
	Skew.	2.69	2.27	2.25	2.39	2.15	0.05	0.11

According to gross of reinsurance distribution, we observe an average CR on the portfolio greater than one because of negative safety loadings in Property, MTPL and GTPL. As already showed for aggregate claim amount characteristics, a higher variability and skewness for Epsilon is confirmed.

Furthermore, the different results related to simulated distribution of combined ratios net of reinsurance can be compared. In particular, in the case of XL strategy, the distribution is heavily affected by reinsurer pricing with a higher combined ratio. On the other hand, this treaty allows the highest reduction of variability and skewness. With regard to proportional treaties, we observe the greater CV in the case of sliding commissions (QSS1) because of both the variance of $\tilde{C}$^re and the dependence with the aggregate claim amount. A very slight reduction of variability and skewness with respect to QSS1 is observed when the QSS2 methodology is considered. In this case, sliding commissions are based on fixed classes with a minimum and a maximum value where if the observed loss ratio falls outside the range, these excesses are not considered in the commissions. The effect is more noticeable for Epsilon because of the higher variability of the company.

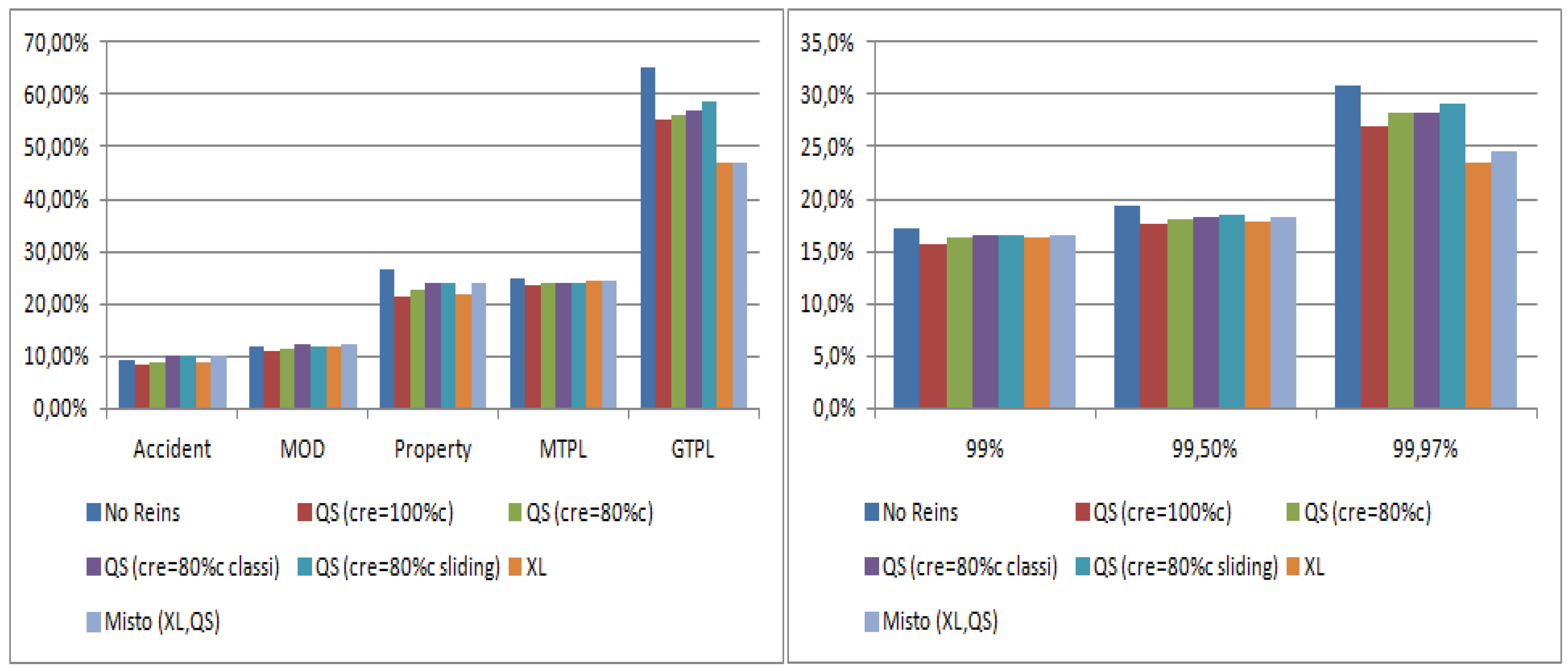
Moving to SCR for Premium risk for Omega, we observe in Figure 3 how all strategies reduce the required capital, but they bring it into effect in a rather different way. In the case of the Quota Share, with fixed commissions equal to expenses loading, we have a reduction of required capital for each LoB equal to the quota to be reinsured $\left(1-{\text{β}}_{\text{h}}\right)$. Other Quota Share treaties are more realistic by assuming lower commission rates or variable commissions, but the unfavorable pricing and the greater variability lead to a reduced saving of capital requirement. The XL strategy is clearly depressing the expected technical results. The assumed XL coverage is indeed more expensive than QS coverage, but it is more effective on reducing the downside risk. In general, it provides a greater saving of capital except when compared to the Quota Share QSF1 with fixed commission rate so that $c^{re}=c$.

The ratio between total capital requirement for Premium Risk and gross premiums ranges indeed between 17.55% of QSF1 Treaty to 19.35% of Gross of Reinsurance case. As expected for lines with high variability and skewness as GTPL, XL is the most efficient treaty, despite the high pricing. For this LoB, the SCR net XL is indeed 47% of gross premiums against a ratio of 65% evaluated gross reinsurance, while the reinsurer applies a safety loading coefficient ${\text{λ}}^{RE}$ equal to roughly 54% of ceded risk premiums for GTPL.

Figure 3. SCR ratio for each LoB and Total SCR ratio according to different reinsurance strategies (Omega insurer).

Figure 3. SCR ratio for each LoB and Total SCR ratio according to different reinsurance strategies (Omega insurer).

Figure 4. SCR ratio for each LoB and Total SCR ratio according to different reinsurance strategies (Epsilon insurer).

Figure 4. SCR ratio for each LoB and Total SCR ratio according to different reinsurance strategies (Epsilon insurer).

When the smaller company is considered (Figure 4), we have noticeable differences between proportional and non-proportional treaties. With respect to a capital ratio gross of reinsurance of roughly 31%, QS treaties settle around 27%–29%, while XL shows a ratio of 21.5%. In this treaty, despite the greater safety loading of reinsurer (${\text{λ}}^{RE}$) for Omega, the higher the reduction of pooling risk, the greater is the saving of capital. We have indeed that in this case, not only GTPL but also Property shows a significant reduction of capital when XL is applied (78% and 35% of premiums for SCR^net against 169% and 67% gross of reinsurance).

Furthermore, we can observe how XL treaties appear very efficient when higher confidence levels are taken into account. For example, when a confidence level of 99.97% is considered, the gross SCR ratio is respectively 31% and 79% for Omega and Epsilon, while the ratio net of XL is equal to 23% and 29% a roughly 15% and 64% less for the two companies. It is clear how the different dimensions lead to different effects when non-proportional treaties are considered.

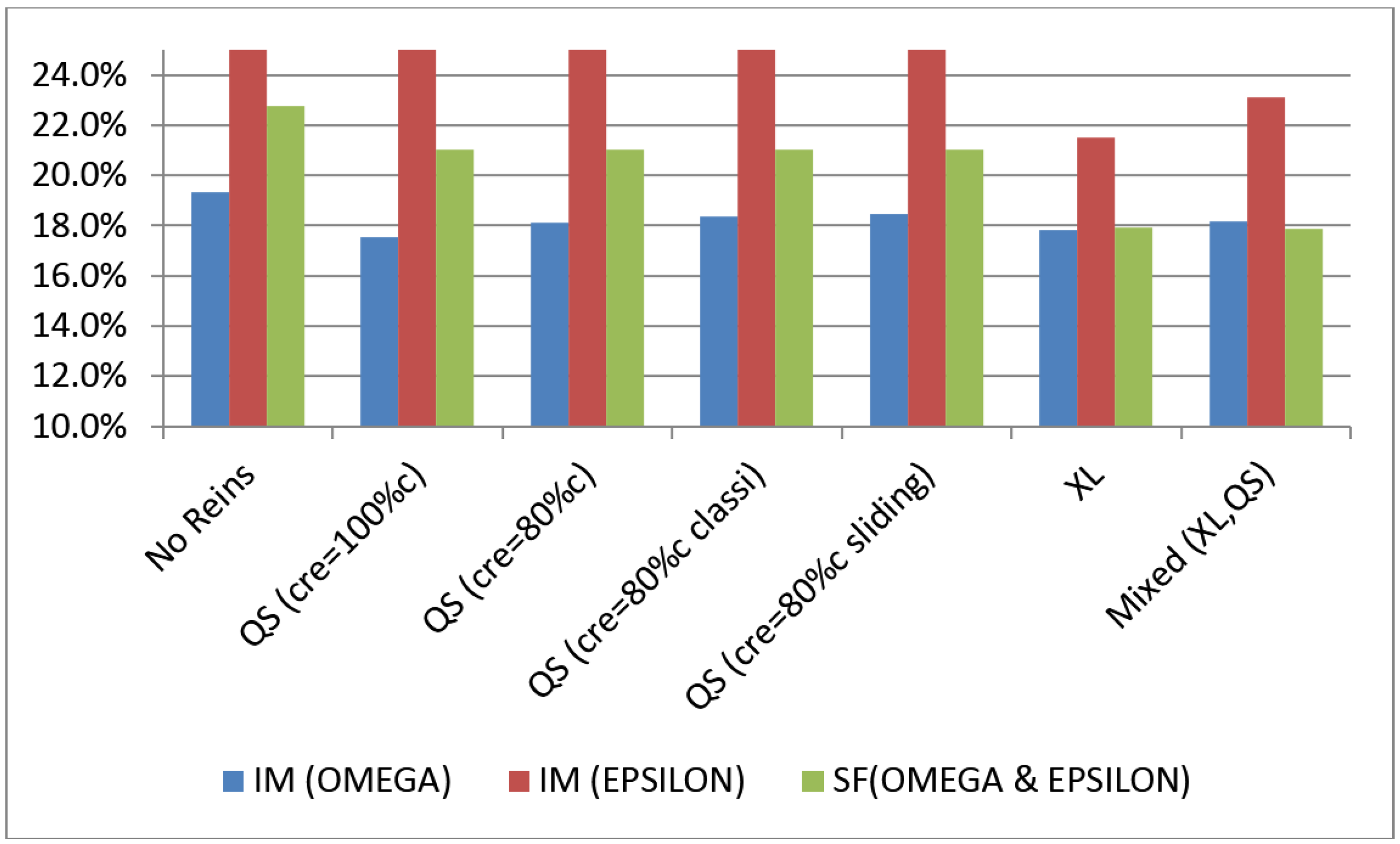
Finally the IM capital requirements can be compared with those obtained by the Standard Formula also for net of reinsurance cases (see Figure 5).

Figure 5. SCR ratios for both insurers derived by Internal Model and market-wide Standard Formula.

Figure 5. SCR ratios for both insurers derived by Internal Model and market-wide Standard Formula.

Both insurers show again the same ratio when SF is considered because of the same mix of portfolio and the same reinsurance strategies. This result emphasizes another pitfall of the market-wide formula that provides, through the fixed NP_lob factor, the same effect of non-proportional reinsurance despite a different size of portfolio. This factor, being independent by the characteristics of the XL treaty (as for example the attachment point of the layer), assumes for some LoBs a greater saving of variability with respect to the effective reduction obtained by analyzing the distribution of aggregate claim amount. We have indeed that the ratio between the variability coefficients net and gross of reinsurance for MTPL is equal to 99.6% for Omega and to 96.7% for Epsilon, while SF allows a NP_lob equal to 80% for this LoB. Considering instead the GTPL, we derive IM ratios equal to respectively 78% and 56% for the insurers because of the high variability of this LoB. This overestimation of the effect of XL, provided by the Standard Formula for MTPL, shows a poor convenience in the development of the Internal Model for Omega when this treaty is applied. On the other hand, the SF provides a significant underestimation of capital requirement when the small insurer is considered.

Moving to Quota Share treaties, the effect of different commission rates is not considered by the Standard Formula that leads to the same capital ratio for all proportional treaties here analyzed.

7. Conclusions

A reliable comparison of different reinsurance covers provided by the real market makes the insurer able to identify the most appropriate strategic planning. Starting from the Collective Risk Theory approach, we extend the relations in order to consider proportional or non-proportional reinsurance strategies. By considering several Quota-Share treaties scenarios, we derive the exact characteristics of combined ratio distribution by considering the effect of alternative methodology on providing ceding commissions.

Moreover, the Monte Carlo Simulation technique has allowed for the comparison of the effect on capital requirements of different strategies. This technique provides a useful insight of the whole complex risk process, with special advantages in cases of portfolios with a large skewness of the loss distribution, whereas the use of approximation formulas are not reliable.

The proposed theoretical model is clearly a simplified version of a more complex model that should be built up, but here suitable analyses about primary insurance aspects have been preferred. In particular, we have focused on the mitigation effect of reinsurance on underwriting Premium Risk, neglecting the additional capital requirement needed to cover the default risk of the reinsurer, since the latter depends clearly on reinsurer reliability as a risk factor and only in terms of volume on the ceded business.

The comparison with the Standard Formula, defined by Delegated Acts, has allowed us to emphasize some technical weaknesses of the market-wide approach, such as the lack of size factor, the use of a default value of the non-proportional factor and the replacement of the LogNormal assumption with a fixed multiplier.