Highly Sensitive Quartz-Based Sensing System for the Detection of Subpercentage Changes in the Relative Permittivity of Liquids Flowing in Microchannels
Abstract
:1. Introduction
2. Materials and Methods
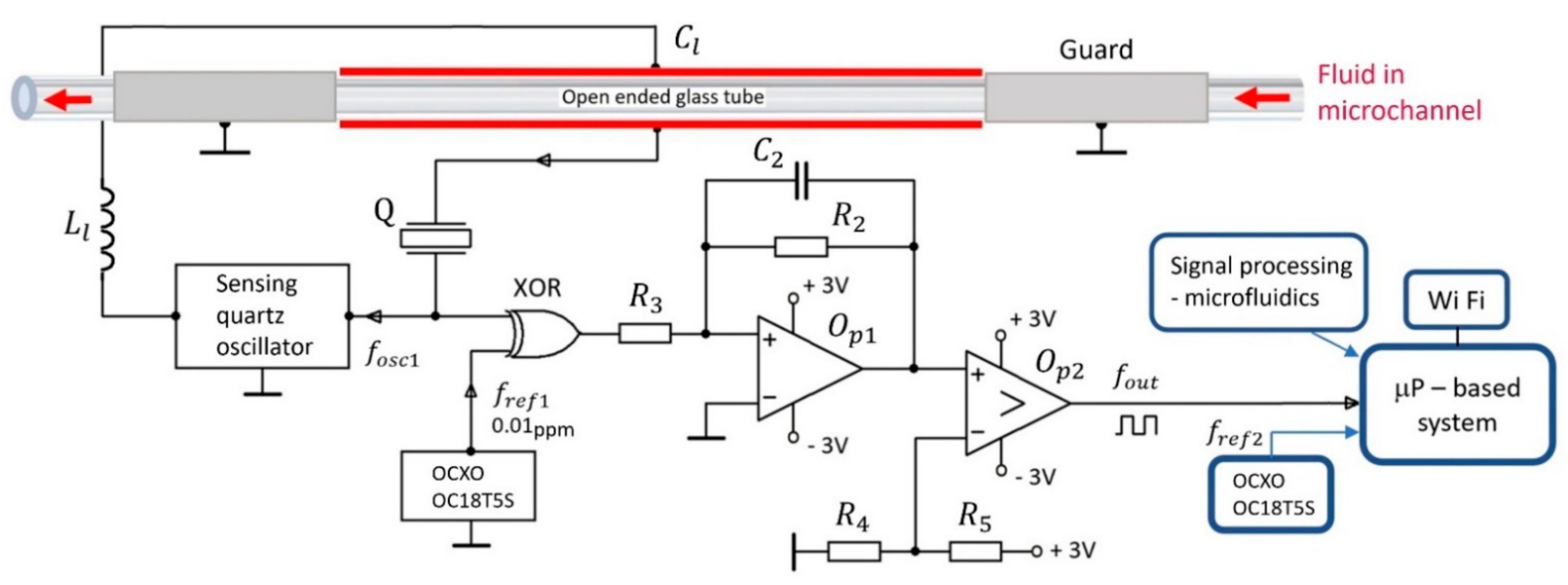
2.1. Experimental Setup
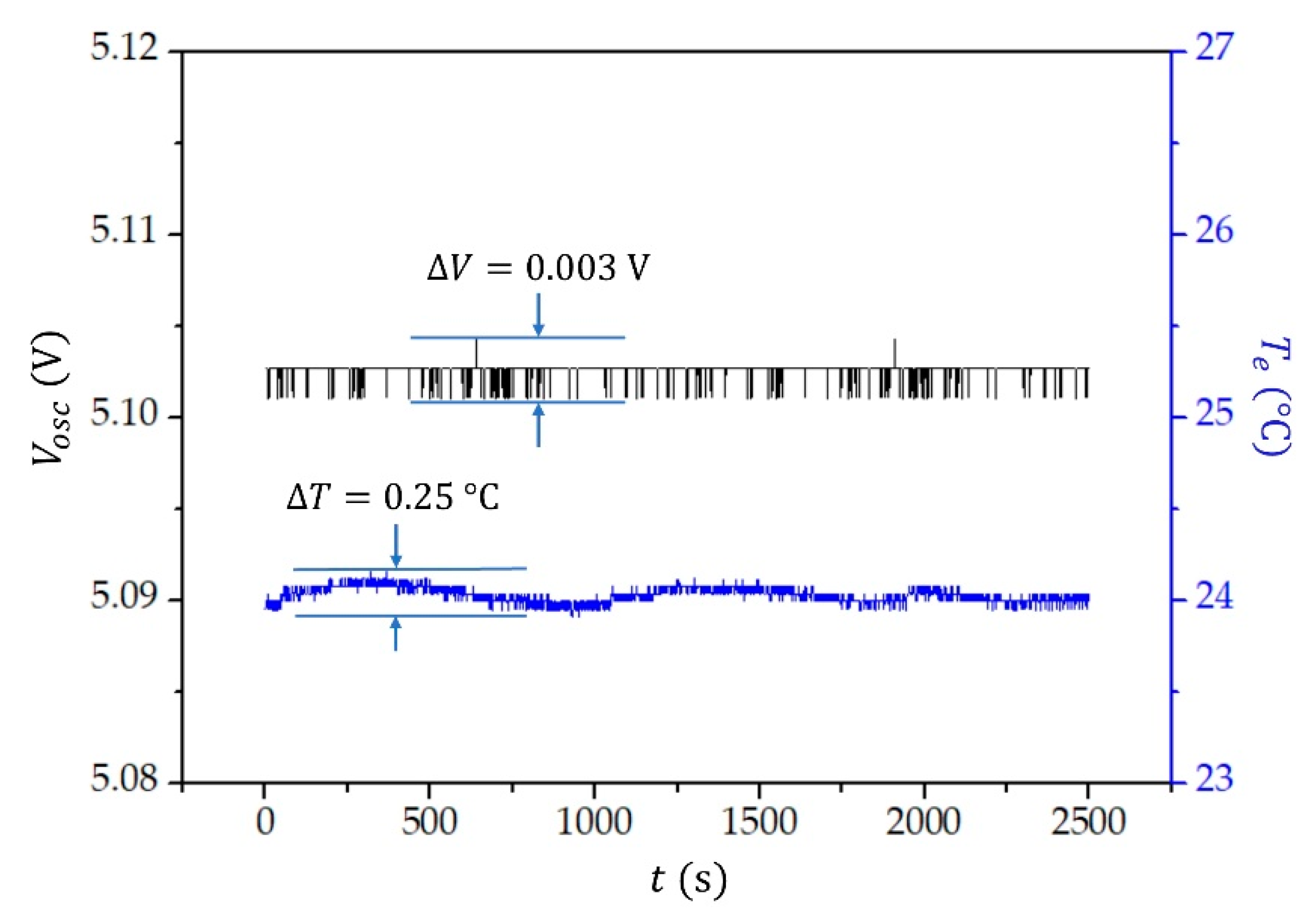
2.1.1. Temperature Stability of the Quartz Oscillator
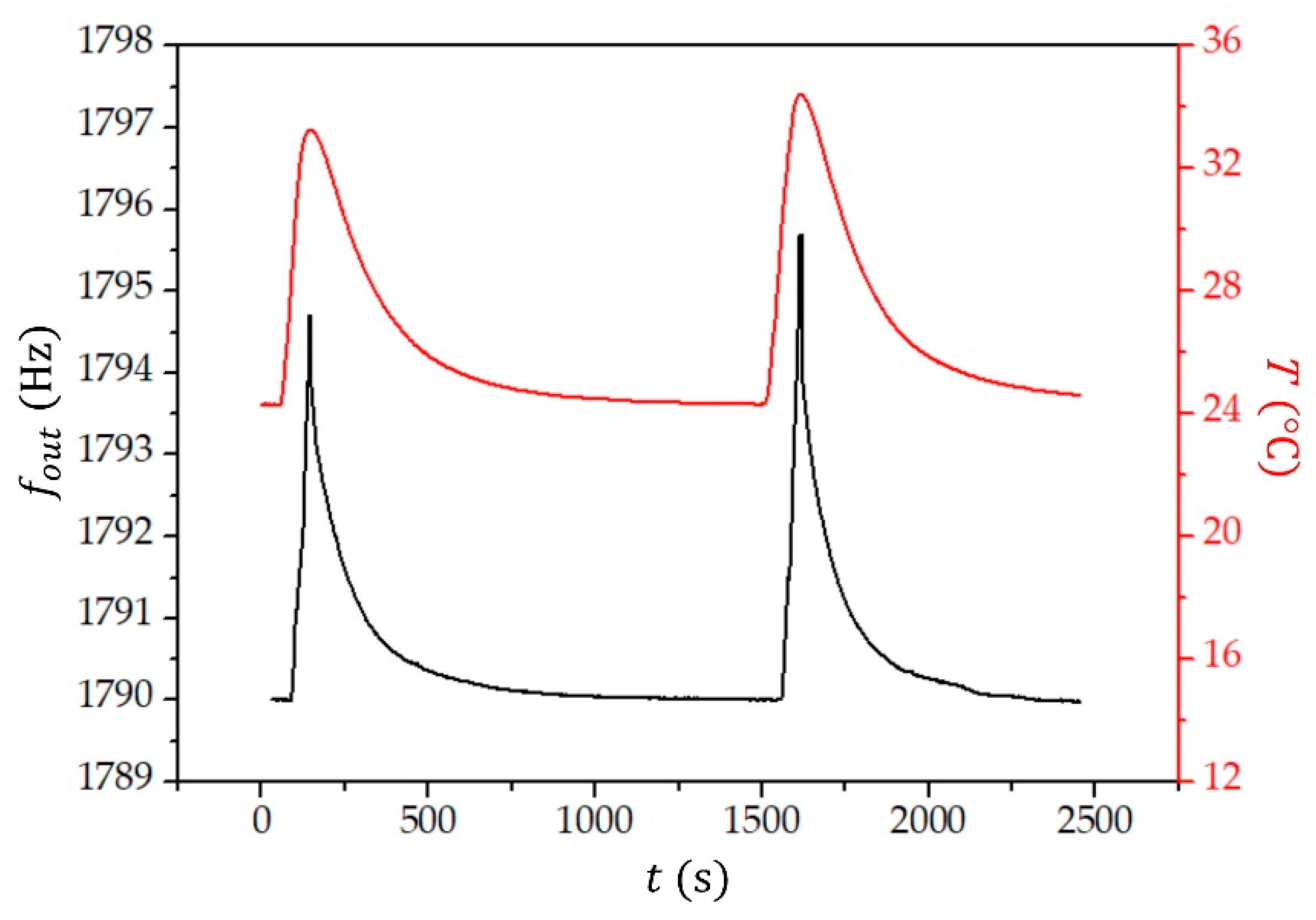
2.1.2. Influence of Quartz Crystal Aging on the Frequency Change
2.1.3. Frequency Measurement Error
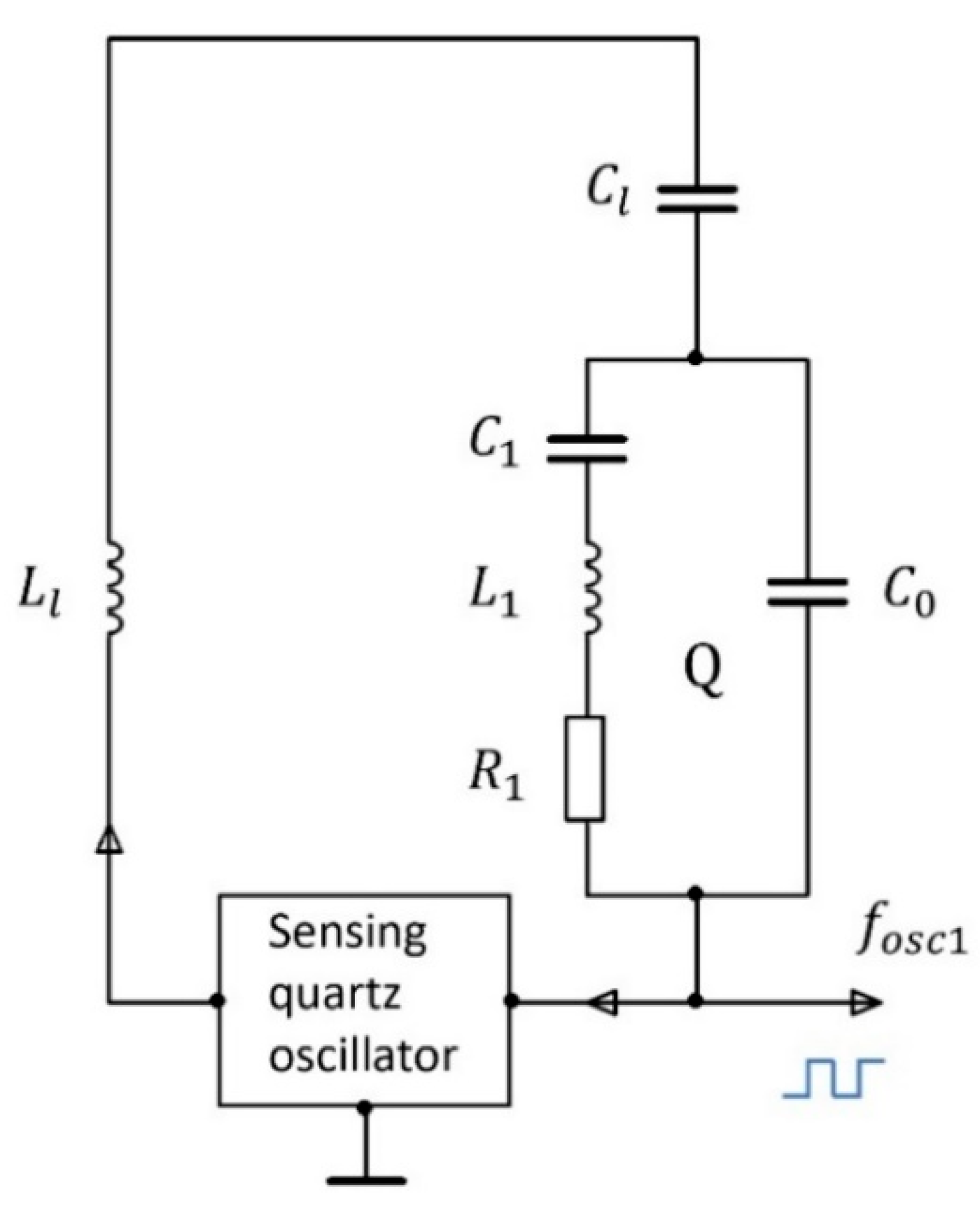
2.2. Influence of the Liquid Impedance on the Quartz Crystal Resonant Frequency
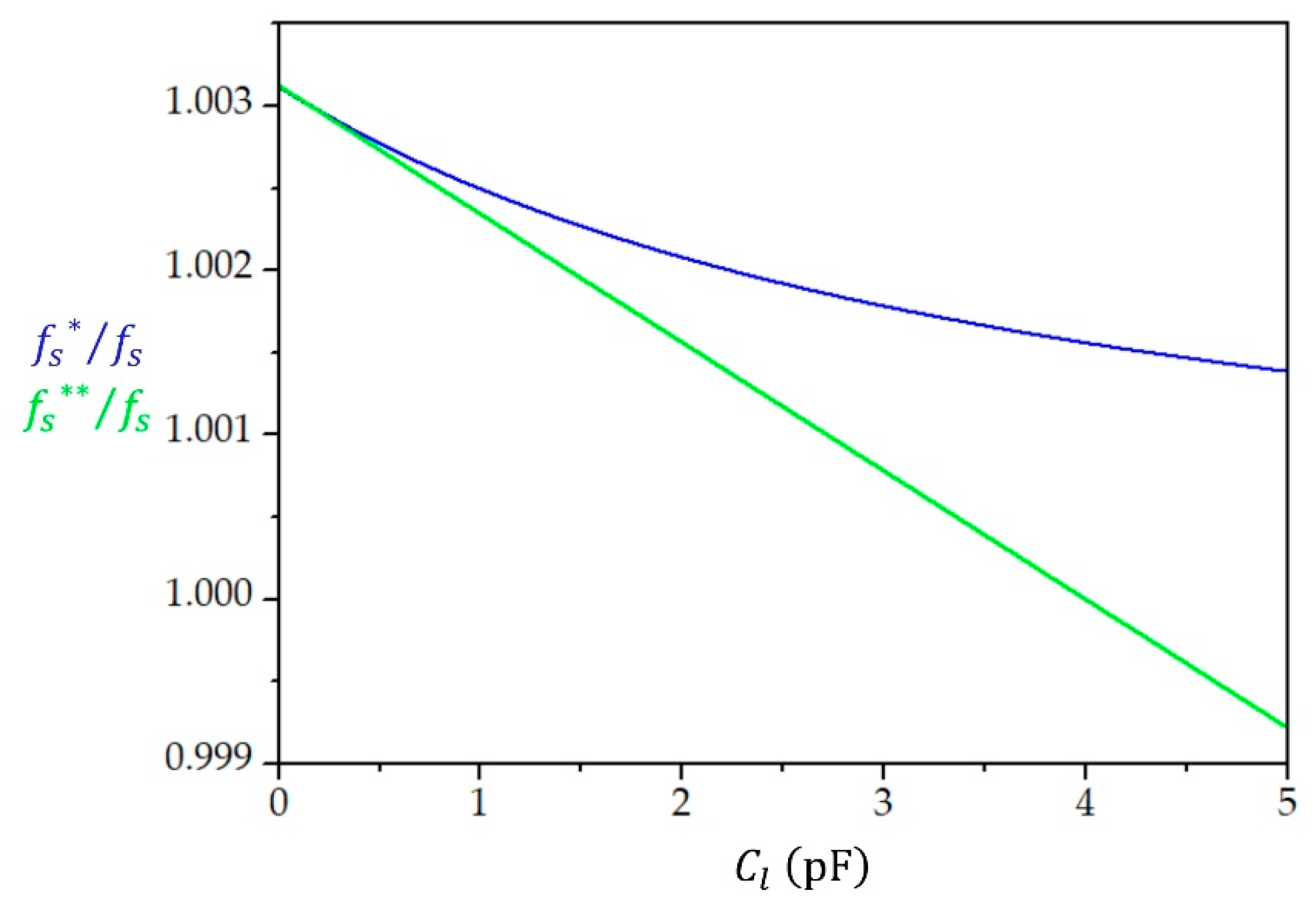
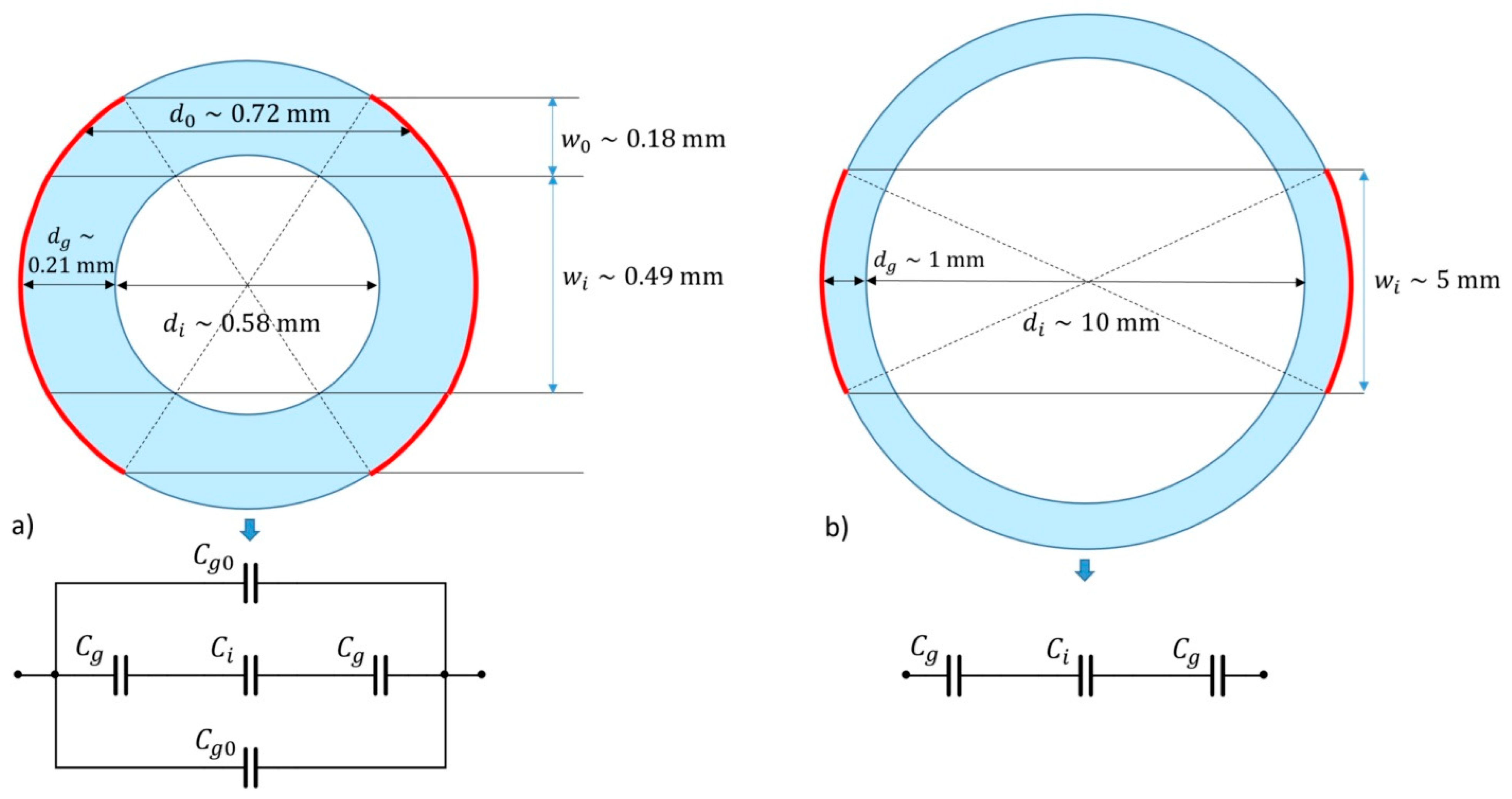
2.3. Dependence of the Load Capacitance on the Relative Permittivity of the Investigated Liquid
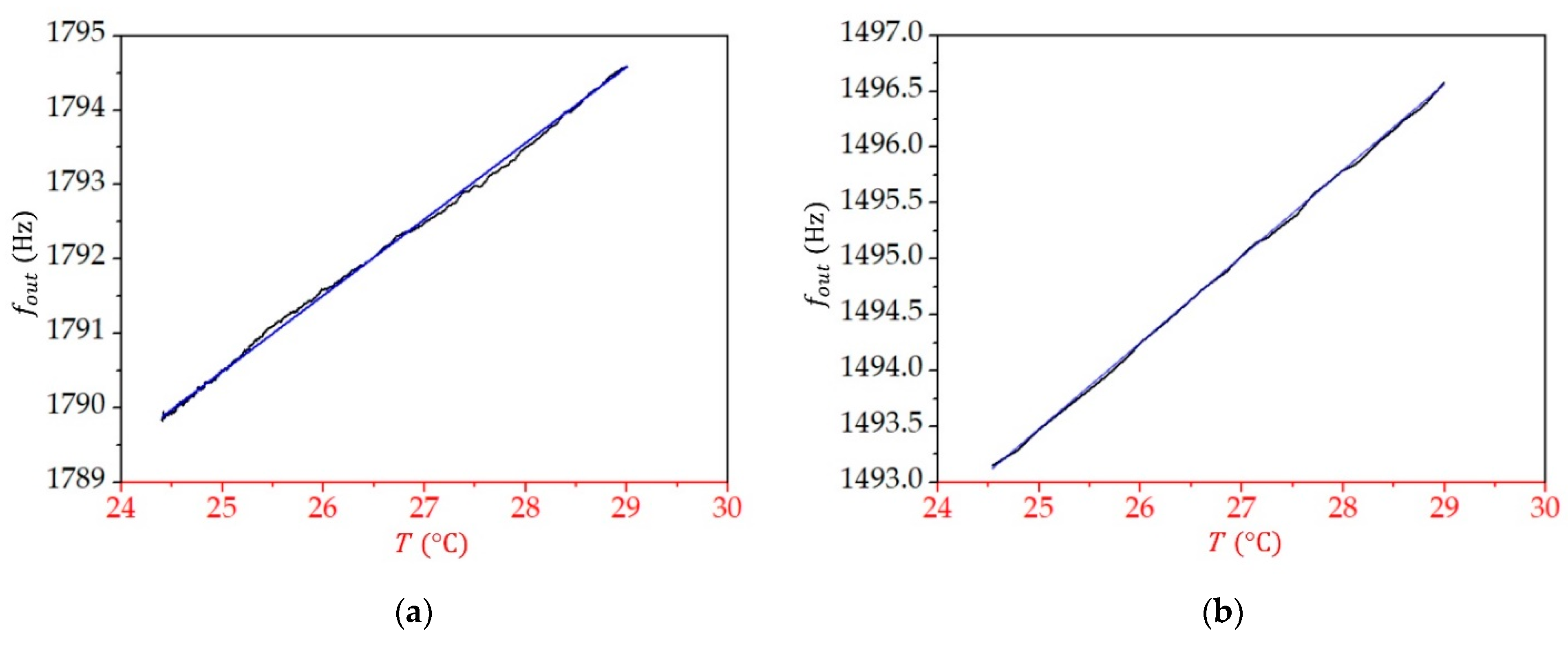
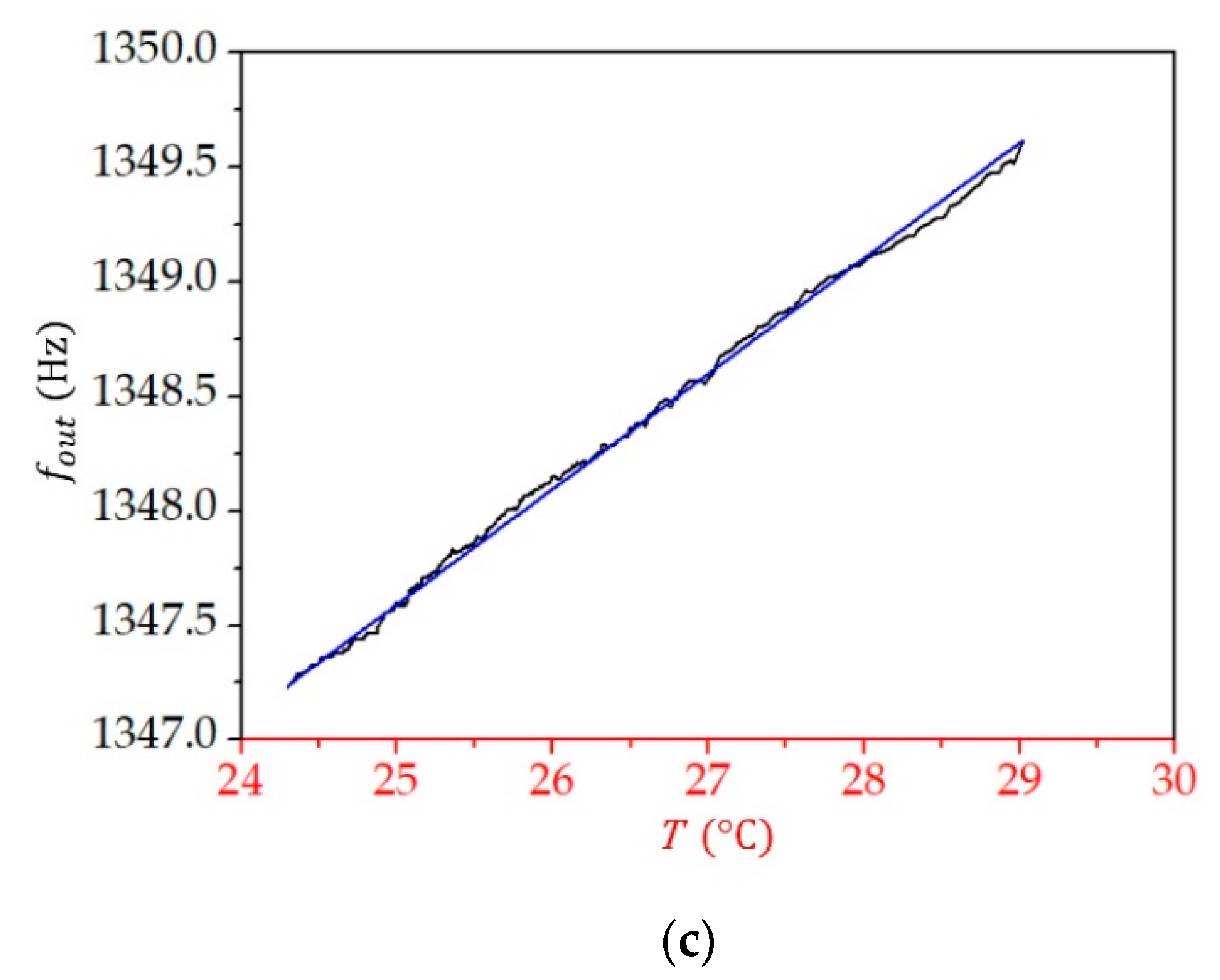
2.4. Temperature Dependence of Relative Permittivity
3. Measurements
4. Discussion
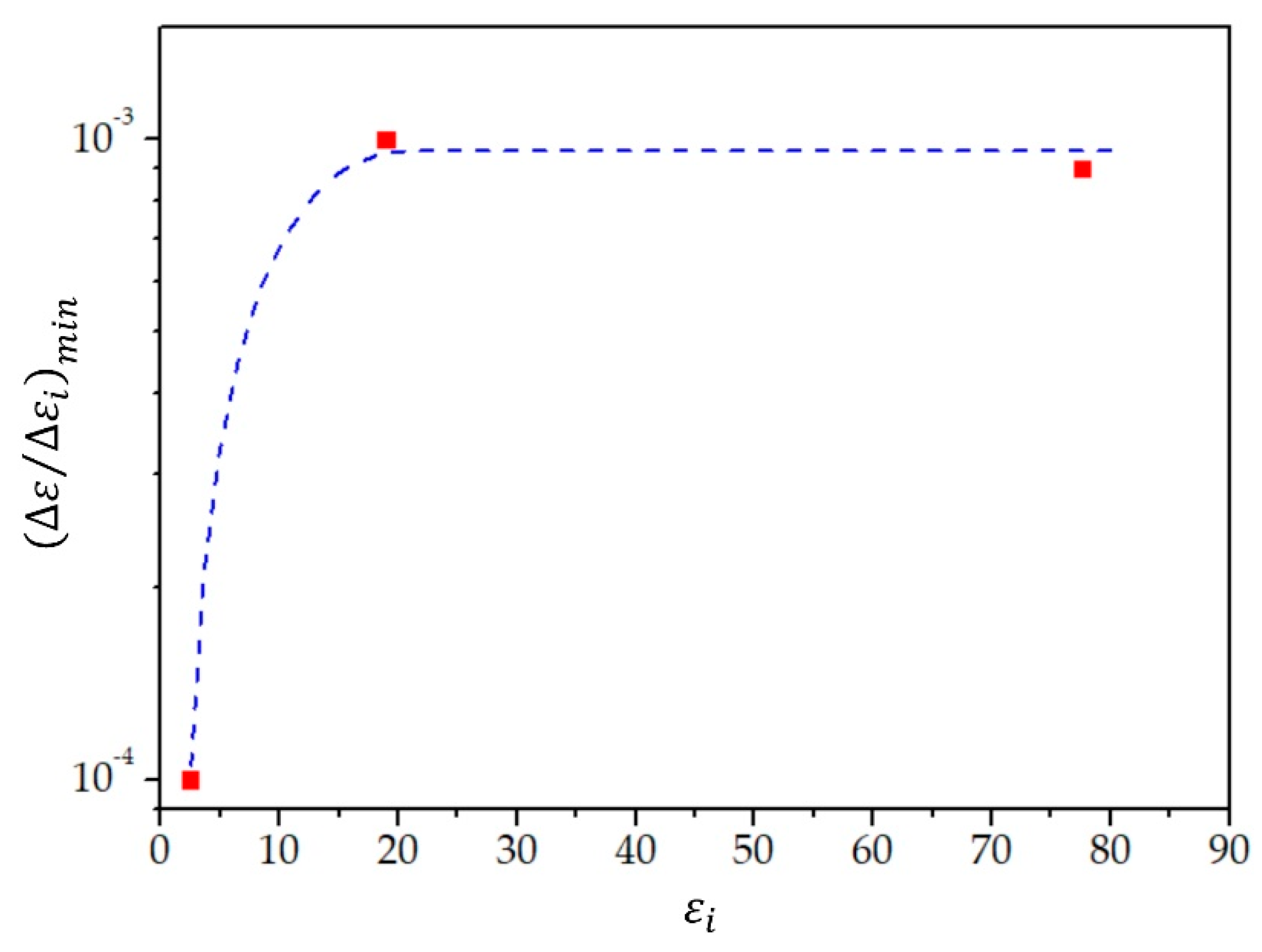
4.1. Sensitivity of the Method
4.2. Detection of Changes in Mixture of Liquids
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Jain, V.; Hole, A.; Deshmukh, R.; Patrikar, R. Dynamic capacitive sensing of droplet parameters in a low-cost open EWOD system. Sens. Actuators A Phys. 2017, 263, 224–233. [Google Scholar] [CrossRef]
- Bilican, I.; Bahadir, T.; Bilgin, K.; Guler, M.T. Alternative screening method for analyzing the water samples through an electrical microfluidics chip with classical microbiological assay comparison of P. aeruginosa. Talanta 2020, 219, 121293. [Google Scholar] [CrossRef] [PubMed]
- Ghafar-Zadeh, E.; Sawan, M.; Chodavarapu, V.P.; Hosseini-Nia, T. Bacteria Growth Monitoring Through a Differential CMOS Capacitive Sensor. IEEE Trans. Biomed. Circuits Syst. 2010, 4, 232–238. [Google Scholar] [CrossRef] [PubMed]
- Stagni, C.; Guiducci, C.; Benini, L.; Ricco, B.; Carrara, S.; Samori, B.; Paulus, C.; Schienle, M.; Augustyniak, M.; Thewes, R. CMOS DNA Sensor Array With Integrated A/D Conversion Based on Label-Free Capacitance Measurement. IEEE J. Solid State Circuits 2006, 41, 2956–2964. [Google Scholar] [CrossRef]
- Iyuke, S.; Ntombenhle Hlongwane, G.; Daramola Michael, O.; Dodoo-Arhin, D.; Moothi, K.; Wamwangi, D. DNA hybridisation sensors for product authentication and tracing: State of the art and challenges. S. Afr. J. Chem. Eng. 2019, 27, 16–34. [Google Scholar]
- Nguyen, N.-T.; Wereley, S.T.; Shaegh, S.A.M. Fundamentals and Applications of Microfluidics, 3rd ed.; Artech House: Norwood, MA, USA, 2019. [Google Scholar]
- Ramana, C.V.V.; Kumar, A.B.V.K.; Kumar, A.S.; Kumar, M.A.; Moodley, M.K. Dielectric and excess dielectric constants in non polar + polar binary liquid mixtures of toluene with alcohols at 303, 313 and 323 K. Thermochim. Acta 2013, 566, 130–136. [Google Scholar] [CrossRef]
- El Khaled, D.; Novas, N.; Gázquez, J.A.; García, R.M.; Manzano-Agugliaro, F. Alcohols and alcohols mixtures as liquid biofuels: A review of dielectric properties. Renew. Sustain. Energy Rev. 2016, 66, 556–571. [Google Scholar] [CrossRef]
- Offenzeller, C.; Hintermüller, M.A.; Hilber, W.; Jakoby, B. A dielectric coating for improved performance of capacitive sensors in all-polymer microfluidic devices. Microelectron. Eng. 2020, 223, 111220. [Google Scholar] [CrossRef]
- Hintermüller, M.A.; Offenzeller, C.; Knoll, M.; Hilber, W.; Jakoby, B. Embedded screen-printed transducers in bulk polymer microfluidic devices. Eingebettete Siebgedruckte Transducer Polym. Mikrofluidischen Syst. 2020, 137, 128. [Google Scholar] [CrossRef] [Green Version]
- Migoń, D.; Wasilewski, T.; Suchy, D. Application of QCM in Peptide and Protein-Based Drug Product Development. Molecules 2020, 25, 3950. [Google Scholar] [CrossRef] [PubMed]
- Pohanka, M. Overview of Piezoelectric Biosensors, Immunosensors and DNA Sensors and Their Applications. Materials 2018, 11, 448. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Li, B.-R.; Shen, M.-Y.; Yu, H.-H.; Li, Y.-K. Rapid construction of an effective antifouling layer on a Au surface via electrodeposition. Chem. Commun. 2014, 50, 6793–6796. [Google Scholar] [CrossRef] [PubMed]
- Matko, V.; Safaric, R. Major Improvements of Quartz Crystal Pulling Sensitivity and Linearity Using Series Reactance. Sensors 2009, 9, 8263–8270. [Google Scholar] [CrossRef] [PubMed]
- Matko, V.; Milanovič, M. Detection Principles of Temperature Compensated Oscillators with Reactance Influence on Piezoelectric Resonator. Sensors 2020, 20, 802. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Euroquartz. Crystal Theory. Available online: https://euroquartz.co.uk/media/1879/tech-notes.pdf (accessed on 26 April 2021).
- Euroquartz. SC-Cut Crystal Reference Oscillator OCXO-OC18T5S. Available online: https://www.wdi.ag/specs/euroquartz/oszillatoren/ocxo/OC18T5S.pdf (accessed on 26 March 2021).
- Vig, J.R. Quartz Crystal Resonators and Oscillators for Frequency Control and Timing Applications. IEEE Ferroelectric and Frequency Control Society. Available online: https://ieee-uffc.org/download/quartz-crystal-resonators-and-oscillators-for-frequency-control-and-timing-applications-a-tutorial-2/ (accessed on 26 March 2021).
- Statek. The Quartz Crystal Model and Its Frequencies. Technical Note 32. Available online: http://statek.com/wp-content/uploads/2018/03/tn32.pdf (accessed on 26 April 2021).
- Schrüfer, E. Electrical Measurement: Quartz as a Frequency Reference, 5th ed.; Carl Hanser Verlag: München, Germany, 1992; pp. 405–414. [Google Scholar]
- Matko, V. Next Generation AT-Cut Quartz Crystal Sensing Devices. Sensors 2011, 11, 4474–4482. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Wang, S.-Y.; Neubig, B.; Sato, K.; Hosoda, T.; Seydel, E.; Wu, J.-H.; Ma, T.-F.; Wang, J. Aging models and parameters of quartz crystal resonators and oscillators. In Proceedings of the 2015 Symposium on Piezoelectricity, Acoustic Waves, and Device Applications (SPAWDA), Jinan, China, 5 January 2015. [Google Scholar] [CrossRef]
- Shuyu, L.; Jie, X. Effect of the Matching Circuit on the Electromechanical Characteristics of Sandwiched Piezoelectric Transducers. Sensors 2017, 17, 329. [Google Scholar]
- Stanford. QCM100-Quartz Microbalance Theory and Calibration. Available online: http://www.thinksrs.com/downloads/PDFs/ApplicationNotes/QCMTheoryapp.pdf (accessed on 26 March 2021).
- Lide, R.D. CRC Handbook of Chemistry and Physics: Permittivity of Liquids; CRC Press LLC: Boca Raton, FL, USA, 2005; pp. 153–175. [Google Scholar]
- Prasad, T.V.; Vishwam, T.; Manjula, V.; Raju, K.C.J.; Aparna, Y. Dielectric relaxation and thermodynamic study of Dimethylformamide/1,4-Butanediol binary mixtures in the temperature range 298K–323K. Phys. B Phys. Condens. Matter 2020, 588, 412142. [Google Scholar] [CrossRef]
- Zhuang, B.; Ramanauskaite, G.; Koa, Z.Y.; Wang, Z.-G. Like dissolves like: A first-principles theory for predicting liquid miscibility and mixture dielectric constant. Sci. Adv. 2021, 7, 1–7. [Google Scholar] [CrossRef] [PubMed]




| Silica glass | Iron glass | |||
| Microchannel | Test tube | Microchannel | Test tube | |
| Water | ||||
| 2-Propanol | ||||
| Toluene | 0.17 | 0.26 | 0.19 | |
| Liquid | ||||
|---|---|---|---|---|
| Toluene | ||||
| 2-Propanol | ||||
| Water |
| 0 | 24.10 | 24.10 |
| 0.1 | 20.91 | 19.14 |
| 0.2 | 17.95 | 15.20 |
| 0.3 | 15.22 | 12.06 |
| 0.4 | 12.71 | 9.58 |
| 0.5 | 10.43 | 7.61 |
| 0.6 | 8.37 | 6.04 |
| 0.7 | 6.54 | 4.80 |
| 0.8 | 4.93 | 3.81 |
| 0.9 | 3.55 | 3.02 |
| 1 | 2.40 | 2.40 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Matko, V.; Milanovič, M. Highly Sensitive Quartz-Based Sensing System for the Detection of Subpercentage Changes in the Relative Permittivity of Liquids Flowing in Microchannels. Chemosensors 2021, 9, 230. https://doi.org/10.3390/chemosensors9080230
Matko V, Milanovič M. Highly Sensitive Quartz-Based Sensing System for the Detection of Subpercentage Changes in the Relative Permittivity of Liquids Flowing in Microchannels. Chemosensors. 2021; 9(8):230. https://doi.org/10.3390/chemosensors9080230
Chicago/Turabian StyleMatko, Vojko, and Miro Milanovič. 2021. "Highly Sensitive Quartz-Based Sensing System for the Detection of Subpercentage Changes in the Relative Permittivity of Liquids Flowing in Microchannels" Chemosensors 9, no. 8: 230. https://doi.org/10.3390/chemosensors9080230
APA StyleMatko, V., & Milanovič, M. (2021). Highly Sensitive Quartz-Based Sensing System for the Detection of Subpercentage Changes in the Relative Permittivity of Liquids Flowing in Microchannels. Chemosensors, 9(8), 230. https://doi.org/10.3390/chemosensors9080230







