The Effect of Counterions on the Detection of Cu2+ Ions in Aqueous Solutions Using Quartz Tuning Fork (QTF) Sensors Modified with L-Cysteine Self-Assembled Monolayers: Experimental and Quantum Chemical DFT Study
Abstract
1. Introduction
2. Materials and Experimental Details
2.1. Chemicals and Materials
2.2. Experimental Setup and Instrumentation
2.3. Experimental Methods
2.4. Density Functional Theory (DFT) Calculations
3. Results and Discussion
3.1. Resonance Frequency Measurements of Au-Coated QTFs Functionalized with L-Cysteine at the Different Concentrations of CuSO4, CuCl2, and Cu(NO3)2
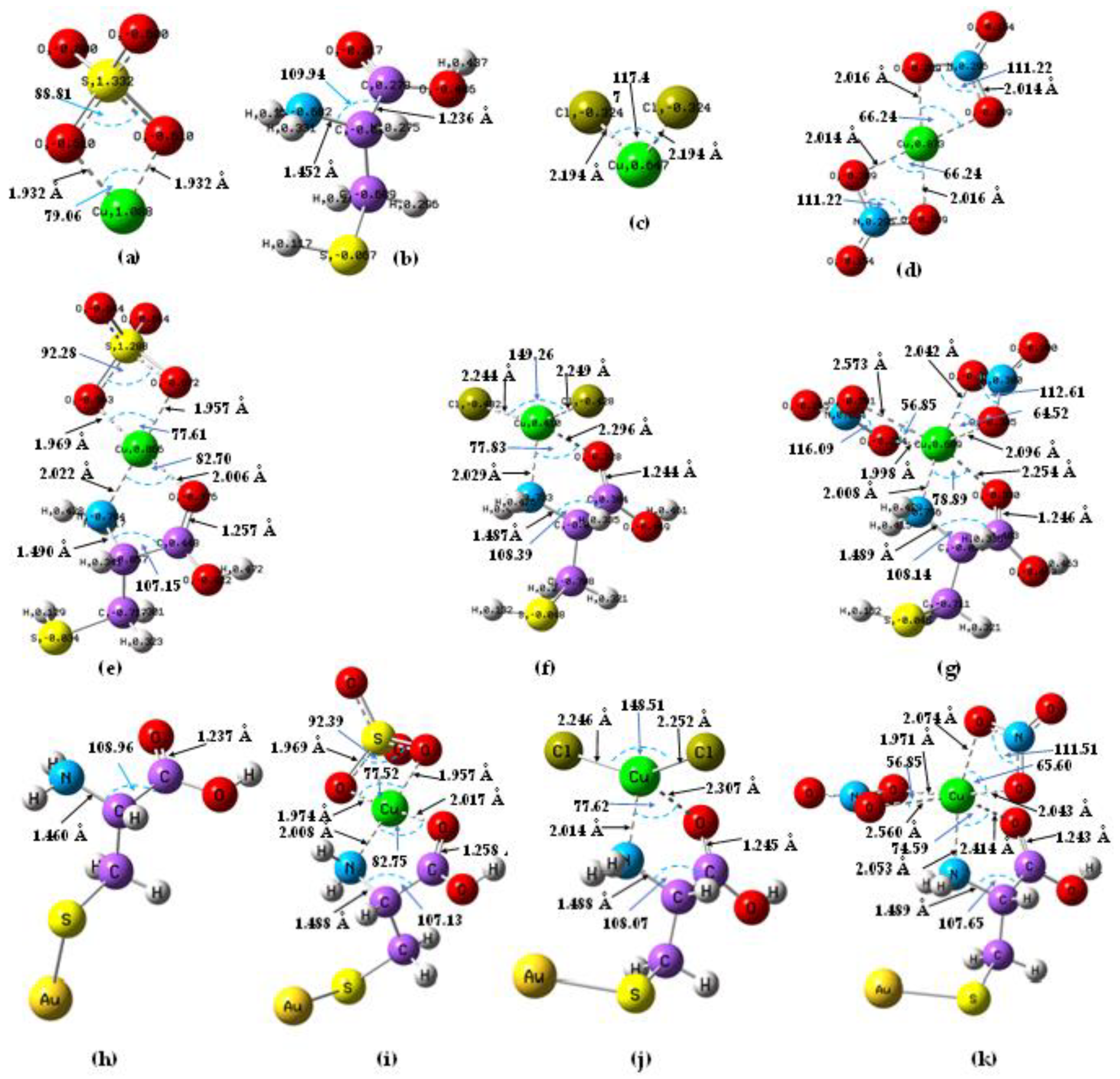
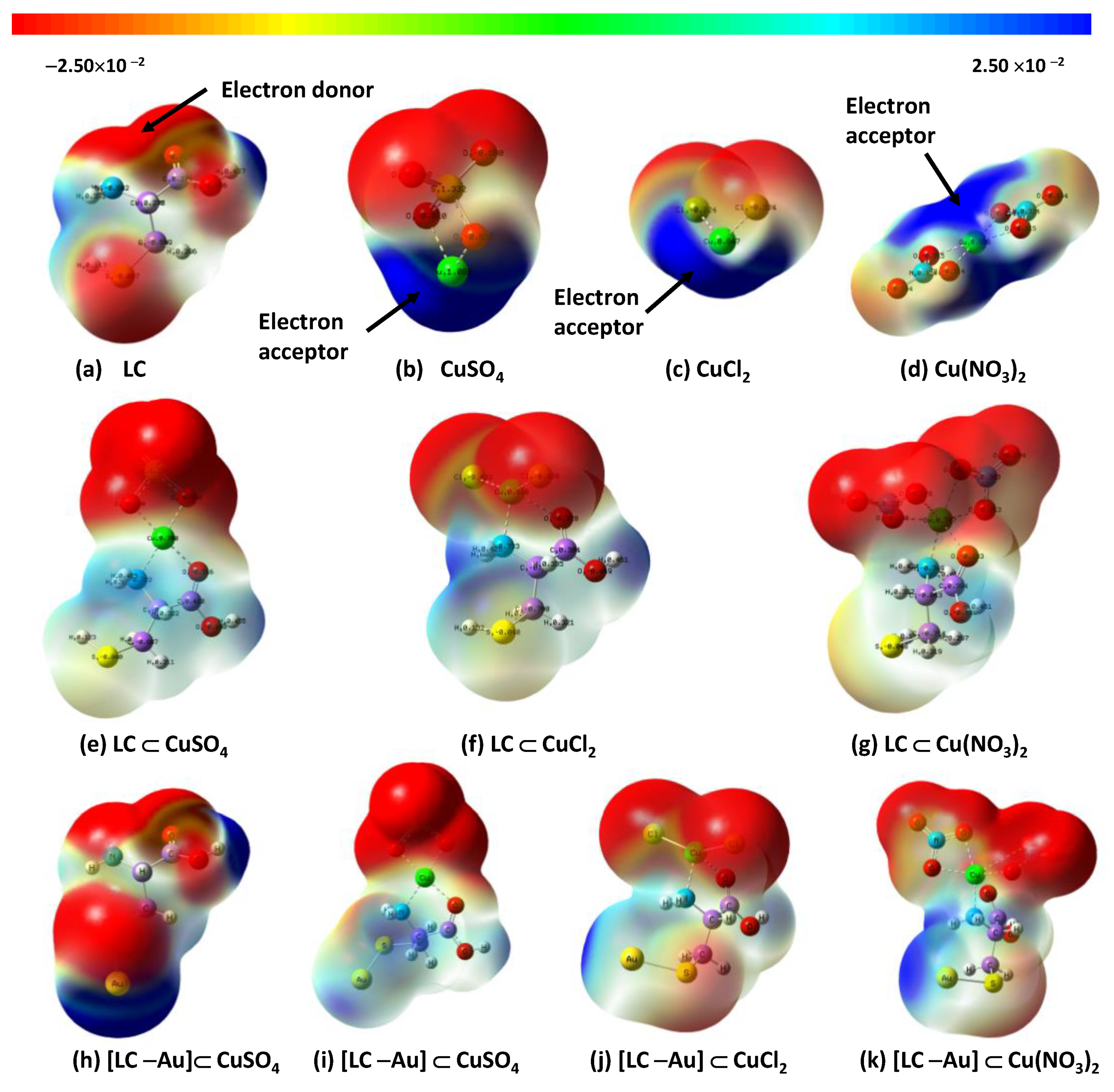
3.2. Quantum Chemical DFT Calculations
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Yoshinari, N.; Kuwamura, N.; Kojima, T.; Konno, T. Development of coordination chemistry with thiol-containing amino acids. Coord. Chem Rev. 2023, 474, 214857. [Google Scholar] [CrossRef]
- Pearson, R.G. Hard and soft acids and bases. J. Am. Chem. Soc. 1963, 85, 3533–3539. [Google Scholar] [CrossRef]
- Pearson, R.G.; Songstad, J. Application of the principle of hard and soft acids and bases to organic chemistry. J. Am. Chem. Soc. 1967, 89, 1827–1836. [Google Scholar] [CrossRef]
- Häkkinen, H. The gold–sulfur interface at the nanoscale. Nat. Chem. 2012, 4, 443–455. [Google Scholar] [CrossRef]
- Love, J.C.; Estroff, L.A.; Kriebel, J.K.; Nuzzo, R.G.; Whitesides, G.M. Self-assembled monolayers of thiolates on metals as a form of nanotechnology. Chem. Rev. 2005, 105, 1103–1169. [Google Scholar] [CrossRef]
- Boisselier, E.; Astruc, D. Gold nanoparticles in nanomedicine: Preparations, imaging, diagnostics, therapies and toxicity. Chem. Soc. Rev. 2009, 38, 1759–1782. [Google Scholar] [CrossRef]
- Xue, Y.; Li, X.; Li, H.; Zhang, W. Quantifying thiol–gold interactions towards the efficient strength control. Nat. Commun. 2014, 5, 1–9. [Google Scholar] [CrossRef]
- Ruiz, L.M.; Libedinsky, A.; Elorza, A.A. Role of copper on mitochondrial function and metabolism. Front. Mol. Biosci. 2021, 8, 711227. [Google Scholar] [CrossRef]
- Festa, R.A.; Thiele, D.J. Copper: An essential metal in biology. Curr. Biol. 2011, 21, R877–R883. [Google Scholar] [CrossRef]
- Kodama, H.; Fujisawa, C. Copper metabolism and inherited copper transport disorders: Molecular mechanisms, screening, and treatment. Metallomics 2009, 1, 42–52. [Google Scholar] [CrossRef]
- Członkowska, A.; Litwin, T.; Dusek, P.; Ferenci, P.; Lutsenko, S.; Medici, V.; Rybakowski, J.K.; Weiss, K.H.; Schilsky, M.L. Wilson disease. Nat. Rev. Dis. Prim. 2018, 4, 1–20. [Google Scholar] [CrossRef] [PubMed]
- Menkes, J.H. Menkes disease and Wilson disease: Two sides of the same copper coin Part 1: Menkes disease. Eur. J. Paediatr. Neurol. 1999, 3, 147–158. [Google Scholar] [CrossRef] [PubMed]
- Denoyer, D.; Masaldan, S.; Fontaine, S.L.; Cater, M.A. Targeting copper in cancer therapy: ‘Copper That Cancer’. Metallomics 2015, 7, 1459–1476. [Google Scholar] [CrossRef] [PubMed]
- Strecker, D.; Mierzecki, A.; Radomska, K. Copper levels in patients with rheumatoid arthritis. Ann. Agric. Environ. Med. 2013, 20, 312–316. [Google Scholar] [PubMed]
- Waggoner, D.J.; Bartnikas, T.B.; Gitlin, J.D. The role of copper in neurodegenerative disease. Neurobiol. Dis. 1999, 6, 221–230. [Google Scholar] [CrossRef] [PubMed]
- Gao, J.; Yin, J.; Tao, Z.; Liu, Y.; Lin, X.; Deng, J.; Wang, S. An ultrasensitive fluorescence sensor with simple operation for Cu2+ specific detection in drinking water. ACS Omega 2018, 3, 3045–3050. [Google Scholar] [CrossRef]
- Guerrini, L.; Alvarez-Puebla, R.A. Surface-enhanced Raman scattering sensing of transition metal ions in waters. ACS Omega 2021, 6, 1054–1063. [Google Scholar] [CrossRef]
- Feist, B.; Mikula, B.; Pytlakowska, K.; Puzio, B.; Buhl, F. Determination of heavy metals by ICP-OES and F-AAS after preconcentration with 2,20-bipyridyl and erythrosine. J. Hazard. Mater. 2008, 152, 1122–1129. [Google Scholar] [CrossRef]
- Yuhana-Ariffin, E.; Sulaiman, S.S.; Abdul Kadir Jilani, N.; Nokarajoo, D.; Abdul Razak, N.H.; Derawi, D.; Hasbullah, S.A. A new sensing material based on tetraaza/SBA15 for rapid detection of copper (II) ion in water. Membranes 2022, 12, 1152. [Google Scholar] [CrossRef]
- Mandal, D.; Banerjee, S. Surface acoustic wave (SAW) sensors: Physics, materials, and applications. Sensors 2022, 22, 820. [Google Scholar] [CrossRef]
- Zheng, C.; Zhu, L.; Wang, J. A review on rapid detection of modified quartz crystal microbalance sensors for food: Contamination, flavour and adulteration. TrAC Trends Anal. Chem. 2022, 157, 116805. [Google Scholar] [CrossRef]
- Xu, X.; Zhang, N.; Brown, G.M.; Thundat, T.G.; Ji, H.F. Ultrasensitive detection of Cu2+ using a microcantilever sensor modified with L-cysteine self-assembled monolayer. Appl. Biochem. Biotechnol. 2017, 183, 555–565. [Google Scholar] [CrossRef] [PubMed]
- Vashist, S.K. For a general review on applications of microcantilevers. J. Nanotechnol. 2007, 3, 1–15. [Google Scholar] [CrossRef]
- Georghiou, P.E.; Rahman, S.; Valluru, G.; Dawe, L.N.; Rahman, S.M.S.; Alodhayb, A.N.; Beaulieu, L.Y. Synthesis of an upper-and lower-rim functionalized calix [4] arene for detecting calcium ions using a microcantilever sensor. New J. Chem. 2013, 37, 1298–1301. [Google Scholar] [CrossRef]
- Valluru, G.; Rahman, S.; Georghiou, P.E.; Dawe, L.N.; Alodhayb, A.N.; Beaulieu, L.Y. Synthesis of a cone-conformer bimodal Calix [4] arene-crown-5 which forms a sensitive cesium ion sensing layer on gold-coated microcantilevers. New J. Chem. 2014, 38, 5868–5872. [Google Scholar] [CrossRef]
- Alodhayb, A.N.; Rahman, S.M.S.; Rahman, S.; Valluru, G.; Georghiou, P.E.; Beaulieu, L.Y. Detection of calcium ions using gold-coated micro-cantilever sensors using upper-and lower-rim functionalized calix [4] arenes. Sens. Actuators B Chem. 2014, 203, 766773. [Google Scholar] [CrossRef]
- Alodhayb, A.N.; Braim, M.; Beaulieu, L.Y.; Valluru, G.; Rahman, S.; Oraby, A.K.; Georghiou, P.E. Metal ion binding properties of a bimodal triazolyl-functionalized Calix [4] arene on a multi-array microcantilever system. Synthesis, fluorescence and DFT computation studies. RSC Adv. 2016, 6, 4387–4396. [Google Scholar] [CrossRef]
- Alodhayb, A.N.; Rahman, S.M.S.; Rahman, S.; Georghiou, P.E.; Beaulieu, L.Y. A 16-microcantilever array sensing system for the rapid and simultaneous detection of the analyte. Sens. Actuators B Chem. 2016, 237, 459–469. [Google Scholar] [CrossRef]
- Al-Gawati, M.A.; Alhazaa, A.; Albrithen, H.; Alnofiay, J.; Alodhayb, A. Effect of surface patterning using femtosecond laser on micromechanical and structural properties of micromechanical sensors. Mater. Res. Express 2020, 7, 085904. [Google Scholar] [CrossRef]
- Aloraini, D.A.; Almuqrin, A.H.; Alanazi, A.; Ain, Q.T.; Alodhayb, A.N. Rapid and sensitive detection of severe acute respiratory syndrome coronavirus 2 in a label-free manner using micromechanical sensors. Sensors 2021, 21, 4439. [Google Scholar] [CrossRef]
- Eliyahu, D.; Gileadi, E.; Galun, E.; Eliaz, N. Atomic force microscope-based meniscus-confined three-dimensional electrodeposition. Adv Mater Technol. 2020, 5, 1900827. [Google Scholar] [CrossRef]
- Barbic, M.; Eliason, L.; Ranshaw, J. Femto-Newton force sensitivity quartz tuning fork sensor. Sens. Actuators A Phys. 2007, 136, 564–566. [Google Scholar] [CrossRef]
- Jahng, J.; Kwon, H.; Lee, E.S. Photo-induced force microscopy by using a quartz tuning-fork sensor. Sensors 2019, 19, 1530. [Google Scholar] [CrossRef] [PubMed]
- Günther, P.; Fischer, U.C.; Dransfeld, K. Scanning near-field acoustic microscopy. Appl. Phys. B Laser Opt. 1989, 48, 89–92. [Google Scholar] [CrossRef]
- Ma, Y.; Hu, Y.; Qiao, S.; Lang, Z.; Liu, X.; He, Y.; Spagnolo, V. Quartz tuning forks resonance frequency matching for laser spectroscopy sensing. Photoacoustics 2022, 25, 100329. [Google Scholar] [CrossRef]
- Alodhayb, A. Quartz tuning fork, a low-cost orthogonal measurement tool for the characterization of low-volume liquid reagents. Measurement 2020, 152, 107313. [Google Scholar] [CrossRef]
- Alshammari, A.; Aldosari, F.; Qarmalah, N.B.; Lsloum, A.; Muthuramamoorthy, M.; Alodhayb, A. Detection of chemical host-guest interactions using a quartz tuning fork sensing system. IEEE Sens. J. 2020, 20, 12543–12551. [Google Scholar] [CrossRef]
- Assaifan, A.K.; Hezam, M.; Al-Gawati, M.A.; Alzahrani, K.E.; Alswieleh, A.; Arunachalam, P.; Al-Mayouf, A.; Alodhayb, A.; Albrithen, H. Label-free and simple detection of trace Pb(II) in tap water using non-Faradaic impedimetric sensors. Sens. Actuators A Phys. 2021, 329, 112833. [Google Scholar] [CrossRef]
- Dennington, R.; Keith, T.A.; Millam, J. GaussView, Version 6.0.16. Semichem Inc.: Shawnee Mission, KS, USA, 2019. [Google Scholar]
- Frisch, M.J.; Trucks, G.W.; Schlegel, H.B.; Scuseria, G.E.; Robb, M.A.; Cheeseman, J.R.; Scalmani, G.; Barone, V.; Petersson, G.A.; Nakatsuji, H.; et al. Gaussian 16, Revision C.01; Gaussian, Inc.: Wallingford, CT, USA, 2019. [Google Scholar]
- Boys, S.; Bernardi, F. The calculation of small molecular interactions by the differences of separate total energies. Some procedures with reduced errors. Mol. Phys. 1970, 19, 553–566. [Google Scholar] [CrossRef]
- Kestner, N.R.; Combariza, J.E. Basis set superposition errors: Theory and practice. In Reviews in Computational Chemistry; Wiley-VCH, John Wiley and Sons, Inc.: New York, NY, USA, 1999; Volume 13, p. 99. [Google Scholar] [CrossRef]
- Murray, J.S.; Politzer, P. The electrostatic potential: An overview. Wiley Interdiscip. Rev. Comput. Mol. Sci. 2011, 1, 153–163. [Google Scholar] [CrossRef]
- Murray, J.S.; Sen, K. Molecular Electrostatic Potentials, Concepts and Applications; Elsevier: Amsterdam, The Netherlands, 1996. [Google Scholar] [CrossRef]
- Politzer, P.; Murray, J. The fundamental nature and role of the electrostatic potential in atoms and molecules. Theor. Chem. Acc. 2002, 108, 134–142. [Google Scholar] [CrossRef]
- Yoosefian, M.; Etminan, N. Leucine/Pd-loaded (5,5) single-walled carbon nanotube matrix as a novel nanobiosensors for in silico detection of protein. Amino Acids 2018, 50, 653–661. [Google Scholar] [CrossRef] [PubMed]


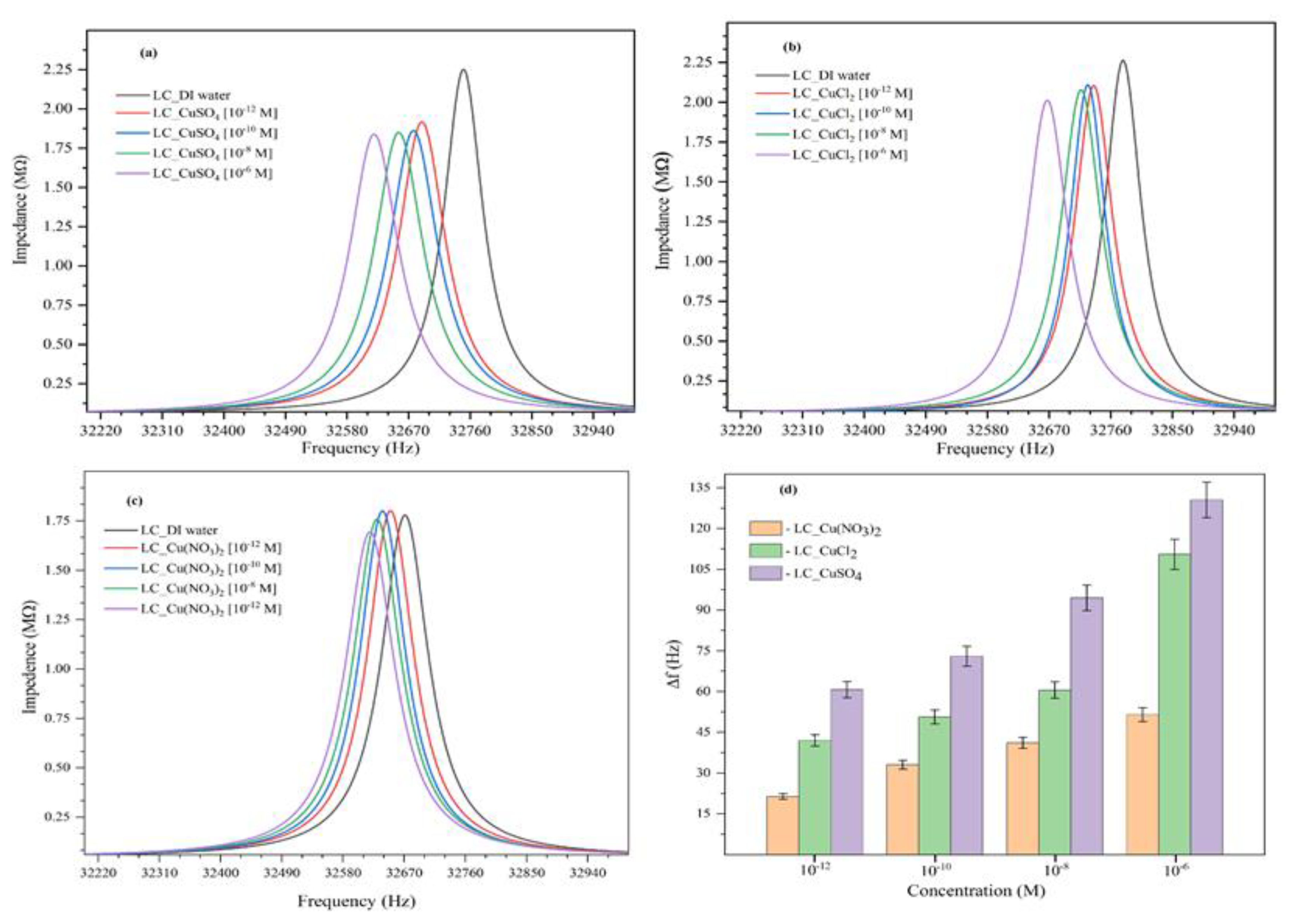
| Analyte Concentration (M) | Resonance Frequency Shift (Hz) | ||
|---|---|---|---|
| CuSO4 | CuCl2 | Cu(NO3)2 | |
| 10−12 | 60.72 ± 3.34 | 42.02 ± 2.10 | 21.36 ± 1.07 |
| 10−10 | 72.99 ± 3.65 | 50.69 ± 2.53 | 33.04 ± 1.65 |
| 10−8 | 94.49 ± 4.72 | 60.51 ± 3.03 | 41.13 ± 2.06 |
| 10−6 | 130.54 ± 6.53 | 110.53 ± 5.53 | 51.52 ± 2.58 |
| Complex | ΔIE and ΔG (kJ/mole) of the Analytes (CuSO4; CuCl2, and Cu(NO3)2 | |||||||
|---|---|---|---|---|---|---|---|---|
| B3LYP/LANL2DZ | ωB97XD/LANL2DZ | |||||||
| Uncorrected BSSE | Corrected BSSE | Uncorrected BSSE | Corrected BSSE | |||||
| ΔIE | ΔG | ΔIE | ΔG | ΔIE | ΔG | ΔIE | ΔG | |
| [LC] ⊃ [CuSO4] | −283.98 | −217.33 | −259.05 | −194.00 | −292.87 | −223.87 | −267.75 | −201.11 |
| [LC] ⊃ [CuCl2] | −133.26 | −115.71 | −112.12 | −95.66 | −172.77 | −112.22 | −151.69 | −107.41 |
| [LC] ⊃ [Cu(NO3)2] | −115.57 | −59.82 | −86.91 | −33.08 | −147.29 | −88.55 | −117.82 | −59.45 |
| [LC-Au] ⊃ [CuSO4] | −299.34 | −229.81 | −274.01 | 205.60 | −314.05 | −243.26 | −288.44 | −218.96 |
| [LC-Au] ⊃ [CuCl2] | −143.86 | −124.68 | −122.29 | −104.06 | −190.82 | −145.04 | −144.89 | −102.19 |
| [LC-Au]⊃[Cu(NO3)2] | −124.16 | −67.75 | −97.81 | −42.49 | −137.48 | −120.39 | −139.86 | −101.80 |
| Complex | ΔIE and ΔG (kJ/mole) of the analytes (CuSO4; CuCl2, and Cu(NO3)2 | |||
|---|---|---|---|---|
| B3LYP/LANL2DZ | ωB97XD/LANL2DZ | |||
| ΔIE | ΔG | ΔIE | ΔG | |
| [LC] ⊃ [CuSO4] | −249.01 | −180.097 | −268.61 | −197.68 |
| [LC] ⊃ [CuCl2] | −167.57 | −112.22 | −198.40 | −119.17 |
| [LC] ⊃ [Cu(NO3)2] | −133.61 | −76.68 | −177.92 | −115.57 |
| [LC-Au] ⊃ [CuSO4] | −264.58 | −195.88 | −292.13 | −220.17 |
| [LC-Au] ⊃ [CuCl2] | −143.86 | −124.68 | −190.82 | −145.04 |
| [LC-Au] ⊃ [Cu(NO3)2] | −142.77 | −85.57 | −180.75 | −118.98 |
| >HC to NH2 (Å) of LC | >HC to C=O (Å) of LC | Cu to NH2 (Å) of LC | Cu to O=C< (Å) of LC | Cu to >S=O(Å) of CuSO4 (Avg) | Cu to Cl (Å) of CuCl2 (Avg) | Cu to O=N (Å) of Cu(NO3)2 (Avg) | −C=O..Cu..NH2 (Å) for LC with Cu2+ (Avg) | Ionic charge of Cu2+ | |
|---|---|---|---|---|---|---|---|---|---|
| LC | 1.452 | 1.236 | − | − | − | − | − | − | − |
| LC-Au | 1.460 | 1.237 | − | − | − | − | − | − | − |
| CuSO4 | − | − | − | − | 1.932 | − | − | − | 1.088 |
| LC⊃CuSO4 | 1.490 | 1.256 | 2.022 | 2.006 | 11.963 | − | − | 2.014 | 0.866 |
| [LC-Au] ⊃CuSO4 | 1.488 | 1.258 | 2.008 | 2.017 | 1.965 | − | − | 2.012 | 0.858 |
| CuCl2 | − | − | − | − | − | 2.194 | − | − | 0.647 |
| LC⊃CuCl2 | 1.487 | 1.244 | 2.029 | 2.296 | − | 2.246 | − | 2.162 | 0.410 |
| [LC-Au] ⊃ CuCl2 | 1.488 | 1.245 | 2.014 | 2.307 | − | 2.249 | − | 2.160 | 0.414 |
| Cu(NO3)2 | − | − | − | − | − | − | 2.015 | − | 0.938 |
| LC⊃Cu(NO3)2 | 1.489 | 1.246 | 2.008 | 2.254 | − | − | 2.177 | 2.131 | 0.689 |
| [LC-Au] ⊃Cu(NO3)2 | 1.489 | 1.243 | 2.029 | 2.414 | − | − | 2.162 | 2.233 | 0.689 |
| Selected Bond Angles (in Degrees). | ||||||
|---|---|---|---|---|---|---|
| O=C−CH−NH2 | O=S=O (CuSO4) | O--Cu--O | −C=O—Cu--NH2− | Cl−Cu−Cl | O=N=O (Avg.) | |
| LC | 109.94 | − | − | − | − | − |
| CuSO4 | − | 88.81 | 79.07 | − | − | − |
| LC⊃CuSO4 | 107.15 | 92.28 | 77.61 | 82.70 | ||
| [LC-Au] ⊃CuSO4 | 107.13 | 92.39 | 77.52 | 82.75 | − | − |
| CuCl2 | − | − | − | − | 117.47 | − |
| LC⊃CuCl2 | 108.39 | − | − | 77.83 | 149.26 | − |
| [LC-Au] ⊃ CuCl2 | 108.07 | − | − | − | 148.51 | − |
| Cu(NO3)2 | − | − | 66.24 | 78.89 | − | 111.22 |
| LC⊃Cu(NO3)2 | − | − | 60.68 | 78.89 | − | 112.61 |
| [LC-Au] ⊃Cu(NO3)2 | − | − | 61.22 | 74.59 | − | 111.51 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rahman, S.; Al-Gawati, M.A.; Alfaifi, F.S.; Muthuramamoorthy, M.; Alanazi, A.F.; Albrithen, H.; Alzahrani, K.E.; Assaifan, A.K.; Alodhayb, A.N.; Georghiou, P.E. The Effect of Counterions on the Detection of Cu2+ Ions in Aqueous Solutions Using Quartz Tuning Fork (QTF) Sensors Modified with L-Cysteine Self-Assembled Monolayers: Experimental and Quantum Chemical DFT Study. Chemosensors 2023, 11, 88. https://doi.org/10.3390/chemosensors11020088
Rahman S, Al-Gawati MA, Alfaifi FS, Muthuramamoorthy M, Alanazi AF, Albrithen H, Alzahrani KE, Assaifan AK, Alodhayb AN, Georghiou PE. The Effect of Counterions on the Detection of Cu2+ Ions in Aqueous Solutions Using Quartz Tuning Fork (QTF) Sensors Modified with L-Cysteine Self-Assembled Monolayers: Experimental and Quantum Chemical DFT Study. Chemosensors. 2023; 11(2):88. https://doi.org/10.3390/chemosensors11020088
Chicago/Turabian StyleRahman, Shofiur, Mahmoud A. Al-Gawati, Fatimah S. Alfaifi, Muthumareeswaran Muthuramamoorthy, Amal F. Alanazi, Hamad Albrithen, Khalid E. Alzahrani, Abdulaziz K. Assaifan, Abdullah N. Alodhayb, and Paris E. Georghiou. 2023. "The Effect of Counterions on the Detection of Cu2+ Ions in Aqueous Solutions Using Quartz Tuning Fork (QTF) Sensors Modified with L-Cysteine Self-Assembled Monolayers: Experimental and Quantum Chemical DFT Study" Chemosensors 11, no. 2: 88. https://doi.org/10.3390/chemosensors11020088
APA StyleRahman, S., Al-Gawati, M. A., Alfaifi, F. S., Muthuramamoorthy, M., Alanazi, A. F., Albrithen, H., Alzahrani, K. E., Assaifan, A. K., Alodhayb, A. N., & Georghiou, P. E. (2023). The Effect of Counterions on the Detection of Cu2+ Ions in Aqueous Solutions Using Quartz Tuning Fork (QTF) Sensors Modified with L-Cysteine Self-Assembled Monolayers: Experimental and Quantum Chemical DFT Study. Chemosensors, 11(2), 88. https://doi.org/10.3390/chemosensors11020088








