Characterization of CdTe Thin Films Using Orthogonal Double-Pulse Laser-Induced Breakdown Spectroscopy
Abstract
1. Introduction
2. Materials and Methods
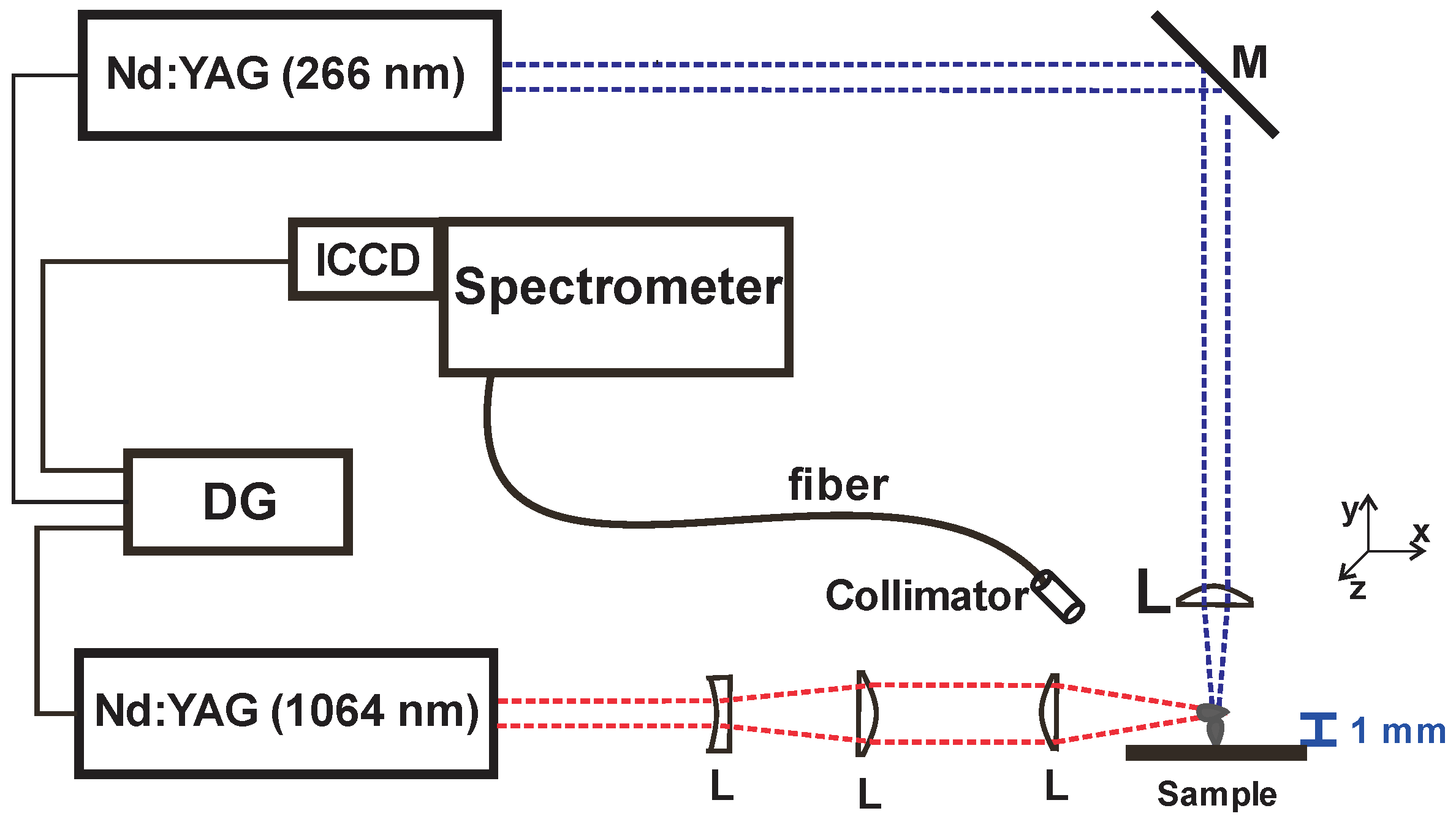
2.1. Experimental Procedure
2.2. Samples
2.3. Methodology
3. Results and Discussion
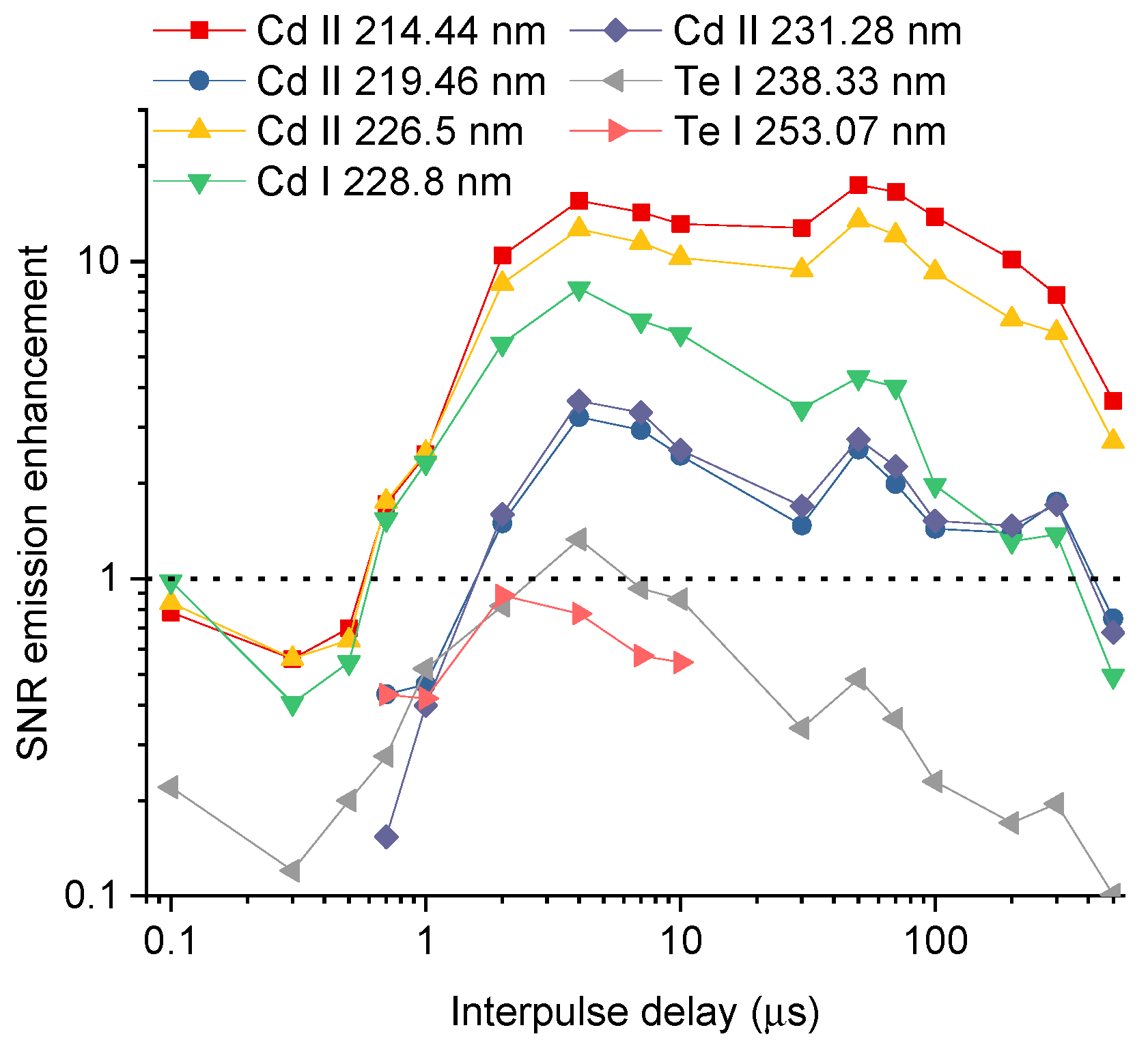
3.1. Optimization of the Experimental Parameters
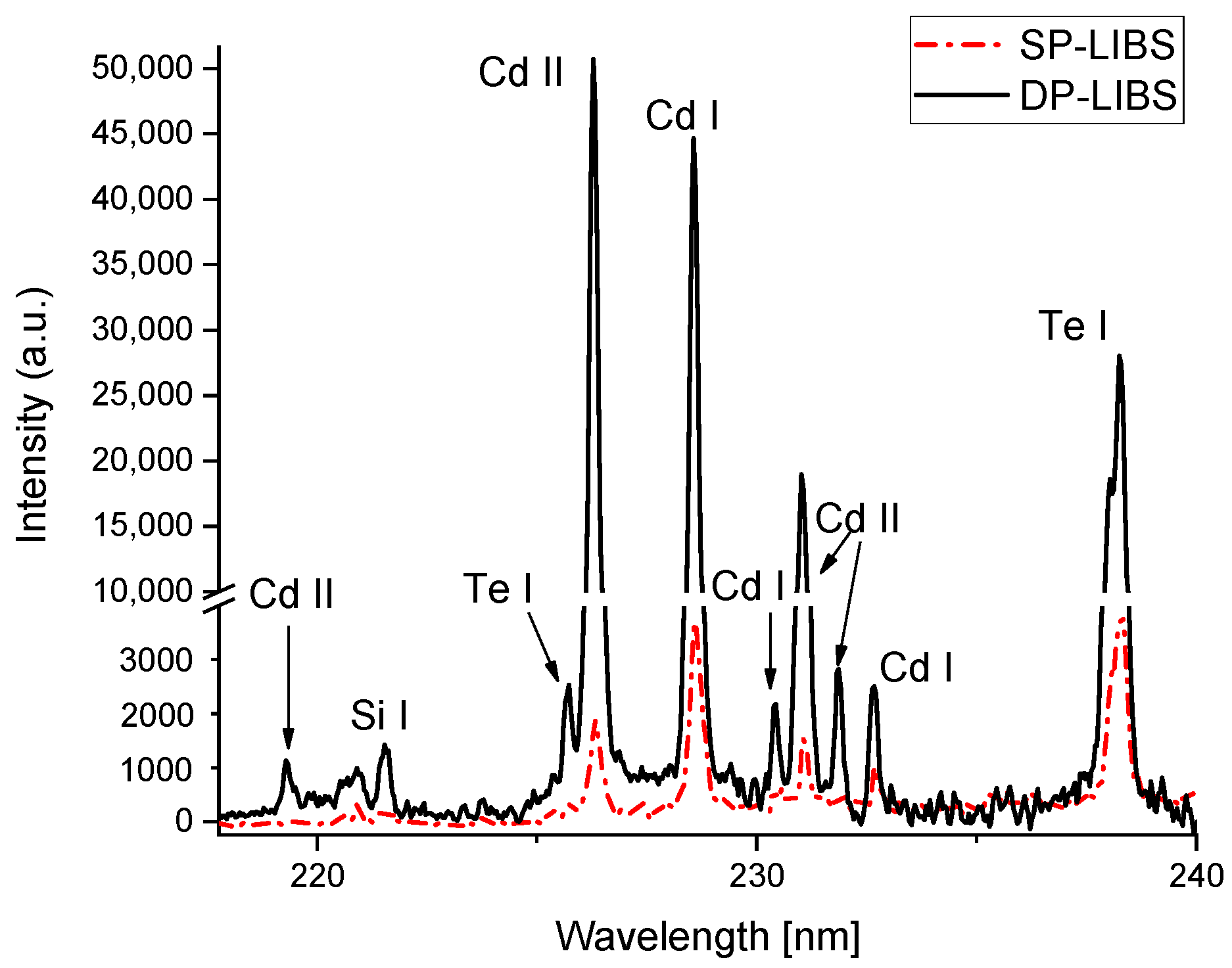
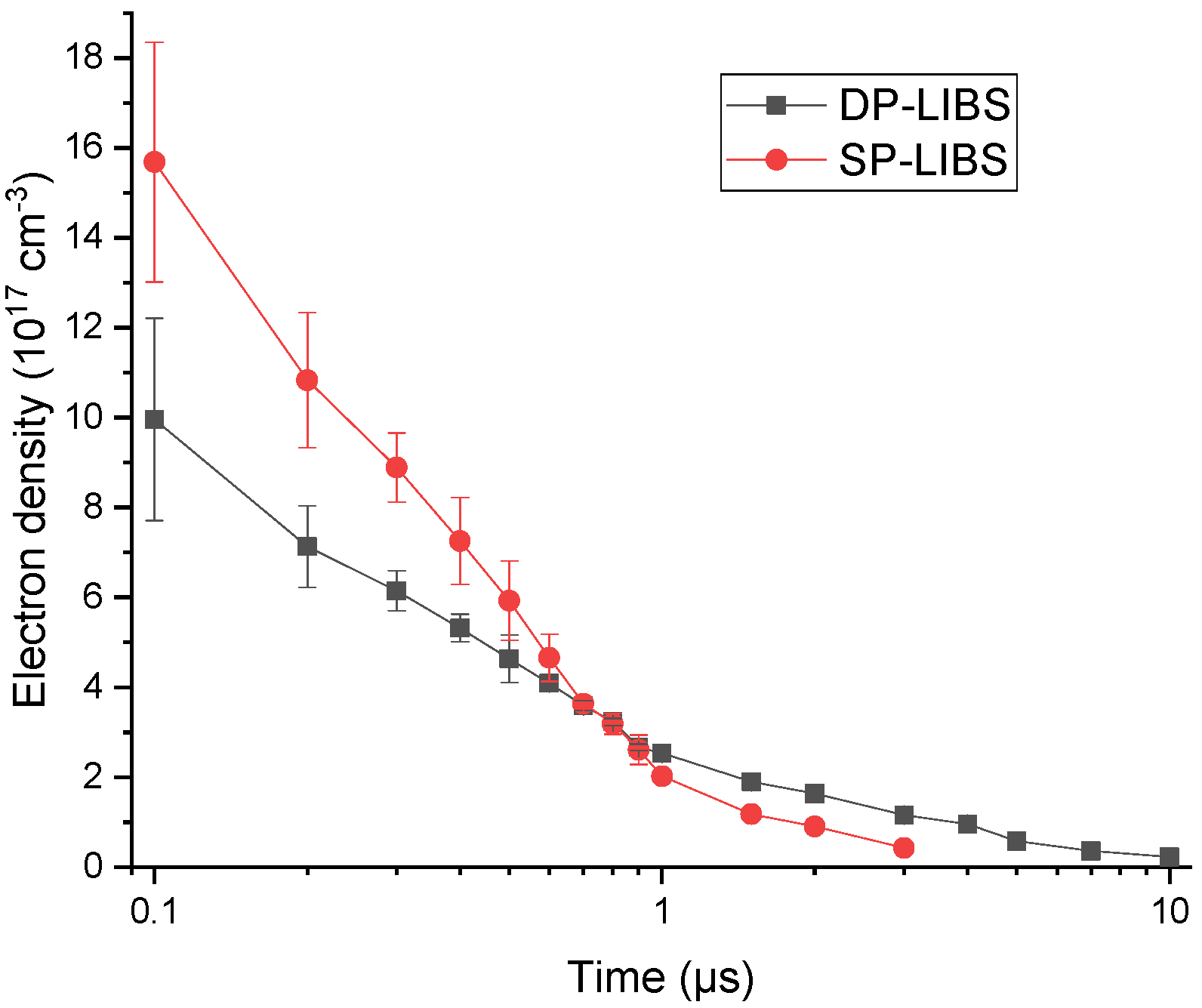
3.2. Plasma Characterization
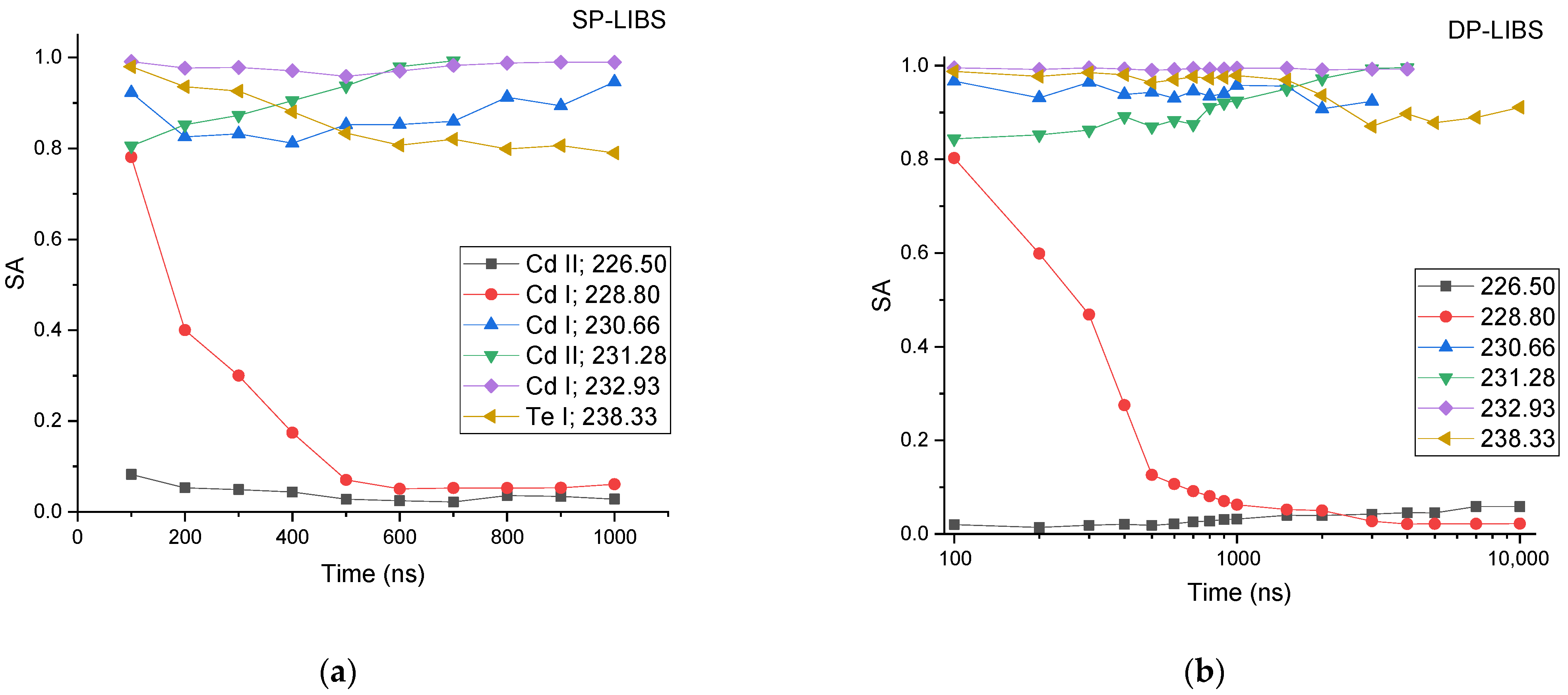
3.3. Determination of Self-Absorption
3.4. Relative Composition of Thin Films
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Manimozhi, T.; Ramamurthi, K.; Sinthiya, M.M.; Karthigeyan, A.; Bhuvanaswari, P.V.; Ramesh Babu, R. Effect of Substrate Temperature on the Properties of Nanocrystalline CdTe Thin Films Coated by Electron Beam Evaporation Method. Int. J. ChemTech Res. 2015, 7, 950–957. [Google Scholar]
- Bosio, A.; Pasini, S.; Romeo, N. The History of Photovoltaics with Emphasis on CdTe Solar Cells and Modules. Coatings 2020, 10, 344. [Google Scholar] [CrossRef]
- Romeo, A.; Artegiani, E. CdTe-Based Thin Film Solar Cells: Past, Present and Future. Energies 2021, 14, 1684. [Google Scholar] [CrossRef]
- Dharmadasa, I.M.; Echendu, O.K.; Fauzi, F.; Abdul-Manaf, N.A.; Olusola, O.I.; Salim, H.I.; Madugu, M.L.; Ojo, A.A. Improvement of Composition of CdTe Thin Films During Heat Treatment in the Presence of CdCl2. J. Mater Sci. Mater Electron. 2017, 28, 2343–2352. [Google Scholar] [CrossRef]
- Ling, J.; Zhang, X.; Mao, T.; Li, L.; Wang, S.; Cao, M.; Zhang, J.; Shi, H.; Huang, J.; Shen, Y.; et al. Electrodeposition of CdTe Thin Films for Solar Energy Water Splitting. Materials 2020, 13, 1536. [Google Scholar] [CrossRef]
- Gu, P.; Zhu, X.; Wu, H.; Yang, D. Regulation of Substrate-Target Distance on the Microstructural, Optical and Electrical Properties of CdTe Films by Magnetron Sputtering. Materials 2018, 11, 2496. [Google Scholar] [CrossRef]
- Arnold, C.B.; Aziz, M.J. Stoichiometry Issues in Pulsed-laser Deposition of Alloys Grown from Multicomponent Targets. Appl. Phys. A. 1999, 69, S23–S27. [Google Scholar] [CrossRef]
- Major, J.D. Grain Boundaries in CdTe Thin Film Solar Cells: A Review. Semicond. Sci. Technol. 2016, 31, 093001. [Google Scholar] [CrossRef]
- Chaudhary, K.; Rizvi, S.Z.H.; Ali, J. Laser-Induced Plasma and its Applications. In Plasma Science and Technology—Progress in Physical States and Chemical Reactions; Mieno, T., Ed.; IntechOpen: London, UK, 2016; Available online: https://www.intechopen.com/chapters/49562 (accessed on 14 September 2022).
- Richardson, M. Laser Produced Plasmas. In Experimental Methods in the Physical Sciences, 1st ed.; Samson, J.A.R., Ederer, D.L., Eds.; Academic Press: Cambridge, MA, USA, 1998; Volume 31, pp. 83–92. [Google Scholar]
- Afonso, C.; Gonzalo, J.; Sena, R.; Solis, J. Pulsed Laser Deposition for Functional Optical Films. In Laser Ablation and its Applications; Phipps, C., Ed.; Springer Science-Business Media: New York, NY, USA, 2007; pp. 315–338. [Google Scholar]
- Stafe, M.; Marcu, A.; Puscas, N.N. Pulsed Laser Ablation of Solids. Basics, Theory and Applications, 1st ed.; Springer: Berlin/Heidelberg, Germany, 2014; pp. 15–51. [Google Scholar]
- Legnaioli, S.; Lorenzetti, G.; Pardini, L.; Cavalcanti, G.H.; Palleschi, V. Applications of LIBS to the Analysis of Metals. In Laser-Induced Breakdown Spectroscopy Theory and Applications, 1st ed.; Musazzi, S., Perini, U., Eds.; Springer: Berlin/Heidelberg, Germany, 2014; Chap. 7; pp. 181–182. [Google Scholar]
- Hahn, D.W.; Omenetto, N. Laser-Induced Breakdown Spectroscopy (LIBS), Part II: Review of Instrumental and Methodological Approaches to Material Analysis and Applications to Different Fields. Appl. Spectrosc. 2012, 66, 347–419. [Google Scholar] [CrossRef]
- Noll, R. Laser-Induced Breakdown Spectroscopy, Fundamentals and Applications, 1st ed.; Springer: Berlin/Heidelberg, Germany, 2012; pp. 7–16. [Google Scholar]
- Li, W.; Li, X.; Li, X.; Hao, Z.; Lu, Y.; Zeng, X. A review of remote laser-induced breakdown spectroscopy. App. Spectrosc. Rev. 2020, 55, 1–25. [Google Scholar] [CrossRef]
- Jolivet, L.; Leprince, M.; Moncayo, S.; Sorbier, L.; Lienemann, C.P.; Motto-Ros, V. Review of the recent advances and applications of LIBS-based imaging. SAB 2019, 151, 41–53. [Google Scholar] [CrossRef]
- Noll, R.; Fricke-Begemann, C.; Connemann, S.; Meinhardt, C.; Sturm, V. LIBS analyses for industrial applications—An overview of developments from 2014 to 2018. J. Anal. At. Spectrom. 2018, 33, 945–956. [Google Scholar] [CrossRef]
- Singh, V.K.; Sharma, J.; Pathak, A.K.; Ghany, C.T.; Gondal, M.A. Laser-induced breakdown spectroscopy (LIBS): A novel technology for identifying microbes causing infectious diseases. Biophys. Rev. 2018, 10, 1221–1239. [Google Scholar] [CrossRef] [PubMed]
- Wu, J.; Qiu, Y.; Li, X.; Yu, H.; Zhang, Z.; Qiu, A. Progress of laser-induced breakdown spectroscopy in nuclear industry applications. J. Phys. D Appl. Phys. 2020, 53, 1–24. [Google Scholar] [CrossRef]
- Liu, C.; Ling, Z.; Zhang, J.; Wu, Z.; Bai, H.; Liu, Y. A Stand-Off Laser-Induced Breakdown Spectroscopy (LIBS) System Applicable for Martian Rocks Studies. Remote Sens. 2021, 13, 4773. [Google Scholar] [CrossRef]
- Papazoglou, D.G.; Papadakis, V.; Anglos, D. In situ interferometric depth and topography monitoring in LIBS elemental profiling of multi-layer structures. J. Anal. At. Spectrom. 2004, 19, 483–488. [Google Scholar] [CrossRef]
- Dwivedi, V.; Marín-Roldán, A.; Karhunen, J.; Paris, P.; Jõgi, I.; Porosnicu, C.; Lungu, C.P.; van der Meiden, H.; Hakola, A.; Veis, P. CF-LIBS quantification and depth profile analysis of Be coating mixed layers. Nucl. Mater. Energy 2021, 27, 100990. [Google Scholar] [CrossRef]
- Canel, T.; Demir, P.; Kacar, E.; Genc Oztoprak, B.; Akman, E.; Gunes, M.; Demir, A. Optimization of parameters for depth resolution of galvanized steel by LIBS technique. Opt. Laser Technol. 2013, 54, 257–264. [Google Scholar] [CrossRef]
- De Bonis, A.; De Filippo, B.; Galasso, A.; Santagata, A.; Smaldone, A.; Teghil, R. Comparison of the performances of nanosecond and femtosecond Laser Induced Breakdown Spectroscopy for depth profiling of an artificially corroded bronze. App. Surf. Sci. 2014, 302, 275–279. [Google Scholar] [CrossRef]
- Sobral, H.; Amador-Mejía, M.; Márquez-Herrera, C. Characterization of Pottery from Teotihuacan by Laser-Induced Breakdown Spectroscopy and Inductively Coupled Plasma-Optical Emission Spectroscopy. Appl. Spectrosc. 2021, 75, 728–738. [Google Scholar] [CrossRef]
- Aragón, C.; Madurga, V.; Aguilera, J.A. Application of laser-induced breakdown spectroscopy to the analysis of the composition of thin films produced by pulsed laser deposition. Appl. Surf. Sci. 2002, 197, 217–223. [Google Scholar] [CrossRef]
- Pender, J.; Pearman, B.; Scaffidi, J.; Goode, S.R.; Michael Angel, S. Laser-induced breakdown spectroscopy using sequential laser pulses. In Laser-Induced Breakdown Spectroscopy (LIBS): Fundamental and Applications, 1st ed.; Miziolek, A.W., Palleschi, V., Schechter, I., Eds.; Cambridge University Press: Cambridge, UK, 2006. [Google Scholar]
- Tognoni, E.; Cristoforetti, G. Signal and noise in Laser Induced Breakdown Spectroscopy: An introductory review. Opt. Laser Technol. 2016, 79, 164–172. [Google Scholar] [CrossRef]
- Li, Y.; Ding, Y.; Yang, G.; Liu, K.; Wanch, C.; Han, X. A review of laser-induced breakdown spectroscopy signal enhancement. Appl. Spectr. Rev. 2018, 53, 1–35. [Google Scholar] [CrossRef]
- Sanginés, R.; Contreras, V.; Sobral, H.; Robledo-Martinez, A. Optimal emission enhancement in orthogonal double-pulse laser-induced breakdown spectroscopy. Spectrochim. Acta Part B 2015, 110, 139–145. [Google Scholar] [CrossRef]
- Popescu, A.C.; Beldjilali, S.; Socol, G.; Craciun, V.; Mihailescu, I.N.; Hermann, J. Analysis of indium zinc oxide thin films by laser-induced breakdown spectroscopy. J. Appl. Phys. 2011, 110, 083116. [Google Scholar] [CrossRef]
- Acquaviva, S.; D’Ann, E.; De Giorgi, M.L.; Moro, F. Laser-induced breakdown spectroscopy for compositional analysis of multielemental thin films. Spectrochim. Acta Part B 2006, 61, 810–816. [Google Scholar] [CrossRef]
- Davari, S.A.; Hu, S.; Pamuac, R.; Mukherjee, D. Calibration-free quantitative analysis of thin-film oxide layers in semiconductors using laser induced breakdown spectroscopy (LIBS). J. Anal. At. Spectrom 2017, 32, 1378–1387. [Google Scholar] [CrossRef]
- Caneve, L.; Colao, F.; Sarto, F.; Spizzichino, V.; Vadrucci, M. Laser-induced breakdown spectroscopy as a diagnostic tool for thin films elemental composition. Spectrochim. Acta Part B 2005, 60, 1098–1102. [Google Scholar] [CrossRef]
- Hermann, J.; Axente, E.; Pelascini, F.; Craciun, V. Analysis of Multi-Elemental Thin Films via Calibration-Free Laser-Induced Breakdown Spectroscopy. Anal. Chem. 2019, 91, 2544–2550. [Google Scholar] [CrossRef]
- Lee, S.H.; Shim, H.S.; Kim, C.K.; Yoo, J.H.; Russo, R.E.; Jeong, S. Analysis of the absorption layer of CIGS solar cell by laser-induced breakdown spectroscopy. Appl. Opt. 2012, 51, B115–B120. [Google Scholar] [CrossRef]
- Banerjee, P.; Sarneta, T.; Siozosb, P.; Loulakisb, M.; Anglosb, D.; Sentis, M. Characterization of organic photovoltaic devices using femtosecond laser induced breakdown spectroscopy. Appl. Surf. Sci. 2017, 418, 542–547. [Google Scholar] [CrossRef]
- Owens, T.; Mao, S.S.; Canfield, E.K.; Grigoropoulos, C.P.; Mao, X.; Russo, R.E. Ultrafast thin-film laser-induced breakdown spectroscopy of doped oxides. Appl. Opt. 2010, 49, C67–C69. [Google Scholar] [CrossRef]
- Nishijima, D.; Hollmann, E.M.; Doerner, R.P. Spatially-offset double-pulse laser-induced breakdown spectroscopy: A novel technique for analysis of thin deposited layers. Spectrochim. Acta Part B 2016, 124, 82–86. [Google Scholar] [CrossRef]
- Sanginés, R.; Sobral, H. Time Resolved Study of the Emission Enhancement Mechanisms in Orthogonal Double-Pulse Laser-Induced Breakdown Spectroscopy. Spectrochim. Acta B 2013, 88, 150–155. [Google Scholar] [CrossRef]
- Harilal, S.S.; Phillips, M.C.; Froula, D.H.; Anoop, K.J.; Isaac, R.C.; Beg, F.N. Optical Diagnostics of Laser-Produced Plasmas. Rev. Mod. Phys. 2022, 94, 035002. [Google Scholar] [CrossRef]
- Rangel-Cárdenas, J.; Sobral, H. Optical Absorption Enhancement in CdTe Thin Films by Microstructuration of the Silicon Substrate. MDPI Materials 2017, 10, 607. [Google Scholar] [CrossRef]
- Bredice, F.O.; Di Rocco, H.O.; Sobral, H.M.; Villagrán-Muniz, M.; Palleschi, V.A. new method for determination of self-absorption coefficients of emission lines in Laser-Induced Breakdown Spectroscopy experiments. Appl. Spectrosc. 2010, 64, 320–323. [Google Scholar] [CrossRef]
- El Sherbini, A.M.; El Sherbini, T.; Hegazy, H.; Cristoforetti, G.; Legnaioli, S.; Pardini, L.; Palleschi, V.; Salvetti, A.; Tognoni, E. Measurement of the Stark Broadening of Atomic Emission Lines in Non–Optically Thin Plasmas by Laser-Induced Breakdown Spectroscopy. Spectrosc. Lett. 2007, 40, 643–658. [Google Scholar] [CrossRef]
- Aguilera, J.A.; Aragón, C. Multi-element Saha–Boltzmann and Boltzmann plots in laser-induced plasmas. Spectrochim. Acta Part B 2007, 62, 378–385. [Google Scholar] [CrossRef]
- Gautier, C.; Fichet, P.; Menut, D.; Lacour, J.L.; L’Hermite, D.; Dubessy, J. Quantification of the intensity enhancements for the double-pulse laser-induced breakdown spectroscopy in the orthogonal beam geometry. Spectrochim. Acta Part B 2005, 60, 265–276. [Google Scholar] [CrossRef]
- Gautier, C.; Fichet, P.; Menut, D.; Lacour, J.L.; L’Hermite, D.; Dubessy, J. Study of the double-pulse setup with an orthogonal beam geometry for laser-induced breakdown spectroscopy. Spectrochim. Acta Part B 2004, 59, 975–986. [Google Scholar] [CrossRef]
- Tognoni, E.; Cristoforetti, G. Basic mechanism of signal enhancement in ns double-pulse laser-induced breakdown spectroscopy in a gas environment. J. Anal. At. Spectrom. 2014, 29, 1318–1338. [Google Scholar] [CrossRef]
- Konjevic, N.; Lesage, A.; Fuhr, J.R.; Wiese, W.L. Experimental Stark Widths and Shifts for Spectral Lines of Neutral and Ionized Atoms (A Critical Review of Selected Data for the Period 1989 Through 2000). J. Phys. Chem. Ref. Data 2002, 31, 819–927. [Google Scholar] [CrossRef]
- Simic, Z.; Dimitrijevic, M.S.; Kovacevic, A. Stark broadening of spectral lines in chemically peculiar stars: Te I lines and recent calculations for trace elements. New Astron. Rev. 2009, 53, 246–251. [Google Scholar] [CrossRef]
- El Sherbini, A.M.; Hegazy, H.; El Sherbini, T.M. Measurement of electron density utilizing the Hα-line from laser produced plasma in air. Spectrochim. Acta Part B 2006, 61, 532–539. [Google Scholar] [CrossRef]
- Scaffidi, J.; Angel, S.M.; Cremers, D. A Emission Enhancement Mechanisms in Dual-Pulse LIBS. Anal Chem. 2006, 78, 24–32. [Google Scholar] [CrossRef]
- St-Onge, L.; Detalle, V.; Sabsabi, M. Enhanced Laser-Induced Breakdown Spectroscopy Using the Combination of Fourth-Harmonic and Fundamental Nd:YAG Laser Pulses. Spectrochim Acta B 2002, 57, 121–135. [Google Scholar] [CrossRef]
- NIST Atomic Spectra Database Lines Form. National Institute of Standards and Technology. Available online: https://www.nist.gov/pml/atomic-spectra-database (accessed on 15 October 2022).
- Atomic Spectral Line Database from CD-ROM 23 of R.L. Kurucz. Available online: https://lweb.cfa.harvard.edu/amp/ampdata/kurucz23/sekur.html (accessed on 15 October 2022).



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Quintana-Silva, G.; Sobral, H.; Rangel-Cárdenas, J. Characterization of CdTe Thin Films Using Orthogonal Double-Pulse Laser-Induced Breakdown Spectroscopy. Chemosensors 2023, 11, 4. https://doi.org/10.3390/chemosensors11010004
Quintana-Silva G, Sobral H, Rangel-Cárdenas J. Characterization of CdTe Thin Films Using Orthogonal Double-Pulse Laser-Induced Breakdown Spectroscopy. Chemosensors. 2023; 11(1):4. https://doi.org/10.3390/chemosensors11010004
Chicago/Turabian StyleQuintana-Silva, Guillermo, Hugo Sobral, and Jesus Rangel-Cárdenas. 2023. "Characterization of CdTe Thin Films Using Orthogonal Double-Pulse Laser-Induced Breakdown Spectroscopy" Chemosensors 11, no. 1: 4. https://doi.org/10.3390/chemosensors11010004
APA StyleQuintana-Silva, G., Sobral, H., & Rangel-Cárdenas, J. (2023). Characterization of CdTe Thin Films Using Orthogonal Double-Pulse Laser-Induced Breakdown Spectroscopy. Chemosensors, 11(1), 4. https://doi.org/10.3390/chemosensors11010004





