Magnetohydrodynamic Hybrid Nanofluid Flow Past an Exponentially Stretching Sheet with Slip Conditions
Abstract
:1. Introduction
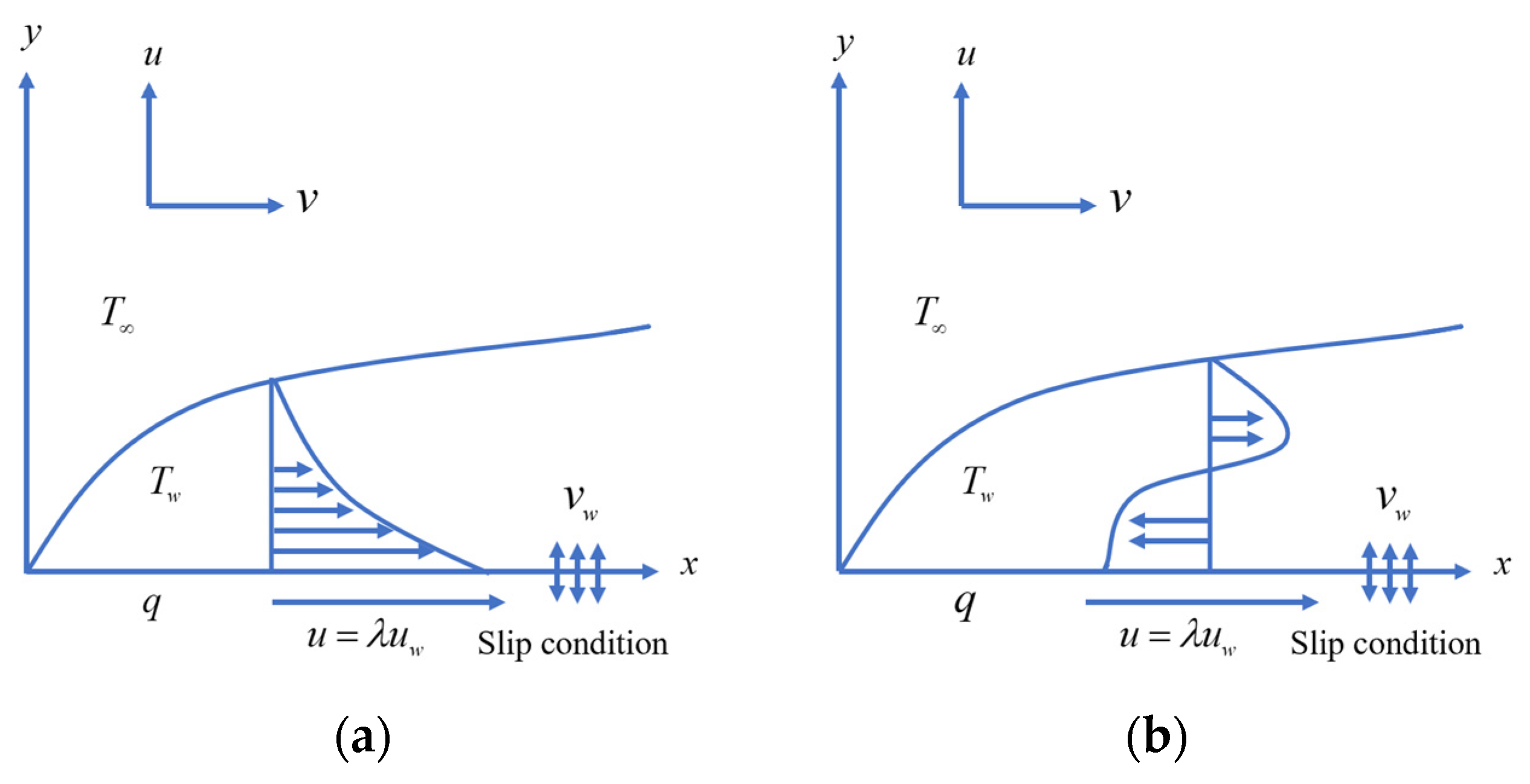
2. Mathematical Formulation
3. Solution of the Problem Using HAM
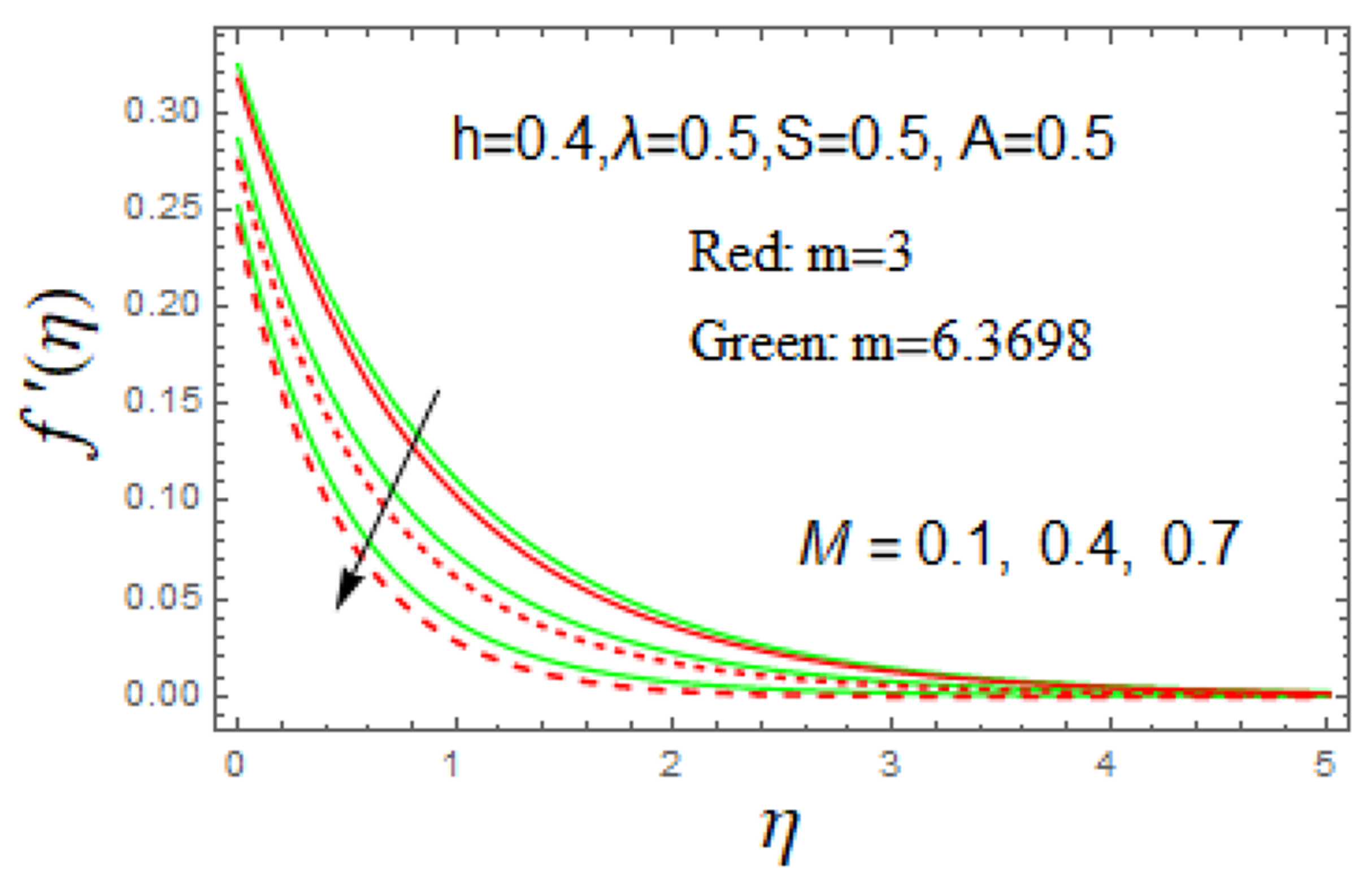
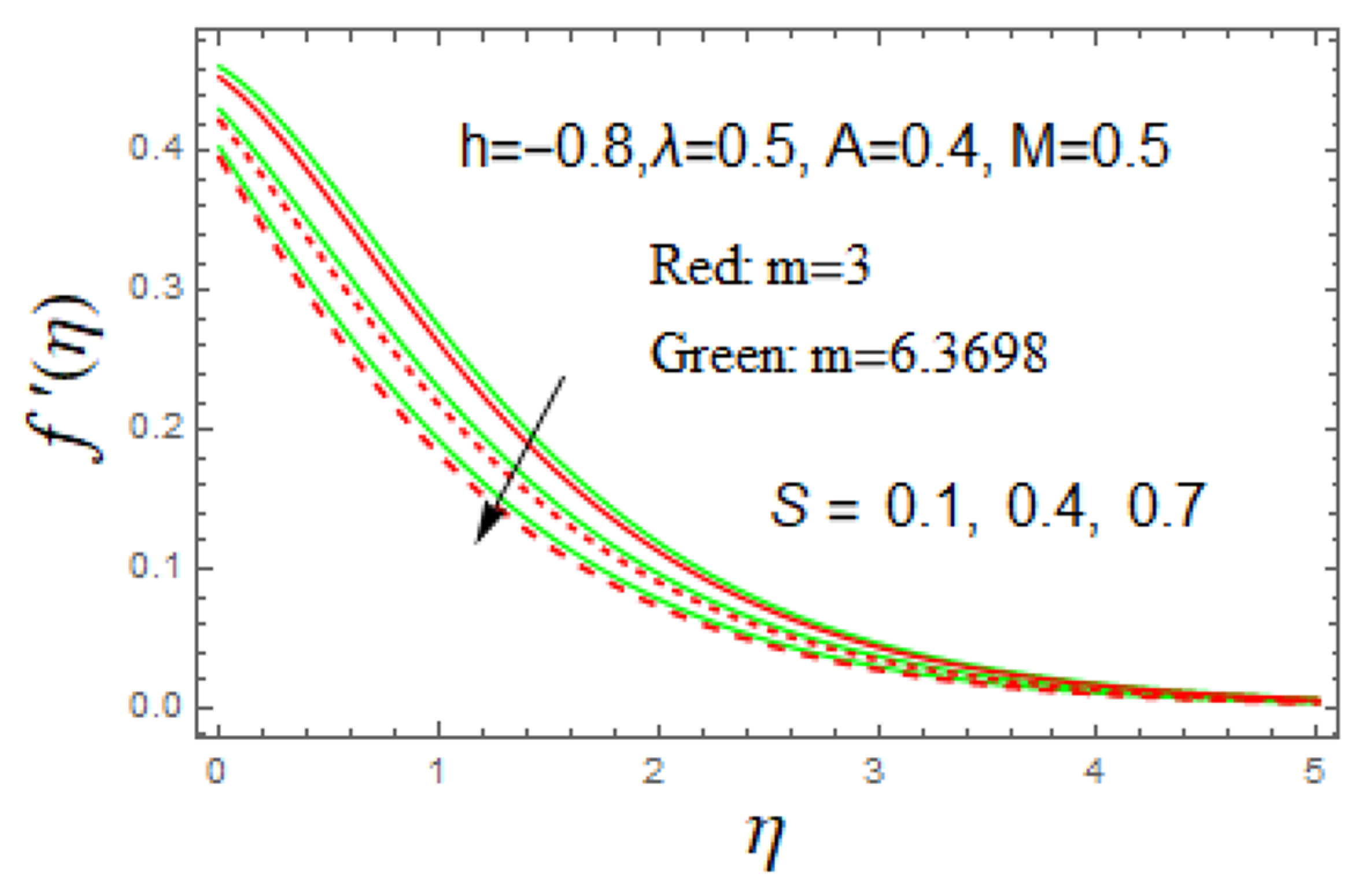
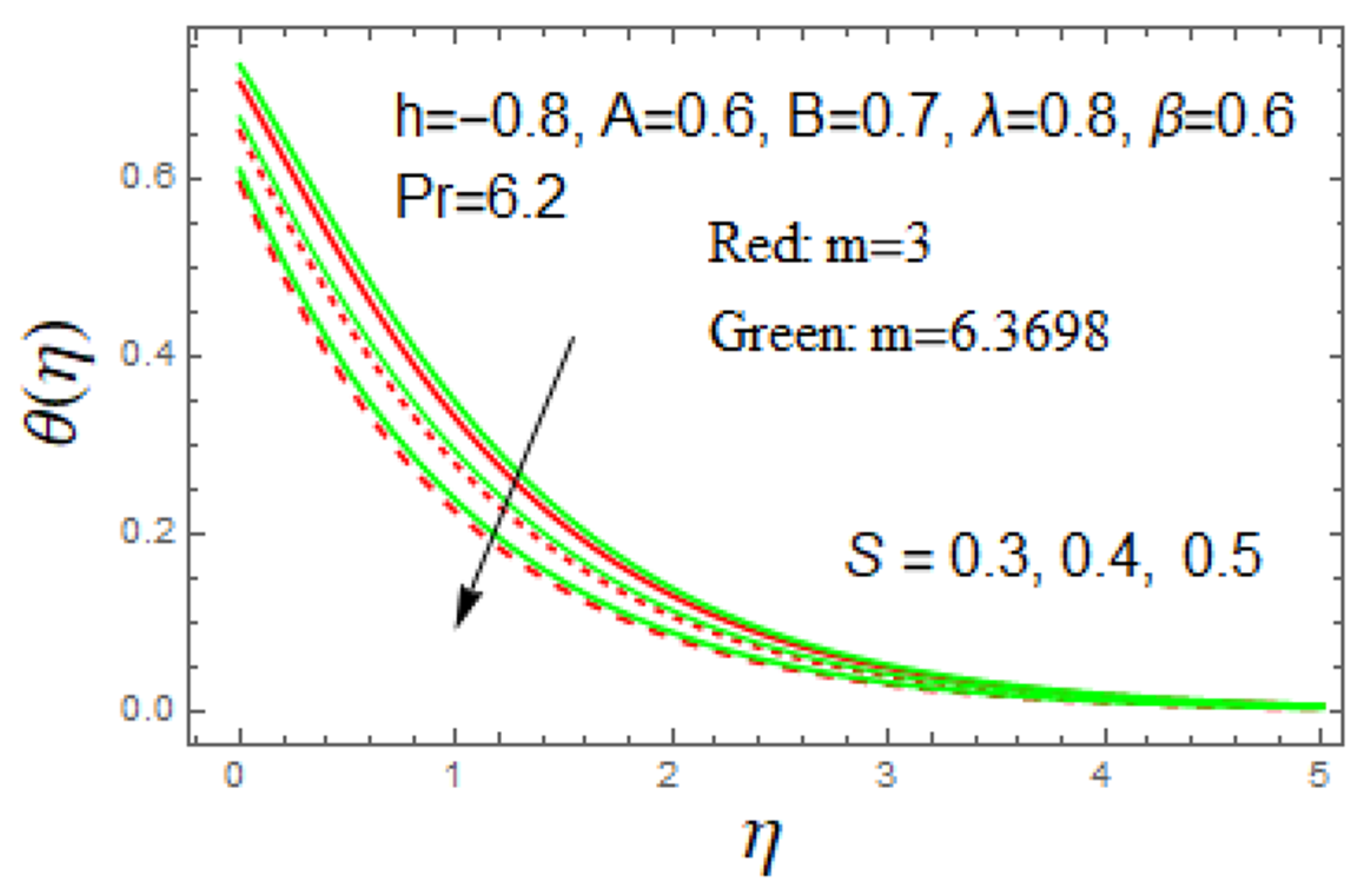
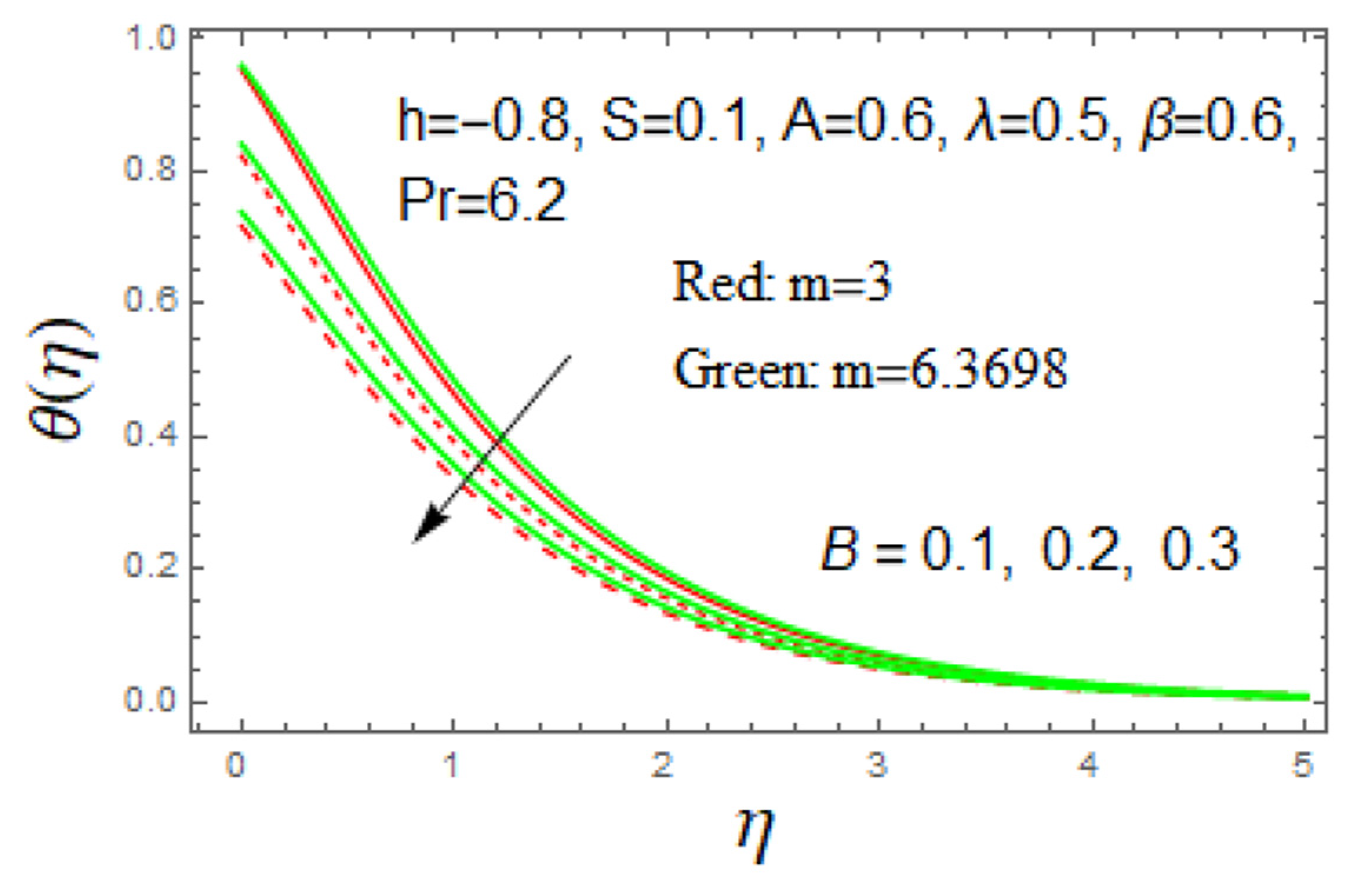
4. Results and Discussion
5. Conclusions
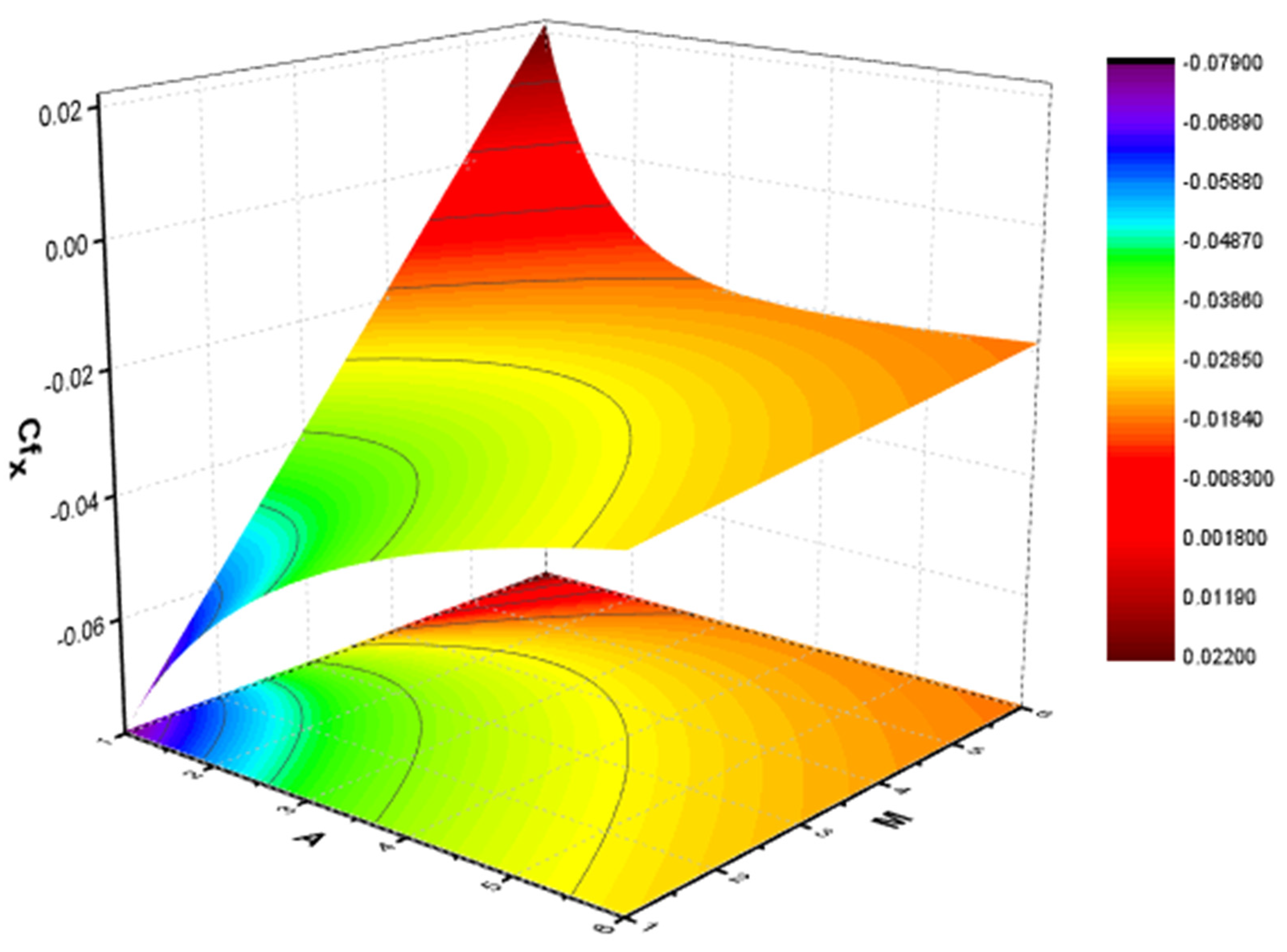
- The rise in velocity slip and the magnetic parameters increases the skin friction. Consequently, it indicates a decline in the velocity of the fluid.
- The rise in the stretching/shrinking parameters decreases the skin friction. Therefore, it shows an increment in the velocity of the fluid.
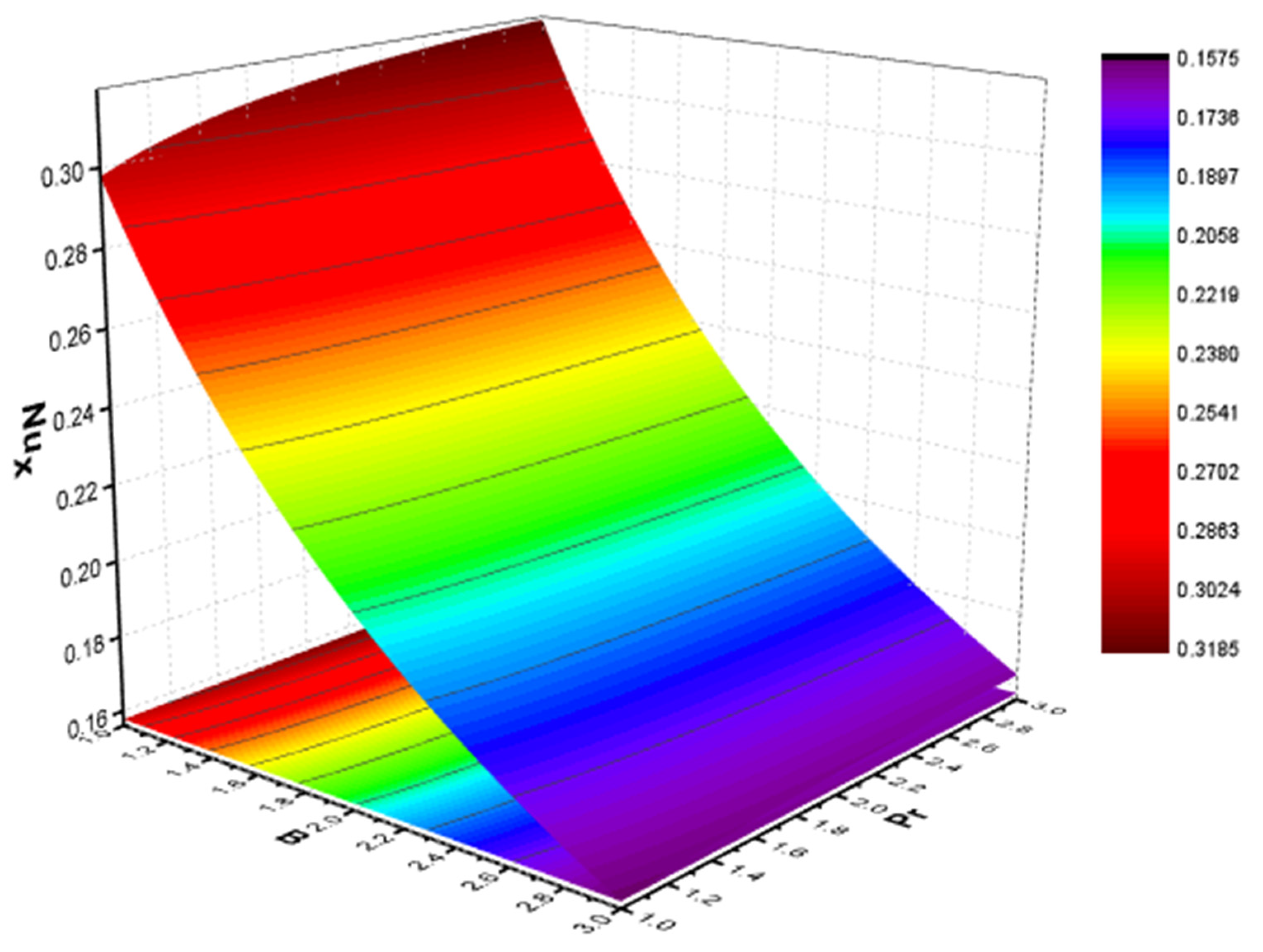
- The Nusselt number is diminished with the increase in the thermal slip parameter and heat generation parameter.
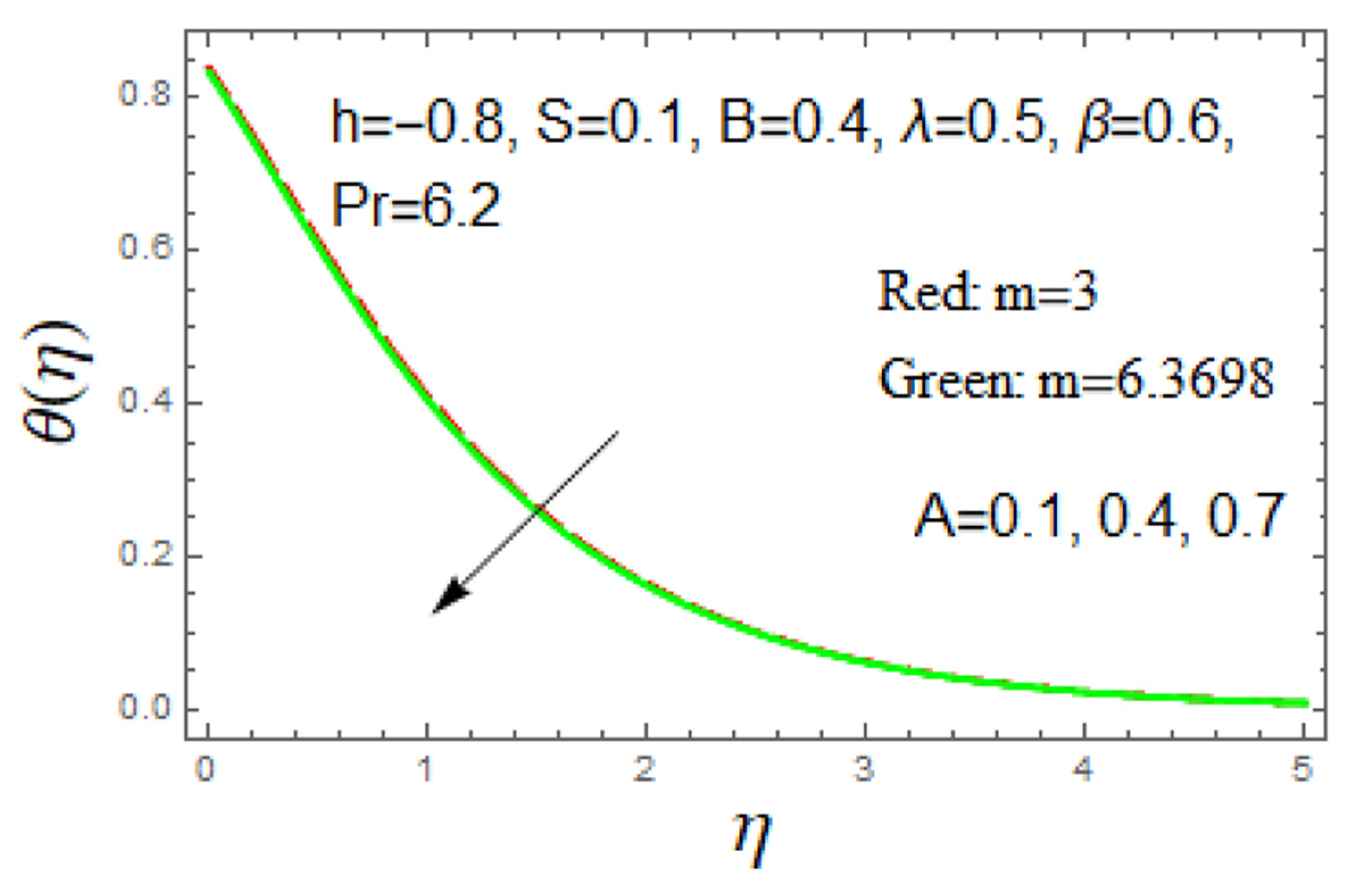
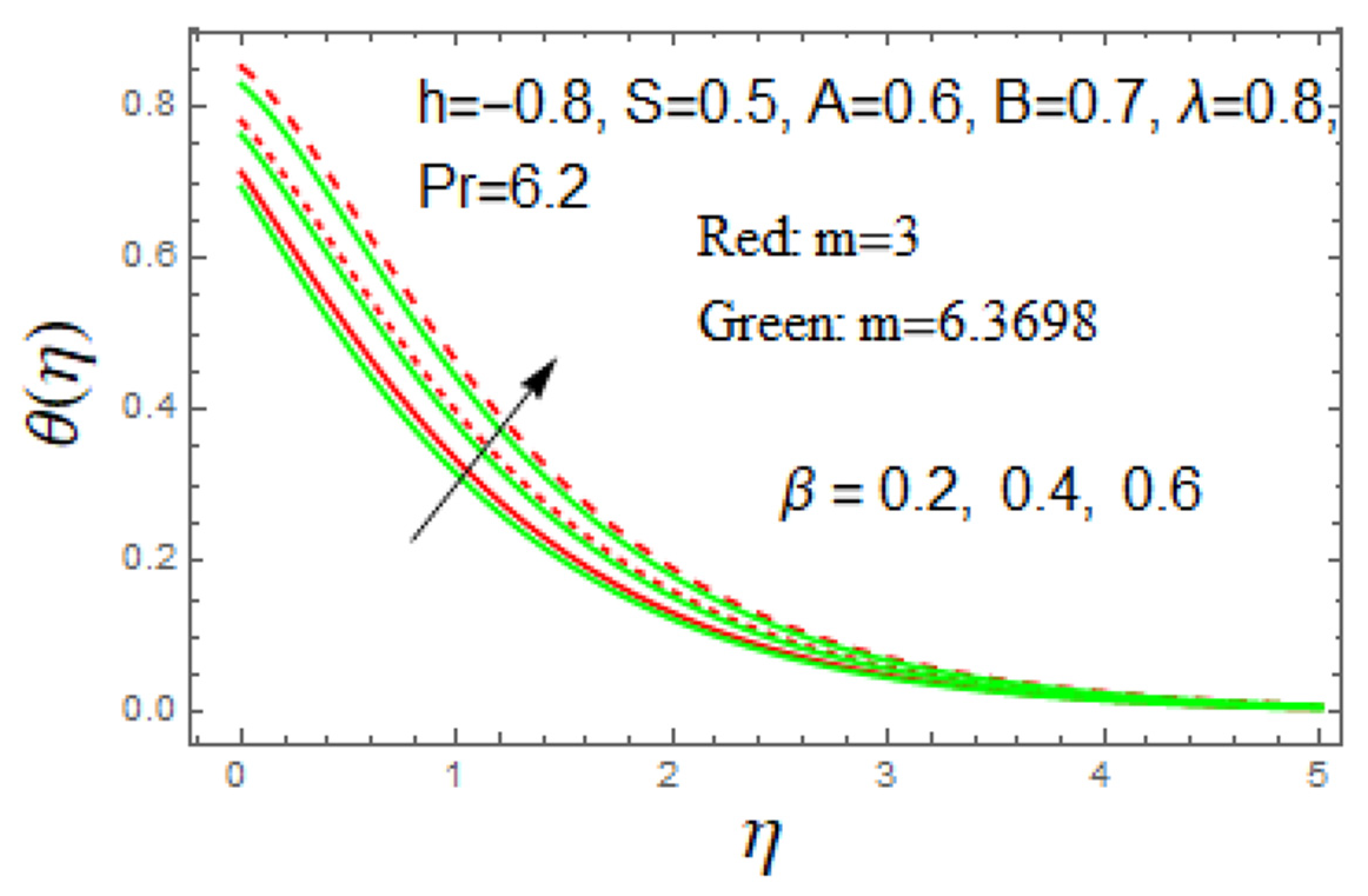
- The temperature distribution rises with the enhancement of the heat generation parameter, while it decreases when the thermal slip parameter and mass transfer parameter rise.
- It is concluded from Table 4 that the heat transfer rate increases with raising , while it decreases with the enhancement of .
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Maxwell, J.C. A Treatise on Electricity and Magnetism; Clarendon Press: Oxford, UK, 1873. [Google Scholar]
- Hamilton, R.L.; Crosser, O.K. Thermal Conductivity of Heterogeneous Two-Component Systems. Ind. Eng. Chem. Fundam. 1962, 1, 187–191. [Google Scholar] [CrossRef]
- Choi, S.U.S.; Eastman, J.A. Enhancing Thermal Conductivity of Fluids with Nanoparticles. ASME Fluids Eng. Div. 1995, 231, 99–106. [Google Scholar]
- Jana, S.; Salehi-Khojin, A.; Zhong, W.-H. Enhancement of fluid thermal conductivity by the addition of single and hybrid nano-additives. Thermochim. Acta 2007, 462, 45–55. [Google Scholar] [CrossRef]
- Jha, N.; Ramaprabhu, S. Thermal conductivity studies of metal dispersed multiwalled carbon nanotubes in water and ethylene glycol based nanofluids. J. Appl. Phys. 2009, 106, 084317. [Google Scholar] [CrossRef]
- Waini, I.; Ishak, A.; Pop, I. Hiemenz flow over a shrinking sheet in a hybrid nanofluid. Results Phys. 2020, 19, 103351. [Google Scholar] [CrossRef]
- Waini, I.; Ishak, A.; Pop, I. Hybrid nanofluid flow and heat transfer over a permeable biaxial stretching/shrinking sheet. Int. J. Numer. Methods Heat Fluid Flow 2019, 30, 3497–3513. [Google Scholar] [CrossRef]
- Waini, I.; Ishak, A.; Pop, I. Mixed convection flow over an exponentially stretching/shrinking vertical surface in a hybrid nanofluid. Alex. Eng. J. 2020, 59, 1881–1891. [Google Scholar] [CrossRef]
- Waini, I.; Ishak, A.; Pop, I. MHD flow and heat transfer of a hybrid nanofluid past a permeable stretching/shrinking wedge. Appl. Math. Mech. 2020, 41, 507–520. [Google Scholar] [CrossRef]
- Waini, I.; Ishak, A.; Pop, I. Hybrid nanofluid flow towards a stagnation point on an exponentially stretching/shrinking vertical sheet with buoyancy effects. Int. J. Numer. Methods Heat Fluid Flow 2021, 31, 216–235. [Google Scholar] [CrossRef]
- Li, H.; Ha, C.-S.; Kim, I. Fabrication of carbon nanotube/SiO2 and carbon nanotube/SiO2/Ag nanoparticles hybrids by using plasma treatment. Nanoscale Res. Lett. 2009, 4, 1384–1388. [Google Scholar] [CrossRef] [Green Version]
- Karthikeyan, N.; Philip, J.; Raj, B. Effect of clustering on the thermal conductivity of nanofluids. Mater. Chem. Phys. 2008, 109, 50–55. [Google Scholar] [CrossRef]
- Sastry, N.N.V.; Bhunia, A.; Sundararajan, T.; Das, S.K. Predicting the effective thermal conductivity of carbon nanotube based nanofluids. Nanotechnology 2008, 19, 055704. [Google Scholar] [CrossRef] [PubMed]
- Paul, G.; Philip, J.; Raj, B.; Das, P.K.; Manna, I. Synthesis, characterization, and thermal property measurement of nano-Al95Zn05 dispersed nanofluid prepared by a two-step process. Int. J. Heat Mass Transf. 2011, 54, 3783–3788. [Google Scholar] [CrossRef]
- Sidik, N.A.C.; Adamu, I.M.; Jamil, M.M. Preparation Methods and Thermal Performance of Hybrid Nanofluids. J. Adv. Res. Appl. Mech. 2020, 66, 7–16. [Google Scholar] [CrossRef]
- Choi, S.U.S.; Zhang, Z.G.; Yu, W.; Lockwood, F.E.; Grulke, E.A. Anomalous thermal conductivity enhancement in nanotube suspensions. Appl. Phys. Lett. 2001, 79, 2252–2254. [Google Scholar] [CrossRef]
- Nor Azwadi, C.S.; Adamu, I. Turbulent force convective heat transfer of hybrid nano fluid in a circular channel with constant heat flux. J. Adv. Res. Fluid Mech. Therm. Sci. 2016, 19, 1–9. [Google Scholar]
- Eastman, J.A.; Choi, S.U.-S.; Li, S.; Yu, W.; Thompson, L.J. Anomalously increased effective thermal conductivities of ethylene glycol-based nanofluids containing copper nanoparticles. Appl. Phys. Lett. 2001, 78, 718–720. [Google Scholar] [CrossRef]
- Xuan, Y.; Li, Q. Investigation on Convective Heat Transfer and Flow Features of Nanofluids. J. Heat Transf. 2003, 125, 151–155. [Google Scholar] [CrossRef] [Green Version]
- Li, D.; Hong, B.; Fang, W.; Guo, Y.; Lin, R. Preparation of Well-Dispersed Silver Nanoparticles for Oil-Based Nanofluids. Ind. Eng. Chem. Res. 2010, 49, 1697–1702. [Google Scholar] [CrossRef]
- Lee, Y.K. The use of nanofluids in domestic water heat exchanger. J. Adv. Res. Appl. Mech. 2014, 3, 9–24. [Google Scholar]
- Park, S.D.; Lee, S.W.; Kang, S.; Bang, I.C.; Kim, J.H.; Shin, H.S.; Lee, D.W. Effects of nanofluids containing graphene/graphene-oxide nanosheets on critical heat flux. Appl. Phys. Lett. 2010, 97, 023103. [Google Scholar] [CrossRef] [Green Version]
- Kang, S.W.; Wei, W.-C.; Tsai, S.-H.; Yang, S.-Y. Experimental investigation of silver nano-fluid on heat pipe thermal performance. Appl. Therm. Eng. 2006, 26, 2377–2382. [Google Scholar] [CrossRef]
- Abubakar, S.; Nor Azwadi, C.S.; Ahmad, A. The use of Fe3O4-H2O4 nanofluid for heat transfer enhancement in rectangular microchannel Heatsink. J. Adv. Res. Mater. Sci. 2016, 23, 15–24. [Google Scholar]
- Yashkun, U.; Zaimi, K.; Abu Bakar, N.A.; Ishak, A.; Pop, I. MHD hybrid nanofluid flow over a permeable stretching/shrinking sheet with thermal radiation effect. Int. J. Numer. Methods Heat Fluid Flow 2021, 31, 1014–1031. [Google Scholar] [CrossRef]
- Lund, L.A.; Omar, Z.; Khan, I.; Seikh, A.H.; Sherif, E.-S.M.; Nisar, K.S. Stability analysis and multiple solution of Cu–Al2O3/H2O nanofluid contains hybrid nanomaterials over a shrinking surface in the presence of viscous dissipation. J. Mater. Res. Technol. 2020, 9, 421–432. [Google Scholar] [CrossRef]
- Lund, L.A.; Omar, Z.; Khan, I.; Sherif, E.-S.M. Dual Solutions and Stability Analysis of a Hybrid Nanofluid over a Stretching/Shrinking Sheet Executing MHD Flow. Symmetry 2020, 12, 276. [Google Scholar] [CrossRef] [Green Version]
- Aladdin, N.A.L.; Bachok, N.; Pop, I. Cu-Al2O3/Water Hybrid Nanofluid Flow over a Permeable Moving Surface in Presence of Hydromagnetic and Suction Effects. Alex. Eng. J. 2020, 59, 657–666. [Google Scholar] [CrossRef]
- Roşca, N.C.; Roşca, A.V.; Jafarimoghaddam, A.; Pop, I. Cross flow and heat transfer past a permeable stretching/shrinking sheet in a hybrid nanofluid. Int. J. Numer. Methods Heat Fluid Flow 2021, 31, 1295–1319. [Google Scholar] [CrossRef]
- Khashi’Ie, N.S.; Arifin, N.M.; Pop, I.; Nazar, R. Melting heat transfer in hybrid nanofluid flow along a moving surface. J. Therm. Anal. Calorim. 2020, 1–12. [Google Scholar] [CrossRef]
- Khashi’Ie, N.S.; Arifin, N.M.; Pop, I.; Nazar, R.; Hafidzuddin, E.H.; Wahi, N. Flow and heat transfer past a permeable power-law deformable plate with orthogonal shear in a hybrid nanofluid. Alex. Eng. J. 2020, 59, 1869–1879. [Google Scholar] [CrossRef]
- Khashi’Ie, N.S.; Arifin, N.M.; Pop, I.; Wahid, N.S. Flow and heat transfer of hybrid nanofluid over a permeable shrinking cylinder with Joule heating: A comparative analysis. Alex. Eng. J. 2020, 59, 1787–1798. [Google Scholar] [CrossRef]
- Khashi’ie, N.S.; Md Arifin, N.; Pop, I. Mixed Convective Stagnation Point Flow towards a Vertical Riga Plate in Hybrid Cu-Al2O3/Water Nanofluid. Mathematics 2020, 8, 912. [Google Scholar] [CrossRef]
- Roy, N.C.; Pop, I. Flow and heat transfer of a second-grade hybrid nanofluid over a permeable stretching/shrinking sheet. Eur. Phys. J. Plus 2020, 135, 1–19. [Google Scholar] [CrossRef]
- Zainal, N.A.; Nazar, R.; Naganthran, K.; Pop, I. MHD flow and heat transfer of hybrid nanofluid over a permeable moving surface in the presence of thermal radiation. Int. J. Numer. Methods Heat Fluid Flow 2021, 31, 858–879. [Google Scholar] [CrossRef]
- Anuar, N.S.; Bachok, N.; Pop, I. Radiative hybrid nanofluid flow past a rotating permeable stretching/shrinking sheet. Int. J. Numer. Methods Heat Fluid Flow 2021, 31, 914–932. [Google Scholar] [CrossRef]
- Gangadhar, K.; Bhargavi, D.N.; Kannan, T.; Rao, M.V.S.; Chamkha, A.J. Transverse MHD flow of Al2O3-Cu/H2O hybrid nanofluid with active radiation: A novel hybrid model. Math. Methods Appl. Sci. 2020, 1–19. [Google Scholar] [CrossRef]
- Wahid, N.S.; Md Arifin, N.; Turkyilmazoglu, M.; Hafidzuddin, M.E.H.; Abd Rahmin, N.A. MHD Hybrid Cu-Al2O3/Water Nanofluid Flow with Thermal Radiation and Partial Slip Past a Permeable Stretching Surface: Analytical Solution. J. Nano Res. 2020, 64, 75–91. [Google Scholar] [CrossRef]
- Khadija, M.; Naeema, M.; Rahmat, I.; Sadiq, M.S. Influence of heat transfer on MHD Carreau fluid flow due to motile cilia in a channel. J. Therm. Anal. Calorim. 2021, 144, 2317–2326. [Google Scholar]
- Rajesh, V.; Sheremet, M.A.; Hakan, F.O. Impact of hybrid nanofluids on MHD flow and heat transfer near a vertical plate with ramped wall temperature. Case Stud. Therm. Eng. 2021, 28, 101557. [Google Scholar] [CrossRef]
- Othman, M.N.; Jedi, A.; Abu Bakar, N.A. MHD Flow and Heat Transfer of Hybrid Nanofluid over an Exponentially Shrinking Surface with Heat Source/Sink. Appl. Sci. 2021, 11, 8199. [Google Scholar] [CrossRef]
- Murugesan, T.; Kumar, M.D. Effects of Thermal Radiation and Heat Generation on Hydromagnetic Flow of Nanofluid over an Exponentially Stretching Sheet in a Porous Medium with Viscous Dissipation. World Sci. News 2019, 128, 130–147. [Google Scholar]
- Ghosh, S.; Mukhopadhyay, S. Stability analysis for model-based study of nanofluid flow over an exponentially shrinking permeable sheet in presence of slip. Neural Comput. Appl. 2020, 32, 7201–7211. [Google Scholar] [CrossRef]
- Tayebi, T.; Chamkha, A.J. Entropy generation analysis during MHD natural convection flow of hybrid nanofluid in a square cavity containing a corrugated conducting block. Int. J. Numer. Methods Heat Fluid Flow 2019, 30, 1115–1136. [Google Scholar] [CrossRef]
- Takabi, B.; Salehi, S. Augmentation of the Heat Transfer Performance of a Sinusoidal Corrugated Enclosure by Employing Hybrid Nanofluid. Adv. Mech. Eng. 2014, 6, 147059. [Google Scholar] [CrossRef]
- Oztop, H.F.; Abu-Nada, E. Numerical study of natural convection in partially heated rectangular enclosures filled with nanofluids. Int. J. Heat Fluid Flow 2008, 29, 1326–1336. [Google Scholar] [CrossRef]
- Waini, I.; Ishak, A.; Pop, I. Hybrid nanofluid flow induced by an exponentially shrinking sheet. Chin. J. Phys. 2020, 68, 468–482. [Google Scholar] [CrossRef]
- Liao, S.L. The Proposed Homotopy Analysis Technique for the Solution of Nonlinear Problems. Ph.D. Thesis, Shanghai Jiao Tong University, Shanghai, China, 1992. [Google Scholar]
- Liao, S. An optimal homotopy-analysis approach for strongly nonlinear differential equations. Commun. Nonlinear Sci. Numer. Simul. 2010, 15, 2003–2016. [Google Scholar] [CrossRef]


| Properties | Hybrid Nanofluid Correlations |
|---|---|
| Density | |
| Heat Capacity | |
| Dynamic Viscosity | |
| Thermal Conductivity |
| Physical Properties | Blood | ||
|---|---|---|---|
| 1053 | 3970 | 8933 | |
| 3594 | 765 | 385 | |
| 0.492 | 40 | 400 |
Cylindrical Shape | Spherical Shape | ||||
|---|---|---|---|---|---|
| 0.4 | 0.3 | 0.5 | 0.4 | −0.117305 * | −0.117305 |
| 0.5 | −0.113748 | −0.113748 | |||
| 0.6 | −0.110191 | −0.110191 | |||
| 0.4 | 0.3 | −0.117305 | −0.117305 | ||
| 0.4 | −0.108694 | −0.108694 | |||
| 0.5 | −0.101673 | −0.101673 | |||
| 0.3 | 0.5 | −0.117305 | −0.117305 | ||
| 0.6 | −0.110741 | −0.110741 | |||
| 0.7 | −0.104883 | −0.104883 | |||
| 0.5 | 0.4 | −0.117305 | −0.117305 | ||
| 0.5 | −0.113921 | −0.113921 | |||
| 0.6 | −0.110592 | −0.110592 |
Cylindrical Shape | Spherical Shape | |||
|---|---|---|---|---|
| 0.7 | 0.1 | 0.3 | 0.444816 * | 0.501535 |
| 0.8 | 0.462944 | 0.512572 | ||
| 0.9 | 0.477043 | 0.521157 | ||
| 0.7 | 0.1 | 0.444816 | 0.501535 | |
| 0.2 | 0.420066 | 0.467725 | ||
| 0.3 | 0.397374 | 0.437983 | ||
| 0.1 | 0.3 | 0.444816 | 0.501535 | |
| 0.4 | 0.439307 | 0.496025 | ||
| 0.5 | 0.433797 | 0.490516 |
| Numerical Iteration (m) | Velocity Profile | Temperature Profile |
|---|---|---|
| 4 | 0.18939 | |
| 8 | 0.0025323 | |
| 12 | ||
| 16 | ||
| 20 |
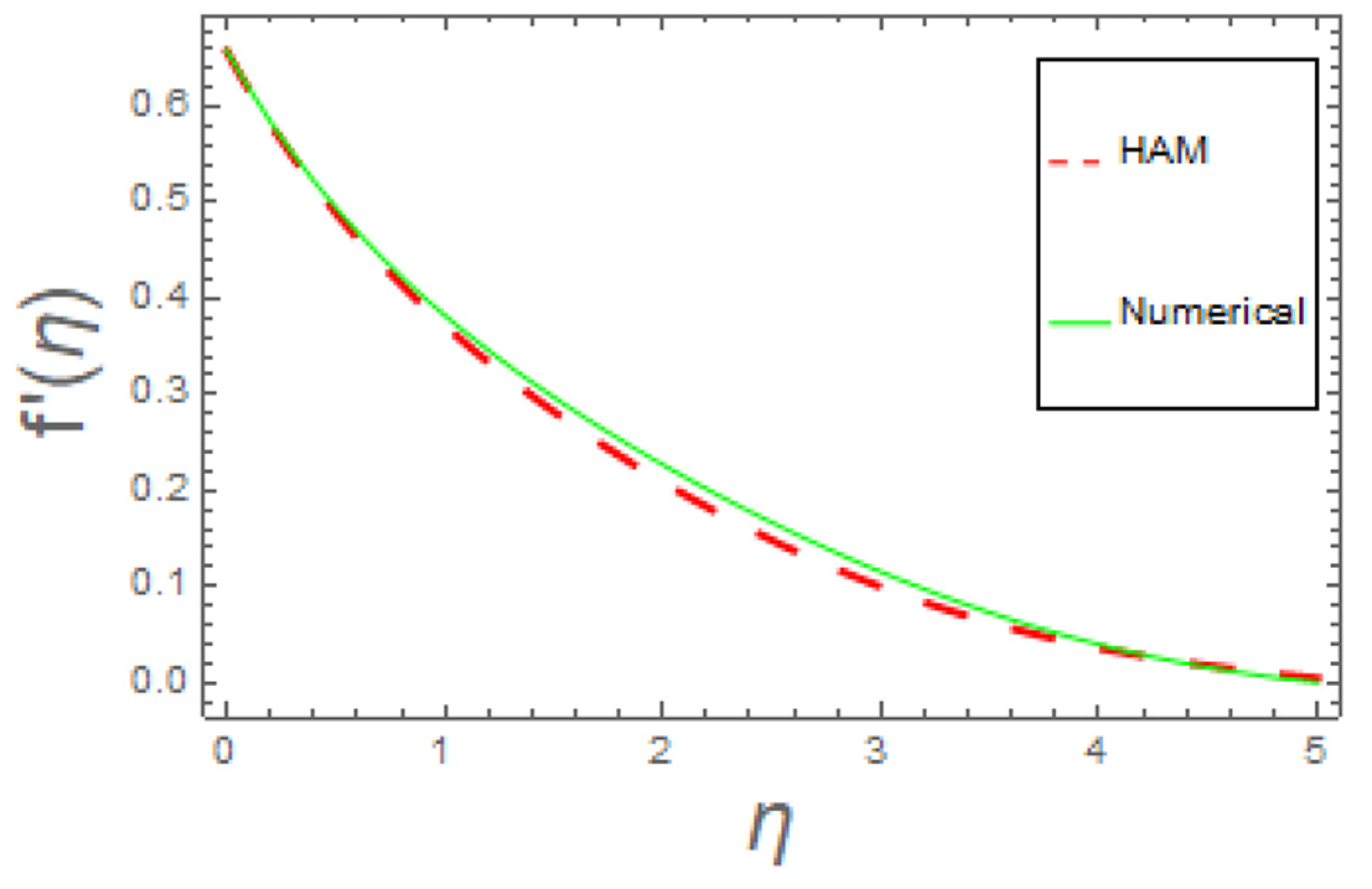
| HAM Solution | Numerical Solution (Shooting Method) | Absolute Error | |
|---|---|---|---|
| 0.0 | 0.658510 | 0.659357 | 0.000847 |
| 0.5 | 0.490030 | 0.495299 | 0.005269 |
| 1.0 | 0.372866 | 0.382860 | 0.009995 |
| 1.5 | 0.283323 | 0.297442 | 0.014119 |
| 2.0 | 0.210825 | 0.227093 | 0.016268 |
| 2.5 | 0.151427 | 0.166819 | 0.015392 |
| 3.0 | 0.103891 | 0.115291 | 0.011399 |
| 3.5 | 0.067335 | 0.072721 | 0.005385 |
| 4.0 | 0.040156 | 0.039517 | 0.000639 |
| 4.5 | 0.019973 | 0.015563 | 0.004410 |
| 5.0 | 0.004288 | 0.004288 |
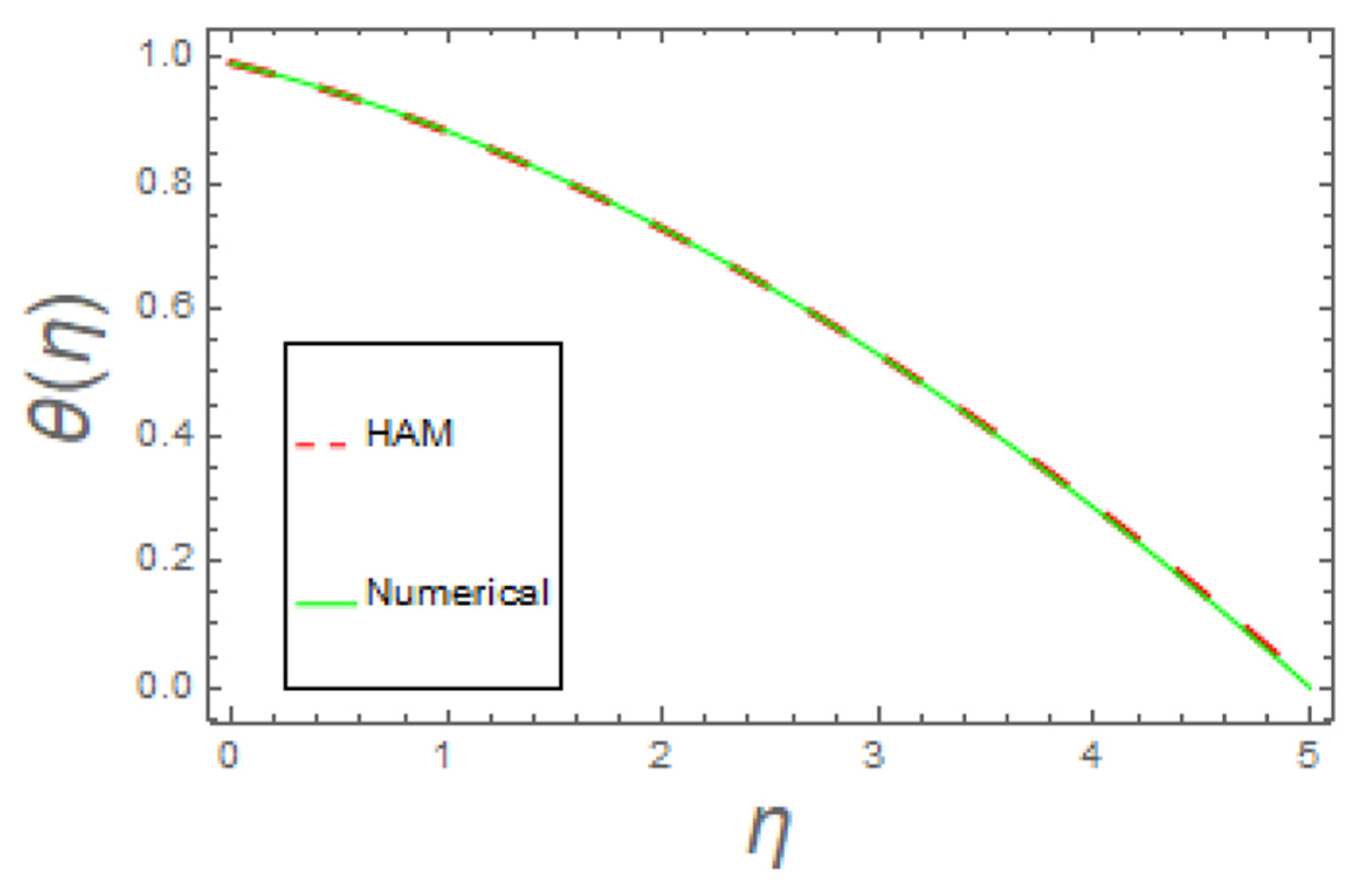
| HAM Solution | Numerical Solution (Shooting Method) | Absolute Error | |
|---|---|---|---|
| 0.0 | 0.942573 | 0.943156 | 0.000583 |
| 0.5 | 0.881382 | 0.882394 | 0.001008 |
| 1.0 | 0.812507 | 0.813847 | 0.001340 |
| 1.5 | 0.736136 | 0.737613 | 0.001477 |
| 2.0 | 0.652458 | 0.653804 | 0.001347 |
| 2.5 | 0.561613 | 0.562549 | 0.000936 |
| 3.0 | 0.463738 | 0.463989 | 0.000251 |
| 3.5 | 0.358965 | 0.358280 | 0.000685 |
| 4.0 | 0.247422 | 0.245590 | 0.001832 |
| 4.5 | 0.129223 | 0.126099 | 0.003123 |
| 5.0 | 0.004492 | 0.004492 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Khan, A.S.; Xu, H.-Y.; Khan, W. Magnetohydrodynamic Hybrid Nanofluid Flow Past an Exponentially Stretching Sheet with Slip Conditions. Mathematics 2021, 9, 3291. https://doi.org/10.3390/math9243291
Khan AS, Xu H-Y, Khan W. Magnetohydrodynamic Hybrid Nanofluid Flow Past an Exponentially Stretching Sheet with Slip Conditions. Mathematics. 2021; 9(24):3291. https://doi.org/10.3390/math9243291
Chicago/Turabian StyleKhan, Abdul Samad, He-Yong Xu, and Waris Khan. 2021. "Magnetohydrodynamic Hybrid Nanofluid Flow Past an Exponentially Stretching Sheet with Slip Conditions" Mathematics 9, no. 24: 3291. https://doi.org/10.3390/math9243291
APA StyleKhan, A. S., Xu, H.-Y., & Khan, W. (2021). Magnetohydrodynamic Hybrid Nanofluid Flow Past an Exponentially Stretching Sheet with Slip Conditions. Mathematics, 9(24), 3291. https://doi.org/10.3390/math9243291






