Mathematical Modeling and Optimal Control of the Hand Foot Mouth Disease Affected by Regional Residency in Thailand
Abstract
:1. Introduction
2. Methodology
2.1. Mathematical Model
2.2. Stability Analysis
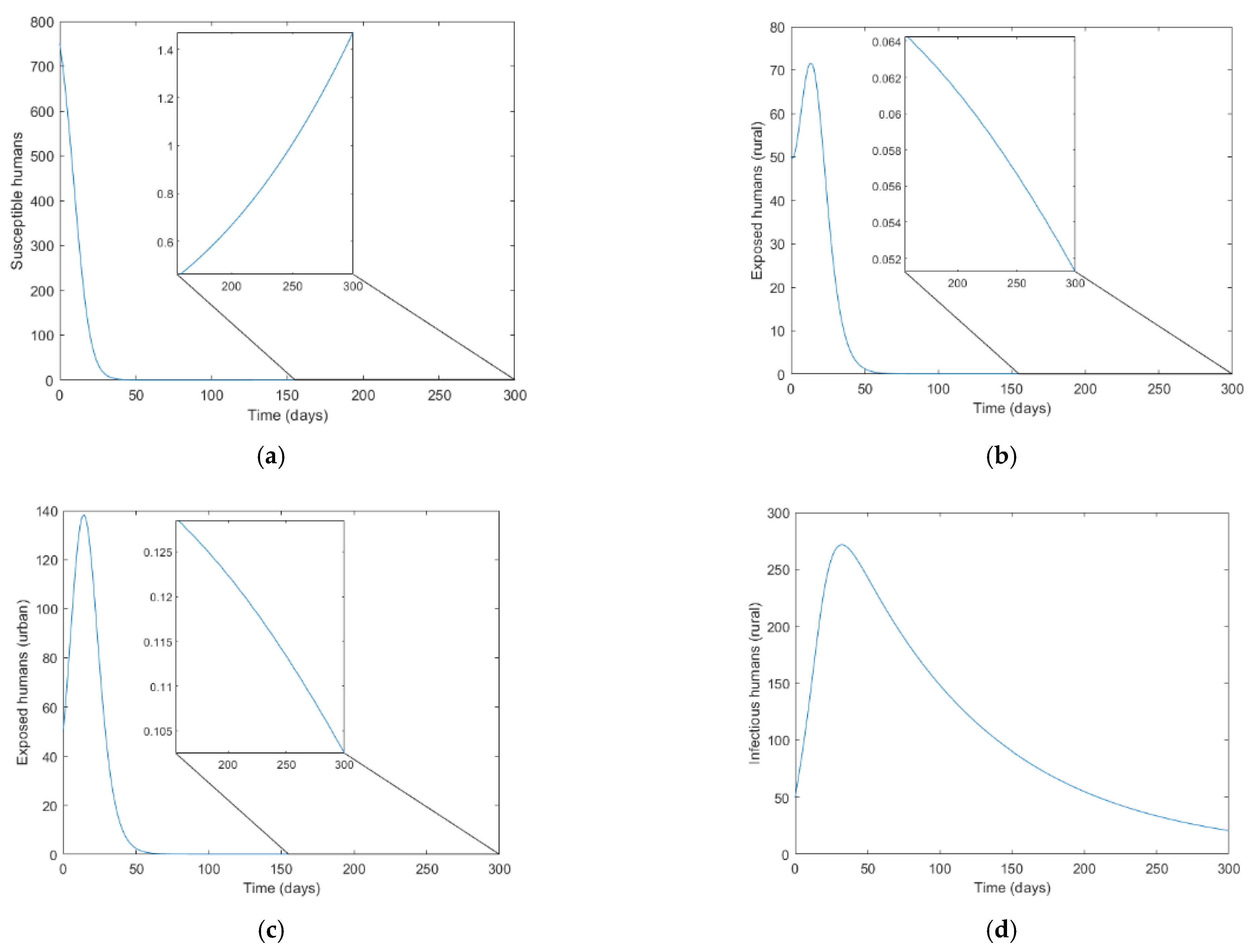
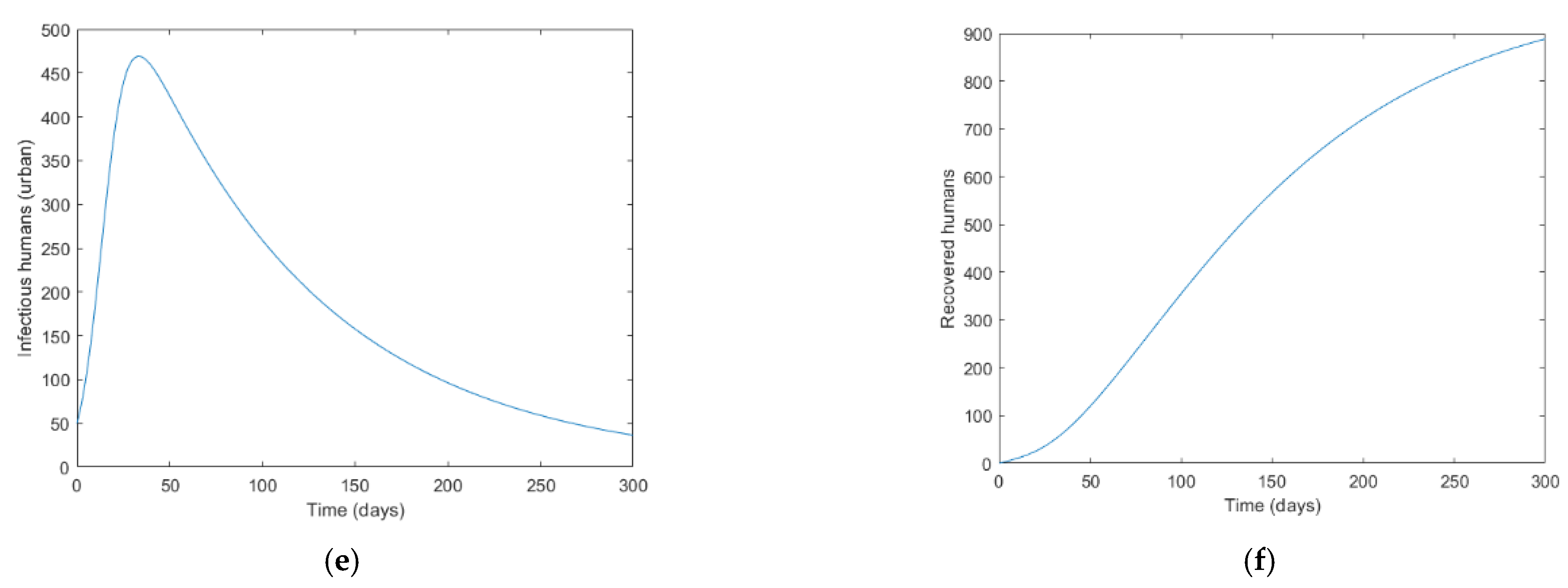
2.3. Numerical Simulation
3. General Settings of the Optimal Control Problem
3.1. Policy 1: Using Treatment Only
3.2. Policy 2: Using Both Treatment and Vaccination
Optimal Control Characterization
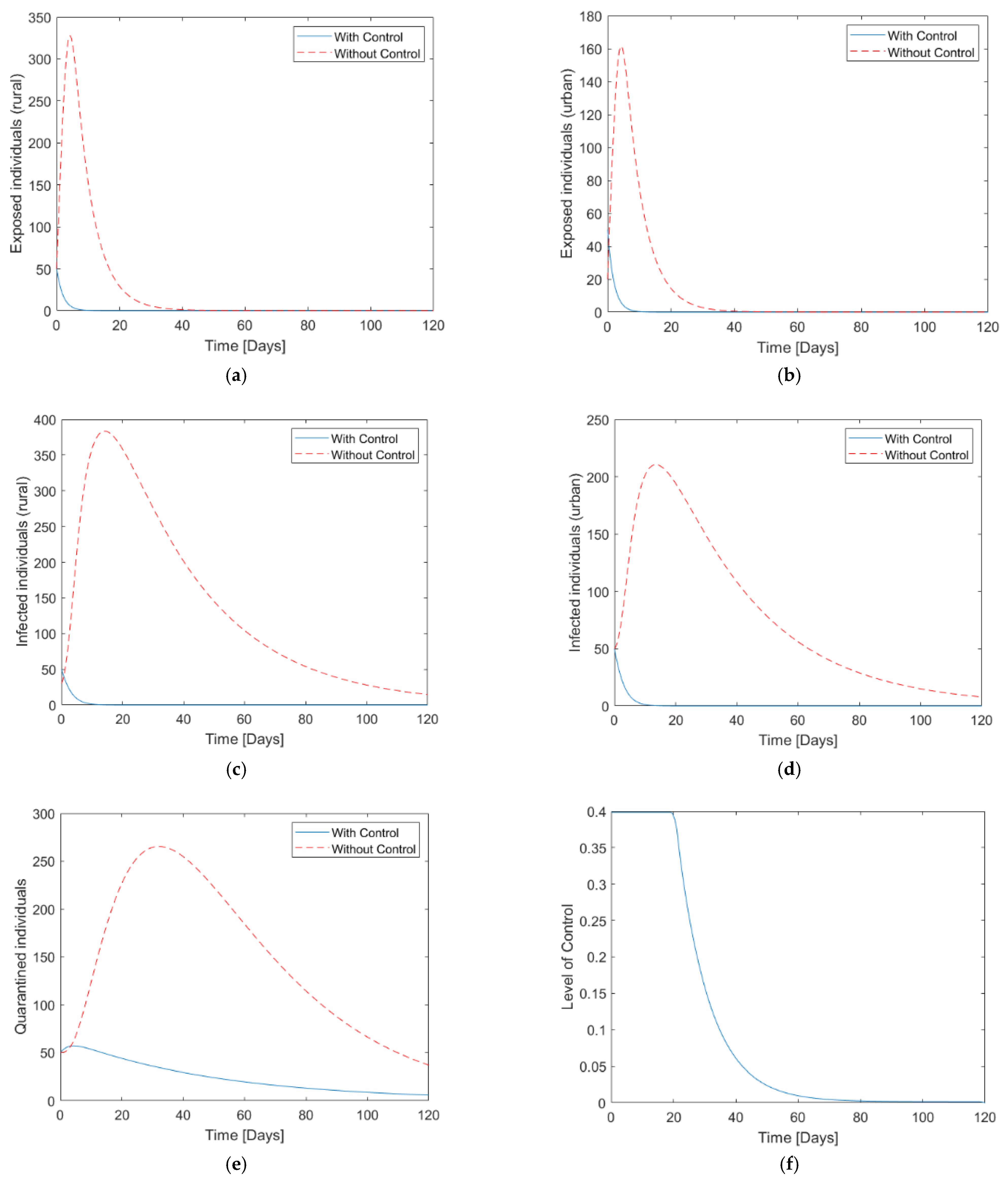
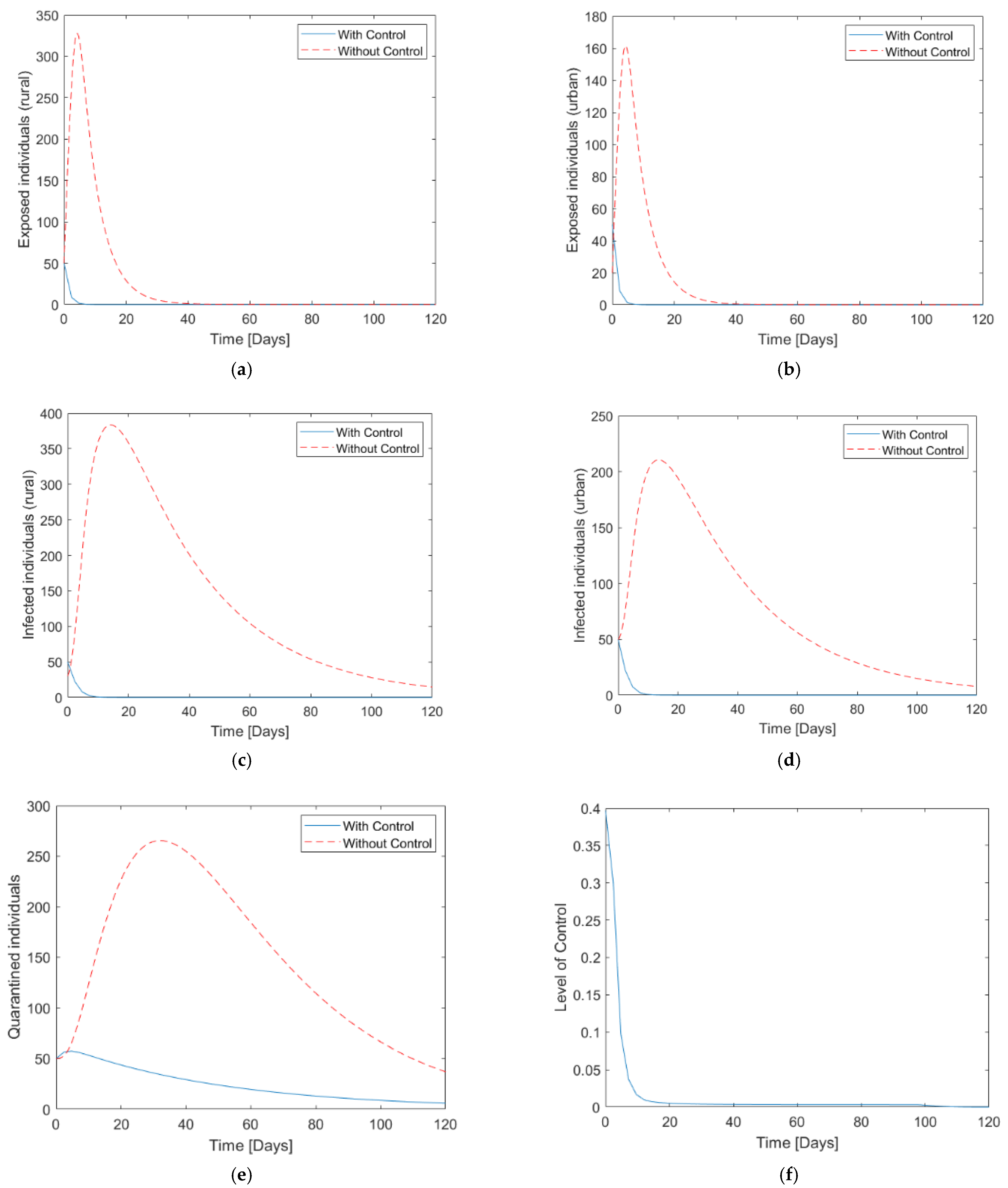
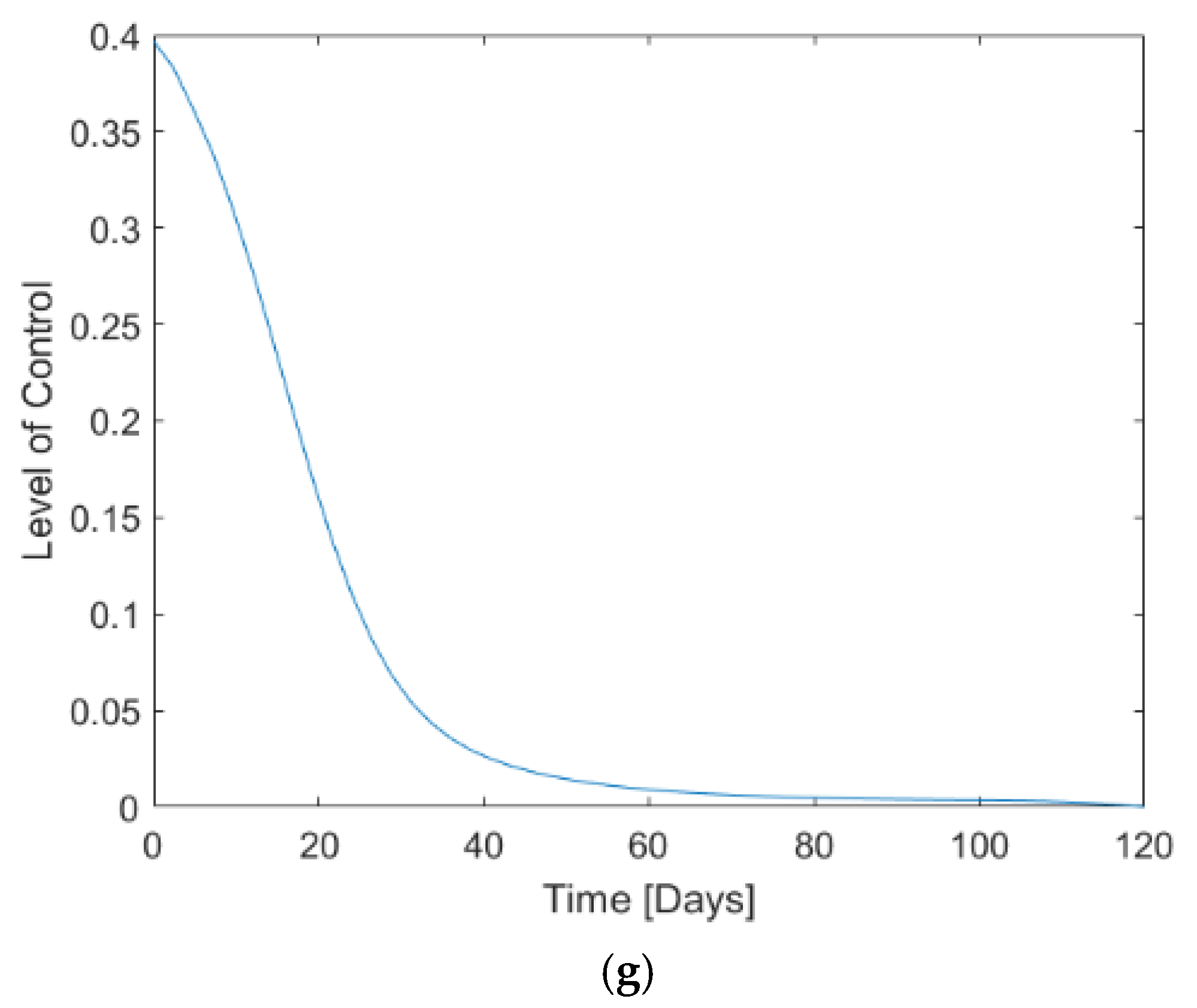
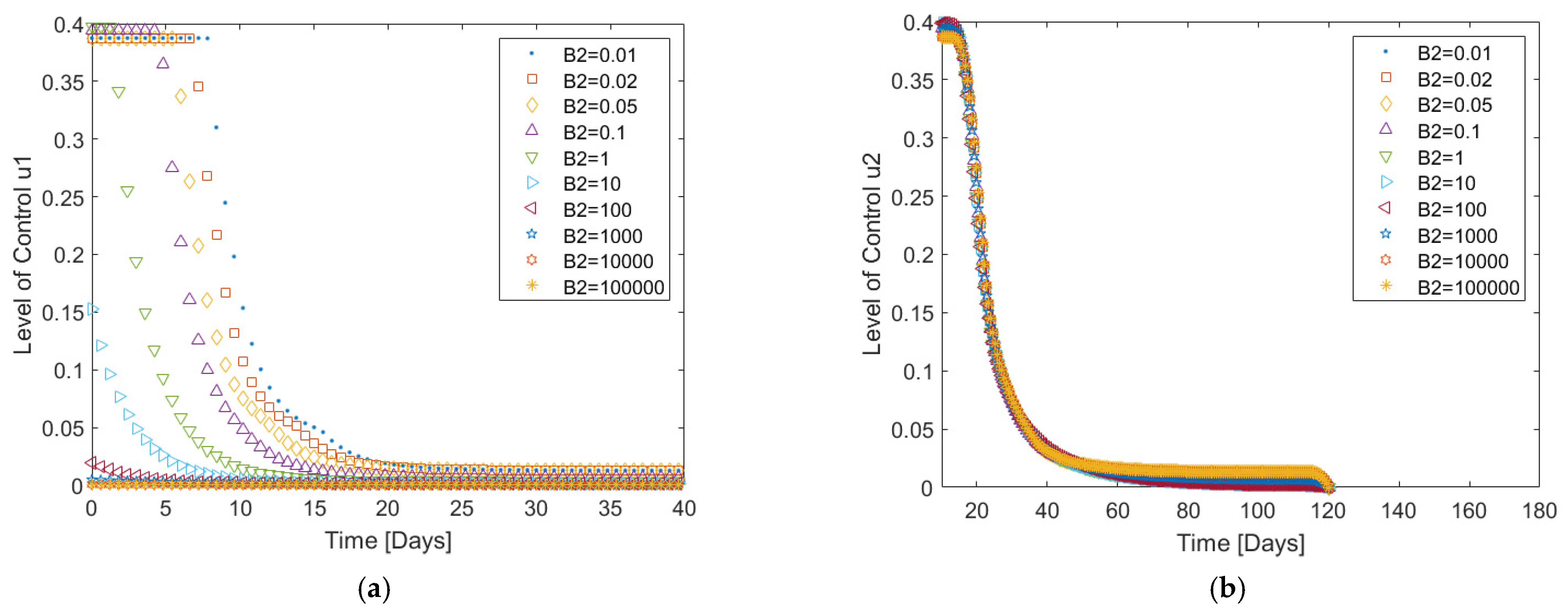
4. Optimal Control Results
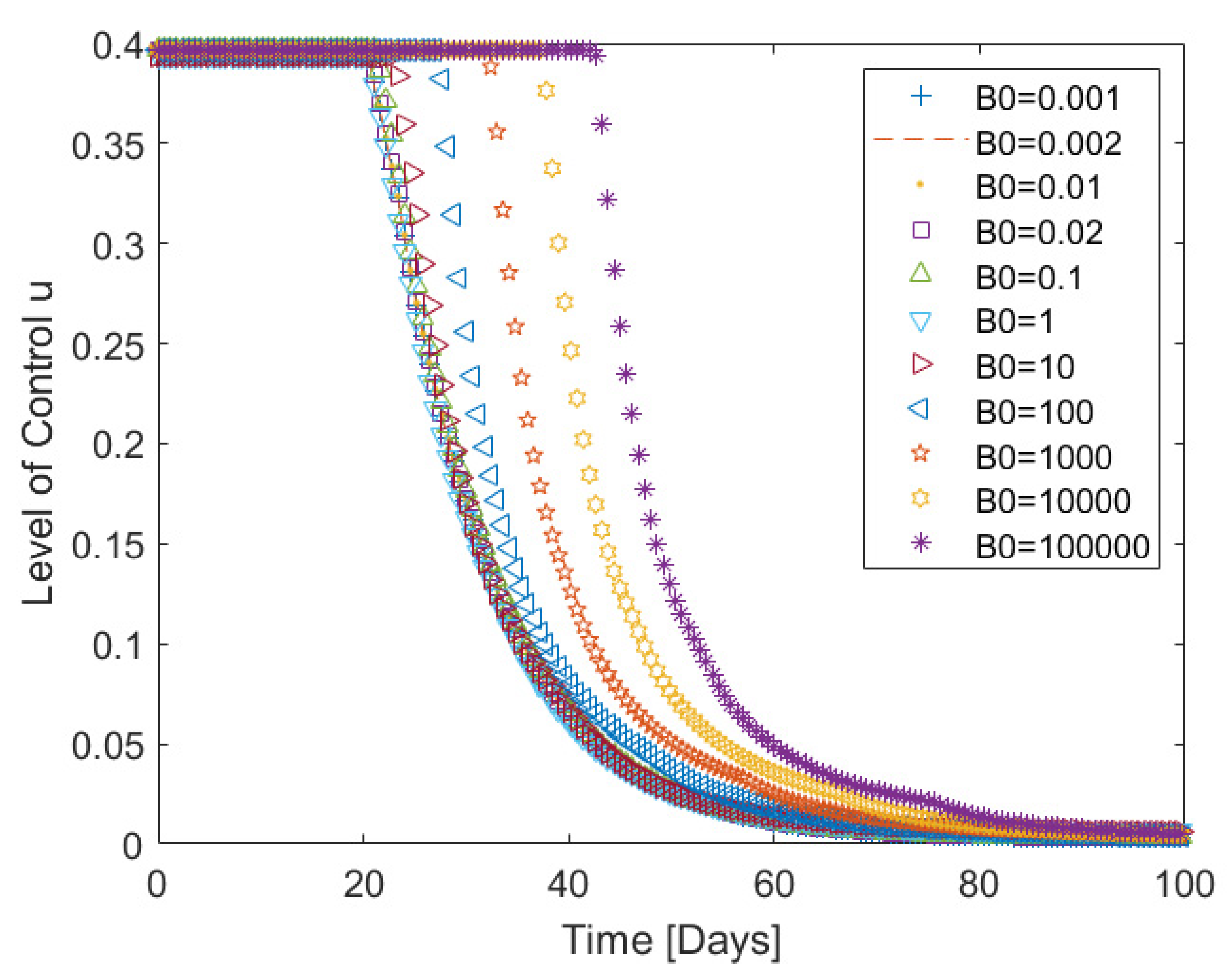
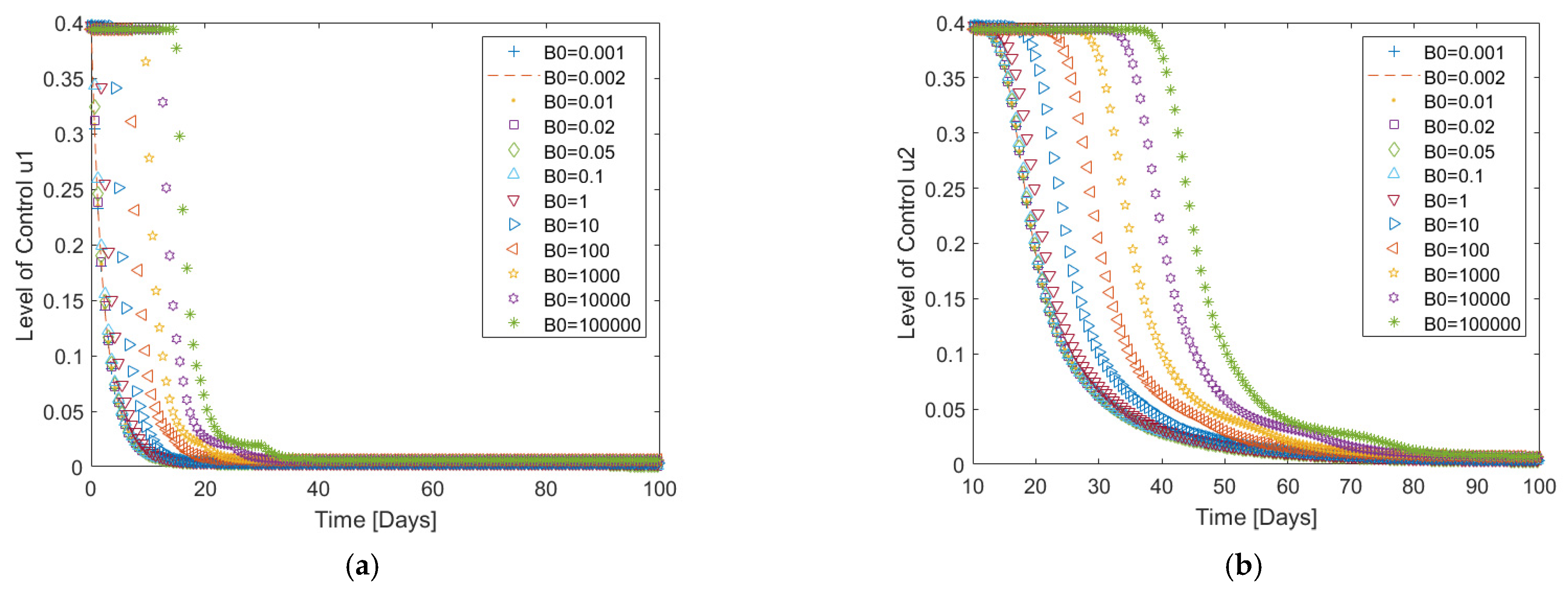
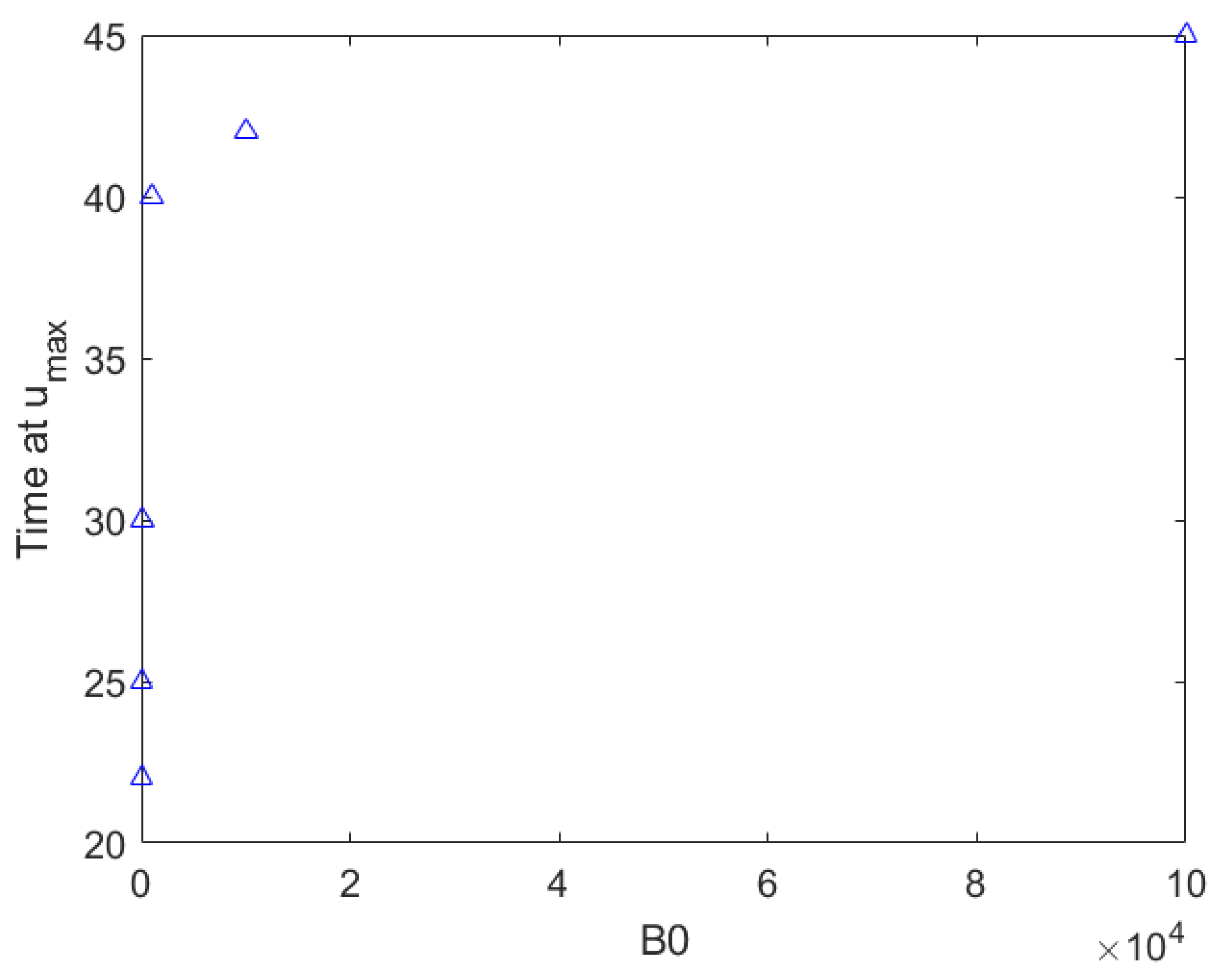
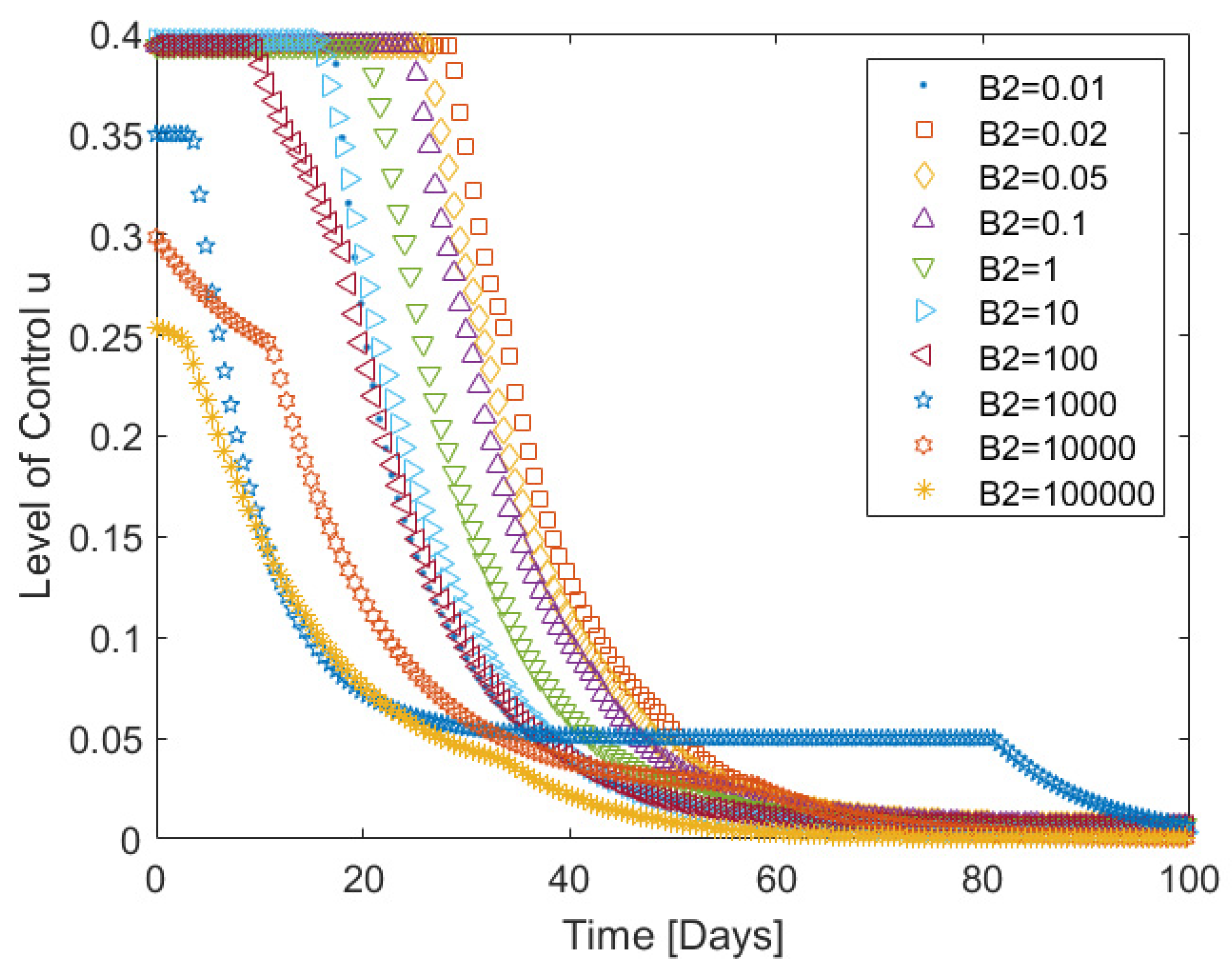
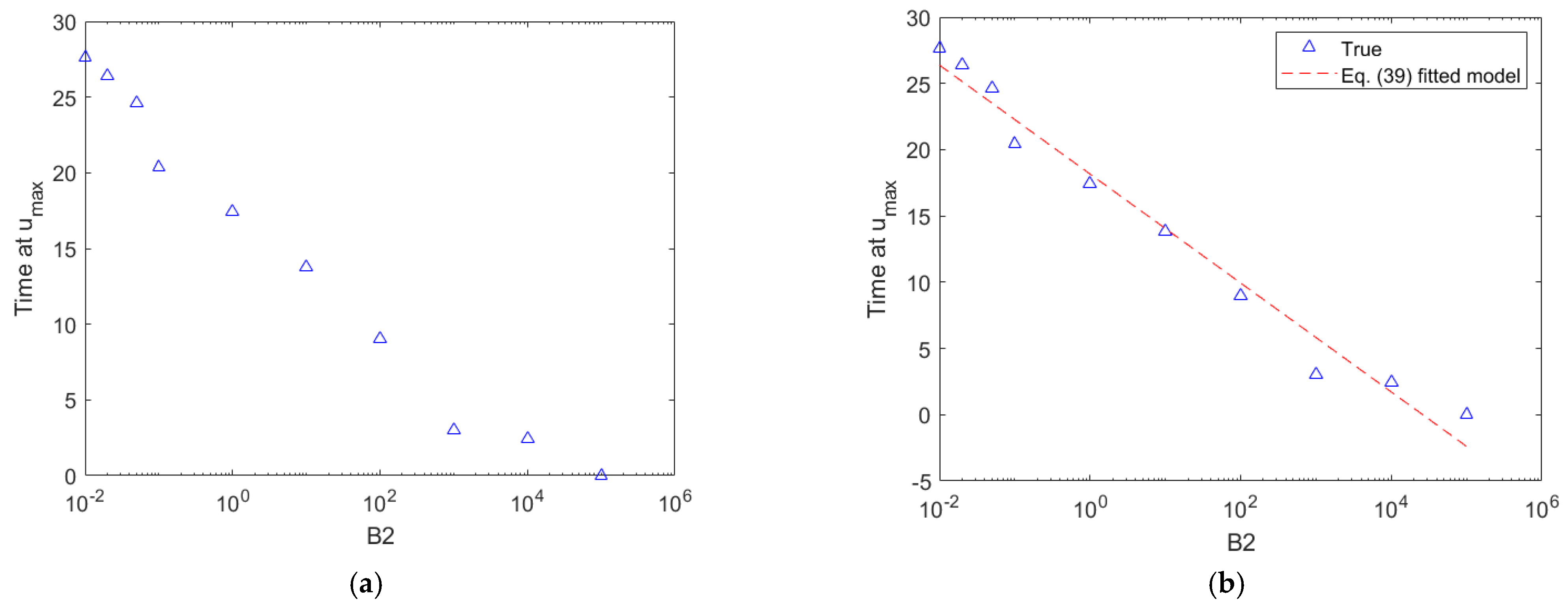
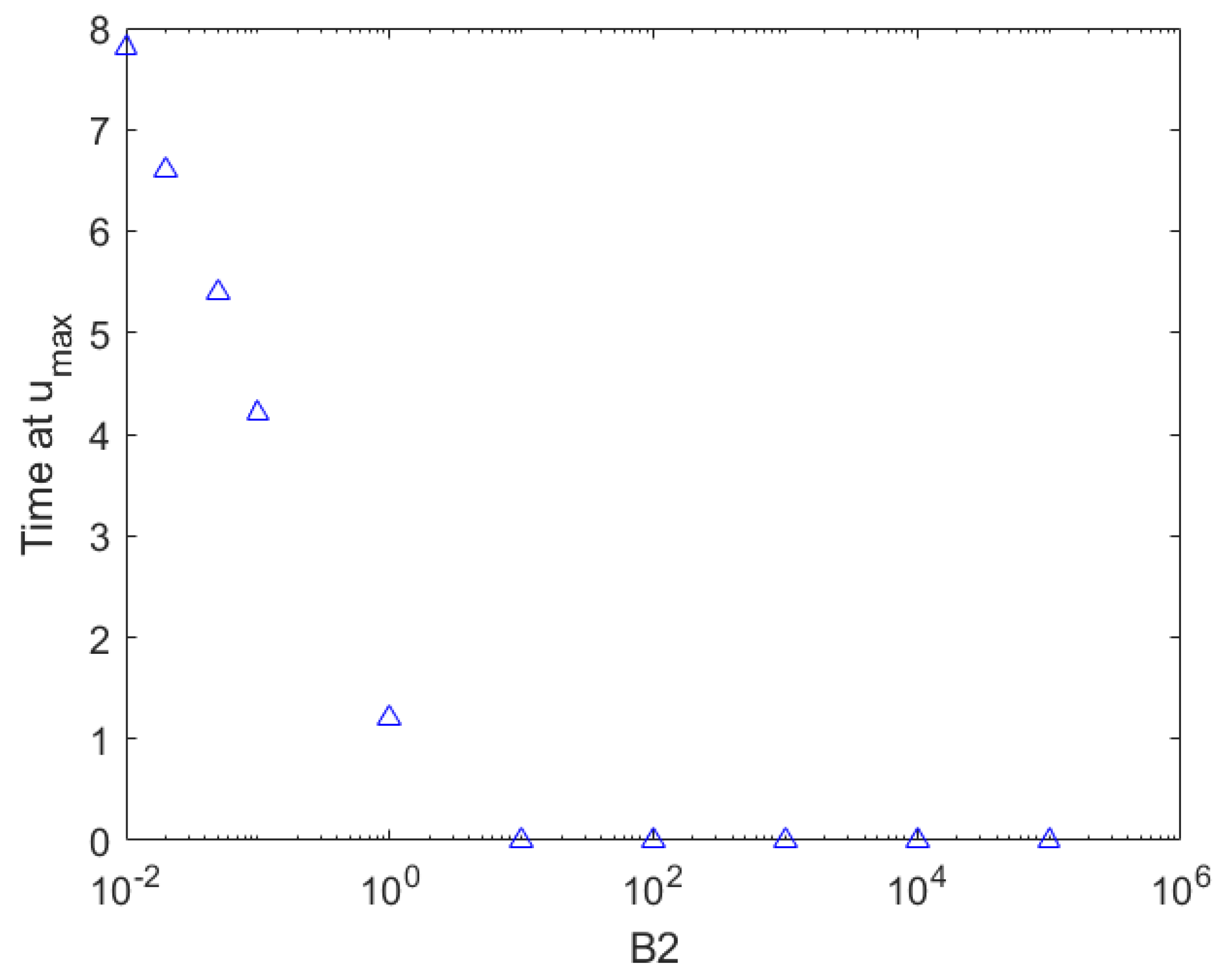
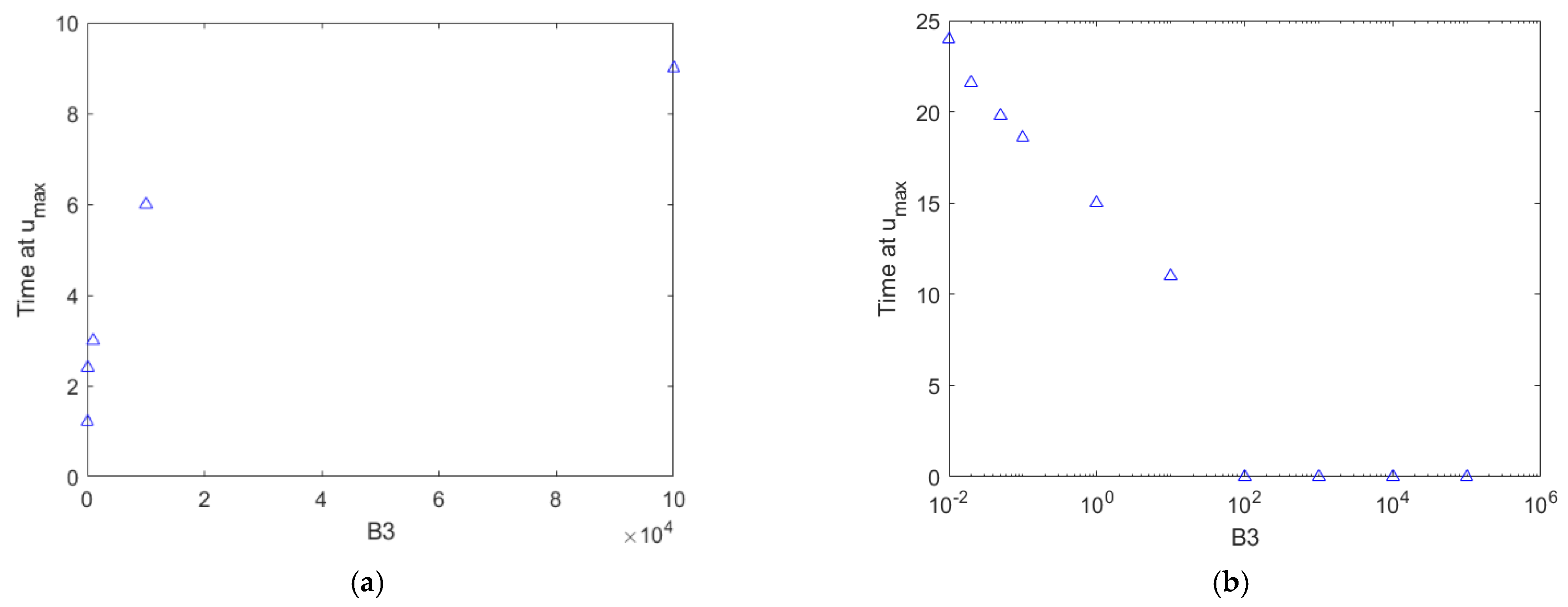
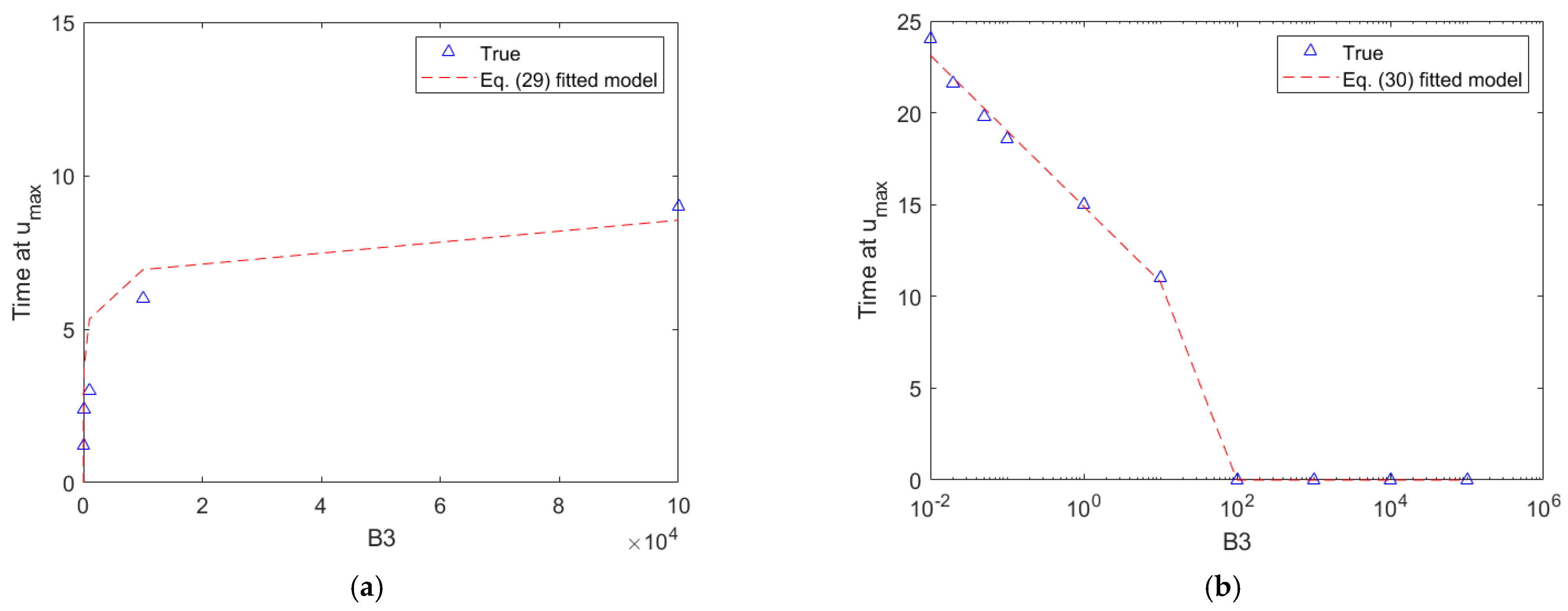
5. Optimal Control Parameters Investigation
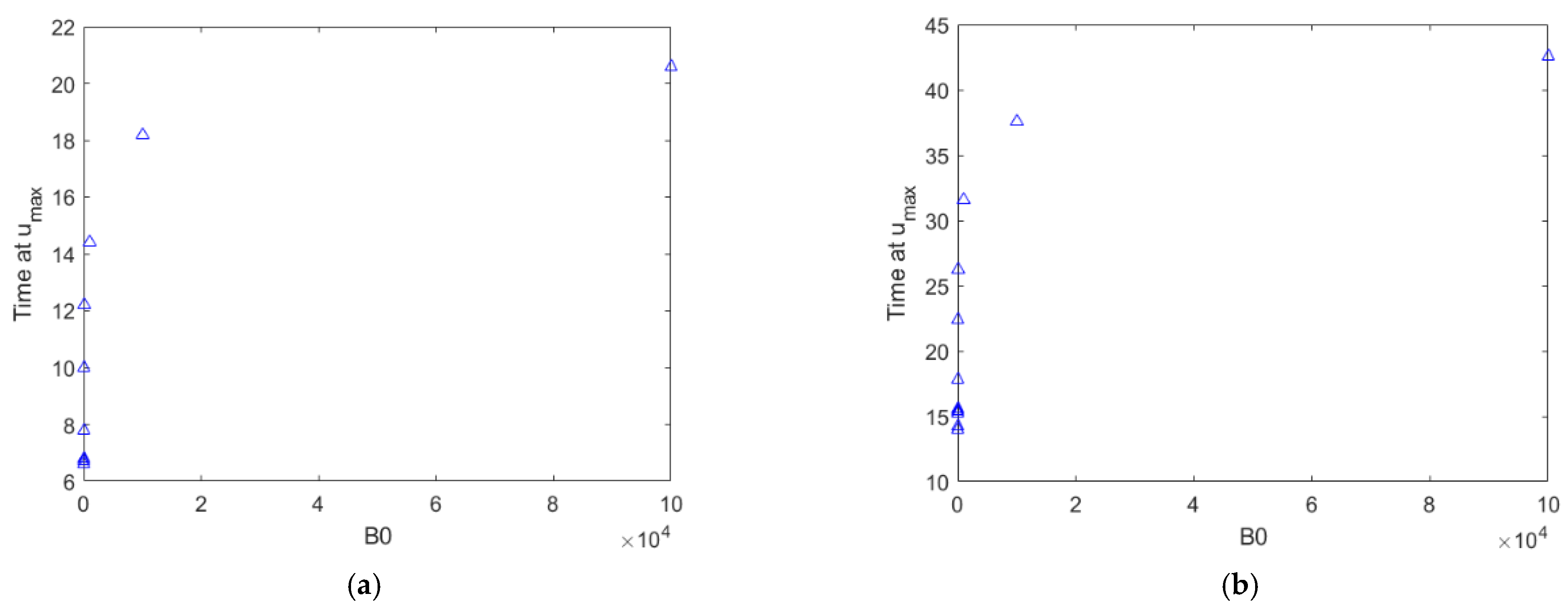
5.1. Changes in B0 Parameters
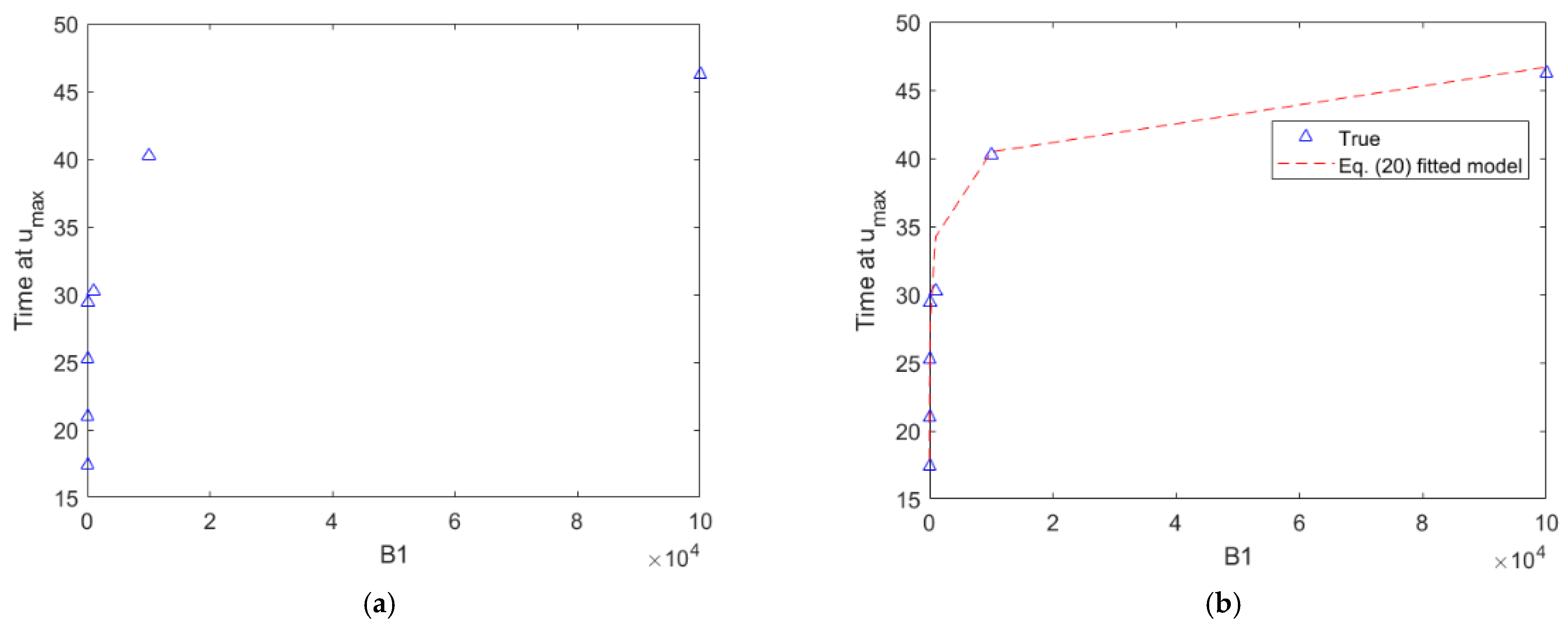
5.2. Changes in B1 Parameters
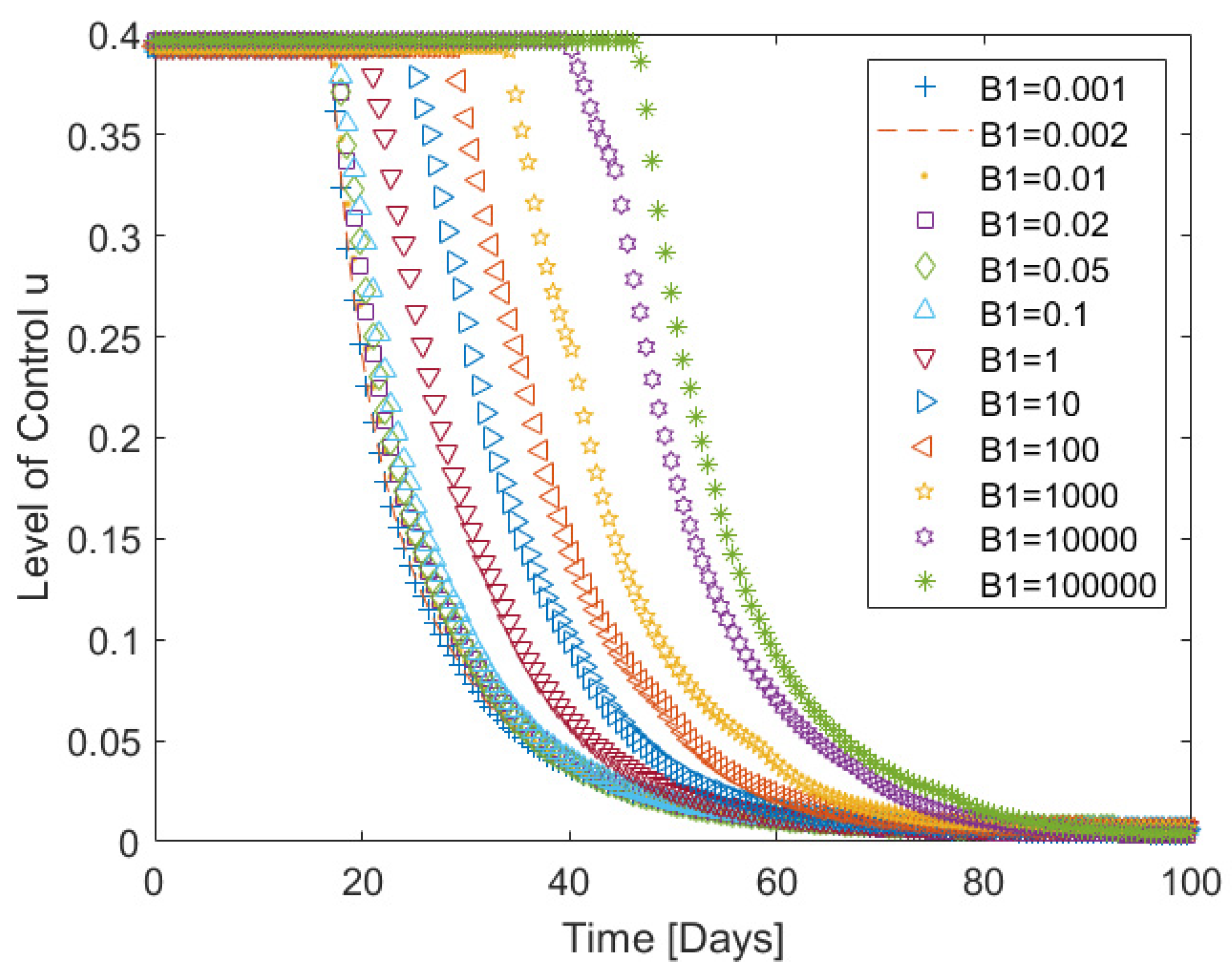
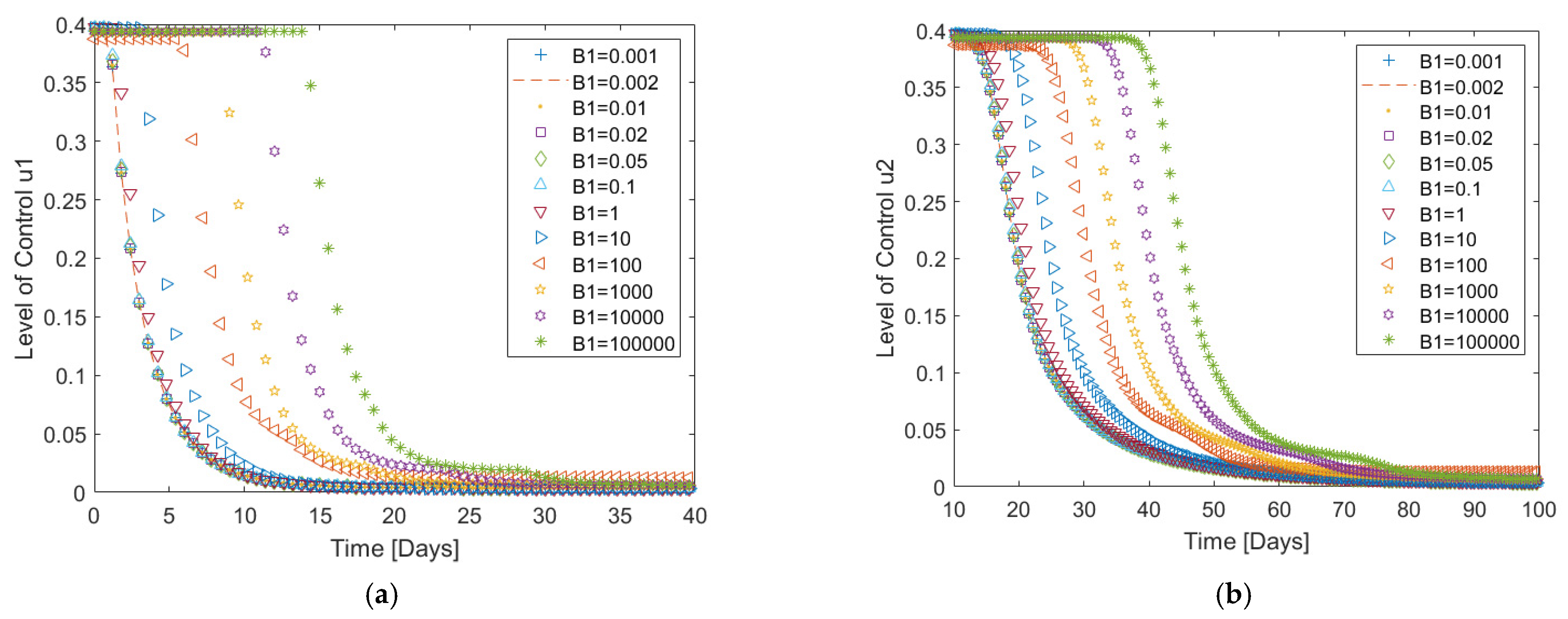
5.3. Changes in the Control Weights
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
Appendix A.1. Proof of Proposition 1
Appendix A.2. Proof of Proposition 2
Appendix A.3. Proof of Theorem 1
Appendix A.4. Proof of Theorem 2
References
- World Health Organization. A Guide to Clinical Management and Public Health Response for Hand, Foot and Mouth Disease (HFMD). 2011. Available online: https://iris.wpro.who.int/handle/10665.1/5521 (accessed on 16 January 2020).
- Wang, M.G.; Sun, H.M.; Liu, X.M.; Deng, X.Q. Clinical analysis of 59 children with hand foot and mouth diseases due to enterovirus EV71 and concomitant viral encephalitis. Euro. Review. Med. Pharm. Sci. 2017, 21, 43–49. [Google Scholar]
- Chin, S. HFMD: A Disease of Epidemic Proportions? The Asean Post. Available online: https://theaseanpost.com/article/hfmd-disease-epidemic-proportions (accessed on 25 December 2019).
- D. Bureau of Epidemiology and MoPH: Report on the Situation of Hand, Foot and Mouth Disease (HFMD) in Thailand. Available online: http://www.boe.moph.go.th/boedb/surdata/disease.php?dcontent=old&ds=71 (accessed on 16 January 2020).
- Puenpa, J.; Auphimai, C.; Korkong, S.; Vongpunsawad, S.; Poovorawan, Y. Enterovirus A71 Infection, Thailand, 2017. Emerg. Infect. Dis. 2018, 24, 1386–1387. [Google Scholar] [CrossRef] [Green Version]
- D. Bureau of Epidemiology and MoPH: Hand, Foot, and Mouth Disease (HFMD) in Thailand, Situation Update, No. 50. 2017. Available online: https://ddc.moph.go.th/uploads/files/333f54af3da87cf66599a0def2c30856.pdf (accessed on 20 January 2020).
- D. Bureau of Epidemiology and MoPH: Hand, Foot, and Mouth Disease (HFMD) in Thailand, Situation Update, No. 10. 2019. Available online: http://dcd.ddc.moph.go.th/2016/informations/view/1262 (accessed on 26 May 2020).
- Ruan, F.; Yang, T.; Ma, H.; Jin, Y.; Song, S.; Fontaine, R.E.; Zhu, B.P. Risk factors for hand, foot, and mouth disease and herpangina and the preventive effect of hand-washing. Pediatrics 2011, 127, e898–e904. [Google Scholar] [CrossRef] [Green Version]
- Park, S.K.; Park, B.; Ki, M.; Kim, H.; Lee, K.; Jung, C.; Sohn, Y.M.; Choi, S.-M.; Kim, D.-K.; Lee, D.S.; et al. Transmission of seasonal outbreak of childhood enteroviral aseptic meningitis and hand-foot-mouth disease. J. Korean Med. Sci. 2010, 25, 677–683. [Google Scholar] [CrossRef]
- Chang, L.Y.; King, C.C.; Hsu, K.H.; Ning, H.C.; Tsao, K.C.; Li, C.C.; Huang, Y.C.; Shih, S.R.; Chiou, S.T.; Chen, P.Y.; et al. Risk factors of enterovirus 71 infection and associated hand, foot, and mouth disease/herpangina in children during an epidemic in Taiwan. Pediatrics 2002, 109, e88. [Google Scholar] [CrossRef] [Green Version]
- Li, Y.; Dang, S.; Deng, H.; Wang, W.; Jia, X.; Gao, N.; Li, M.; Wang, J. Breastfeeding, previous Epstein-Barr virus infection, enterovirus 71 infection, and rural residence are associated with the severity of hand, foot, and mouth disease. Euro. J. Pediatr. 2013, 172, 661–666. [Google Scholar] [CrossRef]
- Ikai, T.; Yamtree, S.; Takemoto, T.; Tamura, T.; Kanayama, H.; Sato, K.; Kusaka, Y.; Hayashi, H.; Terasawa, H. Medical care ideals among urban and rural residents in Thailand: A qualitative study. Int. J. Equity Health 2016, 15, 2. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- D. Bureau of Epidemiology and MoPH: Report on the Situation of the Important Communicable Diseases in Region 9. Available online: http://odpc9.ddc.moph.go.th/hot/situlation.htm (accessed on 30 September 2021).
- Samanta, R. Analysis of a delayed hand foot mouth disease epidemic model with pulse Vaccination. Sys. Sci Contr. Engr. 2014, 2, 61–63. [Google Scholar] [CrossRef]
- Li, Y.; Zhang, J.; Zhang, X. Modeling and Preventive Measures of Hand, Foot and Mouth Disease (HFMD) in China. Int. J. Environ. Res. Pub. Health 2014, 11, 3108–3117. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Zhu, Y.; Xu, B.; Lian, X.; Lin, W.; Zhou, Z.; Wang, W. A Hand-Foot-and-Mouth Disease Model with Periodic Transmission Rate in Wenzhou, China. Abstr. Appl. Anal. 2014, 2014, 1–11. [Google Scholar] [CrossRef]
- Wu, C. Analysis of a Hand-Foot-Mouth Disease Model with Standard Incidence Rate and Estimation for Basic Reproduction Number, Math. Comput. Appl. 2017, 22, 29. [Google Scholar]
- Chadsuthi, S.; Wichapeng, S. The Modelling of Hand, Foot, and Mouth Disease in Contaminated Environments in Bangkok, Thailand. Comput. Math. Methods Med. 2018, 2018, 5168931. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Tan, H.; Cao, H. The Dynamics and Optimal Control of a Hand-Foot-Mouth Disease Model. Comput. Math. Methods Med. 2018, 2018, 9254794. [Google Scholar] [CrossRef] [PubMed]
- Wang, P.; Zhao, H.; You, F.; Zhou, H.; Goggins, W.B. Seasonal modeling of hand, foot and mouth disease as a function of meteorological variations in Chongqink, China. Int. J. Biometeorol. 2017, 61, 1411–1419. [Google Scholar] [CrossRef]
- Ghanbari, B. On approximate solutions for a fractional prey-predator model involving the Atangana-Baleanu derivative. Adv. Differ. Equ. 2020, 679. [Google Scholar] [CrossRef]
- Ghanbari, B. On the modeling of the interaction between tumor growth and the immune system using some new fractional and fractional-fractal operators. Adv. Differ. Equ. 2020, 585. [Google Scholar] [CrossRef]
- Ghanbari, B. A fractional system of delay differential equation with nonsingular kernels in modeling hand-foot-mouth disease. Adv. Differ. Equ. 2020, 536. [Google Scholar] [CrossRef]
- Rahman, G.; Nisar, K.S.; Ghanbari, B.; Abdeljawad, T. On generalized fractional integral inequalities for the monotone weighted Chebyshev functionals. Adv. Differ. Equ. 2020, 368. [Google Scholar] [CrossRef]
- Ghanbari, B.; Kumar, S. A study on fractional predator-prey-pathogen model with Mittag-Leffler kernel-based operators. Numer. Methods Partial Differ. Equ. 2020. [Google Scholar] [CrossRef]
- Ghanbari, B. Mathematical and numerical analysis of a three-species predator-prey model with herd behavior and time fractionalorder derivative. Math. Method Appl. Sci. 2020, 43, 1736–1752. [Google Scholar] [CrossRef]
- Munusamy, K.; Ravichandran, C.; Nisar, K.S. Existence of solutions for some functional integrodifferential equations with nonlocal conditions. Math. Method Appl. Sci. 2020, 43, 10319–10331. [Google Scholar] [CrossRef]
- Ghanbari, B. On forecasting the spread of the COVID-19 in Iran: The second wave. Chaos Solitons Fractals 2020, 140, 110176. [Google Scholar] [CrossRef]
- Djilali, S.; Ghanbari, B. Coronavirus pandemic: A predictive analysis of the peak outbreak epidemic in South Africa, Turkey, and Brazil. Chaos Solitons Fractals 2020, 138, 109971. [Google Scholar] [CrossRef]
- Bichara, D.; Kang, Y.; Castillo-Chavez, C.; Horan, R.; Perrings, C. SIS and SIR epidemic models under virtual dispersal. Bull. Math. Biol. 2015, 77, 2004–2034. [Google Scholar] [CrossRef]
- Castillo-Chavez, C.; Bichara, D.; Morin, B.R. Perspectives on the role of mobility, behavior, and time scales in the spread of diseases. Proc. Natl. Acad. Sci. USA 2016, 113, 14582–14588. [Google Scholar] [CrossRef] [Green Version]
- Moreno, V.; Espinoza, B.; Barley, K.; Paredes, M.; Bichara, D.; Mubayi, A.; Castillo-Chavez, C. The role of mobility and health disparities on the transmission dynamics of Tuberculosis. Theor. Biol. Med. Model. 2017, 14, 3. [Google Scholar] [CrossRef]
- Lolika, P.O.; Mushayabasa, S. On the Role of Short-Term Animal Movements on the Persistence of Brucellosis. Mathematics 2018, 6, 154. [Google Scholar] [CrossRef] [Green Version]
- Rodrigues, H.S.; Monteiro, M.T.; Torres, D.F.M. Dynamics of Dengue epidemics when using optimal control. Math. Comput. Model. 2010, 52, 1667–1673. [Google Scholar] [CrossRef] [Green Version]
- Pongsumpun, P.; Tang, I.M.; Wongvanich, N. Optimal control of the dengue dynamical transmission with vertical transmission. Adv. Differ. Equ. 2019, 2019, 176. [Google Scholar] [CrossRef] [Green Version]
- Imran, M.; Usman, M.; Malik, T.; Ansari, A.R. Mathematical analysis of the role of hospitalization/isolation in controlling the spread of Zika fever. Virus Res. 2018, 255, 95–104. [Google Scholar] [CrossRef]
- Momoh, A.A.; Fuegenschuh, A. Optimal control of intervention strategies and cost effectiveness analysis for a Zika virus model. Oper. Res. Health Care 2018, 18, 99–111. [Google Scholar] [CrossRef]
- Gonzalez-Parra, G.; Díaz-Rodríguez, M.; Arenas, A.J. Mathematical modeling to design public health policies for Chikungunya epidemic using optimal control. Optim. Control. Appl. Methods 2020, 41, 1584–1603. [Google Scholar] [CrossRef]
- González-Parra, G.; Díaz-Rodríguez, M.; Arenas, A.J. Optimization of the Controls against the Spread of Zika Virus in Populations. Computation 2020, 8, 76. [Google Scholar] [CrossRef]
- Okyere, E.; De-Graft Ankamah, J.; Hunkpe, A.K.; Mensah, D. Deterministic epidemic models for ebola infection with time-dependent controls. Discrete. Dyn. Nat. Soc. 2020, 2020, 2823816. [Google Scholar] [CrossRef]
- Wongvanich, N.; Boksuwan, S.; Chesof, A. Simplified modelling and backstepping control of the long arm agricultural rover. Adv. Differ. Equ. 2020, 2020, 701. [Google Scholar] [CrossRef]
- The Thailand Primary Health Care Division. Available online: http://www.thaiphc.net (accessed on 30 May 2021). (In Thai).
- Chanprasopchai, P.; Ming-tang, I.; Pongsumpun, P. SIR Model for Dengue Disease with Effect of Dengue Vaccination. Comput. Math. Methods Med. 2018, 2018, 9861572. [Google Scholar] [CrossRef]
- Chanprasopchai, P.; Pongsumpun, P.; Tang, I.M. Effect of Rainfall for the Dynamical Transmission Model of the Dengue Disease in Thailand. Comput. Math. Methods Med. 2017, 2017, 2541862. [Google Scholar] [CrossRef]
- Herdicho, F.F.; Chukwu, W.; Tasman, H. An optimal control of malaria transmission model with mosquito seasonal factor. Results Phys. 2021, 25, 104238. [Google Scholar]
- The World Bank. Life Expectancy at Birth, Total (Years)—Thailand. Available online: https://data.worldbank.org/indicator/SP.DYN.LE00.IN?locations=TH&view=chart (accessed on 26 May 2020).
- Yang, Z.; Zhang, Q.; Cowling, B.J.; Lau, E.H. Estimating the incubation period of hand, foot and mouth disease for children in different age groups. Sci. Rep. 2017, 7, 16464. [Google Scholar] [CrossRef]
- Guan, X.; Che, Y.; Wei, S.; Li, S.; Zhao, Z.; Tong, Y.; Wang, L.; Gong, W.; Zhang, Y.; Zhao, Y.; et al. Effectiveness and safety of an inactivated Enterovirus 71 vaccines in children aged 6–71 months in a phase IV study. Clin. Infect. Dis. 2019, 71, 2421–2427. [Google Scholar] [CrossRef]
- Yang, Z.; Gao, F.; Wang, X.; Shi, L.; Zhou, Z.; Jiang, Y.; Ma, X.; Zhang, C.; Zhou, C.; Zeng, X.; et al. Development and characterization of an enterovirus 71 (EV-71) virus-like particles (VLPs) vaccines produced in Pichia pastoris. Hum. Vaccin. Immunother. 2019, 16, 1602–1610. [Google Scholar] [CrossRef]
- Jiang, L.; Fan, R.; Sun, S.; Fan, P.; Su, W.; Zhou, Y.; Gao, F.; Xu, F.; Kong, W.; Jiang, C.; et al. A new EV71 VP3 epitope in novovirus P particle vector displays neutralizing activity and protection in vivo in mice. Vaccine 2015, 33, 6596–6603. [Google Scholar] [CrossRef]
- Fleming, W.H.; Richel, R.W. Deterministic and Stochastic Optimal Control; Springer: New York, NY, USA, 1975; Volume 1. [Google Scholar]
- Lukes, D.L. Differential Equations Electronics Resource: Classical to Controlled; Elsevier: Amsterdam, The Netherlands, 1982. [Google Scholar]
- Lenhart, S.; Workman, J.T. Optimal Control Applied to Biological Models; CRC Press: Boca Roton, FL, USA, 2007. [Google Scholar]
- Dufour, J.M. Coefficient of Determination. Available online: https://jeanmariedufour.github.io/ResE/Dufour_1983_R2_W.pdf (accessed on 30 May 2021).






| 9 | Nakhon Ratchasima | Chaiyaphum |
|---|---|---|
| 2018 | 110.70 | 100.42 |
| 2019 | 99.69 | 70.04 |
| 2020 | 5.99 | 5.97 |
| 2021 | 35.16 | 23.81 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wongvanich, N.; Tang, I.-M.; Dubois, M.-A.; Pongsumpun, P. Mathematical Modeling and Optimal Control of the Hand Foot Mouth Disease Affected by Regional Residency in Thailand. Mathematics 2021, 9, 2863. https://doi.org/10.3390/math9222863
Wongvanich N, Tang I-M, Dubois M-A, Pongsumpun P. Mathematical Modeling and Optimal Control of the Hand Foot Mouth Disease Affected by Regional Residency in Thailand. Mathematics. 2021; 9(22):2863. https://doi.org/10.3390/math9222863
Chicago/Turabian StyleWongvanich, Napasool, I-Ming Tang, Marc-Antoine Dubois, and Puntani Pongsumpun. 2021. "Mathematical Modeling and Optimal Control of the Hand Foot Mouth Disease Affected by Regional Residency in Thailand" Mathematics 9, no. 22: 2863. https://doi.org/10.3390/math9222863
APA StyleWongvanich, N., Tang, I.-M., Dubois, M.-A., & Pongsumpun, P. (2021). Mathematical Modeling and Optimal Control of the Hand Foot Mouth Disease Affected by Regional Residency in Thailand. Mathematics, 9(22), 2863. https://doi.org/10.3390/math9222863






