Preventive Maintenance of the k-out-of-n System with Respect to Cost-Type Criterion
Abstract
:1. Introduction and Motivation
- A method for PM investigations of k-out-of-n: F systems, whose failures depend on the positions of failed units developed;
- A new approach based on ordered statistics is used to solve the problem;
- A cost-type criterion for PM strategies comparison is used;
- A study of the sensitivity of decision making to the type of system unit lifetime distribution is carried out.
2. The Problem Set Up, Assumptions, and Notations
2.1. Notations and Assumptions
- At the very beginning the system is absolutely reliable, i.e., it is in zero state ;
- All sequences of r.v.s (unit lifetimes, repair, and PM times) are i.i.d. for each type of r.v. Further, the letters without indexes are used for the representatives of appropriate sequence of r.v.s;
- The mean values of the unit lifetimes, as well as these for repair and PM times are finite,It is supposed that the latter is less than the mean repair time, i.e., that , but may or may not depend on the type of PM;
- It is assumed that the mean unit lifetime, as well as the mean repair and PM times are known to the decision maker (DM);
- After any repair and PM completion, the system starts working “as a new one”, i.e., returns into the zero state. In other words, the model of the perfect PM is considered.
2.2. The Problem Set Up
- System reliability function
- Distributions of the times up to various PM starts and their mean values
- System quality measure for different PM strategies defined for as
3. The Problem Solution and the General Procedure for Comparing the Quality of PM Strategies
3.1. The Problem Solution
3.2. The General Procedure for Comparing the Quality of PM Strategies
| Algorithm 1 The general algorithm for choosing the best strategy. |
Beginning. Determine: - Integers , set of PM strategies ; - Set of system states E; - Subsets of states for the PM beginnings or of the system failure for ; - Distribution of the system unit lifetime, which is defined in Section 2.1; - Mean PM and system repair times ; - Rewards c, cost of repair , cost of l-th PM ; Calculate value according to (14). Step 1. Describe the connection between subsets of the PM and repair beginning states and ordered statistics. Step 2. Represent the time of the subsets destinations in terms of the ordered statistics
Step 3. Calculate distributions of the respective ordered statistics
Step 4. Calculate the distributions of the subsets destination times and their expectations, in terms of distributions of respective ordered statistics,
Step 6. Compare the calculated values with the indicator as advice to a DM in order to choose the best strategy according to inequality (15). Stop. |
4. Numerical Experiments
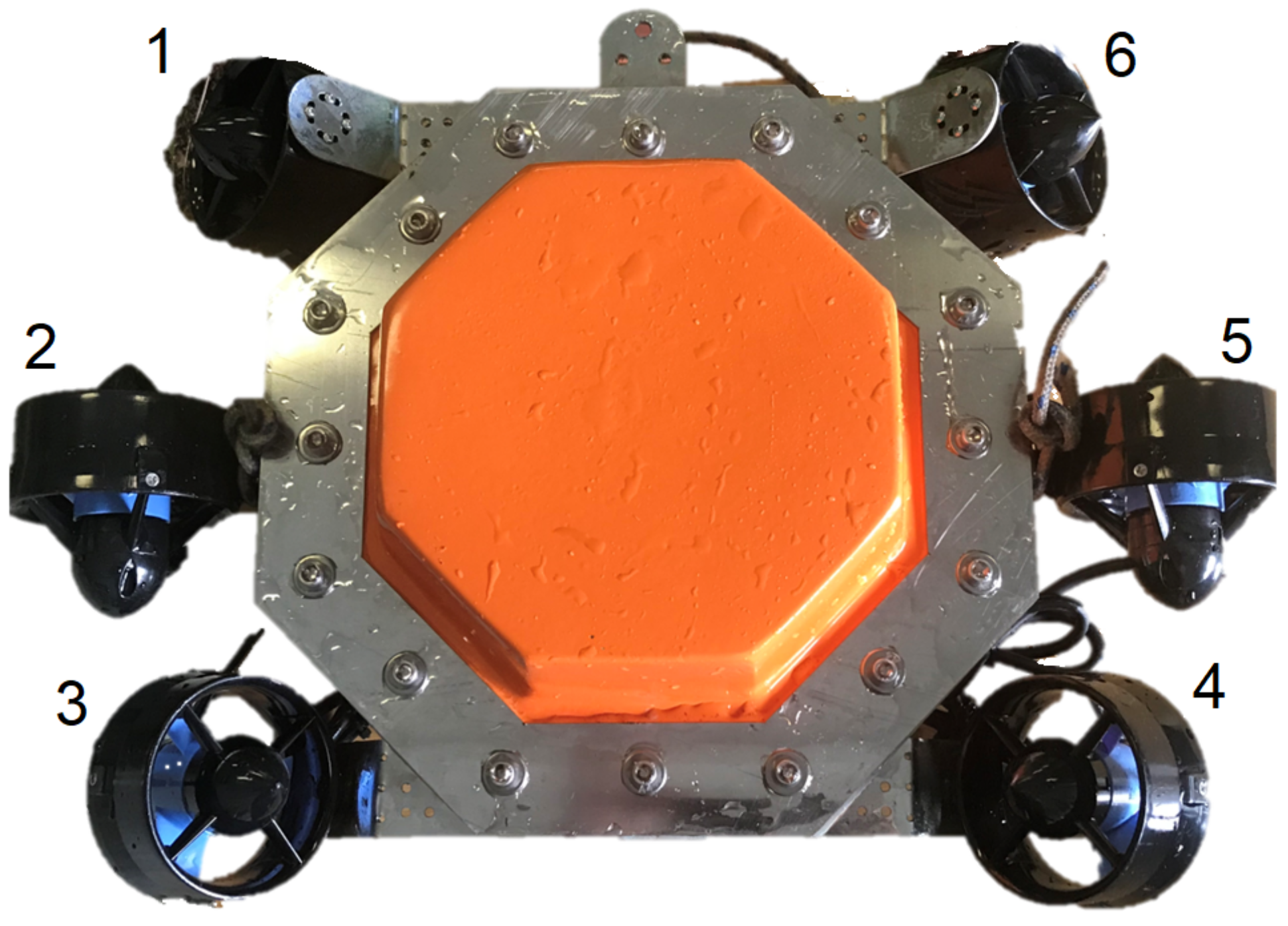
4.1. Preliminary: Description of the Example
- The system failure occurs when any four motors fail regardless of their location. This situation is modeled as a 4-out-of-6: F-system and is presented in Section 4.2;
- The system failure depends on the location of its failed units, and occurs when three motors from one side and one motor from the other side fail (this situation will be denoted as -out-of-6: F system), but the system does not fail when two motors fail on one side, and two motors on the other. Any next unit failure leads to the system failure (this situation will be denoted as a 5-out-of-6: F system).
4.2. PM of a System, Whose Failure Does Not Depend on the Location of Its Failed Units
- 0-strategy is that the system operates up to its failure;
- l-strategy ( 1, 2, 3) is to begin the PM when the system reaches the state l.
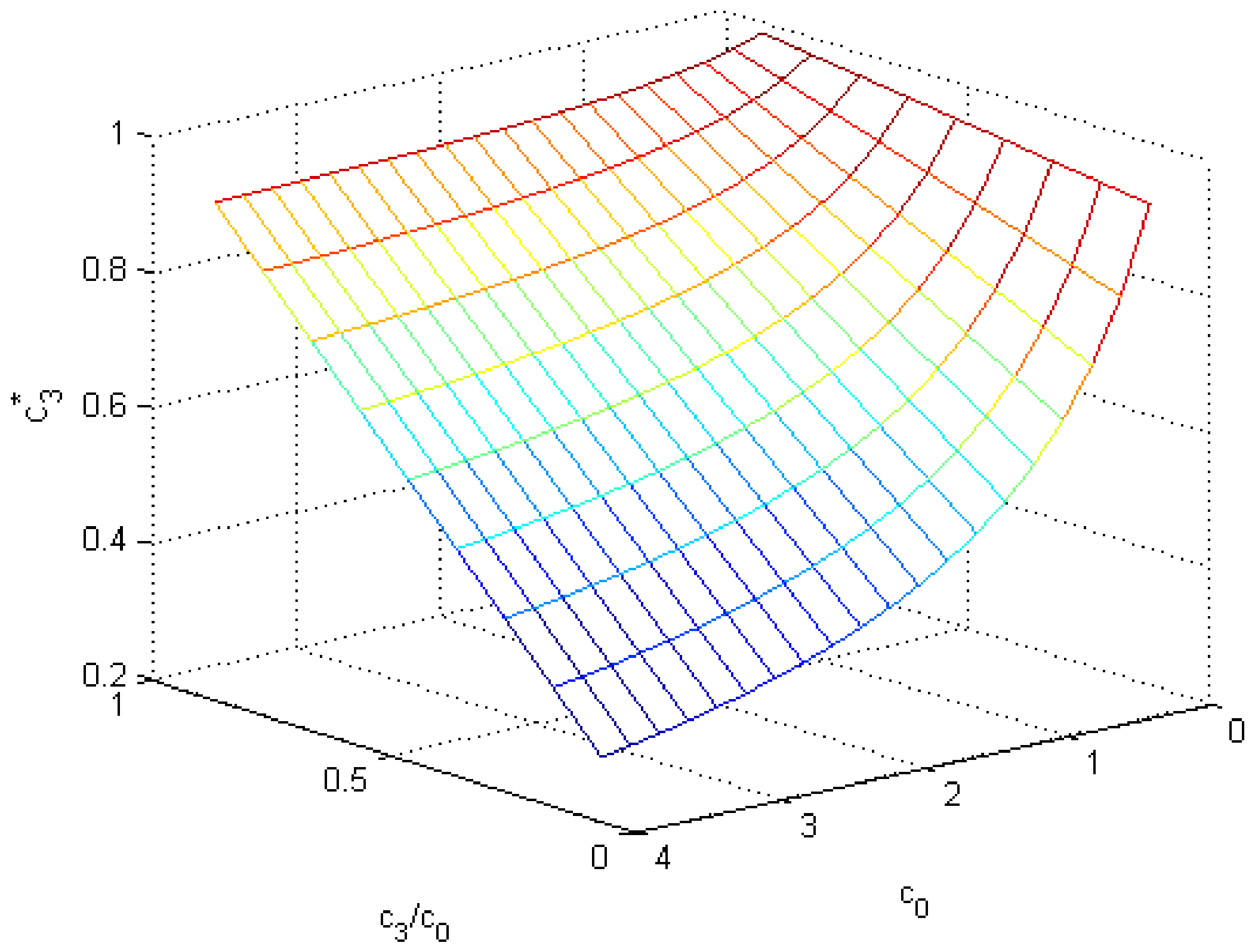
4.3. Special Case: PM of a K-Out-Of-n: F–System, for Exponential Distributions of Unit Lifetimes
- for ,
- for .
4.4. PM of a System, Whose Failure Depends on the Location of the Failed Units
- 0-strategy: run up to the system failure. The subset of the states for the repair beginning is ;
- 3-strategy: start the PM after the failure of any three units. The subset of the states for the PM beginning is .
- Step 1. To form a set of repair start states, consider the set of states with four failed units,In this set, the red states are associated with three failed units on one side and one ion other side, denoted as , when the system failure occurs. The blue states are associated with two units failed one one side and two on the other, denoted as , which leads to the system failure after any next unit failure.The three-strategy starts after the failure of any three units, the subset for it is .
- Step 2. Accordingly to step 1, the times for the system failure coincide with ordered statistics for red states of subset , and coincide with ordered statistics for blue states of subset .The time to the set destination coincide with the relevant ordered statistics namely:
- Step 3. Does not change. The distributions of the j-th ordered statistics are calculated according to (20),
- Step 4. The distribution of the time to the subset destination according to its determination by (29) equals toThe distribution of the subset of states destination is Appropriate expectations of times to the subsets for and destinations are
- Step 5. Does not change: following to step 5 of the Algorithm 1, calculate .
- Step 6. Compare the calculated values with indicator , according to the rule given by (15) as advice to a DM in order to choose the best strategy.
- Stop.
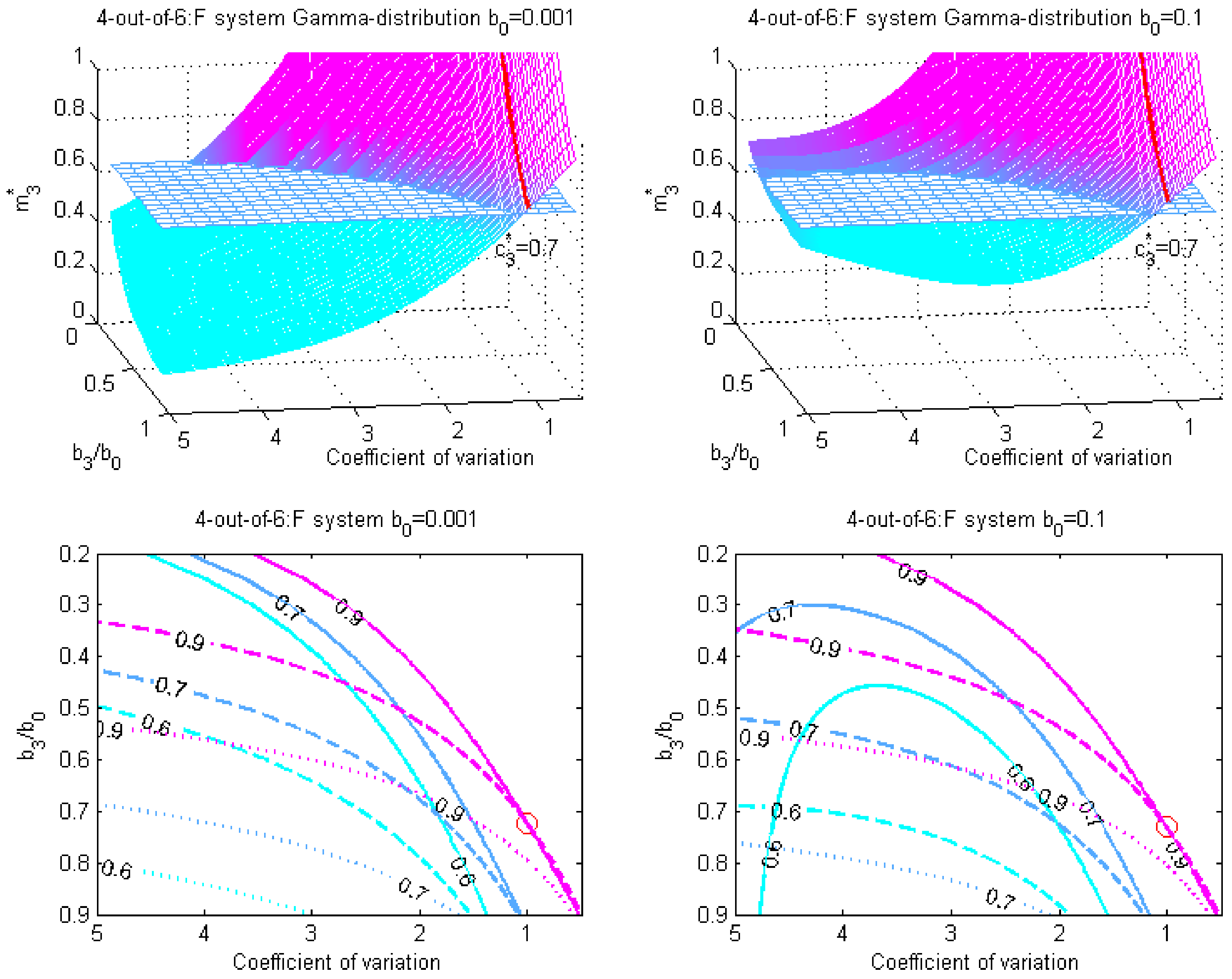
4.5. Special Case: PM of a -Out-Of-6 – System for Exponential Distribution of Unit Lifetimes
- and in the case as
5. Conclusion
- A method for PM investigations of k-out-of-n: F systems, whose failures depend on the positions of failed units developed;
- A new approach based on ordered statistics if used to solve the problem;
- A cost-type criterion for PM strategies comparison is used;
- A study of the sensitivity of decision making to the shape of system unit lifetime distributions is carried out.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| PM | preventive maintenance |
| i.i.d. | independent and identically distributed |
| r.v. | random variable |
| MDP | Markov decision processes |
| DM | decision maker |
| UUV | unmanned underwater vehicle |
References
- Trivedi, K. Probability and Statistics with Reliability, Queuing and Computer Science Applications, 2nd ed.; John Wiley & Sons: New York, NY, USA, 2016. [Google Scholar]
- Chakravarthy, S.; Krishnamoorthy, A.; Ushakumari, P. A k-out-of-n reliability system with an unreliable server and Phase type repairs and services: The (N,T) policy. J. Appl. Math. Stoch. Anal. 2001, 14, 361–380. [Google Scholar] [CrossRef] [Green Version]
- Rykov, V.; Kozyrev, D.; Filimonov, A.; Ivanova, N. On reliability function of a k-out-of-n system with general repair time distribution. Probab. Eng. Inform. Sci. 2020, 35, 885–902. [Google Scholar] [CrossRef]
- Pascual-Ortigosa, P.; Sáenz-de-Cabezón, E. Algebraic Analysis of Variants of Multi-State k-out-of-n Systems. Mathematics 2021, 9, 2042. [Google Scholar] [CrossRef]
- Rykov, V.; Sukharev, M.; Itkin, V. Investigations of the Potential Application of k-out-of-n Systems in Oil and Gas Industry Objects. J. Mar. Sci. Eng. 2020, 8, 928. [Google Scholar] [CrossRef]
- Rykov, V.; Kochueva, O.; Farkhadov, M. Preventive Maintenance of a k-out-of-n System with Applications in Subsea Pipeline Monitoring. J. Mar. Sci. Eng. 2021, 9, 85. [Google Scholar] [CrossRef]
- Vishnevsky, V.; Kozyrev, D.; Rykov, V.; Nguen, Z. Reliability modeling of the rotary-wing flight module of a high-altitude telecommunications platform. Inform. Technol. Comput. Syst. 2020, 4, 26–38. (In Russian) [Google Scholar]
- Shiryaev, A.N. Some new results in theory of controllable random processes. In Trans. Fourth Prague Conf. on Information Theory, Statistical Decision Functions, Random Processes, Prague, Czech Republic, 1965; Academia: Prague, Czech Republic, 1965; pp. 131–203. [Google Scholar]
- Boucherie, R.; vanDijk, N. Markov Decision Processes in Practice; Springer International Publishing: Cham, Switzerland, 2017. [Google Scholar]
- Howard, R. Dynamic Programming and Markov Processes; John Wiley & Sons: New York, NY, USA, 1960. [Google Scholar]
- Wolfe, P.; Dantzig, G. Linear Programming in a Markov Chain. Oper. Res. 1962, 10, 702–710. [Google Scholar] [CrossRef]
- Puterman, M. Markov Decision Processes. In Handbooks in Operations Research and Management Science; Heyman, D., Sobel, M., Eds.; Elsevier: Amsterdam, The Netherlands, 1990; Volume 2, pp. 331–434. [Google Scholar]
- Rykov, V. Controllable Queueing Systems. Itogi Nauk. Tech. Probab. Theory. Math. Stat. Theor. Cybern. 1975, 12, 143–153. (In Russian) [Google Scholar]
- Rykov, V. Controllable Queueing Systems: From the Very Beginning up to Nowadays. Reliab. Theory Appl. 2017, 12, 39–61. [Google Scholar]
- Kitaev, V.; Rykov, V. Controlled queueing systems. Int. J. Stoch. Anal. 1995, 8, 433–435. [Google Scholar]
- Hernandez-Lerma, O.; Lasssrerre, J. Discrete time Markov Control Processes Basic Optimality Criteria; Springer: New York, NY, USA, 1996. [Google Scholar]
- Sennott, L. Stochastic Dynamic Programming and the Control of Queueing Systems; Wiley-Interscience: Hoboken, NJ, USA, 1999. [Google Scholar]
- Gertsbakh, I. Reliability Theory with Applications to Preventive Maintenance; Springer: Berlin/Heidelberg, Germany, 2000. [Google Scholar]
- Dudin, A.; Krishnamoorthy, A.; Viswanath, C. Reliability of a k-out-of-n-system through Retrial Queues. In Proceedings of the Trans. of XXIV International Seminar on Stability Problems for Stochastic Models, Jurmala, Latvia, 10–17 September 2004. [Google Scholar]
- Krishnamoorthy, A.; Viswanath, C.N.; Deepak, T.G. Reliability of a k-out-of-n-system with Repair by a Service Station Attending a Queue with Postponed Work. Int. J. Reliab. Qual. Saf. Eng. 2007, 14, 379–398. [Google Scholar] [CrossRef]
- Krishnamoorthy, A.; Viswanath, C.N.; Deepak, T.G. Maximizing of Reliability of a k-out-of-n-system with Repair by a facility attending external customers in a Retrial Queue. Reliab. Theory Appl. 2007, 2, 21–33. [Google Scholar]
- Krishnamoorthy, A.; Sathian, M.K.; Viswanath, C.N. Reliability of a k-out-of-n system with repair by a single server extending service external customers with pre-emption. Reliab. Theory Appl. 2016, 11, 61–93. [Google Scholar]
- Krishnamoorthy, A.; Sathian, M.K.; Viswanath, C.N. Reliability of a k-out-of-n system with a single server extending non-preemptive service to external customers-Part I. Reliab. Theory Appl. 2016, 11, 62–75. [Google Scholar]
- Krishnamoorthy, A.; Sathian, M.K.; Viswanath, C.N. Reliability of a k-out-of-n system with a single server extending non-preemptive service to external customers-Part II. Reliab. Theory Appl. 2016, 11, 76–88. [Google Scholar]
- Pham, H.; Wang, H. Optimal opportunistic maintenance of a k-out-of-n:G system. Int. J. Reliab. Qual. Saf. Eng. 1997, 4, 369–386. [Google Scholar]
- Pham, H.; Wang, H. Optimal (τ, T) Opportunistic Maintenance of a k-out-of-n:G System with Imperfect PM and Partial Failure. Nav. Res. Logist. 2000, 47, 223–239. [Google Scholar] [CrossRef]
- Geng, J.; Azarian, M.; Pecht, M. Opportunistic maintenance for multi-component systems considering structural dependence and economic dependence. J. Syst. Eng. Electron. 2015, 26, 493–501. [Google Scholar] [CrossRef]
- Finkelstein, M.; Levitin, G. Preventive maintenance for homogeneous and heterogeneous systems. Appl. Stoch. Model. Bus. Ind. 2019, 35, 908–920. [Google Scholar] [CrossRef]
- Finkelstein, M.; Cha, J.H.; Levitin, G. On a new age-replacement policy for items with observed stochastic degradation. Qual. Reliab. Eng. Int. 2020, 36, 1132–1146. [Google Scholar] [CrossRef]
- Park, M.; Lee, J.; Kim, S. An optimal maintenance policy for k-out-of-n system without monitoring component. Qual. Technol. Qual. Manag. 2019, 16, 140–153. [Google Scholar] [CrossRef]
- Endharth, A.J.; Yun, W.Y.; Yamomoto, H. Preventive maintenance policy for linear-consecutive -k-out-of-n:F system. J. Oper. Res. Soc. Jpn. 2016, 59, 334–346. [Google Scholar]
- Hamdan, K.; Tavangar, M.; Asadi, M. Optimal preventive maintenance for repairable weighted k-out-of-n systems. Reliab. Eng. Syst. Safe 2021, 205, 107267. [Google Scholar] [CrossRef]
- Jonge, B.D.; Scarf, P.A. A review on maintenance optimization. Eur. J. Oper. Res. 2020, 285, 805–824. [Google Scholar] [CrossRef]
- Rykov, V. On Reliability of Renewable Systems. In Reliability Engineering. Theory and Applications; Vonta, I., Ram, M., Eds.; CRC Press: Boca Raton, FL, USA, 2018; pp. 173–196. [Google Scholar]
- Rykov, V. Decomposable semi-regenerative processes: Review of theory and applications to queueing and reliability systems. Reliab. Theory Appl. 2021, 16, 157–190. [Google Scholar]
- Klimov, G. An Ergodic Theorem for Regenerating Processes. Theory Probab. Its Appl. 1976, 21, 392–395. [Google Scholar] [CrossRef]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rykov, V.; Kochueva, O.; Rykov, Y. Preventive Maintenance of the k-out-of-n System with Respect to Cost-Type Criterion. Mathematics 2021, 9, 2798. https://doi.org/10.3390/math9212798
Rykov V, Kochueva O, Rykov Y. Preventive Maintenance of the k-out-of-n System with Respect to Cost-Type Criterion. Mathematics. 2021; 9(21):2798. https://doi.org/10.3390/math9212798
Chicago/Turabian StyleRykov, Vladimir, Olga Kochueva, and Yaroslav Rykov. 2021. "Preventive Maintenance of the k-out-of-n System with Respect to Cost-Type Criterion" Mathematics 9, no. 21: 2798. https://doi.org/10.3390/math9212798
APA StyleRykov, V., Kochueva, O., & Rykov, Y. (2021). Preventive Maintenance of the k-out-of-n System with Respect to Cost-Type Criterion. Mathematics, 9(21), 2798. https://doi.org/10.3390/math9212798







