Recovering Density and Speed of Sound Coefficients in the 2D Hyperbolic System of Acoustic Equations of the First Order by a Finite Number of Observations
Abstract
1. Introduction
2. Direct Problem
2.1. Problem Formulation
2.2. Methods for Solving the Direct Problem
- Obtaining the flux values by solving the Riemann problem;
- Updating the state variables on the next time step, using the solution of the Riemann problem.
- Reconstruction of the state variables on the current time step, using piecewise-linear extrapolation;
- Evolution of the reconstructed state variables by conservation laws with a time ;
- Obtaining the flux values for the “midpoint” time step by solving the Riemann problem for evolved state variables;
- Updating the state values from the current time step on the next time step by conservation laws with a time .
3. Inverse Problems
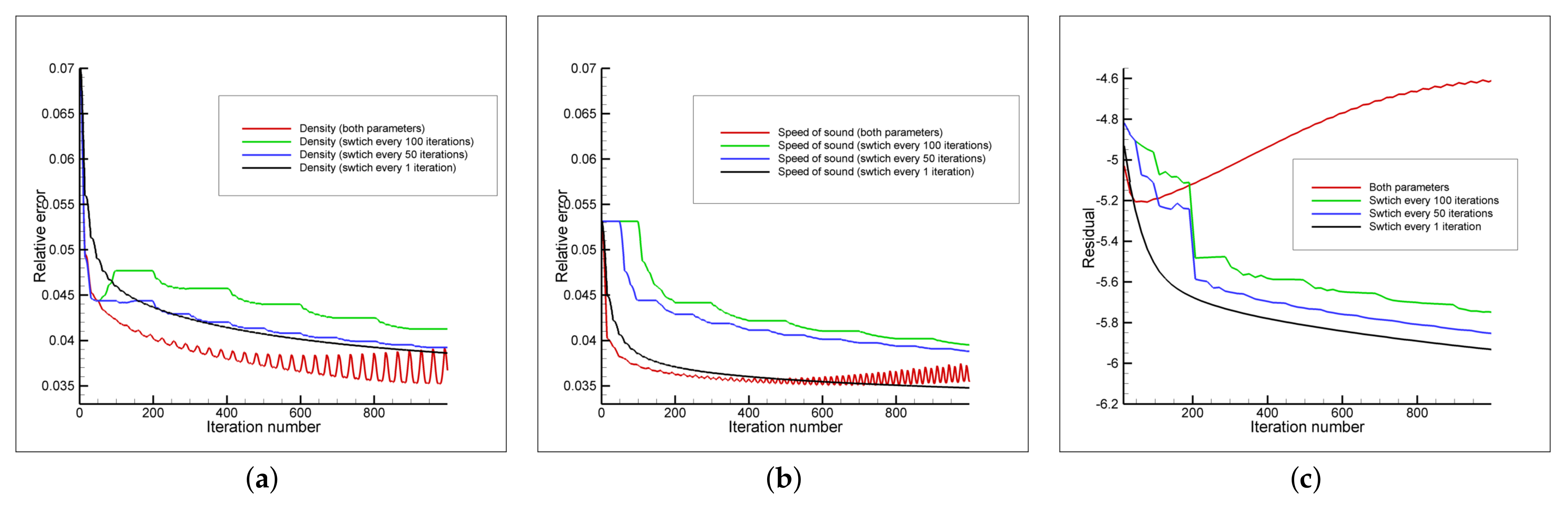
4. Numerical Results
5. Discussion
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Burov, V.A.; Zotov, D.I.; Rumyantseva, O.D. Reconstruction of the sound velocity and absorption spatial distributions in soft biological tissue phantoms from experimental ultrasound tomography data. Acoust. Phys. 2015, 61, 231–248. [Google Scholar] [CrossRef]
- Duric, N.; Littrup, P.; Li, C.; Roy, O.; Schmidt, S.; Janer, R.; Cheng, X.; Goll, J.; Rama, O.; Bey-Knight, L.; et al. Breast ultrasound tomography: Bridging the gap to clinical practice. Proc. SPIE 2012, 8320, 832000. [Google Scholar]
- Jirik, R.; Peterlik, I.; Ruiter, N.; Fousek, J.; Dapp, R.; Zapf, M.; Jan, J. Sound-speed image reconstruction in sparse-aperture 3D ultrasound transmission tomography. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2012, 59, 254–264. [Google Scholar] [CrossRef] [PubMed]
- Wiskin, J.; Malik, B.; Natesan, R.; Lenox, M. Quantitative assessment of breast density using transmission ultrasound tomography. Med. Phys. 2019, 46, 2610–2620. [Google Scholar] [CrossRef]
- Filatova, V.; Danilin, A.; Nosikova, V.; Pestov, L. Supercomputer Simulations of the Medical Ultrasound Tomography Problem. Commun. Comput. Inf. Sci. 2019, 1063, 297–308. [Google Scholar] [CrossRef]
- Beilina, L.; Klibanov, M.V. Synthesis of global convergence and adaptivity for a hyperbolic coefficient inverse problem in 3D. J. Inverse Ill-Posed Probl. 2010, 18, 85–132. [Google Scholar] [CrossRef]
- Klibanov, M.V. Travel time tomography with formally determined incomplete data in 3D. Inverse Probl. Imaging 2019, 13, 1367–1393. [Google Scholar] [CrossRef]
- Klibanov, M.V. On the travel time tomography problem in 3D. J. Inverse Ill-Posed Probl. 2019, 27, 591–607. [Google Scholar] [CrossRef]
- Kabanikhin, S.I.; Kulikov, I.M.; Shishlenin, M.A. An Algorithm for Recovering the Characteristics of the Initial State of Supernova. Comp. Math. Math. Phys. 2020, 60, 1008–1016. [Google Scholar] [CrossRef]
- Kulikov, I.M.; Novikov, N.S.; Shishlenin, M.A. Mathematical modeling of propagation of ultrasonic waves in the medium: Direct and inverse problem. Siberian Electronic Mathematical Reports. 2015, 12, C219–C228. [Google Scholar]
- Kabanikhin, S.I.; Klyuchinskiy, D.V.; Novikov, N.S.; Shishlenin, M.A. Numerics of acoustical 2D tomography based on the conservation laws. J. Inverse Ill-Posed Probl. 2020, 28, 287–297. [Google Scholar] [CrossRef]
- Kabanikhin, S.I.; Klychinskiy, D.V.; Kulikov, I.M.; Novikov, N.S.; Shishlenin, M.A. Direct and Inverse Problems for Conservation Laws. In Continuum Mechanics, Applied Mathematics and Scientific Computing: Godunov’s Legacy; Demidenko, G., Romenski, E., Toro, E., Dumbser, M., Eds.; Springer: Cham, Switzerland, 2020. [Google Scholar]
- Romanov, V.G.; Kabanikhin, S.I. Inverse Problems for Maxwell’s Equations; VSP: Utrecht, The Netherlands, 1994. [Google Scholar]
- Klyuchinskiy, D.; Novikov, N.; Shishlenin, M. A Modification of gradient descent method for solving coefficient inverse problem for acoustics equations. Computation 2020, 8, 73. [Google Scholar] [CrossRef]
- Bastin, G.; Coron, J.-M. Stability and Boundary Stabilization of 1-D Hyperbolic Systems. In Progress in Nonlinear Differential Equations and Their Applications; Birkhauser: Boston, MA, USA, 2016. [Google Scholar]
- Blokhin, A.M.; Trakhinin, Y.L. Well-Posedness of Linear Hyperbolic Problems: Theory and Applications; Nova Publishers: Hauppauge, NY, USA, 2006. [Google Scholar]
- Bellassoued, M.; Jellal, D.; Yamamoto, M. Lipschitz stability in in an inverse problem for a hyperbolic equation with a finite set of boundary data. Appl. Anal. 2008, 87, 1105–1119. [Google Scholar] [CrossRef]
- Imanuvilov, O.Y.; Isakov, V.; Yamamoto, M. An inverse problem for the dynamical Lame system with two sets of boundary data. Comm. Pure Appl. Math. 2003, 56, 1366–1382. [Google Scholar] [CrossRef]
- Li, S. An inverse problem for Maxwell’s equations in bi-isotropic media. SIAM J. Math. Anal. 2005, 37, 1027–1043. [Google Scholar] [CrossRef]
- Li, S.; Yamamoto, M. An inverse source problem for Maxwell’s equations in anisotropic media. Appl. Anal. 2005, 84, 1051–1067. [Google Scholar] [CrossRef]
- Li, S.; Yamamoto, M. An inverse problem for Maxwell’s equations in anisotropic media in two dimensions. Chin. Ann. Math. Ser. B 2007, 28, 35–54. [Google Scholar] [CrossRef]
- Bellassoued, M.; Cristofol, M.; Soccorsi, E. Inverse boundary value problem for the dynamical heterogeneous Maxwell’s system. Inverse Probl. 2012, 28, 095009. [Google Scholar] [CrossRef]
- Beilina, L.; Cristofol, M.; Niinimaki, K. Optimization approach for the simultaneous reconstruction of the dielectric permittivity and magnetic permeability functions from limited observations. Inverse Probl. Imaging 2015, 9, 1–25. [Google Scholar] [CrossRef]
- Beilina, L.; Cristofol, M.; Li, S.; Yamamoto, M. Lipschitz stability for an inverse hyperbolic problem of determining two coefficients by a finite number of observations. Inverse Probl. 2017, 34, 015001. [Google Scholar] [CrossRef]
- Kabanikhin, S.I.; Nurseitov, D.B.; Shishlenin, M.A.; Sholpanbaev, B.B. Inverse problems for the ground penetrating radar. J. Inverse Ill-Posed Probl. 2013, 21, 885–892. [Google Scholar] [CrossRef]
- Godunov, S.K. Differential method for numerical computation of noncontinuous solutions of hydrodynamics equations. Matematicheskiy Sbornik 1959, 47, 271–306. (In Russian) [Google Scholar]
- Godunov, S.K.; Zabrodin, A.V.; Ivanov, M.Y.; Kraikov, A.N.; Prokopov, G.P. Numerical Solution for Multidimensional Problems of Gas Mechanics; Nauka: Moscow, Russia, 1976. [Google Scholar]
- He, S.; Kabanikhin, S.I. An optimization approach to a three-dimensional acoustic inverse problem in the time domain. J. Math. Phys. 1995, 36, 4028–4043. [Google Scholar] [CrossRef]
- Kabanikhin, S.I.; Scherzer, O.; Shishlenin, M.A. Iteration methods for solving a two dimensional inverse problem for a hyperbolic equation. J. Inverse Ill-Posed Probl. 2003, 11, 87–109. [Google Scholar] [CrossRef]
- Beilina, L. Adaptive Finite Element Method for a coefficient inverse problem for the Maxwell’s system. Appl. Anal. 2011, 90, 1461–1479. [Google Scholar] [CrossRef]
- Beilina, L.; Hosseinzadegan, S. An adaptive finite element method in reconstruction of coefficients in Maxwell’s equations from limited observations. Appl. Math. 2016, 61, 253–286. [Google Scholar] [CrossRef]
- Beilina, L.; Klibanov, M.V. A posteriori error estimates for the adaptivity technique for the Tikhonov functional and global convergence for a coefficient inverse problem. Inverse Probl. 2010, 26, 045012. [Google Scholar] [CrossRef][Green Version]
- Xin, J.; Beilina, L.; Klibanov, M. Globally convergent numerical methods for some coefficient inverse problems. Comput. Sci. Eng. 2010, 12, 64–76. [Google Scholar]
- Beilina, L.; Klibanov, M.V. Globally strongly convex cost functional for a coefficient inverse problem. Nonlinear Anal. Real World Appl. 2015, 22, 272–288. [Google Scholar] [CrossRef]
- Klibanov, M.V. Carleman estimates for global uniqueness, stability and numerical methods for coefficient inverse problems. J. Inverse Ill-Posed Probl. 2013, 21, 477–560. [Google Scholar] [CrossRef]
- Kabanikhin, S.I.; Klyuchinskiy, D.V.; Novikov, N.S.; Shishlenin, M.A. On the problem of modeling the acoustic radiation pattern of source for the 2D first-order system of hyperbolic equations. In Journal of Physics: Conference Series; IOP Publishing: Bristol, UK, 2021; Volume 1715, p. 012038. [Google Scholar]
- Van Leer, B. Towards the ultimate conservative difference scheme. V. A second-order sequel to Godunov’s method. J. Comput. Phys. 1979, 32, 101–136. [Google Scholar] [CrossRef]
- Berthon, C. Why the MUSCL-Hancock scheme is L 1 -stable. Numer. Math. 2006, 104, 27–46. [Google Scholar] [CrossRef]
- Toro, E. Riemann Solvers and Numerical Methods for Fluid Dynamics; Springer: Berlin, Germany, 2009. [Google Scholar]
- Hanke, M.; Neubauer, A.; Scherzer, O. A convergence analysis of the Landweber iteration for nonlinear ill-posed problems. Numer. Math. 1995, 72, 21–37. [Google Scholar]
- Kabanikhin, S.I.; Kowar, R.; Scherzer, O. On the Landweber iteration for the solution of a parameter identification problem in a hyperbolic partial differential equation of second order. J. Inverse Ill-Posed Probl. 1998, 6, 403–430. [Google Scholar] [CrossRef]
- Kabanikhin, S.I.; Shishlenin, M.A. Quasi-solution in inverse coefficient problems. J. Inverse Ill-Posed Probl. 2008, 16, 705–713. [Google Scholar] [CrossRef]
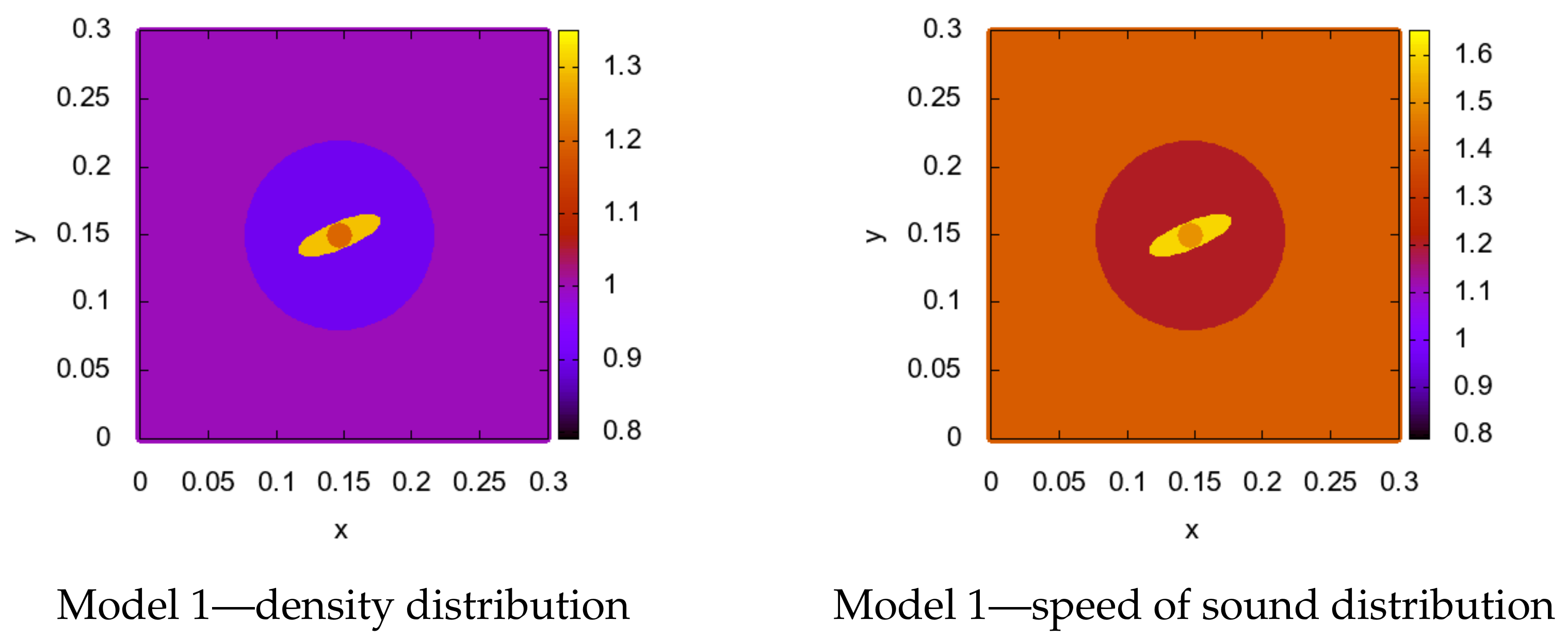
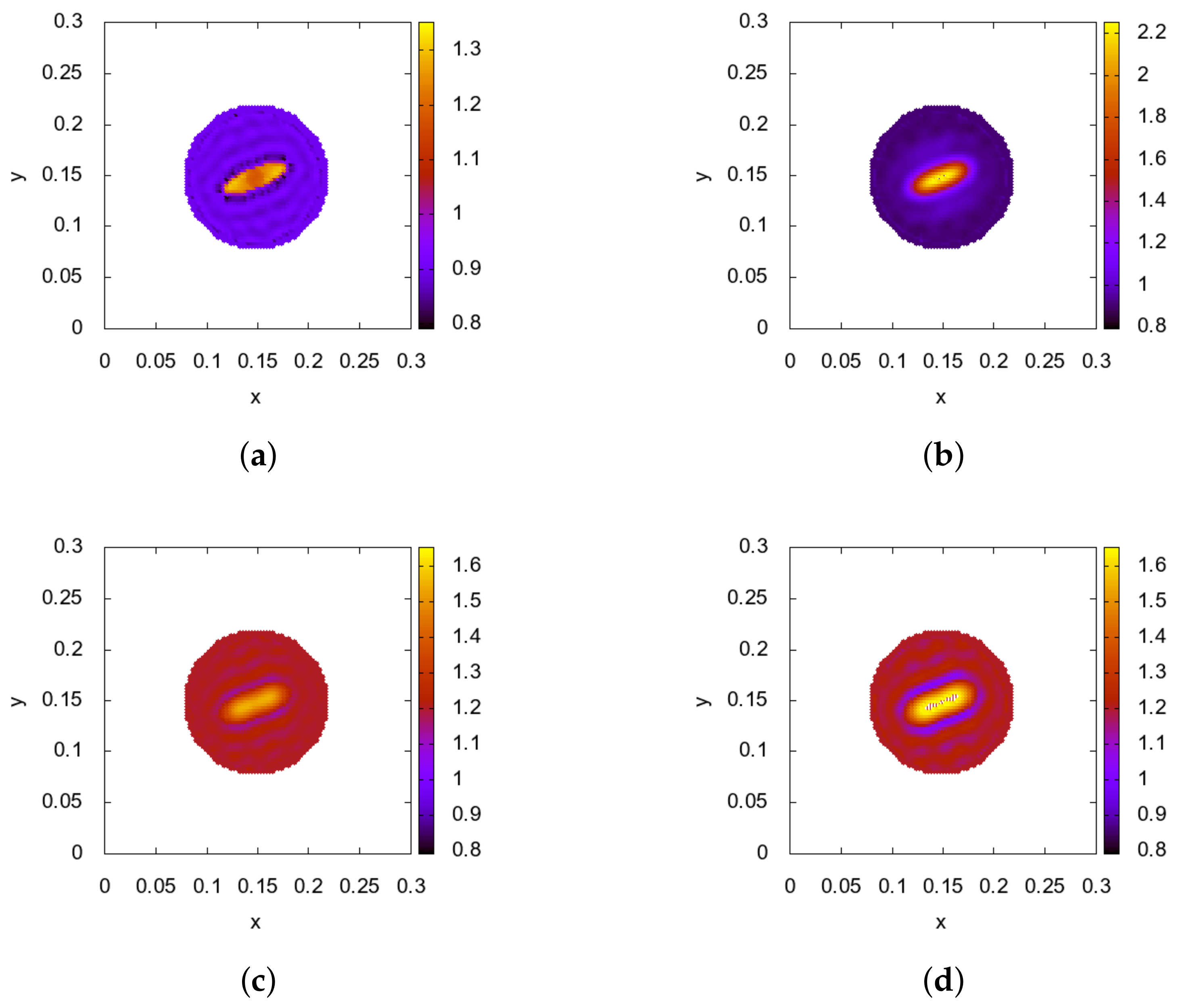
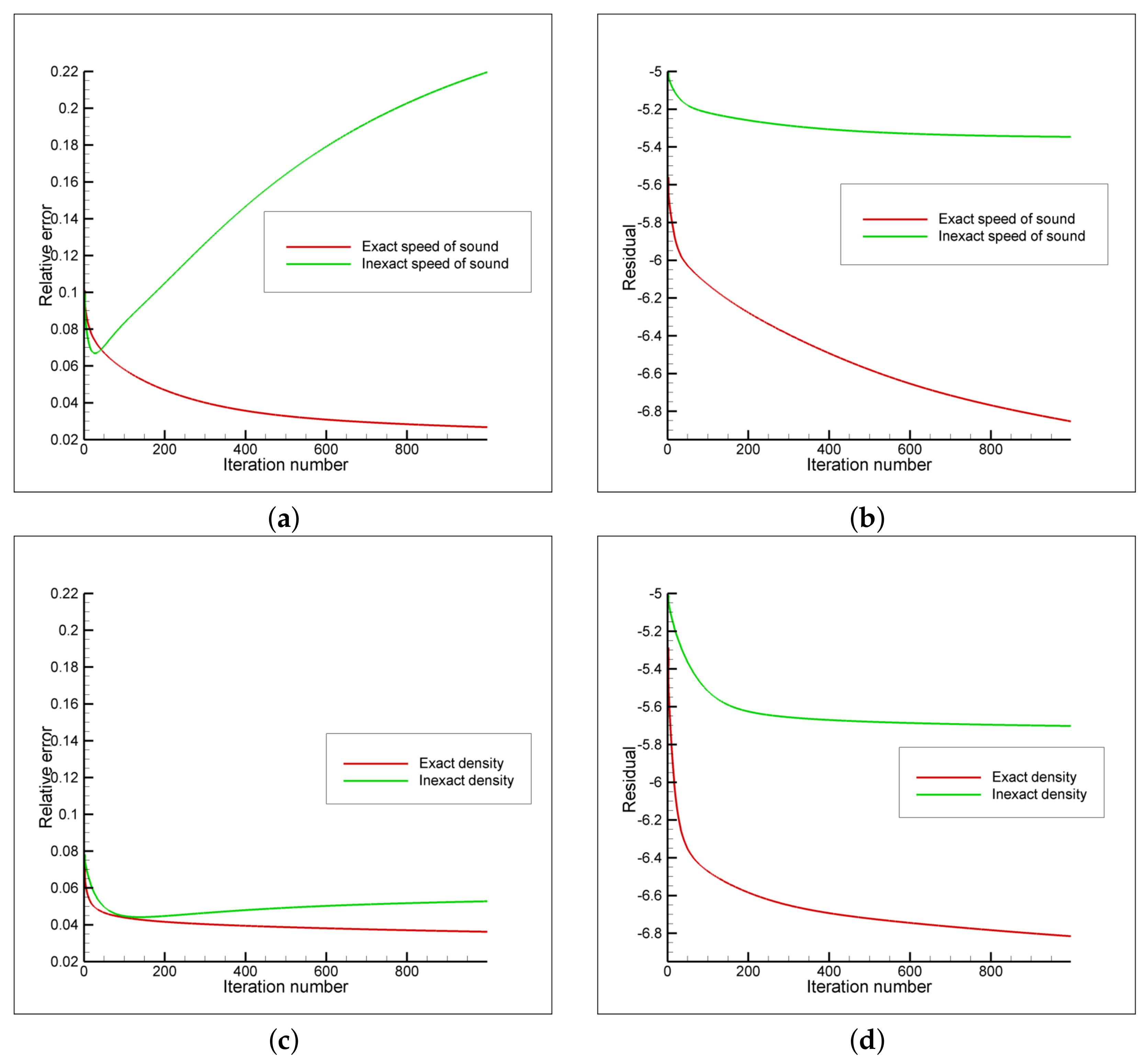
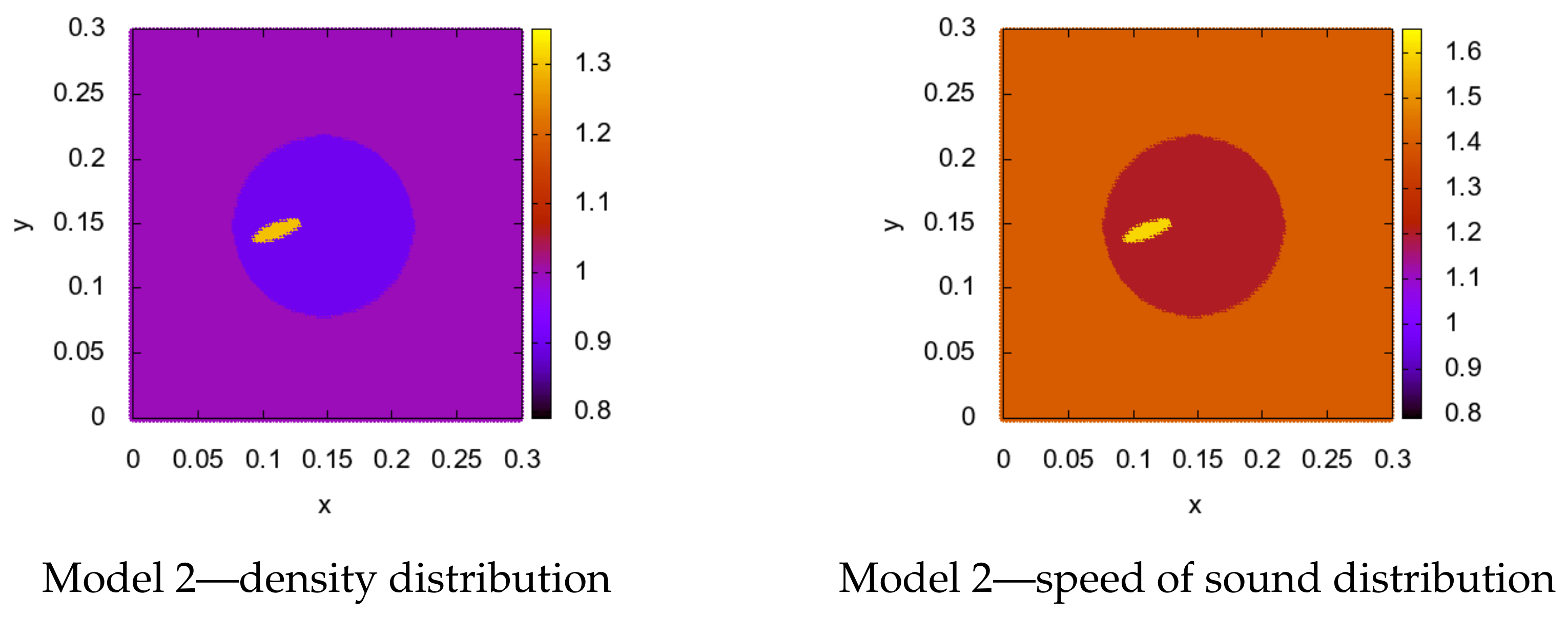


| Mesh Dependence-Exact Data | |||
|---|---|---|---|
| Mesh Size | Residual | Relative Error (Density) | Relative Error (Speed of Sound) |
| −4.95 | 0.056 | 0.049 | |
| −4.88 | 0.045 | 0.039 | |
| −4.85 | 0.034 | 0.029 | |
| Mesh Dependence-5% Noise in the Data | |||
|---|---|---|---|
| Mesh Size | Residual | Relative Error (Density) | Relative Error (Speed of Sound) |
| −3.51 | 0.058 | 0.052 | |
| −3.16 | 0.047 | 0.041 | |
| −2.86 | 0.036 | 0.031 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Klyuchinskiy, D.; Novikov, N.; Shishlenin, M. Recovering Density and Speed of Sound Coefficients in the 2D Hyperbolic System of Acoustic Equations of the First Order by a Finite Number of Observations. Mathematics 2021, 9, 199. https://doi.org/10.3390/math9020199
Klyuchinskiy D, Novikov N, Shishlenin M. Recovering Density and Speed of Sound Coefficients in the 2D Hyperbolic System of Acoustic Equations of the First Order by a Finite Number of Observations. Mathematics. 2021; 9(2):199. https://doi.org/10.3390/math9020199
Chicago/Turabian StyleKlyuchinskiy, Dmitriy, Nikita Novikov, and Maxim Shishlenin. 2021. "Recovering Density and Speed of Sound Coefficients in the 2D Hyperbolic System of Acoustic Equations of the First Order by a Finite Number of Observations" Mathematics 9, no. 2: 199. https://doi.org/10.3390/math9020199
APA StyleKlyuchinskiy, D., Novikov, N., & Shishlenin, M. (2021). Recovering Density and Speed of Sound Coefficients in the 2D Hyperbolic System of Acoustic Equations of the First Order by a Finite Number of Observations. Mathematics, 9(2), 199. https://doi.org/10.3390/math9020199





