The Learning Trajectories of Similarity in Mathematics Curriculum: An Epistemological Analysis of Hong Kong Secondary Mathematics Textbooks in the Past Half Century
Abstract
:1. Introduction
2. Background of the Study and Research Questions
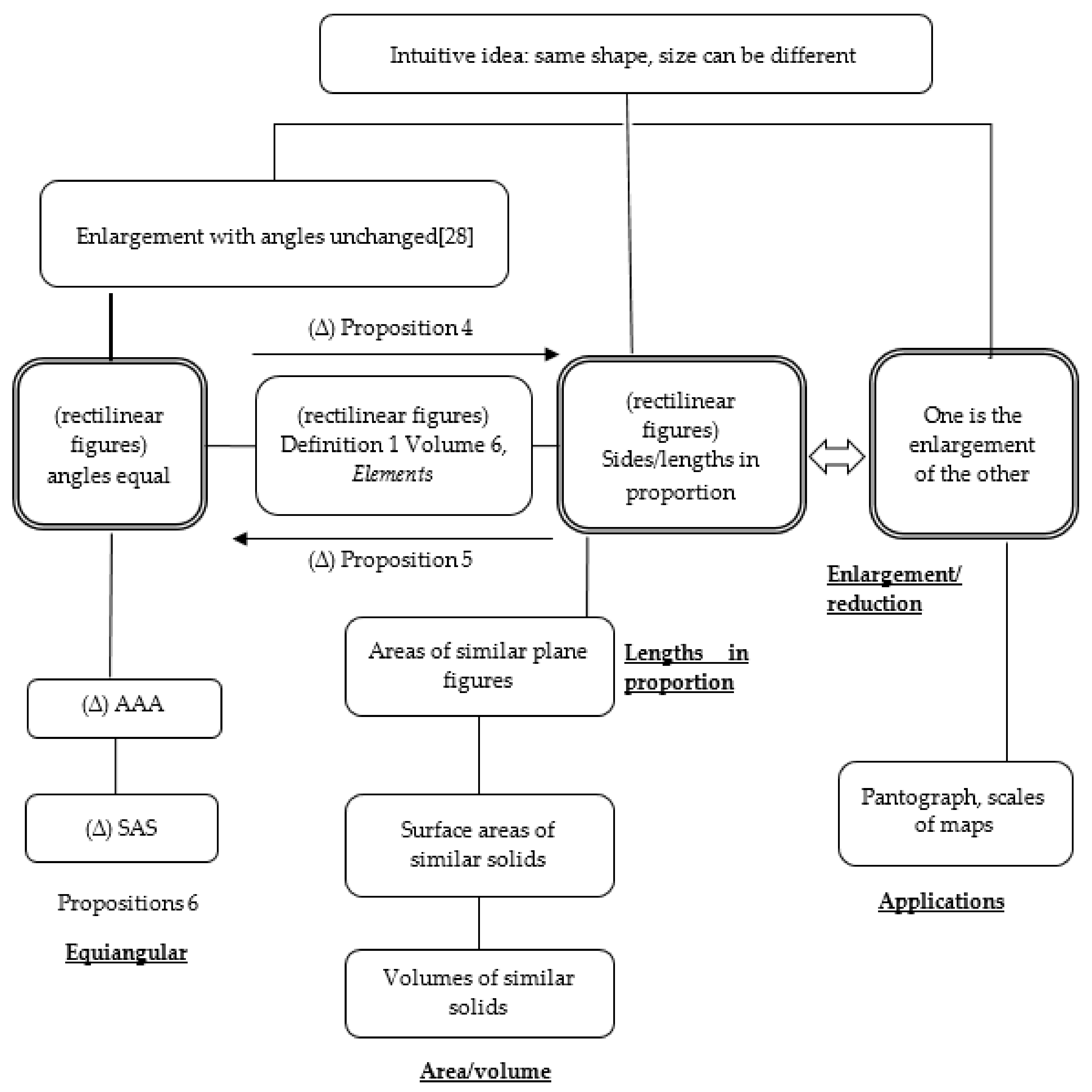
2.1. Definition of Similarity in Mathematics
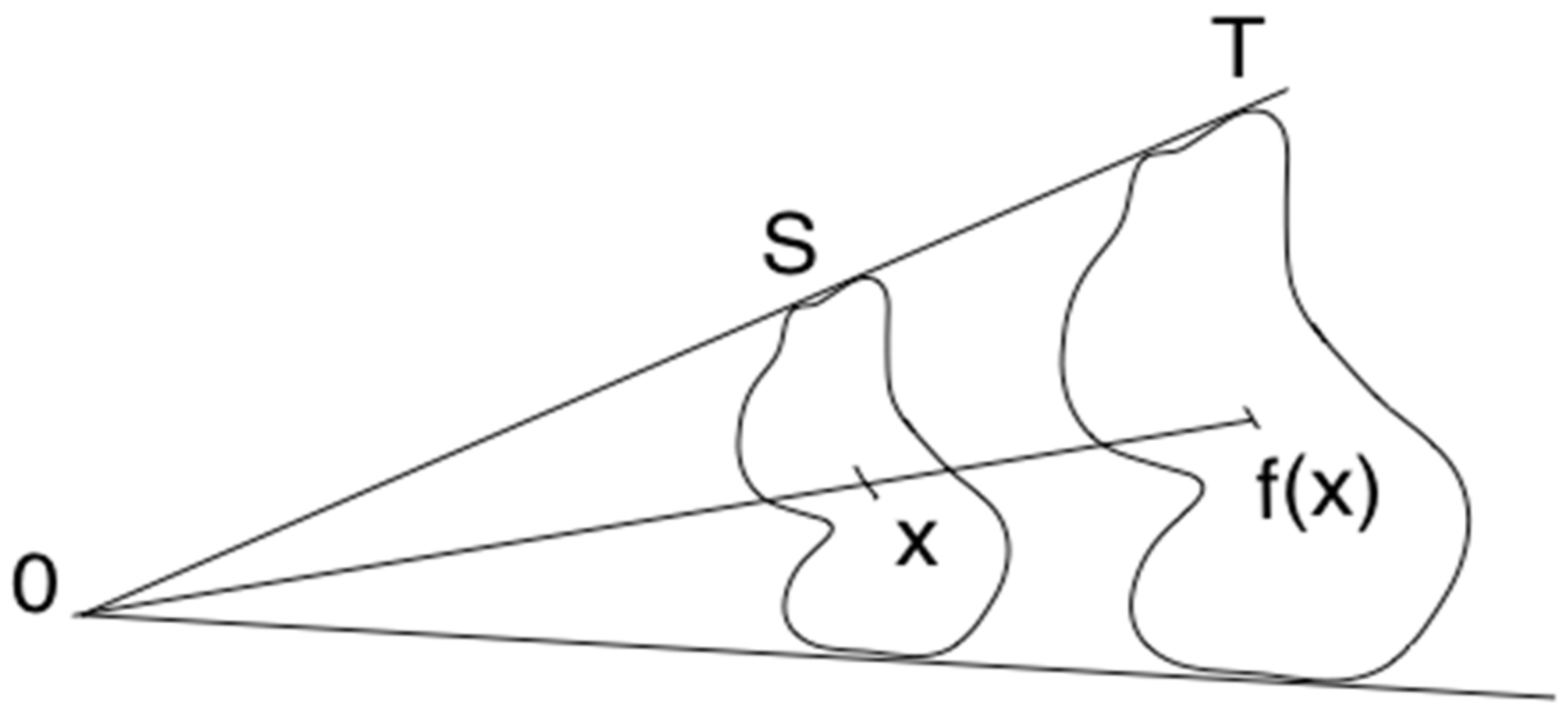
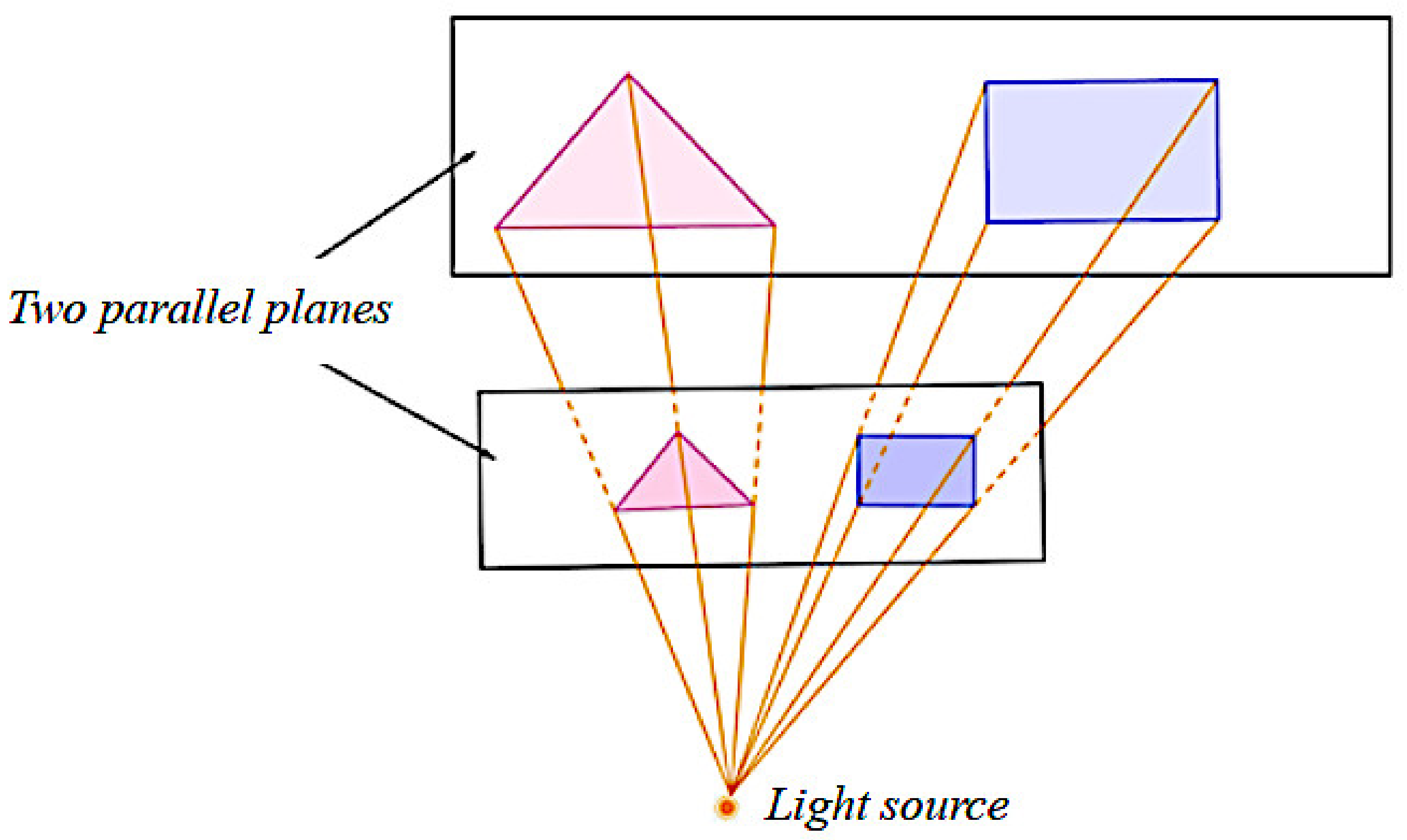
2.1.1. Definition of Similarity in Euclid’s Elements
2.1.2. The Notion of Enlargement: Similarity in 1980s’ Textbooks
2.2. Epistemological Analysis in Mathematics Education
2.3. Historical Development of Mathematics Textbooks: The Case in Hong Kong
3. Methodology
3.1. Content Analysis of Textbooks
3.2. Textbook Sampling
3.3. Data Analysis
4. Results
4.1. Definition: How Similarity Is Defined in Mathematics Textbooks across Time?
4.2. Arrangement: How the Contents of Similarity Are Arranged in Textbooks?
4.2.1. How Was the Content of Similarity Arranged in Current Textbooks?
4.2.2. How Was Similarity Introduced in Previous Textbooks?
- (a)
- Are yellow and brown similar colors?
- (b)
- Are orange and red similar colors?
- (c)
- Are English and Chinese similar languages?
- (d)
- Are all the pupils in your class similar sizes?
- (e)
- Are all circles similar shapes?
- (f)
- Are all triangles similar shapes?
- (g)
- Are all equilateral triangles similar shapes?
- (h)
- Are all pencils similar?
4.3. Proof: How Was the Proof of Similarity Introduced in Previous Textbooks
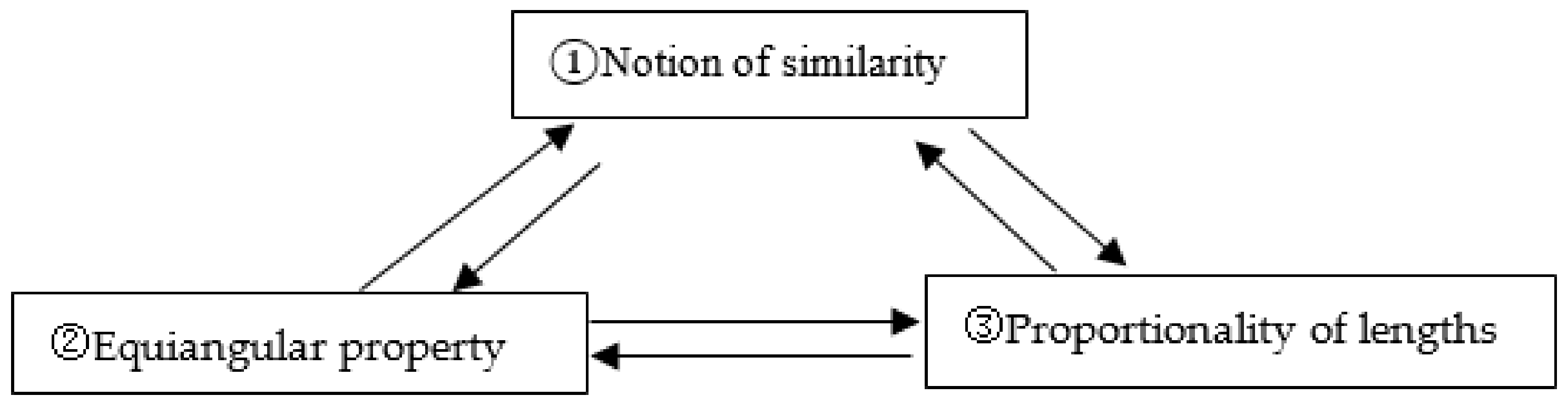
4.4. Topics of Similarity and Its Structure in Geometry Curriculum
5. Discussion
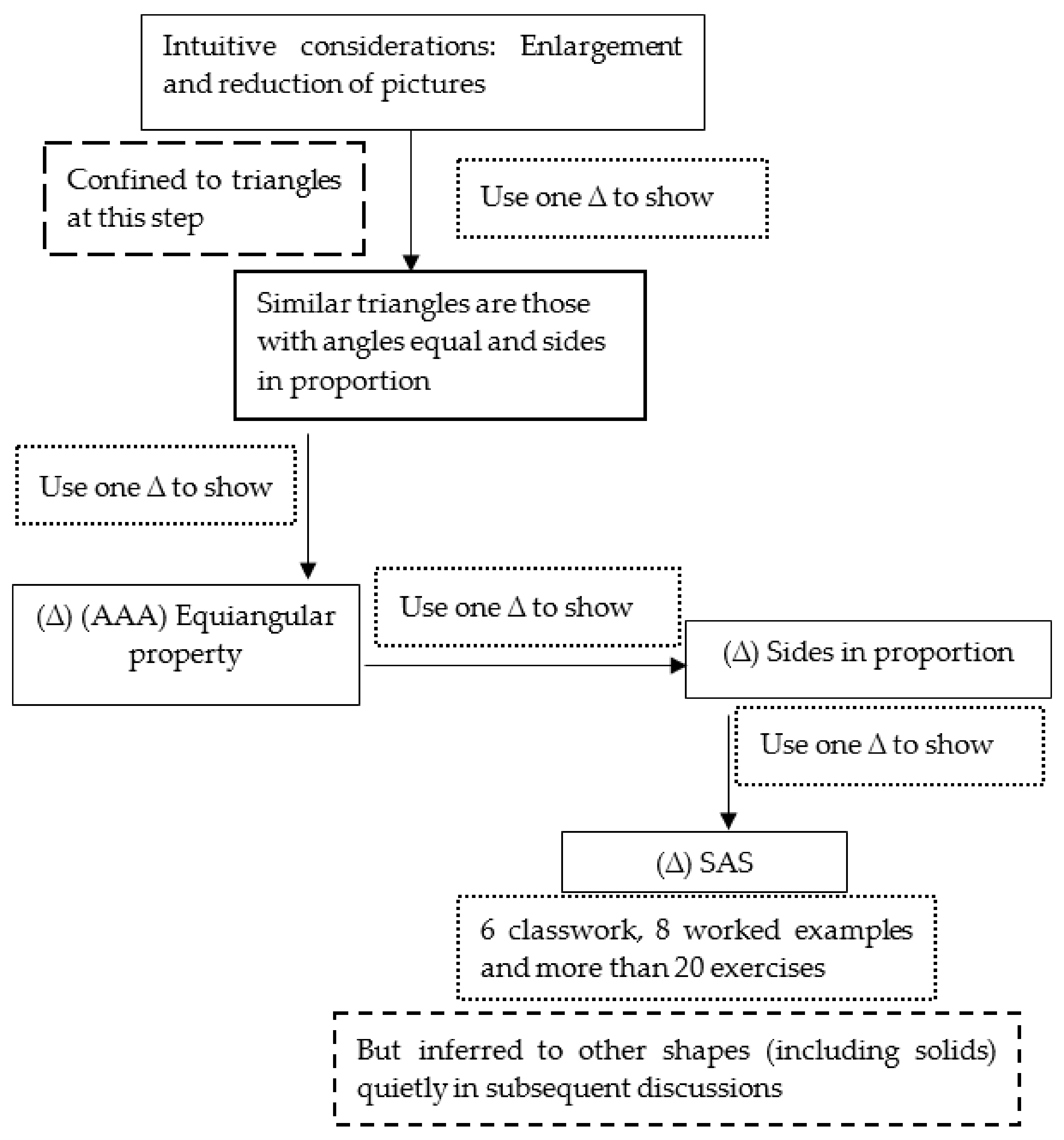
5.1. Approach 1: Using Local Axiomatics and Experimental Geometry
5.2. Approach 2: Using the Idea of Proofs (Idoofs)
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- González, G.; Herbst, P. Competing arguments for the geometry course: Why were American high school students supposed to study geometry in the 20th century? Int. J. Hist. Math. Educ. 2006, 1, 7–33. [Google Scholar]
- Clements, D.H.; Battista, M.T. Geometry and spatial reasoning. In Handbook of Research on Mathematics Teaching and Learning: A Project of the National Council of Teachers of Mathematics; Grouws, D.A., Ed.; Macmillan: New York, NY, USA, 1992; pp. 420–464. [Google Scholar]
- Herbst, P. Establishing a custom of proving in American school geometry: Evolution of the two-column proof in the early 20th century. Educ. Stud. Math. 2002, 49, 283–312. [Google Scholar] [CrossRef]
- Ayalon, M.; Even, R. Mathematics educators’ views on mathematics learning and the development of deductive reasoning. Int. J. Sci. Math. Educ. 2010, 8, 1131–1154. [Google Scholar] [CrossRef]
- Herbst, P.; Cheah, U.H.; Richard, P.R.; Jones, K. (Eds.) International Perspectives on the Teaching and Learning of Geometry in Secondary Schools (ICME-13 Monographs); Springer: Cham, Switzerland, 2018. [Google Scholar]
- Sinclair, N.; Bartolini-Bussi, M.; Villiers, M.D.; Jones, K.; Koretenkamp, U.; Leung, A.; Owens, K. Geometry education, including the use of new technologies: A survey of recent research. In Proceedings of the 13th International Congress on Mathematical Education. ICME-13 Monographs, Hamburg, Germany, 24–31 July 2016; Kaiser, G., Ed.; Springer: Cham, Switzerland, 2017; pp. 277–287. [Google Scholar]
- Vollrath, H.J. The understanding of similarity and shape in classifying tasks. Educ. Stud. Math. 1977, 8, 211–224. [Google Scholar] [CrossRef]
- NCTM. Principles and Standards for School Mathematics; NCTM: Reston, VA, USA, 2000. [Google Scholar]
- Cox, D.C. Similarity at the crossroads of geometry and number. Math. Think. Learn. 2013, 15, 3–23. [Google Scholar] [CrossRef]
- Kallia, M.; Panagiotis, S. The role of teaching in the development of basic concepts in geometry: How the concept of similarity and intuitive knowledge affect student’s perception of similar shapes. In Proceedings of the CERME 6, Lyon, France, 28 January–1 February 2009; Durand-Guerrier, V., Soury-Lavergne, S., Arzarello, F., Eds.; INRP: Lyon, France, 2010; pp. 736–745. [Google Scholar]
- Piaget, J. The Child’s Conception of Space; Routledge: Oxfordshire, UK, 1966. [Google Scholar]
- Lamon, S.J. Ratio and proportion: Connecting content and children’s thinking. J. Res. Math. Educ. 1983, 24, 41–61. [Google Scholar] [CrossRef]
- Dieudonné, J. New Thinking in School Mathematics; The Royaumont Seminar: Asnière-sur-Oise, France, 1959. [Google Scholar]
- Meder, A. What is wrong with Euclid? Math. Teach. 1958, 51, 578–584. [Google Scholar] [CrossRef]
- Wong, N.Y.; Tang, K.C. Mathematics education in Hong Kong under colonial rule. BSHM Bull. J. Br. Soc. Hist. Math. 2012, 27, 119–125. [Google Scholar] [CrossRef]
- Hersh, R.; John-Steiner, V. Loving and Hating Mathematics: Challenging the Myths of Mathematical Life; Princeton University Press: Princeton, NJ, USA, 2011. [Google Scholar]
- Wong, N.Y. (Ed.) The Long and Winding Road of Hong Kong Mathematics Education in the Past Half a Century: Starting the Discourse from the Modern Mathematics Movement; Hong Kong Association for Mathematics Education: Hong Kong, China, 2001. (In Chinese) [Google Scholar]
- Curriculum Development Council. Mathematics Education Key Learning Area Curriculum Guide (Primary 1—Secondary 3); Hong Kong SAR Government Logistic Department: Hong Kong, China, 2002.
- Cheung, K.L.; Wong, N.Y.; Lam, C.C. Reconstructing school geometry curriculum—Why and how should deductive geometry be taught? Mathmedia 2010, 34, 13–33. (In Chinese) [Google Scholar]
- Curriculum Development Council. Mathematics Education Key Learning Area Curriculum Guide (Primary 1—Secondary 6); Hong Kong SAR Government Logistic Department: Hong Kong, China, 2017.
- Reys, R.; Reys, B.; Lapan, R.; Holliday, G.; Wasman, D. Assessing the impact of standards-based middle grades mathematics curriculum materials on student achievement. J. Res. Math. Educ. 2003, 34, 74–95. [Google Scholar] [CrossRef]
- Stein, M.; Remillard, J.; Smith, M. How curriculum influences students’ learning. In Second Handbook of Research on Mathematics Teaching and Learning; Lester, F., Ed.; Information Age: Charlotte, NC, USA, 2007; pp. 557–628. [Google Scholar]
- Hanna, G.; de Villiers, M. (Eds.) Aspects of proof in mathematics education. In Proof and Proving in Mathematics Education: The ICMI Study; Springer: New York, NY, USA, 2012; pp. 1–10. [Google Scholar]
- Miyazaki, M.; Fujita, T.; Jones, K. Students’ understanding of the structure of deductive proof. Educ. Stud. Math. 2017, 94, 223–239. [Google Scholar] [CrossRef] [Green Version]
- Jones, K. Deductive and intuitive approaches to solving geometrical problems. In Perspectives on the Teaching of Geometry for the 21st Century; Mammana, C., Villani, V., Eds.; Kluwer: Dordrecht, The Netherlands, 1998; pp. 78–83. [Google Scholar]
- Seago, N.M.; Jacobs, J.K.; Heck, D.J.; Nelson, C.L.; Malzahn, K.A. Impacting teachers’ understanding of geometric similarity: Results from field testing of the learning and teaching geometry professional development materials. Prof. Dev. Educ. 2014, 40, 627–653. [Google Scholar] [CrossRef]
- Fitzpatrick, R. Euclid’s Elements of Geometry. In The Greek Text of J.L. Heiberg (1883–1885) from Euclidis Element; University of Texas at Austin: Austin, TX, USA, 2007; Available online: http://farside.ph.utexas.edu/Books/Euclid/Elements.pdf (accessed on 9 August 2021).
- Coxeter, H.S.M.; Greitzer, S.L. Geometry Revisited; Mathematical Association of America: Washington, DC, USA, 1967. [Google Scholar]
- Chan, L.K.F.; Leung, C.T.; Wise, S.R. Mathematics for Hong Kong; Canotta Publishing Company: Hong Kong, China, 1988. [Google Scholar]
- Radford, L. Epistemology as a research category in mathematics teaching and learning. In The Didactics of Mathematics: Approaches and Issues; Hodgson, B.R., Kuzniak, A., Lagrange, J.B., Eds.; Springer: Cham, Switzerland, 2016; pp. 31–41. [Google Scholar]
- Barbin, E.; Stehlíková, N.; Tzanakis, C. History and Epistemology in Mathematics Education; Vydavatelský Servis: Prague, Czech, 2008. [Google Scholar]
- Wong, N.Y. The Hong Kong mathematics curriculum: 1997 × MATH × ED = Period of Change. In Reforms and Issues in School Mathematics in East Asia; Leung, F.K.S., Li, Y., Eds.; Sense Publications: Rotterdam, The Netherlands, 2010; pp. 33–57. [Google Scholar]
- Nicol, C.C.; Crespo, S.M. Learning to teach with mathematics textbooks: How preservice teachers interpret and use curriculum materials. Educ. Stud. Math. 2006, 62, 331–355. [Google Scholar] [CrossRef]
- Haggarty, L.; Pepin, B. An investigation of mathematics textbooks and their use in English, French, and German Classrooms: Who gets an opportunity to learn what? Br. Educ. Res. J. 2002, 28, 567–590. [Google Scholar] [CrossRef]
- Simon, M.; Tzur, R. Explicating the role of mathematical tasks in conceptual learning: An elaboration of the hypothetical learning trajectory. Math. Think. Learn. 2004, 6, 91–104. [Google Scholar] [CrossRef]
- Weber, E.; Walkington, C.; McGalliard, W. Expanding notions of “learning trajectories” in mathematics education. Math. Think. Learn. 2015, 17, 253–272. [Google Scholar] [CrossRef]
- Siu, M.K. Concept of function: Its history and teaching. In Learn from the Masters: Proceedings of Workshop on History of Mathematics Kristiansand; Swetz, T.F., Fauvel, J., Bekken, O., Johansson, B., Katz, V., Eds.; Mathematics Association of America: Washington DC, USA, 1995; pp. 105–121. [Google Scholar]
- Mills, A.J.; Durepos, G.; Wiebe, E. (Eds.) Encyclopedia of Case Study Research; Sage: Thousand Oaks, CA, USA, 2010; Volume 1, pp. 225–230. [Google Scholar]
- Mesa, V. Characterizing practices associated with functions in middle school textbooks: An empirical approach. Educ. Stud. Math. 2004, 56, 255–286. [Google Scholar] [CrossRef]
- Weinberg, A.; Wiesner, E. Understanding mathematics textbooks through reader-oriented theory. Educ. Stud. Math. 2011, 76, 49–63. [Google Scholar] [CrossRef]
- Charalambous, C.Y.; Delaney, S.; Hsu, H.Y.; Mesa, V. A comparative analysis of the addition and subtraction of fractions in textbooks from three countries. Math. Think. Learn. 2010, 12, 117–151. [Google Scholar] [CrossRef] [Green Version]
- Durell, C.V. A New Geometry for Schools; G. Bell & Sons Ltd.: London, UK, 1939. [Google Scholar]
- Millo, B.A.; Millo, C.A. School Mathematics Project for Hong Kong; Eastern University Press: Hong Kong, China, 1967. [Google Scholar]
- School Mathematics Monoid. Modern Mathematics; United College Press: Hong Kong, China, 1966. [Google Scholar]
- Parkin, F.; Teng, S.B.; Wills, G. New Curriculum Mathematics; Revised edition; Oxford University Press: Hong Kong, China, 1977. [Google Scholar]
- Leung, K.T.; Chen, D.L.C.; Chan, C.L. Basic Mathematics; Longman Group (Far East) Ltd.: Hong Kong, China, 1975. [Google Scholar]
- Man, P.F.; Yeung, C.M.; Yueng, K.H.; Kowk, Y.F.; Cheung, H.Y. Mathematics in Action, 2nd ed.; Pearson: Hong Kong, China, 2014. [Google Scholar]
- Krippendorff, K. Content Analysis: An Introduction to Its Methodology; Sage Publications: Newbury Park, CA, USA, 1980. [Google Scholar]
- Strauss, A.; Corbin, J.M. Basics of Qualitative Research: Grounded Theory Procedures and Techniques; Sage Publications: Thousand Oaks, CA, USA, 1990. [Google Scholar]
- Fischbein, E. Intuition and proof. For. Learn. Math. 1982, 3, 9–24. [Google Scholar]
- Artigue, M. The role of epistemology in the analysis of teaching/learning relationships in mathematics education. In Proceedings of the 1995 Annual Meeting of the Canadian Mathematics Education Study Group, Ontario, Canada, 26–30 May 1995; Pothier, Y.M., Ed.; University of Western Ontario: London, ON, Canada, 1995; pp. 7–21. [Google Scholar]
- Fischbein, E. Intuition in Science and Mathematics: An Educational Approach; Reidel: Dordrecht, The Netherlands, 1987. [Google Scholar]
- Wong, N.Y.; Han, J.W.; Lee, P.Y. The mathematics curriculum: Towards globalisation or Westernisation? In How Chinese Learn Mathematics: Perspectives from Insiders; Fan, L., Wong, N.Y., Cai, J., Li, S., Eds.; World Scientific: Singapore, 2004; pp. 27–70. [Google Scholar]
- Kuzniak, A. Thinking about the teaching geometry through the lens of the theory of geometric working spaces. In International Perspectives on the Teaching and Learning of Geometry in Secondary Schools (ICME-13 Monographs); Herbst, P., Cheah, U.H., Richard, P.R., Jones, K., Eds.; Springer: Cham, Switzerland, 2018; pp. 5–24. [Google Scholar]
- California State Board of Education. Mathematics Framework for California Public Schools: Kindergarten through Grade Twelve; California State Board of Education: Sacramento, CA, USA, 1999.
- Fujita, T.; Jones, K. The place of experimental tasks in geometry teaching: Learning from the textbook designs of the early 20th century. Res. Math. Educ. 2003, 5, 47–62. [Google Scholar] [CrossRef] [Green Version]
- Jones, K.; Gutiérrez, A.; Mariotti, M.A. (Eds.) Proof in dynamic geometry environments: A special issue. Educ. Stud. Math. 2000, 44, 1–3. [Google Scholar] [CrossRef]
- Leung, A.; Lee, A.M.S. Students’ geometrical perception on a task-based dynamic geometry platform. Educ. Stud. Math. 2013, 82, 361–377. [Google Scholar] [CrossRef]
- Chazan, D. Similarity: Exploring the Understanding of a Geometric Concept; Technical Report 88–15; Educational Technology Center: Cambridge, MA, USA, 1998. [Google Scholar]
- Common Core State Standards Initiative. Common Core States Standards for Mathematics; National Governors Association Center for Best Practices and the Council of Chief State School Officers: Washington, DC, USA, 2010. [Google Scholar]

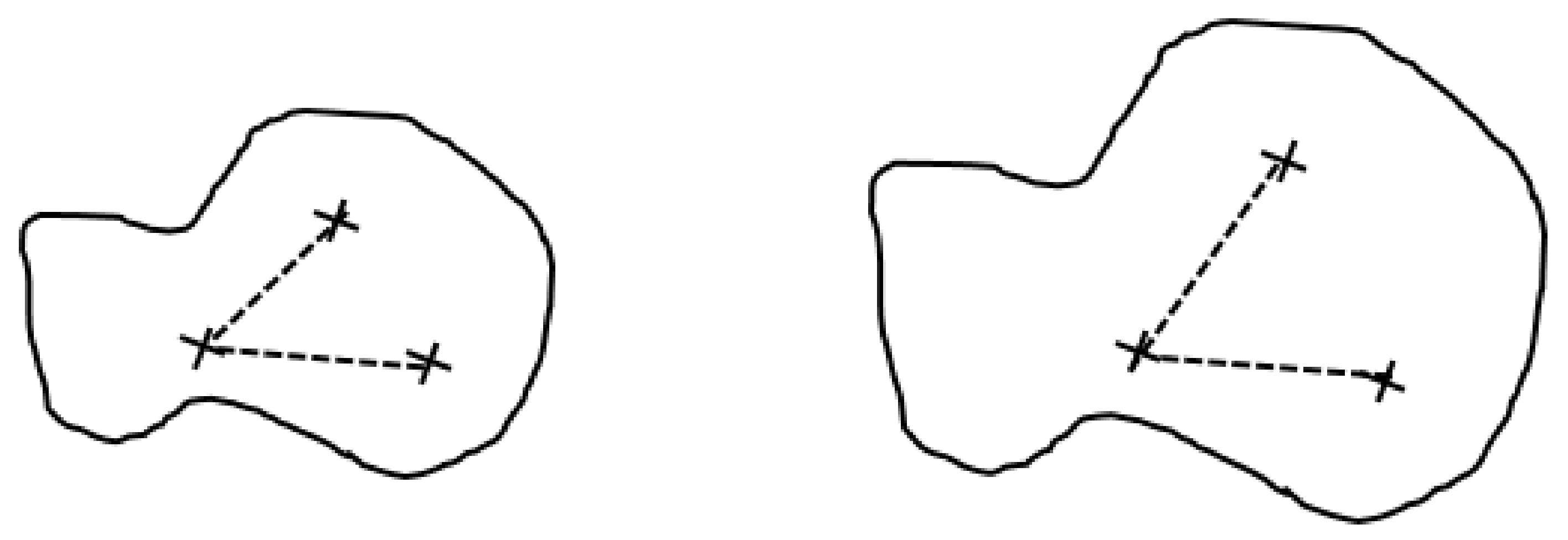
| Period | Textbook and Volumes that Concern Similarity | Reasons for Selection |
|---|---|---|
| 1. Traditional mathematics (1960s) | [I]: A new geometry for schools. Stages A and B [42] | Classic textbooks |
| 2. Modern mathematics (1960s–1980s) | [II]: School mathematics project for Hong Kong. Book 2 [43] | Claimed to have adapted the British SMP to Hong Kong |
| [III]: Modern mathematics. Secondary 1 and 3 [44] | Pioneer of Modern Mathematics movement in Hong Kong | |
| 3. Back to basics (1980s–1990s) | [IV]: New curriculum mathematics (revised edition). Book 3 [45] | Authors were mathematics inspectors, so it aimed to realise the curriculum |
| [V]: Basic mathematics. Book 3 [46] | Authors were lecturers of a mathematics department | |
| 4. Current (Since 2000) | [VI] Mathematics in action (second edition) [47] | Popular textbooks |
| Period | Textbooks That Concern Similarity | How Similarity Is Defined in the Textbook |
|---|---|---|
| 1. Traditional mathematics | [I] Stage A | Uses ② as the definition, confined to triangles right from the start. |
| [I] Stage B | Revisits rectilinear figures. | |
| 2. Modern mathematics | [II] Book 2 | Starts with a discussion of “similar shapes,” without confining them to triangles. Then, by using many examples to generate similar figures that preserve angles, the corresponding lengths of the two figures are proportional. |
| [III] Secondary 1 and 3 | Starts with intuitive considerations, then ④, confined to rectilinear figures. | |
| 3. Back to basics | [IV] Book 3 | Starts with intuitive considerations, then ④, confined to rectilinear figures. Later: similar triangles are those with equal angles and sides in proportion. |
| [V] Book 3 | Starts with intuitive considerations, then ④, confined to triangles. Later: ① for rectilinear figures. |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, Q.-P.; Wong, N.-Y. The Learning Trajectories of Similarity in Mathematics Curriculum: An Epistemological Analysis of Hong Kong Secondary Mathematics Textbooks in the Past Half Century. Mathematics 2021, 9, 2310. https://doi.org/10.3390/math9182310
Zhang Q-P, Wong N-Y. The Learning Trajectories of Similarity in Mathematics Curriculum: An Epistemological Analysis of Hong Kong Secondary Mathematics Textbooks in the Past Half Century. Mathematics. 2021; 9(18):2310. https://doi.org/10.3390/math9182310
Chicago/Turabian StyleZhang, Qiao-Ping, and Ngai-Ying Wong. 2021. "The Learning Trajectories of Similarity in Mathematics Curriculum: An Epistemological Analysis of Hong Kong Secondary Mathematics Textbooks in the Past Half Century" Mathematics 9, no. 18: 2310. https://doi.org/10.3390/math9182310
APA StyleZhang, Q.-P., & Wong, N.-Y. (2021). The Learning Trajectories of Similarity in Mathematics Curriculum: An Epistemological Analysis of Hong Kong Secondary Mathematics Textbooks in the Past Half Century. Mathematics, 9(18), 2310. https://doi.org/10.3390/math9182310






