Fast Localization of Small Inhomogeneities from Far-Field Pattern Data in the Limited-Aperture Inverse Scattering Problem
Abstract
1. Introduction
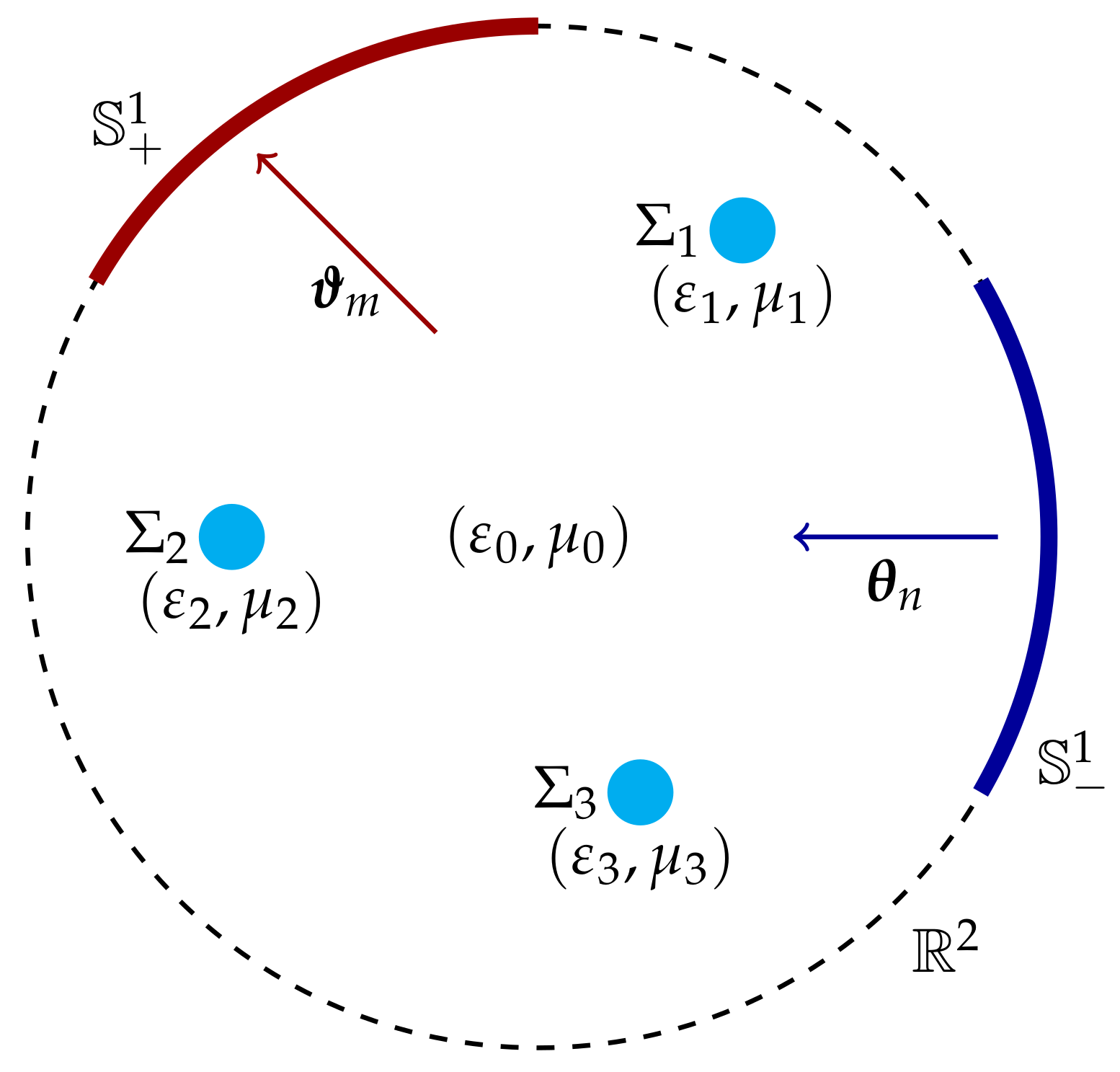
2. Direct Scattering Problem and Far-Field Pattern
3. Introduction and Analysis of Indicator Functions
3.1. Permittivity Contrast Case
3.2. Permeability Contrast Case
3.3. The Case of Both Permittivity and Permeability Contrasts
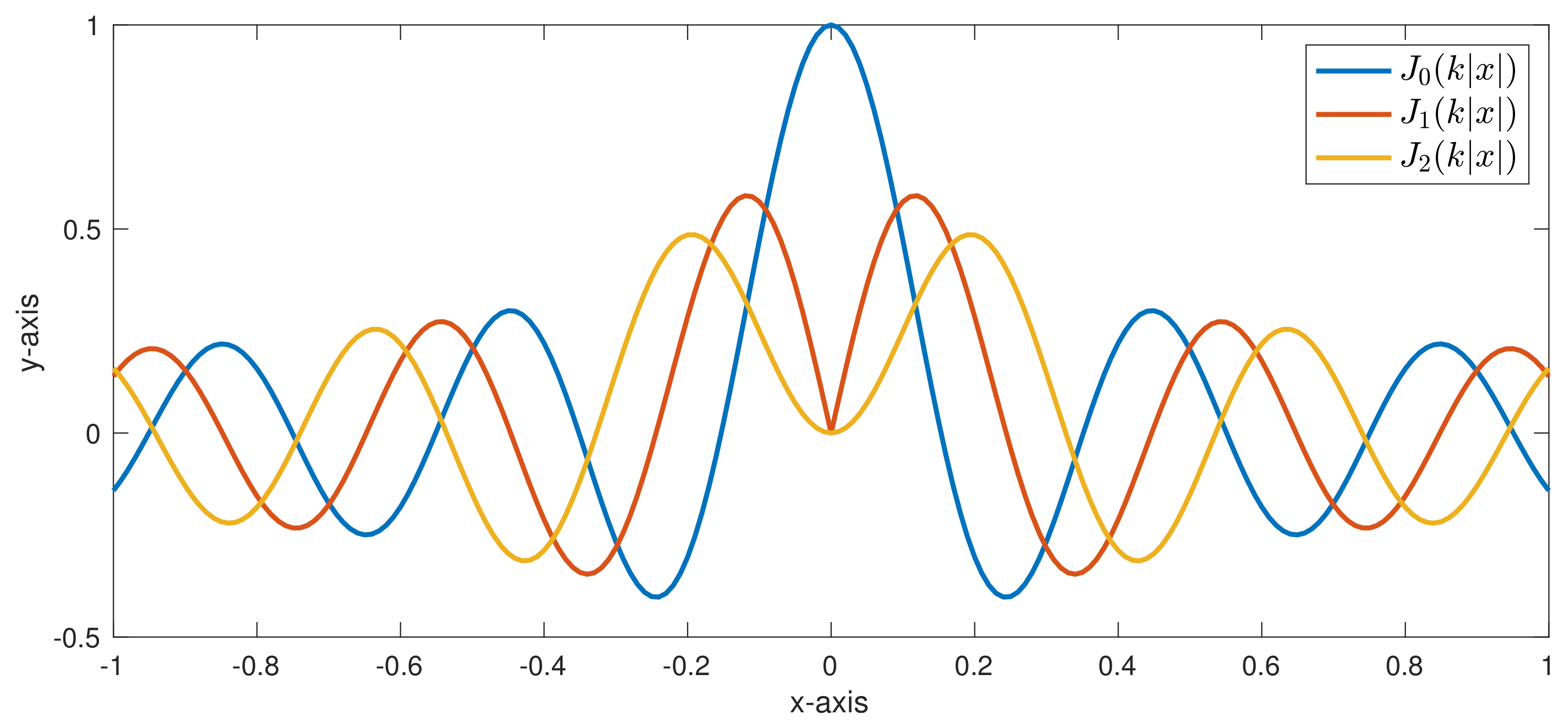
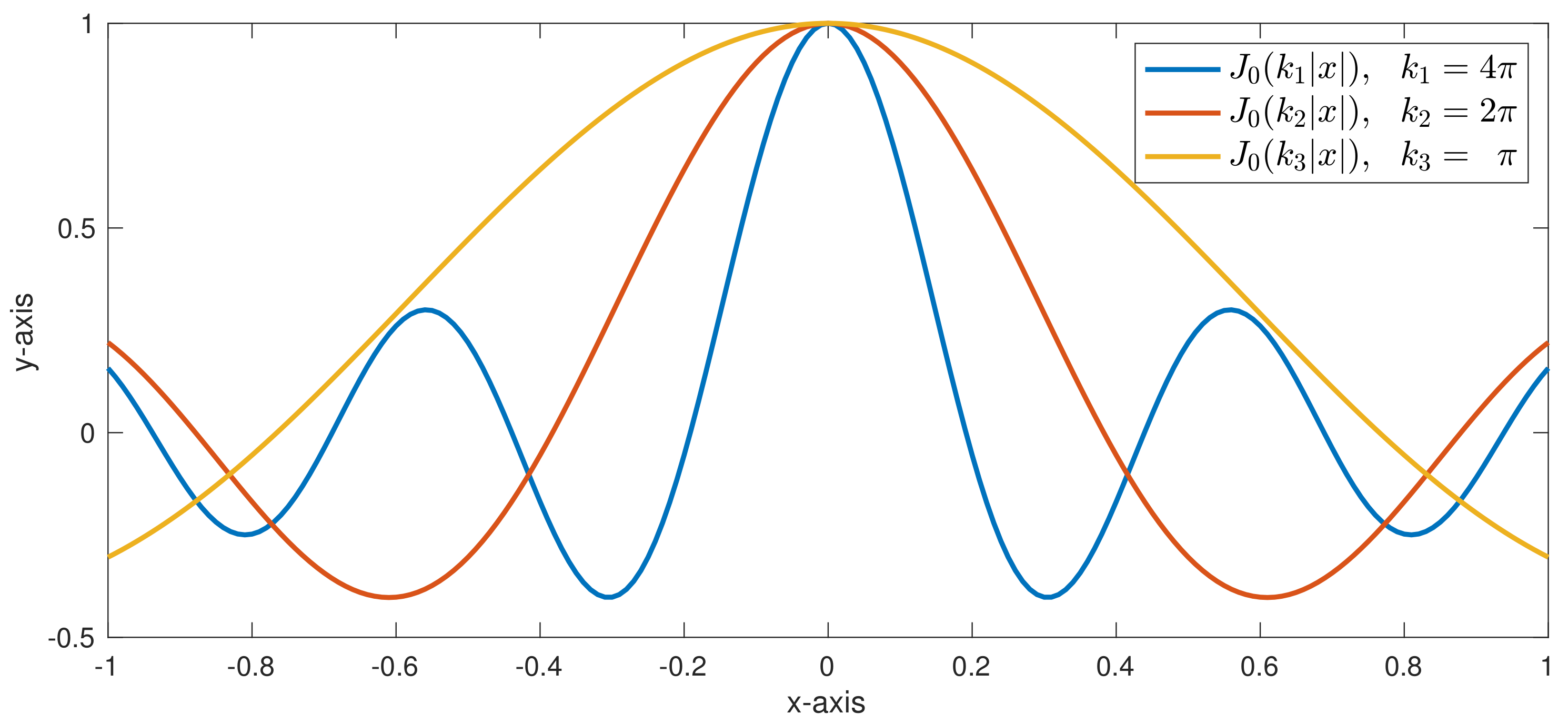
3.4. Properties of the Indicator Functions
4. Simulation Results
5. Conclusions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. Proof of Theorem 1
Appendix B. Proof of Lemma 1
Appendix C. Proof of the Theorem 2
References
- Chew, W.C. Lectures on Theory of Microwave and Optical Waveguides. arXiv 2015, arXiv:2107.09672. [Google Scholar]
- Colton, D.; Kress, R. Inverse Acoustic and Electromagnetic Scattering Problems; Mathematics and Applications Series; Springer: New York, NY, USA, 1998. [Google Scholar]
- Ahn, C.Y.; Chae, S.; Park, W.K. Fast identification of short, sound-soft open arcs by the orthogonality sampling method in the limited-aperture inverse scattering problem. Appl. Math. Lett. 2020, 109, 106556. [Google Scholar] [CrossRef]
- Ahn, C.Y.; Ha, T.; Park, W.K. Direct sampling method for identifying magnetic inhomogeneities in limited-aperture inverse scattering problem. Comput. Math. Appl. 2020, 80, 2811–2829. [Google Scholar] [CrossRef]
- Cox, B.T.; Arridge, S.; Beard, P.C. Photoacoustic tomography with a limited-aperture planar sensor and a reverberant cavity. Inverse Prob. 2007, 23, S95–S112. [Google Scholar] [CrossRef]
- Ikehata, M.; Niemi, E.; Siltanen, S. Inverse obstacle scattering with limited-aperture data. Inverse Probl. Imag. 2012, 1, 77–94. [Google Scholar] [CrossRef]
- Mager, R.; Bleistein, N. An examination of the limited aperture problem of physical optics inverse scattering. IEEE Trans. Antennas Propag. 1978, 26, 695–699. [Google Scholar] [CrossRef]
- Ochs, R.L. The limited aperture problem of inverse acoustic scattering: Dirichlet boundary conditions. SIAM J. Appl. Math. 1987, 47, 1320–1341. [Google Scholar] [CrossRef]
- Park, W.K. Fast imaging of short perfectly conducting cracks in limited-aperture inverse scattering problem. Electronics 2019, 8, 1050. [Google Scholar] [CrossRef]
- Zinn, A. On an optimisation method for the full- and the limited-aperture problem in inverse acoustic scattering for a sound-soft obstacle. Inverse Prob. 1989, 5, 239–253. [Google Scholar] [CrossRef]
- Ammari, H.; Kang, H. Reconstruction of Small Inhomogeneities from Boundary Measurements; Lecture Notes in Mathematics; Springer: Berlin, Germany, 2004; Volume 1846. [Google Scholar]
- Vogelius, M.; Volkov, D. Asymptotic formulas for perturbations in the electromagnetic fields due to Asymptotic formulas for perturbations in the electromagnetic fields due to 34 the presence of inhomogeneities of small diameter the presence of inhomogeneities of small diameter. ESAIM M2AN 2000, 34, 723–748. [Google Scholar] [CrossRef]
- Ammari, H.; Garnier, J.; Kang, H.; Park, W.K.; Sølna, K. Imaging schemes for perfectly conducting cracks. SIAM J. Appl. Math. 2011, 71, 68–91. [Google Scholar] [CrossRef]
- Ammari, H.; Garnier, J.; Kang, H.; Lim, M.; Sølna, K. Multistatic imaging of extended targets. SIAM J. Imag. Sci. 2012, 5, 564–600. [Google Scholar] [CrossRef]
- Park, W.K. Multi-frequency subspace migration for imaging of perfectly conducting, arc-like cracks in full- and limited-view inverse scattering problems. J. Comput. Phys. 2015, 283, 52–80. [Google Scholar] [CrossRef]
- Park, W.K. Reconstruction of thin electromagnetic inhomogeneity without diagonal elements of a multistatic response matrix. Inverse Prob. 2018, 34, 095008. [Google Scholar] [CrossRef]
- Audibert, L.; Haddar, H. The generalized linear sampling method for limited aperture measurements. SIAM J. Imag. Sci. 2017, 10, 845–870. [Google Scholar] [CrossRef]
- Cheney, M. The linear sampling method and the MUSIC algorithm. Inverse Prob. 2001, 17, 591–595. [Google Scholar] [CrossRef]
- Colton, D.; Haddar, H.; Monk, P. The linear sampling method for solving the electromagnetic inverse scattering problem. SIAM J. Sci. Comput. 2002, 24, 719–731. [Google Scholar] [CrossRef][Green Version]
- Haddar, H.; Monk, P. The linear sampling method for solving the electromagnetic inverse medium problem. Inverse Prob. 2002, 18, 891–906. [Google Scholar] [CrossRef]
- Ito, K.; Jin, B.; Zou, J. A direct sampling method to an inverse medium scattering problem. Inverse Prob. 2012, 28, 025003. [Google Scholar] [CrossRef]
- Kang, S.; Lambert, M.; Park, W.K. Multi-frequency direct sampling method in inverse scattering problem. J. Phys. Conf. Ser. 2017, 904, 012018. [Google Scholar] [CrossRef]
- Li, J.; Zou, J. A direct sampling method for inverse scattering using far-field data. Inverse Probl. Imag. 2013, 7, 757–775. [Google Scholar] [CrossRef]
- Park, W.K. Direct sampling method for retrieving small perfectly conducting cracks. J. Comput. Phys. 2018, 373, 648–661. [Google Scholar] [CrossRef]
- Chae, S.; Ahn, C.Y.; Park, W.K. Localization of small anomalies via orthogonality sampling method from scattering parameters. Electronics 2020, 9, 1119. [Google Scholar] [CrossRef]
- Griesmaier, R. Multi-frequency orthogonality sampling for inverse obstacle scattering problems. Inverse Prob. 2011, 27, 085005. [Google Scholar] [CrossRef]
- Harris, I.; Nguyen, D.L. Orthogonality sampling method for the electromagnetic inverse scattering problem. SIAM J. Sci. Comput. 2020, 42, B722–B737. [Google Scholar] [CrossRef]
- Potthast, R. A study on orthogonality sampling. Inverse Prob. 2010, 26, 074015. [Google Scholar] [CrossRef]
- Park, W.K. Fast location search of small anomaly by using microwave. Int. J. Appl. Electromagn. Mech. 2019, 59, 1505–1510. [Google Scholar] [CrossRef]
- Park, W.K. Real-time microwave imaging of unknown anomalies via scattering matrix. Mech. Syst. Signal Proc. 2019, 118, 658–674. [Google Scholar] [CrossRef]
- Park, W.K. Fast imaging of thin, curve-like electromagnetic inhomogeneities without a priori information. Mathematics 2020, 8, 799. [Google Scholar] [CrossRef]
- Chow, Y.T.; Ito, K.; Liu, K.; Zou, J. Direct sampling method for diffusive optical tomography. SIAM J. Sci. Comput. 2015, 37, A1658–A1684. [Google Scholar] [CrossRef]
- Chow, Y.T.; Ito, K.; Zou, J. A direct sampling method for electrical impedance tomography. Inverse Prob. 2014, 30, 095003. [Google Scholar] [CrossRef]
- Park, W.K. Theoretical identification of coupling effect and performance analysis of single-source direct sampling method. Mathematics 2021, 9, 1065. [Google Scholar] [CrossRef]
- Son, S.H.; Lee, K.J.; Park, W.K. Application and analysis of direct sampling method in real-world microwave imaging. Appl. Math. Lett. 2019, 96, 47–53. [Google Scholar] [CrossRef]
- Akinci, M.N. An efficient sampling method for cross-borehole GPR imaging. IEEE Geosci. Remote Sens. Lett. 2018, 15, 1857–1861. [Google Scholar] [CrossRef]
- Akinci, M.N.; Çayören, M.; Akduman, İ. Near-field orthogonality sampling method for microwave imaging: Theory and experimental verification. IEEE Trans. Microwave Theory Tech. 2016, 64, 2489–2501. [Google Scholar] [CrossRef]
- Bevacqua, M.T.; Isernia, T. Shape reconstruction via equivalence principles, constrained inverse source problems and sparsity promotion. Prog. Electromagn. Res. 2017, 158, 47–48. [Google Scholar] [CrossRef]
- Bevacqua, M.T.; Isernia, T. Boundary indicator for aspect limited sensing of hidden dielectric objects. IEEE Geosci. Remote Sens. Lett. 2018, 15, 838–842. [Google Scholar] [CrossRef]
- Bevacqua, M.T.; Isernia, T.; Palmeri, R.; Akinci, M.N.; Crocco, L. Physical insight unveils new imaging capabilities of orthogonality sampling method. IEEE Trans. Antennas Propag. 2020, 68, 4014–4021. [Google Scholar] [CrossRef]
- Huang, K.; Sølna, K.; Zhao, H. Generalized Foldy-Lax formulation. J. Comput. Phys. 2010, 229, 4544–4553. [Google Scholar] [CrossRef]
- Ammari, H.; Garapon, P.; Jouve, F.; Kang, H.; Lim, M.; Yu, S. A new optimal control approach for the reconstruction of extended inclusions. SIAM J. Control Optim. 2013, 51, 1372–1394. [Google Scholar] [CrossRef]
- Bergou, E.; Diouane, Y.; Kungurtsev, V. Convergence and complexity analysis of a Levenberg–Marquardt algorithm for inverse problems. J. Optim. Theory Appl. 2020, 185, 927–944. [Google Scholar] [CrossRef]
- Carpio, A.; Dimiduk, T.G.; Louër, F.L.; Rapún, M.L. When topological derivatives met regularized Gauss–Newton iterations in holographic 3D imaging. J. Comput. Phys. 2019, 388, 224–251. [Google Scholar] [CrossRef]
- Chen, X. Subspace-based optimization method for inverse scattering problems with an inhomogeneous background medium. Inverse Prob. 2010, 26, 074007. [Google Scholar] [CrossRef]
- Kress, R. Inverse scattering from an open arc. Math. Meth. Appl. Sci. 1995, 18, 267–293. [Google Scholar] [CrossRef]
- Liu, Z. A new scheme based on Born iterative method for solving inverse scattering problems with noise disturbance. IEEE Geosci. Remote Sens. Lett. 2019, 16, 1021–1025. [Google Scholar] [CrossRef]
- Palmeri, R.; Bevacqua, M.T.; Crocco, L.; Isernia, T.; Donato, L.D. Microwave imaging via distorted iterated virtual experiments. IEEE Trans. Antennas Propag. 2017, 65, 829–838. [Google Scholar] [CrossRef]
- Timonov, A.; Klibanov, M.V. A new iterative procedure for the numerical solution of coefficient inverse problems. Appl. Numer. Math. 2005, 55, 191–203. [Google Scholar] [CrossRef]
- Wick, T. Modified Newton methods for solving fully monolithic phase-field quasi-static brittle fracture propagation. Comput. Meth. Appl. Mech. Eng. 2017, 325, 577–611. [Google Scholar] [CrossRef]
- Park, W.K.; Lesselier, D. MUSIC-type imaging of a thin penetrable inclusion from its far-field multistatic response matrix. Inverse Prob. 2009, 25, 075002. [Google Scholar] [CrossRef]
- Park, W.K. Application of MUSIC algorithm in real-world microwave imaging of unknown anomalies from scattering matrix. Mech. Syst. Signal Proc. 2021, 153, 107501. [Google Scholar] [CrossRef]
- Ammari, H.; Iakovleva, E.; Lesselier, D.; Perrusson, G. MUSIC type electromagnetic imaging of a collection of small three-dimensional inclusions. SIAM J. Sci. Comput. 2007, 29, 674–709. [Google Scholar] [CrossRef]
- Shea, J.D.; Kosmas, P.; Hagness, S.C.; Veen, B.D.V. Three-dimensional microwave imaging of realistic numerical breast phantoms via a multiple-frequency inverse scattering technique. Med. Phys. 2010, 37, 4210–4226. [Google Scholar] [CrossRef]
- Song, R.; Chen, R.; Chen, X. Imaging three-dimensional anisotropic scatterers in multi-layered medium by MUSIC method with enhanced resolution. J. Opt. Soc. Am. A 2012, 29, 1900–1905. [Google Scholar] [CrossRef]
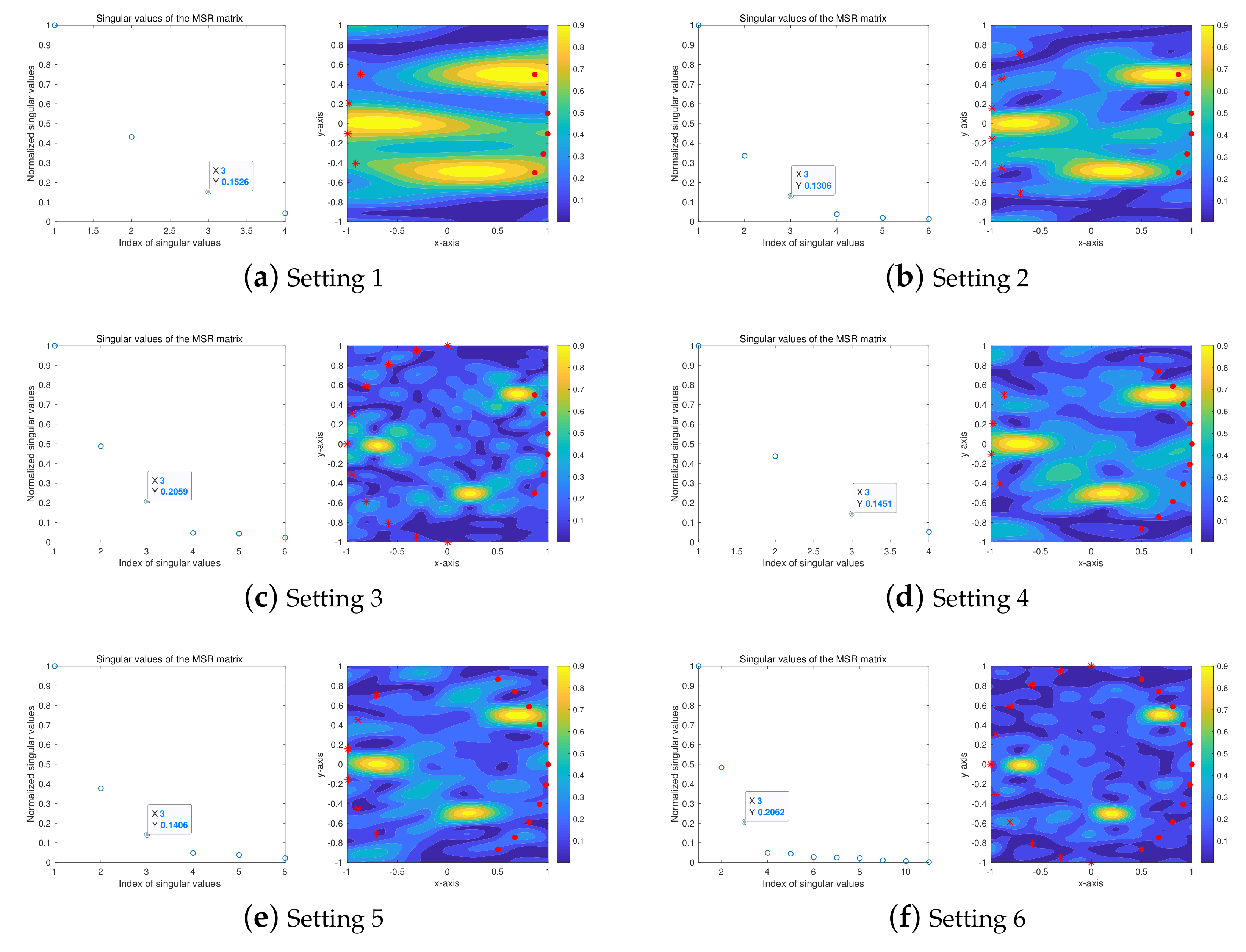
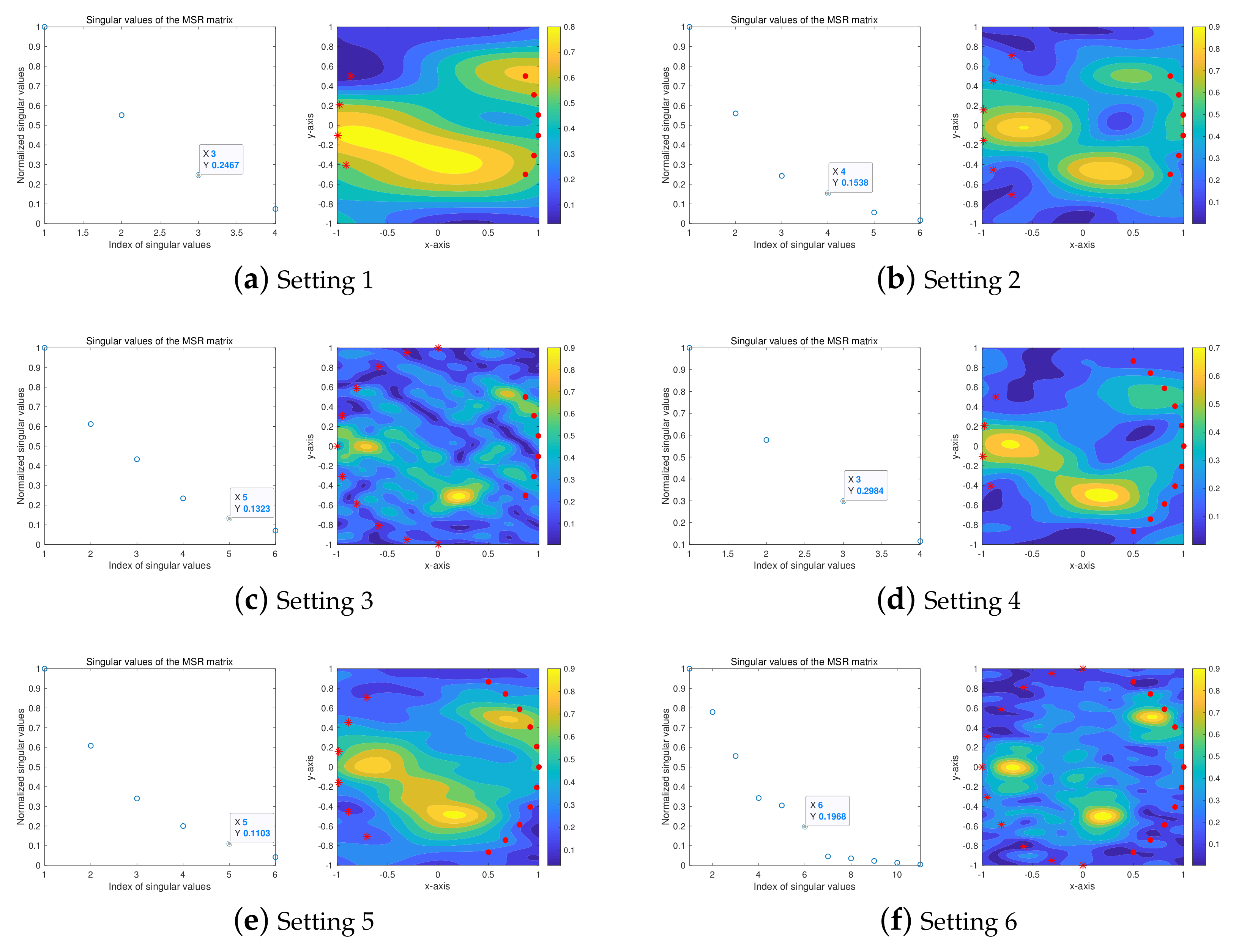


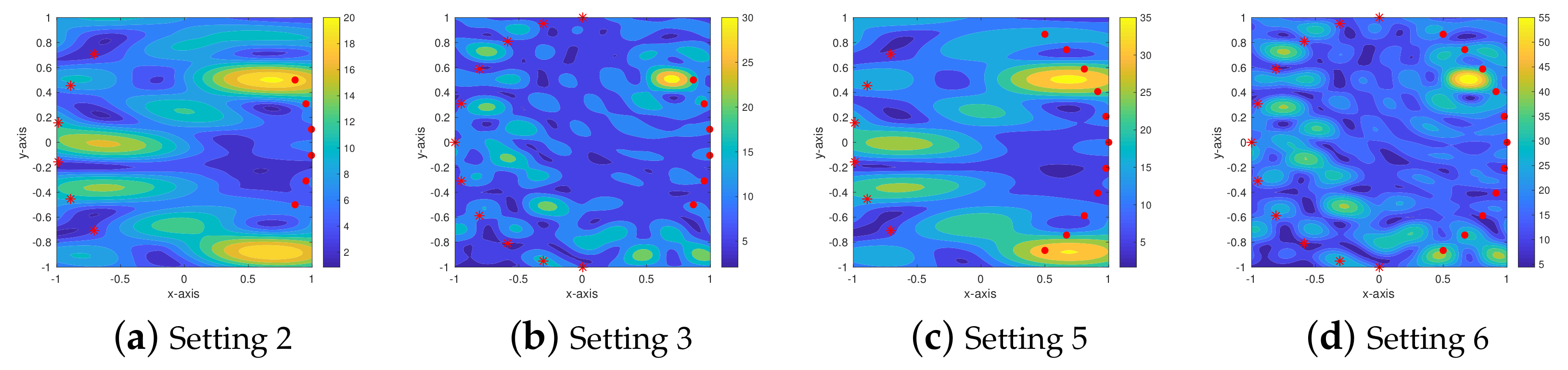
| Setting 1 | Setting 2 | Setting 3 | Setting 4 | Setting 5 | Setting 6 | |
|---|---|---|---|---|---|---|
| P | 4 | 6 | 11 | 4 | 6 | 11 |
| Q | 6 | 6 | 6 | 11 | 11 | 11 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Park, W.-K. Fast Localization of Small Inhomogeneities from Far-Field Pattern Data in the Limited-Aperture Inverse Scattering Problem. Mathematics 2021, 9, 2087. https://doi.org/10.3390/math9172087
Park W-K. Fast Localization of Small Inhomogeneities from Far-Field Pattern Data in the Limited-Aperture Inverse Scattering Problem. Mathematics. 2021; 9(17):2087. https://doi.org/10.3390/math9172087
Chicago/Turabian StylePark, Won-Kwang. 2021. "Fast Localization of Small Inhomogeneities from Far-Field Pattern Data in the Limited-Aperture Inverse Scattering Problem" Mathematics 9, no. 17: 2087. https://doi.org/10.3390/math9172087
APA StylePark, W.-K. (2021). Fast Localization of Small Inhomogeneities from Far-Field Pattern Data in the Limited-Aperture Inverse Scattering Problem. Mathematics, 9(17), 2087. https://doi.org/10.3390/math9172087





