Theoretical and Numerical Aspect of Fractional Differential Equations with Purely Integral Conditions
Abstract
:1. Introduction
2. Notions and Preliminaries
3. Theoretical Study
3.1. Position of the Problem
- Reformulation of the problem into a problem with homogeneous conditions.
- The uniqueness of the solution to the problem using the a priori estimate method.
- The existence of the solution of the problem based on the density of the range of the operator generated by the abstract formulation of the problem.
3.2. Reformulation of the Problem
3.3. Energy Inequality Method and Consequences
3.4. Existence of the Solution
4. Numerical Study
4.1. Finite Difference Method
4.1.1. Discretization of the Problem
4.1.2. General Case
4.2. Stability and Convergence
4.2.1. Stability
4.2.2. Convergence
4.3. Applications
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A
| 5 × 10 | 6 × 10 | 6 × 10 | 7 × 10 | 8 × 10 | 9 × 10 | 10 | 10 | 10 | 10 | 10 |
| 5 × 10 | 6 × 10 | 7 × 10 | 7 × 10 | 8 × 10 | 9 × 10 | 10 | 10 | 10 | 10 | 10 |
| 5 × 10 | 6 × 10 | 7 × 10 | 7 × 10 | 8 × 10 | 9 × 10 | 10 | 10 | 10 | 10 | 10 |
| 6 × 10 | 6 × 10 | 7 × 10 | 7 × 10 | 8 × 10 | 9 × 10 | 10 | 10 | 10 | 10 | 10 |
| 6 × 10 | 6 × 10 | 7 × 10 | 7 × 10 | 8 × 10 | 9 × 10 | 10 | 10 | 10 | 10 | 10 |
| 6 × 10 | 6 × 10 | 7 × 10 | 7 × 10 | 8 × 10 | 9 × 10 | 10 | 10 | 10 | 10 | 10 |
| 6 × 10 | 6 × 10 | 7 × 10 | 8 × 10 | 8 × 10 | 9 × 10 | 10 | 10 | 10 | 10 | 10 |
| 6 × 10 | 6 × 10 | 7 × 10 | 8 × 10 | 8 × 10 | 9 × 10 | 10 | 10 | 10 | 10 | 10 |
| 6 × 10 | 6 × 10 | 7 × 10 | 8 × 10 | 8 × 10 | 9 × 10 | 10 | 10 | 10 | 10 | 10 |
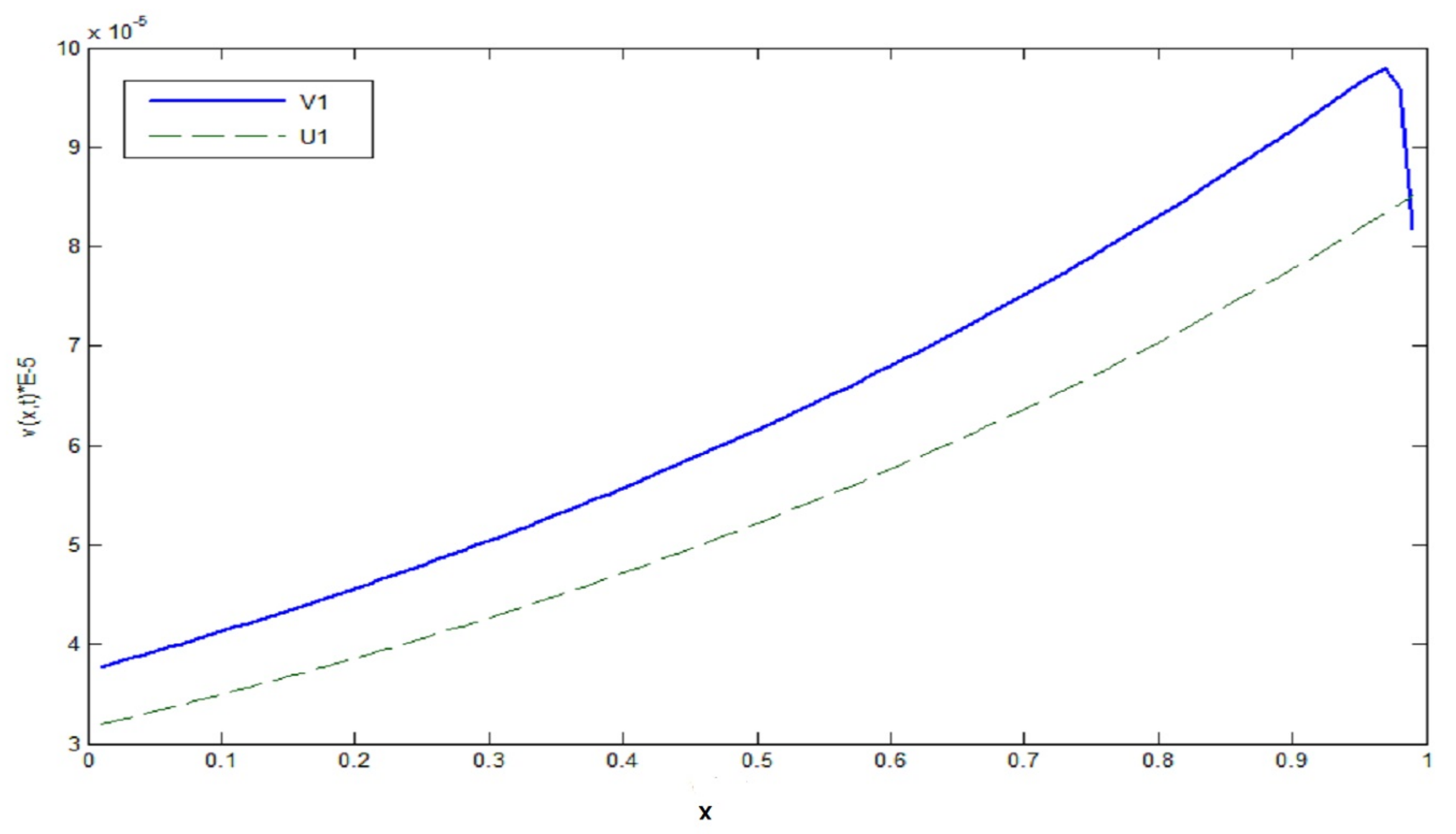
| 5 × 10 | 6 × 10 | 6 × 10 | 7 × 10 | 8 × 10 | 8 × 10 | 9 × 10 | 10 | 10 | 10 | 10 |
| 5 × 10 | 6 × 10 | 6 × 10 | 7 × 10 | 8 × 10 | 9 × 10 | 9 × 10 | 10 | 10 | 10 | 10 |
| 5 × 10 | 6 × 10 | 6 × 10 | 7 × 10 | 8 × 10 | 9 × 10 | 9 × 10 | 10 | 10 | 10 | 10 |
| 5 × 10 | 6 × 10 | 7 × 10 | 7 × 10 | 8 × 10 | 9 × 10 | 10 | 10 | 10 | 10 | 10 |
| 5 × 10 | 6 × 10 | 7 × 10 | 7 × 10 | 8 × 10 | 9 × 10 | 10 | 10 | 10 | 10 | 10 |
| 5 × 10 | 6 × 10 | 7 × 10 | 7 × 10 | 8 × 10 | 9 × 10 | 10 | 10 | 10 | 10 | 10 |
| 6 × 10 | 6 × 10 | 7 × 10 | 7 × 10 | 8 × 10 | 9 × 10 | 10 | 10 | 10 | 10 | 10 |
| 6 × 10 | 6 × 10 | 7 × 10 | 7 × 10 | 8 × 10 | 9 × 10 | 10 | 10 | 10 | 10 | 10 |
| 6 × 10 | 6 × 10 | 7 × 10 | 8 × 10 | 8 × 10 | 9 × 10 | 10 | 10 | 10 | 10 | 10 |
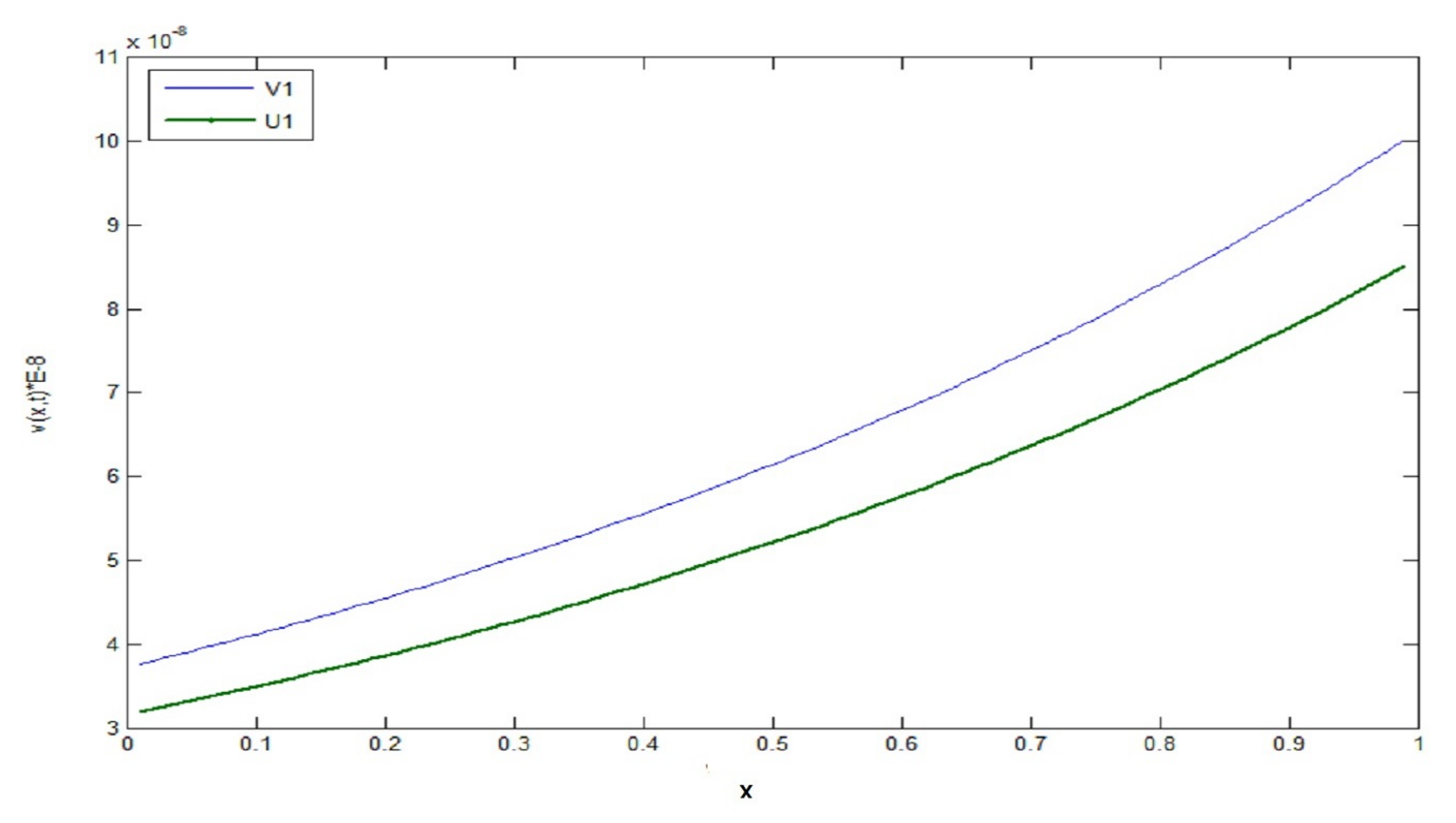
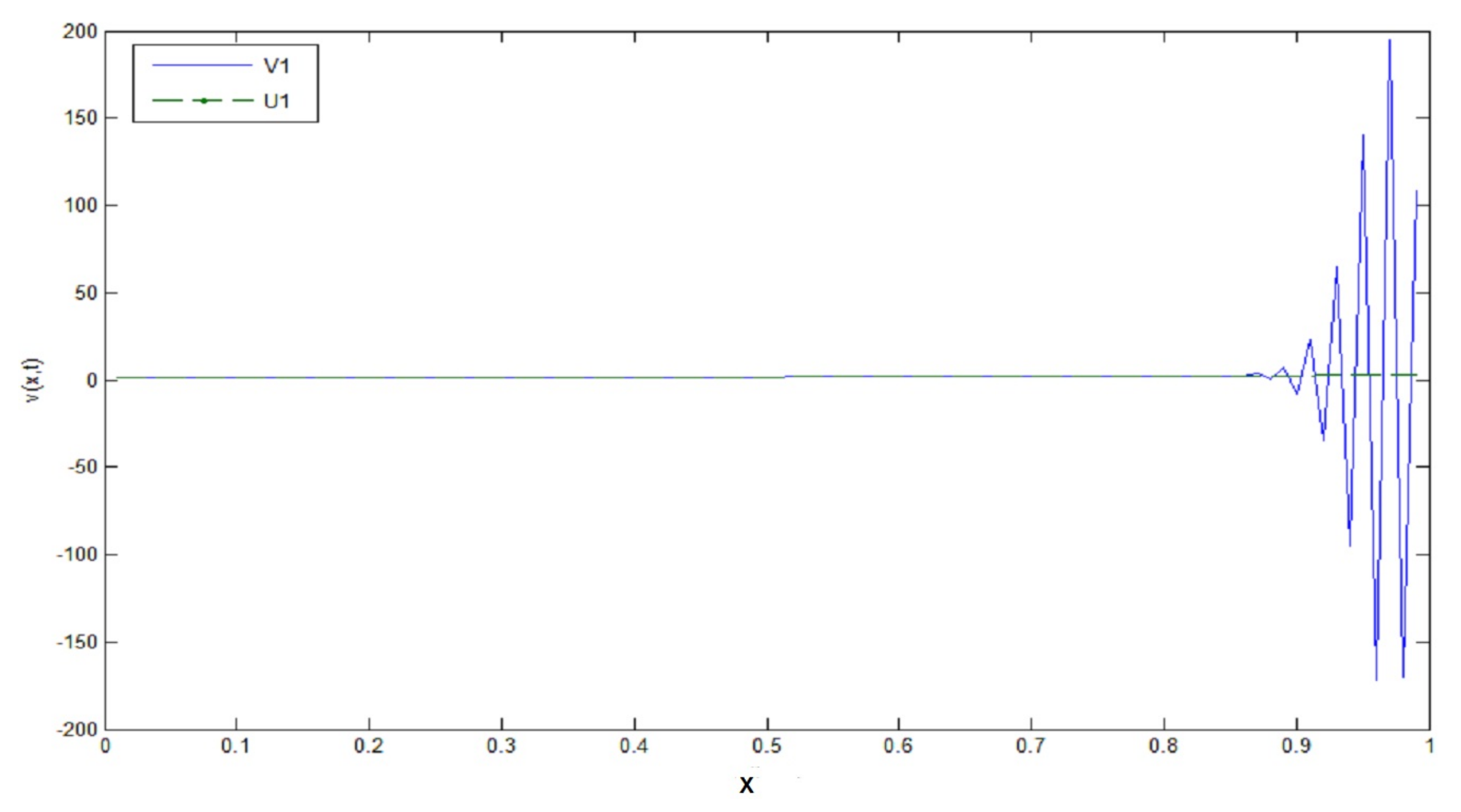

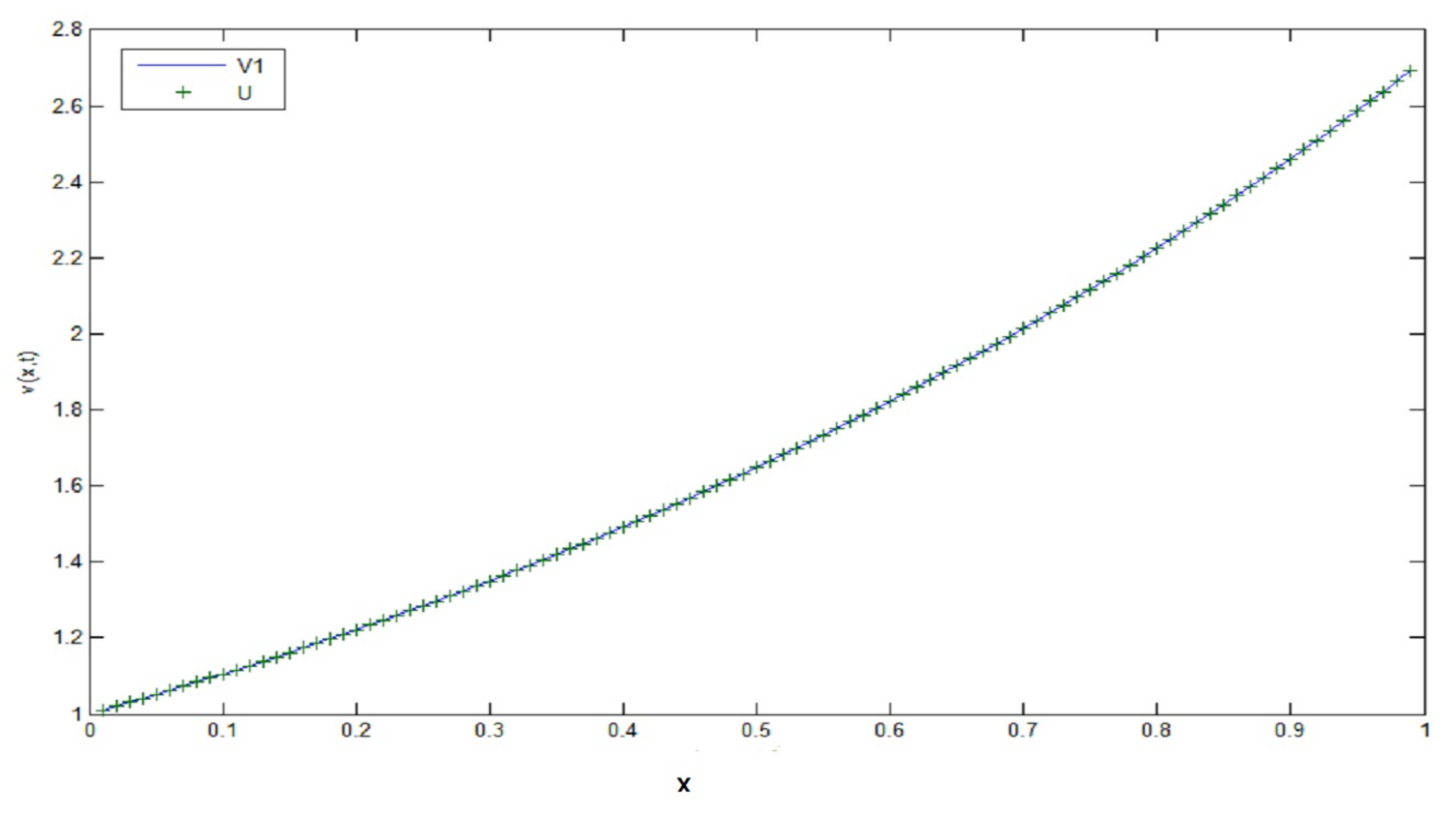
References
- Mainardi, F. Fractional diffusive waves in viscoelastic solids. Nonlinear Waves Solids 1995, 137, 93–97. [Google Scholar]
- Podlubny, I. Fractional Differential Equations; Academic Press: San Diego, CA, USA, 1999. [Google Scholar]
- He, J.H. Approximate analytical solution for seepage flow with fractional derivatives in porous media. Comput. Methods Appl. Mech. Eng. 1998, 167, 57–68. [Google Scholar] [CrossRef]
- Kilbas, A.A.; Srivastava, H.M.; Trujillo, J.J. Theory and Applications of Fractional Differential Equations; Elsevier: Amsterdam, The Netherlands, 2006. [Google Scholar]
- Alikhanov, A.A. Boundary value problems for the di usion equation of the variable order in differential and difference settings. Appl. Math. Comput. 2012, 219, 3938–3946. [Google Scholar]
- Li, X.J.; Xu, C.J. Existence and uniqueness of the weak solution of the space-time fractional diffusion equation and a spectral method approximation. Commun. Comput. Phys. 2010, 8, 1016–1051. [Google Scholar]
- Smit, W.; de Vries, H. Rheological models containing fractional derivatives. Rheol. Acta 1970, 9, 525–534. [Google Scholar] [CrossRef]
- Monje, C.A.; Chen, Y.; Vinagre, B.M.; Xue, D.; Feliu, V. Fractional-Order Systems and Controls: Fundamentals and Applications; Springer: London, UK, 2010. [Google Scholar]
- Enacheanu, O. Fractal Modeling of Electrical Networks. Ph.D. Thesis, Joseph Fourier University, Grenoble, France, October 2008; pp. 47–53. [Google Scholar]
- Kaufmann, E.R.; Mboumi, E. Positive solutions of a boundary value problem for a nonlinear. fractional differential equation. Electron. J. Qual. Theory Differ. Equ. 2007, 3, 1–11. [Google Scholar] [CrossRef]
- Furati, K.M.; Tatar, N. An existence result for a nonlocal fractional differential problem. J. Fract. Calc. 2004, 26, 43–51. [Google Scholar]
- Mesloub, S. Existence and uniqueness results for a fractional two-times evolution problem with constraints of purely integral type. Math. Methods Appl. Sci. 2016, 39, 1558–1567. [Google Scholar] [CrossRef]
- Sosnovskii, L.A.; Komissarov, V.V.; Shcherbakov, S.S. A Method of Experimental Study of Friction in a Active System. J. Frict. Wear 2012, 33, 174–184. [Google Scholar] [CrossRef]
- Shcherbakov, S.S. State of volumetric damage of tribo-fatigue systeme. Strength Mater. 2013, 45, 171–178. [Google Scholar] [CrossRef]
- He, C.; Liu, J.; Wang, W.; Liu, Q. The Tribo-Fatigue Damage Transition and Mapping for Wheel Material under Rolling-Sliding Contact Condition. Materials 2019, 12, 4138. [Google Scholar] [CrossRef] [Green Version]
- Day, W.A. A decreasing property of solutions of parabolic equations with applications to thermoelasticity. Quart. Appl. Math. 1983, 40, 319–330. [Google Scholar] [CrossRef] [Green Version]
- Anguraj, A.; Karthikeyan, P. Existence of solutions for fractional semilinear evolution boundary value problem. Commun. Appl. Anal. 2010, 14, 505–514. [Google Scholar]
- Jesus, M.-V.; Ahcene, M. Existence, uniqueness and numerical solution of a fractional PDE with integral conditions. Nonlinear Anal. Model. Control 2019, 24, 368–386. [Google Scholar]
- Benchohra, M.; Graef, J.R.; Hamani, S. Existence results for boundary value problems with nonlinear fractional differential equations. Appl. Anal. 2008, 87, 851–863. [Google Scholar] [CrossRef]
- Daftardar-Gejji, V.; Jafari, H. Boundary value problems for fractional diffusion-wave equation. Aust. J. Math. Anal. Appl. 2006, 3, 1–8. [Google Scholar]
- Alikhanov, A.A. On the stability and convergence of nonlocal difference schemes. Differ. Equ. 2010, 46, 949–961. [Google Scholar] [CrossRef]
- Alikhanov, A.A. A new difference scheme for the time fractional diffusion equation. J. Comput. Phys. 2015, 280, 424–438. [Google Scholar] [CrossRef] [Green Version]
- Alikhanov, A.A. Stability and convergence of difference schemes for boundary value problems for the fractional-order diffusion equation. Comput. Math. Math. Phys. 2016, 56, 561–575. [Google Scholar] [CrossRef]
- Meerschaert, M.M.; Tadjeran, C. Finite difference approximations for fractional advection dispersion flow equations. J. Comput. Appl. Math. 2004, 172, 65–77. [Google Scholar] [CrossRef] [Green Version]
- Shen, S.; Liu, F. Error analysis of an explicit finite difference approximation for the space fractional diffusion. Anziam J. 2005, 46, C871–C887. [Google Scholar] [CrossRef] [Green Version]
- El-Nabulsi, R.A. Finite Two-Point Space without Quantization on Noncommutative Space from a Generalized Fractional Integral Operator. Complex Anal. Oper. Theory 2018, 12, 1609–1616. [Google Scholar] [CrossRef]
- El-Nabulsi, R.A. The fractional Boltzmann transport equation. Comput. Math. Appl. 2011, 62, 1568–1575. [Google Scholar] [CrossRef] [Green Version]
- El-Nabulsi, R.A. Nonlocal-in-time kinetic energy in nonconservative fractional systems, disordered dynamics, jerk and snap and oscillatory motions in the rotating fluid tube. Int. J. Non-Linear Mech. 2017, 93, 65. [Google Scholar] [CrossRef]
- Yan, B.H.; Yu, L.; Yang, Y.H. Study of oscillating flow in rolling motion with the fractional derivative Maxwell model. Prog. Nucl. Energy 2011, 53, 132–138. [Google Scholar] [CrossRef]
- Li, X.; Yang, X.; Zhang, Y. Error estimates of mixed finite element methods for timefractional Navier-Stokes equations. J. Sci. Comput. 2017, 70, 500–515. [Google Scholar] [CrossRef]
- Yildirim, A. Analytical approach to fractional partial differential equations in fluid mechanics by means of the homotopy perturbation method. Int. J. Numer. Methods Heat Fluid Flow 2010, 20, 186–200. [Google Scholar] [CrossRef]
- Zhou, Y.; Peng, L. On the time-fractional Navier–Stokes equations. Comput. Math. Appl. 2017, 73, 874–891. [Google Scholar] [CrossRef]
- Yu, B.; Jiang, X.Y.; Xu, H.Y. A novel compact numerical method for solving the two-dimensional non-linear fractional reaction-subdiffusion equation. Numer. Algorithms 2015, 68, 923–950. [Google Scholar] [CrossRef]
- Povstenko, Y. Linear Fractional Diffusion-Wave Equation for Scientists and Engineers; Springer: Berlin/Heidelberg, Germany, 2010. [Google Scholar]
- Oussaeif, T.-E.; Bouziani, A. Existence and uniqueness of solutions to parabolic fractional differential equations with integral conditions. Electron. J. Differ. Equ. 2014, 2014, 1–10. [Google Scholar]
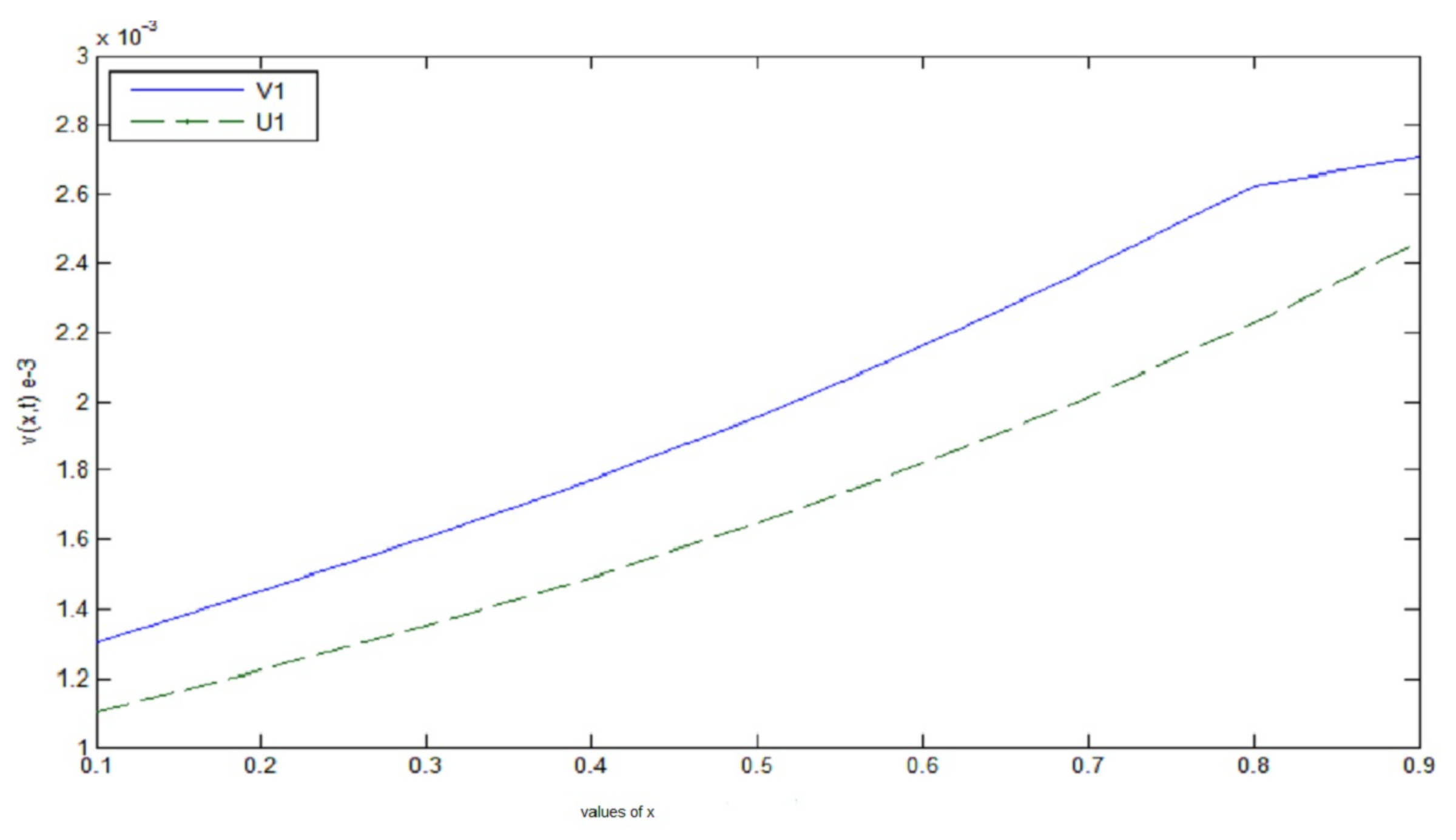
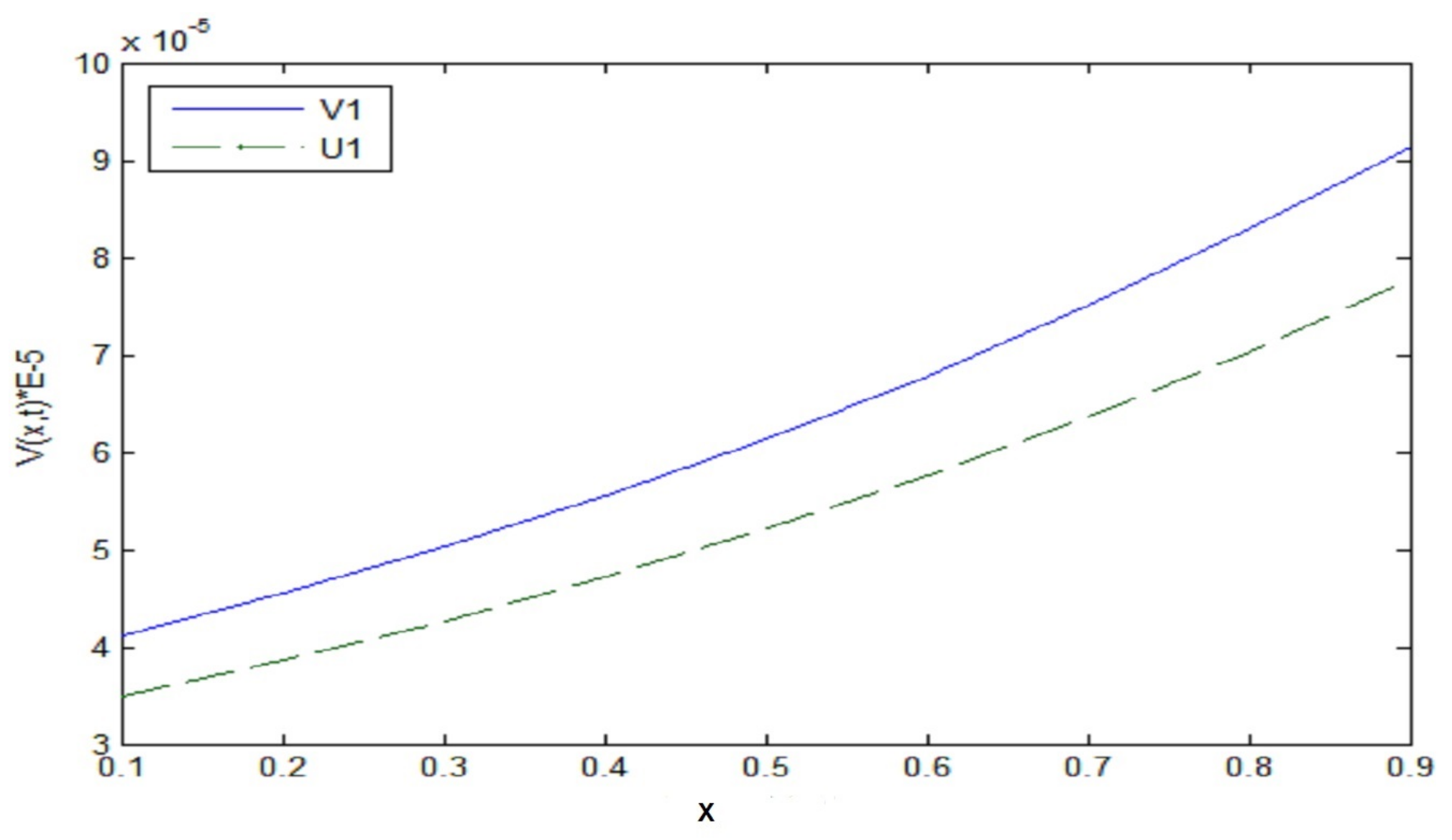
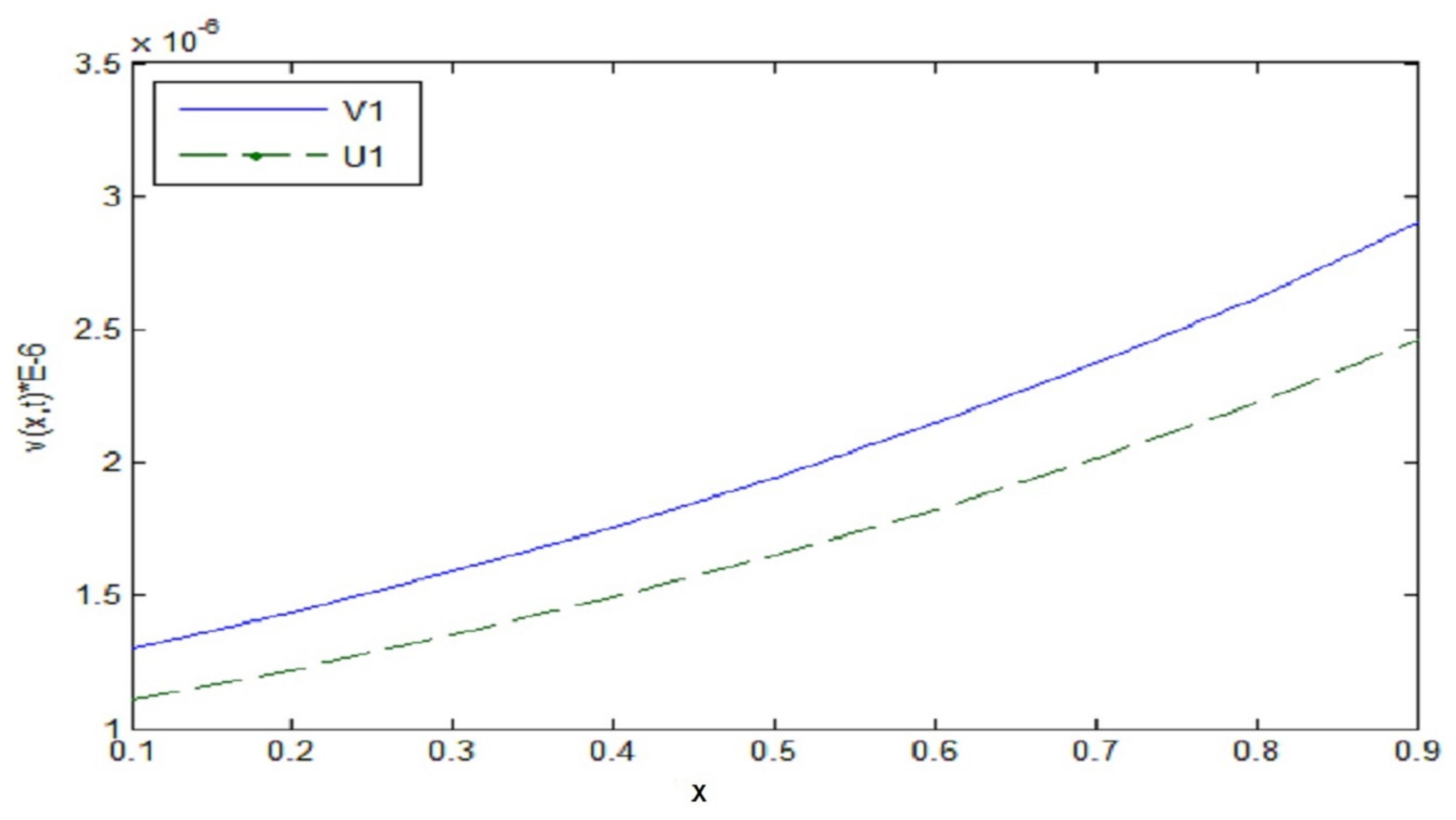
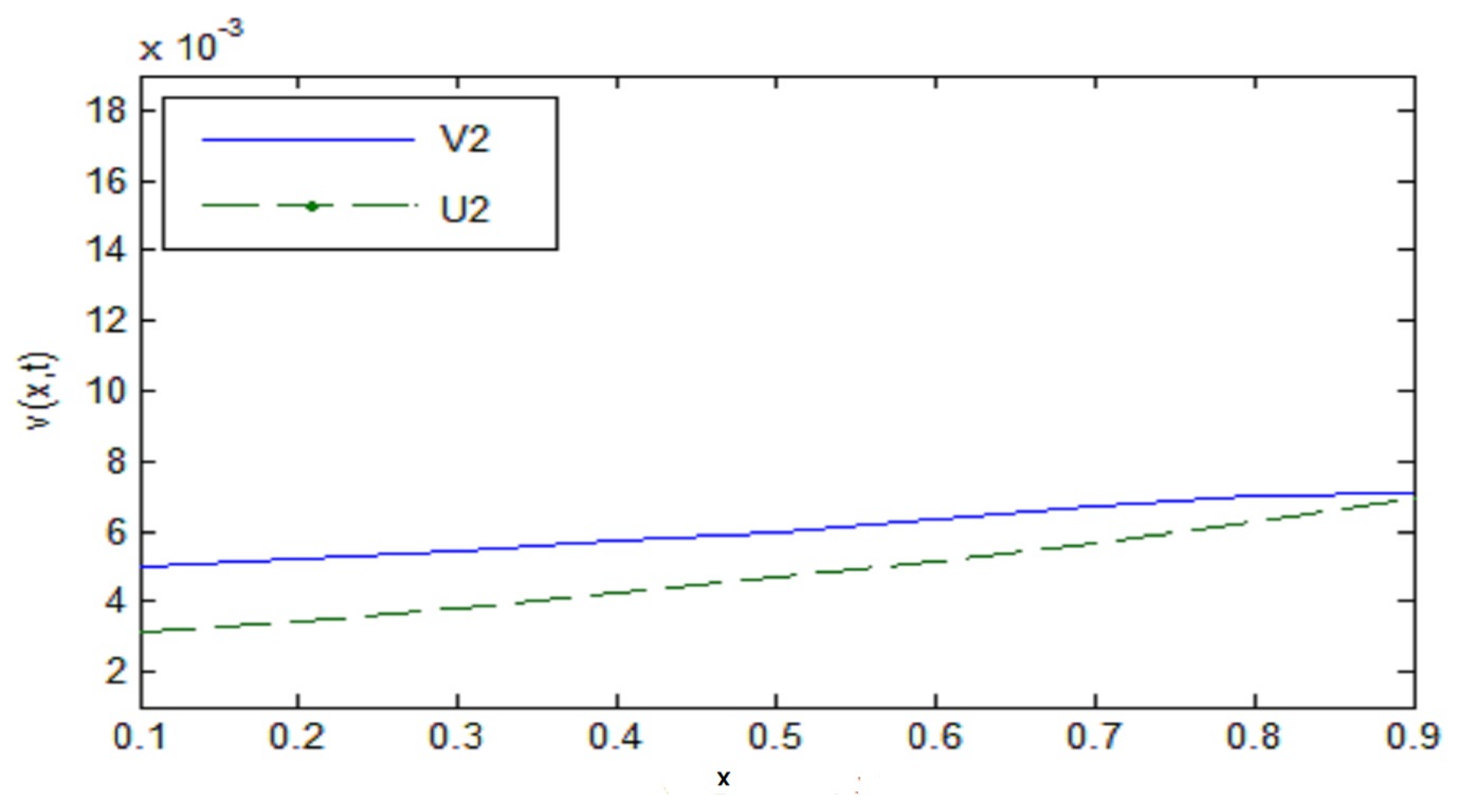
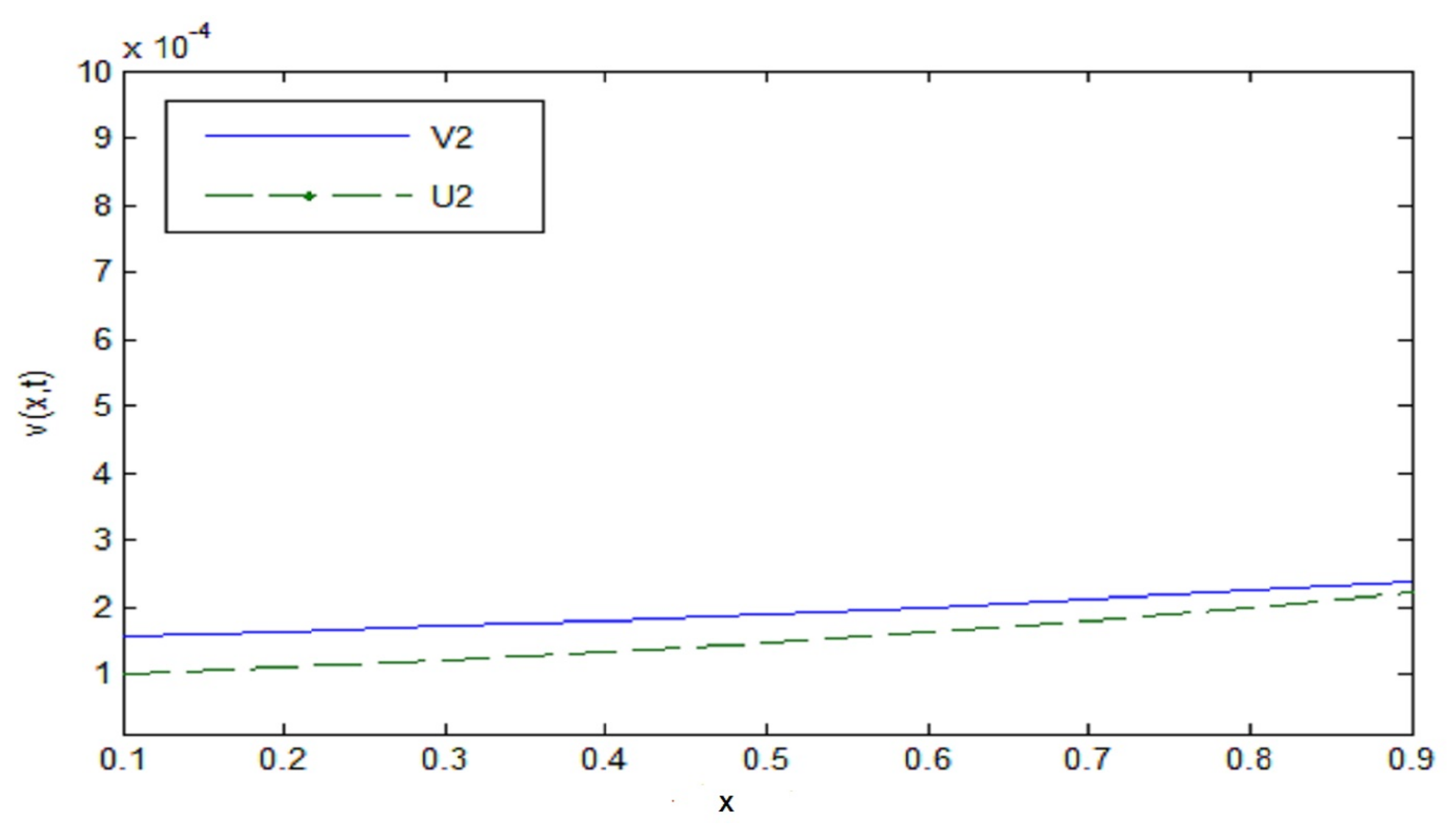
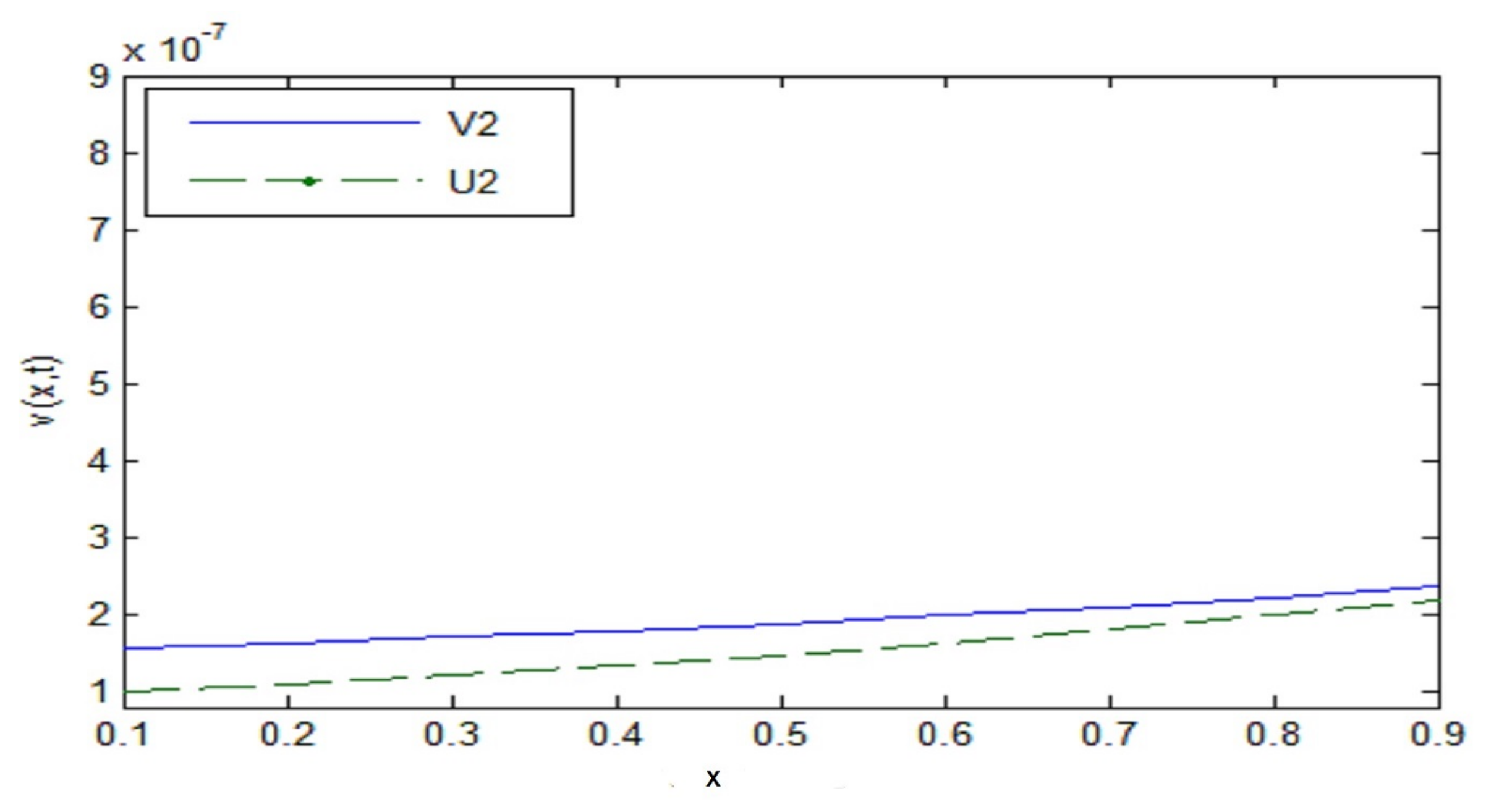
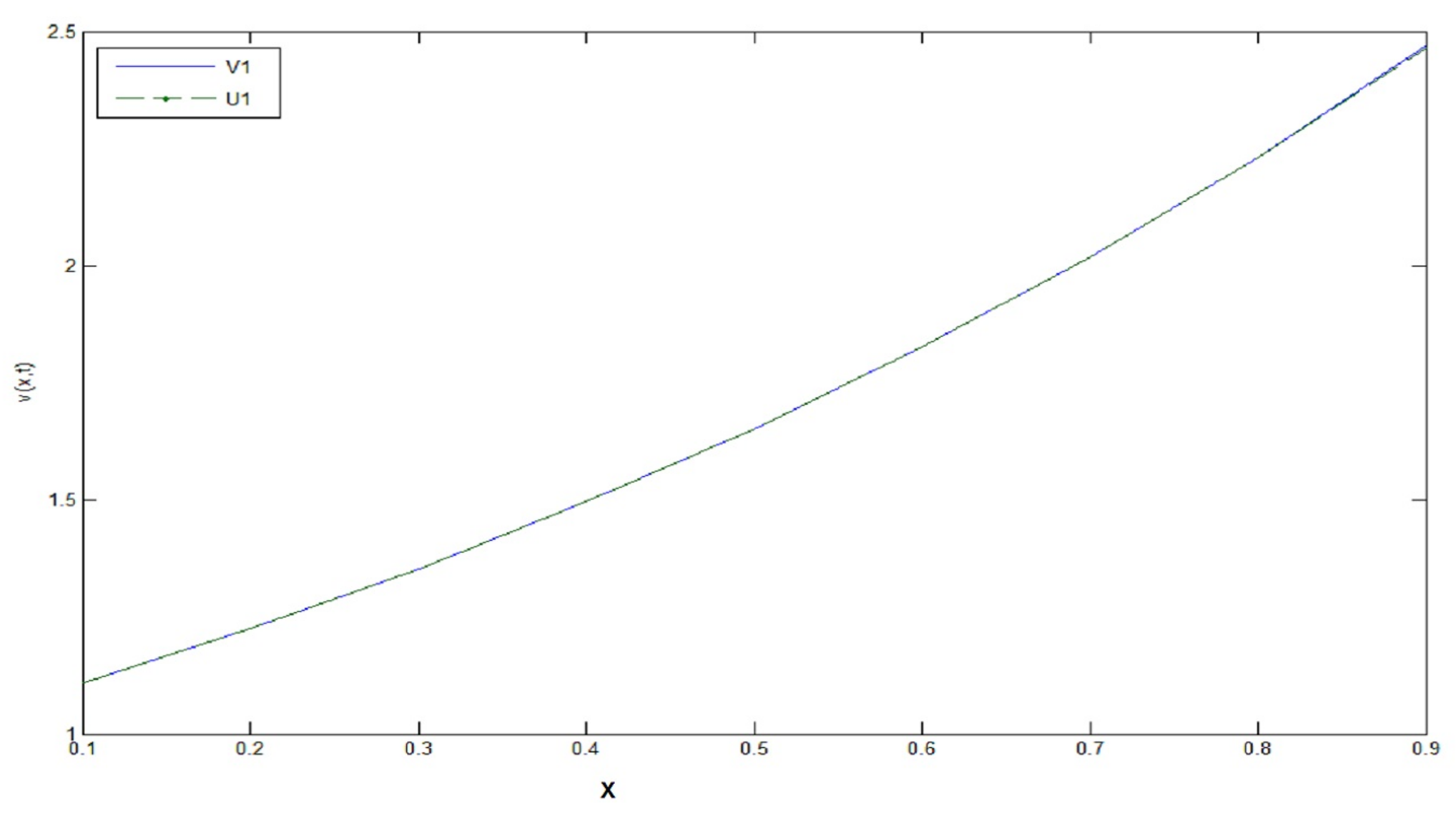
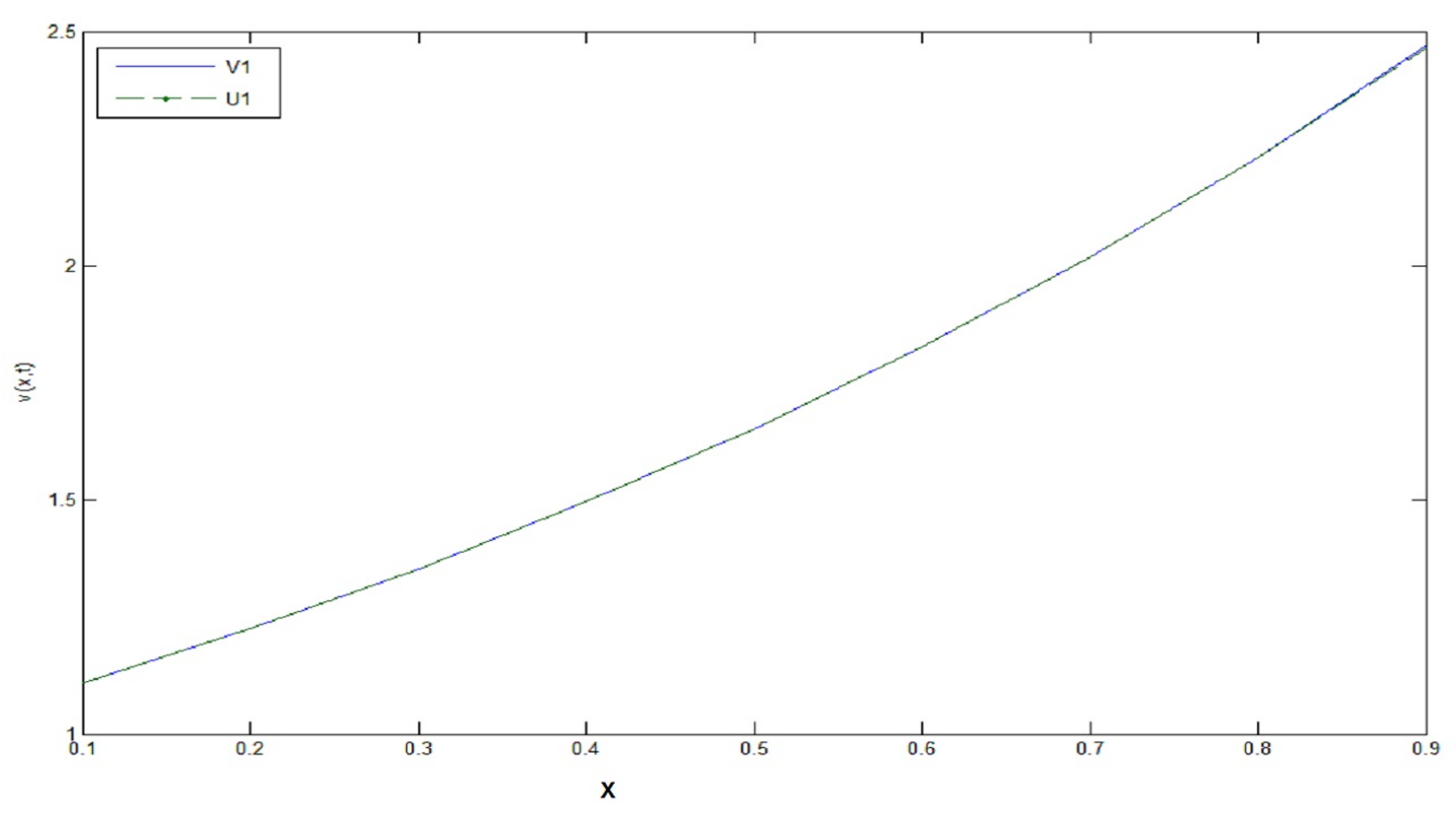

| Value of | ||||
|---|---|---|---|---|
| for | × | |||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Brahimi, S.; Merad, A.; Kılıçman, A. Theoretical and Numerical Aspect of Fractional Differential Equations with Purely Integral Conditions. Mathematics 2021, 9, 1987. https://doi.org/10.3390/math9161987
Brahimi S, Merad A, Kılıçman A. Theoretical and Numerical Aspect of Fractional Differential Equations with Purely Integral Conditions. Mathematics. 2021; 9(16):1987. https://doi.org/10.3390/math9161987
Chicago/Turabian StyleBrahimi, Saadoune, Ahcene Merad, and Adem Kılıçman. 2021. "Theoretical and Numerical Aspect of Fractional Differential Equations with Purely Integral Conditions" Mathematics 9, no. 16: 1987. https://doi.org/10.3390/math9161987
APA StyleBrahimi, S., Merad, A., & Kılıçman, A. (2021). Theoretical and Numerical Aspect of Fractional Differential Equations with Purely Integral Conditions. Mathematics, 9(16), 1987. https://doi.org/10.3390/math9161987







