Stable Optical Solitons for the Higher-Order Non-Kerr NLSE via the Modified Simple Equation Method
Abstract
:1. Introduction
2. Methodology
- Step 1:
- Define the wave variable , where and is the wave speed, to reduce the NLEE (2) into a nonlinear ordinary differential equation (NODE) for as:where , etc., denote the derivatives of v with respect to , and F is a polynomial in v and its total derivatives. Integrate Equation (3) as many times as is applicable;
- Step 2:
- The MSEM [36] expresses the solution of Equation (3) by making an ansatz for as:where the ’s are the parameters to be calculated and N is a positive integer that can be determined by considering the homogeneous balance between the highest-order derivative and the linear term of highest order in the resulting equation of Equation (3). is an unspecified function to be determined subsequently;
- Step 3:
- Step 4:
3. Mathematical Analysis
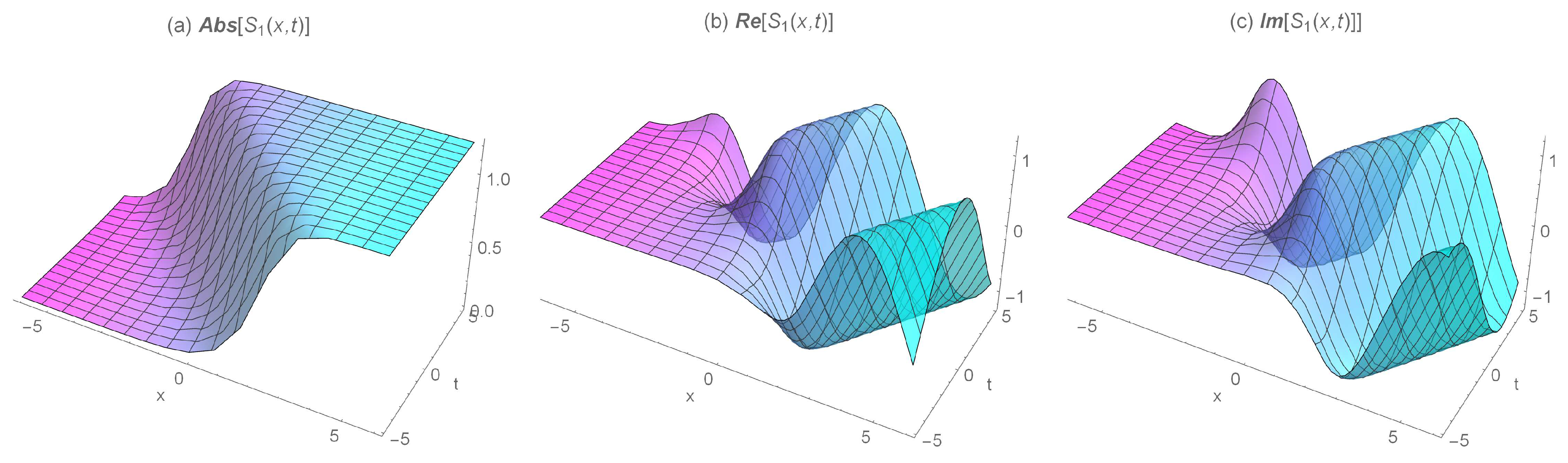
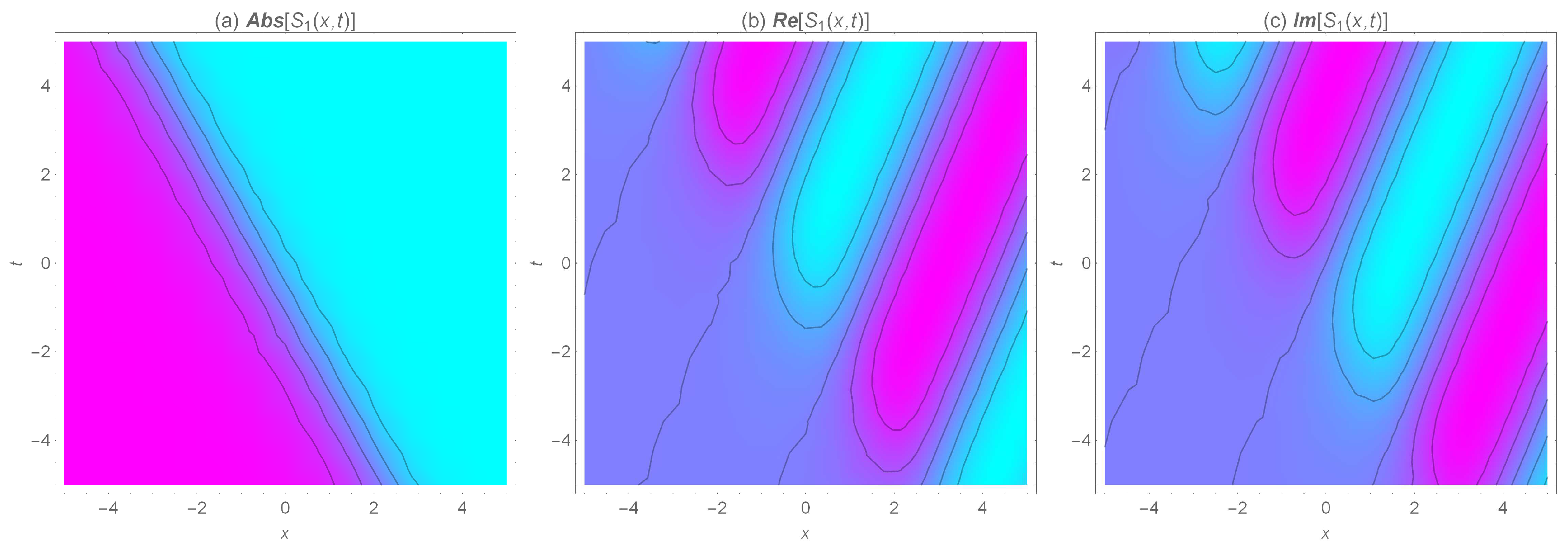
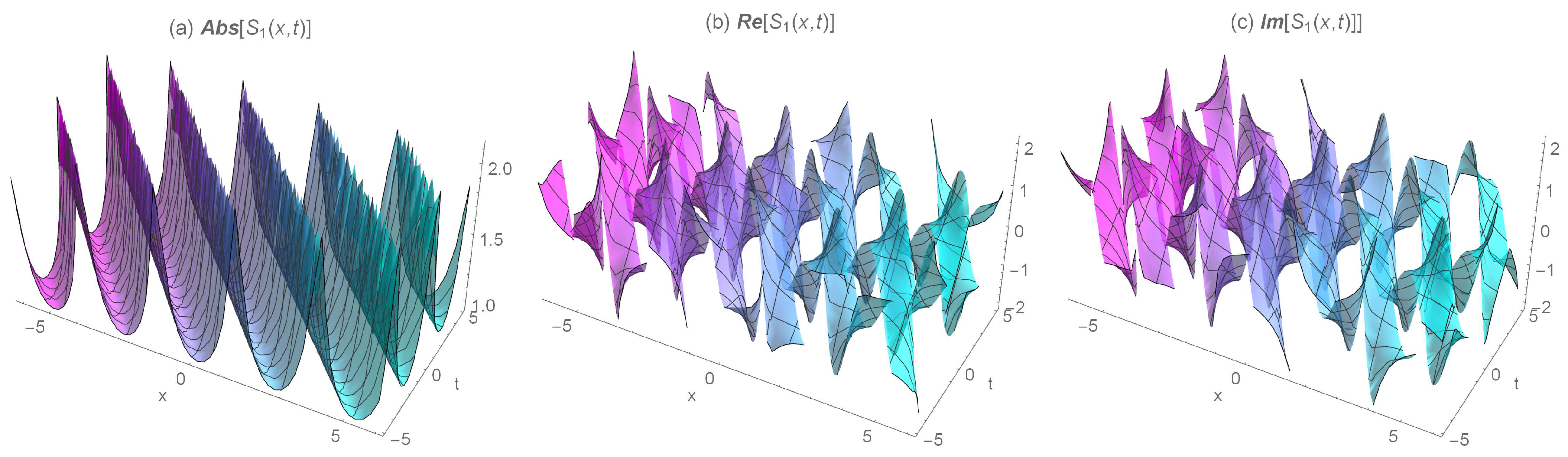
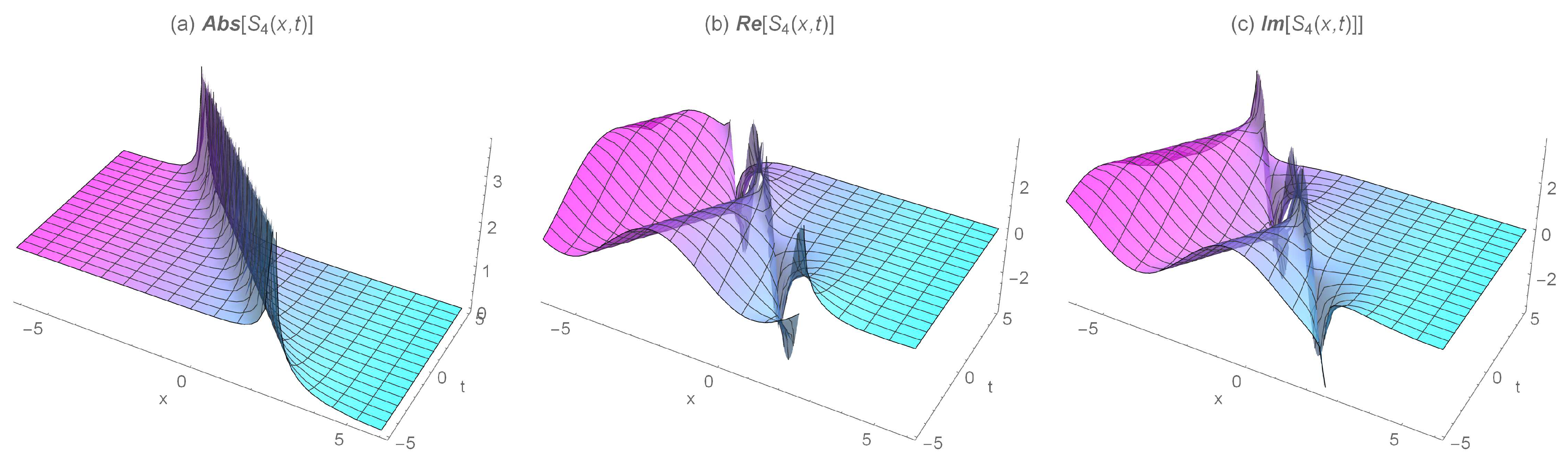
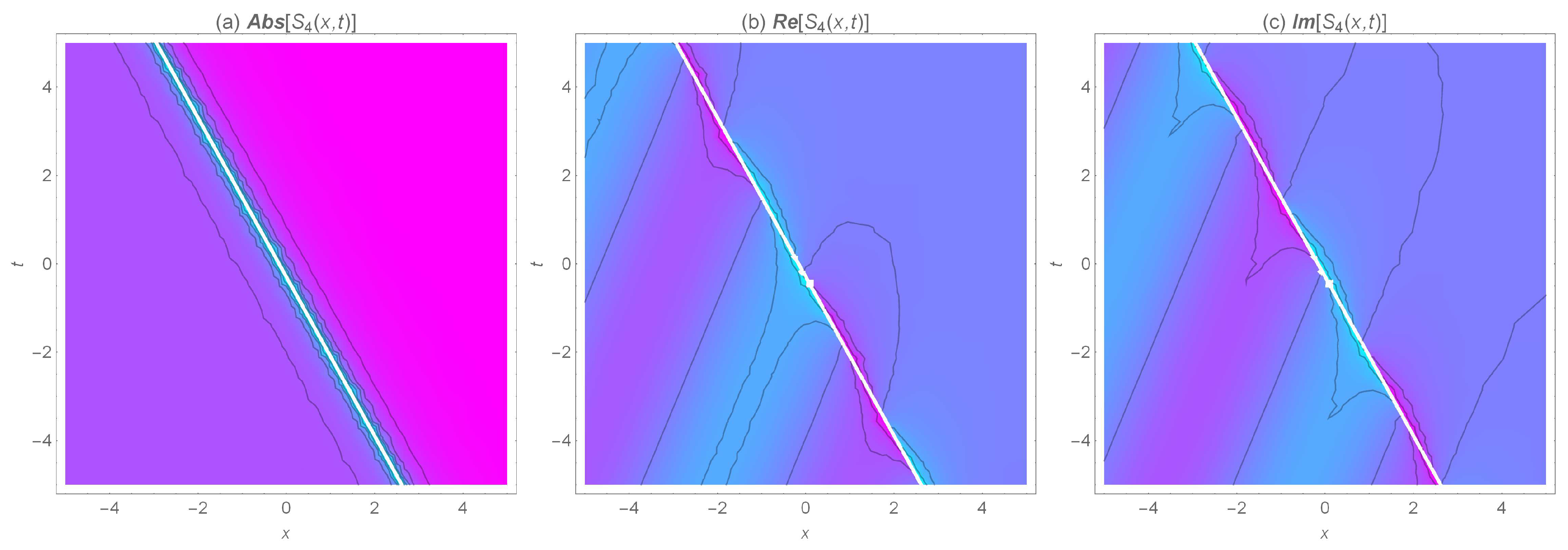
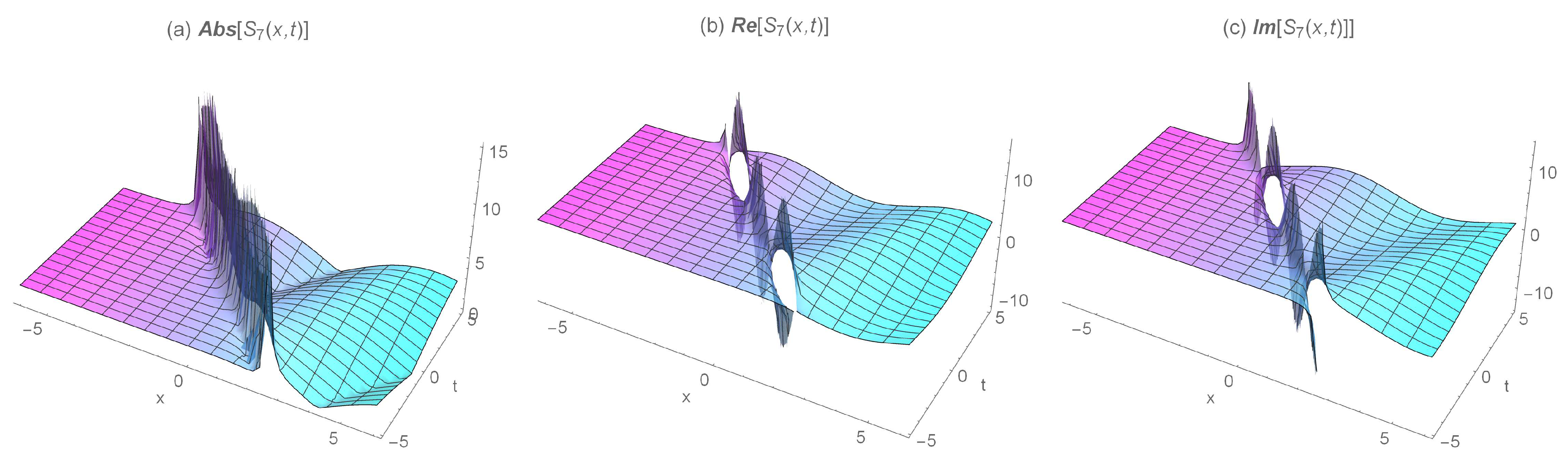
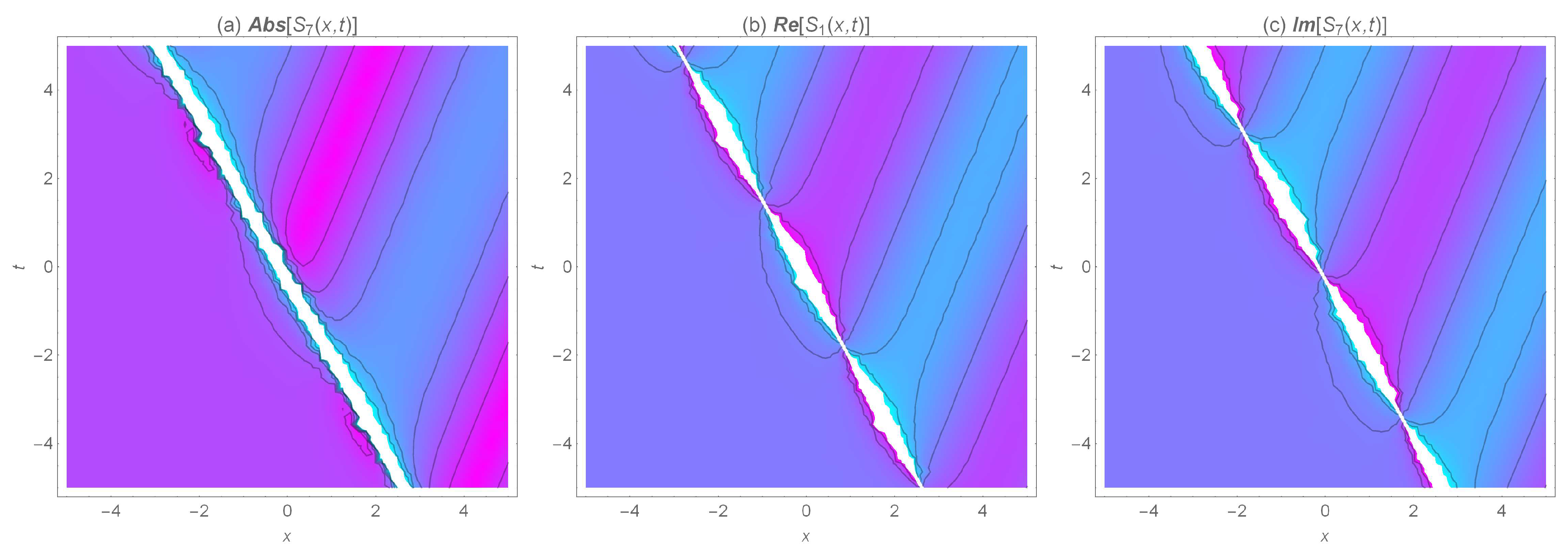
4. Optical Solitons
5. Discussion and Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Hasegawa, A.; Kodama, Y. Solitons in Optical Communications; Oxford University Press: New York, NY, USA, 1995. [Google Scholar]
- Agrawal, G.P. Nonlinear Fiber Optics, 5th ed.; Springer: New York, NY, USA, 2013. [Google Scholar]
- Arshad, M.; Seadawy, A.R.; Lu, D. Exact bright-dark solitary wave solutions of the higher-order cubic-quintic nonlinear Schrodinger equation and its stability. Optik 2017, 128, 40–49. [Google Scholar] [CrossRef]
- Arshad, M.; Seadawy, A.R.; Lu, D.; Wang, J. Optical soliton solutions of unstable nonlinear Schrödinger dynamical equation and stability analysis with applications. Optik 2018, 157, 597–605. [Google Scholar] [CrossRef]
- Az-Zo’bi, E.A. Modified Laplace decomposition method. World Appl. Sci. J. 2012, 18, 1481–1486. [Google Scholar]
- Az-Zo’bi, E.A. An Approximate Analytic Solution for Isentropic Flow by An Inviscid Gas Equations. Arch. Mech. 2014, 66, 203–212. [Google Scholar]
- Pellegrino, E.; Pezza, L.; Pitolli, F. A collocation method in spline spaces for the solution of linear fractional dynamical systems. Math. Comput. Simul. 2020, 176, 266–278. [Google Scholar] [CrossRef] [Green Version]
- Goswami, A.; Rathore, S.; Singh, J.; Kumar, D. Analytical study of fractional nonlinear Schrödinger equation with harmonic oscillator. Discret. Contin. Dyn. Syst. S 2021. [Google Scholar] [CrossRef]
- Arshad, S.; Siddiqui, A.M.; Sohail, A.; Maqbool, K.; Li, Z.-W. Comparison of optimal homotopy analysis method and fractional homotopy analysis transform method for the dynamical analysis of fractional order optical solitons. Adv. Mech. Eng. 2021, 9, 1687814017692946. [Google Scholar] [CrossRef] [Green Version]
- Az-Zo’bi, E.A.; Al Dawoud, K.; Marashdeh, M.F. Numeric-analytic solutions of mixed-type systems of balance laws. Appl. Math. Comput. 2015, 265, 133–143. [Google Scholar] [CrossRef]
- Az-Zo’bi, E.A.; Al-Amr, M.O.; Yıldırım, A.; AlZoubi, W.A. Revised reduced differential transform method using Adomian’s polynomials with convergence analysis. Math. Eng. Sci. Aerosp. 2020, 11, 827–840. [Google Scholar]
- Akinyemi, L.; Iyiola, O.S. A reliable technique to study nonlinear time-fractional coupled Korteweg-de Vries equations. Adv. Differ. Equ. 2020, 2020, 169. [Google Scholar] [CrossRef] [Green Version]
- Rani, M.; Bhatti, H.S.; Singh, V. Exact solitary wave solution for higher order nonlinear Schrodinger equation using He’s variational iteration method. Opt. Eng. 2017, 56, 116103. [Google Scholar] [CrossRef]
- Akgül, A.; Bonyah, E. Reproducing kernel Hilbert space method for the solutions of generalized Kuramoto–Sivashinsky equation. J. Taibah Univ. Sci. 2019, 13, 661–690. [Google Scholar] [CrossRef] [Green Version]
- Akinyemi, L.; Iyiola, O.S. Exact and approximate solutions of time-fractional models arising from physics via Shehu transform. Math. Meth. Appl. Sci. 2020, 43, 7442–7464. [Google Scholar] [CrossRef]
- Az-Zo’bi, E.A. A reliable analytic study for higher-dimensional telegraph equation. J. Math. Comput. Sci. 2018, 18, 423–429. [Google Scholar] [CrossRef]
- Senol, M.; Iyiola, O.S.; Daei Kasmaei, H.; Akinyemi, L. Efficient analytical techniques for solving time-fractional nonlinear coupled Jaulent-Miodek system with energy-dependent Schrödinger potential. Adv. Differ. Equ. 2019, 2019, 1–21. [Google Scholar] [CrossRef]
- Kudryashov, N.A. One method for finding exact solutions of nonlinear differential equations. Commun. Nonlinear Sci. Numer. Simul. 2012, 17, 2248–2253. [Google Scholar] [CrossRef] [Green Version]
- Kudryashov, N.A. Mathematical model of propagation pulse in optical fiber with power nonlinearities. Optik 2020, 212, 164750. [Google Scholar] [CrossRef]
- Kudryashov, N.A. Method for finding highly dispersive optical solitons of nonlinear differential equations. Optik 2020, 206, 163550. [Google Scholar] [CrossRef]
- Az-Zo’bi, E.A.; Alzoubi, W.A.; Akinyemi, L.; Şenol, M.; Masaedeh, B.S. A variety of wave amplitudes for the conformable fractional (2 + 1)-dimensional Ito equation. Mod. Phys. Lett. B 2021, 35, 2150254. [Google Scholar] [CrossRef]
- Salas, A.H.; Martinez, H.L.J.; Ocampo, R.D.L. New Solutions for the Generalized BBM Equation in terms of Jacobi and Weierstrass Elliptic Functions. Abstr. Appl. Anal. 2021, 2021, 5513266. [Google Scholar] [CrossRef]
- Rezazadeh, H.; Adel, W.; Eslami, M.; Tariq, K.U.; Mirhosseini-Alizamini, S.M.; Bekir, A.; Chu, Y.-M. On the optical solutions to nonlinear Schrödinger equation with second-order spatiotemporal dispersion. Open Phys. 2021, 19, 111–118. [Google Scholar] [CrossRef]
- Jia, T.-T.; Chai, Y.-Z.; Hao, H.-Q. Multi-soliton solutions and Breathers for the generalized coupled nonlinear Hirota equations via the Hirota method. Superlattices Microstruct. 2017, 105, 172–182. [Google Scholar] [CrossRef]
- Akinyemi, L.; Senol, M.; Iyiola, O.S. Exact solutions of the generalized multidimensional mathematical physics models via sub-equation method. Math. Comput. Simul. 2021, 182, 211–233. [Google Scholar] [CrossRef]
- Boudoue-Hubert, M.; Nestor, S.; Douvagai; Betchewe, G.; Biswas, A.; Khan, S.; Belic, M. Dispersive solitons in optical metamaterials having parabolic form of nonlinearity. Optik 2018, 179, 1009–1018. [Google Scholar] [CrossRef]
- Akinyemi, L.; Şenol, M.; Rezazadeh, H.; Ahmad, H.; Wang, H. Abundant optical soliton solutions for an integrable (2+1)-dimensional nonlinear conformable Schrödinger system. Results Phys. 2021, 104177. [Google Scholar] [CrossRef]
- Şenol, M. New analytical solutions of fractional symmetric regularized-long-wave equation. Rev. Mex. Fis. 2020, 66, 297–307. [Google Scholar] [CrossRef]
- Ekici, M.; Sonmezoglu, A. Optical solitons with Biswas-Arshed equation by the extended trial function method. Optik 2019, 177, 13–20. [Google Scholar] [CrossRef]
- Ghanbari, B.; Inc, M. A new generalized exponential rational function method to find exact special solutions for the resonance nonlinear Schrödinger equation. Eur. Phys. J. Plus 2018, 133, 142. [Google Scholar] [CrossRef]
- Tikhov, S.V.; Valovik, D.V. Maxwell’s equations with arbitrary self-action nonlinearity in a waveguiding theory: Guided modes and asymptotic of eigenvalues. J. Math. Anal. Appl. 2019, 479, 1138–1157. [Google Scholar] [CrossRef]
- Valovik, D.V. On the existence of infinitely many nonperturbative solutions in a transmission eigenvalue problem for nonlinear helmholtz equation with polynomial nonlinearity. Appl. Math. Model. 2018, 53, 296–309. [Google Scholar] [CrossRef]
- Zahran, E.H.M.; Khater, M.M.A. Modified extended tanh-function method and its applications to the Bogoyavlenskii equation. Appl. Math. Model. 2016, 40, 1769–1775. [Google Scholar] [CrossRef]
- Kudryashov, N.A. Simplest equation method to look for exact solutions of nonlinear differential equations. Chaos Solitons Fractals 2005, 24, 1217–1231. [Google Scholar] [CrossRef] [Green Version]
- Kudryashov, N.A. Exact solitary waves of the Fisher equation. Phys. Lett. A 2005, 342, 99–106. [Google Scholar] [CrossRef]
- Jawad, A.J.; Petkovic, M.D.; Biswas, A. Modified simple equation method for nonlinear evolution equations. Appl. Math. Comput. 2010, 217, 869–877. [Google Scholar]
- Choudhuri, A.; Porsezian, K. Dark-in-the-Bright solitary wave solution of higher-order nonlinear Schrödinger equation with non-Kerr terms. Opt. Commun. 2012, 285, 364–367. [Google Scholar] [CrossRef]
- Arshad, M.; Seadawy, A.R.; Lu, D. Study of soliton solutions of higher-order nonlinear Schrödinger dynamical model with derivative non-Kerr nonlinear terms and modulation instability analysis. Results Phys. 2019, 13, 102305. [Google Scholar] [CrossRef]
- Khater, M.M.A.; Attia, R.A.M.; Abdel-Aty, A.-H.; Abdou, M.A.; Eleuch, H.; Lu, D. Analytical and semi-analytical ample solutions of the higher-order nonlinear Schrödinger equation with the non-Kerr nonlinear term. Results Phys. 2020, 16, 103000. [Google Scholar] [CrossRef]
- Zayed, E.M.E.; Al-Nowehy, A.-G. Many new exact solutions to the higher-order nonlinear Schrodinger equation with derivative non Kerr nonlinear terms using three different techniques. Optik 2017, 143, 84–103. [Google Scholar] [CrossRef]
- Sharma, V.K.; De, K.K.; Goyal, A. Solitary wave solutions of higher-order nonlinear Schrödinger equation with derivative non-Kerr nonlinear terms. Workshop Recent Adv. Photonics WRAP 2013. [Google Scholar] [CrossRef]
- Az-Zo’bi, E.A.; AlZoubi, W.A.; Akinyemi, L.; Şenol, M.; Alsaraireh, I.W.; Mamat, M. Abundant closed-form solitons for time-fractional integro–differential equation in fluid dynamics. Opt. Quant. Electron. 2021, 53, 132. [Google Scholar] [CrossRef]
- Darwish, A.; El-Dahab, E.A.; Ahmed, H.; Arnous, A.H.; Ahmed, M.S.; Biswas, A.; Guggilla, P.; Yıldırım, Y.; Mallawi, F.; Belic, M.R. Optical solitons in fiber Bragg gratings via modified simple equation. Optik 2020, 203, 163886. [Google Scholar] [CrossRef]
- Al-Amr, M.O.; Rezazadeh, H.; Ali, K.K.; Korkmazki, A. N1-soliton solution for Schrödinger equation with competing weakly nonlocal and parabolic law nonlinearities. Commun. Theor. Phys. 2020, 72, 065503. [Google Scholar] [CrossRef]
- Islam, M.N.; Asaduzzaman, M.; Ali, M.S. Exact wave solutions to the simplified modified Camassa-Holm equation in mathematical physics. AIMS Math. 2020, 5, 26–41. [Google Scholar] [CrossRef]
- El-Sheikh, M.M.; Ahmed, H.M.; Arnous, A.H.; Rabie, W.B.; Biswas, A.; Alshomrani, A.S.; Ekici, M.; Zhou, Q.; Belic, M.R. Optical solitons in birefringent fibers with Lakshmanan-Porsezian-Daniel model by modified simple equation. Optik 2019, 192, 162899. [Google Scholar] [CrossRef]
- Az-Zo’bi, E.A. New kink solutions for the van der Waals p-system. Math. Methods Appl. Sci. 2019, 42, 6216–6226. [Google Scholar] [CrossRef]
- Yıldırım, Y. Optical solitons to Kundu–Mukherjee–Naskar model in birefringent fibers with modified simple equation approach. Optik 2019, 184, 121–127. [Google Scholar] [CrossRef]
- Az-Zo’bi, E.A. Peakon and solitary wave solutions for the modified Fornberg-Whitham equation using simplest equation method. Int. J. Math. Comput. Sci. 2019, 14, 635–645. [Google Scholar]
- Kazi, S.; Hossain, A.K.M.; Akbar, M.A.; Wazwaz, A.-M. Closed form solutions of complex wave equations via the modified simple equation method. Cogent Phys. 2017, 4, 1312751. [Google Scholar] [CrossRef]
- Irshad, A.; Mohyud-Din, S.T.; Ahmed, N.; Khan, U. A New Modification in Simple Equation Method and its applications on nonlinear equations of physical nature. Results Phys. 2017, 7, 4232–4240. [Google Scholar] [CrossRef]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rasheed, N.M.; Al-Amr, M.O.; Az-Zo’bi, E.A.; Tashtoush, M.A.; Akinyemi, L. Stable Optical Solitons for the Higher-Order Non-Kerr NLSE via the Modified Simple Equation Method. Mathematics 2021, 9, 1986. https://doi.org/10.3390/math9161986
Rasheed NM, Al-Amr MO, Az-Zo’bi EA, Tashtoush MA, Akinyemi L. Stable Optical Solitons for the Higher-Order Non-Kerr NLSE via the Modified Simple Equation Method. Mathematics. 2021; 9(16):1986. https://doi.org/10.3390/math9161986
Chicago/Turabian StyleRasheed, Noha M., Mohammed O. Al-Amr, Emad A. Az-Zo’bi, Mohammad A. Tashtoush, and Lanre Akinyemi. 2021. "Stable Optical Solitons for the Higher-Order Non-Kerr NLSE via the Modified Simple Equation Method" Mathematics 9, no. 16: 1986. https://doi.org/10.3390/math9161986
APA StyleRasheed, N. M., Al-Amr, M. O., Az-Zo’bi, E. A., Tashtoush, M. A., & Akinyemi, L. (2021). Stable Optical Solitons for the Higher-Order Non-Kerr NLSE via the Modified Simple Equation Method. Mathematics, 9(16), 1986. https://doi.org/10.3390/math9161986







