Mathematics Training in Engineering Degrees: An Intervention from Teaching Staff to Students
Abstract
:1. Introduction
Research Rationale and Research Questions
- How can the mathematical curriculum of an engineering program be adapted to include technological applications?
- How do teachers value this intervention?
- How do students value this intervention?
2. Materials and Methods
2.1. Teachers’ Intervention
2.2. Students’ Intervention
3. Results
3.1. Teachers’ Results
3.1.1. Teachers’ Mathematical Contents
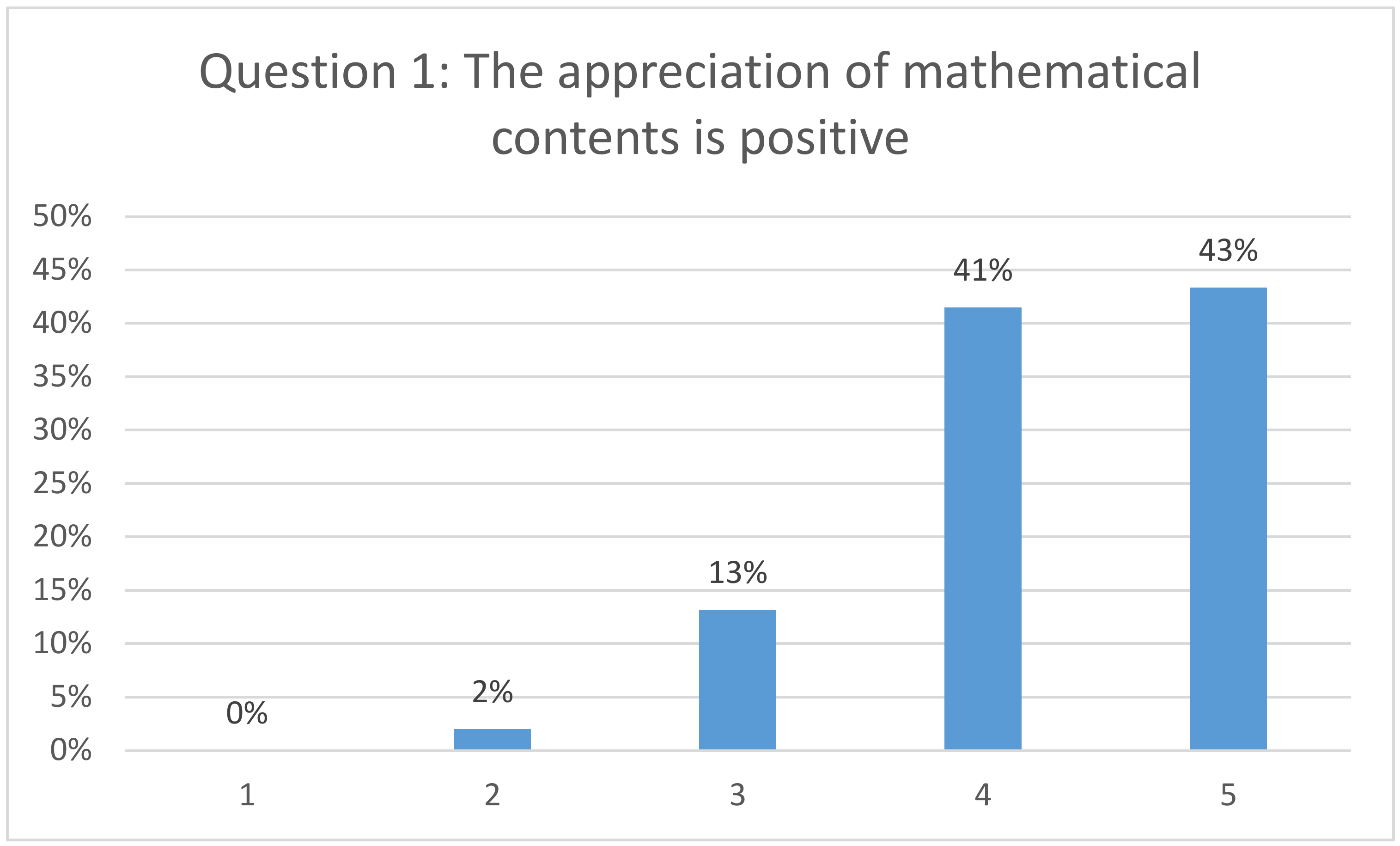
3.1.2. Teachers’ Surveys Results
- Innovative problems.
- Examples with applications in different fields.
- Interesting works linked to social needs.
3.2. Students’ Results
3.2.1. Students’ Mathematical Contents
- –
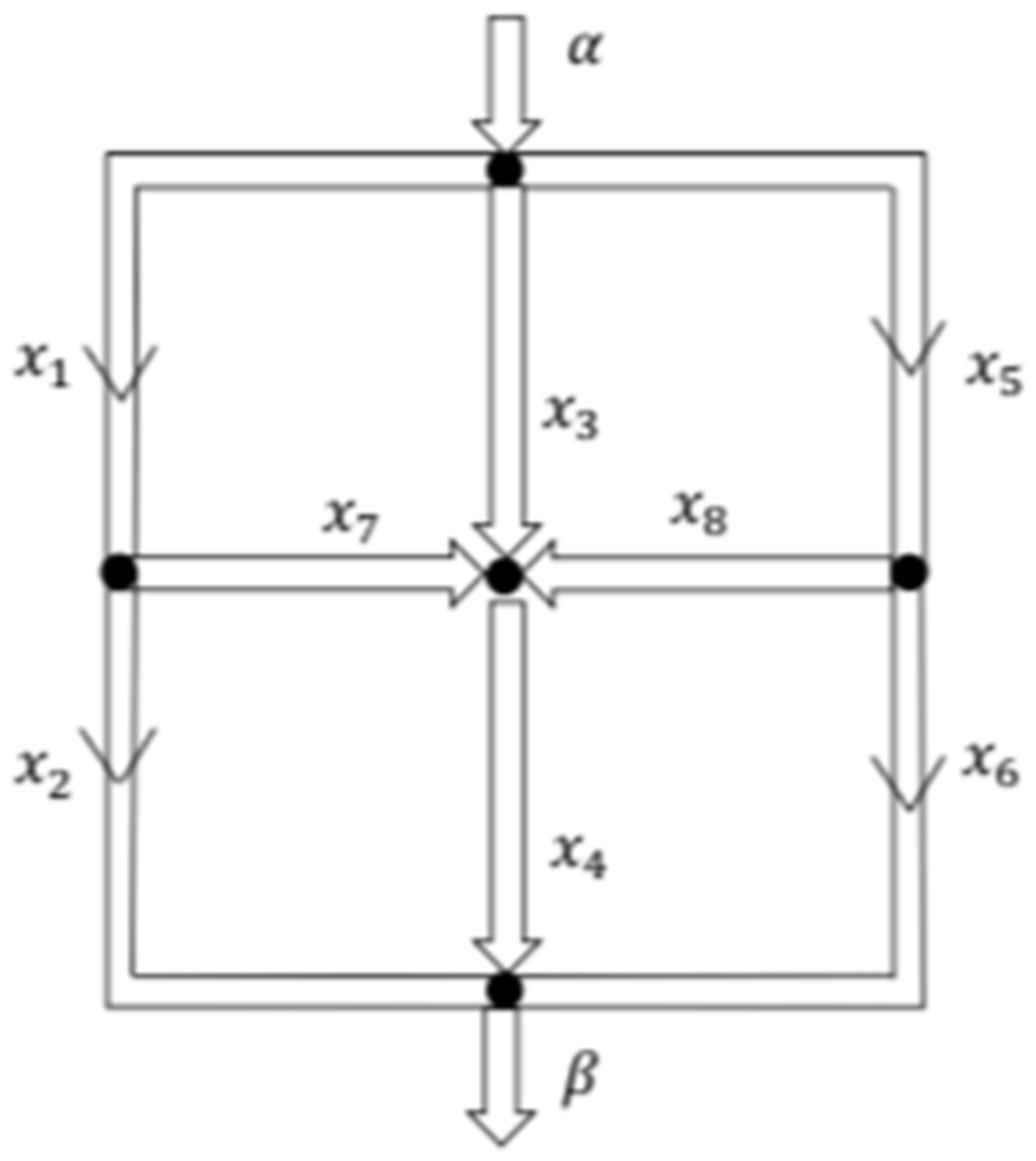
- Analysis of alternating current circuits: an alternating current i(t) must be calculated in a node, knowing the values of three alternating currents in the same node. Kirchhoff’s current law is used, and currents are converted into the complex form.
- –
- Triphasic distribution: phase/neutral voltage and phase/phase voltage must be calculated in a triphasic distribution. To solve it, voltages are converted into the complex form, and phasor representation is used in order to explain the relation between phase/neutral voltage and phase/phase voltage.
- –
- RLC circuit: a circuit with resistance, inductance and capacitor is solved using the complex impedance.
- –
- Resonances: the conditions in which resonance is produced in a parallel circuit must be determined.
- –
- Annulation of reactive power: in this exercise, the capacity of a capacitor must be calculated which has to be in parallel with impedance so that the equivalent impedance is real. That means that reactive power disappears, and performance is optimized.
- Prove that it can be described by the following linear equation system:
- Find and interpret the compatibility conditions.
- In such case, prove that it is a 1-indeterminate system, and a solution basis of the homogeneous system is .
- How many traffic measures are needed to know (?
- Deduce that there exist solutions with and that there exists a unique solution with and some .
- Interpret the solutions with .
- To study the compatibility conditions of the system.
- To determine how many flows must be measured to know the global circulation of the system.
- If global circulation can be calculated measuring the flows of the four peripheric points.
- If global circulation can be calculated measuring the flows of the four intern points.
- To generalize the study to three branches with more the one interconnexion.
- Prove that E is a vector space of dimension 9 and that F is a subspace of E of dimension 4.
- Determinate a basis of F so that (I1, I2, I3, I4) are its coordinates.
- Prove that one of Kirchhoff’s equations is redundant; that is, if it is verified at 5 nodes, it must also be verified at the 6th node.
- –
- With all of the controls, as an addition of subspaces.
- –
- With any of the controls, as an intersection of subspaces.
- G + B = CYAN (C)
- R + B = MAGENTA (M)
- R + G = YELLOW
- R + G + B = WHITE
- MAGENTA + YELLOW = RED
- CYAN + YELLOW = GREEN
- CYAN + MAGENTA = BLUE
- CYAN + MAGENTA + YELLOW = BLACK
- –
- Stations A, B: 1/3 of buses goes to C; 1/3 of buses goes to D; 1/3 of buses remains for maintenance.
- –
- Station C (and respectively D): 1/4 of buses goes to A; 1/4 of buses goes to B; 1/2 of buses goes to D (and respectively C).
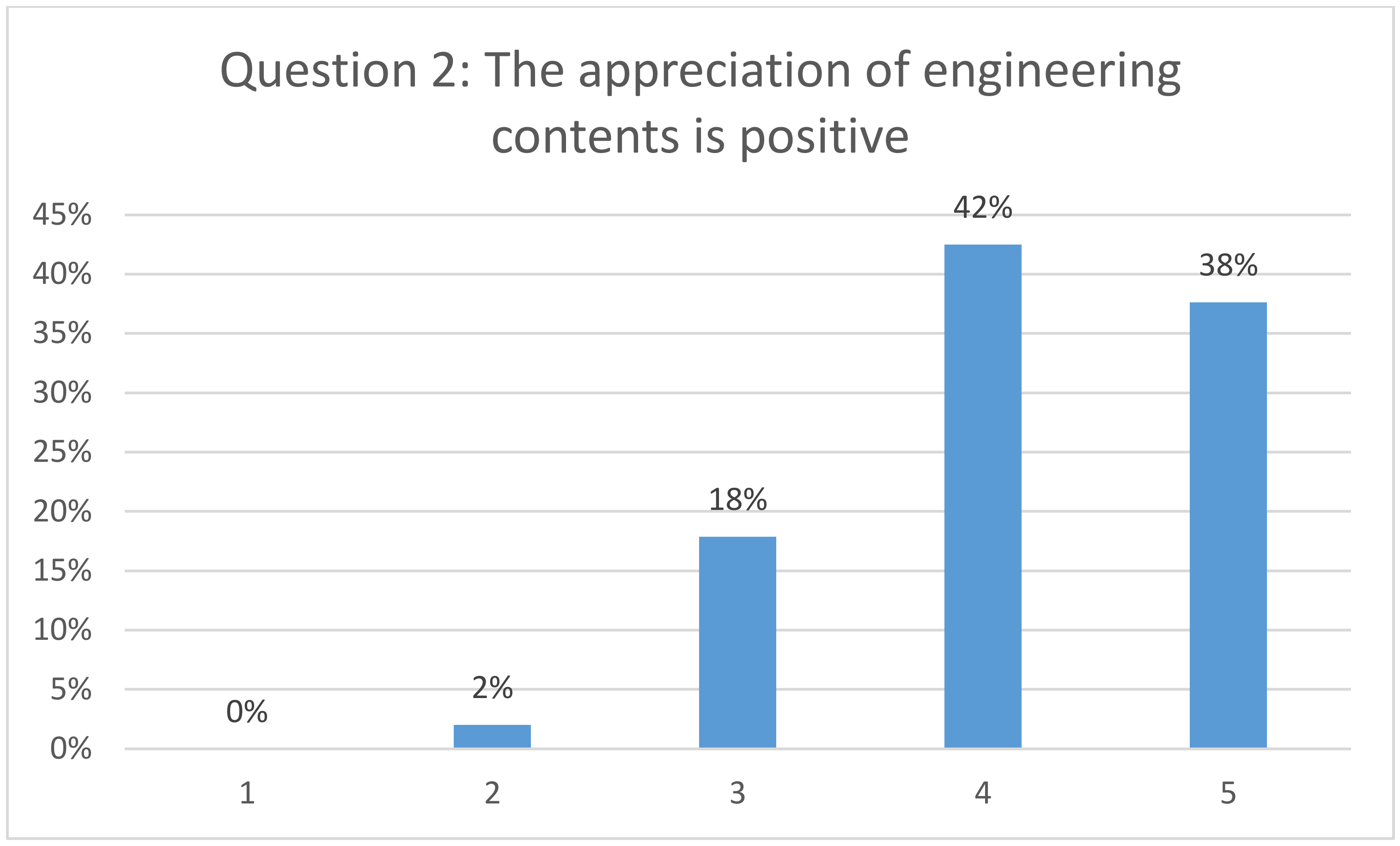
3.2.2. Students’ Surveys and Interviews Results
- Applications let students know that mathematics is necessary.
- These sessions achieve the goal to motivate students and let them realize that linear algebra has real applications.
- Context in mathematics increases the interest and the attention of students, both in university and in secondary school.
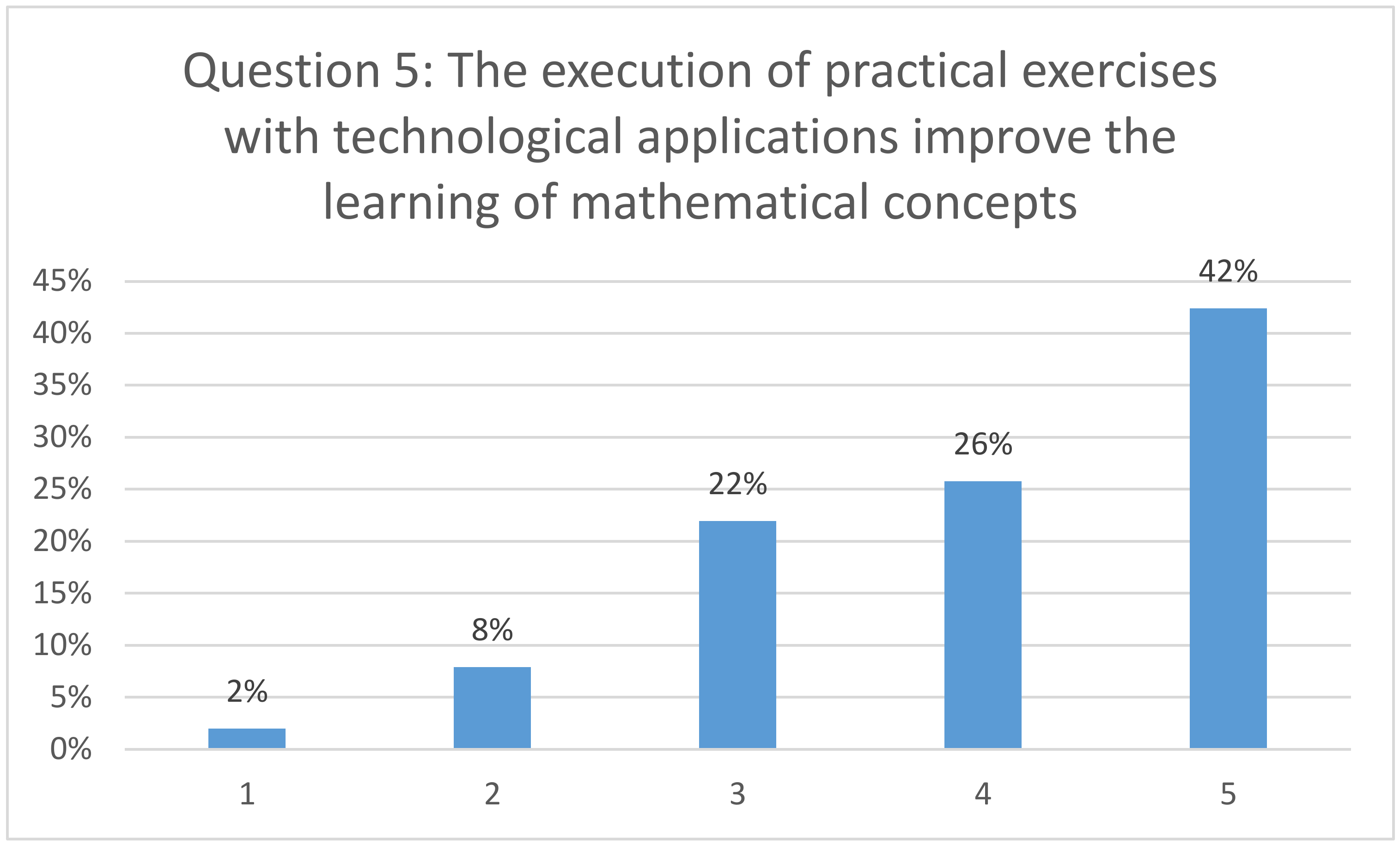
- Applications helped students learn better linear algebra concepts.
- What aspects do you asses more positively of these sessions?
- What applications have been more interesting? Why?
- How have these sessions influenced on your motivation and on your interest toward linear algebra?
- Have these sessions helped you understand mathematical concepts of the subject linear algebra? What applications? What concepts?
- After these sessions, do you consider that mathematics are more important and essential to the development of engineering degrees? How? Why?
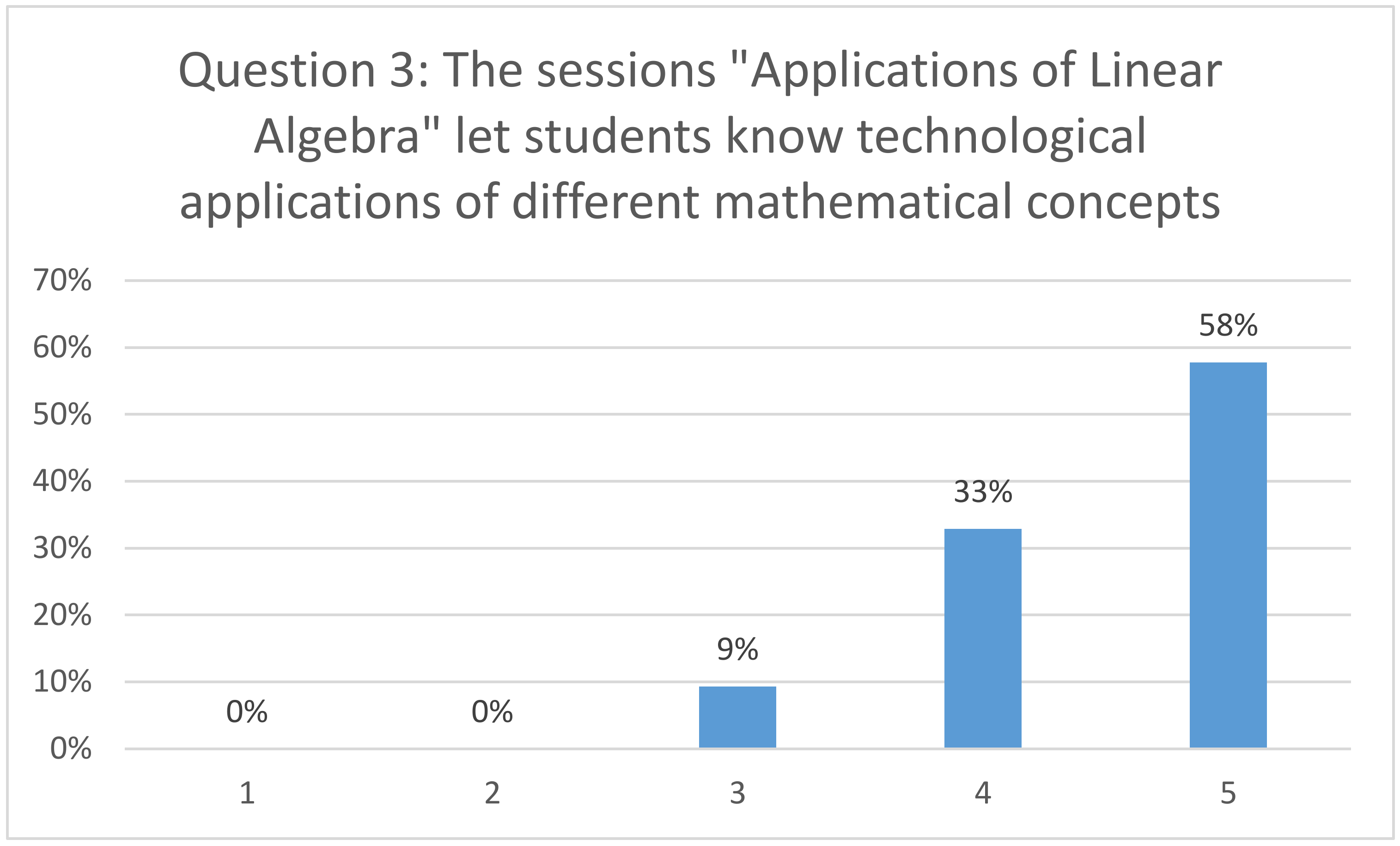
- The sessions “Applications of Linear Algebra in Engineering” let students know real applications in different disciplines of engineering.
- Seeing all these applications let students know what they will be able to do in the following courses and it is very motivating.
- These applications let students realize of how important linear algebra is for engineering degrees and for their future profession.
- Interesting applications: complex numbers (electricity, economy), indeterminate compatible systems (roundabout traffic), vector subspaces (linear control systems), linear applications (change to italics, population fluxes), eigenvectors and eigenvalues (demographic control).
4. Discussion
5. Conclusions and Future Work
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Marra, R.M.; Rodgers, K.A.; Shen, D.; Bogue, B. Leaving engineering: A multi-year single institution study. J. Eng. Educ. 2012, 101, 6–27. [Google Scholar] [CrossRef]
- Vennix, J.; den Brok, P.; Taconis, R. Do outreach activities in secondary STEM education motivate students and improve their attitudes towards STEM? Int. J. Sci. Educ. 2018, 40, 1263–1283. [Google Scholar] [CrossRef] [Green Version]
- Caprile, M.; Palmén, R.; Sanz, P.; Dente, G. Encouraging STEM Studies for the Labour Market, European Parlament; Directorate-General for Research and Innovation: Brussels, Belgium, 2015. [Google Scholar]
- Timms, M.J.; Moyle, K.; Weldon, P.R.; Mitchell, P. Challenges in STEM Learning in Australian Schools: Literature and Policy Review; Australian Council for Educational Research: Camberwell, UK, 2018; ISBN 9781742864990. [Google Scholar]
- Lesh, R.; Doerr, H.M. No beyond Constructivism: Models and Modeling Perspectives on Mathematics Problem Solving, Learning, and Teaching; Lawrence Erlbaum Associates: Mahwah, NJ, USA, 2003. [Google Scholar]
- Fadzil, H.M.; Saat, R.M.; Awang, K.; Adli, D.S.H. Students’ perception of learning stem-related subjects through scientist-teacher-student partnership (STSP). J. Balt. Sci. Educ. 2019, 18, 537–548. [Google Scholar] [CrossRef] [Green Version]
- Bradburn, E.M.; Carroll, C.D. Short-Term Enrollment in Postsecondary Education; National Center for Educational Statistics: Washington, DC, USA, 2002.
- Ministerio de Universidades. Indicators of Undergraduate Students’ Academic Performance, Education Dropout Rate (Indicadores de Rendimiento Académico de Estudiantes de Grado, Tasas de Abandono del Estudio); Ministerio de Universidades: Madrid, Spain, 2019.
- Zumbrunn, S.; McKim, C.; Buhs, E.; Hawley, L.R. Support, belonging, motivation, and engagement in the college classroom: A mixed method study. Instr. Sci. 2014, 42, 661–684. [Google Scholar] [CrossRef]
- Lopez, D. Using service learning for improving student attraction and engagement in STEM studies. In Proceedings of the 45th SEFI Annual Conference 2017—Education Excellence for Sustainability, Azores, Portugal, 18–21 September 2017; Volume 28, pp. 1053–1060. [Google Scholar]
- Wilson, D.; Jones, D.; Bocell, F.; Crawford, J.; Kim, M.J.; Veilleux, N.; Floyd-Smith, T.; Bates, R.; Plett, M. Belonging and Academic Engagement among Undergraduate STEM Students: A Multi-institutional Study. Res. High. Educ. 2015, 56, 750–776. [Google Scholar] [CrossRef]
- Kuh, G.D.; Cruce, T.M.; Shoup, R.; Kinzie, J.; Gonyea, R.M. Unmasking the Effects of Student on First-Year College Engagement Grades and Persistence. J. High. Educ. 2008, 79, 540–563. [Google Scholar] [CrossRef]
- Tinto, V. Dropout from higher education: A theoretical systhesis of recent research. Rev. Educ. Res. 1975, 45, 89–125. [Google Scholar] [CrossRef]
- Gasiewski, J.A.; Eagan, M.K.; Garcia, G.A.; Hurtado, S.; Chang, M.J. From Gatekeeping to Engagement: A Multicontextual, Mixed Method Study of Student Academic Engagement in Introductory STEM Courses. Res. High. Educ. 2012, 53, 229–261. [Google Scholar] [CrossRef] [Green Version]
- Handelsman, M.M.; Briggs, W.L.; Sullivan, N.; Towler, A. A Measure of College Student Course Engagement. J. Educ. Res. 2005, 98, 184–192. [Google Scholar] [CrossRef]
- Chapman, O.; An, S. A survey of university-based programs that support in-service and pre-service mathematics teachers’ change. ZDM Math. Educ. 2017, 49, 171–185. [Google Scholar] [CrossRef]
- Schoenfeld, A.H.; Kilpatrick, J. Toward a Theory of Proficiency in teaching mathematics. In International Handbook of Mathematics Teacher Education; Tirosh, D., Wood, T., Eds.; Brill Sense: Rotterdam, The Netherlands, 2008; Volume 2. [Google Scholar]
- Tinnell, T.L.; Ralston, P.A.S.; Tretter, T.R.; Mills, M.E. Sustaining pedagogical change via faculty learning community. Int. J. STEM Educ. 2019, 6. [Google Scholar] [CrossRef] [Green Version]
- Fischer, C.; Fishman, B.; Dede, C.; Eisenkraft, A.; Frumin, K.; Foster, B.; Lawrenz, F.; Levy, A.J.; McCoy, A. Investigating relationships between school context, teacher professional development, teaching practices, and student achievement in response to a nationwide science reform. Teach. Teach. Educ. 2018, 72, 107–121. [Google Scholar] [CrossRef]
- Sellami, A.; El-Kassem, R.C.; Al-Qassass, H.B.; Al-Rakeb, N.A. A path analysis of student interest in STEM, with specific reference to Qatari students. Eurasia J. Math. Sci. Technol. Educ. 2017, 13, 6045–6067. [Google Scholar] [CrossRef]
- Chalmers, C.; Carter, M.L.; Cooper, T.; Nason, R. Implementing “Big Ideas” to Advance the Teaching and Learning of Science, Technology, Engineering, and Mathematics (STEM). Int. J. Sci. Math. Educ. 2017, 15, 25–43. [Google Scholar] [CrossRef] [Green Version]
- Kumar, S.; Jalkio, J.A. Teaching mathematics from an applications perspective. J. Eng. Educ. 1999, 88, 275–279. [Google Scholar] [CrossRef] [Green Version]
- Dong, Y.; Xu, C.; Song, X.; Fu, Q.; Chai, C.S.; Huang, Y. Exploring the Effects of Contextual Factors on In-Service Teachers’ Engagement in STEM Teaching. Asia-Pac. Educ. Res. 2019, 28, 25–34. [Google Scholar] [CrossRef]
- Kent, P.; Noss, R. Finding a Role for Technology in Service Mathematics for Engineers and Scientists. In The Teaching and Learning of Mathematics at University Level; Kluwer Academic Publishers: Berlin, Germany, 2005; pp. 395–404. [Google Scholar] [CrossRef]
- Perdigones, A.; Gallego, E.; García, N.; Fernández, P.; Pérez-Martín, E.; Del Cerro, J. Physics and Mathematics in the Engineering Curriculum: Correlation with Applied Subjects. Int. J. Eng. Educ. 2014, 30, 1509–1521. [Google Scholar]
- Freeman, S.; Eddy, S.L.; McDonough, M.; Smith, M.K.; Okoroafor, N.; Jordt, H.; Wenderoth, M.P. Active learning increases student performance in science, engineering, and mathematics. Proc. Natl. Acad. Sci. USA 2014, 111, 8410–8415. [Google Scholar] [CrossRef] [Green Version]
- Borda, E.; Schumacher, E.; Hanley, D.; Geary, E.; Warren, S.; Ipsen, C.; Stredicke, L. Initial implementation of active learning strategies in large, lecture STEM courses: Lessons learned from a multi-institutional, interdisciplinary STEM faculty development program. Int. J. STEM Educ. 2020, 7. [Google Scholar] [CrossRef]
- Loyens, S.M.M.; Magda, J.; Rikers, R.M.J.P. Self-directed learning in problem-based learning and its relationships with self-regulated learning. Educ. Psychol. Rev. 2008, 20, 411–427. [Google Scholar] [CrossRef] [Green Version]
- Cetin, Y.; Mirasyedioglu, S.; Cakiroglu, E. An inquiry into the underlying reasons for the impact of technology enhanced problem-based learning activities on students’ attitudes and achievement. Eurasian J. Educ. Res. 2019, 2019, 191–208. [Google Scholar] [CrossRef] [Green Version]
- Mallart, A.; Font, V.; Diez, J. Case study on mathematics pre-service teachers’ difficulties in problem posing. Eurasia J. Math. Sci. Technol. Educ. 2018, 14, 1465–1481. [Google Scholar] [CrossRef]
- Bingolbali, E.; Ozmantar, M.F. Factors shaping mathematics lecturers’ service teaching in different departments. Int. J. Math. Educ. Sci. Technol. 2009, 40, 597–617. [Google Scholar] [CrossRef]
- Harris, D.; Pampaka, M. “They [the lecturers] have to get through a certain amount in an hour” First year students’ problems with service mathematics lectures. Teach. Math. Appl. Int. J. IMA 2016, 35, 144–158. [Google Scholar] [CrossRef] [Green Version]
- Cárcamo Bahamonde, A.; Gómez Urgelles, J.; Fortuny Aymemí, J. Mathematical modelling in engineering: A proposal to introduce linear algebra concepts. J. Technol. Sci. Educ. 2016, 6, 62–70. [Google Scholar]
- Kandamby, G.W.T.C. Enhancement of learning through field study. J. Technol. Sci. Educ. 2018, 8, 408–419. [Google Scholar] [CrossRef] [Green Version]
- Doerr, H.M. What Knowledge Do Teachers Need for Teaching Mathematics through Applications and Modelling. In Modelling and Applications in Mathematics Education; Springer: New York, NY, USA, 2007; pp. 69–78. [Google Scholar] [CrossRef]
- Darhim, P.S.; Susilo, B.E. The effect of problem-based learning and mathematical problem posing in improving student’s critical thinking skills. Int. J. Instr. 2020, 13, 103–116. [Google Scholar] [CrossRef]
- Passow, H. Which ABET Competencies Do Engineering Graduates Find Most Important in their Work? J. Eng. Educ. 2012, 101, 95–118. [Google Scholar] [CrossRef]
- Ferrer, J.; Peña, M.; Ortiz-Caraballo, C. Learning engineering to teach mathematics. In Proceedings of the 40th ASEE/IEEE Frontiers in Education Conference, Arlington, VA, USA, 27–30 October 2010. [Google Scholar]
- Lacuesta, R.; Palacios, G.; Fernández, L. Active learning through problem based learning methodology in engineering education. Proc. Front. Educ. Conf. FIE 2009, 1–6. [Google Scholar] [CrossRef]
- Chen, J.; Kolmos, A.; Du, X. Forms of implementation and challenges of PBL in engineering education: A review of literature. Eur. J. Eng. Educ. 2021, 46, 90–115. [Google Scholar] [CrossRef]
- Amaya Chavez, D.; Gámiz-Sánchez, V.; Cañas Vargas, A. PBL: Effects on Academic Performance and Perceptions of Engineering Students. J. Technol. Sci. Educ. 2020, 10, 306–328. [Google Scholar] [CrossRef]
- Abramovich, S.; Grinshpan, A.Z.; Milligan, D.L. Teaching Mathematics through Concept Motivation and Action Learning. Educ. Res. Int. 2019, 2019. [Google Scholar] [CrossRef]
- Baya’a, N.; Daher, W.; Anabousy, A. The development of in-service mathematics teachers’ integration of ICT in a community of practice: Teaching-in-context theory. Int. J. Emerg. Technol. Learn. 2019, 14, 125–139. [Google Scholar] [CrossRef] [Green Version]
- Fairweather, J. Linking Evidence and Promising Practices in STEM Undergraduate Education; Board of Science Education, National Research Council, The National Academies: Washington, DC, USA, 2010. [Google Scholar]
- Naidoo, J.; Singh-Pillay, A. Teachers’ perceptions of using the blended learning approach for stem-related subjects within the fourth industrial revolution. J. Balt. Sci. Educ. 2020, 19, 583–593. [Google Scholar] [CrossRef]
- Muhtadi, D.; Kartasasmita, B.G.; Prahmana, R.C.I. The Integration of technology in teaching mathematics. J. Phys. Conf. Ser. 2018, 943. [Google Scholar] [CrossRef]
- Johnston, J.; Walshe, G.; Ríordáin, M.N. Supporting Key Aspects of Practice in Making Mathematics Explicit in Science Lessons. Int. J. Sci. Math. Educ. 2020, 18, 1399–1417. [Google Scholar] [CrossRef]
- Gilchrist, P.O.; Alexander, A.B.; Green, A.J.; Sanders, F.E.; Hooker, A.Q.; Reif, D.M. Development of a pandemic awareness stem outreach curriculum: Utilizing a computational thinking taxonomy framework. Educ. Sci. 2021, 11, 109. [Google Scholar] [CrossRef]
- Ferrer, J.; Peña, M.; Ortiz-Caraballo, C. Learning Automation to Teach Mathematics; IntechOpen: London, UK, 2012. [Google Scholar] [CrossRef] [Green Version]
- Peña, M. Applying Dynamical Discrete Systems to Teach Mathematics; ATINER’s Conference Paper Proceedings Series EMS2017-0110; IEEE: Athens, Greece, 2018; pp. 1–27. [Google Scholar]
- Lamberson, R.H.; McKelvey, R.; Noon, B.R.; Voss, C. A Dynamic Analysis of Northern Spotted Owl Viability in a Fragmented Forest Landscape. Conserv. Biol. 1992, 6, 505–512. [Google Scholar] [CrossRef]
- Kertil, M.; Gurel, C. Mathematical Modeling: A Bridge to STEM Education. Int. J. Educ. Math. Sci. Technol. 2016, 4, 44. [Google Scholar] [CrossRef]
- Yildirim, B.; Sidekli, S. STEM applications in mathematics education: The effect of STEM applications on different dependent variables. J. Balt. Sci. Educ. 2018, 17, 200–214. [Google Scholar] [CrossRef]
- Lopez-Diaz, M.T.; Peña, M. Contextualization of basic sciences in technological degrees. In Proceedings of the 2020 IEEE Frontiers in Education Conference (FIE), Uppsala, Sweden, 21–24 October 2020. [Google Scholar]





| Session | Title | Date |
|---|---|---|
| 1 | “Invitation to the Educative Renewal of Mathematics in Engineering degrees” | 10 April 2018 |
| 2 | “Network Flows” | 25 April 2018 |
| 3 | “Engagement with the First Course Students of Civil Engineering” | 15 May 2018 |
| 4 | “Mathematics of Google” | 23 May 2018 |
| 5 | “Numerical Factory: a Numerical Tasting about the Teaching of Mathematics in Engineering” | 5 June 2018 |
| 6 | “How Mathematical Tools help to Manufacture Mechanical Parts” | 3 October 2018 |
| 7 | “One Proposal for the Teaching of Mathematics in Computer Science” | 16 October 2018 |
| 8 | “Mathematical Applications in Elasticity and Resistance of Materials” | 7 November 2018 |
| 9 | “A Historically Problematic Relationship: Mathematics in Engineering” | 27 November 2018 |
| 10 | “Virtual Reality Applications for Biomedical Engineering” | 27 February 2019 |
| 11 | “Fundamental Mathematical Concepts and Tools in Electronic Engineering” | 21 March 2019 |
| 12 | “Modelling and Linear Ordinary Differential Equations Systems” | 10 April 2019 |
| 13 | “Determined Linear Systems for Consecutive Values of States” | 2 May 2019 |
| 14 | “Mathematical Concepts and Tools in Automatic” | 22 May 2019 |
| 15 | “Animated Mathematics” | 16 October 2019 |
| 16 | “Probabilities and Communication Theory: Random Walks in Graphs and Algorithms” | 2 December 2020 |
| 17 | “Cryptography: the Arithmetic of Large Numbers” | 17 March 2021 |
| 18 | “Mathematics at the Service of Engineering Attitudes” | 4 May 2021 |
| Session | Title |
|---|---|
| 1 | “Complex Numbers on the Study of Price Fluctuations” |
| 2 | “Complex Numbers on the Study of Alternating Current” |
| 3 | “Indeterminate Systems: Control Variables” |
| 4 | “Mesh Flushes: a Basis of Conservative Fluxes Vector Subspace” |
| 5 | “Addition and Intersection of Vector Subspaces in Discrete Dynamical Systems” |
| 6 | “Linear Applications and Associated Matrix” |
| 7 | “Basis Changes” |
| 8 | “Eigenvalues, Eigenvectors and Diagonalization in Engineering” |
| 9 | “Modal Analysis in Discrete Dynamical Systems” |
| 10 | “Difference Equations” |
| Applications | Linear Algebra Contents |
|---|---|
| Roundabout traffic | Matrices and determinants. Equation systems. |
| Electrical network | Equation systems. Vectorial spaces. Vectorial subspaces. Linear applications. |
| Bus station | Discrete linear systems: contagious matrix, eigenvectors and eigenvalues. |
| Google: webs classification | Discrete linear systems: contagious matrix, eigenvectors and eigenvalues, Gould accessibility index. |
| Survey Question | Average |
|---|---|
| The assessment of academic aspects is positive | 4.56 |
| The level of satisfaction regarding the speaker is positive | 4.62 |
| General organization of the activity has been appropriate | 4.56 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
López-Díaz, M.T.; Peña, M. Mathematics Training in Engineering Degrees: An Intervention from Teaching Staff to Students. Mathematics 2021, 9, 1475. https://doi.org/10.3390/math9131475
López-Díaz MT, Peña M. Mathematics Training in Engineering Degrees: An Intervention from Teaching Staff to Students. Mathematics. 2021; 9(13):1475. https://doi.org/10.3390/math9131475
Chicago/Turabian StyleLópez-Díaz, María Teresa, and Marta Peña. 2021. "Mathematics Training in Engineering Degrees: An Intervention from Teaching Staff to Students" Mathematics 9, no. 13: 1475. https://doi.org/10.3390/math9131475
APA StyleLópez-Díaz, M. T., & Peña, M. (2021). Mathematics Training in Engineering Degrees: An Intervention from Teaching Staff to Students. Mathematics, 9(13), 1475. https://doi.org/10.3390/math9131475






