Myocardial Fibrosis in a 3D Model: Effect of Texture on Wave Propagation
Abstract
:1. Introduction
2. Materials and Methods
2.1. Electrophysiology Model
Numerical Methods
2.2. Fibrosis Pattern Generation
2.3. Shortest Path Calculation
3. Results
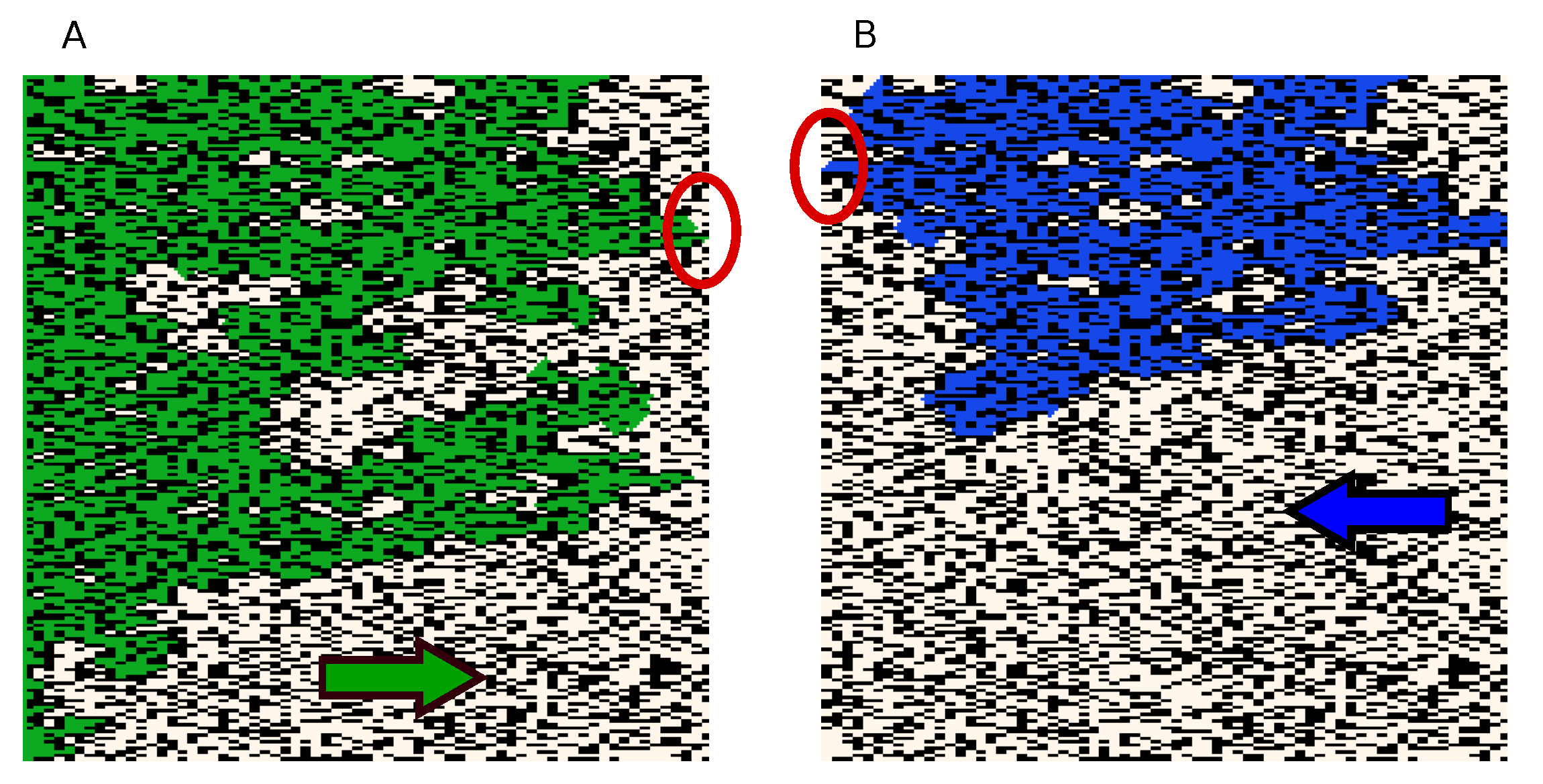
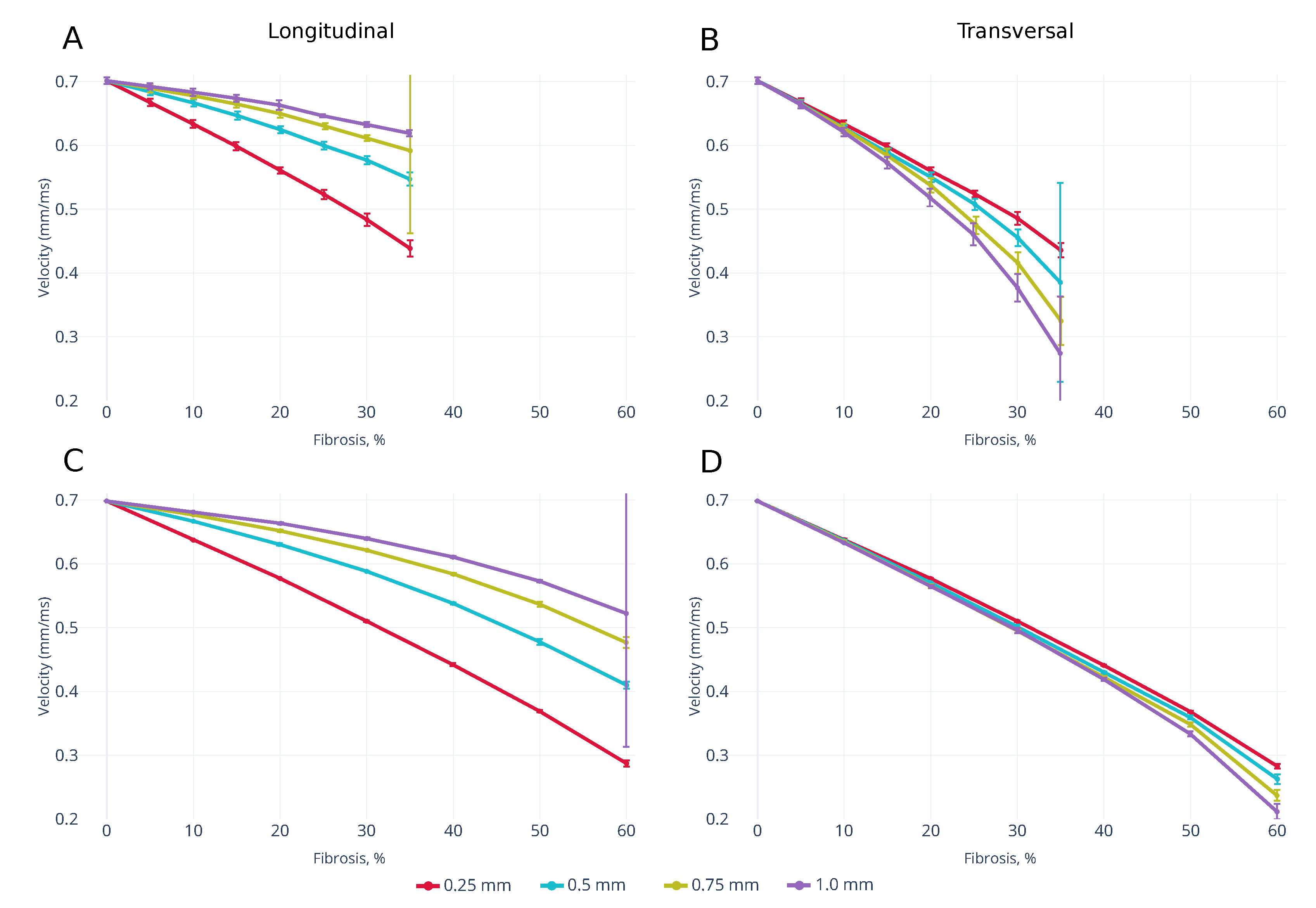
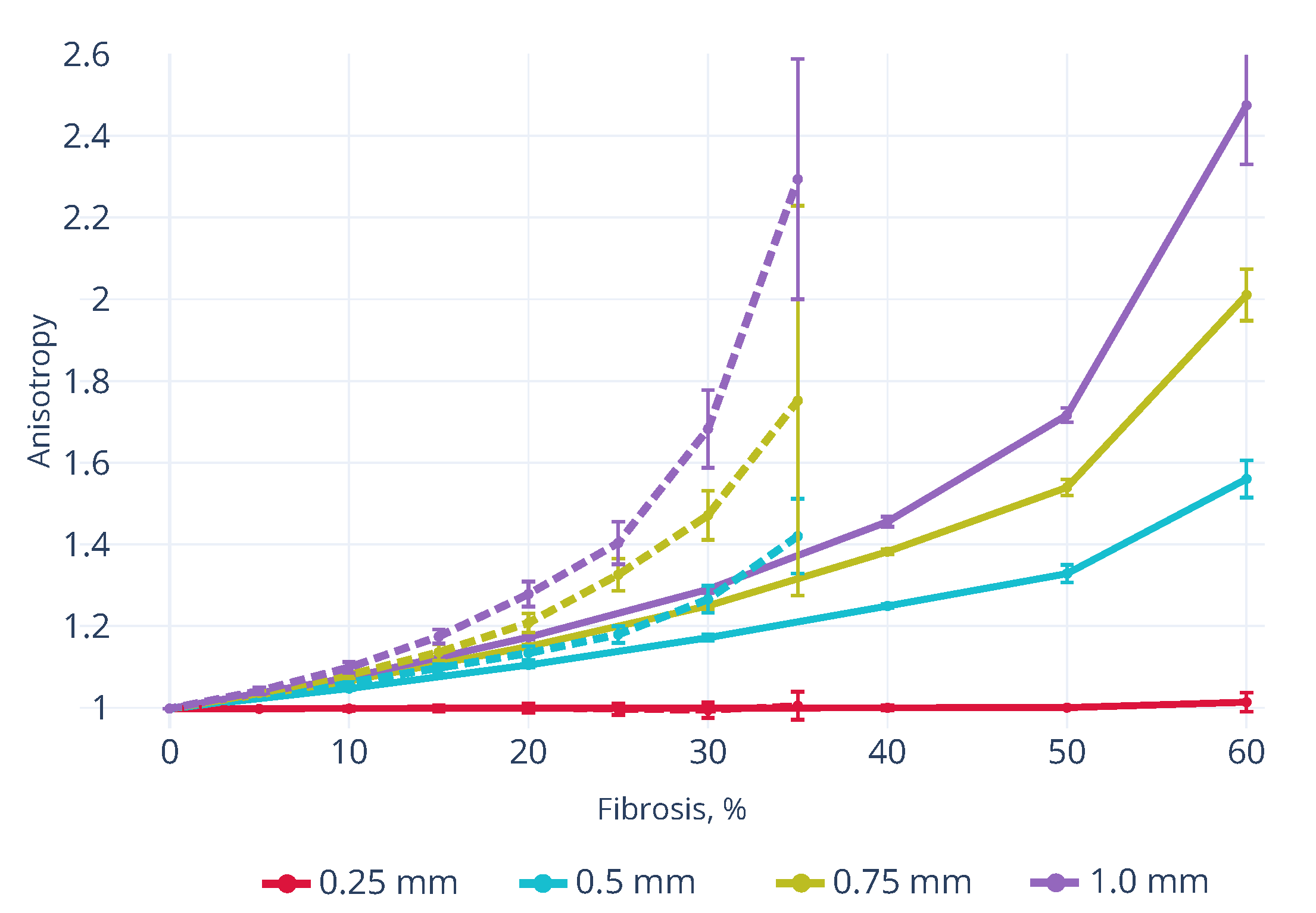
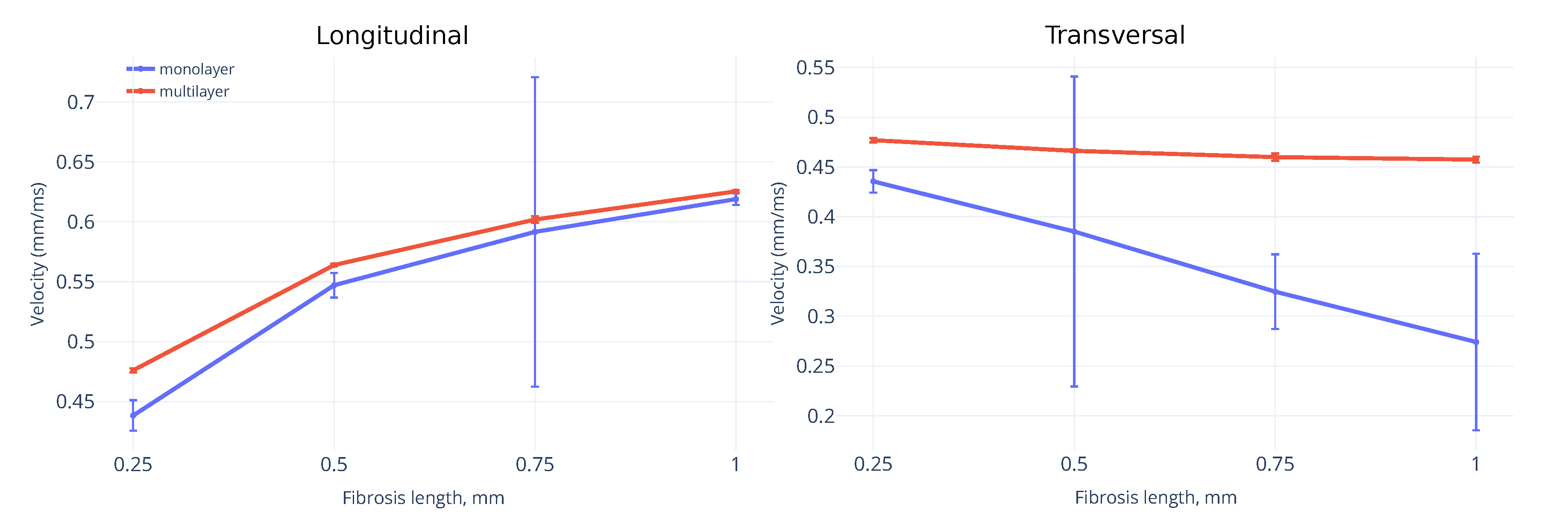
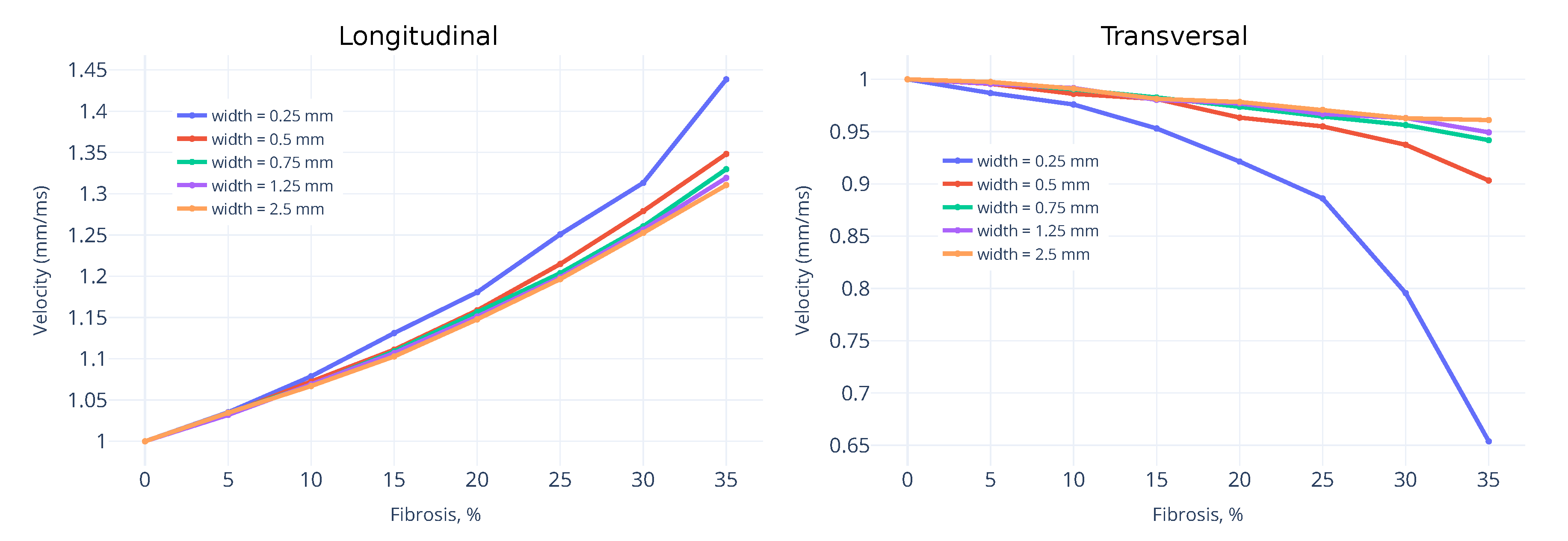
3.1. Wavefront Velocity Depends on Fibrosis Fraction and Propagation Direction
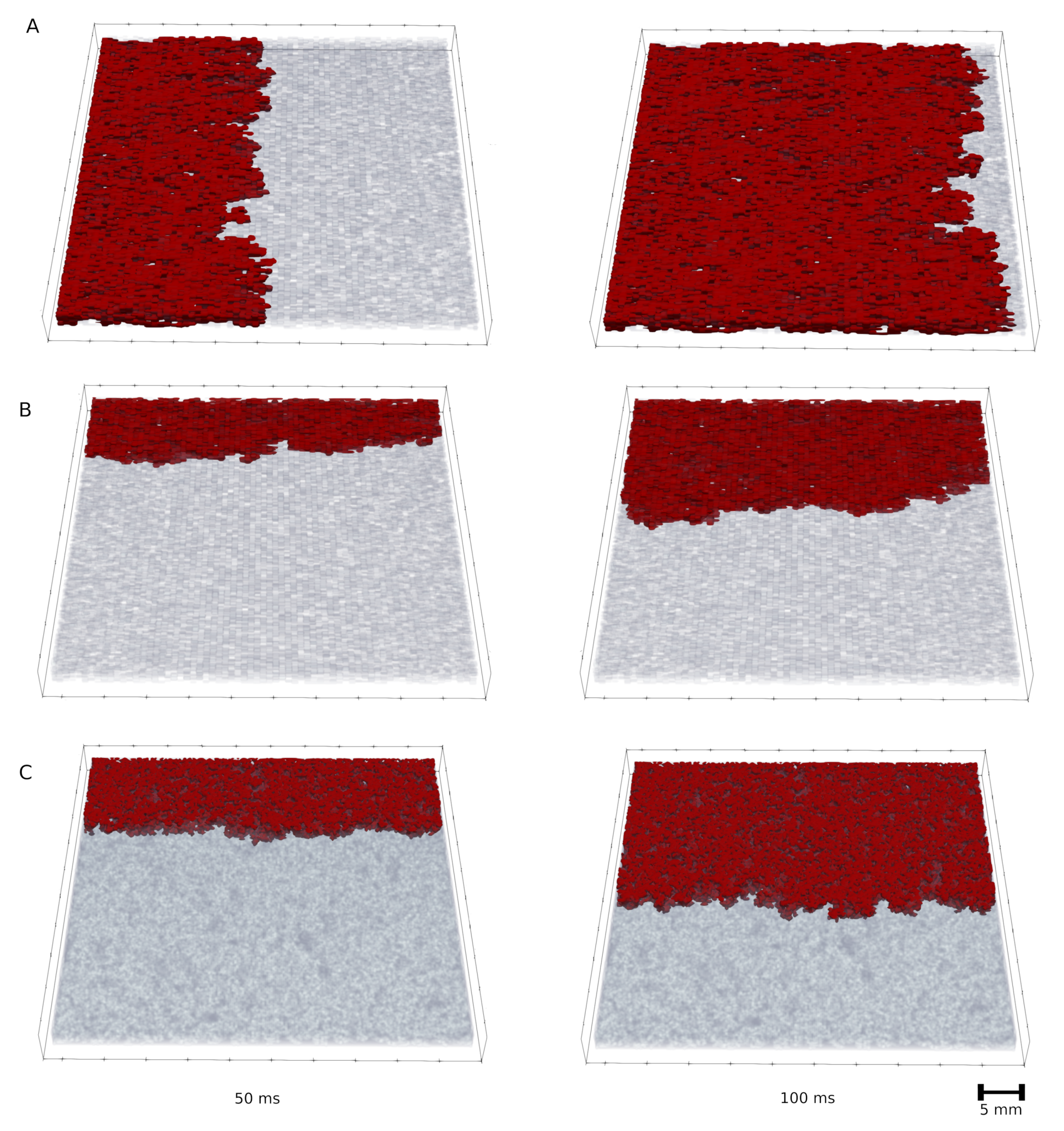
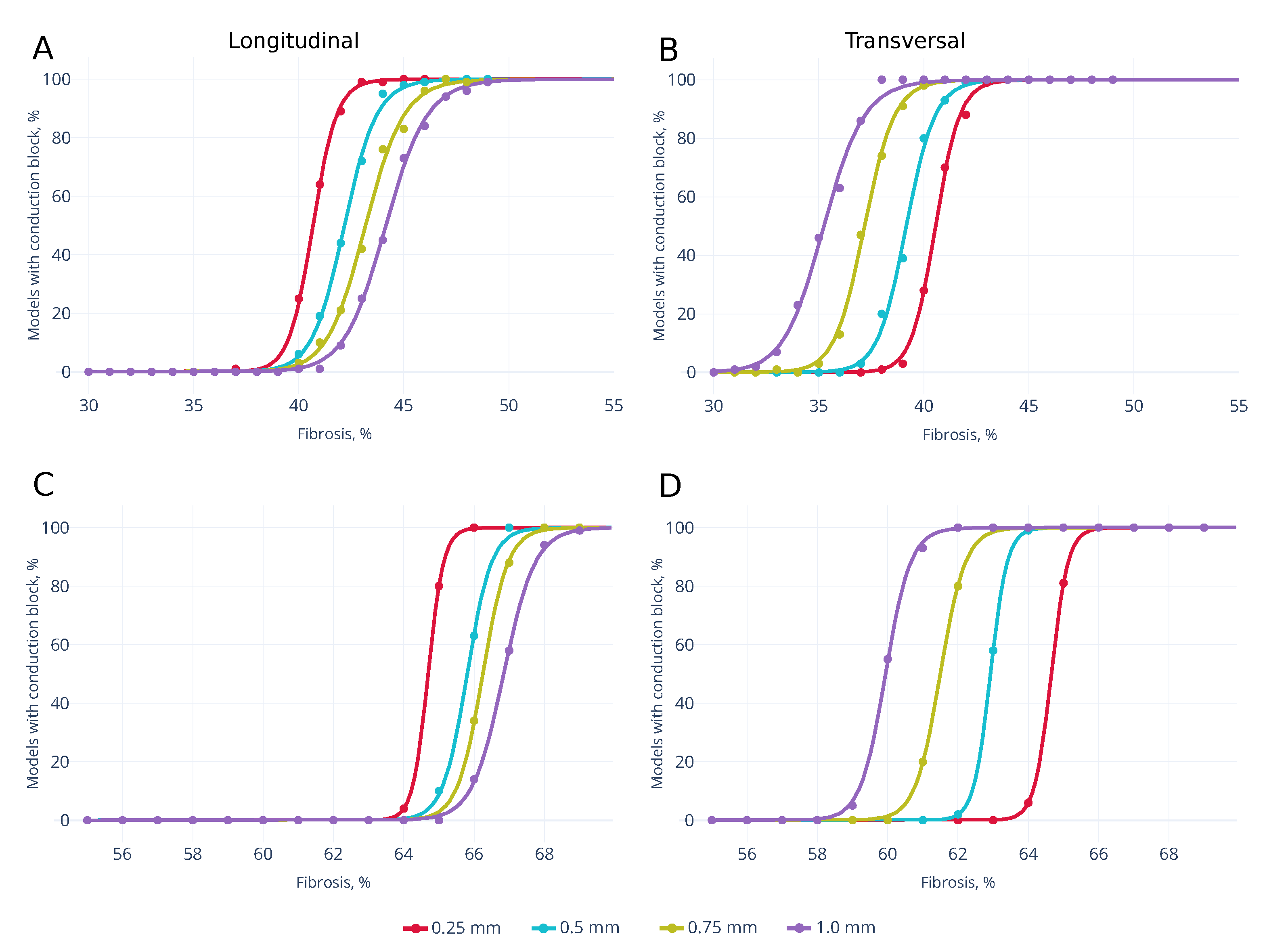
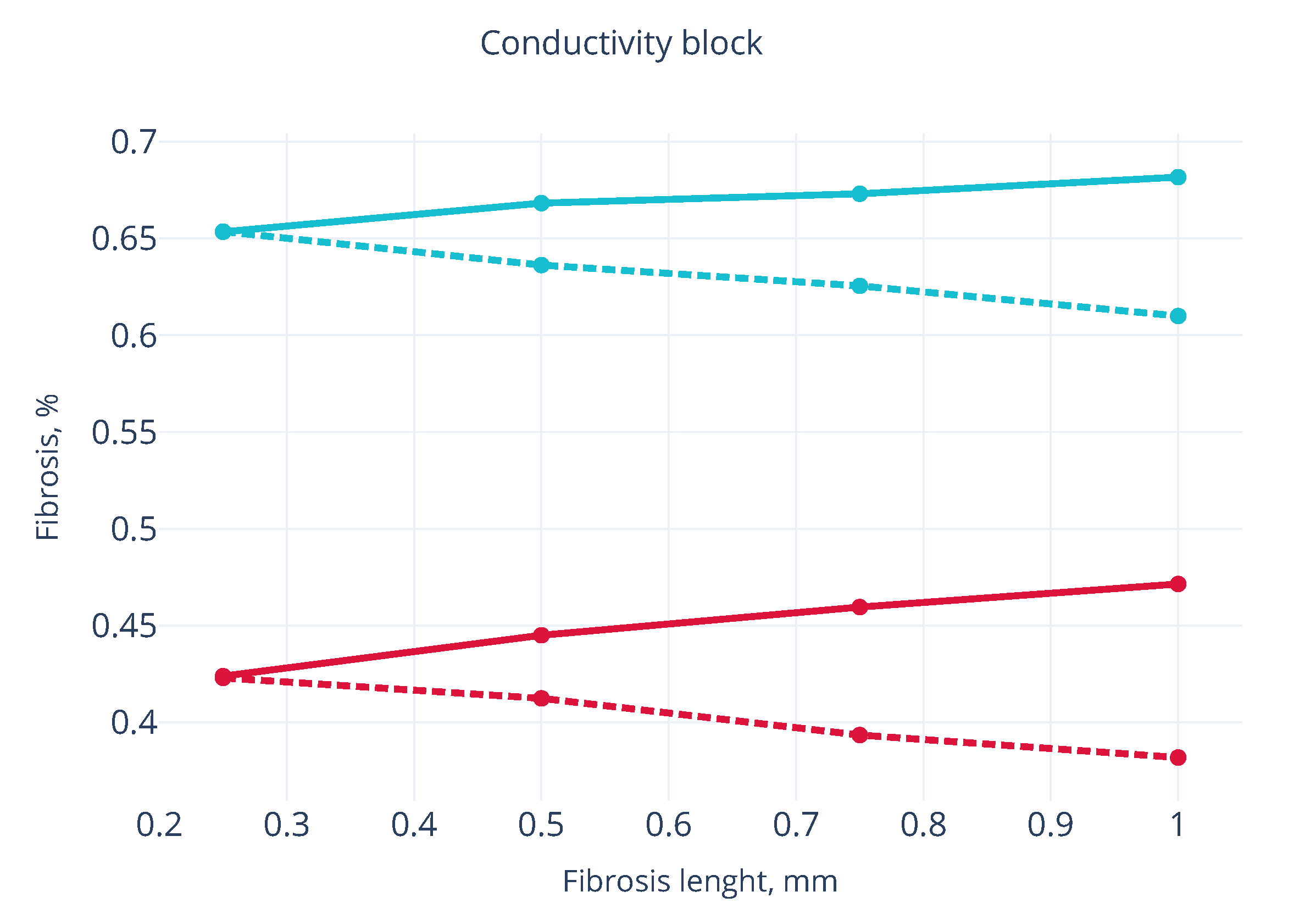
3.2. Wave Propagation Stopping Depends on the Tissue Depth, Fibrosis Percentage and Wavefront Direction
3.3. Effects of Fibrosis on the Conduction Velocity Depends on the Tissue Depth
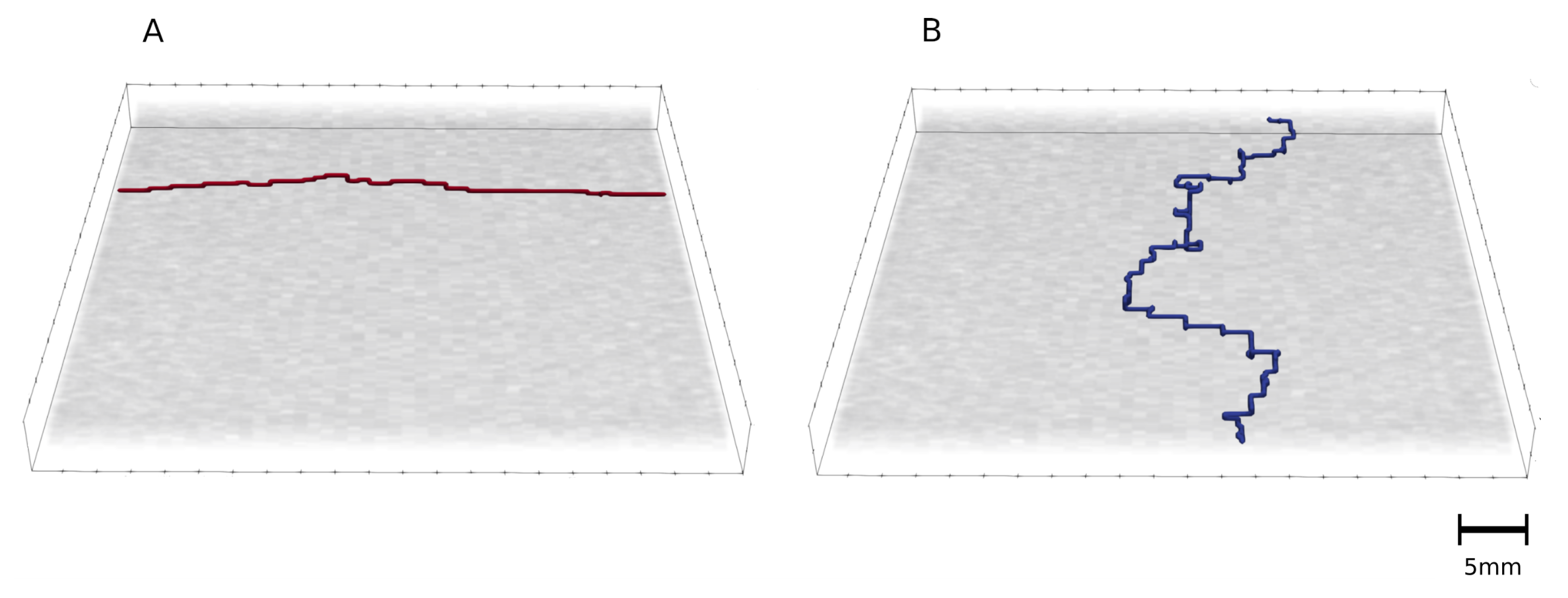
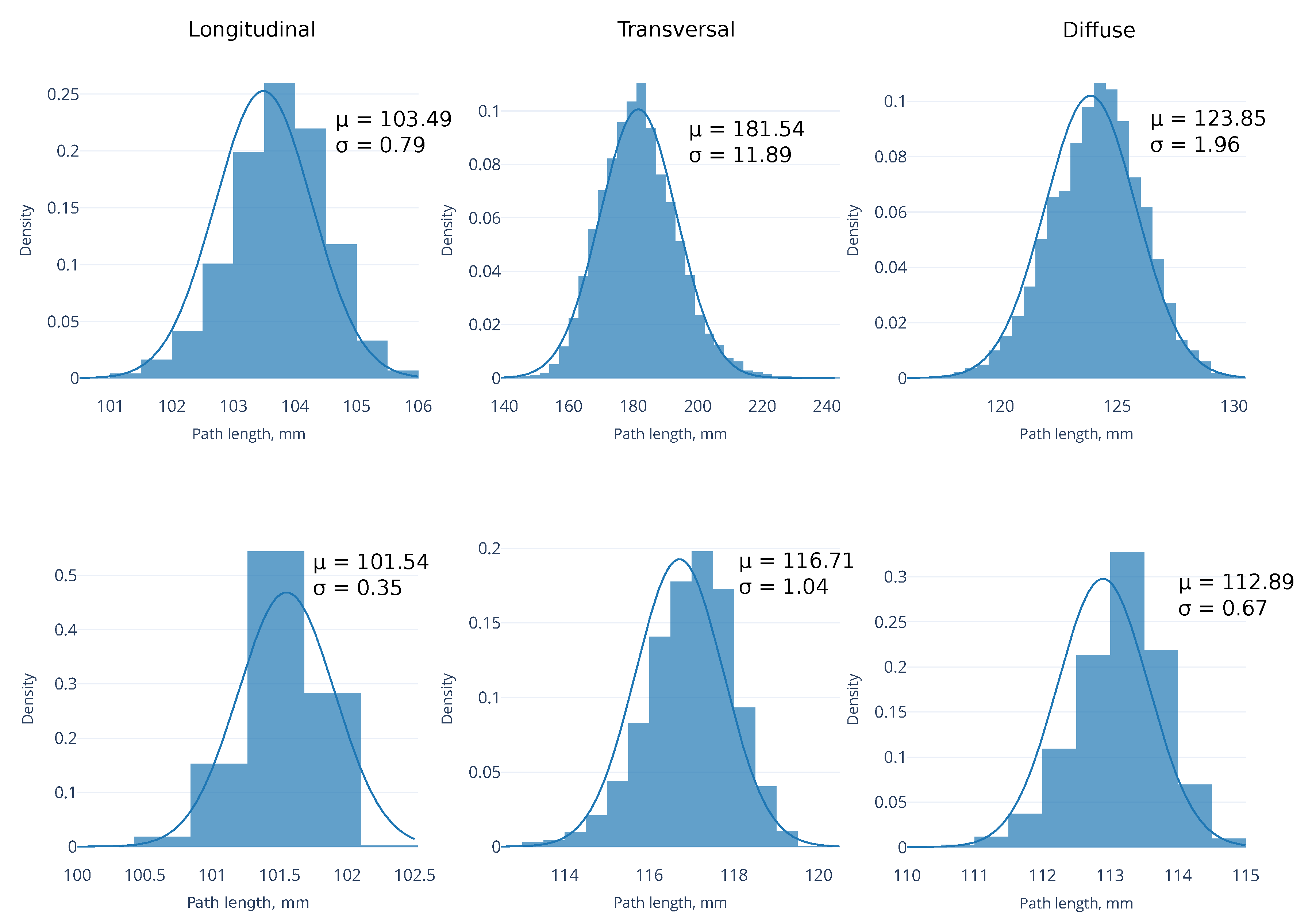
3.4. Shortest Path for Wave Propagation Depends on the Obstacle 0 and the Tissue Depth
4. Discussion
Numerical Implications for Fibrosis Modelling
Supplementary Materials
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Abbreviations
| CV | Conduction velocity |
References
- Volpert, A.; Volpert, V.; Volpert, V. Traveling Wave Solutions of Parabolic Systems: Translations of Mathematical Monographs; American Mathematical Society: Providence, RI, USA, 1994; Volume 140. [Google Scholar]
- Bing, R.; Dweck, M.R. Myocardial fibrosis: Why image, how to image and clinical implications. Heart 2019, 105, 1832–1840. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Nguyen, T.P.; Qu, Z.; Weiss, J.N. Cardiac fibrosis and arrhythmogenesis: The road to repair is paved with perils. J. Mol. Cell. Cardiol. 2014, 70, 83–91. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- De Jong, S.; Van Veen, T.A.; Van Rijen, H.V.; De Bakker, J.M. Fibrosis and cardiac arrhythmias. J. Cardiovasc. Pharmacol. 2011, 57, 630–638. [Google Scholar] [CrossRef]
- Stein, M.; Noorman, M.; van Veen, T.A.; Herold, E.; Engelen, M.A.; Boulaksil, M.; Antoons, G.; Jansen, J.A.; van Oosterhout, M.F.; Hauer, R.N.; et al. Dominant arrhythmia vulnerability of the right ventricle in senescent mice. Heart Rhythm 2008, 5, 438–448. [Google Scholar] [CrossRef] [PubMed]
- Jugdutt, B.I. Ventricular remodeling after infarction and the extracellular collagen matrix. Circulation 2003, 108, 1395–1403. [Google Scholar] [CrossRef] [Green Version]
- Travers, J.G.; Kamal, F.A.; Robbins, J.; Yutzey, K.E.; Blaxall, B.C. Cardiac fibrosis. Circ. Res. 2016, 118, 1021–1040. [Google Scholar] [CrossRef] [Green Version]
- De Bakker, J.M.; Van Capelle, F.J.; Janse, M.J.; Tasseron, S.; Vermeulen, J.T.; De Jonge, N.; Lahpor, J.R. Slow conduction in the infarcted human heart: `Zigzag’ course of activation. Circulation 1993, 88, 915–926. [Google Scholar] [CrossRef] [Green Version]
- Ten Tusscher, K.H.; Panfilov, A.V. Influence of diffuse fibrosis on wave propagation in human ventricular tissue. Europace 2007, 9 (Suppl. 6), 38–45. [Google Scholar] [CrossRef]
- Kazbanov, I.V.; Ten Tusscher, K.H.; Panfilov, A.V. Effects of heterogeneous diffuse fibrosis on arrhythmia dynamics and mechanism. Sci. Rep. 2016, 6, 1–14. [Google Scholar] [CrossRef] [Green Version]
- Alonso, S.; Bär, M. Reentry near the percolation threshold in a heterogeneous discrete model for cardiac tissue. Phys. Rev. Lett. 2013, 110, 158101. [Google Scholar] [CrossRef]
- Alonso, S.; dos Santos, R.W.; Bär, M. Reentry and ectopic pacemakers emerge in a three-dimensional model for a slab of cardiac tissue with diffuse microfibrosis near the percolation threshold. PLoS ONE 2016, 11, e0166972. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Vigmond, E.; Pashaei, A.; Amraoui, S.; Cochet, H.; Hassaguerre, M. Percolation as a mechanism to explain atrial fractionated electrograms and reentry in a fibrosis model based on imaging data. Heart Rhythm 2016, 13, 1536–1543. [Google Scholar] [CrossRef] [PubMed]
- McDowell, K.S.; Vadakkumpadan, F.; Blake, R.; Blauer, J.; Plank, G.; MacLeod, R.S.; Trayanova, N.A. Mechanistic inquiry into the role of tissue remodeling in fibrotic lesions in human atrial fibrillation. Biophys. J. 2013, 104, 2764–2773. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Krueger, M.W.; Rhode, K.S.; O’Neill, M.D.; Rinaldi, C.A.; Gill, J.; Razavi, R.; Seemann, G.; Doessel, O. Patient-specific modeling of atrial fibrosis increases the accuracy of sinus rhythm simulations and may explain maintenance of atrial fibrillation. J. Electrocardiol. 2014, 47, 324–328. [Google Scholar] [CrossRef]
- Varela, M.; Colman, M.A.; Hancox, J.C.; Aslanidi, O.V. Atrial heterogeneity generates re-entrant substrate during atrial fibrillation and anti-arrhythmic drug action: Mechanistic insights from canine atrial models. PLoS Comput. Biol. 2016, 12, e1005245. [Google Scholar] [CrossRef] [Green Version]
- ten Tusscher, K.H.; Panfilov, A.V. Influence of nonexcitable cells on spiral breakup in two-dimensional and three-dimensional excitable media. Phys. Rev. E Stat. Phys. Plasmas Fluids Relat. Interdiscip. Top. 2003, 68, 2–5. [Google Scholar] [CrossRef] [Green Version]
- ten Tusscher, K.H.W.J.; Panfilov, A.V. Wave propagation in excitable media with randomly distributed obstacles. Multiscale Model. Simul. 2005, 3, 265–282. [Google Scholar] [CrossRef] [Green Version]
- Mendonca Costa, C.; Prassl, A.J.; Weber, R.; Campos, F.O.; Prassl, A.J.; Dos Santos, R.W.; Sanchez-Quintana, D.; Ahammer, H.; Hofer, E.; Plank, G.; et al. An efficient finite element approach for modeling fibrotic clefts in the heart. IEEE Trans. Biomed. Eng. 2014, 61, 900–910. [Google Scholar] [CrossRef]
- Arevalo, H.J.; Vadakkumpadan, F.; Guallar, E.; Jebb, A.; Malamas, P.; Wu, K.C.; Trayanova, N.A. Arrhythmia risk stratification of patients after myocardial infarction using personalized heart models. Nat. Commun. 2016, 7, 11437. [Google Scholar] [CrossRef]
- Gao, Y.; Gong, Y.; Xia, L. Simulation of atrial fibrosis using coupled myocyte-fibroblast cellular and human atrial models. Comput. Math. Methods Med. 2017, 2017. [Google Scholar] [CrossRef]
- Mangion, K.; Gao, H.; Husmeier, D.; Luo, X.; Berry, C. Advances in computational modelling for personalised medicine after myocardial infarction. Heart 2018, 104, 550–557. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Majumder, R.; Pandit, R.; Panfilov, A.V. Turbulent electrical activity at sharp-edged inexcitable obstacles in a model for human cardiac tissue. Am. J. Physiol. Heart Circ. Physiol. 2014, 307, H1024–H1035. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Nayak, A.R.; Shajahan, T.K.; Panfilov, A.V.; Pandit, R. Spiral-wave dynamics in a mathematical model of human ventricular tissue with myocytes and fibroblasts. PLoS ONE 2013, 8. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Sridhar, S.; Vandersickel, N.; Panfilov, A.V. Effect of myocyte-fibroblast coupling on the onset of pathological dynamics in a model of ventricular tissue. Sci. Rep. 2017, 7, 1–12. [Google Scholar] [CrossRef] [Green Version]
- Kudryashova, N.; Nizamieva, A.; Tsvelaya, V.; Panfilov, A.V.; Agladze, K.I. Self-organization of conducting pathways explains electrical wave propagation in cardiac tissues with high fraction of non-conducting cells. PLoS Comput. Biol. 2019, 15, e1006597. [Google Scholar] [CrossRef] [Green Version]
- McDowell, K.S.; Arevalo, H.J.; Maleckar, M.M.; Trayanova, N.A. Susceptibility to arrhythmia in the infarcted heart depends on myofibroblast density. Biophys. J. 2011, 101, 1307–1315. [Google Scholar] [CrossRef] [Green Version]
- Pertsov, A.M. Scale of Geometric Structures Responsible for Discontinuous Propagation in Myocardial Tissue. In Discontinuous Conduction in the Heart; Futura Publishing Company: Armonk, NY, USA, 1997. [Google Scholar]
- Nezlobinsky, T.; Solovyova, O.; Panfilov, A.V. Anisotropic conduction in the myocardium due to fibrosis: The effect of texture on wave propagation. Sci. Rep. 2020, 10, 764. [Google Scholar] [CrossRef]
- Anderson, K.P.; Walker, R.; Une, P.; Ershler, P.R.; Lux, R.L.; Karwandee, S.V. Myocardial electrical propagation in patients with idiopathic dilated cardiomyopathy. J. Clin. Investig. 1993, 92, 122–140. [Google Scholar] [CrossRef] [Green Version]
- ten Tusscher, K.H.W.J. Alternans and spiral breakup in a human ventricular tissue model. AJP Heart Circ. Physiol. 2006, 291, H1088–H1100. [Google Scholar] [CrossRef]
- Tusscher, K.H.W.J.T.; Panfilov, A.V. Modelling of the ventricular conduction system. Prog. Biophys. Mol. Biol. 2008, 96, 152–170. [Google Scholar] [CrossRef]
- Rush, S.; Larsen, H. A practical algorithm for solving dynamic membrane equations. IEEE Trans. Biomed. Eng. 1978, BME-25, 389–392. [Google Scholar] [CrossRef] [PubMed]
- Bub, G.; Shrier, A.; Glass, L. Spiral wave generation in heterogeneous excitable media. Phys. Rev. Lett. 2002, 88, 058101. [Google Scholar] [CrossRef] [PubMed]
- Spach, M.S.; Miller, W.T.; Dolber, P.C.; Kootsey, J.M.; Sommer, J.R.; Mosher, C.E. The functional role of structural complexities in the propagation of depolarization in the atrium of the dog. Cardiac conduction disturbances due to discontinuities of effective axial resistivity. Circ. Res. 1982, 50, 175–191. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Li, D.; Fareh, S.; Leung, T.K.; Nattel, S. Promotion of atrial fibrillation by heart failure in dogs. Circulation 1999, 100, 87–95. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Zlochiver, S.; Muñoz, V.; Vikstrom, K.L.; Taffet, S.M.; Berenfeld, O.; Jalife, J. Electrotonic myofibroblast-to-myocyte coupling increases propensity to reentrant arrhythmias in two-dimensional cardiac monolayers. Biophys. J. 2008, 95, 4469–4480. [Google Scholar] [CrossRef] [Green Version]
- Majumder, R.; Feola, I.; Teplenin, A.S.; de Vries, A.A.; Panfilov, A.V.; Pijnappels, D.A. Optogenetics enables real-time spatiotemporal control over spiral wave dynamics in an excitable cardiac system. eLife 2018, 7, 1–17. [Google Scholar] [CrossRef]
- Zuppinger, C. 3D cardiac cell culture: A critical review of current technologies and applications. Front. Cardiovasc. Med. 2019, 6, 1–9. [Google Scholar] [CrossRef] [Green Version]
- Pope, A.J.; Sands, G.B.; Smaill, B.H.; LeGrice, I.J. Three-dimensional transmural organization of perimysial collagen in the heart. Am. J. Physiol. Heart Circ. Physiol. 2008, 295, H1243–H1252. [Google Scholar] [CrossRef]
- Glashan, C.A.; Androulakis, A.F.; Tao, Q.; Glashan, R.N.; Wisse, L.J.; Ebert, M.; De Ruiter, M.C.; Van Meer, B.J.; Brouwer, C.; Dekkers, O.M.; et al. Whole human heart histology to validate electroanatomical voltage mapping in patients with non-ischaemic cardiomyopathy and ventricular tachycardia. Eur. Heart J. 2018, 39, 2867–2875. [Google Scholar] [CrossRef] [Green Version]
- Keldermann, R.H.; Ten Tusscher, K.H.; Nash, M.P.; Hren, R.; Taggart, P.; Panfilov, A.V. Effect of heterogeneous APD restitution on VF organization in a model of the human ventricles. Am. J. Physiol. Heart Circ. Physiol. 2008, 294, 764–774. [Google Scholar] [CrossRef] [Green Version]
- Keldermann, R.H.; Ten Tusscher, K.H.; Nash, M.P.; Bradley, C.P.; Hren, R.; Taggart, P.; Panfilov, A.V. A computational study of mother rotor VF in the human ventricles. Am. J. Physiol. Heart Circ. Physiol. 2009, 296. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Panfilov, A.; Keener, J. Re-entry in an anatomical model of the heart. Chaos Solitons Fractals 1995, 5, 681–689. [Google Scholar] [CrossRef]
- Vandersickel, N.; Kazbanov, I.V.; Nuitermans, A.; Weise, L.D.; Pandit, R.; Panfilov, A.V. A study of early afterdepolarizations in a model for human ventricular tissue. PLoS ONE 2014, 9, e84595. [Google Scholar] [CrossRef]
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dokuchaev, A.; Panfilov, A.V.; Solovyova, O. Myocardial Fibrosis in a 3D Model: Effect of Texture on Wave Propagation. Mathematics 2020, 8, 1352. https://doi.org/10.3390/math8081352
Dokuchaev A, Panfilov AV, Solovyova O. Myocardial Fibrosis in a 3D Model: Effect of Texture on Wave Propagation. Mathematics. 2020; 8(8):1352. https://doi.org/10.3390/math8081352
Chicago/Turabian StyleDokuchaev, Arsenii, Alexander V. Panfilov, and Olga Solovyova. 2020. "Myocardial Fibrosis in a 3D Model: Effect of Texture on Wave Propagation" Mathematics 8, no. 8: 1352. https://doi.org/10.3390/math8081352
APA StyleDokuchaev, A., Panfilov, A. V., & Solovyova, O. (2020). Myocardial Fibrosis in a 3D Model: Effect of Texture on Wave Propagation. Mathematics, 8(8), 1352. https://doi.org/10.3390/math8081352





