A Numerical Method for a System of Fractional Differential-Algebraic Equations Based on Sliding Mode Control
Abstract
1. Introduction
2. Fractional Differential-Algebraic Equations
3. Transformation Based on Sliding Mode Control Theory
- Based on nonlinear control theory, g(x) should satisfy the necessary and sufficient condition of the controllability for the nonlinear system Equation (4), namelywhere, adf·g is Lie bracket operation of vectors f = and g.
- In order to obtain control vector u, Js g(x) should be invertible, where Js is the Jacobi matrix of s(x).
4. The Numerical Algorithm
5. Violation Corrections
6. Results
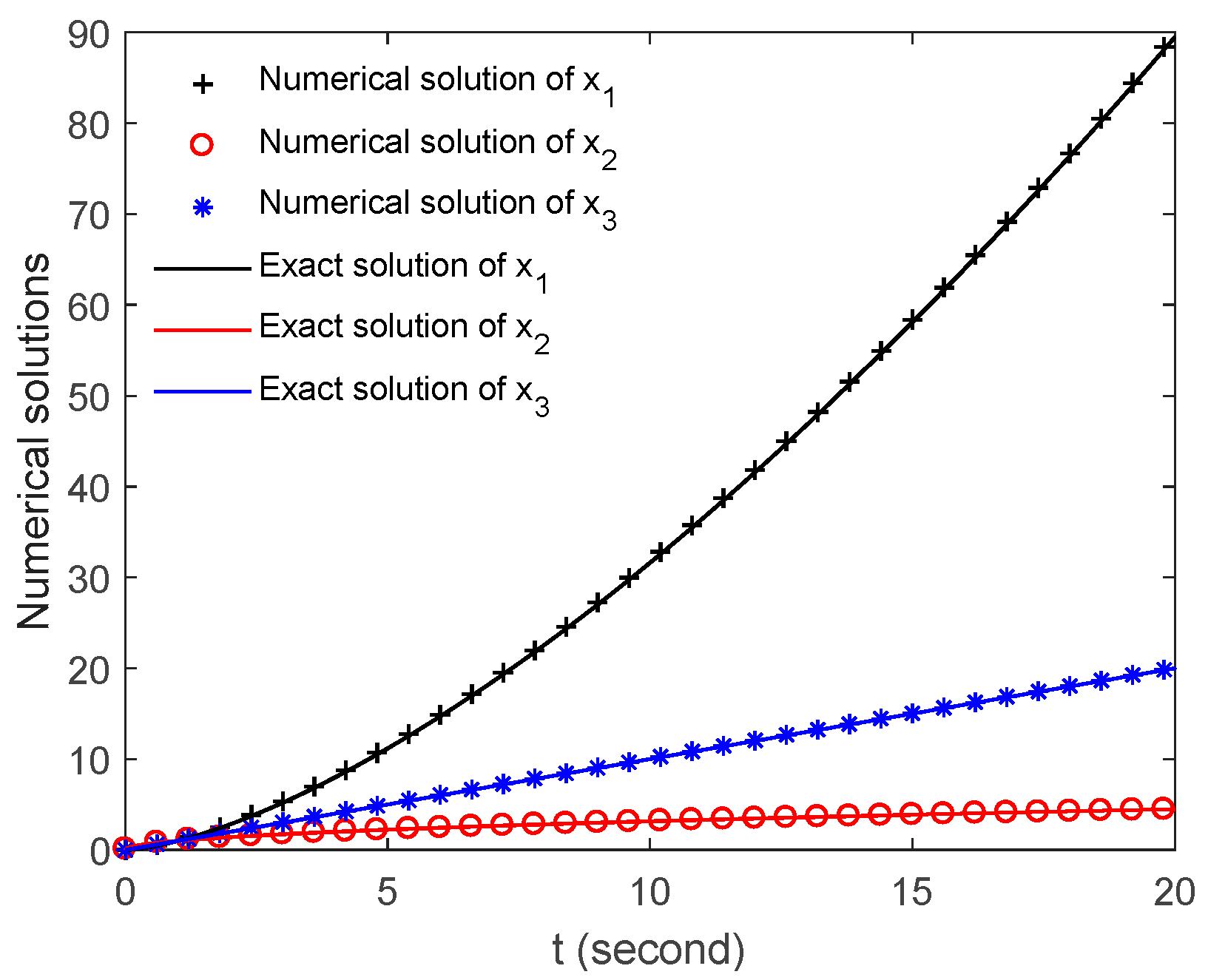
6.1. An Example with Exact Solutions
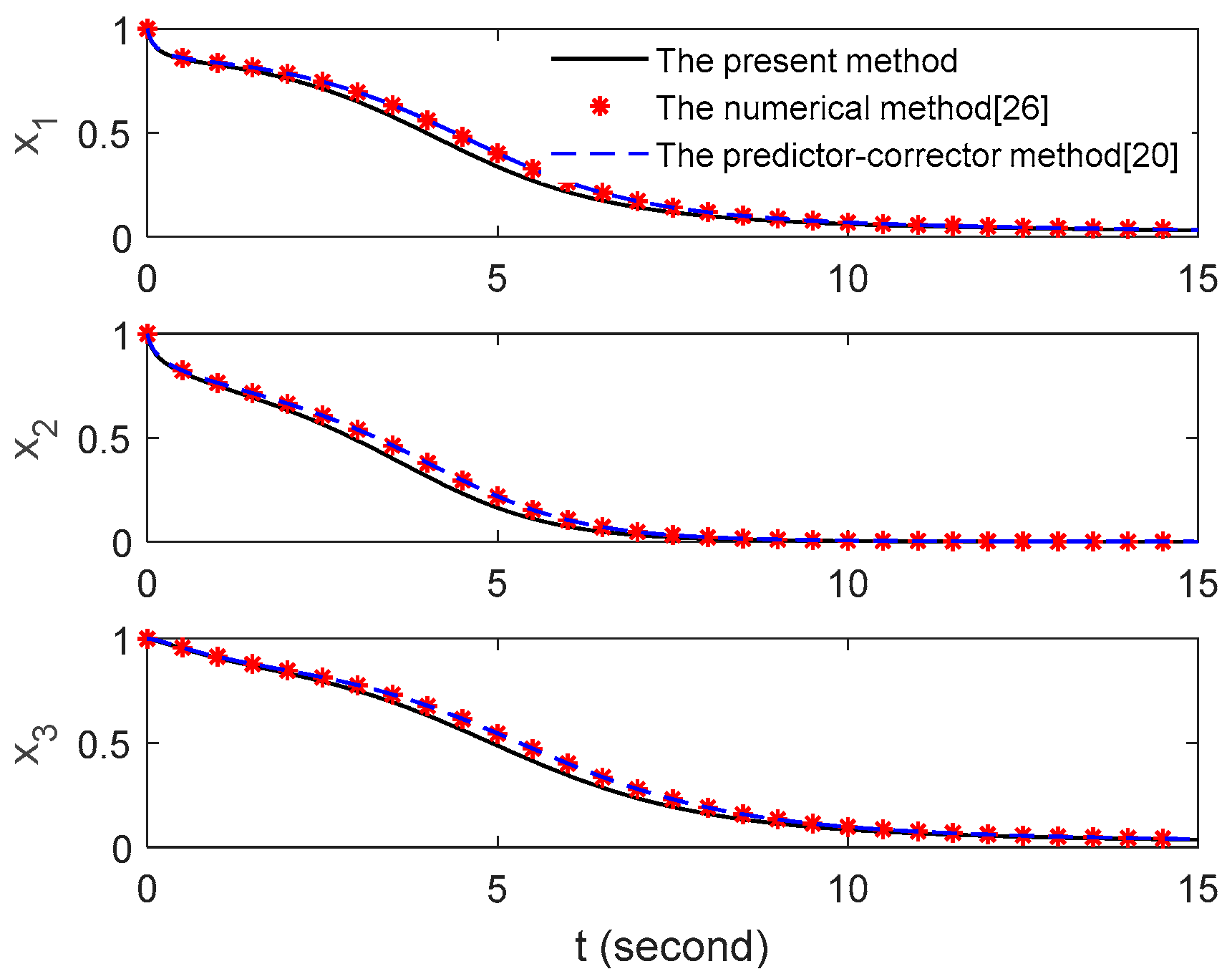
6.2. Comparisons with Other Methods
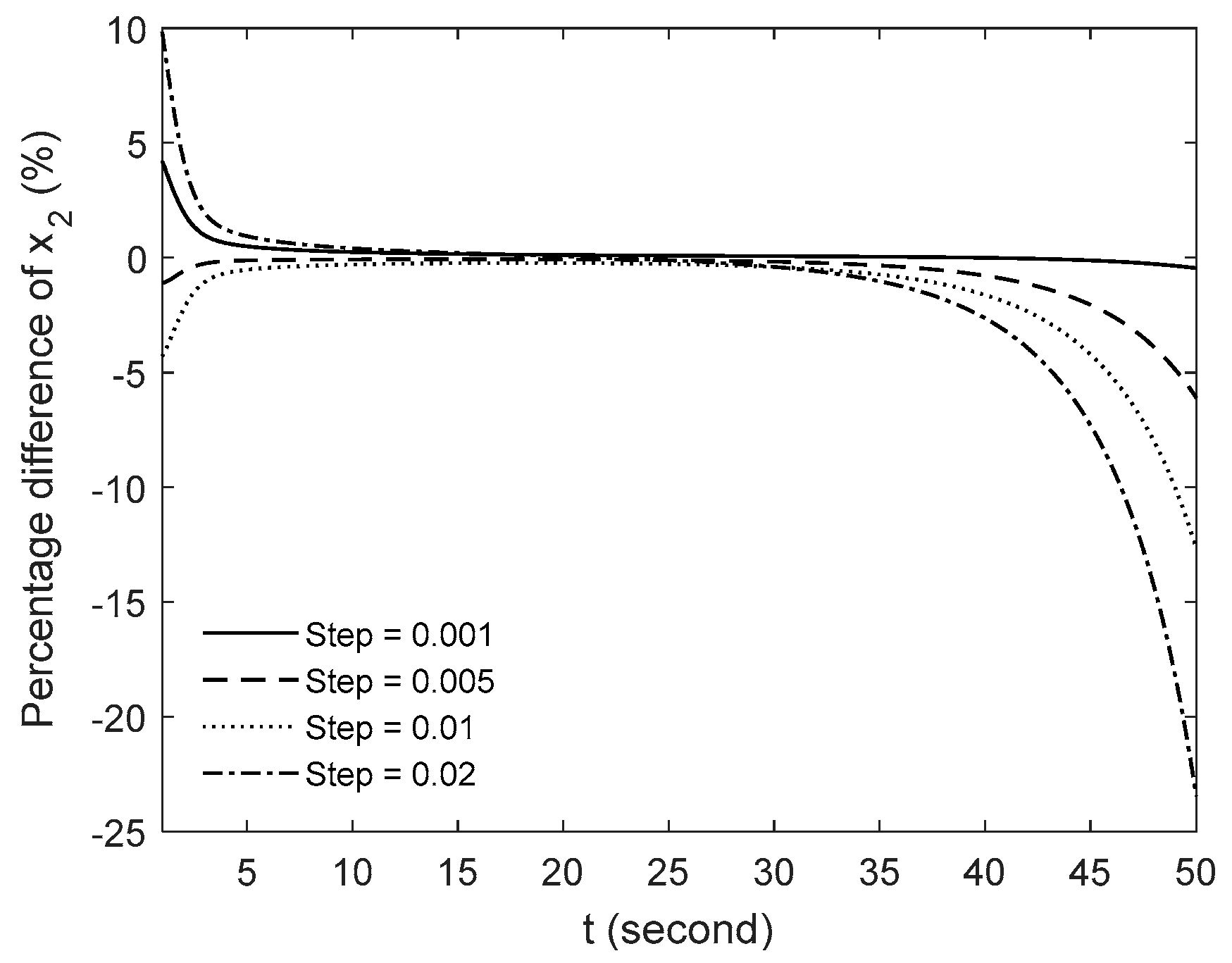
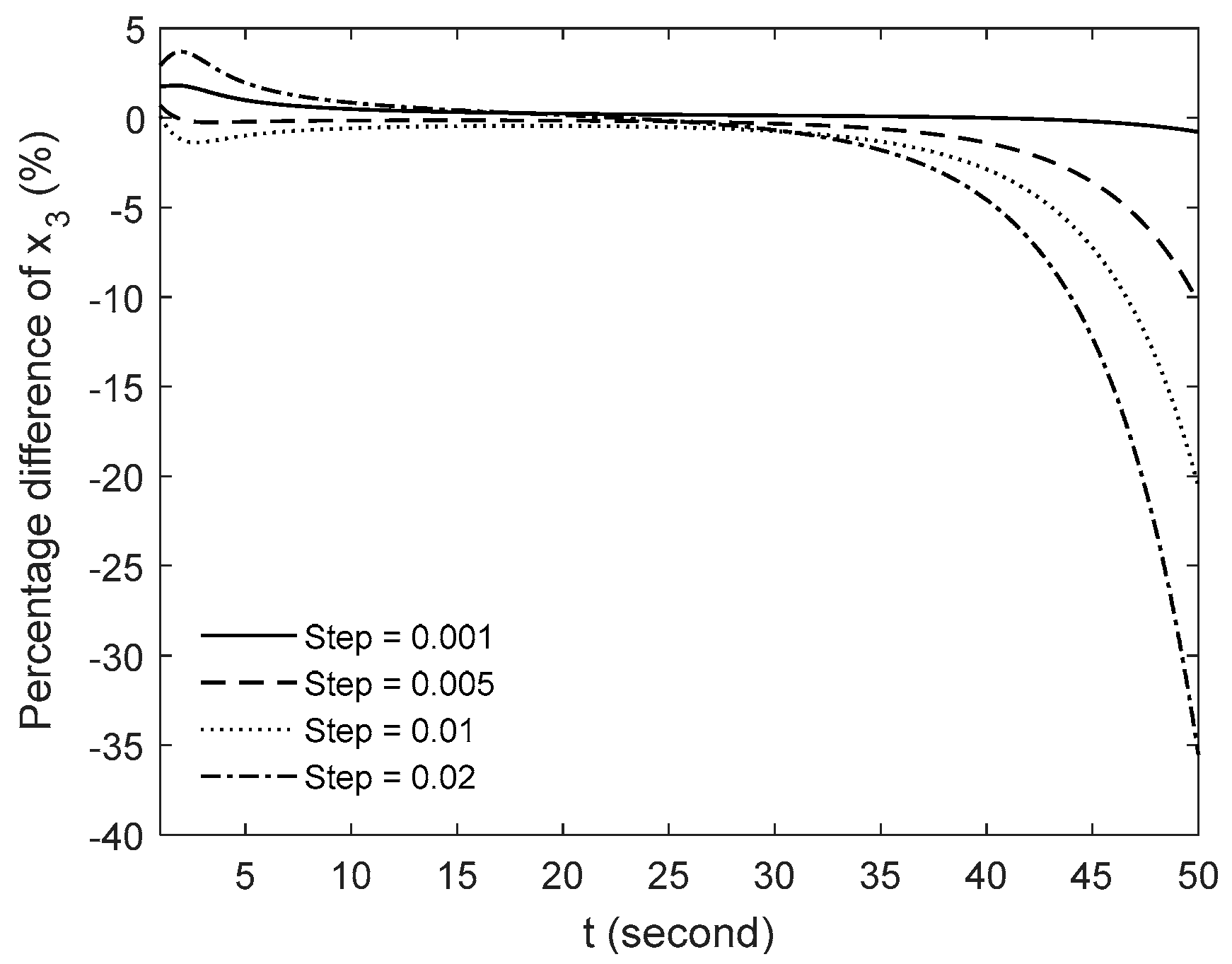
6.3. The Effect of Violation Corrections
7. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Podlubny, I.; Chechkin, A.; Skovranek, T.; Chen, Y.Q.; Jara, B.M.V. Matrix approach to discrete fractional calculus II: Partial fractional differential equations. J. Comput. Phys. 2009, 228, 3137–3153. [Google Scholar] [CrossRef]
- Moshrefi-Torbati, M.; Hammond, J.K. Physical and geometrical interpretation of fractional operators. J. Frankl. Inst. 1998, 335, 1077–1086. [Google Scholar] [CrossRef]
- Xu, J.; Chen, Y.; Tai, Y.; Xu, X.; Shi, G.; Chen, N. Vibration analysis of complex fractional viscoelastic beam structures by the wave method. Int. J. Mech. Sci. 2020, 167, 105204. [Google Scholar] [CrossRef]
- Giona, M.; Cerbelli, S.; Roman, H.E. Fractional diffusion equation and relaxation in complex viscoelastic materials. Phys. A Stat. Mech. Appl. 1992, 191, 449–453. [Google Scholar] [CrossRef]
- Ingman, D.; Suzdalnitsky, J. Application of differential operator with servo-order function in model of viscoelastic deformation process. J. Eng. Mech. 2005, 131, 763–767. [Google Scholar] [CrossRef]
- Povstenko, Y. Fractional heat conduction in a space with a source varying harmonically in time and associated thermal stresses. J. Therm. Stresses 2016, 39, 1442–1450. [Google Scholar] [CrossRef]
- Sun, H.G.; Zhang, Y.; Baleanu, D.; Chen, W.; Chen, Y.Q. A new collection of real world applications of fractional calculus in science and engineering. Commun. Nonlinear Sci. Numer. Simul. 2018, 64, 213–231. [Google Scholar] [CrossRef]
- Podlubny, I. Fractional-order systems and PI/sup /spl lambda//D/sup /spl mu//-controllers. IEEE Trans. Autom. Control 1999, 44, 208–214. [Google Scholar] [CrossRef]
- Vinagre, B.; Petráš, I.; Podlubny, I.; Chen, Y.Q. Using fractional order adjustment rules and fractional order reference models in model-reference adaptive control. Nonlinear Dyn. 2002, 29, 269–279. [Google Scholar] [CrossRef]
- Chen, N.; Tai, Y.; Chen, N. Nonlinear suspension of fractional order calculus in the application of the variable structure sliding mode control. Dyn. Control 2009, 7, 258–263. [Google Scholar]
- Gad, S.; Metered, H.; Bassuiny, A.; Ghany, A.M.A. Multi-objective genetic algorithm fractional-order PID controller for semi-active magnetorheologically damped seat suspension. J. Vib. Control 2017, 23, 1248–1266. [Google Scholar] [CrossRef]
- Mehrmann, V.; Wunderlich, L. Hybrid systems of differential-algebraic equations—Analysis and numerical solution. J. Process. Control 2009, 19, 1218–1228. [Google Scholar] [CrossRef]
- Louca, L.S. Modal analysis reduction of multi-body systems with generic damping. J. Comput. Sci. 2014, 5, 415–426. [Google Scholar] [CrossRef]
- Carpinelli, M.; Gubitosa, M.; Mundo, D.; Desmet, W. Automated independent coordinates’ switching for the solution of stiff DAEs with the linearly implicit Euler method. Multibody Syst. Dyn. 2016, 36, 67–85. [Google Scholar] [CrossRef]
- Ghanbari, F.; Ghanbari, K.; Mokhtary, P. Generalized Jacobi-Galerkin method for nonlinear fractional differential algebraic equations. Comput. Appl. Math. 2018, 37, 5456–5475. [Google Scholar] [CrossRef]
- Ding, X.L.; Jiang, Y.L. Nonnegativity of Solutions of Nonlinear Fractional Differential-Algebraic Equations. Acta Math. Sci. 2018, 38, 756–768. [Google Scholar] [CrossRef]
- Liu, H.; Fu, Y.; Li, B. Discrete Waveform Relaxation Method for Linear Fractional Delay Differential-Algebraic Equations. Discret. Dyn. Nat. Soc. 2017, 2017, 6306570. [Google Scholar] [CrossRef]
- Ghanbari, F.; Mokhtary, P.; Ghanbari, K. Numerical solution of a class of fractional order integro-differential algebraic equations using Muntz-Jacobi Tau method. J. Comput. Appl. Math. 2019, 362, 172–184. [Google Scholar] [CrossRef]
- Ghanbari, F.; Mokhtary, P.; Ghanbari, K. On the numerical solution of a class of linear fractional integro-differential algebraic equations with weakly singular kernels. Appl. Numer. Math. 2019, 144, 1–20. [Google Scholar] [CrossRef]
- Wang, L.; Chen, N. The predictor-corrector solution for fractional order differential algebraic equation. In Proceedings of the 2014 International Conference on Fractional Differentiation and Its Applications (ICFDA 2014), Catania, Italia, 23–25 June 2014; pp. 1–6. [Google Scholar]
- Mortezaee, M.; Ghovatmand, M.; Nazemi, A. Solving variable-order fractional differential algebraic equations via generalized fuzzy hyperbolic model with application in electric circuit modeling. Soft Comput. 2020. [Google Scholar] [CrossRef]
- Ibis, B.; Bayram, M. Numerical comparison of methods for solving fractional differential-algebraic equations (FDAEs). Comput. Math. Appl. 2011, 62, 3270–3278. [Google Scholar] [CrossRef]
- Diethelm, K.; Ford, N.J.; Freed, A.D. A Predictor-Corrector Approach for the Numerical Solution of Fractional Differential Equations. Nonlinear Dyn. 2002, 29, 3–22. [Google Scholar] [CrossRef]
- Podlubny, I. Fractional Differential Equations; ACADEMIC PRESS: San Diego, CA, USA, 1999; p. 198. [Google Scholar]
- Hung, J.Y.; Gao, W.; Hung, J.C. Variable structure control: A survey. IEEE Trans. Ind. Electron. 1993, 40, 2–22. [Google Scholar] [CrossRef]
- Chen, N.; Chen, N.; Tai, Y. A Numerical Scheme for Nonlinear Dynamic System with Fractional Feedback Control. In Proceedings of the ENOC, Rome, Italy, 24–29 July 2011; Volume 7, pp. 24–29. [Google Scholar]


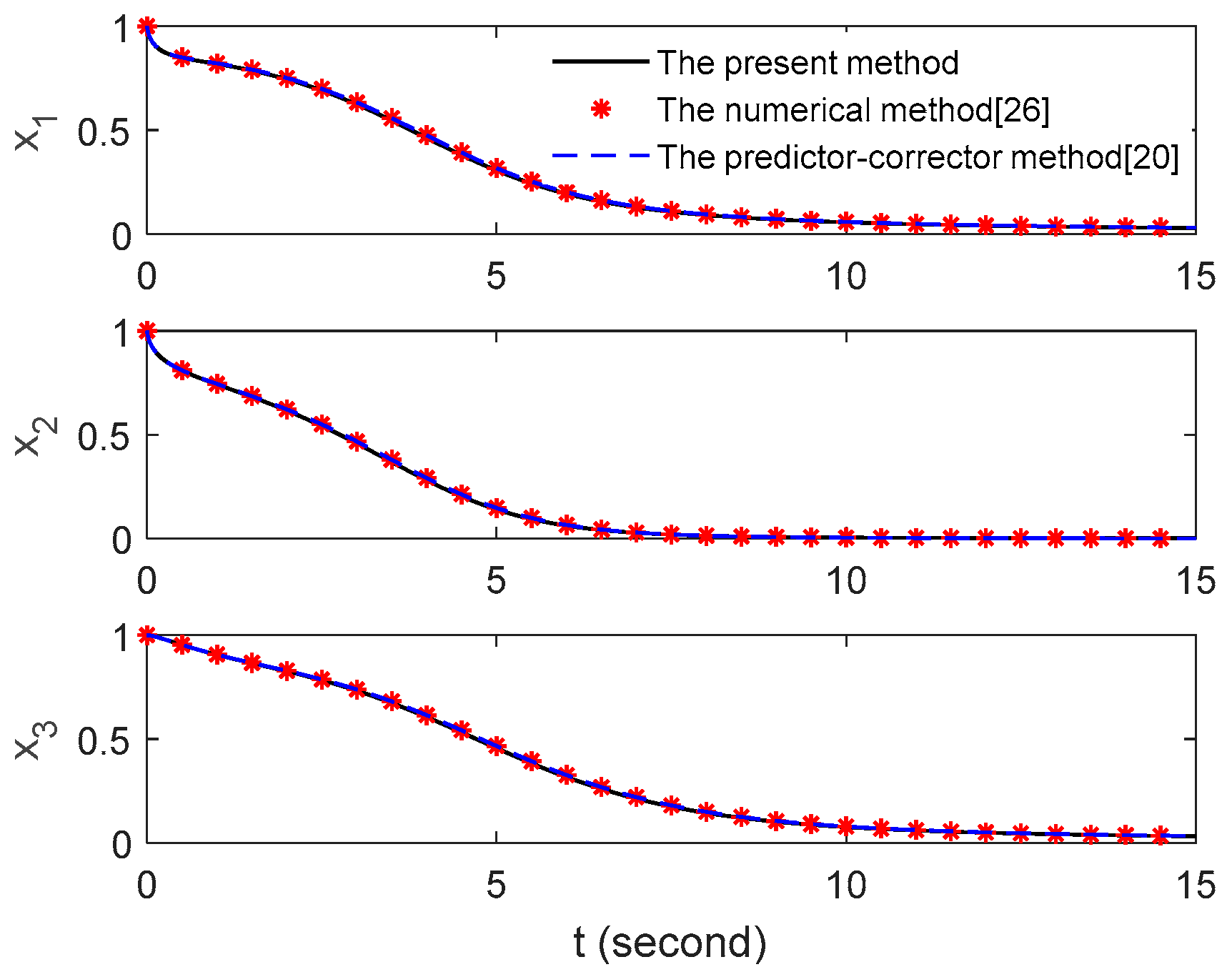

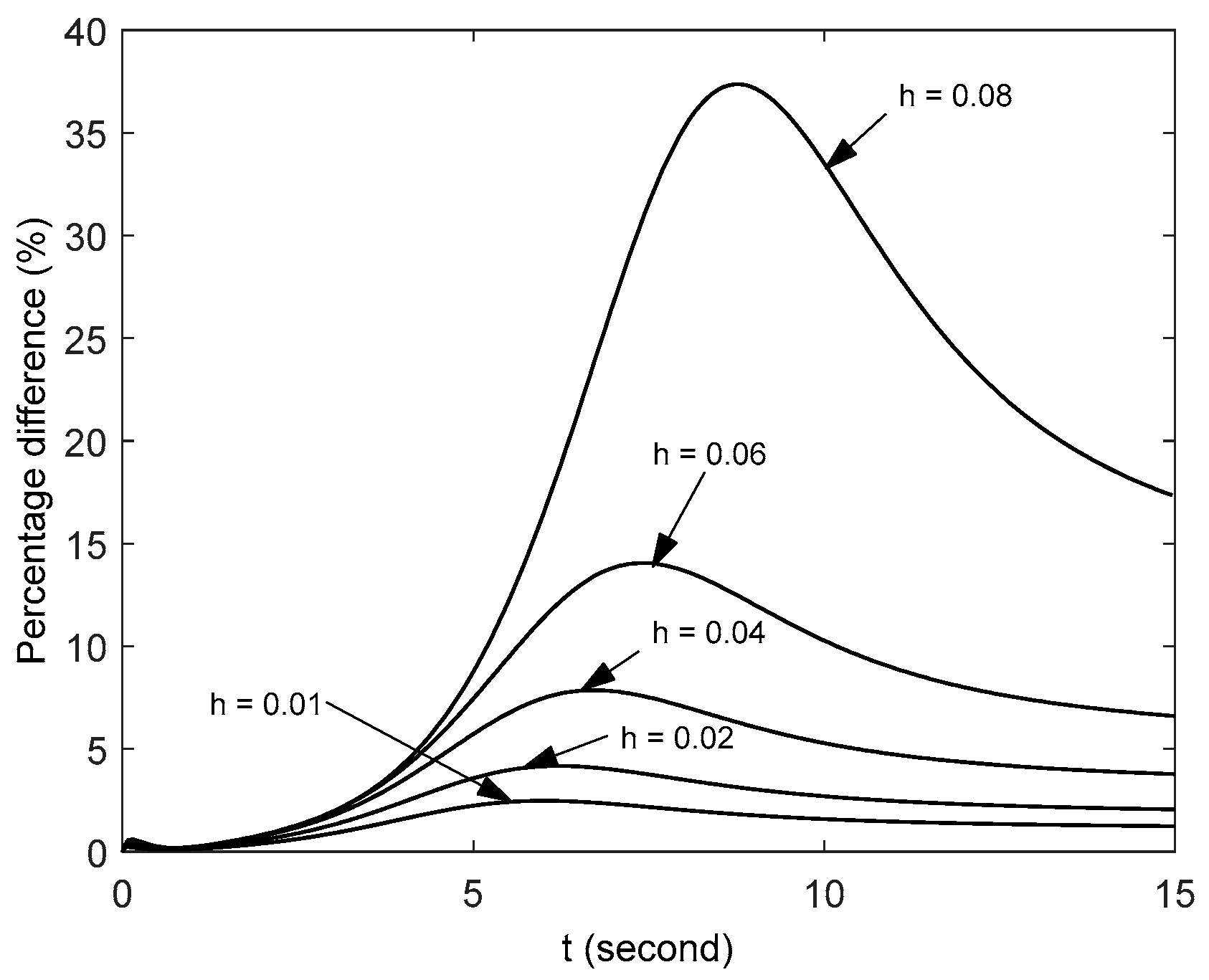

| No. | Method | Maximum Difference (%) | |||
|---|---|---|---|---|---|
| h = 0.01 | h = 0.001 | h = 0.0005 | h = 0.0001 | ||
| 1 | The numerical method [26] | 36.47 | 4.56 | 2.31 | 0 |
| 2 | The predictor-corrector method [20] | 36.94 | 4.57 | 2.31 | 0 |
| 3 | The present method | 11.93 | 0.85 | 0.066 | −0.70 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tai, Y.; Chen, N.; Wang, L.; Feng, Z.; Xu, J. A Numerical Method for a System of Fractional Differential-Algebraic Equations Based on Sliding Mode Control. Mathematics 2020, 8, 1134. https://doi.org/10.3390/math8071134
Tai Y, Chen N, Wang L, Feng Z, Xu J. A Numerical Method for a System of Fractional Differential-Algebraic Equations Based on Sliding Mode Control. Mathematics. 2020; 8(7):1134. https://doi.org/10.3390/math8071134
Chicago/Turabian StyleTai, Yongpeng, Ning Chen, Lijin Wang, Zaiyong Feng, and Jun Xu. 2020. "A Numerical Method for a System of Fractional Differential-Algebraic Equations Based on Sliding Mode Control" Mathematics 8, no. 7: 1134. https://doi.org/10.3390/math8071134
APA StyleTai, Y., Chen, N., Wang, L., Feng, Z., & Xu, J. (2020). A Numerical Method for a System of Fractional Differential-Algebraic Equations Based on Sliding Mode Control. Mathematics, 8(7), 1134. https://doi.org/10.3390/math8071134





